EDITORIAL
Published on 04 Nov 2021
Editorial: Mathematical Modeling of Endocrine Systems
doi 10.3389/fendo.2021.789386
- 1,924 views
- Frontiers in Endocrinology
- Systems Endocrinology
- Research Topics
- Mathematical Modeling of Endocrine Systems
Mathematical Modeling of Endocrine Systems
12k
Total downloads
62k
Total views and downloads
Articles
PERSPECTIVE
Published on 16 Jul 2021
Adapting Protocols or Models for Use in Insulin-Requiring Diabetes and Islet Transplant Recipients
doi 10.3389/fendo.2021.611512
- 1,557 views
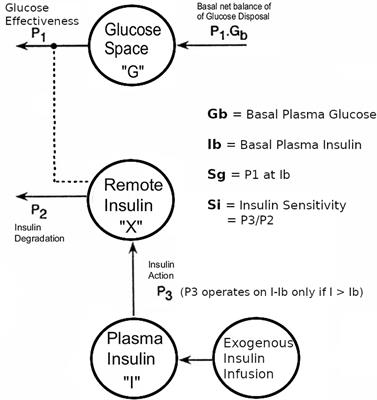
ORIGINAL RESEARCH
Published on 28 Jun 2021
Modeling Challenge Data to Quantify Endogenous Lactate Production
doi 10.3389/fendo.2021.656054
- 1,469 views
ORIGINAL RESEARCH
Published on 29 Mar 2021
Selenium Kinetics in Humans Change Following 2 Years of Supplementation With Selenomethionine
doi 10.3389/fendo.2021.621687
- 2,475 views
- 9 citations
ORIGINAL RESEARCH
Published on 29 Mar 2021
An Analysis of Glucose Effectiveness in Subjects With or Without Type 2 Diabetes via Hierarchical Modeling
doi 10.3389/fendo.2021.641713
- 2,648 views
- 4 citations
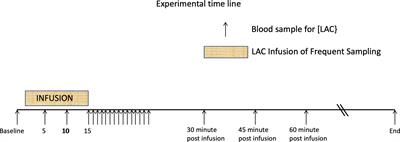
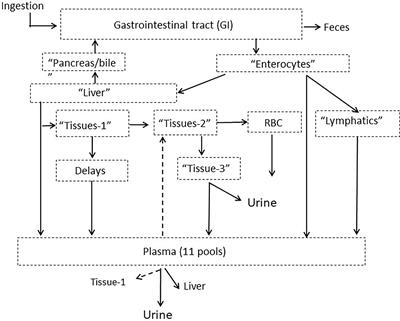
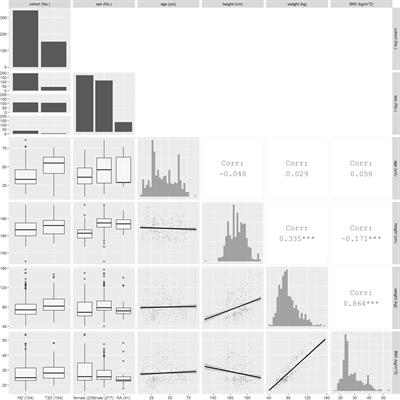
ORIGINAL RESEARCH
Published on 22 Mar 2021
Mathematical Model of Glucagon Kinetics for the Assessment of Insulin-Mediated Glucagon Inhibition During an Oral Glucose Tolerance Test
doi 10.3389/fendo.2021.611147
- 3,770 views
- 19 citations
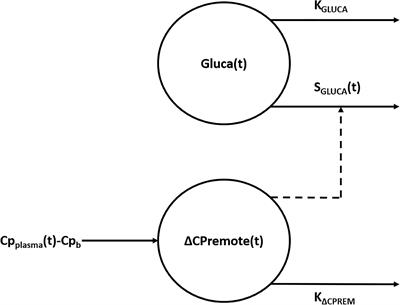
ORIGINAL RESEARCH
Published on 16 Mar 2021
Insulin Action, Glucose Homeostasis and Free Fatty Acid Metabolism: Insights From a Novel Model
doi 10.3389/fendo.2021.625701
- 13,767 views
- 14 citations
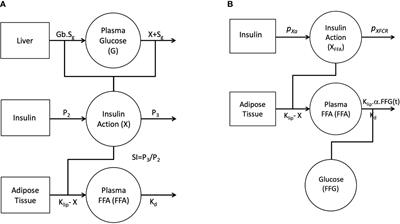
ORIGINAL RESEARCH
Published on 15 Mar 2021
Model-Based Assessment of C-Peptide Secretion and Kinetics in Post Gastric Bypass Individuals Experiencing Postprandial Hyperinsulinemic Hypoglycemia
doi 10.3389/fendo.2021.611253
- 2,594 views
- 7 citations
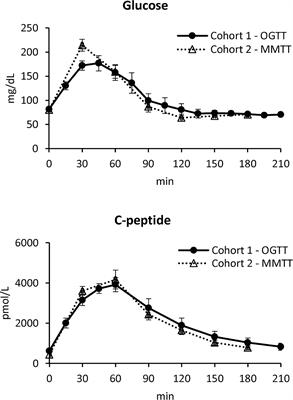
ORIGINAL RESEARCH
Published on 11 Mar 2021
Mathematical Modeling and Simulation Provides Evidence for New Strategies of Ovarian Stimulation
doi 10.3389/fendo.2021.613048
- 12,918 views
- 5 citations
ORIGINAL RESEARCH
Published on 15 Feb 2021
Origins and History of the Minimal Model of Glucose Regulation
doi 10.3389/fendo.2020.583016
- 12,207 views
- 51 citations
