EDITORIAL
Published on 04 Mar 2021
Editorial: Singular and Correlation Optics
doi 10.3389/fphy.2021.651964
- 2,744 views
- 6 citations
7,734
Total downloads
54k
Total views and downloads
You will be redirected to our submission process.
EDITORIAL
Published on 04 Mar 2021
ORIGINAL RESEARCH
Published on 17 Dec 2020
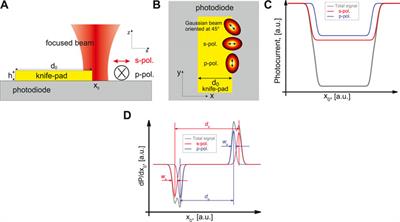
REVIEW
Published on 15 May 2020
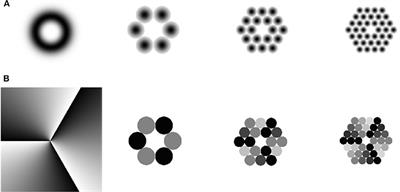
ORIGINAL RESEARCH
Published on 15 May 2020
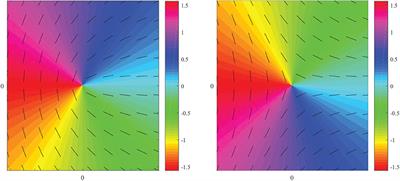
REVIEW
Published on 13 May 2020
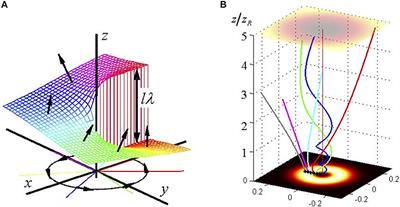
ORIGINAL RESEARCH
Published on 13 May 2020
ORIGINAL RESEARCH
Published on 08 May 2020

