- 1Department of Mechanical Engineering, Faculty of Engineering, Jazan University, Jazan, Saudia Arabia
- 2Department of Mathematics, Quaid-i-Azam University, Islamabad, Pakistan
- 3Mathematics Department, Faculty of Sciences, Northern Border University, Arar, Saudi Arabia
- 4Department of Anatomy, School of Dentistry, Shaheed Zulfiqar Ali Bhutto Medical University, SZABMU, Islamabad, Pakistan
- 5Mechanical Engineering, Faculty of Engineering and Technology, Future University in Egypt, New Cairo, Egypt
In this research, a mathematical model is disclosed that elucidates the peristaltic flow of carbon nanotubes in an elliptic duct with ciliated walls. This novel topic of nanofluid flow is addressed for an elliptic domain for the very first time. The practical applications of current analysis include the customization of the mechanical peristaltic pumps, artificial cilia and their role in flow control, drug delivery and prime biological applications etc. The dimensional mathematical problem is transformed into its non-dimensional form by utilizing appropriate transformations and dimensionless parameters. Exact mathematical solutions are computed over the elliptic domain for the partial differential equations appearing in this convection heat transfer problem. A thorough graphical assessment is performed to discuss the prime results. The graphical visualization of the flow in this elliptic duct is obtained by plotting streamlines. The viscous effects are playing a vital role in the heat enhancement as compared to the molecular conduction. Since the incrementing Brinkman number results in a declined conduction due to viscous dissipation that eventually results in an enhanced temperature profile. This research first time elucidates the impacts of nanofluid flow on the peristaltic pumping through an elliptic domain having ciliated walls. Considering water as base fluid with multi-wall Carbon nanotubes for this ciliated elliptic domain having sinusoidal boundaries.
Introduction
Peristalsis is concerned with fluid dynamics problems involving flow inside ducts with wavelike deformable walls. The flexible walls’ sinusoidal movement eventually aids in the development of flow along the duct’s axis. This fascinating subject has a wide range of functional, engineering, and medical applications, making it a hot research topic. Slurries, various heated mixtures, and chemicals are among its primary industrial applications; see Jaffrin and Shapiro [1]. These are some finest mathematical models furnished by different precise researchers that elucidate the peristaltic flow in distinct geometries like cylinders, Barton [2] had numerically examined the peristaltic flow inside a cylindrical conduit, Siddiqui and Schwarz [3] had conveyed the perturbation solution analysis on Peristaltic pumping of a non-Newtonian fluid, Nadeem et al. [4] had interpreted the Homotopy Analysis solutions on the peristaltic pumping of non-Newtonian Sisko fluid, curved channels, Nadeem and Maraj [5] had disclosed the Homotopy Perturbation solutions on the Peristaltic pumping of Hyperbolic Tangent non-Newtonian fluid thorough a curved domain, Rashid et al. [6] had also utilized the Homotopy Perturbation analysis to interpret the magnetohydrodynamics effects on the peristaltic pumping of Williamson fluid inside a curved domain, rectangular ducts, Nadeem and Akram [7] had provided exact solutions on Jeffrey fluid flow within a rectangular domain having sinusoidal wall fluctuations, Ellahi et al. [8] provided the Homotopy Perturbation analysis on the three dimensional flow inside a rectangular conduit working as a peristaltic pump, Akram and Saleem [9] had interpreted the Carreau fluid transportation inside a rectangular domain operating as a peristaltic pump, asymmetric geometry, Nadeem and Akram [10] had disclosed the peristaltic pumping activity for asymmetric domain by using the regular perturbation expansion method, Akbar et al. [11] had highlighted the impact of nanofluids on peristaltic pumping through asymmetric domain, Akbar [12] had also considered the magnetohydrodynamic effects on the peristaltic pumping through asymmetric domain and the peristaltic pumping through an elliptic duct via exact solutions was interpreted by Saleem et al. [13].
Nanofluids consist of small-scale (1–100 nm in size) nano particles that are scattered in the base fluid. Scientifically, experts prefer multi-walled carbon nanotubes (MWCNTs) been a special form of carbon nanoparticles because they are nested inside one another and possess elongated hollow cylindrical tiny molecules of sp2 carbon [14, 15]. These are useful in industrial problems where thermal conductivity is a prime factor [16]. Akbar and Nadeem [17] had reported a mathematical study that elucidates the combine analysis of peristaltic flow and nanofluids. Akbar and Butt [18] had mathematically elucidated the peristaltic flow of water as a base fluid with nano particles having distinct structures. Akbar [19] had numerically examined the nanofluid flow inside asymmetric domain via Runge-Kutta technique. Nadeem et al. [20, 21] had evaluated the nanofluids impact on the peristaltic pumping through an annular in addition to rectangular domain. Akbar et al. [22] evaluated the magnetohydrodynamic impacts on the nanofluid pumping through a vertically held asymmetric domain.
The consequence of ciliated walls on the peristalsis driven flow is also a subject of keen interest. Cilia are tiny structures (like human hair) that play a prime role in the flow development. The peristaltic flow mechanism is also studied for distinct geometries having ciliated boundaries like ciliated cylindrical tube, Butt et al. [23] had provided exact solutions on the Phan Thien Tanner non-Newtonian flow inside a ciliated cylindrical domain, Saleem et al. [24] had interpreted the effects of hybrid nanofluid on the peristaltic pumping through a ciliated curved domain, Akbar and Khan [25] highlighted the impacts of MHD on the peristaltic pumping via a ciliated asymmetric domain. Sadaf and Nadeem [26] had presented a mathematical model that relates the combine effects of ciliated walls and peristalsis with nanofluid flow. Akbar [27] had reported the mathematical investigation of peristaltic flow of CNT in a symmetric geometry having ciliated boundaries. Akbar and Butt [28] elucidated the impacts of MHD and entropy interpretation on the peristaltic pumping of water based nanofluids through a cylindrical ciliated domain. Nadeem and Sadaf [29] had investigated the trapping phenomenon of nanofluids flow via a ciliated annular domain. Vaidya et al. [30, 31] had highlighted the impacts of chemical reactions on the peristaltic pumping of Ree-Eyring non-Newtonian fluid passing through a permeable domain in addition to an asymmetric domain under MHD effects. Ashraf et al. [32] had examined the MHD impacts on nanofluid flow of Casson fluid through a cylindrical domain with sinusoidal wall fluctuations. Samuel et al. [33–35] had interpreted the effects of MHD, nanofluidic volume fraction with convection analysis through numerical techniques. Saleem et al. [36] highlighted the impacts of activation energy and magnetohydrodynamics on ciliary propelled flow of nanofluid. Ramesh et al. [37] modelled the cilia driven couple stress fluid flow under MHD effects. Farooq et al. [38] had analysed the micro polar flow developed due to cilia propelled rhythmic wave. Akbar et al. [39] modelled the pressure driven flow generated due to rhythmic activity of cilia.
A thorough and careful investigation of the available literature has disclosed that the peristaltic flow of carbon nanotubes in an elliptic duct with ciliated walls is not mathematically reported yet. Thus, the intent of the present mathematical computation is to elucidate this important topic for an elliptic domain for the first time. The base fluid considered in this mathematical study is water and multi-wall carbon nanotubes are taken into account. The viscous dissipation effect is also incorporated for a detailed study of convection heat transfer. Exact mathematical solutions are computed and the results are verified through graphical assessment. Streamlines depict the visualization of flow pattern.
Mathematical Model
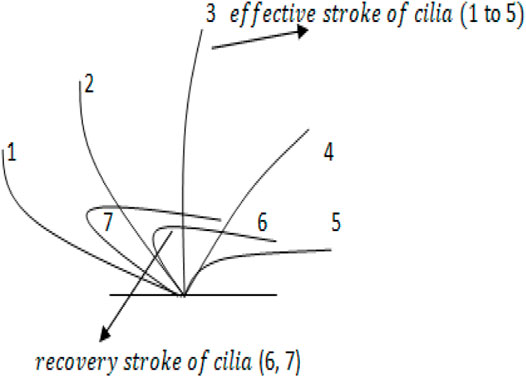
The peristaltic flow of carbon nanotubes in an elliptic duct with ciliated walls is mathematically investigated. A Cartesian coordinate system approach is utilized to interpret this mathematical study. The geometry of the problem is given by Figure 1 providing the model of elliptic domain having ciliated walls. The sinusoidal wall fluctuations are evident in Figure 1 and the ciliated wall are also mentioned. Figure 1 contains the front together with a side view of this geometry, the front view highlights the elliptic domain of this duct.

FIGURE 1. Geometrical model of the flow problem referred as [13].
The envelope model of cilia motion that considers the elliptical shape movements is taken into account. The equations considered for this model incorporates the sinusoidal metachronal wave movements generated due to rhythmic cilia motion. The computational equations that narrate this envelope model of cilia tips are referred as [40].
The following computational equations are used to express the velocities of cilia tips (i.e. axial and radial velocity respectively).
The simplification of Eqs 1, 2 provides the following equations
The above mentioned velocities
The mathematical modelling for the incompressible flow with carbon nanotubes and heat convection is provided in dimensional form as follows
The respective dimensional form of boundary conditions for ciliated walls and elliptic domain are given as
The unsteady and steady frames of reference are related by using the following equations
The non-dimensional variables that are incorporated in present analysis are given as
Whenever we deal with a non-circular domain then the concept of hydraulic diameter needs to be considered. It is considered as four times the cross-sectional area divided by the wetted perimeter of cross-section. The mathematical expression for the hydraulic diameter of elliptic duct is narrated as
The computational equation that narrates the eccentricity of ellipse is
The useful transformations provided in Eq. 10, the dimensionless variables given in Eq. 11 are used in Eqs 5–9 and the approximation
The dimensionless boundary conditions over the elliptic domain are
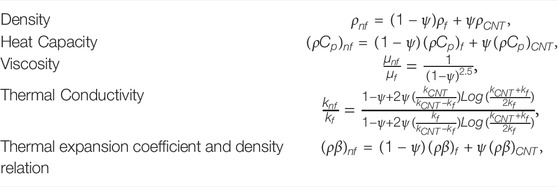
Where
Table 1 Akbar [42], provides the thermophysical properties of base fluid water and nanofluid. Table 2 Akbar [27], shows the mathematical properties of nanofluid.
Exact Solution
The dimensionless form of momentum equation is a non-homogeneous partial differential Eq. 16 subject to non-homogeneous boundary conditions (18). In order to solve this PDE, a polynomial solution method to solve PDE’s over elliptic domain is taken into account. Consider the solution in a six constants polynomial form for velocity profile as narrated below
Substituting Eq. 20 into Eq. 16 and equating the coefficients of
Now using Eq. 20 in boundary condition (18) and equating the coefficients of
The simultaneous solution of above equations gives the following values of constants
The values of these constants is inserted in Eq. 20 and exact mathematical solution is obtained for velocity as follows
The non-dimensional mathematical expression for flow rate
The pressure gradient is calculated by using Eq. 22 and given as
The pressure rise for a single wave-length is computed by using
The technique that was utilized to get an exact velocity solution is employed again to get an exact temperature function given as
Analysis of Results
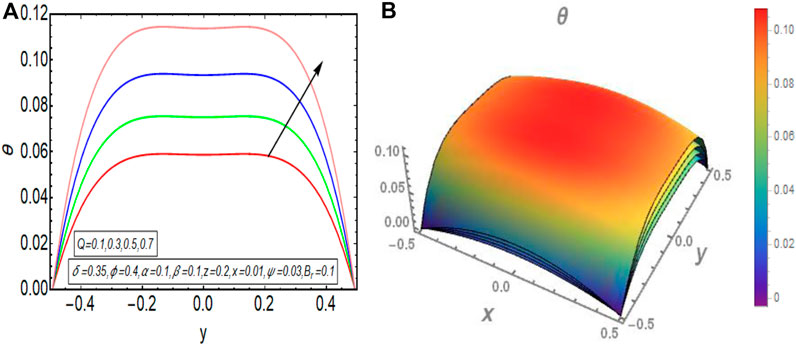
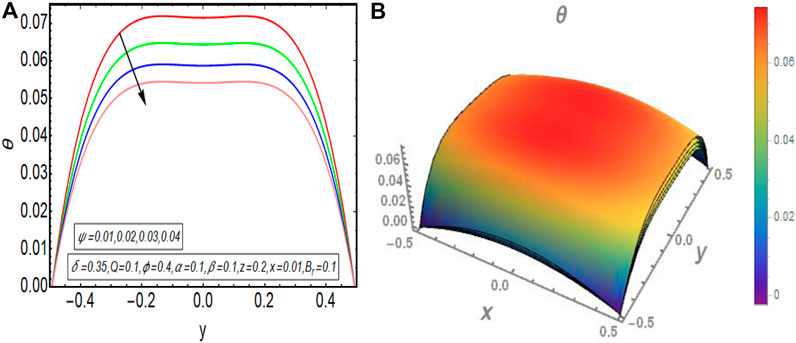
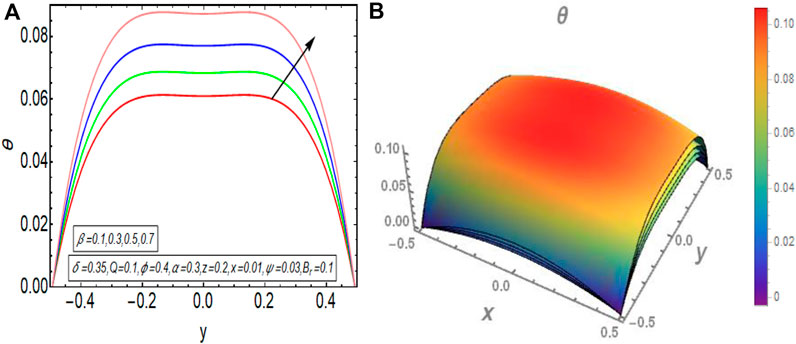
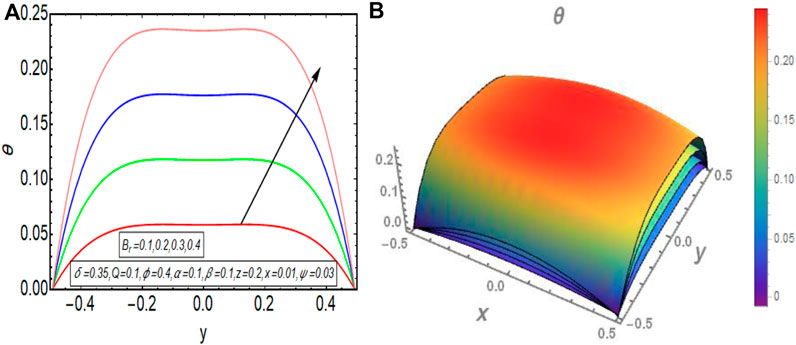
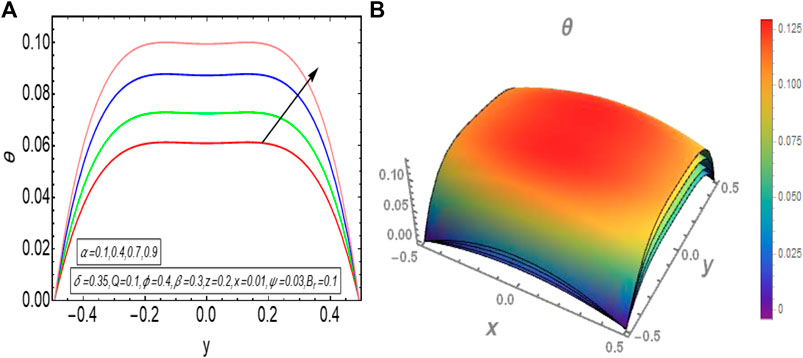
The results obtained in the exact solution section, that is exact solution of momentum equation given by Eq. 21 exactly satisfies the corresponding momentum Eq. 16 and boundary condition (18). Further, the exact solution of temperature profile given by Eq. 25 also satisfies the corresponding energy Eq. 17 with boundary condition (19). The validation of these solutions is also confirmed by using Mathematica software. According to the modelled problem and relevant boundary conditions, the flow profile in addition to the temperature profile should have a maximum value in the centre of elliptic domain and both the profiles should decline towards the walls of channel. Thus, it is evident from the graphical results that flow and temperature are maximum in the centre and reduces towards the boundaries. The temperature graphs show that temperature profile declines toward the boundaries and becomes zero as it was considered in the boundary condition (19). If
Discussion of Results
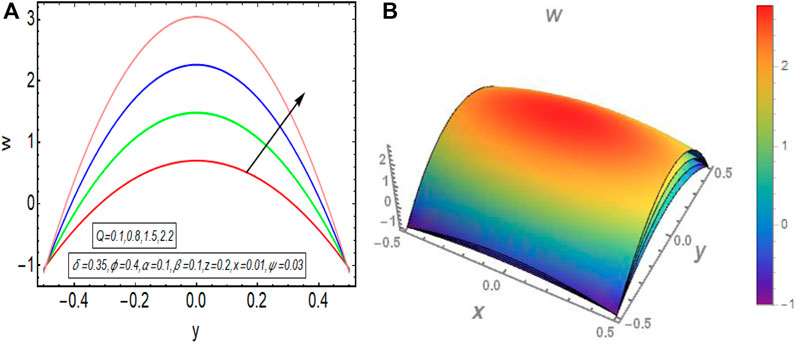
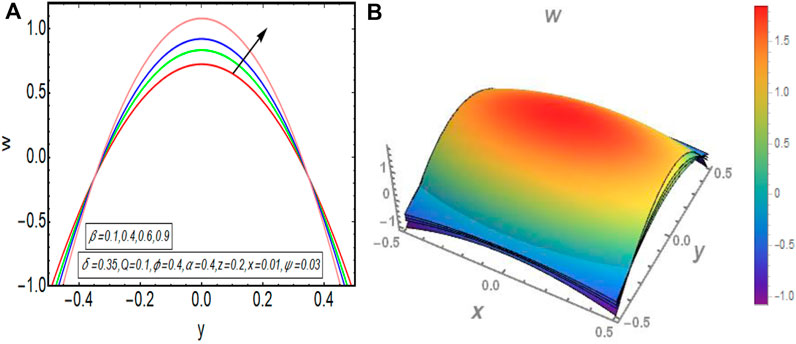
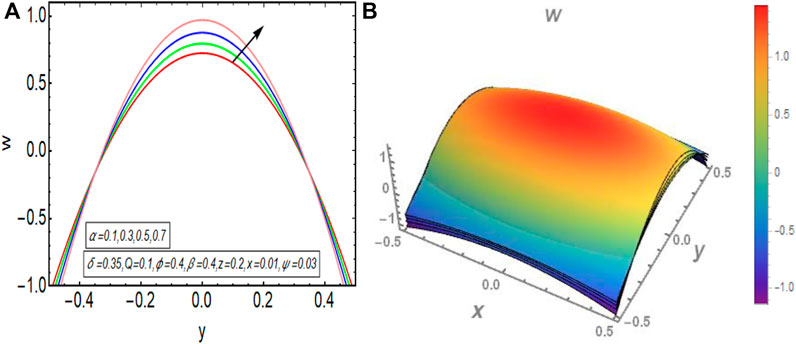
The graphical results that are incorporated in this section provide a comprehensive evaluation of the mathematical computations given in the exact solution segment. A thorough graphical evaluation is considered for velocity, temperature and various physical characteristics of peristalsis. Figures 3–5 unfold the effect of some important physical parameters on the velocity profile. Figure 3 (i.e., Figures 3A,B) provides the graphical outcome of velocity profile for increasing flow rate
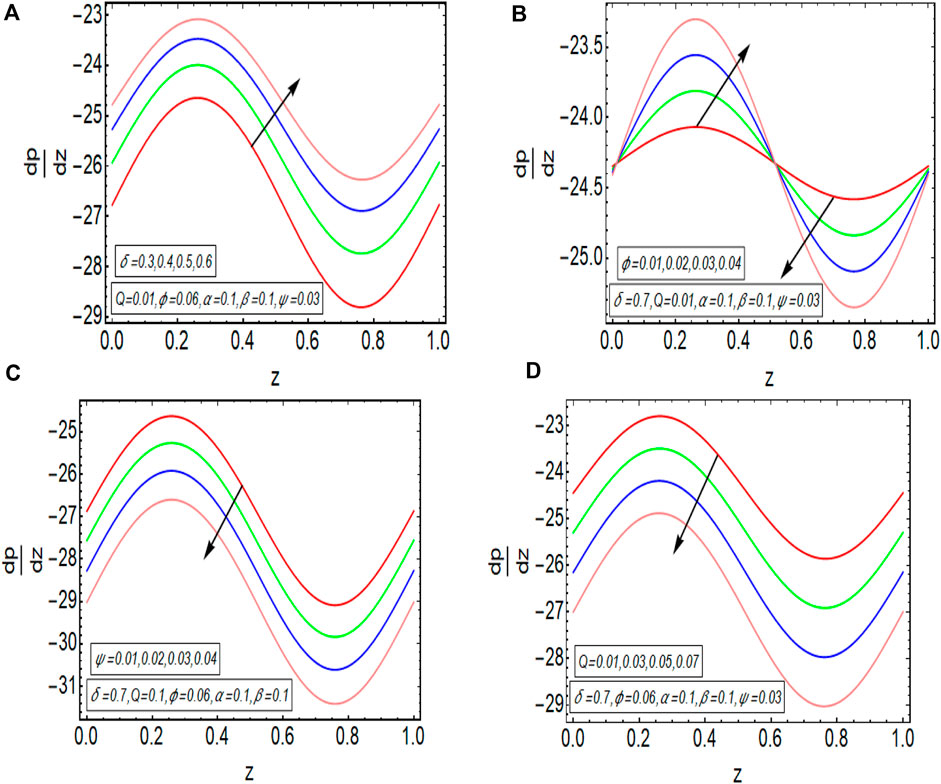
FIGURE 11. (A)
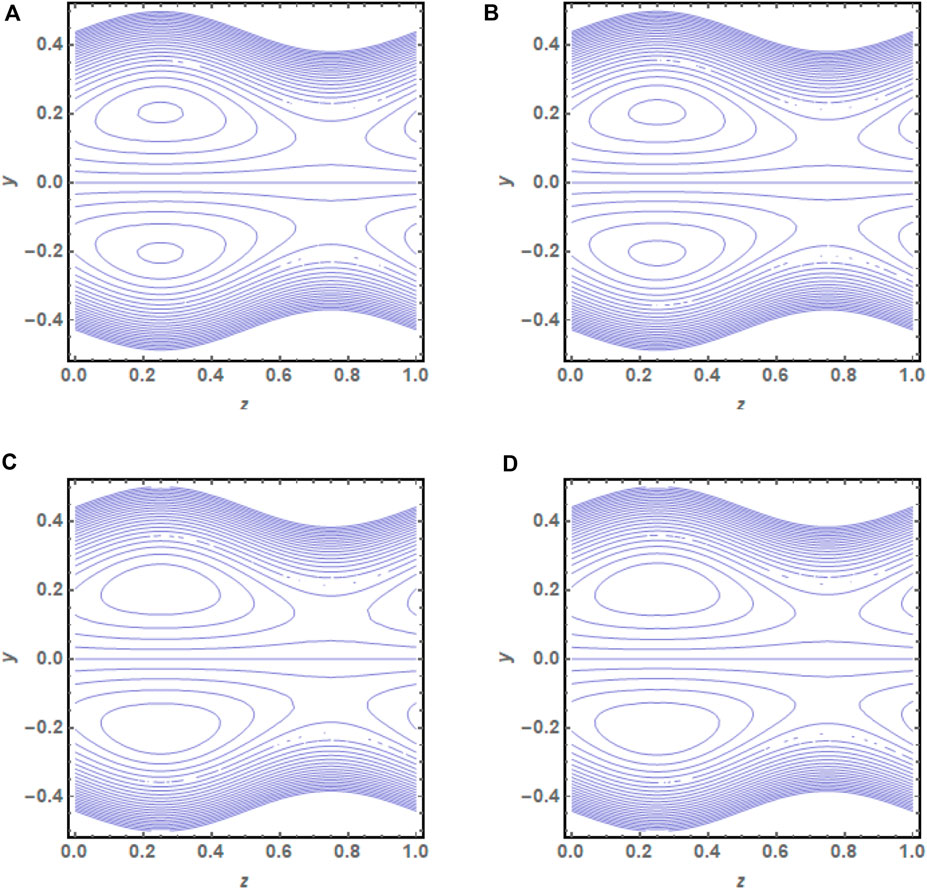
FIGURE 13. (A) Streamlines plot at flow rate
Conclusions
The peristaltic flow of carbon nanotubes in an elliptic duct with ciliated walls is mathematically investigated. A Cartesian coordinate system approach is utilized to interpret this mathematical study. The prime outcomes are given below.
• All the graphical outcomes of velocity reveal an axially symmetry flow that is a fully developed flow and velocity is diminishing towards walls of duct.
• The increasing concentration of carbon nanotubes in the base fluid escalates the thermal conductivity of fluid and this eventually results in decline of temperature of base fluid.
• The inverse relation between the thermal conductivity of fluid and the temperature of fluid is noted.
• All the temperature graphs reveal an axial symmetry profile of temperature. Further, temperature gains maximum escalation in the centre of duct and drops to zero at the duct walls.
• The escalation in the trapping size can be clearly seen in these graphs for incrementing
Data Availability Statement
The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.
Author Contributions
SN and AS are group Heads and suggest the initial theme. SaA has done the mathematical and numerical part where as OM has done the drafting and proof reading.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Jaffrin MY, Shapiro AH. Peristaltic Pumping. Annu Rev Fluid Mech (1971) 3(1):13–37. doi:10.1146/annurev.fl.03.010171.000305
2. Barton C, Raynor S. Peristaltic Flow in Tubes. Bull Math Biophys (1968) 30(4):663–80. doi:10.1007/bf02476682
3. Siddiqui AM, Schwarz WH. Peristaltic Flow of a Second-Order Fluid in Tubes. J Non-Newtonian Fluid Mech (1994) 53:257–84. doi:10.1016/0377-0257(94)85052-6
4. Nadeem S, Akbar NS. Peristaltic Flow of Sisko Fluid in a Uniform Inclined Tube. Acta Mech Sin (2010) 26(5):675–83. doi:10.1007/s10409-010-0356-1
5. Nadeem S, Maraj EN. The Mathematical Analysis for Peristaltic Flow of Hyperbolic tangent Fluid in a Curved Channel. Commun Theor Phys (2013) 59(6):729–36. doi:10.1088/0253-6102/59/6/14
6. Rashid M, Ansar K, Nadeem S. Effects of Induced Magnetic Field for Peristaltic Flow of Williamson Fluid in a Curved Channel. Physica A: Stat Mech its Appl (2020) 553:123979. doi:10.1016/j.physa.2019.123979
7. Nadeem S, Akram S. Peristaltic Flow of a Jeffrey Fluid in a Rectangular Duct. Nonlinear Anal Real World Appl (2010) 11(5):4238–47. doi:10.1016/j.nonrwa.2010.05.010
8. Ellahi R, Riaz A, Nadeem S. Three Dimensional Peristaltic Flow of Williamson Fluid in a Rectangular Duct. Indian J Phys (2013) 87(12):1275–81. doi:10.1007/s12648-013-0340-2
9. Akram S, Saleem N. Analysis of Heating Effects and Different Wave Forms on Peristaltic Flow of Carreau Fluid in Rectangular Duct. Adv Math Phys 2020 (2020):1–14. doi:10.1155/2020/8294318
10. Nadeem S, Akram S. Peristaltic Flow of a Williamson Fluid in an Asymmetric Channel. Commun Nonlinear Sci Numer Simul (2010) 15(7):1705–16. doi:10.1016/j.cnsns.2009.07.026
11. Akbar NS, Raza M, Ellahi R. Interaction of Nanoparticles for the Peristaltic Flow in an Asymmetric Channel with the Induced Magnetic Field. Eur Phys J Plus (2014) 129(7):155. doi:10.1140/epjp/i2014-14155-6
12. Sher Akbar N. Influence of Magnetic Field on Peristaltic Flow of a Casson Fluid in an Asymmetric Channel: Application in Crude Oil Refinement. J Magn Magn Mater (2015) 378:463–8. doi:10.1016/j.jmmm.2014.11.045
13. Saleem A, Akhtar S, Nadeem S, Alharbi FM, Ghalambaz M, Issakhov A. Mathematical Computations for Peristaltic Flow of Heated Non-newtonian Fluid inside a Sinusoidal Elliptic Duct. Phys Scr (2020) 95(10):105009. doi:10.1088/1402-4896/abbaa3
14. Animasaun IL, Shah NA, Wakif A, Mahanthesh B, Sivaraj R, Koriko OK. Ratio of Momentum Diffusivity to thermal Diffusivity: Introduction, Meta-Analysis, and Scrutinization (2022).
15. Rasool G, Saeed AM, Lare AI, Abderrahmane A, Guedri K, Vaidya H, et al. Darcy-Forchheimer Flow of Water Conveying Multi-Walled Carbon Nanoparticles through a Vertical Cleveland Z-Staggered Cavity Subject to Entropy Generation. Micromachines (2022) 13(5):744. doi:10.3390/mi13050744
16. Choi SU, Eastman JA. Enhancing thermal Conductivity of Fluids with Nanoparticles. IL (United States): Argonne National Lab. (1995). (No. ANL/MSD/CP-84938; CONF-951135-29).
17. Akbar NS, Nadeem S. Endoscopic Effects on Peristaltic Flow of a Nanofluid. Commun Theor Phys (2011) 56(4):761–8. doi:10.1088/0253-6102/56/4/28
18. Akbar NS, Butt AW. Ferromagnetic Effects for Peristaltic Flow of Cu-Water Nanofluid for Different Shapes of Nanosize Particles. Appl Nanosci (2016) 6(3):379–85. doi:10.1007/s13204-015-0430-x
19. Akbar NS. Peristaltic Sisko Nano Fluid in an Asymmetric Channel. Appl Nanosci (2014) 4(6):663–73. doi:10.1007/s13204-013-0205-1
20. Nadeem S, Riaz A, Ellahi R, Akbar NS. Effects of Heat and Mass Transfer on Peristaltic Flow of a Nanofluid between Eccentric Cylinders. Appl Nanosci (2014) 4(4):393–404. doi:10.1007/s13204-013-0225-x
21. Nadeem S, Riaz A, Ellahi R, Akbar NS. Mathematical Model for the Peristaltic Flow of Jeffrey Fluid with Nanoparticles Phenomenon through a Rectangular Duct. Appl Nanosci (2014) 4(5):613–24. doi:10.1007/s13204-013-0238-5
22. Akbar NS, Raza M, Ellahi R. Impulsion of Induced Magnetic Field for Brownian Motion of Nanoparticles in Peristalsis. Appl Nanosci (2016) 6(3):359–70. doi:10.1007/s13204-015-0447-1
23. Butt AW, Akbar NS, Mir NA. Heat Transfer Analysis of Peristaltic Flow of a Phan-Thien-Tanner Fluid Model Due to Metachronal Wave of Cilia. Biomech Model Mechanobiol (2020) 19:1925–33. doi:10.1007/s10237-020-01317-4
24. Saleem A, Akhtar S, Alharbi FM, Nadeem S, Ghalambaz M, Issakhov A. Physical Aspects of Peristaltic Flow of Hybrid Nano Fluid inside a Curved Tube Having Ciliated wall. Results Phys (2020) 19:103431. doi:10.1016/j.rinp.2020.103431
25. Akbar NS, Khan ZH. Influence of Magnetic Field for Metachoronical Beating of Cilia for Nanofluid with Newtonian Heating. J Magn Magn Mater (2015) 381:235–42. doi:10.1016/j.jmmm.2014.12.086
26. Sadaf H, Nadeem S. Influences of Slip and Cu-Blood Nanofluid in a Physiological Study of Cilia. Comp Methods Programs Biomed (2016) 131:169–80. doi:10.1016/j.cmpb.2016.04.008
27. Sher Akbar N. Biomathematical Analysis of Carbon Nanotubes Due to Ciliary Motion. Int J Biomath (2015) 08(02):1550023. doi:10.1142/s1793524515500230
28. Akbar NS, Butt AW. Entropy Generation Analysis for Metachronal Beating of Ciliated Cu-Water Nanofluid with Magnetic Field. Int. J. Exergy (2016) 19(1):41–54. doi:10.1504/ijex.2016.074266
29. Nadeem S, Sadaf H. Trapping Study of Nanofluids in an Annulus with Cilia. AIP Adv (2015) 5(12):127204. doi:10.1063/1.4937474
30. Vaidya H, Choudhari R, Mebarek-Oudina F, Animasaun IL, Prasad KV, Makinde OD. Combined Effects of Homogeneous and Heterogeneous Reactions on Peristalsis of Ree-Eyring Liquid: Application in Hemodynamic Flow. Heat Trans (2021) 50(3):2592–609. doi:10.1002/htj.21995
31. Vaidya H, Rajashekhar C, Divya BB, Manjunatha G, Prasad KV, Animasaun IL. Influence of Transport Properties on the Peristaltic MHD Jeffrey Fluid Flow through a Porous Asymmetric Tapered Channel. Results Phys (2020) 18:103295. doi:10.1016/j.rinp.2020.103295
32. Ashraf MU, Qasim M, Wakif A, Afridi MI, Animasaun IL. A Generalized Differential Quadrature Algorithm for Simulating Magnetohydrodynamic Peristaltic Flow of Blood-Based Nanofluid Containing Magnetite Nanoparticles: a Physiological Application. Numer Methods Partial Diff Equations (2020) 38(3): 666–692. doi:10.1002/num.22676
33. Oke AS. Heat and Mass Transfer in 3d mhd Flow of eg-Based Ternary Hybrid Nanofluid Over a Rotating Surface. Arabian J Sci Eng 2022 (2022) 1–17. doi:10.1007/s13369-022-06838-x
34. Kinyanjui Kigio J, Winifred Nduku M, Abayomi Samuel O. Analysis of Volume Fraction and Convective Heat Transfer on MHD Casson Nanofluid over a Vertical Plate. Fluid Mech (2021) 7(1):1. doi:10.11648/j.fm.20210701.11
35. Oke AS, Animasaun IL, Mutuku WN, Kimathi M, Shah NA, Saleem S. Significance of Coriolis Force, Volume Fraction, and Heat Source/sink on the Dynamics of Water Conveying 47 Nm Alumina Nanoparticles over a Uniform Surface. Chin J Phys (2021) 71:716–27. doi:10.1016/j.cjph.2021.02.005
36. Saleem N, Munawar S, Tripathi D. Entropy Analysis in Ciliary Transport of Radiated Hybrid Nanofluid in Presence of Electromagnetohydrodynamics and Activation Energy. Case Stud Therm Eng (2021) 28:101665. doi:10.1016/j.csite.2021.101665
37. Ramesh K, Tripathi D, Anwar Bég O. Cilia-Assisted Hydromagnetic Pumping of Biorheological Couple Stress Fluids. Propul Power Res (2019) 8(3):221–33. doi:10.1016/j.jppr.2018.06.002
38. Farooq AA, Tripathi D, Elnaqeeb T. On the Propulsion of Micropolar Fluid inside a Channel Due to Ciliary Induced Metachronal Wave. Appl Math Comput (2019) 347:225–35. doi:10.1016/j.amc.2018.10.021
39. Akbar NS, Tripathi D, Khan ZH, Bég OA. Mathematical Modelling of Pressure-Driven Micropolar Biological Flow Due to Metachronal Wave Propulsion of Beating Cilia. Math Biosci (2018) 301:121–8. doi:10.1016/j.mbs.2018.04.001
40. Sadaf H, Nadeem S. Fluid Flow Analysis of Cilia Beating in a Curved Channel in the Presence of Magnetic Field and Heat Transfer. Can J Phys (2020) 98(2):191–7. doi:10.1139/cjp-2018-0715
41. Yang ZH, Chu YM, Zhang W. Monotonicity of the Ratio for the Complete Elliptic Integral and Stolarsky Mean. J Inequalities Appl (2016) 2016(1):1–10. doi:10.1186/s13660-016-1113-1
42. Akbar N. Entropy Generation Analysis for a CNT Suspension Nanofluid in Plumb Ducts with Peristalsis. Entropy (2015) 17(3):1411–24. doi:10.3390/e17031411
Nomenclature
MWCNT Multi wall carbon nanotubes
Keywords: peristaltic flow, elliptic duct, nanofluid (CNT), ciliated walls, exact solutions
Citation: Ghazwani HA, Akhtar S, Almutairi S, Saleem A, Nadeem S and Mahmoud O (2022) Insightful Facts on Peristalsis Flow of Water Conveying Multi-Walled Carbon Nanoparticles Through Elliptical Ducts With Ciliated Walls. Front. Phys. 10:923290. doi: 10.3389/fphy.2022.923290
Received: 19 April 2022; Accepted: 25 May 2022;
Published: 24 June 2022.
Edited by:
Animasaun I. L., Federal University of Technology, NigeriaReviewed by:
Abayomi Samuel Oke, Adekunle Ajasin University, NigeriaDharmendra Tripathi, National Institute of Technology Uttarakhand, India
M. D. Shamshuddin, Vaagdevi College of Engineering, India
Copyright © 2022 Ghazwani, Akhtar, Almutairi, Saleem, Nadeem and Mahmoud. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Sohail Nadeem, c29oYWlsQHFhdS5lZHUucGs=
 Hassan Ali Ghazwani1
Hassan Ali Ghazwani1 Salman Akhtar
Salman Akhtar Sohail Nadeem
Sohail Nadeem