- School of Computer Science and Network Security, Dongguan University of Technology, Dongguan, China
Unextendible product basis (UPB), a set of incomplete orthonormal product states whose complementary space has no product state, is very useful for constructing bound entangled states. Naturally, instead of considering the set of product states, Bravyi and Smolin considered the set of maximally entangled states. They introduced the concept of unextendible maximally entangled basis (UMEB), a set of incomplete orthonormal maximally entangled states whose complementary space contains no maximally entangled state [Phys. Rev. A 84, 042,306 (2011)]. An entangled state whose nonzero Schmidt coefficients are all equal to
1 Introduction
Quantum entanglement [1] is an important resource for many quantum information processing, such as quantum teleportation [2, 3] and quantum key distribution [4, 5]. Therefore, it is fundamental to characterize quantum entanglement in quantum information. Bound entangled (BE) states [6, 7] are a special entanglement in nature: non-zero amount of free entanglement is needed to create them but no free entanglement can be distilled from such states under local operations and classical communication.
Unextendible product basis (UPB) [8, 9], a set of incomplete orthonormal product states whose complementary space has no product state, has been shown to be useful for constructing bound entangled states and displaying quantum nonlocality without entanglement [10–12].
As anology of the UPB, Bravyi and Smolin introduced the concept of unextendible maximally entangled basis (UMEB) [13], a set of orthonormal maximally entangled states in
Guo et al. extended these two concepts to the states with fixed Schmidt numbers and studied the complete basis [22] and the unextendible ones [23]. There they introduced the notion of special entangled states of type k: an entangled state whose nonzero Schmidt coefficients are all equal to
The remaining of this article is organized as follows. In Section 2, we first introduce the concept of special unextendible entangled basis and its equivalent form in matrix settings. In Section 3, we present our main idea to construct the SUEBk. In Section 4 and Section 5, based on the combinatoric concept: weighing matrices, we give two constructions of SUEBk whose cardinality varying in a consecutive integer set. Finally, we draw the conclusions and put forward some interesting questions in the last section.
2 Preliminaries
Let [n] denote the set {1, 2, … , n}. Let
where λi > 0 and
Definition 1. (See [22]). A set of states
(i) ⟨ϕi|ϕj⟩ = δij, i, j ∈ [n];
(ii) For any i ∈ [n], the state |ϕi⟩ is a special entangled state of type k;
(iii) If ⟨ϕi|ϕ⟩ = 0 for all i ∈ [n], then |ϕ⟩ can not be a special entangled state of type k.
The concept SUEBk generalizes the UPB (k = 1) and the UMEB (k = d). In order to study SUEBk, it is useful to consider its matrix form. Let |ϕ⟩ be a pure quantum states in
We call the d × d′ matrix
(1) Inner product preserving:
(2) The Schmidt number corresponds to the matrix rank: Sr (|ϕ⟩) = rank (Mϕ);
(3) The nonzero Schmidt coefficients correspond to the nonzero singular values.
With this correspondence, we can restate the concept in definition 2 as follows.
Definition 2. A set of matrices
(i) ⟨Mi, Mj⟩ = δij, i, j ∈ [n];
(ii) The nonzero singular values of Mi are all equal to
(iii) If ⟨Mi, M⟩ = 0 for all i ∈ [n], then some nonzero singular value of M do not equal to
Due to the good correspondence of the states and matrices,
3 Strategy for Constructing SUSVBk
Observation 1. It is usually not easy to calculate the singular values of an arbitrary matrix. However, if there are only k nonzero elements in M (say
where
Observation 2. If there are exactly k nonzero singular values of a matrix, then the rank of that matrix is k. Therefore, the condition rank(M) < k implies that M cannot be a matrix with k nonzero singular values.
With the two observations above, our strategy for constructing an n-members SUSVBk can be roughly described by two steps (note that this is only a sufficient condition, not a necessary and sufficient condition). Firstly, we construct a set of n-members of orthonormal matrices
Let d, d′ be integers such that 2 ≤ d ≤ d′. We define the coordinate set to be
Now we define an order for the set
Then we call
Let (i1, j1), (i2, j2) be two different coordinates in
Let
In order to step forward, we first state the following observation which is helpful for determine the orthogonality of matrices. Let
where Ei,j denote the d × d′ matrix whose (i, j) coordinate is 1 and zero elsewhere.
Lemma 1. Let
(1) If P1 ∩ P2 = ∅, then we have
(2) If P1 = P2 and v, w are orthogonal to each other, then we also have
Proof. Denote
(a) As
we have
The last equality holds as the condition P1 ∩ P2 = ∅ implies δikδjl = 0.
(b) For the second part, we have the following equalities:
4 Constructions of SUEBk
In the following, we try to construct a set of matrices
We start our construction by a simple example.
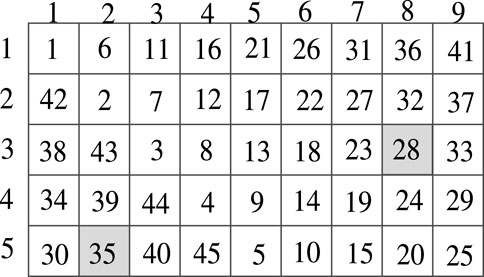
Example 1. There exists a SUEB3 in
Proof. As 41 = 6 × 7–1, we define
Since there are 41 elements in the set
where
where
Let
we have dim V⊥ = 8. Note that the dimension of
One can find that the H3 and O4 play an important role in the proof of the Example 1. We give their generalizations by the following matrix and the weighing matrix in Definition 3. There always exists some complex Hadamard matrix of order d. For example,
where
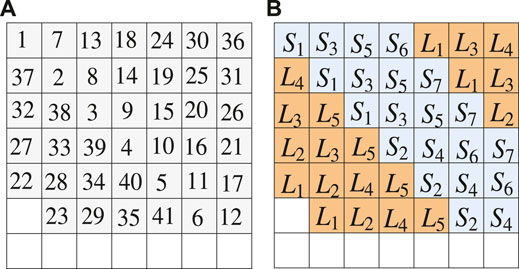
FIGURE 2. (A) shows the order of subset of
Definition 3. (See [27]). A generalized weighing matrix is a square a × a matrix A all of whose non-zero entries are nth roots of unity such that AA† = kIa. It follows that
One can find the following lemma via theorem 2.1.1 on the book “The Diophantine Frobenius Problem” [28]. The related problem is also known as Frobenius coin problem or coin problem.
Lemma 2. ([28]). Let a, b be positive integers and coprime. Then for every integer N ≥ (a − 1) (b − 1), there are non-negative integers x, y such that N = xa + yb.
Now we give one of the main result of this paper.
Theorem 1. Let k be a positive integer. Suppose there exist
Proof. Without loss of generality, we suppose a < b and A ∈ W (m, k, a), B ∈ W (n, k, b). Any integer N ∈ [(d − k + 1)d′, dd’ − 1] can be written uniquely as N = d′q + r where (d − k + 1) ≤ q ≤ d − 1 and r is an integer with 0 ≤ r < d′. Then we have a coordinate set
Since there are N elements in the set
Now let vx be the xth row of
Let
Noticing that Hk ∈ W (k, k, k) for all integer k ≥ 2. Therefore, by Theorem 1, we arrive at the following corollary.
Corollary 1. Let k be an integer such that W (n, k, k + 1) is nonempty for some integer n. Then there exists some SUEBk with cardinality varying from (d − k + 1)d′ to dd’ − 1 in
In the following, we list a result about the weighing matrices proved by Gerald Berman.
Lemma 3. (See [27]). If p, t, r and n are positive integers such that p is prime, n|r (n ≥ 2) and r|(pm − 1). Then there exists a generalized weighing matrix W (n, p(t−1)m, (ptm − 1)/r).
In particular, set t = 2, r = n = pm − 1. If pm > 2, then W (pm − 1, pm, pm + 1) is nonempty. As the set W (pm, pm, pm) is always nonempty, we have the following corollaries.
Corollary 2. Let p be a prime and k = pm > 2 for some positive integer m. Then there exists some SUEBk with cardinality varying from (d − k + 1)d′ to dd’ − 1 in
Corollary 3. Let p1, … , ps be different primes and
5 Second Type of SUEBk
In the following, we try to construct a set of matrices
We also start our construction from a simple example.
Example 2. There exists a SUEB4 in
Proof. As 54 = 7 × 8–2, we can define
Since there are 54 elements in the set
See Figure 3B for an intuitive view of the set Si, Lj. Set
We can easily check that
Let

FIGURE 3. (A) shows the order of subset of
Theorem 2. Let k be a positive integer. Suppose there exist
Proof. Without loss of generality, we suppose a < b and A ∈ W (m, k, a), B ∈ W (n, k, b). We separate the interval
Any integer N ∈ [m1m2, dd’ − 1] lies in one of the above q + r intervals. Without loss of generality, we assume that
Since there are N elements in the set
Now set vx to be the xth row of
Let
Remark: Theorem 1 (the first type) can not obtain from Theorem 2 (the second type) by setting r = 0. In fact, in Theorem 2, we assume d, d′ ≥ max{a, b} + 2 while we only assume d′ ≥ max{a, b} + 1 in Theorem 1.
As application, Theorem 2 give us that there is some SUEB4 in
In fact, we may move further than the results showed in Theorem 2. Here we present some examples (See Example 3) which is beyond the scope of Theorem 2. But their proof can be originated from the main idea of the constructions of SUEBk.
Example 3. For any integer N ∈ [12, 19], there exists a SUEB3 in
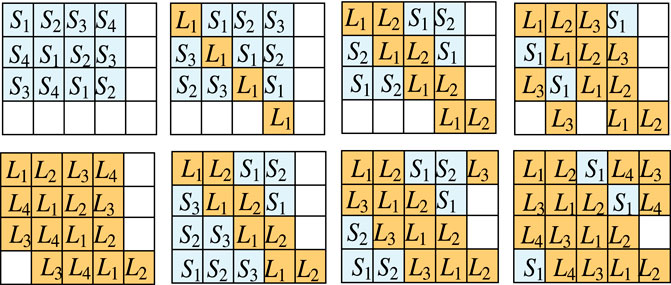
FIGURE 4. This figure shows the distribution of the short states and long states for constructing SUEB3 in
6 Conclusion and Discussion
We presented a method to construct the special unextendible entangled basis of type k. The main idea here is to decompose the whole space into two subspaces such that the rank of each element in one subspace is easily bounded by k and the other can be generated by two kinds of the special entangled states of type k. We presented two constructions of special unextendible entangled states of type k by relating it to a combinatoric concept which is known as weighing matrices. This method is effective when k = pm ≥ 3.
However, there are lots of unsolved cases. Finding out the largest linear subspace such that it does not contain any special entangled states of type k. This is related to determine the minimal cardinality of possible SUEBk. It is much more interesting to find some other methods that can solve the general existence of SUEBk. Note that the concept of SUEBk is a mathematical generalization of the UPB (k = 1) and the UMEB (k = d). Both UPBs and UMEBs are useful for studying some other problems in quantum information. Therefore, another interesting work is to find out some applications of the SUEBk.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Materials, further inquiries can be directed to the corresponding author.
Author Contributions
The author confirms being the sole contributor of this work and has approved it for publication.
Funding
This work is supported by the NSFC with Grant No. 11901084, the Basic and Applied Basic Research Funding Program of Guangdong Province with Grant No. 2019A1515111097, and the Research startup funds of DGUT with Grant No. GC300501-103.
Conflict of Interest
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The handling editor LC declared a past co-authorship with the author YLW.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Acknowledgments
The author thank Mao-Sheng Li for helpful discussion.
References
1. Horodecki R, Horodecki P, Horodecki M, Horodecki K. Quantum Entanglement. Rev Mod Phys (2009) 81:865–942. doi:10.1103/revmodphys.81.865
2. Bennett CH, Brassard G, Crépeau C, Jozsa R, Peres A, Wootters WK. Teleporting an Unknown Quantum State via Dual Classical and Einstein-Podolsky-Rosen Channels. Phys Rev Lett (1993) 70:1895–9. doi:10.1103/physrevlett.70.1895
3. Bouwmeester D, Pan J-W, Mattle K, Eibl M, Weinfurter H, Zeilinger A. Experimental Quantum Teleportation. Nature (1997) 390:575–9. doi:10.1038/37539
4. Bennett CH. Quantum Cryptography Using Any Two Nonorthogonal States. Phys Rev Lett (1992) 68:3121–4. doi:10.1103/physrevlett.68.3121
5. Gisin N, Ribordy G, Tittel W, Zbinden H. Quantum Cryptography. Rev Mod Phys (2002) 74:145–95. doi:10.1103/revmodphys.74.145
6. Horodecki P, Lewenstein M. Bound Entanglement and Continuous Variables. Phys Rev Lett (2000) 85:2657–60. doi:10.1103/physrevlett.85.2657
7. Bruß D, Peres A. Construction of Quantum States with Bound Entanglement. Phys Rev A (2000) 61(R):030301. doi:10.1103/physreva.61.030301
8. Bennett CH, DiVincenzo DP, Mor T, Shor PW, Smolin JA, Terhal BM. Unextendible Product Bases and Bound Entanglement. Phys Rev Lett (1999) 82:5385–8. doi:10.1103/physrevlett.82.5385
9. DiVincenzo DP, Mor T, Shor PW, Smolin JA, Terhal BM. Unextendible Product Bases, Uncompletable Product Bases and Bound Entanglement. Commun Math Phys (2003) 238:379–410. doi:10.1007/s00220-003-0877-6
10. Bennett CH, DiVincenzo DP, Fuchs CA, Mor T, Rains E, Shor PW, et al. Quantum Nonlocality without Entanglement. Phys Rev A (1999) 59:1070–91. doi:10.1103/physreva.59.1070
11. De Rinaldis S. Distinguishability of Complete and Unextendible Product Bases. Phys Rev A (2004) 70:022309. doi:10.1103/physreva.70.022309
12. Horodecki M, Sen A, Sen U, Horodecki K. Local Indistinguishability: More Nonlocality with Less Entanglement. Phys Rev Lett (2003) 90:047902. doi:10.1103/PhysRevLett.90.047902
13. Bravyi S, Smolin JA. Unextendible Maximally Entangled Bases. Phys Rev A (2011) 84:042306. doi:10.1103/physreva.84.042306
14. Chen B, Fei S-M. Unextendible Maximally Entangled Bases and Mutually Unbiased Bases. Phys Rev A (2013) 88:034301. doi:10.1103/physreva.88.034301
15. Li M-S, Wang Y-L, Zheng Z-J. Unextendible Maximally Entangled Bases in $\Mathbb{C}^{d}\bigotimes\Mathbb{C}^{d^{\prime}}$. Phys Rev A (2014) 89:062313. doi:10.1103/PhysRevA.90.034301
16. Wang Y-L, Li M-S, Fei S-M. Unextendible Maximally Entangled Bases in $\Mathbb{C}^{d}\bigotimes\Mathbb{C}^{d}$. Phys Rev A (2014) 90:034301. doi:10.1103/PhysRevA.89.062313
17. Nan H, Tao Y-H, Li L-S, Zhang J. Unextendible Maximally Entangled Bases and Mutually Unbiased Bases in ℂ D ⊗ ℂ d′$\Mathbb{C}^{d}\bigotimes\Mathbb{C}^{d^{\prime}}$. Int J Theor Phys (2015) 54:927–32. doi:10.1007/s10773-014-2288-1
18. Wang Y-L, Li M-S, Fei S-M, Zheng Z-J. Connecting Unextendible Maximally Entangled Base with Partial Hadamard Matrices. Quan Inf. Process (2017) 16:84. doi:10.1007/s11128-017-1537-7
19. Guo Y, Jia Y, Li X. Multipartite Unextendible Entangled Basis. Quan Inf Process (2015) 14:3553–68. doi:10.1007/s11128-015-1058-1
20. Guo Y. Constructing the Unextendible Maximally Entangled Basis from the Maximally Entangled Basis. Phys Rev A (2016) 94:052302. doi:10.1103/physreva.94.052302
21. Zhang G-J, Tao Y-H, Han Y-F, Yong X-L, Fei S-M. Unextendible Maximally Entangled Bases in $${\mathbb {C}}^{pd}\otimes {\mathbb {C}}^{qd}$$ C Pd ⊗ C qd$\Mathbb{C}^{qd}\otimes \Mathbb{C}^{qd}$. Quan Inf. Process (2018) 17:318. doi:10.1007/s11128-018-2094-4
22. Guo Y, Du S, Li X, Wu S. Entangled Bases with Fixed Schmidt Number. J Phys A: Math Theor (2015) 48:245301. doi:10.1088/1751-8113/48/24/245301
23. Guo Y, Wu S. Unextendible Entangled Bases with Fixed Schmidt Number. Phys Rev A (2014) 90:054303. doi:10.1103/physreva.90.054303
24. Shi F, Zhang X, Guo Y. Constructions of Unextendible Entangled Bases. Quan Inf. Process (2019) 18:324. doi:10.1007/s11128-019-2435-y
25. Yong X, Song Y, Tao Y. New Constructions of Unextendible Entangled Bases with Fixed Schmidt Number. Quan Inf. Process (2019) 18:337. doi:10.1007/s11128-019-2451-y
26. Li M-S, Wang Y-L. Construction of Special Entangled Basis Based on Generalized Weighing Matrices. J Phys A: Math Theor (2019) 52:375303. doi:10.1088/1751-8121/ab331b
27. Berman G. Families of Generalized Weighing Matrices. Can J Math (1978) 30:1016–28. doi:10.4153/cjm-1978-086-8
Keywords: unextendible entangled bases, unextendible product bases, entanglement, schmidt number, schmidt coefficients
Citation: Wang Y-L (2022) Constructions of Unextendible Special Entangled Bases. Front. Phys. 10:884327. doi: 10.3389/fphy.2022.884327
Received: 26 February 2022; Accepted: 20 April 2022;
Published: 27 May 2022.
Edited by:
Lin Chen, Beihang University, ChinaReviewed by:
Yuanhong Tao, Zhejiang University of Science and Technology, ChinaZhenhuan Liu, Tsinghua University, China
Hao Dai, Tsinghua University, China
Copyright © 2022 Wang. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Yan-Ling Wang, d2FuZ3lsbWF0aEB5YWhvby5jb20=
 Yan-Ling Wang
Yan-Ling Wang