
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Phys., 06 January 2023
Sec. Atomic and Molecular Physics
Volume 10 - 2022 | https://doi.org/10.3389/fphy.2022.1090346
 Quanjun Wang1,2,3
Quanjun Wang1,2,3 Pengji Ding1*
Pengji Ding1* Shane G. Wilkins4
Shane G. Wilkins4 Michail Athanasakis-Kaklamanakis3,5
Michail Athanasakis-Kaklamanakis3,5 Yuxuan Zhang1
Yuxuan Zhang1 Zuoye Liu1*
Zuoye Liu1* Bitao Hu1
Bitao Hu1We perform a theoretical investigation of a collective emission in tunnel-ionized nitrogen molecules triggered by a coherent seed pulse. A semiclassical theory of superradiance that includes the superradiant temporal profile, characteristic duration, time delay, intensity is achieved. The theoretical predictions of 391-nm forward emission corresponding to the transition between
Tunnel ionization is a fundamental process for atoms/molecules in intense laser fields when the Keldysh parameter is less than unity [1, 2]. In the tunneling regime, not only sequential double ionization but also non-sequential double ionization exist [3, 4]. Tunnel ionization is taken as a foundation to understand other strong-field phenomena including high harmonic generation [5, 6], non-linear filamentation and laser-induced electron acceleration [7–9]. Because of the multielectron effects and the close ionization potentials between the outermost and a few lower-lying orbitals, the electrons from several molecular orbitals can be ionized in strong laser fields [10–12]. A direct consequence of the multiorbital ionization is that the molecular ions are populated not only in the ground but also in the excited electronic states. A good example is the molecular nitrogen ions inside the plasma generated by 800-nm femtosecond laser pulses, which is the subject of interest in this study. The fluorescence measurement of the plasma confirm that the molecular nitrogen ions exist in both ground
Since the ionization rate decays exponentially with the electron binding energy, the populations in the excited states should be much less than those in the ground state, e.g., no population inversion should be expected between B and X state. However, it has been experimentally demonstrated that
An important issue of radiation research is the time evolution of the radiated intensity of the system. Time-resolved measurements of
However, there still exists an understanding gap between the presence of molecular coherence in
After photonization and state couplings by 800-nm laser pulses, the molecular nitrogen ions are populated in B and X states that constitute a two-level system in the plasma. Since the intensity of the 391-nm seed pulse is weak, it can hardly induce ionization or non-resonant excitation. So only the interaction between the seed pulse and the two electronic states is significant. The electron-ion inelastic collisions and the electron-ion recombination can cause the population change (decay or increase) of the two-level system. Both of the them occur on nanosecond timescale [42–49], which is a much slower process compared to the 391-nm lasing emission that takes place in picosecond range. Hence, the population of the two-level system is considered to be conserved during the ultra-fast radiation.
Under the above conditions, the evolution of the two-level system of B (ν′ = 0) and X (ν = 0) in the radiation field can be described by the optical Bloch equations by using the slowly varying amplitude approximation and the rotating-wave approximation [50–52]:
where u and v denote dispersion and absorption terms, respectively, and w is the relative population difference between the upper and lower states. Ω(t) is the Rabi frequency which takes the form
with μ, ℏ,
where z and z′ represent the propagation direction of the seed laser pulse, and c is the speed of light. z and z′ range from 0 to L which is the filament plasma length. A pencil-shaped geometry for the active gain volume is used, i.e.,
with r being the filament radius. It is reasonable because the plasma radius is usually around 50 μm, and the filament plasma length is typically in centimeter range by using a lens of short focal length [53].
In Eqs 1–3, Γ1 = 11.76 × 106 s−1 is the transition rate [44], and the corresponding lifetime 1/Γ1 = 85 ns is about 4 orders of magnitude larger than the time delay and width (∼ps) of the 391-nm retarded emission. Γ2 is the dipole-dephasing rate, which can inhibit the formation of the macroscopic dipole moment. The radiation manifests characteristics of superfluorescence, damped superfluorescence and amplified spontaneous emission (ASE) if 1/Γ2 ≫ τD,
To solve Eqs 1–3, a Bloch angle is defined as
Then, simple solutions of the equations can be obtained as.
with the initial conditions of u (0) = 0, v (0) = 0 and w (0) = w0. By setting the eigen-energy of B and X to be ℏω/2 and −ℏω/2, the energy density of the two-level system can be expressed as
where N is sum of the population densities of the two states.
We define a Bloch vector B as
with eX, eY and eZ denoting the unit vectors of the X-, Y- and Z-axis, respectively. It is easy to obtain
As u = 0, the Bloch vector B with a fixed length w0 rotates around X-axis in Y-Z plane as shown in Figure 1. The rotation determined by the Bloch angle θ describes the evolution of the physical quantities including the energy density of the two-level system.
When t < τr, the Rabi frequency Ωp induced by the seed laser pulse is known, and the Bloch angle θ can be calculated by
where μ0 and ɛ0 denote vacuum permeability and vacuum permittivity, respectively. The radiation field Es (t′, z′) and polarization P (t′, z′) can be written in the form
where
Substituting (t′, z′) with (t, z) into Eq. 17, we get
With the initial condition
This derived expression suggests the amplitude of the radiation field emitted at the end of the plasma at t = τr.
To get a clear physical picture of Eq. 19, Figure 2 illustrates the growth of
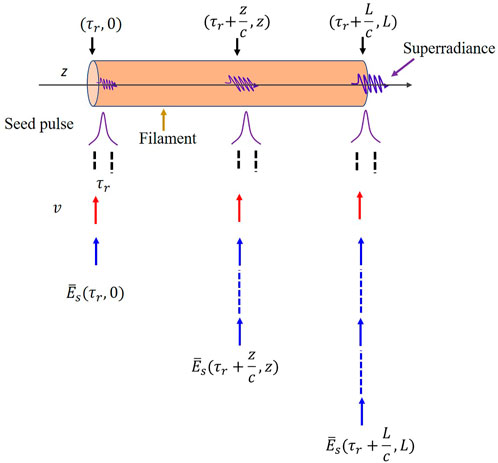
FIGURE 2. The amplitude of the radiation field
At t = τr, the energy flux density S of the radiation field from the cross section of the plasma is
The decrease of energy per unit time of the two-level system at t = τr according to Eq. 11 is
From the law of conservation of energy, the decrease of energy per unit time from the active volume equals the energy flux from the cross section, which reads
By substituting both sides respectively and assuming no external factors affecting the system, one gets the derivative of the Bloch angle with respect to time
with the initial condition (τr, θ(τr)). The solution of Eq. 23 is
with.
The energy density EN(t) of this two-level system according to Eq. 9 and the radiation intensity Is (the same as the energy flux density S) at the end of the plasma can be respectively expressed as.
From above analytical expressions, we can see that the radiation from the two-level system possesses the nature of superradiance [54–56, 60–64] featured with the characteristic duration of τW and delay of τD with respect to the seed pulse. τW is inversely proportional to the population density N and the radiation intensity is proportional to the square of N2, typical signatures of superradiance.
As described by Eq. 27, there is still energy stored in the system after interaction with the seed pulse. The release of remaining energy results in the retarded emission. The initial relative population difference w0 determined by the pump pulse and the Bloch angle θ(τr)caused by the seed pulse govern the temporal evolution of the retarded emission.
In this section, we perform comparisons between the experimental results and theoretical predictions of the temporal profile of the 391-nm lasing emission based on analytical expressions derived. The experimental results are directly taken from previous work [65], where detailed descriptions of the experiments can be found. Figure 3 shows the temporal profiles of 391-nm lasing pulse measured for different nitrogen pressures from 6 to 20 mbar. The interaction time τr for the calculation is set to be 3.6τp, with τp = 0.26 ps being the duration of the seed pulse. The characteristic duration is τW = τFW/1.763 for the hyperbolic secant pulse, where τFW is the experimental FWHM of the 391-nm lasing pulse. By substituting τW and τD at different pressures into Eq. 28, we obtain the corresponding temporal profile as shown by the red line in Figure 3. When the gas pressure exceeds 10 mbar, the theoretical predictions are in agreement with the experimental signals for the main part of superradiance as illustrated in Figures 3D–I. Following the strongest radiation, there are the other two gains at ∼ 4.4 and 8.6 ps that correspond to the revival of the optical gain due to the molecular alignment effect [21, 66], which was not considered in the theoretical derivation. Besides, there exist a gradual decay in the measured temporal profiles at high pressures. By increasing the dephasing rate Γ2 of the transition dipole in
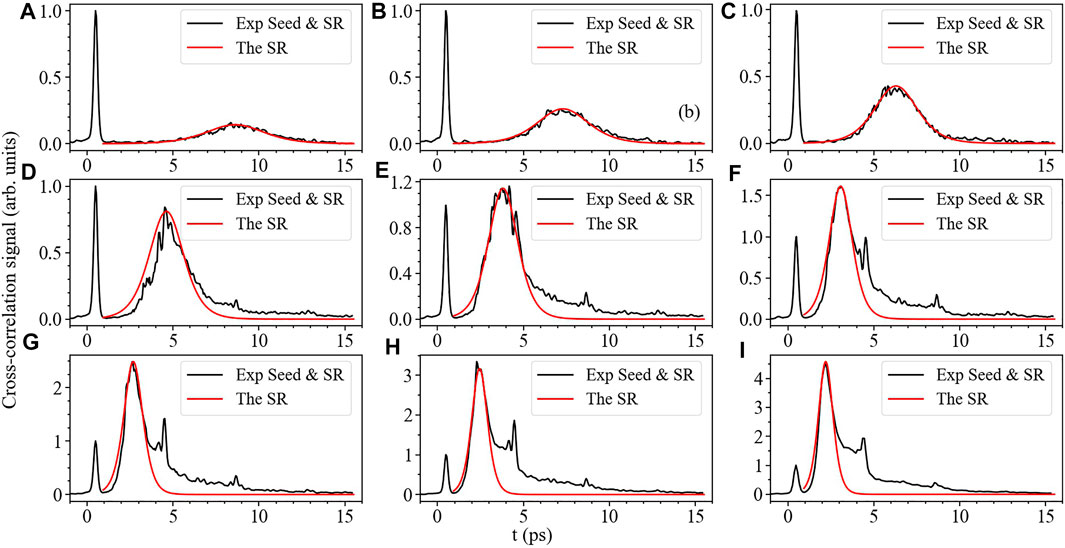
FIGURE 3. Comparisons of the experimental data and theoretical predictions for the temporal profile of the 391-nm lasing emission with different gas pressure of (A) 6 mbar, (B) 7 mbar, (C) 8 mbar, (D) 10 mbar, (E) 12 mbar, (F) 14 mbar, (G) 16 mbar, (H) 18 mbar and (I) 20 mbar, respectively.
The time-dependent quantum-wave-packet calculations show that the initial relative population difference w0 varies from 0 to 0.55 with the 800-nm laser intensity ranging from 2.2 to 4 × 1014 W/cm2 [23]. The 800-nm pump laser intensity in our experiment is estimated to be 2.6 × 1014 W/cm2 with the energy of 1.8 mJ, duration of 45 fs and filament diameter of ∼70 μm. Thus, it is reasonable to set w0 as .1. Since the pump laser pulse energy is unchanged for different gas pressure ranging from 6 to 20 mbar [29, 53], the two-level-system population density is proportional to gas pressure. In actual case, the superradiance will vanish when the pressure is lower than a certain value p0. The minimal gas pressure that causes the superradiance is p0 = 2.5 mbar in our case. The relationship between the population density and pressure can be expressed N = k (p−p0), where k is a scaling factor. Using the experimental point (p = 8 mbar, τW = 1.666 ps), we obtain N = 0.228 × (p−2.5) (mbar) × 1016 cm−3 with L = 10 mm and μ = 1.7 D [67]. Hence, the characteristic duration τW dependent on the gas pressure can be calculated. The calculated τW (red line) as a function of the nitrogen pressure show good agreement with the experimental data (black circle), as shown in Figure 4A.
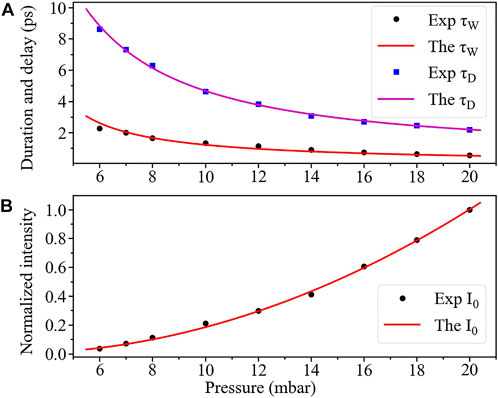
FIGURE 4. Comparisons between (A) the experimental characteristic duration τW and time delay τD, and the calculated τW and τD, and (B) the experimental results of peak intensity I0 and the theoretical calculations of I0 as a function of gas pressure.
The seed pulse in Figure 3 has a peak intensity of 107 W/cm2, and can be well fitted by a Gaussian shape with the pulse duration τp = 0.26 ps. The initial Bloch angle is accurately calculated
which is far smaller than π/2. The two-level system does not experience several cycles of Rabi oscillation under the presence of the seed pulse. Only little energy emits with the seed pulse simultaneously, which explains the almost unchanged intensity of the seed pulse after passing through the filament plasma. It can also be seen that the theoretical calculations for τD are in good agreement with the experimental results (blue square) in Figure 4A.
The peak intensities I0 of the superradiance are investigated as a function of nitrogen pressure. The values at t = τD of the experimental 391-nm emission in Figure 3 are taken as the peak intensities. The theoretical peak intensity is expressed as
From the comparisons in terms of the temporal shape, the characteristic duration, the time delay and the intensity between the theoretical and experimental results above, it can be seen that the assumption 1/Γ2 ≫ τW and 1/Γ2 ≫ τD is reasonable. If the dephasing rate Γ2 is sufficiently large, no macroscopic dipole moment ever develops and each electric dipole simply responds to the instantaneous value of the laser field. In this case a very noise output pulse shows up, of which the pulse duration and the peak intensity no longer scale as 1/N and N2 [54].
In conclusion, we theoretically investigate the evolution of energy in the population-inverted molecular nitrogen ions induced by the coherent seed pulse. By solving the Bloch angle of the two-level system of
Publicly available datasets were analyzed in this study. This data can be found here: https://www.researchgate.net/publication/316084384_Lasing_effect_in_femtosecond_filaments_in_air.
QW conceived the concept. PD provide the experimental data. QW, SW, and MA-K performed the theoretical derivation. QW, PD, YZ, ZL, and BH analyzed the data. The manuscript was prepared by QW and PD, and was discussed among all authors.
This work was supported by China Scholarship Council, the National Natural Science Foundation of China (Grants No. U1932133, No. 11905089, and No. 12004147), and the open project “Study on physical mechanisms of femtosecond laser-induced 337 nm lasing of nitrogen molecules” funded by Shanghai Institute of Optics and Fine Mechanics, Chinese Academy of Sciences.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
1. Keldysh LV. Ionization in the field of a strong electromagnetic wave. Sov Phys JETP (1965) 20:1307–14.
2. Tong XM, Zhao ZX, Lin CD. Theory of molecular tunneling ionization. Phys Rev A (2002) 66:033402. doi:10.1103/physreva.66.033402
3. Kim KY, Alexeev I, Milchberg HM. Single-shot measurement of laser-induced double step ionization of helium. Opt Express (2002) 10:1563–72. doi:10.1364/oe.10.001563
4. Fittinghoff DN, Bolton PR, Chang B, Kulander KC. Observation of nonsequential double ionization of helium with optical tunneling. Phys Rev Lett (1992) 69:2642–5. doi:10.1103/physrevlett.69.2642
5. Corkum PB. Plasma perspective on strong field multiphoton ionization. Phys Rev Lett (1993) 71:1994–7. doi:10.1103/physrevlett.71.1994
6. Lewenstein M, Balcou P, Ivanov MY, L’huillier A, Corkum PB. Theory of high-harmonic generation by low-frequency laser fields. Phys Rev A (1994) 49:2117–32. doi:10.1103/physreva.49.2117
7. Chin SL, Théberge F, Liu W. Filamentation nonlinear optics. Appl Phys B (2007) 86:477–83. doi:10.1007/s00340-006-2455-z
8. Couairon A, Mysyrowicz A. Femtosecond filamentation in transparent media. Phys Rep (2007) 441:47–189. doi:10.1016/j.physrep.2006.12.005
9. Nandan Gupta D, Pal Singh K, Suk H. Optical field-ionization of a neutral gas with inhomogeneous density for electron acceleration by a high-intensity laser. Phys Plasma (2012) 19:023103. doi:10.1063/1.3678202
10. McFarland BK, Farrell JP, Bucksbaum PH, Guhr M. High harmonic generation from multiple orbitals in N2. Science (2008) 322:1232–5. doi:10.1126/science.1162780
11. Smirnova O, Mairesse Y, Patchkovskii S, Dudovich N, Villeneuve D, Corkum P, et al. High harmonic interferometry of multi-electron dynamics in molecules. Nature (2009) 460:972–7. doi:10.1038/nature08253
12. Becker A, Bandrauk AD, Chin SL. S-matrix analysis of non-resonant multiphoton ionisation of inner-valence electrons of the nitrogen molecule. Chem Phys Lett (2001) 343:345–50. doi:10.1016/s0009-2614(01)00705-9
13. Mitryukovskiy S, Liu Y, Ding P, Houard A, Couairon A, Mysyrowicz A. Plasma luminescence from femtosecond filaments in air: Evidence for impact excitation with circularly polarized light pulses. Phys Rev Lett (2015) 114:063003. doi:10.1103/physrevlett.114.063003
14. Wang P, Xu S, Li D, Yang H, Jiang H, Gong Q, et al. Spectroscopic study of laser-induced tunneling ionization of nitrogen molecules. Phys Rev A (2014) 90:033407. doi:10.1103/physreva.90.033407
15. Yao J, Zeng B, Xu H, Li G, Chu W, Ni J, et al. High-brightness switchable multiwavelength remote laser in air. Phys Rev A (2011) 4:051802. (R). doi:10.1103/physreva.84.051802
16. Zhang H, Jing C, Yao J, Li G, Zeng B, Chu W, et al. Rotational coherence encoded in an “air-laser” spectrum of nitrogen molecular ions in an intense laser field. Phys Rev X (2013) 3:041009. doi:10.1103/physrevx.3.041009
17. Ni J, Chu W, Jing C, Zhang H, Zeng B, Yao J, et al. Identification of the physical mechanism of generation of coherent N2+ emissions in air by femtosecond laser excitation. Opt Express (2013) 21:8746–52. doi:10.1364/oe.21.008746
18. Zeng B, Chu W, Li G, Yao J, Zhang H, Ni J, et al. Real-time observation of dynamics in rotational molecular wave packets by use of air-laser spectroscopy. Phys Rev A (2014) 89:042508. doi:10.1103/physreva.89.042508
19. Xie H, Zeng B, Li G, Chu W, Zhang H, Jing C, et al. Coupling of N2+ rotational states in an air laser from tunnel-ionized nitrogen molecules. Phys Rev A (2014) 90:042504. doi:10.1103/physreva.90.042504
20. Liu Y, Brelet Y, Point G, Houard A, Mysyrowicz A. Self-seeded lasing in ionized air pumped by 800 nm femtosecond laser pulses. Opt Express (2013) 21:22791–8. doi:10.1364/oe.21.022791
21. Yao J, Li G, Jing C, Zeng B, Chu W, Ni J, et al. Remote creation of coherent emissions in air with two-color ultrafast laser pulses. New J Phys (2013) 15:023046. doi:10.1088/1367-2630/15/2/023046
22. Li G, Jing C, Zeng B, Xie H, Yao J, Chu W, et al. Signature of superradiance from a nitrogen-gas plasma channel produced by strong-field ionization. Phys Rev A (2014) 89:033833. doi:10.1103/physreva.89.033833
23. Xu H, Lötstedt E, Iwasaki A, Yamanouchi K. Sub-10-fs population inversion in N2+ in air lasing through multiple state coupling. Nat Commun (2015) 6:8347–6. doi:10.1038/ncomms9347
24. Liu Y, Ding P, Lambert G, Houard A, Tikhonchuk V, Mysyrowicz A. Recollision-induced superradiance of ionized nitrogen molecules. Phys Rev Lett (2015) 115:133203. doi:10.1103/physrevlett.115.133203
25. Yao J, Jiang S, Chu W, Zeng B, Wu C, Lu R, et al. Population redistribution among multiple electronic states of molecular nitrogen ions in strong laser fields. Phys Rev Lett (2016) 116:143007. doi:10.1103/physrevlett.116.143007
26. Azarm A, Corkum P, Polynkin P. Optical gain in rotationally excited nitrogen molecular ions. Phys Rev A (2017) 96:051401. doi:10.1103/physreva.96.051401
27. Britton M, Laferriere P, Ko DH, Li Z, Kong F, Brown G, et al. Testing the role of recollision in N2+ air lasing. Phys Rev Lett (2018) 120:133208. doi:10.1103/physrevlett.120.133208
28. Zhang A, Liang Q, Lei M, Yuan L, Liu Y, Fan Z, et al. Coherent modulation of superradiance from nitrogen ions pumped with femtosecond pulses. Opt Express (2019) 27:12638–46. doi:10.1364/oe.27.012638
29. Mysyrowicz A, Danylo R, Houard A, Tikhonchuk V, Zhang X, Fan Z, et al. Lasing without population inversion in N2+. APL Photon (2019) 4:110807. doi:10.1063/1.5116898
30. Ando T, Lötstedt E, Iwasaki A, Li H, Fu Y, Wang S, et al. Rotational, vibrational, and electronic modulations in N2+ lasing at 391 nm: Evidence of coherent (B2 su+)-(X2 Σg+)− (A2Πu) coupling. Phys Rev Lett (2019) 123:203201. doi:10.1103/physrevlett.123.203201
31. Xie H, Lei H, Li G, Zhang Q, Wang X, Zhao J, et al. Role of rotational coherence in femtosecond-pulse-driven nitrogen ion lasing. Phys Rev Res (2020) 2:023329. doi:10.1103/physrevresearch.2.023329
32. Tikhonchuk VT, Liu Y, Danylo R, Houard A, Mysyrowicz A. Theory of femtosecond strong field ion excitation and subsequent lasing in N2+. New J Phys (2021) 23:023035. doi:10.1088/1367-2630/abd8bf
33. Chen J, Yao J, Zhang Z, Liu Z, Xu B, Wan Y, et al. Electronic quantum coherence encoded in temporal structures of N2+ lasing. Phys Rev A (2021) 103:033105. doi:10.1103/physreva.103.033105
34. Zhang X, Lu Q, Zhang Z, Fan Z, Zhou D, Liang Q, et al. Coherent control of the multiple wavelength lasing of N2+: Coherence transfer and beyond. Optica (2021) 8:668–73. doi:10.1364/optica.417804
35. Yuan L, Liu Y, Yao J, Cheng Y. Recent advances in air lasing: A perspective from quantum coherence. Adv Quan Technol (2019) 2:1900080. doi:10.1002/qute.201900080
36. Yao J, Wang L, Chen J, Wan Y, Zhang Z, Zhang F, et al. Photon retention in coherently excited nitrogen ions. Sci Bull (2021) 66:1511–7. doi:10.1016/j.scib.2021.04.001
37. Zhang Z, Zhang F, Xu B, Xie H, Fu B, Lu X, et al. High-sensitivity gas detection with air-lasing-assisted coherent Raman spectroscopy. Ultrafast Sci (2022) 2022:1–8. doi:10.34133/2022/9761458
38. Zhang F, Xie H, Yuan L, Zhang Z, Fu B, Yu S, et al. Background-free single-beam coherent Raman spectroscopy assisted by air lasing. Opt Lett (2022) 47:481–4. doi:10.1364/ol.441602
39. Lei H, Yao J, Zhao J, Xie H, Zhang F, Zhang H, et al. Ultraviolet supercontinuum generation driven by ionic coherence in a strong laser field. Nat Commun (2022) 13:4080–9. doi:10.1038/s41467-022-31824-0
40. Zhang Q, Xie H, Li G, Wang X, Lei H, Zhao J, et al. Sub-cycle coherent control of ionic dynamics via transient ionization injection. Commun Phys (2020) 3:50–6. doi:10.1038/s42005-020-0321-7
41. Xie H, Lei H, Li G, Yao J, Zhang Q, Wang X, et al. Controlling the collective radiative decay of molecular ions in strong laser fields. Photon Res (2021) 9:2046–51. doi:10.1364/prj.434378
42. Bacri J, Medani A. Electron diatomic molecule weighted total cross section calculation: III. Main inelastic processes for N2 and N2+. Physica B+ C (1982) 112:101–18. doi:10.1016/0378-4363(82)90136-x
43. Bahati EM, Jureta JJ, Belic DS, Cherkani-Hassani H, Abdellahi MO, Defrance P. Electron impact dissociation and ionization of N2+. J Phys B: Mol Opt Phys (2001) 34:2963–73. doi:10.1088/0953-4075/34/15/303
44. Johnson AW, Fowler RG. Measured lifetimes of rotational and vibrational levels of electronic states of N2. J Chem Phys (1970) 53:65–72. doi:10.1063/1.1673834
45. Chauveau S, Perrin MY, Riviere P, Soufiani A. Contributions of diatomic molecular electronic systems to heated air radiation. J Quant Spectrosc Radiat Transfer (2002) 72:503–30. doi:10.1016/s0022-4073(01)00141-8
46. Mehr FJ, Biondi MA. Electron temperature dependence of recombination of O2+ and N2+ ions with electrons. Phys Rev (1969) 181:264–71. doi:10.1103/physrev.181.264
47. Johnsen R. Microwave afterglow measurements of the dissociative recombination of molecular ions with electrons. Int J Mass Spectrom Ion Process. (1987) 81:67–84. doi:10.1016/0168-1176(87)80006-x
48. Brian J, Mitchell A. The dissociative recombination of molecular ions. Phys Rep (1990) 186:215–48. doi:10.1016/0370-1573(90)90159-y
49. Jain A, Gupta DN, Suk H. Electron–ion recombination effect on electron acceleration by an intense laser pulse. IEEE Trans Plasma Sci (2019) 47:4891–7. doi:10.1109/tps.2019.2947283
51. Cohen-Tannoudji C, Dupont-Roc J, Grynberg G. Atom-photon interactions: Basic processes and applications. New York: Wiley (1998).
52. Wang Q, Chen R, Zhang Y, Wang X, Sun C, Ding P, et al. Populations of B2Σu+ and X2Σg+ electronic states of molecular nitrogen ions in air determined by fluorescence measurement. Phys Rev A (2021) 103:033117. doi:10.1103/physreva.103.033117
54. Malcuit MS, Maki JJ, Simkin DJ, Boyd RW. Transition from superfluorescence to amplified spontaneous emission. Phys Rev Lett (1987) 59:1189–92. doi:10.1103/physrevlett.59.1189
55. Schuurmans MF, Polder D. Superfluorescence and amplified spontaneous emission: A unified theory. Phys Lett A (1979) 72:306–8. doi:10.1016/0375-9601(79)90477-8
56. Schuurmans MF. Superfluorescence and amplified spontaneous emission in an inhomogeneously broadened medium. Opt Commun (1980) 34:185–9. doi:10.1016/0030-4018(80)90011-5
57. Maki JJ, Malcuit MS, Raymer MG, Boyd RW, Drummond PD. Influence of collisional dephasing processes on superfluorescence. Phys Rev A (1989) 40:5135–42. doi:10.1103/physreva.40.5135
58. Itikawa Y. Cross sections for electron collisions with nitrogen molecules. J Phys Chem Ref Data (2006) 35:31–53. doi:10.1063/1.1937426
60. Dicke RH. Coherence in spontaneous radiation processes. Phys Rev (1954) 93:99–110. doi:10.1103/physrev.93.99
61. Bonifacio R, Schwendimann P, Haake F. Quantum statistical theory of superradiance. I. Phys Rev A (1971) 4:302–13. doi:10.1103/physreva.4.302
62. MacGillivray JC, Feld MS. Theory of superradiance in an extended, optically thick medium. Phys Rev A (1976) 14:1169–89. doi:10.1103/physreva.14.1169
63. Polder D, Schuurmans MF, Vrehen QH. Superfluorescence: Quantum-mechanical derivation of Maxwell-Bloch description with fluctuating field source. Phys Rev A (1979) 19:1192–203. doi:10.1103/physreva.19.1192
64. Bonifacio R, Lugiato LA. Cooperative radiation processes in two-level systems: Superfluorescence. Phys Rev A (1975) 11:1507–21. doi:10.1103/physreva.11.1507
65. Ding P. Lasing effect in femtosecond filaments in air. France: Université Paris-Saclay (2016). Ph.D. thesis.
66. Calegari F, Vozzi C, Gasilov S, Benedetti E, Sansone G, Nisoli M, et al. Rotational Raman effects in the wake of optical filamentation. Phys Rev Lett (2008) 100:123006. doi:10.1103/physrevlett.100.123006
Keywords: optical bloch equations, superradiance (SR), strong-laser-field ionization, 391-nm lasing of N2+, ratarded optical amplification
Citation: Wang Q, Ding P, Wilkins SG, Athanasakis-Kaklamanakis M, Zhang Y, Liu Z and Hu B (2023) Theoretical treatment on externally-seeded superradiance from
Received: 05 November 2022; Accepted: 23 December 2022;
Published: 06 January 2023.
Edited by:
Huipeng Kang, Innovation Academy for Precision Measurement Science and Technology (CAS), ChinaReviewed by:
Xie Hong Qiang, East China University of Technology, ChinaCopyright © 2023 Wang, Ding, Wilkins, Athanasakis-Kaklamanakis, Zhang, Liu and Hu. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Pengji Ding, ZGluZ3BqQGx6dS5lZHUuY24=; Zuoye Liu, enlsQGx6dS5lZHUuY24=
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.