Dual-Band Bandpass Filter With Controllable Stopband Bandwidth

A novel dual-band bandpass filter (BPF) is proposed with independently controllable transmission zeros (TZs) which can realize widely tunable stopband bandwidth (BW). The planar microstrip filter consists of a three-degree L-C ladder lowpass filter loaded with two unsymmetrical shorted stubs which are used to produce different TZs. By tuning the parameters of the two unsymmetrical shorted stubs, the TZs can be independently controlled. Therefore, the BPF has independent controllable center frequencies (CFs), passband bandwidths, and stopband bandwidths between adjacent passbands. All the L-C values in the equivalent circuit of the proposed filter are optimized to fulfill the design specifications. For demonstration, a dual-band BPF is designed. The measured results show good agreement with the simulated ones.

Introduction

Modern wireless communication systems need high performance filters which have controllable CFs, passband bandwidths, stopband bandwidths, and sharp rejection. Different designing structures and methods have been greatly studied [1–17]. Generally, the dual-band bandpass filter (BPF) adjusts the CFs by changing transmission poles (TPs) [1, 2]. To suppress the harmonic responses, the multiple resonant modes excited by a stepped impedance stub-loaded resonator are used to design dual-band BPFs with a wide upper stopband [3]. However, there is only one transmission zero (TZ) in the stopband. Therefore, the stopband bandwidth is narrow. By introducing more TZs in the stopband, the performances of selectivity and stopband bandwidth can be enhanced. Signal-interference principles [4–6] are applied to design single- or dual-band BPFs with broad stopband bandwidths by creating multiple TZs. To design high performance filters with multiple passbands, the method of multiple mono-frequency resonators coupling to a non-resonating node is proposed in [7]. To have more TZs, couplings between the non-resonating nodes are introduced in [8], or couplings and more open/shorted-subs [9, 10] are applied in the configurations. However, it is difficult to realize the coupling for application when the number of passband increases. Moreover, TZs introduced by the coupling matrix need to change all the coupling coefficients, so it is also difficult to independently adjust the position of TZs [11].

This study presents a novel BPF loaded with two unsymmetrical shorted stubs to introduce two different TZs in the stopband to realize the controllable stopband bandwidth. Following our early work [12], the theoretical analysis of the TZs and TPs is shown in this study, which can demonstrate that the design method has advantages of simplicity and effectiveness. A three-degree lowpass filter prototype is chosen for simplicity; the in-band performance will be improved when choosing more degrees of the lowpass filter prototype. A dual-band BPF has been designed and fabricated to verify the proposed concept.

Analysis

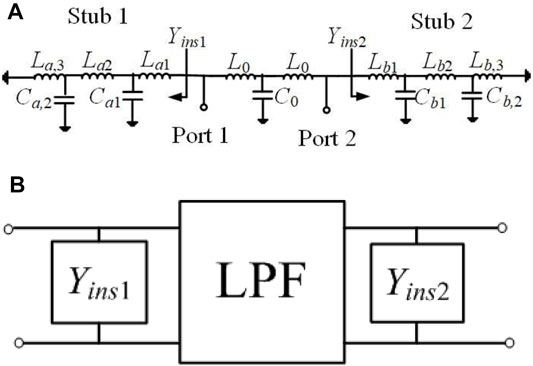
The equivalent circuit and network of the proposed prototype are shown in Figure 1. The network can be divided into three subnetworks, as shown in Figure 1B, which are the lowpass filter (LPF) section in the middle and two unsymmetrical shunt admittance sections at the sides. The LPF section is also a symmetric network and has chosen a three order L-C ladder prototype for simplicity, as shown in Figure 1A.

FIGURE 1

FIGURE 1. Proposed BPF equivalent circuit and network (A) equivalent circuit (B) network model.

From our early analysis in [12], the shorted stub with two equivalent capacitors will introduce two TZs. As shown in Figure 1, the configuration has two unsymmetrical shorted stubs; the shunt admittances of Stub1 and Stub 2 have different LC values, which will produce four different TZs.

The ABCD matrix of the transmission network in Figure 1B is expressed as follows, where M_Y1 and M_Y2 are the ABCD matrices of the Stub 1 and Stub 2, respectively. M_LPF is the ABCD matrix of the middle LPF section. According to the relationship of scattering parameters with the ABCD matrix, the TZs and the reflection zeros (TPs) are the roots of polynomials P(s) and F(s), respectively, where the impedance of the source/load is normalized to be 1. When the root of F(s) is an imaginary number, the reflection zero related to the root represents a TP.

$\begin{array}{l}{M}_{Y1}=\left[\begin{array}{cc}1& 0\\ Y_{ins1}& 1\end{array}\right]=\left[\begin{array}{cc}1& 0\\ \frac{D{}_1\left(s\right)}{N_1\left(s\right)}& 1\end{array}\right]\\ =\frac{1}{N_1\left(s\right)}\left[\begin{array}{cc}{N}_1\left(s\right)& 0\\ D_1\left(s\right)& N_1\left(s\right)\end{array}\right],\end{array}(\mathrm{1a})$

$N_1\left(s\right)=C_{a1}{C}_{a2}{L}_{a1}{L}_{a2}{L}_{a3}{s}^5+\left[C_{a1}{L}_{a1}\left(L_{a2}+L_{a3}\right)+C_{a2}{L}_{a3}\left(L_{a1}+L_{a2}\right)\right]s^3+\left(L_{a1}+L_{a2}+L_{a3}\right)s,(\mathrm{1b})$

$D_1\left(s\right)=C_{a1}{C}_{a2}{L}_{a2}{L}_{a3}{s}^4+\left(C_{a1}{L}_{a2}+C_{a1}{L}_{a3}+C_{a2}{L}_{a3}\right)s^2+1,(\mathrm{1c})$

$\begin{array}{l}{M}_{Y2}=\left[\begin{array}{cc}1& 0\\ Y_{ins2}& 1\end{array}\right]=\left[\begin{array}{cc}1& 0\\ \frac{D{}_2\left(s\right)}{N_2\left(s\right)}& 1\end{array}\right]\\ =\frac{1}{N_2\left(s\right)}\left[\begin{array}{cc}{N}_2\left(s\right)& 0\\ D_2\left(s\right)& N_2\left(s\right)\end{array}\right],\end{array}(\mathrm{2a})$

$N_2\left(s\right)=C_{b1}{C}_{b2}{L}_{b1}{L}_{b2}{L}_{b3}{s}^5+\left[C_{b1}{L}_{b1}\left(L_{b2}+L_{b3}\right)+C_{b2}{L}_{b3}\left(L_{b1}+L_{b2}\right)\right]s^3+\left(L_{b1}+L_{b2}+L_{b3}\right)s,(\mathrm{2b})$

$D_2\left(s\right)=C_{b1}{C}_{b2}{L}_{b2}{L}_{b3}{s}^4+\left(C_{b1}{L}_{b2}+C_{b1}{L}_{b3}+C_{b2}{L}_{b3}\right)s^2+1,(\mathrm{2c})$

$\begin{array}{l}{M}_{LPF}=\left[\begin{array}{cc}{L}_0{C}_0{s}^2+1& L_0^2{C}_0{s}^3+2L_{0}s\\ C_{0}s& L_0{C}_0{s}^2+1\end{array}\right]\\ =\left[\begin{array}{cc}a\left(s\right)& b\left(s\right)\\ c\left(s\right)& d\left(s\right)\end{array}\right],\end{array}(3)$

$\begin{array}{l}\left[\begin{array}{cc}A& B\\ C& D\end{array}\right]=M_{Y1}\times M_{LPF}\times M_{Y2}\\ =\frac{1}{N_1\left(s\right)N_2\left(s\right)}\left[\begin{array}{cc}A\text{'}\left(s\right)& B\text{'}\left(s\right)\\ C\text{'}\left(s\right)& D\text{'}\left(s\right)\end{array}\right]\\ =\frac{1}{P\left(s\right)}\left[\begin{array}{cc}A\text{'}\left(s\right)& B\text{'}\left(s\right)\\ C\text{'}\left(s\right)& D\text{'}\left(s\right)\end{array}\right],\end{array}(\mathrm{4a})$

$P\left(s\right)=N_1\left(s\right)N_2\left(s\right),(\mathrm{4b})$

$\begin{array}{l}F\left(s\right)=A\text{'}\left(s\right)+B\text{'}\left(s\right)-C\text{'}\left(s\right)-D\text{'}\left(s\right)\\ =N_1\left(s\right)N_2\left(s\right)\left[b\left(s\right)-c\left(s\right)\right]\\ -\left[N_1\left(s\right)D_2\left(s\right)+D_1\left(s\right)N_2\left(s\right)\right]a\left(s\right)-D_1\left(s\right)D_2\left(s\right)b\left(s\right).\end{array}(5)$

From (Eq. 4b), polynomial P(s) has 4n+2 roots (n = 2), of which 2 roots are at the origin and others are conjugate and symmetrical on the imaginary axis. Therefore, each shorted stub produces n TZs and the two unsymmetrical shorted stubs produce 2n different TZs on the imaginary axis. From (Eq. 5), the degree of polynomial F(s) is 4n+5. N₁(s), N₂(s), b(s), and c(s) have odd terms only, while D₁(s), D₂(s), and a(s) have constant terms. Therefore, polynomial F(s) has one root at the origin; two real roots and the remaining 4n+2 roots are conjugate and symmetrical on the imaginary axis or in four quadrants. The optimization algorithm is used to obtain the required lumped element values for independently controlling the TZs and the return losses, such as genetic algorithm and so on.

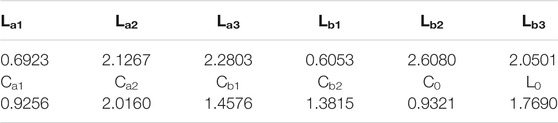
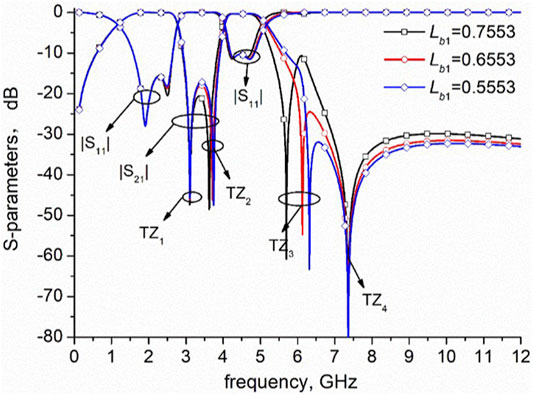
Since one of the two shorted stubs can tune two TZs, a dual-band BPF is presented for demonstration. The optimized L-C values are shown in Table 1. The S-parameters of the circuit with different parameters of the shorted stub are shown in Figures 2, 3. The parameter L_a1 of the shorted stub 1 can tune TZ₁ and TZ₄, which means the first passband CF and stopband bandwidth can be tuned independently, while the second passband width, TZ₂ and TZ₃ keep unchanged. The larger the value of L_a1, the smaller will be the frequencies of TZ₁ and TZ₄. Meanwhile, when the parameter L_b1 of the shorted stub 2 changes, the frequencies of TZ₂ and TZ₃ vary simultaneously. As shown in Figure 3, the value of L_b1 gets large and the frequencies of TZ₂ and TZ₃ decrease, which move at the same direction as that of TZ₁ and TZ₄. Therefore, the bandwidth of the second passband can be tuned independently. Since the position of the last TP is near that of TZ₃, the upper stopband performance is effected by the move of TZ₃.

TABLE 1

TABLE 1. Optimized L-C values for a dual-band BPF (L unit in nH, C unit in pF).

FIGURE 2

FIGURE 2. Tunable TZ₁ and TZ₄ versus different L_a1.

FIGURE 3

FIGURE 3. Tunable TZ₂ and TZ₃ versus different L_b1.

Results

A dual-band BPF is designed and fabricated to demonstrate the design method. The CFs of dual passbands are 1 and 2.8 GHz, respectively. The stopbands are 1.75–2.2 GHz (<25 dB) and >3.55 GHz (<25 dB), respectively. Therefore, the TPs are preset as 1.8, 2.15, 3.6, and 4.4 GHz. The optimized L-C values are L_a1 = 0.5922, L_a2 = 2.5267, L_a3 = 3.3803, L₀ = 3.0691, L_b1 = 1.1153, L_b2 = 2.4080, L_b3 = 3.9501 (unit in nH), C_a1 = 2.8256, C_a2 = 3.2161, C₀ = 1.4321, C_b1 = 2.8576, and C_b2 = 3.6815 (unit in pF). The S-parameters calculated by the optimized L-C values are shown in Figure 4A. The roots of P(s) are 0, 0, ±1.83j, ±2.16j, ±3.59j, and ±4.41j, which are calculated in unit GHz for facility. The positive imaginary roots of P(s) represent the TZs. The L-C values of shunt admittance Y_ins1 control TZ₂ and TZ₄, while the L-C values of shunt admittance Y_ins2 control TZ₁ and TZ₃. The roots of F(s) are 0, −0.05 ± 1.01j, 0.1 ± 1.37j, −0.07 ± 2.59j, 0.04 ± 2.9j, 0.03 ± 3.58j, −8.94, and 15.15. Since the roots 0.03 ± 3.58j of F(s) are close to the roots ±3.59j of P(s), the second passband has two TPs not three TPs [7].

FIGURE 4

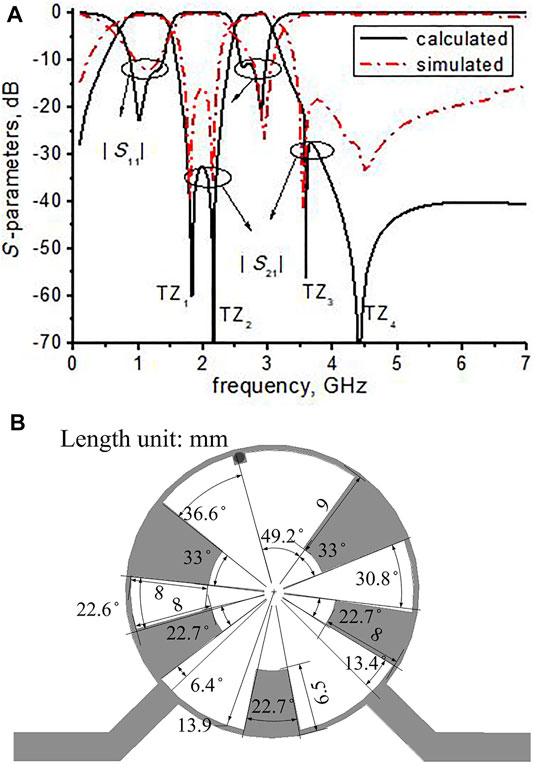
FIGURE 4. Calculated and measured S-parameters and optimized structure (A) calculated and simulated S-parameters (B) optimized BPF structure.

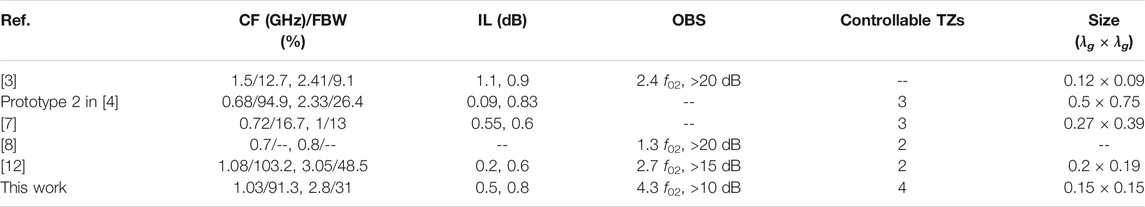
The BPF is simulated and fabricated on a substrate with ε_r = 2.65, tanδ = 0.003, and h = 1 mm. Here, we choose the 117.8 Ω high-impedance line, which has the line-width of 0.5 mm. As shown in Figure 4B, the line width of the open stub which acts as the capacitor is tapered. The characteristic impedance is calculated by the waist width of the taper. Due to the discontinuity of the microstrip line and the minor coupling between the open stubs, the final dimension parameters are optimized by the full-wave electromagnetic simulation software HFSS. The optimized values of parameters are given in Figure 4B. The circuit size is about 0.15 λ_g × 0.15 λ_g, where λ_g is the guided wavelength at f₀₁. The simulated S-parameters agree well with the calculated ones, as shown in Figure 4A. Figure 5 shows the simulated and measured S-parameters with the photograph inset. The measured results are in good agreement with the simulated ones. The discrepancies between the simulated results and the measured results may be due to the ignorance of transmission line discontinuity and fabrication tolerances. The measured CFs, fractional bandwidths (FBWs), insertion losses (ILs) at the CF, out-band suppressions (OBSs), the number of controllable TZs, and the size are listed in Table 2. As shown in Table 2, the proposed dual-band BPF with simple circuit topology has advantages such as relative low IL, wide FBW, wide upper stopband, and more controllable TZs.

FIGURE 5

FIGURE 5. Simulated and measured S-parameters with photograph inset.

TABLE 2

TABLE 2. Comparison of the proposed filter with previous designs.

Conclusion

A dual-band BPF with a controllable stopband bandwidth between the passbands is designed by the method of independently controlling TZs. By tuning the parameters of the two unsymmetrical shorted stubs, the introduced two TZs between adjacent passbands can be independently controlled. After optimizing the equivalent circuit lumped element values, the CFs, passband bandwidths, and stopband bandwidths can be tuned in a certain range. With a simple filter structure and good performance, the proposed method is attractive for application in high-selectivity filter designs.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.

Author Contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Conflict of Interest

Authors NW and HW were employed by the State Grid Shaanxi Electric Power Research Institute. KG and XR were employed by the company State Grid Xi'an Electric Power Corporation. XC was employed by the State Grid Shaanxi Maintenance Company. QX was employed by the State Grid Weinan Electric Power Corporation.

The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. He J, Chen C-m. A Dual-Band BPF with Controllable Center Frequencies and Bandwidths Using a Single SIW Cavity. In: Prceeding of the 2021 IEEE 4th International Conference on Electronics Technology (ICET); 7-10 May 2021; Chengdu, China. IEEE (2021). p. 489–92. doi:10.1109/ICET51757.2021.9451069

CrossRef Full Text | Google Scholar

2. Lin W, Lee T-H, Wu K Dual-Band Bandpass SIW Resonator Filter with Flexible Frequency Ratio. In: Prceeding of the 2019 49th European Microwave Conference (EuMC); 1-3 Oct. 2019; Paris, France. IEEE (2019). p. 364–7. doi:10.23919/EuMC.2019.8910700

CrossRef Full Text | Google Scholar

3. Lin L, Sun SJ, Wu B, Liang CH Dual‐band Bandpass Filter with Wide Upper Stopband Using Quad‐mode Stepped Impedance Stub‐loaded Resonator. Electron Lett (2014) 50(16):1145–6. doi:10.1049/el.2014.2004

CrossRef Full Text | Google Scholar

4. Sanchez-Soriano MA, Gomez-Garcia R Sharp-Rejection Wide-Band Dual-Band Bandpass Planar Filters with Broadly-Separated Passbands. IEEE Microw Wireless Compon Lett (2015) 25(2):97–9. doi:10.1109/LMWC.2014.2382669

CrossRef Full Text | Google Scholar

5. Phani Kumar KV, Karthikeyan SS Compact, High Selectivity and Wideband Bandpass Filter with Multiple Transmission Zeros. AEU - Int J Electronics Commun (2018) 94:79–83. doi:10.1016/j.aeue.2018.06.047

CrossRef Full Text | Google Scholar

6. Yogesh SS, Phani K, Karthikeyan SS Compact Dual-Wideband Bandpass Filter for Wireless Applications. AEU - Int J Electronics Commun (2018) 95:S1434841118304618. doi:10.1016/j.aeue.2018.08.007

CrossRef Full Text | Google Scholar

7. Gomez-Garcia R, Guyette AC Reconfigurable Multi-Band Microwave Filters. IEEE Trans Microwave Theor Techn. (2015) 63(4):1294–307. doi:10.1109/TMTT.2015.2405066

CrossRef Full Text | Google Scholar

8. Gómez-García R, Guyette AC, Psychogiou D, Naglich EJ, Peroulis D Quasi-Elliptic Multi-Band Filters with Center-Frequency and Bandwidth Tunability. IEEE Microwave Wireless Components Lett (2016) 26(3):192–4. doi:10.1109/LMWC.2016.2526026

CrossRef Full Text | Google Scholar

9. Xu K-D, Li D, Liu Y High-Selectivity Wideband Bandpass Filter Using Simple Coupled Lines with Multiple Transmission Poles and Zeros. IEEE Microw Wireless Compon Lett (2019) 29(2):107–9. doi:10.1109/LMWC.2019.2891203

CrossRef Full Text | Google Scholar

10. Xu KD, Zhang F, Liu Y, Nie W High Selectivity Seventh‐order Wideband Bandpass Filter Using Coupled Lines and Open/shorted Stubs. Electron Lett (2018) 54(4):223–5. doi:10.1049/el.2017.4233

CrossRef Full Text | Google Scholar

11. Tang S-C, Chu P-C, Kuo J-T, Wu L-K Dual-band Bandpass Filter Based on Frequency Transformations with Enhanced Inter-band Isolation. In: Prceeding of the 2020 IEEE Asia-Pacific Microwave Conference (APMC); 8-11 Dec. 2020; Hong Kong, Hong Kong. IEEE (2020). p. 758–60. doi:10.1109/APMC47863.2020.9331380

CrossRef Full Text | Google Scholar

12. Yang Q, Jiao YC, Zhang Z Dual‐wideband BPF with Wide Upper Stopband Using Shorted Stepped‐impedance Stub‐loaded Lowpass Filter. Electron Lett (2016) 52(19):1615–6. doi:10.1049/el.2016.2475

CrossRef Full Text | Google Scholar

13. Xu J, Wu W, Miao C Compact and Sharp Skirts Microstrip Dual-Mode Dual-Band Bandpass Filter Using a Single Quadruple-Mode Resonator (QMR). IEEE Trans Microwave Theor Techn. (2013) 61(3):1104–13. doi:10.1109/tmtt.2013.2238949

CrossRef Full Text | Google Scholar

14. Sun S-J, Su T, Deng K, Wu B, Liang C-H Compact Microstrip Dual-Band Bandpass Filter Using a Novel Stub-Loaded Quad-Mode Resonator. IEEE Microw Wireless Compon Lett (2013) 23(9):465–7. doi:10.1109/LMWC.2013.2274038

CrossRef Full Text | Google Scholar

15. Peng Y, Zhang L, Fu J, Wang Y, Leng Y Compact Dual-Band Bandpass Filter Using Coupled Lines Multimode Resonator. IEEE Microw Wireless Compon Lett (2015) 25(4):235–7. doi:10.1109/LMWC.2015.2400936

CrossRef Full Text | Google Scholar

16. Jin X, Wen W, Chen M Compact Microstrip Dual-/Tri-/Quad-Band Bandpass Filter Using Open Stubs Loaded Shorted Stepped-Impedance Resonator. IEEE Trans Microwave Theor Tech (2013) 61(9):3187–99. doi:10.1109/TMTT.2013.2273759

CrossRef Full Text | Google Scholar

17. Kim CH, Chang K Independently Controllable Dual-Band Bandpass Filters Using Asymmetric Stepped-Impedance Resonators. IEEE Trans Microwave Theor Techn. (2011) 59(12):3037–47. doi:10.1109/TMTT.2011.2168973

CrossRef Full Text | Google Scholar