A Fractional Time-Derivative Model for Severe Wear: Hypothesis and Implications

Based on the example of wear of polymers, which exhibit a power-law time variation of the wear loss under constant loading conditions, a fractional time-derivative wear equation has been introduced. The wear contact problem with a fixed contact zone is solved using the known method of separation of spatial and time variables. It is shown that during the wear process, the contact pressure approaches a uniform distribution over the contact area, which is termed as a quasi-steady-state solution, since the mean volumetric wear rate does not tend to become constant. It is of interest that the contact pressure variation displays a decaying oscillatory nature in the case of severe wear, when the mean volumetric wear rate increases with time.

1 Introduction

Wear is a tribological phenomenon that accompanies contact interaction of solids with interfacial sliding between their surfaces and manifests itself primarily in gradual loss of material due to the subsurface damage accumulation and surface degradation (Zmitrowicz, 2006). In wear material testing, the wear loss is usually measured in terms of the volume of lost material, V, which yields the wear depth, w, by relating to the area of contact, A, as

$w=\frac{V}{A}.(1)$

Eq. 1 tentatively assumes that the wear loss is the same at each point of the contact zone, and this condition is characteristic of the steady-state wear process (Dundurs and Comninou, 1980; Páczelt and Mróz, 2007).

Usually, sliding wear tests are performed under constant normal loading conditions, which are characterized by either contact load, P, or mean contact pressure,

$\bar{p}=\frac{P}{A}.(2)$

In the cases of mild or moderate wear, after some initial running-in (Wright and Kukureka, 2001; Khonsari et al., 2021) or wearing-in (Blau, 2005) period of time, the condition of steady state $p(x,t)\equiv \bar{p}$ is realized at the contact interface and maintained for a long time period, during which the wear material characteristics have been measured.

In the steady-state wear process, the linear wear rate, defined as the time derivative $\dot{w}=\mathrm{d}w/\mathrm{d}t$, is constant in time, and moreover, the contact pressure p achieves a constant value over the entire contact zone. So, in the steady-state wear regime, the volumetric wear loss V will be a linear function of the time variable t, and thus, the linear wear rate can be evaluated as

$\dot{w}=\frac{V\left(t_2\right)-V\left(t_1\right)}{A\left(t_2-t_1\right)},(3)$

where t₁ and t₂ are any two different moments of time taken during the steady-state period.

In many cases, the wear equation can be written in the following form (Kragelsky, 1965):

$V=k_{\mathrm{w}}Pvt.(4)$

Here, v is the sliding velocity, and k_w is the wear coefficient. In view of Eqs 1, 2, Eq. 4 can be represented as

$\dot{w}=k_{\mathrm{w}}\bar{p}v,(5)$

which is known as Archard’s equation (Meng and Ludema, 1995), though similar wear equations were introduced earlier by Reye (1860), Khrushchov and Babichev (1941), and Holm (1946). The wear coefficient k_w is determined during the steady-state conditions by using Eqs 3, 5.

A generalization of the wear Eq. 4, which was suggested by Rhee (1970) for polymer-bonded friction materials, takes the form

$V=K_{\mathrm{w}}{P}^{\gamma }{v}^{\beta }{t}^{\alpha },(6)$

where K_w is the wear factor, and α, β, γ are parameters. However, while Eq. 6 has been successfully used to predict the wear resistance of polymer composite materials designed for extreme environmental conditions (Gardos, 1982; Sedakova and Kozyrev, 2021), there is a problem with applying the wear Eq. 6 for solving the wear contact problems with the spatial-temporal variation of the contact pressures (Grzelczyk and Awrejcewicz, 2015; Ciavarella et al., 2020).

In recent years, there has been a growing interest in modeling severe wear (Nguyen et al., 2018; Popov and Pohrt, 2018; Li et al., 2020). In a broad sense, severe wear is defined as a form of wear characterized by a rapid increase in the amount and size of wear particles (Blau, 1992). In many situations, the regime of severe wear can be characterized by the absence of any steady-state regime under constant conditions. This is so, for example, in wear of polymers (Viswanath and Bellow, 1995), when under constant normal load and sliding speed (see Figure 1), the wear volume loss V varies proportionally to some power of time, that is t^α, and therefore, the wear rate $\dot{w}$ will be a function of time (proportional to t^α−1) during the entire testing period.

FIGURE 1

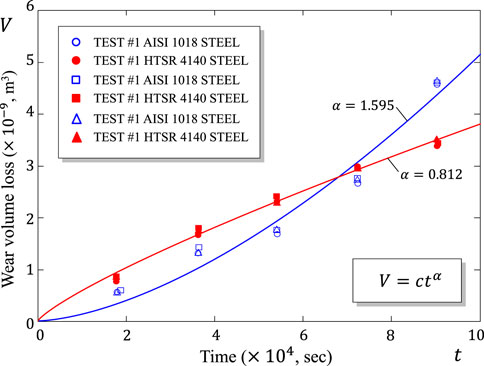
FIGURE 1. Volume loss vs. time on linear coordinates with best fit power-law lines for Delrin against two counterparts (based on the experimental data taken obtained by Viswanath and Bellow (1995)).

It is clear that in experiments like that, whose results are shown in Figure 1, Eq. 3 is not applicable. Instead we can consider the ratio V(t)/t that defines the mean volumetric wear rate. The two cases α = 0.812 and α = 1.595 differ by the decreasing/increasing trend of this quantity. The wear process with increasing in time mean volumetric wear rate (that is when α > 1) will be termed as severe wear in a narrow sense. At the same time, the case α < 1 may called mild wear, as the wear rate decreases towards zero during the wear process.

Further, when comparing the Archard Eq. 4 with the Rhee Eq. 6, we see that for polymers the Archard wear coefficient k_w, which is evaluated as the ratio V/Pvt, is found to depend on time, that is $k_{\mathrm{w}}\sim t^{\left(\right.}\alpha -1\left.\right)$. This means that for polymer-bonded friction materials, for which the Rhee wear equation applies, the concept of wear coefficient as a constant characteristic of tribo-pair is not applicable. However, the two cases α < 1 and α > 1 drastically differ in the time variation of the linear wear rate. In what follows, to avoid misinterpretations of mild and severe wear regimes, these two cases will be termed as the sub-Archard and super-Archard regimes of wear.

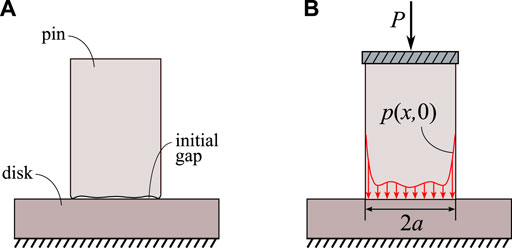
However, a natural concern arises about the effect of the wearing-in period, when the initial contact pressure evolves due to the contact geometry adaptation (Argatov I and Chai, 2020). In a pin-on-disk sliding wear tests, when a wearable pin is put on an abrasive disk, there exists a small initial gap between the surfaces brought into contact (see Figure 2A), which influences the initial contact pressure, p(x, t) at t = 0, in the loaded state (see Figure 2B).

FIGURE 2

FIGURE 2. Initial contact configuration: (A) Unloaded state; (B) Loaded state.

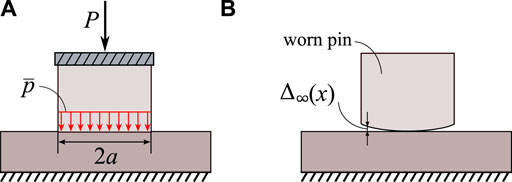
During the wear process, because of the contact geometry adaptation, the contact pressure p(x, t) evolves in time and approaches a steady-state pattern of uniform distribution over the contact zone (see Figure 3A). The corresponding steady-state shape of the pin surface facing the disk is characterized by the limiting gap function, Δ_∞(x), which does not depend on the initial gap (see Figure 3B).

FIGURE 3

FIGURE 3. Steady-state contact configuration: (A) Loaded state; (B) Unloaded state.

When the theoretical modeling framework of the contact geometry adaptation (Figures 2, 3) is applied to the case of super-Archard wear, the main problem to solve in this way is a generalization of the experimentally observed non-stationary relation between $\dot{w}$ and $\bar{p}=\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{s}\mathrm{t}$ for the practically important case p ≠ const. To date there are no publications on generalizations of the Rhee model (6) similar to the Archard wear equation, which follows from Eq. 5 by replacing the mean contact pressure $\bar{p}$ with the contact pressure p. It should be stressed that the same approach does not work for the Rhee wear Eq. 6. To this extent, the concept of fractional time-derivative can be efficiently applied to gain insight into the contact pressure evolution in severe wear.

In recent years, the apparatus of fractional differentiation has been used in mechanics, in particular, to generalize models of diffusion (Mainardi, 1996), rough contact (Argatov, 2003), and viscoelasticity (Su et al., 2021). In the present study, we use the classical Riemann–Liouville derivative. It should be noted that a number of different approaches exist to introduce a more general notion of the derivative (Ortigueira and Machado, 2015). In particular, a so-called fractal fractional derivative (Chen et al., 2010; He, 2011) does not involve the integral convolution and represents a local operator. However, whereas fractals have emerged as a useful mathematical tool in tribological research (Ling, 1990; Borodich and Onishchenko, 1993; Borodich, 1999), the present study pioneers the use of concept of the fractional derivative in modeling wear processes.

Since the theory of wear contact problem is well established (Aleksandrov and Kovalenko, 1980; Kovalenko, 1985), we adopt the approach suggested in the constructive review (Argatov I and Chai Y. S, 2020) and take a general point of view on the description of the pin/disk contact interaction, which is applicable both in the three- and two-dimensional settings. To illustrate the contact pressure evolution, we consider a two-dimensional wear contact problem (Galin, 1976), for which a detailed analysis has been available in the literature (Aleksandrov et al., 1978; Argatov and Fadin, 2011). It is anticipated that with certain modifications the fractional time-derivative approach developed below can be applied to model other temporary-spatial severe damage processes like mechanochemical corrosion (Sedova and Pronina, 2022), wear/scratch damage (Dasari et al., 2009), and wear of metamaterials (Garland et al., 2020).

The rest of the paper is organized as follows. In Section 2, we introduce a fractional time-derivative (FTD) wear equation as a straightforward generalization of the Archard wear equation by replacing the time derivative in the definition of the linear wear rate with appropriate fractional derivative. The FTD wear equation when applied under constant load conditions predicts a power-law time variation for the volumetric wear similar to the Rhee wear equation. This observation explains the main goal of the present study and that is to generalize the Rhee wear equation to the case of non-constant (spatial-temporal) variations of the contact pressure. It is hypothesized that the FTD wear equation provides such a generalization. The main body of the paper is devoted to the analysis of implications drawn from the solution of the model wear contact problem formulated in Section 3. In particular, the existence of a quasi-steady state is identified in Section 4 and, using the method of variables separation (Section 5), the evaluation of the contact pressure towards the quasi-steady state is considered in detail in Section 6. A particularly novel aspect—oscillatory nature—of the contact pressure variations is discussed in Section 7. The spatial-temporal variation of the worn profile is presented in Section 8. Finally, in Section 9, we discuss the obtained results and further generalizations and formulate the conclusions.

2 Fractional Time-Derivative Wear Equation

In its basic version due to Archard (1953), the wear equation linearly relates the wear rate at a point with coordinate x and at time t to the contact pressure as

$\dot{w}\left(x,t\right)=\kappa p\left(x,t\right)(7)$

with some constant κ, which is related to the wear coefficient, k_w, as κ = k_wv, where v is the speed of relative sliding at the contact interface.

Under the assumption that the worn material is absent at the initial moment t = 0 , that is w(x, 0) = 0, Eq. 7 is equivalent to the relation

$w\left(x,t\right)=\kappa \underset{0}{\overset{t}{\int }}p\left(x,\tau \right)\mathrm{d}\tau .(8)$

The integral form Eq. 8 of the wear Eq. 7 is used in formulating the wear contact problems (Galin, 1976; Argatov and Tato, 2012), as the wear depth w(x, t) directly describes the evolution of the contact geometry in the direction normal to the contact interface.

Let us replace the differentiation with respect to time on the left-hand side of Eq. 7 with a more general notion of the derivative. In particular, we make use of the fractional Riemann–Liouville derivative of order α, which will be denoted by ${\mathrm{D}}_t^{\alpha }$. In this way, we straightforwardly arrive at the equation

${\mathrm{D}}_t^{\alpha }w\left(x,t\right)=\kappa p\left(x,t\right),(9)$

which contains two parameters, namely, κ and α.

In the framework of fractional calculus, from Eq. 9, it follows that

$w\left(x,t\right)=\frac{\kappa }{\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{p\left(x,\tau \right)}{{\left(t-\tau \right)}^{1-\alpha }}\mathrm{d}\tau ,(10)$

where Γ(x) is the gamma function.

The right-hand side of Eq. 10 contains the Riemann–Liouville integral of order α which is consistent with the inversion of the respective fractional derivative appearing in Eq. 9.

It is to emphasize that by taking α = 1 in Eq. 10, in view of the normalization condition Γ(1) = 1, we recover the integral form Eq. 8 of the non-fractional Archard wear equation. Therefore, it makes sense to assume that 0 < α < 2, since Eq. 9 generalizes Archard’s Eq. 7, which exactly corresponds to the basic case α = 1.

Remark 1. Observe that in the case of steady state, when $p(x,t)\equiv \bar{p}$, Eq. 10 yields

$w=\frac{\kappa \bar{p}}{\alpha \mathrm{\Gamma }\left(\alpha \right)}{t}^{\alpha },(11)$

and thus, the mean linear wear rate (evaluated from the very onset of the wear process) will be non-constant

$\frac{w}{t}=\frac{\kappa \bar{p}}{\alpha \mathrm{\Gamma }\left(\alpha \right)}{t}^{\alpha -1}.$

In view of Eq. 1, from Eq. 11, it follows that

$V=\frac{\kappa P}{\alpha \mathrm{\Gamma }\left(\alpha \right)}{t}^{\alpha }.(12)$

Eq. 12 can be fitted to the experimental data shown in Figure 1 to uniquely determine both parameters κ and α.

3 Wear Contact Problem Formulation

Following Komogortsev (1985) and Argatov and Fadin (2011), we consider a wear contact problem for an elastic solid (pin) in contact with a rigid base (disk) with a fixed zone of contact, x ∈ ω, under a constant normal load, P. In this case, the resultant of the contact pressure p(x, t) satisfies the equilibrium equation

$\underset{\omega }{\int }p\left(x,t\right)\mathrm{d}x=P,t\ge 0.(13)$

The wear contact problem can be reduced to the following governing integral equation in the domain x ∈ ω, t ≥ 0, which involves two unknowns, namely, the contact pressure p(x, t) and the contact displacement δ₀(t):

$\underset{\omega }{\int }K\left(x,\xi \right)p\left(\xi ,t\right)\mathrm{d}\xi ={\delta }_0\left(t\right)-\left[{\mathrm{\Delta }}_0\left(x\right)+w\left(x,t\right)\right].(14)$

Here, K(x, ξ) is a given surface-influence function (with x and ξ being the points of observation and integration), Δ₀(x) is a known function of initial gap between the contacting surfaces, and w(x, t) is the wear depth which is related to the contact pressure p(x, t) by Eq. 10. It is to note that the gap function is usually subject to the centering condition Δ₀(0) = 0.

We recall that the surface-influence function K(x, ξ) is defined as the normal component of the corresponding vector Green’s function restricted to the surface of the elastic solid, and thus, the equilibrium equations inside the elastic solid (Shillor et al., 2004) are naturally satisfied by the construction of the function K(x, ξ). The reduction of the wear contact problem to the corresponding governing integral equation is well known (Argatov I and Chai Y. S, 2020) and allows applying the boundary element method (Sfantos and Aliabadi, 2006) for the direct evaluation of the contact pressures.

4 Uniform Contact Pressure as a Quasi-Steady State

By integrating Eq. 10 over the contact interval and taking into account the equilibrium Eq. 13, we derive the following the relations for the volumetric wear loss:

$\begin{array}{ll}\hfill V\left(t\right)& =\underset{\omega }{\int }w\left(x,t\right)\mathrm{d}x\hfill \\ \hfill & =\frac{\kappa }{\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{\mathrm{d}\tau }{{\left(t-\tau \right)}^{1-\alpha }}\underset{\omega }{\int }p\left(x,\tau \right)\mathrm{d}x=\frac{\kappa P{t}^{\alpha }}{\alpha \mathrm{\Gamma }\left(\alpha \right)}.\hfill \end{array}(15)$

Thus, making use of Eq. 15 and following the previously introduced method (Komogortsev, 1985), we can reduce the governing integral Eq. 14 to the equation

$\underset{\omega }{\int }\left(K\left(x,\xi \right)-\frac{1}{A}{K}_1\left(\xi \right)\right)\left[p\left(\xi ,t\right)-p\left(\xi ,0\right)\right]\mathrm{d}\xi +w\left(x,t\right)=\frac{\kappa P}{A}\frac{t^{\alpha }}{\alpha \mathrm{\Gamma }\left(\alpha \right)},(16)$

where x ∈ ω and t > 0. Moreover, A denotes the measure of the contact area defined as

$A=\underset{\omega }{\int }\mathrm{d}x,(17)$

and we have introduced the notation

$K_1\left(\xi \right)=\underset{\omega }{\int }K\left(x,\xi \right)\mathrm{d}x.(18)$

The form of Eq. 16 is preferable in wear contact problems (Komogortsev, 1985), as the effect of the initial contact configuration, which is associated with the gap function Δ₀(x), is now incorporated into the initial contact pressure density p(x, 0).

Now, let us introduce the notation for the mean contact pressure and the point-wise deviation of the contact pressure from its mean value

$\bar{p}=\frac{P}{A},q\left(x,t\right)=p\left(x,t\right)-\bar{p}.(19)$

Thus, in view of (Eqs 15, 19), Eq. 16 can be rewritten in the form

$\underset{\omega }{\int }{K}_2\left(x,\xi \right)\left[q\left(\xi ,t\right)-q\left(\xi ,0\right)\right]\mathrm{d}\xi +\frac{\kappa }{\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{q\left(x,\tau \right)}{{\left(t-\tau \right)}^{1-\alpha }}\mathrm{d}\tau =0,(20)$

where x ∈ ω and t > 0, and we have introduced the notation

$K_2\left(x,\xi \right)=K\left(x,\xi \right)-\frac{1}{A}{K}_1\left(\xi \right)-\frac{1}{A}{K}_1\left(x\right).(21)$

We note that in regard to the kernel of the integral Eq. 16, the third term on the right-hand side of Eq. 21 has been introduced for symmetrization (Argatov and Chai, 2019). This is convenient but does not affect the result because of the zero-mean property

$\underset{\omega }{\int }q\left(\xi ,t\right)\mathrm{d}\xi =0,t\ge 0,(22)$

which follows from the definition (19)₂ of the function q(x, t) and the equation of equilibrium Eq. 13.

To this end, the behavior of the residual function q(x, t) as time progresses is shown to be described by Eq. 20. By analogy with the wear contact problems based on the Archard wear equation, it can be anticipated that the deviation of the contact pressure from its mean value diminishes with time. In the case under consideration, we also find that q(x, t) → 0 as t → ∞, and hence the mean contact pressure $\bar{p}$ will be termed as a quasi-steady-state solution.

5 Non-Dimensionalization and Separation of Variables

For the sake of simplicity, we assume that the pin material is isotropic and can be characterized by Young’s modulus, E, and Poisson’s ratio, ν. In contact mechanics, an important role is played by the reduced elastic modulus E* = E/(1 − ν²). Also, let a denote a characteristic size of the contact domain ω, which can be taken to be equal to the half-diameter of ω.

Following Komogortsev (1985), we take advantage of the fact that Eq. 20, in view of Eq. 10, is in a separable form (the kernel function K₂(x, ξ) does not depend on the time variable t) that makes it possible to construct its solution in the form

$q\left(x,t\right)=\sum _{n=1}^{\infty }{b}_n\left(t\right){\varphi }_n\left(x\right),(23)$

where ϕ_n(x) is the nth eigenfunction of the integral operator with the kernel K₂(x, ξ) given by Eq. 21, i.e.,

$\frac{E^{*}}{a}\underset{\omega }{\int }{K}_2\left(x,\xi \right){\varphi }_n\left(\xi \right)\mathrm{d}\xi ={\lambda }_n{\varphi }_n\left(x\right),x\in \omega .(24)$

We note that the non-dimensionalising factor E*/a has been introduced on the left-hand side of Eq. 24 to ensure that the eigenvalues λ₁, λ₂, … are dimensionless. It is pertinent to note here that, in view of Eqs 21, 22, the eigenfunctions possess the zero-mean property

$\underset{\omega }{\int }{\varphi }_n\left(\xi \right)\mathrm{d}\xi =0\left(n=\mathrm{1,2},\dots \right).(25)$

Without loss of generality, we assume that the solutions of the eigenvalue problem Eq. 24 are normalized as

$\frac{1}{A}\underset{\omega }{\int }{\left[{\varphi }_n\left(\xi \right)\right]}^2\mathrm{d}\xi =1\left(n=\mathrm{1,2},\dots \right).(26)$

Thus, taking into account relations (19)₂ and Eq. 23, we can represent the contact pressure in the form

$p\left(x,t\right)=\bar{p}+\sum _{n=1}^{\infty }{b}_n\left(t\right){\varphi }_n\left(x\right),(27)$

where $\bar{p}$ is given by (19)₁, and the set of coordinate functions ϕ_n(x) is uniquely defined by Eqs 24, 26).

By setting t = 0, from Eq. 27, it follows that

$p\left(x,0\right)=\bar{p}+\sum _{n=1}^{\infty }{b}_n\left(0\right){\varphi }_n\left(x\right),(28)$

where p(x, 0) solves the integral equation of initial contact, which is obtained from Eq. 14 by setting t = 0, whereas, in view of Eq. 26, the coefficients of the infinite sum are given by

$b_n\left(0\right)=\frac{1}{A}\underset{\omega }{\int }\left[p\left(x,0\right)-\bar{p}\right]{\varphi }_n\left(x\right)\mathrm{d}x.(29)$

In the next section, we consider the evolution of the functions b_n(t), which satisfy the initial conditions (29). The corresponding equation is simply obtained by substituting the expansion (Eq. 23) into Eq. 20 and utilizing Eq. 24.

6 Evolution of the Contact Pressure Towards the Quasi-Steady State

In view of Eq. 24, the series solution Eq. 23 satisfies Eq. 20, if and only if

$b_n\left(t\right)+\frac{E^{*}\kappa }{{\lambda }_{n}a\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{b_n\left(\tau \right)}{{\left(t-\tau \right)}^{1-\alpha }}\mathrm{d}\tau =b_n\left(0\right).(30)$

Equation 30 is classified as Abel’s integral equation of the second kind (see, e.g., (Gorenflo and Mainardi, 1997; Gorenflo et al., 2014)), and its solution b_n(t) is given in the form

$b_n\left(t\right)=b_n\left(0\right)E_{\alpha }\left(-\frac{E^{*}\kappa }{{\lambda }_{n}a}{t}^{\alpha }\right)(31)$

in terms of the single-parameter Mittag-Leffler function of order α, defined as

$E_{\alpha }\left(x\right)=\sum _{k=0}^{\infty }\frac{x^k}{\mathrm{\Gamma }\left(\alpha k+1\right)},(32)$

where Γ(x) is the gamma function.

It is also convenient to consider the Mittag-Leffler type function

$e_{\alpha }\left(x\right)=E_{\alpha }\left(-x^{\alpha }\right),(33)$

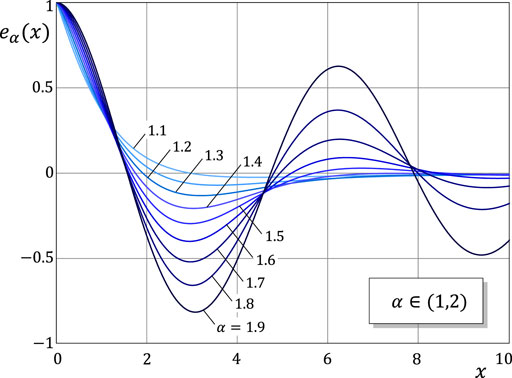
which for α ∈ (0, 1) is known (Mainardi, 2020) to be completely monotone on the positive real semi-axis. The variation of the function e_α(x) for different values of the parameter α ∈ (1, 2) in shown in Figure 4.

FIGURE 4

FIGURE 4. Mittag-Leffler type function.

Moreover, let us introduce a characteristic time of the wearing-in process

${\tau }_{\text{in}}={\left(\frac{a}{E^{*}\kappa }\right)}^{1/\alpha }.(34)$

So, in view of Eqs 33, 34, Eq. 31 can be represented as

$b_n\left(t\right)=b_n\left(0\right)e_{\alpha }\left(\frac{t}{{\lambda }_n^{1/\alpha }{\tau }_{\text{in}}}\right)(35)$

and thus, using Eqs 27, 35, we find that

$p\left(x,t\right)=\bar{p}+\sum _{n=1}^{\infty }{b}_n\left(0\right)e_{\alpha }\left(\frac{t}{{\lambda }_n^{1/\alpha }{\tau }_{\text{in}}}\right){\varphi }_n\left(x\right).(36)$

To characterize the evolution of the contact pressure p(x, t) as the time variable t tends to infinity, we consider the following asymptotic formula for e_α(x), α ∈ (0, 2), α ≠ 1, is known for sufficiently large values of the argument (Mainardi, 2020):

$e_{\alpha }\left(x\right)\sim \frac{\sin \left(\alpha \pi \right)}{\pi }\frac{\mathrm{\Gamma }\left(\alpha \right)}{x^{\alpha }},x\to \infty .(37)$

Thus, in view of Eqs 36, 37, the contact pressure approaches the uniform distribution $\bar{p}$ at large times. It is to emphasize that this conclusion is valid for both cases α ∈ (0, 1) and α ∈ (1, 2). Finally, we note that when α = 1, we have E_α( − x^α) = exp( − x), and thus we recover the known solution for the wear contact problem (Komogortsev, 1985; Argatov and Fadin, 2011), and the approach of p(x, t) to $\bar{p}$ is exponentially fast.

Remark 2. It should be noted that the asymptotic Eq. 37 reflects the decaying behavior of the Mittag-Leffler function E_α(x) on the negative real axis as x → −∞. This formula can be used to analyze the asymptotics of the contact pressure (Eq. 36), provided all eigenvalues λ_n are positive. To prove that this is the fact, we consider the elastic energy, ${\mathcal{E}}_0$, produced by the surface load q(x, 0), which, in view of Eqs 23, 26, can be evaluated as follows:

$\begin{array}{ll}\hfill 2{\mathcal{E}}_0& =\underset{\omega }{\int }q\left(x,0\right)\underset{\omega }{\int }K\left(x,\xi \right)q\left(\xi ,0\right)\mathrm{d}\xi \mathrm{d}x\hfill \\ \hfill & =\sum _{m=1}^{\infty }{b}_m\left(0\right)\underset{\omega }{\int }{\varphi }_n\left(x\right)\left\{\frac{E^{*}}{a}\sum _{n=1}^{\infty }{\lambda }_n{b}_n\left(0\right){\varphi }_n\left(x\right)+\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{s}\mathrm{t}\right\}\mathrm{d}x\hfill \\ \hfill & =\frac{E^{*}A}{a}\sum _{n=1}^{\infty }{\lambda }_n{\left[b_n\left(0\right)\right]}^2.\hfill \end{array}(38)$

Thus, since the elastic energy ${\mathcal{E}}_0$ is positive for any nontrivial set of coefficients b_n(0), n = 1, 2, …, from Eq. 38 it follows that λ_n > 0, n = 1, 2, ….

7 Oscillatory Nature of the Contact Pressure Variation in Super-archard Wear

Equation 37 provides information about the limiting evolution of the contact pressure p(x, t) as the time variable t tends to infinity. During the wearing-in period, the evolution of p(x, t) strongly depends on the variation of the Mittag-Leffler type function e_α(x), which changes its behavior from completely monotonic for α ∈ (0, 1) to decaying oscillatory for α ∈ (1, 2) (see Figure 4).

To illustrate the contact pressure variation during the wearing-in period, we consider the example of two-dimensional contact for an elastic layer and a wearable indenter, which establishes a fixed contact interval x ∈ ( − a, a) of a relatively small half-width, a, compared to the layer thickness. The corresponding wear contact problem in the case of the Archard type wear equation was studied by Aleksandrov et al. (1978), using the Galerkin method for evaluating the eigenfunctions. We refer to the paper by Argatov and Fadin (2011) for more details.

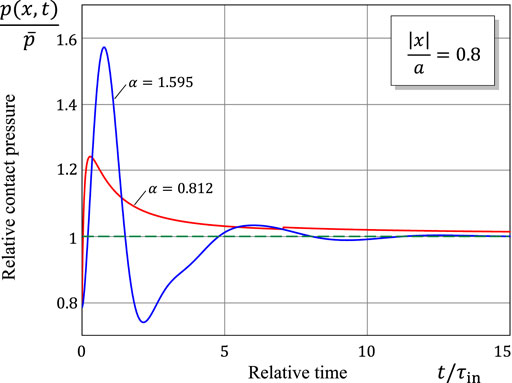
Figure 5 shows the time-variation of the relative contact pressure $p(x,t)/\bar{p}$ at the center of the contact zone, x = 0, for the cases of sub-Archard (α = 0.812) and super-Archard (α = 1.595) wear. It is clear that both curves start from the same point at t = 0 (because of the same initial condition) and tends to a horizontal line of unit value as t → ∞ (because of the same quasi-steady state). A striking difference between them occurs during the wearing-in period that lasts about a few times as much as the characteristic time τ_in, where the super-Archard curve shows a marked decaying oscillatory behavior.

FIGURE 5

FIGURE 5. Time-variation of the contact pressure at the center of the contact zone.

In the example of contact geometry under consideration, which is taken to be almost flat (with a small initial gap between the contacting surfaces), the initial contact pressure shows a strong stress concentration in a periphery region of contact (see curves τ = 0 in Figures 6, 7). That is why, in the initial period of time, the wear rate ${\mathrm{D}}_t^{\alpha }w(x,t)$ will be much higher near the contact contour, and, as a result of the contact geometry adaptation, the evolution of the contact pressure in the periphery region may be non-monotonic even in the sub-Archard wear case (see Figure 8). However, as it is seen from both Figures 5, 8, the oscillatory type of the contact pressure evolution is a characteristic feature of the case of super-Archard wear only.

FIGURE 6

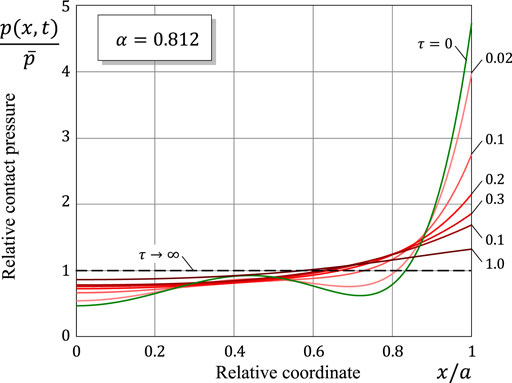
FIGURE 6. Space-variation of the contact pressure for different values of the relative time variable τ = t/τ_in in the sub-Archard wear case.

FIGURE 7

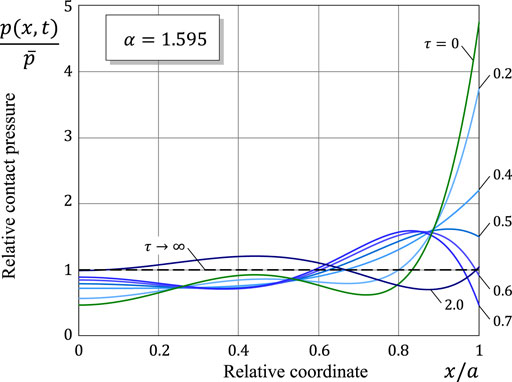
FIGURE 7. Space-variation of the contact pressure for different values of the relative time variable τ = t/τ_in in the super-Archard wear case.

FIGURE 8

FIGURE 8. Time-variation of the contact pressure at some point away from the center.

The discrete-time progression of the spatial variation of the contact pressure is shown in Figures 6, 7 in the cases of sub-Archard and super-Archard wear, respectively. Observe that a drastic reduction in the contact stress concentration occurs during the initial time interval of duration comparable with the characteristic time τ_in. From the comparison of Figures 6, 7, it can be concluded that the wearing-in period in super-Archard wear lasts much longer than in the case of sub-Archard wear.

8 Evolution of the Worn Profile

Due to the wear process, the contact geometry gradually changes, while the contact pressure approaches a uniform distribution of the applied load over the contact area. The initial contact geometry is characterized by the initial gap function Δ₀(x), which is subject to the centering condition Δ₀(0) = 0. The current gap function, Δ(x, t), is defined as follows (Argatov I. I and Chai Y. S, 2020):

$\mathrm{\Delta }\left(x,t\right)=\underset{\omega }{\int }\left[K\left(0,\xi \right)-K\left(x,\xi \right)\right]p\left(\xi ,t\right)\mathrm{d}\xi .(39)$

When the contact pressure density p(x, t) is given by the eigenfunction expansion method in the form Eq. 36, in view of Eqs 18, 24, we will have

$\begin{array}{ll}\hfill \underset{\omega }{\int }K\left(x,\xi \right)p\left(\xi ,t\right)\mathrm{d}\xi & =\bar{p}{K}_1\left(x\right)+\frac{a}{E^{*}}\sum _{n=1}^{\infty }{\lambda }_n{b}_n\left(t\right){\varphi }_n\left(x\right)\hfill \\ \hfill & +\sum _{n=1}^{\infty }\frac{b_n\left(t\right)}{A}\underset{\omega }{\int }{K}_1\left(\xi \right){\varphi }_n\left(\xi \right)\mathrm{d}\xi ,\hfill \end{array}(40)$

where we have used the zero-mean property (Eq. 22) of the eigenfunctions ϕ_n(x), n = 1, 2, …, and the symmetry property of the surface influence function, that is K(x, ξ) = K(ξ, x).

Since b_n(t) → 0 as t → ∞, the limiting gap function is defined only by the first term on the right-hand side of Eq. 40, and thus, we obtain

${\mathrm{\Delta }}_{\infty }\left(x\right)=\bar{p}\left[K_1\left(0\right)-K_1\left(x\right)\right].(41)$

So, from Eqs 39–41, it follows that

$\mathrm{\Delta }\left(x,t\right)={\mathrm{\Delta }}_{\infty }\left(x\right)+\frac{a}{E^{*}}\sum _{n=1}^{\infty }{\lambda }_n{b}_n\left(t\right)\left[{\varphi }_n\left(0\right)-{\varphi }_n\left(x\right)\right].(42)$

The evolution of the relative gap function is illustrated in Figures 9, 10. It is of interest to observe a non-monotonic progression of the worn profile Δ(x, t) towards the limiting shape Δ_∞(x) in the case of super-Archard wear.

FIGURE 9

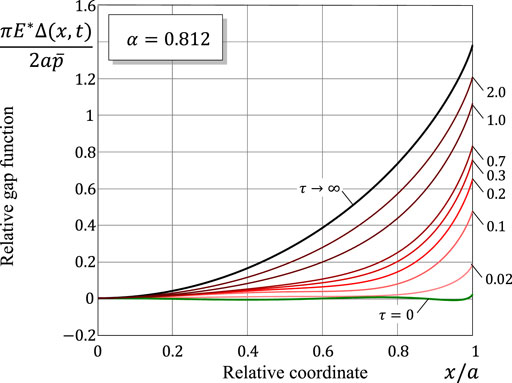
FIGURE 9. Space-variation of the gap function for different values of the relative time variable τ = t/τ_in in the sub-Archard wear case.

FIGURE 10

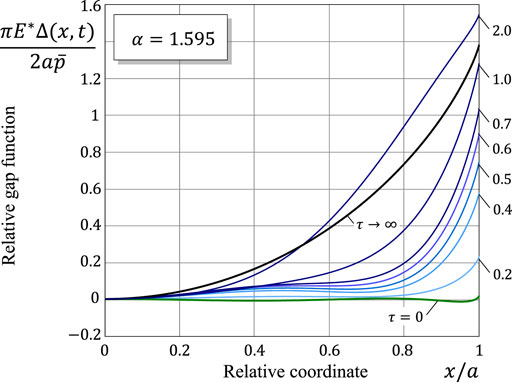
FIGURE 10. Space-variation of the gap function for different values of the relative time variable τ = t/τ_in in the super-Archard wear case.

Figure 11 presents the evolution of wear depth w(0, t), which corresponds to the evolution of the contact pressure at the center of the contact zone shown in Figure 5. It is to note that, in view of Eq. 34, the wear equation transforms as

$w\left(x,t\right)=\frac{a}{E^{*}\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t/{\tau }_{\text{in}}}{\int }}{\left(\frac{t}{{\tau }_{\text{in}}}-\bar{\tau }\right)}^{\alpha -1}p\left(x,\bar{\tau }\right)\mathrm{d}\bar{\tau },$

where $\bar{\tau }$ is a dimensionless integration time-variable. The above formula explains the normalization used in Figures 11–13.

FIGURE 11

FIGURE 11. Time-variation of the wear depth at the center of the contact zone.

Interpretation of the evolution of the relative gap function shown in Figures 9, 10 will be simplified if we consider the evolution of the pin shape described by the function D(x, t) = Δ₀(x) + w(x, t) (see Figures 12, 13). It is of interest to observe that in the case of super-Archard wear, the evolution of the worn profile is also found to be slightly oscillatory (see Figures 10, 13), as it could be predicted based on Eq. 39, which defines the current gap function, and the oscillatory evolution of the contact pressure. It should be emphasized that, though the worn profile progresses non-monotonically in time, this does not mean that locally (in spatial sense) there could exist profile growth, because the fractional wear Eq. 9 predicts a positive wear depth w(x, t) for any positive contact pressure history p(x, τ), τ ∈ (0, t).

FIGURE 12

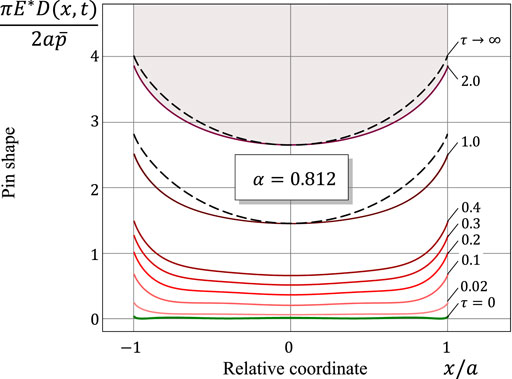
FIGURE 12. Pin shape at different values of the relative time variable τ = t/τ_in in the sub-Archard wear case. The dashed lines correspond to the limiting profile.

FIGURE 13

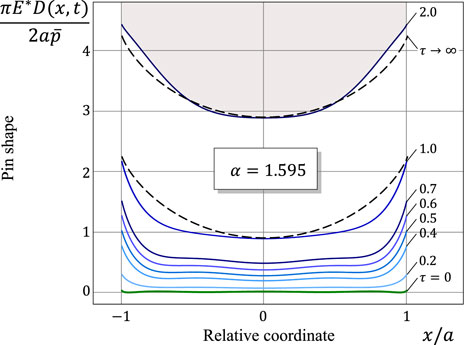
FIGURE 13. Pin shape at different values of the relative time variable τ = t/τ_in in the super-Archard wear case. The dashed lines correspond to the limiting profile.

In the case of sub-Archard wear, when 0 < α < 1, the duration of wearing-in process, when the contact pressure approaches the mean value $\bar{p}$ over the entire contact area, is proportional to the characteristic time parameter τ_in, introduced by Eq. 34. In the case of super-Archard wear, as it is seen from Figure 14, the duration of the wearing-in period, T_in, strongly depends on the value of α ∈ (1, 2), because, if it increases, the amplitude of the decaying oscillations increases as well.

FIGURE 14

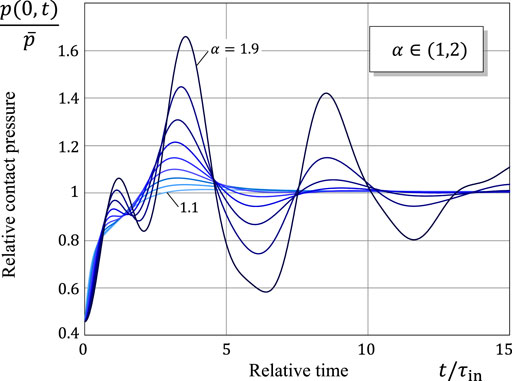
FIGURE 14. Time-variation of the contact pressure at the center of the contact zone for different values of the parameter α ranging from 0.1 to 0.9 with an increment of 0.1.

9 Discussion and Conclusion

First of all, we note that the example of two-dimensional contact has been used for illustrative purposes. A more realistic information about the eigenvalues λ_n, which depend on the global geometry of the wearable pin, can be obtained by means of finite element simulations (Liu et al., 2014).

We would like to emphasize that the numerical results presented in Figures 5–13 were obtained based on the exact solution of the model two-dimensional wear contact problem for an elastic layer (Aleksandrov et al., 1978) with a controllable error determined by the approximate evaluation of the eigenvalues λ_n. In the analysis of real pin-on-disk experiments, the application of FEM-based numerical methods is required (Liu et al., 2014). However, the oscillating nature of the contact pressure variation observed in Figure 5 is caused by the properties of solutions (see Figure 4) of Abel’s integral Eq. 30, which will remain the same in the three-dimensional contact problems. To be more precise, the contact geometry affects the eigenvalue problem Eq. 24, which depends on the surface-influence function (see Eq. 21), whereas the evolution Eq. 30 is fully determined by the adopted fractional time-derivative (FTD) model for wear. This means that while FEM simulations cannot serve for the model verification, spatial-temporal variation of the contact geometry and the contact pressures, if observed experimentally, would support the introduced FDT wear equation. At the same time, though verification experimental studies fall outside the scope of this theoretical work, the simple analytical model presented above will definitely aid in planning such experiments.

Based on the presented analysis, it becomes clear that the temporary evolution of wear process, which is governed by the fractional time-derivative model (10), is determined by the properties of the Mittag-Leffler type function e_α(x), which, in turn, strongly depend on the value of the index α. In particular, for α ∈ (1, 2), the number of zeros, n(α), of the function e_α(x), x ∈ (0, ∞), which determines the number of oscillations, exponentially increases as α approaches 2 (see Figure 15).

FIGURE 15

FIGURE 15. Number of real zeros of the Mittag-Leffler type function e_α(x) based on the numerical results from presented by Hanneken et al. (2007).

Observe that the FTD wear Eq. 9 is linear, and under the constant load condition (Eq. 13) it implies the following relation between the contact load and the worn volume (see Eq. 12):

$V=kP{t}^{\alpha }.(43)$

Here, k = κ/αΓ(α) is a constant coefficient. It is to emphasize again that Eq. 43 is in complete agreement with the Rhee Eq. 6, which in this special case (γ = 1) takes the form

$V=K_{\mathrm{w}}P{v}^{\beta }{t}^{\alpha }.(44)$

Thus, by comparing Eqs 43, 44, we can write that k = K_wv^β, and therefore, we obtain κ = αΓ(α)K_wv^β. In other words, under the condition of constant sliding velocity, the wear coefficient κ in the FTD wear Eq. 9 may be regarded to be parametrically dependent on the sliding velocity v. The effect of surface roughness of the hard counterpart (disk), along which a wearable solid (pin) slides, is incorporated in the value of the coefficient κ as well.

It is of practical interest to have a closer look at the quality of power-law approximations of the experimental data for the volumetric wear loss under constant load (Viswanath and Bellow, 1995), which are presented in Figure 1. In particular, it is instructive to consider the variation of the absolute error as shown in Figure 16, from where it is seen that in the case of super-Archard wear (α > 1), the experimental data exhibits pronounced deviations from the power-law curve. This fact implies that the fractional wear rate ${\mathrm{D}}_t^{\alpha }w(x,t)$ may depend nonlinearly on the contact pressure. In this way, we can generalize Eq. 9 as

${\mathrm{D}}_t^{\alpha }w\left(x,t\right)=\kappa {\left[p\left(x,t\right)\right]}^{\gamma }(45)$

by introducing an additional fitting constant γ.

FIGURE 16

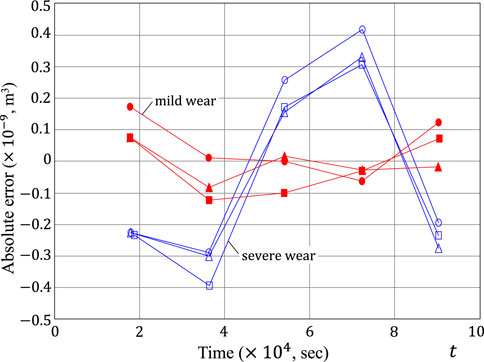
FIGURE 16. Difference between the power-law predictions and the experimental data (Viswanath and Bellow, 1995) shown in Figure 1 (the same legend applies).

Correspondingly, in light of Eq. 45, Eq. 10 can be generalized as follows:

$w\left(x,t\right)=\frac{\kappa }{\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{{\left[p\left(x,\tau \right)\right]}^{\gamma }}{{\left(t-\tau \right)}^{1-\alpha }}\mathrm{d}\tau .(46)$

As a consequence of the nonlinear wear Eq. 46, the volumetric wear loss V(t) will no longer be predicted by the power-law Eq. 15. Indeed, by integrating Eq. 46, we arrive at the equation

$V\left(t\right)=\frac{\kappa }{\mathrm{\Gamma }\left(\alpha \right)}\underset{0}{\overset{t}{\int }}\frac{\mathrm{d}\tau }{{\left(t-\tau \right)}^{1-\alpha }}\underset{\omega }{\int }{\left[p\left(x,\tau \right)\right]}^{\gamma }\mathrm{d}x,(47)$

where the inner integral over the contact domain will no longer be constant under a constant load, but may become oscillatory during the wear process.

It is to note that the nonlinearity in the fractional wear Eqs 45–47 has been introduced in the spirit of the Archard–Kragelsky model for the linear wear rate (Kragelsky, 1965), and thus, they can be applied under the assumption of constant speed of relative sliding at the contact interface with the wear coefficient κ being nonlinearly dependent on the sliding speed.

It should be emphasized that though the simple example of two-dimensional wear contact problem has been employed in the analysis above, the main findings about the decaying oscillatory nature of the approach to the quasi-steady state will hold true for the corresponding three-dimensional contact configurations, since this feature is rooted in the fractional time description of the wear process.

Observe (Soldatenkov, 2010) that the wear Eq. 10 is merely a special case of the more general type

$w\left(x,t\right)=\underset{0}{\overset{t}{\int }}{K}_1\left(\tau \right)K_2\left(t-\tau \right)p\left(x,\tau \right)\mathrm{d}\tau ,(48)$

where K₁(t) and K₂(t) are known functions. In particular, Eq. 48 with K₁(t) ≡ 1 and K₂(t) = (κ/Γ(α))t^α−1 simply coincides with Eq. 10. On the other side, it is of interest to observe that Eq. 48 with K₁(t) = (κ/Γ(α))t^α−1 and K₂(t) ≡ 1 in the case of constant loading also leads to Eq. 11. Moreover, it can be shown that Eq. 9 with ${\mathrm{D}}_t^{\alpha }$ being interpreted as the fractal derivative of the order α is also consistent with Rhee’s Eq. 6, γ = 1, which served as a basis for introducing the fractional wear Eq. 9.

Thus, there are possible different ways of generalizing the Rhee wear Eq. 6 to the case of non-constant (spatial-temporal) variations of the contact pressure, which have the same power-law time behavior in the steady state. While the present study gives no physical motivation for the fractional wear model (9), the hypotheses and implications formulated above can be verified experimentally without much difficulty. Also, a separate study is required to identify key differences and similarities between the wear equations discussed in the previous paragraph.

The systematic analysis of the physical variables that influence the wear process of polymers (Viswanath and Bellow, 1995) is showing an intensive effect of temperature and thus deserves a comment that the thermo-elasticity framework (Yevtushenko and Pyryev, 1999) should be applied for modeling contact deformations once the temperature exceeds a certain threshold.

To conclude, the fractional time-derivative wear rate is introduced to describe a power-law time-growth of the volumetric wear loss under constant loading conditions, which is encountered in wear testing of polymers. The newly introduced fractional time-derivative wear equation allows to extend the experimentally observed dependence to the case of a variable contact pressure. The cases of sub-Archard and super-Archard wear are distinguished based on the value of the time exponent α ∈ (0, 2), which describes decreasing (α < 1, “mild” wear) or increasing (α > 1, “severe” wear) variation of the mean wear rate.

A striking implication of the developed wear contact model is the prediction of a decaying oscillatory behavior for the contact pressure variation in time during the wearing-in period, when it approaches a uniform distribution over the contact area. The main theoretical finding of the paper is supported by the analysis of the power-law approximations for the volumetric wear loss obtained under constant applied loads. The developed fractional time-derivative approach can be applied to modeling other temporary-spatial damage processes that maintain a power-law growth of the accumulated damage under constant loading conditions.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.

Author Contributions

The author confirms being the sole contributor of this work and has approved it for publication.

Conflict of Interest

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

The reviewer EW declared a shared affiliation with the author IV to the handling editor at the time of review.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

The financial support from the Ba-Yu Scholar program of Chongqing City (China) is gratefully acknowledged. The author would like to thank Dr. Dmitry Ponomarev (TU Wien) for useful discussions. Also, the author expresses his gratitude to the Referees for their insightful and constructive comments on this study. We acknowledge support by the German Research Foundation and the Open Access Publication Fund of TU Berlin.

References

Aleksandrov, V. M., and Kovalenko, E. V. (1980). Plane Contact Problems of the Theory of Elasticity for Nonclassical Regions in the Presence of Wear. J. Appl. Mech. Tech. Phys. 21, 421–427.

Google Scholar

Aleksandrov, V. M., Galin, L. A., and Piriev, N. P. (1978). A Plane Contact Problem for an Elastic Layer of Considerable Thickness in the Presence of Wear. Mech. Sol. 4, 60–67.

Google Scholar

Archard, J. F. (1953). Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 24, 981–988. doi:10.1063/1.1721448

CrossRef Full Text | Google Scholar

Argatov, I. I., and Chai, Y. S. (2019). Effective Wear Coefficient and Wearing-In Period for a Functionally Graded Wear-Resisting Punch. Acta Mech. 230, 2295–2307. doi:10.1007/s00707-019-2366-9

CrossRef Full Text | Google Scholar

Argatov, I. I., and Fadin, Y. A. (2011). A Macro-Scale Approximation for the Running-In Period. Tribol Lett. 42, 311–317. doi:10.1007/s11249-011-9775-9

CrossRef Full Text | Google Scholar

Argatov, I., and Tato, W. (2012). Asymptotic Modeling of Reciprocating Sliding Wear - Comparison with Finite-Element Simulations. Eur. J. Mech. - A/Solids 34, 1–11. doi:10.1016/j.euromechsol.2011.11.008

CrossRef Full Text | Google Scholar

Argatov, I., and Chai, Y. S. (2020). Contact Geometry Adaptation in Fretting Wear: A Constructive Review. Front. Mech. Eng. 6, 51. doi:10.3389/fmech.2020.00051

CrossRef Full Text | Google Scholar

Argatov, I. I., and Chai, Y. S. (2020). Wear Contact Problem with Friction: Steady-State Regime and Wearing-In Period. Int. J. Sol. Structures 193-194, 213–221. doi:10.1016/j.ijsolstr.2020.02.019

CrossRef Full Text | Google Scholar

Argatov, I. I. (2003). Theory of Unsaturated Elastic Contact of Rough Surfaces. J. Friction Wear 24, 22–29.

Google Scholar

P. J. Blau (Editor) (1992). ASM Handbook, Volume 18–Friction, Lubrication, and Wear Technology (Russell Township: ASM International).

Google Scholar

Blau, P. J. (2005). On the Nature of Running-In. Tribology Int. 38, 1007–1012. doi:10.1016/j.triboint.2005.07.020

CrossRef Full Text | Google Scholar

Borodich, F. M., and Onishchenko, D. A. (1993). Fractal Roughness in Contact and Friction Problems (The Simplest Models). J. Friction Wear 14, 14.

Google Scholar

Borodich, F. M. (1999). Fractals and Fractal Scaling in Fracture Mechanics. Int. J. Fracture 95, 239–259. doi:10.1007/978-94-011-4659-3_13

CrossRef Full Text | Google Scholar

Chen, W., Zhang, X.-D., and Korošak, D. (2010). Investigation on Fractional and Fractal Derivative Relaxation- Oscillation Models. Int. J. Nonlinear Sci. Numer. Simulation 11, 3–10. doi:10.1515/ijnsns.2010.11.1.3

CrossRef Full Text | Google Scholar

Ciavarella, M., Papangelo, A., and Barber, J. R. (2020). Effect of Wear on the Evolution of Contact Pressure at a Bimaterial Sliding Interface. Tribology Lett. 68, 1–7. doi:10.1007/s11249-020-1269-1

CrossRef Full Text | Google Scholar

Dasari, A., Yu, Z.-Z., and Mai, Y.-W. (2009). Fundamental Aspects and Recent Progress on Wear/scratch Damage in Polymer Nanocomposites. Mater. Sci. Eng. R: Rep. 63, 31–80. doi:10.1016/j.mser.2008.10.001

CrossRef Full Text | Google Scholar

Dundurs, J., and Comninou, M. (1980). Shape of a Worn Slider. Wear 62, 419–424. doi:10.1016/0043-1648(80)90183-0

CrossRef Full Text | Google Scholar

Galin, L. A. (1976). Contact Problems of the Theory of Elasticity in the Presence of Wear. J. Appl. Maths. Mech. 40, 931–936. doi:10.1016/0021-8928(76)90132-5

CrossRef Full Text | Google Scholar

Gardos, M. N. (1982). Self-lubricating Composites for Extreme Environment Applications. Tribology Int. 15, 273–283. doi:10.1016/0301-679x(82)90084-6

CrossRef Full Text | Google Scholar

Garland, A. P., Adstedt, K. M., Casias, Z. J., White, B. C., Mook, W. M., Kaehr, B., et al. (2020). Coulombic Friction in Metamaterials to Dissipate Mechanical Energy. Extreme Mech. Lett. 40, 100847. doi:10.1016/j.eml.2020.100847

CrossRef Full Text | Google Scholar

Gorenflo, R., and Mainardi, F. (1997). “Fractional Calculus: Integral and Differential Equations of Fractional Order,” in Fractals and Fractional Calculus in Continuum Mechanics. Editors A. Carpinteri, and F. Mainardi (Wien and New York: Springer-Verlag), 223–276. 276. doi:10.1007/978-3-7091-2664-6_5

CrossRef Full Text | Google Scholar

Gorenflo, R., Kilbas, A. A., Mainardi, F., and Rogosin, S. V. (2014). Mittag-Leffler Functions, Related Topics and Applications. Berlin, Heidelberg: Springer.

Google Scholar

Grzelczyk, D., and Awrejcewicz, J. (2015). Wear Processes in a Mechanical Friction Clutch: Theoretical, Numerical, and Experimental Studies. Math. Probl. Eng. 2015, 1–25. doi:10.1155/2015/725685

CrossRef Full Text | Google Scholar

Hanneken, J. W., Vaught, D. M., and Achar, B. N. N. (2007). “Enumeration of the Real Zeros of the Mittag-Leffler Function E_α(z), 1 < α < 2,” in Advances in Fractional Calculus. Editors J. Sabatier, O. P. Agrawal, and J. A. Tenreiro Machado (Dordrecht: Springer), 15–26. doi:10.1007/978-1-4020-6042-7_2

CrossRef Full Text | Google Scholar

He, J.-H. (2011). A New Fractal Derivation. Therm. Sci. 15, 145–147. doi:10.2298/tsci11s1145h

CrossRef Full Text | Google Scholar

Holm, R. (1946). Electrical Contacts. Stockholm: H. Gerbers.

Google Scholar

Khonsari, M. M., Ghatrehsamani, S., and Akbarzadeh, S. (2021). On the Running-In Nature of Metallic Tribo-Components: A Review. Wear 474-475, 203871. doi:10.1016/j.wear.2021.203871

CrossRef Full Text | Google Scholar

Khrushchov, M. M., and Babichev, M. A. (1941). “Wear Test of Pure Metals and Antifriction Alloys during Friction on an Abrasive Surface [in Russian],” in Friction and Wear in Machines (Moscow–Leningrad: Publishing House of the Academy of Sciences of the USSR), 1, 89–98.

Google Scholar

Komogortsev, V. F. (1985). Contact between a Moving Stamp and an Elastic Half-Plane when There Is Wear. J. Appl. Maths. Mech. 49, 243–246. doi:10.1016/0021-8928(85)90110-8

CrossRef Full Text | Google Scholar

Kovalenko, E. V. (1985). Study of the Axisymmetric Contact Problem of the Wear of a Pair Consisting of an Annular Stamp and a Rough Half-Space. J. Appl. Maths. Mech. 49, 641–647. doi:10.1016/0021-8928(85)90085-1

CrossRef Full Text | Google Scholar

Kragelsky, I. V. (1965). Friction And Wear. London: Butterworths.

Google Scholar

Li, W., Zhang, L., Chen, X., Wu, C., Cui, Z., and Niu, C. (2020). Fuzzy Modelling of Surface Scratching in Contact Sliding. IOP Conf. Ser. Mater. Sci. Eng. 967, 012022. IOP Publishing. doi:10.1088/1757-899x/967/1/012022

CrossRef Full Text | Google Scholar

Ling, F. F. (1990). Fractals, Engineering Surfaces and Tribology. Wear 136, 141–156. doi:10.1016/0043-1648(90)90077-n

CrossRef Full Text | Google Scholar

Liu, Y., Hoon Jang, Y., and Barber, J. R. (2014). Finite Element Implementation of an Eigenfunction Solution for the Contact Pressure Variation Due to Wear. Wear 309, 134–138. doi:10.1016/j.wear.2013.11.004

CrossRef Full Text | Google Scholar

Mainardi, F. (1996). Fractional Relaxation-Oscillation and Fractional Diffusion-Wave Phenomena. Chaos, Solitons & Fractals 7, 1461–1477. doi:10.1016/0960-0779(95)00125-5

CrossRef Full Text | Google Scholar

Mainardi, F. (2020). Why the Mittag-Leffler Function Can Be Considered the Queen Function of the Fractional Calculus? Entropy 22, 1359. doi:10.3390/e22121359

PubMed Abstract | CrossRef Full Text | Google Scholar

Meng, H. C., and Ludema, K. C. (1995). Wear Models and Predictive Equations: Their Form and Content. Wear 181-183, 443–457. doi:10.1016/0043-1648(95)90158-2

CrossRef Full Text | Google Scholar

Nguyen, V. H., Zheng, D., Schmerwitz, F., and Wriggers, P. (2018). An Advanced Abrasion Model for Tire Wear. Wear 396-397, 75–85. doi:10.1016/j.wear.2017.11.009

CrossRef Full Text | Google Scholar

Ortigueira, M. D., and Tenreiro Machado, J. A. (2015). What Is a Fractional Derivative? J. Comput. Phys. 293, 4–13. doi:10.1016/j.jcp.2014.07.019

CrossRef Full Text | Google Scholar

Páczelt, I., and Mróz, Z. (2007). Optimal Shapes of Contact Interfaces Due to Sliding Wear in the Steady Relative Motion. Int. J. Sol. Structures 44, 895–925. doi:10.1016/j.ijsolstr.2006.05.027

CrossRef Full Text | Google Scholar

Popov, V. L., and Pohrt, R. (2018). Adhesive Wear and Particle Emission: Numerical Approach Based on Asperity-free Formulation of Rabinowicz Criterion. Friction 6, 260–273. doi:10.1007/s40544-018-0236-4

CrossRef Full Text | Google Scholar

Reye, T. (1860). Zur theorie der zapfenreibung. Der Civilingenieur 4, 235–255.

Google Scholar

Rhee, S. K. (1970). Wear Equation for Polymers Sliding against Metal Surfaces. Wear 16, 431–445. doi:10.1016/0043-1648(70)90170-5

CrossRef Full Text | Google Scholar

Sedakova, E. B., and Kozyrev, Y. P. (2021). Estimation of the Tribotechnical Efficiency of Polytetrafluoroethylene Filling. J. Mach. Manuf. Reliab. 50, 236–242. doi:10.3103/s1052618821030146

CrossRef Full Text | Google Scholar

Sedova, O., and Pronina, Y. (2022). The Thermoelasticity Problem for Pressure Vessels with Protective Coatings, Operating under Conditions of Mechanochemical Corrosion. Int. J. Eng. Sci. 170, 103589. doi:10.1016/j.ijengsci.2021.103589

CrossRef Full Text | Google Scholar

Sfantos, G. K., and Aliabadi, M. H. (2006). Wear Simulation Using an Incremental Sliding Boundary Element Method. Wear 260, 1119–1128. doi:10.1016/j.wear.2005.07.020

CrossRef Full Text | Google Scholar

Shillor, M., Sofonea, M., and Telega, J. J. (2004). Models and Analysis of Quasistatic Contact: Variational Methods. Berlin: Springer.

Google Scholar

Soldatenkov, I. A. (2010). Wear-contact Problem with Applications to Engineering Calculation of Wear. Moscow: Fizmatkniga. [in Russian].

Google Scholar

Su, X., Yao, D., and Xu, W. (2021). Processing of Viscoelastic Data via a Generalized Fractional Model. Int. J. Eng. Sci. 161, 103465. doi:10.1016/j.ijengsci.2021.103465

CrossRef Full Text | Google Scholar

Viswanath, N., and Bellow, D. G. (1995). Development of an Equation for the Wear of Polymers. Wear 181-183, 42–49. doi:10.1016/0043-1648(94)07055-5

CrossRef Full Text | Google Scholar

Wright, N. A., and Kukureka, S. N. (2001). Wear Testing and Measurement Techniques for Polymer Composite Gears. Wear 251, 1567–1578. doi:10.1016/s0043-1648(01)00793-1

CrossRef Full Text | Google Scholar

Yevtushenko, A. A., and Pyr'yev, Y. A. (1999). The Applicability of a Hereditary Model of Wear with an Exponential Kernel in the One-Dimensional Contact Problem Taking Frictional Heat Generation into Account. J. Appl. Maths. Mech. 63, 795–801. doi:10.1016/s0021-8928(99)00100-8

CrossRef Full Text | Google Scholar

Zmitrowicz, A. (2006). Wear Patterns and Laws of Wear–A Review. J. Theor. Appl. Mech. 44, 219–253.

Google Scholar