- 1Department of Educational Psychology and Guidance, Faculty of Education, Mahasarakham University, Muang, Mahasarakham, Thailand
- 2Department of Educational Research and Development, Faculty of Education, Mahasarakham University, Muang, Mahasarakham, Thailand
Introduction: Mathematics education development is an urgent topic, with an emphasis on increasing students’ motivation to learn mathematics.
Methods: This study aimed to investigate the impacts of teachers’ mathematical mindset, task values, and instructional practices on students’ mathematical mindset, using students’ perceived instructional practices as a mediator. The participants consisted of 96 teachers and 936 secondary school students. The research instruments included a mathematical mindset scale for teachers and students, a task value scale for teachers, an instructional practice scale for teachers, and a perceived instructional practice scale for students. The statistics for analyzing data included mean, standard deviation, and Structural Equation Modeling (SEM).
Results: The finding revealed that, from most to least, the variables that mutually influenced students’ mathematical growth mindset were students’ perceived instructional practices, teachers’ instructional practices, teachers’ values, and teachers’ mathematical growth mindset.
Discussion: The study’s findings can serve as a framework for developing teachers, who are essential to mathematics education and enhancing secondary school students’ motivation for mathematical learning.
1 Introduction
Mathematics education plays a crucial role in national development as it encourages advancements in technology, creativity, and innovation. Mathematics enables individuals to develop precise and comprehensive analyses of problems or situations, assists in planning, organizing, decision-making, and problem-solving with accuracy and appropriateness, and serves as a foundational tool for the study of science, technology, and other disciplines (Office of the Basic Education Commission, 2017, p.1). There is no doubt that mathematics education is vital in preparing children with the qualities, abilities, and proficiencies needed to construct a powerful nation.
Despite mathematics being acknowledged as crucial, it is often viewed as a difficult subject by many people. Particularly learners who have encountered negative experiences with mathematics. They tend to cultivate negative feelings or beliefs against the subject. This results in a math anxiety, regarded as an emotional response to a mathematical task including stress, concern, or fear (Ashcraft, 2002). Learners with a high level of math anxiety tend to avoid completing the mathematical tasks, which hinders the development and enhancement of their mathematics ability (Saefudin et al., 2023). This, in turn, impacts their academic achievement (Boaler, 2022; Dong et al., 2023). Thus, the cultivation of mathematics ability involves not only intellectual factors but also affective factors, including feelings and beliefs.
In recent years, many researchers have paid close attention to individuals’ motivational beliefs about intellectual abilities, talents, and personality, commonly known as mindset (Dweck, 2006). Mindset can be classified into two types: fixed mindset, which is a belief that intellectual capabilities are rigid and unchangeable; and growth mindset, which is a belief that intellectual abilities can be enhanced and refined by efforts and effective strategies (Yeager and Dweck, 2020). Individuals who have different mindsets exhibit distinct responses to difficulties. According to Dweck (2006), individuals with a fixed mindset tend to avoid difficult tasks, give up quickly in the face of obstacles, and underestimate the importance of making an effort, whereas individuals with a growth mindset focus on developing their abilities, perceive difficulties and setbacks as chances for personal growth, consistently work hard, and use efficient strategies to further their self-development. In the context of the classroom, students with a fixed mindset frequently reject criticism and use inappropriate strategies to uphold a positive self-image and protect their self-worth, while students with a growth mindset are more likely to embrace criticism as a chance for self-development and get inspiration from the accomplishments of others (Dweck, 2010). Hence, cultivating a growth mindset in students is essential for attaining learning goals.
Several studies have primarily concentrated on developing mindsets about life in general, with a few studies addressing mindsets in specific domains, particularly in mathematics. Students with a growth mindset about their life may hold either a growth or a fixed mindset in mathematical achievement, known as mathematical mindsets (Boaler, 2022). In particular, students who have a high level of math anxiety tend to hold a fixed mathematical mindset, in which individuals believe their mathematical qualities are unable to improve (Saefudin et al., 2023). A fixed mathematical mindset is negatively associated with mathematics success (Kaya and Karakoc, 2022), as individuals may engage in avoidance behaviors, experience a decline in confidence, and ultimately attain a lower level of proficiency in mathematics (Boaler, 2022; Boaler et al., 2023; Saefudin et al., 2023). To enhance mathematics education, it is crucial to focus on the formation of a mathematical mindset rather than a general life mindset.
It is evident that students’ mindset beliefs in mathematics can be shaped by teachers in classroom settings (Boaler, 2013; Anderson et al., 2018; Britwum et al., 2024). Teachers have a crucial effect on the learning process, the results of learning, and the overall learning quality (Butler and Shibaz, 2008), as well as students’ thoughts, beliefs, and motivation (Schiefele and Schaffner, 2015; Scales et al., 2020; Khalilzadeh and Khodi, 2021; Mesler et al., 2021). In order to change students’ beliefs regarding mathematics ability, teachers must adapt the learning environment by employing instructional practices that facilitate the formation of a growth mindset. This involves more than simply rewarding effort (Boaler et al., 2021), but teachers must motivate their students to perceive “mathematics as an open, growth, learning subject and themselves as powerful agents in the learning process” (Boaler, 2022, p. 129). To be exact, the primary goal of mathematics instruction is to instill students’ self-belief and to highlight the value of personal growth.
The achievement goals set by teachers for their students have a considerable influence on their instructional practices. According to achievement goal theory, instructional practices can be classified into two types: mastery-oriented instructional practice, where the teacher’s goal is to develop students’ competence; and performance-oriented instructional practice, where the teacher’s goal is to emphasize students’ academic success (Midgley et al., 2000). Different instructional practices have a significant influence on students’ beliefs and motivation. For instance, Park et al. (2016) found that students formed the belief that intelligence and academic abilities were unchangeable when teachers focused more on performance-oriented instructional practice. The students’ fixed belief subsequently impacted their mathematics achievement. Schiefele (2017) discovered that the use of mastery-oriented instructional practice was associated with the motivation of students for the subject and their establishment of goals for self-improvement in their learning. Although the impact of instructional practices on students’ beliefs is increasingly recognized, the ways in which these practices in mathematics classrooms shape students’ mathematical mindsets are still not thoroughly explored. Therefore, it remains essential to investigate how the instructional practices utilized by teachers in mathematics classrooms impact students’ mathematical mindsets.
In accordance with the theory of action, the classroom practices by which teachers convey certain messages to their students are associated with their beliefs (Sun, 2019). This leads to a significant question regarding the factors associated with the different instructional practices employed by teachers, which subsequently influence students’ mindsets about mathematics. In order to respond to this inquiry, we examine the conceptual basis of teachers’ instructional practices, specifically achievement goal structure, which is strongly associated with self-theories that explain mindset (Dweck and Yeager, 2019) and expectancy-value theory, in which task values play a crucial role (Eccles, 2005). It is theoretically posited that teachers’ instructional practices are associated with their mindsets and values, as they employ both implicit and explicit beliefs in intricate classroom settings (Fives and Gill, 2015). Research indicates that teachers’ beliefs about students’ ability impact their teaching strategies (Swann et al., 1980). Teachers with a fixed mindset often establish performance-oriented goals in their classrooms (Lüftenegger and Muth, 2024), whereas those with a growth mindset emphasize mastery-oriented goals in their teaching (Bardach et al., 2024).
In addition to mindset, value in mathematics learning and teaching is another critical aspect that cannot be overlooked. Value refers to the importance placed on certain things and is intricately linked to emotions, desires, and judgments, which together form a continuum (Tiberius, 2018). This concept influences people’s motivation to do or not do certain things. According to the expectancy-value theory, task values can be categorized into four dimensions: attainment value, which emphasizes the importance of completing a task; intrinsic value, which focuses on the enjoyment or interest experienced during task engagement; instrumental or utility value, which involves the relevance of applying knowledge or task completion to future goals; and cost value, which represents the willingness to sacrifice alternative values (Wigfield et al., 2012; Eccles and Wigfield, 2002). Given the influential roles of both mindset and values, these factors are instrumental in shaping teachers’ behaviors within mathematics classrooms. For instance, Piyakun’s (2020) study demonstrated that the interplay between mathematics teachers’ fixed and growth mindsets regarding intelligence, along with task values, specifically cost, intrinsic, and attainment values, significantly predicted performance-oriented instructional practices. In addition, the teachers’ growth mindset and task values, primarily cost and attainment values, were found as predictors of mastery-oriented instructional practices. However, this study continued to emphasize beliefs about intelligence rather than ability in mathematics.
Given the insufficient comprehension of the relationships among teachers’ mathematical mindsets, task values, and instructional practices, it is imperative to examine these variables and their impact on students’ beliefs regarding their mathematics ability. Previous research suggests that teachers’ motivational beliefs and actions in the classroom are not directly associated with students’ mindsets (Haimovitz and Dweck, 2017), but are indirectly related to students’ motivation through students’ perceptions of teachers’ beliefs as a mediator (Gutshall, 2016). Ames (1992) highlights the importance of student perception, noting that students may interpret and perceive teachers’ messages, such as praise, differently based on the differential treatment stemming from teachers’ expectations. This is consistent with motivational climate theory, which elucidates how students’ perceptions of their teachers’ beliefs affect their motivation and academic performance (Kim et al., 2024). The theory identifies three categories of motivational classroom processes: motivational supports, encompassing the behaviors of teachers and students, including speech, actions, and structures within the learning environment and that form the quality, meaning, and extent of individual motivational beliefs; motivational climate, pertaining to students’ shared perceptions of contextual qualities at the group level (classroom or school); and motivational microclimates, referring to students’ individual or subgroup-specific perceptions of contextual qualities (Robinson, 2023). The perception and interpretation of motivational supports by individual students and groups influence their motivational beliefs within the learning context (Robinson, 2023; Kim et al., 2024). For this reason, the investigation of teacher-student interaction must consider how students perceive and interpret their teachers’ behaviors as a mediating factor.
This current study seeks to address the lack of understanding regarding the aspects of teachers and students in mathematics classrooms. The study focuses on the following questions:
(1) How do teachers perceive their mathematical mindset, task values, and instructional practices in the mathematics classrooms?
(2) What mathematical mindset do students possess, and how do they perceive their teacher’s instructional practices in the mathematics classroom?
(3) How are teachers’ mathematical mindset, values on mathematical tasks, and instructional practices related to their students’ mathematical mindset and perceived instructional practices?
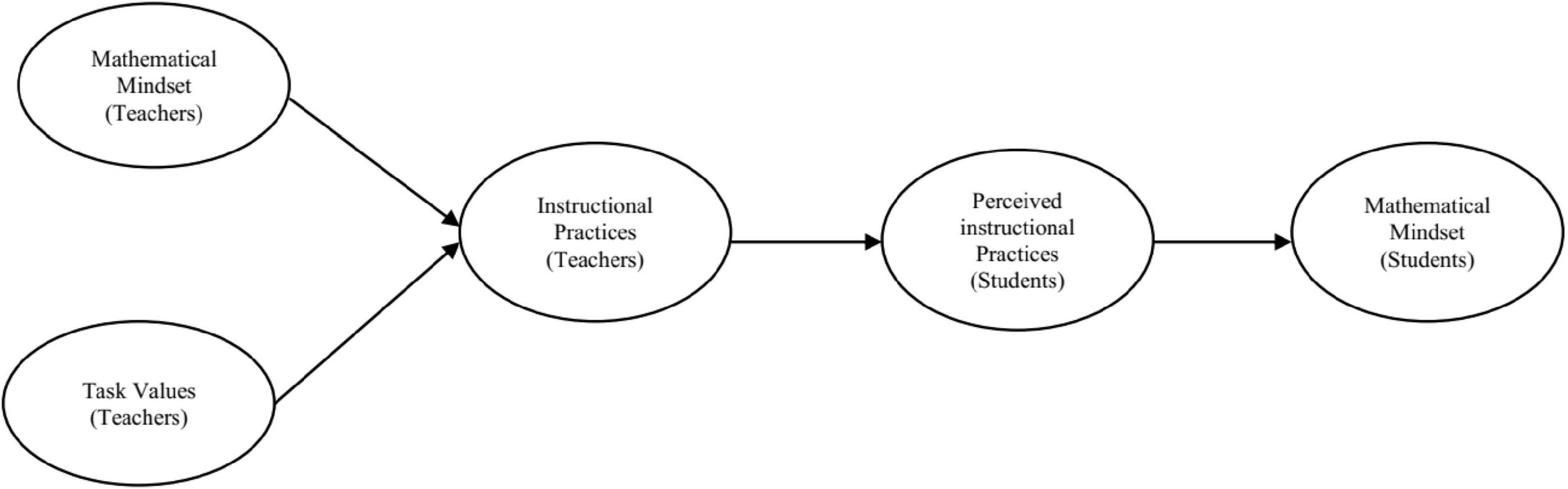
As shown in Figure 1, we aim to examine a latent structural equation model proposing that students’ mathematical mindset is influenced by teachers’ motivational factors (mathematical mindset and task values) and instructional practices, with the students’ perception of instructional practices serving as a mediator. The results of this study will offer crucial insights to improve comprehension and facilitate the strategic creation of methods for enhancing the motivational beliefs of mathematics teachers and students in mathematics classrooms.
2 Materials and methods
2.1 Participants
The participants comprised secondary school teachers and their students. A total of 96 teachers took part in the study, with 66 of them identifying as female, 28 as male, and 2 choosing not to indicate their gender. Among the total, there were 51 teachers working at lower-secondary schools and 45 teachers working at upper-secondary schools. These schools were located in five provinces in the northeastern region of Thailand. In terms of their educational qualifications, 57 teachers earned a bachelor’s degree, 37 had a master’s degree, and 2 possessed a doctoral degree. Out of the total, 88.54% of teachers held a degree in mathematics education.
The students who participated in the study were selected using a random sampling technique. Ten students were randomly selected from the classes of each participating teacher. After obtaining parental consent, the researchers distributed the survey to the students. Upon the exclusion of missing and incomplete responses, the total number of student responses amounted to 936. A total of 489 students were from lower secondary schools, comprising 299 females, 172 males, and 18 who opted not to disclose their gender. In addition, 447 students were from upper secondary schools, including 277 females, 151 males, and 19 who chose not to disclose their gender. Finally, the ratio of students to teachers varied between 8 and 10 students per teacher.
2.2 Instruments
2.2.1 Student mathematical mindset
The mathematical mindset scale for students was developed in accordance with Dweck’s (1999) implicit theories of intelligence. The scale consisted of six items in a six-point Likert format (1 = strongly disagree, 2 = disagree, 3 = somewhat disagree, 4 = somewhat agree, 5 = agree, 6 = strongly agree). To ensure that the research concentrated on a domain-specific mindset, the scale encompassed components concerning aspects of effort, failure, and strategies for learning mathematics. The statements provided as examples were as follows: “I believe that my talent for mathematics can be changed through hardworking and effort”, “Failure experiences in mathematics can provide me an opportunity to enhance my mathematical skills”, and “I can enhance my mathematical abilities by employing effective learning strategies”. These statements reflected a growth mindset in mathematics. All items were positively coded. A high score on the scale signified that students had a growth mindset in mathematics, whereas a low score implied a fixed mindset. Cronbach’s alpha reliability coefficient was computed as α = 0.849 for mathematical mindset.
2.2.2 Teacher mathematical mindset
The mathematical mindset scale for teachers was designed using a comparable approach to the student mathematical mindset scale. A six-point Likert scale with six items examined the teacher’s beliefs about mathematical ability, which included strategies for learning mathematics, failure, and effort. A few instances were provided: “I believe that a competence in mathematics can be developed by consistent practice and effort,” “I believe that failures experienced during learning mathematics can serve as an opportunity for improvement in this subject,” and “I am certain that the application of effective learning strategies can contribute to the improvement of one’s mathematical abilities.” All items were positively coded. A high score on the scale indicated that teachers possessed a growth mindset in mathematics, while a low score suggested a fixed mindset. The reliability coefficient for teachers’ mathematical mindset was calculated as α = 0.923 using Cronbach’s alpha.
2.2.3 Teachers’ task values
The task values scale for teachers was created based on the expectancy-value model of achievement (Eccles and Wigfield, 2002). The scale comprised 16 items rated on a five-point Likert scale, ranging from “not important at all” to “most important”, in order to elicit the participants’ opinions on the importance or value of learning mathematics. The task values were categorized into four dimensions: attainment value, intrinsic value, utility value, and cost value. Four items were used to evaluate the attainment value (e.g., I think that success in mathematics is important for my students), with the Conbach’s alpha value of .815. Four items were assessed for intrinsic value (e.g., I think that learning mathematics with enjoyment is important for my students), with the Conbach’s alpha value of .919. Four items were used to assess the utility value (e.g., I think that applying mathematical knowledge and skills in daily life is significant for my students), with the Conbach’s alpha values of .910. Four items were evaluated for the cost value (e.g., I think that, despite its difficulty, paying attention in mathematics class is important for my students), with the Conbach’s alpha value of 0.876.
2.2.4 Teachers’ instructional practices
The instructional practices scale for teachers was adapted from the Patterns of Adaptive Learning Scales (PALS) by Midgley et al. (2000), which categorized instructional practices based on goal orientation theory into two types: mastery- and performance-oriented instructional practices. The researcher adjusted the scale’s statements to be fitted specifically for mathematics classrooms. This scale employed a five-point Likert scale (ranging from never to always) with a total of 10 items. These items were divided into two categories: 5 items assessed mastery-oriented teaching behaviors (e.g., I facilitate my students to learn independently and stimulate their math competency.), while the remaining 5 items evaluated performance-oriented teaching behaviors (e.g., I ask my students to evaluate their math abilities in order to compare with their peers). The instructional practices scale had a reliability of .798 for mastery-oriented teaching and .724 for performance-oriented teaching.
2.2.5 Students’ perceived instructional practices
The perceived instructional practices scale for students was adapted from the Patterns of Adaptive Learning Scales (PALS) by Midgley et al. (2000), similar to the instructional practices scale for teachers. The researcher modified the questions to reflect students’ opinions on their teachers’ teaching behaviors in mathematics classrooms. This scale used a five-point Likert scale (never / rarely / sometimes / often / always) with 10 items, consisting of 5 items measuring students’ perceptions of mastery-oriented teaching behaviors (e.g., My math teacher facilitates me to learn independently and stimulates my math competency) and 5 items measuring perceptions of performance-oriented teaching behaviors (e.g., My teacher asks me to assess my math abilities in order to compare with my classmates). The perceived instructional practices scale had a reliability of 0.809 for perceived mastery-oriented teaching and 0.756 for perceived performance-oriented teaching.
2.3 Data collection and data analysis
The researchers acquired ethical approval for human study from the study Ethics Committee of Mahasarakham University prior to data collection. The permission was granted with the certification number 244-257/2023. Upon obtaining ethical approval, the researchers continued with the process of participant recruitment. The participants for this study, including mathematics teachers and their students, were selected using a multi-stage random sampling method.
In the initial phase, five provinces in the northeast region of Thailand were randomly selected using simple random sampling. During the second stage, stratified random sampling was employed to select four schools from each province. These schools were separated into four strata based on their size: small, medium, large, and very large. In the third phase, mathematics teachers from each school were selected at random, ensuring the inclusion of both lower- and upper-secondary school teachers. Subsequently, the researchers reached out to the chosen schools to ask for data collection. Upon obtaining consent from the teachers to participate in the study, the researchers randomly selected 10 students from the classes taught by these teachers. After receiving parental consent, the researchers collected data from the selected students.
The researchers distributed the mathematical mindset, task value, and instructional practices scales to 120 teachers and the mathematical mindset and perceived instructional practices scales to 1,200 students. Some of the responses were found to be missing and incomplete by the participants. As a result, a total of 96 complete responses from teachers and 936 from students were analyzed.
The data were analyzed using descriptive statistics, including mean and standard deviation, to demonstrate the mathematical mindset, task values, and instructional practices of the teachers, as well as the mathematical mindset and perceived instructional practices of the students. The study utilized Structural Equation Modeling (SEM) to analyze the effects of the teachers’ mathematical mindset, task values, and instructional practices on the students’ mathematical mindset, using the students’ perceived instructional practices as a mediator.
3 Results
3.1 Descriptive statistics
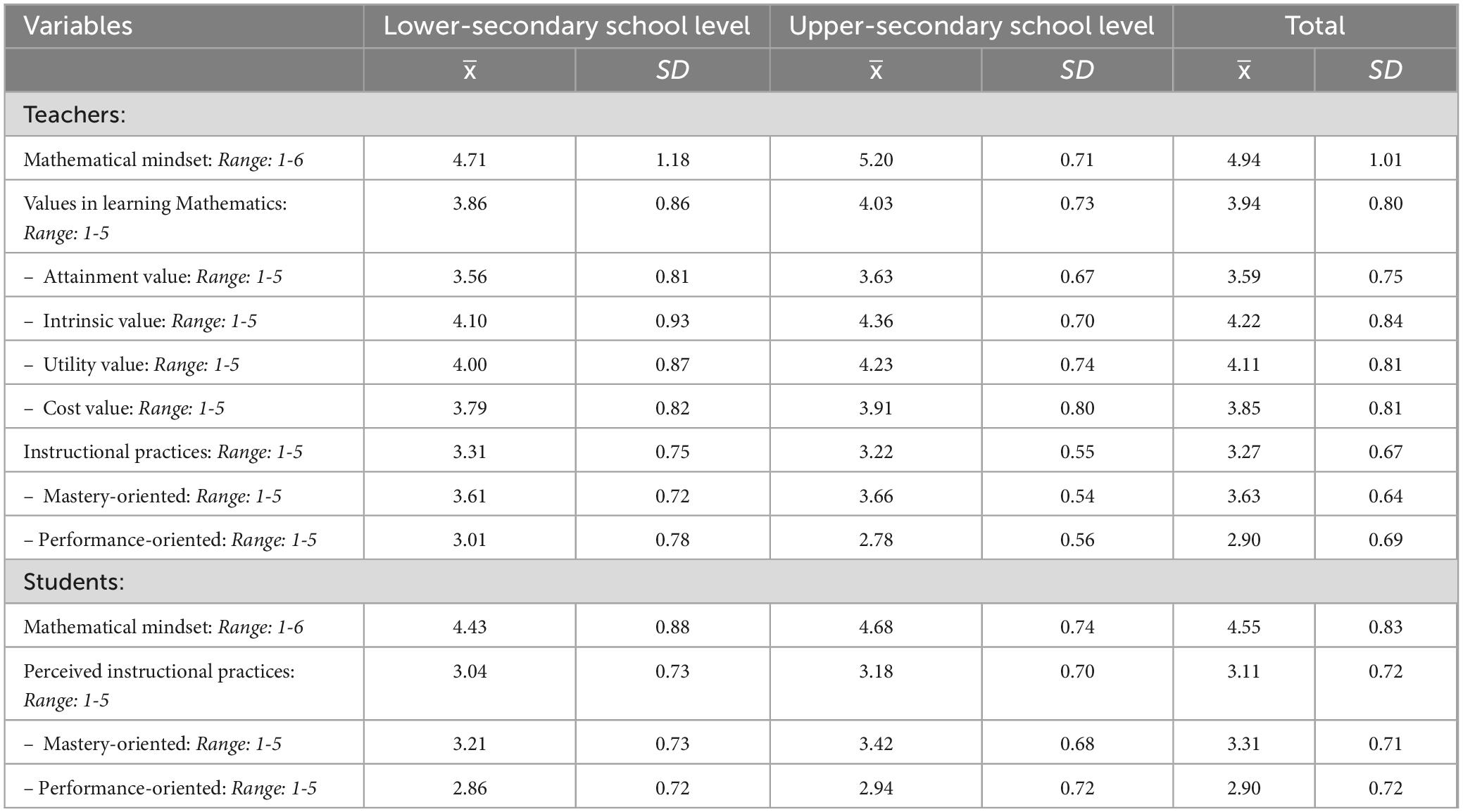
The descriptive data is presented in Table 1 to illustrate the mathematical mindset, the values of learning, and instructional practices of the teachers, as well as the mathematical mindset and the perception of instructional practices of students in the mathematics classroom. The mathematics teachers had a significantly high average score of 4.94 (out of 6.00) indicating their growth mindset in mathematics. When classified by grade levels, teachers of lower-secondary schools had a mean score of 4.71, whereas teachers of upper-secondary schools attained a mean score of 5.20.
In terms of the values of mathematics learning, it was determined that the average score was high ( = 3.94). When classified based on grade level, mathematics teachers teaching upper-secondary school had a higher mean score compared to those teaching lower-secondary school ( = 4.03 and 3.86, respectively). Upon analyzing the different aspects of values, it was discovered that mathematics teachers highly prioritize intrinsic value ( = 4.22). Additionally, attainment, utility, and cost values are also valued highly ( = 3.59, 4.11, and 3.85, respectively).
The mean score for mastery-oriented instructional practice in mathematics classrooms was 3.63, which indicates a high level, whereas the mean score for performance-oriented instructional practice was 2.90, which indicates a moderately low level. Teachers in lower-secondary schools had a mean score of 3.61 for mastery-oriented instructional practice and 3.01 for performance-oriented instructional practice. Conversely, upper-secondary school teachers had a mean score of 3.66 for mastery-oriented instructional practice and 2.78 for performance-oriented instructional practice.
Upon assessing the descriptive data of the students, it was discovered that they exhibited a significantly high average score ( = 4.55) in terms of their growth mindset in mathematics. When analyzing the data based on grade levels, it was discovered that students in both lower and upper secondary school displayed high average scores for a growth mindset in mathematics ( = 4.43 and 4.68, respectively).
In terms of perceived instructional practices, the students had a stronger perception of master-oriented instructional practice, emphasizing deep knowledge, with an average score of 3.31 (out of 5.00), compared to performance-oriented instructional practice, which had an average score of 2.90. Students in lower-secondary schools had a mean score of 3.21 for perceived mastery-oriented instructional practice and 2.86 for perceived performance-oriented instructional practice. The upper-secondary school students indicated a mean score of 3.42 for perceived mastery-oriented instructional practice and 2.94 for perceived performance-oriented instructional practice.
3.2 Testing the hypothesized model
The hypothesized Structural Equation Modeling (SEM) was that students’ mathematical mindset was influenced by teachers’ mathematical mindset, task values, and instructional practices, with students’ perceptions of teachers’ instructional practices as a mediator. The results of SEM analysis are illustrated in Figure 2. The results showed that the hypothetical model was consistent with the empirical data, as detailed in other indices in Table 2.
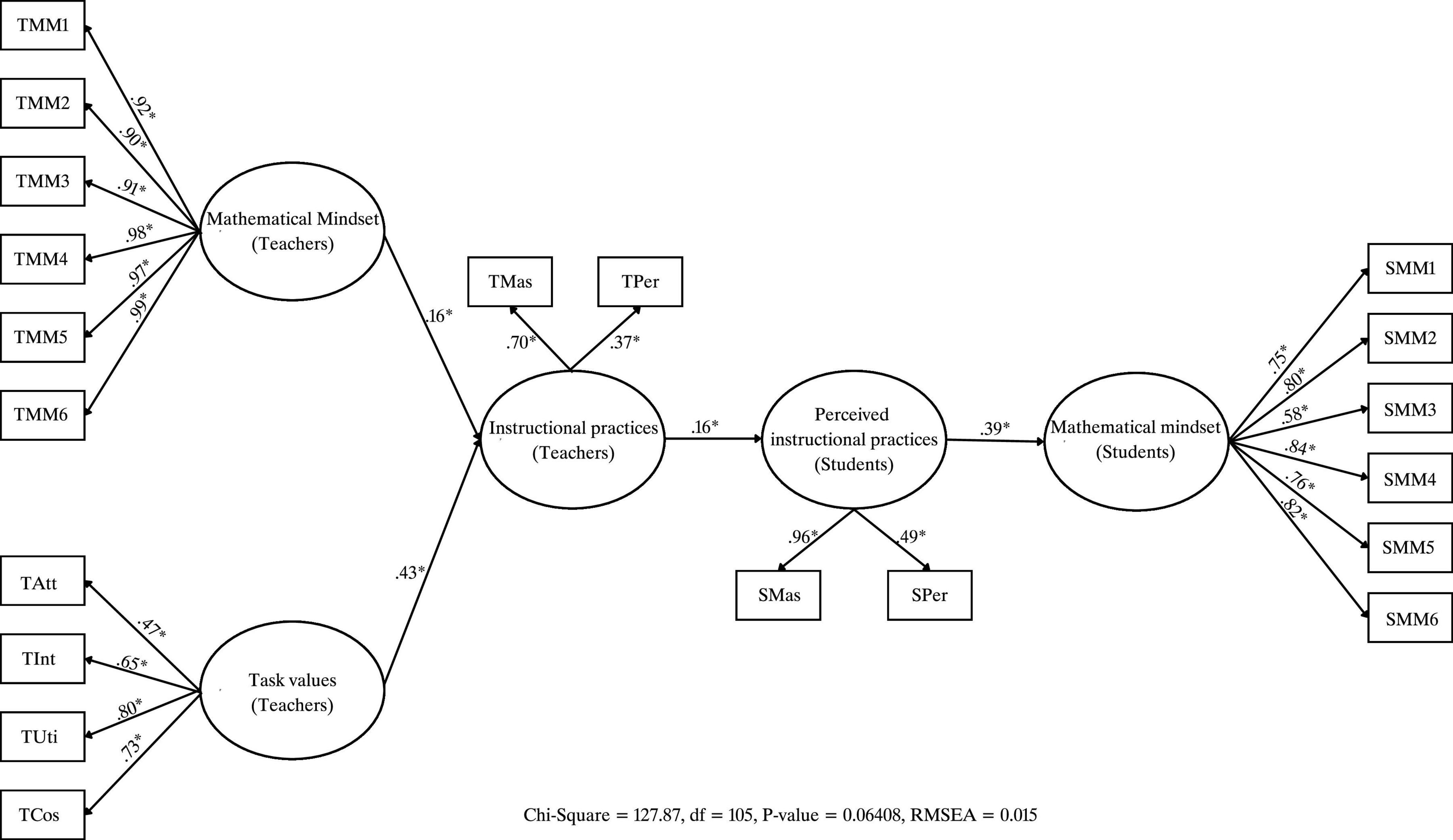
Figure 2. The tested model of relationships between teachers’ variables and students’ variables. TMM, teacher mathematical mindset; TAtt, teacher attainment value; TInt, teacher intrinsic value; TUit, teacher utility value; TCos, teacher cost value; TMas, teacher mastery-oriented; TPer, teacher performance-oriented; SMas, student mastery-oriented; SPer, student performance-oriented; SMM, student mathematical mindset. *p < 0.05.
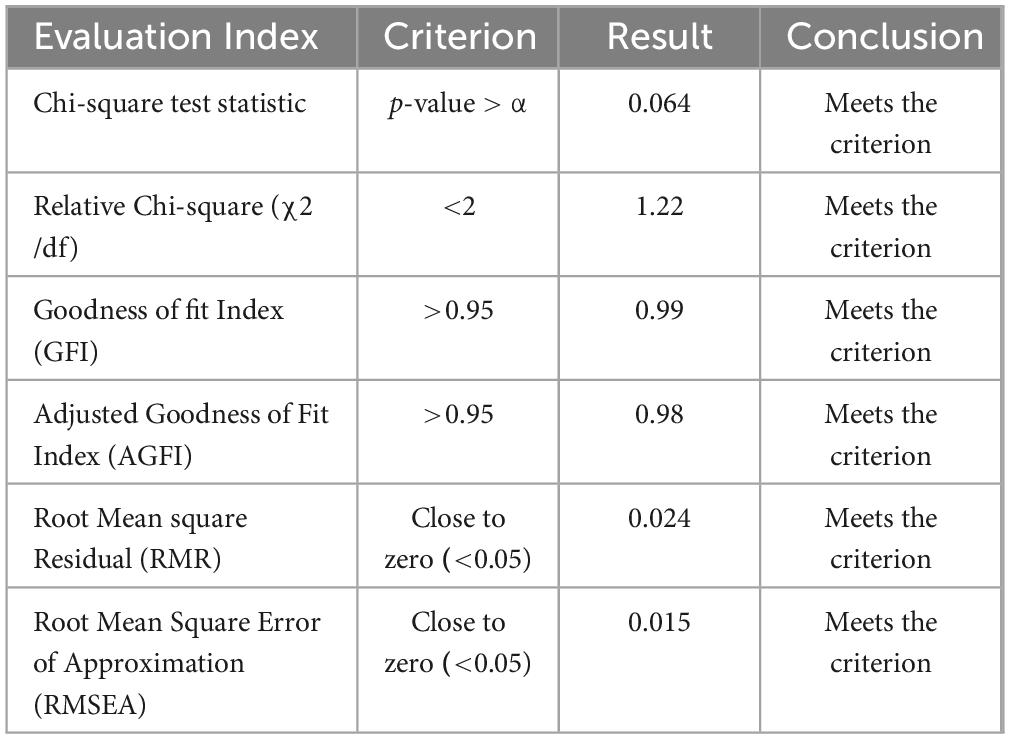
Table 2. Direct influence, indirect influence, and total influence affecting the mathematical mindset of students.
From Table 2, it was found that the indices used to evaluate the consistency of the hypothetical model with the empirical data, including Chi-square, Relative Chi-square (χ2/df), Goodness of Fit Index (GFI), Adjusted Goodness of Fit Index (AGFI), Root Mean Square Residual (RMR), and Root Mean Square Error of Approximation (RMSEA), all met the required criteria. Therefore, the researchers presented the influence values in Table 3. The table shows that the variables influencing students’ mathematical mindset, in order from most to least influence, are: students’ perception of instructional practices, teachers’ instructional practices, teachers’ values of learning mathematics, and teachers’ mathematical mindset.
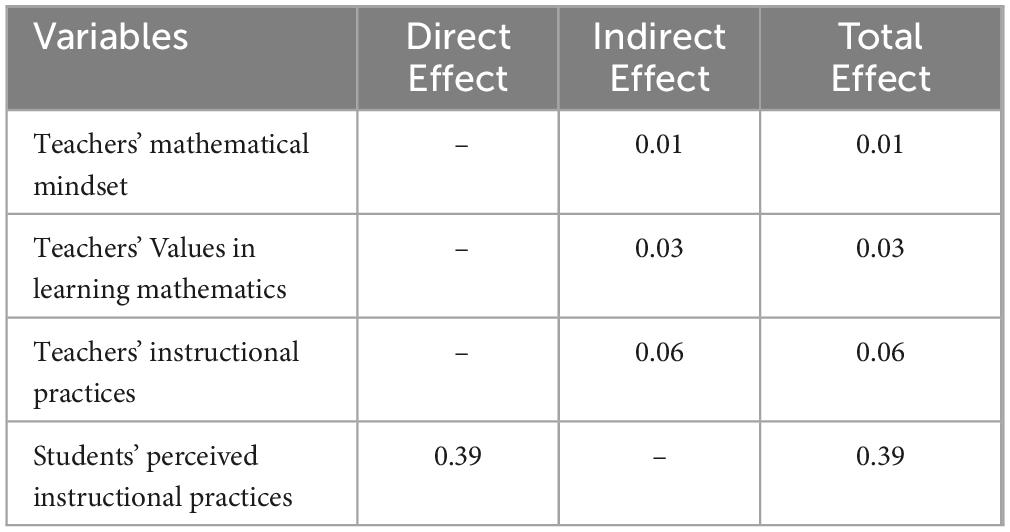
Table 3. The direct influence, indirect influence, and total influence affecting students’ mathematical mindset.
4 Discussion
Upon analyzing the data about teachers’ beliefs and instructional practices in mathematics classroom it was found that the teachers generally had a tendency toward a growth mindset in mathematics education, highly valued mathematics learning and teaching, and displayed more mastery-oriented instructional practice. In terms of a growth mathematical mindset, it suggests that the teachers hold the belief that mathematical abilities can be enhanced by putting in effort and employing effective learning strategies. They emphasize the importance of perseverance in handling difficult mathematical tasks in the process of learning mathematics and consider failure or mistakes as valuable chances to enhance the mathematical skills. The reason for this finding would be that mathematics teachers may possess personal experiences centered on self-improvement and the development of students, which have influenced their belief in the growth mindset concerning mathematical ability. In particular, a majority of teachers who participated in this study (82.29% of all participating teachers) completed their mathematics education programs. This suggests that these programs may have instilled a growth mindset in teachers through their training and ongoing professional development. As a result, these teachers likely possess a profound comprehension of the learning process, emphasizing the importance of making an effort, embracing challenges, and employing effective learning strategies.
In terms of the value of learning mathematics, the teachers made a high score, with intrinsic value at the highest level. It suggests that teachers value the learning of mathematics, particularly the importance of enjoyment in learning mathematics, which aligns with the concept of intrinsic motivation (Upadyaya and Eccles, 2014). This could refer to the fact that the majority of mathematics teachers who participated in the study obtained their degrees in mathematics education. This educational background may have cultivated a deep appreciation for mathematics, a favorable disposition toward learning the subject, and an inherent satisfaction and pleasure in the process of acquiring mathematical knowledge. Eccles (2005) argued that valuing something, such as mastering mathematics and pursuing a career in teaching, is a strong indicator of intrinsic drive and dedication to that job. The teacher professional development program trains teachers to take responsibility for nurturing students’ mathematical abilities and understanding, as well as recognizing the practical applications of mathematics in everyday life and future careers. Additionally, the program instills an awareness of the value of time management and prioritizing learning over other activities when it comes to successfully completing mathematical tasks.
Upon analyzing the instructional practices data, it was discovered that mathematics teachers scored highly on mastery-oriented instructional practice, while performance-oriented instructional practice scored at a moderate level. The teachers reported that they employed pedagogical strategies in mathematics aiming to foster individual learning and emphasize effort and personal growth over academic achievements and comparative performance. The reason would be that the present study gathered data exclusively from secondary school teachers who may encounter reduced pressure from policies that establish success objectives centered on exams. This enables teachers to concentrate on instructional management that aims to enhance students’ capabilities. In addition, the present educational strategy in Thailand is oriented toward enhancing the quality of education. This is achieved by motivating teachers to modify their teaching approaches and arrange activities that facilitate active learning, thus promoting meaningful acquisition of knowledge. The objective is to increase student participation in the classroom, foster teacher-student interactions, promote activities that generate student enthusiasm for learning, and facilitate a diverse range of learning experiences, thereby cultivating a sustained passion for acquiring knowledge (Office of the Basic Education Commission, 2019). This aligns with the aim of achieving learning goals or mastery-oriented instructional practices.
The analysis of the data regarding students’ mathematical mindset and perceived instructional practices revealed that students exhibited a growth mindset in mathematics and interpreted their mathematics classroom goals as centered on enhancing their mathematical competencies. This suggests that the students hold a belief that their mathematical skills can be enhanced through consistent effort, embracing new and challenging tasks, embracing failure, and recognizing opportunities for learning from setbacks. In relation to students’ perception of instructional practices within mathematics classrooms, it was observed that students recognized their teachers’ teaching goals as emphasizing the importance of effort in achieving proficiency in tasks and the ongoing enhancement of mathematical skills. The emphasis on individual growth is regarded as more significant than the comparison of academic performance with peers to demonstrate learning competence (Elliot and Hulleman, 2017). In addition, high school students possess cognitive maturity and are receptive to emotional and social factors that impact their learning (Towner et al., 2023). Consequently, they are more attuned to understanding teachers’ objectives for success through their actions and instructional approaches.
The study investigated the proposed SEM indicating that teachers’ mathematical mindset, task values, and instructional practices impacted students’ mathematical mindset, with students’ perceptions of teachers’ instructional practices serving as a mediator. The results showed that the proposed model was consistent with the empirical data, indicating a strong alignment between theory and observational data. This phenomenon can be explained by the theory of action, which posits that teachers’ teaching behaviors, involving their interactions with students and classroom management, are influenced by their beliefs. These behaviors, in turn, convey their belief in either a fixed or growth mindset to their students, thereby influencing the development of their students’ mindset, which ultimately affects their students’ academic achievement (Sun, 2019). Furthermore, the SEM results validated the role of students’ perceptions of their teachers’ goal-related instructional practices as a mediator in the interactions between teacher and student variables. This result aligns with motivational climate theory, which posits that teachers’ motivational supports - such as speech, actions, and structures in learning context - shape the quality, meanings, and quantity of students’ motivational beliefs through their perceptions of teachers’ behaviors (Robinson, 2023). Thus, the perceptions of students regarding the teachers’ motivational support serve as a significant mediator in the relationship between teachers’ and students’ motivation. The research by Gutshall (2016) showed that student mindset correlated with teacher mindset, with student perception of teacher mindset serving as a mediator. Consistent with the research of Jia et al. (2024), it was found that students’ perceived classroom goal structures strongly influenced the relationship between perceived teachers mindset and school engagement with students’ mindset as a mediator.
Previous research indicates that students’ mindset beliefs are influenced by teachers’ mindsets (Mesler et al., 2021) and their teaching practices (Yu et al., 2022). Students’ perceptions are often overlooked as a mediating factor influencing the relationship between teachers’ motivation and behaviors and students’ beliefs. Due to the complexity of the classroom environment, teachers may inadequately express or fail to communicate their motivational beliefs to students (Haimovitz and Dweck, 2017). Students in the same classroom may perceive and interpret their teachers’ motivational support differently based on their individual experiences and beliefs regarding academic performance (Robinson, 2023). The present study not only examined teachers’ motivation and goal-oriented instructional practices alongside students’ beliefs but also highlighted students’ perceptions of their teachers’ motivation and behaviors in mathematics classrooms to bridge the knowledge gap concerning the relationship between teachers’ and students’ motivation. The study’s conclusions can enhance the comprehension of the complexities of classroom environment processes, providing insights for the improvement of mathematics education. Particular focus should be directed into teacher mindsets and motivations, since these factors impact their goal-oriented instructional practices. Emphasis must be made on cultivating teachers’ growth mindset beliefs and their recognition of the value of mathematics education, while also promoting instructional approaches that prioritize the enhancement of students’ potentials rather than academic results. The students’ backgrounds which influence their perceptions and interpretations of teachers’ motivational support, must not be disregarded. Teachers must take into account the students’ beliefs and characteristics, which affect their interpretation of the messages communicated by their teachers. Thus, the instructional practices enable the students to cultivate a growth mindset for mathematics learning effectively.
5 Conclusion
In summary, the present study discovered that teachers’ mathematical mindset, values in learning mathematics, and instructional practices were able to predict students’ mindsets based on students’ perceived instructional practices. This has significant implications for improving the quality of mathematical education. In their studies, Boaler et al. (2021) and Boaler et al. (2023) identified significant barriers to effective communication between teachers’ and students’ mindsets. These challenges include teaching practices that frequently suggest that students can learn through perseverance and effort, while the mathematics teaching process continues to prioritize short, closed-ended questions. Consequently, students get a rigid conviction about the process of learning mathematics due to the fact that the questions solely offer correct or incorrect solutions, thereby restricting their chances to acquire knowledge or enhance their skills. The lack of clear communication of teachers’ perspectives to students highlights the significance of effectively conveying a mathematical mindset to aid students in cultivating a growth mindset. Teachers should facilitate lessons by assigning inclusive and challenging tasks or questions that are accessible to all students and can be accomplished at advanced levels. They should also offer diverse problem-solving approaches, encourage discussion, and make connections to students’ prior knowledge. This approach will enable students to recognize opportunities for personal growth and foster a growth mindset in mathematics. The evaluation system should be genuine, prioritizing mathematical cognition and logical analysis, and provide feedback to enable students to enhance their learning through self-reflection. When teachers effectively convey the concept of a growth mindset through their teaching practices, students are able to understand and adopt a growth mindset. This aligns with studies that indicate students’ perceptions of how teachers manage their classes have an impact on their growth mindset in mathematics learning. When examining students’ perceptions of teaching practices, it was discovered that students believe that teachers employ instructional techniques and demonstrate behaviors in mathematics classes that prioritize knowledge acquisition. This aligns with the findings of Yu et al. (2022), who found that students are more inclined toward a growth mindset when teachers utilize inquiry-based learning and the school fosters an atmosphere that supports students’ emotional and social growth. The research conducted by Britwum et al. (2024) showed that instructional practices that prioritize student-centered learning had a positive impact on students’ growth mindset. These teaching practices, which involve inquiry-based and student-centered approaches, are in line with knowledge-focused learning. This approach prioritizes the development of students’ abilities by comparing them against their own criteria, engaging with teachers and peers, and utilizing various learning methods that are suitable for each student. Additionally, it grants students the autonomy to determine their own problem-solving strategies.
Regarding the limitations of this study, it is important to note that the current study was only conducted in the northeast region of Thailand. Consequently, the data collected may have limitations in terms of its applicability due to the unique population studied, which may exhibit variations in cultural background. Hence, further investigation could broaden the demographic range to encompass the entirety of Thailand, thereby acquiring more extensive and balanced data and then formulating policy suggestions for the nation.
Data availability statement
The original contributions presented in this study are included in this article/supplementary material, further inquiries can be directed to the corresponding author.
Ethics statement
The studies involving humans were approved by Ethics Committee of Mahasarakham University. The studies were conducted in accordance with the local legislation and institutional requirements. Written informed consent for participation in this study was provided by the participants’ legal guardians/next of kin.
Author contributions
AP: Conceptualization, Funding acquisition, Investigation, Project administration, Writing – original draft, Writing – review and editing. SP-O: Formal analysis, Investigation, Methodology, Writing – original draft.
Funding
The author(s) declare that financial support was received for the research, authorship, and/or publication of this article. This research project was financially supported by the Thailand Science Research and Innovation (TSRI).
Acknowledgments
In this study, a generative artificial intelligence technology called ChatGPT and QuillBot were used only to assist in sentence structure.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Ames, C. (1992). Classrooms: Goals, structures, and student motivation. J. Educ. Psychol. 84, 261–270. doi: 10.1037/0022-0663.84.3.261
Anderson, R. K., Boaler, J., and Dieckmann, J. (2018). Achieving elusive teacher change through challenging myths about learning: A blended approach. Educ. Sci. 8:98. doi: 10.3390/educsci8030098
Ashcraft, M. H. (2002). Math anxiety: Personal, educational, and cognitive consequences. Curr. Dir. Psychol. Sci. 11, 181–185.
Bardach, L., Bostwick, K. C. P., Fütterer, T., Kopatz, M., Hobbi, D. M., Klassen, R. M., et al. (2024). A meta-analysis on teachers’ growth mindset. Educ. Psychol. Rev. 36:84. doi: 10.1007/s10648-024-09925-7
Boaler, J. (2013). Ability and mathematics: The mindset revolution that is reshaping education. Forum 55, 143–152.
Boaler, J., Dieckmann, J. A., LaMar, T., Leshin, M., Selbach-Allen, M., and Pérez-Núñez, G. (2021). The transformative impact of a mathematical mindset experience taught at scale. Front. Educ. 6:784393. doi: 10.3389/feduc.2021.784393
Boaler, J., Dieckmann, J., and Loos, R. (2023). Changing mathematical beliefs and achievement: The synergies of mindset ideas and effective teaching. Quadrante 32, 195–208. doi: 10.48489/quadrante.32534
Britwum, B. A., Ntow, F. D., and Smith, J. A. (2024). Influence of teaching approaches on senior high school students’ mathematical mindset. SAGE Open 14, 1–10. doi: 10.1177/21582440241237060
Butler, R., and Shibaz, L. (2008). Achievement goals for teaching as predictors of students’ perceptions of instructional practices and students’ help seeking and cheating. Learn. Instr. 18, 453–467. doi: 10.1016/j.learninstruc.2008.06.004
Dong, L., Jia, X., and Fei, Y. (2023). How growth mindset influences mathematics achievements: A study of Chinese middle school students. Front. Psychol. 14:1148754. doi: 10.3389/fpsyg.2023.1148754
Dweck, C. S. (1999). Self-Theories: Their Role in Motivation, Personality, and Development. New York: Taylor & Francis Group.
Dweck, C. S. (2006). Mindset: The New Psychology of Success. New York: Random House Publishing Group.
Dweck, C. S., and Yeager, D. S. (2019). Mindsets: A view from two eras. Perspect. Psychol. Sci. 14, 481–496. doi: 10.1177/1745691618804166
Eccles, J. S. (2005). “Subjective task value and the Eccles et al. model of achievement-related choices,” in Handbook of Competence and Motivation, eds A. J. Elliot and C. S. Dweck (New York: The Guilford Press), 105–121.
Eccles, J. S., and Wigfield, A. (2002). Motivational beliefs, values, and goals. Annu. Rev. Psychol. 53, 109–132.
Elliot, A. J., and Hulleman, C. S. (2017). “Achievement goals,” in Handbook of Competence and Motivation: Theory and Application, eds A. J. Elliot, C. S. Dweck, and D. S. Yeager (New York: The Guilford Press), 43–60.
Fives, H., and Gill, M. G. (2015). “Introduction,” in International Handbook of Research on Teachers’ Beliefs, eds H. Fives and M. G. Gill (New York: Routledge), 1–10.
Gutshall, C. A. (2016). Student perception of teachers’ mindset beliefs in the classroom setting. J. Educ. Developmental Psychol. 6, 135–142. doi: 10.5539/jedp.v6n2p135
Haimovitz, K., and Dweck, C. S. (2017). The origins of children’s growth and fixed mindsets: new research and a new proposal. Child Dev. 88, 1849–1859. doi: 10.1111/cdev.12955
Jia, X., Li, P., Zhao, J., and Zhang, Y. (2024). How adolescents’ perceived teachers’ mindset beliefs influence school engagement in China: Roles of perceived classroom goal structures and adolescents’ mindset beliefs. Sch. Psychol. Int. 45, 465–494. doi: 10.1177/01430343231216974
Kaya, S., and Karakoc, D. (2022). Math mindsets and academic grit: How are they related to primary math achievement. Eur. J. Sci. Math. Ed. 10, 298–309. doi: 10.30935/scimath/11881
Khalilzadeh, S., and Khodi, A. (2021). Teachers’ personality traits and students’ motivation: A structural equation modeling analysis. Curr. Psychol. 40, 1635–1650.
Kim, M. H., Han, J., Buford, K. N., Osterhage, J. L., and Usher, E. L. (2024). Undergraduate student perceptions of instructor mindset and academic performance: A motivational climate theory perspective. Contemp. Educ. Psychol. 77:102280. doi: 10.1016/j.cedpsych.2024.102280
Lüftenegger, M., and Muth, J. (2024). Teachers’ mindset meaning system: achievement goals, beliefs and classroom practices. Soc. Psychol. Educ. 27, 2923–2942. doi: 10.1007/s11218-024-09952-w
Mesler, R. M., Corbin, C. M., and Martin, B. H. (2021). Teacher mindset is associated with development of students’ growth mindset. J. Appl. Dev. Psychol. 76:101299. doi: 10.1016/j.appdev.2021.101299
Midgley, C., Maehr, M. L., Hruda, L. Z., Anderman, E., Anderman, L., Freeman, K. E., et al. (2000). Manual for the Patterns of Adaptive Learning Scales. Michigan: The University of Michigan.
Office of the Basic Education Commission (2017). Indicators and Core Content for Mathematics Learning Area (Revised Edition 2017) According to the Basic Education Core Curriculum, B.E. 2551. Bangkok: Cooperative Agricultural Community Printing House of Thailand.
Office of the Basic Education Commission (2019). Guidelines for Supervision to Develop and Promote Active Learning According to the Policy of Reducing Classroom Time and Increasing Learning Time. Available at; http://academic.obec.go.th/images/document/1603180137_d_1.pdf (accessed August 4, 2024).
Park, D., Gunderson, E. A., Tsukayama, E., Levine, S. C., and Beilock, S. L. (2016). Young Children’s motivational frameworks and math achievement: relation to teacher-reported instructional practices, but not teacher theory of intelligence. J. Educ. Psychol. 108:101299. doi: 10.1037/edu0000064
Piyakun, A. (2020). Examining teachers’ mindsets, values, and instructional practices in mathematics classroom. J. Res. Methodol. 33, 219–237.
Robinson, K. A. (2023). Motivational climate theory: Disentangling definitions and roles of classroom motivational support, climate, and microclimates. Educ. Psychol. 58, 92–110. doi: 10.1080/00461520.2023.2198011
Saefudin, A. A., Wijaya, A., Dwiningrum, S. I. A., and Yoga, D. (2023). The characteristics of the mathematical mindset of junior high school students. Eur. J. Math. Sci. Technol. Educ. 19:em2208. doi: 10.29333/ejmste/12770
Scales, P. C., Van Boekel, M., Pekel, K., Syvertsen, A. K., and Roehlkepartain, E. C. (2020). Effects of developmental relationships with teachers on middle-school students’ motivation and performance. Psychol. Schools 57, 646–677.
Schiefele, U. (2017). Classroom management and mastery-oriented instruction as mediators of the effects of teacher motivation on student motivation. Teach. Teach. Educ. 64, 115–126. doi: 10.1016/j.tate.2017.02.004
Schiefele, U., and Schaffner, E. (2015). Teacher interests, mastery goals, and self-efficacy as predictors of instructional practices and student motivation. Contemp. Educ. Psychol. 42, 159–171. doi: 10.1016/j.cedpsych.2015.06.005
Sun, K. L. (2019). The mindset disconnect in mathematics teaching: A qualitative analysis of classroom instruction. J. Math. Behav. 56:100706. doi: 10.1016/j.jmathb.2019.04.005
Swann, J., William, B., and Snyder, M. (1980). On translating beliefs into action: Theories of ability and their application in an instructional setting. J. Pers. Soc. Psychol. 38, 879–888.
Tiberius, V. (2018). Well-Being as Value Fulfillment: How We Can Help Each Other to Live Well. Oxford: Oxford University Press.
Towner, E., Chierchia, G., and Blakemore, S.-J. (2023). Sensitivity and specificity in affective and social learning in adolescence. Trends Cogn. Sci. 27, 642–655. doi: 10.1016/j.tics.2023.04.002
Upadyaya, K., and Eccles, J. S. (2014). How do teachers’ beliefs predict children’s interest in math from kindergarten to sixth grade? Merrill Palmer Quart. 60, 403–430.
Wigfield, A., Hoa, L. W., and Klauda, S. L. (2012). “The Role of achievement values in the regulation of achievement behaviors,” in Motivation and Self-Regulated Learning: Theory, Research, and Applications, eds D. H. Schunk and B. J. Zimmerman (New York: Routledge), 169–195.
Yeager, D. S., and Dweck, C. S. (2020). What can be learned from growth mindset controversies? Am. Psychol. 75, 1269–1284. doi: 10.1037/amp0000794
Keywords: mathematical mindset, attainment value, intrinsic value, utility value, cost value, mastery-oriented achievement goal, performance-oriented achievement goal
Citation: Piyakun A and Phusee-Orn S (2025) The roles of teachers’ beliefs and instructional practices in students’ mathematical mindset. Front. Educ. 10:1480277. doi: 10.3389/feduc.2025.1480277
Received: 13 August 2024; Accepted: 16 January 2025;
Published: 31 January 2025.
Edited by:
Mei-Shiu Chiu, National Chengchi University, TaiwanReviewed by:
Jo Boaler, Stanford University, United StatesLianchun Dong, Minzu University of China, China
Copyright © 2025 Piyakun and Phusee-Orn. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Araya Piyakun, YXJheWEucEBtc3UuYWMudGg=
 Araya Piyakun
Araya Piyakun Songsak Phusee-Orn
Songsak Phusee-Orn