Integrating inquiry and mathematical modeling when teaching a common topic in lower secondary school: an iSTEM approach

The world has been increasingly shaped by Science, Technology, Engineering and Mathematics (STEM). This has resulted in educational systems across the globe implementing STEM education. To reap maximum benefits, researchers are now advocating for the integration of STEM domains. In recent studies, the integration of science and mathematics has become increasingly popular. The domains are much more suitable for integration because of their fields of application and their mutual approach toward problem-solving. However, there is little empirical evidence to drive the development of a practical model for classroom implementation. This study aims to cover that gap through integrating mathematics and science concepts when teaching a common topic to two classes of Form 1 (13–14 years) students. A mathematics and a science teacher went through two cycles of lesson study, integrating and teaching the concept of density. Results show a strong synergy between the BSCS 5E instructional model of inquiry and mathematical modeling; hence the methodological approaches can be used to integrate common topics like density. Further, teacher collaboration, teacher immersion in the iSTEM practices, teacher’s knowledge, and skills of the other subject and an in-depth understanding of a problem and its contextualization, are variables that can be capitalized on to enhance the teacher’s capacity to implement innovative and integrated STEM programs effectively.

1 Introduction

Integrated STEM (iSTEM) education has the potential to increase student’s learning outcomes (Thibaut, 2018; De Loof et al., 2022) as well as their interests in and motivations for STEM careers (Honey et al., 2014; Tati et al., 2017). Despite iSTEM’s apparent benefits, designing qualitative iSTEM projects and implementing these in the classroom is challenging for high-school teachers (Dare et al., 2018; Thibaut, 2018; Spikic et al., 2023). Kelley and Knowles (2016, p. 3) consider iSTEM education to be a situation when content from at least two or more STEM domains, bound by STEM practices within an authentic context is taught in an integrated way to enhance student learning. Recently, the integration of mathematics and science has gained momentum, though literature mostly covers the conceptual underpinnings (see for example, Davison et al., 1995; Berlin and White, 1999; Kiray, 2012; Dorier and Maass, 2020; Treacy, 2021), showing that mathematics and science are well suited for integration. The domains share a similar field of application and approach toward problem-solving. Emphasis has been drawn on the similarity between the processes of inquiry in science learning and the processes of mathematical modeling (MM) in mathematics learning (see for example, Leung, 2018). However, there is little empirical evidence to drive the development of a practical model for classroom implementation (Ríordáin et al., 2016; Srikoom et al., 2018; Treacy, 2021), a situation that is exacerbated by the complexities of school-based research and standard based examination curriculums (Berlin and White, 2012). This study aims to explore how science and mathematics can be integrated when a science and a mathematics teacher collaborate to teach the concept of density at the junior secondary level in Zimbabwe. Most in-service teachers in Zimbabwe received training in either mathematics or science and they have not had experience in implementing integrated STEM pedagogy. Public schools prioritize examination-centered curricula over STEM integration. Further, teachers are overburdened with workload and are lowly motivated due to low remuneration. These contextual factors (see Dong et al., 2019; Fang and Fan, 2023) decrease the likelihood of a successful integration across all STEM domains (Gardner et al., 2019). The authors therefore considered streamlining the integration to two domains, i.e., mathematics and science, with the assumption that it would aid teacher’s confidence and buy-in by reducing practical and pedagogical challenges associated with trying to integrate a large range of subjects. Integrating a large range of domains was cited as one of the main challenges that was experienced in an iSTEM initiative in Finland (Braskén et al., 2020). Some researchers posit that iSTEM education should incorporate two essential elements of STEM integration, namely (1) problem-solving and (2) inquiry (see Clark and Ernst, 2006; Morrison and McDuffie, 2009; Felix and Harris, 2010). Other researchers emphasize the need for integration of content from at least two domains, contextualized to solve real-world problems (Kelley and Knowles, 2016; Kloser et al., 2018), while others stresses the need to conserve the disciplines’ unique characteristics, depth, and rigor (Corlu et al., 2014) when integrating. This underscores the significance of integrating content knowledge, problem-solving abilities, and inquiry-based instruction within iSTEM education (Wang et al., 2011). The current study therefore seeks to explore how a mathematics teacher and a science teacher can integrate mathematics and science concepts of density using the context of a Titanic ship tragedy scenario. Additionally, the study will discuss teacher’s views on how they can enhance the integration of the topic by incorporating the domain of Engineering and Technology when teaching the same topic in future. The study highlights and provides empirical evidence for the model and feasibility of integrating mathematics and science in a normal classroom situation. The need for new models of implementing iSTEM at classroom level and from various school settings have since been echoed by Wang et al. (2010) and Moore et al. (2014). Very few studies have reported such findings within the context of day-to-day classroom teaching, by in-service science and mathematics teachers. Further, the need for research related to teachers and school context when planning for any new educational reform has been overemphasized (el-Deghaidy et al., 2017), and there are no studies on iSTEM education that were done in the context of the Zimbabwean education system and involving in-service teachers.

2 Conceptual framing and related literature

2.1 Integration models

Several models are available that suggest how STEM disciplines can be integrated. The difference in the models mostly depends on the level of connections between the disciplines and to the real-world problem and not necessarily the number of disciplines being integrated (Sanders, 2008; Moore et al., 2014; Kelley and Knowles, 2016), for example: (1) Disciplinary; when subject-based content is learned in separate disciplinary classrooms (2) Multidisciplinary; when content is learned separately but connected a common theme. The metaphor of chicken noodle soup, as described by Lederman and Niess (1997), illustrates this concept of integration, where each ingredient retains its distinct identity while collectively contributing to the overall essence of the soup. The content from the individual disciplines can easily be distinguished within the curriculum and instruction. Learners would be expected to make connections of these content areas (3) Interdisciplinary; when the learning focus is on content and practices from two or more disciplines connected through a common theme or problem. At this integration level, content from the individual disciplines would be difficult to distinguish from one another, just like in the metaphor of “tomato soup” (Lederman and Niess, 1997) where all ingredients that makes up the soup are mixed together and could not easily be identified or separated. In a classroom situation, the lessons are mostly developed from a problem situation that require understanding of content from different subjects (Wang et al., 2011) (4) Transdisciplinary; when content from two or more disciplines is focused and applied to solve real-world problems (Vasquez et al., 2013). Above all, it is crucial that integration of the STEM disciplines is achieved without losing the disciplines’ unique characteristics, depth, and rigor (Corlu et al., 2014).

There are several approaches that can be used to implement iSTEM curriculum, namely project-based activities, STEM camps, STEM clubs, STEM laboratory activities, activities based on the 5E model, activities based on the engineering design, STEM competitions and university–school partnership programs (Le et al., 2023). Every approach has advantages and disadvantages, for example, both the engineering design and project-based approaches offer benefits such as the development of higher-order thinking skills, problem-solving abilities, improved learning outcomes in STEM subjects, and increased interest in STEM (Kelley and Knowles, 2016; Roehrig et al., 2021). However, these approaches typically require more time, and they necessitate the creation of a final product or prototype, which may pose challenges in exam-oriented curriculum and under-resourced school settings. Utilizing problem-based STEM activities, Integrated STEM-lab activities, and STEM activities employing the 5E instructional model can offer significant advantages by fostering knowledge construction and hands-on skills development (Huri and Karpudewan, 2019; Lytle and Shin, 2020; Ultay et al., 2020). These activities can be conveniently organized using already existing school laboratory materials, making it adaptable to various school settings. However, employing these approaches might pose challenges in creating and executing lessons that seamlessly incorporate all STEM disciplines.

2.2 Integrated STEM instructional practices

The successful implementation of a new instructional approach such as iSTEM strongly depends on several teacher related factors (Laboy-Rush, 2011; Ríordáin et al., 2016; Thibaut et al., 2019). Before immersing learners in the key principles of iSTEM, deliberate effort must be made to ensure that their teachers are competent to instruct using these principles themselves. Literature has documented some iSTEM instructional practices a teacher must possess in order to implement high-quality iSTEM education.

2.2.1 Cooperative learning

Collaboration competence is one of the key instructional practices that is essential for an iSTEM teacher. As such, the teacher is naturally expected to surpass mere consultation with colleagues and routine student group work in the classroom (Dare et al., 2018; Thibaut et al., 2018). An iSTEM teacher should be able to create opportunities for collaborative learning among both teachers and students. iSTEM instruction require that teachers integrate content and skills from the other domain, which in most cases they may not be comfortable or competent enough to articulate. Collaborating with colleagues specializing in those subjects enables teachers to acquire and deepen their knowledge and skills from the other domain. For example, a math teacher and a science teacher may team-teach a common topic like density and gain from each other mathematical and scientific concepts related to the concept of density. On the other hand, teachers must be able to organize students to work in small groups on a design task, giving them the opportunity to develop their interpersonal skills while also deepening their knowledge and understanding (Spikic et al., 2023). Collaboration and communication skills help to improve students’ learning achievements by allowing them to learn from their peers and build on each other’s strengths (Batdi et al., 2019). Collaboration and communication skills are some of the few 21^st-century skills that are being promoted in iSTEM education. It is recommendable that teachers generally perceive collaboration time with peer teachers as an important consideration for quality iSTEM implementation (see Al Salami et al., 2017; Herro and Quigley, 2017; Margot and Kettler, 2019).

2.3 Real-world problems

iSTEM education should be contextualized to solve real-world problems (Kelley and Knowles, 2016; Kloser et al., 2018), and the problem solving process is a key component for integration of STEM disciplines (Wang et al., 2011). As such, the teacher should be capable of articulating the problem situation, making sure that it is very clear and well understood by the learners for it determines the whole lesson trajectory. The problem should be authentic, interesting and structured in such a way that it is challenging and attainable through multiple solutions (Moore et al., 2010; Roehrig et al., 2021). The proposed solution should be supported by evidence (Mathis et al., 2016, 2018) and students should explicitly highlight the possible constraints of the proposed solution (Watkins and Mazur, 2013).

2.4 Engineering design

Engineering design is a core activity in iSTEM education (Lin et al., 2022). The common approach is when teachers use hands-on interdisciplinary project-based learning to expose learners in a complete engineering design process to design and produce artifacts, for example a ship or a computer program (Han et al., 2015). The teacher scaffolds learners through the stages of ideation, planning, implementing, and reflecting on the design. Throughout the stages, learners’ choices are given priority and hence during evaluation, learners should be able to justify their designs. The level of teacher’s support provided to learners depends upon the learners’ proficiency and aptitude, allowing space for learners to manage risk, embrace uncertainty, and learn from failure (Moore et al., 2014; Stretch and Roehrig, 2021). As teachers guide learners through the engineering design process, they should consider social, economic, and aesthetic factors as equally important (Roehrig et al., 2021; Roberts et al., 2022). Barak (2012, p. 318) argues that economic, social, cultural, or environmental aspects of a design process fall under the domain of Technology. Through engineering and technology, students can apply their evolving knowledge to real-world problems, allowing them to identify connections and forge links between various STEM fields (Brown et al., 1989). Barak (2012) proposes that engineering and technology should be taught as one unified subject known as Engineering Technology Education (ETE), since the subjects are closely related. This approach also serves to combat the conventional view by many educators who regard technology as mere aids and additional devices that are used during teaching (Czerniak and Johnson, 2007; Felix and Harris, 2010; Wang et al., 2011; Ríordáin et al., 2016). As learners infuse all the design factors during the design process, they become more critical and creative, i.e., skills highly sought after in the 21st century education. The engineering design process may also utilize models to enhance comprehension and facilitate instruction on a particular phenomenon. When using models, emphasis is made on the importance of understanding the assumptions inherent to the model before its use.

2.5 Inquiry

iSTEM curriculum provides a learning platform that prepares learners to think and act like real scientists. The learning environment entails high level of knowledge and engagement from both educators and learners, encourages questioning, thoughtful investigation, making sense of information, and developing new understandings (Kelley and Knowles, 2016). Learners explore new ideas, or test existing ideas by taking things apart, making predictions, observing, and recording explanations (Satchwell and Loepp, 2002). Through this approach, the teacher’s role is to provide guidance by questioning students to help them discover flaws in their reasoning and/or research design, ultimately helping them to arrive at a solution. This can be executed through doing laboratory experiments or field excursions.

2.6 Content integration

Teachers should be able to integrate and align content from different domains into a single curricular activity or unit in order to articulate overarching concepts spanning multiple subject areas. When giving instruction, the teacher should ensure that the connections between the concepts in different domains is made explicit (Moore et al., 2014; English, 2016; Tran and Smith, 2021) since in most cases, learners may not be able to recognize these links on their own (see Tran and Nathan, 2010; Dare et al., 2018; Roehrig et al., 2021). In this way, the teacher has the opportunity to lead a class in comprehending how content from the respective disciplines is crucial in solving a given problem. For example, in this study, teachers lead the classes in discovering how the mathematics and science concepts are applied in the design of ships and also explain how these concepts can be used to explain why the Titanic ship sank. Ultimately, iSTEM learning is said to have been achieved when learners are able to apply content from different domains to the development of design solutions (Tank et al., 2019).

2.7 Science and mathematics integration

Globally, schools have been encouraged to place greater emphasis on problem solving throughout the mathematics curriculum and meaningful integration to other subjects, particularly science (Jerrim and Shure, 2016). Leung (2018) argue that one of the ways to integrate STEM domains is to discern commonalities and differences among the domains. Wherein, commonalities serve to bridge and establish communication while differences serve to maintain the integrity of the individual domain and provide multiple perspectives. Mathematics and science has been regarded to be more suitable for integration because they have similar fields of application and a mutual approach toward problem-solving (Zhang et al., 2015; Leung, 2018). Further, their differences exhibit a symbiotic relationship. Science can often provide concrete or visual examples of abstract mathematical concepts while mathematics can enable students to achieve deeper understanding of science concepts by providing ways to quantify and explain science relationships (McBride and Silverman, 1991; Honey et al., 2014; Ríordáin et al., 2016). Sometimes the synergy can be very strong such that separating the two domains creates a dilemma. Treiber et al. (2023) reports of a scenario where the presence of mathematics in the German Physics Olympiad exam has the potential to demotivate students who are talented in Physics but not very good in mathematics hence leading to the loss of STEM aspirants which are being highly sought-after. They further speculate that Olympians are better prepared to cope with the mathematical challenges of a STEM study, hence removing mathematics will lead to selection of “weak” STEM candidates.

Generally, it has been accepted that science is effectively learnt mostly through the inquiry process while mathematics is through the mathematical modeling process. The two processes has been shown to share exceptional similarities for instance; both processes begin with the problematization of a real-life situation followed by a process to look for the answers using different hypothesis (Artigue and Blomhøj, 2013; Sala Sebastià et al., 2021). Thus, the two approaches can be used as a boundary object (Akkerman and Bakker, 2011) to bridge the epistemological and pedagogical approaches in the learning of mathematics and science (see Figure 1).

Figure 1

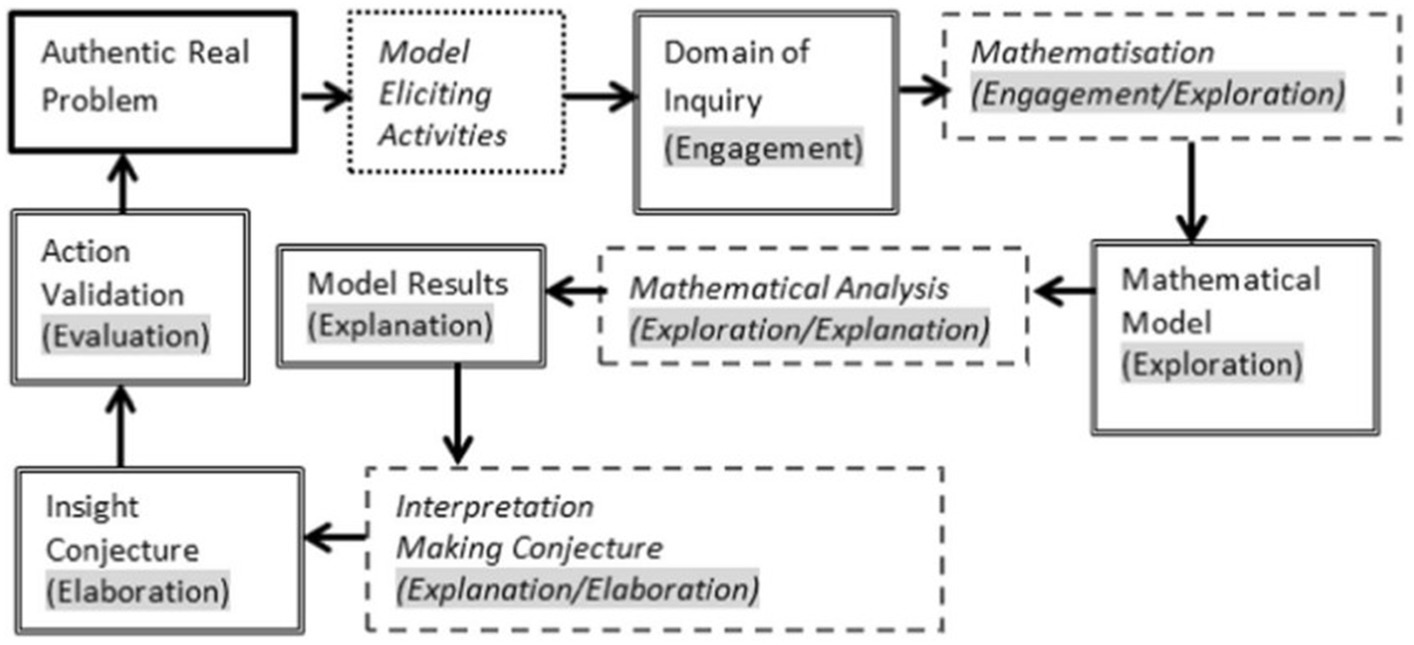
Figure 1. An inquiry-based modeling pedagogical cycle in which elements of mathematical modeling (boxes with solid frame) and inquiry-based learning (shaded) are integrated (Leung, 2018).

2.7.1 Science inquiry

Teaching STEM is considered by several researchers as closely related to inquiry-based teaching (Larsen and Østergaard, 2023). Science inquiry was initially created to outline an effective way for learners to engage with science, and numerous beneficial outcomes have been observed when this approach is utilized in teaching (see Joswick and Hulings, 2023). The commonly used inquiry-based learning model is The BSCS 5E Instructional Model, commonly referred to as the “5Es.” According to Bybee et al. (2006), the five phases of the model can be summarized as below,

• Engage: teacher generates interest and curiosity and helps students to look forward for the lesson.

• Explore: teacher provides resources and time for students to work together, observing, listening, asking probing questions to redirect students’ investigations as they explore.

• Explain: teacher asks for evidence and clarification from students, providing opportunities for learners to demonstrate their conceptual understanding, process skills, or behaviors. The teacher encourages students to explain concepts and definitions in their own words, then provides scientific explanations and vocabulary.

• Elaborate: teacher challenge and extend students’ conceptual understanding and skills, and probe for alternative explanations.

• Evaluation: teacher leads student’s assessment of their understanding. The teacher assesses the student’s abilities and group process skills by looking for evidence that shows change in student’s thinking or behaviors.

A systematic literature review done by Joswick and Hulings (2023) revealed that the 5E is more impactful than traditional instruction. It increases conceptual understanding, problem-solving abilities, self-efficacy, and positive attitudes toward science. The relatively few studies done to implement the 5E in the teaching and learning of mathematics revealed that it promotes flexibility in mathematics instruction and results in increased student conceptual and procedural knowledge, mathematical reasoning and performance (Tuna and Kacar, 2013; Bakri, 2021; Adu and Folson, 2023; Pheaukkhai and Cheausuwantavee, 2023; Alabdulaziz, 2024).

2.7.2 Mathematical modeling

Mathematical modeling (MM) is understood as a common practice of scientists, closely linked to the idea of scientific inquiry. It is also regarded as an approach that may be used to bridge between mathematics, experimental sciences and engineering, since it entails the use of hands-on experiments and the production of a physical prototype (Gilbert, 2004; France, 2017; Carreira and Baioa, 2018; Hallström and Schönborn, 2019). It is a problem solving approach that seek to produce a shareable, manipulatable, modifiable, reusable conceptual tools for constructing, describing, explaining, manipulating or predicting a phenomenon in real life situation (Lesh and Doerr, 2003; Garfunkel and Montgomery, 2019, p. 8). It involves the translation between the real world and mathematics in both directions (Zbiek et al., 2024), prompting students to analyze situations through models and verify that their solutions align with the practical context (Swetz and Hartzler, 1991; Asempapa, 2015). Models and modeling can be used as a basis to foster an integrated and authentic STEM education and STEM literacy (Hallström and Schönborn, 2019). MM promotes quantitative reasoning, problem-solving skills, communication, creativity, innovative abilities, and teamwork, thereby promoting the attainment of the 21st century skills that are being highly sought after in iSTEM education (Blum, 1995; Asempapa, 2015). Through MM, learners appreciate the value and usefulness of mathematics in the real-life situation (Sriraman and English, 2010). Mathematical modeling activities can take a miniature form, making it more appropriate and easier to apply within the existing schooling structures (Kertil and Gurel, 2016). Directly or indirectly, a model is involved in any application of mathematics.

MM consists of several phases, which can vary depending on the complexity of the problem being addressed. The phases are iterative, not always strictly sequential and may involve feedback loops as the modeling process progresses. Without giving much detail of the individual studies, the common phases can be summarized as below (Lesh and Doerr, 2003; Wang et al., 2006; Blum and Ferri, 2009; Maab and Schloglmann, 2009; Roehrig et al., 2012; Torres and Santos, 2015; Leung, 2018).

• Authentic real problem: This phase is also commonly referred to as the “Formulation” stage and it involves simplifying an authentic, real-world situation by defining the problem and establishing objectives to be achieved. The phase ends with the creation of a situation model.

• Domain of inquiry: The phase involves manipulation of the real model that mathematically represents the situation, and it helps to develop equations or functions that describe the relationships between variables. Parameter estimation is then used to determine the best values for parameters that are not precisely known, using available information. In a classroom situation, learners may work in small teams, participating in a range of Model-Eliciting Activities (MEAs) in the quest for data that will help to answer the problem situation. MEAs is a problem-solving approach where learners design or manipulate a given model, situated in realistic, meaningful context to formulate generalizable mathematical equations. The phase mirrors engineering design and it significantly promotes the development of critical thinking, creativity, and communication skills.

• Model results: The phase involves applying mathematical knowledge and strategies to piece together the different equations obtained in the previous stages to solve the problem in the mathematical realm.

• Insight conjecture: This phase involves translating the mathematical results into the real-world situation. It is expected that at this stage, results can be used to explain the solution to the problem in the real-world domain.

• Action validation: In a classroom situation, learners are expected to justify their model/proposed solution or explain how their solution can be iterated to make it batter.

Mathematical modeling is challenging to do and to teach. Teachers are expected to have an in-depth understanding of real-world phenomena and must possess the knowledge of mathematical modeling. Further, they are expected to develop learner’s understanding of mathematical modeling as a mathematical practice, a habit of mind, and an inquiry stance on the world. It is therefore important that teachers are exposed to various learning and teaching experiences through teacher education or professional development (Zbiek et al., 2024, p. 57).

2.7.3 Motivation for selecting density as the lesson topic

The decision to focus on the density topic was driven by the primary researcher’s aspiration to explore instructional approaches that could improve the teaching of this topic. During his tenure as a science educator, a chance dialogue with a mathematics colleague shed light on the shared difficulties students encounter when trying to understand the concept of density in the realm of mathematics. In the Zimbabwean education curriculum, the syllabuses stipulates that the topic of density is learnt at form 2 and 3 in science and form 2 and 4 in mathematics. The authors assumed that the presence of the topic in the mathematics and science syllabuses will increase chances of teacher buy-in and motivate them to collaborate as it is in-line with the goal of the teachers’ test performance. Teachers are unlikely to cooperate when the content in the research is not in line with what will be tested in the national examinations (Schoenfeld and Kilpatrick, 2013; Johnston et al., 2019). Understanding and teaching the concept of density is difficult (Maclin et al., 1997), even teachers were shown to hold misconceptions (Kiray et al., 2015; Kiray and Simsek, 2021). This is because it involves conceptual knowledge of ratios as specifically applied to scientific concepts involving physical quantities and also the mathematical concepts of ratio and proportion (Dawkins et al., 2008), concepts which are abstract and do not involve direct measurements. Literature has revealed that most challenges in understanding the concept of density emanates from a tendency to associate mass, volume, and density with size (Yeend et al., 2001; Zenger and Bitzenbauer, 2022), instead of understanding it as an intensive quantity that requires simultaneous consideration of the object’s mass and its volume (Dole et al., 2013). This study is interested in exploring how a science and a mathematics teacher can integrate mathematics and science concepts to learn the concept of density through hands-on learning experiences, as was recommended by (Nunes et al., 2003). In particular, the study asks:

1. How did a science and a mathematics teacher integrate mathematical modeling and science inquiry when teaching the concept of density?

2. How does the integration by a mathematics teacher and a science teacher compare:

3. a. with each other?

4. b. with models proposed in literature?

3 Materials and methods

3.1 Participants

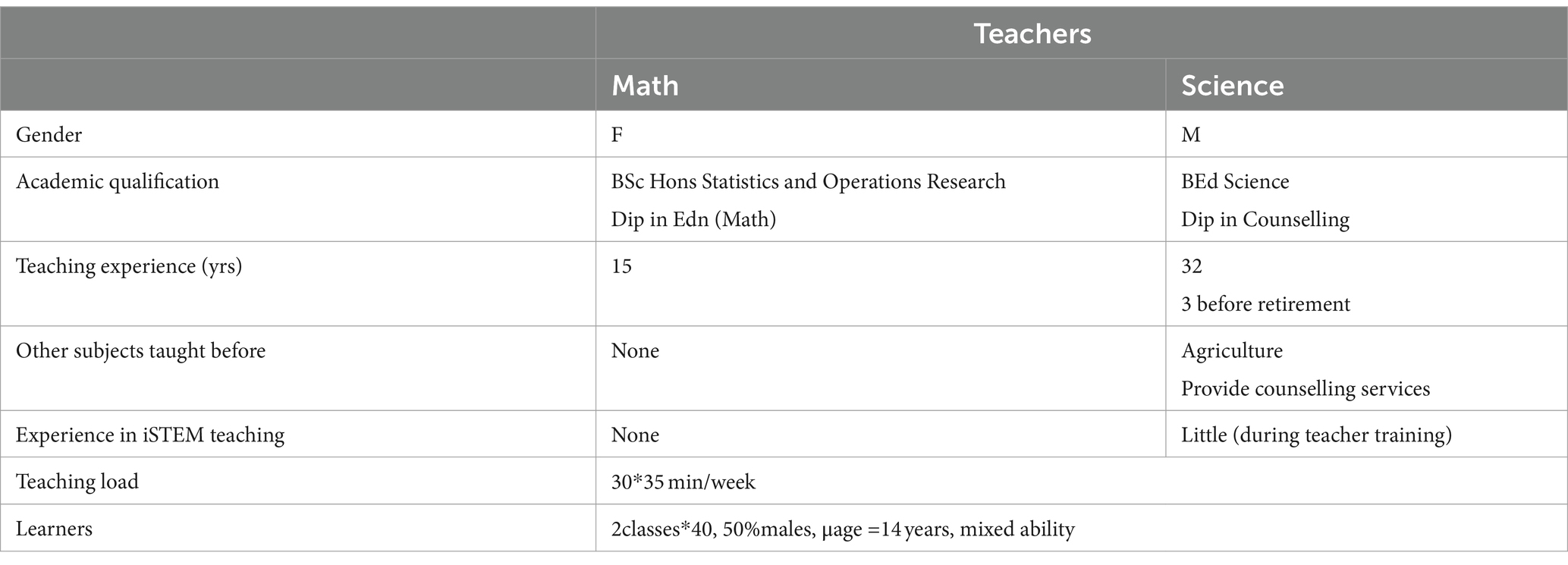
The research employed a lesson study design. A purposive and convenient sampling technique was used to recruit two in-service teacher participants, i.e., a science and a mathematics teacher. Firstly, the first author contacted several teachers in his network who teach mathematics or science at secondary school level in Zimbabwe, inviting them to participate in this study. One of the science teachers showed interest. The science teacher then introduced his colleague, a mathematics teacher. The teachers were willing to collaborate with each other and they taught the same classes, i.e., participants possessed the required characteristics for this research. Teaching the same students and consensus building are some of the critical issues to consider first when planning an effective integrated instruction (Jacobs, 1989). The teachers selected two classes of form one students they were willing to work with. Each class had 40 students, mixed ability and their average age was 14 years. The teachers are registered with the Ministry of Primary and Secondary Education and are highly experienced with extensive years of teaching practice. For example, the mathematics teacher holds a bachelor’s degree in statistics and operations research, a teaching diploma, and had accumulated 15 years of classroom teaching experience. The science teacher has a bachelor’s degree in science, a teaching diploma, and had 32 years of classroom teaching experience, please refer to Table 1 for a summary of participant profiles. In Zimbabwe, all registered teachers hold a teaching diploma as a minimum qualification, with the majority also possessing a first degree relevant to the subject they teach. The school is a government owned, public, urban, day school in Harare. It is one of the largest schools in Zimbabwe in terms of population, with an estimated 2,500 students in form 1-to-6 (13–20 years). The school runs two learning sessions, i.e., morning session and afternoon session. The school has three sets of science laboratories, i.e., for junior secondary learners, for middle secondary learners and for Advanced level learners. The science teachers have their offices in the science laboratories and mathematics teachers share offices with other staff members in the common staff offices unless one has a private office. The school is receptive to authorized academic research.

Table 1

Table 1. Research participant profiles.

3.2 Description of data collection and lesson sequence

3.2.1 Lesson study

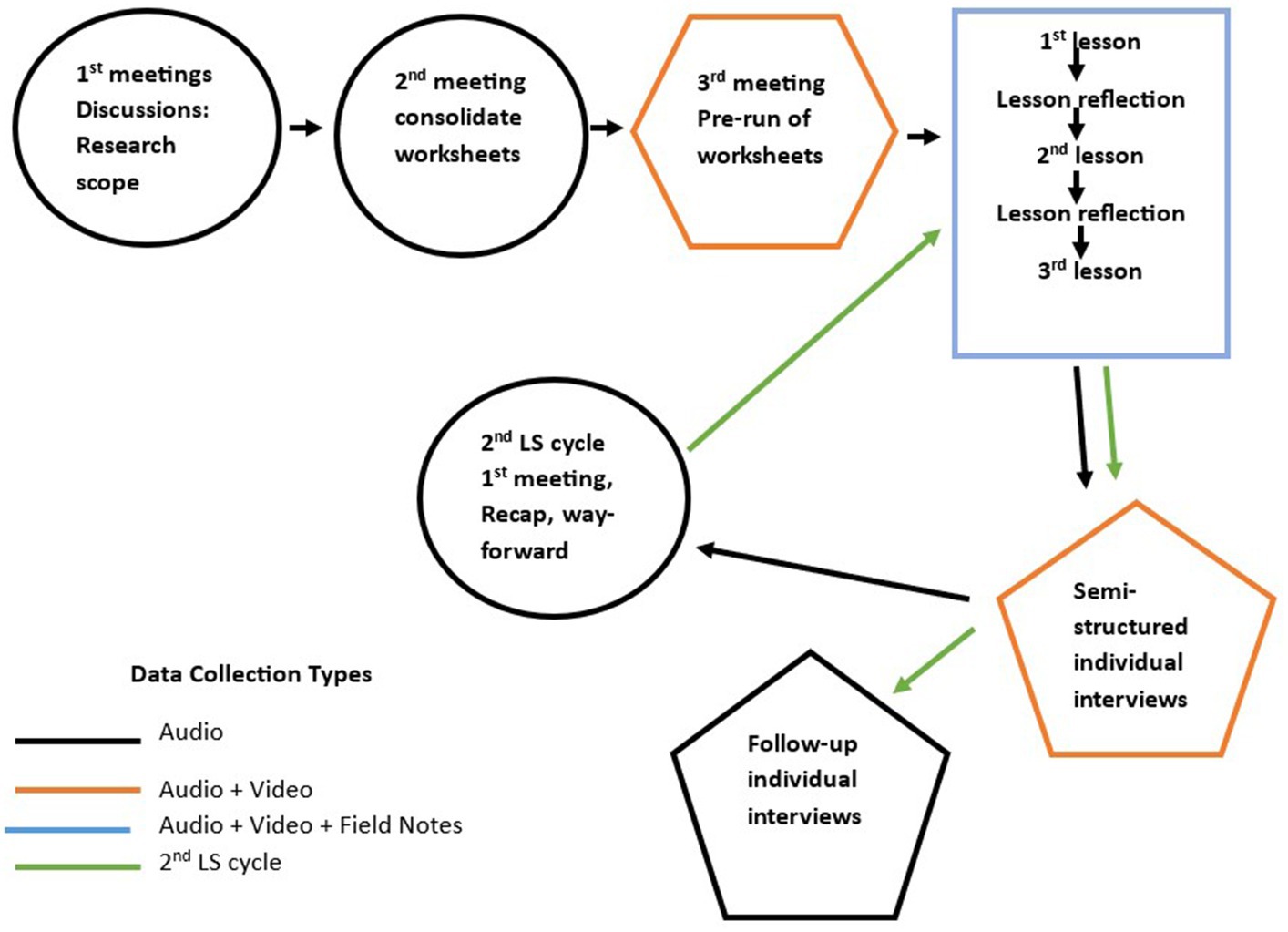
The research followed two cycles of lesson study (LS) format, please refer to Figure 2. LS is a process whereby educators engage in cycles of collaborative planning, teaching, observation, and reflection upon student learning within lessons (Lewis, 2016). This study opted for LS format in order to mitigate the impact of deficiencies in pedagogical content knowledge of the other subject that the participant teachers may face during the lessons (see for example Honey et al., 2014; Ríordáin et al., 2016; Treacy, 2021) whilst at the same time allowing for the conservation of own discipline’s unique characteristics, depth, and rigor (see for example Corlu et al., 2014). The format positioned teachers at the center of the integration process, improving their self-efficacy and giving them a sense of ownership of the integration process. The teachers had the opportunity to model the research lesson to suit the level of their learners, their unique teaching context in relation to the available resources and time, hence bridging the gap between theory and practice (see Murata, 2011; Kieran et al., 2012). For the purposes of describing the data collection procedure, the format is divided into five distinct phases as follows: (i) preparatory phase, (ii) lesson delivery phase, (iii) teacher reflection phase (iv) interviews phase and (v) follow-up interviews phase. The phases are successive rather than concurrent and a preceding phase adds value to the succeeding phase. Data pertaining to the teachers that was collected during lesson delivery and interviews is analyzed using theme analysis.

Figure 2

Figure 2. Data collection procedure.

3.2.2 Data collection instruments

lesson observation and semi-structured teacher interviews were the primary data collection instruments for this study. The first author directly observed all of the six lessons, recording field notes and the lessons were audio and video recorded. Interview protocols were used for all the six interviews, the researcher recorded short field notes and the interviews were audio/video recorded. Details of how the instruments were used are given under the descriptions of the different phases.

The research utilized a research lesson on density that was created by the authors and validated by an expert (refer to Appendix A). It consisted of three 70 min worksheets on density. The worksheets were designed using the Predict Observe Explain (POE) and the Control of Variable Strategy (CVS). The first worksheet develops the concept that mass of a substance affects the substance’s ability to sink or float in water. The second worksheet develops the concept that volume of a substance affects the substance’s ability to sink or float in water. The third worksheet develops the concept of density using the findings from lesson 1 and 2. Teachers demonstrate relative density and evaluate student’s understanding of the concept of density. Some task questions on worksheet three were adapted from Zoupidis et al. (2016).

3.2.3 First phase

In the first phase of data collection, the author and teacher participants held three preparatory meetings, each approximately 90 min long. The teachers suggested the dates, time, and venue for the meetings. All the meetings were held in the science office, which is housed in the Junior Science Laboratory. The meetings were video, and audio recorded. The meetings discussed: (i) consent and logistical issues, (ii) the scope of the research, (iii) the research lesson (Appendix A), (iv) contextualize and implement teacher’s input on the research lessons and (v) pre-running the lessons to test the practical set-up, anticipate learner’s behavior and take corrective measures where necessary. This phase is synonymous with Kyouzai kenkyuu, or the “study of materials for teaching” in the Japanese lesson study. The stage is considered by Murata and Lee (2021, pp. 39–40) as the most important stage in LS because teachers will develop understanding of the instructional materials from their own perspectives and from the perspective of their students. The authors had assumed that if the teachers comprehend and then adopt the research lesson and the basic tenets of integration, the meetings would have achieved in making teachers take greater ownership of the research lesson, its implementation and fostering their self-efficacy. During the meetings, the author took a facilitator role, offering perspectives that lead to deep understanding of the issue being discussed. The atmosphere was conducive for participants to freely express their points of view and perspectives without the author dictating what direction the teachers were supposed to take. All the meetings were audio and video recorded, giving the author the opportunity to concentrate on the discussions and to analyze the recordings in detail after the meetings. Insights from the meetings helped in assessing: the teacher’s initial level of understanding of the concept of integration and how much the authors should share with them, if the teacher’s contribution on the research lesson would not divert it from its gist whilst at the same time giving them the opportunity to own the research lesson, their anticipation and subsequent decision making on how students may possibly behave during lesson delivery. After the three preparatory meetings, there was a 1 week break for teachers to do further research on the concept of iSTEM and to prepare for the lesson delivery phase.

3.2.4 Second phase

In the second phase of data collection, the teachers collaboratively delivered six lessons. Three lessons were delivered in the first LS cycle and were dominantly led by a science teacher while the mathematics teacher assumed a supporting role. In the second LS cycle, the mathematics teacher led the other three lesson deliveries while the science teacher assumed a supporting role. Each lesson was approximately 70 min long, and the lessons were held in the Junior Science Laboratory. The delivery of the lessons was based on the content discussed and covered in the research lesson. The first author observed all six lessons. The lessons were video, and audio recorded. The video camera was stationarily positioned at the back of the classroom, capturing the whole classroom view. The audio recorder was stationarily positioned at the front of the classroom on the teacher demonstration table. Additionally, in the second LS cycle, additional audio recorders were placed on selected group tables to capture the whole group discussion and group-teacher discourse when the teacher visited that specific group. To reduce camera effects, learners were told of the presents and purposes of cameras and audio recorders. The equipment was set before lesson started, was stopped after the learners left the laboratory, and the researcher and teachers did not pay particular attention to the equipment during the class. Recording the lessons using stationery, independent multiple cameras gave the author the opportunity to write detailed lesson observation field notes and to refer to the recordings at a later date and time for detailed analysis. Additionally, it gave the second author the opportunity to analyze the videos without being constrained to the codes selected by the first author. During classroom observations, my focus was to observe the teacher’s classroom practices in integrating science and mathematics and the way they responded to student queries and impromptu incidences. Additionally, the author objectively observed how the teachers were collaborating, the specific mathematics and science content they covered during each lesson, the level of participation by the individual teachers, and aspects of iSTEM skills implemented by the teachers. At the same time, the author avoided interfering with the teaching and learning process, concentrating on taking field notes, capturing as much information as possible of what was transpiring in the classroom. Selected points noted in the field notes were used during teacher reflections and teacher interviews to trigger discussions in areas where the researcher needed clarifications. Data from the lesson delivery was transcribed and analyzed and it formed the basis upon which findings of this research are grounded.

3.2.5 Third phase

Immediately after every lesson, there was a reflection meeting facilitated by the researcher. This was in line with the recommendation by Lewis and Hurd (2011) that reflection meetings should take place as soon as feasible following live lessons. Each reflection meeting lasted around 20 min and was audio recorded. During the meetings, various aspects were considered for discussion, such as teacher collaboration, integration of math and science concepts, student behavior, and using the meeting outcomes to guide future lessons. During the meetings, the researcher contributed to the discussion by asking questions such as “What are your thoughts on the mathematics concepts covered in the lesson? Were they explicit to the learners?.” At the same time, the researcher refrained from dictating way forward or pre-emptying the critical analysis of the observed lesson.

3.2.6 Fourth phase

Since the number of respondents is small, the research utilized interviews to explore in-depth information related to the answering of the research questions. The first author conducted four, individual, face-to-face, semi-structured teacher interviews. Each interview lasted approximately 60 min. Two interviews were done after the first three lessons and the other two interviews were done after the 6th lesson in the second cycle (refer to Figure 2). The interviews were done in the science teacher’s office, in a comfortable, relaxed, and interactive atmosphere. All the interviews were video, and audio recorded. The video camera was placed in a fixed position where the faces were easily seen, and voices were audible. The audio recorder was stationarily positioned on the conversation table. By recording the interviews, the author had the leeway to fully focus on the interview content without the necessity of taking extensive notes during the interview itself. An interview protocol was used, and the interview questions were semi-structured and open-ended as much as possible to allow respondents to demonstrate their unique perspective of an issue being discussed. The overarching objective was to explore the teacher’s perceptions of the lessons, whether they were integrated or not and their suggestions to improve the level of integration. Data from these interviews was transcribed and analyzed and it also formed the basis upon which findings of this research are grounded.

3.2.7 Fifth phase

Initial data analysis indicated the necessity for further data collection, leading to the conduct of two online follow-up telephone interviews during the fifth stage of data collection. The authors opted for online WhatsApp voice call interviews after considering the geographical distance, time and associated flying costs to reach the research participants, factors which rendered in-person interviews impossible. Telephone interviews were found to produce equivalent depth of the responses when compared to face-to-face interviews (Sturges and Hanrahan, 2004; Vogl, 2013). In particular, WhatsApp platform offers speed, cost-effectiveness, and convenience. It is recommended by several researchers as an option to interview participants from around the globe, provided they have access to a smartphone or computer, and a network (Opdenakker, 2006; Squires, 2010; Singer et al., 2020; Gibson, 2022). The follow-up interviews had three main objectives to achieve: (i) to gain teacher’s reflection of the study, (ii) seek clarification on unclear issues that emanated during the preliminary data analysis and (iii) to explore how the teachers perceived to have utilized the iSTEM characteristics and their views on how they can improve the lessons if they were to be given the chance to reteach. On the third objective, before the teachers could narrate their response, the researcher described the basic tenets of the iSTEM characteristic in question. Caution was taken during the description to avoid too much spoon-feeding and, at the same time, helping the interviewees to mobilize their memories and to narrate freely; the approach that was recommended by Jenner et al. (2004 p. 206). Because follow-up interviews were the last set of data collection, the interview questions incorporated teacher reflection questions, follow-up points from the previous interviews and questions that seek to explore how the teachers perceived to have used iSTEM characteristics. Consequently, the interviews naturally took the longest time, approximately 90 min each. At the beginning of the interviews, a consensus was made to take intermittent breaks whenever the interviewee feels exhausted, or has an urgent matter to attend to, or when there is poor communication due to network challenges. Again, prior to commencement of the interviews, the interviewees were informed that the conversation will be recorded for research purposes. The phone’s loudspeaker was activated during the call, enabling simultaneous recording with an external recorder to prevent the possibility of not capturing the conversation. The follow-up interviews served as the third data source which was transcribed and analyzed, also contributing to the overall research findings.

3.3 Teacher collaboration

The “one-teaching-one assisting” collaboration model (see for example Kluth and Straut, 2003; Liu, 2008) was employed in this study. In order to promote integration of mathematics and science content and to boost the teacher’s confidence, the two participant teachers collaborated in planning and teaching the same class of students. The concept of integrated STEM education was new to the participating teachers, and it is also new to the general populace of in-service Zimbabwean teachers who were trained and are often working in a “siloing” environment. The authors therefore posited that collaboration could enhance teachers’ confidence, belief and reduce teacher isolation by facilitating mutual support and learning, thereby helping to address any shortcomings in pedagogical content knowledge related to the other subject that the participating teachers might encounter during lessons. This approach also aimed to preserve the distinctive characteristics, depth, and rigor of mathematics and science during integration (see for example Corlu et al., 2014; Treacy, 2021).

4 Data analysis

4.1 Analysis of the lessons

The authors analyzed the lessons based on the evidence and subsequent length of the processes of the BSCS 5E phases of Inquiry and the phases of mathematical modeling. For example, the teacher’s instruction “Ok, you should have finished recording your observations, now justify your observations” signals the end of exploration phase and the start of explanation phase. Lesson analysis pertaining to that which was dominantly led by the science teacher and that which was dominantly led by the mathematics teacher is analyzed in unison and the difference will be highlighted toward the end of each lesson description. Further, data was also analyzed to reveal the individual teacher’s iSTEM practices.

4.1.1 Lesson 1

The teacher started the lesson by pausing some questions “In everyday life we interact with ships, do you know ships, what is a ship?” One of the students responded, “A mode of transport that travels in water.” The teacher then further describes the immense size of ships and that they carry heavy loads but do not sink. In a small teacher-student discourse, reference to the sinking Titanic ship was given and he asked, “what could have caused the ship to sink?” (Authentic Real Problem). After taking a few responses, the teacher highlighted that they were going to do some experiments to discover how ships float and what can make them sink (contextualizing the lesson). To conclude his introduction, he paused a question. “Are we in the lesson now?” and students responded “Yes!.” The introduction resonates well with the BSCS 5E Engagement phase where teachers are expected to generate interest, curiosity and help learners to become engaged. The teacher managed to focus the students’ minds on the Titanic ship (object), that sank (problem). By revealing that they were going to learn about how ships float or sink, the teacher organized the student’s thinking toward the learning outcomes of current lesson and made students to ponder on what exactly could have happened and how will the situation/event be demonstrated in class. Though the last question “Are we in the lesson now?” sounded more rhetorical, the students confirmed they had been engaged and were ready for the lesson. The question, “what could have caused the ship to sink?,” evoked students to make a Situation Model by mentally imagining a huge Titanic ship (size) carrying lots of passengers, hotels, swimming pools (mass), floating (water).

With the help of the mathematics teacher, students were made to quickly move into their small groups and start doing practical activities following worksheet instructions. Students measured and recorded the mass of the different sets of containers (Real Model) and then made predictions on whether each of the containers will sink or float when placed in a bucket of water provided. The real model was a visual, simplified version of the Titanic ship where the size of containers represented the size/volume of the Titanic ship, mass of the containers represented the mass of the Titanic ship, and a bucket of water represented the ocean. Students enjoyed making predictions which evoked the Domain of Inquiry as the students were now eager to Explore and confirm their predictions. The teachers moved around observing and listening to students as they do the Model Eliciting Activities to explore the variables that make the containers sink or float. Depending on each group’s progress, the teachers probed questions to redirect students’ investigations when necessary. For example, they would say “I do not think you are moving in the right direction, re-read the instructions,” without necessarily telling students what they should do. The teacher’s intervention accorded students the opportunity to puzzle through problems, to interact more and find alternative ways to solve the puzzle. The CVS was used to Mathematise the real model by simultaneously placing container sets of the same size but different mass into a bucket of water and observe how they behave, i.e., sink or float.

Thirty-one minutes into the lesson, the teacher advised students to have started answering question 5 which required learners to Explain their observations. Most groups were able to mathematise the activities culminating in the formation of a Mathematical Model (mass α sinking) i.e., when mass increases, it increases the chances of a container to sink. However, as teachers were moving around, they noticed that some groups claimed that containers with salt solution had more volume than those with distilled water hence containers with salt solution sank. One of the main problems in adopting inquiry learning is that students tend to “simplify inquiry tasks and seek ‘right’ answers rather than to investigate deeply” (Kim et al., 2007). The teachers also noticed that some students were comparing containers from different sets and that made justification of their results to be difficult. One of the students argued that the results seem not to make sense because they are mixed-up to which the teacher responded, “you did not follow the instructions properly.” The student’s concern and the teacher’s response confirm the submission by Boudreaux et al. (2008), that reasoning based on control of variables is challenging for students at all levels and that even teachers may confront difficulties in understanding the underlying reasoning of CVS. To deal with this misconception about volumes of containers, the science teacher decided to Elaborate on the practical activities by providing each group with small measuring cylinders and syringes and instructed them to do additional activities of measuring the volumes of the contents of one set of containers. The teacher’s intention was to let students discover that each set of containers had the same volume of the contents hence directing their attention to the mass variable. The teacher also wanted students to discover that the experimental activity involved controlling one variable, i.e., volume. However, in the proceeding discussion, some students still maintained that salt solution had many elements and therefore it had a higher volume, proving that students’ ideas can be resistant to change and easily interfere with students’ abilities to learn scientific concepts (Hammer, 1996; Jaakkola and Nurmi, 2008; Treagust and Duit, 2008).

Fifty minutes into the lesson, the teacher led the feedback session with different groups presenting their work to the class. The session was characterized by a lengthy teacher-student discourse, and it provided an opportunity for students to communicate and be Evaluated by classmates who are very close to their own level of understanding. A discussion was also centered on how the results can be explained in relation to the everyday real-life applications and the Titanic ship that was introduced at the start of the lesson (Making Conjecture).

At the start of the first lesson, the teacher experienced some glitches in articulating the context of the problem. The teacher confirmed to have not watched the movie on which the problem context was based. The science teacher’s led lesson was characterized by more learner autonomy. Learners progressed from one stage to the next with minimum teacher guidance. During the lesson, some groups missed the prediction stage and the teachers noticed that late into the lesson. The science teacher was also quick to provide alternatives and additional materials for the practical activities when necessary. The lesson that was led by the mathematics teacher was more guided. The teacher took students step by step, following the worksheet instructions in a bid to minimize contingency issues.

To explore the feasibility of collaboration, the original intention was to have a science and a mathematics teacher to collaborate and co-teach using the Team-Teaching model. The teachers were expected to have an equal share of teaching responsibilities by taking turns to facilitate whole-class instruction and leading different activities. However, the team-teaching model proved not to be feasible for this study. In the first lesson, the science teacher was dominant in giving instructions while the mathematics teacher assisted by moving around providing individual assistance to different groups as they were doing practical activities. Assisting duties also included providing additional practical materials to students, helping to resolve challenges arising from students’ interaction with practical materials or interpretation of the worksheet instructions. Only on one occasion during the first LS cycle, the mathematics teacher gave instruction to the whole class to round off their mass measurements of the containers to the nearest whole number. Later, the teacher reported that she was not experienced in leading learners on doing laboratory experiments so she intended to learn from the science teacher “at first I was not sure how the lesson would go, and I did not seem to have understood the research lesson since in mathematics we do not do these activities.” During the teacher reflection meeting, which was held after the first lesson, a consensus was made that one teach/one assist collaboration approach be maintained, and that the mathematics teacher will take a leading role in the second LS cycle while the science teacher will assume the supporting role. Occasionally, teachers were seen having impromptu discussions as learners were doing explorative activities. Effort of teamwork was also evident by the level of organization of learners during lessons which showed that everything for example, the number of groups and the organization of the groups was pre-planned, and this was done in the absence of the researcher. The teachers successfully established a conducive environment for collaborative learning among students by organizing them into small groups. Each group assigned its members some responsibilities like group secretary, group leader and other duties. This approach effectively minimized unnecessary time wastage during the lesson. Fruitful and interesting conversations were evident during group discussions and during whole class feedback.

4.1.2 Lesson 2

The second lesson sequence and activities were almost like that of lesson 1. However, the objective of lesson 2 was to develop the concept that volume is a variable that can influence the sinking or floating of a container, i.e., when volume of container increases, it reduces the chances of the container to sink ( $\mathit{volume}\alpha 1/\mathit{sinking}$ ). The teachers ensured that all the groups recorded their predictions before placing containers in the bucket of water and materials for the lesson activities were prepared with more precision.

4.1.3 Lesson 3

The mathematics teacher led a class discussion to recap the two previous lessons. When students mentioned volume and mass, she probed them to elaborate on how volume was measured. This was crucial because in the previous lessons, there was confusion in referring to volume of container interchangeably with volume of contents. The teacher-student discourse summarized lessons 1 and 2 into two mathematical models.

i. mass α sinking

ii. volume α 1/sinking

Using the concept of proportion, the teacher led a class discussion to connect the two equations (Mathematical Analysis). However, learners seemed to be unfamiliar with the concept of proportion, prompting the teacher to shift her focus of discussion from the Mathematical World View to the Real World View by asking a close-to-home question “if you are to dig an area and if you are ten, you can dig it in ten days, but if you reduce the number of participants to five, then the number of days will increase or decrease?” students responded with “it increases” and she commented “that’s inverse proportion.” Through teacher-student discourse, they developed the formulae: sinking = mass/volume. After that, the teacher formally introduced the term density to replace sinking. The Model Result was therefore written as $\mathit{Density}=\mathit{mass}/\mathit{volume}$ (density model).

Through invitation, the author asked the learners to link what they have learned to the Titanic ship (Elaboration). The intervention initiated a discussion in the interpretation of the mathematical results and to de-mathematise the results into the real world. One learner responded by saying the ship was small, yet it carried too many people hence it became denser, and it sank. When translation of the model results from the mathematics domain to the real world proved difficult, the teacher asked, “can someone who watched the movie tell us what actually happened to the Titanic ship?.” A student narrated the event that led to the sinking of the ship. After that learners were then able to explain that the water that entered the ship made the ship gain mass and become denser causing it to sink. It was interesting to note that interpretation of the model results by starting from a situational model to the model results had a significant impact on the student’s insight of the density model (Insight Conjecture). How students and teachers translate between reality and mathematics when learning the concept of density can be an interesting direction for future studies.

Toward the end of the lesson, the mathematics teacher distributed worksheet 3 and instructed students to quickly answer it as individuals. Worksheet 3 contained questions that serve to evaluate the student’s understanding of all the concepts that were covered in the three lessons. The science teacher led lesson differ with that of the mathematics teacher in that the science teacher quickly explained the development of the density equation and then asked students to write an exercise, see Figures 3A,B for relative time distribution of each stage in the teacher’s lessons. During lesson 3, teachers were supposed to do a demonstration of relative density, but it was not done due to logistical reasons.

Figure 3

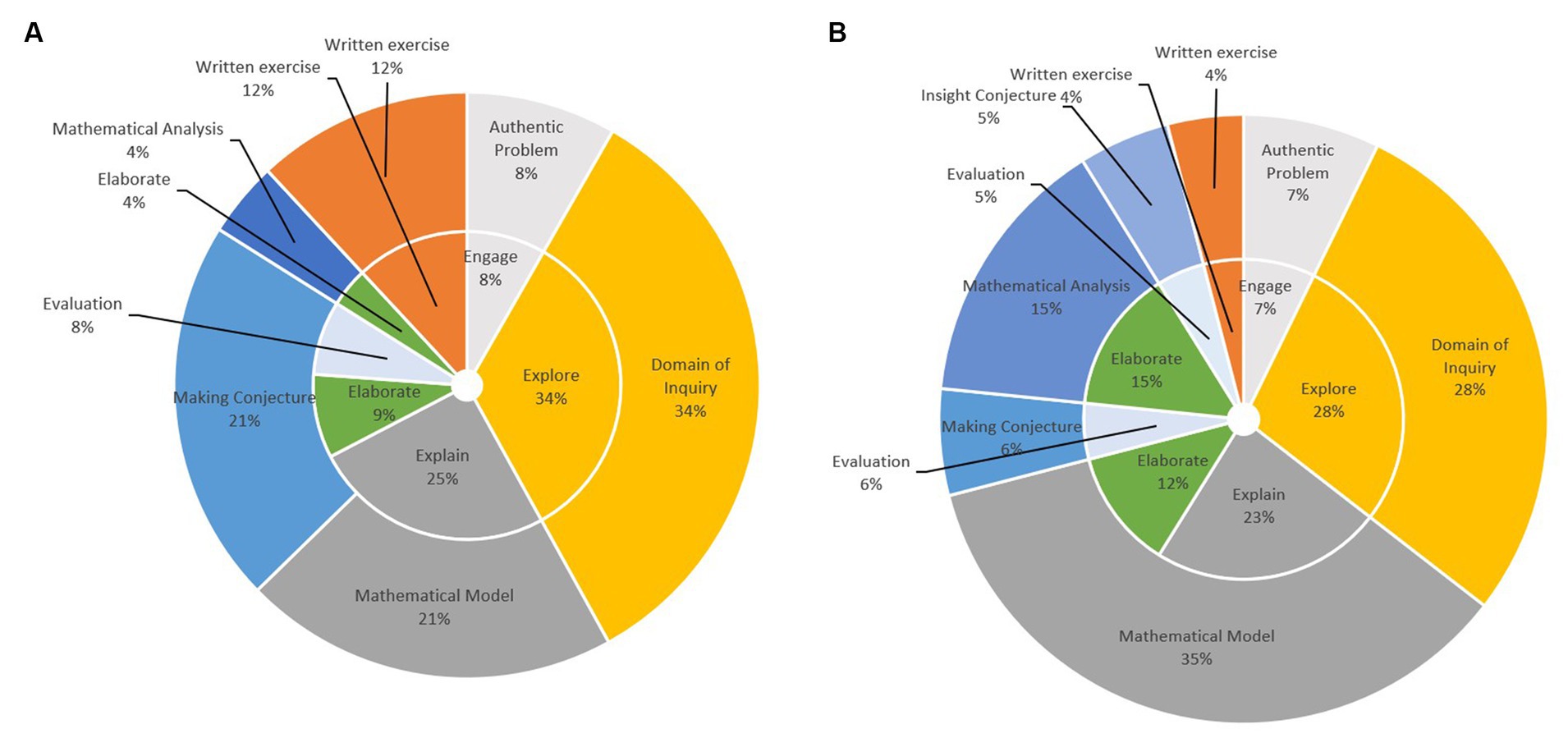
Figure 3. Percentile distribution of phases of inquiry and mathematical modeling: (A) Science Teacher led lesson; (B) Math Teacher led lesson.

4.2 Teacher interviews

After the first cycle of data collection, the mathematics teacher suggested that the integration of science and mathematics was 35% (mathematics):65%(science). She vowed that it can never be 50:50 since all the lesson materials were from the science department and lessons were done in the science laboratory “it can never be 50:50 because all the instruments are from the science department, lessons were done in the science laboratory.” After the second phase of data collection, she stated that it is possible to have a 50:50 integration, arguing that the change in perception could have been influenced by her active role in the lesson “I have changed my perception. I think it was influenced by who is leading the class.” The science teacher argued that the lessons were equally integrated because a lot of science and mathematics concepts were being experienced in the lessons.

In the follow-up interviews, the teachers were asked about the feasibility of the authentic problem used during the lessons. The teachers submitted that some learners did not resonate with the context of the problem because they had not watched the Titanic movie before since it is now an old movie for their generation. To resolve the challenge, the science teacher stated that “I will have to work out a way to clearly express the problem and to make it resonate with learners.” During the course of the study, the researcher noticed that the science teacher had not watched the movie even by the time he conducted the lessons. However, during feedback sessions, most learners were able to link the learned concepts to the problem context. Some explanations by learners surpassed the teacher’s expectations for example, one group of students argued that large ships are able to float because most of the space in ships is filled with empty space that is filled with air hence reducing the ratio of mass to the volume of the ship, hence causing the ship to float. The mathematics teacher suggested that in future she will download the movie before lessons. The teacher also suggested that she can use different problem context that is close to learners, i.e., using small balls, for example cricket ball, pool ball, tennis ball, table tennis ball and baseball balls from her sports office to introduce the problem situation. The teacher stated that her idea was influenced by the resources available in her sports office “I work from the sports office as the school sports organizer, I am surrounded by these balls hence it just came into my mind.”

When the teachers were asked if they had used or developed a model during the lessons, the science teacher stated that the container sets were the model used. The teacher also believes that if he is trained in designing models, his delivery of integrated lessons will improve. The mathematics teacher views the density equation as the model that was developed during the lessons. The teacher also believes that if she gets training in the design of model eliciting activities, her delivery of integrated lessons would improve. The teachers were asked if they had realized, implemented or how they could incorporate Design-Based Learning (DBL) practices in their future lessons when teaching the same topic. The teachers acknowledged that they had not utilized DBL, noting that it might have been possible had they been involved in designing the research lesson from the outset. The science teacher proposed that he will ask learners to watch the movie first and then ask them to make boats using plasticine that simulate how the ship sank and how it can carry maximum load. On the other hand, the mathematics teacher suggested several options of infusing engineering and technology. The teacher proposed enhancing the lesson by having students create two replica ship models—one that sinks due to the presents of a hole and the other that remains floating. She further explained that, as the ship sinks, students can measure the rate of sinking and present their findings using different data presentation formats.

5 Discussion

The integration revealed by the mathematics and science teachers shows great coincidences of specific stages of inquiry and mathematical modeling. Most phases of mathematical modeling and 5E had exact percentile time and sequence/position for example, authentic real problem and engage, domain of inquiry and explore, insight conjecture and evaluation, indicating a strong synergy between inquiry and mathematical modeling. The results are in line with previous studies (see for example, Leung, 2018; Sala Sebastià et al., 2021). The results confirm the argument that there is a close similarity between mathematics and science (Artigue and Blomhøj, 2013). Therefore, mathematics and science are suitable for integration. The results suggest that science inquiry has a shorter ‘life cycle’ than mathematical modeling. In the first two lessons, all the 5E phases were attained in each lesson whilst only three phases, i.e., authentic problem, domain of inquiry and mathematical model were attained for the mathematical model. The additional phases of modeling were realised in the third lesson; however, model validation was not experienced. The research implemented a structured inquiry where a problem and a procedure to solve the problem was given (Zion and Mendelovici, 2012; Bunterm et al., 2014; Schmid and Bogner, 2015). This was possibly the best option than open inquiry because students had no previous experience with the inquiry learning process (see, Schmid and Bogner, 2015), and that the study was constrained by a limited timeframe. Since learner autonomy is restricted in structured inquiry, its “life cycle” becomes short, hence all the 5E stages were attained in each of the first two lessons. An interesting future research direction will be to determine the effect to the integration “model” if the type of inquiry is open.

Model validation was not done by any of the two teachers. The prescriptive model implemented in this research is synonymous with Type 1 case, where validation of the model usually makes no sense (Niss, 2015 p. 78). However, Niss reiterated the importance of prescriptive modeling in science and society and implored teachers to engage students in meta-validation. Initially, the research intended to do a teacher demonstration on relative density in the third lesson but that was not done due to some logistical issues. The authors assume that a demonstration of relative density could have given an opportunity for elements of meta-validation, hence improving the integration of mathematics and science.

In this research, the subject area of specialty appears to have influenced the teacher’s integration model. Deficiencies in teacher knowledge of the “other” subject is one of the threats to integration of science and mathematics (Stinson et al., 2009; Czerniak and Johnson, 2014; Ríordáin et al., 2016; Treacy, 2021). The level and complexity of content knowledge and pedagogical content knowledge of both subjects held by a teacher influences what is done in classrooms, consequently determining what students learn (Frykholm and Glasson, 2005). The science teacher spent more time on the real-world side of teaching density, for example he spent 34% of time in the domain of inquiry/exploration where it requires hands-on manipulation of materials and 4% in mathematical analysis where it requires abstract mathematical reasoning. This can be interpreted as lack of knowledge or confidence to delve much into mathematical area, the area which is not of his specialty. Similar findings were reported by Tekerek et al. (2023) in their research that seek to determine what teachers consider when they plan and implement science and mathematics integration. Tekerek et al., concluded that participant teachers made superficial explanations for the concepts in the other discipline, signaling lack of sufficient content knowledge of the concepts of the other discipline. Previous research has also revealed that most science teachers’ content knowledge, curricular knowledge, and pedagogical content knowledge about models and modeling are often incomplete or inadequate (Justi and Van Driel, 2005). The science teacher’s characteristic is synonymous with a visual (pictorial-holistic) thinking style (Blum and Ferri, 2009), that is presumably more common with science teachers than mathematics teachers. Traditionally, science teaching involves doing science experiments and making observations. The science teacher considered the set of containers used during the practicals as the “model” of density. This is because the science teacher is supposedly confident in dealing with science practicals and less confident on abstract concepts. It is therefore not surprising that his longest sessions were spent on exploration with physical laboratory tools, least on mathematical analysis and did not do insight conjecture. To compensate for the time in the abstract domain, the teacher resorted to giving learners a written exercise.

During lesson delivery, the science teacher exhibited high contingency skills when he spontaneously provided alternative activities to overcome learner’s confusion on size of containers versus volume of contents. Contingency is a vital iSTEM skill (Leung, 2018), especially during exploration when learners can come up with different perspectives of interpreting a problem situation and the teacher should be ready to scaffold them accordingly. Knowing how to organize and manage the classroom during modeling activities (Blum, 2015), the ability to provide strategic interventions and interpretive listening and responding to students’ thinking (Doerr and English, 2006; Doerr and Lesh, 2011) and the ability to recognize the unexpected ways of thinking and developing strategies to cope with crisis situations (Doerr, 2007), are some of the key competencies that a teacher should have in order to implement modeling activities successfully in teaching and learning of mathematics. Contingency goes hand in hand with tools. Resources can influence the teacher’s pedagogical choices (Leung, 2018). Research has also shown that teacher’s efficacy is dependent on the tools at their disposal (Carnine, 1992). During interviews, the science teacher stated that he was able to provide an immediate way to solve students’ misunderstandings because he knew the available laboratory equipment and the exact position they would be found in the laboratory.

In this research, the mathematics teacher exhibited characteristics of an analytical teacher. An analytic thinker can comprehend and express mathematical facts preferably through symbolic or verbal representations and show preferences for a more step-by-step procedure when solving given problems (Blum and Ferri, 2009, p. 50). During lessons 1 and 2, the teacher was seen leading the class step by step following the worksheet procedure in a bid to minimize errors and misconceptions. The teacher’s integration “model” also has a significant percentile time distribution of teaching on the abstract concepts of density for example 35% mathematical model and 15% mathematical analysis as compared to 21 and 4%, respectively, by the science teacher. The teacher’s strength in the mathematical knowledge domain (Blum, 2015), could be a reflection of how the teacher was trained and her experiences in teaching mathematics. In Zimbabwe, traditional mathematics teaching mostly involves solving given equations or mathematical word problems and it rarely involve doing experiments or model eliciting activities. Again, the teacher considered the density equation: D = M/V as the model that was developed during the lessons, reflecting her bias toward thinking in the abstract, versus the set of real containers that was mentioned by the science teacher as the density model.

Tools and physical space seemed to have caused the mathematics teacher to feel alienated during the initial stages of the research. This revealed her lack of confidence in the integration process because of the stated factors, hence her stance in taking a supporting role during the first data collection cycle. In Zimbabwe, and most other countries, the science department has its own specialized buildings/laboratories, and it is mostly separated physically from the mathematics department. The physical separation of departments could be a source of conflict that threaten the prospects of integration (Ball, 1987, p. 42). Research revealed that elimination of physical barriers can support collaboration hence the call to group the science and mathematics faculty (Hart et al., 1982; Wong and Dillon, 2020). On the other hand, the significance of tools is also seen when the mathematics teacher suggested that in future, she may use sports balls to introduce the concept of density claiming that the idea come because she works from a school sports office where she is surrounded by these balls and that the students are more familiar with sports balls than with ships.

The team-teaching model proved to be challenging, possibly due to teachers’ concerns about being compared by students or their lack of time and effort to establish a mutually respectful working relationship. This relationship requires extensive planning, time investment, and coordination (see Jang, 2006). Adoption of the One-Teaching-One Assisting model is in line with Liu (2008) proposal. Liu suggested that collaboration should be done sequentially, starting with that for teachers with little collaboration experience, one-teaching-one assisting followed by alternative teaching, then station teaching and finally team teaching. Sequential adoption ensures that teachers can gradually build up their skills and experience in collaborative teaching. The sentiments have also been echoed by Wong and Dillon (2020) who argue that collaboration is neither straightforward to begin nor to sustain, hence the need to be vigilant especially when planning for a high order collaboration strategy like that of implementing iSTEM. One teach/one assist was advantageous in that it allowed the lead teacher to teach without frequent interruption from individual students who were getting instant help from the assisting teacher. In most cases, the assisting teacher was close-by to offer help in real-time when necessary. The proximity of the assisting teacher to every student when s/he walks around during lesson helped to keep the students on task, aiding classroom management especially in large classes, a common scenario in most schools in Zimbabwe. Additionally, one-teach-one-assist boosted the mathematics teacher’s confidence and she claimed to have learned from the first lessons by the science teacher. The mathematics teacher’s lessons had the mathematics and science content more integrated.

It was not surprising that the science teacher had low motivation of infusing engineering and technology in his future lesson considering that teachers have low levels of confidence for integrating technology and engineering (Smith et al., 2015) and technology is sometimes regarded as the hardest discipline to integrate (Wang et al., 2011) while engineering is usually the least mentioned discipline to be integrated with science (el-Deghaidy et al., 2017). The fact that the mathematics teacher seems to have gained much more than the science teacher, for example, the teacher managed to lead more integrated lessons and she suggested several ways to enhance the lessons to include engineering and technology, is an important direction for further studies to determine factors that could have led to the difference. This is also despite the fact that initially the mathematics teacher had low confidence and belief in iSTEM. Both teachers saw the research lesson as an insight for the design of Continuous Assessment Learning Activities (CALA) assignments. CALA is a national examination requirement that mandates all primary and secondary school candidates in Zimbabwe to develop a long-term project (maximum 6 months) or design an artifact that is aligned with their area of study.

Initially, the mathematics teacher had a negative perception toward the possibility of a balanced integration of mathematics and science. This made her feel alienated to work in a science environment hence she took a less active role during the first lesson. The teacher pictured a Science-centred mathematics-assisted integration (SCMAI) (Tekerek et al., 2023) integration model as the best possible outcome. This signify the strong influence of contextual and teacher related factors (see Dong et al., 2019; Fang and Fan, 2023; Tekerek et al., 2023) on the integration model and the success of implementation. Further, the research has demonstrated that giving teachers the opportunity to experience the iSTEM practices themselves has a significant impact on the teacher’s role in the implementation of new educational programs. For example, the mathematics teacher pictured an interdisciplinary integration model (Vasquez et al., 2013)/ total integration (TI) model (Tekerek et al., 2023) as feasible after she took an active role in implementing the lesson. It is well documented that the most effective professional learning experiences for teachers or the best way to prepare teachers to implement new instructional principles is to immerse them in the respective instructional principles themselves (Rhoton and Stiles, 2002; Loucks-Horsley et al., 2009), hence the change of perception of the mathematics teacher after she took the leading role in lesson delivery.

6 Limitations

Even though the teachers had the support of researchers and had the opportunity to share amongst themselves for a week before lesson delivery started, the time was limited in order for them to gain an in depth understanding of the pedagogical content knowledge of the other subject and how they could possibly connect (Shulman and Sherin, 2004; Czerniak and Johnson, 2014). The authors could have also provided integrated curriculum resources (Czerniak and Johnson, 2014), which could have helped to boost the teacher’s efficacy and the ability to seamlessly cross the boundary between mathematics and science concepts when teaching.

7 Conclusion

This paper explored how a science and a mathematics teacher can integrate mathematics and science concepts when teaching a common topic like density at classroom level and the iSTEM teacher practices that are essential for that purpose. The paper concludes that teachers are able to integrate mathematics and science concepts when teaching a common topic like density. Results have demonstrated the strong synergy between the 5E inquiry and mathematical modeling, findings which are similar to those reported by Leung (2018). Therefore, results provide the support necessary for putting the theory into classroom practices. The integration was made possible through teacher collaboration and persistent exposure to the integration practices. The teachers gained from each other knowledge of the other subject, confidence, and assistance in managing the students especially during the practical activities. Through full immersion in the two LS cycles, the teachers effectively enhanced their practice, leading to notable improvements such as the enhanced integration of content. The level of content integration was also influenced by the teacher’s subject of specialty wherein a science teacher was more leaned toward the real world and manipulation of materials whilst the mathematics teacher was biased toward the mathematics domain, dealing with abstract concepts. In the end, teachers suggested ways to infuse engineering and technology when teaching the same topic, and this can form the basis for further studies to explore how the integration of the full STEM domains can empirically be demonstrated at classroom level. The deliberate attempt by mathematics and science teachers to collaborate provide rich feedback on attempts to integrate STEM teaching across subjects. The big question would be whether such collaboration should happen across more topics in the syllabuses, and if so, how this could potentially be scaled across the whole school in a feasible way without losing the intended benefits. Further, this study revealed that in order to successfully implement a high quality iSTEM program, it is also crucial that the teachers have an in-depth understanding of a problem and its contextualization for it determines the whole lesson trajectory. By immersing teachers in interdisciplinary instructional practices and providing opportunities for experiential learning, educators can enhance their capacity to implement innovative and integrated STEM programs effectively.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Ethics statement

The studies involving humans were approved by Research Ethics Committee (REC): Hong Kong Baptist University. The studies were conducted in accordance with the local legislation and institutional requirements. The participants provided their written informed consent to participate in this study.

Author contributions

KM: Conceptualization, Data curation, Formal analysis, Investigation, Methodology, Visualization, Writing – original draft, Writing – review & editing. AL: Supervision, Visualization, Writing – review & editing.

Funding

The author(s) declare that no financial support was received for the research, authorship, and/or publication of this article.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/feduc.2024.1376951/full#supplementary-material

References

Adu, A., and Folson, D. (2023). Effectiveness of 5E instructional model on students’ performance in mathematics non-routine problem. Asian J. Adv. Res. Rep 17, 22–29. doi: 10.9734/ajarr/2023/v17i5482

Crossref Full Text | Google Scholar

Akkerman, S. F., and Bakker, A. (2011). Boundary crossing and boundary objects. Rev. Educ. Res. 81, 132–169. doi: 10.3102/0034654311404435

PubMed Abstract | Crossref Full Text | Google Scholar

Al Salami, M. K., Makela, C. J., and De Miranda, M. A. (2017). Assessing changes in teachers’ attitudes toward interdisciplinary STEM teaching. Int. J. Technol. Des. Educ. 27, 63–88. doi: 10.1007/s10798-015-9341-0

Crossref Full Text | Google Scholar

Alabdulaziz, M. S. (2024). Designing a heuristic based on flipped classroom approaches aligned with the 5E instructional model to teach mathematics. Kurdish Stud. 12, 3456–3474. doi: 10.58262/ks.v12i2.256

Crossref Full Text | Google Scholar

Artigue, M., and Blomhøj, M. (2013). Conceptualizing inquiry-based education in mathematics. ZDM 45, 797–810. doi: 10.1007/s11858-013-0506-6

PubMed Abstract | Crossref Full Text | Google Scholar

Asempapa, R. S. (2015). Mathematical modeling: essential for elementary and middle school students. J. Math. Educ. 8, 16–29.

Google Scholar

Bakri, S. (2021). Effect of 5E learning model on academic achievement in teaching mathematics: meta-analysis study. Turkish J. Comput. Math. Educ. 12, 196–204. doi: 10.17762/TURCOMAT.V12I8.2783

Crossref Full Text | Google Scholar

Ball, S. (1987) The micropolitics of the school. London: Routledge.

Google Scholar

Barak, M. (2012). Teaching engineering and technology: cognitive, knowledge and problem-solving taxonomies. J. Engin. Design Technol. 11, 316–333. doi: 10.1108/JEDT-04-2012-0020

Crossref Full Text | Google Scholar

Batdi, V., Talan, T., and Semerci, C. (2019). Meta-analytic and meta-thematic analysis of STEM education. Int. J. Educ. Math. Sci. Technol. 7, 382–399.

Google Scholar

Berlin, D., and White, A. (1999) Mathematics and science together: establishing the relationship for the 21st century classroom, In International conference on mathematics into the 21st century: Societal challenges, issues, and approaches, 57–62

Google Scholar

Berlin, D. F., and White, A. L. (2012). A longitudinal look at attitudes and perceptions related to the integration of mathematics, science, and technology education. Sch. Sci. Math. 112, 20–30. doi: 10.1111/j.1949-8594.2011.00111.x

Crossref Full Text | Google Scholar

Blum, W. (1995) Applications and modelling in mathematics teaching and mathematics education - some important aspects of practice and of research: Advances and perspectives in the teaching of mathematical modelling and applications. Yorklyn: Water Street Mathematics Yorklyn

Google Scholar

Blum, W. (2015) Quality teaching of mathematical modelling: what do we know, what can we do?, In The proceedings of the 12th international congress on mathematical education: Intellectual and attitudinal challenges, Seoul, Korea: Springer International Publishing, 73–96

Google Scholar

Blum, W., and Ferri, R. B. (2009). Mathematical modelling: can it be taught and learnt? J. Math. Model. Appl. 1, 45–58.

Google Scholar

Boudreaux, A., Shaffer, P. S., Heron, P. R. L., and McDermott, L. C. (2008). Student understanding of control of variables: deciding whether or not a variable influences the behavior of a system. Am. J. Phys. 76, 163–170. doi: 10.1119/1.2805235

PubMed Abstract | Crossref Full Text | Google Scholar

Braskén, M., Hemmi, K., and Kurtén, B. (2020). Implementing a multidisciplinary curriculum in a Finnish lower secondary school – the perspective of science and mathematics. Scand. J. Educ. Res. 64, 852–868. doi: 10.1080/00313831.2019.1623311

Crossref Full Text | Google Scholar

Brown, J.S., Collins, A., and Duguid, P. (1989) Situated cognition and the culture of learning. Educational Researcher, 18, 32–42.

Google Scholar

Bunterm, T., Lee, K., Ng Lan Kong, J., Srikoon, S., Vangpoomyai, P., Rattanavongsa, J., et al. (2014). Do different levels of inquiry Lead to different learning outcomes? A comparison between guided and structured inquiry. Int. J. Sci. Educ. 36, 1937–1959. doi: 10.1080/09500693.2014.886347

PubMed Abstract | Crossref Full Text | Google Scholar

Bybee, R. W., Taylor, J. A., Gardner, A., Van Scotter, P., Powell, J. C., Westbrook, A., et al. (2006) The BSCS 5E instructional model: origins and effectiveness. Colorado: Colorado Springs: Office of Science Education National Institutes of Health, 1–43

Google Scholar

Carnine, D. (1992). Expanding the notion of teachers’ rights: access to tools that work. J. Appl. Behav. Anal. 25, 13–19. doi: 10.1901/jaba.1992.25-13

PubMed Abstract | Crossref Full Text | Google Scholar

Carreira, S., and Baioa, A. M. (2018). Mathematical modelling with hands-on experimental tasks: on the student’s sense of credibility. ZDM 50, 201–215. doi: 10.1007/s11858-017-0905-1

Crossref Full Text | Google Scholar

Clark, A. C., and Ernst, J. V. (2006). A model for the integration of science, technology, engineering, and mathematics. Technol. Engin. Teacher 66:24.

Google Scholar

Corlu, M. S., Capraro, R. M., and Capraro, M. M. (2014). Introducing STEM education: implications for educating our teachers for the age of innovation. Educ. Sci. 39, 74–85.

Google Scholar

Czerniak, C. M., and Johnson, C. C. (2007). “Interdisciplinary science teaching” in Handbook of Research on Science Education. eds. S. K. Abell and N. G. Lederman, vol. II (New York: Routledge), 537–560.

Google Scholar

Czerniak, C. M., and Johnson, C. C. (2014). “Interdisciplinary science teaching” in Handbook of research on science education. eds. S. K. Abell and N. G. Lederman. 2nd ed (London and New York: Routledge), 395–411.

Google Scholar

Dare, E. A., Ellis, J. A., and Roehrig, G. H. (2018). Understanding science teachers’ implementations of integrated STEM curricular units through a phenomenological multiple case study. Int. J. STEM Educ. 5, 1–19. doi: 10.1186/s40594-018-0101-z

Crossref Full Text | Google Scholar

Davison, D. M., Miller, K. W., and Metheny, D. L. (1995). What does integration of science and mathematics really mean? Sch. Sci. Math. 95, 226–230. doi: 10.1111/j.1949-8594.1995.tb15771.x

PubMed Abstract | Crossref Full Text | Google Scholar

Dawkins, K. R., Dickerson, D. L., McKinney, S. E., and Butler, S. (2008). Teaching density to middle school students: preservice science teachers' content knowledge and pedagogical practices. Clearing House 82, 21–26. doi: 10.3200/TCHS.82.1.21-26

Crossref Full Text | Google Scholar

De Loof, H., Boeve-de Pauw, J., and Van Petegem, P. (2022). Integrated STEM education: the effects of a long-term intervention on students. Eur. J. STEM Educ. 7:13. doi: 10.20897/ejsteme/12738

Crossref Full Text | Google Scholar

Doerr, H. M. (2007). “What knowledge do teachers need for teaching mathematics through applications and modelling?” in Modelling and applications in mathematics education: The 14th ICMI study. eds. W. Blum, P. L. Galbraith, H. W. Henn, and M. Niss (Boston, MA: Springer), 69–78.

Google Scholar

Doerr, H. M., and English, L. D. (2006). Middle grade teachers’ learning through students’ engagement with modeling tasks. J. Math. Teach. Educ. 9, 5–32. doi: 10.1007/s10857-006-9004-x

PubMed Abstract | Crossref Full Text | Google Scholar

Doerr, H. M., and Lesh, R. (2011). “Models and modelling perspectives on teaching and learning mathematics in the twenty-first century” in Trends in teaching and learning of mathematical modelling: ICTMA14. eds. G. Kaiser, W. Blum, R. B. Ferri, and G. Stillman (New York: Springer), 247–268.

Google Scholar

Dole, S., Hilton, G., Hilton, A., and Goos, M. (2013). Considering density through a numeracy lens: implications for science teaching, In International Conference New Perspectives in Science Education, Florence, 14–15.

Google Scholar

Dong, Y., Xu, C., Song, X., Fu, Q., Chai, C. S., and Huang, Y. (2019). Exploring the effects of contextual factors on in-service teachers’ engagement in STEM teaching. Colonial Office J. 28, 25–34. doi: 10.1007/s40299-018-0407-0

Crossref Full Text | Google Scholar

Dorier, J.-L., and Maass, K. (2020). Inquiry-based mathematics education. Encycl. Math. Educ. 384–388. doi: 10.1007/978-3-030-15789-0_176

Crossref Full Text | Google Scholar

el-Deghaidy, H., Mansour, N., Alzaghibi, M., and Alhammad, K. (2017). Context of STEM integration in schools: views from in-service science teachers. Eurasia J. Math. Sci. Technol. Educ. 13, 2459–2484. doi: 10.12973/eurasia.2017.01235a

Crossref Full Text | Google Scholar

English, L. D. (2016). STEM education K-12: perspectives on integration. Int. J. STEM Educ. 3, 1–8. doi: 10.1186/s40594-016-0036-1

Crossref Full Text | Google Scholar

Fang, S.-C., and Fan, S.-C. (2023). ‘Exploring teachers’ conceptions and implementations of STEM integration at the junior secondary level in Taiwan: an interview study. Int. J. Sci. Math. Educ. 21, 2095–2121. doi: 10.1007/s10763-022-10335-w

Crossref Full Text | Google Scholar

Felix, A., and Harris, J. (2010). A project-based, STEM-integrated alternative energy team challenge for teachers. Technol. Engin. Teach. 69:29.

Google Scholar

France, B. (2017) Modeling in technology education: a route to technological literacy. In M. J. VriesDe (Ed.), in Handbook of technology education. Netherlands: Springer.

Google Scholar

Frykholm, J., and Glasson, G. (2005). Connecting science and mathematics instruction: pedagogical context knowledge for teachers. Sch. Sci. Math. 105:127. doi: 10.1111/j.1949-8594.2005.tb18047.x

Crossref Full Text | Google Scholar

Gardner, K., Glassmeyer, D., and Worthy, R. (2019). Impacts of STEM professional development on teachers’ knowledge, self-efficacy, and practice. Front. Educ. 4:26. doi: 10.3389/feduc.2019.00026

Crossref Full Text | Google Scholar

Garfunkel, S., and Montgomery, M. (2019). GAIMME—guidelines for assessment & instruction in mathematical modeling education. SIAM. 7–70. doi: 10.1137/1.9781611975741

Crossref Full Text | Google Scholar

Gibson, K. (2022). Bridging the digital divide: reflections on using WhatsApp instant messenger interviews in youth research. Qual. Res. Psychol. 19, 611–631. doi: 10.1080/14780887.2020.1751902

Crossref Full Text | Google Scholar

Gilbert, J. K. (2004). Models and modelling: routes to more authentic science education. Int. J. Sci. Math. Educ. 2, 115–130. doi: 10.1007/s10763-004-3186-4

PubMed Abstract | Crossref Full Text | Google Scholar

Hallström, J., and Schönborn, K. J. (2019). Models and modelling for authentic STEM education: reinforcing the argument. Int. J. STEM Educ. 6, 1–10. doi: 10.1186/s40594-019-0178-z

Crossref Full Text | Google Scholar

Hammer, D. (1996). More than misconceptions: multiple perspectives on student knowledge and reasoning, and an appropriate role for education research. Am. J. Phys. 64, 1316–1325. doi: 10.1119/1.18376

Crossref Full Text | Google Scholar

Han, S., Capraro, R., and Capraro, M. M. (2015). How science, technology, engineering, and mathematics (STEM) project-based learning (PBL) affects high, middle, and low achievers differently: the impact of student factors on achievement. Int. J. Sci. Math. Educ. 13, 1089–1113. doi: 10.1007/s10763-014-9526-0

Crossref Full Text | Google Scholar

Hart, K., Turner, A., and Booth, L. (1982). Mathematics-science links in the secondary school: collaboration between mathematics and science departments: case studies of four schools: part 2. Math. Sch. 11, 10–12.

Google Scholar

Herro, D., and Quigley, C. (2017). Exploring teachers’ perceptions of STEAM teaching through professional development: implications for teacher educators. Prof. Dev. Educ. 43, 416–438. doi: 10.1080/19415257.2016.1205507

Crossref Full Text | Google Scholar

Honey, M., Pearson, G., and Schweingruber, H. (2014). STEM integration in K-12 education: Status, prospects, and an agenda for research. USA (Washington, DC): National Academies Press, 180.

Google Scholar

Huri, N. H. D., and Karpudewan, M. (2019). Evaluating the effectiveness of integrated STEM-lab activities in improving secondary school students’ understanding of electrolysis. Chem. Educ. Res. Pract. 20, 495–508. doi: 10.1039/C9RP00021F

Crossref Full Text | Google Scholar

Jaakkola, T., and Nurmi, S. (2008). Fostering elementary school students’ understanding of simple electricity by combining simulation and laboratory activities. J. Comput. Assist. Learn. 24, 271–283. doi: 10.1111/j.1365-2729.2007.00259.x

Crossref Full Text | Google Scholar

Jacobs, H. H. (1989). Interdisciplinary curriculum: design and implementation. United States: ERIC.

Google Scholar

Jang, S.-J. (2006). Research on the effects of team teaching upon two secondary school teachers. Educ. Res. 48, 177–194. doi: 10.1080/00131880600732272

Crossref Full Text | Google Scholar

Jenner, B., Flick, U., von Kardoff, E., and Steinke, I. (2004). A companion to qualitative research. London: Sage.

Google Scholar

Jerrim, J., and Shure, N. (2016) Achievement of 15-year-olds in Wales: PISA 2015 National Report. 35–61.

Google Scholar

Johnston, J., Walshe, G., and Ríordáin, M. N. (2019). Supporting key aspects of practice in making mathematics explicit in science lessons. Int. J. Sci. Math. Educ. 18, 1399–1417. doi: 10.1007/s10763-019-10016-1

Crossref Full Text | Google Scholar

Joswick, C., and Hulings, M. (2023). A systematic review of BSCS 5E instructional model evidence. Int. J. Sci. Math. Educ. :22. doi: 10.1007/s10763-023-10357-y

Crossref Full Text | Google Scholar

Justi, R., and Van Driel, J. (2005). The development of science teachers’ knowledge on models and modelling: promoting, characterizing, and understanding the process. Int. J. Sci. Educ. 27, 549–573. doi: 10.1080/0950069042000323773

Crossref Full Text | Google Scholar

Kelley, T. R., and Knowles, J. G. (2016). A conceptual framework for integrated STEM education. Int. J. STEM Educ. 3, 1–11. doi: 10.1186/s40594-016-0046-z

Crossref Full Text | Google Scholar

Kertil, M., and Gurel, C. (2016). Mathematical modeling: a bridge to STEM education. Int. J. Educ. Math. Sci. Technol. 4, 44–55. doi: 10.18404/ijemst.95761

PubMed Abstract | Crossref Full Text | Google Scholar

Kieran, C., Krainer, K., and Shaughnessy, J. M. (2012). “Linking research to practice: teachers as key stakeholders in mathematics education research” in Third international handbook of mathematics education. eds. M. A. (Ken) Clements, A. J. Bishop, C. Keitel, J. Kilpatrick, and F. K. S. Leung (New York: Springer), 361–392.

Google Scholar

Kim, M. C., Hannafin, M. J., and Bryan, L. A. (2007). Technology-enhanced inquiry tools in science education: an emerging pedagogical framework for classroom practice. Sci. Educ. 91, 1010–1030. doi: 10.1002/sce.20219

Crossref Full Text | Google Scholar

Kiray, S. A. (2012). A new model for the integration of science and mathematics: the balance model. Soc. Educ. Stud. 4, 1181–1196.

Google Scholar

Kiray, S. A., and Simsek, S. (2021). Determination and evaluation of the science teacher candidates’ misconceptions about density by using four-tier diagnostic test. Int. J. Sci. Math. Educ. 19, 935–955. doi: 10.1007/s10763-020-10087-5

Crossref Full Text | Google Scholar

Kiray, S. A., Aktan, F., Kaynar, H., Kilinc, S., and Gorkemli, T. (2015). A descriptive study of pre-service science teachers’ misconceptions about sinking–floating. Asia Pacific Forum Sci. Learn. Teach. 16, 1–28.

Google Scholar

Kloser, M., Wilsey, M., Twohy, K. E., Immonen, A. D., and Navotas, A. C. (2018). “We do STEM”: unsettled conceptions of STEM education in middle school STEM classrooms. Sch. Sci. Math. 118, 335–347. doi: 10.1111/ssm.12304

Crossref Full Text | Google Scholar

Kluth, P., and Straut, D. (2003). Do as we say and as we do: teaching and modeling collaborative practice in the university classroom. J. Teach. Educ. 54, 228–240. doi: 10.1177/0022487103054003005

Crossref Full Text | Google Scholar

Laboy-Rush, D. (2011) Integrated STEM education through project-based learning. Available at: http://www.rondout.k12.ny.us/common/pages/DisplayFile.aspx?itemId:p16466975

Google Scholar

Larsen, D.M., and Østergaard, C.H. (2023) Two inquiry approaches to STEM: the role of mathematics, In Thirteenth congress of the European Society for Research in mathematics education (CERME13), Alfréd Rényi Institute of Mathematics; ERME

Google Scholar

Le, H. C., Nguyen, V. H., and Nguyen, T. L. (2023). Integrated STEM approaches and associated outcomes of K-12 student learning: a systematic review. Educ. Sci. 13:297. doi: 10.3390/educsci13030297

Crossref Full Text | Google Scholar

Lederman, N. G., and Niess, M. L. (1997). Integrated, interdisciplinary, or thematic instruction? Is this a question or is it questionable semantics? Sch. Sci. Math. 97:57. doi: 10.1111/j.1949-8594.1997.tb17342.x

PubMed Abstract | Crossref Full Text | Google Scholar

Lesh, R., and Doerr, H. M. (2003). Foundations of a models and modeling perspective on mathematics teaching, learning, and problem solving. Beyond Construct 17, 3–33.

Google Scholar

Leung, A. (2018). Exploring STEM pedagogy in the mathematics classroom: a tool-based experiment lesson on estimation. Int. J. Sci. Math. Educ. 20. doi: 10.1007/s10763-018-9924-9

Crossref Full Text | Google Scholar

Lewis, C. (2016). How does lesson study improve mathematics instruction? ZDM 48, 571–580. doi: 10.1007/s11858-016-0792-x

PubMed Abstract | Crossref Full Text | Google Scholar

Lewis, C.C., and Hurd, J. (2011) Lesson study step by step: How teacher learning communities improve instruction, Portsmouth: Heinemann

Google Scholar

Lin, P.-Y., Di, W., and Wang, X. (2022). Modeling Chinese teachers’ efficacies for the teaching of integrated STEM with interdisciplinary communication and epistemic fluency. Front. Psychol. 13:908421. doi: 10.3389/fpsyg.2022.1023808

PubMed Abstract | Crossref Full Text | Google Scholar

Liu, L. (2008). Co-teaching between native and non-native English teachers: an exploration of co-teaching models and strategies in the Chinese primary school context. Reflect. English Lang. Teach. 7, 103–118.

Google Scholar

Loucks-Horsley, S., Stiles, K. E., Mundry, S., Love, N., and Hewson, P. W. (2009). Designing professional development for teachers of science and mathematics. California, United States: Corwin Press.

Google Scholar

Lytle, A., and Shin, J. E. (2020). Incremental beliefs, STEM efficacy and STEM interest among first-year undergraduate students. J. Sci. Educ. Technol. 29, 272–281. doi: 10.1007/s10956-020-09813-z

Crossref Full Text | Google Scholar

Maab, J., and Schloglmann, W. (2009) Beliefs and attitudes in mathematics education, Austria: University of Linz

Google Scholar

Maclin, D., Grosslight, L., and Davis, H. (1997). Teaching for understanding: a study of students’ Preinstruction theories of matter and a comparison of the effectiveness of two approaches to teaching about matter and density. Cogn. Instr. 15, 317–393. doi: 10.1207/s1532690xci1503_2

Crossref Full Text | Google Scholar

Margot, K. C., and Kettler, T. (2019). Teachers’ perception of STEM integration and education: a systematic literature review. Int. J. STEM Educ. 6, 1–16. doi: 10.1186/s40594-018-0151-2

Crossref Full Text | Google Scholar

Mathis, C. A., Siverling, E. A., Moore, T. J., Douglas, K. A., and Guzey, S. S. (2018). Supporting engineering design ideas with science and mathematics: a case study of middle school life science students. Int. J. Educ. Math. Sci. Technol. 6, 424–442. doi: 10.18404/ijemst.440343

Crossref Full Text | Google Scholar

Mathis, C. A., Siverling, E. A., Glancy, A. W., Guzey, S. S., and Moore, T. J. (2016). Students’ use of evidence-based reasoning in K-12 engineering: a case study (fundamental), In 2016 ASEE Annual Conference & Exposition.

Google Scholar

McBride, J. W., and Silverman, F. L. (1991). Integrating elementary/middle school science and mathematics. Sch. Sci. Math. 91, 285–292.

Google Scholar

Moore, T., Self, B., Miller, R., Hjalmarson, M., Zawojewski, J., and Olds, B. (2010). Special session: model eliciting activities: a construct for better understanding student knowledge and skills, In 2010 Annual Conference & Exposition, 15–1085. doi: 10.18260/1-2—16662

Crossref Full Text | Google Scholar

Moore, T. J., Stohlmann, M. S., Wang, H. H., Tank, K. M., Glancy, A. W., and Roehrig, G. H. (2014). “Implementation and integration of engineering in K-12 STEM education” in Engineering in pre-college settings: Synthesizing research, policy, and practices. eds. S. Purzer, J. Strobel, and M. Cardella (West Lafayette: Purdue University Press).

Google Scholar

Morrison, J., and McDuffie, A. R. (2009). Connecting science and mathematics: using inquiry investigations to learn about data collection, analysis, and display. Sch. Sci. Math. 109, 31–44. doi: 10.1111/j.1949-8594.2009.tb17860.x

Crossref Full Text | Google Scholar

Murata, A. (2011). “Introduction: conceptual overview of lesson study” in Lesson study research and practice in mathematics education. eds. L. C. Hart, A. S. Alston, and A. Murata (Dordrecht: Springer Netherlands), 1–12. doi: 10.1007/978-90-481-9941-9_1

Crossref Full Text | Google Scholar

Murata, A., and Lee, C. K. (Eds.). (2021). Stepping up lesson study: An educator’s guide to deeper learning. New York: Routledge. 124.

Google Scholar

Niss, M. (2015). “Prescriptive modelling – challenges and opportunities” in Mathematical modelling in education research and practice. eds. G. A. Stillman, W. Blum, and M. Salett Biembengut [Cham: Springer International Publishing (International Perspectives on the Teaching and Learning of Mathematical Modelling)], 67–79.

Google Scholar

Nunes, T., Desli, D., and Bell, D. (2003). The development of children’s understanding of intensive quantities. Int. J. Educ. Res. 39, 651–675. doi: 10.1016/j.ijer.2004.10.002

PubMed Abstract | Crossref Full Text | Google Scholar

Opdenakker, R. (2006). “Advantages and disadvantages of four interview techniques in qualitative research” in Forum qualitative Sozialforschung = forum: qualitative social research. Eindhoven University of Technology, 11.

Google Scholar

Pheaukkhai, C., and Cheausuwantavee, C. (2023). Effects of the inquiry mathematics learning (5E) through real life situations on mathematical reasoning based on the Pisa assessment framework. Journal of MCU Nakhondhat 10, 323–331.

Google Scholar

Rhoton, J., and Stiles, K. E. (2002). Exploring the professional development design process: bringing an abstract framework into practice. Sci. Educ. 11, 1–8.

Google Scholar

Ríordáin, M. N., Johnston, J., and Walshe, G. (2016). Making mathematics and science integration happen: key aspects of practice. Int. J. Math. Educ. Sci. Technol. 47, 233–255. doi: 10.1080/0020739X.2015.1078001

Crossref Full Text | Google Scholar

Roberts, T., Maiorca, C., Jackson, C., and Mohr-Schroeder, M. (2022). Integrated STEM as problem-solving practices. Investig. Math. Learn. 14, 1–13. doi: 10.1080/19477503.2021.2024721

PubMed Abstract | Crossref Full Text | Google Scholar

Roehrig, G. H., Dare, E. A., Ellis, J. A., and Ring-Whalen, E. (2021). Beyond the basics: a detailed conceptual framework of integrated STEM. Discip. Interdiscip. Sci. Educ. Res. 3, 1–18. doi: 10.1186/s43031-021-00041-y

Crossref Full Text | Google Scholar

Roehrig, G. H., Moore, T. J., Wang, H. H., and Park, M. S. (2012). Is adding the E enough? Investigating the impact of K-12 engineering standards on the implementation of STEM integration. Sch. Sci. Math. 112, 31–44. doi: 10.1111/j.1949-8594.2011.00112.x

Crossref Full Text | Google Scholar

Sala Sebastià, G., Barquero, B., and Font, V. (2021). Inquiry and modeling for teaching mathematics in interdisciplinary contexts: how are they interrelated? Mathematics 9, 1–19. doi: 10.3390/math9151714

Crossref Full Text | Google Scholar

Sanders, M. E. (2008). Stem, stem education, stemmania. The Technology Teacher 68, 20–26.

Google Scholar

Satchwell, R. E., and Loepp, F. L. (2002). Designing and implementing an integrated mathematics, science, and technology curriculum for the middle school. J. Ind. Teach. Educ. 39, 41–66.

Google Scholar

Schmid, S., and Bogner, F. X. (2015). Effects of students’ effort scores in a structured inquiry unit on long-term recall abilities of content knowledge. Educ. Res. Int. 2015, 1–11. doi: 10.1155/2015/826734

Crossref Full Text | Google Scholar

Schoenfeld, A. H., and Kilpatrick, J. (2013). A US perspective on the implementation of inquiry-based learning in mathematics. ZDM 45, 901–909. doi: 10.1007/s11858-013-0531-5

Crossref Full Text | Google Scholar

Shulman, L. S., and Sherin, M. G. (2004). Fostering communities of teachers as learners: disciplinary perspectives. J. Curric. Stud. 36, 135–140. doi: 10.1080/0022027032000135049

PubMed Abstract | Crossref Full Text | Google Scholar

Singer, B., Walsh, C. M., Gondwe, L., Reynolds, K., Lawrence, E., and Kasiya, A. (2020). WhatsApp as a medium to collect qualitative data among adolescents: lessons learned and considerations for future use. Gates Open Res. 4:130. doi: 10.12688/gatesopenres.13169.2

Crossref Full Text | Google Scholar

Smith, K. L., Rayfield, J., and McKim, B. R. (2015). Effective practices in STEM integration: describing teacher perceptions and instructional method use. J. Agric. Educ. 56, 182–201. doi: 10.5032/jae.2015.04183

Crossref Full Text | Google Scholar

Spikic, S., van Passel, W., Deprez, H., and de Meester, J. (2023). Measuring and activating iSTEM key principles among student teachers in STEM. Educ. Sci. 13:12. doi: 10.3390/educsci13010012

Crossref Full Text | Google Scholar

Squires, L. (2010). Enregistering internet language. Lang. Soc. 39, 457–492. doi: 10.1017/S0047404510000412

Crossref Full Text | Google Scholar

Srikoom, W., Faikhamta, C., and Hanuscin, D. L. (2018). Dimensions of effective STEM integrated teaching practice. K-12 STEM education. IPST 4, 313–330.

Google Scholar

Sriraman, B., and English, L. D. (2010). Theories of mathematics education: Seeking new frontiers. German (Berlin): Springer.

Google Scholar

Stinson, K., Harkness, S. S., Meyer, H., and Stallworth, J. (2009). Mathematics and science integration: models and characterizations. Sch. Sci. Math. 109, 153–161. doi: 10.1111/j.1949-8594.2009.tb17951.x

PubMed Abstract | Crossref Full Text | Google Scholar

Stretch, E. J., and Roehrig, G. H. (2021). Framing failure: leveraging uncertainty to launch creativity in STEM education. Int. J. Learn. Teach. 7, 123–133.

Google Scholar

Sturges, J. E., and Hanrahan, K. J. (2004). Comparing telephone and face-to-face qualitative interviewing: a research note. Qual. Res. 4, 107–118. doi: 10.1177/1468794104041110

PubMed Abstract | Crossref Full Text | Google Scholar

Swetz, F., and Hartzler, J. S. (1991). Mathematical modeling in the secondary school curriculum. National Council of Teachers of Mathematics, USA: ERIC.

Google Scholar

Tank, K., Pleasants, J., and Olson, J. (2019) Elementary teachers’ attempts at integrating science and engineering over the course of a semester, In NARST international meeting, Baltimore, MD, March.

Google Scholar

Tati, T., Firman, H., and Riandi, R. (2017). The effect of STEM learning through the project of designing boat model toward student STEM literacy. J. Phys. Conf. Ser. 895, 4–9. doi: 10.1088/1742-6596/895/1/012157

Crossref Full Text | Google Scholar

Tekerek, B., Haser, Ç., and Işıksal-Bostan, M. (2023). What do teachers consider when they plan and implement science and mathematics integration? Int. J. Math. Educ. Sci. Technol. 54, 2046–2070. doi: 10.1080/0020739X.2022.2143446

Crossref Full Text | Google Scholar

Thibaut, L. (2018) Implementing integrated STEM: Teachers’ attitudes, instructional practices and students’ learning outcomes. KU LEUVEN. Available at: https://lirias.kuleuven.be/retrieve/517135

Google Scholar

Thibaut, L., Ceuppens, S., de Loof, H., de Meester, J., Goovaerts, L., Struyf, A., et al. (2018). Integrated STEM education: a systematic review of instructional practices in secondary education. Eur. J. STEM Educ. 3, 1–12. doi: 10.20897/ejsteme/85525

Crossref Full Text | Google Scholar

Thibaut, L., Knipprath, H., Dehaene, W., and Depaepe, F. (2019). Teachers’ attitudes toward teaching integrated STEM: the impact of personal background characteristics and school context. Int. J. Sci. Math. Educ. 17, 987–1007. doi: 10.1007/s10763-018-9898-7

Crossref Full Text | Google Scholar

Torres, N. V., and Santos, G. (2015). The (mathematical) modeling process in biosciences. Front. Genet. 6:169934. doi: 10.3389/fgene.2015.00354

PubMed Abstract | Crossref Full Text | Google Scholar

Tran, N. A., and Nathan, M. J. (2010). Pre-college engineering studies: an investigation of the relationship between pre-college engineering studies and student achievement in science and mathematics. J. Eng. Educ. 99, 143–157. doi: 10.1002/j.2168-9830.2010.tb01051.x

Crossref Full Text | Google Scholar

Tran, H., and Smith, D. A. (2021). How hard-to-staff rural school districts use state funds to address teacher shortages. J. Educ. Finance 47, 130–156.

Google Scholar

Treacy, P. (2021). A conceptual framework for integrating mathematics and science in the secondary classroom. SN Soc. Sci. 1, 1–17. doi: 10.1007/s43545-021-00166-x

Crossref Full Text | Google Scholar

Treagust, D. F., and Duit, R. (2008). Conceptual change: a discussion of theoretical, methodological and practical challenges for science education. Cult. Stud. Sci. Educ. 3, 297–328. doi: 10.1007/s11422-008-9090-4

Crossref Full Text | Google Scholar

Treiber, E., Neumann, I., and Heinze, A. (2023). What’s mathematics doing here? The role of mathematics in German physics Olympiad tasks. Front. Educ. 8:11. doi: 10.3389/feduc.2023.1196189

Crossref Full Text | Google Scholar

Tuna, A., and Kacar, A. (2013). The effect of 5E learning cycle model in teaching trigonometry on students’ academic achievement and the permanence of their knowledge. Int. J. New Trends Educ. Implic. 4, 73–87.

Google Scholar

Ultay, N., Zıvalı, A., Yılmaz, H., Bak, H. K., Yılmaz, K., Topatan, M., et al. (2020). STEM-focused activities to support student learning in primary school science. J. Sci. Learn. 3, 156–164. doi: 10.17509/jsl.v3i3.23705

Crossref Full Text | Google Scholar

Vasquez, J., Schneider, C., and Comer, M. (2013) STEM lesson essentials, grades 3–8: Integrating science, technology, engineering, and mathematics, Portsmouth, NH: Heinemann

Google Scholar

Vogl, S. (2013). Telephone versus face-to-face interviews: mode effect on semistructured interviews with children. Sociol. Methodol. 43, 133–177. doi: 10.1177/0081175012465967

Crossref Full Text | Google Scholar

Wang, W., Fergola, P., Lombardo, S., and Mulone, G. (2006). Mathematical models of innovation diffusion with stage structure. Appl. Math. Model. 30, 129–146. doi: 10.1016/j.apm.2005.03.011

PubMed Abstract | Crossref Full Text | Google Scholar

Wang, J.-R., Wang, Y. C., Tai, H. J., and Chen, W. J. (2010). Investigating the effectiveness of inquiry-based instruction on students with different prior knowledge and reading abilities. Int. J. Sci. Math. Educ. 8, 801–820. doi: 10.1007/s10763-009-9186-7

Crossref Full Text | Google Scholar

Wang, H. H., Moore, T. J., Roehrig, G. H., and Park, M. S. (2011). STEM integration: teacher perceptions and practice. J-PEER 1, 1–15. doi: 10.5703/1288284314636

Crossref Full Text | Google Scholar

Watkins, J., and Mazur, E. (2013). Retaining students in science, technology, engineering, and mathematics (STEM) majors. J. Coll. Sci. Teach. 42, 36–41.

Google Scholar

Wong, V., and Dillon, J. (2020). Crossing the boundaries: collaborations between mathematics and science departments in English secondary (high) schools. Res. Sci. Technol. Educ. 38, 396–416. doi: 10.1080/02635143.2019.1636024

Crossref Full Text | Google Scholar

Yeend, R.E., Loverude, M.E., and Gonzalez, B.L. (2001) Student understanding of density: a cross-age investigation, in 2001 Physics Education Research Conference Proceedings. 2001 Physics Education Research Conference

Google Scholar

Zbiek, R. M., Peters, S. A., Galluzzo, B., and White, S. J. (2024). Secondary mathematics teachers learning to do and teach mathematical modeling: a trajectory. J. Math. Teach. Educ. 27, 55–83. doi: 10.1007/s10857-022-09550-7

Crossref Full Text | Google Scholar

Zenger, T., and Bitzenbauer, P. (2022). Exploring German secondary school students’ conceptual knowledge of density. Sci. Educ. Int. 33, 86–92. doi: 10.33828/sei.v33.i1.9

Crossref Full Text | Google Scholar

Zhang, D., Orrill, C., and Campbell, T. (2015). Using the mixture Rasch model to explore knowledge resources students invoke in mathematic and science assessments. Sch. Sci. Math. 115, 356–365. doi: 10.1111/ssm.12135

Crossref Full Text | Google Scholar

Zion, M., and Mendelovici, R. (2012). Moving from structured to open inquiry: challenges and limits. Sci. Educ. Int. 23, 383–399.

Google Scholar

Zoupidis, A., Pnevmatikos, D., Spyrtou, A., and Kariotoglou, P. (2016). The impact of procedural and epistemological knowledge on conceptual understanding: the case of density and floating–sinking phenomena. Instr. Sci. 44, 315–334. doi: 10.1007/s11251-016-9375-z

Crossref Full Text | Google Scholar