José L. Rodríguez- Department of Mathematics, University of Almería, Almería, Spain
Virtual reality (VR) enables the creation of immersive and interactive learning environments for students and teachers. This article reports on an exploratory teaching practice conducted with pre-service secondary school teachers using Neotrie, a dynamic geometry software in virtual reality. In small groups, future teachers must learn how to use the software and design a didactic sequence to bring to the classroom. Following a research-action methodology, through this experience it is reported both the advantages and difficulties encountered when starting to use VR to design didactic sequences, as well as when learning a VR sandbox software with interactive tools, like Neotrie. A proposal for assessing the sequences under the premises of the TPaCK model is also included.
1 Introduction
There are currently new technologies that allow us to solve some of society's challenges and entertainment in a completely new way.1 Martín-Gutiérrez et al. (2017) state that “the huge possibilities of accessible virtual technologies will make it possible to break the boundaries of formal education”. In particular, the immersive experience offered by virtual reality in the classroom is highly engaging and motivating for students, with proven advantages in numerous previous experiences and research (Perri et al., 2021; Yünkül, 2022). This is, ultimately, the most important thing and what encourages teachers to dare to implement these new tools and methods in the classroom. Virtual reality “represents a significant opportunity for students to enhance their visual thinking skills through the provision of rich visualizations in both physical and virtual environments” (Bermejo et al., 2023; Cevikbas et al., 2023). It also allows students to see how abstract concepts work in a three-dimensional environment, which facilitates their understanding and retention. In the case of 3D geometry, the use of NeoTrie VR (briefly Neotrie)2 for learning mathematical geometrical concepts leads to better learning outcomes as shown by Rodríguez et al. (2021); see also Su et al. (2022) and Thomsen (2023) for a more recent exploration of the use of VR in mathematics education.
However, as remarked by Cevikbas et al. (2023), current classroom teachers find it complicated to implement virtual reality in the classroom, due to technological failures, cost, initial effort, health issues, and lack of awareness of AR/VR [see also Lai and Cheong (2022) and letter of concern3]. They are not sure of the effectiveness of the teaching and learning processes that are carried out with this new technology and, of course, they foresee the technical complications they will encounter. That is why it is necessary from educational institutions or companies specialized in education and ICT to facilitate real access to these new technologies, providing introductory courses and training.
Fernandez (2017) proposes a 12–15 months methodological process to aid the adoption of these technologies as basic elements within regular education. At that time, the high-quality VR devices available were HTC Vive, Windows Mixed Reality, Oculus Rift, etc., all requiring a gaming computer, at a high cost to schools. Moreover, this long process is intended to generate a limited and restricted solution to concrete learning experiences. It normally produces software with pre-set activities and challenges.
Totally immersive dynamical geometry environments, such us Neotrie, allows users to build their own mathematical activities in VR, although they normally require time and a good mathematical background to take full advantage of the software's capabilities.
Concerning the hardware, the Meta Quest4 is the most affordable standalone (i.e., without a gaming computer) VR system for schools. However, for the proper functioning of a group activity or monitoring by the teacher one must view on an external screen what the player is doing in the game. In order to optimize this experience it is recommended using tablets to casting what the player is seeing. A router Wi-Fi 6 as well to expand the bandwidth (which schools do not normally have) to be able to transmit on non-gaming computers or tablets, via the website http://www.oculus.com/casting or the Meta app directly on the tablet. In some cases, the teachers have opted to use their own personal mobile phones (with unlimited data) to create a local Wi-Fi network, better than the one provided by the school. This technical part of equipment start-up and casting outages is still a waste of time that needs to be improved.
Based on the previous considerations, the following research question is posed:
Is it possible for trainee teachers to produce appropriate activities in Neotrie, in a relatively short time?
A standard Lewin's Action Research methodology (plan, act, observe, reflect) is being employed to investigate and apply the best possible introduction of VR and the use of Neotrie in the master's classroom to produce teaching material, adjusting to time and knowledge constraints (cf. Moral-Sánchez et al., 2022).
In Section 2, the software Neotrie is discussed briefly, including some references to research conducted to date and the new focus of this paper. Section 3 describes the pedagogical guidelines set in our university to plan the new intervention using Neotrie, as well as the assessment informed by the Technological, Pedagogical, and Content Knowledge (TPaCK) framework. The learning environment is then described in Section 4, explaining how the intervention is carried out. The objectives of the intervention are also given, allowing a clear organization of the work to be done by the pre-service teachers in small groups. In Section 5, the observation of our research is done mainly from the didactic sequences provided. On them, a system of objective data and parameters that help to score the sequences is established. As an example, one of the delivered sequences is resumed and analyzed in detail. This yields to draw some preliminary conclusions in Section 5.3. Finally, some discussions answering the posed question are in Section 6, including some adjustments to the implementation plan in subsequent iterations.
2 The virtual reality software Neotrie
Neotrie is a virtual reality software that enables users to create, interact, and manipulate geometrical objects. The available tools allow teachers to deal with many Geometry topics, especially three-dimensional ones, at educational levels ranging from primary school to first years of university. Some of these topics deal with graphs, polyhedra, tessellations, fractals, curves and surfaces, as well as their properties and transformations (see more details at https://www2.ual.es/neotrie/project-neotrie/).
The software has been developed in the video-game engine Unity by the spin-off Virtual Dor of the University of Almería since 2018, updating it to the high quality VR devices on the market over the last years, and more recently on the Meta Quest headsets. These constant updates produce unwanted bugs that appear in the testing in the classrooms, in the never-ending improvements and corrections.
Several studies in recent years show how the use of Neotrie strongly motivates students, develops and implements mathematical thinking in action, improves vision and geometric reasoning in space, stimulates cooperation and teamwork (see Rodríguez et al., 2021; Codina-Sánchez et al., 2022; Moral-Sánchez et al., 2022, 2023; Codina et al., 2023; Romero et al., 2023).
Until now, it was the researchers, doctoral students, and students of mathematics or master degree who mostly designed didactic sequences that were implemented in schools in an exploratory way. Thus, while doing so, data was taken to improve its design, correct the bugs found, and add new functions and tools to increase the scope of use, following a design-research study in cycles as in Collins et al. (2004) and Swan (2014).
On the other hand, the author has used Neotrie in his classes of linear geometry and also of algebraic topology in the Degree of Mathematics, as well as in the Master's Degree in Secondary Education Teaching of the University of Almería (Rodríguez, 2022a,b). In previous experiences, master's students were asked to build a particular figure (mosaic, polyhedron, fractal, ...), which they built manipulatively and also in virtual reality, along with some measurement calculations and study of its geometric or topological properties (Figure 1, left).
Figure 1. Left: Master's students building mosaics with WMR headsets in 2019, complementing manipulative activities. Right: In 2023 working with Meta Quest in multiplayer mode in the same VR scene of Neotrie.
In the academic year 2022–23, the pre-service teachers were asked to design by themselves a didactic sequence using Neotrie and deliver it, in just 2 weeks without the possibility of testing it in real classes, or of being corrected or improved by the author, beyond small suggestions during its design. As a novelty, the students were able to use the first versions of the multiplayer mode, which helped them to learn from each other more quickly in the same VR scene (Figure 1, right).
As remarked before, Neotrie has the flexibility for teachers to create their own activities. This makes it more difficult to use the software at the beginning, but it is worth the initial effort for teachers to propose their own activities, more varied than in other closer software. More details of this experience are provided in Section 4. In the next section, the pedagogical framework of our pilot experience is established, seeing how the freedom offered by Neotrie fits with the guidelines promoted by our university.
3 Pedagogical framework
Our pilot experience was framed in the subject “Practical Tools to develop the Mathematics Syllabus”5 of the Master's Degree in Secondary Education Teaching taught at the University of Almería.
This master is informed by a constructivist pedagogical approach, emphasizing the active construction of knowledge by the pre-service teacher. Future teachers might learn how to design activities and learning environments that promote active student participation and the development of their critical thinking. In this way, a self-centered learning process is facilitated. For that, they must learn how to use technological tools to enhance instruction and student engagement. This includes pedagogical strategies and approaches adapted to the curriculum taught in secondary education, to the characteristics and needs of adolescents. They might be encouraged to reflect on their teaching, adjust their approaches based on student needs, and continue developing as educators throughout their careers. The Master's program also fosters collaboration among future teachers, as well as collaboration with other professionals in the educational field, such as parents, other teachers, and specialists.
In particular, in the subject “Practical Tools to develop the Mathematics Syllabus”, they analyze the teacher's tasks in the mathematics classroom and search for and present materials and resources for teaching, establishing elements to study their function, their didactic interest, and selection criteria. At present, there is a wide range of materials and resources for teaching mathematics. Students are guided to be aware of these sources of classroom activity and to analyze them critically. They can see different methodologies for teaching mathematics, such as cooperative, problem-based and project-based learning. They use various ICT's and computer applications that facilitate the teaching and learning of mathematics (GeoGebra, Wolfram Alpha, Kahoot,...), as well as manipulative activities (polyhedra, tiling, fractals constructions,...).
This subject focuses on technological components, following the suggestions of Cevikbas et al. (2023) in a TPaCK framework, giving importance to mathematical software so that they know it, see its usefulness and ease of use to implement it in their future classes.
The “Technological, Pedagogical, and Content Knowledge (TPaCK) is built on Shulman's construct of Pedagogical Content Knowledge (PCK) to include technology knowledge as situated within content and pedagogical knowledge” (Shulman, 1986; Schmidt-Crawford et al., 2009).
Our assessment of the pre-service teachers' didactic sequences is based on the instrument designed by Schmidt-Crawford et al. (2009) to grade the TPaCK components. Adapted to the use of Neotrie this would be:
• Technological knowledge (TK): General use of Neotrie.
• Content knowledge (CK): Mathematical contents covered by Neotrie.
• Pedagogical knowledge (PK): Pedagogical instruments.
• Pedagogical content knowledge (PCK): Effective pedagogical instruments for teaching the mathematical contents.
• Technological content knowledge (TCK): About the tools of Neotrie appropriate to display the mathematical contents.
• Technological pedagogical knowledge (TPK): How students can use the Neotrie tools to perform effectively the activities.
• Technological pedagogical content knowledge (TPCK): How the lesson is designed for learning the appropriate mathematical contents, using effective pedagogical instruments, with the help of Neotrie.
For the planning of our intervention and subsequent assessing of the didactic sequences, it has taken into account the initiative of the master's students and the peculiarity of Neotrie, and regroup the previous components in four items, giving more importance to the technological components:
1. Originality (TPCK): A learning situation is designed that is easier to carry out using virtual reality, or would be more complicated or tedious otherwise.
2. Mathematical content (PCK): It includes precise instructions, which guide the students in the realization of the tasks, with appropriate mathematical content that promotes a critical thinking.
3. Software mastery (TK, TPK): The guide is accompanied by a list of scaffolding tasks using the appropriate tools of Neotrie. It is important to know how the tools work and which ones to use to perform the tasks. This requires having tried to perform such tasks in order to predict the type of difficulties students will encounter.
4. Presentation (TK, TPK, PCK, TPCK): The pre-service teacher knows how to generate a didactic scene with texts, photos, and videos with the necessary instructions and geometric objects in the software to carry out the task. They also include a rubric with criteria for assessing the tasks.
4 Learning environment
The pilot experience in the subject “Practical Tools to Develop the Mathematics Syllabus” was carried out with a group of 32 pre-service teachers, 25 male and 17 female, mainly aged between 23 and 25 years. They were 21 mathematicians and 11 engineers (4 chemical, 3 civil, 3 computer, and 1 agriculture).
The all course was taught in 18 face-to-face sessions of 2,5 h (two per week), distributed from January to May 2023, with a trial intervention period in real classrooms in March.
Only the last two weeks of May (10 h) were dedicated to designing a didactic sequence using Neotrie in a room with computers with access to the internet and eight Meta Quest headsets. Of course, the sequences could not be tested in real classrooms, as the centers do not normally have VR glasses yet. And as we have indicated, this intervention serves as a first approach to the use of Neotrie, not to test it in real classrooms.
The objectives with these classes on Neotrie were the following:
1. Designing activities, based on real situations, that promote active student participation and critical thinking.
2. Exploring the use of VR to enhance teaching and student engagement.
3. Using VR to create content adapted to the curriculum taught in secondary education.
4. Working in small groups which promotes collaboration between future teachers.
Master's students were left free to organize the groups by themselves, manage their time, and way of working collaboratively.
In the first session, they grouped into eight small groups of 3–6 members. They visited the Neotrie website where they could find a guide to the software, including videos of how to use the tools,6 and many examples of activities available on the community page.7
After this first contact with the software, each group chose a topic and shared it on the board with the rest of the class so that there would be no repetition.
They could consult the criteria and learning standards in the chosen geometric part of the Spanish secondary education curriculum and the most convenient level.
In the following sessions they worked in groups to organize the information, write the proposal in shared documents, and learn to handle the appropriate tools in Neotrie.
They were supported throughout this process, helping them with technical problems, guiding them in the use of Neotrie when necessary (although not much because they helped each other between groups), supervising the methodological proposals, the mathematical content and its assessment.
The four assessing criteria (Originality, Mathematical content, Mastery software, and Presentation) were proposed in general at the beginning of the intervention. Although, these were further refined after observing the work of some groups.
5 Results
Sequences delivered on the last day of the course are listed below (title and short description extracted from their texts). From these documents one can extract some strengths and weaknesses of our intervention.
The first consideration is that the mathematical content was in line with what is expected in the official curriculum. The most commonly chosen age was 13 years old and the sequences included a planning preferable for two sessions. The first session normally is devoted to learning the basics of Neotrie. The most used tools8 were beginner ones (1, 2, 3, 4, 5, 8, 10, 11, 12, 13), but some groups also used experts ones (9, 16) and advanced ones (14, 15). This information was useful to assess the level of mastery.
Another aspect to take into account is if they have tried the tasks by themselves in Neotrie (indicated as Checked). This ensures the mastery of the software and at the same time they check the difficulties that the students would encounter. This is normally detected from the pictures of the activities performed. It should be noted that including a tool or an instruction in the sequence does not imply that it has actually been tested. Therefore, assessing software mastery is not straightforward and the author's observation during the lessons has been taken into account. In each case it is indicated whether the activity is based on a real situation. Some are inspired by a similar activity on the GeoGebra website.
1. Conics sections: the aim is to learn about the conic sections that can be obtained from a complete cone when it is cut by a plane using virtual reality. This is also worked on in GeoGebra. Age: 15; Group size: 3; Sessions: 2; Tools: 1, 2, 3, 4, 6, 12, 14, 16; Checked: Yes; Real situation: No (Geogebra).
2. 3D Puzzles: build a Tangram and a Soma cube in Neotrie, and propose to make different figures. They also use such figures to ask questions about areas and volumes. Age: 14; Group size: 3; Sessions: 3; Tools: 1, 3, 4, 5, 7, 8; Checked: Yes; Real situation: No.
3. Discovering the Koch Snowflake Fractal in 3D: the idea is to create and explore in 3D the Koch's Snowflake fractal, understanding its structure and properties. Age: 14; Group size: 3; Sessions: 2; Tools: 1, 2, 3, 8, 9,10, 12, 13; Checked: Yes; Real situation: No.
4. Creating your ideal house: in this activity, the students must make a plan of their house in 2D, with the Planner 5D program, with simple geometric figures such as squares, rectangles, triangles, circles, ... Then they are asked to build them in Neotrie with real concrete lengths or areas. Age: 13; Group size: 3; Sessions: 4; Tools: 1, 3, 5, 10, 11; Checked: No; Real situation: Yes.
5. Building a playground: the plan is to ask students to design and build in Neotrie a playground with some handrails and a swing. Age: 14; Group size: not-fixed; Sessions: 5; Tools: 1, 3, 4, 16; Checked: No; Real situation: Yes.
6. Pyramids of Giza: the objective is to represent in Neotrie the three pyramids of Giza on both small and real scales. Age: 13; Group size: 3-4; Sessions: 2; Tools: 1, 2, 3, 4, 5, 6, 7, 8, 9; Checked: No; Real situation: Yes.
7. The planetary system: In this proposal, students are asked to draw a series of spheres with certain sizes in Neotrie, color them, and place them at pre-established distances from each other, in order to build a scaled model of the planetary system. Age: 13; Group size: 3; Sessions: 2; Tools: 1, 3, 4, 8, 12, 14, 15; Checked: Yes; Real situation: No, GeoGebra.
8. Demonstrating remarkable identities with Neotrie: Students understand and internalize the remarkable identities of the square of a binomial and cube of a binomial through their geometric development both in the plane and in space in Neotrie. Age: 13; Group size: 3; Sessions: 3; Tools: 1, 4, 5, 9, 12, 16; Checked: Yes; Real situation: Yes.
The Table 1 takes into account the four criteria described in Section 3.
Table 1. Assessment of the sequences over 20 points (each part is over 5).
Next, the assessment process will be illustrated using a specific sequence that aligns with the four items, originality, mathematical content, software mastery and presentation, outlined in Section 3.
5.1 The pyramids of Giza
The following are the essential parts of the sequence Pyramids of Giza, by the group 6 (formed by one chemical engineer, one civil engineer and one mathematician), which will be analyzed in detail in the following section.
“In this sequence, secondary students are asked to follow steps 1–5, accompanied by the pictures in Figure 2.
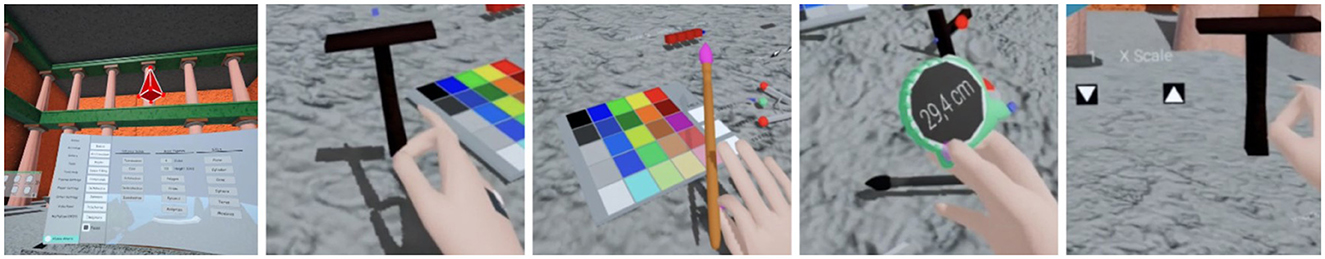
Figure 2. Pictures from the didactic sequence pyramids of Giza: gallery of figures, copy tool, palette and pencil, tape, and scale copy tool.
The objective is to recreate the three pyramids of Giza in Neotrie.
Steps to follow:
1. Basic actions and tools: Follow the guide of Neotrie to learn how to make the basic hand actions and how to use the tools to start working.
2. Insert a pyramid: Load from the gallery a pyramid with height 2 dm.
3. Duplicate objects: Make three copies of the pyramid with the copy tool.
4. Modify their color: red (Keops), blue (Kefren) and green (Micerinos).
5. Scale: Measure, modify, and scale the pyramids to get the them in real size.
In step 5, three tables in the colors red, blue, and green with the measures of the corresponding pyramids are given to the students: For the pyramid of Keops, height 146.6 m, side length 230.3 m, volume 2.592.350 m3 and inclination 51°50'34”; for the pyramid of Kefren, height 143.9 m, side length 215.2 m, volume 2.211.096 m3 and inclination 53°07'48"; and for the pyramid of Micerinos, height 66m, side length 103 m, volume 235.183 m3 and inclination 51°20'00”.
In the didactic sequence, after the proposed task, some comments follow:
Students have to use the tape to measure the proportions of the figures in Neotrie and compare them with those in the table. They realize that the ratio width/height is approximately the golden ratio, which they can calculate as approximately 3/2. Therefore, they must modify the pyramid to have a height 2 dm and base 3 dm.
Once the small pyramids have the correct measurements, the scale factor must be found: 73 for the pyramid of Keops, 72 for the pyramid of Kefren and 33 for the pyramid of Mycerinos.
The objectives sought fixed in this activity are to: Understand what a ratio is and how it relates to proportion; Understand how scale is used to represent objects or drawings in reduced or enlarged proportions; To learn how to scale geometric figures in three dimensions; Understand how the dimensions of a figure are changed by multiplying or dividing its measurements by a scale factor.
The curricular contents intended to be developed are the following: Concepts of ratio and proportion; concept of scale; use of scale to represent objects in reduced or enlarged proportions; and calculation of scaled dimensions using scale factors.
The use of the NeoTrie digital tool is intended to motivate students and develop their technological skills while achieving the aforementioned objectives and contents.”
5.2 Analysis
We next provide some details for marking the sequence:
1. Originality (5 points): An ideal activity is proposed to be carried out in virtual reality, where the 3D figures can be modified and seen in real-time in a totally immersive environment.
2. Mathematical content (3 points): The instructions are precise and guide the students in the construction of the pyramids, encouraging them to think about how to modify the figures, and to look for the corresponding scale factor to obtain the real ones. However, although it is mentioned in the objectives, there are no questions to verify the changes in lengths, areas, and volumes when scaling the pyramid; There is no mention of the inclination, despite it being given to students as data.
3. Software mastery (3 points): The appropriate Neotrie tools to use for each step are listed. It is noted that they have been used and that they know the possible mistakes that could be made by the students. However, it would be necessary to indicate the restriction of movement in the axes, to obtain the small pyramid of height 2 dm and base 3 dm. It would also be missing other options to compare the area and volume measurements, or the use of the protractor to measure the inclination of the pyramids (see Figure 3).
4. Presentation (4 points): The trainee teachers did not generated a scene within Neotrie, not being necessary, as the proposal including pictures and instructions is sufficient to guide the student through the activity.
Figure 3. From left to right: Scales 1:10, and 1:1,000. Comparison of length, area and volume when scaling by 10. Measuring inclination 51.78°. Pyramids models on a satellite picture.
It is interesting to note at this point the software problem that appeared during the design of the activity: The ratio height/width of the Keops pyramid is equal to 146.6/230.3 = 0.635. Then pre-service teachers tried to load from the gallery of figures a pyramid of height 0.625 dm and size of the basis 1 dm. However, there was a software bug in the creation of the pyramid with a given height with decimal numbers. To solve this difficulty, they proposed step 2 which is more successful in that it forces the student to think in step 5 about how to modify the small pyramids to get the scaled real ones. We observe in this case, how thanks to a bug in the software, they have adapted and found an alternative way in the design of the task.
On the other hand, they made a mistake when calculating the change in scale. For the pyramid of Keops, 73 was calculated as 146.6 m/2 dm = 73.3, but it should be 1,466 dm/2 dm = 733. Perhaps they could have detected the error if they had made the pyramids in Neotrie, for being too small compared to the real one.
For the reader's interest, once the bug was solved, for a pyramid of base 1 dm and height 0.635 dm, the correct factor scale to get the Keops pyramid with real measures would be 230,3 m/1 dm = 2,303 dm/1 dm = 2,303. Some pictures following this alternative procedure are in Figure 4. It is appreciated how interesting it can be for students to build these pyramids in virtual reality with real sizes.
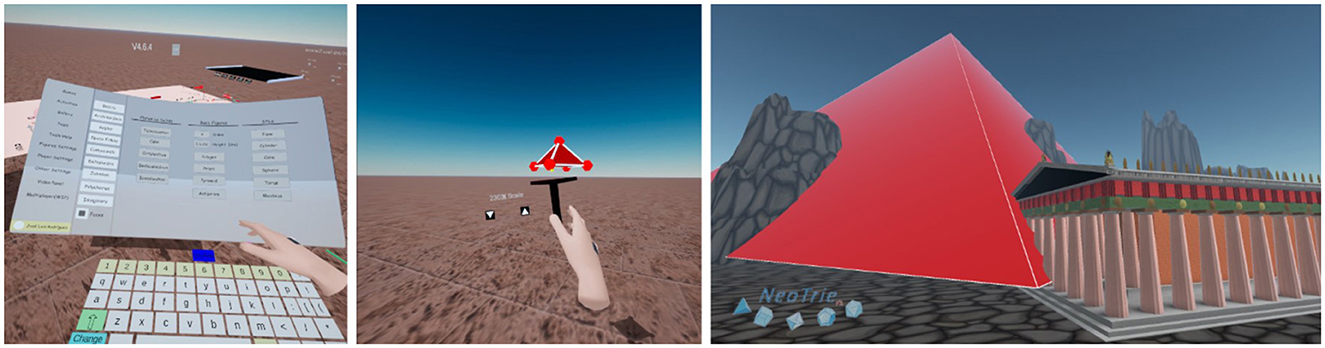
Figure 4. From left to right: Loading Keops pyramid with base size 1 dm and height 0.635 dm. Scale copy tool with factor 2,303. Real size of the Keops pyramid compared with the temple of Neotrie.
5.3 Preliminary conclusions
Our intervention allowed to accomplish the four objectives established in Section 4: (1) Pre-service teachers were able to design activities, many of them based on real situations, that promote active student participation and critical thinking, as they include scaffolding steps and interesting mathematical questions; (2) They have explored the use of VR and its potential to enhance the teaching and learning of geometry; (3) They have used VR to create content adapted to the secondary school curriculum; (4) Working in small groups has fostered their collaboration among pre-service teachers.
However, some drawbacks encountered should be overcome to achieve better training for our future teachers. The technical difficulties and software glitches slowed progress in designing sequences, although acceptable solutions and alternatives were found.
On the other hand, some groups would have needed more time to improve their use of Neotrie. Some of the sequences would have needed more time to be tested by themselves and revised. This would produce a better knowledge of the difficulties that secondary students would encounter in performing them.
It is worth highlighting some comments included in other delivered sequences. These reveal the awareness of the benefits that the use of Neotrie will generate in their future students: “This task allows to advance in spatial reasoning, previously imagining the resulting conic sections and subsequently checking the result, visualizing it in real time” (noted by Group 1); “Students can obtain abstract and complex concepts that are difficult to understand if taught in theoretical classes. [...] it captures students' attention as this software is quite innovative, and students learn through hands-on experience” (by Group 2); “Three-dimensional space offers a unique opportunity to explore mathematical concepts in an interactive and visually appealing way. Virtual reality and the active learning approach encourage the active participation of students, promoting creativity, problem solving and collaborative work” (by Group 3); “It is intended with this learning situation to improve the skills students' spatial and mathematical skills through a critical and appropriate use of technology. From the Neotrie application, a life situation will be exposed daily in which they will have to build elements of a park (handrails and swing) and they will have to justify the geometric figures with which they have carried out the model. Contributing exercises other than the usual ones and manipulative resources such as those exposed in this activity will allow students to generate motivation and interest in the knowledge that they intend to develop” (by Group 5); “Students can generate the geometric representation and also manipulate the different elements in an attractive and fun way” (by Group 7).
What they describe is their perception of how using Neotrie would turn out for their future students. Of course, testing of the sequences in real classrooms would be needed to obtain robust conclusions of these benefits.
6 Discussion
For the past years now, virtual reality has been changing rapidly both hardware and software, which keeps researchers and software developers busy, constantly forced to adapt to changes and avoid failures in the use of both software and hardware. These issues have affected the Neotrie team, which has had to adapt and work to bring their project activities to the classroom.
It was not until the academic year 2022–23 that we were able to conduct pre-service teacher sessions to test the effectiveness of using Neotrie to develop concrete classroom activities in a short time. In this situation, it was not clear how to grade a work of these characteristics in which part of it requires preparing the VR device and casting on computer or tablet, a training period on the use of the software, alignment with a pre-established curriculum, which does not yet take into account virtual reality, or if it does, it is very general within the use of new technologies. With recommendations proposed in the Technological Pedagogical Content Knowledge (TPACK) framework, pre-service teachers were given a rubric with four criteria to assess their sequences: Originality, Mathematical content, Mastery, and Presentation.
In just two weeks (four sessions of 2.5 h), pre-service teachers were able to overcome the mentioned difficulties and to create a fairly complete didactic sequence with Neotrie. The experience gained is good to start creating basic sequences. Pedagogical content learned in other subjects they have taken in the master's degree in teaching can be appreciated. However, the designed scenes can be improved as some groups have not made them themselves completely to realize all possible difficulties. We have shown some details of one of the sequences, “Pyramids of Giza”, to see some limitations that are found when designing this type of sequences in a short time. It is also worth noting in general their belief in the benefits and motivation that VR would generate in their future students.
Therefore, answering the question established at the introduction: more training on Neotrie is needed so that pre-service teachers can introduce more mathematical content using more tools of the software, as well as testing by themselves the activities to detect possible difficulties in their future real classes.
Thus, in the next Master's course, 2 more weeks will be provided to learn how to use Neotrie, to ensure a more effective training. They will also be complemented with GeoGebra activities, approaching a more realistic situation in mathematics classrooms, as these usually have computers but not so many VR glasses. They will also have more time to do the mathematical part themselves and, in general, to complete the activities they ask their students to do, ensuring both the quality of the mathematical content and the mastery of Neotrie.
This type of sandbox software, like Neotrie, gives teachers a great deal of freedom and flexibility to create interesting VR scenes themselves, and even have the students themselves collaboratively generate them and then present them to their peers.
Our pilot experience can serve to help future teachers to use a new technological tool, such as Neotrie, so a final and validated didactic sequence is not expected for now, but it is clear that it serves to introduce them to an effective use of Neotrie in their classes.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Ethics statement
Written informed consent was obtained from the individual(s) for the publication of any potentially identifiable images or data included in this article.
Author contributions
JR: Investigation, Methodology, Software, Visualization, Writing – original draft.
Funding
The author(s) declare financial support was received for the research, authorship, and/or publication of this article. The author has received partial support from the group of Innovation and Research in Science and Mathematics Education (HUM886) of the Regional Government of Andalusia and the Spanish Ministry of Science and Innovation grant PID2020-117971GB-C22.
Acknowledgments
The author would like to thank Isabel Romero, and specially the reviewers, who have contributed to improve the presentation and content of the manuscript.
Conflict of interest
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher's note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Footnotes
1. ^https://www.vrs.org.uk/virtual-reality-applications/
2. ^http://www2.ual.es/neotrie
3. ^https://fairplayforkids.org/wp-content/uploads/2023/04/HorizonLetter.pdf
5. ^https://www.ual.es/estudios/masteres/presentacion/plandeestudios/asignatura/7035/70352118
6. ^https://www2.ual.es/neotrie/guia-2022
7. ^https://www2.ual.es/neotrie/comunidad
8. ^1. Basic hand actions; Create, face, edit, delete, move, grab, extrude; 2. Gallery of figures; 3. Photo camera; 4. Palette and pencil; 5. Tape; 6. Protractor; 7. Figure measures information; 8. Copy tool; 9. Scale copy tool; 10. Parallel tool; 11. Perpendicular tool; 12. Rotation tool; 13. Reflection tool; 14. Coordinate axis; 15. Labeling tool; 16. Sphere, cylinder, cone tool.
References
Bermejo, B., Juiz, C., Cortes, D., Oskam, J., Moilanen, T., Loijas, J., et al. (2023). Ar/vr teaching-learning experiences in higher education institutions (hei): a systematic literature review. Informatics 10, 45. doi: 10.3390/informatics10020045
Cevikbas, M., Bulut, N., and Kaiser, G. (2023). Exploring the benefits and drawbacks of ar and vr technologies for learners of mathematics: recent developments. Systems 11, 244. doi: 10.3390/systems11050244
Codina, A., Rodríguez, J. L., and Morales, C. S. (2023). “Neotrie VR, Realidad Virtual inmersiva para el aprendizaje de la geometría 3D,” in Investigaciones y experiencias en enseñanza de las ciencias y la matemática (Universidad Autónoma del Estado de México, ALDVS), 159–169.
Codina-Sánchez, A., del Mar García López, M., Albaladejo, I. M. R., and Gómez, J. L. L. (2022). Poliedros con el software de realidad virtual inmersiva neotrievr, una experiencia con maestrosen formación. Revista Electrónica Interuniversitaria de Formación del Profesorado 25, 1–14. doi: 10.6018/reifop.531841
Collins, A., Joseph, D., and Bielaczyc, K. (2004). Design research: theoretical and methodological issues. J. Learn. Sci. 13, 15–42. doi: 10.1207/s15327809jls1301_2
Fernandez, M. (2017). Augmented-virtual reality: How to improve education systems. Higher Learn. Res. Commun. 7, 1. doi: 10.18870/hlrc.v7i1.373
Lai, J., and Cheong, K. H. (2022). Adoption of virtual and augmented reality for mathematics education: a scoping review. IEEE Access 10, 1–1. doi: 10.1109/ACCESS.2022.3145991
Martín-Gutiérrez, J., Mora, C. E., Añorbe-Díaz, B., and González-Marrero, A. (2017). Virtual technologies trends in education. Eurasia J. Mathem. Sci. Technol. Educ. 13, 469–486. doi: 10.12973/eurasia.2017.00626a
Moral-Sánchez, S., Sánchez-Compaña, M.-T., and Romero-Albaladejo, I. (2023). Uso de rea-lidad virtual en geometría para el desarrollo de habilidades espaciales. Enseñanza de las Ciencias 41, 125–147. doi: 10.5565/rev/ensciencias.5442
Moral-Sánchez, S. N., Sánchez-Compaña, M. T., and Romero, I. (2022). Geometry with a stem and gamification approach: a didactic experience in secondary education. Mathematics 10, 3252. doi: 10.3390/math10183252
Perri, D., Simonetti, M., Tasso, S., and Gervasi, O. (2021). “Learning mathematics in an immersive way,” in Software Usability, chapter 1, eds. L. M. Castro, D. Cabrero, and R. Heimgärtner (Rijeka: IntechOpen).
Rodríguez, J. L. (2022a). Exploring Dynamic Geometry Through Immersive Virtual Reality and Distance Teaching. Cham: Springer International Publishing, 343–363.
Rodríguez, J. L. (2022b). “Using virtual reality to teach linear algebra with a focus on affine geometry,” in Using Virtual Reality to Teach Linear Algebra With a Focus on Affine Geometry. Virginia: Mathematics and Technology, LLC, 218–230.
Rodríguez, J. L., Romero, I., and Codina, A. (2021). The influence of neotrie vr's immersive virtual reality on the teaching and learning of geometry. Mathematics 9, 2411. doi: 10.3390/math9192411
Romero, I., Rodríguez-Martínez, J., and Rodríguez, J. (2023). Optimizing the surface of orthohedra with virtual reality in primary school. Eurasia J. Mathem. Sci. Technol. Educ. 19, 13508. doi: 10.29333/ejmste/13508
Schmidt-Crawford, D., Baran, E., Thompson, A., Mishra, P., Koehler, M., and Seob, S. (2009). Technological pedagogical content knowledge (tpack): the development and validation of an assessment instrument for preservice teachers. J. Res. Technol. Educ. 42, 123–149. doi: 10.1080/15391523.2009.10782544
Shulman, L. (1986). Those who understand: Knowledge growth in teaching. educational researcher. Educ. Res. 15, 4–14. doi: 10.3102/0013189X015002004
Su, Y. S., Cheng, H. W., and Lai, C. F. (2022). Study of virtual reality immersive technology enhanced mathematics geometry learning. Front. Psychol. 13, 760418. doi: 10.3389/fpsyg.2022.760418
Swan, M. (2014). Design Research in Mathematics Education. Dordrecht: Springer Netherlands, 148–152.
Thomsen, L. (2023). Virtual Reality in Mathematics Education (VRiME): An Exploration of the Integration and Design of Virtual Reality for Mathematics Education (PhD thesis). Denmark: Aalborg University.
Keywords: instruction, education, virtual reality, immersive learning, geometry, TPaCK, action research
Citation: Rodríguez JL (2024) Virtual reality in the classroom: a difficult but exciting adventure for teachers and students. Front. Educ. 9:1294715. doi: 10.3389/feduc.2024.1294715
Received: 15 September 2023; Accepted: 09 February 2024;
Published: 08 March 2024.
Edited by:
Vanda Santos, University of Aveiro, PortugalReviewed by:
Jon Mason, Charles Darwin University, AustraliaWahyu Widada, University of Bengkulu, Indonesia
Copyright © 2024 Rodríguez. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: José L. Rodríguez, amxyb2RyaSYjeDAwMDQwO3VhbC5lcw==