Self-Similar Symmetry Model and Cosmic Microwave Background

In this paper, we present the self-similar symmetry (SSS) model that describes the hierarchical structure of the universe. The model is based on the concept of self-similarity, which explains the symmetry of the cosmic microwave background (CMB). The approximate length and time scales of the six hierarchies of the universe—grand unification, electroweak unification, the atom, the pulsar, the solar system, and the galactic system—are derived from the SSS model. In addition, the model implies that the electron mass and gravitational constant could vary with the CMB radiation temperature.

1. Introduction

What determines the values of the physical constants and whether they will remain constant over time are fundamental questions in physics. A long-standing conundrum associated with the physical constants is that large dimensionless numbers that are seemingly unrelated can be linked by a scale factor of 10³⁹ [1–5]. The Dirac large numbers hypothesis (LNH) tackles this problem. It claims that the gravitational constant G is inversely proportional to the age of the universe. The LNH is based on the coincidences between three very large dimensionless numbers $N_1\simeq N_2\simeq \sqrt{N}$. N₁, the ratio of the radius of the observable universe to the radius of the electron, is approximately 10³⁹; N₂, the ratio of the electromagnetic and gravitational forces between a proton and an electron, is also approximately 10³⁹; and N, the number of protons in the observable universe, is approximately 10⁷⁸. The Dirac LNH argues that “any two of the very large dimensionless numbers occurring in Nature are connected by a simple mathematical relation, in which the coefficients are of the order of unity”[1].

In this paper, we present the self-similar symmetry (SSS) model in which the relationships among these seemingly unrelated physical quantities are represented using a simple geometric sequence for which the first term and the geometric ratio are given by dimensionless ratios of masses. Based on the LNH, the first term of the geometric sequence corresponds to the cosmic microwave background (CMB) radiation temperature, which points to the possibility that the values of the physical constants are determined by the CMB radiation temperature.

2. The Self-Similar Symmetry Model

In the SSS model, the CMB has a symmetrical self-similar structure and the physical constants are dimensionless, otherwise they would not have universality. Therefore, the fundamental dimensionless mass ratios are defined as follows:

$\begin{array}{ll}A=log\alpha =log\left(\frac{m_{\text{pl}}}{m_{\text{pr}}}\right),B=log\beta =log\left(\frac{m_{\text{e}}}{m_{\text{pr}}}\right),& (1)\end{array}$

where m_pr is the proton mass, m_e is the electron mass, and m_pl is the Planck mass, and the fundamental dimensionless time and length ratios are defined as

$\begin{array}{ll}T=log\left(\frac{t}{t_{\text{pl}}}\right),L=log\left(\frac{l}{l_{\text{pl}}}\right),& (2)\end{array}$

where t and l are the time and length scales of the hierarchies and t_pl and l_pl are the Planck time and Planck length, respectively. The similarity dimension D is defined using these dimensionless parameters:

$\begin{array}{ll}D={\left(\frac{T}{L}\right)}^3=\frac{A}{A+B}\approx 1.206.& (3)\end{array}$

We then assume that hierarchical structures are constructed according to the following sequences:

$\begin{array}{rcll}{L}_0& =& 2(A+B)\approx 31.701,& (4)\end{array}$

$\begin{array}{rcll}{L}_n& =& D^n{L}_0\text{for}L>L_0,& (5)\end{array}$

$\begin{array}{rcll}{L}_m& =& (2-D^m)L_0\text{for}L<L_0,& (6)\end{array}$

where n and m are natural numbers that represent the hierarchical level. In addition, the time scales of each hierarchy are calculated using Equation (3).

3. Verification of the SSS Model

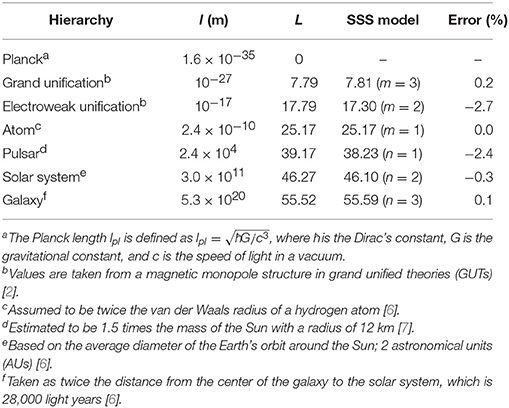
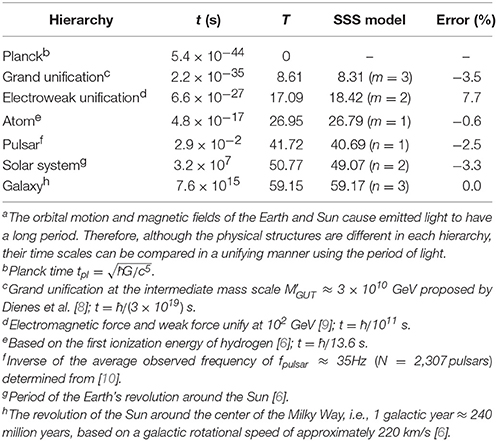
To verify the SSS model, we compared values obtained with it against reference values. Tables 1, 2 summarize the length and time scales, respectively, of the grand unification, electroweak unification, atom, pulsar, solar system, and galactic system hierarchies of the universe. The SSS model values agree well with the reference values. Figure 1 shows the hierarchy time scale as a function of the length scale. The coincidences in the figure confirm the validity of the SSS model.

TABLE 1

Table 1. Length scales of the hierarchies of the universe.

TABLE 2

Table 2. Time Scales of the Hierarchies of the Universe^a.

FIGURE 1

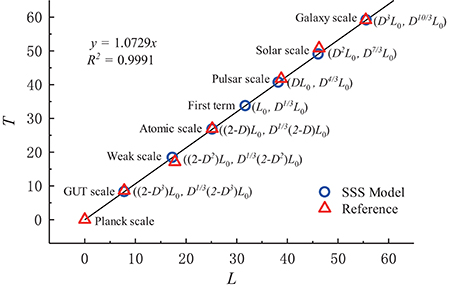
Figure 1. Time scale as a function of the length scale for the SSS model and reference values. The gradient of the fit of the SSS model values to the reference values is 1.0729(R² = 0.9991). Note the symmetry about the first term L₀, which corresponds to the CMB radiation temperature. This symmetry leads to speculation about the approximate length and time scale of the universe, which are 4.1 × 10²⁸ m and 5.2 × 10¹⁶ years, respectively.

4. Discussion

From Equation (1), 2A = −logα_G, where ${\alpha }_{\text{G}}=G{m}_{\text{pr}}^2/\hslash c$ is the gravitational coupling constant, the following coincidences occurs:

$\begin{array}{ll}{L}_{n=2}-L_{m=3}=L_{n=3}-L_{m=2}\approx 2A.& (7)\end{array}$

Equation (7) shows that α_G plays an important role in forming the hierarchical structure of the universe. In addition,

$\begin{array}{ll}{L}_{m=1}-L_0=L_0-L_{n=1}=2B.& (8)\end{array}$

Thus, if L_u is the length of the universe, the following hierarchy holds:

$\begin{array}{ll}{L}_{\text{u}}=2L_0.& (9)\end{array}$

Therefore, the ratios of the coincidences between the length scales of the hierarchies are

$\begin{array}{rcll}{r}_a& =& \frac{L_{n=2}-L_{m=3}}{L_{n=1}}\approx 1.002,& (10)\end{array}$

$\begin{array}{rcll}{r}_b& =& \frac{L_{\text{u}}-L_{n=3}}{L_{n=2}-L_{n=1}}\approx 0.991,& (11)\end{array}$

from which we get

$\begin{array}{ll}D=\frac{r_a-r_b}{1-r_b}.& (12)\end{array}$

From Equation (12), we see that r_a ≠ r_b ≠ 1.

With respect to the first term of the geometric sequence, L₀, we find that

$\begin{array}{ll}{(\alpha \beta )}^{-2}{T}_{\text{pl}}\approx 2.821K,& (13)\end{array}$

where T_pl is the Planck temperature. The value in Equation (13) is consistent with the CMB radiation temperature T_CMB[11]. Assuming the right-hand side of Equation (13) represents the T_CMB, if LNH is applied to Equation (13) and we define the dimensionless temperature ratio τ_CMB = T_CMB/T_pl, we get

$\begin{array}{lll}\hfill {\alpha }_{\text{G}}\approx {\tau }_{\text{CMB}}^D.& & (14)\end{array}$

Similarly,

$\begin{array}{l}\\ \hfill {\beta }^2\approx {\tau }_{\text{CMB}}^{D-1}.& & (15)\end{array}$

Thus, both α_G and β are power functions of T_CMB.

Substituting T_CMB = T_pl, an initial condition of the universe, into Equations (14) and (15) yields α = β = 1, which means that the entire hierarchy was contained in a single point and that the electron, proton, and Planck masses were equivalent. These masses have varied since that initial single point such that m_e ≪ m_pr ≪ m_pl, in response to the changing T_CMB, where T_CMB ≪ T_pl. Assuming that T_CMB → 0 is the ultimate fate of the universe, then α → ∞, β → 0, and L₀ → ∞, indicating that m_e → 0 and G → 0 as the universe expands to infinity.

5. Conclusions

Our SSS model describes the large-scale structure of the universe and shows that the six hierarchies of the universe are self-similar to the CMB, indicating that the CMB is key to unifying quantum theory with general relativity. In addition, the SSS model leads to the conclusion that m_e and G vary with T_CMB. Any errors arising from the SSS model are problems to be tackled in the future.

Author Contributions

TS conceived the study and prepared the manuscript.

Conflict of Interest Statement

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

The author thanks M. B. Greenfield and K. Kitahara for helpful discussions.

References

1. Dirac PAM. The cosmological constants. Nature (1937) 139:323. doi: 10.1038/139323a0

CrossRef Full Text | Google Scholar

2. Barrow JD, Tipler FJ. The Anthropic Cosmological Principle. New York, NY: Oxford University Press (1986). p. 656.

Google Scholar

3. Dicke RH. Dirac's cosmology and Mach's principle. Nature (1961) 192:440–1. doi: 10.1038/192440a0

CrossRef Full Text | Google Scholar

4. Carter B. In: Longair MS, editor. Confrontation of Cosmological Theories with Observational Data. Dordrecht: Reidel (1974), p. 291–8. doi: 10.1007/978-94-010-2220-0_25

CrossRef Full Text

5. Carr BJ, Rees MJ. The anthropic principle and the structure of the physical world. Nature (1979) 278:605–12. doi: 10.1038/278605a0

CrossRef Full Text | Google Scholar

6. National Astronomical Observatory of Japan. Chronological Scientific Tables [in Japanese]. Tokyo: Maruzen Publishing Co. (2013).

7. Lattimer JM Prakash M. The physics of neutron stars. Science (2004) 304:536–42. doi: 10.1126/science.1090720

PubMed Abstract | CrossRef Full Text | Google Scholar

8. Dienes KR, Dudas E, Gherghetta, T. Grand unification at intermediate mass scales through extra dimensions. Nucl Phys B (1999) 537:47–108. doi: 10.1016/S0550-3213(98)00669-5

CrossRef Full Text | Google Scholar

9. Bertone G, Hooper D, Silk J. Particle dark matter: evidence, candidates and constraints. Phys Rep. (2005) 405:279–390. doi: 10.1016/j.physrep.2004.08.031

CrossRef Full Text | Google Scholar

10. Manchester RN, Hobbs GB, Teoh A, Hobb M. The Australia telescope national facility pulsar catalogue. Astron J. (2005) 129:1993–2006. doi: 10.1086/428488

CrossRef Full Text | Google Scholar

11. Fixsen DJ. The temperature of the cosmic microwave background. Astrophys J. (2009) 707:916–20. doi: 10.1088/0004-637X/707/2/916

CrossRef Full Text | Google Scholar