
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Acoust., 02 April 2025
Sec. Acoustic Metamaterials
Volume 3 - 2025 | https://doi.org/10.3389/facou.2025.1529474
This article is part of the Research TopicAcoustic Topological Insulators: Envisioned Applications and Technology IntegrationView all 3 articles
 Hasan B. Al Ba’ba’a1*†
Hasan B. Al Ba’ba’a1*† Jihong A. Ma2,3*†
Jihong A. Ma2,3*†Topological phononics and acoustics have recently garnered significant attention due to their promise of a wide range of advanced wave-controlling applications, including mechanical computing, energy harvesting, and noise isolation. Topological states are vibrational modes emerging inside frequency bandgaps, and typically follow the bulk-boundary correspondence, meaning that the topological features observed at boundaries are determined by the bulk properties—the unit cell. Traditionally, topological states are characterized by analyzing the eigenvectors of the effective Hamiltonian of a given unit cell. However, this approach presents challenges when a rapid and accurate design is needed to achieve desirable topological characteristics as it often involves trial and error to obtain the ideal unit cell parameters. In this study, we propose a rigorous methodology to inversely design one-dimensional diatomic lattices based on the topological properties of complex phase loci, derived from the off-diagonal elements of the effective Hamiltonian. We discuss three representative shapes of complex phase loci: ellipse, epitrochoid, and hypotrochoid. Our methodology can be further expanded to higher dimensions, enabling more complex geometric designs for versatile topological phononic and acoustic features.
Topological insulators (TIs) are materials that possess intrinsic bandgaps while being conducive on non-trivial surfaces, edges, or corners (Haldane, 1988; Hasan and Kane, 2010) due to the emergence of topological states. The number of topological states can typically be characterized using a topological invariant derived from their bulk properties—a concept known as the “bulk-boundary correspondence.” Unlike trivial states, topological states of TIs are resilient to local perturbations and demonstrate high conductivity, making them promising for next-generation electronic and photonic devices (Qi and Zhang, 2011; Xu et al., 2017; Politano et al., 2017).
In recent years, research into acoustoelastic and phononic analogs of TIs has gained momentum due to their unique ability to control phononic waves in unconventional ways. These analogs are robust against disorders, exhibiting no backscattering (Roman and Sebastian, 2015; Nash et al., 2015; Ma et al., 2018; Pal et al., 2016; Fleury et al., 2016), allowing them to function effectively as phononic waveguides (Al Ba’ba’a et al., 2021; Wang et al., 2015; Ma et al., 2019; Mousavi et al., 2015), diodes (Liu et al., 2017; Zhou et al., 2020), logic gates and switches (Pirie et al., 2022; Bahrami and Bahrami, 2024; Li et al., 2024), and acoustic topological field-effect transistors (Lee et al., 2022). Meanwhile, the inverse design of acoustoelastic metamaterials to optimize topological states has also been proposed (Christiansen et al., 2019; Du et al., 2020; Ding et al., 2024). Like their electronic counterparts, phononic topological states also appear to adhere to the principle of bulk-boundary correspondence and can be characterized by a topological invariant derived from the spectral evolution of the mechanical equivalent of Hamiltonian or eigenvector obtained through unit-cell analysis. For instance, the mechanical analog of the Su–Schrieffer–Heeger (SSH) model consists of identical masses,
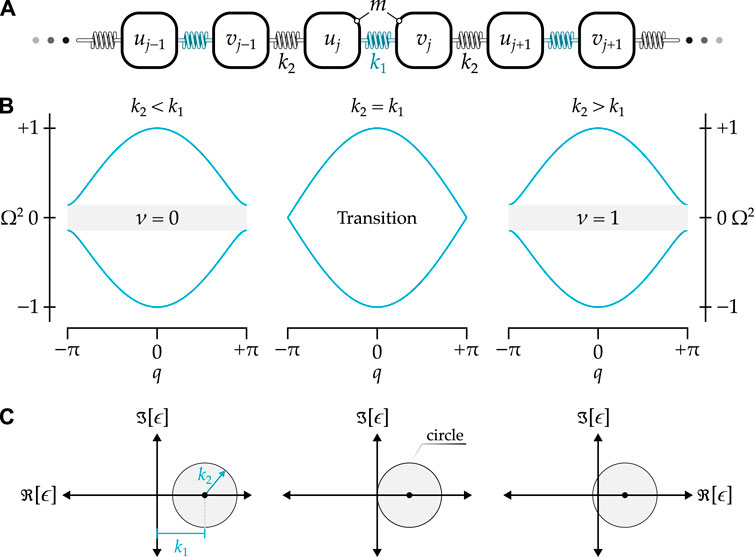
Figure 1. (A) Schematic of a diatomic lattice with a circular complex phase locus. (B) Typical dispersion diagrams with the cases of
However, recent studies have shown that systems with more complex connection networks, such as alternating non-local interactions in SSH models (Rajabpoor Alisepahi et al., 2023; Rajabpoor Alisepahi and Ma, 2024; Grundmann, 2020; Liu et al., 2023), can exhibit multiple domain boundary states due to a higher
While it is now established that coupling beyond nearest neighbors in diatomic lattices uncovers new types of winding trajectories of the complex phase, controlling these trajectories and their overall shapes remains an open challenge. To address this knowledge gap, we propose a novel methodology that enables the selection of complex phase trajectories and facilitates the inverse design of a diatomic lattice exhibiting these intricate phase functions. We begin by revisiting the conventional diatomic lattice, characterized by a circular complex phase locus, and then discuss three representative locus shapes: ellipse, epitrochoid, and hypotrochoid. A step-by-step inverse design process is outlined for creating diatomic lattices that embody these complex loci. Furthermore, we establish connections between the mathematical features of these complex closed-loop locus geometries and the inversely designed diatomic lattices, analyzing their impact on the winding number through detailed parametric studies. By introducing this inverse-design methodology, our study lays the foundation for realizing wave-based acoustic and mechanical devices with complex and unconventional topological features, which are unattainable using simple lattices and structures.
To introduce our inverse design methodology for complex closed-loop locus geometries, we begin our discussion by revisiting the well-established simple 1D mechanical analog of the SSH model with identical masses,
where
As detailed in Appendix A, the eigenvalues of
Results of the normalized eigenvalues
We now focus on how
with
once again, representing a circle with the radius being
where
Here,
For the conventional diatomic lattice, where the complex phase locus is given by Equation 2, the Berry connection can be derived analytically. This involves evaluating the inverse and the
By performing the integral in Equation 6 for the Berry connection (i.e., the integrand) in Equation 8 using MATLAB’s symbolic toolbox, we obtain the winding number:
The topological invariant—the winding number
As observed from Figures 1B, C, if
In the following sections, we will inversely design diatomic lattices with complex networks based on the shape of
In this section, the inverse design methodology for a diatomic lattice based on an elliptical complex phase locus is detailed. Herein, the masses,
Here,
Notice that Equation 12 reduces to Equation 2 when
To inversely design a lattice from the complex phase locus expressed in Equation 12, we need to understand the roles of each term constituting
Thus, we can establish the interconnecting network between the two degrees of freedom based on the following three characteristics of Equation 12:
• Characteristic 1: The term
• Characteristic 2: The second term,
• Characteristic 3: The third term,
Based on these three characteristics, the degrees of freedom are connected as described, resulting in the diatomic lattice in Figure 2A, with the unit-cell equations of motion being:
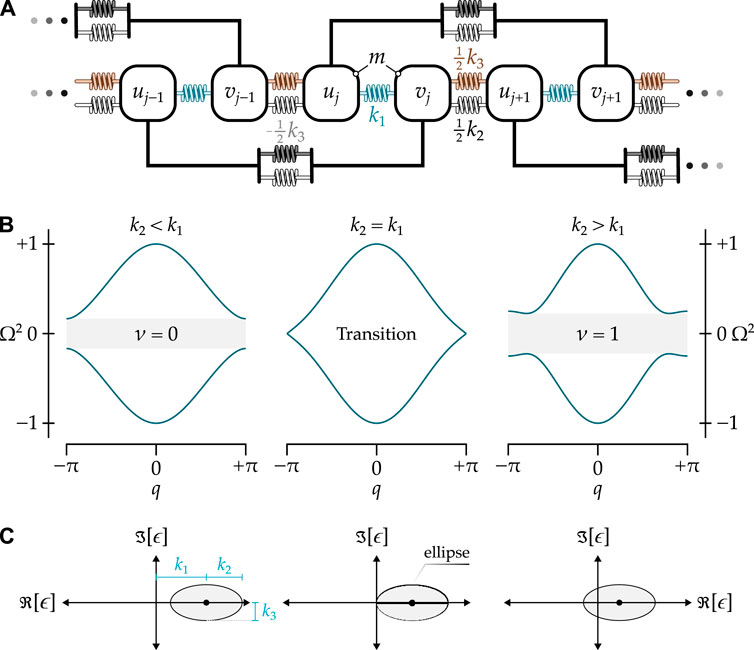
Figure 2. (A) Schematic of a diatomic lattice with a complex phase locus of an ellipse. (B) Dispersion diagrams of the lattice in Subfigure (A) with a constant
We can observe that if the degrees of freedom in Equations 13a, b are condensed via the Bloch theorem, the resulting stiffness matrix will have constant diagonal elements of
To reveal the topological properties of the diatomic lattice with an elliptical phase locus in Figure 2A, the winding number is calculated by performing the integral in Equation 6 for the chiral matrix
As with the conventional diatomic lattice with a circular locus in Figure 1C, the winding number is defined via Equation 14, indicating that it only depends on
Next, we shift our focus to a more complex family of loci known as epitrochoids, whose general form is depicted in Figure 3A. Epitrochoids are closed-loop roulettes generated by tracing a point fixed at a distance
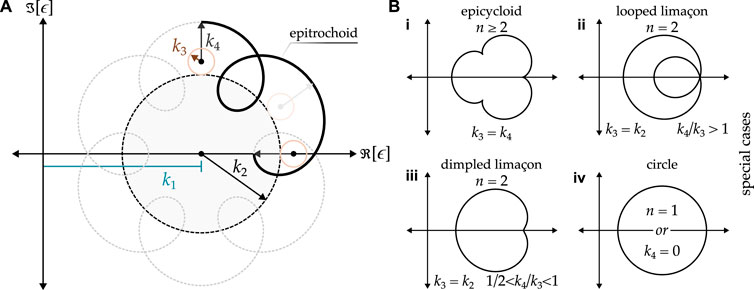
Figure 3. (A) Complex phase locus of an epitrochoid shape, shown for
From the general epitrochoid locus, various special cases can be derived by selecting different sets of parameters. For instance, when
Again, the inverse design of a diatomic lattice with an epitrochoid complex phase locus starts by explicitly stating
To construct the complex phase locus
Substituting Equations 16a, b back into Equation 4 provides the expression of
Following the methodology presented in Section 2.2, the diatomic lattice corresponding to the complex phase locus in Equation 17 is shown in Figure 4. Note that due to the negative stiffness
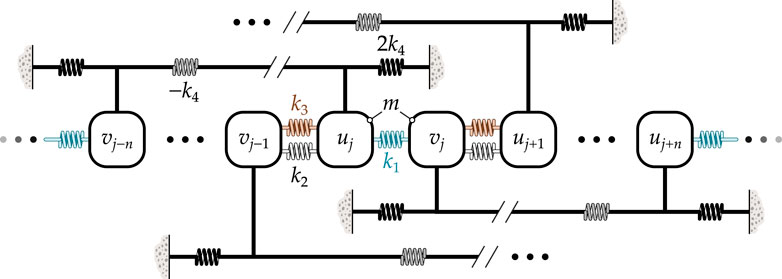
Figure 4. Schematic of a diatomic lattice with coupling beyond next neighbors, resulting in a complex phase locus of epitrochoid shape.
After utilizing
The topology of the diatomic lattice with an epitrochoid locus depends on the choice of the three stiffness parameters
As illustrated in Figure 3, the parameter
Additionally, it is important to note that the maximum number of Dirac points in the first Brillouin zone, corresponding to the number of band crossings when the bandgap is closed, is
For example, consider the case of

Figure 5. Barycentric triangles, showing the winding numbers (left) and bandgap width (right) for
It is worth noting that the bandgap’s width increases significantly near the corners of the Barycentric triangle, which correspond to the cases
To highlight the difference between the topologically distinct regions, five representative combinations of stiffness parameters are selected and listed in Table 2. Their corresponding dispersion relations, calculated using Equation 3 in conjunction with Equation 17, as well as their complex phase loci, are presented in Figure 6.
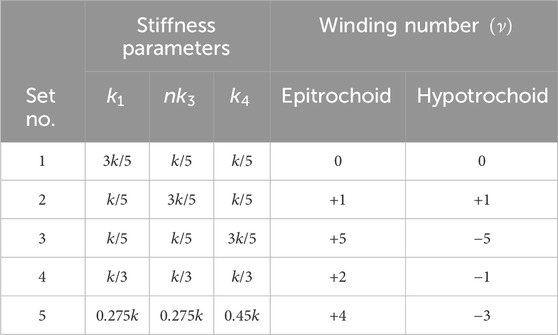
Table 2. Selected combinations of stiffness parameters and their corresponding winding numbers for the lattices with epitrochoid and hypotrochoid complex phase loci, shown in Figures 6 and 10, respectively. Note that

Figure 6. Dispersion relations and complex phase loci of five distinct topological states of epitrochoid diatomic lattices obtained by varying stiffness parameters
This section explores complex phase loci in the shape of hypotrochoids. These closed-loop roulettes are generated like the epitrochoids discussed in Section 2.3, with the definitions of parameters

Figure 7. (A) Complex phase locus of a hypotrochoid shape, shown for
Under special conditions, a generic hypotrochoid complex phase locus can transform into more simplified geometries (Figure 7B). For instance, it becomes a hypocycloid when
Following Equation 4, a hypotrochoid roulette in the complex plane is defined via the following
As in the case of the epitrochoid, the factor multiplied by the wavenumber in the cosine/sine arguments in Equations 20a, b must be an integer. Therefore,
After constructing the diatomic lattice corresponding to
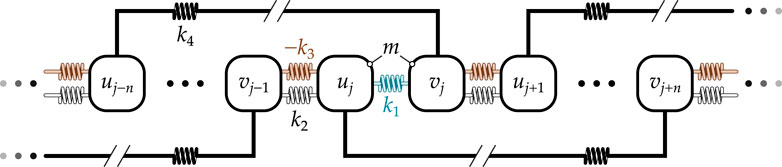
Figure 8. Schematic of a diatomic lattice with coupling beyond next neighbors, resulting in a complex phase locus of hypotrochoid shape.
which, after using
Topological transitions in lattices featuring hypotrochoid loci are achieved by tuning the stiffness parameters
Similar to the epitrochoid complex phase loci, the maximum number of Dirac points in the first Brillouin zone is
Using
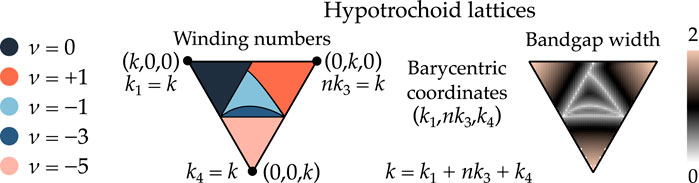
Figure 9. Barycentric triangles, showing the winding numbers (left) and bandgap width (right), for
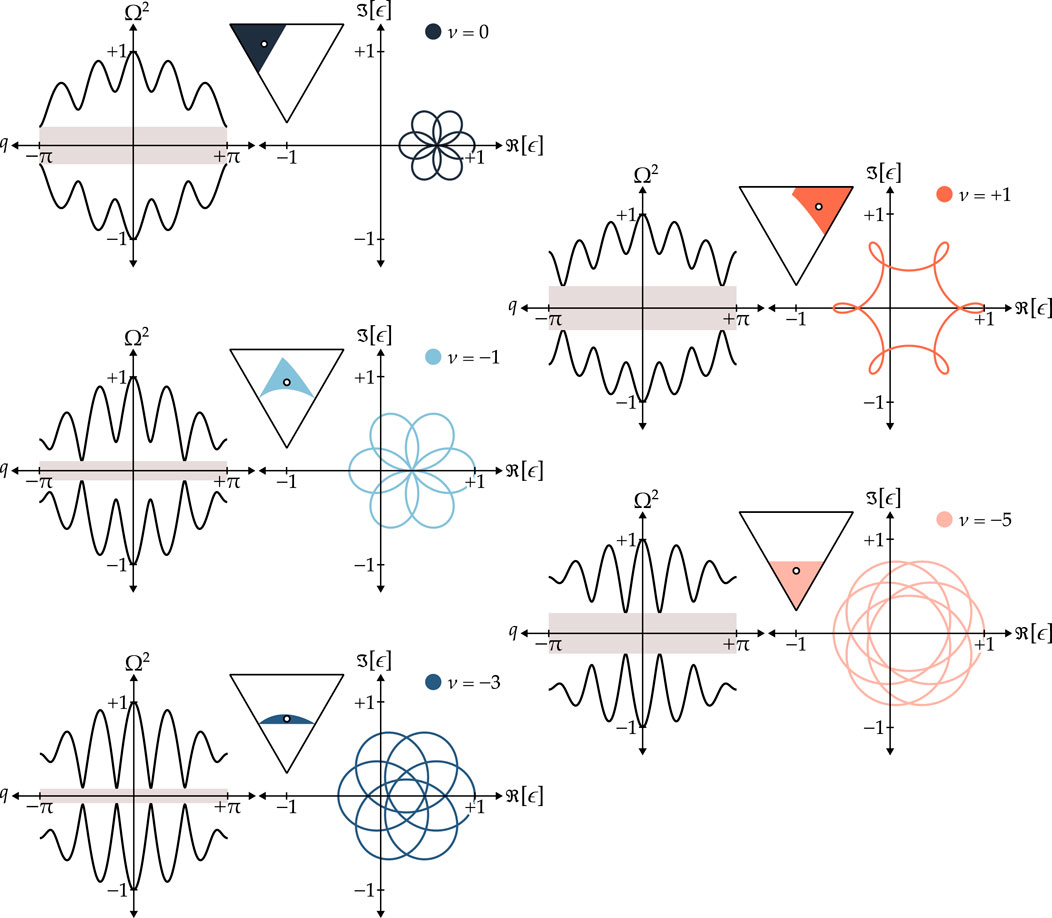
Figure 10. Dispersion relations and complex phase loci of the five distinct topological states of hypotrochoid diatomic lattices when
Previous studies have demonstrated that topological states in one-dimensional phononic crystals are determined not only by the winding numbers but also by the shapes and trajectories of complex phase loci derived from the off-diagonal elements of the chiral stiffness matrices, which is related to the number of Dirac points in the first Brillouin zone (Rajabpoor Alisepahi et al., 2023). In this study, the characteristics that define the shapes and trajectories of complex phase loci are analyzed. Using these characteristics, we inversely designed intricate networks of diatomic lattices. We focused on three categories of complex phase loci: ellipse, epitrochoid, and hypotrochoid. Our analysis revealed that their shapes and trajectories are influenced by interactions between the diatomic lattice’s degrees of freedom beyond the nearest neighbors. The possible winding numbers emerging in each category of complex phase loci have been studied, and parametric studies are compactly represented via barycentric triangles. The implementation of these complex mechanical networks can be achieved by incorporating non-local interactions as shown by Rajabpoor Alisepahi et al. (2023) and Chen et al. (2021).
The methodologies presented in this study can be extended to higher-dimensional systems by analyzing the characteristics of complex phase surfaces or Berry curvatures. These rigorous tools for designing phononic crystals with desired topological characteristics can enable the rapid and accurate design of phononic and acoustic devices with ideal topological properties, such as one-way wave propagation and multiple localized states. These features will advance the development of mechanical and acoustic technologies, including mechanical computing, noise reduction, and energy harvesting, where topological states offer robust solutions for these applications.
The original contributions presented in the study are included in the article/supplementary material; further inquiries can be directed to the corresponding authors.
HA: Conceptualization, Data curation, Formal analysis, Investigation, Methodology, Project administration, Resources, Software, Supervision, Validation, Visualization, Writing–original draft, Writing–review and editing. JM: Conceptualization, Data curation, Formal analysis, Investigation, Methodology, Project administration, Resources, Software, Supervision, Validation, Writing–original draft, Writing–review and editing.
The authors declare that financial support was received for the research, authorship, and/or publication of this article. JM acknowledges the support of the National Aeronautics and Space Administration (NASA Grant 80NSSC23M0071).
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The authors declare that no generative AI was used in the creation of this manuscript.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Al Ba’ba’a, H., Zhu, X., and Wang, Q. (2021). Enabling novel dispersion and topological characteristics in mechanical lattices via stable negative inertial coupling. Proc. R. Soc. A 477, 20200820. doi:10.1098/rspa.2020.0820
Asbóth, J. K., Oroszlány, L., and Pályi, A. (2016). A short course on topological insulators. Lect. notes Phys. 919.
Bahrami, E., and Bahrami, A. (2024). Three-channel acoustic switch based on topological phononic crystals. Eur. Phys. J. Plus 139, 609. doi:10.1140/epjp/s13360-024-05335-4
Cajić, M., Karličić, D., and Adhikari, S. (2024). Inerter-controlled topological interface states in locally resonant lattices with beyond-nearest neighbor coupling. J. Appl. Phys. 135. doi:10.1063/5.0205248
Chen, H., Nassar, H., and Huang, G. L. (2018). A study of topological effects in 1D and 2D mechanical lattices. J. Mech. Phys. Solids 117, 22–36. doi:10.1016/j.jmps.2018.04.013
Chen, Y., Kadic, M., and Wegener, M. (2021). Roton-like acoustical dispersion relations in 3d metamaterials. Nat. Commun. 12, 3278. doi:10.1038/s41467-021-23574-2
Chiu, C.-K., Teo, J. C., Schnyder, A. P., and Ryu, S. (2016). Classification of topological quantum matter with symmetries. Rev. Mod. Phys. 88, 035005. doi:10.1103/revmodphys.88.035005
Christiansen, R. E., Wang, F., and Sigmund, O. (2019). Topological insulators by topology optimization. Phys. Rev. Lett. 122, 234502. doi:10.1103/physrevlett.122.234502
Dal Poggetto, V., Pal, R., Pugno, N., and Miniaci, M. (2024). Topological bound modes in phononic lattices with nonlocal interactions. Int. J. Mech. Sci. 281, 109503. doi:10.1016/j.ijmecsci.2024.109503
Ding, X., Du, Z., Luo, J., Chen, H., Guan, Z., and Guo, X. (2024). Optimal design of fast adiabatic topological pumping in modulated lattices. Appl. Phys. Lett. 124. doi:10.1063/5.0198286
Du, Z., Chen, H., and Huang, G. (2020). Optimal quantum valley hall insulators by rationally engineering berry curvature and band structure. J. Mech. Phys. Solids 135, 103784. doi:10.1016/j.jmps.2019.103784
Fleury, R., Khanikaev, A. B., and Alu, A. (2016). Floquet topological insulators for sound. Nat. Commun. 7, 11744. doi:10.1038/ncomms11744
Grundmann, M. (2020). Topological states due to third-neighbor coupling in diatomic linear elastic chains. Phys. status solidi (b) 257, 2000176. doi:10.1002/pssb.202000176
Haldane, F. D. M. (1988). Model for a quantum hall effect without landau levels: condensed-matter realization of the “parity anomaly”. Phys. Rev. Lett. 61, 2015–2018. doi:10.1103/physrevlett.61.2015
Hasan, M. Z., and Kane, C. L. (2010). Colloquium: topological insulators. Rev. Mod. Phys. 82, 3045–3067. doi:10.1103/revmodphys.82.3045
Jackiw, R., and Rebbi, C. (1976). Solitons with fermion number ½. Phys. Rev. D. 13, 3398–3409. doi:10.1103/physrevd.13.3398
Lee, K. H., Al Ba’ba’a, H., Yu, K., Li, K., Zhang, Y., Du, H., et al. (2022). Magnetoactive acoustic topological transistors. Adv. Sci. 9, 2201204. doi:10.1002/advs.202201204
Li, S.-F., Lu, J.-Y., Zhou, C.-Y.-Y., Zou, X.-Y., and Cheng, J.-C. (2024). Topological logical elements based on defect-mediated sound-wave manipulation. Phys. Rev. Appl. 22, 034025. doi:10.1103/physrevapplied.22.034025
Liu, H., Huang, X., Yan, M., Lu, J., Deng, W., and Liu, Z. (2023). Acoustic topological metamaterials of large winding number. Phys. Rev. Appl. 19, 054028. doi:10.1103/physrevapplied.19.054028
Liu, Y., Xu, Y., Zhang, S.-C., and Duan, W. (2017). Model for topological phononics and phonon diode. Phys. Rev. B 96, 064106. doi:10.1103/physrevb.96.064106
Ma, J., Sun, K., and Gonella, S. (2019). Valley hall in-plane edge states as building blocks for elastodynamic logic circuits. Phys. Rev. Appl. 12, 044015. doi:10.1103/physrevapplied.12.044015
Ma, J., Zhou, D., Sun, K., Mao, X., and Gonella, S. (2018). Edge modes and asymmetric wave transport in topological lattices: experimental characterization at finite frequencies. Phys. Rev. Lett. 121, 094301. doi:10.1103/physrevlett.121.094301
Mousavi, S. H., Khanikaev, A. B., and Wang, Z. (2015). Topologically protected elastic waves in phononic metamaterials. Nat. Commun. 6, 8682. doi:10.1038/ncomms9682
Nash, L. M., Kleckner, D., Read, A., Vitelli, V., Turner, A. M., and Irvine, W. T. (2015). Topological mechanics of gyroscopic metamaterials. Proc. Natl. Acad. Sci. 112, 14495–14500. doi:10.1073/pnas.1507413112
Pal, R. K., Schaeffer, M., and Ruzzene, M. (2016). Helical edge states and topological phase transitions in phononic systems using bi-layered lattices. J. Appl. Phys. 119. doi:10.1063/1.4942357
Pal, R. K., Vila, J., Leamy, M., and Ruzzene, M. (2018). Amplitude-dependent topological edge states in nonlinear phononic lattices. Phys. Rev. E 97, 032209. doi:10.1103/PhysRevE.97.032209
Pirie, H., Sadhuka, S., Wang, J., Andrei, R., and Hoffman, J. E. (2022). Topological phononic logic. Phys. Rev. Lett. 128, 015501. doi:10.1103/physrevlett.128.015501
Politano, A., Viti, L., and Vitiello, M. S. (2017). Optoelectronic devices, plasmonics, and photonics with topological insulators. Apl. Mater. 5. doi:10.1063/1.4977782
Qi, X.-L., and Zhang, S.-C. (2011). Topological insulators and superconductors. Rev. Mod. Phys. 83, 1057–1110. doi:10.1103/revmodphys.83.1057
Rajabpoor Alisepahi, A., and Ma, J. (2024). In-gap edge and domain-wall states in largely perturbed phononic su–schrieffer–heeger lattices. Crystals 14, 102. doi:10.3390/cryst14010102
Rajabpoor Alisepahi, A., Sarkar, S., Sun, K., and Ma, J. (2023). Breakdown of conventional winding number calculation in one-dimensional lattices with interactions beyond nearest neighbors. Commun. Phys. 6, 334. doi:10.1038/s42005-023-01461-0
Roman, S., and Sebastian, D. H. (2015). Observation of phononic helical edge states in a mechanical topological insulator. Science 349, 47–50. doi:10.1126/science.aab0239
Wang, P., Lu, L., and Bertoldi, K. (2015). Topological phononic crystals with one-way elastic edge waves. Phys. Rev. Lett. 115, 104302. doi:10.1103/PhysRevLett.115.104302
Xu, N., Xu, Y., and Zhu, J. (2017). Topological insulators for thermoelectrics. npj Quantum Mater. 2, 51. doi:10.1038/s41535-017-0054-3
Zhou, D., Ma, J., Sun, K., Gonella, S., and Mao, X. (2020). Switchable phonon diodes using nonlinear topological maxwell lattices. Phys. Rev. B 101, 104106. doi:10.1103/physrevb.101.104106
Consider the
Next, we define the degrees of freedom vector
where
where
The constant diagonal elements of
Interestingly, shifting the eigenvalues of matrix
resulting in the following eigenvalue problem:
where
For various values of
Keywords: diatomic lattice, winding number, dispersion analysis, complex phase, inverse design, epitrochoid, hypotrochoid, ellipse
Citation: Al Ba’ba’a HB and Ma JA (2025) Inverse design of topological diatomic lattices based on complex phase locus. Front. Acoust. 3:1529474. doi: 10.3389/facou.2025.1529474
Received: 17 November 2024; Accepted: 18 February 2025;
Published: 02 April 2025.
Edited by:
Raj Kumar Pal, Kansas State University, United StatesReviewed by:
Georgios Theocharis, UMR6613 Laboratoire d’Acoustique de l’Universite du Maine (LAUM), FranceCopyright © 2025 Al Ba’ba’a and Ma. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Hasan B. Al Ba’ba’a, YWxiYWJhYWhAdW5pb24uZWR1; Jihong A. Ma, amlob25nLk1hQHV2bS5lZHU=
†These authors have contributed equally to this work
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.