The prediction of X2B6 monolayers with ultrahigh carrier mobility

Two-dimensional (2D) materials present novel electronic and catalytic performances, showing a promising application as nano-device. In this investigation, a family of 2D material, X₂B₆ (X = K, Na and Rb), is predicted with puckered crystal structure by elemental mutation method. The dynamic and thermal stability of the X₂B₆ monolayer is addressed. The anisotropic mechanical properties of the X₂B₆ monolayer is obtained by the Young’s modulus (296–406 N/m) and the Poisson’s ratio (0.36–0.35). Interestingly, the K₂B₆ and Rb₂B₆ monolayers demonstrate a metallic band structure, while the Na₂B₆ monolayer is a semiconductor with an ultra-narrow bandgap only about 0.42 eV. Then, the ultra-high electron mobility in the Na₂B₆ monolayer is calculated as about 9942 cm².V⁻¹.s⁻¹, and the excellent optical performance of the Na₂B₆ monolayer is also addressed. More importantly, the advantageous catalytic activity in hydrogen evolution reduction (HER) and oxygen evolution reactions (OER) is explored in these X₂B₆ monolayers. Our work suggests a theoretical guidance to use the X₂B₆ monolayer as a high-speed electronic devices and highly efficient catalyst.

Introduction

2D materials have attracted considerable focus after the preparation of the graphene [1], which shows the excellent thermal and catalytic performances [2–4]. While the zero bandgap limits the application of graphene in power devices [5, 6], and then the transition metal dichalcogenides (TMDs) materials are proposed with decent bandgaps larger than that of the bulk one [7]. For example, the MoS₂ monolayer presents novel optical absorption characteristics as a potential photocatalyst [8, 9], which also can be prepared as photoluminescence [10]. In particular, the Janus MoSSe monolayer, as popular asymmetric TMDs, further demonstrates a novel thermal and phononic properties with a polar nature [11–13]. Likewise, 2D Janus materials explain different characteristic on both sides, such as adsorbed [14], catalytic [15], mechanical [16] and electronic properties [17]. All these obtained novel performances of the 2D materials also can be tuned by strain engineering [18, 19], interface coupling [20, 21], external electric field [22] and temperature [23] etc.

Using the nanoscale materials as a catalyst in the hydrogen evolution reaction (HER) and oxygen evolution reaction (OER) is also popular [24–26], because more active sites can be exposed [27–30]. For example, the ability of the OER of the CoO₂ and FeO₂ monolayers can be improved by decreasing 40% overpotential under external strain [31]. The barrier of the biphenylene network in HER is obtained as low as −0.03 eV by the decent atomic doping [32]. The intrinsic defect is also a popular strategy to tune the HER and OER performances of the 2D materials [33]. Besides, to further extend the application range of 2D material, the heterostructure is constructed, which can induce novel electronic and catalytic properties because of the built-in electric field across the interface [17]. PtS₂/arsenene heterostructure is constructed with a −0.487 eV potential for the HER, which is lower than the origin PtS₂ and arsenene monolayers [34]. C₂N/WS₂ heterostructure can facilitates OER with potential of about 1.81 eV [35]. Besides, the prediction of new 2D materials is also an important approach to expand the properties for nano-devices [36, 37]. For example, Ag₂S monolayer acts a semiconductor with auxetic mechanical properties using as nano-electronics [38]. The band edge positions of the SnP₂S₆ monolayer promises the redox potential of the water splitting as a photocatalyst [39]. IV–VI monolayers present ultrahigh carrier mobility, which also act as an excellent HER catalyst [40]. Recently, 2D boron based compound has been proposed to possess excellent electronic and catalytic properties. For example, the Janus B₂P₆ is predicted as potential photocatalyst for water splitting [41], and the band edge energy also can be tuned by external strain [42]. The Li₂B₂ monolayer is calculated with a high hole mobility of 6.8 × 10³ cm²⋅V^{− 1}⋅s^{− 1} using as high-speed electronic devices [43]. The auxetic B₄N monolayer shows an apparent mechanical anisotropy coupled with robust structural stability in future nano-mechanical devices [44]. All these point that exploring the boron (B) based 2D materials as advanced functional material presents significant prospects.

In this work, we propose a novel 2D materials, X₂B₆ (X = K, Na and Rb) monolayer, using elemental mutation method by the prototype of Li₂B₆ monolayer [43]. The stability of the predicted K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is addressed by phonon spectrum and the ab initio molecular dynamics (AIMD) simulations. The mechanical and the electronic performances are investigated by the density functional theory (DFT). Then, the ultra-high electron mobility and the optical light absorption properties are obtained in the Na₂B₆ monolayer. The unique catalytic activity of these X₂B₆ monolayer in HER and OER is studied.

Computational details

All the calculations in this investigation were implemented by Vienna ab initio simulation package (VASP) using first-principle method, which is based on the DFT [45–47]. The core electrons was addressed in the simulations using projector augmented wave potentials (PAW) [48, 49]. The Perdew–Burke–Ernzerhof (PBE) was carried out to demonstrate the exchange correlation method based on the generalized gradient approximation [50–52]. To correct the weak van der Waals interaction in the HER and OER system, the DFT-D3 method was used by Grimme functional [53]. Furthermore, the Heyd–Scuseria–Ernzerhof (HSE06) calculations were explored to investigate the electronic and optical performances of the Na₂B₆ monolayer [54]. It is worth noting that the spin effect was not taken into account in the calculation of electronic properties, because we found that the obtained band structure with the spin turned on and off are exactly the same, shown as Supplementary Figure S1. The Monkhorst–Pack with a k-point grids as 11 × 11 × 1 and 17 × 17 × 1 were used in the relaxation and self-consistent simulations, respectively. The vacuum space was set as 25 Å, which can optimize the interaction of nearby layers. The parameter of the convergence for force and energy are set as 0.01 eVÅ⁻¹ and 0.01 meV, respectively. In the simulation of the phonon spectra, the PHONOPY code was used based on the density functional perturbation theory [55, 56].

Results and discussion

First, the atomic structure of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are predicted with the puckered crystal structure showing a space group of Pca₂₁, by elemental mutation method using the prototype of structure from the Li₂B₆ monolayer [43]. The optimized structure of the X₂B₆ monolayer is presented as Figure 1A and the obtained lattice parameters of the a (or b) in unit-cell of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are 4.311 (or 3.554), 4.313 (or 3.616) Å and 4.312 (or 3.552) Å, respectively, which is smaller than that of the Li₂B₆ monolayer. The bond length of X–B (L_XB) and the B–B (L_BB) in the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are obtained as L_XB = 2.850 Å and L_BB = 5.018 Å, L_XB = 2.523 Å and L_BB = 3.598 Å, L_XB = 2.978 Å and L_BB = 5.450 Å, respectively. Furthermore, the cohesive energy of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is calculated as 6.794 eV/atom, 5.974 eV/atom and 6.048 eV/atom, respectively, which is obtained by (2E_X + 6E_B–E_XB)/8, where E_X, E_B and E_XB are used to present the total energy of an X, B atoms and the X₂B₆ system, respectively. Thus, the calculated cohesive energy of the X₂B₆ system is also larger than that the predicted IV–VI system (about 3.37–3.81 eV/atom) [57] and comparable with the CB monolayer (about 6.13 eV/atom) [58], showing a stability for these K₂B₆, Na₂B₆ and Rb₂B₆ monolayers. Besides, the dynamic stability of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is also studied by phonon spectra obtained in Figure 1B. Obviously, no imaginary frequency can be found in the phonon spectra of these X₂B₆ monolayer, suggesting the dynamic stability of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers. The highest frequency of the optical branch of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is about 34 THz which is smaller than the prototype of the Li₂B₆ system.

Figure 1

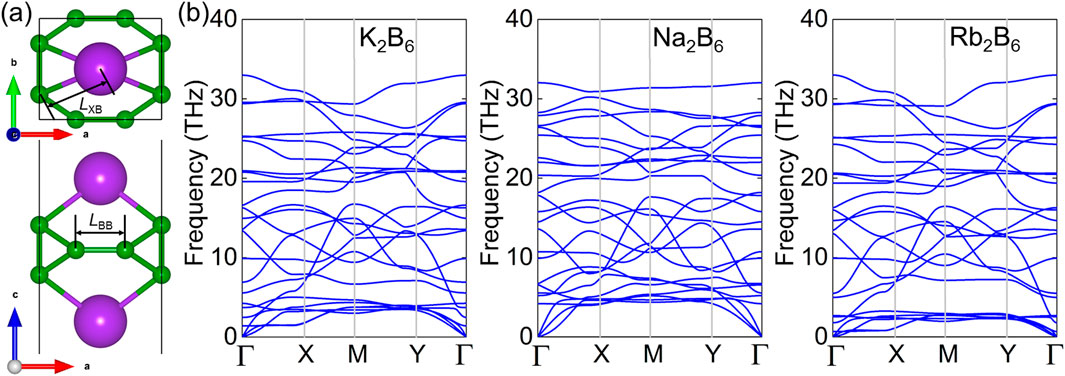
Figure 1. (A) The crystal structure and the (B) phonon spectrum of the X₂B₆ monolayer. The green and the purple atoms are B and X atoms, respectively.

Then, the thermal stability of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is also investigated by the AIMD method by the Nosé−Hoover heat bath functional [59]. The supercell of the X₂B₆ monolayer is obtained as 7 × 4 × 1 to prevent the lattice translational constraints, which also presents 192 atoms [60]. Besides, the structure of the X₂B₆ monolayer is totally relaxed under 300 K and 600 K for 10 ps, after the complete calculations. One can see that the crystal structure of the X₂B₆ monolayer is still undamaged shown as the insets of Figure 2. The temperature and energy of the X₂B₆ monolayer system in the AIMD calculations are also convergent demonstrated as Figure 2, explaining a clear thermal stability at 300 K. Furthermore, the K₂B₆ and Na₂B₆ monolayers are also stable at 600 K because the structure is still intact, while the structure of the Rb₂B₆ monolayer can be melted down at 600 K, shown as Figure 2.

Figure 2

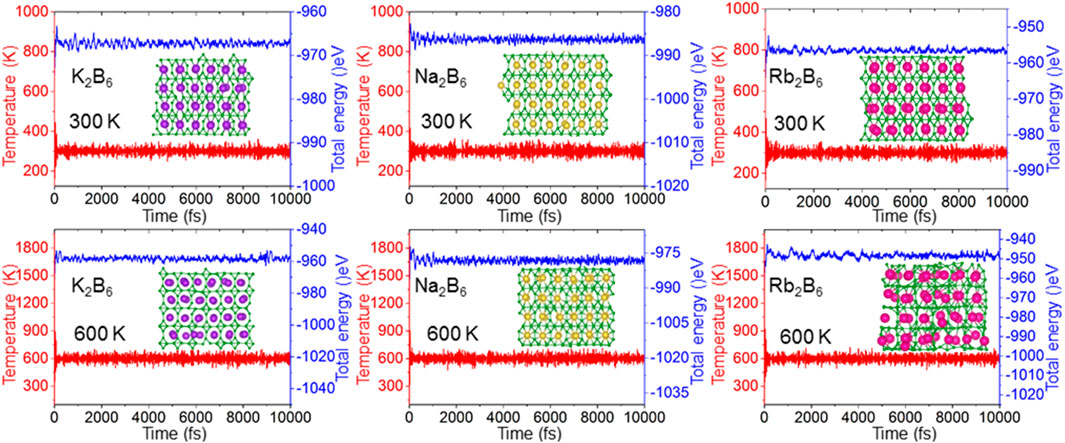
Figure 2. The energy and the temperature of the X₂B₆ monolayer in the AIMD calculations, the inset is the relaxed structure of the X₂B₆ monolayer at 300 K and 600 K for 10 ps.

Then, the mechanical properties of these X₂B₆ monolayer is investigated by the orientation dependences of Young’s modulus using [61] Equation 1 as follows:

$E\left(\theta \right)=\frac{C_{11}{C}_{22}-C_{12}^2}{C_{11}{\sin }^4\theta +C_{22}{\cos }^4\theta +\left(\frac{C_{11}{C}_{22}-C_{12}^2}{C_{66}}-2C_{12}\right){\cos }^2\theta {\sin }^2\theta }(1)$

where θ explains the angle of a direction shown as Figure 1A. The calculated Young’s modulus of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is demonstrated in Figures 3A, C, E, respectively. One can see that K₂B₆, Na₂B₆ and Rb₂B₆ monolayers present the anisotropic Young’s modulus with the maximal and minimal values at θ = 90° and θ = 0°, respectively, shown as Figures 3A, C, E. The obtained maximal Young’s modulus of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are 296 N/m, 397 N/m and 406 N/m, respectively. Then, the orientation dependent Poisson’s ratio of the X₂B₆ monolayer is also studied by [57] Equation 2 as follows:

$v\left(\theta \right)=-\frac{\left(C_{11}+C_{22}-\frac{C_{11}{C}_{22}-C_{12}^2}{C_{66}}\right){\cos }^2\theta {\sin }^2\theta -C_{12}\left({\cos }^4\theta +{\sin }^4\theta \right)}{C_{11}{\sin }^4\theta +C_{22}{\cos }^4\theta +\left(\frac{C_{11}{C}_{22}-C_{12}^2}{C_{66}}-2C_{12}\right){\cos }^2\theta {\sin }^2\theta }(2)$

Figure 3

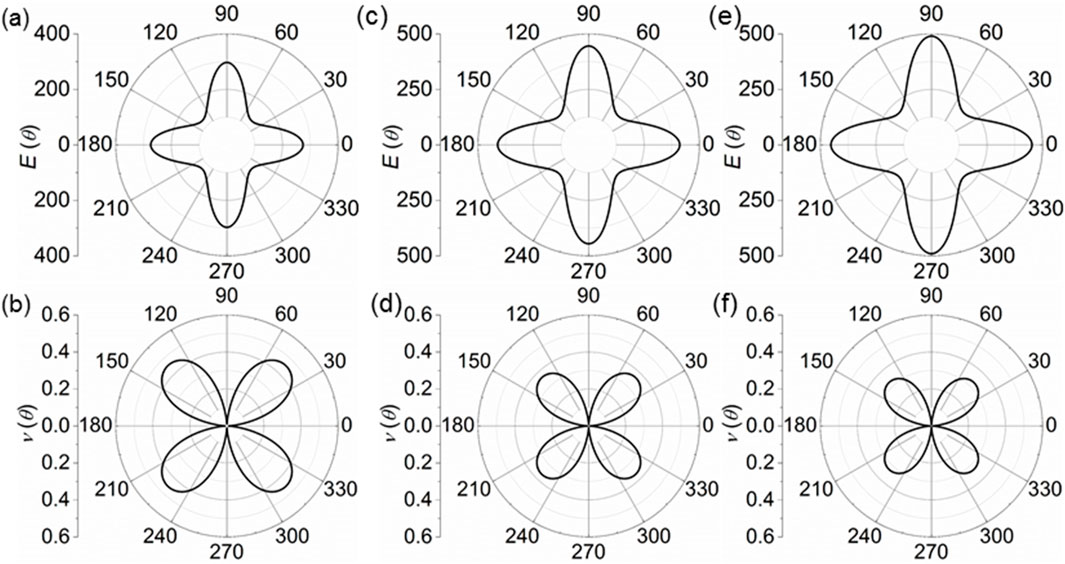
Figure 3. The obtained (A, C, E) Young’s modulus and the (B, D, F) Poisson’s ratio of the (A, B) K₂B₆, (C, D) Na₂B₆ and (E, F) Rb₂B₆ monolayers.

The calculated Poisson’s ratio of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are demonstrated by Figures 3B, D, F, respectively. Obviously, the maximal Poisson’s ratio of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers are obtained as about 0.35, 0.29 and 0.26, respectively, with the θ about 45°. Such obtained Young’s modulus and Poisson’s ratio of the X₂B₆ monolayer is also higher than that of the carbon monochalcogenides [62] and biphenylene [61].

Furthermore, the band structure of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is calculated shown by Figure 4 using PBE method. The K₂B₆ and Rb₂B₆ monolayers present a semi-metallic property, shown as Figures 4A, C, while the Na₂B₆ monolayer suggests semiconductor nature with the direct bandgap that the conduction band minimum (CBM) and the valence band maximum (VBM) are located at the Г point, shown as Figure 4B. In order to obtain a more accurate bandgap of the Na₂B₆ monolayer, HSE06 functional is explored. Interestingly, the Na₂B₆ monolayer presents an ultra-narrow bandgap as about 0.42 eV, smaller than the As₂X₃ system [63], shown as Supplementary Figure S2. It is worth noting that such ultra-narrow bandgap in Na₂B₆ monolayer is also reported in the PbN/CdO heterostructure (about 0.128 eV) [64], which can serve as a promising efficient nano-electronic and catalyst [65, 66]. Besides, the projected band structure of the Na₂B₆ monolayer is also demonstrated by Figure 4B. Obviously, B atoms almost contribute to the band energy comparing with the Na atoms.

Figure 4

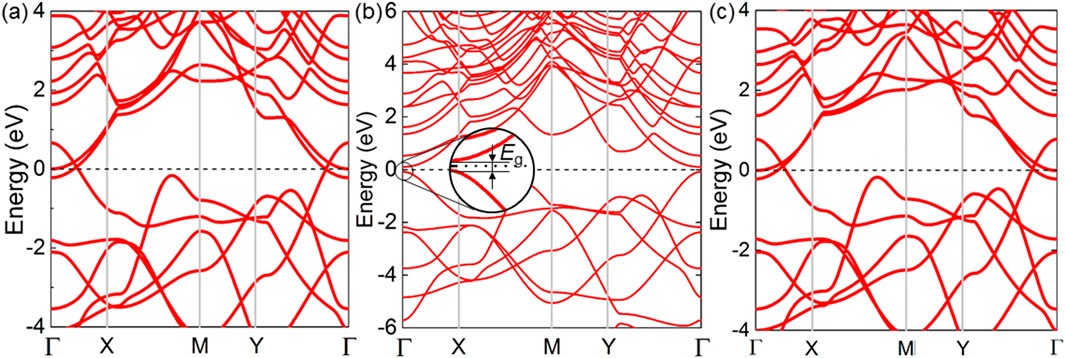
Figure 4. The PBE obtained band structure of the (A) K₂B₆, (B) Na₂B₆ and (C) Rb₂B₆ monolayers. The Fermi level is set as 0 eV.

Since the ultra-narrow bandgap is obtained as about 0.42 eV for the Na₂B₆ monolayer, the potential application as nano-devices is promising. Thus, the carrier mobility of the Na₂B₆ monolayer is necessary to be investigated. The electrons and holes mobility of the Na₂B₆ monolayer along the transport directions (a and b demonstrated in Figure 1A) is explored using the Bardeen-Shockley method [45] which is calculated by Equation 3 as follows:

$\mu =e{\mathrm{\hslash }}^{3}C/\left(k_{\mathrm{B}}T{m}^{*}\sqrt{m_x^{*}{m}_y^{*}}{E}^2\right)(3)$

where elementary charge, the Planck constant and the Boltzmann constant are e, ћ and k_B, respectively. The effective mass of the electron and hole is represented using the m*, which is calculated by Equation 4 as follows:

$m^{*}={\mathrm{\hslash }}^2{\left(\frac{{\mathrm{d}}^2{E}_k}{\mathrm{d}{k}^2}\right)}^{-1}(4)$

where k and E_k are the wave vector and electronic energy, respectively. C represents the elastic modulus of the monolayered Na₂B₆, which is obtained by C = [∂²E/∂((l–l₀)/l₀)/S₀. In this equation, the original lattice constant, the free energy and difference of the lattice constant by the strain are expressed as l, E and l₀, respectively. S₀ is used to represent the area of the Na₂B₆ monolayer. The energy difference of the Na₂B₆ system by the external uniaxial strain is calculated as Figure 5A. Furthermore, E is the potential constant of the Na₂B₆, which is obtained using E = ΔE_edge/((l–l₀)/l₀), where the ΔE_edge is difference of the CBM or VBM energy tuned by external strain in the a or b directions. As shown in Figure 5B, the CBM and the VBM of the Na₂B₆ monolayer can be obviously increased and decreased, respectively, when the external strain is applied. Besides, Figure 5B demonstrates that the dependence of VBM energy of the Na₂B₆ monolayer is obvious under applied strain, suggesting the large potential constant in the Na₂B₆ monolayer for holes.

Figure 5

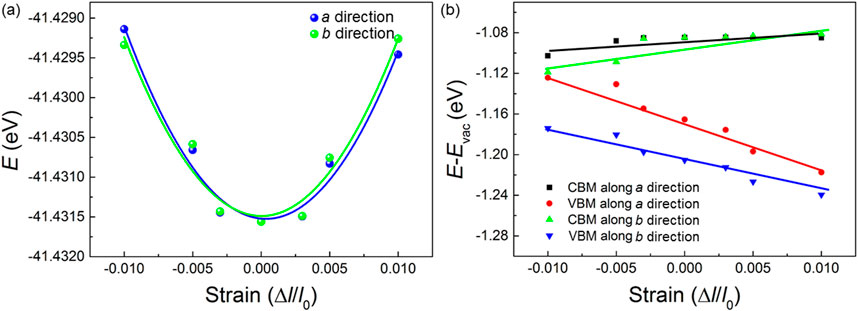
Figure 5. The obtained (A) total and (B) band energy positions of the Na₂B₆ monolayer under different external strain.

Next, the calculated effective mass of the Na₂B₆ monolayer along a and b directions are shown as Table 1. One can see that the effective mass of electrons and holes is relatively uniform in transport direction. The calculated deformation potential constant of the hole is larger than that of the electrons in the Na₂B₆ monolayer shown as Table 1. Besides, the elastic modulus of Na₂B₆ monolayer is also explained as Table 1. It is worth noting that the elastic modulus of the Na₂B₆ monolayer is obtained as 409 N.m⁻¹ and 420 N.m⁻¹, respectively, which is consistent with the previous calculation results of Young’s modulus along a and b directions. Therefore, the apparent isotropic carrier mobility of the Na₂B₆ monolayer is also obtained that electron shows a fast mobility as about 9942 cm².V⁻¹.s⁻¹ and 5486 cm².V⁻¹.s⁻¹ along a and b directions, respectively. While the hole mobility in Na₂B₆ monolayer is calculated as 650 cm².V⁻¹.s⁻¹ and 862 cm² V⁻¹ s⁻¹, along a and b directions, respectively. In the same transport direction, the huge difference between electrons and holes allows them to be effectively separated, about 15 (a direction) times and 6 (b direction) times, suggesting the potential application as photocatalyst. Besides, the calculated electron mobility of the Na₂B₆ monolayer is even higher than that of other 2D materials, such as B₂P₆ monolayer (5888 cm².V⁻¹.s⁻¹) [42], Li₂B₆ monolayer (6800 cm².V⁻¹.s⁻¹) [43] and MoSi₂N₄ (2169 cm².V⁻¹.s⁻¹) [67].

Table 1

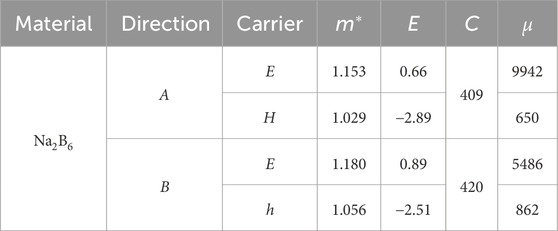
Table 1. The obtained effective mass (m*) and the deformation potential constant (E, eV) of the Na₂B₆ monolayer. The calculated elastic modulus (C, N·m⁻¹) and carrier mobility (μ, cm²·V⁻¹.s⁻¹) of the Na₂B₆ monolayer along transport directions.

Considering the ultra-narrow bandgap obtained for semiconductor of the Na₂B₆ monolayer, the optical absorption spectrum is further calculated by HSE06 method, which is defined as [8] Equation 5 as follows:

$\alpha \left(\omega \right)=\frac{\sqrt{2}\omega }{c}{\left\{{\left[{\epsilon }_1^2\left(\omega \right)+{\epsilon }_2^2\left(\omega \right)\right]}^{1/2}-{\epsilon }_1\left(\omega \right)\right\}}^{1/2}(5)$

where ε₁(ω) shows the real parts and the ε₂(ω) suggests the imaginary part of the dielectric constant. ω is demonstrating the angular frequency. While the complex dielectric function is calculated by ε(ω) = ε₁(ω) + iε₂(ω), where ε₁ can be calculated from ε₂ via the Kramers–Kronig relation. Furthermore, the ε₁(ω) and ε₂(ω) can be decided as Equation 6 as follows:

${\epsilon }_2\left(q\to O_{\widehat{u}},\mathrm{\hslash }\omega \right)=\frac{2e^2\pi }{\mathrm{\Omega }{\epsilon }_0}{\displaystyle \sum _{k,v,c}}\mid \left.⟨|{\mathrm{\Psi }}_k^c\right|{\left.\widehat{u}\cdot r\left|{\mathrm{\Psi }}_k^v\right.〉⟩\right|}^2\times \delta \left(E_k^c-E_k^v-E\right)(6)$

where ${\mathrm{\Psi }}_k$, $E_k$ and $\widehat{u}$ are the wave function, energy and unit vector of the electric field of the incident light. The superscripts (v and c) in ${\mathrm{\Psi }}_k$, $E_k$, label the conduction bands and valence bands, respectively.

Shown as Figure 6A, the optical absorption ability is presented that the light absorption peak of the Na2B6 monolayer is about 11.8 × 105 cm−1 with the wavelength about 335 nm. Such excellent optical absorption performance of the Na2B6 monolayer is more advantages than that of other 2D materials such as AlN/Zr2CO2 heterostructure (3.79 × 105 cm−1) [68], CdO/Arsenene heterostructure (8.47 × 104 cm−1) [69] and SiSe monolayer (7.98 × 105 cm−1) [57].

Figure 6

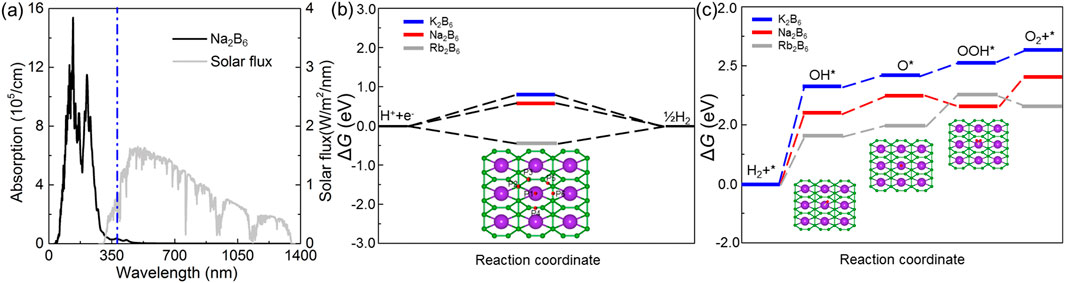
Figure 6. (A) The calculated optical absorption spectrum of the Na₂B₆ monolayer. The free energy of the in the (B) HER and (C) OER of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers. The insets represent the structures of the intermediates in the HER and OER.

Then, the catalytic properties of K2B6, Na2B6 and Rb2B6 monolayers are investigated by calculating the Gibb’s free energy of the system. First, the overall process of HER and the OER in water splitting is demonstrated as Equations 7–10 as follows:

${\mathrm{H}}_2\mathrm{O}+2\mathrm{h}+\to 2{\mathrm{H}}^{+}+1/2\mathrm{O}2(7)$

$2{\mathrm{H}}^{+}+2{\mathrm{e}}^{-}\to {\mathrm{H}}_2(8)$

where the main reactions in the HER process are:

$*+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}\to {\mathrm{H}}^{*}(9)$

${\mathrm{H}}^{*}+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}\to {\mathrm{H}}_2+*(10)$

where * indicates the active site on the Na₂B₆ monolayer. It can be seen that the intermediate product of the HER process is only H*. As an efficient catalyst, its Gibb’s free energy should satisfy ΔG_H = 0 as much as possible. The most excellent Gibb’s free energy in HER of these X₂B₆ monolayer are obtained as Na₂B₆ monolayer, shown as Figure 6B, as about 0.64 eV, which is even lower than that of the MoSi₂N₄ (2.33 eV) [67]. Besides, in the OER reaction, the intermediate products are OH*, O* and OOH*. This process can be expressed as Equations 11–14 as follows:

$*+{\mathrm{H}}_2\mathrm{O}\to {\text{OH}}^{*}+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}(11)$

${\text{OH}}^{*}\to {\mathrm{O}}^{*}+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}(12)$

${\mathrm{O}}^{*}+{\mathrm{H}}_2\mathrm{O}\to {\text{OOH}}^{*}+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}(13)$

${\text{OOH}}^{*}\to *+{\mathrm{O}}_2+{\mathrm{H}}^{+}+{\mathrm{e}}^{–}(14)$

One can see that the rate-determining step in the OER of the K₂B₆, Na₂B₆ and Rb₂B₆ monolayers is first step with the overpotentials about 1.78 eV, 2.19 eV and 2.28 eV, respectively, shown as Figure 6C. The insets in Figure 6C also demonstrated the adsorption configuration of intermediate. Moreover, the calculated OER catalytic activity of these X₂B₆ monolayers is also lower than that of the PtS₂/arsenene heterostructure (5.516 eV) and WSSe monolayer (2.39 eV). It is worth noting that the most stable HER and OER adsorption configuration of these system is demonstrated by binding energy (E_b), which is obtained as E_b = E_system–E_pure–E, where E_system, E_pure and E are the energy of the adsorbed X₂B₆, pure X₂B₆ monolayer and single intermediates, respectively. The lower binding energy imply the more stable configuration of the H*, OH*, O* and OOH*, showing as inset of Figures 6B, C.

Conclusion

In summary, the first-principle calculations are explore to predict the structural, electronic, mechanical, optical and catalytic properties systematically of the novel K₂B₆, Na₂B₆ and Rb₂B₆ monolayers. All these X₂B₆ monolayers present a stability structure, with an anisotropic Young’s modulus (296–406 N/m) and the Poisson’s ratio (0.36–0.35). Then, the ultra-narrow bandgap (0.42 eV) is obtained in the Na₂B₆ monolayer with high electron mobility as about 9942 cm². V⁻¹.s⁻¹. in decent transport direction. Furthermore, the excellent light absorption properties of the Na₂B₆ monolayer is also investigated. All these X₂B₆ monolayers suggest a low Gibb’s free energy in HER and OER, suggesting the potential applications as efficient nanodevice and catalyst.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author contributions

XD: Data curation, Formal Analysis, Funding acquisition, Writing–original draft. ZH: Methodology, Software, Supervision, Writing–review and editing.

Funding

The author(s) declare that financial support was received for the research, authorship, and/or publication of this article. This work was financially supported by Natural science research project of colleges and universities in Anhui Province (Grant No. 2023AH053094); Key teaching research project of Chuzhou Polytechnic (Grant No. 2022jyxm03).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Generative AI statement

The author(s) declare that no Generative AI was used in the creation of this manuscript.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2024.1534301/full#supplementary-material

References

1. Geim AK, Novoselov KS. The rise of graphene. Nat Mater (2007) 6:183–91. doi:10.1038/nmat1849

PubMed Abstract | CrossRef Full Text | Google Scholar

2. Ren K, Chen Y, Qin H, Feng W, Zhang G. Graphene/biphenylene heterostructure: interfacial thermal conduction and thermal rectification. Appl Phys Lett (2022) 121:082203. doi:10.1063/5.0100391

CrossRef Full Text | Google Scholar

3. Wang G, Zhi Y, Bo M, Xiao S, Li Y, Zhao W, et al. 2D hexagonal boron nitride/cadmium sulfide heterostructure as a promising water-splitting photocatalyst. physica status solidi (2020) 257:1900431. doi:10.1002/pssb.201900431

CrossRef Full Text | Google Scholar

4. Ren K, Liu JZ, Palummo M, Sun M. Editorial: theoretical study of two-dimensional materials for photocatalysis and photovoltaics. Front Chem (2024) 12:1387236. doi:10.3389/fchem.2024.1387236

PubMed Abstract | CrossRef Full Text | Google Scholar

5. Jariwala D, Sangwan VK, Lauhon LJ, Marks TJ, Hersam MC. Carbon nanomaterials for electronics, optoelectronics, photovoltaics, and sensing. Chem Soc Rev (2013) 42:2824–60. doi:10.1039/c2cs35335k

PubMed Abstract | CrossRef Full Text | Google Scholar

6. Zhang C, Xu J, Song H, Ren K, Yu ZG, Zhang Y-W. Achieving boron–carbon–nitrogen heterostructures by collision fusion of carbon nanotubes and boron nitride nanotubes. Molecules (2023) 28:4334. doi:10.3390/molecules28114334

PubMed Abstract | CrossRef Full Text | Google Scholar

7. Zhang H, Chhowalla M, Liu Z. 2D nanomaterials: graphene and transition metal dichalcogenides. Chem Soc Rev (2018) 47:3015–7. doi:10.1039/c8cs90048e

PubMed Abstract | CrossRef Full Text | Google Scholar

8. Ren K, Sun M, Luo Y, Wang S, Yu J, Tang W. First-principle study of electronic and optical properties of two-dimensional materials-based heterostructures based on transition metal dichalcogenides and boron phosphide. Appl Surf Sci (2019) 476:70–5. doi:10.1016/j.apsusc.2019.01.005

CrossRef Full Text | Google Scholar

9. Mak KF, Lee C, Hone J, Shan J, Heinz TF. Atomically thin MoS₂: a new direct-gap semiconductor. Phys Rev Lett (2010) 105:136805. doi:10.1103/physrevlett.105.136805

PubMed Abstract | CrossRef Full Text | Google Scholar

10. Splendiani A, Sun L, Zhang Y, Li T, Kim J, Chim C-Y, et al. Emerging photoluminescence in monolayer MoS₂. Nano Lett (2010) 10:1271–5. doi:10.1021/nl903868w

PubMed Abstract | CrossRef Full Text | Google Scholar

11. Ren K, Qin H, Liu H, Chen Y, Liu X, Zhang G. Manipulating interfacial thermal conduction of 2D Janus heterostructure via a thermo-mechanical coupling. Adv Funct Mater (2022) 32:2110846. doi:10.1002/adfm.202110846

CrossRef Full Text | Google Scholar

12. Qin H, Ren K, Zhang G, Dai Y, Zhang G. Lattice thermal conductivity of Janus MoSSe and WSSe monolayers. Phys Chem Chem Phys (2022) 24:20437–44. doi:10.1039/d2cp01692c

PubMed Abstract | CrossRef Full Text | Google Scholar

13. Zhang K, Guo Y, Ji Q, Lu AY, Su C, Wang H, et al. Enhancement of van der Waals Interlayer Coupling through Polar Janus MoSSe. J Am Chem Soc (2020) 142:17499–507. doi:10.1021/jacs.0c07051

PubMed Abstract | CrossRef Full Text | Google Scholar

14. Ren K, Wang K, Zhang G. Atomic adsorption-controlled magnetic properties of a two-dimensional 2D Janus monolayer. ACS Appl Electron Mater (2022) 4:4507–13. doi:10.1021/acsaelm.2c00740

CrossRef Full Text | Google Scholar

15. Wang G-Z, Chang J-L, Tang W, Xie W, Ang YS. 2D materials and heterostructures for photocatalytic water-splitting: a theoretical perspective. J Phys Phys D Appl Phys (2022) 55:293002. doi:10.1088/1361-6463/ac5771

CrossRef Full Text | Google Scholar

16. Ren K, Zhang G, Zhang L, Qin H, Zhang G. Ultraflexible two-dimensional Janus heterostructure superlattice: a novel intrinsic wrinkled structure. Nanoscale (2023) 15:8654–61. doi:10.1039/d3nr00429e

PubMed Abstract | CrossRef Full Text | Google Scholar

17. Zhao L, Huang L, Wang K, Mu W, Wu Q, Ma Z, et al. Mechanical and lattice thermal properties of Si-Ge lateral heterostructures. Molecules (2024) 29:3823. doi:10.3390/molecules29163823

PubMed Abstract | CrossRef Full Text | Google Scholar

18. Zhang L, Ren K, Li J, Cui Z, Cheng H. The First-Principles Study of External Strain Tuning the Electronic and Optical Properties of the 2D MoTe₂/PtS₂ van der Waals Heterostructure. Front Chem (2022) 10:934048. doi:10.3389/fchem.2022.934048

PubMed Abstract | CrossRef Full Text | Google Scholar

19. Wang K, Ren K, Hou Y, Yang D, Zhang G. Strain effects on the magnon-magnon interaction and magnon relaxation time in ferromagnetic CrGeTe₃. Phys Rev Appl (2024) 21:054036. doi:10.1103/physrevapplied.21.054036

CrossRef Full Text | Google Scholar

20. Sun M, Chou J-P, Ren Q, Zhao Y, Yu J, Tang W. Tunable Schottky barrier in van der Waals heterostructures of graphene and g-GaN. Appl Phys Lett (2017) 110:173105. doi:10.1063/1.4982690

CrossRef Full Text | Google Scholar

21. Luo Y, Wang S, Shu H, Chou J-P, Ren K, Yu J, et al. A MoSSe/blue phosphorene vdw heterostructure with energy conversion efficiency of 19.9% for photocatalytic water splitting. Semiconductor Sci Technology (2020) 35:125008. doi:10.1088/1361-6641/abba40

CrossRef Full Text | Google Scholar

22. Shu H, Zhao M, Sun M. Theoretical Study of GaN/BP van der Waals Nanocomposites with Strain-Enhanced Electronic and Optical Properties for Optoelectronic Applications. ACS Appl Nano Mater (2019) 2:6482–91. doi:10.1021/acsanm.9b01422

CrossRef Full Text | Google Scholar

23. Huang L, Ren K, Zhang G, Wan J, Zhang H, Zhang G, et al. Tunable thermal conductivity of two-dimensional SiC nanosheets by grain boundaries: implications for the thermo-mechanical sensor. ACS Appl Nano Mater (2024) 7:15078–85. doi:10.1021/acsanm.4c01803

CrossRef Full Text | Google Scholar

24. Luo Y, Sun M, Yu J, Schwingenschlögl U. Pd4S₃Se₃, Pd4S₃Te₃, and Pd4Se₃Te₃: candidate two-dimensional Janus materials for photocatalytic water splitting. Chem Mater (2021) 33:4128–34. doi:10.1021/acs.chemmater.1c00812

CrossRef Full Text | Google Scholar

25. Luo Y, Ren K, Wang S, Chou J-P, Yu J, Sun Z, et al. First-Principles Study on Transition-Metal Dichalcogenide/BSe van der Waals Heterostructures: A Promising Water-Splitting Photocatalyst. J Phys Chem C (2019) 123:22742–51. doi:10.1021/acs.jpcc.9b05581

CrossRef Full Text | Google Scholar

26. Luo Y, Wang S, Ren K, Chou JP, Yu J, Sun Z, et al. Transition-metal dichalcogenides/Mg(OH)₂ van der Waals heterostructures as promising water-splitting photocatalysts: a first-principles study. Phys Chem Chem Phys (2019) 21:1791–6. doi:10.1039/c8cp06960c

PubMed Abstract | CrossRef Full Text | Google Scholar

27. Wang G, Gong L, Li Z, Wang B, Zhang W, Yuan B, et al. A two-dimensional CdO/CdS heterostructure used for visible light photocatalysis. Phys Chem Chem Phys (2020) 22:9587–92. doi:10.1039/d0cp00876a

PubMed Abstract | CrossRef Full Text | Google Scholar

28. Wang G, Li Z, Wu W, Guo H, Chen C, Yuan H, et al. A two-dimensional h-BN/C₂N heterostructure as a promising metal-free photocatalyst for overall water-splitting. Phys Chem Chem Phys (2020) 22:24446–54. doi:10.1039/d0cp03925j

PubMed Abstract | CrossRef Full Text | Google Scholar

29. Ye H, Ren K, Wang P, Wang L. The investigation of the NH3-SCR performance of a copper-based AEI-CHA intergrown zeolite catalyst. Front Chem (2022) 10:1069824. doi:10.3389/fchem.2022.1069824

PubMed Abstract | CrossRef Full Text | Google Scholar

30. Ren K, Leng B, Zhang C, Sun Q, Tang W. The dynamic investigation of intrinsic vibration characteristics of a stranding machine by the finite element method. Front Phys (2023) 11:203. doi:10.3389/fphy.2023.1159064

CrossRef Full Text | Google Scholar

31. Pu M, Guo Y, Guo W. Strain-mediated oxygen evolution reaction on magnetic two-dimensional monolayers. Nanoscale Horiz (2022) 7:1404–10. doi:10.1039/d2nh00318j

PubMed Abstract | CrossRef Full Text | Google Scholar

32. Ren K, Shu H, Huo W, Cui Z, Xu Y. Tuning electronic, magnetic and catalytic behaviors of biphenylene network by atomic doping. Nanotechnology (2022) 33:345701. doi:10.1088/1361-6528/ac6f64

PubMed Abstract | CrossRef Full Text | Google Scholar

33. Ouyang Y, Ling C, Chen Q, Wang Z, Shi L, Wang J. Activating inert basal planes of MoS₂ for hydrogen evolution reaction through the formation of different intrinsic defects. Chem Mater (2016) 28:4390–6. doi:10.1021/acs.chemmater.6b01395

CrossRef Full Text | Google Scholar

34. Ren K, Tang W, Sun M, Cai Y, Cheng Y, Zhang G. A direct Z-scheme PtS₂/arsenene van der Waals heterostructure with high photocatalytic water splitting efficiency. Nanoscale (2020) 12:17281–9. doi:10.1039/d0nr02286a

PubMed Abstract | CrossRef Full Text | Google Scholar

35. Kumar R, Das D, Singh AK. C₂N/WS₂ van der Waals type-II heterostructure as a promising water splitting photocatalyst. J Catal (2018) 359:143–50. doi:10.1016/j.jcat.2018.01.005

CrossRef Full Text | Google Scholar

36. Zhu Z, Guan J, Liu D, Tománek D. Designing isoelectronic counterparts to layered group V semiconductors. ACS Nano (2015) 9:8284–90. doi:10.1021/acsnano.5b02742

PubMed Abstract | CrossRef Full Text | Google Scholar

37. Li R, Cheng Y, Huang W. Recent progress of Janus 2D transition metal chalcogenides: from theory to experiments. Small (2018) 14:1802091. doi:10.1002/smll.201802091

PubMed Abstract | CrossRef Full Text | Google Scholar

38. Peng R, Ma Y, He Z, Huang B, Kou L, Dai Y. Single-layer Ag₂S: a two-dimensional bidirectional auxetic semiconductor. Nano Lett (2019) 19:1227–33. doi:10.1021/acs.nanolett.8b04761

PubMed Abstract | CrossRef Full Text | Google Scholar

39. Jing Y, Zhou Z, Zhang J, Huang C, Li Y, Wang F. SnP₂S₆ monolayer: a promising 2D semiconductor for photocatalytic water splitting. Phys Chem Chem Phys (2019) 21:21064–9. doi:10.1039/c9cp04143e

PubMed Abstract | CrossRef Full Text | Google Scholar

40. Huang Z, Ren K, Zheng R, Wang L, Wang L. Ultrahigh carrier mobility in two-dimensional IV–VI semiconductors for photocatalytic water splitting. Molecules (2023) 28:4126. doi:10.3390/molecules28104126

PubMed Abstract | CrossRef Full Text | Google Scholar

41. Sun M, Schwingenschlögl U. B₂P₆: a two-dimensional anisotropic Janus material with potential in photocatalytic water splitting and metal-ion batteries. Chem Mater (2020) 32:4795–800. doi:10.1021/acs.chemmater.0c01536

CrossRef Full Text | Google Scholar

42. Ren K, Shu H, Huo W, Cui Z, Yu J, Xu Y. Mechanical, electronic and optical properties of a novel B₂P₆ monolayer: ultrahigh carrier mobility and strong optical absorption. Phys Chem Chem Phys (2021) 23:24915–21. doi:10.1039/d1cp03838a

PubMed Abstract | CrossRef Full Text | Google Scholar

43. Ren K, Yan Y, Zhang Z, Sun M, Schwingenschlögl U. A family of Li_xB_y monolayers with a wide spectrum of potential applications. Appl Surf Sci (2022) 604:154317. doi:10.1016/j.apsusc.2022.154317

CrossRef Full Text | Google Scholar

44. Wang B, Wu Q, Zhang Y, Ma L, Wang J. Auxetic B₄N monolayer: a promising 2D material with in-plane negative Poisson's ratio and large anisotropic mechanics. ACS Appl Mater Inter (2019) 11:33231–7. doi:10.1021/acsami.9b10472

PubMed Abstract | CrossRef Full Text | Google Scholar

45. Van de Walle CG, Martin RM. Absolute’’deformation potentials: formulation and ab initio calculations for semiconductors. Phys Rev Lett (1989) 62:2028–31. doi:10.1103/physrevlett.62.2028

PubMed Abstract | CrossRef Full Text | Google Scholar

46. Capelle K. A bird's-eye view of density-functional theory. Braz J Phys (2006) 36:1318–43. doi:10.1590/s0103-97332006000700035

CrossRef Full Text | Google Scholar

47. Grimme S, Antony J, Ehrlich S, Krieg H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J Chem Phys (2010) 132:154104. doi:10.1063/1.3382344

PubMed Abstract | CrossRef Full Text | Google Scholar

48. Kresse G, Furthmüller J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys Rev B (1996) 54:11169–86. doi:10.1103/physrevb.54.11169

PubMed Abstract | CrossRef Full Text | Google Scholar

49. Kresse G, Joubert D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys Rev B (1999) 59:1758–75. doi:10.1103/physrevb.59.1758

CrossRef Full Text | Google Scholar

50. Oganov AR, Glass CW. Crystal structure prediction using ab initio evolutionary techniques: principles and applications. J Chem Phys (2006) 124:244704. doi:10.1063/1.2210932

PubMed Abstract | CrossRef Full Text | Google Scholar

51. Kresse G, Furthmüller J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comp Mater Sci (1996) 6:15–50. doi:10.1016/0927-0256(96)00008-0

CrossRef Full Text | Google Scholar

52. Perdew JP, Burke K, Ernzerhof M. Generalized gradient approximation made simple. Phys Rev Lett (1996) 77:3865–8. doi:10.1103/physrevlett.77.3865

PubMed Abstract | CrossRef Full Text | Google Scholar

53. Grimme S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J Comput Chem (2006) 27:1787–99. doi:10.1002/jcc.20495

PubMed Abstract | CrossRef Full Text | Google Scholar

54. Heyd J, Peralta JE, Scuseria GE, Martin RL. Energy band gaps and lattice parameters evaluated with the Heyd-Scuseria-Ernzerhof screened hybrid functional. J Chem Phys (2005) 123:174101. doi:10.1063/1.2085170

PubMed Abstract | CrossRef Full Text | Google Scholar

55. Togo A, Tanaka I. First principles phonon calculations in materials science. Scripta Materialia (2015) 108:1–5. doi:10.1016/j.scriptamat.2015.07.021

CrossRef Full Text | Google Scholar

56. Togo A, Oba F, Tanaka I. First-principles calculations of the ferroelastic transition between rutile-type and CaCl₂-type SiO₂ at high pressures. Phys Rev B (2008) 78:134106. doi:10.1103/physrevb.78.134106

CrossRef Full Text | Google Scholar

57. Ren K, Ma X, Liu X, Xu Y, Huo W, Li W, et al. Prediction of 2D IV–VI semiconductors: auxetic materials with direct bandgap and strong optical absorption. Nanoscale (2022) 14:8463–73. doi:10.1039/d2nr00818a

PubMed Abstract | CrossRef Full Text | Google Scholar

58. Ren K, Shu H, Huang L, Wang K, Luo Y, Huo W, et al. Predicted XN (X = C, Si, Ge, and Sn) monolayers with ultrahigh carrier mobility: potential photocatalysts for water splitting. J Phys Chem C (2023) 127:21006–14. doi:10.1021/acs.jpcc.3c06284

CrossRef Full Text | Google Scholar

59. Nosé S. A unified formulation of the constant temperature molecular dynamics methods. J Chem Phys (1984) 81:511–9. doi:10.1063/1.447334

CrossRef Full Text | Google Scholar

60. Ren K, Wang S, Luo Y, Chou J-P, Yu J, Tang W, et al. High-efficiency photocatalyst for water splitting: a Janus MoSSe/XN (X = Ga, Al) van der Waals heterostructure. J Phys Phys D Appl Phys (2020) 53:185504. doi:10.1088/1361-6463/ab71ad

CrossRef Full Text | Google Scholar

61. Luo Y, Ren C, Xu Y, Yu J, Wang S, Sun M. A first principles investigation on the structural, mechanical, electronic, and catalytic properties of biphenylene. Scientific Rep (2021) 11:19008. doi:10.1038/s41598-021-98261-9

PubMed Abstract | CrossRef Full Text | Google Scholar

62. Sun M, Schwingenschlögl U. Unique omnidirectional negative Poisson’s ratio in δ-phase carbon monochalcogenides. J Phys Chem C (2021) 125:4133–8. doi:10.1021/acs.jpcc.0c11555

CrossRef Full Text | Google Scholar

63. Fujiwara K, Shibahara M. Thermal transport mechanism at a solid-liquid interface based on the heat flux detected at a subatomic spatial resolution. Phys Rev (2022) 105:034803. doi:10.1103/physreve.105.034803

PubMed Abstract | CrossRef Full Text | Google Scholar

64. Cheng Z, Wang Y, Zheng R, Mu W. The prediction of two-dimensional PbN: opened bandgap in heterostructure with CdO. Front Chem (2024) 12:1382850. doi:10.3389/fchem.2024.1382850

PubMed Abstract | CrossRef Full Text | Google Scholar

65. Wang J, Li Y, Deng L, Wei N, Weng Y, Dong S, et al. High-performance photothermal conversion of narrow-bandgap Ti₂O₃ nanoparticles. Adv Mater (2017) 29:1603730. doi:10.1002/adma.201603730

PubMed Abstract | CrossRef Full Text | Google Scholar

66. Vo TQ, Kim B. Interface thermal resistance between liquid water and various metallic surfaces. Int J Precision Eng Manufacturing (2015) 16:1341–6. doi:10.1007/s12541-015-0176-0

CrossRef Full Text | Google Scholar

67. Ren K, Shu H, Wang K, Qin H. Two-dimensional MX₂Y₄ systems: ultrahigh carrier transport and excellent hydrogen evolution reaction performances. Phys Chem Chem Phys (2023) 25:4519–27. doi:10.1039/d2cp04224j

PubMed Abstract | CrossRef Full Text | Google Scholar

68. Ren K, Zheng R, Lou J, Yu J, Sun Q, Li J. Ab initio calculations for the electronic, interfacial and optical properties of two-dimensional AlN/Zr₂CO₂ heterostructure. Front Chem (2021) 9:796695. doi:10.3389/fchem.2021.796695

PubMed Abstract | CrossRef Full Text | Google Scholar

69. Ren K, Zheng R, Yu J, Sun Q, Li J. Band bending mechanism in CdO/arsenene heterostructure: a potential direct Z-scheme photocatalyst. Front Chem (2021) 9:788813. doi:10.3389/fchem.2021.788813

PubMed Abstract | CrossRef Full Text | Google Scholar