Olivera J. Đokić
Marija M. Vorkapić- Faculty of Education, Department of Didactics of Mathematics, University of Belgrade, Belgrade, Serbia
Introduction: In their study, the authors delve into the impact of preschool curriculum on the spatial abilities development of children. They specifically explore the significance of spatial understanding through Piaget’s primacy of topological concepts and the increasing emphasis on projective geometry. The research focuses on preschoolers in Serbia, aged 5.5–6.5, as they transition to primary school.
Methods: The study involves 103 children exposed to two distinct preschool curricula: The General Foundations of the Preschool Curriculum (GFPC) and The Foundations of the Preschool Curriculum – Years of Ascent (YA). Drawing on the typology of spatial tasks in the 2 × 2 matrix, the researchers conducted individual structured interviews comprising 8 tasks. These tasks were designed to assess children’s performance across four sub-domains: Intrinsic–Static, Extrinsic–Static, Intrinsic–Dynamic, and Extrinsic–Dynamic.
Results: The findings reveal a general poor performance in solving spatial tasks among the children. Despite inconsistent results in the cognitive structures of spatial ability, the study indicates that the type of preschool program or gender does not significantly influence preschoolers’ spatial abilities in Serbia. Notably, differences were observed in the sub-domains of Static and Dynamic. However, the analysis suggests that the 2 × 2 model may not be an appropriate fit for the Serbian sample.
Discussion: The authors acknowledge the limitations of the 2 × 2 model and propose further exploration of alternative typologies to better characterize children’s spatial skills development in Serbia. They advocate for a model that accurately describes preschoolers’ spatial abilities and can inform curriculum creators in enriching mathematics education. The study underscores the importance of spatial reasoning for success in STEM fields, as supported by meta-analyses of children’s spatial abilities.
1 Introduction
The tradition of the preschool institutions in Serbia is over 150 years long and, in this period, there have been conceptual changes in the curricula. During this period many twists and turns occurred, conditioned by the situation in Serbia, as well as the leading pedagogical thought and the philosophy of education. As Stamatović (2021) and Stojanović and Bogavac (2016) note, in the long tradition and multi-decade trends toward modern pedagogical discourse in the preschool education, what we have now is the construction and co-construction of children’s knowledge and understanding of the world around them.
According to Buđevac and Kovačević (2020) and Stojanović et al. (2018), the long-standing tradition of preschool education in Serbia is reflected in the establishment of first “playschools” in which educational work was organized under the influence of the prevailing European pedagogical movements based first on Froebelian, and then on Montessori traditions. The main goal and purpose of these playschools was to educate children of preschool age (up to the age of 7 in Serbia). The position and function of the preschools was defined along with the task of teaching the children of ages 5–7 through play and organized activities, with an emphasis on the importance of physical and mental life, as well as the enculturation of the preschool children. In the period between the two World Wars, Froebel’s system of preschool education continued to influence considerably the development of kindergartens throughout Europe and the world, and soon the term ‘kindergarten’ substituted the term ‘playschool’. Further development of organized preschool education followed the development of pedagogical thought and translations of pertinent literature from foreign languages. After the Word War II, Soviet pedagogy provided conditions for the overall development and education of children in state educational institutions and it exerted great influence on the concept of preschool institutions. The focus was on the elements of the socio-cultural historical theory of learning, which incorporates the experience of children and the adults (Stojanović et al., 2018). Initial curriculums for children’s education included some didactic-methodological instructions as a form of educational work with preschool children, and it was not earlier than 1959 that the function of the preschool institutions was defined. Serbia entered a period of turbulent socio-political, economic, and cultural transformations. Actually, the development of the preschool institutions in Serbia, as in the majority of European countries, went in two directions: institutions with a predominantly social (care) function (infant, nursery) and institutions with a predominantly educational function (kindergartens, preschools).
This means that nowadays in Serbia we have the understanding of the child’s development in the sociocultural approach in the synthesis of the ideas of Piaget, Vygotsky, and Dewey, and the perception of childhood as a worthy and visible social category (Stojanović et al., 2018). The new strategy for the development of preschool education in Serbia was adopted in 2018 (ME, 2018). However, as we shall see, as pedagogical priorities shifted, mathematical content in the preschool curricula did not remain stable.
2 Theoretical background
2.1 Curriculum transformations in the early childhood education in Serbia
Olson (1994) observes that the constructivist or developmental-humanistic paradigm was accepted as the basis for theoretical concepts of preschool education, while Stojanović et al. (2018) claim that it lead to the implementation of appropriate methodological programs for educational work in nurseries and kindergartens in Serbia. Since 2006, the preschool education of the children of age 3–7 in Serbia has provided a detailed description of two equal models, Model A and Model B (ME, 2006) which derive from official Organization for Economic Co-operation and Development (OECD) and European Union (EU) documents. The two approaches reflect the dichotomy of the preschool curriculum, i.e., constructivists vs. instructivists in which the role of children and the way of learning differs from the role of the teacher. The Model A curriculum follows the constructivist approach where children’s learning through interaction with their environment, construct their knowledge independently and in cooperation with others. This ‘open’ approach follows children’s choices rather than pre-offered stimuli prepared by teachers in opportunities for learning. The Model B curriculum follows the instructivist approach where the preschool teachers have a critical role in encouraging kids to participate in thoughtfully organized activities that promote systematic learning and advancement in a number of developmental domains. These objectives are sometimes expressed as educational standards or learning outcomes, which are linked to the fundamental know-how and abilities that act as the foundation for further education and learning (Bertrand, 2007).
Since 2018, the curriculum-related changes have occurred (ME, 2018). According to Stojanović et al. (2018), the new idea is centered on preschoolers’ general well-being as well as a thorough understanding of integrated learning as the result of activity such as games, real-world activities, and purposeful learning. The previous practice of thematic planning, where a preschool teacher (as an expert on the subject) was responsible for children’s learning, while the goal of learning was the acquisition of new or expansion of the existing knowledge, was replaced with project planning, in which the goal of learning is to seek answers to questions that children perceive as challenging, while teachers and children share responsibility. In other words, in the previous concept (ME, 2006), the structure of the content was taken from some school subjects, for example, in mathematics, geometric shapes and figures, spatial relations (left–right, up-down, in front of-behind, and so on), and the success of the curriculum was measured by the level of the adoption of the specific knowledge. Milinković and Vorkapić (2021) claim that, in the new concept, preschool teachers do not work on the content of separate areas, they create opportunities for learning where children explore and get to know different areas of human knowledge and make connections between old and new experience. These are old and new preschool education curricular frameworks.
Clearly, the two above-mentioned concepts of preschool education are in a certain contrast in the conceptual sense. Namely, when it comes to mathematical contents, in the old concept (Model A and Model B), the goals, content, and even activities contributing to the development of mathematical concepts are clearly stated. Specifically, the goals are “the ability to perceive space in relation to one’s own body and the position of certain parts of the body… the positions of various objects and the positions of objects in relation to other objects,” “the elementary ability to transfer a real (natural) appearance of the terrain and the arrangement of objects on it onto a model,” “the ability to identify directions of movement through space in practical situations” (ME, 2006, p. 158–159). These goals provide an array of different aspects of spatial abilities that children should develop at preschool age, but one can actually discern an idea of different spatial skills that, put together, will eventually form children’s spatial abilities (further in the paper these are, for example: spatial scaling, perspective-taking, and so on.). On the other hand, the new curriculum does not state concrete goals, nor the contents that should be operationalized in order to develop mathematical, specifically spatial, concepts, thus preventing children’s spatial thinking. The new curriculum states that mathematical competencies of children are developed through practical manipulation, problem-solving, research activities, games, and practical situations useful in life that encourage logical-mathematical thinking and understanding of mathematical concepts (ME, 2018).
2.2 Children’s spatial reasoning in early education
Based on the review of the recent research within the Proceedings of the Eleventh Congress of the European Society for Research in Mathematics Education [CERME11], conference “A Mathematics Education Perspective on Early Mathematics Learning between the Poles of Instruction and Construction “[POEM4] and 13th International Congress on Mathematical Education [ICME13], we follow the directions of Björklund et al. (2020) for the research on early mathematics teaching and learning how the spatial and geometric thinking (reasoning) of children develops.
In the period of early childhood there is still not a sufficient focus on the development of spatial and geometry thinking (e.g., Uttal and Cohen, 2012; Sinclair and Bruce, 2014). However, there are several reasons to believe that this situation is changing for the better. A first indication of this trend is extensive recent research showing a strong link between children’s spatial abilities and their performance in mathematics (e.g., Newcombe, 2010; Gilligan et al., 2019; Tam and Chan, 2022), which in turn increases the possibility of a better performance in STEM disciplines (science, technology, engineering and math) (e.g., Wai et al., 2009; Young et al., 2018). Secondly, there is an increasing number of indicators that children start school with a developed informal spatial reasoning (e.g., Bryant, 2008; Ishikawa and Newcombe, 2021), which in further mathematics education is frequently not supported by the curricula in which the requirements for the development of numerical and algebraic thinking are predominant.
In short, there is an extensive literature pointing to the link between the performance in mathematics and specific spatial skills (e.g., Lubinski, 2010; Mix and Cheng, 2012; Young et al., 2018; Tam and Chan, 2022). When examining the mechanism of the association between spatial ability in general and mathematical performance, each domain of the ability (or specific spatial skills) should be separately examined, as shown in the meta-analysis of Xie et al. (2020). However, spatial skills such as mental rotation, spatial visualization, and others are widely elaborated on in the literature. There is little evidence for other specific spatial skills, such as perspective taking, map reading, and others such (e.g., Clements and Sarama, 2007; Hawes et al., 2019). The literature is also focused on older children and adolescents (e.g., Hawes et al., 2017; Young et al., 2018), leaving us with relatively little information on the relationship between spatial abilities and mathematics performance in younger children. The paper with which we started our research on the spatial skills of preschool children in Serbia is the paper that looks at the skills of the preschool children in perspective taking (Vorkapić et al., 2022; Đokić and Vorkapić, 2023). With this paper, we continue this practice, taking the steps in terms of ongoing monitoring and critical assessment of the practice of the new preschool curriculum in Serbia.
2.3 The model for the typology of children’s spatial skills used in the research
From the review of the results of factor and meta-analyses (in the period 1925–1979), we singled out the following spatial skills identified by the factor analysts: (1) Spatial Orientation, (2) Mental Rotation and (3) Spatial Visualization (Mix and Cheng, 2012); and based on the review of the results of the meta-analysis (in the period 1975–2011) the spatial skills are: (1) Disembedding, (2) Spatial Visualisation, (3) Mental Rotation, (4) Spatial Perception and (5) Perspective Taking (adapted from Uttal et al., 2013); and five domains of spatial ability have been identified in the current research, a meta-analysis of 73 studies: (1) Intrinsic-Dynamic, (2) Intrinsic-Static, (3) Extrinsic-Dynamic, (4) Extrinsic-Static and (5) Visual–Spatial memory (Xie et al., 2020).
In Harris (2021) opinion, despite the evidence that spatial reasoning of children is the key for mathematics, especially in early childhood, the clear absence of the development of spatial abilities and spatial skills is still evident in mathematics classes. Thus Davis and Spatial Reasoning Group (2015) state that the fact that mathematics curricula do not emphasize spatial thinking gives ample reason for concern. According to Clements and Sarama (2011), geometry content is often limited to sorting and naming 2D shapes, while Frick et al. (2013) note that children are able to investigate geometric transformations of 2D and 3D shapes through mental rotation or in other similar ways.
Earlier, Newcombe (1989) found that many studies rejected Piagets’ idea of egocentrism and age limitation, and Newcombe and Huttenlocher (1992) showed that even younger preschoolers could show the ability to take different perspectives of the object. Apart from Newcombe’s and her associates’ extensive research on the limitations of the primacy of Piaget’s topological concepts in younger children, the critiques of the topological primacy theory can also be found, for example, in the papers of Thom et al. (2021) and Alkouri (2022). This opens the necessary path in terms of research of the ways in which Piaget’s theory and other theories emphasizing the intuitive nature of knowledge can notify children’s spatial reasoning by means of projective concepts formed almost simultaneously with the topological ones.
Thom et al. (2021) claim that the studies of mathematics education clarify the physical aspects of children’s spatial reasoning, including movements, drawings, and such like. Therefore, body and perception are actually the tools that children conceptual thinking stems from and changes. Thom et al. (2021) emphasize that there is a significant research opportunity in this area for advancing spatial reasoning in projective geometry, particularly in terms of the spatial processes that are familiar to children. Note that Thom et al. (2021) point out that not only the perception of two-dimensional and three-dimensional shapes and their transformations are part of early mathematics education. There should be more than only two-dimensional shapes used in geometry education. Opposite to that, initial geometry education should be grounded in a close examination of the three-dimensional reality of the surroundings. Later, as youngsters get older, the observation of the three-dimensional situation should be expanded to (and at the same time, reinforced with) other activities, namely focusing on the connection between the three-dimensional space and its two-dimensional plane representation (Đokić, 2018). According to Poincaré (1905), objects should be portrayed exactly as they are, that is, how they appear on the retina of our eyes, on a piece of paper, on a computer or TV screen.
Therefore, we looked for answers in the studies and meta-analyses of children’s spatial abilities (Mix and Cheng, 2012; Uttal et al., 2013; Xie et al., 2020), as well as in the history of mathematics education (Chorlay et al., 2022; Đokić and Vorkapić, 2023). We are following investigatively the conceptual change approach from topological primacy to projective geometry. According to Beth and Piaget (1974), the primacy of topology evident in the children of preschool and early primary school age, and Poincaré’s and Piaget’s views on physical and geometrical space point to the reliance on intuition directly reflected in sensation and imagination, and later on the process of induction. It represents “an awareness of space, at a level not yet formalized” (Piaget and Inhelder, 1956, p. 448). All this precedes geometrical reasoning. Jahnke et al. (2022, p. 1424) state that “Poincaré was of the opinion that students should somehow follow the ‘trail of the explorer’ to cultivate the faculty of intuition.” Our conclusion is that studying the concept of geometrical space is very important for teaching and learning geometry. Although three-dimensional objects are a part of everyday life, in geometry teaching they are most often represented by using two-dimensional images. Our understanding of the three-dimensional geometry is closely related to understanding the two-dimensional geometry. Therefore, the mismatch between the knowledge of geometrical space and intuitive representative space can be the root of a deep misunderstanding of space (Đokić, 2014).
This idea lead us to the Newcombe’s study on pupils’ spatial abilities (2018), whose typology of spatial tasks we consider suitable for the age we are examining and in accordance with the ideas of physical and geometric space of Piaget and Poincaré. Relying on Poincaré’s and Piaget’s understandings, as well as on the 2 × 2 typology of spatial skills by Newcombe (2018), we designed models of mathematics tasks to examine the children’s spatial abilities. Based on the reasoning of Uttal et al. (2013) and adopting the Newcombe and Shipley’s (2015) theory-driven framework for classifying spatial tasks, the static-dynamic domain from Newcombe’s categorization of spatial skills (2018) reflects the observation that spatial tasks sometimes involve objects arranged in stable positions (static), and sometimes involve moving objects in movement (dynamic). At the same time, the intrinsic-extrinsic domain reflects the observation that spatial relations can be inherent to an object and its parts (intrinsic) or they can exist among several objects or between an object and its context (extrinsic). By crossing the two dimensions, we get a typology matrix 2 × 2 with four types of spatial skills (see Table 1).
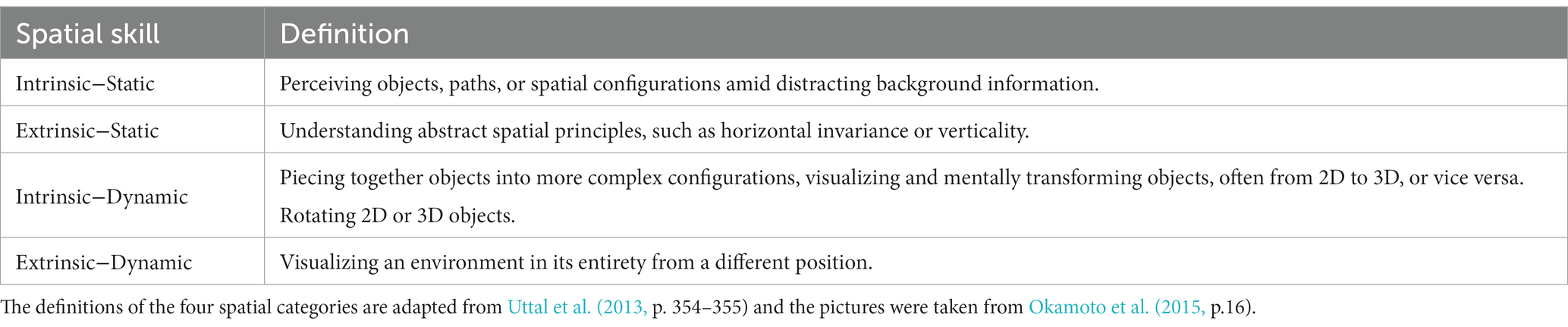
Table 1. Definitions and examples of the tasks in the typology matrix 2 × 2 for four types of spatial skills.
As the literature on spatial knowledge offers strong support to the typology of the tasks in the 2 × 2 matrix (Newcombe, 2018), we selected this one to see if the model can be suitable as a guideline for enriching the mathematics curriculum in Serbia by including children’s spatial reasoning as its important element in the process of mathematical reasoning based on the development of four spatial skills in children (in the domains of the 2 × 2 typology, through four stated sub-domains). This led to our research questions:
1. How was the 2 × 2 typology model tested as a potential classification of the preschool children’s spatial skills, and what associations were observed between the curriculum type and spatial skills, between spatial skills and gender, between spatial skill domains, and between spatial skill sub-domains in this study?
2. What were the findings when testing the performance on tasks related to different domains of spatial skills and the performance on tasks related to different subdomains of spatial skills?
3 Methodology
The aim of the research is to examine Serbian preschool children’s spatial abilities using the typology model 2 × 2 of spatial skills.
The research sample involved the children from Serbia, age 5.5–6.5 (n = 103, 51 boys). The children were included in two different curricula. The General Foundations of the Preschool Curriculum (GFPC) was the official curriculum from 2006 until 2022 and the implementation of The Foundations of the Preschool Curriculum–Years of Ascent (YA) began in 2019 (preschool institutions used one of two possible curricula from 2019 until 2022, and then gradually all kindergartens adopted the new YA curriculum, lasting 3 years; we opted for the last year in which some kindergartens were still working in line with the old curriculum, GFPC). As of 2022, only one curriculum has been in use, the YA. Both curricula were official legislative documents that were valid at the same time during the three-year period. The first part of the research was conducted during May 2021, when the children attended the preparatory preschool program, while the second part of the research was conducted during May and June 2022, when the same children attended the first grade of primary school. Out of five kindergartens in the deliberate sample, there were two kindergartens involved in the YA (55 children). One of the kindergartens was in the rural part of Serbia (37 children), while the other four were in suburban areas.
We used interview as the research technique, along with using the protocol of the structured interview in which children’s responses were recorded. The questions of the interview can be found in the Supplementary Appendix 1. The list of interview tasks was originally compiled for the purposes of our research. Each of the tasks is taken from the relevant literature (see Table 2) intended for preschool children age 5–6. We used a 2 × 2 typology matrix for the four types of spatial skills from Newcombe (2018). The interview involved 2–5 questions for each task, based on which we received qualitative answers from the children. The children were not time-limited when it came to answers, while the next question was asked when the child expressed that he/she did not know the answer. Each interview lasted 12–15 min (the total time depends on whether or not the child continues with answering a question, i.e., if he/she declines to answer the question in full). Spatial skills are measured by the variable that is made regarding the number of the correct answers on all tasks. Hence it is an ordinal variable with values 0 (0 correct answers) to 6 (max number of correct answers that children provided). A task with a correct answer was scored with 1, while incorrect ones were scored with 0. We also monitored children’s reasoning that could lead to a correct answer, but when they did not, they were scored as incorrect. The aspects of children’s spatial reasoning will not be discussed in this paper.
Table 2. Spatial tasks for preschool children.
4 Results
First, we will present the data for the correlations on the spatial skills tasks, followed by performances on the tasks of different spatial skills domains and sub-domains.
4.1 Correlations on the spatial skills in the matrix 2 × 2
Table 3 shows the data on the mean and standard deviations on each of the 8 tasks in the matrix 2 × 2 and Table 2 comprises all spatial tasks for preschool children used in this research.
Table 3. Descriptive statistics for the spatial tasks for preschool children.
The range of points in all 8 tasks is 0–6 (out of 8 points). Not a single child did all the tasks. The domain of Dynamic skills and the domain of Intrinsic skills do not have all the tasks completed.
We calculated the Cronbach’s alpha coefficient of 2 × 2 items (0.088) and it is very low. When we calculated the Inter-Item Correlation (the scale with the number of items less than 10), we got the value 0.009, and among the tasks from −0.28 to 0.26. This means that the correlations among all 8 tasks is small. However, given the heterogenous nature of spatial items, such low Cronbach’s alpha coefficient can be expected (number of items 8) (Cortina, 1993).
Table 3 shows that the lowest score was in the Spatial Scaling task, while the highest score was in the Spatial Tests of Horizontal task. Both tasks belong to the same sub-domain of spatial skills − Extristic−Static. In the task Spatial Tests of Horizontal (Table 2) children were supposed to identify which of the five tilted jars with water is in good position relative to horizontal plane. Experience of this kind is quite common among the children of preschool age. Unlike the Spatial Tests of Horizontal, the task Spatial Scaling (Table 2) required that children should identify which of the four smaller images on the computer screen matches the one on a piece of paper (on the piece of paper, next to the boy there is a square marked on the square grid 6 × 6, while the images of the four pieces of paper on the computer screen do not contain square grids, only the square in a certain position). Spatial Scaling tasks generally undergo some serious changes among the children of this age, therefore it is not surprising that they underachieved (Newcombe et al., 2018). We can wonder how often we put children in such learning situations. Generally speaking, the children solved the tasks poorly, even in terms of the domains and sub-domains of spatial skills.
4.2 Association between the spatial skills in the matrix 2 × 2 and curriculum type
Chi-square Test for independence was used for examining the association between the curriculum type and the spatial skills of children. Given that, in some cells of the contingency table 2 × 2 the frequencies were under 5, we used Fisher’s Exact Test. The results show that there is no statistically significant association between the curriculum type and the spatial skills (p = 0.443), that is, children’s spatial skills do not depend on either of the two curricula applied in their learning process among the children in our sample. This study has failed to show such a connection.
4.3 Association between the spatial skills tasks in the matrix 2 × 2 and gender
Chi-Square Tests, respectively Fisher’s Exact Test were also used for examining the association between the gender and the spatial skills of children. The value of p is 0.332 and this indicates that there is no statistically significant association between gender and spatial skills among the children in our sample. This research has failed to show such a connection.
4.4 Association between the spatial skills domains in the matrix 2 × 2 and association between the spatial skills sub-domains in the matrix 2 × 2
The scores obtained on all measurements are not significantly associated. This study has failed to show such a connection. Intrinsic-Dynamic and Extrinsic-Dynamic skills are the only exception.
It can be concluded that the associations among the spatial skill sub-domains are present only in Intrinsic-Dynamic and Extrinsic-Dynamic skills (p = 0.052). The association between them is small (effect size is 0.194). The tasks Mental Rotation and Mental Folding are significantly associated with the tasks Perspective-taking Task − Appearance: Object-to-Object Orientation and Perspective-taking Task − Appearance: Self-to-Object Orientation. The tasks of imagining the position of two boys on a merry-go-round and imagining what a folding paper looks like are associated with the tasks of identifying what the back of the house looks like when viewed from its front side and identifying which of the photos represent what the boy who occupies a certain position in space can see.
4.5 Performance on tasks of different spatial skills domains in the matrix 2 × 2 and performance on tasks of different spatial skill sub-domains in the matrix 2 × 2
We investigated whether there are differences between some domains and sub-domains of the preschool children’s spatial skills. Table 4 shows if there are differences in the distribution of frequencies for the domains of Intrinsic and Extrinsic skills, as well as Static and Dynamic ones.
Table 4. Differences in performance on domains of spatial skills.
As far as the domains of Intrinsic and Extrinsic skills are concerned, the results show the following: the value of the Chi-Square Test of Homogeneity is low, there is no statistically significant difference in the distribution of frequencies of the two domains (p = 0.530). This means that children equally frequently did the tasks belonging to both domains of Intrinsic and Extrinsic skills. On the other hand, regarding the domains of Static and Dynamic skills, the value of the Chi-Square Test of Homogeneity is high, there is a statistically significant difference in the distribution of frequencies (p = 0.001).
We used a cross-tabulation to analyze the relationship between these two variables, Static and Dynamic skills (Table 5). A contingency table (or cross-tabulation) is a type of table in a matrix format that displays the (multivariate) frequency distribution of the variables of the Static and Dynamic skills.
Table 5. Differences in performance on static and Dynamic spatial domains.
In the cases where children did not solve any of the four Static or Dynamic tasks respectively, more children did not solve Dynamic tasks, which means that these tasks were more difficult for them than the Static ones. The children were more successful at solving the Static tasks and at solving one or two tasks, but not three or four tasks in both domains. Although the children did better on the Static skills tasks, no child solved all the tasks in this domain, and yet one child solved all four Dynamic skills tasks. We can say that the children solved all four tasks extremely poorly as far as the domains of Static-Dynamic skills are concerned, but the same was true for the domain of Intrinsic-External skills (where there was not even a statistically significant difference).
Apart from differences in the above-mentioned domains, we investigated if there were differences related to all sub-domains of spatial skills. The results are presented in Table 6.
Table 6. Differences in performance on sub-domains of spatial skills.
Statistically significant differences in the distribution of the sub-domains (p > 0.05) have been identified. These are the differences between Intrinsic-Static and Intrinsic-Dynamic skills (p = 0.143), as well as differences between Intrinsic-Static and Extrinsic-Dynamic skills (p = 0.348).
Table 7 shows the differences between Intrinsic-Static and Extrinsic-Static skills.
Table 7. Differences in performance on intrinsic-static and extrinsic-static skills.
The value of the Chi-Square Test of Homogeneity is high, there is a statistically significant difference in the distribution of frequencies of these sub-domains (p = 0.004). A cross-tabulation shows the relationship between the Intrinsic-Static and Extrinsic-Static skills. In the cases where children did not solve two tasks belonging to both sub-domains respectively, more children did not solve the tasks from the sub-domain of Intrinsic-Static skills, compared to Extrinsic-Static skills. This sub-domain was equally difficult for children even when they solved one task from both sub-domains. The smallest number of children solved both tasks in both sub-domains, whereby children underperformed again when solving the tasks from the sub-domain of Intrinsic-Static skills. Therefore, children are more successful at solving the tasks of Extrinsic-Static skills, compared to the Intrinsic-Static skills tasks.
Regarding the differences between Intrinsic-Dynamic and Extrinsic-Static skills (Table 8), there is a statistically significant difference in the distribution of frequencies in these sub-domains (p = 0.029).
Table 8. Differences in performance on intrinsic-dynamic and extrinsic-static skills.
In cases where children did not solve two tasks from both sub-domains respectively, there are more unsolved tasks from the sub-domain of Intrinsic-Dynamic skills. When the children solved one task from these sub-domains, the tasks involving the Intrinsic-Dynamic skills were more difficult for the children. In the end, the children who solved two tasks from both sub-domains were more successful at solving the Intrinsic-Dynamic skills tasks. Therefore, both the children whose performance was poor and the children whose performance was good are from the sub-domain of Intrinsic-Dynamic skills in relation to Extrinsic-Static skills.
In addition, we observed that there is a statistically significant difference in the frequency distribution of Intrinsic-Dynamic and Extrinsic-Dynamic skills (p = 0.010) (Table 9).
Table 9. Differences in performance on intrinsic-dynamic and extrinsic-dynamic skills.
When the children did not solve the two tasks belonging to both sub-domains, there were more unsolved tasks on Extrinsic-Dynamic skills. They were equally difficult for children when they solved one task from both sub-domains, as well as when they solved all tasks. Therefore, the children were more successful at solving the tasks of Intrinsic-Dynamic skills compared to Extrinsic-Dynamic skills.
Finally, the value of the Chi-Square Test of Homogeneity is high, there is a statistically significant difference in the frequency distribution between Extrinsic-Dynamic and Extrinsic-Static skills (p = 0.000) (Table 10).
Table 10. Differences in performance on extrinsic-dynamic and extrinsic-static skills.
Among the children who did not solve any of the two tasks in both sub-domains, more children did not solve the tasks of Extrinsic-Dynamic skills. The performance of the children who solved one task from these two sub-domains, respectively, was higher for Extrinsic-Static skills, as well as when they solved both tasks in these two sub-domains. Therefore, the children are more successful at solving the Extrinsic-Static skills tasks in relation to Extrinsic-Dynamic skills.
5 Discussion and conclusion
Now we will summarize the results of our research on the sample of children from Serbia and open some questions for its further course. The Structure Interview and the tasks with questions were based on the cited references. Based on the obtained data, our conclusion is that the children solved the tasks poorly (M = 2.37, SD = 1.30) (max. 8 pts. on 8 tasks), and not a single child from our sample did all the tasks (the range of points is 0–6). The most difficult tasks are from the Dynamic and Intrinsic domains. There were children who tried to solve these tasks, but they failed.
The children from our sample had the lowest performance in two tasks: Spatial Scaling and Mental Rotation. Despite the poor results of the children from our sample in these tasks, this is not the case in some other research dealing with the preschoolers (e.g., Mix et al., 2018). Our results related to Spatial Scaling also do not match the results of the preschoolers in Gilligan et al. (2019). Generally speaking, children have a problem with this skill (e.g., Mix et al., 2018; Newcombe et al., 2018). Our results on Mental Rotation tasks do not match the results on the same tasks for preschoolers in Yang et al. (2020). However, the mixed findings about spatial scaling skill and mental rotation skill put us in a position of questioning. Mix et al. (2018) found that training in a specific sub-skill transfers learning that occurs later, including in school. For example, Mix et al. (2016) found that mental rotation was the best predictor of mathematical performance in the kindergarten. Each of the aforementioned studies used spatial skills tasks in the same 2 × 2 typology intended for children aged 5–6 years. Although three-dimensional objects are a part of everyday life, in geometry teaching they are most often represented by using two-dimensional images. In everyday situations, a child has to imagine and give an answer to the question of how he/she sees the view from his/her or somebody else’s position on the objects, going through the entire process of imagining and representing from 3D space to its 2D representation. The tasks in our interview involve the stimulus of a 2D picture representing a group of objects physically located in a 3D space. These objects are first perceived and then connected to the 2D representation (as the eye sees it, in Poincaré’s words (1905)). Thus, Đokić and Vorkapić (2023) tried to find the answer in the history of mathematics, particularly in Poincaré’s conceptual definition of space as geometric one and its representation in physical space; that is the idea of the formation of the concept of space by examining the interrelations of objects. In doing this, we are helped by physical space and the intuitive knowledge that it offers, representing an image, the representation of the geometric space. Children’s spatial skills and the development of these skills are important because they enable them to form geometric space. Only then, for example, Piaget’s task (“Three Mountains Task,” Table 1 Extrinsic−Dynamic spatial skill) involving 3D space and the relations in it can be placed in the 2D plane, as well as the image of the relations among the objects in it. Poincaré gives us a broader concept that we trace in the history of mathematics. Assembling simple objects into complex one, their visualizing and mental transformation, from two-dimensional to three-dimensional or vice versa, as well as rotating two-dimensional or three-dimensional objects is a complex task for preschool children. This means that the researchers need to focus on two understandings of the formation of geometrical space: Poincaré’s, which highlights the important role of experiments for children, complemented by Piaget’s activities that help the development of the representative space. Therefore, it is necessary to understand the substructure of cognitive domains and spatial skills in order to improve the child’s development of spatial ability.
There is no association between the children’s spatial skills and the preschool education curriculum that the children attended, nor is there an association between the children’s spatial skills and the gender of the children. An increasing number of studies does not report gender-based effects (Hodgkiss et al., 2021). Neither of the two curricula, YA or GFPC, enabled the development of children’s spatial skills, and we should look for the elements of the curriculum that need to be improved in order to develop children’s spatial abilities, thereby improving children’s spatial reasoning and creating a good basis for the school curriculum (although Vorkapić et al. (2022), in their results, also state the weak foundations of the school curriculum for spatial reasoning of children in primary education in Serbia). This raises the question of the curriculum in Serbia from the perspective of developing spatial abilities, especially if we bear in mind that it is one of the essential requirements for STEM disciplines (e.g., Newcombe et al., 2013; Gilligan et al., 2019; Cui and Guo, 2022; Tam and Chan, 2022). Gilligan-Lee et al. (2022) provide a broader view of curricular changes from the perspective of developing children’s spatial abilities. Despite the data provided, many curricula throughout the world continue to place insufficient emphasis on spatial thinking, as Gilligan-Lee et al. (2022) observed. Notably, neither the curriculum in England (UK) nor the Common Core State Standards for Mathematics, which are used in many U.S. states, specifically specify any particular spatial abilities. In contrast, spatial information is given more weight in Australian basic curriculum. It is important to note that lately a number of nations have included spatial thinking in their curricula. As an illustration, the 2020 Ontario (Canada) Mathematics curriculum contains a specific ‘spatial sense’ sub-domain for all age groups and clearly acknowledges spatial thinking as essential for success in STEM areas. Similar approaches can be found in the Netherlands curruculum (Van den Heuvel-Panhuizen and Buys, 2008) and Cyprus curriculum (Cyprus Ministry of Education and Culture, 2010). As evidenced earlier, in the long tradition of preschool education in Serbia, spanning more than 150 years, mathematics contents in the curriculum have not been stable and the normative approach to children’s development has been lost. What is the continuity in the mathematics curriculum for primary grades for the development of spatial thinking? Here are the results of the analysis of Serbian curriculum that relate to spatial thinking according to Vorkapić et al. (2022):
1. At preschool age, children are focused on exploring the space around them, that is, in a real environment.
2. In the 1st grade of primary school, the outcomes for seven-year-old students state that they are able to determine the mutual position of objects and beings and their positions in relation to the ground, as well as to notice and name the geometric shapes of objects in the immediate environment.
3. In the 2nd and 3rd grades of primary school, the outcomes that explicitly refer to the development of spatial thinking are not stated.
4. In the 4th grade of primary school, the outcome related to the recognition of the pictorial representation of the body viewed from different sides is stated.
The question arises as to how many educators/teachers put the students in different situations that require positions of perception and description of different spatial situations. For example, Pollitt et al. (2020) found that when the spatial reasoning assessment activities were enacted frequently and with consistency, teacher beliefs about spatial thinking changed rapidly. From pre-school onwards, children/students should be provided with learning opportunities to further develop their spatial skills. This is an indicator that students, in the continuity of mathematics education through curricula in Serbia, are not clearly supported in the development of spatial reasoning. Consequently, Đerić et al. (2021) observe that the fourth-grade students from Serbia showed significant deficiencies in the form and space domains compared to other mathematical sub-domains on international tests such as TIMSS 2015 and 2019. The results show that Serbian students’ achievement on tasks of three-dimensional shapes, including the connection to their two-dimensional representations, is below the TIMSS average. According to Vorkapić et al. (2022), in Serbia, such approaches in mathematics curricula are not sufficiently recognized and, therefore, represent potentially unequal opportunities for learning and developing both spatial ability and spatial reasoning ability. This is consistent with what Sinclair and Bruce (2014) claim, children are expected to master the competencies that are increasingly (if not entirely) becoming irrelevant, while the ones that are increasingly becoming necessary are ignored, such as spatial abilities. Ironically, it seems that the instability of the curricula is reflected in the opposite interests of the interested parties, forgetting that the curriculum is the result, not an input. In this sense, as Sinclair and Bruce (2014) point out, it cannot serve as a mechanism for effecting changes, it can only co-evolve with shifts in beliefs and expectations.
Out of all measured associations for domains and sub-domains, a statistically significant association, and a relatively small one, was found only for Intrinsic-Dynamic and Extrinsic-Dynamic skills (p = 0.052). Obviously, there were no significant associations, which was surprising and it is contrary to the results of Mix et al. (2018).
According to the children’s answers to 8 interview tasks/questions, the following differences between the domains were identified. Statistically significant differences exist only between the domains of Static and Dynamic skills (χ2 = 18.70; p = 0.001). Regarding the pairs of the spatial skill sub-domains, statistically significant differences were found in four pairs: Extrinsic-Dynamic and Extrinsic-Static skills (χ2 = 22.53; p = 0.000), Intrinsic-Static and Extrinsic-Static skills (χ2 = 11.24; p = 0.004); Intrinsic-Dynamic and Extrinsic-Dynamic skills (χ2 = 9.26; p = 0.010) and Intrinsic-Dynamic and Extrinsic-Static skills (χ2 = 7.06; p = 0.029).
The domains of Dynamic skills and Intrinsic skills were the two domains where children did not solve all the tasks. This means that the tasks which develop the skills related to these domains are too difficult for preschool children in Serbia. We observed that some children, although they did well on the tasks related to one domain, for instance, the Static skills domain, underperformed on all tasks in the Dynamic skills domain. We also noticed that some sub-domains included both the children who solved the tasks well (as extremes) and the children who did not, for example, in the sub-domain of Intrinsic-Dynamic skills relative to Extrinsic-Static ones.
Given the heterogeneous nature of spatial items, such a low Cronbach’s alpha coefficient (0.088) was not surprising. Alpha is not the extent to which the items are interrelated (Cortina, 1993; Hoekstra et al., 2019). It is a function of the extent to which the items in a test have high communalities and thus low uniqueness. This does not imply unidimensionality or homogeneity. Cognitive structures of spatial ability yield inconsistent results (Mix et al., 2018). And yet, the research by Mix et al. confirms the theoretical model of the typology of 2 × 2 tasks. Our results show that the 2 × 2 model does not give a frequency distribution that is statistically significant. The only differences we found were for the sub-domains Static and Dynamic. The analysis indicated that the model 2 × 2 (Newcombe, 2018) was not the fitting model considering the statistics for our sample from Serbia. According to the results of our sample, although there are different spatial abilities (internal, external, and others), they are not connected and do not represent the aspects of one ability that children have. The children did not have any intervention, namely, no systematic work was done on the development of these abilities/skills. The question is whether the systematic work with children would result in their increased ability in every aspect so that it would affect the connection between abilities/skills, and therefore a better ‘fit’ of the model 2 × 2 on such a sample. We open the question of a model that would describe better the development of spatial abilities of preschool children in Serbia.
To learn more about possible links between spatial abilities and performance in STEM subjects, it was important to testing the 2 × 2 model (Mix et al., 2018). If spatial skills have several dimensions, these dimensions could have different correlations with mathematics in general or with particular mathematical tasks. The results encourage future research into the relationship between static-dynamic processing and STEM achievement in the early phases of the development. Though Mix et al. (2018) got intrinsic-extrinsic domain differences, we did not obtain statistically significant differences in the frequency distribution of children’s responses to tasks from these domains but we did in static-dynamic. We found for static-dynamic processing that it reflects the observation of the spatial tasks that:
1. Sometimes involve objects arranged in stable positions - static:
1.1 A complex geometric figure and the simple ones from which it is composed;
1.2 A straight line and its features;
1.3 Forward and backward counting;
1.4 An object and its features;
1.5 A figure in a square grid and its features, position of a figure on a square grid and so on.
2. And sometimes involve moving object in movement - dynamic:
2.1 Assemble/disassemble a complex figure consisting of the simple ones;
2.2 Draw a straight line using a ruler;
2.3 Count with a skip;
2.4 Measure the length of an object with a given, non-standard unit of measure, extending it without making gaps, and so on;
2.5 Map the points and figures in a square grid based on the given instructions.
Recently, researchers have pointed to a solid research base linking spatial ability to a long-term achievement in STEM courses and argue that the time has come for educators to capitalize on this powerful connection (e.g., Lubinski, 2010; Newcombe, 2010; Uttal et al., 2013; Xie et al., 2020).
There is also a problem of spatial skills typology (and the requirements or tasks we give to children) in specific domains and sub-domains. Newcombe (2018) claims that one way to conceptualize, for example, “the crucial difference between navigation and object manipulation is that navigation concerns the extrinsic spatial relations among objects, with wider frames of reference, whereas object manipulation acts upon the intrinsic spatial relations that constitute the structure of objects” (Newcombe, 2018, p. 524). The distinction between these relations is one key aspect of recent proposals about the structure of spatial skills (illustrated in Table 1). However, the seriousness of the issue of the typology of children’s spatial skills model from the perspective of which task develops a specific skill, is reflected in the argument of Newcombe et al. (2018) that spatial scaling (which belongs to one sub-domain) may engage similar spatial transformation processes as mental rotation (which belongs to another sub-domain), which leave the intrinsic structure and configuration of an object or layout intact while changing its spatial relation to the observer (apparent distance or orientation, respectively). More generally, a task might match multiple categories because different strategies for solving a spatial task engage different processing, leading to individual differences in the categorization itself. This means that children’s strategies for solving spatial tasks should be monitored while solving tasks and classified later into a specific (primary) category. Thus, Newcombe and Shipley (2015) discussed the importance of both intrinsic and extrinsic spatial skills for STEM success. One possible explanation could be that success in each domain of spatial ability (in general) involves a series of spatial processes and specific spatial skills (Newcombe et al., 2018). Moreover, various spatial skills are not independent but related, which may help an effective integration of information for faster decision making.
These results should be examined on a larger sample. The larger sample of children from Serbia, as well as the application of the factor analysis, will provide more convincing evidence for some further research and a model for the typology of children’s spatial skills. Some other directions for further research should also be pointed out, for example, on developing a more detailed model for the typology of children’s spatial skills that could explain the case in Serbia or similar cases in other countries. Moreover, as the multidimensional structure of spatial ability remains a debated issue, the developmental trajectories of spatial skills may give some possible new directions in the research of children’s spatial abilities (Hodgkiss et al., 2021).
Data availability statement
The original contributions presented in the study are included in the article/Supplementary material, further inquiries can be directed to the corresponding author/s.
Ethics statement
The studies involving human participants were carried out with the consent/approval of the participating schools. Written informed consent to participate in this study was provided by the participants’ legal guardian/next of kin.
Author contributions
OĐ and MV conceived and planned the interviews with preschool children, planned and carried out the simulations, contributed to sample preparation, contributed to the interpretation of the results, and provided critical feedback and helped shape the research, analysis, and manuscript. MV carried out the interviews with preschool children. OĐ took the lead in writing the manuscript. All authors contributed to the article and approved the submitted version.
Acknowledgments
The authors owe a great debt of gratitude to Milana Dabić Boričić for useful suggestions in the methodological part of the work when resolving some procedures and dilemmas.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Supplementary material
The Supplementary material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/feduc.2024.1223022/full#supplementary-material
References
Alkouri, Z. (2022). Developing spatial abilities in young children: implications for early childhood education. Cog. Educ. 9:1. doi: 10.1080/2331186X.2022.2083471
Bertrand, J. (2007). “Preschool programs: effective curriculum. Comments on Kagan and Kauerz and on Schweinhart,” in Encyclopedia on early childhood development. R. E. Tremblay, R. G. Barr, and R. V. Petersde (Montreal, Quebec: Center of Excellence for Early Childhood Development).
Beth, E. W., and Piaget, J. (1974). “General psychological problems of Logico-mathematical thought” in Mathematical epistemology and psychology (Dordrecht: Springer), 163–190.
Björklund, C., van den Heuvel-Panhuizen, M., and Kullberg, A. (2020). Research on early childhood mathematics teaching and learning. ZDM 52, 607–619. doi: 10.1007/s11858-020-01177-3
Bryant, P. (2008). “Paper 5: understanding spaces and its representation in mathematics” in Key understanding in mathematics learning: A report to the Nuffield Foundation. eds. T. Nunez, P. Bryant, and A. Watson (London: Nuffield Foundation).
Buđevac, N., and Kovačević, Z. (2020). “Sagledavanje deteta i procesa učenja u novim osnovama programa predškolskog vaspitanja i obrazovanja [“children and learning process from the perspective of the new preschool education curricular framework”]” in Curricular (re)forms in education – Challenges and perspectives. eds. Z. Opačić and G. Zeljić (Belgrade: Teacher Education Faculty), 391–406.
Chorlay, R., Clark, K. M., and Tzanakis, C. (2022). History of mathematics in mathematics education: recent developments in the field. ZDM 54, 1407–1420. doi: 10.1007/s11858-022-01442-7
Clements, D. H., and Sarama, J. (2007). Effects of a preschool mathematics curriculum: summative research on the building blocks project. J. Res. Math. Educ. 38:2. doi: 10.2307/30034954
Clements, D. H., and Sarama, J. (2011). Early childhood teacher education: the case of geometry. J. Math. Teach. Educ. 14, 133–148. doi: 10.1007/s10857-011-9173-0
Cortina, J. M. (1993). What is coefficient alpha? An examination of theory and applications. J. Appl. Psychol. 78:1.
Cui, X., and Guo, K. (2022). Supporting mathematics learning: a review of spatial abilities from research to practice. Curr. Opin. Behav. Sci. 46:101176. doi: 10.1016/j.cobeha.2022.101176
Cyprus Ministry of Education and Culture (2010). Program of studies in mathematics. Nicosia: Cyprus Ministry of Education and Culture.
B. Davis and Spatial Reasoning Group (2015). Spatial reasoning in the early years: Principles, assertions and speculations. UK: Routledge.
Đerić, I., Gutvajn, N., Jošić, S., and Ševa, N. (2021). Nacionalni izveštaj: TIMSS 2019 u Srbiji - pogled osnovnih nalaza [National Report: TIMSS 2019 in Serbia - An Overview of the Main Findings]. Beograd: Institut za pedagoška istraživanja.
Đokić, O. J. (2014). Realno okruženje u početnoj nastavi geometrije [realistic mathematics education in initial geometry teaching]. Teach Innov 27, 7–21. doi: 10.5937/inovacije1402007D
Đokić, O. (2018). Research perspectives of initial geometry education. J Pedag Res 2:2. doi: 10.13140/RG.2.2.12247.78249
Đokić, O., and Vorkapić, M. (2023). “The conceptual change approach in mathematics education: from topological primacy to projective geometry,” Proceedings of the 13th congress of the European Society for Research in mathematics education (CERME13). (Budapest, Hungary: ERME).
Frick, A., Hansen, M. A., and Newcombe, N. S. (2013). Development of mental rotation in 3- to 5-year-old children. Cogn. Dev. 28, 386–399. doi: 10.1016/j.cogdev.2013.06.002
Gilligan, K. A., Hodgkiss, A., Thomas, M. S. C., and Farran, E. K. (2019). The developmental relations between spatial cognition and mathematics in primary school children. Dev. Sci. 22:e12786. doi: 10.1111/desc.12786
Gilligan-Lee, K. A., Hawes, Z. C. K., and Mix, K. S. (2022). Spatial thinking as the missing piece in mathematics curricula. NPJ Sci. Learn. 7:10. doi: 10.1038/s41539-022-00128-9
Harris, D. (2021). “Spatial ability, skills, reasoning and thinking: what does it mean for mathematics?” In Excellence in Mathematics Education: Foundations & Pathways (Proceedings of the 43th Annual Conference of the Mathematics Education Research Group of Australasia). Eds. Y. H. Leong, B. Kaur, B. H. Choy, J. B. W. Yeo, and S. LN (Singapore: MERGA), 385–392.
Harris, J., Hirsh-Pasek, K., and Newcombe, N. S. (2013). Understanding spatial transformations: similarities and differences between mental rotation and mental folding. Cogn. Process. 14, 105–115. doi: 10.1007/s10339-013-0544-6
Hawes, Z., Moss, J., Caswell, B., Naqvi, S., and MacKinnon, S. (2017). Enhancing children’s spatial and numerical skills through a dynamic spatial approach to early geometry instruction: effects of a 32-week intervention. Cogn. Instr. 35, 236–264. doi: 10.1080/07370008.2017.1323902
Hawes, Z., Moss, J., Caswell, B., Seo, J., and Ansari, D. (2019). Relations between numerical, spatial, and executive function skills and mathematics achievement: a latent-variable approach. Cogn. Psychol. 109, 68–90. doi: 10.1016/j.cogpsych.2018.12.002
Hodgkiss, A., Gilligan, K. A., Tolmie, A. K., Thomas, M. S. C., and Farran, E. K. (2018). Spatial cognition and science achievement: the contribution of intrinsic and extrinsic spatial skills from 7 to 11 years. Br. J. Educ. Psychol. 88, 675–697. doi: 10.1111/bjep.12211
Hodgkiss, A., Gilligan, K. A., Tolmie, A. K., Thomas, M. S. C., and Farran, E. K. (2021). The developmental trajectories of spatial skills in middle childhood. Br. J. Dev. Psychol. 39, 566–583. doi: 10.1111/bjdp.12380
Hoekstra, R., Vugteveen, J., Warrens, M. J., and Kruyen, P. M. (2019). An empirical analysis of alleged misunderstandings of coefficient alpha. Int. J. Soc. Res. Methodol. 22, 351–364. doi: 10.1080/13645579.2018.1547523
Ishikawa, T., and Newcombe, N. S. (2021). Why spatial is special in education, learning, and everyday activities. Cog. Res. 6:20. doi: 10.1186/s41235-021-00274-5
Jahnke, H. N., Jankvist, U. T., and Kjeldsen, T. H. (2022). Three past mathematicians’ views on history in mathematics teaching and learning: Poincaré, Klein, and Freudenthal. ZDM 54, 1421–1433. doi: 10.1007/s11858-022-01376-0
Lubinski, D. (2010). Spatial ability and STEM: a sleeping giant for talent identification and development. Personal. Individ. Differ. 49, 344–351. doi: 10.1016/j.paid.2010.03.022
Mathematical Society of Serbia. (2023). Mathematical Society of Serbia Available at: https://dms.rs/ (Accessed May 15, 2023).
Milinković, J., and Vorkapić, M. (2021). Stavovi budućih vaspitača o integrativnom pristupu razvoju matematičkog mišljenja [pre-service preschool teachers’ attitudes towards integrative approach to the development of mathematical thinking]. Teach. Innov. 34, 124–134. doi: 10.5937/inovacije2103124M
ME (2006). Pravilnik o opštim osnovama predškolskog programa [rulebook on the general basics of the preschool curriculum]. Beograd: Službeni glasnik 14/2006.
ME (2018). Osnove programa predškolskog vaspitanja i obrazovanja [basics of preschool education and education curriculum]. Beograd: Ministarstvo prosvete, nauke i tehnološkog razvoja. Available at: https://prosveta.gov.rs/wp-content/uploads/2018/09/OSNOVE-PROGRAMA-.pdf (Accessed May 10, 2023).
Mix, K., and Cheng, Y. (2012). The relation between space and math. Developmental and educational implications. Adv. Child Dev. Behav. 42, 197–243. doi: 10.1016/B978-0-12-394388-0.00006-X
Mix, K. S., Hambrick, D. Z. V., Satyam, R., Burgoyne, A. P., and Levine, S. C. (2018). The latent structure of spatial skill: a test of the 2 × 2 typology. Cognition 180, 268–278. doi: 10.1016/j.cognition.2018.07.012
Mix, K. S., Levine, S. C., Cheng, Y. L., Young, C., Hambrick, D. Z., Ping, R., et al. (2016). Separate but correlated: the latent structure of space and mathematics across development. J. Exp. Psychol. Gen. 145, 1206–1227. doi: 10.1037/xge0000182
Newcombe, N. (1989). The development of spatial perspective taking. Adv. Child Dev. Behav. 22, 203–247. doi: 10.1016/S0065-2407(08)60415-2
Newcombe, N. (2010). Picture this: increasing math and science learning by improving spatial thinking. Am. Educ. 34:2.
Newcombe, N. (2018). “Three kinds of spatial cognition” in Stevens' handbook of experimental psychology and cognitive neuroscience. ed. J. Wixted. 4th ed (Hoboken, NJ: John Wiley & Sons Inc), 1–31.
Newcombe, N., and Huttenlocher, J. (1992). Children’s early ability to solve perspective-taking problems. Dev. Psychol. 28, 635–643. doi: 10.1037/0012-1649.28.4.635
Newcombe, N. S., Möhring, W., and Frick, A. (2018). “How big is many? Development of spatial and numerical magnitude understanding” in Heterogeneity of function in numerical cognition. eds. A. Henik and W. Fias (Cambridge: Elsevier Academic Press), 157–176.
Newcombe, N. S., and Shipley, T. F. (2015). “Thinking about spatial thinking: new typology, new assessments” in Studying visual and spatial reasoning for design creativity. ed. J. Gero (Dordrecht: Springer), 179–192.
Newcombe, N. S., Uttal, D. H., and Sauter, M. (2013). “Spatial development” in Oxford handbook of developmental psychology: Body and mind. ed. P. D. Zelazo (New York: Oxford University Press), 564–590.
Okamoto, Y., Kotsopoulos, D., McGarvey, L., and Hallowel, D. (2015). “The development of spatial reasoning in young children” in Spatial reasoning in the early years: Principles, assertions and speculations. eds. B. Davis and Spatial Reasoning Group (UK: Routledge), 15–28.
Olson, G. (1994). “Preparing early childhood educators for constructivist teaching” in New perspectives in early childhood teacher education: Bringing practitioners into the debate. eds. S. G. Goffin and D. Day (New York: Teachers College), 37–47.
Piaget, J., and Inhelder, B. (1956). The Child’s conception of space. UK:Routledge & Kegan Paul Ltd.
Poincaré, H. (1905). Science and hypothesis (with a preface by J. Larmor). NewYork: The Science Press.
Pollitt, R., Cohrssen, C., and Seah, W. T. (2020). Assessing spatial reasoning during play: educator observations, assessment and curriculum planning. Math. Educ. Res. J. 32, 331–363. doi: 10.1007/s13394-020-00337-8
Raven, J. J. (2003). “Raven progressive matrices” in Handbook of nonverbal assessment. ed. R. S. McCallum (Boston: Springer), 223–237.
Sinclair, N., and Bruce, C. D. (2014). “Spatial reasoning for young learners,” Proceedings of the Joint Meeting of PME 38 and PME-NA 36. Eds. P. Liljedahl, C. Nicol, S. Oesterle, and D. Allan (Vancouver, Canada: PME), 173–203.
Stamatović, J. (2021). Osnove programa predškolskog vaspitanja i obrazovanja - mogućnosti i izazovi [“preschool education program - opportunities and challenges”] in Zbornik radova sa 16. Konferencije Vaspitač u 21. Veku “Izazovi primene osnova programa predškolskog vaspitanja i obrazovanja” [book of proceedings from the 16th conference twenty-first century preschool teacher “the importance of preschool teachers’ competence for the quality of education of preschool age children”]. Ed. P. M. Jašović (Aleksinac: Akademija vaspitačko-medicinskih strukovnih studija), 17–22.
Stojanović, A., and Bogavac, D. (2016). Metodičke strategije razvoja dečjih potencijala u savremenoj predškolskoj ustanovi [Methodological Strategies for the Development of Children’s Potential in a Modern Preschool Institution]. Beograd: Učiteljski fakultet.
Stojanović, A., Kovačević, Z., and Bogavac, D. (2018). From obsolete to contemporary preschool education (experiences in Serbia). Early Years 38, 363–377. doi: 10.1080/09575146.2018.1444584
Tam, Y. P., and Chan, W. W. L. (2022). The differential relations between sub-domains of spatial abilities and mathematical performance in children. Contemp. Educ. Psychol. 71, 102101–102111. doi: 10.1016/j.cedpsych.2022.102101
Thom, J. S., McGarvey, L. M., and Lineham, N. D. (2021). “Perspective taking: spatial reasoning and projective geometry in the early years,” In Excellence in Mathematics Education: Foundations & Pathways (Proceedings of the 43th Annual Conference of the Mathematics Education Research Group of Australasia). Eds. Y. H. Leong, B. Kaur, B. H. Choy J. B. W. Yeo, and S. L. Chin. (Singapore: MERGA). 219–226.
Uttal, D. H., and Cohen, C. A. (2012). “Spatial thinking and STEM education: when, why, and how?” in The psychology of learning and motivation. ed. B. H. Ross (Cambridge: Elsevier Academic Press), 147–181.
Uttal, D. H., Meadow, N. G., Tipton, E., Hand, L. L., Alden, A. R., Warren, C., et al. (2013). The malleability of spatial skills: a meta-analysis of training studies. Psychol. Bull. 139, 352–402. doi: 10.1037/a0028446
Van den Heuvel-Panhuizen, M., and Buys, K. (2008). Young children learn measurement and geometry. Rotterdam: Sense.
Vorkapić, M. M., Milošević, M. S., and Đokić, O. J. (2022). Young pupils’ ability to solve perspective-taking problems. Uzdanica XIX, 71–94. doi: 10.46793/Uzdanica19.S.071V
Wai, J., Lubinski, D., and Benbow, C. P. (2009). Spatial ability for STEM domains: aligning over 50 years of cumulative psychological knowledge solidifies its importance. J. Educ. Psychol. 101, 817–835. doi: 10.1037/a0016127
Xie, F., Zhang, L., Chen, X., and Xin, Z. (2020). Is spatial ability related to mathematical ability: a Meta-analysis. Educ. Psychol. Rev. 32, 113–155. doi: 10.1007/s10648-019-09496-y
Yang, J., Wu, D., Luo, J., Xie, S., Chang, C., and Li, H. (2020). Neural correlates of mental rotation in preschoolers with high or low working memory capacity: an fNIRS study. Front. Psychol. 11:568382. doi: 10.3389/fpsyg.2020.568382
Keywords: spatial ability, spatial skills, preschool children, curriculum, model for the typology of children’s spatial skills, STEM, Serbia
Citation: Đokić OJ and Vorkapić MM (2024) Spatial skills of preschool children from Serbia and a possible model for the typology of children’s spatial skills. Front. Educ. 9:1223022. doi: 10.3389/feduc.2024.1223022
Edited by:
Subramaniam Ramanathan, Nanyang Technological University, SingaporeReviewed by:
Gavin Duffy, Technological University Dublin, IrelandJietong Luo, East China Normal University, China
Copyright © 2024 Đokić and Vorkapić. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Olivera J. Đokić, b2xpdmVyYS5kam9raWNAdWYuYmcuYWMucnM=