Daniel Chazan1*
Patricio Herbst2- 1Department of Teaching and Learning, Policy and Leadership, Center for Mathematics Education, College of Education, University of Maryland, College Park, MD, United States
- 2GRIP Lab, School of Education, University of Michigan, Ann Arbor, MI, United States
This article considers what teacher education candidates can show their instructors as evidence that they are learning what they are supposed to learn, and how that might be described as like what happens in mathematics classrooms in compulsory settings. To support this effort, the article extends the use of the construct of instructional exchanges from the analysis of mathematics instruction to the analysis of mathematics teacher education. We describe four ways in which teacher candidates exchange their work for teacher educators' assessments of their learning of mathematics teaching and illustrate how such exchanges are a subset of a more general kind of activity, a transaction of practice. Section 4 looks at ways in which the theorizing in the study might have practical implications. The examination of teacher education closes by looking back at what the attention to the transaction of practice might suggest as ways to develop a greater range of tasks for use in mathematics teaching in compulsory settings; the use of a wider range of tasks might allow mathematics teachers in compulsory settings to make a wider variety of claims about what it is that their students have learned. The article also highlights ways in which technological tools can be considered as an important infrastructure to develop to support instructional exchanges in mathematics teacher education.
1. Introduction
Throughout their careers, many mathematics educators have had opportunities to teach mathematics in formal institutions of schooling when working as a schoolteacher. Many have also had opportunities to teach prospective teachers when working as mathematics teacher educators. On rare occasions, some of us have served in both of these capacities in the same period. Regardless of the timing, the career pathway from teaching to teacher education is common enough that experience as teacher is often conceptualized as an extremely useful resource for mathematics teacher educators working in universities (Reys and Dossey, 2008).
In this section, we begin this article by informally calling on the first author's conversations with two instructors, with much experience teaching mathematics at both school and university, about their first forays into teacher education. We use the descriptions of these experiences to generate a rough description of some differences in the dynamics present in these two teaching contexts, against the backdrop of similarities in instruction. We then use those differences to justify theoretical work that aims to give descriptive purchase on differences between teaching mathematics and teaching mathematics teaching. Ultimately, the article aims to consider tasks posed by teacher educators that ask teacher candidates to do work that can function as evidence that teacher candidates are learning what they are supposed to learn about teaching, and how that might be described as similar to and different from what happens in classrooms in compulsory settings where mathematics is the knowledge at stake.
1.1. How do teaching mathematics and teaching mathematics teaching feel similar and different?
In talking to colleagues familiar with teaching mathematics but new to teacher education, the first author has noticed that grading often comes up as a topic of conversation. In both kinds of instruction, the goals of grading student work included both feedback to students and distinguishing student performances one from another in some way that is deemed fair. Yet, having experience with the teaching of mathematics did not seem to immediately provide the first author's colleagues with resources for their work as teacher educators. As one colleague defines it, when teaching mathematics, they could write a test, give it to students, assign point totals to responses comparatively easily, add up the points to assign grades, and then use the resulting grades to indicate to students how their performances differed; the work proceeded relatively efficiently and students typically seemed satisfied with the process; though, on occasion, there might be a question about a particular element of the scoring.
By contrast, as new teacher educators, the process of grading student work was, for the first author's two colleagues, far more time-consuming and often seemed fraught with potential for conflict. The instructions for the assignments in the teacher education context did not seem as self-explanatory as in the mathematics classroom and often required much clarification and negotiation with the students (indeed such negotiation was often a site for substantial learning). Scoring responses did not seem straightforward, often felt difficult to warrant, and took much thought. These instructors often wrestled with justifying ranking different responses. Once grades were shared, students seemed to have many questions about how the grading was done, and whether it was fair or not.
Perhaps some part of an explanation for these experiences of novice teacher educators might lie in public perceptions of knowledge of mathematics and mathematics teaching. Public perceptions of mathematics include a description of mathematics as a hard science where the answers to questions are correct or incorrect. Indeed, the correct answers to mathematical questions are so certain that mathematics is sometimes suggested as a way to communicate with sentient beings elsewhere in the universe (see, e.g., Lakoff and Núñez, 2000, for what they call “The Romance of Mathematics”). Knowledge of mathematics teaching, by contrast, is not typically deemed a hard science, and perhaps not even a science at all at this stage. Instead, teacher education is a context in which hard truths are thought to be less prevalent and where individuals might have a good reason for holding different opinions based on different experiences, differing commitments, different contexts, or even simply personal style (Fenstermacher, 2007).
Like many mathematics education researchers, we consider this descriptive contrast between mathematical knowledge and knowledge of teaching that we have just offered insufficiently nuanced and a misrepresentation both of mathematical knowledge and of knowledge about mathematics teaching. However, we value consideration of the question of how teacher educators create contexts where teacher candidates can show what they are learning from their instructors. In this study, we consider what teacher education candidates can show their instructors as evidence that they are learning what they are supposed to learn, and how that might be described as similar to and different from what happens in mathematics classrooms in compulsory settings. This theoretical article extends the construct of instructional exchanges from the analysis of the work of mathematics teaching (Herbst and Chazan, 2012) to mathematics teacher education. Our aim was to understand better the differences between teaching mathematics to students and teaching mathematics teaching to teacher candidates by extending the use of theorizing about one aspect of mathematics instruction to the instruction about mathematics teacher education (see Figure 1).
Figure 1. Relating teaching mathematics and teaching to teach mathematics.
We seek purchase on the exchange between teacher candidates' (as students) work and teacher educators' (as instructors) judgments of what their candidates have learned about teaching. In Section 3, we begin with instructional exchanges that seem easily analogous to instructional exchanges in mathematics instruction, exchanges where teacher educators provide teacher candidates with tasks that allow teacher candidates to demonstrate that they have learned to carry out certain actions (teacher moves) expected of teachers. We then move on to tasks where teacher candidates are asked to identify such actions in teaching that either they observed or they have enacted. Such exchanges allow the teacher educator to claim that teacher candidates have learned to notice aspects of teaching (Chieu et al., 2015; Ghousseini and Herbst, 2016) within the enormous number of stimuli that are present in classroom life (Jackson, 1968/1990). Finally, we move to instructional exchanges where teacher educators expect teacher candidates to reflect on their own instruction and identify room for growth and development. Teacher candidate reflections allow teacher educators to argue that their candidates are becoming reflective practitioners who will continue to learn and to grow and change by reflecting on their experiences as teachers.
In Section 4, we consider ways in which the use of instructional exchanges analytically in the contexts of mathematics teaching and mathematics teacher education generates insights into each context. Stimulated in part by our understanding of the role of student work formats central to instructional exchanges in mathematics classroom instruction (e.g., two-column proofs Herbst, 2002), in the teacher education context, we consider how students and teachers transact practice through representations of teaching (Herbst and Chazan, 2023). In the context of teacher education and exchanges between teacher candidates or practicing teachers and teacher educators or professional development providers, this focus on formats of student work leads us to argue for the importance of developing infrastructure to support the transaction of instructional practice using the variety of representations of practice now available.
Similarly, we look back at what the extension of instructional exchanges to the analysis of teacher education might suggest as ways to develop a greater range of tasks for use in mathematics teaching in compulsory settings. We suggest that a wider range of tasks in the mathematics classroom, beyond having students work on mathematics problems, could allow mathematics teachers in compulsory settings to make a wider variety of claims about what it is that their students have learned.
2. Instruction in mathematics teaching from the perspective of practical rationality
Since 2003, under the label of Practical Rationality (Herbst and Chazan, 2003, 2012, 2020; Chazan et al., 2016), we have been engaged in a process of building a middle-range theory of mathematics teaching as a practice (Herbst and Chazan, 2023). Our theory is meant to provide a language for the description of teaching that captures the work of the teacher, as well as teachers' perspectives on this work, from an observer's perspective. In the development of this theory, we have used as resources accounts of dynamics of teaching that are familiar or credible to mathematics teachers and patterns in mathematics teachers' responses to scenario-based surveys.
This theory views teaching as accountable to the interests and agendas of a variety of stakeholders (students and parents, administrators, the disciplines that are the source of the content taught, the societies in which schooling exist, and the institutions in which mathematics instruction is deployed) and sees the norms that describe the kind of teaching done in school as representing a point of equilibrium in the complex space created by the competing agendas of stakeholders (see Figure 2).
Figure 2. Instruction has obligations to stakeholders of schooling.
Within this complex space, a notion central to Practical Rationality is that a key aspect of the work of the teacher in instruction is that of managing instructional exchanges. Instructional exchanges are processes particularly easy to notice when considering formative and summative assessment, though these processes are not limited only to assessment. These processes are present regardless of whether a teacher is interacting with an individual student, a small group, or the whole class and regardless of whether the judgments they make are about the progress of an individual student, a group, or the whole class.
Instructional exchanges involving judgments about the progress of the whole class are particularly useful for understanding formative assessment in the context of teacher decision-making about when and why to deviate from plans. The notion of an instructional exchange captures both how teachers make the accomplishment of instructional goals possible through posing mathematics problems to students and how examination of students' work on these problems is a performance that can indicate whether and how a goal has been met or is on its way to being met. In other words, teachers operationalize the content they are to teach in tasks through which students can show that they have learned the content teachers are supposed to teach; and students work on tasks and submit the results of their work to their teacher who then has ways of conveying the value that they see in this work (Doyle, 1988). In instruction, for example when teachers are monitoring students' actual responses to tasks (Stein et al., 2008), teachers monitor what the class has done and manage the exchange of the results of student work for their judgments that the class has learned, or is learning, the mathematics at stake, or not. The results of these teacher judgments are then used to make instructional decisions; if the class is not learning the mathematics it is supposed to learn, then reteaching or additional tasks may be called for (see Figure 3 above).
Figure 3. Teachers manage instructional exchanges related to mathematical knowledge.
We offer three observations about the instructional exchange construct. First, instructional exchanges do not describe exchanges of things of the same kind (as in a currency exchange where one currency is changed for another); students cannot offer the teacher the knowledge the teacher seeks to teach students; instructional exchanges always deal in exchanges of things of different kinds (like the purchasing of food with currency, or the barter of food for services).
Relatedly, these exchanges are meant to describe the nature of interaction in terms of what is being exchanged, not just a moment of physical exchange or the way an exchange takes place. Instructional exchanges are interpretive processes the teacher does based on curricular, behavioral, cognitive, and discursive evidence; as such they may be elicited from classroom interaction but are not reducible to moment-to-moment analysis of classroom interaction. Thus, using this construct to describe teaching is a more subtle matter of interpretation than describing transactions one might observe in a marketplace; particularly, we are interested in considerations that engage a teacher as they teach that may not be directly accessible to an observer, but whose impact may be seen in instructional decisions. For example, as a teacher monitors groups of students doing mathematical work, it may not be obvious to the observer exactly what aspects of students' work are being monitored which lead to an instructional decision then taken by the teacher.
In other circumstances, however, instructional exchanges may be easier to observe because they have been standardized in some way. There may be aspects of instructional interactions that signal the transactional dimension of instruction and communicate that certain behaviors and actions are expected in what we call instructional situations (For example, we have explored doing proofs, Herbst et al., 2009, and solving equations, Chazan and Lueke, 2009). For example, the canonical method for solving equations' format (Buchbinder et al., 2015) and the two-column proof format (Herbst, 2002) are formats for student work that help teachers manage instructional exchanges by standardizing student work and making it less time-consuming for teachers to judge that students' work can be taken as evidence that students have learned, or are learning, the mathematics at stake. Such student work formats are one piece of evidence for the existence of an instruction situation with normative expectations and can help make instructional exchanges evident to observers. Other evidence for the construct of instructional situation, and for classroom norms related to the particular mathematics at stake, can be found in our empirical examinations of teachers' expectations about the nature of classroom activity in the context of the teaching of the solving of equations and the doing of proofs (e.g., Herbst et al., 2009, 2010; Buchbinder et al., 2019).
Our second observation about instructional exchanges is that managing these exchanges is part of what teachers are expected to do. Within the context of schooling, teachers must be ready to justify both in what ways the tasks they have chosen to give students represent the mathematics at stake and in what ways student's work should count as evidence that students have learned this mathematics (Herbst and Chazan, 2011, 2012). Principals, lead teachers, parents, and students may all have reasons to ask for such justifications. In addition, in reflecting on their own work, some of that need for justification may be internal. For example, teachers may feel that students have not produced work that exchanges for the claim of having learned the mathematics at stake, because the tasks offered by the teacher do not provide students with sufficient opportunities to learn, and to show they have learned, that content.
The construct of instructional exchanges is helpful to us as researchers of mathematics instruction for two main reasons. First, this construct captures that teachers are responsible to teach students particular mathematics and have institutional obligations to do so as a part of their role as teachers. The construct assumes the institutional embeddedness of instruction; teachers and students have obligations to the institution that shapes the work that students can do and how teachers can call for that work and interpret its meanings. The notion of instructional exchanges captures that teachers as employees of educational institutions must pursue instructional goals through which they are accountable to stakeholders and why teachers feel obligations to achieve those goals (e.g., what teachers often call “covering the curriculum” and Lampert, 2001, reifies as an important component of the work of teaching).
Our third observation about instructional exchanges is that this construct allows analysts of teaching to focus on patterned ways in which instructional exchanges go forward. For instance, instructional exchanges help capture ways in which the content of instruction is related to ways in which teaching differs across the content of school mathematics (e.g., how high school geometry and algebra instruction differ). For example, the two-column proof student work format supports exchanges between students' proving particular mathematical assertions and the claim that they are learning how to do proofs (Herbst, 2002). Similarly, students' work showing their use of the canonical solution method for linear equations supports exchanges for the claim that students are learning to solve equations (Buchbinder et al., 2019). These formats help students know what they must do and ease the instructor's burden when assessing what students have done. Much like the use of standardized coins, rather than barter, these formats grease the wheels of instructional exchanges. The ways in which these work formats differ for the different mathematical content being taught in Euclidean geometry and introductory algebra illustrate that instructional exchanges are a mechanism that allows researchers to describe how teaching is responsive to the particular content being taught.
3. Theorizing instructional exchanges in the context of mathematics teacher education
Teacher education takes place in different institutional contexts than compulsory schooling. There can be a range of settings where this work takes place (e.g., in the US, a common distinction is between university-based and alternative certification settings). Typically, teacher candidates (also known as prospective or preservice teachers), unlike students of mathematics in compulsory settings, choose to follow teacher education as a course of studies (in some countries, teacher candidates pay for their professional education) and are free to leave programs whenever they like, and teacher education has different stakeholders. For example, in the context of university-based teacher education, there are norms related to academic freedom of concern to particular stakeholders (e.g., members of the faculty) that are often in tension with expectations of attention to program accreditation and certification of graduates of deep concern to other stakeholders (e.g., accreditation agencies).
While we are conscious of these differences between mathematics teaching and mathematics teacher education, and of the differences alluded to in the introduction between the societal status of the knowledge that is the source of the curriculum of compulsory schooling and teacher education, in this section, we seek to identify some similarities. One aspect of the similarity we will build on is the presence of didactical contracts (Brousseau, 1997) in both contexts. In both contexts, some instructors seek to teach their clients some expected knowledge and skills. In both contexts, at least in principle, students are supposed to learn what it is that their instructors teach. Building on the presence of didactical contracts in these two contexts, we extend the use of the construct of instructional exchanges from mathematics instruction to the context of mathematics teacher education.
We start with academic tasks in teacher education that might be considered analogous to the mathematics problems (whether they be familiar tasks, e.g., Doyle, 1988, or novel tasks, e.g., Herbst, 2003) that are so ubiquitous in mathematics classrooms. In the same way that mathematics problems ask students to do mathematics, we begin with academic tasks that ask teacher candidates to teach and consider examples where teaching done by candidates trades as having achieved some particular curricular goal for teacher educators (e.g., competence in doing some aspect of the work of teaching).
An activity of long-standing in teacher education involves asking teacher candidates to engage in instruction, albeit often in contexts at a distance from settings of compulsory schooling. In what has been called microteaching (McKnight, 1971), teacher candidates are asked to instruct their peers in the university classroom; a related practice, in which teacher candidates enact student-centered practices that have been documented under the name of rehearsals (Lampert et al., 2013) or enactments; various forms of simulations exist, including having teacher candidates interact with avatars that represent students, or some other digital representation of students, and teach a lesson (Amador et al., 2021); or teacher candidates may actually instruct children in schools in the context of a methods course that takes place in a school (Kazemi and Wæge, 2015), an early field experience (Hughes et al., 2020), or in the context of student teaching, an internship, or a residency.
These various contexts are created to help teacher candidates have an opportunity to practice some components of the work of instruction that their instructors seek to have them learn. Let us consider, for a moment, the work of one individual teacher candidate. The work the teacher candidate does is an actual instruction on a specific topic to a specific group in a specific context. This work is then offered in exchange for a claim that the teacher candidate is learning some aspect of teaching that has generality beyond the particulars of that content and that context. When the teacher educator can see the instruction take place, the work the student is exchanging is the instruction itself; when the teacher educator cannot experience the instruction in person, the teacher candidate may need to submit their work in some proxy format, for example, with an annotated video record of their instruction, but, regardless, the candidate is offering the instruction as their work and the teacher educator seeks evidence in the instruction, or its representation, that the teacher candidate has learned to carry out the aspects of the work of teaching that are at stake, typically some sort of teacher moves [e.g., the discourse moves described in Herbel-Eisenmann et al. (2013)] or practices.
In teacher education, teacher candidates are also given opportunities to engage in preparation for instruction, rather than simply instruction itself. For example, many methods courses provide the opportunity for teacher candidates to practice the work of planning for instruction. Whether or not teacher candidates are going to engage in instruction on a particular topic, teacher candidates may be asked by teacher educators to plan a lesson and to prepare a lesson plan to capture their plans. Such lesson plans are very much like two-column proofs in that they are an artificial work format created for instruction, not work formats that exist in this way either in the practice of doing mathematics or the practice of teaching mathematics. The lesson plans that a teacher candidate offers their instructor are meant to exchange as evidence that teacher candidates are learning, or have learned, to plan for instruction in particular desirable ways, for example, considering the types of responses students of mathematics in compulsory settings may give to a particular question or task. The assumption is that if teacher candidates have learned to plan for instruction, then when they work as teachers, they will plan in ways that will allow their instruction to be supportive of their student's learning.
Mathematics instruction is routinely organized around mathematical problems that ask students to engage in mathematical activity, even if it is reported in a work format designed for instruction in mathematical practice (say doing proofs as a part of the school subject of mathematics) that is at odds with how that practice is carried out in the discipline of mathematics (Popkewitz, 2004). By contrast, besides instruction and planning for instruction, other varieties of teachers' work come to the fore in teacher education. These are varieties of work that are not directly dictated by occupying the instructional role of teacher, but instead, ancillary practices required by teachers' institutional position within schools and that indirectly support teachers' instructional roles. Much in the way that writing formal lesson plans is not a common practice of practicing teachers, there are other practices requested of teacher candidates by teacher educators that focus on learning habits that are intended to make teachers better instructors, even if those habits are less directly specified by the nature of instruction itself.
For example, increasingly over the last two decades, researchers on teacher learning have focused on what it is that teachers notice when they watch teaching, either live or as recorded on videotape (Sherin and Han, 2004). More recently, this research has sought to find ways to increase teachers' attention to the mathematics thinking of their students (van Es and Sherin, 2008). Put more generally, building on Goodwin's (1994) construct of Professional Vision, researchers on teacher learning have come to articulate the importance of noticing aspects of teaching within classroom life, not just student thinking (Walkoe and Luna, 2020). As this noticing work comes to influence the curriculum of teacher education, in the words of Grossman et al. (2009), teacher educators aim to teach teacher candidates a particular way of decomposing practice as one way to understand key constituent parts of the work. This development is reflected in the increasing prevalence of instructional exchanges in teacher education where the work that teacher candidates do is to comment on or annotate representations of practice in some way: teacher candidates can be asked to say what they see in a classroom video of their own, or someone else's, teaching. Teacher candidate comments or annotations then can be taken as evidence that they are learning, or are not learning, to view teaching in the ways that their instructors seek to teach them to view it. The hope is that being able to decompose teaching in this particular way will help teacher candidates as practitioners then act in particular ways in classrooms, for example, by paying greater attention to students' algebraic thinking (Walkoe and Levin, 2018).
In the context of teacher education, there is another kind of student work that has been central to descriptions of aims of teacher education: to produce reflective practitioners, teacher candidates are asked to reflect on practice (Falk, 2006). Once again, with this kind of work, students are asked to consider practice; in this case, teacher candidates are often asked to consider their own practice and to reflect on how particular actions in particular contexts do or do not represent the particular commitments that guide their image of how they seek to teach. However, what is at stake here for the teacher educator in this sort of interaction is not evidence that teacher candidates have learned to decompose practice in a particular way, or to carry out specific instructional moves, but rather that teacher candidates are able to stand back from the particulars of instruction to identify ways in which their instruction in an examined instance does or does not represent teaching that the candidate seeks to do. Students whose reflections do not address aspects of an interaction that a teacher educator sees as in conflict with the commitments articulated by a teacher candidate would therefore have a lower trade value than reflections that address those aspects of an interaction, even should they lead to a teacher candidate's harsh self-assessment. A harsh self-assessment, after all, maybe an accurate judgment of the instruction a candidate has carried out and will thus trade as a good example of the capacity to engage in reflection.
In sum, what we have begun to sketch in this section are instructional exchanges that can be found in the context of teacher preparation (see Figure 4) and have identified four types of instructional exchanges.
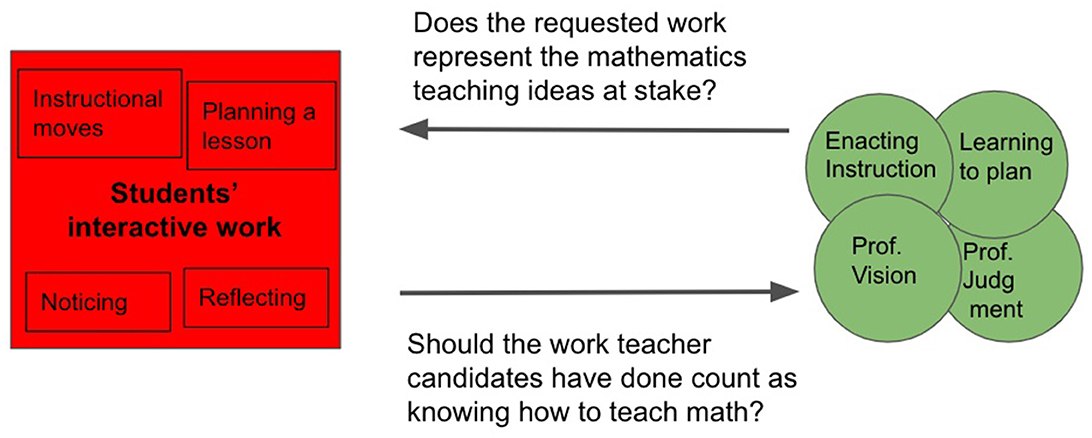
Figure 4. Teacher educators manage instructional exchanges related to mathematics teaching that involve different kinds of student work.
Each of these kinds of instructional exchanges can be instantiated by a range of exchanges that are specific to the particular instructional goals of a teacher educator. Thus, exchanges focused on the enactment of teaching can be focused on a range of practices of teaching: e.g., giving instructional explanations, responding to student ideas, launching a task, and supporting students' sense of competence. Similarly, instructional exchanges related to planning can focus on the choice of tasks, planning for interaction with students' ideas, and more. Instructional exchanges focused on enlarging teacher candidates' professional vision may direct teacher candidates' attention to a student's thinking of particular kinds, on patterns of who it is who speaks in mathematics classrooms, on racial inequities in classroom interaction, and more. Finally, teacher candidates can be asked to reflect on, and critique, a variety of aspects of their teaching.
4. Using an instructional exchange lens to cross-fertilize understandings of mathematics teaching and mathematics teacher education
Section 2 of this article laid out prior work using the construct of instructional exchanges as part of a descriptive theory of mathematics teaching. Building on this earlier work, Section 3 worked on extending the use of instructional exchanges to mathematics teacher education and articulated instructional exchanges built around four initial sorts of teacher candidate work. Further efforts may identify other such instructional exchanges in teacher education.
Section 4 moves away from this theoretical focus and explores the practical ramifications of the extension of the construct of instructional exchanges to mathematics teacher education. In this section, we consider mathematics teaching and mathematics teacher education both as instances of teaching in institutional contexts. We use contrasts between mathematics teaching and mathematics teacher education brought to the surface by the extension of instructional exchanges to mathematics teacher education to shed further light on each instructional context. While many have considered what the practice of mathematics teaching can suggest for the practice of mathematics teacher education, we begin with the practice of mathematics teacher education that might suggest for the practice of mathematics teaching.
4.1. From mathematics teacher education to mathematics teaching: revisiting instructional exchanges in mathematics teaching
Extension of the use of instructional exchanges from mathematics teaching to mathematics teacher education suggests that, in the context of teacher education, there is a widespread use of exchanges that involve different kinds of instructional goals on the part of the teacher educator and different kinds of student interactive work being exchanged for those goals. In some cases, like learning to enact instruction by practicing teacher moves or learning to plan instruction by writing lesson plans, the work that is exchanged between teacher candidates and teacher educators is connected directly to accomplishing instruction. However, in other cases, like achieving facility with a particular decomposition of practice or learning to reflect on one's own teaching, what it is that teacher candidates learn in teacher education does not teach them to do instruction directly, but instead involves work that seeks to improve their capacity to notice and reflect on aspects of teaching practice. By contrast, mathematics problems seem central to work that mathematics students offer most typically in the context of the instructional exchanges that take place in schools (even with novel tasks; Herbst, 2003).
Having noticed this difference between mathematics teacher education and mathematics teaching, we highlight how this contrast raises useful questions. For example, are there, perhaps less common, instructional exchanges in which student work does not consist of solving mathematics problems? One of those can be located in what Herbst (2010) called installing a definition or installing a theorem (see also Herbst et al., 2011): when the teacher formally enables a new item of knowledge as part of what the class can use, students are expected to do some work, albeit not work that involves solving problems. This work might be described behaviorally as paying attention, but we know that the work might be described mathematically as well, even if aspirationally. For example, the statement of a theorem could be engaged by translating it mentally into what it says about an instance; the statement of a definition could be engaged by thinking of examples and non-examples. The installation of theorems and definitions could demand from students at least a mental production of questions, and if the questions were uttered, they could indicate to the teacher whether the students are on their way to understanding the item of knowledge being installed.
Beyond this particular kind of exchange, the question of exchanges, when students are not doing problems, might also draw our attention to academic tasks in which students assess the work of other students, either grading the work as correct or incorrect or indicating whether they agree or disagree with assertions attributed to another student. With such tasks, it can be a difficult call to determine what precisely is different in the exchange: has the content at stake changed, or is the same mathematical knowledge still at stake and there is simply a different kind of interactive student work being requested? How about if the assessments are self-assessments of a student's own work? Do such academic tasks shift the knowledge at stake from the mathematics itself to a student's learning to assess their own understanding of the mathematics at stake? Is this sort of capacity to assess their own understanding perhaps analogous to a teacher candidate's capacity to reflect on instruction?
Moving to another kind of academic task that also feels different from the typical mathematics problem: how about academic tasks, alluded to above, that ask students to submit examples (e.g., of the equations of lines that are tangent to the graph of a given function) and defend that what they have submitted is an appropriate example [Yerushalmy and Olsher (2020) call these example eliciting tasks]? With such tasks, has the instructional exchange remained anchored in understandings of tangency or has the mathematics at stake become instead something else about coordinating examples and non-examples? If the instructional exchange remains about tangency, is there nonetheless a difference in the mathematics at stake in exchanges around such tasks and exercises involving the use of a computational method initially demonstrated by a teacher for identifying the equations of such lines? These questions seem like useful ones to push the further development of the construction of instructional exchanges in mathematics instruction.
Beyond tasks that are already present within mathematics classrooms, even if infrequently, could there be tasks designed to support instructional exchanges that are closer analogs to those sketched for mathematics teacher education and that would be new to mathematics teaching? For example, could students of mathematics in compulsory settings be presented with animations or storyboards that depict interactive mathematical work in classrooms with the request that mathematics students learn to decompose such classroom mathematical practice (e.g., using the Common Core's Standards for Mathematical Practice) and provide evidence of such learning by annotating these animations or storyboards? Or could tasks be developed for students of mathematics in compulsory settings that would engage them in creating alternatives to an existing storyboard that would demonstrate particular mathematical practices? Thinking about the instructional goals of mathematics teaching: Could such tasks help students of mathematics develop and strengthen their capacity to engage in the sorts of mathematical practices outlined in policy documents such as the US Common Core Standards for Mathematical Practice? With such academic tasks, would such work trade as learning mathematics, or not?
These questions suggest that the constructs in the descriptive theory of teaching that we are exploring can be a tool for considering new kinds of development for mathematics teaching in compulsory settings. In the next section, we outline ways in which consideration of the extension of this descriptive theory to instruction on mathematics teaching sheds new light on teacher education.
4.2. From mathematics teaching to mathematics teacher education: considering infrastructure to support instructional exchanges in teacher education
Problems in Teaching Secondary-School Mathematics (Breslich, 1931) is an artifact from a time when compulsory schooling at the elementary school level was already widely established in the United States, there were increasing enrollments at the secondary school level, and the need to educate high school mathematics teachers was also understood as a corollary of a broader education for members of the society. The book is the second volume of two focusing on the teaching of mathematics in Grades 7–12 in the United States. Both books are based on the work of the author at the Laboratory Schools of the University of Chicago; while the first volume examined general issues, the second volume discusses issues that arise in the teaching of specific topics and principles.
In the second volume, the author reports on a variety of student work formats that were under development at the time, for example, the development of systematic arrangements of the facts in word problems (p. 195–199), such as motion problems (see Figure 5).
Figure 5. A format for student work on word problems.
Although student work formats can be critiqued for many reasons, and though this sort of arrangement of the information provided in a word problem seems to have fallen out of favor (see Chazan et al., 2012), we find such work formats an illuminating artifact for understanding teaching. From our theoretical orientation, we consider student work formats as infrastructure that supports the work of teachers in managing particular instructional exchanges. Student work formats are intended both to support students in doing their work (in this case, to write equations to show how students use algebra to solve a word problem) and articulating it in a way that makes it easy for the teacher to monitor students' work and consider students' progress (in this case, toward learning to use algebra to solve word problems). We view the active engagement of mathematics teacher educators, like Breslich, in the production of student work formats as a contribution to the development of supports for instruction by providing teachers with tools to help them manage instructional exchanges.
Mathematics teacher education is an even newer societal phenomenon than mathematics teaching in compulsory schooling. Over slightly more than the last 100 years, teacher education has grown, and mathematics teaching itself has become the content of formal instruction within teacher education. Reflecting on the potential role of student work formats in helping teachers manage instructional exchanges in the mathematics classroom, in this section, we seek to use our understandings of the role of student work formats in mathematics instruction to raise questions about analogous infrastructure for instruction in mathematics teacher education. Specifically, we ask here the following: what kinds of resources might be useful infrastructure that could support teacher educators in managing the instructional exchanges that have developed in teacher education? We bring to this question experience designing technology to support teacher educators with the use of rich media in teacher education.
Considering where to start, in Figure 4 in Section 3, we outlined four different kinds of instructional exchanges in which teacher candidates offer their work to teacher educators. One of the four involves planning. Teacher educators create circumstances where teacher educators offer teacher candidates contexts in which teacher candidates can do work that will demonstrate that they have learned to plan for instruction. Any of the many forms used for creating lesson plans is an example of a work format in some ways analogous to student work formats in mathematics teaching. However, other than the forms for lesson planning, we are less aware of commonly used standardized student work formats in the context of teacher education.
Therefore, instead of maintaining a focus on student work formats, we now consider other instructional artifacts that support instructional exchanges by providing ways for learners to create work. Considering the kinds of technology that have been developed for mathematics instruction, like graphing calculators and interactive whiteboards, we describe technologies that can be considered as providing elements of infrastructure for teacher education by supporting teacher candidates in creating work to share with their instructors. Given the increasing centrality of representations of practice, like video records (Brophy, 2004), in teacher education, we focus on infrastructure for supporting interaction between teacher educators and teacher candidates around representations of practice. In this section, we describe technological tools that form the infrastructure for exchanging meanings around representations of practice and for authoring multimedia representations of teaching and learning that show, rather than tell (e.g., as written cases do), what happens in classrooms. For each of these elements of infrastructure, we will illustrate how they can support the sorts of teacher education instructional exchanges we outlined in Section 3.
We begin with Anotemos (www.anotemos.org; Herbst et al., 2019), a collaborative software application for annotating representations of classroom interaction, such as video clips, that supports exchanges of meaning around such representations. With this software application, client users can put pins at various moments in a timeline that represents the video clip and also select intervals on the timeline. In a collaborative commentary, each participant has their own timeline, and participants can see all the timelines and their markings for a single video clip. Each pin or interval is linked to a text field that can be used to house comments about that moment or interval. The application has a range of functionalities for supporting threaded discussion for each tagged moment or interval. We consider a commentary of a representation of classroom interaction to be the full set of annotations (including markings and comments associated with moments and intervals) in the video clip (see Figure 6).
Figure 6. A screenshot of Anotemos.
A teacher educator using the application can view the work of all of the client users on their timelines in real-time and can thus monitor that work in a way that is analogous to walking around a classroom. Thus, teacher educators can use the application to manage instructional exchanges at the level of an individual teacher candidate or the whole class. When interested in seeing whether a teacher education class has learned teaching moves associated with a particular decomposition of practice (e.g., launching a task; Wieman and Webel, 2019), the teacher educator can provide labeled pins of different colors and can monitor what moments or intervals are pinned as examples of particular component moves of the practice being taught. When interested in learning whether their students have incorporated taught decompositions of practice into their professional vision, teacher educators can leave the naming of the pins more open to interpretation and ask students simply to pin moments that they notice. The moments then noticed in a video by a class of students may indicate whether a particular decomposition has been taken on board. Finally, when teacher educators seek insight into teacher candidates' professional judgment, another use of pins might be to ask students to identify teacher actions in a video and then to comment on the pros and cons of each action. The exchange of such meanings may help a teacher educator develop a sense of the capacity of a class of teacher candidates to reflect on practice.
In contrast to Anotemos, LessonDepict (www.lessondepict.org; Herbst et al., 2020) is a software application designed to support the authoring of multimedia representations of teaching and learning using non-descript cartoon characters. LessonDepict asks users to utilize conventions (e.g., like how to order the placement of speech bubbles in a frame) from the world of comics (McCloud, 2000) to represent key elements of the enactment of a lesson in school by producing a storyboard of the lesson. LessonDepict supports collaborative storyboarding and the creation of alternative developments within a storyboard.
A storyboard produced with LessonDepict is a set of frames that represent a classroom scenario as an interaction among non-descript, but customizable, cartoon characters. When creating a storyboard, the user begins by choosing a classroom template and dragging it onto a frame. All of the elements on the frame can be dragged and tweaked to create desired effects. For example, once chosen, the template can be customized by personalizing a character's facial expression, skin color, and what the character holds in its hands. Finally, the user can add dialogue by positioning and filling speech bubbles and zooming into specific parts of the frame that they have created. To reduce the overhead in making storyboards, frames can be copied and then edited to create continuity between consecutive frames. A storyboard consists of a sequence of frames that can be shown, one frame at a time, as a slideshow or exported as a storyboard (see Figure 7).
Figure 7. A storyboard of instruction.
As suggested by the description above, LessonDepict supports both teacher educators and teacher candidates in authoring, rather than recording, representations of practice (either of their practice or some other teacher's practice). Storyboards produced with LessonDepict show, rather than tell, what happened in classroom interaction with little reliance on descriptions. Thus, like videos of teaching that focus on classroom interaction and do not have interviews with teachers about what they were doing superimposed over the classroom footage, speech bubbles feature the kinds of words that capture talk in mathematics classrooms and do not rely heavily on a shared technical descriptive vocabulary about teaching.
Teacher educators and teacher candidates can use LessonDepict to author storyboards with different sorts of purposes. For example, teacher candidates can represent what they did in classroom instruction in compulsory settings, perhaps highlighting instructional moves that they made. Or teacher candidates can use storyboards to represent what they saw in classroom placement. Rather than showing what happened, as part of learning to plan to teach, teacher candidates could also represent what they think would happen in future. Such predictions can then be compared with storyboards of what did happen, and teacher candidates can reflect on differences between the prediction and the actual event (e.g., see Amidon et al., 2017). Alternatively, teacher educators can use storyboards to represent how they decompose instruction into particular practices (see Wieman and Webel, 2019).
In sum, we see these two applications as offering teacher educators ways to have students author representations of practice and annotations of representations of practice that can be used in the context of the sorts of instructional exchanges we have outlined in teacher education. Beyond supporting instructional exchanges in teacher education, in our view, these technological tools are an important part of an infrastructure for supporting transaction of practice more generally [Herbst et al. (2023) outline other elements of such an infrastructure], including, for example, between researcher on instruction and practicing teachers [see Herbst and Chazan (2015) for use of storyboards in survey research on teaching].
5. Concluding thoughts
This article has ranged crosses between mathematics instruction and mathematics teacher education in an exploration of the utility of the construct of instructional exchanges to understand both similarities and differences between these two practices. For us, and we hope for readers as well, this exploration has underscored ways in which teacher education does not have one single analog to the mathematics problem within instruction in mathematics classrooms, at least on the surface. Similarly, teacher education does not seem to have as many widely used student work formats as high school mathematics teaching, but, as the examples of Anotemos and LessonDepict suggest, teacher education may benefit from technological tools that support the transaction of practice. We suggest that investment in such infrastructure could be valuable for the mathematics teacher education community. Finally, we also hope that this article illustrates how the theory might contribute to practice by helping us understand why particular work formats and technologies can be useful for instruction.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Author contributions
DC wrote the first draft of the manuscript with PH contributing paragraphs as needed. Both authors conceptualized the paper together, contributed to manuscript revision, read, and approved the submitted version.
Funding
Funding for work with teacher educators referenced in this paper was provided by National Science Foundation, DRL1316241, Developing Rich Media-Based Materials for Practice-Based Teacher Education. This article represents the thoughts of the authors, rather than of the foundation.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher's note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Amador, J. M., Estapa, A., Kosko, K., and Weston, T. (2021). Prospective teachers' noticing and mathematical decisions to respond: Using technology to approximate practice. Int. J. Math. Educ. Sci. Technol. 52, 3–22. doi: 10.1080/0020739X.2019.1656828
Amidon, J., Chazan, D., Grosser-Clarkson, D., and Fleming, E. (2017). Meet me in Azul's room: designing a virtual field placement for learning to teach mathematics. Math. Teach. Educ. 6, 52–66. doi: 10.5951/mathteaceduc.6.1.0052
Breslich, E. (1931). Problems in Teaching Secondary School Mathematics. Chicago, IL: University of Chicago.
Brophy, J. (2004). Using Video in Teacher Education (Vol. 10). Stamford, CT: JAI Press. doi: 10.1016/S1479-3687(2003)10
Brousseau, G. (1997). Theory of Didactical Situations in Mathematics: Didactique des Mathématiques, 1970-1990 (Transl. by N. Balacheff, M. Cooper, R. Sutherland, and V. Warfield). Dordrecht: Kluwer.
Buchbinder, O., Chazan, D., and Capozzoli, M. (2019). Solving equations: exploring instructional exchanges as lenses to understand teaching and its resistance to reform. J. Res. Math. Educ. 50, 18–51. doi: 10.5951/jresematheduc.50.1.0051
Buchbinder, O., Chazan, D., and Fleming, E. (2015). Insights into the school mathematics tradition from solving linear equations. For Learn. Math. 35, 2–9.
Chazan, D., Herbst, P., and Clark, L. (2016). “Research on the teaching of mathematics: a call to theorize the role of society and schooling in mathematics instruction,” in Handbook of Research on Teaching, 5th ed., eds D. Gitomer, and C. Bell (Washington, DC: American Educational Research Association), 1039–1097. doi: 10.3102/978-0-935302-48-6_17
Chazan, D., and Lueke, H. M. (2009). “Exploring relationships between disciplinary knowledge and school mathematics: implications for understanding the place of reasoning and proof in school mathematics,” in Teaching and Learning of Proof Across the Grades: A k-16 Perspective, eds D. Stylianou, E. Knuth, and M. Blanton (New York, NY: Routledge), 21–39. doi: 10.4324/9780203882009-2
Chazan, D., Sela, H., and Herbst, P. (2012). Is the role of equations in the doing of word problems in school algebra changing? Initial indications from teacher study groups. Cogn. Instr. 30, 1–38. doi: 10.1080/07370008.2011.636593
Chieu, V. M., Kosko, K. W., and Herbst, P. (2015). An analysis of evaluative comments in teachers' online discussions of representations of practice. J. Teach. Educ. 66, 35–50. doi: 10.1177/0022487114550203
Doyle, W. (1988). Work in mathematics classes: the context of students' thinking during instruction. Educ. Psychol. 23, 167–180. doi: 10.1207/s15326985ep2302_6
Falk, B. (2006). A conversation with lee shulman—signature pedagogies for teacher education: defining our practices and rethinking our preparation. New Educ. 2, 73–82. doi: 10.1080/15476880500486145
Fenstermacher, G. D. (2007). Teaching educational foundations: multiple explorations of pedagogy in teacher education. New Educ. 3, 95–102. doi: 10.1080/15476880701309807
Ghousseini, H., and Herbst, P. (2016). Pedagogies of practice and opportunities to learn about classroom mathematics discussions. J. Math. Teach. Educ. 19, 79–103. doi: 10.1007/s10857-014-9296-1
Goodwin, C. (1994). Professional vision. Am. Anthropol. 96, 606–633. doi: 10.1525/aa.1994.96.3.02a00100
Grossman, P., Compton, C., Igra, D., Ronfeldt, M., Shahan, E., Williamson, P., et al. (2009). Teaching practice: a cross-professional perspective. Teach. Coll. Rec. 111, 2055–2100. doi: 10.1177/016146810911100905
Herbel-Eisenmann, B. A., Steele, M. D., and Cirillo, M. (2013). (Developing) teacher discourse moves: a framework for professional development. Math. Teach. Educ. 1, 181–196. doi: 10.5951/mathteaceduc.1.2.0181
Herbst, P. (2002). Establishing a custom of proving in american school geometry: evolution of the two-column proof in the early twentieth century. Educ. Stud. Math. 49, 283–312. doi: 10.1023/A:1020264906740
Herbst, P. (2003). Using novel tasks in teaching mathematics: three tensions affecting the work of the teacher. Am. Educ. Res. J. 40, 197–238. doi: 10.3102/00028312040001197
Herbst, P. (2010). “Practical rationality and the justification for actions in mathematics teaching,” in Proceedings of the 2010 Annual PME-NA Conference (Columbus, OH: Ohio State).
Herbst, P., and Chazan, D. (2003). Exploring the practical rationality of mathematics teaching through conversations about videotaped episodes: the case of engaging students in proving. For Learn. Math. 23, 2–14.
Herbst, P., and Chazan, D. (2011). Research on practical rationality: Studying the justification of action in mathematics teaching. Math. Enthus. 8, 405–462. doi: 10.54870/1551-3440.1225
Herbst, P., and Chazan, D. (2012). On the instructional triangle and the sources of justification for the actions of the mathematics teacher. ZDM 44, 601–612. doi: 10.1007/s11858-012-0438-6
Herbst, P., and Chazan, D. (2015). Using multimedia scenarios delivered online to study professional knowledge use in practice. Int. J. Res. Method Educ. 38, 272–287. doi: 10.1080/1743727X.2015.1025742
Herbst, P., and Chazan, D. (2020). Mathematics teaching has its own imperatives: mathematical practice and the work of mathematics instruction. ZDM Math. Educ. 52, 1149–1162. doi: 10.1007/s11858-020-01157-7
Herbst, P., and Chazan, D. (2023). “Keeping theorizing in touch with practice: practical rationality as a middle range theory of mathematics teaching,” in Theorizing Teaching: Bringing Together Expert Perspectives to Move the Field Forward, eds A.-K. Praetorius, and C. Charalambos (Cham: Springer), 189–224. doi: 10.1007/978-3-031-25613-4_7
Herbst, P., Chazan, D., and Lavu, S. (2019). Anotemos. Web-based Collaborative Software Tool for the Annotation of Video. Disclosed to the Office of Technology Transfer, University of Michigan. Available online at: www.anotemos.com (accessed June 30, 2023).
Herbst, P., Chazan, D., and Lavu, S. (2020). LessonDepict. Web-based Collaborative Software Tool for the Creation of Storyboards and Storyboard Maps. Disclosed to the Office of Technology Transfer, University of Michigan. Available online at: www.lessondepict.org (accessed June 30, 2023).
Herbst, P., Chazan, D, and Schleppegrell, M. (2023), “A semiotic infrastructure for the transaction of instructional practice: Toward a theory of representation of practice,” in Handbook of Digital Resources in Mathematics Education, eds B. Pepin, G. Gueudet, J. Choppin (Cham: Springer).
Herbst, P., Chen, C., Weiss, M., González, G., Nachlieli, T., Hamlin, M., et al. (2009). “Doing proofs,” in Teaching and Learning of Proof Across the Grades: A K-16 Perspective, eds D. A. Stylianou, M. L. Blanton, and E. J. Knuth (New York, NY: Routledge), 250–268. doi: 10.4324/9780203882009-15
Herbst, P., Nachlieli, T., and Chazan, D. (2011). Studying the practical rationality of mathematics teaching: what goes into “installing” a theorem in geometry? Cogn. Instr. 29, 218–255. doi: 10.1080/07370008.2011.556833
Herbst, P., with González, G., Hsu, H. Y., Chen, C., Weiss, M., and Hamlin, M. (2010). Instructional Situations and Students' Opportunities to Reason in the High School Geometry Class. Manuscript. Deep Blue at the University of Michigan. Available online at: http://hdl.handle.net/2027.42./78372 (accessed June 30, 2023).
Hughes, K. K., Welch, A. R., and Moreland, A. L. (2020). “The uteach instructional program, elements, and courses,” in Preparing STEM Teachers: The UTeach Replication Model, eds J. E. Goodell, and S. Koç (Charlotte, NC: IAP), 163–174.
Kazemi, E., and Wæge, K. (2015). Learning to teach within practice-based methods courses. Math. Teach. Educ. Dev. 17, 125–145.
Lakoff, G., and Núñez, R. E. (2000). Where Mathematics Comes from: How the Embodied Mind Brings Mathematics into Being. New York, NY: Basic Books.
Lampert, M. (2001). Teaching Problems and the Problems of Teaching. New Haven, CT: Yale University Press.
Lampert, M., Franke, M. L., Kazemi, E., Ghousseini, H., Turrou, A. C., Beasley, H., et al. (2013). Keeping it complex using rehearsals to support novice teacher learning of ambitious teaching. J. Teach. Educ. 64, 226–243. doi: 10.1177/0022487112473837
McCloud, S. (2000). Reinventing Comics: How Imagination and Technology are Revolutionizing an art Form. New York, NY: Harper Paperbacks.
McKnight, P. C. (1971). Microteaching in teacher training. Res. Educ. 6, 24–38. doi: 10.1177/003452377100600103
Popkewitz, T. (2004). The alchemy of the mathematics curriculum: inscriptions and the fabrication of the child. Am. Educ. Res. J. 41, 3–34. doi: 10.3102/00028312041001003
Reys, R. E., and Dossey, J. A. (2008). US Doctorates in Mathematics Education: Developing Stewards of the Discipline. Providence, RI: American Mathematical Society. doi: 10.1090/cbmath/015
Sherin, M. G., and Han, S. Y. (2004). Teacher learning in the context of a video club. Teach. Teach. Educ. 20, 163–183. doi: 10.1016/j.tate.2003.08.001
Stein, M. K., Engle, R. A., Smith, M. S., and Hughes, E. K. (2008). Orchestrating productive mathematical discussions: five practices for helping teachers move beyond show and tell. Math. Think. Learn. 10, 313–340. doi: 10.1080/10986060802229675
van Es, E. A., and Sherin, M. G. (2008). Mathematics teachers'“learning to notice” in the context of a video club. Teach. Teach. Educ. 24, 244–276. doi: 10.1016/j.tate.2006.11.005
Walkoe, J., and Levin, D. M. (2018). Using technology in representing practice to support preservice teachers' quality questioning: the roles of noticing in improving practice. J. Technol. Teach. Educ. 26, 127–147.
Walkoe, J. D., and Luna, M. J. (2020). What we are missing in studies of teacher learning: a call for microgenetic, interactional analyses to examine teacher learning processes. J. Learn. Sci. 29, 285–307. doi: 10.1080/10508406.2019.1681998
Wieman, R., and Webel, C. (2019). Patterns linking interpreting and deciding how to respond during the launch of a lesson: noticing from an integrated perspective. Math. Teach. Educ. Dev. 21, 28–50.
Keywords: instruction, teacher noticing, teacher reflection, teacher moves, instructional exchanges, practical rationality
Citation: Chazan D and Herbst P (2023) Extending use of instructional exchanges to research on teacher education. Front. Educ. 8:1163396. doi: 10.3389/feduc.2023.1163396
Received: 10 February 2023; Accepted: 20 June 2023;
Published: 14 July 2023.
Edited by:
Stefinee Pinnegar, Brigham Young University, United StatesReviewed by:
Yael Nurick, Ludwigsburg University of Education, GermanyGrace Ukasoanya, University of Manitoba, Canada
Dina Tirosh, Tel Aviv University, Israel
Copyright © 2023 Chazan and Herbst. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Daniel Chazan, dchazan@umd.edu