Anti-Kekulé number of the {(3, 4), 4}-fullerene*

A {(3,4),4}-fullerene graph G is a 4-regular plane graph with exactly eight triangular faces and other quadrangular faces. An edge subset S of G is called an anti-Kekulé set, if G − S is a connected subgraph without perfect matchings. The anti-Kekulé number of G is the smallest cardinality of anti-Kekulé sets and is denoted by $ak\left(G\right)$. In this paper, we show that $4\le ak\left(G\right)\le 5$; at the same time, we determine that the {(3, 4), 4}-fullerene graph with anti-Kekulé number 4 consists of two kinds of graphs: one of which is the graph ${\mathcal{H}}_1$ consisting of the tubular graph $Q_n\left(n\ge 0\right)$, where Q_n is composed of $n\left(n\ge 0\right)$ concentric layers of quadrangles, capped on each end by a cap formed by four triangles which share a common vertex (see Figure 2 for the graph Q_n); and the other is the graph ${\mathcal{H}}_2$, which contains four diamonds D₁, D₂, D₃, and D₄, where each diamond $D_i\left(1\le i\le 4\right)$ consists of two adjacent triangles with a common edge $e_i\left(1\le i\le 4\right)$ such that four edges e₁, e₂, e₃, and e₄ form a matching (see Figure 7D for the four diamonds D₁ − D₄). As a consequence, we prove that if $G\in {\mathcal{H}}_1$, then $ak\left(G\right)=4$; moreover, if $G\in {\mathcal{H}}_2$, we give the condition to judge that the anti-Kekulé number of graph G is 4 or 5.

1 Introduction

A {(3,4),4}-fullerene graph G is a 4-regular plane graph with exactly eight triangular faces and other quadrangular faces. This concept of the {(3, 4), 4}-fullerene comes from Deza’s {(R,k)}-fullerene (Deza and Sikirić, 2012). Fixing R ⊂ N, a {(R, k)}-fullerene graph is a k-regular $\left(k\ge 3\right)$, and it is mapped on a sphere whose faces are i-gons $\left(i\in R\right)$. A {(a,b),k}-fullerene is {(R, k)}-fullerene with $R=\left\{a,b\right\}\left(1\le a\le b\right)$. The {(a, b), k}-fullerene draws attention because it includes the mostly widely researched graphs, such as fullerenes (i.e.,{(5, 6), 3}-fullerenes), boron–nitrogen fullerenes (i.e.,{(4, 6), 3}-fullerenes), and (3,6)-fullerenes (i.e.,{(3, 6), 3}-fullerenes) (Yang and Zhang, 2012).

The anti-Kekulé number of a graph was introduced by Vukičević and Trinajstić (2007). They introduced the anti-Kekulé number as the smallest number of edges that have to be removed from a benzenoid to remain connected but without a Kekulé structure. Here, a Kekulé structure corresponds to a perfect matching in mathematics; it is known that benzenoid hydrocarbon has better stability if it has a lower anti-Kekulé number. Veljan and Vukičević (2008) found that the anti-Kekulé numbers of the infinite triangular, rectangular, and hexagonal grids are 9, 6 and 4, respectively. Zhang et al. (2011) proved that the anti-Kekulé number of cata-condensed phenylenes is 3. For fullerenes, Vukičević (2007) proved that C₆₀ has anti-Kekulé number 4, and Kutnar et al. (2009) showed that the leapfrog fullerenes have the anti-Kekulé number 3 or 4 and that for each leapfrog fullerene, the anti-Kekulé number can be established by observing the finite number of cases independent of the size of the fullerene. Furthermore, this result was improved by Yang et al. (2012) by proving that all fullerenes have anti-Kekulé number 4.

In general, Li et al. (2019) showed that the anti-Kekulé number of a 2-connected cubic graph is either 3 or 4; moreover, all (4,6)-fullerenes have the anti-Kekulé number 4, and all the (3,6)-fullerenes have anti-Kekulé number 3. Zhao and Zhang (2020) confirmed all (4,5,6)-fullerenes have anti-Kekulé number 3, which consist of four sporadic (4,5,6)-fullerenes (F₁₂, F₁₄, F₁₈, and F₂₀) and three classes of (4,5,6)-fullerenes with at least two and at most six pentagons.

Here, we consider the {(3, 4), 4}-fullerene graphs. In the next section, we recall some concepts and results needed for our discussion. In Section 3, by using Tutte’s Theorem on perfect matching of graphs, we determine the scope of the anti-Kekulé number of the {(3, 4), 4}-fullerene. Finally, we show that the {(3, 4), 4}-fullerene with anti-Kekulé number 4 consists of two kinds of graphs ${\mathcal{H}}_1,{\mathcal{H}}_2$. As a consequence, we prove that if $G\in {\mathcal{H}}_1$, then $ak\left(G\right)=4$. Moreover, if $G\in {\mathcal{H}}_2$, we give the condition to judge that the anti-Kekulé number of graph G is 4 or 5.

2 Definitions and preliminary results

Let $G=\left(V,E\right)$ be a simple and connected plane graph with vertex set V(G) and edge set E(G). For $V^{\prime }\subseteq V\left(G\right)$, G − V′ denotes the subgraph obtained from G by deleting the vertices in V′ together with their incident edges. If V′ = v, we write G − v. Similarly, for $E^{\prime }\subseteq E\left(G\right)$, G − E′ denotes the graph with vertex set V(G) and edge set $E\left(G\right)-E^{\prime }$. If E′ = e, we write G − e. Let V′ be a non-empty set; $G\left[V^{\prime }\right]$ denotes the induced subgraph of G induced by the vertices of V′; similarly, if $E^{\prime }\subseteq E\left(G\right)$, $G\left[E^{\prime }\right]$ denotes the induced subgraph of G induced by the edges of E′.

For a subgraph H of G, the induced subgraph of G induced by vertices of $V\left(G\right)-V\left(H\right)$ is denoted by $\bar{H}$. A plane graph G partitions the rest of the plane into a number of arcwise-connected open sets. These sets are called the faces of G. A face is said to be incident with the vertices and edges in its boundary, and two faces are adjacent if their boundaries have an edge in common. Let $F\left(G\right)$ be the set of the faces of G.

An edge-cut of a connected plane graph G is a subset of edges $C\subseteq E\left(G\right)$ such that G − C is disconnected. A k-edge-cut is an edge-cut with k edges. A graph G is k-edge-connected if G cannot be separated into at least two components by removing less than k edges. An edge-cut C of a graph G is cyclic if its removal separates two cycles. A graph G is cyclically k-edge-connected if G cannot be separated into at least two components, each containing a cycle, by removing less than k edges. A cycle is called a facial cycle if it is the boundary of a face.

For subgraphs H₁ and H₂ of a plane graph G, $E\left(H_1,H_2\right)=E\left(V\left(H_1\right),V\left(H_2\right)\right)$ represents the set of edges whose two end vertices are in $V\left(H_1\right)$ and $V\left(H_2\right)$ separately. If $V\left(H_1\right)$ and $V\left(H_2\right)$ are two non-empty disjoint vertex subsets such that $V\left(H_1\right)\cup V\left(H_2\right)=V\left(G\right)$, then $E\left(H_1,H_2\right)$ is an edge-cut of G, and we simply write $\nabla \left(H_1\right)=\nabla \left(V\left(H_1\right)\right)$ or $\nabla \left(H_2\right)=\nabla \left(V\left(H_2\right)\right)$. We use $\partial \left(G\right)$ to denote the boundary of G, that is, the boundary of the infinite face of G.

A matching M of a graph G is a set of edges of G such that no two edges from M have a vertex in common. A matching M is perfect if it covers every vertex of G. A perfect matching is also called a Kekulé structure in chemistry.

Let G be a connected graph with at least one perfect matching. For $S\subseteq E\left(G\right)$, we call S an anti-Kekulé set if G − S is connected but has no perfect matchings. The smallest cardinality of anti-Kekulé sets of G is called the anti-Kekulé number and denoted by $ak\left(G\right)$.

For the edge connectivity of the {(3, 4), 4}-fullerene, we have the following results.

Lemma 2.1. ((Yang et al., 2023) Lemma 2.3) Every {(3, 4), 4}-fullerene is cyclically 4-edge-connected.

Lemma 2.2. ((Yang et al., 2023) Corollary 2.4) Every {(3, 4), 4}-fullerene is 4-edge-connected.

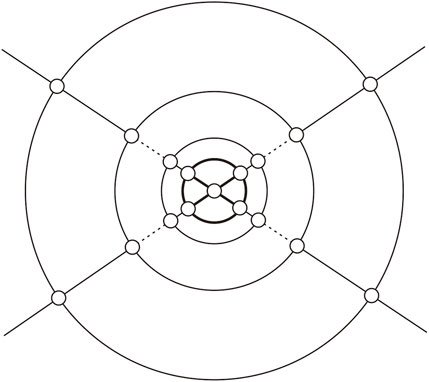
Q_n is the graph consisting of n concentric layers of quadrangles, capped on each end by a cap formed by four triangles which share a common vertex as shown in Figure 2. In particular, Q₀ is what we call an octahedron (see Figure 5F).

Lemma 2.3. ((Yang et al., 2023) Lemma 2.5) If G has a cyclical 4-edge-cut $E=\left\{e_1,e_2,e_3,e_4\right\}$, then $G\cong Q_n\left(n\ge 1\right)$, where the four edges e₁, e₂, e₃, and e₄ form a matching, and each e_i belongs to the intersection of two quadrilateral faces for i = 1, 2, 3, 4.

Tutte’s theorem plays an important role in the process of proof.

Theorem 2.4. (Lovász and Plummer, 2009) (Tutte’s theorem) A graph G has a perfect matching if and only if for any $X\subseteq V\left(G\right)$, $o\left(G-X\right)\le \left|X\right|$, where $o\left(G-X\right)$ denotes the number of odd components of G − X.

Here, an odd component of G − X is trivial if it is just a single vertex and non-trivial otherwise.

All graph-theoretical terms and concepts used but unexplained in this article are standard and can be found in many textbooks, such as Lovász and Plummer (2009).

3 Main results

From now on, let G always be a {(3, 4), 4}-fullerene; we called a 4-edge-cut E in G trivial if $E=\nabla \left(v\right)$, that is, E consists of the four edges incident to v. By Lemma 2.3, if E is a cyclical 4-edge-cut, then the four edges in E form a matching. Moreover, if E is not a cyclical 4-edge-cut, then E is trivial. So, we have the following lemma.

Lemma 3.1. Let G be a {(3, 4), 4}-fullerene, $E=\left\{e_1,e_2,e_3,e_4\right\}$ be an 4-edge-cut, but it is not cyclical, then E is trivial.

Proof. Since $E=\left\{e_1,e_2,e_3,e_4\right\}$ is an 4-edge-cut, G − E is not connected. Then, G − E has at least two components. Moreover, as G is 4-edge-connected by Lemma 2.2, G − E has at most two components. So, G − E has exactly two components.

Let G₁, G₂ be two components of G − E. Since E is not cyclical, without loss of generality, we suppose that G₁ is a forest; then, we have

$n-e=l,(1)$

where n, e, l is the number of vertices, edges, and trees in G₁, respectively. Furthermore, since each vertex of G is of degree 4, we have

$4n-4=2e.(2)$

Combing with equalities 1) and 2), we know n = l = 1 and e = 0, which means G₁ only consists of a single vertex. So, E is trivial. □

Lemma 3.1 plays an important role in the proof of the following theorem. Next, we explore the scope of the anti-Kekulé number of {(3, 4), 4}-fullerene.

Theorem 3.2. Let G be a {(3, 4), 4}-fullerene, then $4\le ak\left(G\right)\le 5$.

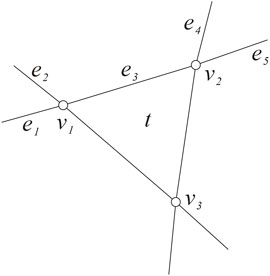
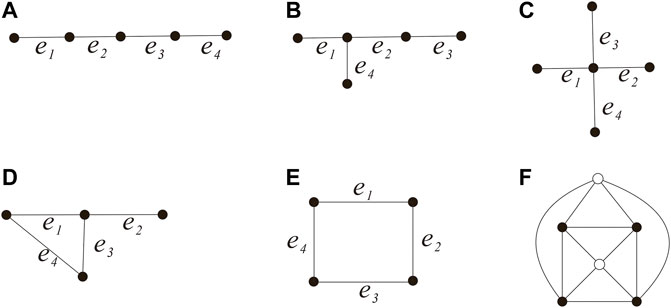
Proof. First, we show $ak\left(G\right)\le 5$. Let t be any triangle in G and the boundary of t was labeled v₁v₂v₃ along the clockwise direction. Denote the other two edges incident to $v_1\left(v_2\right)$ by $e_1,e_2\left(e_4,e_5\right)$, set e₃ = v₁v₂, then e₁, e₂, e₃, e₄, and e₅ are pairwise different, set $E^{\prime }=\left\{e_1,e_2,e_3,e_4,e_5\right\}$ (see Figure 1) and G′ = G − E′.

In order to show $ak\left(G\right)\le 5$, we only need to prove that G′ is connected and has no perfect matchings. Then, G′ has no perfect matchings since the two edges v₁v₃, v₂v₃ cannot be covered by a perfect matching at the same time in G′.

In the following, we show that G′ is connected. We proved this using reduction to absurdity, suppose G′ is not connected, then G′ has a component (say G₁) containing vertices v₁, v₂, and v₃, as v₁, v₂, and v₃ are connected by the path v₁v₃v₂ in G₁. On the other hand, since e₃ = v₁v₂ connects two vertices v₁, v₂ in G and $E^{\prime }=\left\{e_1,e_2,e_3,e_4,e_5\right\}$ is an edge cut of G, even if we remove five edges, e₁, e₂, e₃, e₄, and e₅, to disconnect G, it is actually the same as removing four edges, e₁, e₂, e₄, and e₅ (see Figure 1); that is, $E_1=\left\{e_1,e_2,e_4,e_5\right\}$ is an 4-edge-cut. Moreover, due to Lemma 2.3, E₁ cannot be a cyclical 4-edge-cut as e₁, e₂, e₄, and e₅ is not a matching. Then, according to Lemma 3.1, E₁ is a trivial 4-edge-cut. Thus, G₁ or $\overline{G_1}$ is a single vertex, both of which are impossible by the definition of G. So G′ is connected. Thus,

$ak\left(G\right)\le 5.(3)$

Finally, we show $ak\left(G\right)\ge 4$. By the definition of an anti-Kekulé set, suppose $E_1^{\prime }=\left\{e_1^{\prime },e_2^{\prime },e_3^{\prime },\dots ,e_k^{\prime }\right\}$ was the smallest anti-Kekulé set of G, that is, $ak\left(G\right)=k$. Then, $G_1^{\prime }=G-E_1^{\prime }$ was connected and has no perfect matching. Hence, according to Theorem 2.4, there exists a non-empty subset $X_0\subseteq V\left(G_1^{\prime }\right)$ such that $o\left(G_1^{\prime }-X_0\right)>\left|X_0\right|$, since $\left|V\left(G_1^{\prime }\right)\right|=\left|V\left(G\right)\right|$ and $\left|V\left(G\right)\right|$ is even, $o\left(G_1^{\prime }-X_0\right)$ and $\left|X_0\right|$ have the same parity. Consequently,

$o\left(G_1^{\prime }-X_0\right)\ge \left|X_0\right|+2(4)$

For the sake of convenience, we let $\alpha =o\left(G_1^{\prime }-X_0\right)$. If we chose an X₀ with the maximum size, then $G_1^{\prime }-X_0$ has no even components. On the contrary, we suppose there exists an even component (say F) of $G_1^{\prime }-X_0$. For any vertex $v\in V\left(F\right)$, $o\left(F-v\right)\ge 1$. Let $X^{\prime }=X_0\cup \left\{v\right\}$, thus $o\left(G_1^{\prime }-X^{\prime }\right)=o\left(G_1^{\prime }-X_0\right)+o\left(F-v\right)\ge \left|X_0\right|+2+1=\left|X^{\prime }\right|+2$, which is a contradiction to the choice of X₀.

In addition, $E_1^{\prime }$ is the smallest anti-Kekulé set of G, then $G_1^{\prime }+e_i^{\prime }$ has perfect matchings for any edge $e_i^{\prime }\in E_1^{\prime }$ for 1 ≤ i ≤ k. On the other hand, the number of odd components of $G_1^{\prime }-X_0$ was not decreased or decreased by at most one or two if we add one edge $e_i^{\prime }$ to $G_1^{\prime }$, that is,

$\left|X_0\right|\ge o\left(G_1^{\prime }+e_i^{\prime }-X_0\right)\ge \alpha -2.(5)$

By inequality (4), we have

$\left|X_0\right|\le \alpha -2.(6)$

Combined with inequalities (5) and (6), we have $\alpha =\left|X_0\right|+2$ and each edge $e_i^{\prime }\in E_1^{\prime }$ connects two odd components of $G_1^{\prime }-X_0$. Let H₁, H₂, H₃, …, H_α be the odd components of $G_1^{\prime }-X_0$. Then, due to Lemma 2.2, $\left|\nabla \left(H_i\right)\right|\ge 4\left(1\le i\le \alpha \right)$; therefore,

$4\alpha -2k\le \sum _{i=1}^{\alpha }\left|\nabla \left(H_i\right)\right|-2\left|E_1^{\prime }\right|\le 4\left|X_0\right|=4\left(\alpha -2\right).(7)$

Thus, k ≥ 4, that is, $ak\left(G\right)\ge 4$. We know that $4\le ak\left(G\right)\le 5$.

By Theorem 3.2, we know that $4\le ak\left(G\right)\le 5$. Next, we give the characterization of {(3, 4), 4}-fullerenes with anti-Kekulé number 4. Before, we define ${\mathcal{H}}_1=\left\{Q_n\left|n\ge 0\right.\right\}$, where Q_n is shown in Figure 2. The structure of two adjacent triangles is called a diamond. In a diamond, the common edge of the two triangles is called the diagonal edge. The subgraph consisting of four diamonds such that the four diagonal edges form a matching is denoted by D, that is, $D=\bigcup _{i=1}^4{D}_i$ (see Figure 7D for the four diamonds D₁ − D₄). Let ${\mathcal{H}}_2=\left\{G|D\subseteq G\right\}$. So, we have the following theorem.

FIGURE 1

FIGURE 1. Edges e₁e₂, e₃, e₄, and e₅.

FIGURE 2

FIGURE 2. {(3,4),4}-Fullerene Q_n, where the bold segments indicate the cap of Q_n (n ≥0).

Theorem 3.3. Let G be a {(3, 4), 4}-fullerene, if $ak\left(G\right)=4$, then $G\in {\mathcal{H}}_1$ or $G\in {\mathcal{H}}_2$.

Proof. Let E₀ be the anti-Kekulé set of G such that $\left|E_0\right|=4$, set G₀ = G − E₀. Then, G₀ is connected without perfecting matchings. Thus, by Theorem 2.4, there exists a non-empty subset $X_0\subseteq V\left(G_0\right)$ such that $o\left(G_0-X_0\right)>\left|X_0\right|$. For convenience, let $\alpha =o\left(G_0-X_0\right)$, since α and $\left|X_0\right|$ have the same parity, that is,

$\alpha \ge \left|X_0\right|+2.(8)$

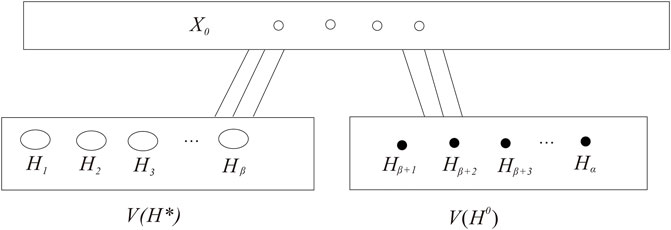
We choose an X₀ satisfying Ineq. (8) with the maximum size. Then, a proof similar to the proof of Theorem 3.2 is used to prove $ak\left(G\right)\ge 4$. We can know G₀ − X₀ has no even components. Let H₁, H₂, H₃, …, H_α be all the odd components of G₀ − X₀, set $H=\underset{i=1}{\overset{\alpha }{\cup }}{H}_i$.

Let H₁, H₂, H₃, …, H_β be the non-trivial odd components of G₀ − X₀, set $H^{*}=\underset{i=1}{\overset{\beta }{\cup }}{H}_i$. Let H_β+1, H_β+2, H_β+3, …, H_α be the trivial odd components of G₀ − X₀, set $H^0=\underset{i=\beta +1}{\overset{\alpha }{\cup }}{H}_i$. Then, $V\left(G\right)$ is divided into X₀, $V\left(H^{*}\right)$, $V\left(H^0\right)$(see Figure 3 the partition of $V\left(G\right)$).

Since $ak\left(G\right)=4$, all equalities in Ineq. (7) of Theorem 3.2 hold. The first equality in Ineq. (7) holds if and only if $\left|\nabla \left(H_i\right)\right|=4\left(1\le i\le \alpha \right)$, and the second equality in Ineq. 7) holds if and only if there is no edge in the subgraph $G_0\left[X_0\right]$; that is, X₀ is an independent set of G₀. Moreover, each edge of E₀ connects two components in H and $\left|X_0\right|=\alpha -2$. Since $\left|\nabla \left(H_j\right)\right|=4\left(1\le j\le \alpha \right)$, $\nabla \left(H_j\right)$ is a cyclical 4-edge-cut of G or not.

Next, we distinguish the following two cases to complete the proof of Theorem 3.3.

Case 1: There exists one H_j such that $\nabla \left(H_j\right)$ is a cyclical 4-edge-cut.

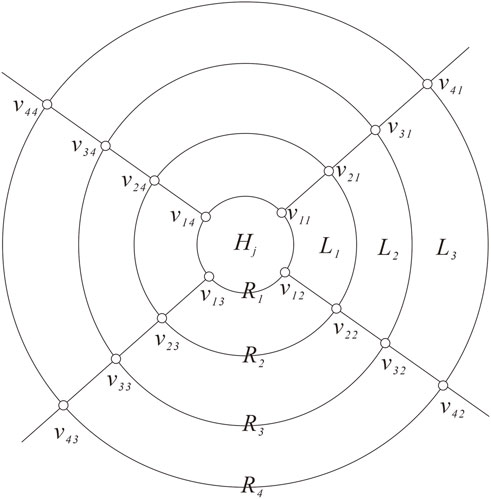
By Lemma 2.3, $G\cong Q_n\left(n\ge 1\right)$, which means the four edges of $\nabla \left(H_j\right)$ form a matching. Without loss of generality, we supposed H_j consists of s layers of quadrangular faces and the cap of H_j is entirely in the interior of the boundary cycle $\partial \left(H_j\right)$. Then, $G\left[V\left(\overline{H_j}\cup \partial \left(H_j\right)\right)\right]$ induced by the vertices of $\overline{H_j}$ and the boundary of $\partial \left(H_j\right)$ consists of n − s layers of quadrangular faces and a cap, for convenience, set m = n − s, let L₁, L₂, L₃, …, L_m be all the layers and C be the cap of $G\left[V\left(\overline{H_j}\cup \partial \left(H_j\right)\right)\right]$, where quadrangular layer L_i is adjacent to L_i−1 and L_i+1 for 2 ≤ i ≤ m − 1, L₁ is adjacent to H_j, and L_m is adjacent to C. Set R₁ = H_j ∩ L₁ and R_m+1 = C ∩ L_m. For 2 ≤ i ≤ m, let R_i = L_i−1 ∩ L_i. The vertices on $R_i\left(i=\mathrm{1,2,3},\dots ,m+1\right)$ are recorded as v_i1, v_i2, v_i3, and v_i4 (i = 1, 2, 3, …, m + 1) in a clockwise direction and v_i1, v_i3, and v_i2, v_i4, are on the same line, respectively (see Figure 4). Since $\nabla \left(H_j\right)$ is a cyclical 4-edge-cut, set $\nabla (H_j)=\{e_1^{\prime },e_2^{\prime },e_3^{\prime },e_4^{\prime }\}$. Without loss of generality, set $e_i^{\prime }=v_{1i}{v}_{2i}(1\le i\le 4)$. The vertices shared by the four triangles on the two caps are represented by v′, v^″, respectively, such that v′ is in H_j and v^″ is in $\overline{H_j}$.

Next, we analyze whether the edges of $\nabla \left(H_j\right)$ belongs to E₀ or not, which is divided into the following five subcases.

Subcase 1.1: All the edges of $\nabla \left(H_j\right)$ belong E₀.

That is, $e_i^{\prime }\in E_0$ for all i = 1, 2, 3, 4. Since each edge of E₀ connects two components of H and there are four edges $e_1^{\prime },e_2^{\prime },e_3^{\prime },e_4^{\prime }$ belonging to E₀. All the vertices of $\overline{H_j}$ belong to $V\left(H^{*}\right)$, which means X₀ = ∅, a contradiction.

Subcase 1.2: Exactly three edges of $\nabla \left(H_j\right)$ belong to E₀.

Without loss of generality, suppose $e_1^{\prime },e_2^{\prime },e_3^{\prime }\in E_0$, then v₂₄ ∈ X₀ and $v_{21},v_{22},v_{23}\in V\left(H\right)$, that is, v₂₁, v₂₂, v₂₃ belong to $V\left(H^{*}\right)$ or $V\left(H^0\right)$.

If all of v₂₁, v₂₂, and v₂₃ belong to $V\left(H^0\right)$, then v₂₁v₂₂, v₂₂v₂₃ ∈ E₀, immediately $\left|E_0\right|>4$, which contradicts $\left|E_0\right|=4$. This contradiction means at least one of v₂₁, v₂₂, and v₂₃ belongs to $V\left(H^{*}\right)$ (say $V\left(H_1\right)$), then by Lemma 2.3 and Lemma 3.1, either $\nabla \left(H_1\right)$ is a cyclical 4-edge-cut and the four edges in $\nabla \left(H_1\right)$ form a matching or $\nabla \left(H_1\right)$ is trivial. However, since H₁ is a non-trivial odd component of G₀ − X₀, $\left|V\left(H_1\right)\right|\ge 3$. Thus, $\nabla \left(H_1\right)$ is not a trivial 4-edge-cut. That is, $\nabla \left(H_1\right)$ is a cyclical 4-edge-cut, and the four edges in $\nabla \left(H_1\right)$ form a matching. Now, if v₂₁ (or v₂₃) belong to $V\left(H_1\right)$, then v₂₁v₂₄, v₂₁v₁₁ (or v₂₃v₂₄, v₂₃v₁₃) belong to $\nabla \left(H_1\right)$, but they do not form a matching, a contradiction. Thus, both $v_{21},v_{23}\in V\left(H^0\right)$ and $v_{22}\in V\left(H_1\right)$. Immediately, we have v₂₁v₂₂, v₂₂v₂₃ ∈ E₀ and $\left|E_0\right|>4$, which contradicts $\left|E_0\right|=4$. This contradiction means there cannot be three edges of $\nabla \left(H_j\right)$ belonging to E₀.

Subcase 1.3: Exactly two edges of $\nabla \left(H_j\right)$ belong to E₀.

Then, by symmetry, $e_1^{\prime },e_2^{\prime }\in E_0$ or $e_1^{\prime },e_3^{\prime }\in E_0$.

First, if $e_1^{\prime },e_2^{\prime }\in E_0$, then v₂₃, v₂₄ ∈ X₀ and $v_{23}{v}_{24}\in E\left(X_0\right)$, which contradicts that $E\left(X_0\right)=\varnothing$.

Claim 1: For a quadrangular face q with $\partial \left(q\right)=abcda$ with clock direction such that $a\in X_0,b\in V\left(H^0\right)$, then $c,d\in V\left(H^0\right)$ or $c,d\in V\left(H^{*}\right)$ or $c\in X_0,d\in V\left(H^0\right)$.

FIGURE 3

FIGURE 3. $V\left(G\right)$ is divided into X₀, $V\left(H^{*}\right)$, and $V\left(H^0\right)$.

FIGURE 4

FIGURE 4. Labeling of $G\left[V\left(\overline{H_j}\cup \partial \left(H_j\right)\right)\right]$.

Proof. Since $E\left(X_0\right)=\varnothing$, $d\in V\left(H^0\right)\cup V\left(H^{*}\right)$. If $d\in V\left(H^0\right)$, then $c\notin V\left(H^{*}\right)$ by Lemma 2.3 and Lemma 3.1, thus c ∈ X₀ or $c\in V\left(H^0\right)$.

If $d\in V\left(H^{*}\right)$, then also by Lemma 2.3 and Lemma 3.1, we can know $c\in V\left(H^{*}\right)$ and the claim holds.

By Claim 1, next, if $e_1^{\prime },e_3^{\prime }\in E_0$, then $v_{22},v_{24}\in V\left(X_0\right)$, $v_{21},v_{23}\in V\left(H\right)$. If all the vertices of v₂₁, v₂₂, v₂₃, and v₂₄ belong to the cap of $\overline{H_j}$, that is, all of v₂₁, v₂₂, v₂₃, and v₂₄ are adjacent to v^″, then as $\left|E_0\right|=4$ and $e_1^{\prime },e_3^{\prime }\in E_0$, we can know v^″ ∈ H⁰ and v₂₁v^″, v₂₃v^″ ∈ E₀, and we have the {(3, 4), 4}-fullerenes Q_s+1, that is, m = 1.

If all the vertices of v₂₁, v₂₂, v₂₃, and v₂₄ do not belong to the cap of $\overline{H_j}$, that is, the layer L₂ consists of four quadrangular faces, then, for the quadrangular face $q\in F\left(L_2\right)$, the vertices on $\partial \left(q\right)$ belong to X₀, H⁰, H⁰, H⁰ or X₀, H⁰, H*, H* or X₀, H⁰, X₀, H⁰ by Claim 1.

If the former case holds, that is, there exists one face $q\in F\left(L_2\right)$ such that the boundary of q is of the form X₀, H⁰, H⁰, and H⁰, then immediately we can have $\left|E_0\right|>4$, a contradiction.

If the second case holds, that is, there exists one face $q\in F\left(L_2\right)$ such that the boundary of q is of the form X₀, H⁰, H*, and H*, then by Claim 1 and since $\left|E_0\right|=4$, we can know all the faces of L₂ are of the form X₀, H⁰, H*, and H*, that is, all the vertices of $\overline{H_j\cup L_1}$ belong to $V\left(H^{*}\right)$. In this case, we also have $G\in {\mathcal{H}}_1$.

By the aforementioned discussion and Claim 1, next, we suppose all the quadrangular faces of L₂ are of the form X₀, H⁰, X₀, and H⁰. Then, we can use the aforementioned same analysis to the layer L₃ as L₂, since G ≅ Q_n and H_j consists of s layers of quadrangular faces; after finite steps (say t steps), we obtain t layers L₂, L₃, …, L_t+1 such that all the faces of $L_i\left(2\le i\le t+1\right)$ are of form X₀, H⁰, X₀, and H⁰ and either the four vertices on $\partial \left(R_{t+2}\right)$ are adjacent to $v^{\prime \prime }(v^{\prime \prime }\in V\left(H^0\right))$ or all the vertices of $\overline{H_j\cup L_1\cup L_2\cup \cdots \cup L_{t+1}}$ belong to $V\left(H^{*}\right)$.

If the four vertices on $\partial \left(R_{t+2}\right)$ are adjacent to $v^{\prime \prime }(v^{\prime \prime }\in V\left(H^0\right))$, then m = t + 1, n = s + t + 1 and $G\in {\mathcal{H}}_1$. If all the vertices of $\overline{H_j\cup L_1\cup L_2\cup \cdots \cup L_{t+1}}$ belong to $V\left(H^{*}\right)$ (say $V\left(H_1\right)$), suppose H₁ consists of p layers of quadrangular faces, then m = t + p + 2, n = s + t + p + 2, and also $G\in {\mathcal{H}}_1$.

To sum up, if exactly two edges of $\nabla \left(H_j\right)$ belong to E₀, then $G\in {\mathcal{H}}_1$.

Subcase 1.4: Exactly one edge of $\nabla \left(H_j\right)$ belong to E₀.

Without loss of generality, suppose $e_1^{\prime }\in E_0$, then v₂₂, v₂₃, v₂₄ ∈ X₀, $v_{22}{v}_{23},v_{23}{v}_{24}\in E\left(X_0\right)$, which contradicts that X₀ is an independent set of G₀.

Subcase 1.5: No edge of $\nabla \left(H_j\right)$ belongs to E₀.

Thus, $\bigcup _{i=1}^4{v}_{2i}\subseteq X_0$, so $v_{21}{v}_{22},v_{22}{v}_{23},v_{23}{v}_{24},v_{24}{v}_{21}\in E\left(X_0\right)$, which contradicts $E\left(X_0\right)=\varphi$.

Case 2: $\nabla \left(H_j\right)$ is not a cyclical 4-edge-cut of G for all 1 ≤ j ≤ α.

For convenience, set $E_0=\left\{e_1,e_2,e_3,e_4\right\}$. Here, first, we give the idea of proof, then we will show that G₀ = G − E₀ is bipartite by proving $\left|V\left(H_i\right)\right|=1\left(1\le i\le \alpha \right)$. Since G has exactly eight triangular faces and $\left|E_0\right|=4$, which implies that each edge e_i of E₀ is the common edge of two triangles, by discussing all possible subgraphs formed by facial cycles containing an edge of E₀, we show that $G\in {\mathcal{H}}_1$ or $G\in {\mathcal{H}}_2$.

Since $\nabla \left(H_j\right)$ is not a cyclical 4-edge-cut of G for all 1 ≤ j ≤ α, H_j or $\overline{H_j}$ is a singleton by Lemma 3.1. Since X₀ is non-empty and $\alpha =\left|X_0\right|+2$, which means H_j is a singleton vertex, that is, $\left|V\left(H_j\right)\right|=1\left(1\le j\le \alpha \right)$.

Let Y₀ denote the set of all singletons y_i from each $H_i\left(1\le i\le \alpha \right)$, and denote the vertices of X₀ by $x_i\left(1\le i\le \left|X_0\right|\right)$, so $G_0=\left(X_0,Y_0\right)$ is bipartite. For convenience, we color the vertices white in X₀ and black in Y₀.

Next, we consider possible subgraphs of G containing all edges of E₀. By the Euler theorem, G has exactly eight triangular faces because $G_0=\left(X_0,Y_0\right)$ is bipartite; each edge e_i of E₀ is the common edge of two triangles and connects two vertices in Y₀, that is, every edge e_i ∈ E₀ belongs to a diamond, say D_i, i = 1, 2, 3, 4 and $F\left(D_i\right)\cap F\left(D_j\right)=\varnothing \left(i\ne j,i,j=\mathrm{1,2,3,4}\right)$.

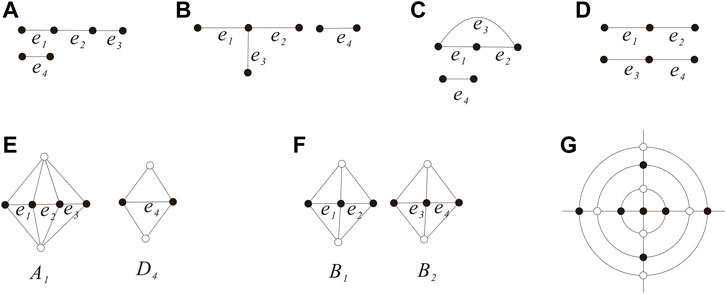
Claim 2: If $G\left[E_0\right]$ has one component, then G ≅ Q₀, where Q₀ is the octahedron.

Proof. If $G\left[E_0\right]$ has one component, then we have the subgraphs shown in Figures 5A, B, C) if $G\left[E_0\right]$ is a tree and Figures 5D, E if $G\left[E_0\right]$ has cycles. If $G\left[E_0\right]$ is isomorphism to the graph shown in Figure 5A, then the two diamonds D₁, D₂ are adjacent and they form one cap of Q_n. Set D₁₂ = D₁ ∪ D₂, then $\nabla \left(D_{12}\right)$ forms an 4-edge-cut. On the other hand, by Lemma 2.3 and Lemma 3.1, $\nabla \left(D_{12}\right)$ is a cyclical 4-edge-cut and G ≅ Q_p or $\nabla \left(D_{12}\right)$ is trivial. If $\nabla \left(D_{12}\right)$ is a cyclical 4-edge-cut, then $G\cong Q_p\left(p\ge 1\right)$ and e₃ belongs to a quadrangular face, which contradicts that the two faces containing e₃ are triangles. If $\nabla \left(D_{12}\right)$ is a trivial 4-edge-cut, that is, $\overline{D_{12}}$ is a singleton, which is impossible as the two vertices of e₄ belong to $V\left(\overline{D_{12}}\right)$. Thus, $G\left[E_0\right]$ cannot be isomorphism to the subgraph shown in Figure 5A. All the situations of Figures 5B–D contradicts $F\left(D_i\right)\cap F\left(D_j\right)=\varnothing \left(i\ne j,i,j=\mathrm{1,2,3,4}\right)$.

If $G\left[E_0\right]$ is isomorphic to the graph shown in Figure 5E, then in order to guarantee $F\left(D_i\right)\cap F\left(D_j\right)=\varnothing \left(i\ne j,i,j=\mathrm{1,2,3,4}\right)$, the four diamonds D₁, D₂, D₃, and D₄ forms two caps of Q_n such that the cycle induced by E₀ is exactly the intersecting of the two caps. Immediately, we have the graph Q₀ (see Figure 5F the octahedron Q₀), that is, G ≅ Q₀ if $G\left[E_0\right]$ has one component, so $G\in {\mathcal{H}}_1$.

In accordance with Claim 2, next, we assume that $G\left[E_0\right]$ is not connected, so $G\left[E_0\right]$ has at least two and at most four components. Then, we have the following three cases.

Subcase 2.1: $G\left[E_0\right]$ has exactly two components.

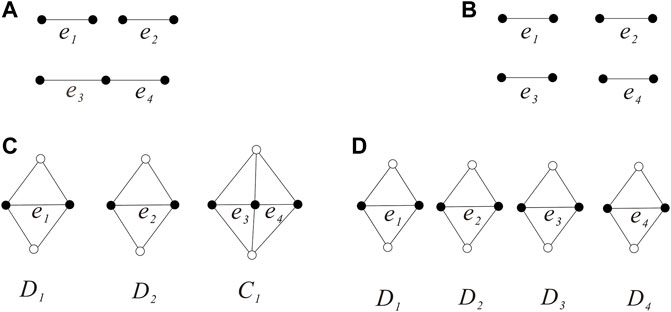
By symmetry, the subgraph induced by E₀ has four cases as shown in Figures 6A–D. Then, the graph G which contains the subgraphs shown in Figure 6B contradicts $F\left(D_i\right)\cap F\left(D_j\right)=\varnothing \left(i\ne j,i,j=\mathrm{1,2,3,4}\right)$. If G contains the subgraph shown in Figure 6C, then the three edges e₁, e₂, and e₃ belong to the same triangular face as every 3-length cycle of a {(3, 4), 4}-fullerene must be the boundary of a triangular face by Lemma 2.2, which contradicts that $F\left(D_i\right)\cap F\left(D_j\right)=\varnothing \left(i\ne j,i,j=\mathrm{1,2,3,4}\right)$.

If $G\left[E_0\right]$ is isomorphic to the graph as shown in Figure 6A, then the three edges e₁, e₂, and e₃ belong to three diamonds D₁, D₂, and D₃, respectively, and we have the subgraph A₁ consisting of D₁, D₂, and D₃ (see Figure 6E) such that $\left|\nabla \left(A_1\right)\right|=2$ and A₁, D₄ are disjoint. By the definition of G, we can know the two 3-degree vertices on $\partial \left(A_1\right)$ must be adjacent and we obtain G ≅ Q₀, which contradicts that A₁, D₄ are disjoint.

If $G\left[E_0\right]$ is isomorphic to the graph as shown in Figure 6D, then D₁, D₂ are adjacent, and D₃, D₄ are adjacent. Set B₁ = D₁ ∪ D₂, B₂ = D₃ ∪ D₄. Since the two edges e₁, e₂ are disjoint, the edges e₃, e₄, B₁, B₂ are disjoint. Then, $\nabla \left(B_i\right)\left(i=\mathrm{1,2}\right)$ forms a cyclical 4-edge-cut (see Figure 6F), by Lemma 2.3, $G\cong Q_l\left(l\ge 1\right)$.

Since $G_0=\left(X_0,Y_0\right)$ is bipartite, it should be noted that each edge e_i of E₀ is in these eight triangles and connects two vertices in Y₀; thus, the edges of $E\left(G\right)-E\left(B_1\right)-E\left(B_2\right)$ are X₀Y₀ − edges and G − B₁ − B₂ has only quadrangles (see Figure 6G). Moreover, by Lemma 2.3, we can know G − B₁ − B₂ consists of $l-2\left(l\ge 2\right)$ layers of quadrangles (each layer is made up of four quadrangles). Thus, we have $G\in {\mathcal{H}}_1$.

Subcase 2.2: $G\left[E_0\right]$ has exactly three components.

Then, both of the two components of $G\left[E_0\right]$ are K₂, and one component is K_1,2 (see Figure 7A). Without loss of generality, we suppose the component K_1,2 is induced by the edges e₃, e₄. Then, the two diamonds D₃, D₄ are adjacent, and D₁, D₂ are disjoint. Set C₁ = D₃ ∪ D₄ (see Figure 7C).

Then, due to Lemma 2.3 and Lemma 3.1, $\nabla \left(C_1\right)$ forms a cyclical 4-edge-cut, thus, G≅Q_s, where Q_s is the tubular {(3, 4), 4}-fullerene as shown in Figure 2, which means each of the two caps of Q_s must contain two adjacent diamonds, contradicts that D₁, D₂ are disjoint.

Subcase 2.3: $G\left[E_0\right]$ has four components.

Then, the four diagonal edges e₁, e₂, e₃, and e₄ are disjoint (see Figure 7B), that is, the four diamonds D₁, D₂, D₃, and D₄ cannot intersect at the diagonal edges. We have the four diamonds D₁, D₂, D₃, and D₄ as shown in Figure 7D. Then, $G\in {\mathcal{H}}_2$.

So far, we have completed the proof of Theorem 3.3.

Inspired by Theorem 3.3, we immediately get the following theorems.

FIGURE 5

FIGURE 5. $G\left[E_0\right]$ has one component and the {(3,4),4}-fullerene Q₀ (A–F).

FIGURE 6

FIGURE 6. $G\left[E_0\right]$ has two components and the {(3,4),4}-fullerenes $Q_l\left(l\ge 1\right)$ (A–G).

FIGURE 7

FIGURE 7. $G\left[E_0\right]$ has three components (A,C) or four components (B,D).

Theorem 3.4. Let G be a {(3, 4), 4}-fullerene, if $G\in {\mathcal{H}}_1$, then $ak\left(G\right)=4$.

Proof. Let $G\in {\mathcal{H}}_1$, that is, $G\cong Q_n\left(n\ge 0\right)$. By Theorem 3.2 and the definition of the anti-Kekulé number, we only need to find an anti-Kekulé set E₀ of G such that $\left|E_0\right|=4$.

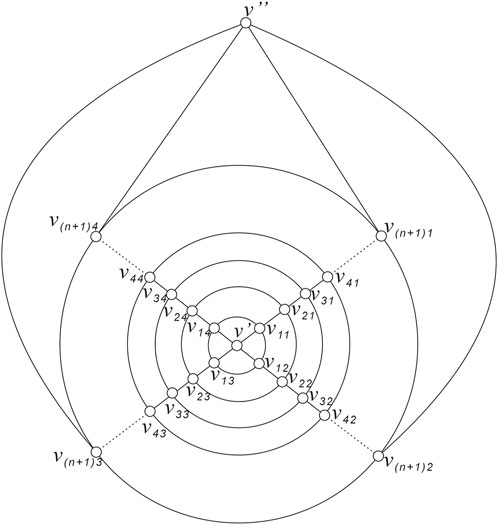
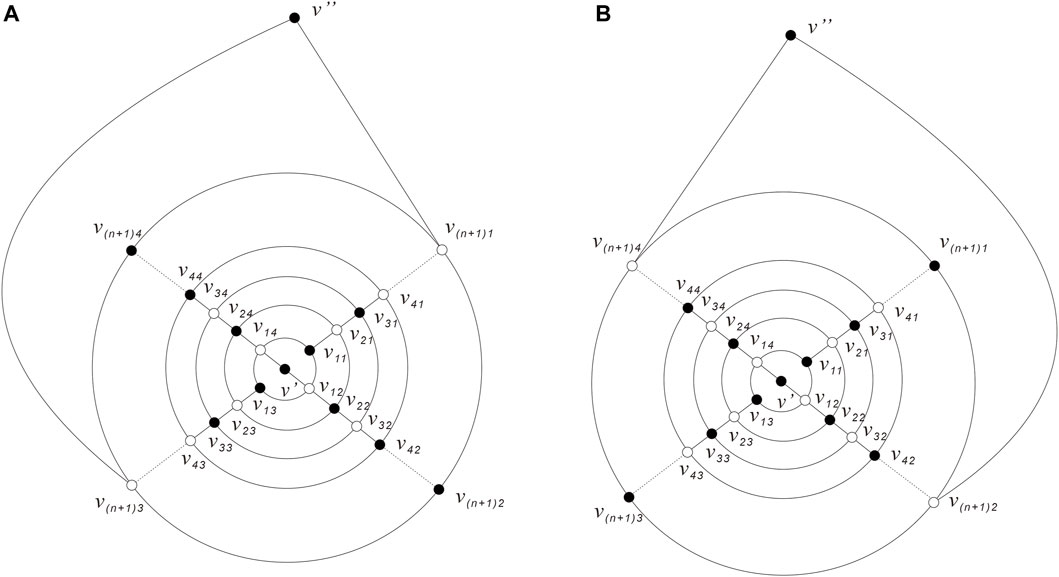
For convenience, let the plane embedding graph of Q_n as shown in Figure 8. Q_n consist of n + 1 concentric rings with four vertices on each ring and two vertices on two caps; these n + 1 concentric rings are recorded as R₁, R₂, R₃, …, R_n+1 from the inside to the outside. Next, the vertices of Q_n are labeled as follows: the vertices shared by the four triangles on the two caps are represented by v′, v^″, respectively, and the vertices on $R_i\left(i=\mathrm{1,2,3},\dots ,n+1\right)$ are recorded as v_i1, v_i2, v_i3, and v_i4 (i = 1, 2, 3, …, n + 1) in a clockwise direction such that v_i1, v_i3 (v_i2,, and v_i4) are on the same line (see Figure 8 the labeling of Q_n).

Next, we will prove Theorem 3.4 in two cases.

Case 1: n is an odd number.

Let $E_0=\left\{v^{\prime }{v}_{11},v^{\prime }{v}_{13},v^{\prime \prime }{v}_{n+\mathrm{1,2}},v^{\prime \prime }{v}_{n+\mathrm{1,4}}\right\}$ (see Figure 9A), and set G₁ = G − E₀. Then, E₀ is not a cyclically 4-edge-cut of G by Lemma 2.3. Moreover, E₀ is not a trivial 4-edge-cut as the four edges in E₀ are not incident with a common vertex. That is, G₁ is connected.

Then, we prove that G₁ = G − E₀ has no perfect matching, and there are only quadrangular faces in G₁, so, G₁ is bipartite. We color the vertices of G₁ with black and white such that adjacent vertices in G₁ are assigned two distinct colors (see Figure 9A). Let M₀ denote the set of white vertices and N₀ denote the set of black vertices, then $G_1=G_1\left(M_0,N_0\right)$, $\left|M_0\right|=2n+2$, $\left|N_0\right|=2n+4$. In accordance with Theorem 2.4, there exist $M_0\subseteq V\left(G_1\right)$ such that $o\left(G_1-M_0\right)=\left|N_0\right|=2n+4>\left|M_0\right|=2n+2$, so G₁ has no perfect matching.

Case 2: n is an even number.

Let $E_0=\left\{v^{\prime }{v}_{11},v^{\prime }{v}_{13},v^{\prime \prime }{v}_{n+\mathrm{1,1}},v^{\prime \prime }{v}_{n+\mathrm{1,3}}\right\}$ (see Figure 9B), and set G₂ = G − E₀. Also, G₂ is connected.

There are only quadrangular faces in G₂; so, G₂ is also bipartite with one bipartition 2n + 2 vertices and the other bipartition 2n + 4 vertices, which means G₂ has no perfect matching.

Therefore, we find the anti-Kekulé set E₀ of G with $\left|E_0\right|=4$, which means $ak\left(G\right)=4$, if $G\in {\mathcal{H}}_1$.

Due to Theorem 3.4, if $G\in {\mathcal{H}}_1$, then $ak\left(G\right)=4$. However, the anti-Kekulé number of G can be 4 or 5 if $G\in {\mathcal{H}}_2$. Next, we use a method to judge whether the anti-Kekulé number of G can be 4 or 5 when $G\in {\mathcal{H}}_2$. Before we give some definitions of G if $G\in {\mathcal{H}}_2$. Let $G\in {\mathcal{H}}_2$, the four diamonds of G be D₁, D₂, D₃, and D₄ and the four diagonal edges be e₁, e₂, e₃, and e₄ such that $e_i\in E\left(D_i\right)$, i = 1, 2, 3, 4. Set $E_0=\left\{e_1,e_2,e_3,e_4\right\}$ and e₁ = v₁v₂, e₂ = v₃v₄, e₃ = v₅v₆, and e₄ = v₇v₈. The eight vertices of the four diagonal edges are called eight stars, and their union is denoted by $V_0=\bigcup _{i=1}^8{v}_i$.

Set G₀ = G − E₀. Then, G₀ is bipartite, without loss of generality, we supposed the bipartitions of G₀ were V₁, V₂. Then, by the proof of Theorem 3.3, we can know if $ak\left(G\right)=4$, then V₀ ⊂ V₁ or V₀ ⊂ V₂, which means $ak\left(G\right)=5$ when V₀⊄V₁ and V₀⊄V₂. Thus, we have the following theorem.

FIGURE 8

FIGURE 8. {(3,4),4}-Fullerenes Q_n.

FIGURE 9

FIGURE 9. Graph G − E₀; n is an odd number (A), and n is an even number (B).

Theorem 3.5. Let G be a {(3, 4), 4}-fullerene, $G\in {\mathcal{H}}_2$, if V₀ ⊂ V₁ or V₀ ⊂ V₂, then $ak\left(G\right)=4$, otherwise, $ak\left(G\right)=5$.

Proof. By Theorem 3.2, we only need to show if V₀ ⊂ V₁ or V₀ ⊂ V₂, then $ak\left(G\right)=4$. Without loss of generality, suppose V₀ ⊂ V₁. Then, $G\left[V_1\right]$ consists of the four edges e₁, e₂, e₃, and e₄ and some singleton vertices. Since the four edges e₁, e₂, e₃, and e₄ cannot be incident with a common vertex, E₀ is not a trivial 4-edge-cut. However, E₀ also cannot be a cyclical 4-edge-cut by Lemma 2.3, as e_i belongs to the intersection of two triangular faces for i = 1, 2, 3, 4. Thus, G₀ = G − E₀ is connected.

On the other hand, by the degree-sum formula $4\left|V_2\right|=4\left|V_1\right|-8$, which means $\left|V_1\right|\ne \left|V_2\right|$. Thus, G₀ cannot have perfect matchings by Theorem 2.4. So, we find the anti-Kekulé set E₀ with $\left|E_0\right|=4$. Immediately, we have $ak\left(G\right)=4$. Otherwise, by Theorem 3.2, $ak\left(G\right)=5$.

By Theorem 3.5, for a {(3, 4), 4}-fullerene G with $G\in {\mathcal{H}}_2$, we can give the method to judge the anti-Kekulé number of graph G is 4 or 5 as follows:

Step 1: Delete the four diagonal edges e₁, e₂, e₃, and e₄.

Step 2: Color the vertices of $G_0=G-\left\{e_1,e_2,e_3,e_4\right\}$ with black and white.

Step 3: If we find the eight stars are in the same color, then $ak\left(G\right)=4$, otherwise, $ak\left(G\right)=5$.

4 Conclusion

In this paper, we have obtained the scope of the anti-Kekulé number of {(3, 4), 4}-fullerenes in Theorem 3.2; at the same time, we characterized {(3, 4), 4}-fullerenes with anti-Kekulé number 4 in Theorem 3.3, which includes two kinds of graphs ${\mathcal{H}}_1,{\mathcal{H}}_2$.

As a consequence, we proved that if $G\in {\mathcal{H}}_1$, then $ak\left(G\right)=4$. Interestingly, by the proof of Theorem 3.3, we found the {(3, 4), 4}-fullerene G belongs to ${\mathcal{H}}_2$, but the anti-Kekulé number of G is not always 4; therefore, at the end of this paper, we gave a condition for judging whether the anti-Kekulé number of graph G is 4 or 5.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.

Author contributions

RY performed the ideas and the formulation of overarching research goals and aims. HJ wrote the first manuscript draft and performed the review and revision of the first draft.

Funding

This work was supported by the National Natural Science Foundation of China (grant nos. 11801148 and 11626089) and the Foundation for the Doctor of Henan Polytechnic University (grant no. B2014-060).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Deza, M., and Sikirić, M. (2012). Spheric analogs of fullerenes. Ecole Normale Superieure, Pairs, Rudjer Boskovic Inst. 16.

Google Scholar

Kutnar, K., Sedlar, J., and Vukičević, D. (2009). On the anti-Kekulé number of leapfrog fullerenes. J. Math. Chem. 45, 431–441. doi:10.1007/s10910-008-9416-1

CrossRef Full Text | Google Scholar

Li, Q., Shiu, W. C., Sun, P. K., and Ye, D. (2019). On the anti-Kekulé problem of cubic graphs. Art Discrete Appl. Math. 2, 1–14. doi:10.26493/2590-9770.1264.94b

CrossRef Full Text | Google Scholar

Lovász, L., and Plummer, M. D. (2009). Matching theory. Providence, RI, USA: American Mathematical Society.

Google Scholar

Veljan, D., and Vukičević, D. (2008). The anti-Kekulé number of the infinte triangular, rectangular, and hexagonal grids. Glas. Mat. 43, 243–252. doi:10.3336/gm.43.2.02

CrossRef Full Text | Google Scholar

Vukičević, D., and Trinajstić, N. (2007). On the anti-forcing number of benzenoids. J. Math. Chem. 42, 575–583. doi:10.1007/s10910-006-9133-6

CrossRef Full Text | Google Scholar

Vukičević, D. (2007). Anti-Kekulé number of C₆₀. Australas. J. Comb. 38, 47–62.

Google Scholar

Yang, Q., Ye, D., Zhang, H., and Lin, Y. (2012). On the anti-Kekulé number of fullerenes. Match Commun. Math. Comput. Chem. 67, 281–288.

Google Scholar

Yang, R., Liu, C., and Wu, S. (2023). The facial resonance of {(3, 4), 4}-fullerene. J. Comb. Math. Comb. Comput. Accepted.

Google Scholar

Yang, R., and Zhang, H. (2012). Hexagonal resonance of (3,6)-fullerenes. J. Math. Chem. 50 (1), 261–273. doi:10.1007/s10910-011-9910-8

CrossRef Full Text | Google Scholar

Zhang, Q., Bian, H., and Vumar, E. (2011). On the anti-Kekulé and anti-forcing number of cata-condensed phenylenes. Match Commun. Math. Comput. Chem. 65 (3), 799–806.

Google Scholar

Zhao, L., and Zhang, H. (2020). On the anti-Kekulé number of (4,5,6)-fullerenes. Discrete Appl. Math. 283, 577–589. doi:10.1016/j.dam.2020.02.006

CrossRef Full Text | Google Scholar