
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Appl. Math. Stat. , 02 October 2024
Sec. Mathematical Physics
Volume 10 - 2024 | https://doi.org/10.3389/fams.2024.1472401
This article is part of the Research Topic Quasi-Normal Modes, Non-Selfadjoint Operators and Pseudospectrum: an Interdisciplinary Approach View all 12 articles
 C. M. Warnick1,2*
C. M. Warnick1,2*We consider how the quasinormal spectrum for the conformal wave operator on the static patch of de Sitter changes in response to the addition of a small potential. Since the quasinormal modes and co-modes are explicitly known, we are able to give explicit formulae for the instantaneous rate of change of each frequency in terms of the perturbing potential. We verify these exact computations numerically using a novel technique extending the spectral hyperboloidal approach of Jaramillo et al. (2021). We propose a definition for a family of pseudospectra that we show capture the instability properties of the quasinormal frequencies.
For asymptotically de Sitter and anti-de Sitter black hole spacetimes, the problem of defining the quasinormal frequencies has been satisfactorily resolved based on making use of a hyperboloidal foliation of the spacetime [1, 2].1 For asymptotically flat black hole spacetimes, the situation is not as fully developed, but nevertheless in many cases a suitably robust mathematical definition exists either through casting the problem in terms of scattering resonances and making use of the method of complex scaling [3, 5] or through using a hyperboloidal slicing [4, 6, 7]. In all cases, the quasinormal frequencies can ultimately be understood as eigenvalues of some operator which is not self-adjoint.
A feature of operators which are not self-adjoint is that their spectra can be unstable to “small” perturbations. For a simple example in finite dimensions, consider the matrices
Clearly for 0 < ϵ ≪1, A′−A is “small” by any reasonable notion of smallness; however, A has a repeated eigenvalue at 0, while A′ has eigenvalues ±ϵ−1, so the spectra diverge as ϵ → 0.
In the context of black hole quasinormal spectra, it was noticed already by Aguirregabiria–Vishveshwara Nollert–Price in the 90's [8–11] that seemingly innocuous changes to the operators used in defining quasinormal modes could have dramatic effects on the spectrum. Motivated in part by mathematical [2, 4, 6, 12] and numerical [13–15] studies which cast the problem of finding the quasinormal spectrum as an eigenvalue problem for the time evolution operator on a hyperboloidal foliation, there has been a resurgence of interest in the problem of quasinormal spectral stability, see [16, 17] in the specific context of instability arising from non-self-adjoint operators as well as [18–23] and references therein for many other studies.
In this short article, we shall consider the problem of the conformal wave equation on the static patch of de Sitter space. The high degree of symmetry enjoyed by the de Sitter spacetime means that the problem of determining the quasinormal spectrum is completely solvable, and the various objects involved can be computed explicitly. This makes this a helpful test-bed for understanding the effects on the spectrum of small perturbations. The perturbations we consider consist of stationary modifications to the potential. In a more physically motivated situation, we should consider the linearized gravitational field (rather than a conformal scalar field) and permit perturbations to the geometry of the background rather than just a potential. Our methods can, in principle, be applied in this situation, but for simplicity, we focus on the toy model.2
We are able to compute exactly the first order correction to each quasinormal frequency in terms of the perturbing potential. We find that the answer to the question of whether an individual quasinormal frequency is stable to “small” perturbations depends sensitively on what is meant by “small” [cf [25, footnote 18]]. In particular, the relevant notion of smallness varies depending on which modes we are considering, and those representing more rapidly decaying modes require a more stringent notion of smallness. One may alternatively view this by first fixing the notion of smallness considered and then one observes that the more rapidly decaying modes are more unstable to small perturbations, consistent with expectations going back to [9].3
To confirm the analytic computations, we also perform some numerics. For this, we make use of a spectral method on a hyperboloidal (or null) slicing, similar to that used in [16], but applied to an enlarged system obtained by differentiating the equation by hand k-times motivated by the analysis of [2]. This has a doubly beneficial effect—First, it stabilizes the numerical computation of quasinormal frequencies; second, it permits us to stably compute a family of pseudospectra that we define, associated with the problem, which allow the stability properties to be directly visualized. In this context, we should also mention the forthcoming study [26] which also provides a numerically stable computation of pseudospectra.
We consider the static patch of the de Sitter spacetime, written in coordinates that are regular at the future horizon. This is a metric on ℝ4 = {(t, x):t ∈ ℝ, x ∈ ℝ3}
with δij the usual Kronecker delta and κ > 0 a constant. The static patch is the region where B = {x ∈ ℝ3:|x| < κ−1} is the ball of radius κ−1, and the future cosmological horizon is . This metric is Einstein with cosmological constant Λ = 3κ2. We will keep track of κ for later discussion, but nothing is lost by setting κ = 1 throughout.
The wave operator in these coordinates takes the form
We shall consider the following family of equations on this background
Here, Vh is a time-independent potential depending on some small parameter |h| < ϵ, and we assume that the map (h, x) ↦ Vh(x) is smooth on (−ϵ, ϵ) × ℝ3. We are interested in particular in the quasinormal ring-down behavior of solutions to this equation. To discuss this, we introduce the Laplace transformed operator which acts on functions u:B → ℂ
We define the quasinormal frequencies through the solvability properties of this operator. More precisely, for k = 0, 1, 2, … we define an inner product and norm on functions u, w:B → ℂ by
Here, ∇(l)u is the rank l-tensor ∇i1⋯∇ilu, and · means contraction on all indices.4 Notice that (u, w)0 is the usual L2−inner product. We define Hk, the Sobolev space of order k, to consist of those functions u:B → ℂ with ||u||k < ∞. This is a Hilbert space with the corresponding inner product. We define the domain of Ls to be
It can be shown that Hk+2 ⊂ Dk ⊂ Hk+1, so that for all s, h.
With this definition in hand, we can state the basic theorem we shall require, which follows straightforwardly from Vasy, Warnick, and Hintz and Xie [1, 2, 27, 28]:
Theorem 1. Fix |h| < ϵ, k ∈ ℕ and let . Then, the operator is invertible for s ∈ Uk, except at a discrete set Λk(h) ⊂ Uk. Moreover, for each σ ∈ Λk(h) there is an integer d>0 such that:
1. There exists a d-dimensional space of smooth functions w:B → ℂ which extend smoothly to ∂B and satisfy .
2. There exists a d-dimensional space of distributions X ∈ ′(ℝ3) which satisfy
for some c > 0 and all test functions .
3. As s varies, the meromorphic family of operators has a pole at σ.
It follows from the characterization of points in Λk(h) that Λk+1(h) ∩ Uk = Λk(h). We call any σ ∈ Λk(h) for some k a quasinormal frequency of L(h), with geometric multiplicity d. A corresponding smooth solution to is a quasinormal mode, and a distribution X satisfying ii) above we call a co-mode. Notice that the condition on X implies that X is supported in and so can be uniquely extended to act on test functions in .
The residue of at s = σ is a finite rank operator, and we identify the rank of this residue with the algebraic multiplicity of σ. As in the familiar case of matrices, the algebraic multiplicity is an upper bound for the geometric multiplicity. We say that a quasinormal frequency σ ∈ Λk(h) is simple if it has algebraic multiplicity one.
The result above holds for h fixed. The question we shall consider in this study, that of quasinormal spectral instability, amounts to trying to understand how the set Λk(h) changes as h varies.
Let us suppose that for the unperturbed operator, that is, at h = 0, we can compute the quasinormal frequencies, modes, and co-modes, and we consider some simple σ ∈ Λk(0) with corresponding quasinormal mode w and co-mode X. It was shown in Joykutty [29] that that as h varies, there is some smooth curve of quasinormal frequencies σ(h) ∈ Λk(h), with σ(0) = σ, together with an associated curve of quasinormal modes w(h) with w(0) = w, depending smoothly on h such that
holds for all |h| < ϵ. Moreover, in Joykutty [29] an explicit power series expansion for σ(h) is given in terms of the trace of certain operator valued contour integrals. We shall take a more elementary approach to find a formula for σ′(0).
Since depends smoothly on its arguments, we can differentiate with respect to h at h = 0 to find:
By assumption, we know and w, but we do not know anything about w′(0). If, however, we act on Equation 6 with the co-mode X, the term involving w′(0) will be annihilated. We find then:
or, rearranging
This formula gives us an exact expression for the velocity of the curve of quasinormal frequencies σ(h) as it passes through σ.
We observe that only the numerator of Equation 7 depends on the perturbation—the denominator can be computed from the unperturbed operator alone. Recalling that the operator norm of a linear map A:V→W between normed spaces is given by
we can estimate σ′(0) in terms of an operator norm of the linearized perturbation as
Here, the sensitivity, or condition number, γσ depends only on the unperturbed operator and is given by
where . We can think of the expression for γ as a generalization of the formula for the sensitivity of a matrix eigenvalue.
At this stage, it is worth commenting on the role of k in the discussion. Increasing k increases the region of the complex plane in which we can study the quasinormal frequencies; however, the price we pay for this in Equation 8 is an increase in the control that we require on the perturbation. We can mitigate this by choosing k to be as small as possible, consistent with σ ∈ Λk(0). Even doing this we see that to bound the rate of change of a quasinormal frequency σ, we (roughly speaking) need control of more than −κ−1(Reσ) derivatives of the perturbation. We shall see this more explicitly later on. We should note that ‘control of higher derivatives' may appear to be an unphysical condition, but one can also view this condition as asking that the perturbations should not have too much of their energy at high wavenumbers.5
The arguments above do not rely strongly on the particular form of the metric, or the family of operators we consider. As long as a result broadly analogous to the conclusions of Theorem 1 holds, we can expect to be able to repeat this argument.
In the discussion above, we made the assumption that the quasinormal frequency σ was simple, which was needed to establish that σ sits on a smooth curve of quasinormal frequencies. If σ is not simple, then this need not be the case in general—see Figure 3 for a situation where this arises in our numerics. It does follow from [29] that the number of QNFs, counted with suitable multiplicity, inside a small circle around σ is independent of h for small h, so that QNFs in particular cannot be locally “created” or “destroyed” by small perturbations of the type we consider—QNFs can only appear from infinity or by splitting off from a QNF with algebraic multiplicity greater than one.
In general, it does not appear to be a straightforward question to determine whether a particular non-simple quasinormal frequency lies on a smooth curve. In some cases, however, it may be that evolution under L(h) leaves invariant some subspace (such as an angular momentum eigenspace) so we can consider the problem of finding quasinormal frequencies restricted to this subspace. If σ is a simple quasinormal frequency of the restricted problem, then the results of the previous section will apply.
In Jaramillo et al. [16] and subsequently [see Boyanov et al. [23] and references therein], the instability of the quasinormal spectrum has been investigated using the notion of pseudospectrum, comparing the results from this approach to computations with explicit perturbations. Recall that for a matrix A we can define the ϵ−pseudospectrum to be6
where we define whenever A−sι is not invertible. It can be shown [30–32] that Λϵ corresponds to the set of points which can appear in the spectrum of A + δA, where δA is a perturbation satisfying .
This notion generalizes to operators on infinite dimensional spaces in the obvious way. However, this definition cannot immediately be applied to our problem above because is not of the form A−sI for some operator A. There are two possible approaches to resolve this. The approach taken by Jaramillo et al. [16] is to follow [2, 13, 14] and recast the problem of finding the quasinormal frequencies as a genuine eigenvalue problem by writing
where Lj(h) is a differential operator of order j. Then, we can verify that has a solution if and only if
has a smooth solution. Thus, the set Λk(h) can be identified with the part of the spectrum of
in Uk, where is thought of as a closed unbounded operator on . This motivates one definition of the ϵ−pseudospectrum7 as
This has the advantage of being the standard definition applied to , but the disadvantage that in numerical computations one has to double the dimension of the approximation space to account for the two functions w, v. Moreover, since does not have compact resolvent, approximation by matrices can be more challenging.
An alternative approach is to generalize the notion of ϵ−pseudospectrum by declaring
This has the advantage that is compact, but the disadvantage that since it is not the standard definition of pseudospectrum, one cannot readily make use of existing numerical libraries. We note as an aside that we could also consider the Hk → Dk norm in place of the Hk → Hk norm in Equation 9, but it will not make a significant difference for the type of perturbations we consider.
We shall take Equation 9 as our definition of the pseudospectrum for the rest of the study [see Besson et al. [26] for an alternative approach]. A modification of the usual arguments for pseudospectra [31, 32] shows that is precisely the set of points in Uk which can occur as quasinormal frequencies of L(s, 0)+E for some operator E:Hk → Hk satisfying . One can verify that the fact that s does not appear linearly in L(s, 0) does not affect this argument. In particular, provided we have .
We note that this definition agrees with that for the null slicing in Cownden et al. and Boyanov et al. [22, 23]; however, we do not assume that the slicing is everywhere null.
To give a concrete demonstration of the ideas above, we will work in a setting where the quasinormal frequencies, modes, and co-modes of the operator are known explicitly at h = 0. In particular, from now on we assume that we perturb about the conformal wave operator on de Sitter, in our language:
Under this assumption, we have [27, 28]:
Lemma 2. Suppose V0(x) = 2. Then:
1. Λk(0) = {−κ, −2κ, −3κ, …, −kκ}.
2. The quasinormal frequency σn: = −nκ ∈ Λk(0) has geometric and algebraic multiplicity n2, and a basis for the corresponding space of quasinormal frequencies is given in terms of the standard spherical polar coordinates (r, θ, ϕ) on Bκ by
Here, Yl, m are the spherical harmonics, 2F1 is the hypergeometric function and the integers m, l satisfy |m| ≤ l ≤ n.
3. For each σn ∈ Λk(0), the corresponding quasinormal co-modes are supported on ∂B. A basis for the space of co-modes is given in terms of the action on a smooth test function by
where |m| ≤ l ≤ n, are constants, and φl, m(r) is the projection of φ onto the (l, m)−spherical mode.
While it is possible to specify the constants explicitly, see Hintz and Xie; Joykutty [27, 28, 33], for the purposes of our results below it is more computationally efficient to find Xn, l, m for any particular choice of n, l, m by simply using Equation 10 as an ansatz in Equation 5 and solving the resulting linear system for . Doing so using Mathematica to perform the computations, we find the results in Table 1.
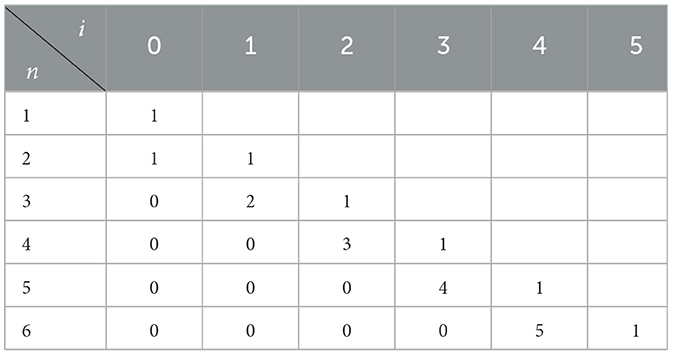
Table 1A. Coefficients for the first six quasinormal frequencies with in each of the angular sectors l = 0, 1, 2. (A) l = 0.
Since for σ ≠ −κ the quasinormal frequencies are not simple, to make use of Equation 7 to estimate the change in the QNF, we shall make the additional assumption that the potential Vh is spherically symmetric. Under this assumption, the QNFs are simple once we restrict our attention to a single fixed angular mode. If we fix l, m with |m| ≤ l, then for k ≥ l, the unperturbed quasinormal spectrum restricted to the l, m angular mode is and all the QNFs are simple. We can compute the rate of change of the QNF at −κ n by
To use this formula, we also need and . For the particular case of interest, with L(h) given by Equation 2, we have
so that
and
where we introduce , the first order perturbation to the potential.
We now have all that is required to compute . In view of the structure of the operator Xn, l, m, we can write
for some constants . Note that this is independent of m due to the spherical symmetry of the perturbing potential. We can again use Mathematica to compute these constants and present the results for the first few modes in the l = 0, 1, 2 angular sectors in Table 2.
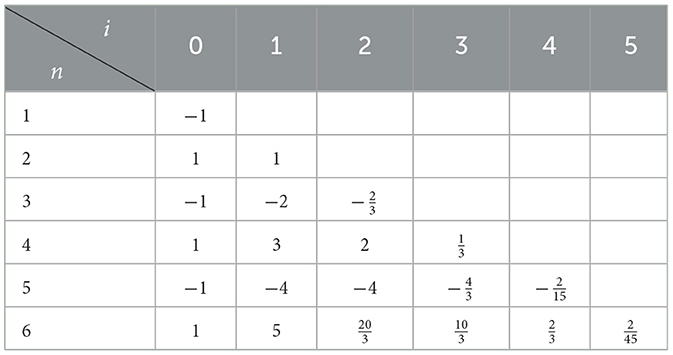
Table 2A. Coefficients for the first six quasinormal frequencies within each of the angular sectors l = 0, 1, 2. (A) l = 0.
Picking two cases as examples, we can read off from the tables that
We see very explicitly here and from Table 8 that the rate of change of the quasinormal frequency depends on higher derivatives of the perturbing potential, and the larger n, that is, the deeper into the stable plane we go, the more derivatives that are required. Equivalently, the deeper into the stable plane, the more control we require on the high wavenumber component of our perturbation. The increasing order of the operator norm that appears on the right-hand side of Equation 8 as we probe deeper into the plane is not simply an artifact of our framework but is necessary.
To see why it is necessary to use higher order norms to constrain the perturbations, let us consider the case κ = 1 and consider a family of perturbations . We clearly have that
so in particular as ϵ → 0, we see that in the ‘energy norm', that is, the operator norm associated to the H1 norm we have that the perturbation tends to zero. However, W″(1) = (4ϵ−1 − 2ϵ)exp(−1/ϵ2) ~ ϵ−1 as ϵ → 0, so that using the expression above we see that the l = m = 1, n = 3 mode is displaced (to first order) by a term proportional to ϵ−1. Hence, smallness of the perturbation in the energy norm is no guarantee of stability of the quasinormal modes lying sufficiently deep in the stable half-plane.
For the choice of potential , with κ = 1, which we study numerically below, we have computed for n ≤ 20, l ≤ 2 and presented the results graphically in Figure 1. Noting the logarithmic scale on the y−axis, we see that for this choice of perturbing potential grows roughly exponentially in n, consistent with our expectation that modes deeper in the stable plane become more and more unstable.
To test numerically the computations above, we have computed the quasinormal frequencies for the choice . We first present the results and then comment on the methods used below.
Since the equation is real, as is the quasinormal spectrum of , frequencies can only move off the real axis in complex conjugate pairs. Restricted to each angular sector the QNFs are simple, so each QNF must remain real for a range of h values near 0. Accordingly, in Figure 2 we show the directly computed real part of the quasinormal frequencies as a function of h. Superposed on this, we also plot the linear approximation to the QNFs given by
with computed using the exact methods of §5 and we see very good agreement with the full numerical computation. We have experimented and this result is robust to changes to the potential, provided it remains smooth. We have thus verified the results of §5. We note that this is a non-trivial test of our numerical scheme (described below) as it correctly predicts the values of the QNFs for h = 0 and agrees with the analytical computations for the gradient of the blue curves at these points.
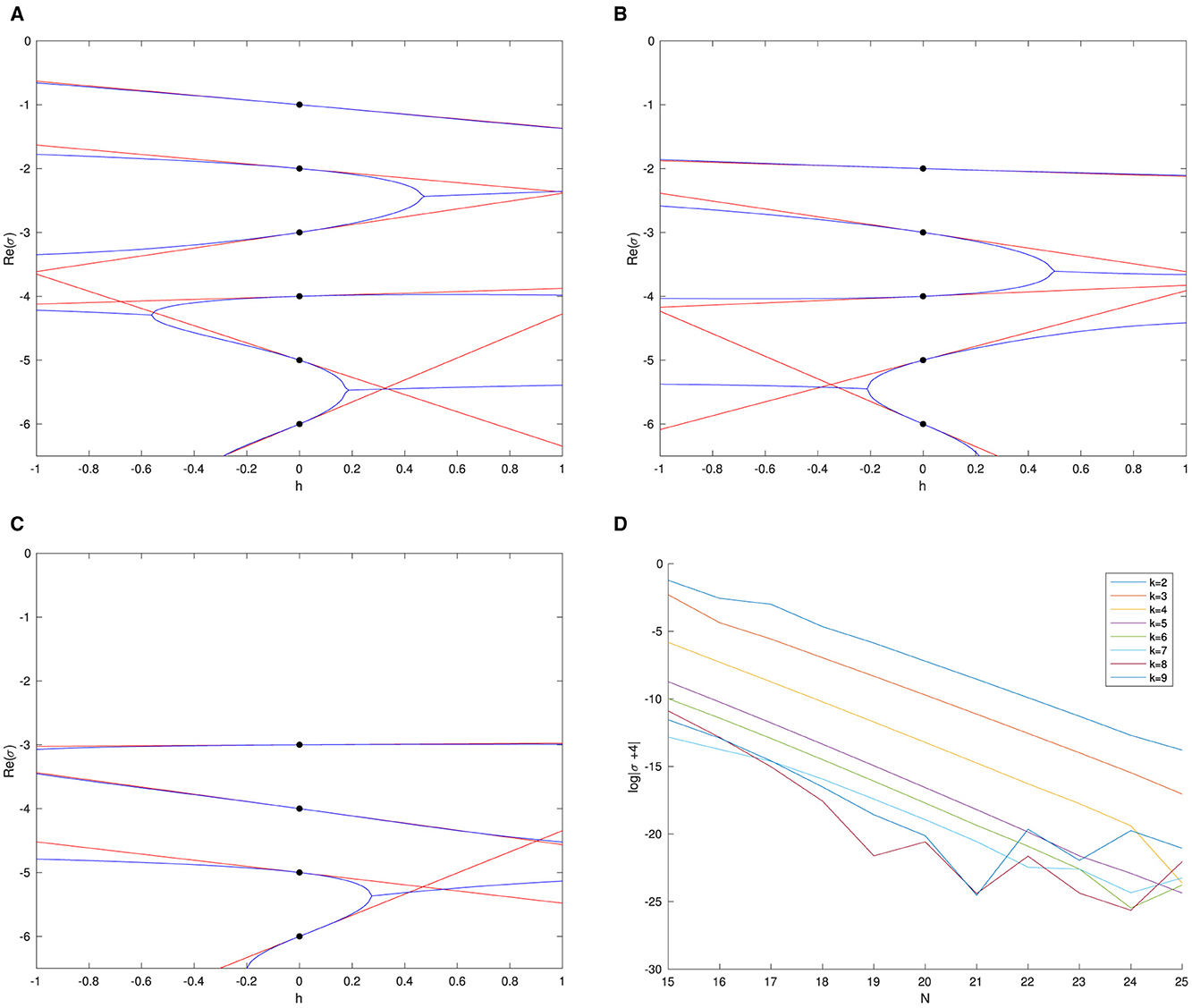
Figure 2. Re(σ(h)) plotted against h for numerically computed QNFs for (blue lines) together with the linear approximations (red lines) in the l = 0, 1, 2 sector. The black dots mark the location of the analytically known QNFs for h = 0. (A) l = 0. (B) l = 1. (C) l = 2. (D) Logarithmic error against N for k = 2, … ,9.
Figure 2 shows that pairs of QNFs do eventually meet and move off the real axis. In Figure 3, we show an example of one such interaction in the complex plane, which occurs when the quasinormal frequencies with l = 0, σ(0) = −2, −3 coalesce and move into the complex plane at h ≈ 0.4645. We note that (within the accuracy of the numerics) it appears that we cannot identify a smooth curve σ(h) of QNFs passing through the point at which the QNFs meet (and hence cease to be simple). Whichever branch we pick, the curve will have to turn through an angle of π/2 as h passes the critical value. The choices of h to plot were determined by setting hi = 0.4645+ϵi|ϵi|, and taking ϵi to be spaced uniformly in [−1, 1]. This figure was computed with a depth k = 3 and N = 25 gridpoints, see §6.2.
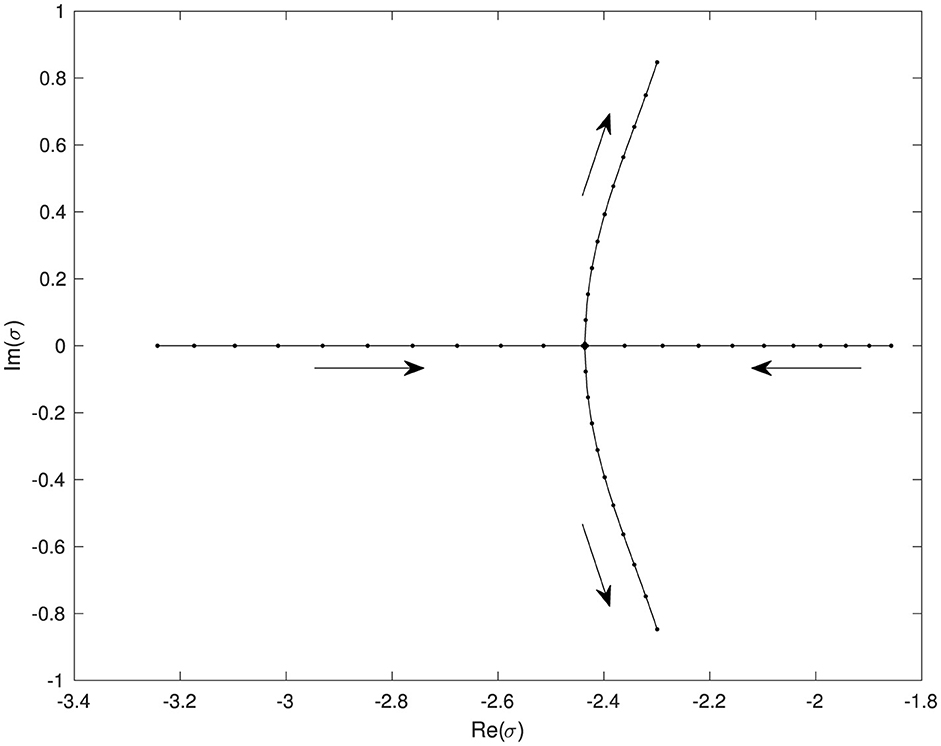
Figure 3. Numerically determined QNFs in a neighborhood of the transition point at h ≈ 0.4645, at which the two real QNFs with l = 0, σ(0) = −2, −3 meet and branch into a conjugate pair of complex QNFs. Arrows indicate the direction of increasing h.
The numerics in this section are performed using a null slicing, rather than the spacelike slicing introduced above, but the scheme can be readily adapted for a spacelike slicing. For convenience, we will take κ = 1 from now on. Setting
the metric takes the form
To find the quasinormal frequencies, we seek solutions to of the form
If we define
then
so that to find quasinormal frequencies, we are led to consider the solvability of
for given f, with f(0) = R(0) = 0 and R regular r = 1. Rather than directly discretize Equation 12, we first expand to a system of equations by differentiating the equation. We have the commutation relation
Let , , and . Then using the commutation relation, we can show that Equation 12 implies
where are numerical (indeed integer) constants determined recursively by
with for all i. Next, we set for all i, j and recursively define
with
otherwise. Finally, set , for all i ≠ 0 and
We can verify that , which we expect as a consequence of the enhanced redshift effect (see Warnick and Dafermos–Rodninski [2, 34]).
To construct our numerical scheme, we fix an integer k ≥ 0, which we call the depth of the scheme. If Equation 12 holds, then the system of equations:
will also hold. Here, we have used the fact that to arrange that we have the operator acting as the principle differential operator on all components. This is the approach taken to increase the working regularity in the analysis of Warnick [2].
We now treat R0, …, Rk as independent functions, and we discretize on the interval [0, 1] using a pseudospectral method, following Trefethen [35]. The constants α, β, γ are found recursively, and the derivatives are computed exactly using Matlab's Symbolic Math Toolbox before discretization. We note that Rp(0) = 0 which gives a Dirichlet boundary condition at one end of our interval, and we do not need a boundary condition at r = 1 as the pseudospectral discretization will impose smoothness there automatically.
After discretizing on N points, Equation 13 becomes
for (kN) × (kN)-matrices A, B, C and column vectors V, F which represent the discretization of (R0, …, Rk), (f0, …, fk), respectively. We work throughout at standard machine precision.
If σ is a quasinormal frequency, then we expect the generalized eigenvalue problem
to have an eigenvalue near σ. Thus, we can find the quasinormal frequencies by applying Matlab's generalized eigenvalue finder to Equation 14. However, by enlarging the original problem to a system, we may have introduced spurious eigenvalues which correspond to vectors V for which the condition
does not hold. To enforce this condition, we select only those eigenvalues of 14 for which (the discretized version of)
holds, where e is a sufficiently small threshold parameter, which we take to be 10−1 for the computations in this study.
Plots 2a–c show the numerically computed quasinormal spectrum in the l = 0, 1, 2 sector as h varies, computed using k = 6, N = 25. Plot 2d shows the error in the scheme when computing the eigenvalue at σ = −4 for various values of k. We see (as has been observed in other situations [16]) that for a given value of k, the pseudospectral method in fact can accurately find quasinormal frequencies even outside the domain Uk in which we expect the numerics to converge.
To compute pseudospectra for different k, we need to numerically approximate . We can approximate this by computing
where Π is the L2−orthogonal projector onto the space of vectors F of the form (f0, …, fk), where . This projection is necessary to account for the enlargement of our space by considering the system of higher derivatives. Since for such an F we have8 we can approximately compute the Hk operator norm of by the ℓ2 operator norm of the approximating matrix.
In Figure 4, we show the numerically computed pseudospectra for k = 1, …, 6. We see that in all cases the pseudospectrum is well-behaved in the region Uk, but that the contours open out significantly once we leave this region. We expect that the fact that the contour curves can leave Uk at all is a feature of the finite truncation. We observe the phenomenon noted above that the spectral method finds quasinormal frequencies accurately, even in the region of the plane that we expect significant numerical instability. For example, in Figure 4A we see the first five frequencies accurately computed, even though only the first is actually in U1.
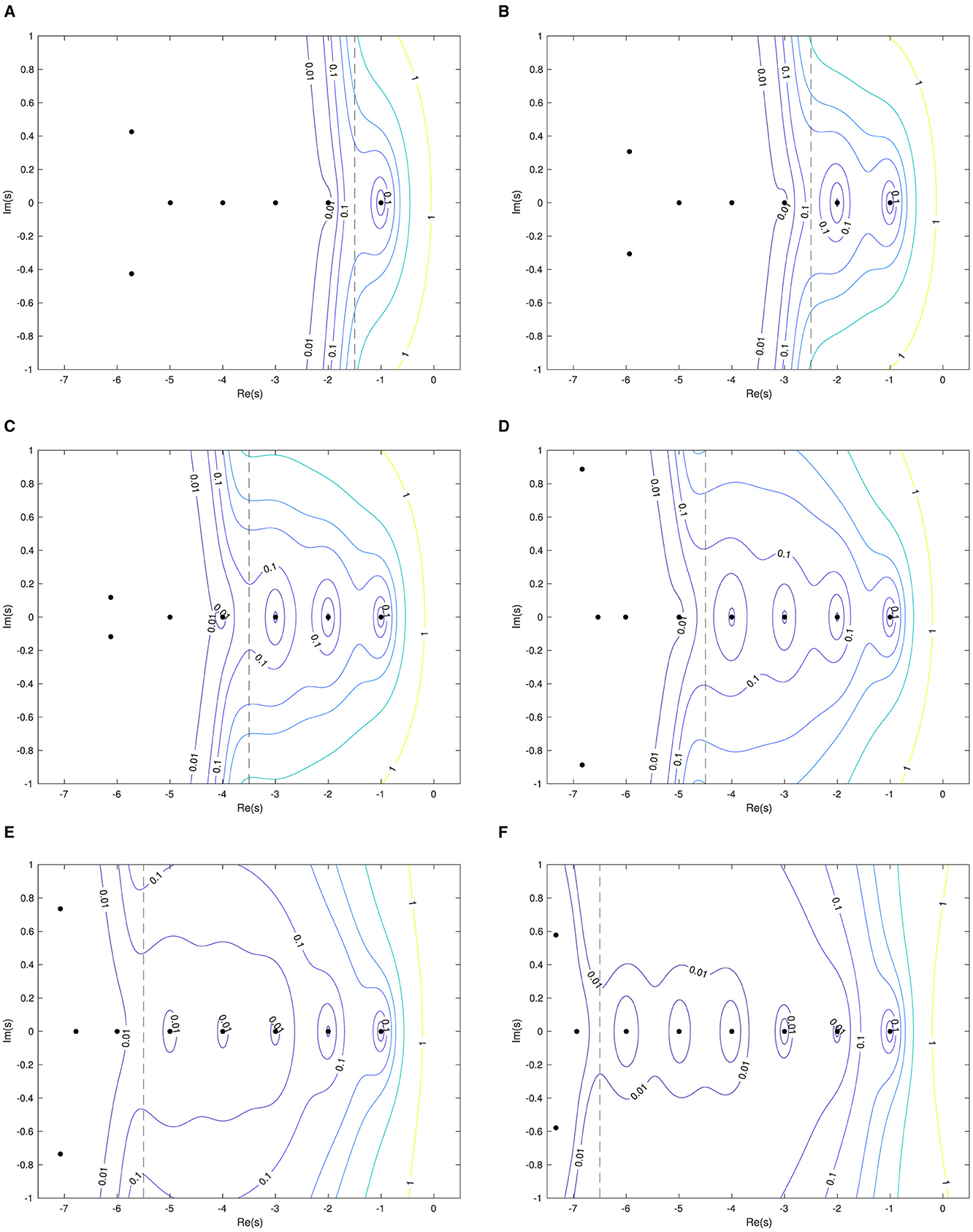
Figure 4. Numerically computed contour lines for 1 ≤ k ≤ 6. The black dashed line indicates the boundary of Uk. Black dots are the QNFs of computed by the numerical algorithm with N = 35. (A) k = 1. (B) k = 2. (C) k = 3. (D) k = 4. (E) k = 5. (F) k = 6.
To verify convergence of the numerical operator norm, in Figure 5 we show the approximated values of at s = −4 + i for k = 2 and k = 4 as N varies. As expected, in the k = 2 case we see divergence, since for this k our choice of s does not belong to Uk. For the case k = 4, we are in the region Uk, and we see good convergence. This can be compared to Figure 7 of [23]. We should mention that the pseudospectrum for this operator according to the standard definition has been computed for k = 1 in [36], which appeared slightly before this study—see their Figure 11.
We have investigated the stability of the quasinormal spectrum of the conformal wave equation on the static patch of de Sitter. We find that the quasinormal frequencies are stable, provided the perturbing potential is small in a sufficiently high regularity norm. Conversely, one could instead interpret this as a spectral instability for perturbing potentials which are not sufficiently regular at the cosmological horizon. We numerically verify our computations using a spectral method and propose a definition for a family of pseudospectra that demonstrate good convergence properties and capture the (in)stability of the quasinormal frequencies.
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
CW: Writing – original draft, Writing – review & editing.
The author(s) declare that no financial support was received for the research, authorship, and/or publication of this article.
I am grateful to Jason Joykutty and Dejan Gajic for several valuable discussions on the topic of pseudospectral instability of black holes. I am especially grateful to José Luis Jaramillo for discussions and very helpful comments on this manuscript. I am also grateful to the The Erwin Schrödinger International Institute for Mathematics and Physics in Vienna for hosting the programme “Spectral Theory and Mathematical Relativity” in Summer 2023, during which I started thinking about this problem.
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
1. ^see [3, 4] and references therein for a historical overview of this problem
2. ^A further complication can arise where the perturbations are presumed to have a time dependence with a typical timescale much shorter than the quasinormal frequencies, in which case one may hope to attempt some averaging procedure (see [24, §3.5 d]).
3. ^“...we find that the fundamental mode is, in general, insensitive to small changes in the potential, whereas the higher modes could alter drastically.”
4. ^Derivatives should be understood in the distributional sense.
5. ^Roughly speaking, for a perturbation in Hk, the fraction of the total energy carried by wavenumbers greater than μ is bounded by a constant multiple of μ−2k for large μ.
6. ^The pseudospectrum is usually defined as a closed set, with ≥ in place of >; however, the open condition generalizes more straightforwardly to the infinite dimensional case.
7. ^The pseudospectrum is a property of the unperturbed operator; hence, we set h = 0.
8. ^In fact this is the discretized version of a weighted Sobolev nor; however, since the weights only degenerate near r = 0, this is adequate for our purposes.
1. Vasy A. Microlocal analysis of asymptotically hyperbolic and Kerr-de Sitter spaces (with an appendix by Semyon Dyatlov). Inventiones Mathematicae. (2013) 194:381–513. doi: 10.48550/arXiv.1012.4391
2. Warnick CM. On quasinormal modes of asymptotically Anti-de Sitter black holes. Commun Math Phys. (2015) 333:5760. doi: 10.48550/arXiv.1306.5760
3. Dyatlov S, Zworski M. Mathematical Theory of Scattering Resonances. Graduate Studies in Mathematics. (2019). Available at: https://api.semanticscholar.org/CorpusID:216573983
4. Gajic D, Warnick C. Quasinormal modes in extremal Reissner—Nordström Spacetimes. Commun Math Phys. (2021) 385:8479. doi: 10.48550/arXiv.1910.08479
6. Gajic D, Warnick C. A model problem for quasinormal ringdown of asymptotically flat or extremal black holes. J Math Phys. (2020) 61:8481. doi: 10.48550/arXiv.1910.08481
8. Aguirregabiria JM, Vishveshwara CV. Scattering by black holes: a simulated potential approach. Phys Lett A. (1996) 210:251–4.
9. Vishveshwara CV. On the black hole trail ...: a personal journey. In: 18th Conference of the Indian Association for General Relativity and Gravitation (1996). p. 11–22.
10. Nollert HP. About the significance of quasinormal modes of black holes. Phys Rev D. (1996) 53:4397–402.
11. Nollert HP, Price RH. Quantifying excitations of quasinormal mode systems. J Math Phys. (1999) 40:980–1010.
12. Bizoń P, Chmaj T, Mach P. A toy model of hyperboloidal approach to quasinormal modes. Acta Phys Polon B. (2020) 51:1007. doi: 10.48550/arXiv.2002.01770
13. Ansorg M, Macedo RP. Spectral decomposition of black-hole perturbations on hyperboloidal slices. Phys Rev D. (2016) 93:124016. doi: 10.48550/arXiv.1604.02261
14. Panosso Macedo R, Jaramillo JL, Ansorg M. Hyperboloidal slicing approach to quasi-normal mode expansions: the Reissner-Nordström case. Phys Rev D. (2018) 98:124005. doi: 10.48550/arXiv.1809.02837
15. Panosso Macedo R. Hyperboloidal framework for the Kerr spacetime. Class Quant Grav. (2020) 37:65019. doi: 10.48550/arXiv.1910.13452
16. Jaramillo JL, Panosso Macedo R, Al Sheikh L. Pseudospectrum and black hole quasinormal mode instability. Phys Rev X. (2021) 11:31003. doi: 10.48550/arXiv.2004.06434
17. Al Sheikh L. Scattering Resonances and Pseudospectrum: Stability and Completeness Aspects in Optical and Gravitational Systems. Besançon: Université Bourgogne Franche-Comté (2022).
18. Cheung MHY, Destounis K, Macedo RP, Berti E, Cardoso V. Destabilizing the fundamental mode of black holes: the elephant and the flea. Phys Rev Lett. (2022) 128:111103. doi: 10.48550/arXiv.2111.05415
19. Sarkar S, Rahman M, Chakraborty S. Perturbing the perturbed: stability of quasinormal modes in presence of a positive cosmological constant. Phys Rev D. (2023) 108:104002. doi: 10.48550/arXiv.2304.06829
20. Areán D, Fariña DG, Landsteiner K. Pseudospectra of holographic quasinormal modes. J High Energy Phys. (2023) 2023:187. doi: 10.48550/arXiv.2307.08751
21. Destounis K, Duque F. Black-hole spectroscopy: quasinormal modes, ringdown stability and the pseudospectrum. In:Papantonopoulos E, Mavromatos N, , editors. Compact Objects in the Universe. Cham: Springer (2024). doi: 10.1007/978-3-031-55098-0_6
22. Cownden B, Pantelidou C, Zilhão M. The pseudospectra of black holes in AdS. J High Energy Phys. (2024) 2024:202. doi: 10.48550/arXiv.2312.08352
23. Boyanov V, Cardoso V, Destounis K, Jaramillo JL, Panosso Macedo R. Structural aspects of the anti-de Sitter black hole pseudospectrum. Phys Rev D. (2024) 109:64068. doi: 10.48550/arXiv.2312.11998
24. Jaramillo JL. Pseudospectrum and binary black hole merger transients. Class Quant Grav. (2022) 39:217002. doi: 10.48550/arXiv.2206.08025
25. Gasperín E, Jaramillo JL. Energy scales and black hole pseudospectra: the structural role of the scalar product. Class Quant Grav. (2022) 39:115010. doi: 10.48550/arXiv.2107.12865
26. Besson J, Boyanov V, Jaramillo JL. Black Hole Quasi-Normal Modes as Eigenvalues: Definition and Stability Problem, in Preparation.
27. Hintz P, Xie Y. Quasinormal modes and dual resonant states on de Sitter space. Phys Rev D. (2021) 104:64037. doi: 10.48550/arXiv.2104.11810
28. Hintz P, Xie Y. Quasinormal modes of small Schwarzschil—de Sitter black holes. J Math Phys. (2022) 63:2347. doi: 10.48550/arXiv.2105.02347
29. Joykutty J. Existence of zero-damped quasinormal frequencies for nearly extremal black holes. Annales Henri Poincaré. (2022) 23:4343–90. doi: 10.48550/arXiv.2112.05669
30. Trefethen LN. Spectra and pseudospectra. In:Ainsworth M, Levesley J, Marletta M, , editors. The Graduate Student's Guide to Numerical Analysis 98: Lecture Notes from the VIII EPSRC Summer School in Numerical Analysis. Berlin; Heidelberg: Springer Berlin Heidelberg (1999). p. 217–50.
31. Embree M, Trefethen LN. Pseudospectra Gateway. Available at: http://www.comlab.ox.ac.uk/pseudospectra (accessed July 28, 2024).
32. van Dorsselaer JLM, Kraaijevanger JFBM, Spijker MN. Linear stability analysis in the numerical solution of initial value problems. Acta Numerica. (1993) 2:199–237.
33. Joykutty J. Quasinormal Modes of Nearly Extremal Black Holes. Cambridge: Department of Applied Mathematics And Theoretical Physics, Cambridge University (2024).
34. Dafermos M, Rodnianski I. Lectures on black holes and linear waves. Clay Math Proc. (2013) 17:97–205. doi: 10.48550/arXiv.0811.0354
35. Trefethen LN. Spectral Methods in MATLAB. Society for Industrial and Applied Mathematics (2000). doi: 10.1137/1.9780898719598
Keywords: quasinomal modes of black holes, black holes, spectral instability, non-normal, wave equation
Citation: Warnick CM (2024) (In)stability of de Sitter quasinormal mode spectra. Front. Appl. Math. Stat. 10:1472401. doi: 10.3389/fams.2024.1472401
Received: 29 July 2024; Accepted: 11 September 2024;
Published: 02 October 2024.
Edited by:
Jose Luis Jaramillo, Université de Bourgogne, FranceReviewed by:
Piotr Bizon, Jagiellonian University, PolandCopyright © 2024 Warnick. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: C. M. Warnick, Yy5tLndhcm5pY2tAbWF0aHMuY2FtLmFjLnVr
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.