- 1Hubei key laboratory of energy storage and power battery, School of Mathematics, Physics and Optoelectronic Engineering, Hubei University of Automotive Technology, Shiyan, China
- 2School of Physics and Electronic Information, Weifang University, Weifang, China
- 3Anhui Province Key Laboratory of Photo-Electronic Materials Science and Technology, and College of Physics and Electronic Information, Anhui Normal University, Wuhu, China
- 4School of Physics, Zhejiang University, Hangzhou, China
- 5Department of Physics, Wenzhou University, Wenzhou, China
This study proposes a hybrid quantum system of an ensemble of collective spins coupled to a surface acoustic wave (SAW) cavity through a sideband design. Assisted by a dichromatic optical drive with a phase-dependent control, this spin ensemble can effectively mimic different types of long-range Lipkin–Meshkov–Glick (LMG) interactions and then undergo quantum phase transitions (QPTs) due to phase-induced spontaneous symmetry breaking (SSB). In addition, this phase-controlled scheme also ensures the dynamical preparation of the spin-squeezed state (SSS), which may be a useful application in quantum measurement. This study is a fresh attempt at quantum manipulation based on acoustic control and also provides a promising route toward useful applications in quantum information processing, especially the adiabatic preparation of multiparticle-entangled ground states via QPTs; i.e., the Greenberger–Horne–Zeilinger (GHZ) or W-type states.
1 Introduction
In the field of quantum information processing (QIP) and quantum manipulation (QM), the quantized electromagnetic field has always been considered the most reliable medium and means, such as in cavity quantum electrodynamics (QED) system and the superconducting circuits (SC) system (Xiang et al., 2013; Blais et al., 2020; Carusotto et al., 2020; Clerk et al., 2020; Haroche et al., 2020; Blais et al., 2021). However, with the rapid development of cryogenic and micro-nano processing technologies, traditional mechanical or acoustic devices have gradually re-entered the quantum world (O’Connell et al., 2010; Aspelmeyer et al., 2014; Forn-Díaz et al., 2019). In particular, quantum acoustic devices (QADs) have become among the most valuable quantum units owing to their unique advantages (Naber et al., 2006; Gustafsson et al., 2014; Schuetz et al., 2015). First, for the suppression of quantum noise, QADs can isolate environmental phononic noise at a much higher efficiency compared to other electromagnetic systems. Second, QADs are more convenient to design and fabricate because of their inherent larger dimensions (Schuetz et al., 2015; Kuzyk and Wang, 2018). Third, they originate from mature magnetostrictive or piezoelectric technologies, which can further improve their adaptive capacity to establish various hybrid quantum systems (Manenti et al., 2016; Knörzer et al., 2018). Finally, QADs can induce strong coupling to different qubits; this coupling mechanism is mainly similar to the ion (or atom) trap system and is naturally applicable to multiple QIP schemes, which were first proposed in ion trap systems (Andrew Golter et al., 2016a; Andrew Golter et al., 2016b). Therefore, an acoustic-based hybrid system can provide a promising platform to implement QIP and QM targets to ensure the efficient tailoring of spin–phonon and spin–spin interactions (Rabl et al., 2009; Bennett et al., 2013). Working as a quantum data bus or transducer, QADs can provide strong interactions in a wide range of qubits, especially at the single-quantum level (Li et al., 2020; Zhou et al., 2021; Leng et al., 2022; Zhou et al., 2022). Taking the surface acoustic wave (SAW) cavity, for example, strong coupling to different qubits can be achieved, with an estimated cooperativity C ∼ g2/(γκ) of C ∼ 10–100 (Schuetz et al., 2015).
Recently, other kinds of artificial qubits, including nitrogen-vacancy (NV), silicon-vacancy (SiV), and hexagonal boron nitride (hBN) color centers have been introduced into the field of quantum science (Gao et al., 2015; Lemonde et al., 2018; Tan et al., 2022; Zhao et al., 2022). Without an additional trap, a SAW-based device may also induce strong stressful interactions directly to this type of solid-state spin, which increased interest for QIP and QM. This study proposes a general hybrid quantum system of an ensemble of collective spins coupled to a surface acoustic wave (SAW) cavity through a sideband design. Assisted by a dichromatic optical drive with a phase-dependent control, this spin ensemble effectively mimicked different types of long-range spin–spin interactions, namely the Lipkin–Meshkov–Glick (LMG) model with different types (Lipkin et al., 1965; Castaños et al., 2006; Morrison and Parkins, 2008; Ma and Wang, 2009; Zhang et al., 2017). Through a phase-dependent modification of this optical drive, this ensemble of spins undergoes quantum phase transitions (QPTs) because of the so-called spontaneous symmetry breaking (SSB). In addition, this phase-controlled scheme can also supply both the dynamical preparation of spin-squeezed state (SSS) (Kitagawa and Ueda, 1993; Wineland et al., 1994) and the adiabatic preparation of the multiparticle entangled ground states via QPTs (Vidal et al., 2004a; Vidal et al., 2004b); i.e., the Greenberger–Horne–Zeilinger (GHZ) or W-type states (Anders and Mølmer, 1999; Mølmer and Anders, 1999; Zheng, 2001; Unanyan and Fleischhauer, 2003). Given the goal of performing realistic QIP and QM, the results of our investigations are a fresh attempt using an acoustic control and may also provide general and useful applications.
2 Setup and Hamiltonian
Here we consider the basic proposal for this hybrid system and the coupling mechanism, which are illustrated in Figures 1A, B, C. Without a loss of generality, we take an ensemble of the NV centers as an example. In Figure 1A, an ensemble of solid-state spins is set near the surface of the SAW cavity with a fundamental frequency ωm/2π ∼ 0.1–1.0 GHz. A phase-dependent dichromatic field (λ ∼ 700 nm) is applied to transmit the optional classical field (θ1, ω1, Ω1) or (θ2, ω2, Ω2). According to the previous investigation, this type of spin–phonon coupling is analogous to the ion trap system, and this SAW mode is similar to a harmonic oscillator potential as shown in Figure 1B. For an NV center, the energy-level design is plotted in Figure 1C, where |e(g)⟩ denotes the excited (ground) state with the energy split ω0 ≫ ωm. We can obtain only the longitudinal coupling to each NV center via the SAW cavity, based on the expression
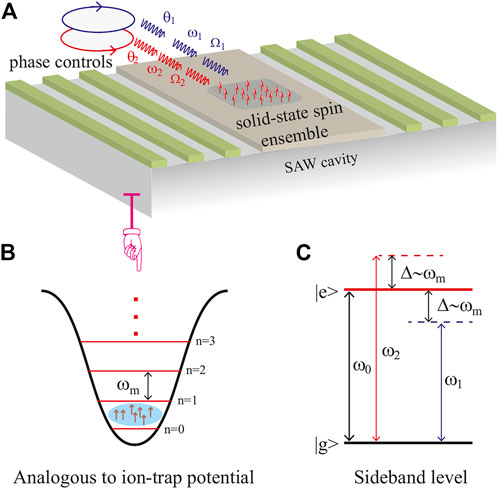
FIGURE 1. (Color online) Scheme diagrams. (A) An ensemble of solid-state spins is set near the surface of the SAW cavity with the phonon annihilation (creation) operator
Therefore, the systemic Hamiltonian for each NV spin (i.e., we consider the jth NV spin) is expressed as (ℏ = 1)
where
in which the collective spin operators are defined as
In this scheme, we assume the following relations: ν ≫ λ, |Δ|≫|Ω1,2|, and {ν, |Δ|, |Δ ± ν|}≫ η|Ω1,2| and can then eliminate this phonon mode adiabatically (according to Appendix A) (James, 1998; James and Jerke, 2012). Ignoring the items for the energy shift caused by this acoustic mode (
where the relevant coefficients are
3 LMG model
The Lipkin–Meshkov–Glick (LMG) model was first proposed in the area of nuclear physics to describe monopole-monopole interactions and is one kind of solvable long-range spin–spin model (Lipkin et al., 1965). This model, not only obeys the conservation of angular momentum but also satisfies the
and the relevant tunable parameters are ϵ, V, and W, respectively (Lipkin et al., 1965). Here, Eq. 5 satisfies the conservation of angular momentum, namely,
4 Discussion of the phase-dependent control
First, according to the previous investigation of this long-range spin model, the isotropy LMG model is (see Appendix B)
In this Hamiltonian,
Second, the more interesting and novel point lies in the phase-dependent control of this proposal, which we discuss briefly. Here, we assume
with
For single solid-state spin, we first define
When
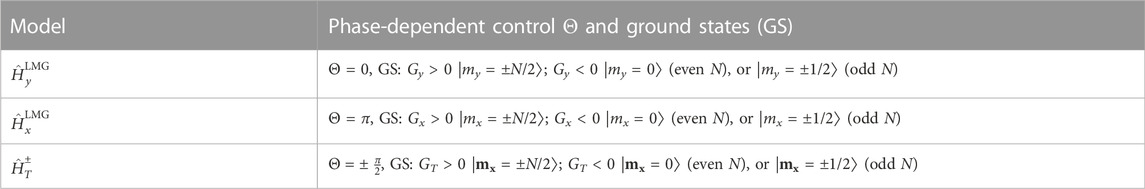
TABLE 1. Phase-dependent LMG models and ground states (GS) (Zhou et al., 2018).
While for case (2), when Θ = π, we can also get another one-axis twisting LMG model along the x direction,
Equivalently, when
Then, for case (3), as illustrated in the third and fourth lines of Table 1, when Θ = ±π/2, we obtain a novel transverse-type LMG model (in the x−y plane) as follows:
This type of spin–spin interaction belongs to the one-axis twisting model. For example, if we define a linear transformation in this model; i.e.,
Thus, despite the different representations, we can always get the one-axis twisting LMG model, which can also lead to the preparation of the spin-squeezed state (SSS) through a dynamic process. Two main definitions have been proposed to describe the squeezed degree of this spin ensemble, (Sidles et al., 1995). First, Masahiro Kitagawa and Masahito Ueda defined
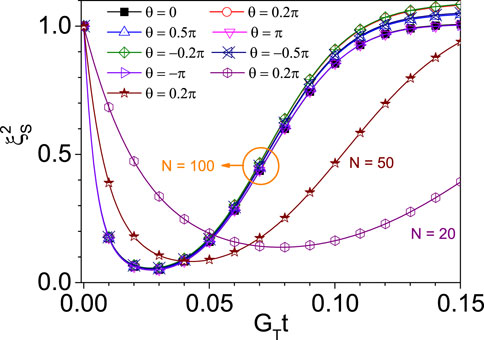
FIGURE 2. (Color online) Dynamical squeezing parameter
From which we note that this general LMG model can always engineer collective spins into the SSS dynamically at time
5 Quantum phase transitions (QPTs)
Utilizing the standard average-field approximation, we can determine the expected value of the collective spin components via the definition
in which we assume that all spins are homogenous and that their relative dephasing and decay rates are uniform factors; i.e., we assume that γdc ∼Γdc/(−GT) and γdp ∼Γdp/(−GT). By discarding the quantum fluctuations of
Together with the total spins’ conservation relation X2+Y2+Z2 = 1, we obtain the analytical solutions of the relevant order parameters, i.e., X, Y, and Z, by solving this group of equations. Then, we can determine the analytical solutions as (1) the trivial solution X = Y = 0, and Z = ±1 for the normal phase; and (2) the non-trivial solutions
Assuming γdp ≈ 0.1γdc, we get Z = −0.05 and plot the numerical average-value order parameters X and Y in Figure 3. Owing to the Z2 symmetry of this general LMG model
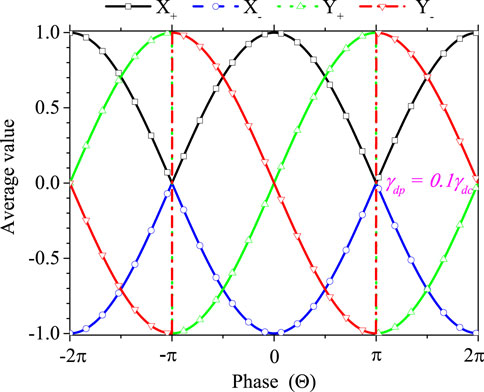
FIGURE 3. (Color online) Steady-state order parameters X and Y varying with Θ ∈ [−2π, 2π], with γdp ≈ 0.1γdc.
More specifically, when Θ approaches points such as 0, ± 2π, and even 2kπ (k is any integer number), X+ → 1, X− → −1, and Y± → 0 simultaneously. This type of QPT is mainly induced by the transformation of the representation; i.e., z ↔ x or x ↔ y. We consider this to correspond to phase-induced spontaneous symmetry breaking (SSB). When Θ → ±π, we get a different QPT effect, namely, X± → 0; however, its n-order derivative at this point
6 Applications for engineering multiparticle entanglement
Therefore, no matter in case (1) and in cases (2) and (3), these long-range spin–spin interactions belong to the one-axis twisting LMG model under their different y, x, and x representations. This type of spin model can also play an important role in the preparation of multiparticle entanglement. Starting from the original and general LMG model (4), we briefly discuss this topic.
Initially, we can easily get the isotropy LMG model such as Eq. 6, which belongs to the FM order. Its ground state is the disentangled and unique state |mz = S⟩ or |mz = −S⟩, which is also decided by the sign of
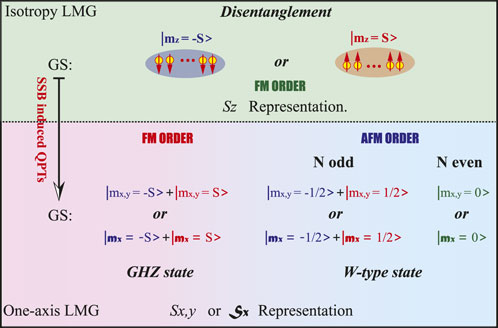
FIGURE 4. (Color online) Basic transformation schematic for generating the multiparticle entanglement via QPTs induced by SSB.
7 Experimental considerations
In this proposal, we first considered an ensemble of solid-state spins located on the surface of the SAW cavity with a fundamental frequency of ωm/2π ∼ 0.1–1.0 GHz (Schuetz et al., 2015). SAW-based quantum devices can induce strong or ultra-strong interactions in many kinds of atoms, spins, and even other artificial atoms, as shown in Table 2. Taking the nitrogen-vacancy (NV) spins for example, we can define the ground state |0⟩ ≡|g⟩ and excited state |Ey⟩ ≡|e⟩, with energy-splitting ω0/2π ∼ 470 THz. The estimated coherent spin–phonon coupling at the single-quantum level is approximately g0/2π ∼ 100 kHz; thus, we can determine the collective coupling strength
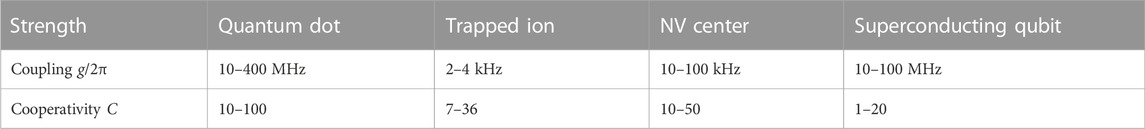
TABLE 2. Basic coupling of the SAW-based platform to different qubits (Schuetz et al., 2015).
8 Conclusion
Utilizing a hybrid system of collective spins coupled to a SAW cavity, we study a proposal for simulating the general long-range LMG model with a phase-dependent control. Our analysis and discussion show that this scheme can not only ensure the generation of the SSS via a dynamical evolution governed by the general one-axis twisting interactions but also supply a potential route toward multiparticle GHZ or W-type states through their FM or AFM phase transitions. We also studied the open-system critical behavior of this general LMG model by using the average-field method. For the target of carrying out realistic QIP and QM, our findings may be considered a fresh attempt by using an acoustic control, especially a phase-dependent control. Moreover, this proposed system might have various applications.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material. Further inquiries can be directed to the corresponding author.
Author contributions
YZ propose the proposal and write the whole paper; Q-LW support this investigation and provide the necessary financial assistance; L-ZC, C-SH, Z-CZ, WX, and Q-LW participate in the discussions.
Funding
This investigation was supported by the National Key Research and Development Program of China (NKRD) (2020YFC2200500), Natural National Science Foundation (NSFC) (11774285, 12047524, and 11774282); the China Postdoctoral Science Foundation (2021M691150); the Natural Science Foundation of Hubei Province (2020CFB748); the Natural Science Foundation of Shandong Province (ZR2021MA042 and ZR2021MA078); the Research Project of Hubei Education Department (B2020079); the Doctoral Scientific Research Foundation of Hubei University of Automotive Technology (HUAT) (BK201906, BK202113, and BK202008); the Innovation Project of University Students in HUAT (DC2022097, DC2022100, DC2021107, and DC2021108); an Open Fund of HUAT (QCCLSZK 2021A07); and the Foundation of Discipline Innovation Team of HUAT. Part of the simulations is coded in Python using the QuTiP library (Johansson et al., 2012; Johansson et al., 2013).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Anders, S., and Mølmer, K. (1999). Quantum computation with ions in thermal motion. Phys. Rev. Lett. 82, 1971–1974. doi:10.1103/physrevlett.82.1971
Andrew Golter, D., Oo, T., Amezcua, M., Stewart, K. A., and Wang, H. (2016). Optomechanical quantum control of a Nitrogen-Vacancy center in diamond. Phys. Rev. Lett. 116, 143602. doi:10.1103/physrevlett.116.143602
Andrew Golter, D., Oo, T., Amezcua, M., Lekavicius, I., Stewart, K. A., and Wang, H. (2016). Coupling a surface acoustic wave to an electron spin in diamond via a dark state. Phys. Rev. X 6, 041060. doi:10.1103/physrevx.6.041060
Aspelmeyer, M., Tobias, J., and Marquardt, F. (2014). Cavity optomechanics. Rev. Mod. Phys. 86, 1391–1452. doi:10.1103/revmodphys.86.1391
Bennett, S. D., Yao, N. Y., Otterbach, J., Zoller, P., Rabl, P., and Lukin, M. D. (2013). Phonon-induced spin-spin interactions in diamond nanostructures: Application to spin squeezing. Phys. Rev. Lett. 110, 156402. doi:10.1103/physrevlett.110.156402
Blais, A., Girvin, S. M., and Oliver, W. D. (2020). Quantum information processing and quantum optics with circuit quantum electrodynamics. Nat. Phys. 16, 247–256. doi:10.1038/s41567-020-0806-z
Blais, A., Arne, L., and Grimsmo, S. M. (2021). Circuit quantum electrodynamics. Rev. Mod. Phys. 93, 025005. doi:10.1103/revmodphys.93.025005
Carusotto, I., Houck, A. A., Kollár, A. J., Roushan, P., and Simon, J. (2020). Photonic materials in circuit quantum electrodynamics. Nat. Phys. 16, 268–279. doi:10.1038/s41567-020-0815-y
Castaños, O., López-Peña, R., Hirsch, J. G., and López-Moreno, E. (2006). Classical and quantum phase transitions in the Lipkin-Meshkov-Glick model. Phys. Rev. B 74, 104118. doi:10.1103/physrevb.74.104118
Clerk, A. A., Lehnert, K. W., Bertet, P., Petta, J. R., and Nakamura, Y. (2020). Hybrid quantum systems with circuit quantum electrodynamics. Nat. Phys. 16, 257–267. doi:10.1038/s41567-020-0797-9
Forn-Díaz, P., Lamata, L., Rico, E., Kono, J., and Solano, E. (2019). Ultrastrong coupling regimes of light-matter interaction. Rev. Mod. Phys. 91, 025005. doi:10.1103/revmodphys.91.025005
Gao, W. B., Imamoglu, A., Bernien, H., and Hanson, R. (2015). Coherent manipulation, measurement and entanglement of individual solid-state spins using optical fields. Nat. Photonics 9, 363–373. doi:10.1038/nphoton.2015.58
Gustafsson, M. V., Aref, T., Kockum, A. F., Ekström, M. K., and Johansson, G. (2014). Propagating phonons coupled to an artificial atom. Science 346, 207–211. doi:10.1126/science.1257219
Haroche, S., Brune, M., and Raimond, J. M. (2020). From cavity to circuit quantum electrodynamics. Nat. Phys. 16, 243–246. doi:10.1038/s41567-020-0812-1
James, D. F. V., and Jerke, J. (2012). Effective Hamiltonian theory and its applications in quantum information. Can. J. Phys. 85 (8), 625–632. doi:10.1139/p07-060
James, D. F. V. (1998). Quantum dynamics of cold trapped ions with application to quantum computation. Appl. Phys. B Lasers Opt. 66, 181–190. doi:10.1007/s003400050373
Johansson, J. R., Nation, P. D., and Nori, F. (2012). QuTiP: An open-source Python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 183, 1760–1772. doi:10.1016/j.cpc.2012.02.021
Johansson, J. R., Nation, P. D., and Nori, F. (2013). Qutip 2: A python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 184, 1234–1240. doi:10.1016/j.cpc.2012.11.019
Kitagawa, M., and Ueda, M. (1993). Squeezed spin states. Phys. Rev. A 47, 5138–5143. doi:10.1103/physreva.47.5138
Knörzer, J., Schuetz, M. J. A., Giedke, G., Huebl, H., Weiler, M., Lukin, M. D., et al. (2018). Solid-state magnetic traps and lattices. Phys. Rev. B 97, 235451. doi:10.1103/physrevb.97.235451
Kuzyk, M. C., and Wang, H. (2018). Scaling phononic quantum networks of solid-state spins with closed mechanical subsystems. Phys. Rev. X 8, 041027. doi:10.1103/physrevx.8.041027
Lemonde, M. A., Meesala, S., Sipahigil, A., Schuetz, M. J. A., Lukin, M. D., Loncar, M., et al. (2018). Phonon networks with silicon-vacancy centers in diamond waveguides. Phys. Rev. Lett. 120, 213603. doi:10.1103/physrevlett.120.213603
Leng, S-Y., Lü, D-Y., Yang, S-L., Ma, M., Dong, Y-Z., Zhou, B-F., et al. (2022). Simulating the dicke lattice model and quantum phase transitions using an array of coupled resonators. J. Phys. Condens. Matter 34, 415402. doi:10.1088/1361-648x/ac84bd
Li, P-B., Zhou, Y., Gao, W-B., and Nori, F. (2020). Enhancing spin-phonon and spin-spin interactions using linear resources in a hybrid quantum system. Phys. Rev. Lett. 125, 153602. doi:10.1103/physrevlett.125.153602
Lipkin, H. J., Meshkov, N., and Glick, A. J. (1965). Validity of many-body approximation methods for a solvable model:(i). exact solutions and perturbation theory. Nucl. Phys. 62, 188–198. doi:10.1016/0029-5582(65)90862-x
Ma, J., and Wang, X. (2009). Fisher information and spin squeezing in the Lipkin-Meshkov-Glick model. Phys. Rev. A 80, 012318. doi:10.1103/physreva.80.012318
Manenti, R., Peterer, M. J., Nersisyan, A., Magnusson, E. B., Patterson, A., and Leek, P. J. (2016). Surface acoustic wave resonators in the quantum regime. Phys. Rev. B 93, 041411. doi:10.1103/physrevb.93.041411
Mølmer, K., and Anders, S. (1999). Multiparticle entanglement of hot trapped ions. Phys. Rev. Lett. 82, 1835–1838. doi:10.1103/physrevlett.82.1835
Morrison, S., and Parkins, A. S. (2008). Dynamical quantum phase transitions in the dissipative Lipkin-Meshkov-Glick model with proposed realization in optical cavity QED. Phys. Rev. Lett. 100, 040403. doi:10.1103/physrevlett.100.040403
Naber, W. J. M., Fujisawa, T., Liu, H. W., and van der Wiel, W. G. (2006). Surface-acoustic-wave-induced transport in a double quantum dot. Phys. Rev. Lett. 96, 136807. doi:10.1103/physrevlett.96.136807
O’Connell, A. D., Hofheinz, M., Ansmann, M., Bialczak, R. C., Lenander, M., Lucero, E., et al. (2010). Quantum ground state and single-phonon control of a mechanical resonator. Nature 464, 697–703. doi:10.1038/nature08967
Rabl, P., Cappellaro, P., Gurudev Dutt, M. V., Jiang, L., Maze, J. R., and Lukin, M. D. (2009). Strong magnetic coupling between an electronic spin qubit and a mechanical resonator. Phys. Rev. B 79, 041302. doi:10.1103/physrevb.79.041302
Rabl, P., Kolkowitz, S. J., Koppens, F. H. L., Harris, J. G. E., Zoller, P., and Lukin, M. D. (2010). A quantum spin transducer based on nanoelectromechanical resonator arrays. Nat. Phys. 6, 602–608. doi:10.1038/nphys1679
Schuetz, M. J. A., Kessler, E. M., Giedke, G., Vandersypen, L. M. K., Lukin, M. D., and Cirac, J. I. (2015). Universal quantum transducers based on surface acoustic waves. Phys. Rev. X 5, 031031. doi:10.1103/physrevx.5.031031
Sidles, J. A., Garbini, J. L., Bruland, K. J., Rugar, D., Züger, O., Hoen, S., et al. (1995). Magnetic resonance force microscopy. Rev. Mod. Phys. 67, 249–265. doi:10.1103/revmodphys.67.249
Tan, Q., Lai, J-M., Liu, X-L., Guo, D., Xue, Y., Dou, X., et al. (2022). Donor-acceptor pair quantum emitters in hexagonal boron nitride. Nano Lett. 22, 1331–1337. doi:10.1021/acs.nanolett.1c04647
Tsomokos, D. I., Ashhab, S., and Nori, F. (2008). Fully connected network of superconducting qubits in a cavity. New J. Phys. 10, 113020. doi:10.1088/1367-2630/10/11/113020
Unanyan, R. G., and Fleischhauer, M. (2003). Decoherence-free generation of many-particle entanglement by adiabatic ground-state transitions. Phys. Rev. Lett. 90, 133601. doi:10.1103/physrevlett.90.133601
Vidal, J., Palacios, G., and Mosseri, R. (2004). Entanglement in a second-order quantum phase transition. Phys. Rev. A 69, 022107. doi:10.1103/physreva.69.022107
Vidal, J., Mosseri, R., and Dukelsky, J. (2004). Entanglement in a first-order quantum phase transition. Phys. Rev. A 69, 054101. doi:10.1103/physreva.69.054101
Wineland, D. J., Bollinger, J. J., Itano, W. M., Moore, F. L., and Heinzen, D. J. (1992). Spin squeezing and reduced quantum noise in spectroscopy. Phys. Rev. A 46, R6797–R6800. doi:10.1103/physreva.46.r6797
Wineland, D. J., Bollinger, J. J., Itano, W. M., and Heinzen, D. J. (1994). Squeezed atomic states and projection noise in spectroscopy. Phys. Rev. A 50, 67–88. doi:10.1103/physreva.50.67
Xiang, Z-L., Ashhab, S., You, J. Q., and Nori, F. (2013). Hybrid quantum circuits: Superconducting circuits interacting with other quantum systems. Rev. Mod. Phys. 85, 623–653. doi:10.1103/revmodphys.85.623
Zhang, Y-C., Zhou, X-F., Guo, G. C., and Zhou, Z. W. (2017). Cavity-assisted single-mode and two-mode spin-squeezed states via phase-locked atom-photon coupling. Phys. Rev. Lett. 118, 083604. doi:10.1103/physrevlett.118.083604
Zhao, M., Cai, H., Chen, D., Kenny, J., Jiang, Z., Ru, S., et al. (2022). Excited-state optically detected magnetic resonance of spin defects in hexagonal boron nitride. Phys. Rev. Lett. 128, 216402. doi:10.1103/PhysRevLett.128.216402
Zheng, S-B. (2001). One-step synthesis of multiatom Greenberger-Horne-Zeilinger states. Phys. Rev. Lett. 87, 230404. doi:10.1103/physrevlett.87.230404
Zhou, Y., Li, B., Li, X-X., Li, F-L., and Li, P-B. (2018). Preparing multiparticle entangled states of nitrogen-vacancy centers via adiabatic ground-state transitions. Phys. Rev. A 98, 052346. doi:10.1103/physreva.98.052346
Zhou, Y., Lü, D-Y., Wang, G-H., Fu, Y-H., He, M-Y., and Ren, H-T. (2021). Improvement on the manipulation of a single nitrogen-vacancy spin and microwave photon at single-quantum level. Commun. Theor. Phys. 73, 065101. doi:10.1088/1572-9494/abec3a
Zhou, Y., Hu, C-S., Lü, D-Y., Li, X-K., Huang, H-M., Xiong, Y-C., et al. (2022). Synergistic enhancement of spin-phonon interaction in a hybrid system. Phot. Res. 10, 1640–1649. doi:10.1364/prj.459794
Appendix A: Derivation of the effective Hamiltonian
According to Eq. 3, we can rewrite
where δ2 = ωm+Δ, δ3 = ωm−Δ, and
Utilizing the method of effective Hamiltonian, we get (James, 1998; James and Jerke, 2012)
Thus, we can rewrite the total systemic Hamiltonian with an effective form
Utilizing the relation
with the coefficients
In general, if we may assume
Appendix B: Isotropy LMG model
From Eqs. A9, A11, we assume r1 = 0, r2 ≠ 0 or r2 = 0, r1 ≠ 0; thus, the isotropy LMG model is
with coefficients (η ∼ 0.1–0.2)
Keywords: hybrid quantum system, surface acoustic wave, Lipkin–Meshkov–Glick (LMG) model, quantum phase transitions (QPTs), spin-squeezed state
Citation: Zhou Y, Cao L-Z, Wang Q-L, Hu C-S, Zhang Z-C and Xiong W (2023) Phase-dependent strategy to mimic quantum phase transitions. Front. Quantum Sci. Technol. 1:1078597. doi: 10.3389/frqst.2022.1078597
Received: 24 October 2022; Accepted: 07 December 2022;
Published: 12 January 2023.
Edited by:
Youngik Sohn, Korea Advanced Institute of Science and Technology (KAIST), South KoreaCopyright © 2023 Zhou, Cao, Wang, Hu, Zhang and Xiong. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Yuan Zhou, emhvdXl1YW5AaHVhdC5lZHUuY24=
 Yuan Zhou
Yuan Zhou Lian-Zhen Cao2
Lian-Zhen Cao2 Chang-Sheng Hu
Chang-Sheng Hu