Chaotic and fractal maps in higher-order derivative dynamical systems

Hamiltonian maps are considered a class of dynamical systems that hold meticulous properties used to model a large number of complex dynamical systems. When time flows in dynamical systems with two-dimensional degrees of freedom, the trajectories in phase space can be analyzed within bidimensional surfaces known as Poincaré sections. The Chirikov–Taylor standard map for two canonical dynamical variables (momentum and coordinate) is the most renewed map characterized by a family of area-preserving maps with a single parameter that controls the degree of chaos. In this study, a generalization of the standard map for two different problems is presented and discussed. The first problem deals with the higher-order derivative Hamiltonian system (up to the fourth order) since the fourth-order characteristic provides the possibility of chaotic behavior at all scales including nanoscales where high-order derivatives take place in nanosystems. The second problem concerns the time-dependent δ-kicked rotor in fractal dimensions characterized by a time-dependent potential due to its important implications in quantum chaos. This study shows that higher-order derivative maps and fractal dimensional δ-kicked rotor maps apparently exhibit a large number of chaotic orbits and fractal patterns, including the spiral fractal patterns comparable to the Julia set. Moreover, these problems are characterized by additional parameters which can be used to control chaos. Some of these parameters lead to chaos, and others lead to fractal patterns.

Introduction

Classical mechanics is successfully described based on the Lagrangian and Hamiltonian formalisms, which fulfill the locality basic property. The trajectory of any body and its associated derivatives depend on a single point. The most significant results of both formalisms, including Noether’s theorem for dynamical systems with an infinite number of degrees of freedom, are well-known in the literature [1]. A classical local dynamical system consists of a set of possible local states described by one or a set of second-order differential equations. A basic account of these equations can be obtained by means of the discrete time framework modeled through maps, e.g., the kicked-rotor problem, which plays an important role in dynamical systems. These discrete maps alternate a system of differential equations and are practical in computational modeling of complex dynamical systems [2, 3]. In case of higher-order differential equations, e.g., the fourth-order differential equations, they offer the possibility of chaotic behavior in contrast to second-order autonomous differential equations, principally since such systems do not have an adequate amount of degrees of freedom. Hence, one naturally expects that nth-order differential equations will offer a rich variety of patterns and chaotic structures than the second-order differential equations. In general, any system described by a second-order Lagrangian leads to a fourth-order Lagrangian [4–8]. A supplementary advance in the theory of differential equations both facilitates applications and offers new insights in applied mathematics. The penultimate motive for this study is to understand the causal structure of higher-order differential equations, in particular of fourth-order equations, to study their associated standard map, and finally to understand the impacts of fractal dimensions on them. The reason to consider fractal dimensions in this study is based on the fact that fractals are a type of dynamic system generated by recursion. They depend on initial conditions and generate a kind of non-periodic orbits. Fractals, therefore, belong to chaotic dynamical systems. Let us stress that not all chaotic systems are fractals, e.g., stochastic dynamical systems. In phase space, a chaotic orbit traces out a fractal dimensional strange attractor, i.e., strange attractors exhibit the fractal structure [9]. Substantial attention is given, in this study, to two main types of problems, which generalize the basic standard map, known as the Chirikov–Taylor standard map [2]. These problems are expressed as follows:

1. The problem of the fourth-order differential equation is expressed as follows:

$-\gamma u^{\left(4\right)}\left(x\right)+\beta u^{″}\left(x\right)+K\sin u\left(x\right)=0,(1)$

where $u=u\left(x\right)$ is a function of the space $x$ and $\beta \in {\mathbb{R}}^{+}$ and $\gamma \in {\mathbb{R}}^{+}$ are assumed to be small parameters. We will prove that the limit of the problem will lead to the “kicked rotor” problem in higher-order derivative theory. Higher-order dynamical systems play a pertinent role in theoretical physics, applied mathematics, and numerical analysis. This problem is of particular importance since it may have motivating implications in the understanding of the kicked-rotor problem, formed by periodically pulsing on the optical higher-order standing waves [10, 11].

2. The problem of “time-dependent δ-kicked rotor” formulated in fractal dimensions. We are interested on fractal calculus concepts introduced in [12, 13], where the derivatives of two given functions are given by $f\left(x\right)$ and $g\left(t\right)$, which are expressed as $d^{\alpha }f\left(x\right)/d{x}^{\alpha }=\left(x^{1-\alpha }/\alpha \right)\left(df\left(x\right)/dx\right),\alpha >0$ and $d^{\beta }g\left(t\right)/d{t}^{\beta }=\left(t^{1-\beta }/\beta \right)\left(dg\left(t\right)/dt\right),\beta >0$, respectively. Here, $\alpha$ and $\beta$ are their associated fractal dimensions. It is obvious that the fractal length measure $x$ and the fractal time measure $t$ are scaled according to the laws $x^{\alpha }$ and $t^{\beta }$. This approach has motivating implications in various fields of sciences and engineering and at different lengths and time scales [14–20]. These fractal derivatives are considered a non-Newtonian simplification of the derivative defined in fractal medium or topology. Self-similarity and scale invariance are two important concepts in physics and any complex dynamical systems governed by Lagrangian and Hamiltonian mechanics laws. In general, the notion of non-integer fractal dimension has been spread over all fields of sciences since Mandelbrot introduced the notion of fractals or self-similar sets [21]. It is notable that fractal calculus is of practical importance in various fields of physics since it is considered, to some extent, trouble-free, helpful, and algorithmic [22]. It is also used in the theory of differential equations to study stability problems [23–25] besides its relevance in stochastic differential equations [26, 27] and transforms approaches such as the Laplace, Fourier, and Sumudu fractal transforms [28, 29]. The relevance of fractal calculus in sciences is well-appreciated based on a large number of outcomes obtained by researchers [30–47]. In the literature, there are various types of fractal derivatives where various inequalities have been obtained, and new classes of differential equations have been derived and analyzed accordingly [34, 48–57]. However, He’s fractal derivative is an extension of Leibniz’s derivative for discontinuous fractal media and is less tricky in mathematical analysis, but with relevance in various fields of sciences, as mentioned previously, including geometric analysis [58], attachment oscillator arising from nanotechnology [59], variational study of the time–space fractal Bogoyavlenskii equation [59], non-linear vibrations [60], and fractal nano/microelectromechanical system [61]

The purpose of this paper is to study the whole dynamical behavior of maps generated by these problems. We investigate the chaotic and complex behavior of standard maps by selecting various control parameters. From a practical point of view, we show that in addition to the usual stochastic parameter, there are additional parameters in each model, which can be used as chaos control parameters.

Before elaborating our analysis, two points deserve to be elucidated:

1. The investigation of higher-order derivative Hamiltonian systems (up to the fourth-order) is relevant as it offers insights into chaotic behavior across multiple scales, including the nanoscale. This shows potential to enhance our understanding of physical systems like micro-electromechanical systems (MEMS), where high-order derivatives play a crucial role. For example, in MEMS devices, the mechanical behavior at small scales can be highly non-linear and may exhibit chaotic dynamics that could be better understood through the study of such higher-order systems [62–69].

2. In our approach, we used the two-scale fractal development: in fact, the two-scale dimension is of great importance to describe any physical properly of a complex system. It is used to evaluate the degree of complexity of a given discontinuous pattern between two neighboring dissimilar scales of observation [70]. It is considered an alternative definition of fractal dimension. It is notable that physical laws are scale-dependent, and dissimilar outcomes may be obtained at different scales. The two-scale theory is practical since it treats any physical or dynamical problem with two different scales applied respectively for continuous and porous structures media: the conventional classic calculus can be successfully applied for the large scale, whereas for the smaller scale, the effect of the porous structure on the physical properties of the system can be effortlessly explained and, hence, reveal a number of hidden properties beyond the classical assumption. The validation of this new methodology has been proved using qualitative and quantitative/numerical techniques [70–80]. Therefore, it is motivating to consider, in this study, two-scale dimensions since they reveal a number of hidden properties and features not found within the conventional formalism.

Problem 1: To start, we introduce the Hamiltonian of Equation 1, which is written as

$H\left(u,v,p_u,p_v\right)=\frac{1}{2\gamma }{p}_v^2+v{p}_u-\frac{\beta }{2}{v}^2+\sin u,$

where $v=u^{\prime }$, $p_u=\beta u^{\prime }-\gamma u^{‴}$, and $p_v=\gamma u^{″}$ [5]. The associated Hamilton’s equations of motion are $v=u^{\prime }=\mathrm{\partial }H/\mathrm{\partial }{p}_u$, $v^{\prime }=\mathrm{\partial }H/\mathrm{\partial }{p}_v$, $p_u^{\prime }=-\mathrm{\partial }H/\mathrm{\partial }u$, and $p_v^{\prime }=-\mathrm{\partial }H/\mathrm{\partial }v$. To construct a map, we introduce a small number $\epsilon \ll 1$ such that

$u_{n+1}=u\left(x_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}u\left(x\left(n+1\right)-\epsilon \right),(2)$

$v_{n+1}=v\left(x_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}v\left(x\left(n+1\right)-\epsilon \right),(3)$

${}_{u}p_{n+1}={}_{u}p\left(x_{n+1}\right)\equiv p\left(x_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}p\left(x\left(n+1\right)-\epsilon \right),(4)$

${}_{v}P_{n+1}={}_{v}P\left(x_{n+1}\right)\equiv P\left(x_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}P\left(x\left(n+1\right)-\epsilon \right),(5)$

where $x_{n+1}=\left(n+1\right)x$. Integrating the Hamilton’s equation yields

$p_{n+1}=p_n+K\sin u_n,(6)$

$u_{n+1}=u_n+p_{n+1},(7)$

$P_{n+1}-P_n=-p_{n+1}+\beta \left(u_{n+1}-u_n\right),(8)$

$\gamma \left(v_{n+1}-v_n\right)=P_{n+1}=\gamma \left(u_{n+1}-2u_n+u_{n-1}\right),(9)$

which, after arrangement, also yields the modified standard map:

$p_{n+1}=\beta \left(u_{n+1}-u_n\right)-\gamma \left(u_{n+1}-3u_n+3u_{n-1}-u_{n-2}\right),(10)$

$p_{n+1}=p_n+K\sin u_n.(11)$

For $\beta =1$ and $\gamma =0$, Equations 2–9 reduce Equations 10, 11 to the Chirikov–Taylor standard map. In order to examine the typical features of the dynamics determined by the modified standard map, let us start our analysis with the results of the numerical observations of the particle motions in the phase space. We plot in Figure 1 accordingly, by running a program in MATLAB, the following figures (Poincaré sections) for different numerical values of the parameters $\beta$ and $\gamma$.

Figure 1

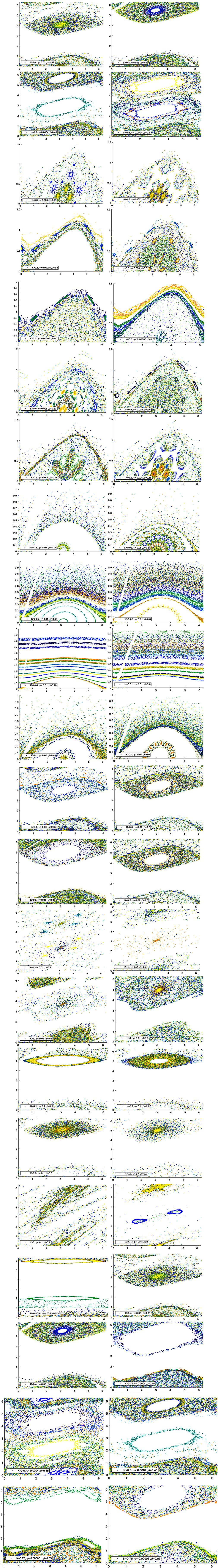
Figure 1. Particle orbits of the modified standard map for the different stochastic parameter $K$and parameters $\beta$and $\gamma$.

We observe the emergence of a family of patterns, including fractals and chaotic patterns. Decreasing $K$ suppresses the deterministic diffusion significantly and may lead to chaotic maps. Decreasing both $K$ and $\gamma$ also suppresses the formation of islands around islands. There is a classic evidence of stickiness in these plots and transition to chaos (chaotic sea) in some. Orbits are subject to consecutive traps, filling regions more densely than others. In some cases, we observe the Kolmogorov–Arnold–Moser (KAM) secondary islands corresponding to a certain resonance. We recall that the KAM theorem states that for non-integrable Hamiltonian systems, only non-destroyed orbits have quasiperiodic irrational winding numbers. We also observe islands in the chaotic sea, while islands of certain resonance are inside the corresponding last KAM curve. Small islands also emerge for certain values of $K$, besides unstable and stable orbits. There is an emergence of chaotic orbits depending on the values of the system parameters. For lower values of $K$, the size of the central island is reduced and is limited by a number of unstable periodic orbits. For some values of $K$ which are close to unity, there is emergence of spiral fractal patterns comparable to the Julia set [81, 82]. An arbitrary small variation in the parameters causes radical changes in the patterns. We recall that, in general, the standard map has an attractive property; it has a fractal behavior. Further consecutive amplifications would confirm the fractal-like structure of this model. Chaotic regions with various chaoticities are also observed, besides the chaotic chains whose chaoticities are weaker than other chaotic seas. These maps exhibit chaotic and fractal behaviors separately or together in the available phase space as the control parameters change. The breakdown of the ergodicity of this map may lead to a deformation of the statistical mechanical framework [83]. In fact, non-linear dynamical systems exhibit fractal structures in the phase space, and they are very sensitive to initial conditions [84]. This problem, however, has received less attention, for higher-order derivative theories. In this problem, we proved that fractal structures arise in fourth-order derivative theories, although the geometrization of Hamiltonian formalisms was developed for autonomous and non-autonomous mechanical systems [85–87].

Problem 2: We are concerned with the classical global momentum transport in the kicked rotor governed by the time-dependent Hamiltonian (time-dependent δ-kicked rotor):

$H\left(x,p,t\right)=\frac{p^2}{2}+KTV\left(x,t\right){\displaystyle \sum _{n=-\infty }^{n=+\infty }}\delta \left(t-n\right),(12)$

where $K$ is the amplitude of the pulse, $T$ is the period of oscillations, $p$ is the momentum, $V\left(x,t\right)$ is a time-dependent potential, and $\delta$ is the Dirac delta-function. Equation 12 is subject to the initial conditions $x\left(0\right)=x_0$ and $\dot{x}\left(0\right)=p\left(0\right)=p_0$ [1–3]. When $K$ is adequately large, no KAM invariant circles bound the motion. The presence of the Dirac function is suitable since the equations of motion can be reduced to a simple discrete Chirikov–Taylor standard map. It is notable that during the kick, the potential term dominates the kinetic term, the potential is zero between kicks, and the motion is that of a free rotor. One can, therefore, integrate easily the equations of motion over one temporal period of the Hamiltonian [2, 3, 88, 89]. However, in our approach, we considered a time-dependent potential due to their motivating implications in controlling quantum chaos [90–95]. In this study, we consider $V\left(x,t\right)=t^{\eta }\cos x$, with $\eta$ being a real parameter. In fact, periodic time-dependent Hamiltonian systems are said to be of $n$ and half degrees of freedom, and one way to study them is the stroboscopic map, which is a special case of a Poincaré map for driven systems [96]. It is considered in quantum-kicked top [97, 98]. Hamilton’s equations of motion in fractal dimensions are given by

$\frac{1}{\beta }{t}^{1-\beta }\frac{dp}{dt}=-\frac{1}{\alpha }{x}^{1-\alpha }\frac{\mathrm{\partial }H}{\mathrm{\partial }x}=-\frac{1}{\alpha }{x}^{1-\alpha }{t}^{\eta }KT\sin x{\displaystyle \sum _{n=-\infty }^{\infty }}\delta \left(t-nT\right),(13)$

$\frac{1}{\beta }{t}^{1-\beta }\frac{\mathrm{\partial }x}{\mathrm{\partial }t}=p.(14)$

The special case where $\eta =1-\beta$ is motivating since it does not lead to divergent series when performing the integration of Equation 13. To construct a map in fractal dimensions before the $n^{\text{th}}$-kick, we again introduce a small number $\epsilon \ll 1$ such that

$x_{n+1}=x\left(t_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}x\left(T\left(n+1\right)-\epsilon \right)(15)$

and

$p_{n+1}=p\left(t_{n+1}\right)={\lim }_{\epsilon \to 0^{+}}p\left(T\left(n+1\right)-\epsilon \right).(16)$

Here, $t_{n+1}=\left(n+1\right)T$. Accordingly, we integrate Equations 13, 14 using Equations 15, 16 as follows:

${\displaystyle \underset{t_n-\epsilon }{\overset{t_{n+1}-\epsilon }{\int }}}\frac{\mathrm{\partial }p}{\mathrm{\partial }t}dt=\frac{KT\beta }{\alpha }{x}^{1-\alpha }\sin x{\displaystyle \underset{t_n-\epsilon }{\overset{t_{n+1}-\epsilon }{\int }}}{t}^{\beta -1+\eta }{\displaystyle \sum _{n=1}^{\infty }}\delta \left(t-nT\right)dt,(17)$

${\displaystyle \underset{t_n-\epsilon }{\overset{t_{n+1}-\epsilon }{\int }}}\frac{\mathrm{\partial }x}{\mathrm{\partial }t}dt=\beta {\displaystyle \underset{t_n-\epsilon }{\overset{t_{n+1}-\epsilon }{\int }}}{t}^{\beta -1}pdt.(18)$

Equations 17, 18 yield, in particular for $\eta =1-\beta$:

$p_{n+1}=p_n-\frac{KT\beta }{\alpha }{x}_n^{1-\alpha }\sin x_n\equiv p_n+F_{\alpha }\left(x_n\right).(19)$

$x_{n+1}=x_n+\beta {\left(nT\right)}^{\beta -1}T{p}_{n+1}\equiv x_n+G_{\beta }\left(p_{n+1}\right).(20)$

Observe that when $\alpha =\beta =1$, Equations 19, 20 are reduced to the Chirikov–Taylor standard map. In addition, FSM differs from the fractional standard map obtained in [87, 88]. The stability of the fixed points is determined from the residue of the tangent map:

$\left(\begin{array}{l}\Delta p_{n+1}\\ \Delta x_{n+1}\end{array}\right)=\Delta M\left(\begin{array}{l}\Delta p_n\\ \Delta x_n\end{array}\right),(21)$

where

$\Delta M=\left(\begin{array}{cc}1& \frac{\mathrm{\partial }{F}_{\alpha }\left(x_n\right)}{\mathrm{\partial }{x}_n}\\ \frac{\mathrm{\partial }{G}_{\beta }\left(p_{n+1}\right)}{\mathrm{\partial }{p}_n}& 1+\frac{\mathrm{\partial }{F}_{\alpha }\left(x_n\right)}{\mathrm{\partial }{x}_n}\frac{\mathrm{\partial }{G}_{\beta }\left(p_{n+1}\right)}{\mathrm{\partial }{p}_n}\end{array}\right)(22)$

is the tangent map [39]. The stability of the system arises (using Equations 21, 22) if the residue given by

$R=\frac{1}{2}-\frac{1}{4}\text{Tr}\left(\Delta M\right)=-\frac{1}{4}\frac{\mathrm{\partial }{F}_{\alpha }\left(x_n\right)}{\mathrm{\partial }{x}_n}\frac{\mathrm{\partial }{G}_{\beta }\left(p_{n+1}\right)}{\mathrm{\partial }{p}_n}(23)$

is constrained by $0<R<1$, which yields at the fixed point $x_m=2\pi m,m\mathbb{\in }\mathbb{Z}$, $0<K<4\alpha {\left(2\pi m\right)}^{1-\alpha }/T\beta$, and stability occurs, phase-space trajectories lie on invariant curves, and the variation in momentum based on Equation 23 is restricted. We examine particle motions in the phase space by plotting in Figure 2 the Poincaré sections, where the orbits may have different behaviors depending on the values of the parameters $K$ and $T$ and the fractal dimensions $\alpha$ and $\beta$.

Figure 2

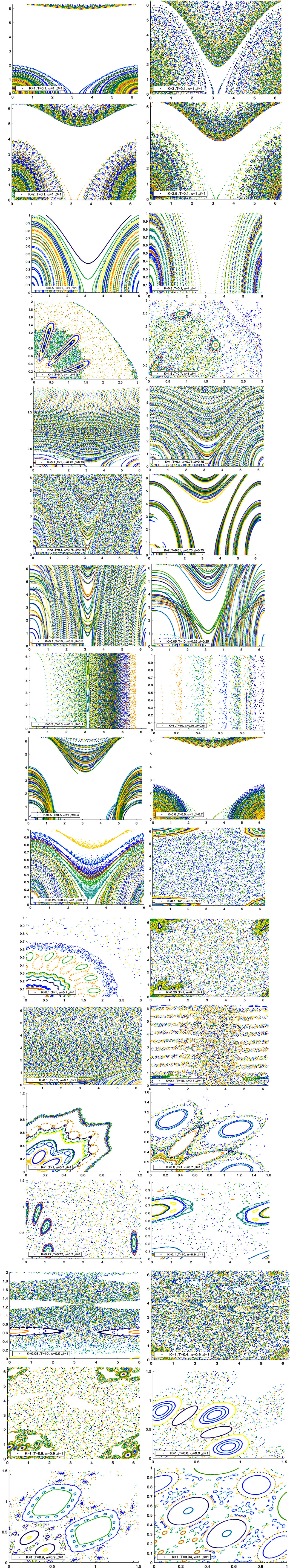
Figure 2. Particle orbits of the fractal standard map for different numerical values of the parameters $K$and $T$and the fractal dimensions $\alpha$and $\beta$.

We observe that all the parameters play an important role in the formation of islands around islands and that low fractal dimensions suppress the deterministic diffusion and lead to chaotic maps. KAM secondary islands corresponding to a certain resonance emerge in some particular cases. Trajectories demonstrate island chains connected with an assortment of elliptic periodic orbits. For small $K$ and spatial fractal dimension $\alpha$, chaotic behavior dominates larger phase space areas, i.e., a large area of the phase space is occupied by a single chaotic sea due to the large amount of non-integrability of the system. The fundamental reason for this behavior is related to destroying the stability islands. This leads to a decrease in the non-integrability of the fractal dynamical system. An increase in $K$ and fractal dimensions shows regions of chaotic behavior rising around stable and unstable manifolds, besides the emergence of fractal patterns. These orbits are characterized by a dense collection of points with no obvious order. These chaotic orbits can have positive Lyapunov exponents but free from any kind of fractal structure. The stabilization of these orbits may be achieved in some particular cases due to the presence of parameters that may help control the chaotic behavior. There is a critical value of $K$ and fractal dimensions from which the chaotic regions are no longer separated. For fractal dimensions close to unity, a central island with a chain of smaller islands around it is revealed, comparable to its larger-scale version. Additional successive amplifications would prove the fractal structure. It is notable that this fractal map verifies the twist condition $\mathrm{\partial }{x}_{n+1}/\mathrm{\partial }{p}_n\ne 0$, which is the analog of the non-degeneracy condition from Hamiltonian systems for KAM’s theorem applicability to the map [99, 100].

To conclude, we have constructed maps for two different dynamical problems: the first one describes higher-order derivative dynamical systems, and the second one, the time-dependent δ-kicked rotor in fractal dimensions. The first problem is characterized, for particular values of $K$, by the emergence of spiral fractal patterns comparable to the Julia set, besides the emergence in some cases of strange chaotic orbits which are thickly interfaced with regular regions. On the contrary, the second problem, which is dominated by fractal dimensions, reveals the emergence of invariant curves, islands, and fractal and chaotic trajectories. The transition to chaos is shown by varying control parameters. Some of these parameters lead to chaos, and others lead to fractal patterns. The range of convergence and stability can be made to increase considerably. The difference between the fractal time-dependent δ-kicked rotor and the conventional one with the integer derivative is the emergence of various quasiperiodic and periodic windows, intermittency, and chaotic structures, which depend on the numerical values of fractal dimensions. The dynamic system displays a rich assortment of non-linear behaviors as fractal dimensions are varied. We observe the occurrence of chaotic regions exhibiting fractal features (islands around islands) in regions confined between the other types of trajectories. In several cases, KAM secondary islands corresponding to a certain resonance emerge. The second problem is, therefore, very sensitive to fractal dimensions. These new standard maps might be used to achieve better results to study quantum chaos. This work addresses challenges in incorporating Hamiltonian systems with higher-order derivatives and fractal derivatives into the analysis of complex systems that go beyond the standard map. It offers new tools and models to enhance our understanding of how higher-order dynamics and fractal patterns affect complex systems by providing additional accurate representations than traditional models. It will be of interest to apply these models to relativistic systems governed by higher-order derivatives and dissipation, and to time-dependent quantum Hamiltonian systems. In fact, the relativistic generalization of the Chirikov–Taylor standard map is based on various aspects, e.g., acceleration of the particle in an electric field [98, 100], dynamics of particles in magnetic relativistic field [101–103], and acceleration of charged particles in the electric field of an electromagnetic wave packet subject to temperature effects [104]. The emergence of chaos and fractal structures in relativistic systems is also considered motivating since these will support physicists and mathematicians to better understand several hidden properties arising in quantum and high energetic Hamiltonian chaos, besides their relevance in ergodic theory [105–107]. Additionally, it will be of interest to study the frequency property of Equation 1 using the one-step frequency formulation for non-linear oscillators introduced in [108], with an emphasis on odd non-linearity. Based on the outcomes of [108], we believe that this approach will offer several additional insights into the dynamics described by Equation 1, mainly related to the variations and effects of the frequency characteristics of the system for different parameter values and their correlations with chaotic and fractal behaviors. Work in these directions will be the aim of our future study.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author contributions

RE-N: conceptualization, investigation, methodology, software, and writing–original draft. WA: resources, validation, and writing–review and editing.

Funding

The author(s) declare that financial support was received for the research, authorship, and/or publication of this article. RE-N has received funding from the Czech National Agency of Agricultural 533 Research, project QK22020134 and from Chiang Mai University.

Acknowledgments

The authors are indebted to the anonymous referees for useful comments and suggestions.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Generative AI statement

The author(s) declare that no Generative AI was used in the creation of this manuscript.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. Goldstein H. Classical mechanics. 3rd ed. San Francisco: Addison-Wesley (2002).

Google Scholar

2. Chirikov BV. A universal instability of many dimensional oscillator systems. Phys Rep (1979) 52(5):263–379. doi:10.1016/0370-1573(79)90023-1

CrossRef Full Text | Google Scholar

3. Zaslavsky GM. Hamiltonian chaos and fractional dynamics. Oxford: Oxford University Press (2005).

Google Scholar

4. van den Berg JB, van der Vorst RCAM. Stable patterns for fourth order parabolic equations. Duke Math J (2002) 115:513–58. doi:10.1215/s0012-7094-02-11534-8

CrossRef Full Text | Google Scholar

5. van der Berg JB. Dynamics and equilibria of fourth order differential equations. Universiteit Leiden, Netherlands (2000) PhD Thesis.

Google Scholar

6. Peletier LA, Troy WC. Spatial patterns: higher order models in physics and mechanics. Boston: Birkhäuser (2001).

Google Scholar

7. Peletier LA, Troy WC, van der Vorst RCAM. Stationary solutions of a fourth order nonlinear diffusion equation. Differential’nye Uravneniya (1995) 31:327–37. Available from: ///C:/Users/user/Downloads/download%20(9).pdf

Google Scholar

8. Coban E, Gahramanov I, Kosva D. Variational symmetries of Lagrangian systems with second-order derivatives. Eur Phys J Plus (2023) 138:605. doi:10.1140/epjp/s13360-023-04241-5

CrossRef Full Text | Google Scholar

9. Buzug T, van Stamm J, Pfister G. Fractal dimensions of strange attractors obtained from the Taylor-Couette experiment. Phys A: Stat Mech and Appl (1992) 191:559–63. doi:10.1016/0378-4371(92)90583-C

CrossRef Full Text | Google Scholar

10. Sakkaravarthi K, Kanna T, Babu Mareeswaran R. Higher-order optical rogue waves in spatially inhomogeneous multimode fiber. Phys D: Nonlinear Phenom (2022) 435:133285. doi:10.1016/j.physd.2022.133285

CrossRef Full Text | Google Scholar

11. Malos JT, Dykstra R, Vaupel M, Weiss CO. Vortex streets in a cavity with higher-order standing waves. Opt Lett (1997) 22:1056–8. doi:10.1364/ol.22.001056

PubMed Abstract | CrossRef Full Text | Google Scholar

12. He JH. A new fractal derivation. Therm Sci (2011) 15:S145–7. doi:10.2298/tsci11s1145h

CrossRef Full Text | Google Scholar

13. He JH. Fractal calculus and its geometrical explanation. Res Phys (2018) 10:272–6. doi:10.1016/j.rinp.2018.06.011

CrossRef Full Text | Google Scholar

14. He JH, Ain QT. New promises and future challenges of fractal calculus: from two-scale thermodynamics to fractal variational principle. Therm Sci (2020) 24:659–81. doi:10.2298/tsci200127065h

CrossRef Full Text | Google Scholar

15. He JH, Liu C. Fractal dimensions of a porous concrete and its effect on the concrete’s strength. Series: Mech Eng (2023) 21:137–50. doi:10.22190/fume221215005h

CrossRef Full Text | Google Scholar

16. He JH, He YQ. A tutorial introduction to the two-scale fractal calculus and its application to the fractal Zhiber-Shabat oscillator. Fractals (2021) 29:2150268. doi:10.1142/s0218348x21502686

CrossRef Full Text | Google Scholar

17. El-Nabulsi RA. Fractal diffusion from a geometric Ricci flow. J Elliptic and Parabolic Equat (2022) 8:837–52. doi:10.1007/s41808-022-00169-6

CrossRef Full Text | Google Scholar

18. El-Nabulsi RA, Anukool W. Foam drainage equation in fractal dimensions: breaking and instabilities. Eur Phys J (2023) E46:110. doi:10.1140/epje/s10189-023-00368-6

PubMed Abstract | CrossRef Full Text | Google Scholar

19. El-Nabulsi RA, Anukool W. Modelling von Bertalanffy growth function of fish with fractals. Hydrobiologia (2024) 851:2543–59. doi:10.1007/s10750-024-05487-5

CrossRef Full Text | Google Scholar

20. El-Nabulsi RA, Anukool W. Spiral waves in fractal dimensions and their elimination in λ − ω systems with less damaging intervention. Chaos, Solitons and Fractals (2024) 178:114317. doi:10.1016/j.chaos.2023.114317

CrossRef Full Text | Google Scholar

21. Mandelbrot BB. The fractal geometry of nature. New York: W. H. Freeman (1982).

Google Scholar

22. Golmankhaneh AK. Fractal calculus and its applications. World Scientific (2022). doi:10.1142/12988

CrossRef Full Text | Google Scholar

23. Cetinkaya FA, Golmankhaneh AK. General characteristics of a fractal Sturm-Liouville problem. Turk J Math (2021) 45:1835–46. doi:10.3906/mat-2101-38

CrossRef Full Text | Google Scholar

24. Golmankhaneh AK, Tunç C, Şevli H. Hyers-Ulam stability on local fractal calculus and radioactive decay. Eur Phys J Spec Top (2021) 230:3889–94. doi:10.1140/epjs/s11734-021-00316-5

CrossRef Full Text | Google Scholar

25. Strichartz RS. Differential equations on fractals. Princeton University Press (2018).

Google Scholar

26. Golmankhaneh AK, Fernandez A. Random variables and stable distributions on fractal cantor sets. Fractal Fract (2019) 3:31. doi:10.3390/fractalfract3020031

CrossRef Full Text | Google Scholar

27. Golmankhaneh AK, Tunç C. Stochastic differential equations on fractal sets. Stochastics (2020) 92:1244–60. doi:10.1080/17442508.2019.1697268

CrossRef Full Text | Google Scholar

28. Golmankhaneh AK, Tunç C. Sumudu transform in fractal calculus. Appl Math Comput (2019) 350:386–401. doi:10.1016/j.amc.2019.01.025

CrossRef Full Text | Google Scholar

29. Golmankhaneh AK, Ali K, Yilmazer R, Kaabar M. Local fractal Fourier transform and applications. Comput Meth Differ Equ (2021) 10:595–607. doi:10.22034/cmde.2021.42554.1832

CrossRef Full Text | Google Scholar

30. Parvate A, Gangal AD. Calculus on fractal subsets of real line–I: formulation. Fractals17 (2009) 17:53–81. doi:10.1142/s0218348x09004181

CrossRef Full Text | Google Scholar

31. Parvate A, Gangal AD. Calculus on fractal subsets of real line–II: conjugacy with ordinary calculus. Fractals (2011) 19:271–90. doi:10.1142/s0218348x11005440

CrossRef Full Text | Google Scholar

32. Golmankhaneh AK, Welch K. Equilibrium and non-equilibrium statistical mechanics with generalized fractal derivatives: a review. Mod Phys Lett (2021) A36:2140002. doi:10.1142/s0217732321400022

CrossRef Full Text | Google Scholar

33. Golmankhaneh AK, Jørgensen PET, Prodanov D, Fractal calculus to derive fractal Frenet equations for fractal curves, arXiv: 2404.07996.

Google Scholar

34. El-Nabulsi RA, Golmankhaneh AK, Agrawal P. On a new generalized local fractal derivative operator. Chaos, Solitons and Fractals (2022) 161:112329. doi:10.1016/j.chaos.2022.112329

CrossRef Full Text | Google Scholar

35. El-Nabulsi RA, Golmankhaneh AK. Generalized heat diffusion equations with variable coefficients and their fractalization from the Black-Scholes equation. Comm Theor Phys (2021) 73:055002. doi:10.1088/1572-9494/abeb05

CrossRef Full Text | Google Scholar

36. El-Nabulsi RA, Golmankhaneh AK. Nonstandard and fractal electrodynamics in Finsler-Randers space. Int J Geom Meth Mod Phys (2022) 19:2250080. doi:10.1142/s0219887822500803

CrossRef Full Text | Google Scholar

37. Deppman A, Megias E, Pasechnik R. Fractal derivatives, fractional derivatives and q-deformed calculus. Entropy (2023) 25:1008. doi:10.3390/e25071008

PubMed Abstract | CrossRef Full Text | Google Scholar

38. El-Nabulsi RA, Anukool W. Fractal dimensions in fluid dynamics and their effects on the Rayleigh problem, the burger’s vortex and the Kelvin–Helmholtz instability. Acta Mech (2022) 233:363–81. doi:10.1007/s00707-021-03128-9

CrossRef Full Text | Google Scholar

39. El-Nabulsi RA, Golmankhaneh AK. Propagation of waves in fractal spaces. Compl Med (2022) 1–23. doi:10.1080/17455030.2023.2184640

CrossRef Full Text | Google Scholar

40. El-Nabulsi RA, Golmankhaneh AK. Dynamics of particles in cold electrons plasma: fractional actionlike variational approach versus fractal spaces approach, Waves Rand. Compl Med (2024) 34:350–71. doi:10.1080/17455030.2021.1909779

CrossRef Full Text | Google Scholar

41. El-Nabulsi RA, Anukool W. Vlasov equation, waves and dispersion relations in fractal dimensions: landau damping and the toroidal ion temperature gradient instability problem. Compl Med (2022) 1–21. doi:10.1080/17455030.2022.2155321

CrossRef Full Text | Google Scholar

42. El-Nabulsi RA. Transition from circular to spiral waves and from Mexican hat to upside-down Mexican hat-solutions: the cases of local and nonlocal lambda-omega reaction-diffusion-convection fractal systems with variable coefficients. Chaos, Solitons and Fractals (2024) 189:115737. doi:10.1016/j.chaos.2024.115737

CrossRef Full Text | Google Scholar

43. El-Nabulsi RA. Modelling nematic liquid crystal in fractal dimensions. Med Nov. Tech. Dev. (2024) 24:100334. doi:10.1016/j.medntd.2024.100334

CrossRef Full Text | Google Scholar

44. El-Nabulsi RA, Anukool W. Schwarzschild spacetime in fractal dimensions: deflection of light, supermassive black holes and temperature effects. Mod Phys Lett (2024) A39:2450124. doi:10.1142/s0217732324501244

CrossRef Full Text | Google Scholar

45. El-Nabulsi RA, Anukool W. Time-dependent heating problem of the solar corona in fractal dimensions: a plausible solution. Adv Space Res (2024) 74:2510–29. doi:10.1016/j.asr.2024.06.015

CrossRef Full Text | Google Scholar

46. El-Nabulsi RA. Thermal transport equations in porous media from product-like fractal measure. J Therm Stresses (2021) 44:899–918. doi:10.1080/01495739.2021.1919585

CrossRef Full Text | Google Scholar

47. El-Nabulsi RA. Fractal Pennes and Cattaneo–Vernotte bioheat equations from product-like fractal geometry and their implications on cells in the presence of tumour growth. J R Soc Interf (2021) 18:20210564. doi:10.1098/rsif.2021.0564

PubMed Abstract | CrossRef Full Text | Google Scholar

48. Chen W. Time-space fabric underlying anomalous diffusion. Chaos, Solitons and Fractals (2006) 28:923–9. doi:10.1016/j.chaos.2005.08.199

CrossRef Full Text | Google Scholar

49. Li J, Ostoja-Starzewski M. Fractal solids, product measures and fractional wave equations. Proc Roy Soc (2009) A465:2521–36. doi:10.1098/rspa.2009.0101

CrossRef Full Text | Google Scholar

50. Li J, Ostoja-Starzewski M. Thermo-poromechanics of fractal media. Phil Trans Roy Soc (2020) A378:20190288. doi:10.1098/rsta.2019.0288

PubMed Abstract | CrossRef Full Text | Google Scholar

51. Ostoja-Starzewski M, Li J, Joumaa H, Demmie PN. From fractal media to continuum mechanics. J Appl Math Mech (2014) 94:373–401. doi:10.1002/zamm.201200164

CrossRef Full Text | Google Scholar

52. Ostoja-Starzewski M. Continuum mechanics models of fractal porous media: integral relations and extremum principles. J Mech Mat Struct (2009) 4:901–12. doi:10.2140/jomms.2009.4.901

CrossRef Full Text | Google Scholar

53. Jiang H, Su W. Some fundamental results of calculus on fractal sets. Comm Nonlinear Sci Numer Simul (1998) 3:22–6. doi:10.1016/s1007-5704(98)90054-5

CrossRef Full Text | Google Scholar

54. Bongiorno D. Derivatives not first return integrable on a fractal set. Ric di Mat (2018) 6:597–604. doi:10.1007/s11587-018-0390-z

CrossRef Full Text | Google Scholar

55. Balankin AS, Mena B. Vector differential operators in a fractional dimensional space, on fractals, and in fractal continua. Chaos Solitons and Fractals (2023) 168:113203. doi:10.1016/j.chaos.2023.113203

CrossRef Full Text | Google Scholar

56. Aerts D, Czachor M, Kuna M. Simple fractal calculus from fractal arithmetic. Rep Math Phys (2018) 81:359–72. doi:10.1016/s0034-4877(18)30053-3

CrossRef Full Text | Google Scholar

57. He J-H. A new fractal derivation. Therm Sci (2011) 15:S145–7. doi:10.2298/tsci11s1145h

CrossRef Full Text | Google Scholar

58. El-Nabulsi RA. Fractal diffusion from a geometric Ricci flow. J Elliptic Parabol Equ (2022) 8:837–52. doi:10.1007/s41808-022-00169-6

CrossRef Full Text | Google Scholar

59. Lu J, Ma L. Analysis of fractal modification of attachment oscillator. Therm Sci (2024) 28:2153–63. doi:10.2298/TSCI2403153L

CrossRef Full Text | Google Scholar

60. Lu J, Shen S, Chen L. Variational approach for time-space fractal Bogoyavlenskii equation. Alex Eng J (2024) 97:294–301. doi:10.1016/j.aej.2024.04.031

CrossRef Full Text | Google Scholar

61. Sun Y, Lu J, Alsolami AA. Numerical analysis of fractional nonlinear vibrations of a restrained cantilever beam with an intermediate lumped mass, J. Low Frequency Noise. Vib and Active Control (2024) doi:10.1177/14613484241285502

CrossRef Full Text | Google Scholar

62. He C-H. A variational principle for a fractal nano/microelectromechanical (N/MEMS) system. Int J Numer Meth Heat and Fluid Flow (2023) 33:351–9. doi:10.1108/hff-03-2022-0191

CrossRef Full Text | Google Scholar

63. Perez Polo MF, Perez Molina M, Chica JG. Chaotic dynamic and control for micro-electro-mechanical systems of massive storage with harmonic base excitation, Chaos. Solitons and Fractals (2009) 39:1356–70. doi:10.1016/j.chaos.2007.06.010

CrossRef Full Text | Google Scholar

64. Buscarino A, Famoso C, Fortuna L, Frasca M. A new chaotic electro-mechanical oscillator. Int J Bifurc Chaos (2016) 26:1650161. doi:10.1142/s0218127416501613

CrossRef Full Text | Google Scholar

65. Defoort M, Rufer L, Fesquet L, Basrour S. A dynamical approach to generate chaos in a micromechanical resonator. Microsyst Nanoeng (2021) 7:17. doi:10.1038/s41378-021-00241-6

PubMed Abstract | CrossRef Full Text | Google Scholar

66. Luo S, Li S, Tajaddodianfar F. Chaos and nonlinear feedback control of the arch micro-electro-mechanical system. J Syst Sci Complex (2018) 31:1510–24. doi:10.1007/s11424-018-7234-5

CrossRef Full Text | Google Scholar

67. Pourmahmood AM. Chaos in a fractional-order micro-electro-mechanical resonator and its suppression. Chin Phys (2012) B21:100505. doi:10.1088/1674-1056/21/10/100505

CrossRef Full Text | Google Scholar

68. DeMartini BE, Butterfield HE, Moehlis J, Foster KL. Chaos for a microelectromechanical oscillator governed by the nonlinear Mathieu equation. J Electromechanical Syst (2007) 16:1314–23. doi:10.1109/jmems.2007.906757

CrossRef Full Text | Google Scholar

69. Park K, Chen Q, Lai Y-C. Energy enhancement and chaos control in microelectromechanical systems. Phys Rev (2008) E77:026210. doi:10.1103/physreve.77.026210

PubMed Abstract | CrossRef Full Text | Google Scholar

70. Tul Ain Q, He J-H. On two-scale dimension and its application. Therm Sci (2019) 23:1707–12. doi:10.2298/TSCI190408138A

CrossRef Full Text | Google Scholar

71. Anjum N, He C-H, He J-H. Two-scale fractal theory for the population dynamics. Fractals (2021) 29:2150182. doi:10.1142/s0218348x21501826

CrossRef Full Text | Google Scholar

72. He J-H, El-Dib YO. A tutorial introduction to the two-scale fractal calculus and its application to the fractal Zhiber-Shabat oscillator. Fractals (2021) 29:2150268. doi:10.1142/s0218348x21502686

CrossRef Full Text | Google Scholar

73. Liu F, Wang S, Nadeem M. A fractal solution of Camassa-Holm and Degasperis-Procesi models under two-scale dimension approach. Fractals (2023) 31:2350053. doi:10.1142/s0218348x23500536

CrossRef Full Text | Google Scholar

74. Yang T, Lai J, Yan W, Zhao Y, Wu Z, Ji Y, et al. Fractal two-scale method for calculating the laser scattering distribution of asphalt pavement. Opt Express (2023) 31:17792–808. doi:10.1364/oe.494025

PubMed Abstract | CrossRef Full Text | Google Scholar

75. Tul Ain Q, He J-H, Qiang X-L, Kou Z. The two-scale fractal dimension: a unifying perspective to metabolic law. Fractals (2024) 32:2450016. doi:10.1142/s0218348x24500166

CrossRef Full Text | Google Scholar

76. Wei C-F. Two-scale transform for 2-D fractal heat equation in a fractal space. Therm Sci (2021) 25:2339–45. doi:10.2298/tsci190918124w

CrossRef Full Text | Google Scholar

77. He J-H, Tul Ain Q. New promises and future challenges for fractal calculus: from two-scale thermodynamics to fractal variational principle. Therm Sci (2020) 24:659–81. doi:10.2298/TSCI200127065H

CrossRef Full Text | Google Scholar

78. Obara R. Two-scale fractal method for characterization of worn surfaces from internal combustion engine cylinder bores. SAE Tech Paper (2018) 36:0014. doi:10.4271/2018-36-0014

CrossRef Full Text | Google Scholar

79. El-Nabulsi RA, Anukool W. On fractal thermodynamics of the superconducting transition and the roles of specific heat, entropy and critical magnetic field in disordered superconductors. Phys C: Superconduct Appl (2023) 611:1354302. doi:10.1016/j.physc.2023.1354302

CrossRef Full Text | Google Scholar

80. He J-H, Ji F-Y. Two-scale mathematics and fractional calculus for thermodynamics. Therm Sci (2019) 23:2131–3. doi:10.2298/tsci1904131h

CrossRef Full Text | Google Scholar

81. Falconer KJ. Fractal geometry-mathematical foundations and applications. New York: Wiley (2003).

CrossRef Full Text | Google Scholar

82. Cetin K, Tirnakli U, Boghosian BM. A generalization of the standard map and its statistical characterization. Sci Rep (2022) 12:8575. doi:10.1038/s41598-022-12213-5

PubMed Abstract | CrossRef Full Text | Google Scholar

83. Mathias AC, Mugnaine M, Santos MS, Szezech JD, Caldas IL, Viana RL. Fractal structures in the parameter space of nontwist area-preserving maps. Phys Rev (2019) E100:052207. doi:10.1103/physreve.100.052207

PubMed Abstract | CrossRef Full Text | Google Scholar

84. Cantrijn F, Crampin M, Sarlet W. Higher-order differential equations and higher-order Lagrangian mechanics. Math Proc Cambridge Philos Soc (1986) 99(3):565–87. doi:10.1017/s0305004100064501

CrossRef Full Text | Google Scholar

85. Galor O. Higher-order and non-autonomous systems, springer books. In: Discrete dynamical systems. Edition 1. Springer (2007) p. 107–13. Chapter 0.

Google Scholar

86. Prieto-Martinez PD, Roman-Roy N. Unified formalism for higher order non-autonomous dynamical systems. J Math Phys (2012) 53(2):032901. doi:10.1063/1.3692326

CrossRef Full Text | Google Scholar

87. Tarasov VE. Differential equations with fractional derivative and universal map with memory. J Phys A: Math Theor (2009) 42:465102. doi:10.1088/1751-8113/42/46/465102

CrossRef Full Text | Google Scholar

88. Tarasov VE, Zaslavsky GM. Fractional equations of kicked systems and discrete maps. J Phys A: Math Theor (2008) 41:435101. doi:10.1088/1751-8113/41/43/435101

CrossRef Full Text | Google Scholar

89. Tomsovic S, Urbina JD, Richter K. Controlling quantum chaos: time-dependent kicked rotor. Phys Rev (2023) E108:044202. doi:10.1103/physreve.108.044202

PubMed Abstract | CrossRef Full Text | Google Scholar

90. Da Costa DR, Livorati ALP, Leonel ED. Critical exponents and scaling properties for the chaotic dynamics of a particle in a time-dependent potential barrier. Int J Bifur Chaos (2012) 22:1250250. doi:10.1142/s0218127412502501

CrossRef Full Text | Google Scholar

91. Da Costa DR, Dettmann CP, Leonel ED. Escape of particles in a time dependent potential well. Phys Rev (2011) E83:066211. doi:10.1103/physreve.83.066211

PubMed Abstract | CrossRef Full Text | Google Scholar

92. Da Costa DR, Caldas IL, Leonel ED. Dynamical properties for an ensemble of classical particles moving in a driven potential well with different time perturbation. Phys Lett (2013) A377:1814–21. doi:10.1016/j.physleta.2013.05.050

CrossRef Full Text | Google Scholar

93. Casati G, Molinari L. Quantum chaos with time-periodic Hamiltonians. Prog Theor Phys Suppl (1989) 98(98):287–322. doi:10.1143/ptps.98.287

CrossRef Full Text | Google Scholar

94. Chaudhury S, Smith A, Anderson BE, Ghose S, Jessen PS. Quantum signatures of chaos in a kicked top. Nature (2009) 461:768–71. doi:10.1038/nature08396

PubMed Abstract | CrossRef Full Text | Google Scholar

95. Jorba-Cuscó M. Periodic time dependent Hamiltonian systems and applications, PhD thesis, department of mathematics and informatics. Barcelona: Universitat de Barcelona (2018).

Google Scholar

96. Haake F, Kus M, Scharf R. Classical and quantum chaos for a kicked top. Z Phys B: Condensed Matter (1987) 65:381–95. doi:10.1007/bf01303727

CrossRef Full Text | Google Scholar

97. Anand A, Davis J, Ghose S. Quantum recurrences in the kicked top. Phys Rev Res (2024) 6:023120. doi:10.1103/physrevresearch.6.023120

CrossRef Full Text | Google Scholar

98. Nomura Y, Ichikawa YH, Horton W. Nonlinear dynamics of the relativistic standard map. Phys Rev (1992) A45:1103–15. doi:10.1103/physreva.45.1103

PubMed Abstract | CrossRef Full Text | Google Scholar

99. del-Castillo-Negrete D, Greene J, Morrison P. Area preserving nontwist maps: periodic orbits and transition to chaos. Phys. (1996) D91:1–23. doi:10.1016/0167-2789(95)00257-x

CrossRef Full Text | Google Scholar

100. Souza FG, Grime GC, Caldas IL, Bidimensional symplectic maps, arXiv: 2301.06526.

Google Scholar

101. de Sousa MC, Steffens FM, Pakter R, Rizzato FB. Standard map in magnetized relativistic systems: fixed points and regular acceleration. Phys Rev (2010) E82:026402. doi:10.1103/physreve.82.026402

PubMed Abstract | CrossRef Full Text | Google Scholar

102. Zugasti MA, Gomez JME, Miro DG-P, Llorente FL, Ranada AF. Chaotic magnetic fields. Chaos, Solitons and Fractals (1994) 4:1943–59. doi:10.1016/0960-0779(94)90009-4

CrossRef Full Text | Google Scholar

103. Khazanov GV, Tel’nikhin AA, Kronberg TK. Chaotic motion of relativistic electrons driven by whistler waves. Plasma Phys Control Fusion (2007) 49:447–66. doi:10.1088/0741-3335/49/4/008

CrossRef Full Text | Google Scholar

104. Ciubotariu C, Bădeliţă L, Stancu V. Chaos in dissipative relativistic standard maps. Chaos, Solitons and Fractals (2002) 13:1253–67. doi:10.1016/s0960-0779(01)00122-9

CrossRef Full Text | Google Scholar

105. Percival IC. Order and chaos in Hamiltonian systems. In: S Lundqvist, NH March, and MP Tosi, editors. Order and chaos in nonlinear physical systems. Physics of solids and liquids. Boston, MA: Springer (1998) doi:10.1007/978-1-4899-2058-4_13

CrossRef Full Text | Google Scholar

106. Buzzi J. Chaos and ergodic theory. In: R Meyers, editor. Encyclopedia of complexity and systems science. New York, NY: Springer (2009) doi:10.1007/978-0-387-30440-3_64

CrossRef Full Text | Google Scholar

107. Eckmann J-P, Ruelle D. Ergodic theory of chaos and strange attractors. Rev Mod Phys (1985) 57:617–56. doi:10.1103/revmodphys.57.617

CrossRef Full Text | Google Scholar

108. He J-H. Frequency formulation for nonlinear oscillators (part 1). Sound and Vibration (2024) 59:1687. doi:10.59400/sv1687

CrossRef Full Text | Google Scholar