- 1Institute of Space Science and Applied Technology, Harbin Institute of Technology, Shenzhen, China
- 2Shenzhen Key Laboratory of Numerical Prediction for Space Storm, Institute of Space Science and Applied Technology, Harbin Institute of Technology, Shenzhen, China
- 3Key Laboratory of Solar Activity and Space Weather, National Space Science Center, Chinese Academy of Sciences, Beijing, China
- 4Mengcheng National Geophysical Observatory, University of Science and Technology of China (or Anhui Earthquake Agency), Mengcheng, China
Electron firehose instabilities can be excited at dipolarization fronts and in the magnetic reconnection outflow in the terrestrial magnetotail, but their occurrence rate in the plasma sheet is unclear. Here, we investigate the characteristics of electron firehose unstable conditions in the magnetotail plasma sheet based on observations of the Magnetospheric Multiscale mission. We find an Alfvénic magnetic field fluctuation accompanied by a strong field-aligned current during a flapping motion. This fluctuation occurs where the local plasma is electron firehose unstable, indicating that the electron firehose instability in the plasma sheet can occur in the region besides dipolarization fronts and magnetic reconnection outflow. We statistically find that the local plasma near the neutral sheet has a small probability with the maximum value <1.4% to be electron firehose unstable, which mainly occurs in the central plasma sheet with BXY/BL < 0.3. The maximum probability of Tef > 0 (electron firehose unstable condition) is ∼1.36% (1.32%) at BXY/BL ≈ 0.05 (0.15) during fast (non-fast) flows. During fast flows, the plasma near the neutral sheet tends to have a higher probability of Tef > 0 when the local VT is larger. During non-fast flows, the plasma near the neutral sheet tends to have a higher probability of Tef > 0 when Te is larger. The probability of Tef > 0 shows a dawn-dusk asymmetry during fast flows and non-fast flows. In addition, the probability of Tef > 0 during fast flows tends to be larger when the ambient BZ is weak, which shows opposite characteristics during non-fast flows. These findings help to assess the importance of the role of electron firehose instabilities in the magnetotail plasma sheet.
1 Introduction
Fast flows are essential to the transport of mass, magnetic flux, and energy in the terrestrial magnetotail [1, 2]. They might originate from magnetic reconnections [3, 4] or interchange instabilities [5]. Temperature anisotropies can be caused during fast flows [6, 7], which are able to provide free energy to excite various instabilities [8–11]. For example, ion firehose instabilities can be driven when Ti,|| > Ti,⊥, where Ti,|| and Ti,⊥ are the parallel and perpendicular ion temperatures with respect to the ambient magnetic field [10, 11]. Such instabilities also exist in the solar wind [12–15] and the terrestrial magnetosheath [16, 17].
Ion firehose instabilities include parallel and oblique modes [10, 11, 18–20]. In the terrestrial magnetotail, parallel firehose instabilities are more likely to occur near the neutral sheet [21], and can generate Pi2-band (40–150 s) Alfvénic fluctuations during fast flows [22, 23]. [24] further found that the parallel firehose unstable condition can affect the wave power of the Pi1-band (10–40 s) and Pi2-band fluctuations during fast flows. The probability of the plasma being parallel firehose unstable condition tends to be larger for the faster flow, and is positively correlated with the wave power of the Pi1/2-band fluctuations [24]. Oblique firehose instabilities can generate compressional fluctuations, which are linear-polarized and have a zero frequency [10]. A flapping motion of the current sheet was reported to might originate from the oblique firehose instability during a fast flow [19]. Later, [25] statistically found that both probabilities of the fast flows accompanied by large-amplitude neutral sheet oscillations and the plasma being oblique firehose unstable condition near the neutral sheet tend to be larger for faster flows. In addition, the oblique firehose unstable condition can affect the period of these oscillations. These results support that oblique firehose instabilities are a generation mechanism of some flapping motions [25].
Similar to ions, electron firehose instabilities driven by electron temperature anisotropy also have two modes based on linear theory and 2D Particle-In-Cell (PIC) simulation [26–29]. The parallel electron firehose mode is a parallel propagation with respect to the ambient magnetic field and is non-resonant with respect to electrons, while the oblique electron firehose mode is characterized by a lower instability threshold and higher growth rate, which is a non-propagated and is resonant with both electrons and ions [27, 29]; [26, 30, 31]. In the magnetotail, electron firehose instabilities can be excited at dipolarization fronts [32] and in the magnetic reconnection outflow [31]. These instabilities are believed to lead the electron to isotropization by cooling (heating) the electron in the parallel (perpendicular) direction with respect to the ambient field [31–33]. The magnetotail current sheet can become thin to the sub-ion scale [34, 35]. Energy conversion processes take place in the thin current sheet, where the anisotropic electrons can excite electron-dominated instabilities [34–36]. The occurrence rate of electron firehose instabilities helps to evaluate their impact on electrons in the plasma sheet, however, it is still unclear.
In this study, we statistically investigate the electron firehose unstable conditions in the plasma sheet using the data obtained from the Magnetospheric Multiscale (MMS) mission. We first show a magnetic field fluctuation event associated with the electron firehose instability in the plasma sheet, then statistically analyze the probability of the plasma being electron firehose unstable during fast flows and non-fast flows.
2 Observation
The MMS spacecraft, launched on March 2015, consists of four identical probes with an interspacecraft distance of 10–400 km [37]. In the present study, only the magnetic field and plasma data of the MMS1 probe from 2015 to 2022 are used without other statement since the interspacecraft distances among the probes are very small compared with the thickness of the plasma sheet. The used magnetic field data with a resolution of 16 Hz are from the fluxgate magnetometer (FGM) instrument [38], and the used plasma moment data with a resolution of 4.5 s are from the Fast Plasma Investigation (FPI) instrument [39].
2.1 An event associated with electron firehose instabilities
Figure 1 shows the magnetic field and ion moments observed by MMS1 between 15:00 and 16:00 UT on 20 July 2017. The MMS1 probe is located at [-23.3, 7.0, 3.0] RE in the geocentric solar magnetospheric (GSM) coordinate system at 15:30 UT. The ion beta βi, ratio of the ion thermal pressure to the magnetic pressure, is >0.5 during the whole interval, indicating that this probe is in the plasma sheet [40]. Figure 1A shows that BX had a maximum variation from ∼9.4 nT to −21 nT in the interval of 15:21–15:33 UT, and the sign of BX has one reversal. Such a large variation of BX meets the expectation of a flapping motion of the current sheet [41–43]. At ∼15:24:20 UT, BX suddenly changes from ∼4 nT to −4 nT with almost unchanged of BT. Figure 1D, E show that Vi,X and Vi,Y are dominant and the maximum value of the total ion velocity is ∼216.9 km/s, suggesting that the sudden change of BX occurs during a weak fast flow.
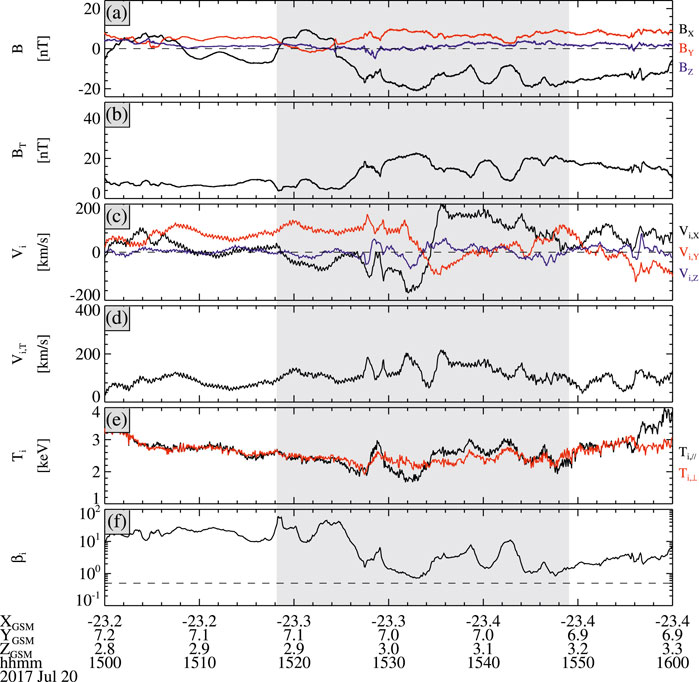
Figure 1. A fast flow event observed by MMS1 between 15:00 and 16:00 UT on 20 July 2017. From top to bottom: (A) the magnetic field in GSM, (B) the magnetic field strength, (C) ion velocities in GSM, (D) the total ion velocity, (E) the parallel (black) and perpendicular (red) ion temperatures, (F) ion beta. The gray region indicates the interval of a fast flow event.
Timing analysis can be used to determine the propagation velocity along the normal direction of a one-dimensional current sheet [44, 45]. Assuming that the magnetic field fluctuation between 15:24:10 and 15:24:30 UT is one-dimensional, its propagation velocity is ∼39.7 km/s determined by timing analysis. Thus, its length is ∼794 km along the normal direction. At this time, the local ion gyroradius ρi is ∼1001.9 km estimated by using the ambient ion temperature (∼2.37 keV) and BT (∼4.96 nT). Thus, the size of the magnetic field fluctuation is ∼0.79 ρi along the normal direction, indicating that this fluctuation is sub-ion scale.
Figure 2 shows the electron moments in the interval of 15:23–15:26 UT. BX changes up to ∼9.3 nT between 15:24:10 and 15:24:30 UT, while BT is almost a constant, indicating that this fluctuation has an Alfvénic characteristic. The electron number density, velocities, and perpendicular temperature (Te,⊥) have no significant change during the whole interval in Figure 2, while the parallel electron temperature (Te,||) has a significant change. Te,|| is > Te,⊥ between 15:23 and 15:25 UT. The electron temperature anisotropy can excite electron firehose instabilities [27, 31, 33]. Based on the linear dispersion theory, the threshold of electron firehose instabilities is derived to be Tef =
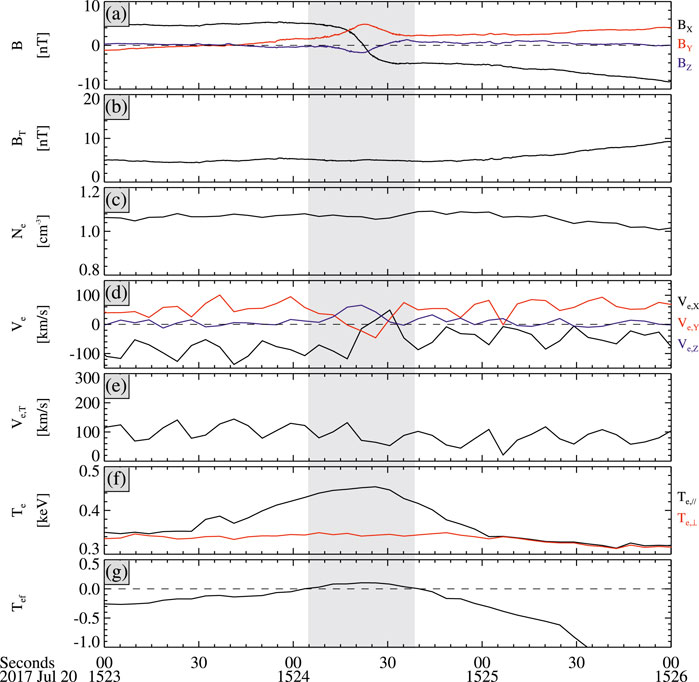
Figure 2. From top to bottom: (A) the magnetic field in GSM, (B) the magnetic field strength, (C) electron number density, (D) electron velocities in GSM, (E) the total electron velocity, (F) the parallel (blue) and perpendicular (red) electron temperatures, (G) the threshold of the electron firehose instability. The gray region indicates the interval of the magnetic field structure.
Figure 3 shows the current density between 15:23 and 15:26 UT, which is calculated by the curlometer technique [46]. It is regarded as reliable when the ratio of |∇·B| to |∇×B| is <0.2 [43, 47]. Thus, the current density is reliable in the interval 15:24:11.2–15:24:25.1 UT. The total current density tends to be larger with the maximum value of ∼26.2 nA/m2 when the MMS1 probe is closer to the neutral sheet. As shown in the shaded area, the parallel current density is dominant in this interval, indicating that the magnetic field fluctuation is accompanied by a strong field-aligned current. Field-aligned currents play a significant role in the process of the ionosphere-magnetosphere coupling [48–51]. In addition, electron firehose instabilities can cause the electron to be isotropic [31, 32]. To evaluate the importance of electron firehose instabilities in the plasma sheet, a question is raised, namely, what is the probability of the plasma being electron firehose unstable in the plasma sheet.

Figure 3. (A) The magnetic field in GSM, (B) the parallel and perpendicular components of the current density, (C) the total current density and (D) the ratio of |∇·B| to |∇×B| between 15:23 and 15:26 UT.
2.2 Electron firehose unstable conditions during fast flows and non-fast flows
To figure out the details of the electron firehose unstable conditions during fast flows and non-fast flows in the plasma sheet, we first select the fast flow events at XGSM < −10 RE and |YGSM| < 12 RE using the following criteria, which are modified based on the selection criteria of bursty bulk flows proposed by Angelopoulos et al. [40]. A fast flow event is defined to be a segment of the continuous ion flow with a magnitude of |Vi| ≥ 100 km/s, during which |Vi| exceeds 150 km/s at least one sample. If two adjacent events are observed within 2 min, they are regarded to belong to the same fast flow event. In total, 5,675 fast flow events are selected.
Figure 4 shows the distribution of the data points in the space of (βe,||, Te,||/Te,⊥) during the fast flows (a) and non-fast flows (b), where both the bin sizes of the logarithm of βe,|| and Te,||/Te,⊥ are 0.02. About ∼79.3% (84.8%) of the data points are observed at Te,||/Te,⊥ > 1 during the fast flows (non-fast flows). The gray dashed line denotes the threshold of the electron firehose instability, i.e.,
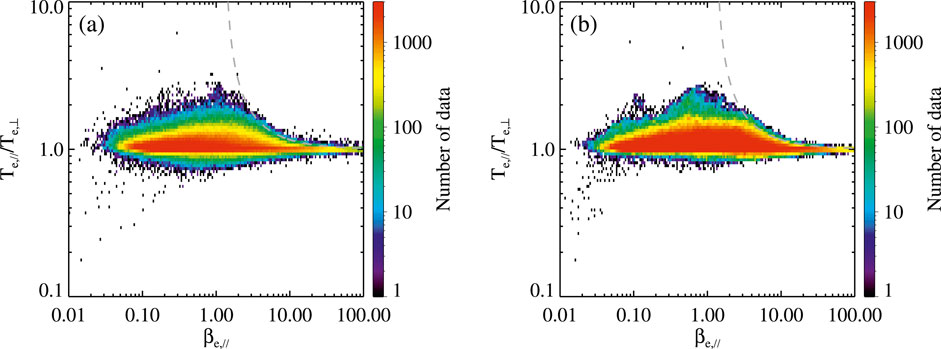
Figure 4. Number of data points in the space of (βe,||, Te, ||/Te,⊥) during the fast flows (A) and non-fast flows (B). The color bar denotes the number of data points. The gray dashed line in each panel denotes
In the magnetotail, firehose instabilities are believed to be more likely to occur near the neutral sheet [21, 24, 52]. We consider the parameter BXY/BL as the relative distance away from the neutral sheet [53], where BXY =
Figure 5 shows the percentages of 2 ≤ βe,|| ≤ 25 (a) and Tef > 0 (b) at different values of (BX/|BX|)⋅BXY/BL with a step length of 0.05 during all the fast flows (black) and non-fast flows (orange). In Figure 5A, the percentage in each bin is determined by the data counts with 2 ≤ βe,|| ≤ 25 divided by the total counts in that bin. (BX/|BX|)⋅BXY/BL < (>) 0 denotes that the satellite is located on the south (north) side of the neutral sheet. One can find that the electrons with 2 ≤ βe,|| ≤ 25 mainly occur in the region within BXY/BL < 0.3. The percentages of 2 ≤ βe,|| ≤ 25 during the fast flows are approximately symmetrically distributed relative to (BX/|BX|)⋅BXY/BL = 0, and have the maximum value at BXY/BL ≈ 0.15 instead of BXY/BL = 0. The characteristics of the percentages of 2 ≤ βe,|| ≤ 25 during the non-fast flows are similar to those during the fast flows. Since the threshold of the electron firehose instability Tef (=
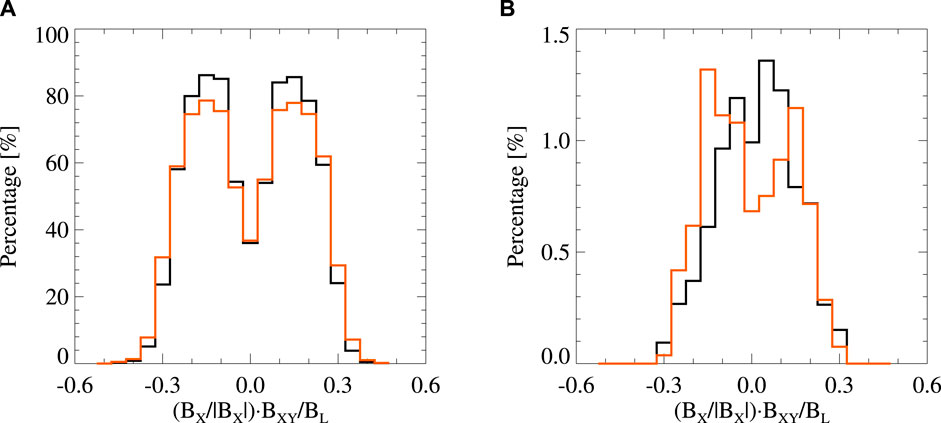
Figure 5. Percentages of 2 ≤ βe,|| ≤ 25 (A) and Tef > 0 (B) at different values of (BX/|BX|)⋅BXY/BL during all the fast flows (black) and non-fast flows (orange). The step length of (BX/|BX|)⋅BXY/BL is 0.05.
Figure 5B shows that the percentages of Tef > 0 during the fast flows (non-fast flows) have the maximum value at BXY/BL ≈ 0.05 (0.15). In each bin, the percentage is determined by the data counts with Tef > 0 and 2 ≤ βe,|| ≤ 25 divided by the total counts with 2 ≤ βe,|| ≤ 25 in that bin. The maximum percentage of Tef > 0 during the fast flows is ∼1.36%. And the plasma during the fast flows tends to have a higher probability of Tef > 0 when closer to the neutral sheet. By contrast, the percentage of Tef > 0 during the non-fast flows has the maximum value of ∼1.32% at BXY/BL ≈ 0.15.
Figure 6 shows the percentages of Tef > 0 during the fast flows at different values of (BX/|BX|)⋅BXY/BL under different conditions of the local ion speed VT. At BXY/BL < 0.05, the maximum percentages of Tef > 0 are ∼1.23%, 1.45%, and 1.86% when VT is in the range of <100 km/s, 100–400 km/s, and >400 km/s, respectively, indicating that the plasma near the neutral sheet tends to have a slightly higher probability of being electron firehose unstable with the increase of the local VT. The percentage of Tef > 0 has the maximum value at BXY/BL ≈ 0.05 when VT < 100 km/s. Under the condition of the local VT > 400 km/s, the percentage of Tef > 0 has the maximum value at BXY/BL ≈ 0.1. Obviously, the local VT can affect the electron firehose unstable conditions during fast flows.

Figure 6. Percentages of Tef > 0 at different values of (BX/|BX|)⋅BXY/BL during the fast flows when the local VT is in the range of <100 km/s (black), 100–400 km/s (cyan), and >400 km/s (orange), respectively.
Figure 7 shows the percentages of Tef > 0 during the fast flows (a) and non-fast flows (b) at different values of (BX/|BX|)⋅BXY/BL under different conditions of the electron number density Ne. Figure 7A shows that the percentage of Tef > 0 has no significant change when Ne is in different range during the fast flows. As shown in Figure 7B, the maximum percentages of Tef > 0 are ∼2.58%, 1.07%, and 1.31% at BXY/BL < 0.2 when Ne is in the range of <0.2 cm−3, 0.2–0.4 cm-3, and >0.4 cm−3 during the non-fast flows, respectively. Under the condition of Ne < 0.2 cm−3, the percentage of Tef > 0 has the maximum value at BXY/BL ≈ 0.15.

Figure 7. Percentages of Tef > 0 at different values of (BX/|BX|)⋅BXY/BL during the fast flows (A) and non-fast flows (B) when Ne is in the range of <0.2 cm−3 (black), 0.2–0.4 cm−3 (cyan), and >0.4 cm−3 (orange), respectively.
Figure 8 shows the percentages of Tef > 0 during the fast flows (a) and non-fast flows (b) under different conditions of the electron temperature Te. During the fast flows, the percentage of Tef > 0 has no significant change when Te is in the range of <0.8 keV, 0.8–1.4 keV, and >1.4 keV, respectively. During the non-fast flows, the maximum percentages of Tef > 0 are ∼0.97%, 1.51%, and 1.89% at BXY/BL < 0.15 when Te is in the range of <0.8 keV, 0.8–1.4 keV, and >1.4 keV, respectively, indicating that the plasma in this region tends to have a slightly higher probability of being electron firehose unstable with the increase of Te. Under the condition of Te > 0.8 keV, the percentage of Tef > 0 has the maximum value at BXY/BL ≈ 0.15.
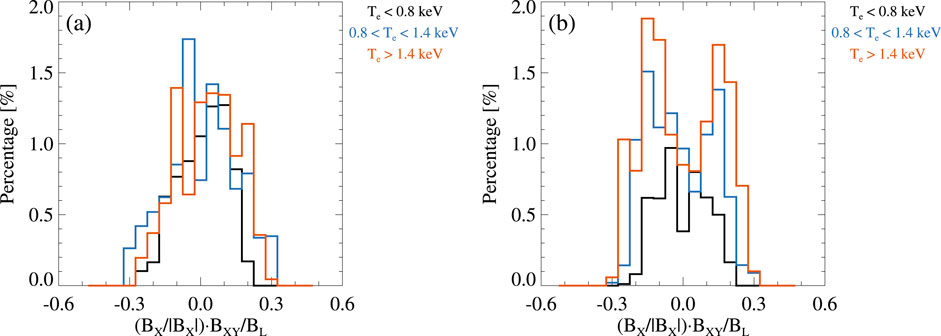
Figure 8. Percentages of Tef > 0 at different values of (BX/|BX|)⋅BXY/BL during the fast flows (A) and non-fast flows (B) when Te is in the range of < 0.8 keV (black), 0.8–1.4 keV (cyan), and >1.4 keV (orange), respectively.
According to the distribution of the percentages of Tef > 0 in Figure 5, one can find that electron firehose instabilities are more likely to be excited at BXY/BL < 0.3 during the fast flows and non-fast flows. Next, we only analyze the characteristics of Tef > 0 within BXY/BL < 0.3 in Figure 9 as well as in Figure 10. Figure 9A shows that the percentages of Tef > 0 are ∼0.65%, 0.90% and 0.66% (0.67%, 0.86% and 0.79%) during the fast flows (non-fast flows) at −15 < XGSM < −10 RE, −20 < XGSM < −15 RE and −30 < XGSM < −20 RE, respectively. The percentages of Tef > 0 at −20 < XGSM < −15 RE is somewhat larger than that at −15 < XGSM < −10 RE and −30 < XGSM < −20 RE. Figure 9B shows the percentages of Tef > 0 are ∼0.80%, 0.74%, and 0.55% (0.87%, 1.38% and 0.38%) during the fast flows (non-fast flows) at 4 < YGSM < 12 RE, −4 < YGSM < 4 RE and −12 < YGSM < −4 RE, respectively. This suggests that both electron firehose unstable conditions during the fast flows and non-fast flows have a dawn-dusk asymmetry.
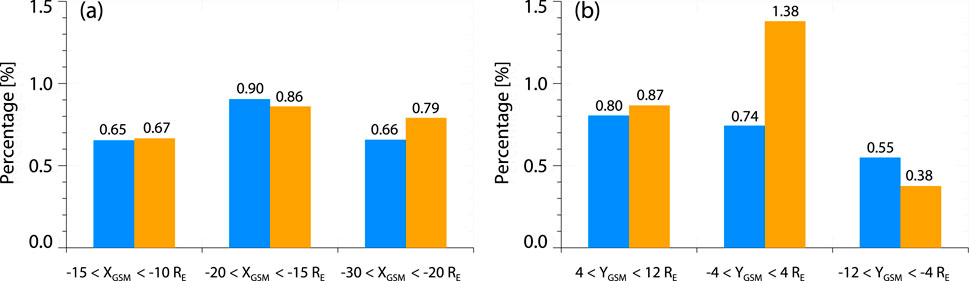
Figure 9. (A) Percentages of Tef > 0 within BXY/BL < 0.3 during the fast flows (blue) and non-fast flows (orange) at −15 < XGSM < −10 RE, −20 < XGSM < −15 RE and −30 < XGSM < −20 RE, respectively. (B) Percentages of Tef > 0 within BXY/BL < 0.3 during the fast flows (blue) and non-fast flows (orange) at 4 < YGSM < 12 RE, −4 < YGSM < 4 RE and −12 < YGSM < −4 RE, respectively.
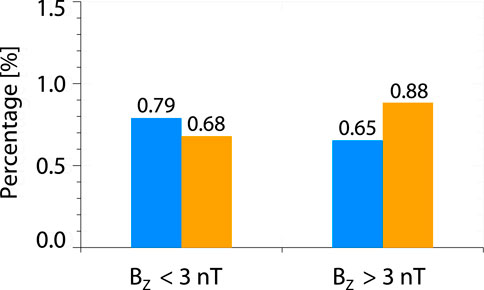
Figure 10. Percentages of Tef > 0 under the condition of BXY/BL < 0.3 during the fast flows (blue) and non-fast flows (orange) when the ambient BZ < 3 nT and BZ > 3 nT, respectively.
We regard the smoothed BZ with a temporal window of 20 min as the ambient BZ. Figure 10 shows that the percentages of Tef > 0 during the fast flows (blue) are ∼0.79% and 0.65% when the ambient BZ is <3 nT and >3 nT, respectively. This indicates that the probability of the electron firehose unstable condition is somewhat larger when the ambient BZ is <3 nT than that when the ambient BZ is >3 nT. One may expect that the waves generated by electron firehose instabilities during fast flows are more likely to occur during the stretch process of the plasma sheet than that during the dipolarization process. By contrast, the percentages of Tef > 0 during the non-fast flows (orange) are ∼0.68% and 0.88% when BZ is <3 nT and >3 nT. This indicates that the ambient BZ has an opposite effect on the electron firehose unstable condition during the non-fast flows.
3 Summary and discussion
Using the MMS1 data from 2015 to 2022, we investigate the electron firehose unstable condition in the magnetotail plasma sheet. Our findings are as follows:
a. A magnetic field fluctuation accompanied by a field-aligned current is found during a flapping motion. The fluctuation occurs near the neutral sheet, where the local plasma is electron firehose unstable, suggesting that this fluctuation might be generated by the electron firehose instability.
b. According to the theory of [27], the plasma being electron firehose unstable (Tef > 0) mainly occurs within BXY/BL < 0.3. The probability of the plasma with Tef > 0 tends to be larger with a maximum value of ∼1.36% when closer to the neutral sheet during the fast flows. By contrast, the maximum probability is ∼1.32% at BXY/BL ≈ 0.15 during the non-fast flows.
c. During the fast flows, the plasma near the neutral sheet tends to have a higher probability of Tef > 0 when the local VT is larger. During non-fast flows, the plasma near the neutral sheet tends to have a higher probability of Tef > 0 when Te is larger.
d. Within BXY/BL < 0.3, the probability of Tef > 0 shows a dawn-dusk asymmetry during the fast flows as well as during the non-fast flows. During the fast flows, the probability of Tef > 0 is larger when the ambient BZ is <3 nT than that when the ambient BZ is >3 nT, which shows opposite characteristics during the non-fast flows.
Flapping motions are a large movement of the current sheet in the north-south direction [41, 43]. Field-aligned currents are reported to occur near the neutral sheet during flapping motions [54, 55]. Some flapping motions can create Pi2 (period: 40–150 s) pulsations on the ground via field-aligned currents flowing into the ionosphere along the magnetic field line [42, 56]. During flapping motions, the current carriers of the current density are dominant by electrons [55], and some field-aligned currents can be explained by the chaotic motion of electrons near the neutral sheet [51]. So far, the origin of the field-aligned current during flapping motions is still not fully understood. Figure 1 shows a magnetic field fluctuation observed at the neutral sheet during a flapping motion. We find that this fluctuation is sub-ion scale, and accompanied by a strong field-aligned current. This sub-ion scale fluctuation is Alfvénic, and occurs in the region where the local plasma is electron firehose unstable. These results suggest that this Alfvénic fluctuation is possibly generated by the electron firehose instability, which might be the origin of the field-aligned current during the flapping motion in Figure 1.
In the central thin current sheet, electrons have a weak temperature anisotropy with Te,||/Te,⊥ ≈ 1.06, and Te,||/Te,⊥ is mainly in the range of 1–1.2 [57]. Here, we mainly focus on the electrons with 2 ≤ βe,|| ≤ 25, which mainly occur at BXY/BL < 0.3. The average Te,||/Te,⊥ of these electrons during the fast (non-fast) flows is ∼1.07 (1.09), and ∼61.6% (62.6%) of these electrons have the value of Te,||/Te,⊥ in the range of 1–1.2. Our findings suggest that the electrons at the central current sheet have a weak parallel temperature anisotropy regardless of whether the current sheet is thin or not. Although the probability of Te,||/Te,⊥ > 1 for the electrons with 2 ≤ βe,|| ≤ 25 is up to ∼73.4% (78.9%) during the fast (non-fast) flows, these electrons have a very low probability of being firehose unstable (see Figure 4).
Fast flows can cause plasma temperature anisotropies to excite various instabilities, such as mirror instabilities and ion firehose instabilities [10, 11, 20]. Similar to ions, the plasma near the neutral sheet has the maximum probability of being electron firehose unstable overall during fast flows (see Figure 5). Thus, one may expect that instabilities in the plasma sheet are more likely to occur during fast flows. However, Figure 5B shows that the probability of the plasma being electron firehose unstable during the fast flows is very close to that during the non-fast flows. This suggests that fast flows have no significant contribution to the excitation of electron firehose instabilities in the plasma sheet. The plasma tends to have a slightly higher probability of being electron firehose unstable with the increase of Te during the non-fast flows (see Figure 8). According to the definition of Tef, Tef tends to be larger with the increase of Te if we assume that the other plasma parameters are constant. This might explain why the electrons during the non-fast flows tend to have a higher probability of being firehose unstable when Te is larger.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Author contributions
JW: Writing–original draft. GW: Writing–review and editing. PZ: Writing–review and editing.
Funding
The author(s) declare that financial support was received for the research, authorship, and/or publication of this article. This work was supported by Key-Area Research and Development Program of Guangdong Province (2020B0303020001), NSFC (42130204, 42241155), the Guangdong Basic and Applied Basic Research Foundation (Grant Nos 2019A1515011067, 2023B1515040021, 2022A1515011698, and 2023A1515030132), Shenzhen Natural Science Fund (the Stable Support Plan Program GXWD20201230155427003-20200822192703001), Shenzhen Science and Technology Research Program (JCYJ20210324121412034, JCYJ20210324121403009), National Key Research and Development Program of China (grant No. 2022YFF0503904), Shenzhen Key Laboratory Launching Project (ZDSYS20210702140800001), the Fundamental Research Funds for the Central Universities (HIT.OCEF.2022041), and Joint Open Fund of Mengcheng National Geophysical Observatory (No. MENGO-202315).
Acknowledgments
We acknowledge the data from the NASA MMS mission. We also acknowledge MMS project FGM and FPI teams.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Angelopoulos V, Coroniti FV, Kennel CF, Kivelson MG, Walker RJ, Russell CT, et al. (1997) Correction to Multipoint analysis of a bursty bulk flow event on April 11, 1985. J Geophys Res Space Phys 102(A1):211–2. doi:10.1029/96JA03217
2. Cao JB, Ma YD, Parks G, Reme H, Dandouras I, Nakamura R, et al. (2006) Joint observations by Cluster satellites of bursty bulk flows in the magnetotail. J Geophys Res 111(A4):A04206. doi:10.1029/2005JA011322
3. Machida S, Miyashita Y, Ieda A, Nishida A, Mukai T, Saito Y, et al. (1999). GEOTAIL observations of flow velocity and north-south magnetic field variations in the near and mid-distant tail associated with substorm onsets. Geophys Res Lett 26(6):635–8. doi:10.1029/1999GL900030
4. Nagai T, Machida S. Magnetic reconnection in the near-Earth magnetotail (1998) Geophys Monograph-American Geophys Union 105:211–24. doi:10.1029/GM105p0211
5. Chen CX, Wolf RA (1993) Interpretation of high-speed flows in the plasma sheet. J Geophys Res 98(A12):21409–19. doi:10.1029/93JA02080
6. Kim H-S, Lee D-Y, Ohtani S-I, Lee E-S, Ahn B-H (2010) Some statistical properties of flow bursts in the magnetotail. J Geophys Res Space Phys 115(A12):A12229. doi:10.1029/2009JA015173
7. Runov A, Angelopoulos V, Sitnov M, Sergeev VA, Nakamura R, Nishimura Y, et al. (2011) Dipolarization fronts in the magnetotail plasma sheet. Planet Space Sci 59(7):517–25. doi:10.1016/j.pss.2010.06.006
8. Gary SP, Fuselier SA, Anderson BJ (1993) Ion anisotropy instabilities in the magnetosheath. J Geophys Res Space Phys 98(A2):1481–8. doi:10.1029/92JA01844
9. Hasegawa A. Drift mirror instability in the magnetosphere. (1969) Phys Fluids 12(12):2642–50. doi:10.1063/1.1692407
10. Hellinger P, Matsumoto H. New kinetic instability: oblique Alfvén fire hose (2000) J Geophys Res Space Phys 105(A5):10519–26. doi:10.1029/1999JA000297
11. Quest KB, Shapiro VD. (1996) Evolution of the fire-hose instability: linear theory and wave-wave coupling. J Geophys Res Space Phys 101(A11):24457–69. doi:10.1029/96JA01534
12. Hellinger P, Trávníček P, Kasper JC, Lazarus AJ. (2006) Solar wind proton temperature anisotropy: linear theory and WIND/SWE observations. Geophys Res Lett 33(9):2006GL025925. doi:10.1029/2006GL025925
13. Hellinger P, Trávníček P. Parallel and oblique proton fire hose instabilities in the presence of alpha/proton drift: hybrid simulations (2006) J Geophys Res Space Phys 111(A1):2005JA011318. doi:10.1029/2005JA011318
14. Markovskii SA, Vasquez BJ. The effect of solar wind turbulence on parallel and oblique firehose instabilities (2022) Astrophysical J 924(2):111. doi:10.3847/1538-4357/ac3754
15. Opie S, Verscharen D, Chen CHK, Owen CJ, Isenberg PA (2022) Conditions for proton temperature anisotropy to drive instabilities in the solar wind. Astrophysical J 941(2):176. doi:10.3847/1538-4357/ac982f
16. DeWeese H, Maruca BA, Qudsi RA, Chasapis A, Pultrone M, Johnson E, et al. (2022) Alpha particle temperature anisotropy in earth’s magnetosheath. Astrophysical J 941(1):12. doi:10.3847/1538-4357/ac9791
17. Maruca BA, Chasapis A, Gary SP, Bandyopadhyay R, Chhiber R, Parashar TN, et al. (2018) MMS observations of beta-dependent constraints on ion temperature anisotropy in earth’s magnetosheath. Astrophysical J 866(1):25. doi:10.3847/1538-4357/aaddfb
18. Astfalk P, Jenko F. Parallel and oblique firehose instability thresholds for bi-kappa distributed protons (2016) J Geophys Research-Space Phys 121(4):2842–52. doi:10.1002/2015ja022267
19. Wang C, Liu Y, Xing X, Runov A, Artemyev A, Zhang X. An event study of simultaneous earthward and tailward reconnection exhaust flows in the earth’s midtail (2020) J Geophys Res Space Phys 125(6):e2019JA027406. doi:10.1029/2019JA027406
20. Wu M, Volwerk M, Lu Q, Voeroes Z, Nakamura R, Zhang T. The proton temperature anisotropy associated with bursty bulk flows in the magnetotail (2013) J Geophys Research-Space Phys 118(8):4875–83. doi:10.1002/jgra.50451
21. Treumann RA, Baumjohann W. Advanced space plasma physics. London, United Kingdom: Imperial College Press (2001). p. 51–2.
22. Horton W, Xu BY, Wong HV (2004) Firehose driven magnetic fluctuations in the magnetosphere. Geophys Res Lett 31(6):L06807. doi:10.1029/2003GL018309
23. Horton W, Xu BY, Wong HV, Van Dam JW. Nonlinear dynamics of the firehose instability in a magnetic dipole geotail (2004) J Geophys Research-Space Phys 109(A9):A09216. doi:10.1029/2003JA010288
24. Wei J, Wang G, Zuo P. Statistical study of the relationship between pi1/2-band wave powers and firehose instability criterion during fast flows in the magnetotail plasma sheet (2022) J Geophys Res Space Phys 127(11):e2022JA030567. doi:10.1029/2022JA030567
25. Wei J, Wang G, Zuo P. Study of the relationship between large-amplitude neutral sheet oscillations and oblique firehose instabilities during fast flows in the terrestrial magnetotail (2024). Phys Fluids 36(5):056616. doi:10.1063/5.0206101
26. Camporeale E, Burgess D. Electron firehose instability: kinetic linear theory and two-dimensional particle-in-cell simulations (2008) J Geophys Res 113(A7):A07107. doi:10.1029/2008JA013043
27. Gary SP, Nishimura K (2003) Resonant electron firehose instability: particle-in-cell simulations. Phys Plasmas 10(9):3571–6. doi:10.1063/1.1590982
28. Hellinger P, Trávníček PM, Decyk VK, Schriver D (2014) Oblique electron fire hose instability: particle-in-cell simulations. J Geophys Res Space Phys 119(1):59–68. doi:10.1002/2013JA019227
29. Li X, Habbal SR. Electron kinetic firehose instability. J Geophys Res (2000) 105(A12):27377–85. doi:10.1029/2000JA000063
30. López RA, Micera A, Lazar M, Poedts S, Lapenta G, Zhukov AN, et al. (2022) Mixing the solar wind proton and electron scales. Theory and 2d-PIC simulations of firehose instability. Astrophysical J 930(2):158. doi:10.3847/1538-4357/ac66e4
31. Cozzani G, Khotyaintsev YV, Graham DB, André M (2023) Direct observations of electron firehose fluctuations in the magnetic reconnection outflow. J Geophys Res Space Phys 128(5):e2022JA031128. doi:10.1029/2022JA031128
32. Zhang X, Angelopoulos V, Artemyev AV, Liu J (2018) Whistler and electron firehose instability control of electron distributions in and around dipolarizing flux bundles. Geophys Res Lett 45(18):9380–9. doi:10.1029/2018GL079613
33. Artemyev AV, Angelopoulos V, Vasko IY, Petrukovich AA, Runov A, Saito Y, et al. (2020) Contribution of anisotropic electron current to the magnetotail current sheet as a function of location and plasma conditions. J Geophys Res Space Phys 125(1):e2019JA027251. doi:10.1029/2019JA027251
34. Nakamura R, Baumjohann W, Fujimoto M, Asano Y, Runov A, Owen CJ, et al. (2008) Cluster observations of an ion-scale current sheet in the magnetotail under the presence of a guide field. J Geophys Res Space Phys 113(A7):2007JA012760. doi:10.1029/2007JA012760
35. Zelenyi LM, Malova HV, Leonenko MV, Grigorenko EE, Popov VY (2022) Equilibrium configurations of super-thin current sheets in space plasma: characteristic scaling of multilayer structures. J Geophys Res Space Phys 127(11):e2022JA030881. doi:10.1029/2022JA030881
36. Kamaletdinov SR, Yushkov EV, Artemyev AV, Lukin AS, Vasko IY (2020) Superthin current sheets supported by anisotropic electrons. Phys Plasmas 27(8):082904. doi:10.1063/5.0018063
37. Burch JL, Moore TE, Torbert RB, Giles BL (2016) Magnetospheric Multiscale overview and science objectives. Space Sci Rev 199(1–4):5–21. doi:10.1007/s11214-015-0164-9
38. Russell CT, Anderson BJ, Baumjohann W, Bromund KR, Dearborn D, Fischer D, et al. (2016) The magnetospheric Multiscale magnetometers. Space Sci Rev 199(1–4):189–256. doi:10.1007/s11214-014-0057-3
39. Pollock C, Moore T, Jacques A, Burch J, Gliese U, Saito Y, et al. (2016) Fast plasma investigation for magnetospheric Multiscale. Space Sci Rev 199(1–4):331–406. doi:10.1007/s11214-016-0245-4
40. Angelopoulos V, Kennel CF, Coroniti FV, Pellat R, Kivelson MG, Walker RJ, et al. (1994) Statistical characteristics of bursty bulk flow events. J Geophys Res 99(A11):21257–80. doi:10.1029/94JA01263
41. Zhang TL, Baumjohann W, Nakamura R, Balogh A, Glassmeier K -H (2002) A wavy twisted neutral sheet observed by CLUSTER. Geophys Res Lett 29(19) doi:10.1029/2002GL015544
42. Wang GQ, Volwerk M, Nakamura R, Boakes P, Zhang TL, Yoshikawa A, et al. (2014) Flapping current sheet with superposed waves seen in space and on the ground. J Geophys Res Space Phys 119(12). doi:10.1002/2014JA020526
43. Wang GQ, Zhang TL, Wu MY, Schmid D, Cao JB, Volwerk M (2019) Solar wind directional change triggering flapping motions of the current sheet: MMS observations. Geophys Res Lett 46(1):64–70. doi:10.1029/2018GL080023
45. Sergeev V, Runov A, Baumjohann W, Nakamura R, Zhang TL, Balogh A, et al. (2004) Orientation and propagation of current sheet oscillations. Geophys Res Lett 31(5):L05807. doi:10.1029/2003GL019346
46. Dunlop MW, Balogh A, Glassmeier K-H, Robert P (2002) Four-point cluster application of magnetic field analysis tools: the curlometer. J Geophys Res 107(A11):1384. doi:10.1029/2001JA005088
47. Wang GQ, Volwerk M, Zhang TL, Schmid D, Yoshikawa A (2017) High-latitude Pi2 pulsations associated with kink-like neutral sheet oscillations. J Geophys Res Space Phys 122(3):2889–99. doi:10.1002/2016JA023370
48. Kaufmann RL, Paterson WR, Frank LA. Magnetization of the plasma sheet (2004) J Geophys Res 109(A9). doi:10.1029/2003ja010148
49. Keiling A, Takahashi K. Review of Pi2 models. Space Sci Rev (2011) 161(1–4):63–148. doi:10.1007/s11214-011-9818-4
50. Milan SE, Clausen LBN, Coxon JC, Carter JA, Walach M-T, Laundal K, et al. (2017) Overview of solar wind-magnetosphere-ionosphere-atmosphere coupling and the generation of magnetospheric currents. Space Sci Rev 206(1–4):547–73. doi:10.1007/s11214-017-0333-0
51. Wang GQ, Zhang TL, Wu MY, Xiao SD, Wang G, Chen YQ, et al. (2021) Field-aligned currents originating from the chaotic motion of electrons in the tilted current sheet: MMS observations. Geophys Res Lett 48(9). doi:10.1029/2020GL088841
52. Hietala H, Drake JF, Phan TD, Eastwood JP, McFadden JP (2015) Ion temperature anisotropy across a magnetotail reconnection jet. Geophys Res Lett 42(18):7239–47. doi:10.1002/2015GL065168
53. Xiao S, Zhang T, Wang G, Volwerk M, Ge Y, Schmid D, et al. (2017) Occurrence rate of dipolarization fronts in the plasma sheet: cluster observations. Ann Geophysicae 35(4):1015–22. doi:10.5194/angeo-35-1015-2017
54. Shen C, Rong ZJ, Li X, Dunlop M, Liu ZX, Malova HV, et al. (2008) Magnetic configurations of the tilted current sheets in magnetotail. Ann Geophysicae 26(11):3525–43. doi:10.5194/angeo-26-3525-2008
55. Vasko IY, Artemyev AV, Petrukovich AA, Nakamura R, Zelenyi LM (2014) The structure of strongly tilted current sheets in the Earth magnetotail. Ann Geophysicae 32(2):133–46. doi:10.5194/angeo-32-133-2014
56. Sergeev V, Angelopoulos V, Carlson C, Sutcliffe P (1998) Current sheet measurements within a flapping plasma sheet. J Geophys Research-Space Phys 103(A5):9177–87. doi:10.1029/97ja02093
Keywords: electron firehose unstable, magnetic field fluctuation, plasma sheet, fast flow, non-fast flow
Citation: Wei J, Wang G and Zuo P (2024) Study of the characteristics of electron firehose unstable conditions in the terrestrial magnetotail plasma sheet. Front. Phys. 12:1446646. doi: 10.3389/fphy.2024.1446646
Received: 10 June 2024; Accepted: 13 September 2024;
Published: 26 September 2024.
Edited by:
Andrey Samsonov, University College London, United KingdomReviewed by:
Anton Artemyev, University of California, Los Angeles, United StatesNikolai Erkaev, Institute of Computational Modelling SB RAS, Russia
Copyright © 2024 Wei, Wang and Zuo. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Guoqiang Wang, d2FuZ2dxQGhpdC5lZHUuY24=; Pingbing Zuo, enVvcGJAaGl0LmVkdS5jbg==
 Jiayun Wei
Jiayun Wei Guoqiang Wang
Guoqiang Wang Pingbing Zuo1,2,3*
Pingbing Zuo1,2,3*