Modification entropy of Kerr–Sen-like black hole in Lorentz-breaking bumblebee gravity

The Lorentz symmetry breaking theory not only affects the space–time background but also the dynamic behavior of bosons and fermions in curved space–time. Therefore, the Lorentz symmetry breaking theory will affect the quantum tunneling rate, Hawking temperature, black hole entropy, and other physical quantities of black holes. According to the modification of the space–time background and the modification of the particle dynamic equations, the quantum tunneling radiation of the Kerr–Sen-like black hole in bumblebee gravitational theory and its related contents are deeply studied. The research methods and a series of new results obtained in this paper are discussed. This makes the research methods and conclusions in this paper of more astrophysical significance and reference value.

1 Introduction

Lorentz symmetry is the basic relationship of general relativity and quantum field theory. General relativity is a gravity theory that cannot be renormalized. Therefore, researchers put forward string theory, M-theory, and loop quantum gravity theory for studying quantum gravity theory. The studies on string theory and quantum gravity theory show that the Lorentz symmetry needs to be modified on the Planck scale in the case of high energy. The theory of Lorentz symmetry breaking in high energy physics includes the Horava–Lifshitz theory proposed by Horava in 2009. Another Lorentz symmetry breaking theory of gravity is the Einstein-aether theory. Since Einstein gravity theory cannot be renormalized, the quantum gravity theory combined with gravity theory and quantum theory as well as the grand unified theory in physics cannot be constructed so far. On the other hand, significant amounts of dark matter and dark energy are observed in cosmology, but these results still cannot be reasonably explained by current gravity theory. Since the publication of general relativity more than 100 years ago, researchers of physics and astronomy have always been studying Einstein’s gravity theory and the modified gravity theory. The supersymmetry theory, scalar tensor gravity theory, f(R) theory, f (R, T) gravity theory, Rastall gravity theory, and Finsler gravity theory are all gravity theories with modification. This paper studies the modification of Bekenstein–Hawking entropy in the Kerr–Sen-like black hole in the bumblebee gravity theory and its related projects [1, 2]. We consider two impacts of Lorentz symmetry breaking in curved space–time, namely, the impact on the space–time background and the influence on the dynamic equations of bosons and fermions in curved space–time. Without the Lorentz-breaking modification for the space–time background, people have modified the dynamic equations of bosons and fermions in static, stationary, and non-stationary black hole space–time with Lorentz-breaking modification and carried out a series of meaningful research works on related topics [3–16]. [1, 2] show that in the Einstein–bumblebee gravity theory, due to the influence of Lorentz-breaking on the space–time background, the space–time metric of the stationary Kerr–Sen-like black hole is modified to a certain extent. In this modified space–time background, the modifications to the dynamic equation of fermions by Lorentz-breaking and related projects are studied. Section 2 introduces the modification of space–time background and the modified dynamic equation of fermions. Section 3 describes the modified form of black hole entropy. The final section presents the research results.

2 The dynamic equation of fermions in Einstein–bumblebee gravity and Kerr–Sen-like black hole space–time

The Einstein–bumblebee gravity action including the term Lorentz-breaking is expressed as follows [1, 2].

$S_{EB}=\int d^{4}x\sqrt{-g}\left\{\frac{1}{16\pi G_N}\left[R+\lambda B^{\mu }{B}^{\nu }{R}_{\mu \nu }-\frac{1}{4}{B}^{\mu \nu }{B}_{\mu \nu }-V\left(B^{\mu }\right)\right]\right\}.(1)$

In Eq. 1, λ is a non-minimal coupling constant between gravity and the bumblebee vector field B^μ. Obviously, when B_μ = 0, Eq. 1 is back to the action of the Einstein gravitational field. B_μν in Eq. 1 and the following Eq. 2 is the field strength tensor corresponding to the bumblebee field reads [1, 2].

$B_{\mu \nu }={\partial }_{\mu }{B}_{\nu }-{\partial }_{\nu }{B}_{\mu }.(2)$

$V\left(B_{\mu }\right)$ in Eq. 1 is the potential, and the functional form of the potential $V\left(B_{\mu }\right)$ that induces Lorentz-breaking is

$V=V\left(B_{\mu }{B}^{\mu }\pm b^2\right),(3)$

where b² is a real positive constant and b² provides field B_μ, a non-vanishing vacuum expectation value (VEV). Eq. 3 is assumed to have a minimum through the condition B_μB^μ ± b² = 0, which ensures a non-zero VEV. $⟨B^{\mu }⟩=b^{\mu }$ will be supplied to the field B_μ by the potential V. b^μ is a function of the space–time coordinates, satisfying the equation b_μb^μ = ±b²,; here the plus–minus sign indicates that b^μ may have a time-like as well as space-like nature depending upon the choice of the sign. According to [1, 2, 17–19], assuming a space-like bumblebee field, which acquires a pure radial VEV, and naming ℓ = λb_μb^μ, we can express the space–time line element of the Kerr–Sen-like black hole in bumblebee gravity theory as

$\begin{array}{cc}\hfill d{s}^2& =-\left(1-\frac{2Mr}{{\rho }^2}\right)d{t}^2-\frac{4Mra\sqrt{1+\ell }}{{\rho }^2}si{n}^2\theta dtd\phi \hfill \\ \hfill & +\frac{{\rho }^2}{\mathrm{\Delta }}d{r}^2+{\rho }^{2}d{\theta }^2+\frac{Asi{n}^2\theta }{{\rho }^2}d{\phi }^2,\hfill \end{array}(4)$

where

$\mathrm{\Delta }=\frac{r\left(r+b\right)-2Mr}{1+\ell }+a^2,(5)$

$\begin{array}{cc}\hfill {\rho }^2& =r\left(r+b\right)+\left(1+\ell \right)a^{2}co{s}^2\theta \hfill \\ \hfill & =r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^{2}si{n}^2\theta ,\hfill \end{array}(6)$

$A={\left[r\left(r+b\right)+\left(1+\ell \right)a^2\right]}^2-\mathrm{\Delta }{\left(1+\ell \right)}^2{a}^{2}si{n}^2\theta ,(7)$

In the above Eqs 4–7, M is the mass of such a black hole, a = J/M is the angular momentum per unit mass, J is the angular momentum, and ℓ and b are expressed in the above content. Equation 4 is the form in the Boyer–Lindquist coordinate and the space–time metric of the stationary axisymmetric Kerr–Sen-like black hole after the Lorentz-breaking modification. Such space–time background is not only the modification of the stationary Kerr–Sen black hole but also the development of Kerr–Sen metric. When ℓ = 0 and b = 0, Kerr–Sen-like metric is back to Kerr metric. According to Eq. 4, the components of non-zero covariant metric tensors are shown as follows, respectively:

$\begin{array}{cc}\hfill g_{tt}& =-\frac{1}{{\rho }^2}\left({\rho }^2-2Mr\right)=-\frac{1}{{\rho }^2}\left[r\left(r+b\right)-2Mr+\left(1+\ell \right)a^{2}co{s}^2\theta \right],\hfill \\ \hfill g_{rr}& =\frac{{\rho }^2}{\mathrm{\Delta }},\hfill \\ \hfill g_{\theta \theta }& ={\rho }^2,\hfill \\ \hfill g_{\phi \phi }& =\frac{1}{{\rho }^2}Asi{n}^2\theta ,\hfill \\ \hfill g_{t\phi }& =g_{\phi t}=-\frac{2Mra\sqrt{1+\ell }}{{\rho }^2}si{n}^2\theta .\hfill \end{array}(8)$

Then, the metric determinant g corresponding to g_μν is expressed as

$\begin{array}{cc}\hfill g& =\left|\begin{array}{cc}\hfill \begin{array}{cc}\hfill g_{tt}\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill g_{rr}\hfill \end{array}\hfill & \hfill \begin{array}{cc}\hfill 0\hfill & \hfill g_{t\phi }\hfill \\ \hfill 0\hfill & \hfill 0\hfill \end{array}\hfill \\ \hfill \begin{array}{cc}\hfill 0\hfill & \hfill 0\hfill \\ \hfill g_{\phi t}\hfill & \hfill 0\hfill \end{array}\hfill & \hfill \begin{array}{cc}\hfill g_{\theta \theta }\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill g_{\phi \phi }\hfill \end{array}\hfill \end{array}\right|\hfill \\ \hfill & =-\left(1+\ell \right)si{\mathrm{n}}^2\theta {\left[r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^{2}si{\mathrm{n}}^2\theta \right]}^2.\hfill \end{array}(9)$

According to Eqs 8, 9, we can calculate the components of the non-zero inverse metric tensors as follows:

$\begin{array}{cc}\hfill g^{tt}& =-\frac{{\rho }^2}{\mathrm{\Delta }}\frac{{\left[r\left(r+b\right)+\left(1+\ell \right)a^2\right]}^2-{\left(1+\ell \right)}^2{a}^{2}si{n}^2\theta }{\left(1+\ell \right){\left[r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^{2}si{n}^2\theta \right]}^2},\hfill \\ \hfill g^{rr}& =\frac{\mathrm{\Delta }}{{\rho }^2},\hfill \\ \hfill g^{\theta \theta }& =\frac{1}{{\rho }^2},\hfill \\ \hfill g^{\phi \phi }& =\frac{{\rho }^2}{\mathrm{\Delta }}\frac{r\left(r+b\right)-2Mr+\left(1+\ell \right)a^2\left(1-si{n}^2\theta \right)}{\left(1+\ell \right)si{n}^2\theta \left[r\left(r+b\right)+\left(1+\ell \right)a^2+\left(1+\ell \right)a^{2}si{n}^2\theta \right]},\hfill \\ \hfill g^{t\phi }& =-\frac{{\rho }^2}{\mathrm{\Delta }}\frac{2Mra\sqrt{1+\ell }si{n}^2\theta }{g}\hfill \\ \hfill & =\frac{{\rho }^2}{\mathrm{\Delta }}\frac{2Mra}{{\left(1+\ell \right)}^{1/2}{\left[r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^{2}si{n}^2\theta \right]}^2}.\hfill \end{array}(10)$

With the expression g^μν, according to a hypersurface equation $F\left(x^{\mu }\right)=0$ based on the four-dimensional curved space–time together with the normal vector $n_{\mu }=\frac{\partial F}{\partial x^{\mu }}$, we can get $n_{\mu }{n}^{\mu }=g^{\mu \nu }{n}_{\mu }{n}_{\nu }=g^{\mu \nu }\frac{\partial F}{\partial x^{\mu }}\frac{\partial F}{\partial x^{\nu }}$. If n_μn^μ = 0, such a hypersurface is the null hypersurface that can determine the event horizon of the black hole, namely,

$g^{\mu \nu }\frac{\partial F}{\partial x^{\mu }}\frac{\partial F}{\partial x^{\nu }}=0.(11)$

Substituting Eq. 10 into Eq. 11, it can be concluded that

$g^{rr}{\left(\frac{\partial F}{\partial r}\right)}^2=0,(12)$

namely,

$\mathrm{\Delta }{|}_{r=r_H}=\frac{r_H\left(r_H+b\right)-2M{r}_H}{1+\ell }+a^2=0.(13)$

This is the equation that is satisfied by the event horizon of the Kerr–Sen-like black hole. From this equation, it can be seen that the outer horizon of this black hole, the event horizon, is expressed as

$r_{+}=r_H=M-\frac{b}{2}+{\left[{\left(2M-b\right)}^2-4\left(1+\ell \right)a^2\right]}^{\frac{1}{2}}.(14)$

According to Eqs 12–14, the event horizon r_H of the black hole is modified due to the influence of Lorentz-breaking on the space–time background.

In order to research the quantum tunneling radiation characteristics of the Kerr–Sen-like black hole, we can consider the expression of the action quantity of spin 1/2 fermions, which has been modified by Lorentz-breaking theory. Due to the particularity of spin 1/2 fermions, one of the action modification terms is the chiral, and the other two are aether-like field vector and Carroll–Field–Jackiw (CFJ). In fact, the first discovered Lorentz-breaking term is the CFJ term [20–22]. In the later research process, researchers proposed the aether-like vector modification term u^μ for the scalar field. For the spinor field, the chiral term and the aether-like term were proposed. The Lorentz-breaking modification of field theory models has attracted widespread attention [23–29]. There have been some reports on the use of Lorentz-breaking theory to modify fermion dynamic equations in flat and curved space–time [30–33]. Now, based on the particularity of the Kerr–Sen-like black hole metric and considering the fermions of spin 1/2, we can express the modified spinor field action as [29–32]

$\begin{array}{cc}\hfill S_F& =\int d^{4}x\sqrt{-g}\bar{\psi }\hfill \\ \hfill & \left\{i{\gamma }^{\mu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left[1-\frac{d{\hslash }^2}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)\right]\right.\hfill \\ \hfill & \left.+\frac{c\hslash }{m}{\mu }^{\mu }{\mu }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)+\frac{f}{\hslash m}{\gamma }^5-\frac{m}{\hslash }\right\}\psi ,\hfill \end{array}(15)$

where Ω_μ and Ω_ν are spinor contacts, $\bar{\psi }$ is the conjugate of ψ, γ^μ and γ^β are the gamma metrics of Kerr–Sen-like space–time, m is the mass of spin 1/2 fermions, and d, c, and f separately correspond to the CFJ term, aether-like term, and chiral term. The covariant derivative in Eq. 15 is ∂_;μ = ∂_μ + iΩ_μ. It should be noted that a, b, and c are all dimensionless real small quantities, and $\frac{d}{m}\ll 1$, $\frac{f}{m}\ll 1,\frac{c}{m}\ll 1$. According to Eq. 15 and the variational principle, we can obtain the spinor field equation. Applying the variational principle to Eq. 15, from

$\begin{array}{c}\hfill \delta S_F=0,\hfill \end{array}(16)$

we can obtain

$\begin{array}{cc}\hfill & \delta \left\{\bar{\psi }\left\{i{\gamma }^{\mu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left[1-\frac{d{\hslash }^2}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)\right]\right.\right.\hfill \\ \hfill & \left.+\frac{c\hslash }{m}{u}^{\mu }{u}^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)+\frac{f}{\hslash m}{\gamma }^5-\frac{m}{\hslash }\right\}\psi =0.\hfill \end{array}(17)$

Due to $\delta \bar{\psi }=\frac{\partial \bar{\psi }}{\partial x^{\mu }}\delta x^{\mu },\delta \psi =\frac{\partial \psi }{\partial x^{\mu }}\delta x^{\mu }$, and δx^μ ≠ 0, and according to Eqs 16, 17, the matrix form of the modified fermion dynamic equation for spin 1/2 is obtained as follows:

$\begin{array}{cc}\hfill & \left\{i{\gamma }^{\mu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left[1-\frac{d{\hslash }^2}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)\right]\right.\hfill \\ \hfill & \left.+\frac{c\hslash }{m}{u}^{\mu }{u}^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)+\frac{f}{\hslash m}{\gamma }^5-\frac{m}{\hslash }\right\}\psi =0.\hfill \end{array}(18)$

Multiplying by $\left[1+\frac{d{\hslash }^2}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)\right]$ on both sides and omitting higher-order small quantities of d², Eq. 18 can be rewritten as

$\begin{array}{cc}\hfill & \left\{i{\gamma }^{\mu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)+\left[1+\frac{d{\hslash }^2}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)\right]\right.\hfill \\ \hfill & \left.\times \left[\frac{c\hslash }{m}{u}^{\mu }{u}^v\left({\partial }_{\mu }+i{\mathrm{\Omega }}_{\mu }\right)\left({\partial }_{\nu }+i{\mathrm{\Omega }}_{\nu }\right)+\frac{f}{\hslash m}{\gamma }^5-\frac{m}{\hslash }\right]\right\}\psi =0.\hfill \end{array}(19)$

This is the modified form of the dynamic equation of spin 1/2 fermions, also known as a matrix equation. According to the WKB approximation theory, the wave function ψ is expressed as

$\psi =\left(\begin{array}{c}\hfill \lambda \hfill \\ \hfill \xi \hfill \end{array}\right)e^{\frac{i}{\hslash }S},(20)$

where S is the action of spin 1/2 fermions. Substituting Eq. 20 into Eq. 19, an equation in the matrix form can be obtained as follows:

$\begin{array}{cc}\hfill & \left[-{\gamma }^{\mu }{\partial }_{\mu }S+\left(1-\frac{d}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }{\partial }_{\mu }S{\partial }_{\nu }S\right)\right.\hfill \\ \hfill & \left.\left(-\frac{c}{m}{u}^{\mu }{u}^{\nu }{\partial }_{\mu }S{\partial }_{\nu }S+\frac{f}{m}{\gamma }^5-m\right)\right]\left(\begin{array}{c}\hfill \lambda \hfill \\ \hfill \xi \hfill \end{array}\right)=0,\hfill \end{array}(21)$

where ℏΩ_μ and ℏΩ_ν are omitted. Equation 21 is a matrix equation, which is actually an eigenmatrix. Accordingly, the condition for nontrivial solutions in Eq. 21 is that the value of the determinant corresponding to the matrix is 0.

$\begin{array}{cc}\hfill & -{\gamma }^{\mu }{\partial }_{\mu }S+\left(1-\frac{d}{m^2}{\gamma }^{\mu }{\gamma }^{\nu }{\partial }_{\mu }S{\partial }_{\nu }S\right)\left(-\frac{c}{m}{u}^{\mu }{u}^{\nu }{\partial }_{\mu }S{\partial }_{\nu }S+\frac{f}{m}{\gamma }_0^5-m\right)=0,\hfill \end{array}(22)$

where γ^μ is the gamma matrix in curved space–time, γ⁵ is the matrix in the chiral modification term, and ${\gamma }_0^5$ is the coefficient corresponding to γ⁵. The relationship between γ⁵ and γ^μ is as follows:

${\gamma }^{\mu }{\gamma }^{\nu }+{\gamma }^{\nu }{\gamma }^{\mu }=2g^{\mu \nu }I,(23)$

${\gamma }^5{\gamma }^{\mu }+{\gamma }^{\mu }{\gamma }^5=0.(24)$

where I in Eq. 23 is a unit matrix. After multiplying both sides of Eq. 22 by γ^ν∂_νS and omitting the higher-order small quantities, the following field equation is obtained:

$g^{\mu \nu }\left(1-2d\right){\partial }_{\mu }S{\partial }_{\nu }S+2c{u}^{\mu }{u}^{\nu }{\partial }_{\mu }S{\partial }_{\nu }S+m^2-2f{r}_0^5=0.(25)$

This is the spinor field equation for fermions with spin 1/2 in Kern–Sen-like black hole space–time. Calculate S from Eq. 25; then, according to the WKB approximation theory and the black hole quantum tunneling radiation theory, we can research the quantum tunneling radiation characteristics of such black holes.

3 Modification of the Kerr–Sen-like black hole entropy by Lorentz-breaking theory

To solve the field in Eq. 25 in the Kerr–Sen-like space–time background, the four components of the aether-like field vector u^μ are selected as follows:

$\begin{array}{cc}\hfill u^t& =\frac{c_t}{\sqrt{g_{tt}}},u^t{u}_t=u^t{u}^t{g}_{tt}=C_t^2,\hfill \\ \hfill u^r& =\frac{c_r}{\sqrt{g_{rr}}},u^r{u}_r=u^r{u}^r{g}_{rr}=C_r^2,\hfill \\ \hfill u^{\theta }& =\frac{c_{\theta }}{\sqrt{g_{\theta \theta }}},u^{\theta }{u}_{\theta }=u^{\theta }{u}^{\theta }{g}_{\theta \theta }=C_{\theta }^2,\hfill \\ \hfill u^{\phi }& =\frac{c_{\phi }}{\sqrt{g_{\phi \phi }}},u^{\phi }{u}_{\phi }=u^{\phi }{u}^{\phi }{g}_{\phi \phi }=C_{\phi }^2.\hfill \end{array}(26)$

Obviously, u^μu_μ is a constant and meets the basic conditions of the aether-liker field vector. In Eq. 25, ${\gamma }_0^5$ is determined by the matrix corresponding to the Kerr–Sen-like space–time metric. Based on the characteristics of such a black hole metric, the four components of the gamma matrix are constructed as follows:

$\begin{array}{cc}\hfill {\gamma }^t& =\sqrt{g^{tt}}\left(\begin{array}{cc}\hfill I\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill -I\hfill \end{array}\right),\hfill \\ \hfill {\gamma }^r& =\sqrt{g^{rr}}\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^1\hfill \\ \hfill {\sigma }^1\hfill & \hfill 0\hfill \end{array}\right),\hfill \\ \hfill {\gamma }^{\theta }& =\sqrt{g^{\theta \theta }}\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^2\hfill \\ \hfill {\sigma }^2\hfill & \hfill 0\hfill \end{array}\right),\hfill \\ \hfill {\gamma }^{\phi }& =\frac{g^{t\phi }}{\sqrt{g^{tt}}}\left(\begin{array}{cc}\hfill I\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill -I\hfill \end{array}\right)+\sqrt{g^{\phi \phi }-\frac{{\left(g^{t\phi }\right)}^2}{g^{tt}}}\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^3\hfill \\ \hfill {\sigma }^3\hfill & \hfill 0\hfill \end{array}\right),\hfill \end{array}(27)$

where σ¹, σ², σ³ are Pauli matrices, namely,

$\begin{array}{c}\hfill {\sigma }^1=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill 1\hfill & \hfill 0\hfill \end{array}\right),{\sigma }^2=\left(\begin{array}{cc}\hfill 0\hfill & \hfill -i\hfill \\ \hfill i\hfill & \hfill 0\hfill \end{array}\right),{\sigma }^3=\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill -1\hfill \end{array}\right).\end{array}(28)$

From Eqs 27, 28, we obtain

$\begin{array}{c}\hfill {\gamma }^t{\gamma }^{\phi }+{\gamma }^{\phi }{\gamma }^t=2g^{t\phi }I,\\ \hfill {\gamma }^r{\gamma }^r=g^{rr}I,\\ \hfill {\gamma }^{\theta }{\gamma }^{\theta }=g^{\theta \theta }I,\\ \hfill {\gamma }^{\phi }{\gamma }^{\phi }=g^{\phi \phi }I.\end{array}(29)$

Equations 23 and 29 are completely consistent. According to Eq. 27 and the Kerr–Sen-like metric feature, we can construct γ⁵ as follows:

$\begin{array}{cc}\hfill {\gamma }^5& =\frac{\sin \theta }{\sqrt{g}}\left(\begin{array}{cc}\hfill I\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill -I\hfill \end{array}\right)\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^1\hfill \\ \hfill {\sigma }^1\hfill & \hfill 0\hfill \end{array}\right)\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^2\hfill \\ \hfill {\sigma }^2\hfill & \hfill 0\hfill \end{array}\right)\left(\begin{array}{cc}\hfill 0\hfill & \hfill {\sigma }^3\hfill \\ \hfill {\sigma }^3\hfill & \hfill 0\hfill \end{array}\right)\hfill \\ \hfill & =\frac{{\left(1+\ell \right)}^{-\frac{1}{2}}}{r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^2{\sin }^2\theta }\left(\begin{array}{cc}\hfill I\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill I\hfill \end{array}\right)\hfill \\ \hfill & \begin{array}{c}\hfill ={\gamma }_0^5\left(\begin{array}{cc}\hfill I\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill I\hfill \end{array}\right)\hfill \end{array}.\hfill \end{array}(30)$

where γ⁵ is a Hermitian matrix. From Eqs 27, 30, we can obtain

$\begin{array}{cc}\hfill & {\gamma }^t{\gamma }^5+{\gamma }^5{\gamma }^t=0,\hfill \\ \hfill & {\gamma }^r{\gamma }^5+{\gamma }^5{\gamma }^r=0,\hfill \\ \hfill & {\gamma }^{\theta }{\gamma }^5+{\gamma }^5{\gamma }^{\theta }=0,\hfill \\ \hfill & {\gamma }^{\phi }{\gamma }^5+{\gamma }^5{\gamma }^{\phi }=0.\hfill \end{array}(31)$

Obviously, γ⁵ in Eq. 31 completely meets the requirements of Eq. 24, and correct γ⁵ is merely constructed by correctly choosing γ^μ. By substituting Eq. 26 and g^μν into Eq. 25, Eq. 25 can be simplified as

$\begin{array}{cc}\hfill & g^{tt}\left(1-2d\right){\left(\frac{\partial S}{\partial t}\right)}^2+g^{rr}\left(1-2d\right){\left(\frac{\partial S}{\partial r}\right)}^2+2g^{t\phi }\left(1-2d\right)\frac{\partial S}{\partial t}\frac{\partial S}{\partial \phi }\hfill \\ \hfill & +g^{\theta \theta }{\left(\frac{\partial S}{\partial t}\right)}^2+g^{\phi \phi }{\left(\frac{\partial S}{\partial \phi }\right)}^2+2c{u}^{\mu }{u}^{\nu }\frac{\partial S}{\partial x^{\mu }}\frac{\partial S}{\partial x^{\nu }}+m^2-2f{\gamma }_0^5=0,\hfill \end{array}(32)$

where the modification term corresponding to Kerr–Sen-like space–time is expressed as

$\begin{array}{cc}\hfill & 2c{u}^{\mu }{u}^{\nu }\frac{\partial S}{\partial x^{\mu }}\frac{\partial S}{\partial x^{\nu }}=2c{u}^{\mu }{u}_{\lambda }{g}^{\lambda \nu }\frac{\partial S}{\partial x^{\mu }}\frac{\partial S}{\partial x^{\nu }}\hfill \\ \hfill & =2c\left[C_t^2{g}^{tt}{\left(\frac{\partial S}{\partial t}\right)}^2+C_r^2{g}^{rr}{\left(\frac{\partial S}{\partial r}\right)}^2+2C_t^2{g}^{t\phi }\frac{\partial S}{\partial t}\frac{\partial S}{\partial \phi }\right.\hfill \\ \hfill & +\left.C_{\theta }^2{g}^{\theta \theta }{\left(\frac{\partial S}{\partial \theta }\right)}^2+C_{\phi }^2{g}^{\phi \phi }{\left(\frac{\partial S}{\partial \phi }\right)}^2\right]+4c\left[u^r\left(\frac{\partial S}{\partial t}\right)\left(\frac{\partial S}{\partial r}\right)\right.\hfill \\ \hfill & \left.+u^{\theta }\left(\frac{\partial S}{\partial r}\right)\left(\frac{\partial S}{\partial \theta }\right)+u^{\phi }\left(\frac{\partial S}{\partial r}\right)\left(\frac{\partial S}{\partial \phi }\right)+u^{\theta }\left(u^t\frac{\partial S}{\partial t}\frac{\partial S}{\partial \theta }+u^{\phi }\frac{\partial S}{\partial \theta }\frac{\partial S}{\partial \phi }\right)\right].\hfill \end{array}(33)$

Killing vector ${\left(\frac{\partial }{\partial \phi }\right)}^{\alpha }$ exists in Kerr–Sen-like space–time and then $\frac{\partial S}{\partial \phi }=n$ (constant). The S in Eq. 32 can be separated as

$S=-\omega t+R\left(r\right)+\mathrm{\Theta }\left(\theta \right)+n\phi .(34)$

By substituting Eqs 10, 33, 34 into Eq. 32 and multiplying both sides of Eq. 32 by ${\left[r\left(r+b\right)+\left(1+\ell \right)a^2-\left(1+\ell \right)a^2{\sin }^2\theta \right]}^2$, ρ², and Δ respectively, by separating variables and considering

${\left.\mathrm{\Delta }\right|}_{r\to r_H}=0,(35)$

we can get the radial dynamic equation of Eq. 32 at r_H as follows:

$\begin{array}{cc}\hfill & -\left(1-2d+2c{C}_t^2\right){\left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]}^2{\omega }^2\hfill \\ \hfill & +\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right){\left.\left[{\mathrm{\Delta }}^2{\left(\frac{dR}{dr}\right)}^2\right]\right|}_{r\to r_H}\hfill \\ \hfill & -\left(1-2d+2c{C}_{\phi }^2\right)a^2{n}^2\hfill \\ \hfill & \begin{array}{c}\hfill +2\left(1-2d+2c{C}_t^2\right)\left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]\omega n=0\hfill \end{array}.\hfill \end{array}(36)$

From this Eqs 35, 36, we can obtain

$\begin{array}{cc}\hfill & {\left.{\left(\frac{dR}{dr}\right)}^2\right|}_{r\to r_H}\hfill \\ \hfill & =\frac{1}{{\mathrm{\Delta }}^2\left|r\to r_H\right.}\frac{\left(1-2d+2c{C}_t^2\right){\left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]}^2}{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}\hfill \\ \hfill & \left[{\omega }^2-2\frac{\omega n}{r_H\left(r_H+b\right)+\left(1+\ell \right)a^2}\right.\left.+\frac{a^2{n}^2}{{\left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]}^2}\right]\hfill \\ \hfill & =\frac{1}{{\mathrm{\Delta }}^2\left|r\to r_H\right.}\frac{\left(1-2d+2c{C}_t^2\right){\left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]}^2}{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}{\left(\omega -{\omega }_0\right)}^2,\hfill \end{array}(37)$

where

${\omega }_0=\frac{an}{r_H\left(r_H+b\right)+\left(1+\ell \right)a^2}.(38)$

Taking square roots on both sides of Eq. 37, we can obtain

$\begin{array}{cc}\hfill & \frac{d{R}^{\pm }}{dr}\left|r\to r_H\right.\hfill \\ \hfill & =\pm \frac{1}{{\left.\mathrm{\Delta }\right|}_{r\to r_H}}\sqrt{\frac{\left(1-2d+2c{C}_t^2\right)}{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}}\hfill \\ \hfill & \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]\left(\omega -{\omega }_0\right).\hfill \end{array}(39)$

In the above Eq. 39, ω₀ is shown in Eq. 38. Using the residue theorem integrals, we can obtain

$\begin{array}{c}\hfill R^{\pm }=\pm i2\pi \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]\sqrt{\frac{\left(1-2d+2c{C}_t^2\right)}{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}}\left(\omega -{\omega }_0\right).\hfill \end{array}(40)$

According to the WKB approximation theory and the quantum tunneling radiation theory, we can get that the quantum tunneling rate of the spin 1/2 fermions at the event horizon of the black hole is

$\mathrm{\Gamma }\sim exp\left(-2Im{S}^{\pm }\right)=exp\left(-2Im{R}^{\pm }\right)=exp\left[-\frac{\omega -{\omega }_0}{T_H}\right],(41)$

where

$T_H=\frac{{\left[\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)\right]}^{1/2}}{2\pi \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]{\left(1-2d+2c{C}_t^2\right)}^{1/2}}.(42)$

This is the modified expression for Hawking temperature, where ℓ and b are the modification terms due to the influence of Lorentz-breaking on the space–time background. d is the modification term of the CFJ term. c, C_t, and C_r are modification terms caused by the aether-like field vector. The new significance of Eqs 41, 42 is including the modification for background and also for the dynamic behavior of spin 1/2 fermions in the curved space–time backgrounds, where ω₀ is the chemical potential, due to the rotation of the black hole; a ≠ 0; and ω₀ ≠ 0. So ω₀ is related to ℓ and b and also to a = J/M. This is the basic feature of the stationary Kerr–Sen-like black hole thermodynamics.

In order to more intuitively represent the influence of ℓ, b, d, c, C_r, and C_t on the quantum tunneling rate of the Kerr–Sen-like black hole, the figures Γ − (ω − ω₀) are drawn.

As can be seen from Figures 1, 2, the Lorentz-breaking bumblebee gravity theory has modified the space–time background of the Kerr–Sen-like black hole. After the correction, the Kerr–Sen black hole space–time background becomes the Kerr–Sen-like black hole. Figure 1 shows the comparison of the quantum tunneling rates of the Kerr–Sen-like black hole and Kerr-Sen black hole. Figure 2 shows a schematic diagram of the quantum tunneling rate of the Kerr–Sen-like black hole affected by the CFJ and the aether-like correction terms.

Figure 1

Figure 1. Variation of the quantum tunneling rate for different parameters such as ℓ and b.

Figure 2

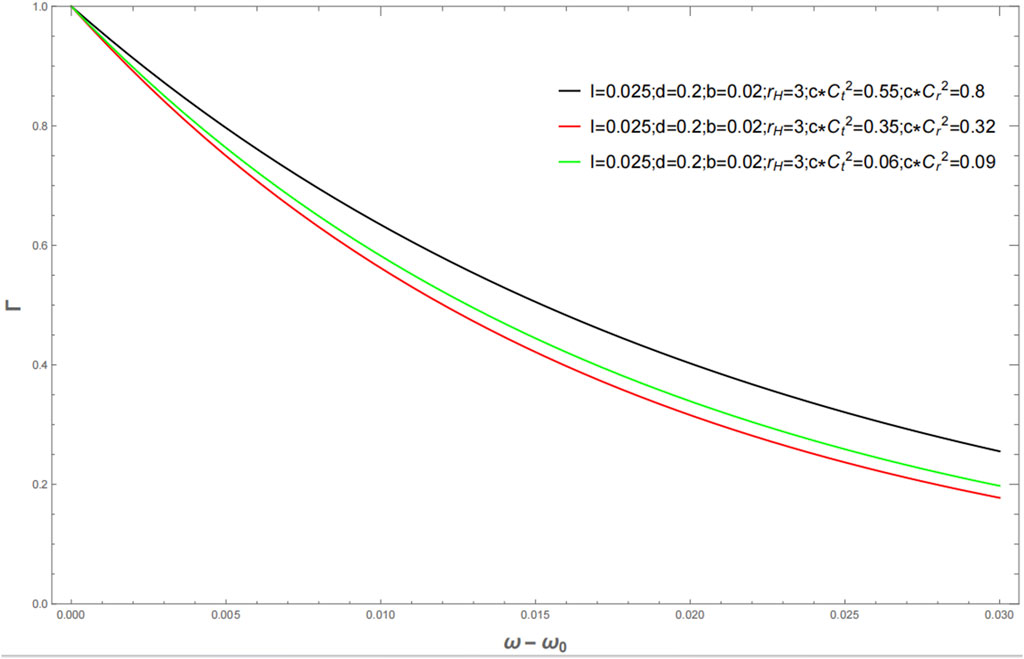
Figure 2. Variation of the quantum tunneling rate for different parameters such as d, c, C_r, and C_t.

Another major physical quantity in black hole thermodynamics is black hole entropy. The entropy of black holes is closely related to Hawking temperature. Due to the modified Hawking temperature expressed in Eq. 42, there should be corresponding modified black hole entropy. According to the first law of black hole thermodynamics, the Bekenstein–Hawking entropy S_BH of the black hole is related to the Hawking temperature of the black hole as

$dM=T_{H}d{S}_{BH}+\mathrm{\Omega }dJ,(43)$

where Ω is the rotational angular velocity. From Eq. 43, we obtain the following equation:

$\begin{array}{cc}\hfill & S_{BH}=\int \frac{dM-VdJ}{T_H}\hfill \\ \hfill & \approx \int \frac{dM-VdJ}{T_h}{\left[\frac{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}{1-2d+2c{C}_t^2}\right]}^{\frac{1}{2}}\left(1-\frac{r_{H}b+\ell a^2}{r_H^2+a^2}+\cdots \right).\hfill \end{array}(44)$

From Eq. 44, we obtain the following equation:

$T_h=\frac{1}{2\pi \left(r^2+a^2\right)}.(45)$

In the above Eq. 45, T_h is the Hawking temperature at the event horizon of the Kerr black hole before modification, so Eq. 44 with the integral is

$S_{BH}=S_{BH}^{\prime }{\left[\frac{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}{1-2d+2c{C}_t^2}\right]}^{\frac{1}{2}}\left(1-\frac{r_{H}b+\ell a^2}{r_H^2+a^2}+\cdots \right),(46)$

where $S_{BH}^{\prime }$ is the Bekenstein–Hawking entropy before modification. From the analysis of the quantum theory, the entropy of the black hole is proportional to the area of the event horizon measured in Planck area. This means that the black hole has an entropy proportional to its area, that is $S_{BH}^{\prime }\propto A_H$, which is the specific form of the entropy increasing principle in black hole thermodynamics. According to the second law of black hole thermodynamics, entropy never decreases in the clockwise direction. This is a merely physical law that can show the arrow of time. $S_{BH}^{\prime }$ in Eq. 46 can be obtained by calculating the area A_H. From Eq. 4, r = r_H, when considering dt = 0 and dr = 0, the two-dimensional line element is obtained as follows:

$d{\sigma }^2={\rho }^{2}d{\theta }^2+\frac{Asi{n}^2\theta }{{\rho }^2}d{\phi }^2.(47)$

The metric determinant corresponding to Eq. 47 is

$\begin{array}{c}\hfill g_{\sigma }=\left|\begin{array}{cc}\hfill g_{\theta \theta }\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill g_{\phi \phi }\hfill \end{array}\right|=Asi{n}^2\theta .\end{array}(48)$

Therefore, according to Eq. 48, the event horizon area A_H of such a black hole is

$A_H=\int \sqrt{g_{\sigma }}d\theta d\phi =\sqrt{A}\int sin\theta d\theta d\phi =4\pi \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right].(49)$

Then,

$S_{BH}^{\prime }=\frac{A_H}{4}=\pi \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right].(50)$

Substituting Eq. 50 into Eq. 46, the expression of the modification entropy of such a black hole is

$\begin{array}{cc}\hfill S_{BH}& =\pi \left[r_H\left(r_H+b\right)+\left(1+\ell \right)a^2\right]\hfill \\ \hfill & {\left[\frac{\left(1+\ell \right)\left(1-2d+2c{C}_r^2\right)}{1-2d+2c{C}_t^2}\right]}^{\frac{1}{2}}\left(1-\frac{r_{H}b+\ell a^2}{r_H^2+a^2}+\cdots \right).\hfill \end{array}(51)$

It can be seen that the modified entropy S_BH of the black hole is related to d, b, ℓ, c, C_t, and C_r. If using ΔS_BH to express the Bekenstein–Hawking entropy transformation, then the expression of the quantum tunneling rate, as shown in Eq. 41, can be rewritten as

$\mathrm{\Gamma }=\exp \left(\mathrm{\Delta }{S}_{BH}\right).(52)$

Eqs 40–42, 49–52 indicate that due to Lorentz-breaking, both the space–time background and quantum tunneling radiation are affected to some extent. A series of conclusions obtained above are of certain practical significance for research on black hole thermodynamics.

4 Conclusion

First, it should be noted that this term containing f in Eq. 25 does not appear in the abovementioned series of results, which is because $\mathrm{\Delta }{|}_{r\to r_H}=0$ makes $\mathrm{\Delta }\left(m^2-2f{\gamma }_0^5\right){|}_{r\to r_H}=0$. However, if we research the non-thermal radiation of the black hole, there must be a term containing f in the distribution of Dirac positive and negative energy levels of spin 1/2 fermions in the space–time of such a black hole. This term appearing in the expression of the Dirac energy level distribution will have a certain influence on the particle energy level distribution. Since ${\gamma }_0^5\propto \frac{1}{r^2}$ and ${\gamma }_0^5{|}_{r\to \infty }=0$, there is still a correct conclusion that the Dirac energy level, when r → ∞, is approaching to ± m. Second, when ℓ = 0, the abovementioned series of results correspond to the Kerr–Sen black hole. When ℓ = 0 and b = 0, the abovementioned results correspond to the relevant results of the classical Kerr black hole. When ℓ ≠ 0, a = 0 and b = 0, the abovementioned results correspond to the results of Schwarzschild-like black hole. When ℓ = 0, a = 0 and b = 0, the abovementioned results correspond to the Schwarzschild black hole.

In the previous literature on the modification of the quantum tunneling radiation of the black hole by Lorentz-breaking, the modification of the space–time background by Lorentz-breaking was generally not considered [12–15]. Therefore, the novelty of the abovementioned research content is that the Lorentz-breaking modification of space–time background and the fermion dynamics equation are taken into account at the same time, and a series of novel outcomes are obtained. In a future in-depth research process, we should consider the effects of Lorentz-breaking theory on the space–time background of static, stationary, and non-stationary black holes. In the process of measuring the shadow of the black hole, for example, we should take into account the effect of Lorentz-breaking theory. All these research contents should continuously enrich the content of quantum gravity theory research.

For bosons, the abovementioned methods cannot be used to research the dynamic characteristics of bosons, and it is necessary to research the dynamic behavior of bosons from the modified form of the scalar field equation. For other static, stationary, and non-stationary black holes, the abovementioned research methods can be used to study the quantum tunneling radiation characteristics of fermions and the physical significance of black hole temperature and black hole entropy modification. We need to deeply understand the conclusions mentioned above, especially the profound meaning of black hole entropy. Black hole entropy is one of the important physical quantities in black hole physics, and it is directly proportional to the area of the black hole. According to the second law of black hole thermodynamics, the area of a black hole will never decrease in the clockwise direction, which means that black hole entropy will not decrease. When we explore black hole entropy from the perspective of quantum theory, black hole entropy is directly proportional to the area of the event horizon measured in the Planck area, which is a scientific project worthy of in-depth research.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.

Author contributions

XT: formal analysis, software, validation, writing–original draft, and writing–review and editing. CW: software and writing–review and editing. S-ZY: conceptualization, data curation, methodology, and writing–original draft.

Funding

The authors declare that financial support was received for the research, authorship, and/or publication of this article. This work is supported by the National Natural Science Foundation of China (No. 12373109).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. Jha SK, Rahaman A. Bumblebee gravity with a kerr-sen like solution and its shadow. Eur Phys J C (2021). 81:345. doi:10.1140/epjc/s10052-021-09132-6

CrossRef Full Text | Google Scholar

2. Carleo A, Lambiase G, Mastrototaro L. Energy extraction via magnetic reconnection in lorentz breaking kerr-sen and kiselev black holes. Eur Phys J C (2022). 82:776. doi:10.1140/epjc/s10052-022-10751-w

CrossRef Full Text | Google Scholar

3. Banerjee R, Majhi BR. Connecting anomaly and tunneling methods for the hawking effect through chirality. Phys Rev D (2009). 79:064024. doi:10.1103/physrevd.79.064024

CrossRef Full Text | Google Scholar

4. Kruglov SI. Modified Dirac equation with lorentz invariance violation and its solutions for particles in an external magnetic field. Phys Lett B (2012). 718:228–31. doi:10.1016/j.physletb.2012.10.037

CrossRef Full Text | Google Scholar

5. Amelino-Camelia G. Phenomenology of planck-scale lorentz-symmetry test theories. New J Phys (2004). 6:188. doi:10.1088/1367-2630/6/1/188

CrossRef Full Text | Google Scholar

6. Magueijo J, Smolin L. Generalized lorentz invariance with an invariant energy scale. Phys Rev D (2003). 67:044017. doi:10.1103/physrevd.67.044017

CrossRef Full Text | Google Scholar

7. Ellis JR, Mavromatos NE, Sakharov VS. Synchrotron radiation from the crab nebula discriminates between models of space-time foam. Astropart Phys (2004). 20:669–82. doi:10.1016/j.astropartphys.2003.12.001

CrossRef Full Text | Google Scholar

8. Kruglov SI. Modified wave equation for spinless particles and its solutions in an external magnetic field. Mod Phys Lett A (2013). 28:1350014. doi:10.1142/s0217732313500144

CrossRef Full Text | Google Scholar

9. Yang SZ, Chen DY. A new method to study hawking tunneling radiation of the charged particles from ressiner-nordström black hole. Int J Theor Phys (2007). 46:1747–52. doi:10.1007/s10773-006-9306-x

CrossRef Full Text | Google Scholar

10. Yang SZ, Lin K. Hawking tunneling radiation in lorentz-violating scalar field theory. Acta Phys Sin (2019). 68:060401. doi:10.7498/aps.68.20182050

CrossRef Full Text | Google Scholar

11. Li R, Yu ZH, Yang SZ. Modification of bekenstein-hawking entropy of cylindrical symmetric black hole by lorentz breaking theory. EPL (2023). 141:50001. doi:10.1209/0295-5075/acbb9b

CrossRef Full Text | Google Scholar

12. Tan X, Zhang J, Li R. Correction of lorentz breaking theory to quantum tunneling radiation and entropy of black hole in gravity’s rainbow. Phys Scripta (2023). 98:105015. doi:10.1088/1402-4896/acf810

CrossRef Full Text | Google Scholar

13. Tan X, Liu YZ, Liu ZE, Sha B, Zhang J, Yang S. Modification of the dynamic equation and tunneling radiation of fermions with arbitrary spin in kerr-newman-kasuya black hole space-time. Mod Phys Lett A (2020). 35:2050168. doi:10.1142/s0217732320501680

CrossRef Full Text | Google Scholar

14. Zhang J, Liu ZE, Sha B. Influence of lorentz invariation violation on arbitrarily spin fermions tunneling radiation in the vaidya-bonner spacetime. Adv High Energ Phys. (2020). 2020:2742091. doi:10.48550/arXiv.2002.05946

CrossRef Full Text | Google Scholar

15. Sha B, Liu ZE, Liu YZ, Tan X, Zhang J, Yang SZ. Accurate correction of arbitrary spin fermion quantum tunneling from non-stationary kerr-de sitter black hole based on corrected lorentz dispersion relation. Chin Phys C (2020). 44:125104. doi:10.1088/1674-1137/abb4d6

CrossRef Full Text | Google Scholar

16. Liu ZE, Tan X, Liu YZ, Sha B, Zhang J, Yang SZ. Hawking radiation of reissner-nordström-de sitter black hole with a global monopole der ived from modified hamilton-jacobi equation. Can J Phys (2021). 99:451–4. doi:10.1139/cjp-2020-0402

CrossRef Full Text | Google Scholar

17. Javed F, Mustafa G, Mumtaz S, Atamurotov F. Thermal analysis with emission energy of perturbed black hole in f(Q) gravity. Nucl Phys B (2023). 990:116180. doi:10.1016/j.nuclphysb.2023.116180

CrossRef Full Text | Google Scholar

18. Rakhimova G, Atamurotov F, Javed F, Abdujabbarov A, Mustafa G. Thermodynamical analysis of charged rotating black hole surrounded by perfect fluid dark matter. Nucl Phys B (2023). 996:116363. doi:10.1016/j.nuclphysb.2023.116363

CrossRef Full Text | Google Scholar

19. Abbas G, Ali RH, Mustafa G. Thermodynamical analysis with extended phase transition of ads hairy black hole in gravitational decoupling theory. Phys Scr (2024). 99:045025. doi:10.1088/1402-4896/ad340a

CrossRef Full Text | Google Scholar

20. Carrol S, Field G, Jackiw R. Limits on a lorentz-and parity-violating modification of electrodynamics. Phys Rev D (1990). 41:1231–40. doi:10.1103/physrevd.41.1231

CrossRef Full Text | Google Scholar

21. Jackiw R, Kostelecky VA. Radiatively induced lorentz and violation in electrodynamics. Phys.Rev.Lett. (1999). 82:3572–5. doi:10.1103/physrevlett.82.3572

CrossRef Full Text | Google Scholar

22. Coleman S, Glashow SL. High-energy tests of lorentz invariance. Phys Rev D (1999). 59:116008. doi:10.1103/physrevd.59.116008

CrossRef Full Text | Google Scholar

23. Kostelecky VA. Gravity, lorentz violation, and the standard model. Phys Rev D (2004). 69:105009. doi:10.1103/physrevd.69.105009

CrossRef Full Text | Google Scholar

24. Belich H, Costa-Soares T, Ferreira MM, Helayel-Neto JA. Non-minimal coupling to a Lorentz-violating background and topological implications. Eur Phys J C (2005). 41:421–6. doi:10.1140/epjc/s2005-02240-y

CrossRef Full Text | Google Scholar

25. Belich H, Colatto LP, Costa-Soares J, Helayël-Neto JA, Orlando MTD. Magnetic moment generation from non-minimal couplings in a scenario with lorentz-symmetry violation. Eur Phys J C (2009). 62:425–32. doi:10.1140/epjc/s10052-009-1017-z

CrossRef Full Text | Google Scholar

26. Klinkhammer FR, Schrec KM. Consistency of isotropic modified maxwell theory: microcausality and unitarity. Nucl Phys B (2011). 848:90–107. doi:10.1016/j.nuclphysb.2011.02.011

CrossRef Full Text | Google Scholar

27. Klinkhammer FR, Schrec KM. Models for low-energy lorentz violation in the photon sector: addendum to ’consistency of isotropic modified maxwell theory. Nucl Phys B (2012). 856:666–9. doi:10.1016/j.nuclphysb.2011.11.019

CrossRef Full Text | Google Scholar

28. Nascimento JR, Passos E, Petrov AY, Brito FA. Lorentz-cpt violation, radiative corrections and finite temperature. JHEP (2007). 0706:016. doi:10.1088/1126-6708/2007/06/016

CrossRef Full Text | Google Scholar

29. Gomes M, Nascimento JR, Petrov AY, da Silva AJ. Aetherlike lorentz-breaking actions. Phys Rev D (2010). 81:045018. doi:10.1103/physrevd.81.045018

CrossRef Full Text | Google Scholar

30. Nascimento JR, Yu Petrov A, Reyes CM. Lorentz-breaking theory with higher derivatives in spinor sector. Phys Rev D (2015). 92:045030. doi:10.1103/physrevd.92.045030

CrossRef Full Text | Google Scholar

31. Pu J, Yang SZ, Lin K. Lorentz-violating theory and tunneling radiation characteristics of Dirac particles in curved spacetime of vaidya black hole. Acta Phys Sin (2019). 68:190401. doi:10.7498/aps.68.20190437

CrossRef Full Text | Google Scholar

32. Yang SZ, Lin K. Modified fermions tunneling radiation from Kerr-Newman-de Sitter black hole. Sci Sin-phy Mech Astron (2019). 49:019503. doi:10.1360/sspma2018-00307

CrossRef Full Text | Google Scholar

33. Yang SZ, Lin K, Li J, Jiang QQ. Lorentz invariance violation and modified hawking fermions tunneling radiation. Adv High Energ phys. (2016). 2016:1–7. doi:10.1155/2016/7058764

CrossRef Full Text | Google Scholar