- 1Theoretical Division, Los Alamos National Laboratory, Los Alamos, NM, United States
- 2MPA-Q, Los Alamos National Laboratory, Los Alamos, NM, United States
Parity or quadratic spin (e.g.,
1 Introduction
In the context of optical interferometry with non-classical states of light, the optical twin Fock (TF) state was introduced as a candidate probe state that minimizes phase fluctuations between the arms of an interferometer [1]. The operating principle of the interferometer is that although the input TF state has maximal phase uncertainty prior to entering a Mach–Zehnder (MZ) interferometer, its phase variance after the first beamsplitter is lower by a factor of O(I−1) compared to a probe state consisting of single-mode lasers of the same total intensity I per measurement interval (lowering the optical standard quantum limit sensitivity of O(I−1) to optical Heisenberg limit sensitivity O(I−2)). For present-day laser-based gravitational wave detectors, suppression of phase and amplitude fluctuations across the operating spectrum motivates the use of novel non-classical electromagnetic field probe states. In the LIGO interferometer, for example, phase fluctuations in the quantum electromagnetic field give rise to the photonic shot noise that constitutes the primary limitation to sensitivity in the high-frequency domain. Fluctuations in the amplitude quadrature give rise to the radiation pressure noise that constitutes the primary limitation to sensitivity in the low-frequency domain [2, 3]. Therefore, preparation of a probe field with frequency-dependent quadrature squeezing enables globally improved noise spectral densities [4, 3]. However, even at fixed wavelength, state-of-the-art optical TF states with O(1) photons are produced only probabilistically [5] and not with intensities large enough to be relevant for application in LIGO.
On the other hand, matter–wave interferometry with ensembles of phase-coherent atoms provides an alternative framework for gravitational wave detection [6, 7]. Analogous to the optical TF state, a TF state of massive bosons produced by strong repulsion of atoms in a double-well optical trap could potentially improve the sensitivity of such matter–wave interferometers beyond the atomic standard quantum limit (1/N scaling of the optimal estimator variance, where N is the number of atoms). While traditional atom interferometers apply optimal Bragg-splitting schemes to non-entangled states such as a Bose–Einstein condensate to produce an optimal non-entangled probe state [8, 9], the input TF state exhibits particle entanglement [10] and remains entangled throughout the MZ interferometer sequence. As far as experimental atom interferometry is concerned, the sensitivity boost provided by TF states can allow smaller MZ loops to be used in the interferometry sequence. TF states of neutral atoms in an optical dipole trap have been prepared with O(104) atoms [11].
This work focuses on the parameter estimation setting defined using an MZ interferometer [12], which applies the operation
where
In the remainder of this section, we review relevant background on quantum sensing aspects of twin Fock states. In Section 2, we move on to considering the possibility of saturating the inequality (Eq. 1) when applying practical readout schemes to pure, but imperfectly prepared, twin Fock states. We generalize the method of moments error of a single readout observable to a generalized signal-to-noise ratio that quantifies correlated errors of a list of non-commuting observables. Observables are identified for which the generalized signal-to-noise ratio globally saturates the QFI (i.e., saturates the QFI for all θ). Sections 3 and 4, respectively, identify lists of observables associated with a generalized signal-to-noise ratio which globally saturates the QFI for the case of lossy twin Fock probe states and for instances of lossy, spatially extended twin Fock probe states. The spatially extended twin Fock states probe multiple spatially separated interferometric phase shifts, which define a multiparameter sensing setting relevant to, for example, spatially resolved gravimetry and magnetometry. Section 5 summarizes the results and discusses directions for future research.
We now define the TF state and provide background on their known properties related to quantum metrology. The TF state of N bosonic atoms distributed between two orthogonal single-particle modes |0⟩ and |1⟩ is the zero Jz weight vector in a spin-N/2 representation of SU(2). It is the
where x = x1…xN ∈ {0,1}N is a binary string identified with a computational basis element of N qubits and Ham(x) is its Hamming weight. The notation in the left-hand side owes to the Schwinger boson form of the Jz operator: 2Jz = a†a − b†b so that
Our model of the MZ interferometer is the standard one: a two-mode, mode-separable bosonic state |ψ⟩ is parametrized by a phase according to the following equation:
Eq. 3 is an example of the shift model of parameter estimation [13]. The TF state possesses the following optimality property for sensing the phase difference in the arms of the MZ interferometer: it has the largest QFI on the unitary path generated by Jy over all mode-separable probe states with a fixed total number of particles N = a†a + b†b, where N is even [15]. The operator Jy generates the phase difference dynamics of the MZ interferometer.
As pointed out by Lang and Caves, mode-separable states (or product states in the setting of optical MZ interferometry) are the only sensible input states to consider in single-parameter MZ interferometry. The whole purpose of the first beamsplitter is to generate the quantum coherence required for the phase difference sensing task. If one has access to mode-entangled states at the input of the MZ interferometer, an optimal input state is a Greenberger–Horne–Zeilinger (GHZ) state (recall that for N atoms, the GHZ states are a class of states defined as an equal amplitude superposition of the highest and lowest eigenvectors of a total spin operator
Recent work has shown that Dicke states |N − m, m⟩ can be prepared with
2 Pure imperfect TF probes
Unlike other entangled probe states of two-mode bosons such as one-axis-twisted states [30], the physical origin of the sensitivity of TF states for interferometry is not spin-squeezing [31]. Rather, it is the property of low uncertainty in the difference of phase (near a phase difference of zero or π) appearing between the paths of the MZ interferometer after the TF state undergoes the first beamsplitting [32]. In fact, the Dicke state amplitudes of the π/2-rotated TF state are greatest on the |N, 0⟩ and |0, N⟩ states, similar to an approximate GHZ state. Although a GHZ state in the arms of the MZ interferometer allows obtaining the minimal uncertainty of an estimator of the MZ phase, the input state required to generate such a configuration is simply a rotated GHZ state, which is clearly not any easier to generate. By contrast, in the case of ultracold atoms, the TF state can be prepared as the ground state of N atoms in a double-well optical trap [33, 34], which makes it practically advantageous compared to a GHZ probe. Furthermore, loss of a single particle from a GHZ state renders it useless for interferometry beyond O(N) scaling of the QFI [i.e., standard quantum limit (SQL) scaling]. Photon-loss-robust optical states for MZ interferometry were explored as GHZ alternatives in [35].
In this section, we consider the case of pure, but imperfectly prepared, twin Fock states. Such states coincide with sequences of Dicke states of the form
which is independent of θ. The particle number dependence in Eq. 4 indicates the possibility of Heisenberg scaling, i.e., O(N2), but not the Heisenberg limit N2, which is only achievable by a GHZ probe state. In fact, this value of the QFI is attained for any spin generator
To locally saturate the QFI, we first review two practical measurement schemes that have appeared in the literature. A Bayesian estimation scheme locally achieving the same scaling as the QFI was discussed in [1, 38]. However, a single-mode parity measurement (we will use
the method of moments error
where we recall the formula for the method of moments error
of a phase estimator

Figure 1. (A) Error of the method of moments estimator
It should be noted that a parity measurement requires single-particle resolution at a detector. For high-intensity optical systems or a high-occupation two-mode bosonic system, such an idealistic measurement is not always feasible. By contrast, it would be desirable to use low moments of a measurement of a total spin operator to extract a high-precision estimator. It has been shown that for a TF state input, the method of moments error for an estimator obtained from the measurement of
Theorem 1. Let
If m scales with N as
Proof: We use the moments of Jz in Supplemental Section 1 to find that at θ = 0, the method of moments error
The ratio of the reciprocal of Eq. 9 to the QFI is given by the following equation:
which satisfies Eq. 8. The last statement in the theorem follows from taking m to satisfy the scaling assumption in the statement and noting that Eq. 10 implies that
It should be noted that
holds for general parameterized states ρθ, and K = 2 with
It is worth noting that the N-particle one-axis-twisted probe state [30]
at oversqueezed interaction times t = O(N−α), with 0 < α < 1/2, has asymptotic QFI equal to
Last, we note that a measurement in the Jy phase basis
(eigenvectors of
3 Phase-diffused and lossy TF probes
Interatomic interactions during the interferometric sequence lead to the phenomenon of bosonic phase diffusion [47, 48, 49]. Unlike optical phase diffusion, which is modeled by applying random unitary optical rotations to a continuous-variable probe state [50, 51], bosonic phase diffusion is a unitary error which reduces the phase coherence in the arms of the interferometer. In a modern context, the fact that one-axis twisting can reduce the performance of atom interferometry may seem surprising given that the one-axis-twisted split Bose–Einstein condensate
Applying the MZ interferometer to Eq. 14 and using the fidelity with
We now analyze the QFI after loss of K atoms from the TF state, with the aim of identifying an asymptotic scaling of K with N that allows better-than-SQL scaling to persist. Analyses of mixtures of Dicke states with an uncertain total number of particles appear in [38] and with uncertain spin projection in [54]. The performance of the
where p0 = (0,…,0,1)⊺ is a vector of length m + 1, Qk is a sequence of (m + 1) × (m + 1) bidiagonal, bistochastic matrix defined by non-zero elements
for i = 1, …, m, and nk = N − k is the particle number after the loss of k particles, k = 0, …, K. The dynamics occurs on the space of probability distributions on the discrete set {0, …, m} due to the fact that loss of an atom cannot increase the Hamming weight that defines a Dicke state. To apply Eq. 15 to the TF state, we take m = N/2 and numerically compute pK and note that the final state of N − K particles
where
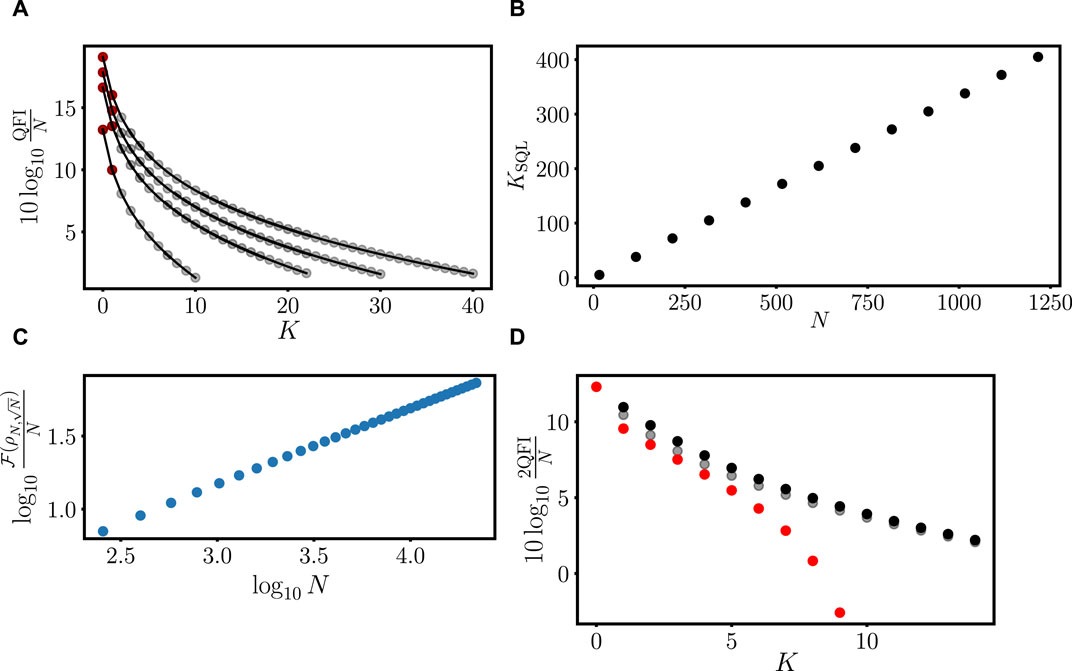
Figure 2. (A) QFI after the application of
Unlike the case for the noiseless TF probe in Section 2, it is not possible to saturate the QFI
is the probability of observing ℓ particles in the second mode, it can be found that a particle number-resolving measurement does not provide enough information to construct an estimator of θ with error saturating the inverse QFI for K > 1. Similar to [54], we find that the greatest sensitivity is obtained away from θ = 0, even for a combined
These results indicate that neither a measurement in the occupation number basis nor a measurement in the Jy phase state basis produces enough information to saturate the QFI for a lossy twin Fock probe. However, we verified numerically that there indeed exists a minimal list
is sufficient, and for
is sufficient. Remarkably, a phase observable with eigenvectors in Eq. 13 or the non-linear parity observable
From the discussion in Section 2, we recall that a sequence of corrupted (but pure) TF states
4 Gradiometry with doubled TF and states
Advantages of using pure, spatially split spin-squeezed atomic ensembles for magnetic gradiometry (estimation of the difference θ1 − θ2) beyond SQL were outlined in [20]. Generally, when using atom interferometers for distributed sensing (i.e., sensing a field at many different spatial points), there are two classes of quantum sensing strategies that can be used, which are straightforward generalizations of their single-parameter sensing counterparts [42]. The parallel strategies use identical copies of a probe state or a single global entangled state as a probe which addresses the various points of interest. The probe state is locally parametrized by phases corresponding to the local field values, with the parametrization usually modeled by a tensor product of parametrized quantum channels. By contrast, the sequential strategies expose an initial probe state (which may be entangled with an ancilla register) to the points of interest in temporal succession. The preference of strategy depends on the cost of probe state preparation and the dwell time of the transient that must be sensed. It has been shown that in the parallel generalization of the MZ interferometry protocol which uses general linear optical unitaries in lieu of the
One possible sequential strategy to estimate
However, it should be noted that for any global rotation
where the spin operators act in a spin-N/2 representation on the left-hand side and a spin-
Instead, we consider two parallel strategies in which globally entangled states are used to estimate linear functions of the phases θj, j = 1, 2. The first parallel strategy is especially relevant when the initial TF state is a single atomic cloud with N/2 occupation in each of two internal modes, for example, atomic nuclear spin states. The initial TF cloud is then spatially split (we assume perfect splitting) to give the TF probe state in the superposition of spatial modes.
It should be noted that the original internal modes a and b of the TF state have been spatially split to spatial modes a1 and a2 and b1 and b2, respectively. The local phase shifts are imprinted on |ψsplit⟩ by the operation
The second parallel strategy uses the same local phase shift operation UMZ(θ) but uses as probe the doubled TF state
in which two spin modes and two spatial modes are occupied symmetrically. The state (Eq. 24) can also be considered as two copies of bosonic-independent and identically distributed (b.i.i.d.) TF states [58] which, mathematically, correspond to taking the Young product of two copies of the symmetric subspace of
The property of the QFI matrix being a scalar multiple of the identity holds also for the probe state obtained by losing K particles from Eq. 24. This fact follows because taking the trace over K particles produces a statistical mixture of four-mode Dicke states, and the 1 ↔ 2 label symmetry of the state is preserved. It should be noted that atom loss from Eq. 24 is not a strictly local process to the MZ interferometers and cannot be described by, for example, applying the loss channels
The black dots of Figure 2D show the gain over the SQL
5 Discussion
We analyzed the method of moments readouts for the TF state and its images under a particle loss channel or unitary phase-diffusion channel. The traditional parity measurement, which saturates the QFI for MZ interferometry near θ = 0 for all Dicke states, can be dispensed with if one can measure both quadratic spin observables
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.
Author contributions
TV: conceptualization, data curation, formal analysis, investigation, methodology, resources, software, supervision, validation, visualization, writing–original draft, and writing–review and editing. CR: conceptualization, funding acquisition, resources, and writing–review and editing.
Funding
The authors declare that financial support was received for the research, authorship, and/or publication of this article. The authors acknowledge support from the Laboratory Directed Research and Development (LDRD) Program at Los Alamos National Laboratory (LANL). Los Alamos National Laboratory is managed by Triad National Security, LLC, for the National Nuclear Security Administration of the U.S. Department of Energy under Contract No. 89233218CNA000001.
Acknowledgments
The authors thank Katarzyna Krzyzanowska, Sivaprasad Omanakuttan, and Jonathan Gross for helpful discussions.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Supplementary material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2024.1369786/full#supplementary-material
References
1. Holland MJ, Burnett K. Interferometric detection of optical phase shifts at the Heisenberg limit. Phys Rev Lett (1993) 71:1355–8. doi:10.1103/PhysRevLett.71.1355
2. Caves CM. Quantum-mechanical noise in an interferometer. Phys Rev D (1981) 23:1693–708. doi:10.1103/PhysRevD.23.1693
3. Ganapathy D, Jia W, Nakano M, Xu V, Aritomi N, Cullen T, et al. Broadband quantum enhancement of the LIGO detectors with frequency-dependent squeezing. Phys Rev X (2023) 13:041021. doi:10.1103/PhysRevX.13.041021
4. McCuller L, Whittle C, Ganapathy D, Komori K, Tse M, Fernandez-Galiana A, et al. Frequency-dependent squeezing for advanced LIGO. Phys Rev Lett (2020) 124:171102. doi:10.1103/PhysRevLett.124.171102
5. Cooper M, Wright LJ, Söller C, Smith BJ. Experimental generation of multi-photon Fock states. Opt Express (2013) 21:5309–17. doi:10.1364/OE.21.005309
6. Dimopoulos S, Graham PW, Hogan JM, Kasevich MA, Rajendran S. Atomic gravitational wave interferometric sensor. Phys Rev D (2008) 78:122002. doi:10.1103/PhysRevD.78.122002
7. Abe M, Adamson P, Borcean M, Bortoletto D, Bridges K, Carman SP, et al. Matter-wave atomic gradiometer interferometric sensor (MAGIS-100). Quan Sci Technol (2021) 6:044003. doi:10.1088/2058-9565/abf719
8. Kasevich M, Chu S. Atomic interferometry using stimulated Raman transitions. Phys Rev Lett (1991) 67:181–4. doi:10.1103/PhysRevLett.67.181
9. Wu S, Wang YJ, Diot Q, Prentiss M. Splitting matter waves using an optimized standing-wave light-pulse sequence. Phys Rev A (2005) 71:043602. doi:10.1103/PhysRevA.71.043602
10. Wiseman HM, Vaccaro JA. Entanglement of indistinguishable particles shared between two parties. Phys Rev Lett (2003) 91:097902. doi:10.1103/PhysRevLett.91.097902
11. Lücke B, Scherer M, Kruse J, Pezzé L, Deuretzbacher F, Hyllus P, et al. Twin matter waves for interferometry beyond the classical limit. Science (2011) 334:773–6. doi:10.1126/science.1208798
12. Yurke B, McCall SL, Klauder JR. SU(2) and SU(1,1) interferometers. Phys Rev A (1986) 33:4033–54. doi:10.1103/PhysRevA.33.4033
13. Holevo A. Probabilistic and statistical aspects of quantum theory. Amsterdam: North-Holland (1982).
14. Yu S. Quantum Fisher information as the convex roof of variance. arXiv preprint arXiv:1302.5311 (2013).
15. Lang MD, Caves CM. Optimal quantum-enhanced interferometry. Phys Rev A (2014) 90:025802. doi:10.1103/PhysRevA.90.025802
16. Duivenvoorden K, Terhal BM, Weigand D. Single-mode displacement sensor. Phys Rev A (2017) 95:012305. doi:10.1103/PhysRevA.95.012305
17. Vaneph C, Tufarelli T, Genoni MG. Quantum estimation of a two-phase spin rotation. Quan Measurements Quan Metrology 1 (2013) 12–20. doi:10.2478/qmetro-2013-0003
18. Fujiwara A. Estimation of SU(2) operation and dense coding: an information geometric approach. Phys Rev A (2001) 65:012316. doi:10.1103/PhysRevA.65.012316
19. Gessner M, Smerzi A, Pezzè L. Multiparameter squeezing for optimal quantum enhancements in sensor networks. Nat Commun (2020) 11:3817. doi:10.1038/s41467-020-17471-3
20. Fadel M, Yadin B, Mao Y, Byrnes T, Gessner M. Multiparameter quantum metrology and mode entanglement with spatially split nonclassical spin ensembles. New J Phys (2023) 25:073006. doi:10.1088/1367-2630/ace1a0
21. Bärtschi A, Eidenbenz S. Short-depth circuits for Dicke state preparation. IEEE Int Conf Quan Comput Eng (Qce) (2022) 87–96. doi:10.1109/QCE53715.2022.00027
22. Kajtoch D, Witkowska E. Quantum dynamics generated by the two-axis countertwisting Hamiltonian. Phys Rev A (2015) 92:013623. doi:10.1103/PhysRevA.92.013623
23. Jiang Z, Rieffel EG, Wang Z. Near-optimal quantum circuit for Grover’s unstructured search using a transverse field. Phys Rev A (2017) 95:062317. doi:10.1103/PhysRevA.95.062317
24. Lange K, Peise J, Lücke B, Kruse I, Vitagliano G, Apellaniz I, et al. Entanglement between two spatially separated atomic modes. Science (2018) 360:416–8. doi:10.1126/science.aao2035
25. Krischek R, Schwemmer C, Wieczorek W, Weinfurter H, Hyllus P, Pezzé L, et al. Useful multiparticle entanglement and sub-shot-noise sensitivity in experimental phase estimation. Phys Rev Lett (2011) 107:080504. doi:10.1103/PhysRevLett.107.080504
26. Zhou L, Kong J, Lan Z, Zhang W. Dynamical quantum phase transitions in a spinor Bose-Einstein condensate and criticality enhanced quantum sensing. Phys Rev Res (2023) 5:013087. doi:10.1103/PhysRevResearch.5.013087
27. Niezgoda A, Kajtoch D, Dziekańska J, Witkowska E. Optimal quantum interferometry robust to detection noise using spin-1 atomic condensates. New J Phys (2019) 21:093037. doi:10.1088/1367-2630/ab4099
28. Guan Q, Biedermann GW, Schwettmann A, Lewis-Swan RJ. Tailored generation of quantum states in an entangled spinor interferometer to overcome detection noise. Phys Rev A (2021) 104:042415. doi:10.1103/PhysRevA.104.042415
29. Mao TW, Liu Q, Li XW, Cao JH, Chen F, Xu WX, et al. Quantum-enhanced sensing by echoing spin-nematic squeezing in atomic Bose-Einstein condensate. Nat Phys (2023) 19:1585–90. doi:10.1038/s41567-023-02168-3
30. Kitagawa M, Ueda M. Squeezed spin states. Phys Rev A (1993) 47:5138–43. doi:10.1103/PhysRevA.47.5138
31. Lücke B, Peise J, Vitagliano G, Arlt J, Santos L, Tóth G, et al. Detecting multiparticle entanglement of Dicke states. Phys Rev Lett (2014) 112:155304. doi:10.1103/PhysRevLett.112.155304
32. Campos RA, Gerry CC, Benmoussa A. Optical interferometry at the Heisenberg limit with twin Fock states and parity measurements. Phys Rev A (2003) 68:023810. doi:10.1103/PhysRevA.68.023810
33. Gietka K, Chwedeńczuk J. Atom interferometer in a double-well potential. Phys Rev A (2014) 90:063601. doi:10.1103/PhysRevA.90.063601
34. Grond J, Hohenester U, Mazets I, Schmiedmayer J. Atom interferometry with trapped Bose–Einstein condensates: impact of atom–atom interactions. New J Phys (2010) 12:065036. doi:10.1088/1367-2630/12/6/065036
35. Dorner U, Demkowicz-Dobrzanski R, Smith BJ, Lundeen JS, Wasilewski W, Banaszek K, et al. Optimal quantum phase estimation. Phys Rev Lett (2009) 102:040403. doi:10.1103/PhysRevLett.102.040403
36. Pezzè L, Smerzi A, Oberthaler MK, Schmied R, Treutlein P. Quantum metrology with nonclassical states of atomic ensembles. Rev Mod Phys (2018) 90:035005. doi:10.1103/RevModPhys.90.035005
37. Hyllus P, Gühne O, Smerzi A. Not all pure entangled states are useful for sub-shot-noise interferometry. Phys Rev A (2010) 82:012337. doi:10.1103/PhysRevA.82.012337
38. Meiser D, Holland MJ. Robustness of Heisenberg-limited interferometry with balanced Fock states. New J Phys (2009) 11:033002. doi:10.1088/1367-2630/11/3/033002
39. Bollinger JJ, Itano WM, Wineland DJ, Heinzen DJ. Optimal frequency measurements with maximally correlated states. Phys Rev A (1996) 54:R4649–52. doi:10.1103/PhysRevA.54.R4649
40. Gerry CC. Heisenberg-limit interferometry with four-wave mixers operating in a nonlinear regime. Phys Rev A (2000) 61:043811. doi:10.1103/PhysRevA.61.043811
41. Gerry CC, Campos RA, Benmoussa A. Comment on “Interferometric detection of optical phase shifts at the Heisenberg limit”. Phys Rev Lett (2004) 92:209301. doi:10.1103/PhysRevLett.92.209301
42. Giovannetti V, Lloyd S, Maccone L. Quantum metrology. Phys Rev Lett (2006) 96:010401. doi:10.1103/PhysRevLett.96.010401
43. Kim T, Pfister O, Holland MJ, Noh J, Hall JL. Influence of decorrelation on Heisenberg-limited interferometry with quantum correlated photons. Phys Rev A (1998) 57:4004–13. doi:10.1103/PhysRevA.57.4004
44. Volkoff TJ, Martin MJ. Saturating the one-axis twisting quantum Cramér-Rao bound with a total spin readout. J Phys Commun (2024) 8:015004. doi:10.1088/2399-6528/ad1dc8
45. Davis E, Bentsen G, Schleier-Smith M. Approaching the Heisenberg limit without single-particle detection. Phys Rev Lett (2016) 116:053601. doi:10.1103/PhysRevLett.116.053601
46. Volkoff TJ, Martin MJ. Asymptotic optimality of twist-untwist protocols for Heisenberg scaling in atom-based sensing. Phys Rev Res (2022) 4:013236. doi:10.1103/PhysRevResearch.4.013236
47. Javanainen J, Wilkens M. Phase and phase diffusion of a split Bose-Einstein condensate. Phys Rev Lett (1997) 78:4675–8. doi:10.1103/PhysRevLett.78.4675
48. Sanders BC, Milburn GJ. Optimal quantum measurements for phase estimation. Phys Rev Lett (1995) 75:2944–7. doi:10.1103/PhysRevLett.75.2944
49. Jo GB, Shin Y, Will S, Pasquini TA, Saba M, Ketterle W, et al. Long phase coherence time and number squeezing of two Bose-Einstein condensates on an atom chip. Phys Rev Lett (2007) 98:030407. doi:10.1103/PhysRevLett.98.030407
50. Vidrighin MD, Donati G, Genoni MG, Jin XM, Kolthammer WS, Kim MS, et al. Joint estimation of phase and phase diffusion for quantum metrology. Nat Commun (2014) 5:3532. doi:10.1038/ncomms4532
51. Genoni MG, Olivares S, Paris MGA. Optical phase estimation in the presence of phase diffusion. Phys Rev Lett (2011) 106:153603. doi:10.1103/PhysRevLett.106.153603
52. Pezzé L, Smerzi A. Phase sensitivity of a Mach-Zehnder interferometer. Phys Rev A (2006) 73:011801. doi:10.1103/PhysRevA.73.011801
53. Uys H, Meystre P. Quantum states for Heisenberg-limited interferometry. Phys Rev A (2007) 76:013804. doi:10.1103/PhysRevA.76.013804
54. Apellaniz I, Lücke B, Peise J, Klempt C, Tóth G. Detecting metrologically useful entanglement in the vicinity of Dicke states. New J Phys (2015) 17:083027. doi:10.1088/1367-2630/17/8/083027
55. Braunstein SL, Caves CM. Statistical distance and the geometry of quantum states. Phys Rev Lett (1994) 72:3439–43. doi:10.1103/PhysRevLett.72.3439
56. Ge W, Jacobs K, Eldredge Z, Gorshkov AV, Foss-Feig M. Distributed quantum metrology with linear networks and separable inputs. Phys Rev Lett (2018) 121:043604. doi:10.1103/PhysRevLett.121.043604
57. Proctor TJ, Knott PA, Dunningham JA. Multiparameter estimation in networked quantum sensors. Phys Rev Lett (2018) 120:080501. doi:10.1103/PhysRevLett.120.080501
58. Volkoff TJ. Distillation of maximally correlated bosonic matter from many-body quantum coherence. Quantum (2020) 4:330. doi:10.22331/q-2020-09-24-330
59. Gessner M, Pezzè L, Smerzi A. Sensitivity bounds for multiparameter quantum metrology. Phys Rev Lett (2018) 121:130503. doi:10.1103/PhysRevLett.121.130503
Keywords: quantum metrology, atom interferometry, gradiometry, non-Gaussian states, quantum Cramér–Rao inequality
Citation: Volkoff TJ and Ryu C (2024) Globally optimal interferometry with lossy twin Fock probes. Front. Phys. 12:1369786. doi: 10.3389/fphy.2024.1369786
Received: 12 January 2024; Accepted: 22 February 2024;
Published: 20 March 2024.
Edited by:
Nan Li, Chinese Academy of Sciences (CAS), ChinaReviewed by:
Ma Hongyang, Qingdao University of Technology, ChinaLu Zhou, East China Normal University, China
Copyright © 2024 Volkoff and Ryu. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: T. J. Volkoff, dm9sa29mZkBsYW5sLmdvdg==
 T. J. Volkoff
T. J. Volkoff Changhyun Ryu
Changhyun Ryu