- 1Department of Physics, Mekdela Amba University, Dessie, Ethiopia
- 2Department of Physics, Dilla University, Dilla, Ethiopia
The main objective of this manuscript is to focus on the computational study of the interplay of spin density wave (SDW) and superconductivity using a two-band model for SrFe2−xNixAs2. We derived mathematical statements for the superconducting critical temperature, SDW critical temperature, superconductivity order parameter, and the SDW order parameter using the Hamiltonian model and Green’s function formalism for the SrFe2−xNixAs2 superconductor. A mathematical expression for the dependence of transition temperatures on the SDW order parameter was obtained for SrFe2−xNixAs2. Using these mathematical statements, transition temperatures versus the SDW order parameter phase diagrams were plotted to show the dependence of the SDW order parameter on transition temperatures. By merging these diagrams, we have depicted the intriguing possibility of the interplay of superconductivity and magnetism for the SrFe2−xNixAs2 superconductor. Phase diagrams of temperature versus superconducting order parameters and the SDW order parameter were also plotted to show the dependence of order parameters on temperature for the SrFe2−xNixAs2 superconductor.
1 Introduction
Superconductivity was observed for the first time by a Dutch physicist Heike Kamerlingh Onnes. He found in 1911 that the electrical resistivity of Hg abruptly dropped to zero [1]. After 20 years of his discovery, in 1933, W. Meissner and his student R. Ochsenfeld found that the magnetic field is repelled by superconducting materials, called the Meissner effect [2]. This effect suggests a fundamental property of the superconducting state called perfect diamagnetism. In 1957, L. N. Cooper, J. Bardeen, and J. R. Schrieffer, the three American physicists, developed a quantum theory, called BCS theory, to elucidate the behaviour of superconducting materials at the microscopic level [3]. This theory claims that the formation of quasiparticles termed Cooper pairs by the electrons in a material leads to superconductivity [4]. Cooper pairs are bosons and can aggregate in the same low-energy fundamental state (ground state energy), which is the superconducting state. It bases on a critical assumption that there is an attractive force between electrons. Electrons are fermions and are subject to the Pauli exclusion principle [5].
In the development of condensed matter physics, the discovery of high-transition temperature Tc superconductors marks a turning point. In 1979, the discovery of superconductivity in the heavy-fermion compound, CeCu2Si2 [6], came as a surprise because magnetic spin–spin interactions bind the superconducting charge carriers in pair and is highly unlikely by BCS theory [7]. The heavy-fermion system is the first class of unconventional superconductors. K. A. Muller and J. G. Bednorz discovered cuprates, the second class of high-temperature superconductors, in La2−xBaxCuO4 with Tc = 35K [8]. Iron-based superconductors (FebSc), with Tc = 26K in LaOFeAs, are the other family of high-temperature materials. They were identified by Hosono et al., in 2008 [9].
FebScs have various distinct systems that significantly enlarge the class of unconventional superconducting materials. Numerous FebSc systems with various compositions and crystal structure classes are found still now. Different systems are distinguished for convenience by the stoichiometric proportions of the chemical components of their parent molecules [10, 11]. The most common FebSc systems that use this nomenclature are 245, 1144, 42622, 12442, 1111, 111, 11, and 122 (for example, Rb2Fe4Se5, CsEuFe4As4, Sr4V2O6Fe2As2, RbCa2Fe4As4F2, SmO1−xFxFeAs, NaFeAs, Fe1+yTe1−xSex, and SrFe2−xNixAs2) [12–16]. In the 245 FebSc system, Rb2Fe4Se5, there is a unique phase separation phenomenon, unlike most other FebSc systems, and each phase separation has arrived from the competition between magnetic and superconducting ordering in the material [17, 18]. Despite having various structural variations, all FebSc systems have one thing in common: iron-based square-planar sheets [19]. FebSc systems contain iron-based layers, which are essential for their superconductivity, similar to copper oxide-based superconductors, in which oxygen—together with copper—creates the superconductivity layer [20].
According to theories, the parent materials of FebSc are semi-metallic, and the density of state close to Fermi’s surface is primarily supplied by the iron 3d electrons (orbitals) and all five of the 3d orbitals cross Fermi’s surface [21]. Just from the most experimental results and the band structure calculations, the results reveal three iron 3d orbitals (dxy, dyz, and dxz) provide the primary contribution to the density of states near the Fermi level and they ruffle weakly in the z direction [22]. Two electron pockets are situated at the margin of the Brillouin zone (BZ) point, and two hole pockets are distributed evenly throughout the resultant Fermi surface. The weights of the three orbitals, (
Within the two-orbital model, the electron Fermi pocket is close to the (π, 0) point and is made up of the orbital dyz, whereas the hole Fermi pocket around the (0, 0) point is made up of a combination of dxz and dyz orbitals. The inter-band spin fluctuation resulting from this nesting is actually the particle hole scattering that occurs between the dxz and dyz orbitals since the component of the hole Fermi pocket coupled by the nesting wave vector (π, 0) is primarily of the dxz orbital characteristic [24]. The amount of doping affects how the electrical band structure is shaped. In electron-doped materials, such as 122 Fe-based superconductor compounds, the Fermi surface has many quasi 2D warped cylinders centred Γ point around (k = 0, 0) and M (k = π, π), as well as a potential quasi 3D pocket close to kz = π. For electron-doped 122 systems such as SrFe2−xNixAs2, the electron Fermi pocket expands as the doping level rises, while the hole Fermi pocket contracts until at the strongly doped level, where the hole Fermi pocket eventually disappears. The opposite is true for hole-doped systems [20].
The spin density wave (SDW) state, first proposed by Overhauser in 1962, is where the electronic spin density forms a static wave and makes it a type of anti-ferromagnetic state [25]. It happens in anisotropic low-dimensional compounds at low temperatures. The spin and SDW are coupled. It describes the spin density periodic modulation specified by the Fermi wave number [26]. There is no net magnetization over the entire volume with the density varying perpendicularly as a function of position. When delocalized or itinerant electrons, rather than localized electrons, are responsible for the spatial spin density modulation and the SDW transition takes place. Its origin can be electron–hole pairing or finite wave vector singularities of the magnetic susceptibility [27]. It is Fermi surface nesting that is responsible for SDW stabilization. Nesting of the Fermi surface and SDW are observed in SrFe2As2 [28]. Although the SDW transition leads to the establishment of AFM order, which causes the moment of the iron atoms to become still along the longer axis in the orthorhombic phase, and the orthorhombic deformation of the crystal lattice transforms the structure of the crystal from the tetragonal to the orthorhombic phase [29].
The FeAs tetrahedral layers are present in the parent compounds of the 122 iron pnictide superconductors, which also show structural transitions from tetragonal to orthorhombic and from paramagnetic (PM) to anti-ferromagnetic (AFM) structures [30]. It has been demonstrated that the AFM orthorhombic phase is the parent phase of the pnictide superconductor that produces the SDW state, which can be suppressed to produce superconductivity by applying pressure or through chemical doping. It has been demonstrated that the parent pnictide compound structural and magnetic phase transitions are crucial in causing superconductivity [31]. The structural and magnetic transitions in our compound SrFe2−xNixAs2 occur simultaneously at TS = TN ≈ 205K [25, 32, 33]. The transition can be tracked to the concentration x = 0.15, where TS (TN) ≈ 40K [34], but TS (TN) disappears for x > 0.15 or it is not observed in x = 0.16, which leads to maximum Tc [35]. In the concentration range 0.10 ≤ x ≤ 0.22, superconductivity is observed for SrFe2−xNixAs2. As the doping level [x in SrFe2−xNixAs2] increases, the hole Fermi pocket continuously shrinks, whereas the electron pocket becomes larger, and the hole pocket finally vanishes in the heavily doped region, where superconductivity also disappears. Magnetic transition is strongly coupled with the structural distortion. Superconductivity has been demonstrated that the result from the suppression of the AFM state of SrFe2As2 by nickel substitution. The suppression of the long-range magnetic ordering and the sudden appearance of the Sc state at the same time are correlated, which suggests that the spin fluctuation of the Fe moments is crucial in creating the superconductive state [36]. The closeness of structural and magnetic transitions suggests that spin and lattice coupling has occurred. Lower symmetry enables the spins to organise and, therefore, reduce magnetic frustrations, which is assumed to be the source of the crystal deformation [37].
FebSc are so interesting because they show the coexistence of superconductivity and magnetism. Therefore, they show a lot of promise for applications. They are desirable for electrical power stations and magnetic applications (Maglev trains) because they have a substantially larger critical magnetic field than cuprates or heavy fermions and high isotropic critical currents. Superconductivity shows potential to have a significant impact on our community and the realisation of a world with minimal carbon emissions [38]. In this article, the researchers study the coexistence of superconductivity and SDW in a two-band model for the iron-based superconductor SrFe2−xNixAs2 by using Green’s function formalism.
Based on the concepts of electronic structure, this article is investigated theoretically on the coexistence of superconductivity and SDW in nickel substitution of the strontium–iron–arsenide (SrFe2−xNixAs2) superconductor in a two-band model. By considering a two-band model of Hamiltonian and using the double-time temperature-dependent Green’s function formalism methodology, researchers tried to discover the mathematical statements for the critical temperatures (Tc and TM) and order parameters (ΔSc and M).
2 Mathematical formulation of the problem
We explore a two-band model that encapsulates the fundamental physics of the multi-band unconventional superconducting state and SDW order and has a self-consistent solution in order to investigate the coexistence of superconductivity and SDW. The mean-field model Hamiltonian for the interplay of SDW and superconductivity in our compound in a two-band model (dyz, say s and dxz, say d) can be expressed as [39–41]
where the first term indicates the energy of conduction electrons in the s-band. The second term indicates the energy of conduction electrons in the d-band. The third and fourth terms are the energies involving superconductivity due to the intra-band interactions at the s- and d-bands, respectively. The fifth and sixth terms are the energies involving superconductivity due to the inter-band interaction between bands s and d. The last term is the mean-field Hamiltonian which describes the magnetic interactions. ɛs(k) and ɛd(k) are energies of an electron measured with respect to the Fermi energy in s and d bands, respectively.
where Us is the intra-band interaction potential in the s-band.
where Ud is the intra-band interaction potential in the d-band.
where Usd is the inter-band interaction potential between the two bands.
The double-time temperature-dependent Green’s function is used to find the equation of motion. This formalism is defined as
Rr (t − t′) is boson operators’ retarded response function.
The Dirac’s delta function δ(t − t′) is related to the Heaviside step function as
This implies the following:
Now let us introduce the Heisenberg equations of motion as
Substituting Eq. 8 and Eq. 9 into Eq. 6, we have the following:
Let Rr(ω) be the Fourier transform of Rr (t − t′), which will be given by
The inverse Fourier transform is
The first-order derivative of Eq. 11 with time will be
The Dirac delta function will be defined as
Substituting Eqs 13, 14 into Eq. 10, we have
Last but not the least, the formalism for the double-time temperature-dependent Green’s function is
Here,
For simplification, Eq. 1 can be written as
where
This is s intra-band interaction’s mean-field Hamiltonian.
This is d intra-band interaction’s mean-field Hamiltonian.
This is the mean-field Hamiltonian in the inter-band interaction
This is the mean-field Hamiltonian due to the magnetic interaction of conduction electrons.
2.1 Superconducting order parameters in the pure superconducting region
2.1.1
To describe the superconducting order parameter due to the intra-band interaction within the s-band in the pure superconducting region, one can use the equation of motion for the correlation
The commutation relations in Eq. 19 can be solved as follows:
Ignoring Eq. 23 and inserting Eq. 20 to Eq. 22 into Eq. 19, we get
The equation of motion for the homologous
Evaluating the commutation relations in Eq. 29 which yields,
Inserting Eq. 30 to Eq. 32 in Eq. 29, we get
Substituting Eq. 36 in Eq. 27, we get
By ignoring
By decoupling Eq. 43 using the partial fraction decomposition method, we have
Using the expression ω → iωn, where ωn is the Matsubara frequency and written as
Substituting Eq. 50 in Eq. 51, we have
Let
Let us change summation into integration in the region −ℏωF < ɛs(k) < ℏωF, and at the Fermi level, the density of state, Ns(o), is
Let α = UsNs(o), which is called the superconducting coupling constant in the s-band intra-band interaction.
Case I: If T → 0, β → ∞ this implies that
By applying the integral relation
From the concept of BCS theory, the superconducting order parameter
This gives
where Us = 0.323meV, Ns(o) = 1.5 (meV)−1, kB = 0.086 meV/K and ℏωF = 6 meV [29]. Substituting these experimental values, we get Tc = 10.09K that agrees with the experiment.
Case 2: If 0 < T < Tc, then Eq. 57 is simplified as follows:
From the Laplacian transform with the Matsubara relation result, we can write Eq. 65 as
Applying the following equality
From the above equality relations
For low temperature,
From Eq. 74, we have
Using logarithmic series
Leaving the high-order terms, we get the following.
This equation tells us the superconducting order parameter
2.1.2
By applying the same procedure as above, the superconducting transition temperature Tc due to the intra-band interactions within the d-band is written as
where Ud = 0.267meV, Nd(o) = 1.80 (meV)−1, kB = 0.086 meV/K and ℏωF = 6 meV [29]. Substituting these experimental values in this equation, we get Tc = 9.92K that agrees with the experiment. The dependence of the superconducting order parameter
Eq. 88 indicates the dependence of the superconducting order parameter on temperature in the d-band intra-band interaction in the pure superconducting region. The superconducting order parameter decreases as the temperature increases. It vanishes at Tc (9.92K). At T = 0,
2.1.3
The inter-band interaction between the s- and d-bands causes the superconducting order parameter, which can be connected to Green’s function as
Applying the same procedure, the equation of motion for the correlations
Ignoring the intra-band superconducting order parameter terms and by decoupling these equations using the partial fraction decomposition method, we will have
where
Let ɛs(k) = ɛd(k) in the inter-band interaction between the two bands, and Eq. 90 becomes
Let
Changing summation into integration in the region −ℏF < ɛd(k) < ℏωF, at the Fermi level, the density of state in the inter-band interaction is Nsd(o), that is,
After a couple of steps, the superconducting transition temperature Tc is given by
where Usd = 0.297meV, Nd(o) = 1.80 (meV)−1, Ns(o) = 1.5 (meV)−1, kB = 0.086 meV/K, and ℏωF = 6 meV [29]. Eq. 96 clearly shows that the superconducting transition temperature depends on the SDW order parameter.
In the pure diamagnetism region M = 0, and Eq. 91 becomes
This equation shows that the dependence of the superconducting order parameter on the temperature in the inter-band interactions between the s- and d-bands. The superconducting order parameter suppresses as the temperature increases. It vanishes at the superconducting transition temperature (Tc = 10.20K). If
2.2 SDW order parameter (M)
Using the double-time temperature-dependent Green’s function to the equation of motion for the correlation
The commutation relations in Eq. 99 can be solved as follows:
Substituting Eq. 100 to Eq. 103 in Eq. 99, it gives
Now, let us find the equation of motion for the correlation
Solving the commutation relations in Eq. 105, we get
Substituting Eq. 106 to Eq. 109 in Eq. 105, we get
Substituting Eq. 110 into Eq. 104 and after some mathematics, we get
where
The magnetic order parameter is given by
Substituting Eq. 112 into Eq. 113; transforming summation into integration in the boundary −ℏωF < ɛs(k) < ℏωF and by presenting the density of state (DOS) at the Fermi level is N(o), that is,
After some steps, we get
For small values of M, we ignore the M2 term. Thus, Eq. 116 reduces to
where UN(o) = 1.68 [29] and it is the SDW coupling parameter. Eq. 117 shows that the SDW order parameter increases as the SDW transition temperature increases.
For the pure magnetic region
This is simplified to
For a little value of M, M2 → 0 and T → TM. Eq. 119 reduces
This implies that
Eq. 121 simplifies to
Eq. 123 indicates that if temperature rises, the SDW order parameter suppresses.
3 Results and discussion
In this part, we discussed how temperature (T) affects superconducting order parameters (ΔSc) and the SDW order parameter (M), and M affects on both the SDW transition temperature (TM) and superconducting transition temperature (Tc). We expand on the analysis. In a two-band model for SrFe2−xNixAs2, we created the theoretical examination of the coexistence of superconductivity and SDW. With the aid of a two-band Hamiltonian model and the double-time temperature-dependent Green’s function formal consideration, we were able to derive the mathematical expressions for the superconducting transition temperature (Tc), superconducting order parameters for each intra- and inter-band interactions, SDW order parameter (M), and SDW transition temperature (TM).
From Eqs 63, 87, 96, we obtain the superconducting transition (critical) temperatures for each intra- and inter-band interactions of SrFe2−xNixAs2. Using these (Tc) values and Eqs 85, 88, 97, respectively, we plotted the phase diagram of ΔSc versus T within each intra-band and inter-band interactions, Figure 1A.

FIGURE 1. Superconducting order parameters vs. temperature for each interactions of the SrFe2−xNixAs2 superconductor (A) and SDW order parameter (M) vs. temperature in the pure magnetic region of the SrFe2−xNixAs2 superconductor (B).
As illustrated in Figure 1A, the superconducting order parameter decreases as the temperature increases. It vanishes as the temperature is equal to the critical temperature. For the s intra-band interaction, the maximum value of the superconducting order parameter,
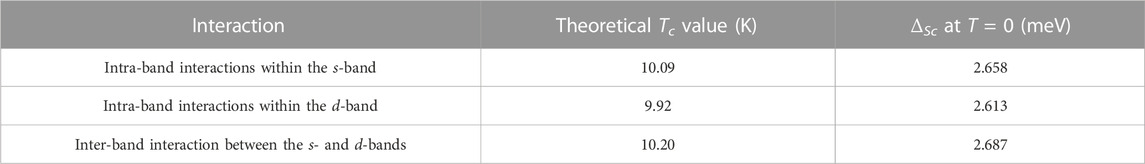
TABLE 1. Superconducting transition temperature Tc values and the superconducting order parameter at T = 0 in different interactions for our compound SrFe2−xNixAs2. The mean value of Tc is nearly 10K.
Based on Eq. 123, we plotted the phase illustration for the dependence of the magnetic order parameter (M) on temperature in the pure magnetic region illustrated in Figure 1B. As illustrates from this figure, magnetism decreases as the temperature enhances and vanishes at the SDW transition temperature TM = 205K. The maximum value of the SDW order parameter, M = 54 meV, occurs at T = 0. This finding is also in agreement with experimental observations [34, 37].
Based on Eq. 96, we plotted the phase diagrams of Tc versus M. As illustrated from Figure 2A, when the value of the SDW order parameter enhances, the superconducting transition temperature is suppressed for SrFe2−xNixAs2. From this figure, one can see the SDW order parameter promotes the magnetic nature and suppresses superconductivity in the system.

FIGURE 2. Superconducting transition temperature (TC) versus the SDW order parameter(M) for the inter-band interaction of the SrFe2−xNixAs2 superconductor (A) and SDW transition temperature (TM) versus SDW order parameter(M) of SrFe2−xNixAs2 (B).
Using Eq. 117, we also plotted the phase plotting of the SDW transition temperature (TM) versus the SDW order parameter (M), as seen in Figure 2B. As demonstrated from this figure, (TM) progressively gets bigger with the SDW order parameter of the SrFe2−xNixAs2 superconductor.
Finally, by combining Figures 2A, B, this article depicted a region where both SDW and superconductivity coexist, as shown in Figure 3. Because of their coexistence, the iterating superconducting electrons and spins are thought to have a weak exchange coupling for SrFe2−xNixAs2. This figure shows that the possible interplay of superconductivity and SDW for SrFe2−xNixAs2. As indicated in this figure, our finding is in agreement with experimental observations [34, 37]. This figure also depicts there are regions that show the superconducting and anti-ferromagnetic states segregate, which indicates that there are regions where magnetic and superconducting phases are not mixed.
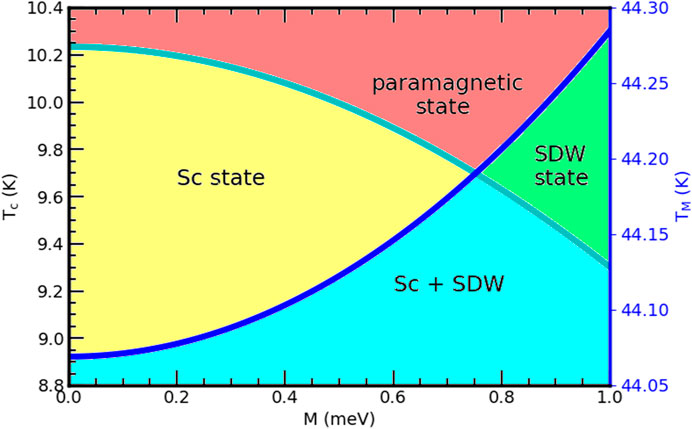
FIGURE 3. Superconducting critical temperature and magnetic transition temperature vs. the SDW order parameter for the SrFe2−xNixAs2 superconductor.
4 Conclusion
In this work, we have studied the possibility of coexisting superconductivity and magnetism for the iron-based superconductor SrFe2−xNixAs2. The superconductivity order parameter for FebSc SrFe2−xNixAs2 is suppressed as the temperature raises and vanishes at the superconducting critical temperature. The magnitude of the SDW order parameter for SrFe2−xNixAs2 is suppressed as the superconducting critical temperature increases and increases with increasing the SDW transition temperature. We depicted the possibility of coexisting superconductivity and magnetism in the SrFe2−xNixAs2 superconductor. For the iron-based superconductor SrFe2−xNixAs2, we further studied the reliance of the SDW order parameter M on temperature in the pure magnetic region. When temperature increases, the SDW order parameter is suppressed and is zero at the SDW transition temperature of the SrFe2−xNixAs2 superconductor.
Data availability statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author contributions
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. John R. Solid state physics 1st ed. India: New Delhi McGraw-Hill Education (2014). p. 24. 110016.
2. Kim CJ, Kim CJ. History of superconductivity Singapore. Superconductor Levitation: Concepts and Experiments (2019). p. 1–11. doi:10.1007/978-981-13-6768-7_1
3. Raychaudhuri P, Dutta S. Phase fluctuations in conventional superconductors. J Phys Condensed Matter (2021) 34(8):083001. doi:10.1088/1361-648X/ac360b
4. Zhu Z, Papaj M, Nie XA, Xu HK, Gu YS, Yang X, et al. Discovery of segmented Fermi surface induced by Cooper pair momentum. Science (2021) 374(6573):1381–5. doi:10.1126/science.abf1077
5. Qiu D, Gong C, Wang S, Zhang M, Yang C, Wang X, et al. Recent advances in 2D superconductors. Adv Mater (2021) 33(18):2006124. doi:10.1002/adma.202006124
6. Naqib SH, Islam RS. Possible quantum critical behavior revealed by the critical current density of hole doped high-T c cuprates in comparison to heavy fermion superconductors. Scientific Rep (2019) 9(1):14856. doi:10.1038/s41598-019-51467-4
7. Bussmann-Holder A, Keller H. High-temperature superconductors: underlying physics and applications. Z für Naturforschung B (2020) 75(1-2):3–14. doi:10.1515/znb-2019-0103
8. Maksimovic N. Advances in nearly-magnetic superconductivity. Berkeley: University of California (2022).
9. Li H. Spectroscopic–imaging scanning tunneling microscopy studies on cuprates high temperature superconductors. United States: Stony Book Doctoral dissertation, State University of New York at Stony Brook (2021).
10. Rasaki SA, Thomas T, Yang M. Iron based chalcogenide and pnictide superconductors: from discovery to chemical ways forward. Prog Solid State Chem (2020) 59:100282. doi:10.1016/j.progsolidstchem.2020.100282
11. Carretta P, Prando G. Iron-based superconductors: tales from the nuclei. La Rivista Del Nuovo Cimento (2020) 43(1):1–43. doi:10.1007/s40766-019-0001-1
12. Fernandes RM, Coldea AI, Ding H, Fisher IR, Hirschfeld PJ, Kotliar G. Iron pnictides and chalcogenides: a new paradigm for superconductivity. Nature (2022) 601(7891):35–44. doi:10.1038/s41586-021-04073-2
13. Kreisel A, Hirschfeld PJ, Andersen BM. On the remarkable superconductivity of FeSe and its close cousins. Symmetry (2020) 12(9):1402. doi:10.3390/sym12091402
14. Roter B, Ninkovic N, Dordevic SV. Clustering superconductors using unsupervised machine learning. Physica C: Superconductivity its Appl (2022) 598:1354078. doi:10.1016/j.physc.2022.1354078
15. Kim TK, Pervakov KS, Evtushinsky DV, Jung SW, Poelchen G, Kummer K, et al. Electronic structure and coexistence of superconductivity with magnetism in RbEuFe4As4. Phys Rev B (2021) 103(17):174517. doi:10.1103/PhysRevB.103.174517
16. Yu AB, Huang Z, Zhang C, Wu YF, Wang T, Xie T, et al. Superconducting anisotropy and vortex pinning in CaKFe4As4 and KCa2Fe4As4F2. Chin Phys B (2021) 30(2):027401. doi:10.1088/1674-1056/abcf98s
17. Park JT, Friemel G, Li Y, Kim JH, Tsurkan V, Deisenhofer J, et al. Magnetic resonant mode in the low energy spin-excitation spectrum of superconducting Rb2Fe4Se5 single crystals. Phys Rev Lett (2011) 107(17):177005. doi:10.1103/physrevlett.107.177005
18. Charnukha A, Cvitkovic A, Prokscha T, Propper D, Ocelic N, Suter A, et al. Nanoscale layering of antiferromagnetic and superconducting phases in Rb2Fe4Se5 single crystals. Phys Rev Lett (2012) 109(1):017003. doi:10.1103/PhysRevLett.109.017003
19. Gui X, Lv B, Xie W. Chemistry in superconductors. Chem Rev (2021) 121(5):2966–91. doi:10.1021/acs.chemrev.0c00934
20. Bogale GM, Shiferaw DA. High entropy materials-microstructures and properties” in iron-based superconductors. Rijeka: Croatia IntechOpen (2022). p. 1–19. doi:10.5772/intechopen.109045
21. Ma X, Wang G, Liu R, Yu T, Peng Y, Zheng P, et al. Correlation-corrected band topology and topological surface states in iron-based superconductors. Phys Rev B (2022) 106(11):115114. doi:10.1103/PhysRevB.106.115114
22. Tam DW, Berlijn T, Maier TA. Stabilization of s-wave superconductivity through arsenic p-orbital hybridization in electron-doped BaFe 2 as 2. Phys Rev B (2018) 98(2):024507. doi:10.1103/PhysRevB.98.024507
23. Zhang R. Neutron scattering studies of the magnetic excitations in 122 family of iron pnictides (doctoral dissertation. Houston: Texas Rice University (2022).
24. Christensen MH, Kang J, Fernandes RM. Intertwined spin-orbital coupled orders in the iron-based superconductors. Phys Rev B (2019) 100(1):014512. doi:10.1103/PhysRevB.100.014512
25. Gruner G. Density waves in solids. Boca Raton: Florida CRC Press (2018). doi:10.1201/9780429501012
26. Kokanova SV, Maksimov PA, Rozhkov AV, Sboychakov AO. Competition of spatially inhomogeneous phases in systems with nesting-driven spin-density wave state. Phys Rev B (2021) 104(7):075110. doi:10.1103/PhysRevB.104.075110
27. Araujo RDMT, Zarpellon J, Mosca DH. Unveiling ferromagnetism and antiferromagnetism in two dimensions at room temperature. J Phys D: Appl Phys (2022) 55(28):283003. doi:10.1088/1361-6463/ac60cd
28. Ali K, Adhikary G, Thakur S, Patil S, Mahatha SK, Thamizhavel A, et al. Hidden phase in parent Fe-pnictide superconductors. Phys Rev B (2018) 97(5):054505. doi:10.1103/PhysRevB.97.054505
29. Chen X, Liu N, Guo J, Chen X. Direct response of the spin-density wave transition and superconductivity to anion height in SrFe2As2. Phys Rev B (2017) 96(13):134519. doi:10.1103/PhysRevB.96.134519
30. Gati E, Xiang L, Bud’ko SL, Canfield PC. Hydrostatic and uniaxial pressure tuning of iron-based superconductors: insights into superconductivity, magnetism, nematicity, and collapsed tetragonal transitions. Annalen der Physik (2020) 532(10):2000248. doi:10.1002/andp.202000248
31. Wu JJ, Lin JF, Wang XC, Liu QQ, Zhu JL, Xiao YM, et al. Pressure-decoupled magnetic and structural transitions of the parent compound of iron-based 122 superconductors BaFe2As2. Proc Natl Acad Sci (2013) 110(43):17263–6. doi:10.1073/pnas.1310286110
32. Chong SV, Tallon JL, Fang F, Kennedy J, Kadowaki K, Williams GVM. Surface superconductivity on SrF e2As2 single crystals induced by ion implantation. EPL (Europhysics Letters) (2011) 94(3):37009. doi:10.1209/0295-5075/94/37009
33. Hosono H, Fukuyama H, Akai H. Iron-based superconductors. Handbook Superconductivity: Fundamentals Mater One (2022) 1:293–301. doi:10.1201/9780429179181-30
34. Butch NP, Saha SR, Zhang XH, Kirshenbaum K, Greene RL, Paglione J. Effective carrier type and field dependence of the reduced-T c superconducting state in SrFe2−xNixAs2. Phys Rev B (2010) 81(2):024518. doi:10.1103/PhysRevB.81.024518
35. Saha SR, Butch NP, Kirshenbaum K, Paglione J. Annealing effects on superconductivity in SrFe2−xNixAs2. Physica C, Superconductivity (2010) 470:S379–81. doi:10.1016/j.physc.2009.11.079
36. Xie T, Gong D, Ghosh H, Ghosh A, Soda M, Masuda T, et al. Neutron spin resonance in the 112-type iron-based superconductor. Phys Rev Lett (2018) 120(13):137001. doi:10.1103/PhysRevLett.120.137001
37. Saha SR, Butch NP, Kirshenbaum K, Paglione J. Evolution of bulk superconductivity in SrFe2As2 with Ni substitution. Phys Rev B (2009) 79(22):224519. doi:10.1103/PhysRevB.79.224519
38. Yao C, Ma Y. Superconducting materials: challenges and opportunities for large-scale applications. Iscience (2021) 24(6):102541. doi:10.1016/j.isci.2021.102541
39. Kidanemariam T, Kahsay G, Mebrahtu A. Theoretical investigation of the coexistence of superconductivity and spin density wave (SDW) in two-band model for the iron-based superconductor BaFe2(As1−xPx)2. The Eur Phys J B (2019) 92(2):39–12. doi:10.1140/epjb/e2019-80638-9
40. Han Q, Chen Y, Wang ZD. A generic two-band model for unconventional superconductivity and spin-density-wave order in electron-and hole-doped iron-based superconductors. EPL (Europhysics Letters) (2008) 82(3):37007. doi:10.1209/0295-5075/82/37007
Keywords: superconductivity, superconducting transition temperature, superconducting order parameters, SDW transition temperature, SDW order parameter, magnetism
Citation: Bogale GM and Shiferaw DA (2023) Theoretical study of the interplay of spin density wave and superconductivity in nickel substitution of the strontium–iron–arsenide (SrFe2−xNixAs2) superconductor in a two-band model. Front. Phys. 11:1235105. doi: 10.3389/fphy.2023.1235105
Received: 05 June 2023; Accepted: 20 November 2023;
Published: 07 December 2023.
Edited by:
Jeffrey W. Lynn, National Institute of Standards and Technology (NIST), United StatesCopyright © 2023 Bogale and Shiferaw. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Gedefaw Mebratie Bogale, Z2VkZWZhd21lYnJhdGllMjJAZ21haWwuY29t
†These authors have contributed equally to this work
 Gedefaw Mebratie Bogale
Gedefaw Mebratie Bogale Dagne Atnafu Shiferaw2†
Dagne Atnafu Shiferaw2†