- 1College of Education, Zhejiang University, Hangzhou, China
- 2School of Education, Tianjin University, Tianjin, China
- 3Education Science Research Center, Tianjin University, Tianjin, China
Various mechanisms have been proposed to promote cooperation in evolutionary games, including aspiration and social influence. However, the joint effects of aspiration and social influence have so far been ignored in the literature. Thus, we discuss the joint effects of aspiration and social influence on promoting cooperation in the Prisoner’s Dilemma Game (PDG). We introduce different aspiration tags as different aspirations and different types of neighborhoods as different social influences to reveal how the level of cooperation changes in four heterogeneous micro-environments composed of diverse aspirations and social influences. The Fermi function is adopted as the priority strategy updating rule. Simulation results show that aspiration and social influence mechanisms are jointly effective at improving cooperation in the PDG. More precisely, the aspiration mechanism promotes cooperation unconditionally, but that the social influence mechanism promotes it conditionally.
1 Introduction
Cooperation is found in many biological and social systems and typically plays a very important role in the development of these systems [1, 2], so much so that in 2005, Science listed “how did cooperative behavior evolve?” as one of the 25 most critical scientific questions of the 21st century. Indeed, over the past several decades, evolutionary game theory has attracted considerable attention in explaining and exploring the evolution of cooperation [3–6]. Nowak, for example, has devoted his efforts to introducing spatial structure into evolutionary game theory and has put forth five promotion mechanisms for cooperation: kin selection, group selection, direct reciprocity, indirect reciprocity, and network reciprocity [7]. Since this pioneering work, various mechanisms have been proposed to promote cooperation in complex networks, such as reputation [8–11], conformity [12–14], reward and punishment [15–17], heterogeneous investment [18, 19], teaching activity [20, 21], migration [22, 23], noise [24, 25], and memory [26–28]. Aspiration and social influence mechanisms have also begun to garner the attention of many researchers.
In the extant literature, aspiration as an important endogenous feature of individuals has become a vital concern among researchers. Many researchers defined aspiration as the expectation of payoff and introduced a control parameter as the average aspiration level for every individual. A moderate aspiration level has been found to be able to favor cooperative behavior on small-world networks [29] and square lattices [30, 31], when individuals update their strategies synchronously. On the contrary, cooperative behavior is inhibited when the strategy updating is switched to an asynchronous manner. Still, the involvement of extortioners [32] and the mechanism of migration [33] can facilitate the evolution of cooperation. In addition, aspiration mechanism has usually been studied together with imitation rule. For example, Liu et al. indicated that the invasion of aspiration-driven individuals can boost the cooperation of the imitation-driven individuals [34]. Wang et al. established a new mixed model of combining imitation and aspiration and discussed the effects of aspiration on improving the level of cooperation in different populations, including well-mixed population, structured population, and random matching model [35]. Arefin et al. investigated the coevolution of strategy updating rules of both aspiration and imitation [36]. Furthermore, some researchers proposed a different definition of aspiration, which means the willingness to choose the strategy of a neighbor whose payoff is the highest. They pointed out that heterogeneous aspirations can enhance network reciprocity and resolve social dilemmas [37–39].
Another mechanism, social influence, is also regarded as a crucial factor in an individual’s strategy selection. Some researchers believe that the evolution of cooperation can be enhanced when social influence is integrated into the strategy updating process, whether in the PDG [40], PGG [41, 42], and Snowdrift Games (SDG) [43]. Nevertheless, Zong et al. [44] argued that social influence does not always promote cooperation. They classified social influence into weak social influence and strong social influence, and indicated that weak social influence usually inhibits cooperation, while strong social influence has a mediating effect. Inspired by the classification of social influence of Zong et al., we also divided social influence into strong social influence and weak social influence in this study in order to explore the effect of social influence mechanism in the PDG.
Although the effects of aspiration and social influence mechanisms have been explored extensively, there are still three research gaps in the existing literature. First, aspiration has always linked to payoffs, either the expected payoffs of the individual or the highest payoffs of the individual’s neighbor. In the real world, however, payoff may not be the only interest for individuals when they interact with others [39]. The other meanings of aspiration have not received much attention. Second, the joint effects of social influence and other mechanisms have yet to be sufficiently studied, and the joint effects of aspiration and social influence specifically have so far been ignored. Third, the simulation models in previous papers are almost entirely at the individual level. The effects of various mechanisms on facilitating the evolution of cooperation at the group level have yet to be fully illustrated.
In an attempt to remedy these research gaps, we define aspiration as the individual’s preference for strategy (cooperation or defection) and focus on the joint effects of aspiration and social influence mechanisms at both the individual and group levels in the PDG. Specifically, we consider aspiration as the internal preferences of each individual, which divides the population into positive aspiration (preference for cooperation strategy) group and negative aspiration (preference for defection strategy) group, and we consider social influence as the external characteristic of each individual, which divides the population into strong social influence group and weak social influence group. Based on this classification, individuals with different aspirations and different social influences constitute four kinds of groups, or four heterogeneous micro-environments. This paper investigates the evolution of cooperation in these four micro-environments and reveals the joint effects of aspiration and social influence mechanisms on promoting cooperation in the PDG.
The rest of this paper is structured as follows. In the Section 2 we introduce the details of our model. Large-scale simulation results are used to explain the evolution of cooperation in the Section 3. Finally, we summarize the main findings in the Section 4.
2 Model
We arrange the evolutionary game on a square lattice with periodic boundary conditions. Each node signifies an individual, and each individual has 4 or 8 neighbors. An individual with 4 neighbors is in the “von Neumann” neighborhood, which is considered to have weak social influence (W). An individual with 8 neighbors is in the “Moore” neighborhood, which is considered to have strong social influence (S). We introduce r as the ratio of strong social influence individuals to investigate the effect of social influence mechanism on improving the level of cooperation. Moreover, in our real life, for example, the extravert usually maintains a positive relation to the outside world and enjoys making friends, while the introvert tends to focus more on the inner world and withdraws from social activities [45]. Thus, we introduce an aspiration tag (+ or -) for each individual. An individual with the positive aspiration tag (+) tends to choose cooperation strategy, while an individual with the negative aspiration tag (−) tends to choose defection strategy.
We set the population size as N = L × L (L = 100). Initially, each individual selects one of the two strategies, cooperation (C) or defection (D) with equal probability. During the process of the evolutionary game, if both individuals choose cooperation, they both receive the reward R. If both individuals choose defection, they both receive the punishment P. If one individual chooses the defection strategy and the other chooses the cooperation strategy, the defector gains the temptation T while the cooperator has to bear the cost S. For simplicity, we follow previous studies [46–49] and adopt a variant of the PDG, which is also known as the “weak PDG” [4]. The payoff matrix of this model is as follows:
We use Eq. 2 below to calculate an individual’s accumulated payoff after interacting with all his neighbors. For each individual i, Pi is the accumulated payoff, Ωi is the set of individual i’s neighbors, j is one of individual i’s neighbor in his neighborhood, ui and uj denote the strategies of individual i and his neighbor j, respectively.
During the process of strategy updating, the Fermi function [50] is the priority. That is, after the first step in which an individual chooses cooperation or defection with equal probability, he updates his strategy by learning the strategy of a randomly selected neighbor with probability:
where K refers to environmental noise introduced to permit irrational choices. Without loss of generality, we set K = 0.1. Si is individual i’s strategy, Sj is neighbor j’s strategy (j is a randomly selected neighbor from i’s neighborhood), Pi is individual i’s accumulated payoff, and Pj is neighbor j’s accumulated payoff.
According to Eq. 3, the probability of individual i adopting his neighbor j’s strategy is divided into 3 cases. (1) If Pj < Pi, the probability is between 0 and 1/2. (2) If Pj = Pi, the probability is equal to 1/2. (3) If Pj > Pi, the probability is between 1/2 and 1. When the probability is between 0 and 1/2, the individual maintains his own strategy. When the probability is between 1/2 and 1, he changes the strategy by copying his neighbor’s strategy. However, when the probability is equal to 1/2, the individual updates his strategy according to his aspiration tag. If the aspiration tag is positive (+), the individual chooses cooperation strategy. If the aspiration tag is negative (−), the individual chooses defection strategy. Thus, the strategy updating rule is defined as:
where i denotes the focal individual, and j denotes one of i’s neighbors who was selected at random in i’s neighborhood. Pi and Pj represent the accumulated payoff of i and j, respectively. Si and Sj represent the strategies of i and j, respectively. C refers to cooperation strategy, and D refers to defection strategy.
We use the Monte Carlo method to simulate the evolution of cooperation with 10,000 Monte Carlo steps (MCS). Our focus is on how the fraction of cooperators (Fc) changes under four different micro-environments and the joint effect of aspiration and social influence mechanisms on the evolution of cooperation.
3 Results
The purpose of this work is to explore the joint effects of aspiration and social influence mechanism on promoting cooperation in the PDG and to explain how the level of cooperation changes under heterogeneous micro-environments composed of different aspirations and social influences. In our model, individuals with different aspirations and social influences give us four cases of heterogeneous micro-environments: +S + W (all the individuals have positive aspirations), -S-W (all the individuals have negative aspirations), +S-W (strong social influence individuals have positive aspirations, but weak social influence individuals have negative aspirations), and -S + W (strong social influence individuals have negative aspirations, but weak social influence individuals have positive aspirations). The color plots below show the level of cooperation in the simulation of each of the four different cases.
Figure 1A shows the highest level of cooperation, followed by Figures 1B, C, D. Moreover, as shown in Figure 1A, when b is at a medium-high level, the larger the ratio of strong social influence individuals is, the higher the fraction of cooperators. Thus, we conclude that of the case with positive aspiration and strong social influence results in the most cooperation.
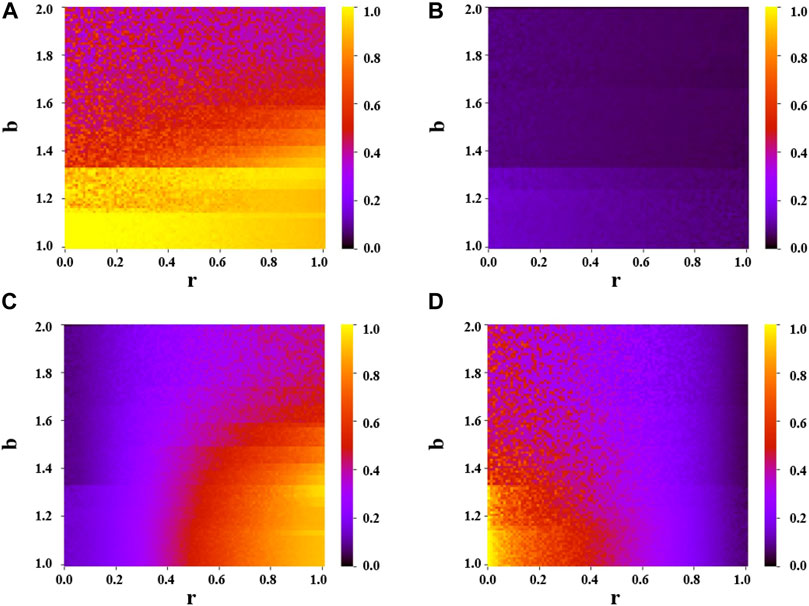
FIGURE 1. Color plots of the level of cooperation in the (A) +S + W case, (B) -S-W case, (C) +S-W case, and (D) -S + W case. The horizontal axis r in each plot signifies the ratio of strong social influence individuals and satisfies 0 ≤ r ≤ 1. The vertical axis b in each plot signifies the temptation to defect and satisfies 1 < b ≤ 2.
Furthermore, we observe that the aspiration mechanism plays a decisive role in promoting cooperation. Positive aspiration always promoted cooperation. Comparing Figures 1A, B, we find that the fraction of cooperators decreases rapidly when the tag of aspiration changes from positive to negative. The same phenomenon can also be seen by comparing Figures 1C, D. The high level of cooperation emerges in the lower right area of Figure 1C, and in Figure 1D, the level of cooperation in the lower left area is high. These two areas are the regions where individuals have the positive aspiration tag. This result tells us that increasing the proportion of individuals with positive aspiration and inspiring the aspiration of all the individuals to cooperate are fundamental to improving the level of cooperation. Additionally, comparing Figures 1A, D, we find that the level of cooperation in the right area of Figure 1D is very low, which is because the strong social influence individuals have negative aspiration to cooperate. That is, although individuals have strong social influence, without positive aspiration to cooperate, they cannot enhance cooperation and may even inhibit it. Likewise, the level of cooperation in the left area of Figure 1D is high when compared to Figure 1B. That is, although individuals have weak social influence, as long as they have positive aspiration to cooperate, they can improve the surrounding environment and promote cooperation. As the Chinese proverb says, do not do evil things though they may be insignificant and do not give up good things though they may be minor matters. Therefore, individuals should maintain positive aspiration to cooperate, no matter the social influence is strong or weak.
By contrast, we observed that the social influence mechanism only promoted cooperation conditionally. Comparing Figures 1C, D, the area of the high level of cooperation in the case of +S-W is larger than that in the case of -S + W, which indicates that strong social influence individuals have a greater effect on facilitating the level of cooperation than weak social influence individuals. However, the effect does not always promote cooperation. As shown in Figures 1A, B, we find that when b is at a medium-high level, as the proportion of strong social influence individuals increases, the fraction of cooperators increases in the case of +S + W but decreases in the case of -S-W. That is, when all the individuals have positive aspirations to cooperate, improving their social influence can promote cooperation, but when all the individuals have negative aspirations to cooperate, improving their social influence undermines cooperation. Therefore, transforming the strong social influence individuals with negative aspiration into positive ones or improving the social influence of individuals with positive aspiration can effectively promote cooperation.
In order to explore the changes of cooperation levels over time, we plot the time evolution of Fc with r = 0.0, 0.2, 0.4, 0.6, 0.8, 1.0, as shown in Figure 2. Here we set b = 1.5 since according to Figure 1, the differences in the four cases are most obvious at this value of temptation.
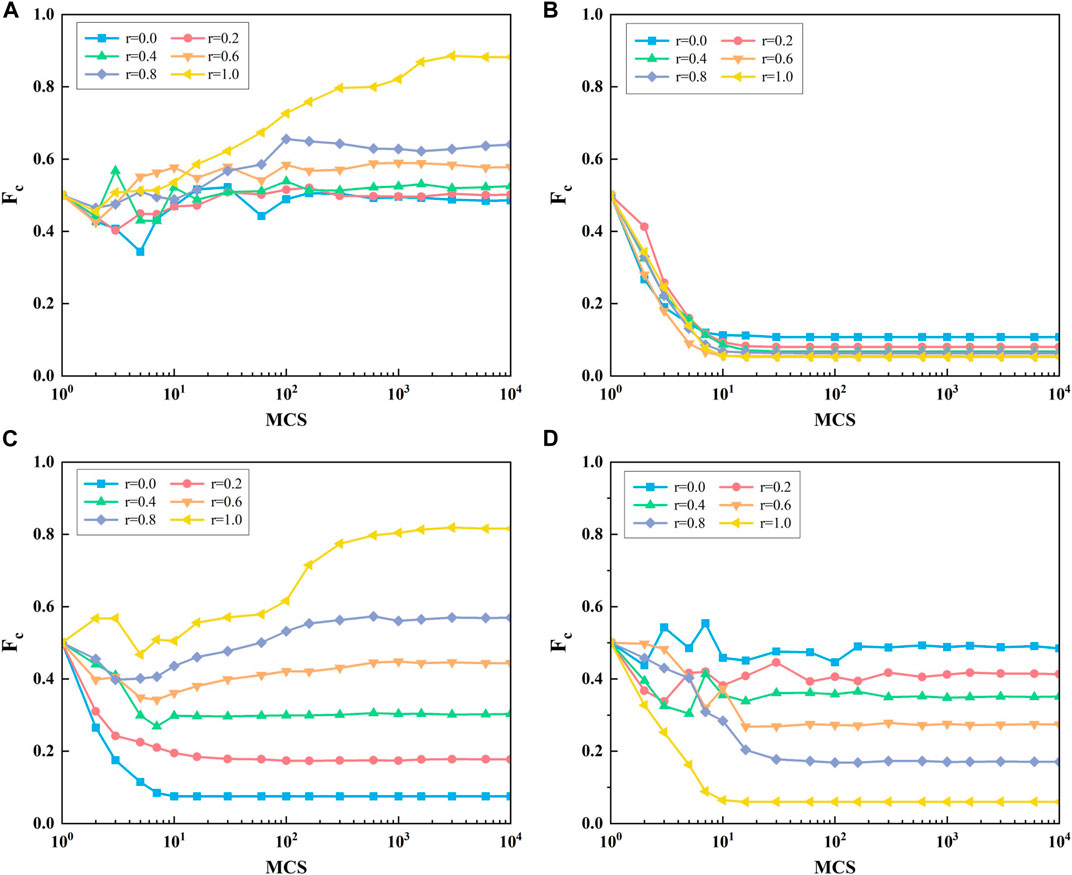
FIGURE 2. Time evolution of Fc with different r values at b = 1.5 in the (A) +S + W case, (B) -S-W case, (C) +S-W case, and (D) -S + W case.
Here we find that after a brief period of fluctuation, at approximately 30 MCS, most of the Fc values gradually become stable. After 1000 MCS all Fc values reach the steady state. The case of +S + W had the highest Fc (close to 0.9) when r = 1.0. This result proves the above conclusion. Furthermore, although these four cases of heterogeneous aspirations and social influences are four micro-environments, they together constitute a macro-environment. Any small change will thus affect the whole environment. For example, as shown in Figures 2B, D, we find that even transforming the aspiration of weak social influence individuals from negative to positive can greatly facilitate cooperation. According to the theory of Parsons [51], society is a unified system composed of many interdependent parts, and if one part changes, it will influence the other parts as well as the whole. In our model, changes in an individual’s behavior can affect their surrounding micro-environment and thus the macro-social system. As the saying goes, no snowflake in an avalanche ever feels responsible. The behavior of individuals is especially critical. Therefore, each individual should make his efforts to maintain a positive aspiration and improve his social influence.
In order to compare the changes of Fc values in the four cases more clearly, we calculated the average Fc values of the 10,000 MCS with different r values and present the variation of Fc, in Figure 3.
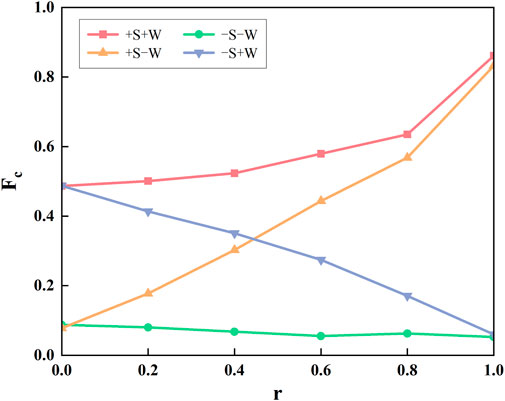
FIGURE 3. The variation of Fc with different r values at b = 1.5 in four heterogeneous micro-environments of different aspirations and social influences.
As shown in Figure 3, the Fc values of individuals with positive aspirations are higher than the Fc values of individuals with negative aspirations, which indicates the unconditional promotion effect on cooperation of aspiration mechanism. However, comparing the lines of +S-W and -S + W, we find that in the case of +S-W, the Fc values increase with r, but in the case of -S + W it decreases with r. This result tells us that the promotion effect on cooperation of social influence mechanism is conditional. When the strong social influence individuals have positive aspirations, increasing their proportion can indeed facilitate more cooperation, but when the strong social influence individuals have negative aspirations, increasing their proportion destroys cooperation.
In order to show the evolution of cooperation in the four cases visually, we selected 16 evolutionary snapshots (see Figure 4) of four kinds of individuals (strong social influence cooperator, strong social influence defector, weak social influence cooperator, weak social influence defector) at r = 0.5.
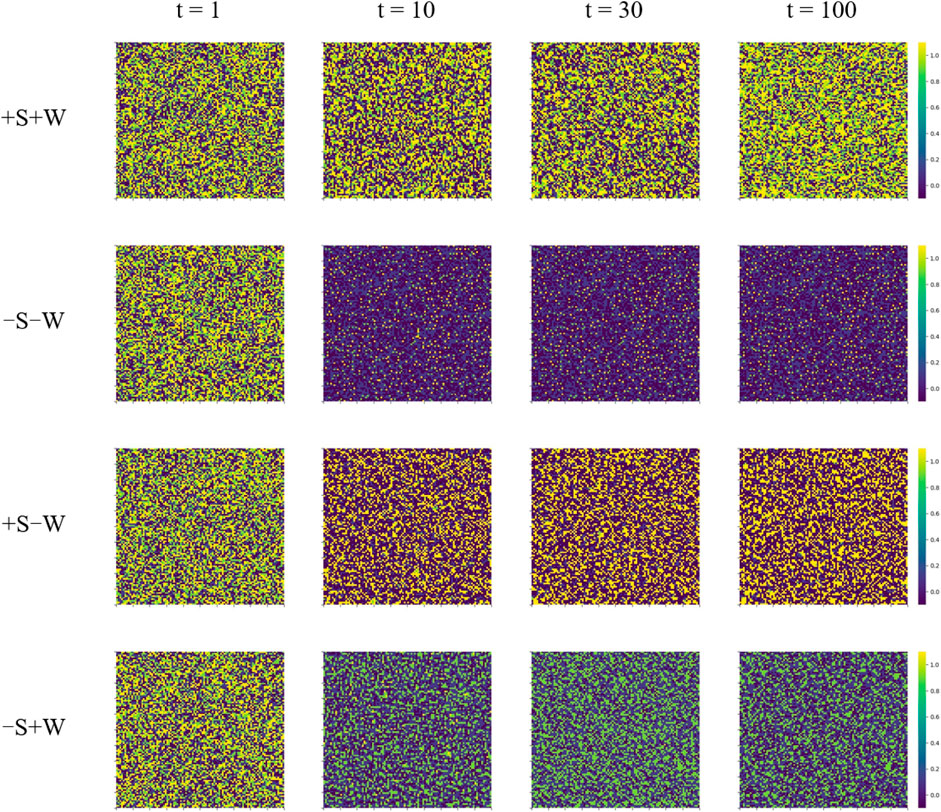
FIGURE 4. Evolutionary snapshots of individuals distribution at various MCS for b = 1.5 and r = 0.5. Strong social influence cooperators, strong social influence defectors, weak social influence cooperators, weak social influence defectors are colored yellow, green, dark blue, and purple black, respectively. The rows depict results for the case of +S + W, -S-W, +S-W, and -S + W from top to bottom. The MCS for each row are 1, 10, 30, and 100 from left to right.
As shown in Figure 4, the fraction of cooperators in the case of +S + W is significantly lower than that in the case of -S-W, which shows the pivotal role of positive aspiration in improving the level of cooperation. Moreover, the level of cooperation in the case of +S-W is higher than that in the case of -S + W, which reveals the important effect of strong social influence on facilitating the evolution of cooperation. These results provide new evidence to illustrate the effects of aspiration and social influence, and prove the previous conclusions once again from a different angle.
4 Conclusion
In this work, we discussed the joint effect of aspiration and social influence on promoting cooperation and how the level of cooperation changes in four heterogeneous micro-environments in the PDG. Different aspirations are the internal preferences of each individual, and were reflected by different aspiration tags, Different social influences are the external characteristics of each individual, and were reflected by different types of neighborhoods. Our simulation results show that in all the micro-environments the aspiration mechanism always promoted cooperation, but that the social influence mechanism only did so in certain circumstances. That is, the aspiration mechanism promotes cooperation unconditionally, while the social influence mechanism promotes it conditionally. The joint effect of aspiration and social influence is thus effective way to improve the level of cooperation. Transforming individual’s aspiration from negative to positive as well as improving the ratio of strong social influence individuals with positive aspirations are two effective ways that directly enhanced cooperation.
For the aspiration mechanism, no matter whether the social influence of an individual was strong or weak, positive aspiration always promoted cooperation. Even if individuals have strong social influence, they cannot promote cooperation without positive aspiration. However, even if individuals have weak social influence, they can improve their surrounding micro-environment and promote cooperation as long as they have positive aspirations. Therefore, inspiring the aspirations of all individuals to cooperate leads to increased cooperation. For the social influence mechanism, strong social influence did not always promote cooperation. When all the individuals had positive aspirations to cooperate, improving their social influence promoted cooperation, but when they all had negative aspirations to cooperate, improving their social influence actually undermined cooperation. Therefore, changing the negative aspirations of strong social influence individuals to positive ones or strengthening the social influence of individuals with positive aspirations can effectively promote cooperation.
Finally, from a sociological perspective, changes of individual behavior can affect their surrounding micro-environment and thus the macro-social system. We hope our work can deepen the understanding of the joint effects of aspiration and social influence on the evolution of cooperation and provide some insights into exploring the emergence and maintenance of cooperation under heterogeneous micro-environments composed of other internal preferences and external characteristics. In the future, we will also explore the evolution of cooperation at larger population sizes.
Data availability statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author contributions
CL: conceptualization, methodology, data analysis, and writing-original draft. HW: visualization, data analysis and writing-original draft. YD: conceptualization, methodology, writing-original draft and review, and supervision.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Colman AM. Game theory and its applications: In the social and biological sciences. Oxford: Butterworth-Heinemann Press (1995).
2. Dugatkin LA. Cooperation among animals: An evolutionary perspective. New York, NY: Oxford University Press (1997).
3. Axelrod R, Hamilton WD. The evolution of cooperation. Science (1981) 211:1390–6. doi:10.1126/science.7466396
4. Nowak MA, May RM. Evolutionary games and spatial chaos. Nature (1992) 359:826–9. doi:10.1038/359826a0
5. Perc M, Szolnoki A. Social diversity and promotion of cooperation in the spatial prisoner’s dilemma game. Phys Rev E (2008) 77:011904. doi:10.1103/physreve.77.011904
6. Amaral MA, Javarone MA. Heterogeneous update mechanisms in evolutionary games: Mixing innovative and imitative dynamics. Phys Rev E (2018) 97:042305. doi:10.1103/physreve.97.042305
7. Nowak MA. Evolutionary dynamics: Exploring the equations of life. Cambridge, MA: The Belknap Press of Harvard University Press (2006).
8. Brandt H, Hauert C, Sigmund K. Punishment and reputation in spatial public goods games. P Roy Soc B-biol Sci (2003) 270:1099–104. doi:10.1098/rspb.2003.2336
9. Chen MH, Wang L, Sun SW, Wang J, Xia CY. Evolution of cooperation in the spatial public goods game with adaptive reputation assortment. Phys Lett A (2016) 380:40–7. doi:10.1016/j.physleta.2015.09.047
10. Wang Z, Wang L, Yin ZY, Xia CY. Inferring reputation promotes the evolution of cooperation in spatial social dilemma games. PLOS ONE (2012) 7:e40218. doi:10.1371/journal.pone.0040218
11. Dai Y, Lu P. When does inferring reputation probability countervail temptation in cooperative behaviors for the prisoners’ dilemma game? Chaos, Solitons Fractals (2015) 78:238–44. doi:10.1016/j.chaos.2015.07.030
12. Pena J, Volken H, Pestelacci E, Tomassini M. Conformity hinders the evolution of cooperation on scale-free networks. Phys Rev E (2009) 80:016110. doi:10.1103/physreve.80.016110
13. Szolnoki A, Perc M. Leaders should not be conformists in evolutionary social dilemmas. Sci Rep (2016) 6:23633. doi:10.1038/srep23633
14. Liu C, Wang HX, Dai Y. How do reputation and conformity promote cooperation in the prisoner’s dilemma? EPL (2022) 140:41001. doi:10.1209/0295-5075/aca3d0
15. Jimenez R, Lugo H, Cuesta JA, Sanchez A. Emergence and resilience of cooperation in the spatial prisoner’s dilemma via a reward mechanism. J Theor Biol (2008) 250:475–83. doi:10.1016/j.jtbi.2007.10.010
16. Fehr E, Gachter S. Altruistic punishment in humans. Nature (2002) 415:137–40. doi:10.1038/415137a
17. Wu Y, Zhang B, Zhang SH. Probabilistic reward or punishment promotes cooperation in evolutionary games. Chaos, Solitons Fractals (2017) 103:289–93. doi:10.1016/j.chaos.2017.06.015
18. Cao XB, Du WB, Rong ZH. The evolutionary public goods game on scale-free networks with heterogeneous investment. Physica A (2010) 389:1273–80. doi:10.1016/j.physa.2009.11.044
19. Wang Q, Wang HC, Zhang ZX, Li YM, Liu Y, Perc M. Heterogeneous investments promote cooperation in evolutionary public goods games. Physica A (2018) 502:570–5. doi:10.1016/j.physa.2018.02.140
20. Szolnoki A, Szabo G. Cooperation enhanced by inhomogeneous activity of teaching for evolutionary prisoner’s dilemma games. EPL (2007) 77:30004. doi:10.1209/0295-5075/77/30004
21. Szolnoki A, Perc M. Coevolution of teaching activity promotes cooperation. New J Phys (2008) 10:043036. doi:10.1088/1367-2630/10/4/043036
22. Wang J, Chen XJ, Wang L. Effects of migration on the evolutionary game dynamics in finite populations with community structures. Physica A (2010) 389:67–78. doi:10.1016/j.physa.2009.09.003
23. Jiang LL, Wang WX, Lai YC, Wang BH. Role of adaptive migration in promoting cooperation in spatial games. Phys Rev E (2010) 81:036108. doi:10.1103/physreve.81.036108
24. Szolnoki A, Vukov J, Szabo G. Selection of noise level in strategy adoption for spatial social dilemmas. Phys Rev E (2009) 80:056112. doi:10.1103/physreve.80.056112
25. Du WB, Cao XB, Hu MB, Yang HX, Zhou H. Effects of expectation and noise on evolutionary games. Physica A (2009) 388:2215–20. doi:10.1016/j.physa.2009.02.012
26. Posch M. Win–stay, lose–shift strategies for repeated games—Memory length, aspiration levels and noise. J Theor Biol (1999) 198:183–95. doi:10.1006/jtbi.1999.0909
27. Wang WX, Ren J, Chen G, Wang BH. Memory-based snowdrift game on networks. Phys Rev E (2006) 74:056113. doi:10.1103/physreve.74.056113
28. Luo C, Zhang XL, Liu H, Shao R. Cooperation in memory-based prisoner’s dilemma game on interdependent networks. Physica A (2016) 450:560–9. doi:10.1016/j.physa.2016.01.032
29. Chen XJ, Wang L. Promotion of cooperation induced by appropriate payoff aspirations in a small-world networked game. Phys Rev E (2008) 77:017103. doi:10.1103/physreve.77.017103
30. Lin YT, Yang HX, Wu ZX, Wang BH. Promotion of cooperation by aspiration-induced migration. Physica A (2011) 390:77–82. doi:10.1016/j.physa.2010.07.034
31. Liu YK, Chen XJ, Wang L, Li B, Zhang WG, Wang HF. Aspiration-based learning promotes cooperation in spatial prisoner's dilemma games. EPL (2011) 94:60002. doi:10.1209/0295-5075/94/60002
32. Wu ZX, Rong ZH. Boosting cooperation by involving extortion in spatial prisoner’s dilemma games. Phys Rev E (2014) 90:062102. doi:10.1103/physreve.90.062102
33. Yang HX, Wu ZX, Wang BH. Role of aspiration-induced migration in cooperation. Phys Rev E (2010) 81:065101. doi:10.1103/physreve.81.065101
34. Liu XS, He MF, Kang YB, Pan QH. Aspiration promotes cooperation in the prisoner’s dilemma game with the imitation rule. Phys Rev E (2016) 94:012124. doi:10.1103/physreve.94.012124
35. Wang XJ, Gu CL, Zhao JH, Quan J. Evolutionary game dynamics of combining the imitation and aspiration-driven update rules. Phys Rev E (2019) 100:022411. doi:10.1103/physreve.100.022411
36. Arefin MR, Tatsukawa Y, Tanimoto J. Evolution of cooperation under the coexistence of imitation and aspiration dynamics in structured populations. Nonlinearity (2023) 36:2286–309. doi:10.1088/1361-6544/acc500
37. Perc M, Wang Z. Heterogeneous aspirations promote cooperation in the prisoner’s dilemma game. PLOS ONE (2010) 5:e15117. doi:10.1371/journal.pone.0015117
38. Tanimoto J, Nakata M, Hagishima A, Ikegaya N. Spatially correlated heterogeneous aspirations to enhance network reciprocity. Physica A (2012) 391:680–5. doi:10.1016/j.physa.2011.08.039
39. Zou QS, Hu KP. Heterogeneous aspiration resolves social dilemma in structured populations. Chaos, Solitons Fractals (2020) 134:109711. doi:10.1016/j.chaos.2020.109711
40. Portillo IG. Cooperative networks overcoming defectors by social influence. Physica A (2014) 394:198–210. doi:10.1016/j.physa.2013.10.008
41. Wu T, Fu F, Dou PX, Wang L. Social influence promotes cooperation in the public goods game. Physica A (2014) 413:86–93. doi:10.1016/j.physa.2014.06.040
42. Allen JM, Skeldon AC, Hoyle RB. Social influence preserves cooperative strategies in the conditional cooperator public goods game on a multiplex network. Phys Rev E (2018) 98:062305. doi:10.1103/physreve.98.062305
43. Li WJ, Jiang LL, Gu CG, Yang HJ. Influentials promote cooperation in spatial snowdrift games. J Stat Mech Theor Exp. (2018) 2018:063406. doi:10.1088/1742-5468/aac91b
44. Zong HS, Jia GZ, Cheng Y. Exploring social influence on evolutionary prisoner’s dilemma games in networks. Mod Phys Lett B (2015) 29:1550184. doi:10.1142/s0217984915501845
46. Tanimoto J, Sagara H. Relationship between dilemma occurrence and the existence of a weakly dominant strategy in a two-player symmetric game. BioSystems (2007) 90:105–14. doi:10.1016/j.biosystems.2006.07.005
47. Wang Z, Kokubo S, Jusup M, Tanimoto J. Universal scaling for the dilemma strength in evolutionary games. Phys Life Rev (2015) 14:1–30. doi:10.1016/j.plrev.2015.04.033
48. Ito H, Tanimoto J. Scaling the phase-planes of social dilemma strengths shows game-class changes in the five rules governing the evolution of cooperation. R Soc Open Sci (2018) 5:181085. doi:10.1098/rsos.181085
49. Liu YD, Wang HX, Ding Y, Yang X, Dai Y. Can weak diversity help in propagating cooperation? Invasion of cooperators at the conformity-conflict boundary. Chaos, Solitons Fractals (2022) 156:111787. doi:10.1016/j.chaos.2021.111787
50. Szabo G, Toke C. Evolutionary prisoner's dilemma game on a square lattice. Phys Rev E (1998) 58:69–73. doi:10.1103/physreve.58.69
Keywords: prisoner’s dilemma, aspiration, social influence, cooperation, evolutionary game theory
Citation: Liu C, Wang H and Dai Y (2023) How do the joint effects of aspiration and social influence promote cooperation in the prisoner’s dilemma game?. Front. Phys. 11:1222489. doi: 10.3389/fphy.2023.1222489
Received: 14 May 2023; Accepted: 10 July 2023;
Published: 19 July 2023.
Edited by:
Chengyi Xia, Tiangong University, ChinaReviewed by:
Zhixi Wu, Lanzhou University, ChinaJun Tanimoto, Kyushu University, Japan
Xiaojie Chen, University of Electronic Science and Technology of China, China
Copyright © 2023 Liu, Wang and Dai. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Yu Dai, eXUuZGFpQHRqdS5lZHUuY24=
 Chao Liu1
Chao Liu1 Hexin Wang
Hexin Wang Yu Dai
Yu Dai