Abundant optical solutions for the Sasa-Satsuma equation with M-truncated derivative

Here, we look at the Sasa-Satsuma equation with M-truncated derivative (SSE-MTD). The analytical solutions in the form of trigonometric, hyperbolic, elliptic, and rational functions are constructed using the Jacobi elliptic function and generalizing Riccati equation mapping methods. Because the Sasa–Satsuma equation is applied to explain the propagation of femtosecond pulses in optical fibers, the acquired solutions can be employed to explain a wide range of important physical phenomena. Moreover, we apply the MATLAB tool to generate a series of graphs to address the effect of the M-truncated derivative on the exact solution of the SSE-MTD.

1 Introduction

Many authors have centered their attention on fractional nonlinear differential equations (FNLDEs) in the noble age of technology and science to examine complex mathematical models that are used in research area and real life, such as neuroscience, robotics, fluid dynamics, quantum mechanics, plasma physics, optical fibers, and so on. A lot studies have been published about some aspects of fractional differential equations, such as finding exact and numerical solutions, the existence and uniqueness of solutions, and the stability of solutions [1–7]. Therefore, it is essential to discover the exact solutions to these equations in order to understand the physical phenomenon and overcome the resulting obstacles. Recently, acquiring soliton solutions to important equations has emerged as a major field of study. Numerous researchers defended numerous novel methods to evaluate soliton solutions including (G′/G, 1/G)-expansion method [8] (G′/G)-expansion [9, 10], generalized (G′/G)-expansion [11], exp-function method [12], Jacobi elliptic function expansion [13], sine-cosine procedure [14], auxiliary equation scheme [15], first-integral method [16], sine-Gordon expansion technique [17], generalized Kudryashov approach [18], exp(−ϕ(ς))-expansion method [19], homotopy perturbation method with Aboodh transform [20], He–Laplace method, He’s variational iteration method [21, 22], and others.

In contrast, a new differentiation operator has grown up, that includes the concepts of fractional differentiation and fractal derivative. Therefore, various kinds of fractional derivatives were proposed by several mathematicians. The most well-known ones are the ones proposed by Grunwald-Letnikov, He’s fractional derivative, Atangana-Baleanu’s derivative, Riemann-Liouville, Marchaud, Riesz, Caputo, Hadamard, Kober, and Erdelyi [23–26]. The bulk of fractional derivative types do not follow classic derivative equations like the chain rule, quotient rule, and product rule. Sousa et al. [27] have developed a new derivative known as the M-truncated derivative (MTD), which is a natural extension of the classical derivative. The MTD for $u:[0,\infty )\to \mathbb{R}$ of order δ ∈ (0, 1] is indicated as

${\mathcal{M}}_{j,t}^{\delta ,\beta }u\left(t\right)=\underset{h\to 0}{\lim }\frac{u\left(t{\mathcal{E}}_{j,\beta }\left(h{t}^{-\delta }\right)\right)-u\left(t\right)}{h},$

where ${\mathcal{E}}_{j,\beta }(t)$, for $t\in \mathbb{C}$ and β > 0, is the truncated Mittag-Leffler function and is defined as:

${\mathcal{E}}_{j,\beta }\left(t\right)=\sum _{k=0}^j\frac{t^k}{\mathrm{\Gamma }\left(\beta k+1\right)}.$

The MTD has the following characteristics for any real integers a and b [27]:

(1) ${\mathcal{M}}_{j,t}^{\delta ,\beta }(au+bv)=a{\mathcal{M}}_{j,t}^{\delta ,\beta }(u)+b{\mathcal{M}}_{j,t}^{\delta ,\beta }(v),$

(2) ${\mathcal{M}}_{j,t}^{\delta ,\beta }(u◦v)(t)=u^{\prime }(v(t)){\mathcal{M}}_{j,t}^{\delta ,\beta }v(t)$,

(3) ${\mathcal{M}}_{j,t}^{\delta ,\beta }(uv)=u{\mathcal{M}}_{j,t}^{\delta ,\beta }v+v{\mathcal{M}}_{j,t}^{\delta ,\beta }u,$

(4) ${\mathcal{M}}_{j,t}^{\delta ,\beta }(u)(t)=\frac{t^{1-\delta }}{\mathrm{\Gamma }(\beta +1)}\frac{du}{dt},$

(5) ${\mathcal{M}}_{j,t}^{\delta ,\beta }(t^{\nu })=\frac{\nu }{\mathrm{\Gamma }(\beta +1)}{t}^{\nu -\delta }$.

There are many authors have considered some nonlinear partial differential equations with M-truncated derivative such as [28–31] and the references therein. In this article, we examine the Sasa-Satsuma equation with M-truncated derivative (SSE-MTD):

$i{\mathcal{M}}_{j,t}^{\delta ,\beta }\mathcal{W}+\frac{1}{2}{\mathcal{W}}_{xx}+i\left[{\alpha }_1{\mathcal{W}}_{\mathit{xxx}}+{\alpha }_2\mathcal{W}{\left({\left|\mathcal{W}\right|}^2\right)}_x+{\alpha }_3{\left|\mathcal{W}\right|}^2{\mathcal{W}}_x\right]+{\alpha }_4{\left|\mathcal{W}\right|}^2\mathcal{W}=0,(1)$

where $\mathcal{W}=\mathcal{W}(x,t)$ is the optical soliton profile,$i=\sqrt{-1}.{\alpha }_k$, for k = 1, 2, 3, 4, are real constants. ${\mathcal{W}}_t$ defines the temporal evolution of optical soliton molecules, ${\mathcal{W}}_{xx}$ is the group velocity dispersion. ${\mathcal{W}}_{\mathit{xxx}}$ represents the third-order dispersion, while $\mathcal{W}{({\left|\mathcal{W}\right|}^2)}_x$ provides the stimulated Raman scattering, ${\left|\mathcal{W}\right|}^2{\mathcal{W}}_x$ is the self-steepening and ${\left|\mathcal{W}\right|}^2\mathcal{W}$ is Kerr-law fiber nonlinearity.

If we set δ = 1 and β = 0, then we have the Sasa-Satsuma (SS) equation (32, 33):

$i{\mathcal{W}}_t+\frac{1}{2}{\mathcal{W}}_{xx}+i\left[{\alpha }_1{\mathcal{W}}_{\mathit{xxx}}+{\alpha }_2{\left|\mathcal{W}\right|}^2{\mathcal{W}}_x+{\alpha }_3\mathcal{W}{\left({\left|\mathcal{W}\right|}^2\right)}_x\right]+{\alpha }_4{\left|\mathcal{W}\right|}^2\mathcal{W}=0.(2)$

The SS Eq. 2, which was found while studying the integrability of Schrödinger equation, reduced to nonlinear Schrödinger equation when α₁ = α₂ = α₃ = 0 as follows:

$i{\mathcal{W}}_t+\frac{1}{2}{\mathcal{W}}_{xx}+{\alpha }_4{\left|\mathcal{W}\right|}^2\mathcal{W}=0.(3)$

In 1991, Sasa and Satsuma [34] created Eq. 2. This equation has additional components that explain third-order dispersion, self-steepening, and self-frequency shift, which are prevalent in many areas of physics, such as ultrashort pulse propagation in optical fibers [35, 36]. Due to the importance of SS Eq. 2, many authors have obtained its exact solutions by using various methods such as new auxiliary equation method [37], extended trial equation and generalized Kudryashov methods [38], inverse scattering transform [39], improved F-expansion methods and improved auxiliary [40], Riemann problem method [41], unified transform method [42], Bäcklund transformation [43], Darboux transformation [44].

Our main objective of this work is to find the exact solutions of the SSE-MTD (1). The solutions in the form of hyperbolic, trigonometric, elliptic, and rational functions are constructed by utilizing the Jacobi elliptic function method (JEF-method) and generalizing Riccati equation mapping method (GREM-method). Because the Sasa–Satsuma equation is applied to clarify the propagation of femtosecond pulses in optical fibers, the solutions obtained can be employed to study a wide range of important physical phenomena. Furthermore, we utilize the MATLAB tool to generate a series of graphs to examine the effect of the M-truncated derivative on the exact solution of the SSE-MTD (1).

The following is how the paper is organized: In the next section, we describe the methods employed in this paper. The wave equation for the SSE-MTD (1) is developed in Section 3. In Section 4, we employ the JEF-method and the GREM-method to get the precise solutions of the SSE-MTD (1). In Section 5, we study the effect of the MTD on the solution of Eq. 1. Finally, the findings of the article are presented.

2 Description of the methods

In this section, we describe the methods employed in this paper.

2.1 GREM-method

It is useful to outline the essential steps of GREM-method mentioned in [45] as follows:

1. We begin by looking at a general kind of PDEs with MTD as follows

$\mathcal{P}\left(\mathcal{W},{\mathcal{M}}_{j,t}^{\delta ,\beta }\mathcal{W},{\mathcal{W}}_x,{\mathcal{W}}_{xx},\dots \right)=0.(4)$

2. We use Eq. 4 to obtain the traveling wave solution

$\mathcal{W}\left(t,x\right)=\mathcal{X}\left({\eta }_{\delta }\right),{\eta }_{\delta }={\eta }_{1}x+\frac{\mathrm{\Gamma }\left(\beta +1\right){\eta }_2}{\delta }{t}^{\delta }.(5)$

3. Using the next changes

$\begin{array}{ll}\hfill {\mathcal{M}}_{j,t}^{\delta ,\beta }\mathcal{W}& ={\eta }_2{\mathcal{X}}^{\prime },\hfill \\ \hfill u_x& ={\eta }_1{\mathcal{X}}^{\prime },\hfill \\ \hfill & ⋮⋮⋮\hfill \\ \hfill u_{x^n}& ={\eta }_1^n{\mathcal{X}}^{\left(n\right)}.\hfill \end{array}(6)$

4. After then, substituting (6) into (4) to get ordinary differential equation (ODE)

$\mathcal{P}\left({\eta }_2{\mathcal{X}}^{\prime },{\eta }_1{\mathcal{X}}^{\prime },{\eta }_1^n{\mathcal{X}}^{\left(n\right)}\right)=0.(7)$

5. Putting the following Riccati-Bernoulli equation

${\mathcal{X}}^{\prime }=s{\mathcal{X}}^2+r\mathcal{X}+p,(8)$

where s, r, p are constants, into Eq. 8. Then we balance each coefficient of ${\mathcal{X}}^k$ to zero to get a system of ODE. We solve this system to attain the value of s, r and p. It is straightforward to obtain the non-traveling wave solutions to Eq. 4 by solving Eq. 8 and utilizing Eq. 5.

2.2 JEF-method

While, we summarize here the main steps of the JEF-method described by Fan et al. [46] as follows.

1. We repeat the first four steps from the previous subsection in order to obtain Eq. 7.

2. Assuming the solution of Eq. 7 in this type

$\mathcal{X}\left({\eta }_{\delta }\right)=\sum _{k=0}^N{a}_k{\left[\mathcal{F}\left({\eta }_{\delta }\right)\right]}^k,(9)$

where N is a positive integer that will be determined and $\mathcal{F}({\eta }_{\delta })=\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)$ or $\mathcal{F}({\eta }_{\delta })=cn({\mathcal{K}\eta }_{\delta },m)$ or $\mathcal{F}({\eta }_{\delta })=dn({\mathcal{K}\eta }_{\delta },m)$ for 0 < m < 1. The Jacobi elliptic functions $\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)$, $cn({\mathcal{K}\eta }_{\delta },m),dn({\mathcal{K}\eta }_{\delta },m)$ are periodic and have features of triangular functions as follows: ${\mathrm{sn}}^2({\mathcal{K}\eta }_{\delta },m)+c{n}^2({\mathcal{K}\eta }_{\delta },m)=1$, $d{n}^2({\mathcal{K}\eta }_{\delta },m)=1-m^2{\mathrm{sn}}^2({\mathcal{K}\eta }_{\delta },m)$, ${[\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)]}^{\prime }=cn({\mathcal{K}\eta }_{\delta },m)dn({\mathcal{K}\eta }_{\delta },m)$,${[cn({\mathcal{K}\eta }_{\delta },m)]}^{\prime }=-\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)dn({\mathcal{K}\eta }_{\delta },m)$, ${[dn({\mathcal{K}\eta }_{\delta },m)]}^{\prime }=-m^2\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)cn({\mathcal{K}\eta }_{\delta },m)$.

If m → 1, then $\mathrm{sn}({\mathcal{K}\eta }_{\delta },1)\to \tanh ({\mathcal{K}\eta }_{\delta })$, $cn({\mathcal{K}\eta }_{\delta },1)\to$ sech$({\mathcal{K}\eta }_{\delta })$ and $dn({\mathcal{K}\eta }_{\delta },1)\to$sech$({\mathcal{K}\eta }_{\delta })$.

3. Usually, to determine the parameter N, we balance the highest order linear terms in the resulting equation with the highest order nonlinear terms. To determine the order, we follow these steps: Firstly, we define the degree of $\mathcal{F}$ as $D[\mathcal{F}]=N$. Secondly, we calculated the highest order nonlinear terms and the highest order nonlinear terms as

$D\left[\frac{d^n\mathcal{F}}{d{\eta }^n}\right]=N+n,$

and

$D\left[{\mathcal{F}}^p{\left(\frac{d^n\mathcal{F}}{d{\eta }^n}\right)}^s\right]=pN+s\left(N+n\right).$

4. After we determine N, we substitute (9) into the ODE (7) in order to attain an equation in powers of $\mathcal{F}$.

5. Equating each coefficients of powers of $\mathcal{F}$ in the resulting equation to zero. This will provide a set of equations containing the a_k (k = 0, 1, …N) and $\mathcal{K}$. We solve these equations to attain the values of a_k (k = 0, 1, …N) and $\mathcal{K}$ and substitute with these value into Eq. 9.

3 Traveling wave Eq. For SSE-MTD

To derive the wave equation for SSE-MTD (1), we use

$\mathcal{W}\left(x,t\right)=\mathcal{X}\left({\eta }_{\delta }\right)e^{i{\mu }_{\delta }},{\mu }_{\delta }={\mu }_{1}x+\frac{{\mu }_2\mathrm{\Gamma }\left(\beta +1\right)}{\delta }{t}^{\delta }\text{\hspace{0.17em}\hspace{0.17em}and\hspace{0.17em}\hspace{0.17em}}{\eta }_{\delta }={\eta }_{1}x+\frac{{\eta }_2\mathrm{\Gamma }\left(\beta +1\right)}{\delta }{t}^{\delta },(10)$

where$\mathcal{X}$ is a real function, μ₁, μ₂, η₁, and η₂ are non-zero constants. We note that

$\begin{array}{ll}\hfill {\mathcal{M}}_{j,t}^{\delta ,\beta }\mathcal{W}& =\left[{\eta }_2{\mathcal{X}}^{\prime }+i{\mu }_2\mathcal{X}\right]e^{i{\mu }_{\delta }},\hfill \\ \hfill {\mathcal{W}}_x& =\left({\eta }_1{\mathcal{X}}^{\prime }+i{\mu }_1\mathcal{X}\right)e^{i{\mu }_{\delta }}\text{,\hspace{0.17em}}{\left({\left|\mathcal{W}\right|}^2\right)}_x={\eta }_1{\left({\mathcal{X}}^2\right)}^{\prime }{e}^{i{\mu }_{\delta }},\hfill \\ \hfill {\mathcal{W}}_{xx}& =\left({\eta }_1^2{\mathcal{X}}^{\prime \prime }+2i{\mu }_1{\eta }_1{\mathcal{X}}^{\prime }-{\mu }_1^2\mathcal{X}\right)e^{i{\mu }_{\delta }},\hfill \\ \hfill {\mathcal{W}}_{\mathit{xxx}}& =\left({\eta }_1^3{\mathcal{X}}^{\prime \prime \prime }+3i{\mu }_1{\eta }_1^2{\mathcal{X}}^{\prime \prime }-3{\eta }_1{\mu }_1^2{\mathcal{X}}^{\prime }-i{\mu }_1^3\mathcal{X}\right)e^{i{\mu }_{\delta }}.\hfill \end{array}(11)$

Inserting Eq. 11 into Eq. 1, we have for real part

$\left(\frac{1}{2}{\eta }_1^2-3{\alpha }_1{\mu }_1{\eta }_1^2\right){\mathcal{X}}^{\prime \prime }+\left(-{\mu }_2-\frac{1}{2}{\mu }_1^2+{\alpha }_1{\mu }_1^3\right)\mathcal{X}+\left[{\alpha }_4-{\alpha }_1{\mu }_1\right]{\mathcal{X}}^3=0,(12)$

and for imaginary part

${\alpha }_1{\eta }_1^3{\mathcal{X}}^{\prime \prime \prime }+\left({\eta }_2+{\mu }_1{\eta }_1-3{\alpha }_1{\eta }_1{\mu }_1^2\right){\mathcal{X}}^{\prime }+{\eta }_1\left({\alpha }_2+2{\alpha }_3\right){\mathcal{X}}^2{\mathcal{X}}^{\prime }=0.(13)$

Integrating (13) once, we get

$[{\alpha }_1{\eta }_1^3{\mathcal{X}}^{\prime \prime }+\left({\eta }_2+{\mu }_1{\eta }_1-3{\alpha }_1{\eta }_1{\mu }_1^2\right)\mathcal{X}+\frac{1}{3}{\eta }_1\left({\alpha }_2+2{\alpha }_3\right){\mathcal{X}}^3=C,(14)$

where C is the integral constant. If we compare the coefficients of Eqs (12) and (14), we have

${\eta }_1=\frac{1}{2{\alpha }_1}-3{\mu }_1,$

${\eta }_2=-2{\gamma }_1{\mu }_1{\eta }_1+3{\alpha }_1{\eta }_1{\mu }_1^2-{\mu }_2-{\gamma }_1{\mu }_1^2+{\alpha }_1{\mu }_1^3,$

${\alpha }_4={\alpha }_1{\mu }_1+\frac{1}{3}{\eta }_1\left({\alpha }_2+2{\alpha }_3\right),$

and

$C=0.$

Now, we can rewrite Eq. 12 as

${\mathcal{X}}^{\prime \prime }-{\ell }_1{\mathcal{X}}^3-{\ell }_2\mathcal{X}=0,(15)$

where

${\ell }_1=\frac{2{\alpha }_1{\mu }_1-2{\alpha }_4}{\left({\eta }_1^2-6{\alpha }_1{\mu }_1{\eta }_1^2\right)}\text{,\hspace{0.17em}and\hspace{0.17em}}{\ell }_2=\frac{\left(2{\mu }_2+{\mu }_1^2-2{\alpha }_1{\mu }_1^3\right)}{\left({\eta }_1^2-6{\alpha }_1{\mu }_1{\eta }_1^2\right)}.(16)$

Balancing ${\mathcal{X}}^{\prime \prime }$ with ${\mathcal{X}}^3$ in Eq. 15 to calculate the parameter N as

$N+2=3N\Rightarrow N=1.$

4 Exact solutions of SSE-MTD

Two various methods such as GREM-method and JEF-method are used to attain the solutions to Eq. 15. The solutions to the SSE-MTD (1) are then determined.

4.1 REM-method

Utilizing Eq. 8, we obtain

${\mathcal{X}}^{\prime \prime \prime }=2s^2{\mathcal{X}}^3+3sr{\mathcal{X}}^2+\left(2sp+r^2\right)\mathcal{X}+rp.(17)$

Substituting (17) into (15), we have

$\left(2s^2-{\ell }_1\right){\mathcal{X}}^3+3sr{\mathcal{X}}^2+\left(2sp+r^2-{\ell }_2\right)\mathcal{X}+rp=0.$

We put each coefficient of ${\mathcal{X}}^i$ equal zero in order to get

$2s^2-{\ell }_1=0,3sr=0,2sp+r^2-{\ell }_2=0,\text{\hspace{0.17em}and\hspace{0.17em}}rp=0.$

Solving these equations, we have

$s=\pm \sqrt{\frac{{\ell }_1}{2}},(18)$

$r=0,(19)$

and

$p=\frac{{\ell }_2}{2s}=\pm \frac{{\ell }_2}{\sqrt{2{\ell }_1}},(20)$

where ℓ₁ and ℓ₂ are stated in Eq. 16. There are different sets for the solution of Eq. 8 relying on p and s as follows:

Set I: When ps > 0, thus the solutions of Eq. 8 are:

${\mathcal{X}}_1\left({\eta }_{\delta }\right)=\sqrt{\frac{p}{s}}\tan \left(\sqrt{ps}{\eta }_{\delta }\right),$

${\mathcal{X}}_2\left({\eta }_{\delta }\right)=-\sqrt{\frac{p}{s}}\cot \left(\sqrt{ps}{\eta }_{\delta }\right),$

${\mathcal{X}}_3\left({\eta }_{\delta }\right)=\sqrt{\frac{p}{s}}\left(\tan \left(\sqrt{4ps}{\eta }_{\delta }\right)\pm \sec \left(\sqrt{4ps}{\eta }_{\delta }\right)\right),$

${\mathcal{X}}_4\left({\eta }_{\delta }\right)=-\sqrt{\frac{p}{s}}\left(\cot \left(\sqrt{4ps}{\eta }_{\delta }\right)\pm \csc \left(\sqrt{4ps}{\eta }_{\delta }\right)\right),$

${\mathcal{X}}_5\left({\eta }_{\delta }\right)=\frac{1}{2}\sqrt{\frac{p}{s}}\left(\tan \left(\frac{1}{2}\sqrt{ps}{\eta }_{\delta }\right)-\cot \left(\frac{1}{2}\sqrt{ps}{\eta }_{\delta }\right)\right),$

Then, SSE-MTD (1) has the trigonometric functions solution:

${\mathcal{W}}_1\left(x,t\right)=\sqrt{\frac{p}{s}}\tan \left(\sqrt{ps}{\eta }_{\delta }\right)e^{i{\mu }_{\delta }},(21)$

${\mathcal{W}}_2\left(x,t\right)=-\sqrt{\frac{p}{s}}\cot \left(\sqrt{ps}{\eta }_{\delta }\right)e^{i{\mu }_{\delta }},(22)$

${\mathcal{W}}_3\left(x,t\right)=\sqrt{\frac{p}{s}}\left(\tan \left(\sqrt{4ps}{\eta }_{\delta }\right)\pm \sec \left(\sqrt{4ps}{\eta }_{\delta }\right)\right)e^{i{\mu }_{\delta }},(23)$

${\mathcal{W}}_4\left(x,t\right)=-\sqrt{\frac{p}{s}}\left(\cot \left(\sqrt{4ps}{\eta }_{\delta }\right)\pm \csc \left(\sqrt{4ps}{\eta }_{\delta }\right)\right)e^{\left(\gamma \mathcal{W}\left(t\right)-\frac{1}{2}{\gamma }^{2}t\right)},(24)$

${\mathcal{W}}_5\left(x,t\right)=\frac{1}{2}\sqrt{\frac{p}{s}}\left(\tan \left(\frac{1}{2}\sqrt{ps}{\eta }_{\delta }\right)-\cot \left(\frac{1}{2}\sqrt{ps}{\eta }_{\delta }\right)\right)e^{i{\mu }_{\delta }},(25)$

where ${\eta }_{\delta }={\eta }_{1}x+\frac{{\eta }_2\mathrm{\Gamma }(\beta +1)}{\delta }{t}^{\delta }$.

Family II: When ps < 0, thus the solutions of Eq. 8 are:

${\mathcal{X}}_6\left({\eta }_{\delta }\right)=-\sqrt{\frac{-p}{s}}\tanh \left(\sqrt{-ps}{\eta }_{\delta }\right),$

${\mathcal{X}}_7\left({\eta }_{\delta }\right)=-\sqrt{\frac{-p}{s}}\coth \left(\sqrt{-ps}{\eta }_{\delta }\right),$

${\mathcal{X}}_8\left({\eta }_{\delta }\right)=-\sqrt{\frac{-p}{s}}\left(\tanh \left(\sqrt{-4ps}{\eta }_{\delta }\right)\pm i\text{sech}\left(\sqrt{-4ps}{\eta }_{\delta }\right)\right),$

${\mathcal{X}}_9\left({\eta }_{\delta }\right)=-\sqrt{\frac{-p}{s}}\left(\coth \left(\sqrt{-4ps}{\eta }_{\delta }\right)\pm \text{csch}\left(\sqrt{-4ps}{\eta }_{\delta }\right)\right),$

${\mathcal{X}}_{10}\left({\eta }_{\delta }\right)=\frac{-1}{2}\sqrt{\frac{-p}{s}}\left(\tanh \left(\frac{1}{2}\sqrt{-ps}{\eta }_{\delta }\right)+\coth \left(\frac{1}{2}\sqrt{-ps}{\eta }_{\delta }\right)\right).$

Then, SSE-MTD (1) has the hyperbolic functions solution:

${\mathcal{W}}_6\left(x,t\right)=-\sqrt{\frac{-p}{s}}\tanh \left(\sqrt{-ps}{\eta }_{\delta }\right)e^{i{\mu }_{\delta }},(26)$

${\mathcal{W}}_7\left(x,t\right)=-\sqrt{\frac{-p}{s}}\coth \left(\sqrt{-ps}{\eta }_{\delta }\right)e^{i{\mu }_{\delta }},(27)$

${\mathcal{W}}_8\left(x,t\right)=-\sqrt{\frac{-p}{s}}\left(\tanh \left(\sqrt{-4ps}{\eta }_{\delta }\right)\pm i\text{sech}\left(\sqrt{-4ps}{\eta }_{\delta }\right)\right)e^{i{\mu }_{\delta }},(28)$

${\mathcal{W}}_9\left(x,t\right)=-\sqrt{\frac{-p}{s}}\left(\coth \left(\sqrt{-4ps}{\eta }_{\delta }\right)\pm \text{csch}\left(\sqrt{-4ps}{\eta }_{\delta }\right)\right)e^{i{\mu }_{\delta }},(29)$

${\mathcal{W}}_{10}\left(x,t\right)=\frac{-1}{2}\sqrt{\frac{-p}{s}}\left(\tanh \left(\frac{1}{2}\sqrt{-ps}{\eta }_{\delta }\right)+\coth \left(\frac{1}{2}\sqrt{-ps}{\eta }_{\delta }\right)\right)e^{i{\mu }_{\delta }},(30)$

where ${\eta }_{\delta }={\eta }_{1}x+\frac{{\eta }_2\mathrm{\Gamma }(\beta +1)}{\delta }{t}^{\delta }$.

Family III: When p = 0, s ≠ 0, then the solution of Eq. 8 is

${\mathcal{X}}_{11}\left({\eta }_{\delta }\right)=\frac{-1}{s{\eta }_{\delta }}.$

Then, we get the rational function solution of SSE-MTD (1) as

${\mathcal{W}}_{11}\left(x,t\right)=\left(\frac{-1}{s\left({\eta }_{1}x+\frac{{\eta }_2\mathrm{\Gamma }\left(\beta +1\right)}{\delta }{t}^{\delta }\right)}\right)e^{i{\mu }_{\delta }}.(31)$

Remark 1. If we Put β = 0 and δ = 0 in Eqs. (21) and (26), then we get the solutions (13) and (14) that stated in [40].

4.2 JEF-method

We assume the solutions of Eq. 15, with N = 1, are

$\mathcal{X}\left({\eta }_{\delta }\right)=a+b\mathcal{F}\left({\eta }_{\delta }\right).(32)$

First, let $\mathcal{F}({\eta }_{\delta })=\mathit{sn}({\mathcal{K}\eta }_{\delta },m)$. Differentiate Eq. 32 two times, we have

${\mathcal{X}}^{\prime \prime }\left({\eta }_{\delta }\right)=-\left(m^2+1\right)b{\mathcal{K}}^2\mathit{sn}\left({\mathcal{K}\eta }_{\delta },m\right)+2m^{2}b{\mathcal{K}}^2{\mathit{sn}}^3\left({\mathcal{K}\eta }_{\delta },m\right).(33)$

Setting Eqs 32, 33 into Eq. 15, we obtain

$\left(2m^{2}b{\mathcal{K}}^2-{\ell }_1{b}^3\right){\mathit{sn}}^3\left({\mathcal{K}\eta }_{\delta },m\right)-3{\ell }_{1}a{b}^2{\mathit{sn}}^2\left({\mathcal{K}\eta }_{\delta },m\right)$

$-\left[\left(m^2+1\right)b{\mathcal{K}}^2+3{\ell }_1{a}^{2}b+{\ell }_{2}b\right]\mathit{sn}\left({\mathcal{K}\eta }_{\delta },m\right)-\left({\ell }_1{a}^3+a{\ell }_2\right)=0.$

Plugging each coefficient of ${[\mathrm{sn}({\mathcal{K}\eta }_{\delta },m)]}^n$ equal zero, we attain

${\ell }_1{a}^3+a{\ell }_2=0,$

$\left(m^2+1\right)b{\mathcal{K}}^2+3{\ell }_1{a}^{2}b+{\ell }_{2}b=0,$

$3{\ell }_{1}a{b}^2{\mathit{sn}}^2=0,$

and

$2m^{2}b{\mathcal{K}}^2-{\ell }_1{b}^3=0.$

We obtain when we solve these equations

$a=0,b=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(m^2+1\right){\ell }_1}}{\mathcal{K}}^2=\frac{-{\ell }_2}{\left(m^2+1\right)}.$

Consequently, the solution of Eq. 15 is

$\mathcal{X}\left({\eta }_{\delta }\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(m^2+1\right){\ell }_1}}\mathit{sn}\left(\sqrt{\frac{-{\ell }_2}{\left(m^2+1\right)}}{\eta }_{\delta },m\right).$

As a result, the solution of the SSE-MTD (1), for ℓ₂ < 0 and ℓ₁ > 0, is

$\mathcal{W}\left(x,t\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(m^2+1\right){\ell }_1}}\mathit{sn}\left(\sqrt{\frac{-{\ell }_2}{\left(m^2+1\right)}}{\eta }_{\delta },m\right)e^{i{\mu }_{\delta }},(34)$

where ${\eta }_{\delta }={\eta }_{1}x+\frac{{\eta }_2\mathrm{\Gamma }(\beta +1)}{\delta }{t}^{\delta }$. When m → 1, the solution (34) tends to:

$\mathcal{W}\left(x,t\right)=\pm \sqrt{\frac{-{\ell }_2}{{\ell }_1}}\tanh \left(\sqrt{\frac{-{\ell }_2}{2}}\left({\eta }_{1}x+{\eta }_{2}t\right),m\right)e^{i{\mu }_{\delta }}.(35)$

Similarly, we can replace $\mathcal{F}({\eta }_{\delta })$ in (32) with $cn({\mathcal{K}\eta }_{\delta },m)$ or$dn({\mathcal{K}\eta }_{\delta },m)$ to derive the solutions of Eq. 15 as follows:

$\mathcal{X}\left({\eta }_{\delta }\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(2m^2-1\right){\ell }_1}}cn\left(\sqrt{\frac{{\ell }_2}{\left(2m^2-1\right)}}{\eta }_{\delta },m\right),$

and

$\mathcal{X}\left({\eta }_{\delta }\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(2-m^2\right){\ell }_1}}dn\left(\sqrt{\frac{{\ell }_2}{\left(2-m^2\right)}}{\eta }_{\delta },m\right).$

Consequently, the solutions of the SSE-MTD (1) are as follows:

$\mathcal{W}\left(x,t\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(2m^2-1\right){\ell }_1}}cn\left(\sqrt{\frac{-{\ell }_2}{\left(2m^2-1\right)}}{\eta }_{\delta },m\right)e^{i{\mu }_{\delta }},(36)$

for $\frac{{\ell }_2}{(2m^2-1)}>0$, ℓ₁ < 0, and

$\mathcal{W}\left(x,t\right)=\pm \sqrt{\frac{-2m^2{\ell }_2}{\left(2-m^2\right){\ell }_1}}dn\left(\sqrt{\frac{{\ell }_2}{\left(2-m^2\right)}}{\eta }_{\delta },m\right)e^{i{\mu }_{\delta }},(37)$

for ℓ₂ > 0, ℓ₁ < 0, respectively. If m → 1, then the solutions (36) and (37) turn to:

$\mathcal{W}\left(x,t\right)=\pm \sqrt{\frac{-2{\ell }_2}{{\ell }_1}}\text{sech}\left(\sqrt{{\ell }_2}\left({\eta }_{1}x+{\eta }_{4}t\right)\right)e^{i{\mu }_{\delta }},(38)$

for ℓ₂ > 0, ℓ₁ < 0.

Remark 2. If we Put β = 0 and δ = 0 in Eqs. (34) and (36), then we get the solutions (48) and (49) that stated in [40].

5 Discussion and effects of M-truncated derivative

Discussion: For the Sasa-Satsuma equation with a M-truncated derivative, we found the optical solutions in this paper. Two effective methods, the REM-method and JEF-method, were used to arrive at these results. The REM-method has provided optical singular periodic (21) and (22), singular optical solution (27), and dark optical solution (26). While JEF-method has provided elliptic solutions. Dark optical solution can interpret solitary waves (SW) with less intensity than the background [47]. SW with discontinuous derivatives can be illustrated using singular solitons [48, 49]. These kinds of SW are effective because of their efficacy and applicability in optical long-distance communications. Optical fibers can be thought of as thin, long strands of pure-ultra glass that allow electromagnetic radiations to travel unimpeded from one location to another.

Effects of M-truncated derivative: Now, we examine the influence of MTD on the exact solution of the SSE-MTD (1). Several graphical representations depict the behavior of some obtained solutions, including (26) (34) and (38). Let us fix the parameters ${\alpha }_1=\frac{1}{2},{\mu }_1={\mu }_2={\alpha }_4={\eta }_1=1,{\alpha }_2=2,{\eta }_2=-2,x\in [\mathrm{0,4}]$ and t ∈ [0, 2] to simulate these graphs.

Now, we deduce from Figures 1, 2, 3 that when the derivative order δ of M-truncated derivative increases, the surface moves into the right.

FIGURE 1

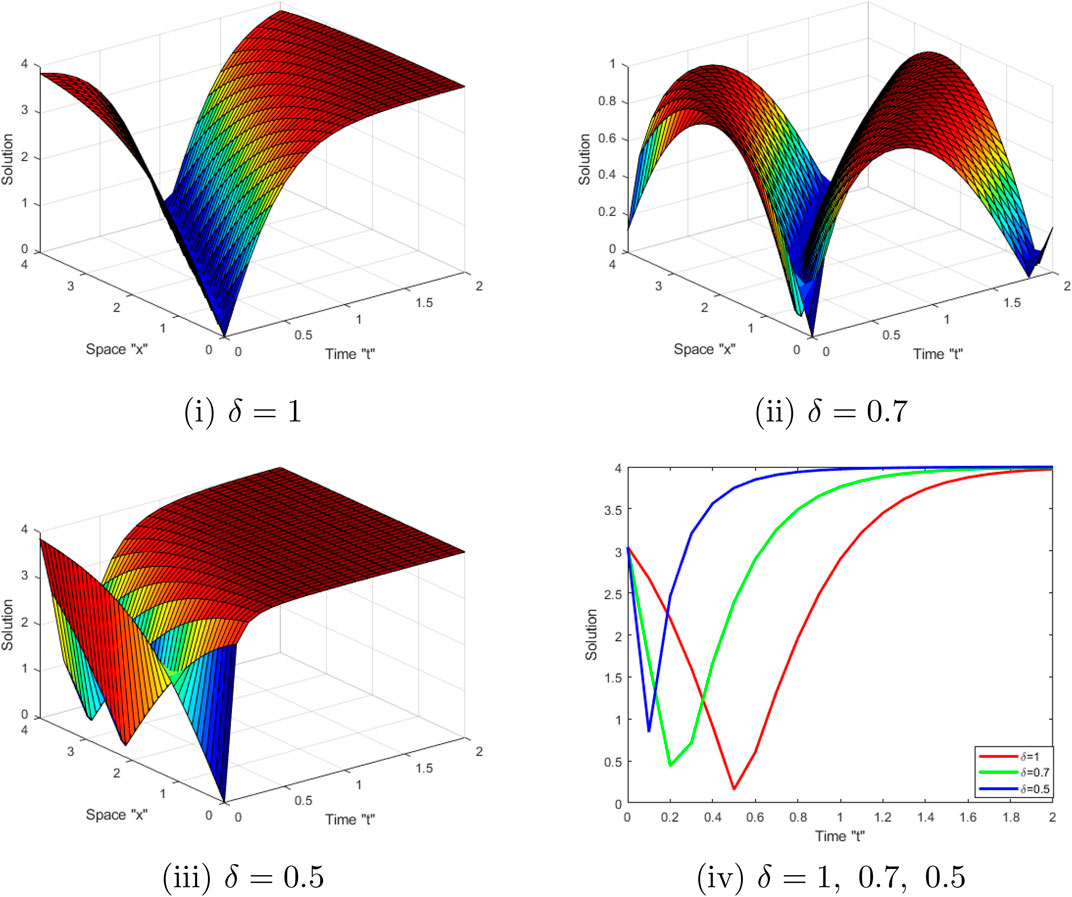
FIGURE 1. (i–iii) display 3D-shape of solution $\left|\mathcal{W}(x,t)\right|$ in Eq. 26 with δ =1,0.7,0.5 (iv) display 2D-shape of Eq. 26 with different values of δ.

FIGURE 2

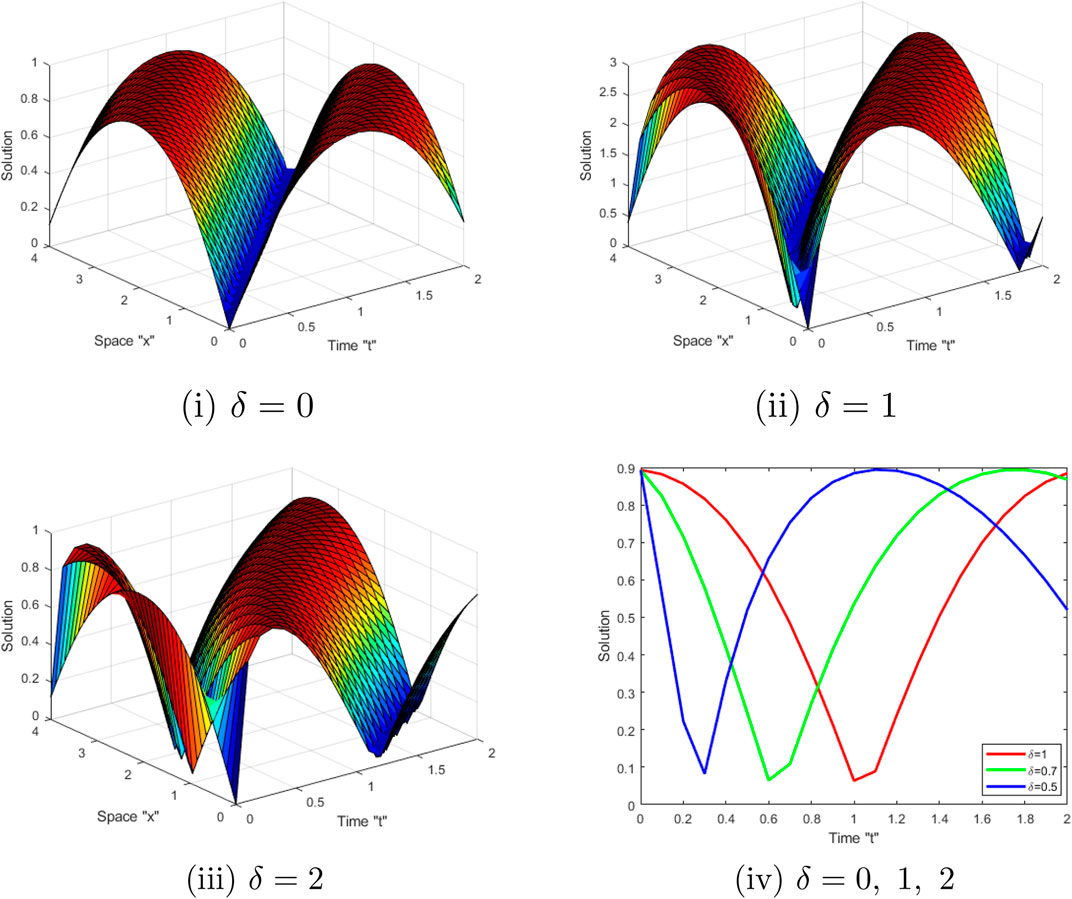
FIGURE 2. (i–iii) display 3D-shape of solution $\left|\mathcal{W}(x,t)\right|$ in Eq. 34 with δ = 1,0.7,0.5 (iv) display 2D-shape of Eq. 34 with different values of δ.

FIGURE 3

FIGURE 3. (i–iii) display 3D-shape of solution $\left|\mathcal{W}(x,t)\right|$ in Eq. 38 with δ = 1, 0.7,0.5 (iv) display 2D-shape of Eq. 38 with different values of δ.

6 Conclusion

In this study, the Sasa-Satsuma equation with M-truncated derivative (SSE-MTD) was examined. We acquired the exact solutions by utilizing Jacobi elliptic function and generalizing Riccati equation mapping methods. Because of the application of the Sasa–Satsuma equation in explaining the propagation of femtosecond pulses in optical fibers, these solutions may explain a wide range of interesting and complex physical phenomena. Furthermore, using the MATLAB program, the M-truncated derivative effects on the exact solutions of SSE-MTD (1) were illustrated. We concluded that when the derivatives order increases the surface moves into the right. In the future work, we can consider Sasa-Satsuma equation with stochastic term.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.

Author contributions

WM: software, data curation, formal analysis, investigation, methodology, writing—original draft. FA-A: data curation, investigation, formal analysis, writing—original draft. All authors contributed to the article and approved the submitted version.

Acknowledgments

Princess Nourah bint Abdulrahman University Researcher Supporting Project number (PNURSP2023R 273), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Abbreviations

2D, Two dimension; 3D, Three dimension; FNLDEs, Fractional nonlinear differential equations; GREM-method, generalizing Riccati equation mapping method; JEF-method, Jacobi elliptic function method; MTD, M-truncated derivative; ODE, Ordinary differential equation; SSE, Sasa-Satsuma equation; SW, Solitary waves.

References

1. Lyu W, Wang Z. Global classical solutions for a class of reaction-diffusion system with density-suppressed motility. Electron Res Archive (2022) 30(3):995–1015. doi:10.3934/era.2022052

CrossRef Full Text | Google Scholar

2. Xie X, Wang T, Zhang W. Existence of solutions for the (p,q)-Laplacian equation with nonlocal Choquard reaction. Appl Math Lett (2023) 135:108418. doi:10.1016/j.aml.2022.108418

CrossRef Full Text | Google Scholar

3. Zhang J, Xie J, Shi W, Huo Y, Ren Z, He D. Resonance and bifurcation of fractional quintic Mathieu–Duffing system. Chaos: Interdiscip J Nonlinear Sci (2023) 33(2):023131. doi:10.1063/5.0138864

CrossRef Full Text | Google Scholar

4. Alshammari M, Iqbal N, Botmart T. The solution of fractional-order system of KdV equations with exponential-decay kernel. Results Phys (2022) 38:105615. doi:10.1016/j.rinp.2022.105615

CrossRef Full Text | Google Scholar

5. Hussain S, Madi EN, Iqbal N, Botmart T, Karaca Y, Mohammed WW. Fractional dynamics of vector-borne infection with sexual transmission rate and vaccination. Mathematics (2021) 9(23):3118. doi:10.3390/math9233118

CrossRef Full Text | Google Scholar

6. Alshammari M, Mohammed WW, Yar M. Novel Analysis of fuzzy fractional Klein-Gordon model via Semianalytical method. J Funct Spaces (2022) 2022:1–9. doi:10.1155/2022/4020269

CrossRef Full Text | Google Scholar

7. Qt Ain QT, Anjum N, Din A, ZebDjilali AS, Khan ZA. On the analysis of Caputo fractional order dynamics of Middle East Lungs Coronavirus (MERS-CoV) model. Alex Eng J (2022) 61(7):5123–31. doi:10.1016/j.aej.2021.10.016

CrossRef Full Text | Google Scholar

8. Akbulut A, Kaplan M, Tascan F. Conservation laws and exact solutions of Phi-four (Phi-4) equation via the (G′/G, 1/G) -expansion method. Z für Naturforschung A (2016) 71(5):439–46. doi:10.1515/zna-2016-0010

CrossRef Full Text | Google Scholar

9. Wang ML, Li XZ, Zhang JL. The (G′/G)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys Lett A (2008) 372:417–23. doi:10.1016/j.physleta.2007.07.051

CrossRef Full Text | Google Scholar

10. Zhang H. New application of the (G′/G)-expansion method. Commun Nonlinear Sci Numer Simul (2009) 14:3220–5. doi:10.1016/j.cnsns.2009.01.006

CrossRef Full Text | Google Scholar

11. Naher H, Abdullah FA. New approach of (G′/G) -expansion method and new approach of generalized (G′/G) -expansion method for nonlinear evolution equation. AIP Adv (2013) 3(3):032116. doi:10.1063/1.4794947

CrossRef Full Text | Google Scholar

12. He JH, Wu XH. Exp-function method for nonlinear wave equations. Chaos Solitons Fractals (2006) 30(3):700–8. doi:10.1016/j.chaos.2006.03.020

CrossRef Full Text | Google Scholar

13. Yan ZL. Abundant families of Jacobi elliptic function solutions of the (2+1)-dimensional integrable Davey–Stewartson-type equation via a new method. Chaos Solitons Fractals (2003) 18:299–309. doi:10.1016/s0960-0779(02)00653-7

CrossRef Full Text | Google Scholar

14. Wazwaz AM. The sine-cosine method for obtaining solutions with compact and noncompact structures. Appl Math a Comput (2004) 159(2):559–76. doi:10.1016/j.amc.2003.08.136

CrossRef Full Text | Google Scholar

15. Jiong S. Auxiliary equation method for solving nonlinear partial differential equations. Phys Lett A (2003) 309:387–96. doi:10.1016/S0375-9601(03)00196-8

CrossRef Full Text | Google Scholar

16. Lu B. The first integral method for some time fractional differential equations. J Math Anal Appl (2012) 395(2):684–93. doi:10.1016/j.jmaa.2012.05.066

CrossRef Full Text | Google Scholar

17. Baskonus HM, Bulut H, Sulaiman TA. New complex hyperbolic structures to the lonngren-wave equation by using sine-gordon expansion method. Appl Math Nonlinear Sci (2019) 4(1):129–38. doi:10.2478/amns.2019.1.00013

CrossRef Full Text | Google Scholar

18. Arnous AH, Mirzazadeh M. Application of the generalized Kudryashov method to the Eckhaus equation. Nonlinear Anal Modell Control (2016) 21(5):577–86. doi:10.15388/na.2016.5.1

CrossRef Full Text | Google Scholar

19. Khan K, Akbar MA. The exp(−ϕ(ς))-expansion method for finding travelling wave solutions of Vakhnenko-Parkes equation. Int J Dyn Syst Differ Equ (2014) 5:72–83. doi:10.1504/ijdsde.2014.067119

CrossRef Full Text | Google Scholar

20. Tao H, Anjum N, Yang Y-J. The Aboodh transformation-based homotopy perturbation method: New hope for fractional calculus. Front Phys (2023) 11:1168795. doi:10.3389/fphy.2023.1168795

CrossRef Full Text | Google Scholar

21. Anjum N, He C-H, He J-H. Two-scale fractal theory for the population dynamics. Fractals (2021) 29:2150182. doi:10.1142/S0218348X21501826

CrossRef Full Text | Google Scholar

22. Anjum N, Ain QT, Li XX. Two-scale mathematical model for tsunami wave. Int J Geomath (2021) 12:10. doi:10.1007/s13137-021-00177-z

CrossRef Full Text | Google Scholar

23. Katugampola UN. New approach to a generalized fractional integral. Appl Math Comput (2011) 218(3):860–5. doi:10.1016/j.amc.2011.03.062

CrossRef Full Text | Google Scholar

24. Katugampola UN. New approach to generalized fractional derivatives. Bull Math Anal Appl B (2014) 6(4):1–15.

Google Scholar

25. Kilbas AA, Srivastava HM, Trujillo JJ. Theory and applications of fractional differential equations. Amsterdam, Netherlands: Elsevier (2016).

Google Scholar

26. Samko SG, Kilbas AA, Marichev OI. Fractional integrals and derivatives, theory and applications. Yverdon, Switzerland: Gordon and Breach (1993).

Google Scholar

27. Sousa JV, de Oliveira EC. A new truncated M fractional derivative type unifying some fractional derivative types with classical properties. Int J Anal Appl (2018) 16(1):83–96. doi:10.28924/2291-8639-16-2018-83

CrossRef Full Text | Google Scholar

28. Al-Askar FM, Cesarano C, Mohammed WW. Abundant solitary wave solutions for the boiti–leon–manna–pempinelli equation with M-truncated derivative. Axioms (2023) 12(5):466. doi:10.3390/axioms12050466

CrossRef Full Text | Google Scholar

29. Mohammed WW, Cesarano C, Al-Askar FM. Solutions to the (4+1)-dimensional time-fractional fokas equation with M-truncated derivative. Mathematics (2023) 11(1):194. doi:10.3390/math11010194

CrossRef Full Text | Google Scholar

30. Yusuf A, Inc M, Baleanu D. Optical solitons with M-truncated and beta derivatives in nonlinear optics. Front Phys (2019) 7:126. doi:10.3389/fphy.2019.00126

CrossRef Full Text | Google Scholar

31. Ozkan A, Ozkan EM, Yildirim O. On exact solutions of some space–time fractional differential equations with M-truncated derivative. Fractal and Fractional (2023) 7(3):255. doi:10.3390/fractalfract7030255

CrossRef Full Text | Google Scholar

32. Bogning JR, Tchaho CT, Kafne TC. Solitary wave solutions of the modified sasa-satsuma nonlinear partial differential equation. Am J Comput Math (2013) 3:131–7. doi:10.5923/j.ajcam.20130302.11

CrossRef Full Text | Google Scholar

33. Yildirim Y. Optical solitons to sasa-satsuma model in birefringent fibers with trial equation approach. Optik (2019) 185:269–74. doi:10.1016/j.ijleo.2019.03.016

CrossRef Full Text | Google Scholar

34. Sasa N, Satsuma J. New-type of soliton solutions for a higher-order nonlinear Schrödinger equation. J Phys Soc Jpn (1991) 60:409–17. doi:10.1143/jpsj.60.409

CrossRef Full Text | Google Scholar

35. Mihalache D, Truta N, Crasovan LC. Painlevé analysis and bright solitary waves of the higher-order nonlinear Schrödinger equation containing third-order dispersion and self-steepening term. Phys Rev E (1997) 56(1):1064–70. doi:10.1103/physreve.56.1064

CrossRef Full Text | Google Scholar

36. Solli D, Ropers C, Koonath P, Jalali B. Optical rogue waves. Nature (2007) 450(7172):1054–7. doi:10.1038/nature06402

PubMed Abstract | CrossRef Full Text | Google Scholar

37. Khater MM, Seadawy AR, Lu D. Dispersive optical soliton solutions for higher order nonlinear Sasa-Satsuma equation in mono mode fibers via new auxiliary equation method. Superlatt Microstruct (2018) 113:346–58. doi:10.1016/j.spmi.2017.11.011

CrossRef Full Text | Google Scholar

38. Chen S. Twisted rogue-wave pairs in the Sasa-Satsuma equation. Phys Rev E (2013) 88(2):023202. doi:10.1103/physreve.88.023202

CrossRef Full Text | Google Scholar

39. Tuluce DS, Pandir Y, Bulut H. New soliton solutions for Sasa–Satsuma equation. Waves Random Complex Medium (2015) 25(3):417–28. doi:10.1080/17455030.2015.1042945

CrossRef Full Text | Google Scholar

40. Seadawy AR, Nasreen N, Dian-chen LU. Optical soliton and elliptic functions solutions of Sasa-satsuma dynamical equation and its applications. Appl Math J Chin Univ. (2021) 36(2):229–42. doi:10.1007/s11766-021-3844-0

CrossRef Full Text | Google Scholar

41. Xu T, Wang D, Li M, Liang H. Soliton and breather solutions of the Sasa–Satsuma equation via the Darboux transformation. Phys Scr (2014) 7:075207. doi:10.1088/0031-8949/89/7/075207

CrossRef Full Text | Google Scholar

42. Xu J, Fan E. The unified transform method for the Sasa–Satsuma equation on the half-line. Proc R Soc A (2013) 469(2159):20130068. doi:10.1098/rspa.2013.0068

PubMed Abstract | CrossRef Full Text | Google Scholar

43. Liu Y, Gao YT, Xu T, Lü X, Sun ZY, Meng XH, et al. Soliton solution, bäcklund transformation, and conservation laws for the Sasa-Satsuma equation in the optical fiber communications. Z Nat A (2010) 65(4):291–300. doi:10.1515/zna-2010-0405

CrossRef Full Text | Google Scholar

44. Wright OC. Sasa-Satsuma equation, unstable plane waves and heteroclinic connections. Chaos Soliton Fract (2007) 33(2):374–87. doi:10.1016/j.chaos.2006.09.034

CrossRef Full Text | Google Scholar

45. Zhu SD. The generalizing Riccati equation mapping method in non-linear evolution equation: Application to (2+1)-dimensional boiti–leon–pempinelle equation. Chaos, Solitons Fractals (2008) 37:1335–42. doi:10.1016/j.chaos.2006.10.015

CrossRef Full Text | Google Scholar

46. Fan E, Zhang J. Applications of the Jacobi elliptic function method to special-type nonlinear equations. Phys Lett A (2002) 305:383–92. doi:10.1016/s0375-9601(02)01516-5

CrossRef Full Text | Google Scholar

47. Scott AC. Encyclopedia of nonlinear science. New York, NY: Routledge, Taylor and Francis Group (2005).

Google Scholar

48. Rosenau P, Hyman JM, Staley M. Multidimensional compactons. Phys Rev Lett (2007) 98:024101. doi:10.1103/PhysRevLett.98.024101

PubMed Abstract | CrossRef Full Text | Google Scholar

49. Camassa R, Holm DD. An integrable shallow water equation with peaked solitons. Phys Rev Lett (1993) 71:1661–4. doi:10.1103/PhysRevLett.71.1661

PubMed Abstract | CrossRef Full Text | Google Scholar

Nomenclature