- 1Department of Mathematical Sciences, Federal Urdu University of Arts, Sciences & Technology, Karachi, Pakistan
- 2Department of Mathematics, College of Science and Humanities in Al-Kharj, Prince Sattam Bin Abdulaziz University, Al-Kharj, Saudi Arabia
- 3Center of Research, Faculty of Engineering, Future University in Egypt New Cairo, New Cairo, Egypt
- 4Department of Mathematics, Abdul Wali Khan University, Mardan, Khyber Pakhtunkhwa, Pakistan
The amalgamation of microorganisms in the nanofluid is significant in beautifying the thermal conductivity of several systems, such as microfluid devices, chip-shaped microdevices, and enzyme biosensors. The current investigation studies mixed convective flow of the entropy minimization of unsteady MHD tangent hyperbolic nanoliquid because a stretching surface has motile density via convective and slip conditions. For the novelty of this work, the variable transport characteristics caused by dynamic viscosity, thermal conductivity, nanoparticle mass permeability, and microbial organism diffusivity are considered. It is considered that the vertical sheet studying the flow. By using the appropriate alteration, the governing equations for the most recent flow analysis were altered into a non-dimension relation. Through MATLAB Software bvp4c, the PDE model equations have been made for these transformed equations. Engineering-relevant quantities against various physical variables include force friction, Nusselt number, Sherwood number, and microorganism profiles. The results showed good consistency compared to the current literature. Moreover, these outcomes revealed that augmentation in the magnitude of the magnetic field and velocity slip parameter declines the velocity profile. The reverse impact is studied in
1 Introduction
A passion sparked by the study of non-Newtonian fluids in recent decades. Several researchers have various features in the technological, chemical, and mechanical industries. The most convenient applications to encounter non-Newtonian fluids like polymeric relation, atomic rector, solvent, biomedical in tumor, and many more. Because of the complexity of their design and subsequent management according to the model of power kind, non-Newtonian fluids can produce a connection between shear stress and shear rate that is non-linear. Because of the complexity of these fluids, it is not possible to understand the rheological properties of fluids of this type using a straightforward relation, in contrast to the Newtonian fluid. In order to determine the nature of these occurrences, the researchers offered a number of mathematical studies to investigate the material associated with non-Newtonian fluids. The tangent hyperbolic fluid model illustrates a model in which an increase in the shear effect reduces the effective viscosity of the fluid. References [1, 2] first described the flow of tangent hyperbolic fluid in a boundary layer caused by a stretched sheet. Hayat et al. [3] described the boundary-layer flow of tangent hyperbolic fluid under mass flux conditions. The double solution of tangent hyperbolic was probed by Nagendramma et al. [4]. The effect of convective conditions on tangent hyperbolic fluid induced by the homogenous–heterogeneous reaction was completed by Kumar et al. [5]. The bioconvection flow of tangent hyperbolic due to a movable surface using numerical methods was completed by Kamel et al. [6]. The effect of numerical analysis of the bioconvection flow on the tangent hyperbolic fluid past Riga plate was scrutinized by Waqas et al. [7]. The impact of the zero mass flux condition for the tangent hyperbolic nanoparticles past an exponential surface due to statistical analysis was found by Shafiq et al. [8]. The unsteady flow on the tangent hyperbolic fluid past a wedge surface was conducted by Ali et al. [9]. Hussain et al. [10] scrutinized the unsteady flow of permeable surfaces for the tangent hyperbolic fluid under slip conditions. Shafiq et al. proposed the influence of Newtonian heating having microorganisms on tangent hyperbolic nanofluid [11]. Ramzan et al. presented the 3D tangent hyperbolic nanofluid of the heat flux theory with activation energy [12].
According to experts, nanoliquid is the most innovative and compelling technology currently used in engineering and technological products, including cooling agents, vehicles, catalyst reactions, and computer chips. The thermophysical characteristics of a nanoliquid are more important than those of a regular liquid. The presence of the nanoparticle in the regular fluid is investigated for potential effects on the fluid’s thermophysical characteristics. Choi [13] created the main invention in this field. The Williamson nanofluid due to the permeable surface was done by Bhatti and Rashidi [14]. Hayat et al. [15] described the melting heat transfer with thermal radiation induced by the variable thickness of the carbon nanotubes (CNT). Kumar et al. studied the effect of semiconductors on the thermal properties of CuO-H2O with the base fluid of nanoparticles [16]. The influence of thermal radiation using Sisko nanofluid with activation energy was evaluated by Ijaz et al. [17]. Khan and Shehzad [18] investigated the joule heating effect for the flow of third-grade nanomaterials due to the periodic motion sheet. The induction of nanoparticles in solar energy was discussed by Fayaz et al. [19]. Faizan et al. [20] examined how fluids move thermally and generate entropy during the tangent hyperbolic flow with the Darcy–Forchheimer flow across a stretching surface. Olanrewaju et al. [21] exploited Maxwell nanofluid due to the permeable surface by second law analysis. The heat transfer in the porous surface for the second law analysis of Maxwell nanofluid was reported by Alagumalai et al. [22], elaborating on applying nanofluid with thermal energy and technological applications. Tayebi et al. [23] discovered the natural convection flow of nanofluids with entropy generation due to annular enclosure. Chamka et al. [24] constituted an MHD natural convection flow and thermal radiation in the cavity enclosure using the finite element method. Seyyedi et al. [25] examined the MHD natural convection flow of nanofluids on entropy minimization in a wavy porous enclosure. Dogonchi et al. [26] explained the combined effect of thermal radiation and joule heat on the natural convection of nanoliquid between two disks. Eshaghi et al. [27] discussed the Baffle length H-shaped cavity with the addition of nanoparticles. Afshar et al. [28] probed the free convection flow of nanofluids inside the porous wavy enclosure. A titanium dioxide nanofluid based on 10 W40 subjected to electroosmotic forces and peristaltic propulsion in a curved microchannel is the subject of the current study, which focuses on the fluid flow analysis and heat transfer properties of the material explored by Akram et al. [29]. Maraj et al. [30] analyzed the oscillatory pressure-driven MHD flow of a hybrid nanofluid in a vertical rotating channel with velocity slip, thermal periodic limitations, and the effect of hall current, which are the main topics of the current mathematical investigation. Akram et al. [31] investigated the fluid flow properties and heat transmission by the drilling muds augmented with nanoparticles flowing through the drilling pipes under different physical conditions. Shah et al. [32] investigated how energy is transferred as engine oil-based Prandtl–Eyring nanofluid flows across a heated stretching surface. The influence of slip velocity across a vertically declining sheet, heat generation and absorption, and convection in a two-dimensional magnetic nanofluid are all statistically investigated by Asghar et al. [33]. Some other important studies can be found [34–39].
Researchers have used the oxytactic and erratic motion of microorganisms as a model to examine the processes of bioconvection. Slanting swimming cells are related to bioconvection, which focuses on different types of microorganisms. Ecological systems, biofuels, biosensors, and many more technologies successfully use the physical meaning of bioconvection. Due to the interaction of microorganisms, the bioconvection of nanoparticles is correlated with density. It has been noted that gyrotactic bacteria successfully increased the stability of nanoparticles. In recent years, several researchers have examined significant events. The effect of bioconvection employing nanoparticles past a permeable sheet was studied by Saini and Sharma [40]. Dhanai et al. [41] investigated the MHD flow gyrotactic microorganism on the nanoparticles past an inclined sheet. The force connection with bioconvection resulting from a vertical wedge plate was performed by Mahdy [42]. Avinash et al. [43] found the magnetic field of natural convective and microorganisms together with nanoliquid. Makinde and Animasaun [44] analyzed the impact of non-linear thermal radiation induced by the microorganism with a magnetic field and nanofluid. The impact of the slip effect with MHD flow on gyrotactic microorganisms was conducted by Khan et al. [45]. The mixed convective flow of bioconvection past a spongy plate was designed by Mutuku and Makinde [46]. The impact of convective conditions on the magnetic field flow of bioconvection was worked on by Khan et al. [47].
It has been brought to our attention that the second law analysis of bioconvection phenomena in the flow of tangent hyperbolic nanofluids with a slip effect under thermal radiation has not yet been considered. The motive is to fill the gap. This was discovered after careful observation of the review highlighted previously. In contrast to the standard approach, the flow, in this case, is caused by a vertical surface. It has been thoroughly reviewed and determined that such characteristics are not researched. Fluid flow past vertical stretching sheets has been proven to have numerous applications in current times due to physical and technological applications, such as the processing of polymers, the petrochemical sector, various production processes, glass production, and rolling hot. Because of these motivating factors, we decided to direct our attention to this substantial research gap. The present investigation was performed by simulating the features of thermal radiation, which has studied a large variety of features in thermal extrusion processing, solar energy, defense system, heavy automatic equipment, atomic chain reactions, and many other processes. The answer to the inserted problem is approached by methodically computing it using the BVP4c approach. The graphical results of various emerging variables besides the flow field are studied and evaluated.
2 Problem formulation
We presume a two-dimensional, laminar, unsteady, velocity effect of an MHD incompressible tangent hyperbolic nanofluid comprising bioconvection with entropy analysis. The fluid system is surrounded by a stretched sheet that is saturated with motile microorganisms and has a velocity of
The constitutive equation for the flow of the model (continuity, momentum, energy, concentration, and microorganism) is represented as follows [48–53]:
The corresponding boundary conditions are
Here, the component of velocity is
By considering the appropriate transformation [20],
The application of the suitable alteration in Eqs 2–5, subject to the boundary conditions in Eq. 6, is rendered into a system of ODEs [48]:
The boundary conditions are as follows [20], [42], [48]:
The dimensionless variables in the aforementioned equations are the unsteady parameter denoted by
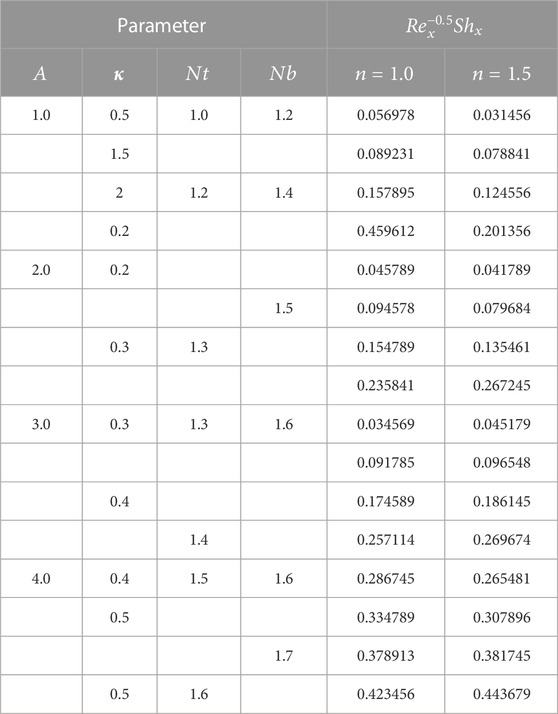
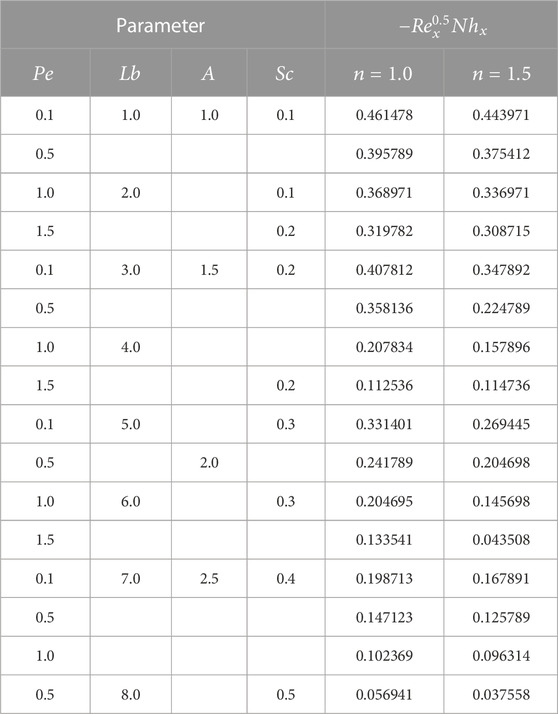
Skin friction, Nusselt number, Sherwood number, and motile microorganism are as follows:
The values for the aforementioned quantities are as follows:
Equation 14 and Eq. 15 by using Eq. 7
3 Implemented strategy
It is obvious that the presented problem Eqs. (8–11) with boundary assumptions Eq. 12 cannot be handled analytically due to the problem’s extreme non-linearity. Hence, we employ the well-known BVP4c technique to simulate the numerical solution. Actually, BVP4c is used as a powerful method to achieve accurate results. The ordinary differential boundary value problem is resolved using this method. Each method, such as bvp4c MATLAB, has advantages and disadvantages. The main advantage is that it accepts multipoint BVPs natively, in contrast to many other solvers. As the source code is public, we can easily and gratis modify it to function with already-existing equations. MATLAB is an interpreted language; thus, making corrections is easy. Moreover, there are several issues concerning its ability to resolve first-order differential equations. You must first reduce your system to the first order before utilizing the bvp4c MATLAB method to solve higher orders. MATLAB executes more slowly than C and C++ because, as was already mentioned, it is an interpreted language. The technique turns the initial BVP into an IVP by applying the following presumptions: we will assign a few extra variables to accomplish this goal:
The criteria for new variable boundaries include
The far boundary is
4 Entropy generation
The influence of the magnetic field, thermal radiation and diffusion, and entropy generation
Eq. 20 shows the formation of entropy through the first phrase, which represents heat transfer, the second term, fluid friction, and the third term through the sixth term, diffusive irreversibility. The importance of entropy production can be expressed mathematically as
Using Eq. 6, it is possible to modify the rate of entropy minimization using tangent hyperbolic [54]:
5 Result and discussion
The numerous variables over
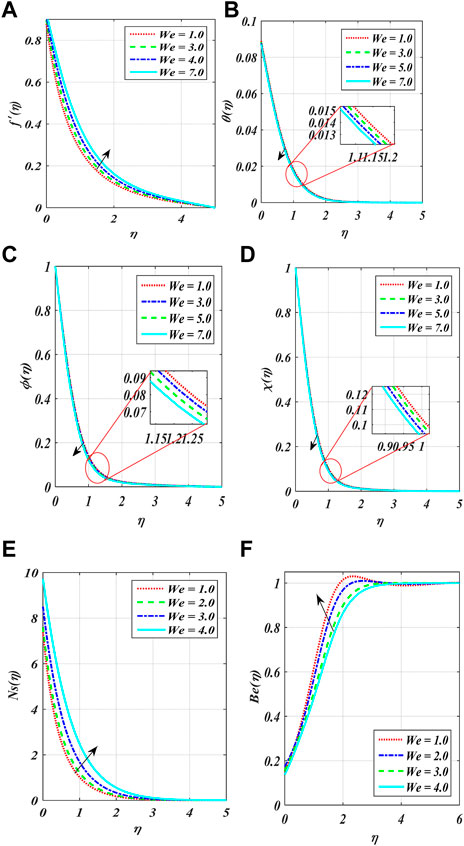
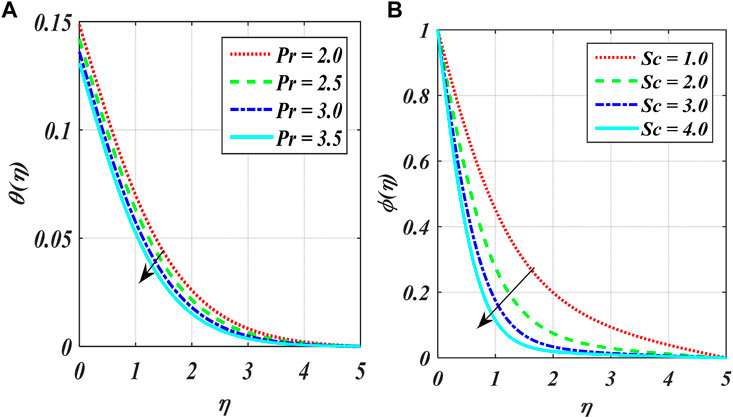
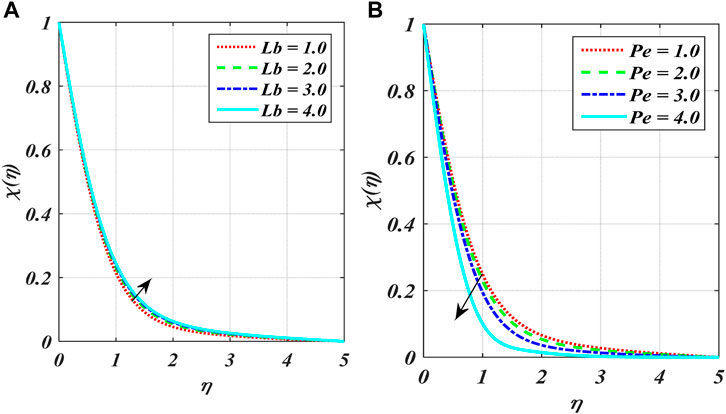
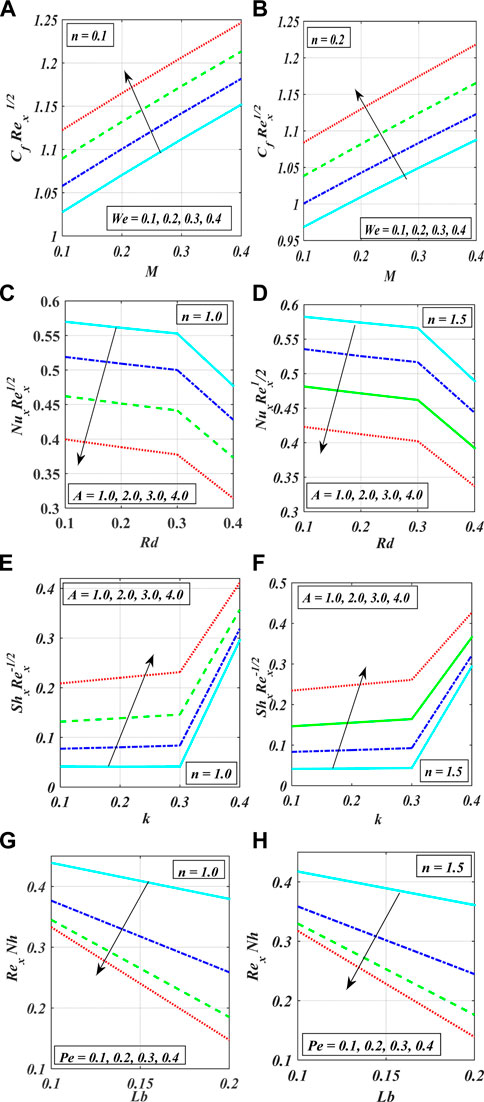
Figures 2A–F show the variation in power index number
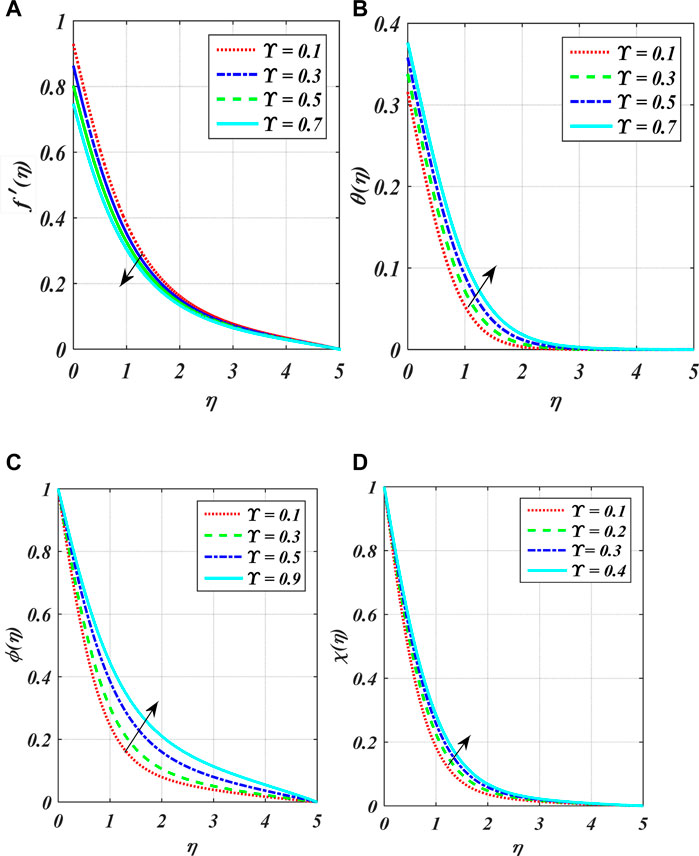
The impact of
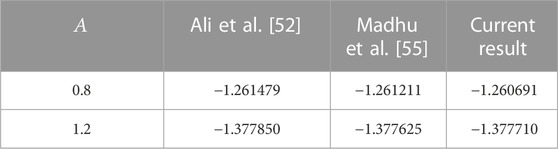
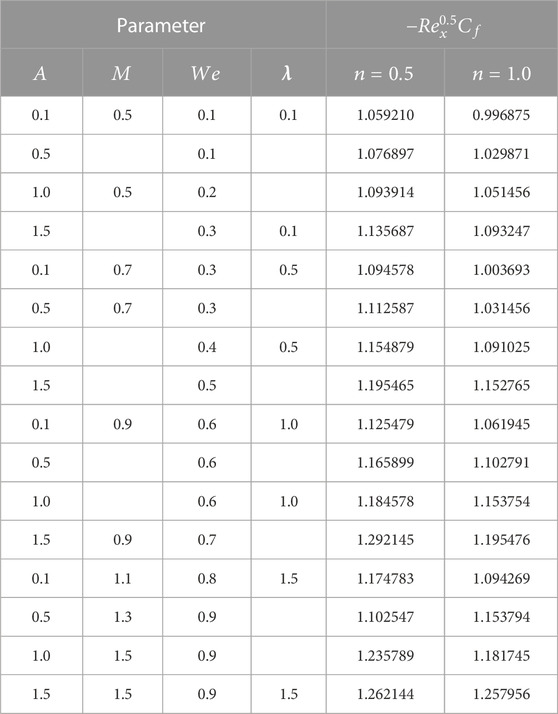
Table 1 compares the current problem with the published results of Ali et al. [52] and Madhu et al. [55]. In this comparison, the current outcomes present excellent achievement with the existing published results. Table 2 shows the variation
6 Final remarks
This study measured the impact of velocity slip on the erratic flow of a mixed convection MHD tangent hyperbolic nanofluid containing moving microorganisms. The articulated ordinary differential equation and specified boundary conditions generated from the similarity solutions are resolved using the bvp4c solver in MATLAB. MATLAB bvp4c is time-saving, efficient, durable, fast-converging, and consistent with earlier studies. The effects of a few associated control parameters are highlighted. The physical variables over
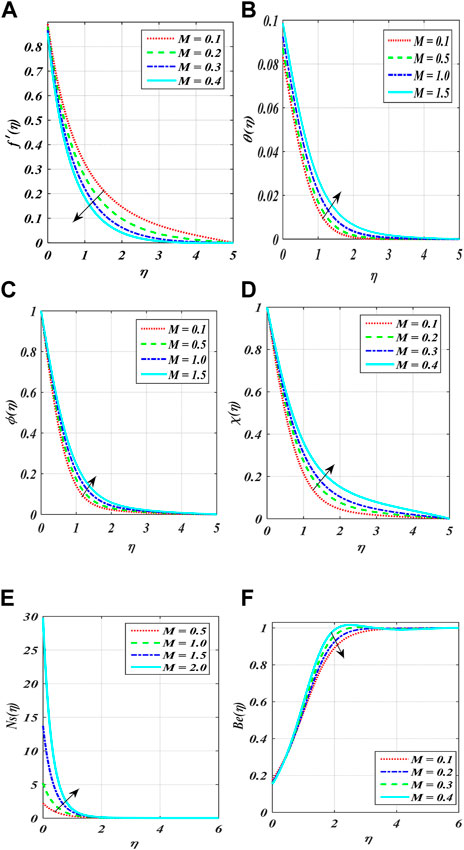
• Boundary-layer thickness augments for temperature, concentration, and density distributions, although the velocity profile decays as an enhancement in
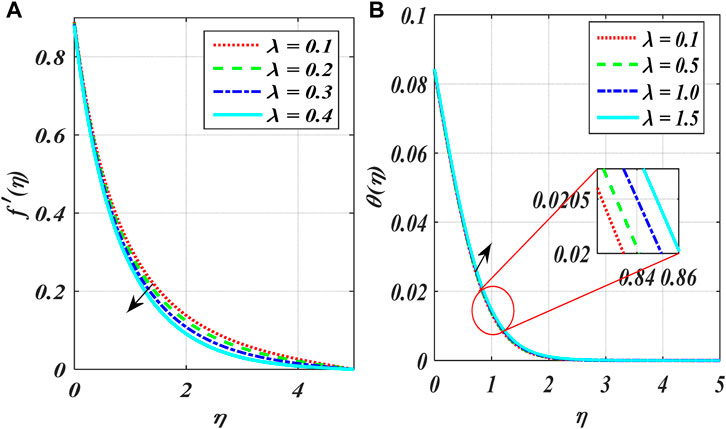
• The velocity profile declines in contrast to the increasing value of the power law index. Since the dimensionless parameter
• When the unsteady variable values increase, the temperature profile increases; however, when the Weissenberg number increases, it decreases.
• The microorganism field has diminished due to the change in
• Rising the values of
• At higher quantities of
•
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material. Further inquiries can be directed to the corresponding authors.
Author contributions
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
Funding
This study was funded by Prince Sattam Bin Abdulaziz University (Project no. PSAU/2023/R/1444).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Nadeem S, Hussain ST. Changhoon lee, “flow of a williamson fluid over astretching sheet. Brazillian J Chemeng (2013) 30(03):619–25. July - September.
2. ZakirUllah GZ. Lie group analysis of magnetohydrodynamic tangent hyperbolic fluid flow towards a stretching sheet with slip conditions. Cite as: Heliyon (2017) 3:e00443. doi:10.1016/j.heliyon.2017.e00443
3. Hayat T, Ullah I, Alsaedi A, Ahmad B. Modeling tangent hyperbolic nanoliquid flow with heat and mass flux conditions. Eur Phys J Plus (2017) 132:112. doi:10.1140/epjp/i2017-11369-0
4. Nagendramma V, Leelarathnam A, Raju CSK, Shehzad SA, Hussain T. Doubly stratified MHD tangent hyperbolic nanofluid flow due to permeable stretched cylinder. Res Phys (2018) 9:23–32. doi:10.1016/j.rinp.2018.02.019
5. Geethan Kumar S, Varma SVK, Kiran Kumar RVMSS, Raju CSK, Shehzad SA, Bashir MN. Three-dimensional hydromagnetic convective flflow of chemically reactive Williamson fluid with non-uniform heat absorption and generation. Int J Chem Reactor Eng (2019).
6. Al-KhaledKhanKhan KSUI. Chemically reactive bioconvection flow of tangent hyperbolic nanoliquid with gyrotactic microorganisms and nonlinear thermal radiation. Heliyon (2020) 6:e03117. doi:10.1016/j.heliyon.2019.e03117
7. Waqas H, Kafait A, Muhammad T, Farooq U. Numerical study for bio-convection flow of tangent hyperbolic nanofluid over a Riga plate with activation energy. Alexandria Eng J (2022) 61:1803–14. doi:10.1016/j.aej.2021.06.068
8. Shafq A, Lone SA, Sindhu TN, Al-Mdallal QM, Rasool G. Statistical modeling for bioconvective tangent hyperbolic nanofluid towards stretching surface with zero mass flux condition. Sci Rep () 11:13869. doi:10.1038/s41598-021-93329-y
9. Ali B, sHussain , Naqvi SIR, Habib D, Abdal S. Aligned magnetic and bioconvection effects on tangent hyperbolic nanofluid flow across faster/slower stretching wedge with activation energy: Finite element simulation. Int J Appl Comput Math (2021) 7:149. doi:10.1007/s40819-021-01097-0
10. Hussain S, Ahmad F, Ayed H, Malik MY, Waqas H, Al-Sawalha MM, et al. Combined magnetic and porosity effects on flow of time-dependent tangent hyperbolic fluid with nanoparticles and motile gyrotactic microorganism past a wedge with second-order slip. Case Stud Therm Eng (2021) 26:100962. doi:10.1016/j.csite.2021.100962
11. Shafiq A, Hammouch Z, Sindhu TN, “Bioconvective MHD flow of tangent hyperbolic nanofluidwithnewtonian heating,” International Journal of Mechanical SciencesVolume 133, November 2017, Pages 759–66. doi:10.1016/j.ijmecsci.2017.07.048
12. Ramzan M, Chu YM, Gul H, Kadry S, Yu-Ming C. Role of bioconvection in a three dimensional tangent hyperbolic partially ionized magnetized nanofluid flow with Cattaneo-Christov heat flux and activation energy. Int Commun Heat Mass TransferVolume (2021) 120:104994. bioconvection in a three dimensional tangent hyperbolic partially ionized magnetized nanofluid flow with Cattaneo-Christov heat flux and activation energy doi:10.1016/j.icheatmasstransfer.2020.104994
13. Choi SUS. Enhancing thermal conductivity of fluids with nanoparticles. Int Mech Eng Cong Exp Asme, FED (1995) 66:99–105.
14. Bhatti MM, Rashidi MM. Effects of thermo-diffusion and thermal radiation on Williamson nanofluid over a porous shrinking/stretching sheet. J Mol Liq September (2016) 21:567–73. doi:10.1016/j.molliq.2016.05.049
15. Hayat T, Muhammad K, Farooq M. AlsaediA.Melting heat transfer in stagnation point flow of carbon nanotubes towards variable thickness surface. AIP Adv (2019) 6015214.
16. Kumar PCM, Kuma CMA. Numerical evaluation of cooling performances of semiconductor using CuO/water nanofluids. Heliyon (2019) 5:5e02227. doi:10.1016/j.heliyon.2019.e02227
17. Ijaz M, Ayub M, Khan H. Entropy generation and activation energy mechanism in non-linear radiative flow ofSiskonanofluid: Rotating disk. Heliyon (2019) 5:5e01863. doi:10.1016/j.heliyon.2019.e01863
18. Khan SU, Shehzad SA. Brownian movement and thermophoretic aspects in third grade nanofluid over oscillatory moving sheet. Phys Scr (2019) 94:94095202. doi:10.1088/1402-4896/ab0661
19. Fayaz H, Nasrin R, Rahim NA, Hasanuzzaman M. Energy and exergy analysis of the PVT system: Effect of nanofluid flow rate. Solar Energy (2018) 169:217–30. doi:10.1016/j.solener.2018.05.004
20. Ahmed MF, Khalid M, Ali F, Alduais FS, Al-Bossly A, Saeed A. Second law analysis in Darcy- Forchheimer flow of tangent hyperbolic nanofluid in the presence of gyrotacticisroorganisms. Z Angew Math Mech (2023) e202200466.
21. Olanrewaju AM, Salawu SO, Olanrewaju PO, Amoo SA. Unsteady radiativemagnetohydromagnetic flow and entropy generation ofmaxwellnanofluidin a porous medium with arrhenius chemical kinetic. Cogent Eng (2021) 8(1):1942639. doi:10.1080/23311916.2021.1942639
22. Alagumalai A, Qin C, Vimal KEK, Evgeny S, Liu . Y, Zhang P, et al. Conceptual analysis framework development to understand barriers of nanofluid commercialization. Nano Energy (2022) 92:106736. doi:10.1016/j.nanoen.2021.106736
23. Tayebi T, SattarDogonchi A, Karimi N, Ge-JiLe H, Chamkha AJ, Elmasry Y. “2021, Thermo-economic and entropy generation analyses of magnetic natural convective flow in a nanofluid-filled annular enclosure fitted with fins.” Sustainable Energ Tech Assessments 46 (2021): 101274. doi:10.1016/j.seta.2021.101274
24. Chamkha AJ, Dogonchi AS, Ganji DD. MagnetohydrodynamicNanofluid natural convection in a cavity under thermal radiation and shape factor of nanoparticles impacts: A numerical study using cvfem. Appl Sci (2018) 8:2396–12. doi:10.3390/app8122396
25. Seyyedi SM, Dogonchi AS, Hashemi-Tilehnoee M, Ganji DD, Chamkha AJ. Second law analysis of magneto-natural convection in a nanofluid filled wavy-hexagonal porous enclosure. Int J Numer Methods Heat Fluid Flow (2020) 30:4811–36. 11 (January 1, 2020): 4811–36. doi:10.1108/HFF-11-2019-0845
26. Dogonchi AS, Waqas M, Afshar SR, Seyyedi SM, Hashemi-Tilehnoee M, Chamkha AJ, et al. Investigation of magneto-hydrodynamic fluid squeezed between two parallel disks by considering joule heating, thermal radiation, and adding different nanoparticles. Int J Numer Methods Heat Fluid Flow (2019) 30(2):659–80. doi:10.1108/HFF-05-2019-0390
27. Eshaghi S, Farhad I, Sattar Dogonchi A, Chamkha AJ, Mohamed B, Ben H, et al. 2021. “The optimum double diffusive natural convection heat transfer in H-shaped cavity with a baffle inside and a corrugated wall.” Case Stud Therm Eng 28 (2021): 101541. doi:10.1016/j.csite.2021.101541
28. Afshar SR, Mishra SR, SattarDogonchi A, Karimi N, Chamkha AJ, Abulkhair H. Dissection of entropy production for the free convection of NEPCMs-filled porous wavy enclosure subject to volumetric heat source/sink. J Taiwan Inst Chem Eng (2021) 128:98–113. doi:10.1016/j.jtice.2021.09.006
29. Akram J, Akbar NS, Monairah A, Tripathi D, “Electroosmotically modulated peristaltic propulsion of TiO2/10W40 nanofluid in curved microchannel” Intternational Commun Heat Mass Transfer, Vol. 136, 106208, doi:10.1016/j.icheatmasstransfer.2022.1062082022, 1062.
30. Maraj EN, Zehra I, Akbar NS, “Rotatory flow of MHD (MoS2-SiO2)/H2O hybrid nanofluid in a vertical channel owing to velocity slip and thermal periodic conditions(MoS2-SiO2)/H2Ohybrid nanofluid in a vertical channel owing to velocity slip and thermal periodic conditions,” Colloids Surf A: Physicochemical Eng Aspects, Vol. 639, 2022, 128383, doi:10.1016/j.colsurfa.2022.128383
31. Akram J, Akbar NS. Mathematical modeling of Aphron drilling nanofluid driven by electroosmotically modulated peristalsis through a pipe. Math Model Nat Phenom (2022) 17:19. doi:10.1051/mmnp/2022012
32. Shah Z, Rooman M, Shutaywi M. Computational analysis of radiative engine oil-based Prandtl–Eyring hybrid nanofluid flow with variable heat transfer using the Cattaneo–Christov heat flux model. RSC Adv Issue (2023) 13:3552–60. doi:10.1039/d2ra08197k
33. Asghar A, Chandio AF, Shah Z, Vrinceanu N, Deebani W, Shutaywi M, et al. Magnetized mixed convection hybrid nanofluid with effect of heat generation/absorption and velocity slip condition. Heliyon (2023) 9(issue 2):e13189. doi:10.1016/j.heliyon.2023.e13189
34. Akbar NS, Maraj EN, Noor NFM, Habib MB. Exact solutions of an unsteady thermal conductive pressure driven peristaltic transport with temperature – dependent nanofluid viscosity. Case Stud Therm Eng (2022) 35:102124. doi:10.1016/j.csite.2022.102124
35. Habib MB, Akbar NS. New trends of nanofluids to combat Staphylococcus aureus in clinical isolates. J Therm Anal Calorim (2021) 143:1893–9. doi:10.1007/s10973-020-09502-4
36. Akram J, Akbar NS, Tripathi D, “ Electroosmosis augmented MHD peristaltic transport of SWCNTs suspension in aqueous media,” J Therm Anal Calorim, 2022, Vol. 147 Issue 3, p2509-26. doi:10.1007/s10973-021-10562-3
37. Shah Z, Ullah A. Ferrofluid treatment with insertion of electric field inside a porous cavity considering forced convection. Waves in Random and Complex Media (2023) 1–19. doi:10.1080/17455030.2023.2169386
38. Tang T-Q, Rooman M, Shah Z, MA , Vrinceanu N, Racheriu M, “2023, Computational study and characteristics of magnetized gold-blood Oldroyd-B nanofluid flow and heat transfer in stenosis narrow arteries,” J Magnetism Magn Mater, Vol. 569, 2023, 170448, doi:10.1016/j.jmmm.2023.170448
39. Khan I, Raja MAZ, Khan MAR, Shoaib M, Islam S, Shah Z. Design of backpropagated intelligent networks for nonlinear second-order lane–emden pantograph delay differential systems. Arabian J Sci Eng (2022) 47:1197–210. doi:10.1007/s13369-021-05814-1
40. Saini S, Sharma YD. Double-diffusive bioconvection in a suspension of gyrotactic microorganisms saturated by nanofluid. Int J Appl Fluid Mech (2019) 12:271–80. doi:10.29252/jafm.75.253.29097
41. Dhanai R, Rana P, Kumar L. Lie group analysis for bioconvection MHD slip flow and heat transfer of nano fluid over an inclined sheet: Multiple solutions. J Taiwan Instchem Eng (2016) 66:283–91. doi:10.1016/j.jtice.2016.07.001
42. Mahdy A. Gyrotactic microorganisms mixed convection nanofluid flow along an isothermal vertical wedge in porous media. Int J Mech Aero Indus Mechatronmanuf Eng (2017) 11(4):840–50.
43. Avinash K, Sandeep N, Makinde OD, Animasaun IL. Aligned magnetic field effect on radiativebioconvection flow past a vertical plate with thermophoresis and Brownian motion. Defect Diffus Forum (2017) 377:127–40. doi:10.4028/www.scientific.net/ddf.377.127
44. Makinde OD, Animasaun IL. Thermophoresis and Brownian motion effects on MHD bioconvection of nanofluid with non-linear thermal radiation and quartic chemical reaction past an upper horizontal surface of a paraboloid of revolution. J Mol Liq (2016) 21:733–43. doi:10.1016/j.molliq.2016.06.047
45. Khan WA, Makinde OD, KhaN ZH. MHD boundary layer flow of a nanofluid containing gyrotactic microorganisms past a vertical plate with Navier slip. Int J Heat Mass Transf (2014) 74:285–91. doi:10.1016/j.ijheatmasstransfer.2014.03.026
46. Mutuku WN, Makinde OD. Hydromagnetic bioconvection of nanofluid over a permeable vertical plate due to gyrotactic microorganisms. Comput Fluids (2014) 95:88–97. doi:10.1016/j.compfluid.2014.02.0262014
47. Khan WA, Makinde OD. MHD nanofluidbioconvection due to gyrotactic microorganisms over a convectively heat stretching sheet. Int J Therm Sci (2014) 81:118–24. doi:10.1016/j.ijthermalsci.2014.03.009
48. Shafiq A, Sindhu T, Masood Khalique C. Numerical investigation and sensitivity analysis on bioconvective tangent hyperbolic nanofluid flow towards stretching surface by response surface methodology. Alexandria Eng J (2020) 59(6):4533–48. doi:10.1016/j.aej.2020.08.007
49. Atif SM, Khan WA, Abbas M, Rashid U. BioconvectionMangnetohydrodynamic tangent hyperbolic nanofluid flow with quartic chemical reaction past a paraboloid surface. Comput Model Eng Sci (2022) 130(1):205–20. doi:10.32604/cmes.2022.017304
50. Ashraf MZ, Rehman SU, Farid S, Hussein AK, Ali B, Shah NA, et al. Insight into significance of bioconvection on MHD tangent hyperbolic nanofluid flow of irregular thickness across a slender elastic surface. Mathematics (2022) 10:2592. doi:10.3390/math10152592
51. Reddy CS, Ali F, Al-Farhany K, Sridhar W. Numerical analysis of gyrotactic microorganisms in MHDradiative Eyring–Powell nanofluid across a static/movingwedge with Soret and Dufour effects. Z Angew Math Mech (2022) 102:e202100459. doi:10.1002/zamm.202100459
52. Ali F, Padmavathi T, Hemalatha B. Entropy minimization in Darcy Forchheimer on Sutterbynanofluid past a stretching surface with swimming of gyrotactic microorganisms. Waves in Random and Complex Media (2022) 1–24. doi:10.1080/17455030.2022.2112635
53. Ali F, Reddy CS, Summayya N, Ahmed MF. Effect of nonlinear thermal radiation on unsteady MHD flow of Maxwell nanoliquid over a porous vertical sheet with chemical reaction. Heat Transfer (2021) 50:8259–79. doi:10.1002/htj.22276
54. Zhao T, Khan MR, Chu Y, Issakhov A, Ali R, Khan S. Entropy generation approach with heat and mass transfer in magnetohydrodynamic stagnation point flow of a tangent hyperbolic nanofluid. Appl Maths Mech (2021) 42:1205–18. doi:10.1007/s10483-021-2759-5
Madhu M, Kishan N, Chamkha AJ. Unsteady flow of a Maxwell nanofluid over a stretching surface in thepresence of magnetohydrodynamic and thermal radiation effects. Purpul Power Res (2015) 6:31–40. doi:10.1016/j.jppr.2017.01.002
Nomenclature
Greeks
Keywords: nanofluids, entropy minimization, gyrotactic microorganism, MHD, thermal radiation
Citation: Faizan Ahmed M, Khalid M, Ali F, Al-Bossly A, Alduais FS, Eldin SM and Saeed A (2023) Importance of bioconvection flow on tangent hyperbolic nanofluid with entropy minimization. Front. Phys. 11:1154478. doi: 10.3389/fphy.2023.1154478
Received: 30 January 2023; Accepted: 20 March 2023;
Published: 07 April 2023.
Edited by:
Felix Sharipov, Federal University of Paraná, BrazilReviewed by:
Noreen Sher Akbar, National University of Sciences and Technology (NUST), PakistanEbenezer Bonyah, University of Education, Winneba, Ghana
Copyright © 2023 Faizan Ahmed, Khalid, Ali, Al-Bossly, Alduais, Eldin and Saeed. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Sayed M. Eldin, c2F5ZWQuZWxkaW4yMkBmdWUuZWR1LmVn; Anwar Saeed, YW53YXJzYWVlZDc2OUBnbWFpbC5jb20=
 M. Faizan Ahmed
M. Faizan Ahmed M. Khalid1
M. Khalid1 Farhan Ali
Farhan Ali Sayed M. Eldin
Sayed M. Eldin Anwar Saeed
Anwar Saeed