Impacts of Generalized Uncertainty Principle on the Black Hole Thermodynamics and Phase Transition in a Cavity

In this work, we conduct a study regarding the thermodynamic evolution and the phase transition of a black hole in a finite spherical cavity subject to the generalized uncertainty principle. The results demonstrate that both the positive and negative generalized uncertainty principle parameters β₀ can significantly affect the thermodynamic quantities, stability, critical behavior, and phase transition of the black hole. For β₀ > 0, the black hole forms a remnant with finite temperature, finite mass, and zero local heat capacity in the last stages of evolution, which can be regarded as an elementary particle. Meanwhile, it undergoes one second-order phase transition and two Hawking-Page-type phase transitions. The Gross-Perry-Yaffe phase transition occurs for both large black hole configuration and small black hole configuration. For β₀ < 0, the Gross-Perry-Yaffe phase transition occurs only for large black hole configuration, and the temperature and heat capacity of the black hole remnant is finite, whereas its mass is zero. This indicates the remnant is metastable and would be in the Hawking-Page-type phase transition forever. Specifically, according to the viewpoint of corpuscular gravity, the remnant can be interpreted as an additional metastable tiny black hole configuration, which never appears in the original case and the positive correction case.

1 Introduction

The Planck scale is well known as the minimum scale in nature. In the vicinity of it, many works expected that the quantum theory and gravity would merge into a theory of quantum gravity (QG) [1–4]. Therefore, the Planck scale can be regarded as a demarcation line between classical gravity and QG. For a long time, it is believed that the properties of different physical systems would be properly changed at the Planck scale due to the effect of QG. For example, when the Heisenberg uncertainty principle (HUP) approaches the Planck scales, it should be modified to the so-called generalized uncertainty principle (GUP) [5–7]. In this sense, Kempf, Mangano, and Mann [5] proposed one of the most adopted GUP, as follows:

$\mathrm{\Delta }x\mathrm{\Delta }p\ge \frac{\hslash }{2}\left[1+{\beta }_0\frac{{\ell }_p^2\mathrm{\Delta }{p}^2}{{\hslash }^2}\right],(1)$

where ℓ_p is the Planck length, β₀ is a dimensionless GUP parameter, while that ℏ corresponds to the reduced Planck constant. Meanwhile, inequality (1) is equivalent to the modified fundamental commutation relation $\left[x_i,p_i\right]=i\hslash {\delta }_{ij}\left[1+{\beta }_0{\ell }_p^2{p}^2{\hslash }^2\right]$ with the position operator x_i and momentum operator p_i. For the sake of simplicity, the GUP parameter is always taken to be positive and of the order of unity, so that the results are only efficient at the Planck length. However, if this assumption is ignored, Eq. 1 can be treated by phenomenology for constraining bound of GUP parameter from experimental and observational, such as gravitational bar detectors, electroweak measurement, ⁸⁷Rb cold-atom-recoil experiment, and Shapiro time delay [8–17]. With this in mind, it is believed that some new physics may appear [18].

Despite the GUP with a positive parameter plays an important role in many physical systems, such as gravitational theory and astrophysics [19–24], black hole physics [25–36], cosmology [37–42], quantum physics [43–46]. It is still beneficial to investigate how GUP with the negative parameters affects the classical theories [10, 47–49]. Recently, it has been proposed that the Chandrasekhar limit fails with a positive GUP parameter and leads to the mass of white dwarfs being arbitrarily large [50, 51]. For solving this paradoxical situation, Ong suggests taking a negative GUP parameter, which naturally restores the Chandrasekhar limit [52]. In this regard, to be compatible with the previous works of thermodynamics of black holes, the Hawking temperatures with both positive and negative GUP parameters have been substantively revised in Ref. [53], which can be expressed as follows:

$T_H^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)=\frac{M{c}^2}{4\pi {\beta }_0}\left(1-\sqrt{1-\frac{{\beta }_0\hslash c}{G{M}^2}}\right),(2)$

$T_H^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)=-\frac{M{c}^2}{4\pi {\beta }_0}\left(1+\sqrt{1-\frac{{\beta }_0\hslash c}{G{M}^2}}\right),(3)$

where β₀ is the GUP parameter, M is the mass of the Schwarzschild (SC) black hole, whose line element is $\mathrm{d}{s}^2=-f\left(r\right)\mathrm{d}{t}^2+-f^{-1}\left(r\right)\mathrm{d}{r}^2+r^2\mathrm{d}{\theta }^2+r^2{\sin }^2\theta \mathrm{d}{\phi }^2$ with $f\left(r\right)=1-2GM/r$. It is notable that the modified Hawking temperatures reproduce the original cases for β₀ → 0. Moreover, based on the first law of black hole thermodynamics dM = TdS, the GUP corrected entropy associated with Eq. 2 and Eq. 3 read

$S^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)=2\pi \left\{\frac{G{M}^2}{c^3\hslash }\left(1+\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right)\right.-\frac{{\beta }_0}{c^2}\left.\ln \left[M\left(1+\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right)\right]\right\},(4)$

$S^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)=2\pi \left\{\frac{G{M}^2}{c^3\hslash }\left(1+\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right)\right.-\frac{{\beta }_0}{c^2}\left.\ln \left[M\left(1+\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right)\right]\right\},(5)$

where the logarithmic corrections on the right-hand side of Eq. 4 and Eq. 5 are consistent with the expectation of QG theories [49]. The original area law of the entropy $S=4\pi G{M}^2/c^3\hslash$ is recovered in HUP. According to Eqs 2–5, it is worth noting that whether β₀ > 0 or β₀ < 0, the pictures of Hawking radiation are deviate from the classical one (see Refs. [52, 53]).

On the other hand, the thermodynamic properties and evolution of black holes can be described not only by the Hawking temperature and the corresponding entropy but also by their phase structures and critical phenomena [54–71, 74–83]. To our knowledge, the study of the thermodynamic phase transition of the black hole started with Hawking and Page, who pointed out the existence of a thermodynamic phase transition (known as “Hawking-Page phase transition”) in the asymptotically anti-de Sitter (AdS) SC black hole when its temperature reaches a certain value. This pioneering work demonstrates the deeper-seated relation between confinement and deconfinement phase transition of the gauge field in the AdS/CFT correspondence [56]. Moreover, this correspondence can be used to investigate the behavior of various condensed matter phenomena [57, 58]. Therefore, inspired by the classical theory of Hawking-Page phase transition, similar investigations were extended to a variety of complicated AdS spacetimes [59–66]. Beyond these achievements, people are also exploring the phase structures and critical phenomena of the non-AdS spacetimes. The biggest obstacle to achieving this is the lack of reflective surfaces (e.g., AdS term), which leads to the thermodynamic instability of non-AdS black holes. To overcome this issue, York suggests placing the non-AdS black holes inside a spherical cavity, so that the boundary of the spherical cavity can ensure the black holes are in a quasi-static thermally stable structure and makes the study of their phase behavior possible. In Ref. [67], York showed that the stable structure of the Schwarzschild black in a spherical cavity is similar to those of the Schwarzschild AdS black hole. Subsequently, the phase behavior of RN black holes in the cavity is shown to have extensive similarity to that of Reissner-Nordström (R-N) AdS black holes in the grand canonical ensemble [68]. In Refs. [69–71], the phase structure and critical phenomena of a class of brane spacetimes in a cavity have been investigated by a similar method. Those results in the existence of Hawking-Page phase transitions in these thermodynamic systems. Moreover, by studying the thermodynamic properties of bosonic stars and hairy black holes in a cavity, it is found that they are very similar to holographic superconductors in AdS gravity [72, 73]. In addition, the author in Ref. [74] found a non-equilibrium second-order phase transition in the charged R-N spacetime. Subsequently, the phenomena of phase transition of the Kerr-Newman black hole were proved by Davies [75]. By using the path-integral formulation of Einstein’s theory, the Gross-Perry-Yaffe (GPY) phase transition, which occurs for a hot flat space decays into the large black hole state [76]. So far, the thermodynamic phase transition and critical phenomena of black holes are still a topic of concern [84–95]. More recently, the quantum gravity corrections to the thermodynamic phase transition and critical behavior of black holes have attracted a lot of attention [96–102]. In particular, when considering the effect of GUP, the modified thermodynamics of black holes in cavities are different from the original case, whereas the corresponding thermodynamic phase transition and critical behavior are similar to those of the AdS black hole [103, 104]. Hence, those results may provide a new perspective on thermodynamic properties and evolution of black holes.

Recently, many works showed that the negative GUP parameters may appear in the nontrivial structures of spacetimes, for example, the discreteness of space [105–108]. This indicates that the spacetimes with negative GUP parameters have different properties from that of positive GUP cases. As we know, the thermodynamic phase transitions and critical behavior of spacetimes are related to their structure. Therefore, it is believed that the negative GUP parameter could lead to many new physical phenomena and results. To this end, the purpose of this study is to explore how the positive/negative GUP effect changes the thermodynamic properties of the SC black hole. However, most investigations pertain to the positive GUP parameters, while the cases of negative GUP parameters case have seen comparatively little development. To this end, we would like to consider this issue and study the local thermodynamic evolution, critical behavior, and phase transition of SC black holes in the framework of GUP with positive/negative parameters, respectively. It turns out that, the positive/negative GUP parameter can change the thermodynamics and phase structure of black holes in varying degrees, which are different from those of the standard cases.

The rest of this article is organized as follows: in Section 2, we investigate the GUP corrected Hawking temperature and the specific heat of the SC black hole in a cavity. Then, the issue of black hole remnants and the corresponding thermodynamic stability are discussed. According to the modified local thermodynamic quantities, the thermodynamic critically and phase transition of SC black hole are analyzed in detail in Section 3. The conclusion and discussion are contained in Section 4. Throughout this article we adopt the convention ℏ = c = k_B = 1.

2 The GUP Corrected Thermodynamic Quantities in a Cavity

In order to detect the thermodynamic evolution and the phase transition of a black hole, one should enclose it in a cavity to keep it in a quasilocal thermally stable [105]. Essentially, the boundary of the cavity acts as a reflecting surface to retain the radiation particles in this thermodynamic ensemble. Now, supposing the radius of the cavity is R and using Eqs 2, 3, the GUP corrected local temperature of the SC black hole for an observer on the cavity can be expressed as follows [105]:

$T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)=\frac{T_H^{\mathrm{G}\mathrm{U}\mathrm{P}}}{\sqrt{f\left(R\right)}}=\frac{M}{4\pi {\beta }_0}{\left(1-\frac{2GM}{R}\right)}^{-\frac{1}{2}}\left(1-\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right),(6)$

$T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)=\frac{T_H^{\mathrm{G}\mathrm{U}\mathrm{P}}}{\sqrt{f\left(R\right)}}=-\frac{M}{4\pi {\beta }_0}{\left(1-\frac{2GM}{R}\right)}^{-\frac{1}{2}}\left(1-\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right),(7)$

The aforementioned equation is implemented by the blue-shifted factor of the metric of the SC black hole. Mathematically, those modifications are not only sensitively dependent on mass M but also the GUP parameters β₀. They respect the original local temperature $T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{o}\mathrm{r}\mathrm{i}\mathrm{g}\mathrm{i}\mathrm{n}\mathrm{a}\mathrm{l}}=\left(c^3\hslash /8\pi GM\right){\left(1-2GM/R\right)}^{-\frac{1}{2}}$ in the limit β₀ = 0. For β₀ > 0, the GUP corrected local temperature $T_{\text{local}}^{\text{GUP\hspace{0.17em}}}$ is physical as far as the mass satisfies $M_{\text{0}}^{\prime }>\sqrt{{\beta }_0/G}=m_p\sqrt{{\beta }_0}$ since $T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\in \mathbb{R}$. This means that, due to the effect of GUP, the black hole terminates evaporating as its mass approaches $M_{\text{0}}^{\prime }$, which leads to a thermodynamically inert remnant with mass $M_{{\beta }_0>0}^{\mathrm{r}\mathrm{e}\mathrm{s}}=M_0^{\prime }$ and temperature is given by

$T_{{\beta }_0>0}^{\mathrm{r}\mathrm{e}\mathrm{s}}=T_{\text{0}}^{\prime }=\frac{m_p}{4\pi \sqrt{{\beta }_0}}\left(1-\sqrt{1-\frac{1}{G{m}_p^2}}\right),(8)$

where m_p represents the Planck length. It should be noted that this kind of remnant is consistent with previous works [109–112]. Hence, one can find that the remnant has zero specific heat (see Eq. 13 for more discussions), which means it does not exchange the energy with the surrounding space. In particular, the behavior of the remnant is more likely an elementary particle. Therefore, the temperature of the remnant can be considered as the energy of the particle [53]. However, if β₀ < 0, the result shows an “unconventional” black hole remnant, which has no rest mass but only pure temperature $T_0^{\prime \prime }=1/\sqrt{4\pi }{\beta }_0$. Despite the remnant with zero rest mass is quite different from those of previous works, it still has been discussed in Refs. [10, 99, 106]. Meanwhile, this remnant is regarded as reasonable when considering the evolution equation of the SC black hole and its sparsity of Hawking radiation. In Ref. [53], the author demonstrates that the black hole cannot evaporate completely in finite time, and the corresponding Hawking radiation becomes extremely sparse. In other words, at the end of evaporation, there indeed exists a metastable, long-lived remnant that approaches zero rest mass asymptotically for β₀ < 0. Moreover, for investigating the possibility of critical behavior, it is necessary to calculate the critical points by considering the radius of the cavity R as an invariable quantity, which satisfies the following conditions

${\left(\frac{\partial T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}}{\partial M}\right)}_R=0,{\left(\frac{{\partial }^2{T}_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}}{\partial M^2}\right)}_R=0.(9)$

In the aforementioned equation, we setting R = 10 and G = 1, the critical values of GUP parameters, the mass of the black hole, and the local temperature are

$\left|{\beta }_{0c}\right|\approx 20.710,M_c^{\mathrm{G}\mathrm{U}\mathrm{P}}\approx 4.615,T_c^{\mathrm{G}\mathrm{U}\mathrm{P}}\approx 0.053.(10)$

It is clear that the critical ratio ${\rho }_c\left(\text{GUP}\right)=M_c\left|{\beta }_{0c}\right|/T_c^{\text{GUP}}\approx 1803.333$ is different from the universal ratio ${\rho }_c\left(\text{RN}-\text{AdS}\right)=P_c{\nu }_c/T_c=3/8$ for the Van der Waals fluid/RN-AdS black hole [59], the ratio ${\rho }_c\left(\text{K}-\text{N}-\text{AdS}\right)=P_c{\nu }_c/T_c=5/12$ for Kerr-Newman-AdS black hole [113], and the ratio ${\rho }_c\left(\text{RainbowGravity}\right)=M_c{\gamma }_c/T_c^{\text{GUP}}\approx 266.478$ for the black hole in the rainbow gravity [98], where P_c, ν_c, and γ_c are the pressure, the specific volume, and the critical values of quantum gravity parameter, respectively.

Since the phase transition would occurs with $0<{\beta }_0<\left|{\beta }_{0c}\right|$, hence, in order to further investigate the relationship between the local temperature and mass for different GUP parameters, for example, β₀ = ±1, we plot Figure 1 by fixing R = 10 and G = 1.

FIGURE 1

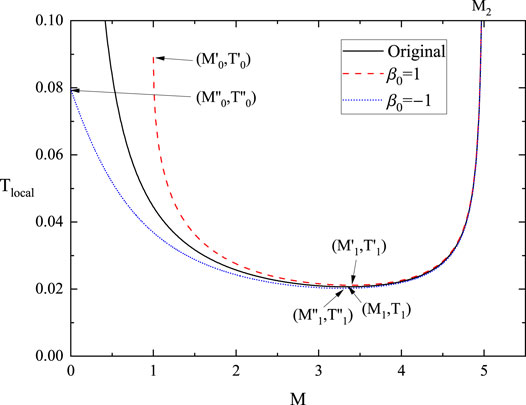
FIGURE 1. The original and GUP corrected local temperature (β₀ = ±1) as a function of mass. We set R = 10 and G = 1.

As seen from Figure 1, the black solid curve corresponds to the original local temperature while the red dashed curve and the blue dotted curve represent the GUP corrected cases with β₀ = 1 and β₀ = −1, respectively. It is obvious that all three kinds of local temperature have the minimum values in the “T_local − M” plane, which can be easy numerically obtained if needed (i. e., $\left(M_1,T_1\right)=(3.333,0.021)$ for the original case; $\left(M_1^{\prime },T_1^{\prime }\right)=(3.384,0.0212)$ for β₀ = 1; $\left(M_1^{\prime \prime },T_1^{\prime \prime }\right)=(3.284,0.020)$ for β₀ = −1). Those lowest points naturally divide the evolution of the black hole into two branches. The right branch represents the early stages of evolution, one can see that the original and the GUP corrected local temperatures follow the same qualitative behavior, which diverge at M₂ = R/2G, and gradually decrease with the decrease of mass before they reach the lowest points. This implies the effect of GUP is negligible at a large scale. The left branches show the final destination of the black hole: the original local temperature diverges as M → 0, which eventually leads to the “information paradox.” However, under the influence of GUP, SC black hole stops radiating particles and leaves the remnant at the end of evolution. For β₀ > 0, the red dashed curve terminates at $M_0^{\prime }=M_{{\beta }_0>0}^{\mathrm{r}\mathrm{e}\mathrm{s}}=1$ with the $T_0^{\prime }=T_{{\beta }_0>0}^{\mathrm{r}\mathrm{e}\mathrm{s}}\approx 0.089$, whereas the remnant with β₀ < 0 has an infinitely small value $M_0^{\prime \prime }=M_{{\beta }_0<0}^{\mathrm{r}\mathrm{e}\mathrm{s}}$ with the temperature $T_0^{\prime \prime }=T_{{\beta }_0<0}^{\mathrm{r}\mathrm{e}\mathrm{s}}\approx 0.282$, as we have discussed earlier.

According to the previous works, one can classify the SC black hole as a large black hole and a small black hole depending on the two branches in Figure 1. For confirming this viewpoint, it is necessary to study the thermodynamic stability of the black hole, which is determined by the heat capacity. First, by using the first law of thermodynamics, the local thermal energy is given by

$E_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)={\int }_{M_0^{\prime }}^M{T}_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}d{S}^{\mathrm{G}\mathrm{U}\mathrm{P}}=\frac{R}{G}\left[\sqrt{1-\frac{2{\left({\beta }_{0}G\right)}^{\frac{1}{2}}}{R}}-\sqrt{1-\frac{2GM}{R}}\right],(11)$

$E_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)={\int }_{M_0^{\prime \prime }}^M{T}_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}d{S}^{\mathrm{G}\mathrm{U}\mathrm{P}}=\frac{R}{G}\left(1-\sqrt{1-\frac{2GM}{R}}\right).(12)$

Note that, due to the mass of remnant of black hole, the lower limit of integration for β₀ > 0 is $M_0^{\prime }=m_p\sqrt{{\beta }_0}$, while it becomes to $M_0^{\prime \prime }=0$ for β₀ < 0. When β₀ → 0, the original local free energy $E_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{o}\mathrm{r}\mathrm{i}\mathrm{g}\mathrm{i}\mathrm{n}\mathrm{a}\mathrm{l}}=R\left(1-\sqrt{1-2GM/r}\right)/G$ is recovered. Next, according to the definition $\mathcal{C}={\left(\partial E_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}/\partial T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}\right)}_r$, the GUP corrected local heat capacity within the boundary r is given by

$C_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)=\frac{4\pi M{\beta }_0\left(R-2GM\right)\sqrt{1-\frac{{\beta }_0}{G{M}^2}}}{{\beta }_0-M\left(R-GM\right)\left(1-\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right)},(13)$

$C_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)=\frac{4\pi M{\beta }_0\left(R-2GM\right)\sqrt{1+\frac{{\beta }_0}{G{M}^2}}}{{\beta }_0+M\left(R-GM\right)\left(1-\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right)}.(14)$

By setting R = 10 and G = 1, the original specific heat and the GUP corrected specific heat as a function of mass for different values of β₀ is reflected in Figure 2.

FIGURE 2

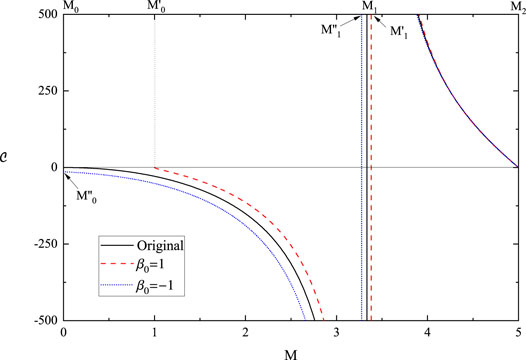
FIGURE 2. The original and GUP corrected local temperature. The original specific heat and GUP corrected specific heat (β₀ = ±1) as a function of mass. We set R = 10 and G = 1.

As seen from Figure 2, the black solid curve for the original heat capacity $C^{\mathrm{o}\mathrm{r}\mathrm{i}\mathrm{g}\mathrm{i}\mathrm{n}\mathrm{a}\mathrm{l}}=8\pi G{M}^2\left(R-2GM\right)/\left(3GM-R\right)$ goes to zero when M = 0, whereas the red dotted curve for ${\mathcal{C}}_{{\beta }_0>0}^{\mathrm{G}\mathrm{U}\mathrm{P}}$ vanishes at $M_0^{\prime }$, resulting in a thermodynamically inert remnant. We are more concerned about what happens when β₀ < 0. When the mass of the black hole is large enough, the blue dotted curve for the modified heat capacity with negative GUP parameter is larger than zero, its behavior is the same as those of β₀ = 0 and β₀ > 0. However, the modified heat capacity approaches a non-zero value as the mass becomes $M_0^{\prime \prime }$, which is caused by the thermal interaction between metastable remnant and the environment [106]. In particular, the similar results can be found in the framework of rainbow gravity (RG) [97, 114], which implies GUP and RG have a deeper connection. Furthermore, all the curves have vertical asymptotes at the locations (i. e., M₁, $M_1^{\prime }$ and $M_1^{\prime \prime }$) where the local temperatures reach the minimum values. Therefore, it implies a second-order phase transition from positive to negative heat capacity at around the vertical asymptote.

Now, armed with the discussions on the modified local temperature and heat capacity, one can classify the SC black hole into two configurations depending on its mass scale, namely, the large black hole (LBH) configuration and the small black hole (SBH) configuration. The stability, the region of the heat capacity, and the region of the mass of small/large black holes with the positive and negative GUP parameters are depicted in Table 1.

TABLE 1

TABLE 1. Stability and region of the mass of the small/large black hole with different values of GUP parameter.

From Table 1, it is found that whether β₀ > 0 or β₀ < 0, the system always has one SBH with positive heat capacity and one LBH with negative heat capacity. Obviously, the stability determines that SBH cannot exist for a long time, it would decay into the remnant or the stable LBH. In this process, some interesting thermodynamic phase transitions that never appeared in the original case can be found by analyzing the Helmholtz free energy of LBH and SBH.

3 Helmholtz Free Energy and Phase Transition

In this section, it is necessary to analyze the corrections to thermodynamic criticality and phase transition due to the GUP. To this aim, one needs to calculate the Helmholtz free energy in an isothermal cavity defined as F_on = E_local − T_localS. According to Eqs 4–12, the GUP corrected Helmholtz free energy is given by:

$F_{\mathrm{o}\mathrm{n}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0>0\right)=\frac{R}{G}\left(\sqrt{1-\frac{2{\left({\beta }_{0}G\right)}^{\frac{1}{2}}}{R}}-\sqrt{1-\frac{2GM}{R}}\right)-\frac{M\mathrm{\Theta }}{2\sqrt{1-\frac{2GM}{R}}},(15)$

$F_{\mathrm{o}\mathrm{n}}^{\mathrm{G}\mathrm{U}\mathrm{P}}\left({\beta }_0<0\right)=\frac{R}{G}\left(1-\sqrt{1-\frac{2GM}{R}}\right)-\frac{M\mathrm{\Xi }}{2\sqrt{1-\frac{2GM}{R}}},(16)$

where $\mathrm{\Theta }=1-\left(1-\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right)\ln \left[M\left(1+\sqrt{1-\frac{{\beta }_0}{G{M}^2}}\right)\right]$ and $\mathrm{\Xi }=1-\left(1-\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right)\ln \left[M\left(1+\sqrt{1+\frac{{\beta }_0}{G{M}^2}}\right)\right]$. In the limit β₀ → 0, this agrees with the original Helmholtz free energy $F_{\mathrm{o}\mathrm{n}}=R\left(1-\sqrt{1-2GM/R}\right)/G-\left(M/2\sqrt{1-2GM/R}\right)$. By fixing R = 10 and G = 1, the original and modified Helmholtz free energy versus their local temperatures are presented in Figures 3–5.

FIGURE 3

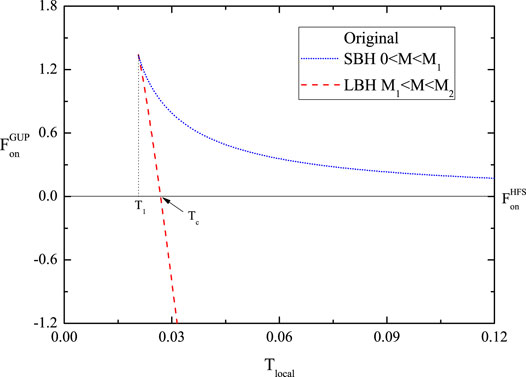
FIGURE 3. The original Helmholtz free energy versus the original local temperature, we take R = 10 and G = 1.

Figure 3 reveals the relationship between the original local free energy and its local temperature. Note that the curve of free energy is not continuous, there is a cusp between the free energies of SBH and LBH, resulting in a second-order phase transition appearing at the critical point T₁ corresponding to the mass M₁. The horizontal line refers to the Helmholtz free energy of hot flat space (HFS) $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{F}\mathrm{S}}=0$ in the Minkowski spacetime without a black hole. Following the viewpoint of York, the SC black hole in an isothermal cavity should reach the thermal equilibrium between the HFS and the black hole through the Hawking-Page phase transition [105]. Hence, one can see a first-order Hawking-Page phase transition at T_c since the Helmholtz free energy of LBH (red dashed curve) intersects the $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{F}\mathrm{S}}$ there. On the contrary, the Helmholtz free energy of SBH tends to but never reaches the line of HFS, so then there is only one Hawking-Page phase transition in the $F_{\mathrm{o}\mathrm{n}}^{\mathrm{G}\mathrm{U}\mathrm{P}}-T_{\mathrm{l}\mathrm{o}\mathrm{c}\mathrm{a}\mathrm{l}}$ plane. In the range of T₁ < T < T_c, the HFS is most probable since $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}$ and $F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$ are higher than $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{F}\mathrm{S}}$. However, the relation of Helmholtz free energy changes to $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{F}\mathrm{S}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$ for T > T_c, which implies that the HFS and the unstable SBH eventually collapses into the stable LBH. Moreover, according to the viewpoints in Ref. [76], it finds that a GPY phase transition occurs for the LBH.

Next, let us focus on Figure 4 and Figure 5 for the modified cases with different GUP parameters, for example, β₀ = ±1. It is worth noting that spacetime is always curved due to the remnant of the black hole. Hence, there is no HFS in the framework of GUP. In the following discussion, we should use the hot curved (HCS) space to replace the HFS. More specifically, one can find that the free energy of HCS $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{C}\mathrm{S}}$ tends to zero when the mass of M = M_res. Hence, the HFS should be replaced by the hot curved space (HCS) in the following discussion. As the counterpart of the original one, the HCS and $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{C}\mathrm{S}}$ effectively influence the phase transition of the SC black hole.

FIGURE 4

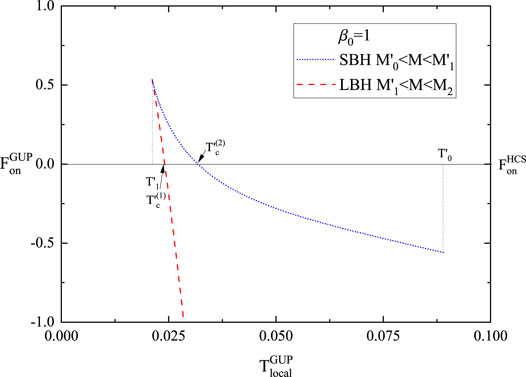
FIGURE 4. The GUP corrected Helmholtz free energy versus the modified temperature for β₀ = 1, we take R = 10 and G = 1.

FIGURE 5

FIGURE 5. The GUP corrected Helmholtz free energy versus the modified temperature for β₀ = −1, we take R = 10 and G = 1.

As seen from Figure 4, when the temperature is lower than $T_c^{\prime \left(2\right)}$, the behavior of the modified Helmholtz free energy is analogous to that in the case of Figure 3, namely, a second-order phase transition point and a Hawking-Page-type phase transition occur at the inflection point $T_1^{\prime }$ and $T_c^{\prime \left(1\right)}$, respectively. The Helmholtz free energy of LBH is smaller than those of SBH and HCS in the range of $T_c^{\prime \left(1\right)}<T<T_c^{\prime \left(2\right)}$, resulting in a GPY phase transition appears for the LBH. However, for $T>T_c^{\prime \left(2\right)}$, due to the effect of GUP with the positive parameter, one can explore some interesting results that different from the original one:

1) An additional Hawking-Page-type phase transition can be found at $T_c^{\prime \left(2\right)}$ since the Helmholtz free energy of SBH intersect with $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{C}\mathrm{S}}$.

2) As long as $T_c^{\prime \left(2\right)}<T<T_0^{\prime }$, the energy of SBH decreases below $F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{C}\mathrm{S}}$, which indicates that the HCS decay into SBH via the GPY phase transition. Therefore, when considering the case β₀ > 0, the GPY phase transition also occurs for SBH.

3) The $F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{H}\mathrm{B}}$ is always higher than the Helmholtz free energy of LBH and the black hole remnant. In this case, the unstable SBH would not only decay into the stable LBH but also into the remnant.

Regarding Figure 5, one can observe that:

1) There is a Hawking-Page-type phase transition from $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}>0$ to $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}<0$ at the inflection point $T_c^{\prime \prime \left(1\right)}$. In addition, one can see a second-order phase transition since far the left end of the blue curve for $F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$ meets the red dashed curve at the cusp temperature $T_1^{\prime \prime }$.

2) The $F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$ cannot reach the intersection point $T_c^{\prime \prime \left(2\right)}$ in a finite time. According to the arguments about the remnant without rest mass in Section 3, it turns out the black hole remnant caused by the negative GUP parameter is metastable and can be trapped in the Hawking-Page-type phase transition for a long time, which is consistent with the analysis of the Hawking temperature in Eq. 9 and the specific heat in Eq. 14.

3) Even more remarkably, the aforementioned results are reminiscent of viewpoints of the black hole in corpuscular gravity (CG), which states that the black holes can be interpreted as a condensate at the critical point of a quantum phase transition [115, 116]. Therefore, along the line of CG theory, the remnant can be interpreted as an additional metastable tiny black hole (TBH) configuration of the system. With this, both the unstable SBH and metastable TBH (or the remnant) would collapse into stable LBH eventually since the relation of free energies obey $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{T}\mathrm{B}\mathrm{H}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$.

4) The Helmholtz free energy of LBH is always lower than those of SBH and HCS for $T>T_c^{\prime \prime \left(1\right)}$, showing the GPY phase transition only appears for the LBH.

4 Conclusion

In this study, we have explored how the GUP with positive/negative parameters affects the local thermodynamic quantities, thermal stability, and phase transitions of SC black holes in a cavity. Our results show that the positive/negative corrections have their own unique properties and are unambiguously distinguished from the original case. For β₀ > 0, the SC black hole leaves a thermodynamically inert remnant with a finite temperature, a finite mass, and a zero local heat capacity. By analyzing the F_on − T_local, it was found two Hawking-Page-type phase transitions and one second-order phase transition, whereas the original case only has one Hawking-Page phase transition. As long as $T_c^{(1)}<T<T_c^{\prime \left(2\right)}$, the free energy obeys $F_{\mathrm{o}\mathrm{n}}^{\mathrm{L}\mathrm{B}\mathrm{H}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}<F_{\mathrm{o}\mathrm{n}}^{\mathrm{H}\mathrm{F}\mathrm{S}}$, which implies that the GPY phase transition does not only occur for the LBH but also for the SBH. Meanwhile, the relation of free energy also shows that unstable SBH collapses into the stable LBH or remnant eventually. However, for β₀ < 0, the remnant becomes metastable, which has a non-zero heat capacity and a finite temperature but a zero rest mass. Furthermore, this thermodynamic ensemble exists one Hawking-Page-type critical point and one second-order phase transition critical point in the range of $T>T_0^{\prime \prime }$ (see Figure 5). Due to the $F_{\mathrm{o}\mathrm{n}}^{\mathrm{S}\mathrm{B}\mathrm{H}}$ is infinitely close to the horizontal line at $T=T_0^{\prime \prime }$, we confirmed that the remnant is metastable and be trapped in the Hawking-Page-type phase transition for a long time. Interestingly, from the CG point of view, the remnant can be interpreted as an additional TBH configuration, which never appears in the original case and the positive correction case. Last, we found the unstable SBH and the metastable remnant eventually collapse into stable the LBH since the free energy of LBH is always higher than those of the SBH and the remnant, so the GPY phase transition only occurs for LBH.

For a long time, people are focused on the GUP with a positive parameter. However, our work shows that the GUP with a negative parameter is as important as the positive one since it can significantly affect the thermodynamics, stability, and phase structures of a black hole. These results can reasonably and consistently describe the thermodynamic evolution of a black hole, and avoid the information paradox. Specifically, the zero mass remnant can be regarded as a candidate of dark matter (see Refs. [53, 117]), which could be found in further astronomical observations. Therefore, it would be very interesting to explore these phenomena in the context of black holes with the negative GUP. Finally, we only focused on how the GUP with both positive and negative parameters affects the thermodynamic properties and phase transition of the SC black hole in this present work. It should be noted that our work can be applied to more generic black holes, such as SC-AdS black holes. The relevant issues will be discussed in detail in our future work.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.

Author Contributions

Z-WF and XZ completed all the derivation and manuscript writing. S-QZ checked the errors in equations.

Funding

This work was supported by the National Natural Science Foundation of China (Grant No. 12105231), the Guiding Local Science and Technology Development Projects by the Central Government of China (Grant No. 2021ZYD0031), and the Sichuan Youth Science and Technology Innovation Research Team (Grant No. 21CXTD0038) the Fundamental Research Funds of China West Normal University (Grant No. 20B009).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. Konishi K, Paffuti G, Provero P. Minimum Physical Length and the Generalized Uncertainty Principle in String Theory. Phys Lett B (1990) 234:276–84. doi:10.1016/0370-2693(90)91927-4

CrossRef Full Text | Google Scholar

2. Maggiore M. The Algebraic Structure of the Generalized Uncertainty Principle. Phys Lett B (1993) 319:83–6. doi:10.1016/0370-2693(93)90785-g

CrossRef Full Text | Google Scholar

3. Garay LJ. Quantum Gravity and Minimum Length. Int J Mod Phys A (1995) 10:145–65. doi:10.1142/s0217751x95000085

CrossRef Full Text | Google Scholar

4. Amelino-Camelia G. Relativity in Spacetimes with Short-Distance Structure Governed by an Observer-independent (Planckian) Length Scale. Int J Mod Phys D (2002) 11:35–59. doi:10.1142/s0218271802001330

CrossRef Full Text | Google Scholar

5. Kempf A, Mangano G, Mann RB. Hilbert Space Representation of the Minimal Length Uncertainty Relation. Phys Rev D (1995) 52:1108–18. doi:10.1103/physrevd.52.1108

CrossRef Full Text | Google Scholar

6. Scardigli F. Generalized Uncertainty Principle in Quantum Gravity from Micro-black Hole Gedanken experiment. Phys Lett B (1999) 452:39–44. doi:10.1016/s0370-2693(99)00167-7

CrossRef Full Text | Google Scholar

7. Adler RJ, Santiago DI. On Gravity and the Uncertainty Principle. Mod Phys Lett A (1999) 14:1371–81. doi:10.1142/s0217732399001462

CrossRef Full Text | Google Scholar

8. Das S, Vagenas EC. Universality of Quantum Gravity Corrections. Phys Rev Lett (2008) 101:221301. doi:10.1103/PhysRevLett.101.221301

PubMed Abstract | CrossRef Full Text | Google Scholar

9. Ghosh S. Quantum Gravity Effects in Geodesic Motion and Predictions of Equivalence Principle Violation. Class Quan Grav. (2014) 31:025025. doi:10.1088/0264-9381/31/2/025025

CrossRef Full Text | Google Scholar

10. Scardigli F, Casadio R. Gravitational Tests of the Generalized Uncertainty Principle. Eur Phys J C (2015) 75:425. doi:10.1140/epjc/s10052-015-3635-y

CrossRef Full Text | Google Scholar

11. Gao D, Zhan M. Phys Rev A (2016) 94:013607. doi:10.1103/physreva.94.013607

CrossRef Full Text

12. Feng Z-W, Yang S-Z, Li H-L, Zu X-T. Constraining the Generalized Uncertainty Principle with the Gravitational Wave Event GW150914. Phys Lett B (2017) 768:81–5. doi:10.1016/j.physletb.2017.02.043

CrossRef Full Text | Google Scholar

13. Kouwn S. Implications of Minimum and Maximum Length Scales in Cosmology. Phys Dark Universe (2018) 21:76–81. doi:10.1016/j.dark.2018.07.001

CrossRef Full Text | Google Scholar

14. Bushev P, Bourhill J, Goryachev M, Kukharchyk N, Ivanov E, Galliou S, et al. Phys Rev D (2019) 100:066020. doi:10.1103/physrevd.100.066020

CrossRef Full Text

15. Giardino S, Salzano V arXiv:2006.01580.

16. Neves JCS. Upper Bound on the GUP Parameter Using the Black Hole Shadow. Eur Phys J C (2020) 80:343. doi:10.1140/epjc/s10052-020-7913-y

CrossRef Full Text | Google Scholar

17. Ökcü Ö, Aydiner E. Observational Tests of the Generalized Uncertainty Principle: Shapiro Time Delay, Gravitational Redshift, and Geodetic Precession. Nucl Phys B (2021) 964:115324. doi:10.1016/j.nuclphysb.2021.115324

CrossRef Full Text | Google Scholar

18. Marin F, Marino F, Bonaldi M, Cerdonio M, Conti L, Falferi P, et al. Gravitational Bar Detectors Set Limits to Planck-Scale Physics on Macroscopic Variables. Nat Phys (2013) 9:71–3. doi:10.1038/nphys2503

CrossRef Full Text | Google Scholar

19. Salah M, Hammad F, Faizal M, Ali AF. Non-singular and Cyclic Universe from the Modified GUP. J Cosmol Astropart Phys (2017) 2017:035. doi:10.1088/1475-7516/2017/02/035

CrossRef Full Text | Google Scholar

20. Scardigli F, Lambiase G, Vagenas EC. GUP Parameter from Quantum Corrections to the Newtonian Potential. Phys Lett B (2017) 767:242–6. doi:10.1016/j.physletb.2017.01.054

CrossRef Full Text | Google Scholar

21. Buoninfante L, Luciano GG, Petruzziello L. Generalized Uncertainty Principle and Corpuscular Gravity. Eur Phys J C (2019) 79:663. doi:10.1140/epjc/s10052-019-7164-y

CrossRef Full Text | Google Scholar

22. Moradpour H, Ziaie AH, Ghaffari S, Feleppa F. The Generalized and Extended Uncertainty Principles and Their Implications on the Jeans Mass. Mon Not R Astron Soc (2019) 488:L69–L74. doi:10.1093/mnrasl/slz098

CrossRef Full Text | Google Scholar

23. Casadio R, Scardigli F. Generalized Uncertainty Principle, Classical Mechanics, and General Relativity. Phys Lett B (2020) 807:135558. doi:10.1016/j.physletb.2020.135558

CrossRef Full Text | Google Scholar

24. Chen P, Ong YC, Yeom D-H. Black Hole Remnants and the Information Loss Paradox. Phys Rep (2015) 603:1–45. doi:10.1016/j.physrep.2015.10.007

CrossRef Full Text | Google Scholar

25. Sakalli I, Övgün A, Jusufi K. GUP Assisted Hawking Radiation of Rotating Acoustic Black Holes. Astrophys Space Sci (2016) 361:330. doi:10.1007/s10509-016-2922-x

CrossRef Full Text | Google Scholar

26. Feng ZW, Li HL, Zu XT, Yang SZ. Quantum Corrections to the Thermodynamics of Schwarzschild-Tangherlini Black Hole and the Generalized Uncertainty Principle. Eur Phys J C (2016) 76:212. doi:10.1140/epjc/s10052-016-4057-1

CrossRef Full Text | Google Scholar

27. Scardigli F, Blasone M, Luciano G, Casadio R. Modified Unruh Effect from Generalized Uncertainty Principle. Eur Phys J C (2018) 78:728. doi:10.1140/epjc/s10052-018-6209-y

PubMed Abstract | CrossRef Full Text | Google Scholar

28. Li H-L, Feng Z-W, Yang S-Z, Zu X-T. The Remnant and Phase Transition of a Finslerian Black Hole. Eur Phys J C (2018) 78:768. doi:10.1140/epjc/s10052-018-6252-8

CrossRef Full Text | Google Scholar

29. Vagenas EC, Alsaleh SM, Ali AF. GUP Parameter and Black-Hole Temperature. EPL (2018) 120:40001. doi:10.1209/0295-5075/120/40001

CrossRef Full Text | Google Scholar

30. Kanzi S, Sakallı İ. GUP Modified Hawking Radiation in Bumblebee Gravity. Nucl Phys B (2019) 946:114703. doi:10.1016/j.nuclphysb.2019.114703

CrossRef Full Text | Google Scholar

31. Barman S. The Hawking Effect and the Bounds on Greybody Factor for Higher Dimensional Schwarzschild Black Holes. Eur Phys J C (2020) 80:50. doi:10.1140/epjc/s10052-020-7613-7

CrossRef Full Text | Google Scholar

32. Hassanabadi H, Maghsoodi E, Chung WS. Analysis of Black Hole Thermodynamics with a New Higher Order Generalized Uncertainty Principle. Eur Phys J C (2019) 79:358. doi:10.1140/epjc/s10052-019-6871-8

CrossRef Full Text | Google Scholar

33. Iorio A, Lambiase G, Pais P, Scardigli F. Generalized Uncertainty Principle in Three-Dimensional Gravity and the BTZ Black Hole. Phys Rev D (2020) 101:105002. doi:10.1103/PhysRevD.101.105002

CrossRef Full Text | Google Scholar

34. Blanchette K, Das S, Rastgoo S. Effective GUP-Modified Raychaudhuri Equation and Black Hole Singularity: Four Models. J High Energ Phys. (2021) 2021:62. doi:10.1007/JHEP09(2021)062

CrossRef Full Text | Google Scholar

35. Kanzi S, Sakallı İ. GUP-modified Hawking Radiation and Transmission/reflection Coefficients of Rotating Polytropic Black Hole. Eur Phys J Plus (2022) 137:14. doi:10.1140/epjp/s13360-021-02245-7

CrossRef Full Text | Google Scholar

36. Sakallı İ, Kanzi S. Physical Properties of Brane-World Black Hole Solutions via a Confining Potential. Ann Phys (2022) 439:168803. doi:10.1016/j.aop.2022.168803

CrossRef Full Text | Google Scholar

37. Chen Y, Li H-L arXiv:2001.11193.

38. Ma M-S, Liu Y-S arXiv:1810.06955.

39. Zhu T, Ren J-R, Li M-F. Influence of Generalized and Extended Uncertainty Principle on Thermodynamics of FRW Universe. Phys Lett B (2009) 674:204–9. doi:10.1016/j.physletb.2009.03.020

CrossRef Full Text | Google Scholar

40. Chemissany W, Das S, Ali AF, Vagenas EC. Effect of the Generalized Uncertainty Principle on post-inflation Preheating. J Cosmol Astropart Phys (2011) 2011:017. doi:10.1088/1475-7516/2011/12/017

CrossRef Full Text | Google Scholar

41. Zeynali K, Darabi F, Motavalli H. Multi-dimensional Cosmology and GUP. J Cosmol Astropart Phys (2012) 2012:033. doi:10.1088/1475-7516/2012/12/033

CrossRef Full Text | Google Scholar

42. Atazadeh K, Darabi F. Einstein Static Universe from GUP. Phys Dark Universe (2017) 16:87–93. doi:10.1016/j.dark.2017.04.008

CrossRef Full Text | Google Scholar

43. DasMann SRB, Mann RB. Planck Scale Effects on Some Low Energy Quantum Phenomena. Phys Lett B (2011) 704:596–9. doi:10.1016/j.physletb.2011.09.056

CrossRef Full Text | Google Scholar

44. Verma H, Mitra T, Prasad Mandal B. Schwinger's Model of Angular Momentum with GUP. Epl (2018) 123:30009. doi:10.1209/0295-5075/123/30009

CrossRef Full Text | Google Scholar

45. Vagenas EC, Alasfar L, Alsaleh SM, Ali AF. The GUP and Quantum Raychaudhuri Equation. Nucl Phys B (2018) 931:72–8. doi:10.1016/j.nuclphysb.2018.04.004

CrossRef Full Text | Google Scholar

46. Park D, Jung E. Phys Rev D (2020) 101:066007. doi:10.1103/physrevd.101.066007

CrossRef Full Text

47. Jizba P, Kleinert H, Scardigli F. Phys Rev D (2010) 81:084030. doi:10.1103/physrevd.81.084030

CrossRef Full Text

48. Carr BJ, Mureika J, Nicolini P. J High Energ Phys. (2015) 07:052.

49. Chatterjee A, Ghosh A. Phys Rev Lett (2020) 125:041302. doi:10.1103/physrevlett.125.041302

PubMed Abstract | CrossRef Full Text

50. Moussa M. Adv High Energ Phys. (2015) 2015:343284. doi:10.1155/2015/343284

CrossRef Full Text

51. Rashidi R. Generalized Uncertainty Principle and the Maximum Mass of Ideal white Dwarfs. Ann Phys (2016) 374:434–43. doi:10.1016/j.aop.2016.09.005

CrossRef Full Text | Google Scholar

52. Ong YC. Generalized Uncertainty Principle, Black Holes, and white Dwarfs: a Tale of Two Infinities. J Cosmol Astropart Phys (2018) 2018:015. doi:10.1088/1475-7516/2018/09/015

CrossRef Full Text | Google Scholar

53. Ong YC. An Effective Black Hole Remnant via Infinite Evaporation Time Due to Generalized Uncertainty Principle. J High Energ Phys (2018) 2018:195. doi:10.1007/jhep10(2018)195

CrossRef Full Text | Google Scholar

54. Kastor D, Ray S, Traschen J. Enthalpy and the Mechanics of AdS Black Holes. Class Quan Grav. (2009) 26:195011. doi:10.1088/0264-9381/26/19/195011

CrossRef Full Text | Google Scholar

55. Kastor D, Ray S, Traschen J. Mass and Free Energy of Lovelock Black Holes. Class Quan Grav. (2011) 28:195022. doi:10.1088/0264-9381/28/19/195022

CrossRef Full Text | Google Scholar

56. Hawking SW, Page DN. Thermodynamics of Black Holes in Anti-de Sitter Space. Commun.Math Phys (1983) 87:577–88. doi:10.1007/BF01208266

CrossRef Full Text | Google Scholar

57. Hartnoll SA. Lectures on Holographic Methods for Condensed Matter Physics. Class Quan Grav. (2009) 26:224002. doi:10.1088/0264-9381/26/22/224002

CrossRef Full Text | Google Scholar

58. McGreevy J. Adv High Energ Phys. (2010) 2010:723105. doi:10.1155/2010/723105

CrossRef Full Text

59. Kubizňák D, Mann RB. J High Energ Phys. (2012) 07:033.

60. Wei S-W, Liu Y-X. Insight into the Microscopic Structure of an AdS Black Hole from a Thermodynamical Phase Transition. Phys Rev Lett (2015) 115:111302. doi:10.1103/physrevlett.115.111302

PubMed Abstract | CrossRef Full Text | Google Scholar

61. Hendi SH, Mann RB, Panahiyan S, Panah BE. Phys Rev D (2017) 95:021501. doi:10.1103/physrevd.95.021501

CrossRef Full Text

62. Ma M-S, Wang R-H. Phys Rev D (2017) 96:024052. arXiv:1707.09156.

63. Dehyadegari A, Sheykhi A. Phys Rev D (2017) 98:024011. arXiv:1711.01151.

64. Wei S-W, Liu Y-X. Extended Thermodynamics and Microstructures of Four-Dimensional Charged Gauss-Bonnet Black Hole in AdS Space. Phys Rev D (2020) 101:104018. doi:10.1103/physrevd.101.104018

CrossRef Full Text | Google Scholar

65. Rostami M, Sadeghi J, Miraboutalebi S. The Static Black Hole in F(R) Gravity with thermal Corrections and Phase Transition. Phys Dark Universe (2020) 29:100590. doi:10.1016/j.dark.2020.100590

CrossRef Full Text | Google Scholar

66. Rostamia M, Sadeghibac J, Miraboutalebi S. Phys Rev D (2020) 101:044001. doi:10.1103/PhysRevD.101.044001

CrossRef Full Text

67. York JW. Black-hole Thermodynamics and the Euclidean Einstein Action. Phys Rev D (1986) 33:2092–9. doi:10.1103/PhysRevD.33.2092

PubMed Abstract | CrossRef Full Text | Google Scholar

68. Braden HW, Brown JD, Whiting BF, York JW. Charged Black Hole in a Grand Canonical Ensemble. Phys Rev D (1990) 42:3376–85. doi:10.1103/PhysRevD.42.3376

CrossRef Full Text | Google Scholar

69. Lu JX, Roy S, Xiao Z. Phase Transitions and Critical Behavior of Black Branes in Canonical Ensemble. J High Energ Phys. (2011) 2011:133. doi:10.1007/jhep01(2011)133

CrossRef Full Text | Google Scholar

70. Lu JX, Wei R. J High Energ Phys (2013) 2013:134. doi:10.1007/jhep04(2013)100

CrossRef Full Text

71. Zhou D, Xiao Z. J High Energ Phys. (2015) 2015:134. doi:10.1088/0264-381/6/12/018

CrossRef Full Text

72. Peng Y. Scalar Field Configurations Supported by Charged Compact Reflecting Stars in a Curved Spacetime. Phys Lett B (2018) 780:144–8. doi:10.1016/j.physletb.2018.02.068

CrossRef Full Text | Google Scholar

73. Kiczek B, Rogatko M. Phys Rev D (2020) 101:084035. doi:10.1103/physrevd.101.084035

CrossRef Full Text

74. Pavón D. Phys Rev D (1991) 43:2495. doi:10.1088/0264-381/6/12/018

CrossRef Full Text

75. Davies PCW. Thermodynamic phase transitions of Kerr-Newman black holes in de Sitter space. Class Quan Grav. (1989) 6:1909–14. doi:10.1088/0264-9381/6/12/018

CrossRef Full Text | Google Scholar

76. Gross DJ, Perry MJ, Yaffe LG. Instability of Flat Space at Finite Temperature. Phys Rev D (1982) 25:330–55. doi:10.1103/physrevd.25.330

CrossRef Full Text | Google Scholar

77. Mandal R, Bhattacharyya S, Gangopadhyay S. Rainbow Black Hole Thermodynamics and the Generalized Uncertainty Principle. Gen Relativ Gravit (2018) 50:143. doi:10.1007/s10714-018-2468-z

CrossRef Full Text | Google Scholar

78. Gangopadhyay S, Dutta A. Adv High Energ Phys. (2018) 2018:7450607. doi:10.1155/2018/7450607

CrossRef Full Text

79. Kumar N, Bhattacharyya S, Gangopadhyay S. Phase Transitions in Born-Infeld AdS Black Holes in D-Dimensions. Gen Relativ Gravit (2020) 52:20. doi:10.1007/s10714-020-02674-2

CrossRef Full Text | Google Scholar

80. Haroon S, Hennigar RA, Mann RB, Simovic F. Phys Rev D (2020) 101:084051. doi:10.1103/physrevd.101.084051

CrossRef Full Text

81. Promsiri C, Hirunsirisawat E, Liewrian W. Phys Rev D (2021) 104:064004. doi:10.1103/physrevd.104.064004

CrossRef Full Text

82. Dehyadegari A, Sheykhi A. Thermodynamic Geometry and Phase Transition of Spinning AdS Black Holes. Phys Rev D (2021) 104:104066. doi:10.1103/physrevd.104.104066

CrossRef Full Text | Google Scholar

83. Liu Y, Lyu H-D, Raju A. Black Hole Singularities across Phase Transitions. J High Energ Phys. (2021) 2021:140. doi:10.1007/jhep10(2021)140

CrossRef Full Text | Google Scholar

84. Carlip S, VaidyaClass S. Quan Gravity (2001) 20:3827. arXiv:gr-qc/0306054.

85. Lundgren AP. Phys Rev D (2008) 77:044014. doi:10.1103/physrevd.77.044014

CrossRef Full Text

86. Dolan SR, Ponglertsakul S, Winstanley E. Stability of Black Holes in Einstein-Charged Scalar Field Theory in a Cavity. Phys Rev D (2015) 92:124047. doi:10.1103/physrevd.92.124047

CrossRef Full Text | Google Scholar

87. Ponglertsakul S, Winstanley E, Dolan SR. Phys Rev D (2016) 94:024031. doi:10.1103/physrevd.94.024031

CrossRef Full Text

88. Sanchis-Gual N, Degollado JC, Herdeiro C, Font JA, Montero PJ. Phys Rev D (2016) 94:044061. doi:10.1103/physrevd.94.044061

CrossRef Full Text

89. Wang P, Yang H, Ying S. Phys Rev D (2020) 101:064045. doi:10.1103/physrevd.101.064045

CrossRef Full Text

90. Wang P, Wu H, Yang H. Thermodynamics and Phase Transition of a Nonlinear Electrodynamics Black Hole in a Cavity. J High Energ Phys. (2019) 2019:2. doi:10.1007/jhep07(2019)002

CrossRef Full Text | Google Scholar

91. Wang P, Wu H, Yang H, Yao F. Extended Phase Space Thermodynamics for Black Holes in a Cavity. J High Energ Phys. (2020) 2020:154. doi:10.1007/jhep09(2020)154

CrossRef Full Text | Google Scholar

92. Simovic F, Mann RB. Critical phenomena of charged de Sitter black holes in cavities. Class Quan Grav. (2019) 36:014002. doi:10.1088/1361-6382/aaf445

CrossRef Full Text | Google Scholar

93. Haroon S, Hennigar RA, Mann RB, Simovic F. Phys Rev D (2020) 101:084051. doi:10.1103/physrevd.101.084051

CrossRef Full Text

94. Wei S-W, Liu Y-X, Mann RB. Ruppeiner Geometry, Phase Transitions, and the Microstructure of Charged AdS Black Holes. Phys Rev D (2019) 100:124033. doi:10.1103/physrevd.100.124033

CrossRef Full Text | Google Scholar

95. Simovic F, Mann RB. Critical phenomena of charged de Sitter black holes in cavities. Class Quan Grav. (2019) 36:014002. doi:10.1088/1361-6382/aaf445

CrossRef Full Text | Google Scholar

96. Gim Y, Kim W. Thermodynamic Phase Transition in the Rainbow Schwarzschild Black Hole. J Cosmol Astropart Phys (2014) 2014:003. doi:10.1088/1475-7516/2014/10/003

CrossRef Full Text | Google Scholar

97. Gim Y, Kim W. Black Hole Complementarity in Gravity's Rainbow. J Cosmol Astropart Phys (2015) 2015:002. doi:10.1088/1475-7516/2015/05/002

CrossRef Full Text | Google Scholar

98. Feng Z-W, Yang S-Z. Thermodynamic Phase Transition of a Black Hole in Rainbow Gravity. Phys Lett B (2017) 772:737–42. doi:10.1016/j.physletb.2017.07.057

CrossRef Full Text | Google Scholar

99. Kim Y-W, Kim SK, Park Y-J. Thermodynamic Stability of Modified Schwarzschild-AdS Black Hole in Rainbow Gravity. Eur Phys J C (2016) 76:557. doi:10.1140/epjc/s10052-016-4393-1

CrossRef Full Text | Google Scholar

100. Upadhyay S, Hendi SH, Panahiyan S, Panah BE. Prog Theor Exp Phys (2018) 2018:093E01. doi:10.1093/ptep/pty093

CrossRef Full Text

101. Shahjalal M. Phys Lett B (2018) 784:6. doi:10.1016/j.physletb.2018.07.032

CrossRef Full Text

102. Feng Z-W, Tang D-L, Feng D-D, Yang S-Z. The Thermodynamics and Phase Transition of a Rainbow Black Hole. Mod Phys Lett A (2020) 35:2050010. doi:10.1142/s0217732320500108

CrossRef Full Text | Google Scholar

103. Ma M-S, Liu Y-S. Adv High Energ Phys. (2018) 2018:1257631. doi:10.1155/2018/1257631

CrossRef Full Text

104. Chen Y, Li H-L arXiv:2001.11193.

105. Jizba P, Kleinert H, Scardigli F. Phys Rev D (2010) 81:084030. doi:10.1103/physrevd.81.084030

CrossRef Full Text

106. Kanazawa T, Lambiase G, Vilasi G, Yoshioka A. Noncommutative Schwarzschild Geometry and Generalized Uncertainty Principle. Eur Phys J C (2019) 79:95. doi:10.1140/epjc/s10052-019-6610-1

CrossRef Full Text | Google Scholar

107. Nenmeli V, Shankaranarayanan S, Todorinov V, Das S. Maximal Momentum GUP Leads to Quadratic Gravity. Phys Lett B (2021) 821:136621. doi:10.1016/j.physletb.2021.136621

CrossRef Full Text | Google Scholar

108. Das S, Fridman M, Lambiase G, Vagenas EC. Phys Lett B (2021) 824:136841. arXiv:2107.02077.

109. Adler RJ, Chen P, Santiago DI. The Generalized Uncertainty Principle and Black Hole Remnants. Gen Relativity Gravitation (2001) 33:2101–8. doi:10.1023/a:1015281430411

CrossRef Full Text | Google Scholar

110. Chen DY, Wu HW, Yang HT. Adv. High Energy Phys. (2013) 2013:432412. doi:10.1155/2013/432412

CrossRef Full Text

111. Chen DY, Jiang QQ, Wang P, Yang H. J. High Energy Phys. (2012) 2013:176. doi:10.1007/JHEP11(2013)176

CrossRef Full Text

112. Feng Z, Zhang L, Zu X. The remnants in Reissner-Nordström-de Sitter quintessence black hole. Mod. Phys. Lett. A (2014) 29:1450123. doi:10.1142/S0217732314501235

CrossRef Full Text | Google Scholar

113. Gunasekaran S, Kubizňák D, Mann RB. Extended Phase Space Thermodynamics for Charged and Rotating Black Holes and Born-Infeld Vacuum Polarization. J High Energ Phys (2012) 2012:110. doi:10.1007/jhep11(2012)110

CrossRef Full Text | Google Scholar

114. Kim Y-W, Kim SK, Park Y-J. Thermodynamic Stability of Modified Schwarzschild-AdS Black Hole in Rainbow Gravity. Eur Phys J C (2016) 76:557. doi:10.1140/epjc/s10052-016-4393-1

CrossRef Full Text | Google Scholar

115. Casadio R, Orlandi A. J High Energ Phys. (2013) 1308:025. arXiv:1302.7138.

116. Casadio R, Giugno A, Micu O, Orlandi A. Phys Rev D (2014) 90:084040. doi:10.1103/physrevd.90.084040

CrossRef Full Text

117. Nozari K, Hamid Mehdipour S. Gravitational Uncertainty and Black Hole Remnants. Mod Phys Lett A (2005) 20:2937–48. doi:10.1142/s0217732305018050

CrossRef Full Text | Google Scholar