
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Phys. , 04 March 2022
Sec. Condensed Matter Physics
Volume 10 - 2022 | https://doi.org/10.3389/fphy.2022.851322
This article is part of the Research Topic Spintronic Materials and Devices View all 6 articles
Two-dimensional generalized XY spin model on a triangular lattice is studied by means of Monte-Carlo simulations. The critical temperatures of Berezinskii-Kosterlitz-Thouless (BKT) phase transition are obtained by the method of helicity modulus. It is found that the results are consistent with those obtained by other methods. The vortex density and the vortex-antivortex pair formation energy are also obtained. The result shows that the critical temperature decreases with the increase of the generalization parameter
The critical phase transition behavior of two-dimensional spin models has been the subject of intense study in recent years. The two-dimensional (2D) XY spin model is one of the most intensively studied due to it’s a paradigmatic example of phase transitions mediated by topological defects [1–4]. It is well known that there is a Berezinskii-Kosterlitz-Thouless (BKT) phase transition in the XY spin model. The BKT phase transition is caused by the unbinding of vortex-antivortex pairs [3, 4]. The XY model has a rich variety of applications in condensed matter physics and statistical physics. For example, it can be used to describe magnetic films with planar anisotropy, two-dimensional solids, thin-film super fluids or superconductors [5, 6].
Besides the two-dimensional XY model, transitions of the BKT type exist in other models such as the classical Heisenberg antiferromagnet model [7], spin model of Long-Range ferromagnetic interactions [8], the ice-type F model [9], 2D spin models with nonmagnetic impurities [10–12] and even spin model with anisotropic interaction [13]. Thus a thorough and quantitative understanding of XY model is very important for the expansion of theoretical physics knowledge. Recently, a generalized XY model has been proposed and attracted a lot of interest [14]. The generalized XY model Hamiltonian is described by
where spin vector
A hybrid MC approach that includes Wolff cluster [19] and Metropolis single spin updates [20] has been used to calculate some thermodynamic quantities for the model defined by Eq. 1. The initial spin configuration is constructed by randomly assigning a spin value to each lattice point. The simulations were performed on a triangular lattice with periodic boundary conditions for system size
In the process of Monte Carlo simulation, different methods are often used to determine the phase transition temperature (or critical temperature)
where
Here
where C is an undetermined fitting constant. According to finite-size scaling formula of helicity modulus in Eq. 4, the critical temperature can be obtained by fitting the data under different sizes.
Figures 2, 3 show the finite-size scaling plot of helicity modulus for
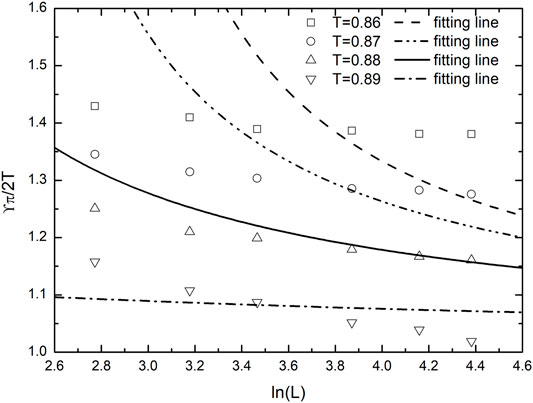
FIGURE 2. Application of the finite-size scaling of
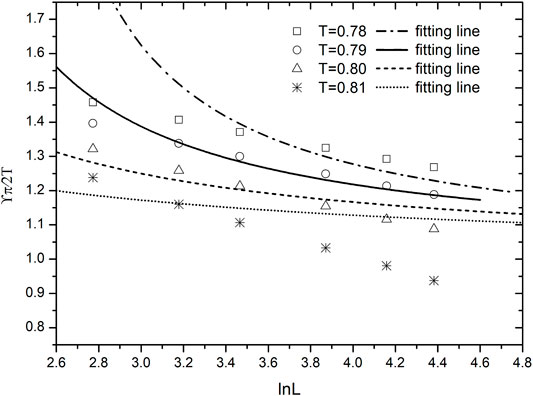
FIGURE 3. Application of the finite-size scaling of
It is well known that the XY model supports topological excitation and shows a BKT phase transition related to the unbinding of vortex–antivortex pairs. However, whenever the BKT transition temperature is reached, the bound pairs appear to dissociate. In the present work, each vortex or antivortex was counted with L = 80. Each vortex core consists of three spins on each unit triangular lattice. When the sum of all spin angles on the unit lattice is equal to
where
There has been great interest in the discussion of the first-order phase transition of the model. The previous work shows that the 2D generalized XY model has both BKT phase transition and first-order phase transition. When
In this paper, with the application of MC simulation, the helicity modulus and vortex density of a 2D generalized XY model on a triangular lattice is discussed. The helicity modulus and vortex density were obtained as a function of temperature for
The original contributions presented in the study are included in the article/supplementary materials, further inquiries can be directed to the corresponding authors.
Y-ZS conceived this idea and provided most of the calculations. QW and QA made the overall modification of the article and assigned some calculation tasks. L-YZ and QC provided their help for the calculation of the vortex part, and X-LY and YZ provided help in algorithm and calculation.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
The authors thank the three reviewers for their constructive comments.
1. Berezinskii VL. Destruction of Long-Range Order in One-Dimensional and Two-Dimensional Systems Possessing a Continuous Symmetry Group. II. Quantum Systems. Sov Phys JEPT (1972) 34:610–6.
2. Kosterlitz JM, Thouless DJ. Ordering, Metastability and Phase Transitions in Two-Dimensional Systems. J Phys C: Solid State Phys (1973) 6:1181–203. doi:10.1088/0022-3719/6/7/010
3. Weber H, Minnhagen P. Monte Carlo Determination of the Critical Temperature for the Two-dimensionalXYmodel. Phys Rev B (1988) 37:5986–9. doi:10.1103/physrevb.37.5986
4. Evertz HG, Landau DP. Critical Dynamics in the Two-Dimensional classicalXYmodel: A Spin-Dynamics Study. Phys Rev B (1996) 54:12302–17. doi:10.1103/PhysRevB.54.12302
6. Chamati H, Romano S, Mól LAS, Pereira AR. Three-dimensional Generalized Xy Models: A Monte Carlo Study. Europhys Lett (2005) 72:62–8. doi:10.1209/epl/i2005-10197-8
7. Romano S. Computer Simulation Evidence for Berezhinskiǐ-kosterlitz-thouless-like Transitions in One Dimension. Int J Mod Phys B (1997) 11:2043–56. doi:10.1142/s0217979297001052
8. Capriotti L, Vaia R, Cuccoli A, Tognetti V. Phase Transitions Induced by Easy-Plane Anisotropy in the Classical Heisenberg Antiferromagnet on a Triangular Lattice: A Monte Carlo Simulation. Phys Rev B (1998) 58:273–81. doi:10.1103/PhysRevB.58.273
9. Weigel M, Janke W. The Square-latticeFmodel Revisited: a Loop-Cluster Update Scaling Study. J Phys A: Math Gen (2005) 38:7067–92. doi:10.1088/0305-4470/38/32/002
10. Wysin GM. Vacancy Effects in an Easy-Plane Heisenberg Model: Reduction of Tc and Doubly Charged Vortices. Phys Rev B (2005) 71:094423. doi:10.1103/PhysRevB.71.094423
11. Sun Y-Z, Yi L, Wysin GM. Berezinskii-Kosterlitz-Thouless Phase Transition for the Dilute Planar Rotator Model on a Triangular Lattice. Phys Rev B (2008) 78:155409. doi:10.1103/PhysRevB.78.155409
12. Paula FM, Pereira AR, Wysin GM. Contribution of Impurity-Pinned Vortices to the Response Function in a Randomly Diluted Easy-Plane Ferromagnet on a Square Lattice. Phys Rev B (2005) 72. doi:10.1103/PhysRevB.72.094425
13. Sun Y-Z, Yi L, Wang J-S. Effects of Dzyaloshinsky-Moriya Interaction on Planar Rotator Model on Triangular Lattice. Commun Comput Phys (2012) 11:1169–81. doi:10.4208/cicp.100410.020211s
14. Romano S, Zagrebnov V. On the Xy Model and its Generalizations. Phys Lett A (2002) 301:402–7. doi:10.1016/s0375-9601(02)01077-0
15. Mól LAS, Pereira AR, Chamati H, Romano S. Monte Carlo Study of 2D Generalized XY-Models. Eur Phys J B (2006) 50:541–8. doi:10.1140/epjb/e2006-00172-3
16. Mól LAS, Pereira AR, Moura-Melo WA. On Phase Transition and Vortex Stability in the Generalized XY Models. Phys Lett A (2003) 319:114–21. doi:10.1016/j.physleta.2003.10.009
17. Van Enter ACD, Romano S, Zagrebnov VA. First-order Transitions for Some Generalized XY Models. J Phys A: Math Gen (2006) 39. doi:10.1088/0305-4470/39/26/l03
18. Sun YZ, Wu Q, Li JY, Chen Q. Thermodynamic Quantities and Phase Transition of a Generalized XY Model on Triangular Lattice. IOP Conf Series: Earth Environ Sci (2018) 128. doi:10.1088/1755-1315/128/1/012106
19. Wolff U. Collective Monte Carlo Updating for Spin Systems. Phys Rev Lett (1989) 62:361–4. doi:10.1103/physrevlett.62.361
20. Metropolis N, Rosenbluth AW, Rosenbluth MN, Teller AH, Teller E. Equation of State Calculations by Fast Computing Machines. J Chem Phys (1953) 21:1087–92. doi:10.1063/1.1699114
21. Sun Y-Z, Liang J-C, Xu S-L, Yi L. Berezinskii-Kosterlitz-Thouless Phase Transition of 2D Dilute Generalized Model. Physica A: Stat Mech its Appl (2010) 389:1391–9. doi:10.1016/j.physa.2009.12.023
22. Olsson P. Two Phase Transitions in the Fully FrustratedXYModel. Phys Rev Lett (1995) 75:2758–61. doi:10.1103/physrevlett.75.2758
23. Lee KW, Lee CE, Kim I-m. Helicity Modulus and Vortex Density in the Two-Dimensional Easy-Plane Heisenberg Model on a Square Lattice. Solid State Commun (2005) 135:95–8. doi:10.1016/j.ssc.2005.03.054
24. Wysin GM, Pereira AR, Marques IA, Leonel SA, Coura PZ. Extinction of the Berezinskii-Kosterlitz-Thouless Phase Transition by Nonmagnetic Disorder in Planar Symmetry Spin Models. Phys Rev B (2005) 72. doi:10.1103/physrevb.72.094418
25. Lee DH, Joannopoulos JD, Negele JW, Landau DP. Symmetry Analysis and Monte Carlo Study of a Frustrated Antiferromagnetic Planar (XY) Model in Two Dimensions. Phys Rev B (1986) 33:450–75. doi:10.1103/physrevb.33.450
26. Gupta R, Baillie CF. Critical Behavior of the Two-dimensionalXYmodel. Phys Rev B (1992) 45:2883–98. doi:10.1103/PhysRevB.45.2883
Keywords: Monte—Carlo method, XY model, vortex density, phase transition, helicity modulus
Citation: Sun Y-Z, Wu Q, Yang X-L, Zhou Y, Zhu L-Y, Chen Q and An Q (2022) Numerical Studies of Vortices and Helicity Modulus in the Two-Dimensional Generalized XY Model. Front. Phys. 10:851322. doi: 10.3389/fphy.2022.851322
Received: 09 January 2022; Accepted: 14 February 2022;
Published: 04 March 2022.
Edited by:
Guoying Gao, Huazhong University of Science and Technology, ChinaReviewed by:
Zhongbing Huang, Hubei University, ChinaCopyright © 2022 Sun, Wu, Yang, Zhou, Zhu, Chen and An. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Qin Wu, bnVlLXFpbnd1QHFxLmNvbQ==; Qing An, NDkwNzU2NzI5QHFxLmNvbQ==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.