Effective Dirac Neutrino Mass Operator in the Standard Model With a Local Abelian Extension

We present 48 types of solutions to the anomaly cancellation conditions of local Abelian extensions of the Standard Model (SM) with right-handed SM-singlet chiral fermions. At least two of them acquire effective light Dirac neutrino masses, while the others get heavy masses from the spontaneous symmetry breaking of the local Abelian symmetry, forming a dark sector with multi-component and multi-generational fermionic dark matter. The corresponding effective Dirac neutrino mass operator can be realized at tree-level or radiatively by introducing extra scalars, and in some cases after imposing extra scotogenic conditions. The Dirac Zee model with Dirac fermionic dark matter is presented as an example of model where the neutrino and dark matter phenomenology are basically independent of each other.

1 Introduction

We can relate fermionic dark matter (DM) with neutrinos in two ways, through the fermions inside the loops in scotogenic models [1] or through anomaly-free gauge extensions of the SM with the extra chiral fermions required to cancel out the anomalies [2]. With Dirac neutrinos, we can have the two ways simultaneously. In fact, regarding neutrinos, the oscillation data is compatible with both Majorana or Dirac neutrino masses. To explain Dirac neutrino masses, right-handed neutrinos have to be introduced. Additionally, an extra local symmetry is also required to guarantee proper total lepton number conservation. Moreover, to have a consistent anomaly-free local symmetry, extra right-handed SM-singlet chiral fields are required in a dark sector which may include dark matter candidates.

In this work we propose a general framework for the systematic study of Dirac neutrino masses in the context of a gauge Abelian symmetry with multi-component dark matter and massive gauge bosons, including the possibility of dark photons.

By using an effective operator approach with up to 10 extra SM-singlet chiral fields, we found a well defined set of 48 types of solutions with effective Dirac neutrino masses, multi-component and multi-generational dark matter, and either a dark photon or a heavy gauge boson mediator.

It is worth noticing that the types of solutions comprise around one thousand specific charge assignments, each one constituting a self-consistent model with its own phenomenology worth to be analysed by itself. In fact, the phenomenology of some of them has been already studied in the literature (see [3, 4] and references therein). Concerning the realization of effective Dirac neutrino mass operator, it can always be realized at least within scalar extensions of each model, like the tree-level Type-II Dirac seesaw [4] or the Dirac Zee model [5], which do not affect the anomaly cancellation conditions at all.

This work is organized as follows. The anomaly conditions for the gauge Abelian symmetry are presented in Section 2, and expressed in terms of the charges of a set of right-handed SM-singlet chiral fields. In Section 3, a subset of them are used as the right-handed neutrino component of the effective Dirac neutrino mass operator. Then, the charge of the complex SM-singlet scalar that breaks spontaneously the gauge Abelian symmetry is fixed. In Section 4 we search for the solutions in which the remaining subset of right-handed SM-singlet chiral fermions obtain heavy masses from the SM-singlet scalar vacuum expectation value. There, they are classified in 48 types of solutions and their main features are highlighted. In Section 5 the solution with the least number of chiral fields is discussed and used as an example of the realization of the effective Dirac neutrino mass operator through SM scalar extensions. Finally, in Section 6 our conclusions are presented.

2 Anomaly Conditions

We consider an extension of the SM with an additional Abelian gauge symmetry and extra right-handed chiral fields singlets under the SM SU(3)_c ⊗ SU(2)_L ⊗ U (1)_Y group. We assume that they do not form vector-like pairs so that all of them are massless before the spontaneous symmetry breaking of the Abelian gauge symmetry. On the other hand, we consider a set of N integers, n₁, n₂, … , n_N, which satisfy the following Diophantine equations

$\sum _{\rho =1}^N{n}_{\rho }=0\text{and}\sum _{\rho =1}^N{n}_{\rho }^3=0.(1)$

In [3] it is shown that.

• The extra gauge Abelian symmetry can be identified as one dark symmetry, U (1)_D, with N_chiral = N right-handed SM-singlet chiral fermions with D-charges n₁, n₂, … n_N.

• The full set of anomaly cancellations conditions for the SM model can be rewritten just as Eq. 1. In fact, if the set of integers in Eq. 1 has one integer, m, repeated three times, the extra gauge Abelian symmetry can be identified as one active symmetry, U (1)_X, with N_chiral = N − 3 right-handed SM-singlet chiral fermions with X-charges $n_1,n_2,\dots n_{N_{\text{chiral}}}$. If the SM right-handed chiral fermions X-charges are denoted with the same name of the field ¹, they can be written in terms of m and a free parameter that we choose to be the X-charge of the conjugate of the SM lepton doublet, L, such that

$u=\frac{4L}{3}-m,d=m-\frac{2L}{3},Q=-\frac{L}{3},e=m-2L,h=L-m,(2)$

where h is the SM Higgs X-charge, and Q is the X-charge of the conjugate of the SM quark doublet. If in addition we choose L such that h = 0, then we obtain the well known U (1)_B−L symmetry, while if we choose L = 0 we obtain a symmetry where the SM fermion doublets are neutral, U (1)_R [6].

We are interested in chiral solutions: the ones in which there is no D or X opposite sign charges for the right-handed chiral fermions. This is motivated by the fact that there are only chiral fermions in the SM.

The simple solution to obtain an active symmetry, U (1)_X, is just to have m = 1 and three right-handed SM-singlet chiral fermions. When h = 0, such that L = 1, this is the well known U (1)_B−L set of N integers satisfying Eq. 1 (−1,−1,−1, 1, 1, 1), and any multiplicative factor of it (−m,−m,−m, m, m, m)². Note that we can make the direct sum of this simple solution with any other solution with N_chiral integers that does not contains m, to obtain an active gauge Abelian symmetry with three extra right handed chiral fermions with X-charges −m [4]. The same procedure can be used to obtain active symmetries for any chiral solution of Eq. 1, by identifying a subset of its integers as m. For example, from the well known solution (1, 1, 1,−4,−4, 5) [7, 8] of Eq. 1, we can build the following extra active solutions with their corresponding m

$\begin{array}{ll}\hfill \left(\mathrm{1,1,1},-4,-\mathrm{4,5}\right)\oplus \left(-m,-m,-m,m,m,m\right)=& \left(\mathrm{1,1,1},-4,-\mathrm{4,5},-m,-m,-m,m,m,m\right),\text{\hspace{0.17em}with}m\ne \pm 1\left(\mathrm{1,1,1},-4,-\mathrm{4,5}\right)\oplus \left(\mathrm{5,5},-5,-5\right)=\left(\mathrm{1,1,1},-4,-\mathrm{4,5,5,5},-\mathrm{5,5}\right),\text{\hspace{0.17em}with}m=5\left(\mathrm{1,1,1},-4,-\mathrm{4,5}\right)\oplus \left(-\mathrm{4,4}\right)=\left(\mathrm{1,1,1},-4,-4,-\mathrm{4,4,5}\right),\text{\hspace{0.17em}with}m=-4.\hfill \end{array}(3)$

Finally, for any given solution, we also have a new solution from the direct sum with itself. For example

$\left(\mathrm{1,1,1},-4,-\mathrm{4,5}\right)\oplus \left(\mathrm{1,1,1},-4,-\mathrm{4,5}\right)=\left(\mathrm{1,1,1,1,1,1},-4,-4,-4,-\mathrm{4,5,5}\right),\text{\hspace{0.17em}with}m=1orm=-4.(4)$

In the next section we will apply phenomenological conditions upon all the solutions with 5 ≤ N ≤ 12 integers obtained with the method explained in [9] and implemented in the python package anomalies [10] ³. The corresponding dataset with 390,074 solutions to Eq. 1, with a maximum absolute value of 30, is in [11]. They are complemented with the direct sums as in Eqs. 3, 4 for each solution while keeping the maximum number of integers less or equal than 12.

3 Effective Dirac Neutrino Mass Operator

In this section, we look for anomaly-free Abelian gauge extensions of the SM, with right-handed SM-singlet chiral fermions, realizing the effective Dirac neutrino mass operator at a given dimension [12, 13]. In the two-component spinor notation, this can be written as

${\mathcal{L}}_{\text{eff}}=h_{\nu }^{\alpha i}{\left({\nu }_{R\alpha }\right)}^{†}{ϵ}_{ab}{L}_i^a{H}^b{\left(\frac{S^{\ast }}{\mathrm{\Lambda }}\right)}^{\delta }+\text{H.c.},\text{with}i=\mathrm{1,2,3},(5)$

and δ = 1, 2, … for dimension d = 4 + δ operators. Here $h_{\nu }^{\alpha i}$ are dimensionless induced couplings with at least a rank-2 matrix structure, ν_Rα are at least two right-handed neutrinos (α = 1, 2, … ) with equal Abelian charge ν extracted from the full set of right-handed SM-singlet chiral fields, L_i are the lepton doublets with Abelian charge −L, H is the SM Higgs doublet with Abelian charge h = L−m, S is the complex SM-singlet scalar responsible for the spontaneous symmetry breaking (SSB) of the anomaly-free gauge symmetry with Abelian charge

$s=-\left(\nu +m\right)/\delta .(6)$

Finally, Λ is a scale of new physics.

In general, after the SSB, several discrete symmetry are left, which may guarantee the stability of at least one potential DM candidate [14]. m ≠ 0 define an active symmetry where the collider restrictions on Z′ are rather strong because its allowed couplings to both light quarks and light leptons, while m = 0 corresponds to a dark symmetry with the corresponding dark photon.

The tree-level realization of the renormalizable operator for Dirac neutrino masses through the Higgs mechanism is obtained when δ = 0, such that ν = −m. Therefore, it is only possible for active symmetries. The simple realization is for the U (1)_B−L solution (−1,−1,−1, 1, 1, 1) with m = 1, ν = −1, and s = 3 to avoid a Majorana mass for ν_R. Therefore, the remnant Z₃ symmetry guarantees the Diracness of the tree-level neutrino masses. However, such a solution imply very tiny Yukawa coupling $h_{\nu }^{\alpha i}\sim \mathcal{O}(10^{-11})$, and therefore we do not consider this kind of solutions here because their lack of explanation for the smallness of neutrino masses.

For δ > 0 we can have tree-level [4] or radiative [3] realizations of the non-renormalizable effective Dirac neutrino mass operator. The specific realization can imply additional phenomenological conditions besides Eq. 6 [3]. However, there are realizations that only involve extra scalars beyond the SM [15] which do not affect the anomaly cancellation conditions. Therefore, any found solution satisfying Eq. 6 can be embedded into a SM scalar extension which realize the effective Dirac neutrino mass operator, like the tree-level Type-II Dirac seesaw [4]. In this way, we can focus into find the self-consistent solutions with at least two repeated charges, ν, which are compatible with the effective Dirac neutrino mass operator (5). This self-consistent solutions are defined such that the only particles beyond the SM are ν_Rα, S and the set of SM-singlet chiral fermions which acquires masses from ⟨S⟩ and that can play the role of (multi-component) dark matter. The solutions up to N = 9 were already found in [4] in the context of type-II Dirac seesaw realizations of the effective Dirac neutrino mass operator for δ = 1. Here, we extend the search of self-consistent solutions in the more general framework of the effective operator up to N = 12 and all the relevant values for δ.

4 Dark Sector With Multi-Component and Multi-Generational Dark Matter

Starting from the extended dataset for the solutions with N integers to the Diophantine Eq. 1, we apply the following steps.

• Check that the solution has two (three) repeated integers to be identified as ν and fix N_ν = 2 (N_ν = 3).

• For δ = 1, 2, … and all the possible combinations for m and ν in the solution, including m = 0, find the s value compatible with the effective Dirac neutrino mass operator of d = 4 + δ according to Eq. 6.

• Interpret the integers in the solution that are different from m and ν as the D-charges for m = 0 or the X-charges for m ≠ 0, of a set of SM-singlet chiral fermions: ψ_i, i = 1, … , N_chiral − N_ν. Then select the solutions for which the condition

$|n_i+n_j|=|s|,(7)$

which guarantees that all the SM-singlet chiral fermions, ψ_i, acquire masses after the spontaneous symmetry breaking of the gauge Abelian symmetry through ⟨S⟩.

We found 1 122 solutions where all the SM-singlet chiral fermions have either one effective mass through the operator in Eq. 5 or from the vacuum expectation value of S after the spontaneous symmetry breaking of either the dark or the active Abelian gauge symmetry. As shown in Figure 1, most of the solutions are for δ = 1 and N = 10.

FIGURE 1

FIGURE 1. Distribution of solutions with N integers to the Diophantine Eq. 1 which allow the effective Dirac neutrino mass operator at d = (4 + δ) for at least two right-handed neutrinos and have non-vanishing Dirac o Majorana masses for the other SM-singlet chiral fermions in the solution.

Concerning the solutions with multi-component DM, we also explore the cases which feature at least two DM candidates with unconditional stability [4, 16]. This happens when the remnant symmetry ${\mathbb{Z}}_{|s|}$ contains two subgroups ${\mathbb{Z}}_p\otimes {\mathbb{Z}}_q$ with p and q coprimes and |s| = pq, which guarantee the stability of each lightest state under ${\mathbb{Z}}_p$ and ${\mathbb{Z}}_q$ respectively. For the two DM candidates associated to the set of chiral fields ψ_i and χ_j, we consider below the following two possibilities for |s| [4, 16].

• ${\mathbb{Z}}_6\cong {\mathbb{Z}}_2\otimes {\mathbb{Z}}_3$: solutions with at least a set of chiral fields with ${\psi }_i\sim \left[{\omega }_6^2\vee {\omega }_6^4\right]$ under ${\mathbb{Z}}_6$, and at least a set of chiral fields with ${\chi }_i\sim {\omega }_6^3$ under ${\mathbb{Z}}_6$,

• ${\mathbb{Z}}_{14}\cong {\mathbb{Z}}_2\otimes {\mathbb{Z}}_7$: solutions with at least a set of chiral fields with ${\psi }_i\sim \left[{\omega }_{14}^2\vee {\omega }_{14}^6\vee {\omega }_{14}^8\vee {\omega }_{14}^{10}\vee {\omega }_{14}^{12}\right]$ under ${\mathbb{Z}}_{14}$ and at least a set of chiral fields with ${\chi }_i\sim {\omega }_{14}^7$ under ${\mathbb{Z}}_{14}$,

where ω_|s| = e^{i 2π/|s|}.

The solutions can be classified in 48 types depending on the dimension of the effective Dirac neutrino mass operator, d = (4 + δ), for δ = 1, 2 values ⁴; the number of Dirac or Majorana massive SM-singlet fermions; the multiplicity of generations for each one of them; and the unconditional stability of at least two of the multi-component dark matter candidates. The most representative solutions (with the lower possible maximum charge) for each type, are presented in Table 1. The column m there, label the solutions of Eq. 1 which are interpreted as one dark symmetry as a solution with m = 0 (absence of m), and the ones with three repeated integers to be interpreted as an active symmetry, as a solution with m ≠ 0 (identified m). Note that the dark symmetry solution in the third row of Table 1 could be also identified as an active symmetry with m = −7.

TABLE 1

TABLE 1. Set of charges satisfying the Diophantine Eq. 1 together with the conditions enumerated in the text, for solutions with N_chiral massive fermions, including the Dirac neutrinos masses allowed by the effective operator of d = 4 + δ, the solution with unconditional stability through ${\mathbb{Z}}_{|s|}$ is highlighted with a bold font for the charge of |s| = 6, 14. N_D and N_M are the number of massive Dirac and Majorana SM-singlet fermions in each solution, while G_D and G_M are the corresponding number of maximum generations in the set.

In this way, in Table 1, the list of N integers in column “Solution” satisfy the Diophantine Eq. 1 for an active symmetry if m ≠ 0 or for a dark symmetry if m = 0 (in such a case the number of new SM-singlet chiral fermions is increased by 3). In each solution there are N_chiral SM-singlet chiral fermions, from which N_ν can develop effective Dirac neutrino masses from the operator in Eq. 5 with d = 4 + δ. The remaining SM-singlet chiral field acquires Dirac or Majorana masses from the Yukawa couplings with the complex SM-singlet scalar, S. Finally, the solutions with unconditional stability are highlighted with a bold font in the column s of Table 1. The last four columns correspond to the number of massive Dirac (Majorana) fermions N_D (N_M) and the maximum number of generations for the Dirac (Majorana) fermions G_D (G_N) in each solution.

There are not types of solutions with a single massive Dirac or Majorana SM-singlet fermion in several generations: N_DM = 1 and G_DM > 1, which would point out to a pure scotogenic realization of the Dirac neutrino mass operator without extra fermionic DM candidates. In fact, in general, most of the solutions feature multi-component dark matter with the lighter of all them protected by at least one remnant discrete symmetry, ${\mathbb{Z}}_{|s|}$. Each DM candidate can be the lighter in a sector with several generations, and for some cases highlighted with a bold s, with at least two of them protected by ${\mathbb{Z}}_p\otimes {\mathbb{Z}}_q$ with p and q coprimes and |s| = pq.

In Figure 2 it is illustrated the variety in which the number of Majorana and Dirac massive SM-singlet fermions appears in the several types of solutions. We can have types of solutions with up to four Majorana and two Dirac fermions for dark Abelian gauge symmetries of d = 5 (magenta discs) and four Dirac fermions and two Majorana ones for dark or active Abelian gauge symmetries of d = 5 (magenta and yellow discs respectively).

FIGURE 2

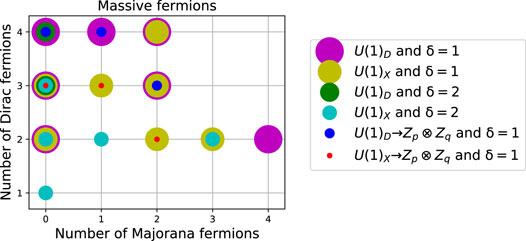
FIGURE 2. Number of massive Dirac and Majorana fermions of the full set of solutions in Figure 1. The discs magenta and green (yellow and cyan) are for dark (active) symmetries which allows the effective Dirac neutrino mass operator for at least two right-handed neutrinos for d = 4 + δ, with δ = 1, two respectively. Similarly, the blue and red points are the type of solution which satisfy the unconditional stability conditions for at least two DM candidates.

Each massive SM-singlet fermion can belong to a set of generations with the same quantum numbers but different masses. In Figure 3 it is shown the way in which each dark matter candidate is grouped in generations for the several types of solutions. From a phenomenological point of view, this is important because this allows to have the rank of the effective neutrino mass matrix sufficiently high in scotogenic realizations of Dirac neutrino mass operator [3]. Moreover, the interplay between several generations can boost the direct and indirect signals of the DM candidate [17]. We can have types of solutions with until four generations of Majorana SM-singlet fermions or with until three generations of Dirac SM-singlet fermions, for dark symmetries of d = 5 (magenta discs in the plot).

FIGURE 3

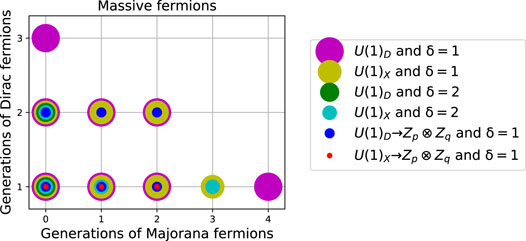
FIGURE 3. Same as Figure 2 but for number generations of massive Dirac and Majorana fermions of the full set of solutions in Figure 1.

Note also that dark symmetries with δ = 1 and unconditional stability, represented as blue dots in Figures 2, 3, are typically associated to a high number of massive SM-singlet Dirac fermions, Figure 2, in sectors of at most two generations of massive Dirac or Majorana SM-singlet fermions, Figure 3. On the other hand, active symmetries with δ = 1 and unconditional stability, represented as red dots in Figures 2, 3, are associated to at least two Dirac SM-singlet fermions and less than three Majorana SM-singlet fermions, Figure 2, without extra generations, Figure 3.

In any scotogenic realization of the effective Dirac neutrino mass operator, a set of the heavy SM-singlet fermions must participate directly in the generation of the light neutrino masses. In this way, the corresponding fermion Yukawa couplings are also constrained by neutrino physics. Extra conditions are required to check if a solution can have a scotogenic realization. In fact, all the solutions up to N = 9 for the one-loop scotogenic realization of the effective Dirac neutrino mass operator at d = 5 and d = 6 were presented in [3]. As an example, the solution in the first row of Table 1 was analyzed there, corresponding to one scotogenic realization of the d = 5 operator at one-loop through a dark symmetry with two independent Dirac fermionic dark matter candidates (magenta disc with coordinates (0, 2) in Figure 2). In general, for each type of solution we can have extra DM sectors which may be independent of the neutrino physics. It is worth noticing that in the absence of scotogenic conditions, the realization of the effective Dirac neutrino mass operator is always possible for SM extensions with only extra scalars at tree-level [4, 18], one-loop [15, 19] and two and three-loops [15]. Therefore, it is well motivated to make a systematic study of multi-component Dirac or Majorana fermionic DM, independent of the specific realization of the effective Dirac neutrino mass operator. Moreover, since the neutrino physics is in general independent of the DM phenomenology, we expect that the General Neutrino Interactions (GNI) can be large and well suited to be explored in neutrino experiments [19]. Additionally, the dark symmetry models contains a dark photon which can be searched in both neutrino and direct detection experiments. Alongside, the active symmetry contains a Z′ with both quark and lepton couplings which have strong dilepton signals at the LHC. It turns out that the Z′ portal also allows us to probe the models with m ≠ 0 through modifications of the cosmological history of the Universe, namely, via additional contributions from the right handed neutrinos to the effective number of relativistic degrees of freedom N_eff, with constraints that are competitive with the ones obtained from colliders [20].

In general, we can see that multi-component and multi-generational DM candidates are the trend for gauge Abelian extensions of the SM with massive SM-singlet chiral fermions compatible with the effective Dirac neutrino mass operator of d = 4 + δ, for δ > 0.

5 Simplest Realization: Dirac Zee Model With Dirac Fermionic DM

As an illustration, consider the type of solution as the one in the second row of Table 1 (3, 3, 3, −5, −5, −7, 8). This features the one with the least N_chiral = 4, as summarized in Table 2. This solution is the simplest of the types of solutions with U (1)_X and d = 6 (δ = 2), represented by the cyan dot with coordinates (0, 1) in Figures 2, 3.

TABLE 2

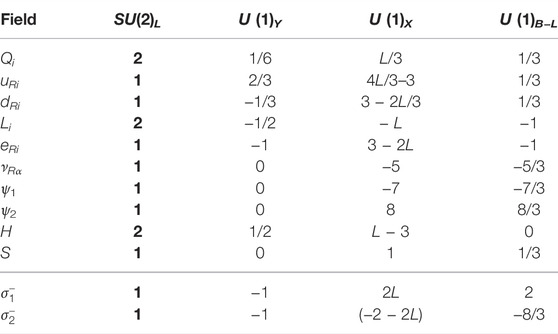
TABLE 2. X and proper B−L normalized charges for the first solution in Table 1 (3, 3, 3, −5, −5, −7, 8), for which m = 3, ν = −5, δ = 2 and therefore from Eq. 6, s = 1. For the column U (1)_B−L we fix L = 3 and change X → X/3. Moreover, i = 1, 2, 3 and α = 1, 2. The electroweak quantum numbers are also shown for each one of SM fields, the extra SM-singlet chiral fermions, and the scalars in the last four rows.

The solution corresponds to an extension of the SM with m = 3 that for h = 0 give rise to a local U (1)_B−L up to a global factor of − 1/3: u = d = −Q = 1 and e = −L = −3. This factor has been included in the column U (1)_B−L of Table 2. The effective Dirac neutrino mass operator of d = 6 is compatible with two light Dirac neutrino masses through the right-handed neutrino, ν_R, with a (B− L)-charge of ν = −5/3. Without further conditions, this can be realized if we include two weak-singlet charged scalars, denoted as ${\sigma }_1^{-}$ and ${\sigma }_2^{-}$ in Table 2. Then, we can have the one-loop realization of the effective Dirac neutrino mass operator at d = 6 [15, 19] illustrated in Figure 4, which features the Dirac Zee model. From the diagram there, we have σ₁ = −2L and σ₂ = e + ν = −2 − 2L, with the corresponding U (1)_B−L charges in Table 2. The neutrino related phenomenology of the model has been analysed in detail in [19], featuring large GNI along with charged lepton flavour violating processes, and strong dilepton signals at the LHC.

FIGURE 4

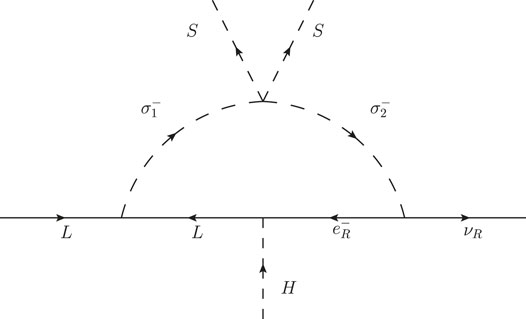
FIGURE 4. Diagram with the X-charge flux of the fields in the one-loop Dirac Zee model which realizes the effective Dirac neutrino mass operator at d = 6

In the new realization of the Dark Zee model presented here, we have the novelty that the model can also have a Dirac fermionic dark matter candidate. In fact, the Lagrangian includes a new Yukawa term

$\mathcal{L}\supset y_D{\psi }_1{\psi }_2{S}^{\ast }+\text{H.c,}(8)$

with two SM-singlet chiral fermions of (B− L)-charges −7/3 and 8/3 respectively. After the spontaneous symmetry breaking of the active gauge symmetry, this give rise to a Dirac heavy fermion mass

${\mathcal{L}}_{\text{DM}}=M_{\mathrm{\Psi }}\bar{\mathrm{\Psi }}\mathrm{\Psi },(9)$

where $\mathrm{\Psi }={\left(\begin{array}{cc}\hfill {\left({\psi }_1\right)}^{†}\hfill & \hfill {\psi }_2\hfill \end{array}\right)}^T$ and $M_{\mathrm{\Psi }}=y_D⟨S⟩/\sqrt{2}$.

Therefore, in contrary to the scotogenic models, the DM phenomenology can be studied independently of the neutrino physics. And therefore, previous analysis of simplified dark matter models with Dirac fermionic dark matter in extensions of the SM with U (1)_B−L, fully apply here. In particular, the annihilation cross section for $\bar{\psi }\psi \to {Z^{\prime }}^{*}\to \bar{f}f$ is given in [21]. As we implemented this model in SARAH [22], we can have the relic density calculation directly from micrOMEGAs [23].

The corresponding spin-independent direct detection cross section of dark matter per nucleon is given by [21, 24].

${\sigma }_{\psi N}=\frac{{\mu }_N^2}{\pi }\frac{g_X^4}{M_{Z^{\prime }}^4}{\left({\psi }_1-{\psi }_2\right)}^2{L}^2,(10)$

where ψ₁ = −7/3, ψ₂ = 8/3 and L = 1, are the U (1)_B−L charges in Table 2, and

${\mu }_N=\frac{M_N{M}_{\mathrm{\Psi }}}{M_N+M_{\mathrm{\Psi }}},(11)$

is the reduced mass.

The cross section can be written as

${\sigma }_{\psi N}^{\text{SI}}\left({\mathrm{c}\mathrm{m}}^2\right)=4.8\times 10^{-47}{\left(\frac{{\mu }_N}{1\mathrm{G}\mathrm{e}\mathrm{V}}\right)}^2{\left(\frac{0.1}{g_X}\right)}^4{\left(\frac{M_{Z^{\prime }}}{4\text{TeV}}\right)}^4{\left({\psi }_1-{\psi }_2\right)}^2{L}^2{\mathrm{c}\mathrm{m}}^2.(12)$

We can illustrate the DM phenomenology for a specific benchmark point which is compatible with collider, ΔN_eff [20] and the current direct detection currents constraints [25]. We choose g_X = 0.1, M_Z’ = 4 TeV, m_S = 5 TeV. For this specific benchmark point, the main constraint arises from the current upper bound on ΔN_eff for which M_Z’ ≳ 40 TeV. This kind of constraint has the potential to probe the full model with the next generation of CMB experiments [20]. From Figure 5 we can see that the proper relic density can be obtained for M_Ψ ≈ 1840 GeV or M_Ψ ≈ 2050 GeV.

FIGURE 5

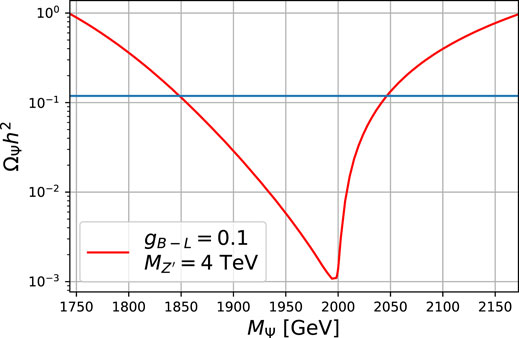
FIGURE 5. Dirac fermionic Dark matter relic density as a function of its mass for U (1)_B−L with copuling g_B−L = g_X = 0.1, M_Z’ = 4 TeV, m_S = 5 TeV.

6 Conclusion

We found around one thousand solutions to the anomaly free conditions of local Abelian extensions of the SM with N integers and N_chiral right handed SM-singlet fermions which include the ones forming the effective set of Dirac neutrinos and a dark sector with massive fermions. We classify the solutions in 48 types in Table 1 depending if the symmetry is dark (N_chiral = N) or active (N_chiral = N − 3), the dimension of the effective Dirac neutrino mass operator (d = 4 + δ), the number of independent dark matter candidates, and the number of generations of each massive fermion. The scalar realizations of the effective Dirac neutrino mass operator feature a set of parameters which explain independently the neutrino oscillations and the phenomenology of a multi-component and multi-generational dark matter sector. This allows for large GNI and charged lepton flavor violation observables while keeping the features of the simplified dark matter models. We have illustrated this separation explicitly with a simple one-loop Dirac Zee model which has a Dirac fermionic dark matter candidate. We could expect enhanced effects in scalar realizations of the effective Dirac neutrino mass operator at two-loops and three-loops [15], which are not yet fully explored in the literature.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author

Author Contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Funding

The work of DR is supported by Sostenibilidad UdeA, and the UdeA/CODI Grants 2017-16286 and 2020-33177.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2022.838531/full#supplementary-material

Footnotes

¹Q and L are the X-charges of the fermion doublets Q^† and L^†, respectively.

²This solutions cannot be interpreted as a dark symmetry because it would contain three pairs of vector-like SM-singlet fermions.

³https://pypi.org/project/anomalies/.

⁴For larger δ there a few other solutions with similar features.

References

1. Ma E. Verifiable Radiative Seesaw Mechanism of Neutrino Mass and Dark Matter. Phys Rev D (2006) 73:077301. arXiv:hep-ph/0601225. doi:10.1103/physrevd.73.077301

CrossRef Full Text | Google Scholar

2. Patra S, Werner R, Yaguna CE. A New B − L Model without Right-Handed Neutrinos. JHEP (2016) 09:076. arXiv:1607.04029 [hep-ph]. doi:10.1007/jhep09(2016)076

CrossRef Full Text | Google Scholar

3. Bernal N, Calle J, Restrepo D. Anomaly-free Abelian Gauge Symmetries with Dirac Scotogenic Models. Phys Rev D (2021) 103:095032. arXiv:2102.06211 [hep-ph]. doi:10.1103/physrevd.103.095032

CrossRef Full Text | Google Scholar

4. Bernal N, Restrepo D. Anomaly-free Abelian Gauge Symmetries with Dirac Seesaws. Eur Phys J C (2021) 81:11. arXiv:2108.05907 [hep-ph].

CrossRef Full Text | Google Scholar

5. Nasri S, Moussa S. Model for Small Neutrino Masses at the TeV Scale. Mod Phys Lett A (2002) 17:771–8. arXiv:hep-ph/0106107. doi:10.1142/s0217732302007119

CrossRef Full Text | Google Scholar

6. Jana S, Vishnu PK, Saad S. Minimal Dirac Neutrino Mass Models from $$\hbox {U}(1)_{\Mathrm{R}}$$ Gauge Symmetry and Left-Right Asymmetry at Colliders. Eur Phys J C (2019) 79:916. arXiv:1904.07407 [hep-ph]. doi:10.1140/epjc/s10052-019-7441-9

CrossRef Full Text | Google Scholar

7. Appelquist T, Dobrescu BA, Hopper AR. Nonexotic Neutral Gauge Bosons. Phys Rev D (2003) 68:035012. arXiv:hep-ph/0212073. doi:10.1103/physrevd.68.035012

CrossRef Full Text | Google Scholar

8. Montero JC, Pleitez V. Gauging U(1) Symmetries and the Number of Right-Handed Neutrinos. Phys Lett B (2009) 675:64–8. arXiv:0706.0473 [hep-ph]. doi:10.1016/j.physletb.2009.03.065

CrossRef Full Text | Google Scholar

9. Costa DB, Dobrescu BA, Fox PJ. General Solution to the U(1) Anomaly Equations. Phys Rev Lett (2019) 123:151601. arXiv:1905.13729 [hep-th]. doi:10.1103/physrevlett.123.151601

PubMed Abstract | CrossRef Full Text | Google Scholar

10. Restrepo D. Restrepo/Anomalies: Zenodo Version (2021). doi:10.5281/zenodo.5526558

CrossRef Full Text | Google Scholar

11. Restrepo D. Set of N Integers between − 30 and 30 with Sum and Cubic Sum up to Zero for 4 < N < 13 (2021). doi:10.5281/zenodo.5526707

CrossRef Full Text | Google Scholar

12. Cleaver G, Cvetič M, Espinosa JR, Everett L, Langacker P. Intermediate Scales, μ Parameter, and Fermion Masses from String Models. Phys Rev D (1998) 57:2701–15. arXiv:hep-ph/9705391. doi:10.1103/physrevd.57.2701

CrossRef Full Text | Google Scholar

13. Gu P-H, He H-J. Neutrino Mass and Baryon Asymmetry from Dirac Seesaw. JCAP (2006) 2006:010. arXiv:hep-ph/0610275 [hep-ph]. doi:10.1088/1475-7516/2006/12/010

CrossRef Full Text | Google Scholar

14. Batell B. Dark Discrete Gauge Symmetries. Phys Rev D (2011) 83:035006. arXiv:1007.0045 [hep-ph]. doi:10.1103/physrevd.83.035006

CrossRef Full Text | Google Scholar

15. Saad S. Simplest Radiative Dirac Neutrino Mass Models. Nucl Phys B (2019) 943:114636. arXiv:1902.07259 [hep-ph]. doi:10.1016/j.nuclphysb.2019.114636

CrossRef Full Text | Google Scholar

16. Yaguna CE, Zapata Ó. Multi-component Scalar Dark Matter from a Z_N Symmetry: a Systematic Analysis. JHEP (2020) 2020:109. arXiv:1911.05515 [hep-ph]. doi:10.1007/jhep03(2020)109

CrossRef Full Text | Google Scholar

17. Herms J, Ibarra A. Production and Signatures of Multi-Flavour Dark Matter Scenarios with T-Channel Mediators. J Cosmology Astroparticle Phys (2021) 2021(10):026. arXiv:2103.10392 [hep-ph]. doi:10.1088/1475-7516/2021/10/026

CrossRef Full Text | Google Scholar

18. Ma E. Linkage of Dirac Neutrinos to Dark U(1) Gauge Symmetry. Phys Lett B (2021) 817:136290. arXiv:2101.12138 [hep-ph]. doi:10.1016/j.physletb.2021.136290

CrossRef Full Text | Google Scholar

19. Calle J, Restrepo D, Zapata Ó. Phenomenology of the Zee Model for Dirac Neutrinos and General Neutrino Interactions. Phys Rev D (2021) 104:015032. arXiv:2103.15328 [hep-ph]. doi:10.1103/physrevd.104.015032

CrossRef Full Text | Google Scholar

20. Calle J, Restrepo D, Zapata Ó. Dirac Neutrino Mass Generation from a Majorana Messenger. Phys Rev D (2020) 101:035004. arXiv:1909.09574 [hep-ph]. doi:10.1103/physrevd.101.035004

CrossRef Full Text | Google Scholar

21. Duerr M, Perez PF, Smirnov J. Simplified Dirac Dark Matter Models and Gamma-Ray Lines. Phys Rev D (2015) 92:083521. arXiv:1506.05107 [hep-ph]. doi:10.1103/physrevd.92.083521

CrossRef Full Text | Google Scholar

22. Staub F. SARAH 4: A Tool for (Not Only SUSY) Model Builders. Comput Phys Commun (2014) 185:1773–90. arXiv:1309.7223 [hep-ph]. doi:10.1016/j.cpc.2014.02.018

CrossRef Full Text | Google Scholar

23. Bélanger G, Boudjema F, Goudelis A, Pukhov A, Zaldívar B. micrOMEGAs5.0 : Freeze-In. Comput Phys Commun (2018) 231:173–86. arXiv:1801.03509 [hep-ph]. doi:10.1016/j.cpc.2018.04.027

CrossRef Full Text | Google Scholar

24. Duerr M, Perez PF. Theory for Baryon Number and Dark Matter at the LHC. Phys Rev D (2015) 91:095001. arXiv:1409.8165 [hep-ph]. doi:10.1103/physrevd.91.095001

CrossRef Full Text | Google Scholar

25. Yue M, Wang Z, Tao Y, Abdukerim A, Bo Z, Chen W, et al. Dark Matter Search Results from the PandaX-4T Commissioning Run. PandaX-4T. Phys Rev Lett (2021) 127(26):261802. arXiv:2107.13438 [hep-ex]. doi:10.1103/PhysRevLett.127.261802

PubMed Abstract | CrossRef Full Text | Google Scholar