Xinmin Fan1,2
Jianxin Zhang1,2,3*Sensen Li4Shun Li1,2Yan Wang1,2Fei Du1,2Xiaodong Huang1,2Yingde Li1Lujun Zhang1,2Chunyan Wang1,2*- 1School of Physics and Electronic Information, Weifang University, Weifang, China
- 2Weifang Key Laboratory of Laser Technology and Application, Weifang University, Weifang, China
- 3Weifang Academy of Advanced Opto-Electronic Circuits, Weifang, China
- 4Science and Technology on Electro-Optical Information Security Control Laboratory, Tianjin, China
A symmetric grating is proposed to obtain higher output power in spectral beam combination by increasing the number of lasers and spectral utilization. The grating allows laser beams to be incident from both sides of the grating normal to achieve coaxial beam combining, so the number of beams and the combined output power are doubled compared with the traditional grating under the same spectral line-width. The grating is designed with the central wavelength of 4.65 μm, and the calculation results show that this grating is very advantageous for spectral beam combining, especially for the light waves in the range 4.55–4.71 μm, where their diffraction efficiencies are high (over 80%) and correspond to a wide and linear range of incidence angles. Meanwhile, based on the symmetric gratings we further propose a circular grating to achieve the same frequency spectral beam combining. This beam combining design will not increase the laser spectral line width while enhancing the laser power, reducing the requirements for the unit laser spectral line width, which is very meaningful in some application fields and will further enrich the research of spectral beam combining.
1 Introduction
The output power of a single laser beam is often difficult to meet scientific and industrial needs due to the gain saturation, non-linear effects and device damage. Combining multiple laser beams is an effective way to obtain higher output power to solve this problem [1]. In 1986, Wilfrid B. Veldkamp of Lincoln Laboratory first proposed a method for coherent beam combining of multi-channel lasers using binary phase gratings and achieved 6 GaAlAs laser beams combination [2, 3]. In 2011, Thales Research and Technology in France reported the coherent beam combining of 5 quantum-cascade lasers by a binary phase or Dammann grating [4]. All of the above solutions are coherent beam combining, which requires a high degree of coherence between different channel lasers and is hard to accomplished experimentally, especially when the number of lasers is large [5–9]. The feasibility of a spectral beam combining (incoherent beam combining) scheme was proposed by Christopher C. Cook et al. at Lincoln Laboratory in 1999 [10] and was experimentally achieved for a semiconductor laser array in 2000, where the combined beam quality was almost identical to that of the single emitter [11], and in the same year, they have also successfully achieved spectral beam combining of 11-channel lasers [12]. Since then, the study of spectral beam combination has attracted extensive attention [13, 14].
Theoretically, the more lasers, the higher the combined beam output power, but increasing the number of lasers will bring many problems, mainly in: 1) difficulty in optical path arrangement, because the existing spectral beam combination schemes require that all emitters (or laser arrays) can only be on the same side of the grating normal; 2) the spectral of each emitter must be broadband, and the more the emitters are, the wider their spectral must be, otherwise the efficiency of the spectral beam combination will be reduced.[15]
To overcome these above problems, we propose a symmetric grating for the spectral beam combining, and also develop a circular grating (two-dimensional grating) to achieve the same frequency spectral beam combining, which greatly enriches the study of spectral beam combining.
2 Novel spectral beam combining scheme
2.1 Symmetric grating and its spectral beam combining scheme
Compared with traditional grating, our symmetric grating has the following features: The diffracted light can be emitted perpendicular to the grating plane if the laser beam is incident on the grating at a suitable angle, so it can also be emitted perpendicular to the grating plane when the laser beam incident at the symmetrical position (or angle) on the other side of the grating normal. This allows multiple laser beams to be incident from both sides of the grating normal to achieve spectral beam combining. Meanwhile, the incident light can only be on one side of the normal of the traditional grating for spectral beam combining. Take the example of the three-channel lasers L1, L2, and L3, the differences between the two spectral combining schemes of the traditional grating and the symmetrical grating are given in Figures 1A,B respectively. DG and SG are the traditional diffraction grating and the symmetric grating respectively, and OC is the coupling output mirror that partially reflects and partially transmits the spectral lines of the unit lasers L1, L2, and L3 so that the three channels lasers are locked to the central wavelengths λ1, λ2 and λ3 respectively and are coaxially combined.
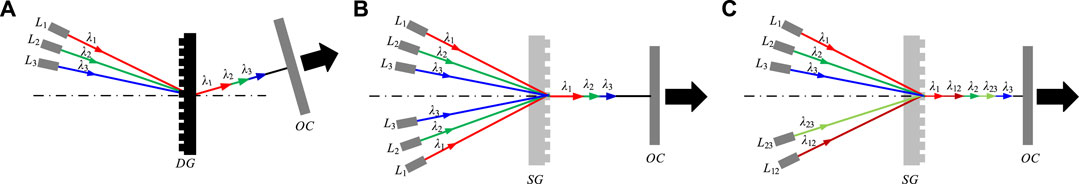
FIGURE 1. Comparison of the two spectral beam combining schemes of (A) the traditional grating and (B) the symmetrical grating. (C) The second spectral beam combination scheme of the symmetric grating.
Compared with traditional gratings, it can be seen that the symmetrical grating has the advantages of a larger number of lasers, higher spectral utilisation, smaller space requirement and the ability to combine beam at the same frequency. As shown in Figure 1C, there is also another spectral beam combining scheme where the positions of the lasers on either side of the grating normal can be asymmetrical compared with Figure 1B, L12 and L23 are located between L1 and L2, L2, and L3 respectively, which their corresponding wavelengths λ12 and λ23 are between λ1 and λ2, λ2, and λ3 respectively.
2.2 Parameter design and the spectral beam combining effect
A theoretical study and parametric design have been carried out for the new grating proposed above. In order to obtain high first-order diffraction efficiency, we have designed a sub-wavelength dielectric grating assuming the central wavelength of 4.56 μm [16]. As shown in Figure 2, the simulation unit is in the black box, and the refractive index of grating material
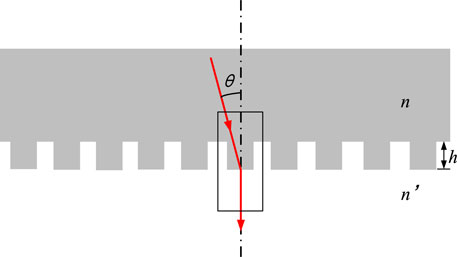
FIGURE 2. Schematic diagram of the ray trace in the grating (the simulation unit is in the black box).
As shown in Figure 3A, calculations show that this grating is ideally suited for spectral beam combining, as it has a high diffraction efficiency (>80%) for the incident light in the range 4.55–4.71 μm, with a maximum diffraction efficiency of 91.4% at 4.66 μm, and a relatively significant difference in the incidence angle of these lights while their diffracted lights are emitted perpendicular to the grating plane. Figure 3B shows the electric field distribution of incident and diffracted light in the grating for the laser beam of 4.6 μm, the purple arrows represent the direction of incident light (33.2°) and the green arrows represent the direction of diffracted light, which is consistent with the initial assumptions and calculations made earlier. Due to the left-right symmetry of the grating structure (see Figure 2), the beam incident from the right side of the grating normal has the same direction of emission.
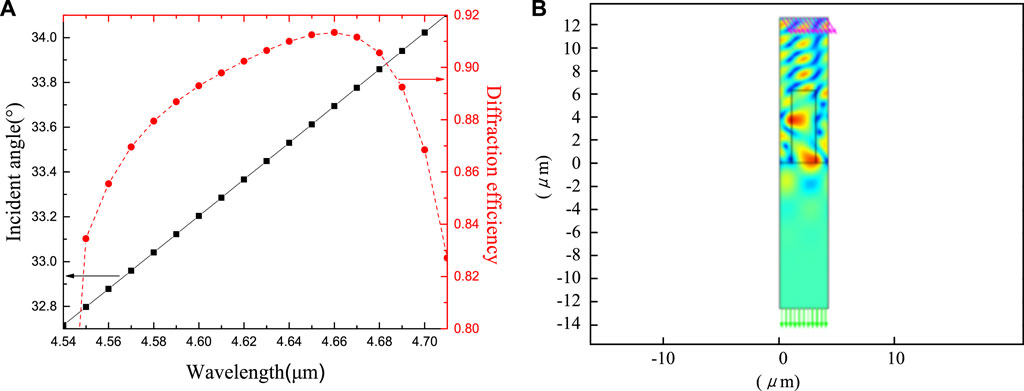
FIGURE 3. (A) Variations of the optimal incidence angle and diffraction efficiency with laser wavelength; (B) The electric field distribution for the laser beam of 4.6 μm at the optimal incidence angle of 33.2°, the purple and the green arrows represent the directions of incident light and diffracted light respectively.
From the previous simulation and analysis, it is clear that the symmetrical grating is perfectly feasible for spectral beam combining, and has the advantages of high spectral utilisation and small space occupation compared with traditional grating combining scheme. But as shown in Figure 1B, this grating can only combine two beams of the same frequency (or same wavelength). If a grating could combine multiple beams of light at the same frequency, the spectrum of each laser does not need to be broadband, and the combined laser beam is not broadband anymore which is very meaningful in some application fields [17]. To this end, we further develop a circular grating.
2.3 Circular grating and its spectral beam combining scheme
The so-called circular grating means that the grating grooves are not straight but circular, so that the same frequency beam is incident on the same solid angle to the center of the circular grating to achieve coaxial same frequency spectral beam combining. A schematic diagram of a 4-channel lasers spectral beam combining using the circular grating is given in Figure 4, CG is the circular grating and OC is the coupling output mirror respectively. Obviously, the overall output power of the combined beam can be increased by increasing the number of the same frequency lasers at this solid angle, and further increased by increasing the number of different wavelength lasers at other solid angles.
FIGURE 4. The same frequency spectral beam combining scheme based on the circular grating.
3 Conclusion
To increase the number of lasers and spectral utilisation for greater output power of the combined beam, we propose a symmetric (one-dimensional) grating and a circular grating (two-dimensional). Compared with traditional spectral combining scheme, the symmetrical grating spectral beam combining scheme can double the total output power of the combined beam by doubling the number of lasers for the same spectral line-width. The grating was designed with a central wavelength of 4.65 µm, and the calculations show that this grating is ideally suited for spectral beam combining with a high diffraction efficiency (>80%) and a relatively significant difference in the incidence angle for the incident light in the range 4.55–4.71 μm. In addition, a circular grating is further proposed for the same frequency spectral beam combining. The ideas of symmetrical grating, circular grating and same frequency spectral beam combining proposed in this study will further enrich the study of spectral beam combination.
Data availability statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author contributions
XF: Conceptualization, Original draft, Review and Editing JZ: Numerical simulation, Software SEL: Conceptualization SHL: Numerical simulation YW: Investigation FD: Review and Editing XH: Numerical simulation, Review and Editing YL: Review LZ: Writing, Review and Editing CW: Original draft, Review, Funding acquisition.
Funding
This work is supported by the Shandong Provincial Natural Science Foundation, China (No. ZR2020QA072).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Yan A, Liu L, Dai E, Sun J, Zhou Y. Simultaneous beam combination and aperture filling of coherent laser arrays by conjugate Dammann gratings. Opt Lett (2010) 35:1251. doi:10.1364/OL.35.001251
2. Veldkamp WB, Leger JR, Swanson GJ. Coherent summation of laser beams using binary phase gratings. Opt Lett (1986) 11:303. doi:10.1364/OL.11.000303
3. Leger JR, Swanson GJ, Veldkamp WB. Coherent beam addition of GaAlAs lasers by binary phase gratings. Appl Phys Lett (1986) 48:888–90. doi:10.1063/1.96648
4. Bloom G, Larat C, Lallier E, Lehoucq G, Bansropun S, Lee-Bouhours MSL, et al. Passive coherent beam combining of quantum-cascade lasers with a Dammann grating. Opt Lett (2011) 36:3810. doi:10.1364/OL.36.003810
5. Nabors CD. Effects of phase errors on coherent emitter arrays. Appl Opt (1994) 33:2284. doi:10.1364/AO.33.002284
6. Lucas-Leclin G, Schimmel G, Albrodt P, Hanna M, Georges P. Coherent combining architectures for high-brightness laser diodes. In: 2017 IEEE High Power Diode Lasers and Systems Conference (HPD) (2017). p. 49. doi:10.1109/HPD.2017.8261095
7. Chang H, Chang Q, Xi J, Hou T, Su R, Ma P, et al. First experimental demonstration of coherent beam combining of more than 100 beams. Photon Res (2020) 8:1943. doi:10.1364/PRJ.409788
8. Sakakibara R, Mio N. Simplified system for relative phase control between two input beams for coherent polarization beam combination. Appl Opt (2020) 59:10289. doi:10.1364/AO.405132
9. Albrodt P, Niemeyer M, Crump P, Hamperl J, Moron F, Georges P, et al. Coherent beam combining of high power quasi continuous wave tapered amplifiers. Opt Express (2019) 27:27891. doi:10.1364/OE.27.027891
10. Cook CC, Fan TY. Spectral beam combining of Yb-doped fiber lasers in an external cavity[C]. Adv Solid State Lasers (1999) 26:PD5. doi:10.1364/ASSL.1999.PD5
11. Daneu V, Sanchez A, Fan TY, Choi HK, Cook CC. Spectral beam combining of a broad-stripe diode laser array in an external cavity. Opt Lett (2000) 25:405. doi:10.1364/OL.25.000405
12. Tso Yee F, Sanchez A, Daneu V, Aggarwal RL, Buchter SC, Goyal A, et al. Laser beam combining for power and brightness scaling[C]. In: 2000 IEEE Aerospace Conference Proceedings (Cat No00TH8484) (2000). p. 49. doi:10.1109/AERO.2000.879830
13. Huang RK, Chann B, Missaggia LJ, Donnelly JP, Harris CT, Turner GW, et al. High-Brightness wavelength beam combined semiconductor laser diode arrays. IEEE Photon Technol Lett (2007) 19:209–11. doi:10.1109/LPT.2006.890717
14. Cao Y, Shu S, Sun F, Zhao Y, Tong C, Wang L. Development of beam combining technology in mid-infrared semiconductor lasers(invited). 红外与激光工程 (2018) 47:1003002. doi:10.3788/irla201847.1003002
15. Sun S, Tan H, Meng H, Guo L, Gao S, Wu D, et al. High brightness diode laser by coupler free grating external cavity spectral beam combining. Infrared Laser Eng (2019) 48:306006. doi:10.3788/irla201948.0306006
16. Pan T, Ye J, Zhang Z, Xu Y. Inverse design of coupled subwavelength dielectric resonators with targeted eigenfrequency and Q factor utilizing deep learning. Opt Lett (2022) 47:3359. doi:10.1364/OL.463040
Keywords: spectral beam combination, symmetrical grating, circular grating, same frequency spectral beam combination, coaxial beam combining
Citation: Fan X, Zhang J, Li S, Li S, Wang Y, Du F, Huang X, Li Y, Zhang L and Wang C (2022) A method to achieve spectral beam combining based on a novel symmetric grating. Front. Phys. 10:1102323. doi: 10.3389/fphy.2022.1102323
Received: 18 November 2022; Accepted: 25 November 2022;
Published: 08 December 2022.
Edited by:
Liyuan Chen, Hilase Center, CzechiaReviewed by:
Yajun Pang, Hebei University of Technology, ChinaPibin Bing, North China University of Water Conservancy and Electric Power, China
Li Xing, Shanghai Jiao Tong University, China
Copyright © 2022 Fan, Zhang, Li, Li, Wang, Du, Huang, Li, Zhang and Wang. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Jianxin Zhang, zhjx_aa@126.com; Chunyan Wang, wangcy@bnu.edu.cn