Finite-time synchronization of Kuramoto-oscillator networks with a pacemaker based on cyber-physical system

In this paper, we study the finite-time synchronization problem of a Kuramoto-oscillator network with a pacemaker. By constructing a cyber-physical system (CPS), the finite-time phase agreement and frequency synchronization of the network are explored for identical and non-identical oscillators, respectively. According to the Lyapunov stability analysis, sufficient conditions are deduced for ensuring the phase agreement and frequency synchronization for arbitrary initial phases and/or frequencies under distributed strategies. Furthermore, the upper bound estimations of convergence time are obtained accordingly, which is related to the initial phases and/or frequencies of oscillators. Finally, numerical examples are presented to verify the effectiveness of the theoretical results.

1 Introduction

Synchronization of complex networks has been extensively investigated by researchers due to its numerous practical applications. As one of the most celebrated periodic-oscillator models, Kuramoto model [1] and its variations have been widely used for explaining various synchronization phenomena, and they have attracted considerable attention from researchers in diverse fields ranging from biology [2, 3], mathematics [4], physics [5, 6] and engineering [7–10]. In the past decade, many progresses concerning on the synchronization of Kuramoto-oscillator networks have been made by researchers in the control community [10–20], where synchronization criteria with respect to constraints on coupling strengths and initial phases have been developed. For example, in [10], the relationship between the algebraic connectivity of a connected Kuramoto-oscillator network and critical coupling was revealed. In [11], Chopra and Spong showed that initial relative phases should be confined to π/2 and a critical coupling strength should be satisfied, which guaranteed the frequency synchronization of an all-to-all connected Kuramoto network.

In [14, 15, 17, 20], researchers have taken the pacemaker (i.e. the so-called leader) into consideration, where synchronization criteria were related to not only the constraint on coupling strengths and initial phases, but also the selection of direct controlled oscillators. Since the interactions between oscillators are usually in the sinusoidal form of phase differences, the theoretical results mentioned above were based on the requirements of initial phases, and only local stability analyses were provided. Based on the framework of cyber-physical systems [21, 22], distributed linear controllers have been adopted to synchronize Kuramoto-oscillator networks in [23, 24], where the derived stability conditions were independent of the initial phases such that the global synchronization was achieved. In [24], sufficient criteria were established for the Kuramoto-oscillator network with a pacemaker under distributed linear control.

The results aforementioned merely focused on the asymptotical synchronization, which indicated that synchronization was realized when t → ∞. Recently, in [18, 19, 25–27], more researchers have focused on the finite-time synchronization of Kuramoto-oscillator networks, which is also of significance in practical applications. For example, power girds need to get rid of local power failures as soon as possible in order to avoid the cascading failure. In [27], Wu and Li investigated the finite-time and fixed-time synchronization of Kuramoto-oscillator networks by employing a novel multiplex control. However, the finite-time synchronization of Kuramoto-oscillator network in present of a pacemaker has not been investigated so far.

Inspired by the above literatures, it is worth investigating the finite-time synchronization of Kuramoto-oscillator network with a pacemaker. In this paper, we aim to explore finite-time synchronization criteria of such network by adopting distributed schemes based on CPS. The main contributions of this paper are summarized as follows: Firstly, effective criteria are established to deal with finite-time phase agreement and frequency synchronization for Kuramoto-oscillator network with a pacemaker, and the upper bound of synchronization time is also provided; Secondly, synchronization can be achieved for arbitrary initial phases, which only influence the upper bound of synchronization time; Finally, the requirement on the connectivity of physical system is relaxed, even if it is an unconnected network.

The remainder of this paper is organized as follows. In Section 2, the framework of CPS is constructed, which consists of the physical Kuramoto-oscillator network system and the cyber (controlling) system. Furthermore, two definitions and some necessary mathematical preliminaries are encompassed in Section 2. Finite-time phase agreement in an identical Kuramoto-oscillator network and frequency synchronization in a non-identical Kuramoto-oscillator network cover the heart body of Section 3 and Section 4, respectively. Section 5 presents the numerical simulation results, and Section 6 concludes the whole paper.

2 Model and preliminaries

In the framework of CPS, a Kuramoto-oscillator network consisting of N oscillators with control input u _i can be described as

${\dot{\theta }}_i={\omega }_i+\sum _{j=1}^N{a}_{ij}\sin \left({\theta }_j-{\theta }_i\right)+u_i,i\in I,(1)$

where $I=\left\{1,\dots ,N\right\}$ , θ _i and ω _i are the phase and natural frequency of the ith oscillator, respectively. $A={(a_{ij})}_{N\times N}$ denotes the adjacency matrix of an undirected network, where a _ij = a _ji (i ≠ j) > 0 iff there is an edge between oscillator i and oscillator j; otherwise, a _ij = 0. Let ${\mathcal{L}}_A=D^A-A$ be the Laplacian matrix associated with the adjacency matrix A, where D ^A ∈ R ^N×N is a diagonal matrix with $D_{ii}^A={\sum }_{j=1}^N{a}_{ij}(\forall i\in I)$ . The network associated with the adjacency matrix A is called physical network.

Assume that there is a pacemaker with dynamics

${\dot{\theta }}_0={\omega }_0,$

where θ ₀ and ω ₀ are the phase and natural frequency of the pacemaker, respectively.

In this paper, we concern phase agreement and frequency synchronization with respect to the pacemaker in finite time.

Definition 1. Network Eq. 1 with control input u _i achieves (pacemaker-based) finite-time phase agreement, if there exists a settling time T > 0 depending on the initial states θ _i (0) (i ∈ {0}⋃I), such that

$\underset{t\to T}{\lim }\left({\theta }_i-{\theta }_0\right)=0,i\in I,(2)$

and θ _i − θ ₀ ≡ 0 for t ≥ T.

Definition 2. Network Eq. 1 with control input u _i achieves (pacemaker-based) finite-time frequency synchronization, if there exists a settling time T > 0 depending on the initial states ${\dot{\theta }}_i(0)(i\in \{0\}\bigcup I)$ , such that

$\underset{t\to T}{\lim }\left(\dot{{\theta }_i}-\dot{{\theta }_0}\right)=0,i\in I,(3)$

and $\dot{{\theta }_i}-\dot{{\theta }_0}\equiv 0$ for t ≥ T.In order to obtain the sufficient conditions, the following Lemmas are needed.

Lemma 1. [28] For an undirected graph $\mathcal{G}$ with N nodes, $x^T\mathcal{L}x=\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{(x_i-x_j)}^2$ holds, where $x={(x_1,x_2,\dots ,x_N)}^T$ and $\mathcal{L}$ is the Laplacian matrix of $\mathcal{G}$ .

Lemma 2. [29] Consider the system of differential equation

$\dot{x}\left(t\right)=f\left(x\left(t\right)\right)(4)$

where $f:\mathcal{D}\to {\mathbb{R}}^n$ is continuous on an open neighborhood $\mathcal{D}\subseteq {\mathbb{R}}^n$ of the origin and f (0) = 0. A continuously differentiable function $x:I\to \mathcal{D}$ is said to be a solution of Eq. 4 on the interval $I\subset \mathbb{R}$ if x satisfies Eq. 4 for all t ∈ I.If there exists a continuous function V(x): $\mathcal{D}\to \mathbb{R}$ such that(1) V(x) is positive definite;(2) There exist real numbers c > 0, 0 < ρ < 1, and an open neighborhood ${\mathcal{D}}_0\subseteq \mathcal{D}$ of the origin such that $\dot{V}(x)\le -c{V}^{\rho }(x),x\in {\mathcal{D}}_0\backslash \{0\}$ .Then, the origin is a finite-time stable equilibrium of Eq. 4 and the finite settling time T satisfies

$T\le \frac{V^{1-\rho }\left(x\left(0\right)\right)}{c\left(1-\rho \right)}.$

If in addition ${\mathcal{D}}_0=\mathcal{D}={\mathbb{R}}^N$ , the origin is globally finite-time stable equilibrium.For the sake of convenience, let ξ _i = θ _i − θ ₀, then $\dot{{\xi }_i}=\dot{{\theta }_i}-\dot{{\theta }_0}=\dot{{\theta }_i}-{\omega }_0$ . For a real symmetric matrices $\mathcal{L}$ , let ${\lambda }_{\min }(\mathcal{L})$ be the minimum eigenvalue of matrix $\mathcal{L}$ . Denote $sig{(x)}^{\alpha }=\mathrm{sign}(x){\left|x\right|}^{\alpha }$ , where the signum function sign(x) is defined as

$sign\left(x\right)=\left\{\begin{array}{c}\hfill 1,\forall x>0,\hfill \\ \hfill 0,x=0,\hfill \\ \hfill -1,\forall x<0.\hfill \end{array}\right.$

3 Finite-time phase agreement for identical Kuramoto oscillators

In this section, we first concentrate on the case of oscillators with identical natural frequency, i.e., ω _i = ω ₀, ∀i ∈ I. Thus, network Eq. 1 with control input u _i becomes

${\dot{\theta }}_i=\omega +\sum _{j=1}^N{a}_{ij}\sin \left({\theta }_j-{\theta }_i\right)+u_i,i\in I.(5)$

For achieving finite-time phase agreement, a distributed control strategy is constructed as

$u_i=\sum _{i=1}^N{b}_{ij}\left({\theta }_j-{\theta }_i\right)+f_{i}sig{\left({\theta }_0-{\theta }_i\right)}^{\alpha },(6)$

where $B={(b_{ij})}_{N\times N}$ denotes the adjacency matrix of an undirected network with elements b _ij defined similar to a _ij , f _i ≥ 0, and parameter 0 < α < 1. The network associated with the connections between the oscillators in the controller Eq. 6 is called cyber network. Let ${\mathcal{L}}_B$ be the Laplacian matrix associated with the adjacency matrix B, where its elements are defined similar to ${\mathcal{L}}_A$ .

By transforming θ _i into ξ _i , network Eq. 5 with distributed control strategy Eq. 6 becomes

${\dot{\xi }}_i=\sum _{j=1}^N{a}_{ij}\sin \left({\xi }_j-{\xi }_i\right)+\sum _{j=1}^N{b}_{ij}\left({\xi }_j-{\xi }_i\right)-f_{i}sig{\left({\xi }_i\right)}^{\alpha }.(7)$

Theorem 1. Network Eq. 1 with identical oscillator under distributed control strategy Eq. 6 achieves finite-time phase agreement with the settling time bounded by

$T_1\le \frac{{‖\xi \left(0\right)‖}^{1-\alpha }}{\left(1-\alpha \right)f_{\min }},(8)$

if

${\lambda }_{\min }\left(\cos \gamma \cdot {\mathcal{L}}_A+{\mathcal{L}}_B\right)\ge 0,$

where f _min = min{f ₁, …, f _N }, ${\Vert \xi (0)\Vert }^2=\sum _{i=1}^N{[{\xi }_i(0)]}^2$ and γ ∈ (π, 2π) satisfies tan γ = γ.

Proof 1. Consider the following Lyapunov functional candidate

$V_1=\frac{1}{2}{\xi }^T\xi =\frac{1}{2}\sum _{i=1}^N{\xi }_i^2.$

The derivation of V ₁ along trajectories Eq. 7 gives

$\begin{array}{c}\hfill {\dot{V}}_1=\sum _{i=1}^N{\xi }_i{\dot{\xi }}_i=\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{\xi }_i\sin \left({\xi }_j-{\xi }_i\right)+\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\xi }_i\sin \left({\xi }_j-{\xi }_i\right)-\sum _{i=1}^N{f}_i{\xi }_{i}sig{\left({\xi }_i\right)}^{\alpha }.\end{array}(9)$

According to < href="statement-Lemma_1">Lemma 1 and the fact $\frac{\sin ({\theta }_j-{\theta }_i)}{({\theta }_j-{\theta }_i)}\in [\cos \gamma ,1]$ , we can obtain

$\begin{array}{ll}\hfill \sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{\xi }_i\sin \left({\xi }_j-{\xi }_i\right)& =\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}\left({\xi }_i-{\xi }_j\right)\sin \left({\xi }_j-{\xi }_i\right)=-\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}\frac{\sin \left({\xi }_j-{\xi }_i\right)}{\left({\xi }_j-{\xi }_i\right)}{\left({\xi }_j-{\xi }_i\right)}^2\hfill \\ \le -\frac{\cos \gamma }{2}\cdot \sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{\left({\xi }_j-{\xi }_i\right)}^2\hfill \\ \hfill & =-\cos \gamma \cdot {\xi }^T{\mathcal{L}}_A\xi .\hfill \end{array}(10)$

And,

$\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\xi }_i\left({\xi }_j-{\xi }_i\right)=-\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\left({\xi }_j-{\xi }_i\right)}^2=-{\xi }^T{\mathcal{L}}_B\xi ,(11)$

$\sum _{i=1}^N{f}_i{\xi }_{i}sig{\left({\xi }_i\right)}^{\alpha }=\sum _{i=1}^N{f}_i{\xi }_i\mathit{sign}\left({\xi }_i\right){\left|{\xi }_i\right|}^{\alpha }\le \sum _{i=1}^N{f}_i{\left|{\xi }_i\right|}^{1+\alpha }.(12)$

Combining Eqs. 10–12, Eq. 9 yields

${\dot{V}}_1\le -{\xi }^T\left(\cos \gamma \cdot {\mathcal{L}}_A+{\mathcal{L}}_B\right)\xi -\sum _{i=1}^N{f}_i{\left|{\xi }_i\right|}^{1+\alpha }.$

If ${\lambda }_{\min }(\cos \gamma \cdot {\mathcal{L}}_A+{\mathcal{L}}_B)\ge 0$ , we get

$\begin{array}{cc}\hfill {\dot{V}}_1& \le -\sum _{i=1}^N{f}_i{\left|{\xi }_i\right|}^{1+\alpha }\hfill \\ \hfill & =-\sum _{i=1}^N{f}_i{\left[{\left|{\xi }_i\right|}^2\right]}^{\frac{1+\alpha }{2}}\hfill \\ \hfill & \le -f_{\min }{2}^{\frac{1+\alpha }{2}}{\left[\frac{1}{2}\sum _{i=1}^N{\left|{\xi }_i\right|}^2\right]}^{\frac{1+\alpha }{2}}\hfill \\ \hfill & =-2^{\frac{1+\alpha }{2}}{f}_{\min }{V_1}^{\frac{1+\alpha }{2}}.\hfill \end{array}$

By < href="statement-Lemma_2">Lemma 2 and < href="statement-Definition_1">Definition 1 , network Eq. 1 with identical oscillator under distributed control strategy Eq. 6 achieves finite-time phase agreement with the settling time bounded by

$T_1\le \frac{{\left[\sum _{i=1}^N{\left({\xi }_i\left(0\right)\right)}^2\right]}^{\frac{1-\alpha }{2}}}{\left(1-\alpha \right)f_{\min }}=\frac{{‖\xi \left(0\right)‖}^{1-\alpha }}{\left(1-\alpha \right)f_{\min }}.$

This completes the proof.

Remark 1. According to (8), we find that the upper bound of synchronization time is proportionate to initial state ‖ξ(0)‖, and is inversely proportional to f _min . According to < href="statement-Theorem_1">Theorem 1 , it is sufficient to achieve finite-time phase agreement if ${\lambda }_{\min }(\cos \gamma \cdot {\mathcal{L}}_A+{\mathcal{L}}_B)\ge 0$ . Therefore, even if the physical network is not connected, phase agreement could be also achieved with the help of cyber network, which relaxes the requirement on the connectivity of the physical network.

4 Finite-time frequency synchroniztion for non-identical Kuramoto oscillators

Now we further concentrate on the case of oscillators with non-identical natural frequencies, i.e., there exists some i ∈ I such that ω _i ≠ ω ₀. For achieving finite-time frequency synchronization, a distributed control strategy u _i is designed as

$u_i=\sum _{j=1}^N{b}_{ij}\left({\theta }_j-{\theta }_i\right)+U_i,(13)$

where $\dot{U_i}=f_{i}sig{({\dot{\theta }}_0-{\dot{\theta }}_i)}^{\alpha }$ , f _i ≥ 0, parameter 0 < α < 1, and b _ij denotes the same as that in Eq. 6. Let ${\mathcal{L}}_B$ be the Laplacian matrix associated with the adjacency matrix B, where its elements are defined similar to ${\mathcal{L}}_A$ .

Theorem 2. Network Eq. 1 with non-identical oscillators under distributed control strategy Eq. 13 achieves frequency synchronization with the settling time bounded by

$T_2\le \frac{{‖\dot{\xi }\left(0\right)‖}^{1-\alpha }}{\left(1-\alpha \right)f_{\min }},(14)$

if

${\lambda }_{\mathit{min}}\left({\mathcal{L}}_B-{\mathcal{L}}_A\right)\ge 0,$

where ${\Vert \dot{\xi }(0)\Vert }^2=\sum _{i=1}^N{[{\dot{\xi }}_i(0)]}^2$ .

Proof 2. By taking the derivation of Eq. 1 , we obtain

$\ddot{{\xi }_i}=\ddot{{\theta }_i}=\sum _{j=1}^N{a}_{ij}\cos \left({\xi }_j-{\xi }_i\right)\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)+{\dot{u}}_i.(15)$

Consider the following Lyapunov functional candidate

$V_2=\frac{1}{2}\dot{{\xi }^T}\dot{\xi }=\frac{1}{2}\sum _{i=1}^N{\dot{{\xi }_i}}^2.$

The derivation of V ₂ along trajectories Eq. 15 gives

$\begin{array}{ll}\hfill {\dot{V}}_2& =\sum _{i=1}^N{\dot{\xi }}_i{\ddot{\xi }}_i=\sum _{i=1}^N{\dot{\xi }}_i\left[\sum _{j=1}^N{a}_{ij}\cos \left({\xi }_j-{\xi }_i\right)\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)+{\dot{u}}_i\right]\hfill \\ \hfill & =\sum _{i=1}^N{\dot{\xi }}_i\left[\sum _{j=1}^N{a}_{ij}\cos \left({\xi }_j-{\xi }_i\right)\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)+\sum _{j=1}^N{b}_{ij}\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)-f_{i}sig{\left({\dot{\xi }}_i\right)}^{\alpha }\right]\hfill \\ \hfill & =\sum _{i=1}^N\sum _{j=1}^N{\dot{\xi }}_i{a}_{ij}\cos \left({\xi }_j-{\xi }_i\right)\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)+\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\dot{\xi }}_i\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)-\sum _{i=1}^N{\dot{\xi }}_{i}sig{\left({\dot{\xi }}_i\right)}^{\alpha }.\hfill \end{array}(16)$

According to < href="statement-Lemma_1">Lemma 1 and the fact $\left|\cos ({\theta }_j-{\theta }_i)\right|\le 1$ , we can obtain

$\begin{array}{cc}\hfill \sum _{i=1}^N\sum _{j=1}^N{\dot{\xi }}_i{a}_{ij}\cos \left({\xi }_j-{\xi }_i\right)\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)& =-\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)}^2\cos \left({\xi }_j-{\xi }_i\right)\le \frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{a}_{ij}{\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)}^2\hfill \\ ={\dot{\xi }}^T{\mathcal{L}}_A\dot{\xi }.\hfill \end{array}(17)$

And,

$\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\dot{\xi }}_i\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)=-\frac{1}{2}\sum _{i=1}^N\sum _{j=1}^N{b}_{ij}{\left({\dot{\xi }}_j-{\dot{\xi }}_i\right)}^2=-{\dot{\xi }}^T{\mathcal{L}}_B\dot{\xi },(18)$

$\sum _{i=1}^N{\dot{\xi }}_{i}sig{\left({\dot{\xi }}_i\right)}^{\alpha }=\sum _{i=1}^N{f}_i{\dot{\xi }}_i\mathit{sign}\left({\dot{\xi }}_i\right){\left|{\dot{\xi }}_i\right|}^{\alpha }\le \sum _{i=1}^N{f}_i{\left|{\dot{\xi }}_i\right|}^{\alpha +1}.(19)$

Combining Eqs. 17–19, Eq. 16 yields

${\dot{V}}_2\le -{\dot{\xi }}^T\left({\mathcal{L}}_B-{\mathcal{L}}_A\right)\dot{\xi }-\sum _{i=1}^N{f}_i{\left|{\dot{\xi }}_i\right|}^{\alpha +1}.$

If ${\lambda }_{\min }({\mathcal{L}}_B-{\mathcal{L}}_A)\ge 0$ , we get

$\begin{array}{ll}\hfill {\dot{V}}_2& \le -\sum _{i=1}^N{f}_i{\left|{\dot{\xi }}_i\right|}^{\alpha +1}\hfill \\ \hfill & =-\sum _{i=1}^N{f}_i{\left[{\left|{\dot{\xi }}_i\right|}^2\right]}^{\frac{1+\alpha }{2}}\hfill \\ \hfill & =-f_{\min }{2}^{\frac{1+\alpha }{2}}{\left[\frac{1}{2}\sum _{i=1}^N{\left|{\dot{\xi }}_i\right|}^2\right]}^{\frac{1+\alpha }{2}}\hfill \\ \hfill & =-2^{\frac{1+\alpha }{2}}{f}_{\min }{V}_2^{\frac{1+\alpha }{2}}.\hfill \end{array}$

By < href="statement-Lemma_2">Lemma 2 and < href="statement-Definition_2">Definition 2 , network Eq. 1 with non-identical oscillators under distributed control strategy Eq. 13 achieves finite-time frequency synchronization with the settling time bounded by

$T_2\le \frac{{\left[\sum _{i=1}^N{\left(\dot{{\xi }_i}\left(0\right)\right)}^2\right]}^{\frac{1-\alpha }{2}}}{\left(1-\alpha \right)f_{\min }}=\frac{{‖\dot{\xi }\left(0\right)‖}^{1-\alpha }}{\left(1-\alpha \right)f_{\min }}.$

This completes the proof.

Remark 2. According to Eq. 14 , we find that the upper bound of synchronization time is proportionate to initial state $\Vert \dot{\xi }(0)\Vert$ , and is inversely to the f _min . According to < href="statement-Theorem_2">Theorem 2 , it is sufficient to achieve finite-time frequency synchronization if ${\lambda }_{\min }({\mathcal{L}}_B-{\mathcal{L}}_A)\ge 0$ . Therefore, even if the physical network is not connected, frequency synchronization could be also achieved with the help of cyber network, which relaxes the requirement on the connectivity of the physical network.

5 Numerical simulation

In this section, we assume networks associated with adjacency matrices A and B as shown in Figures 1A,B, respectively.

FIGURE 1

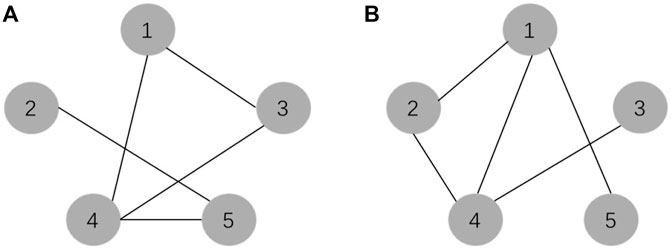
FIGURE 1. (A) Network associated with adjacency matrix A. (B) Network associated with adjacency matrix B.

We first verify < href="statement-Theorem_1">Theorem 1. Obviously, ${\lambda }_{\min }(\cos \gamma \cdot {\mathcal{L}}_A+{\mathcal{L}}_B)=0$ . For simplicity, set ω _i = 0 (i = 0, 1, 2, 3, 4, 5), α = 0.5, f _i = 2 (i = 1, 2, 3, 4, 5), and (θ ₀ (0), θ ₁ (0), θ ₂ (0), θ ₃ (0), θ ₄ (0), θ ₅ (0)) = (0.25,−0.1028,28.8866,−10.0289,5.3575,−17.3534) ^T . In Figure 2A, phase differences θ _i − θ ₀ converge to zero, which means finite-time phase agreement is achieved. Besides, it also shows phase agreement is achieved about 2.2s, which is less than the upper bound of settling time T ₁ = 5.9600s. Time evolutions of the controller Eq. 6 of each oscillator are showed in Figure 2B.

FIGURE 2

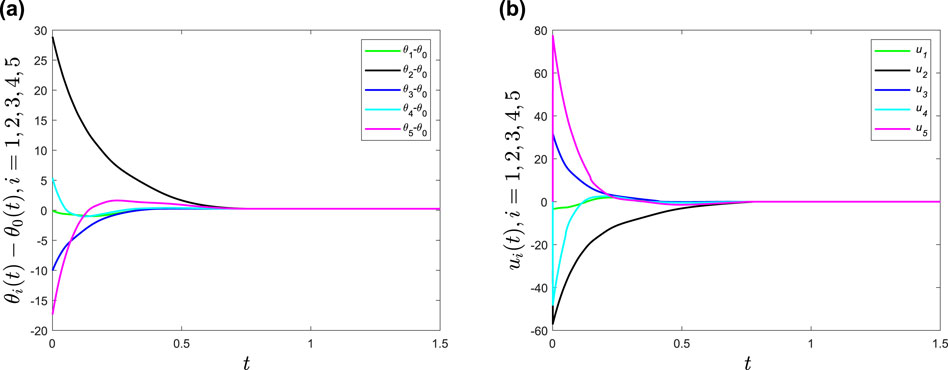
FIGURE 2. (A) Time evolutions of phase differences θ _i − θ ₀ (i = 1, 2, 3, 4, 5) under distributed control strategy Eq. 6. (B) Time evolutions of the distributed control strategy Eq. 6.

Secondly, we verify < href="statement-Theorem_2">Theorem 2. Obviously, ${\lambda }_{\min }({\mathcal{L}}_B-{\mathcal{L}}_A)=0$ . Set α = 0.5, f _i = 2 (i = 1, 2, 3, 4, 5), ${\dot{\theta }}_0(0)={\omega }_0=2$ , (ω ₁, ω ₂, ω ₃, ω ₄, ω ₅) = (−10,−4,0,4,10) ^T , and $({\dot{\theta }}_1(0),{\dot{\theta }}_2(0),{\dot{\theta }}_3(0),{\dot{\theta }}_4(0),{\dot{\theta }}_5(0))={(44.5324,-4.2299,17.8956,-39.9282,-18.2699)}^T$ . In Figure 3Afrequency differences $\dot{{\theta }_i}-\dot{{\theta }_0}$ converge to zero, which means finite-time frequency synchronization is achieved. Besides, it also shows frequency synchronization is achieved about 0.825s, which is less than the upper bound of settling time bound T ₂ = 2.1147s. Time evolutions of the controller Eq. 13 of each oscillator are showed in Figure 3B.

FIGURE 3

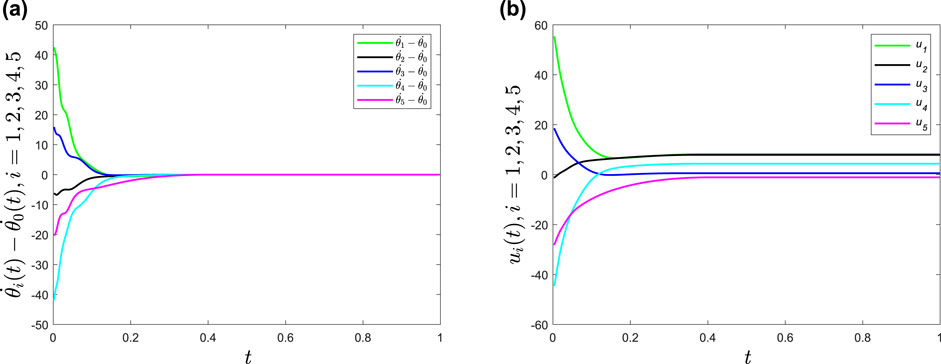
FIGURE 3. (A) Time evolutions of frequencies differences ${\dot{\theta }}_i-{\dot{\theta }}_0(i=\mathrm{1,2,3,4,5})$ under distributed control strategy Eq. 13. (B) Time evolutions of the distributed control strategy Eq. 13.

Finally, we move to see the influence of parameter α on synchronization time. In the simulations, we set α = 0.1, 0.3, 0.5, 0.7, 0.9. In Figure 4, it is showed that the synchronization time decreases as α grows.

FIGURE 4

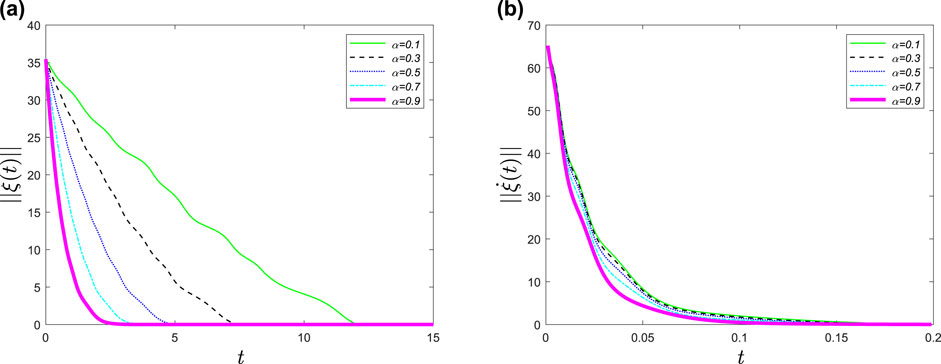
FIGURE 4. (A) Time evolutions of ‖ξ(t)‖ under the distributed control strategy Eq. 6 with respect to different parameters α = 0.1, 0.3, 0.5, 0.7, 0.9. (B) Time evolutions of $\Vert \dot{\xi }(t)\Vert$ under the distributed control strategy Eq. 13 with respect to different parameters α = 0.1, 0.3, 0.5, 0.7, 0.9.

6 Conclusion

In this paper, the problems of finite-time phase agreement and frequency synchronization of Kuramoto-oscillator networks with a pacemaker have been investigated. Two distributed control strategies, based on the CPS, have been designed to drive the Kuramoto-oscillator networks. In the light of finite-time stability theory, the sufficient criteria have been derived for guaranteeing the phase agreement and frequency synchronization of identical and non-identical Kuramoto-oscillator networks with a pacemaker. At the same time, the upper bounds estimation of convergence time of Kuramoto-oscillator networks have been given accordingly. Numerical examples have validated the effectiveness of the derived theoretical results.

However, the convergence time estimations of this paper are heavily related to initial phases and/or frequencies of oscillators. Therefore, it is urgent to explore the fixed-time synchronization of Kuramoto model with a pacemaker in the future.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.

Author contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Funding

This work is supported by National Natural Science Foundation of China (Grant No. 61903142), the Science and Technology Project of Jiangxi Education Department (Grant No. GJJ180356).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. Kuramoto Y. Chemical oscillators, waves, and turbulence. Berlin, Germany: Springer (1984).

Google Scholar

2. Liu C, Weaver DR, Strogatz SH, Reppert SM. Cellular construction of a circadian clock: period determination in the suprachiasmatic nuclei. Cell (1997) 91:855–60. doi:10.1016/s0092-8674(00)80473-0

PubMed Abstract | CrossRef Full Text | Google Scholar

3. Chen C, Liu S, Shi X-Q, Chaté H, Wu YL. Weak synchronization and large-scale collective oscillation in dense bacterial suspensions. Nature (2017) 542:210–4. doi:10.1038/nature20817

PubMed Abstract | CrossRef Full Text | Google Scholar

4. Kim J-H, Park J-H. Exponential synchronization of Kuramoto oscillators using spatially local coupling. Physica D: Nonlinear Phenomena (2014) 277:40–7. doi:10.1016/j.physd.2014.03.006

CrossRef Full Text | Google Scholar

5. Wiesenfeld K, Colet P, Strogatz SH. Frequency locking in josephson arrays: connection with the Kuramoto model. Phys Rev E (1998) 57:1563–9. doi:10.1103/physreve.57.1563

CrossRef Full Text | Google Scholar

6. Daido H. Quasientrainment and slow relaxation in a population of oscillators with random and frustrated interactions. Phys Rev Lett (1992) 68:1073–6. doi:10.1103/physrevlett.68.1073

PubMed Abstract | CrossRef Full Text | Google Scholar

7. Sun W, Lü JH, Yu XH, Chen Y, Chen SH. Cooperation of multiagent systems with mismatch parameters: a viewpoint of power systems. IEEE Trans Circuits Syst (2016) 63:693–7. doi:10.1109/tcsii.2016.2530178

CrossRef Full Text | Google Scholar

8. Seyboth GS, Wu JB, Qin JH, Yu CB, Allgöwer F. Collective circular motion of unicycle type vehicles with non-identical constant velocities. IEEE Trans Control Netw Syst (2014) 1:167–76. doi:10.1109/tcns.2014.2316995

CrossRef Full Text | Google Scholar

9. Sepulchre R, Paley DA, Leonard NE. Stabilization of planar collective motion: all-to-all communication. IEEE Trans Automat Contr (2007) 52:811–24. doi:10.1109/tac.2007.898077

CrossRef Full Text | Google Scholar

10. Dörfler F, Bullo F. Synchronization in complex networks of phase oscillators: a survey. Automatica (2014) 50:1539–64. doi:10.1016/j.automatica.2014.04.012

CrossRef Full Text | Google Scholar

11. Chopra N, Spong MW. On exponential synchronization of Kuramoto oscillators. IEEE Trans Automat Contr (2009) 54:353–7. doi:10.1109/tac.2008.2007884

CrossRef Full Text | Google Scholar

12. Ha S-Y, Li ZC, Xue XP. Formation of phase-locked states in a population of locally interacting Kuramoto oscillators. J Differential Equations (2013) 255:3053–70. doi:10.1016/j.jde.2013.07.013

CrossRef Full Text | Google Scholar

13. Li HQ, Chen G, Liao XF, Huang TW. Attraction region seeking for power grids. IEEE Trans Circuits Syst (2017) 64:201–5. doi:10.1109/tcsii.2016.2561410

CrossRef Full Text | Google Scholar

14. Li X, Rao PC. Synchronizing a weighted and weakly-connected Kuramoto oscillator digraph with a pacemaker. IEEE Trans Circuits Syst (2015) 62:899–905. doi:10.1109/tcsi.2014.2382193

CrossRef Full Text | Google Scholar

15. Rao PC, Li X, Ogorzalek MJ. Stability of synchronous solutions in a directed Kuramoto-Oscillator network with a pacemaker. IEEE Trans Circuits Syst (2017) 64:1222–6. doi:10.1109/tcsii.2017.2679216

CrossRef Full Text | Google Scholar

16. Jadbabaie A, Motee N, Barahona M. On the stability of the Kuramoto model of coupled nonlinear oscillators. Proc Amer Control Conf (2004) 5.

CrossRef Full Text | Google Scholar

17. Tong DB, Rao PC, Chen QY, Ogorzalek MJ, Li X. Exponential synchronization and phase locking of a multilayer Kuramoto-oscillator system with a pacemaker. Neurocomputing (2018) 308:129–37. doi:10.1016/j.neucom.2018.04.067

CrossRef Full Text | Google Scholar

18. Wu J, Liu MQ, Wang XF, Ma R-R. Achieving fixed-time synchronization of the Kuramoto model via improving control techniques. J Korean Phys Soc (2021) 79:998–1006. doi:10.1007/s40042-021-00302-z

CrossRef Full Text | Google Scholar

19. Wu J, Li X. Global stochastic synchronization of Kuramoto-oscillator networks with distributed control. IEEE Trans Cybern (2021) 51:5825–35. doi:10.1109/tcyb.2019.2959854

PubMed Abstract | CrossRef Full Text | Google Scholar

20. Wang YQ, Doyle FJ. Exponential synchronization rate of Kuramoto oscillators in the presence of a pacemaker. IEEE Trans Automat Contr (2013) 58:989–94. doi:10.1109/tac.2012.2215772

PubMed Abstract | CrossRef Full Text | Google Scholar

21. Derler P, Lee EA, Vincentelli AS. Modeling cyber-physical systems. Proc IEEE (2012) 100:13–28. doi:10.1109/jproc.2011.2160929

CrossRef Full Text | Google Scholar

22. Lee EA. Computing foundations and practice for cyber-physical systems: a preliminary report. California: University of California (2007).

Google Scholar

23. Zhang W-Y, Yang C, Guan Z-H, Liu Z-W, Chi M, Zheng G-L. Bounded synchronization of coupled Kuramoto oscillators with phase lags via distributed impulsive control. Neurocomputing (2016) 218:216–22. doi:10.1016/j.neucom.2016.08.054

CrossRef Full Text | Google Scholar

24. Rao PC, Li X. Pacemaker-based global synchronization of Kuramoto oscillators via distributed control. IEEE Trans Circuits Syst (2018) 65:1768–72. doi:10.1109/tcsii.2017.2763184

CrossRef Full Text | Google Scholar

25. Dong J-G, Xue XP. Finite-time synchronization of Kuramoto-type oscillators. Nonlinear Anal Real World Appl (2015) 26:133–49. doi:10.1016/j.nonrwa.2015.05.006

CrossRef Full Text | Google Scholar

26. Zhang XX, Sun ZY, Yu CB. Finite-time synchronization of networked Kuramoto-like oscillators. In: Proceedings of Australian Control Conference; 3-4 Nov; Newcastle (2016).

CrossRef Full Text | Google Scholar

27. Wu J, Li X. Finite-time and fixed-time synchronization of kuramoto-oscillator network with multiplex control. IEEE Trans Control Netw Syst (2019) 6:863–73. doi:10.1109/tcns.2018.2880299

CrossRef Full Text | Google Scholar

28. Olfati-Saber R, Murray RM. Consensus problems in networks of agents with switching topology and time-delays. IEEE Trans Automat Contr (2004) 49:1520–33. doi:10.1109/tac.2004.834113

CrossRef Full Text | Google Scholar

29. Bhat SP, Bernstein DS. Finite-time stability of continuous autonomous systems. SIAM J Control Optim (2000) 38:751–66. doi:10.1137/s0363012997321358

CrossRef Full Text | Google Scholar