Abstract
As an important branch of quantum secure multi-party computation, quantum secret sharing (QSS) can distribute secret information among dishonest network nodes without revealing the secrets. In this study, a new four-party QSS protocol based on locally indistinguishable orthogonal product (LIOP) states is first proposed for quantum network communication. Then, the general multiparty QSS model based on LIOP states will be expanded. Combined with the property of LIOP states and obfuscating operation, the source node can send the secrets to different destination nodes in the quantum network. Accordingly, it is proven that the destination nodes have to work together to recover the shared secrets against some existing attacks. Furthermore, no entangled resources and complicated operations are required in the presented protocol. We hope the results could make positive effects to the development of quantum secure communication in the future.
1 Introduction
With the rapid development of the Internet, the security of information is becoming more and more important. Cryptography, as one of the fastest developing fields in modern science, is the basic theory to guarantee information security. Due to the development of quantum algorithms [1, 2], classical cryptographic protocols based on computational complexity are facing great security threats. Applying quantum theory to the research of cryptography, quantum cryptography has made a scientific breakthrough in cryptography. In 2002, Long et al. first discussed the quantum secure direct communication idea and analyzed its application in further quantum networks [3]. In 2008, Ma et al. proposed a group quantum communication network based on quantum secret sharing (QSS) among multiple nodes [4]. Afterward, QSS is becoming an important application in the quantum network [5–12].
QSS is the use of quantum technology to distribute secrets to a group of sharers. In QSS, a secret can only be recovered by all authorized sharers working together. As an important branch of quantum secure multi-party computation, QSS has attracted much attention. In 1999, Hillery et al. proposed the first QSS protocol [13]. On this basis, Karlsson et al. designed a Bell state secret sharing protocol [14]. In 2004, Xiao et al. generalized Hillery’s protocol to arbitrary multi-parties, effectively solving the limitation to secret sharing among multiple parties [15]. In 2017, Qin et al. proposed a QSS protocol using the n-qudit GHZ states [16]. In 2019, Zhang et al. gave an n-party QSS model based on multiparty entangled states [17]. In 2020, Mansour et al. presented a QSS protocol using maximally entangled multi-qudit states [18]. In 2021, Hu et al. proposed a novel dynamic QSS protocol in the high-dimensional quantum system based on transmitted particles and local unitary operations [19].
During the study, it can be seen that most of the existing QSS protocols are achieved by entangled states. As we know, the preparation of entangled states is difficult. It is necessary to propose more practical QSS protocols. The local indistinguishability of orthogonal product states is one of the hot topics in quantum information field recently. In 2015, Yu et al. constructed a set of orthogonal product states which cannot be perfectly distinguished by local operations and classical communication (LOCC) [20]. The indistinguishable orthogonal product (LIOP) states are easier to prepare than the entangled ones. It exhibits the overall non-locality of a wide range of applications in quantum cryptographic protocols. For example, Guo et al. proposed a quantum key distribution (QKD) protocol based on LIOP states in 2001 [21]. In 2007, Yang et al. presented a QSS protocol based on LIOP states [22]. In 2019, Jiang et al. proposed a quantum voting protocol based on LIOP states [23]. In 2020, Jiang et al. implemented a trusted third-party e-payment protocol on LIOP states [24].
In this study, we proposed a practical new four-party QSS protocol for LIOP states in quantum networks. First, the source node encodes the secret information into LIOP states. Second, the source node safely obfuscates the particles in the sequence and sends the corresponding particles to different destination nodes. Finally, all destination nodes work together to recover the secrets. Then, we generalize the protocol to any number of parties. According to the property of LIOP states, even if an attacker obtains n − 1 (n ≥ 3) particles of orthogonal product states, it is impossible to determine the shared messages.
The rest of the study is organized as follows. In Section 2, we introduce two LIOP states: X-LIOP states and F-LIOP states. With the introduced LIOP states, a new specific four-party QSS protocol and an extended multi-party QSS protocol are presented in Section 3 and Section 4. The security of the protocol is discussed in Section 5. A brief conclusion is given in Section 6.
2 Preliminaries
Here, we introduce the following specific form and properties of LIOP states, which will be used in the following protocols. It is well known that a set of orthogonal states is locally indistinguishable if it cannot be completely distinguished by LOCC [25].
Definition 1In a 2 ⊗ 2 ⊗⋯⊗ 2 quantum system, the product basis that contains the following 2n orthogonal product statescannot be perfectly distinguished by LOCC, where n ≥ 3, and the subscript i of the state denotes that the corresponding subsystem belong to the i-th party. In order to simplify the following protocol, the states aforementioned are named X-LIOP states.We can get the special case of n = 3, i.e., the following Definition 2.
Definition 2In a 2 ⊗ 2 ⊗ 2 quantum system, the product basis that contains the following six orthogonal product statescannot be perfectly distinguished by LOCC. In order to simplify the subsequent protocol, the states aforementioned are named F-LIOP states.In Refs. [26, 27], these states are proven not to be perfectly distinguished by LOCC. We can find some properties of them.
Property 1Even if n − 1 (n ≥ 3) particles of orthogonal product states are obtained, the exact form cannot be determined.
Property 2Each particle can be transmitted independently.
Property 3An operation on one of the particles does not affect the other particles.
3 Four-party quantum secret sharing protocol based on F-LIOP states
3.1 Proposed protocol
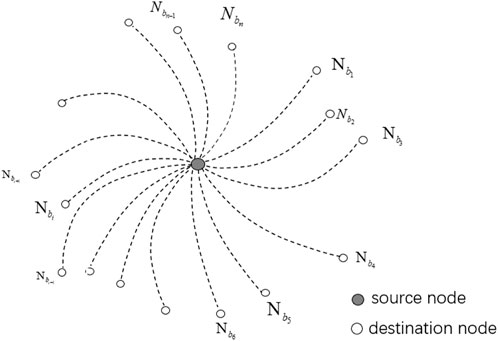
In this section, a four-party QSS protocol applied in the quantum network based on F-LIOP states is proposed. The network graph has two types of nodes (
Figure 1): the source node (
Na) wants to distribute secrets, and destination nodes
receive secrets. The secrets can only be recovered if all destination nodes collaborate. The specific description is as follows:
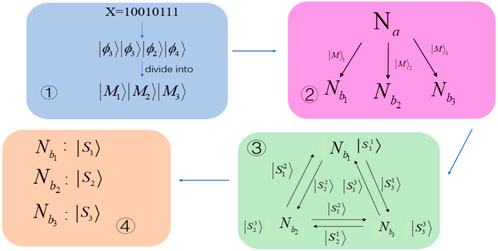
Step 1) Na divides the secret message X into n groups, i.e., x1, …, xn, where xi ∈ {00, 01, 10, 11}, i = 1, 2, …, n.
Step 2) Na encodes the secret message X to a quantum sequence |S⟩, and according to the following rules, it should be accepted by all the nodes:
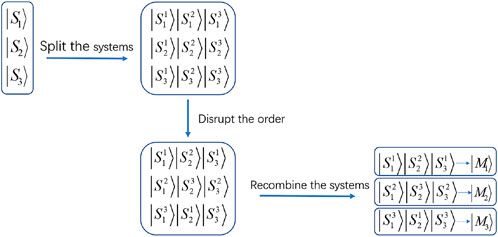
Step 3) Na generates three identical sequences |S⟩, where the i-th sequence is denoted by |Si⟩, i = 1, 2, 3. Na splits |Si⟩ into three subsystems, i.e., . Then, Na generates three sequences |M1⟩, |M2⟩, and |M3⟩, where , and . The distribution of the particles is shown in Figure 2.
Step 4) Na takes the left states composed of |ϕ5⟩, |ϕ6⟩ as decoy states to randomly insert the quantum sequence |Mt⟩ to form |Mt⟩′, where t = 1, 2, 3. Finally, |Mt⟩′ is sent to randomly, where l = 1, 2, 3. In this case, does not know which particle they receive.
Step 5) After receiving the sequence |Mt⟩′ from Na, sends an acknowledgment to Na. Then, Na announces both the basis and the positions of the decoy photons in |Mt⟩′. measures the decoy states. According to the measurement results of , Na performs eavesdropping detection. If no eavesdropping is detected, the protocol will continue to the next step. Otherwise, it will be aborted and will restart from Step 1.
Step 6) After the eavesdropping check, has the sequence |Mt⟩. Then, sends the j-th group of particles |Mt⟩ with the decoy states to , where the decoy states are chosen from {| + ⟩, | − ⟩, |0⟩, |1⟩}, and where j = 1, 2, 3.
Step 7) After receiving the sequences from , sends him a confirmation. announces both the basis and the positions of the decoy photons. According to the measurement results of , performs eavesdropping detection. If no eavesdropping is detected, the protocol will continue to the next step; otherwise, it will be aborted.
Step 8) After the eavesdropping check, has , i.e., |Sl⟩. Then, the quantum sequence |Sl⟩ is measured under the basis of Eq. 2, and is recovered. Na announces the measurement basis and order of all sequences.
Step 9) and hold the same particles and perform the same operations. Therefore, if the protocol is valid, and the secret X must be the same. Intuitively, if , the protocol will be valid; otherwise, the protocol fails.
FIGURE 1
FIGURE 2
3.2 Example
To illustrate our protocol more clearly, the following example is proposed. For convenience, eavesdropping detection is ignored. Suppose secret is 10010111, it can been encoded as |ϕ3⟩, |ϕ2⟩, |ϕ2⟩, |ϕ4⟩.
Therefore,
,
, and
.
Then, we get
,
, and
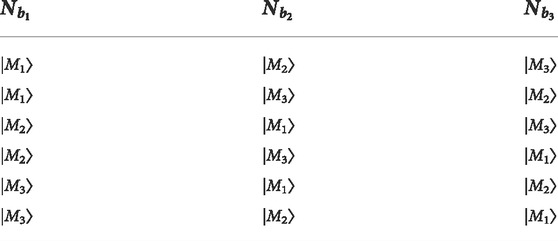
Here, we assume that Na sends |M1⟩, |M2⟩, |M3⟩ to , respectively (This is just one of the cases; see Table 1).
TABLE 1
| |M1⟩ | |M2⟩ | |M3⟩ |
| |M1⟩ | |M3⟩ | |M2⟩ |
| |M2⟩ | |M1⟩ | |M3⟩ |
| |M2⟩ | |M3⟩ | |M1⟩ |
| |M3⟩ | |M1⟩ | |M2⟩ |
| |M3⟩ | |M2⟩ | |M1⟩ |
received the sequence |Mt⟩.
Then, sends to (). In the same way, () sends () to . () holds on its own. Then, gets, gets |S2⟩, and gets |S3⟩. () measures the quantum sequence |S1⟩(|S2⟩, |S3⟩). According to the measurement basis and order of all sequences announced by Na, the secret can be obtained. The specific procedures can be seen in Figure 3.
FIGURE 3
4 Multi-party quantum secret sharing protocol based on X-LIOP states
In this section, we generalize the QSS protocol to any multi-party based on X-LIOP states applied in the quantum network. There are source node (
Na) and
ndestination nodes
. The secrets can be recovered only when the destination nodes cooperate together. The protocol can be described as follows. Here, we denote different
m-bit sequences as
111 ⋯ 111, where
m= ⌊ log
2n⌋.
Step1)Na divides the secret message X into n groups, i.e., x1, …, xn, where .
Step2)Na encodes the secret message X to a quantum sequence |S⟩ according to the following rules accepted by all the nodes:
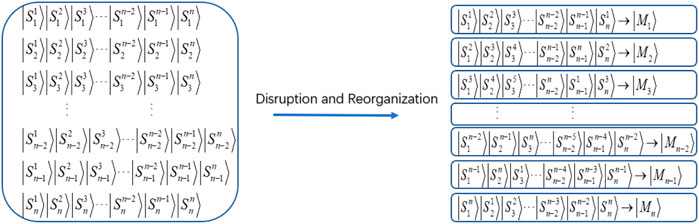
Step3)Na creates n identical sequences |S⟩, where the i-th sequence is denoted by |Si⟩ and i = 1, 2, …, n. Na splits |Si⟩ into n systems, i.e., . Na generates n sequences |M1⟩, |M2⟩, …, |Mn⟩, where , , …, and (Figure 4).
Step4)Na randomly inserts n unencoded orthogonal products into the quantum sequence as the decoy states and generates |Mt⟩′, where t = 1, 2, 3, …, n. Finally, |Mt⟩′ is given to randomly, where l = 1, 2, 3, …, n. Therefore, does not know which particle it gains.
Step5)After getting the sequence |Mt⟩′ from Na, sends an acknowledgment to the sender. Na announces the basis and the positions of the decoy photons in |Mt⟩′, and measures these decoy states. According to the measurement results of , Na checks eavesdropping. If Na does not detect eavesdropping, the protocol will continue to perform the next step. Otherwise, it will stop and restart from Step 1.
Step6)After detecting eavesdropping, gets sequence |Mt⟩, and generates n decoy states that are selected from {| + ⟩, | − ⟩, |0⟩, |1⟩}. Then, decoy states are randomly inserted into the sequence |Mt⟩, and then, the j-th group of particles is sent to , where j = 1, 2 …, n.
Step7)After receiving the sequences from , sends a confirmation. Then, announces the basis and the positions of the decoy photons. According to the measurement results of , performs eavesdropping detection. If no eavesdropping is detected, the protocol will continue to the next step; otherwise, it will stop.
Step8)After the eavesdropping check, gets , i.e., |Sl⟩. Then, the quantum sequence |Sl⟩ is measured under the basis of Eq. 1, and is recovered. Na announces the measurement basis and order of all sequences.
Step9) keep the same particles and perform the same operations. Therefore, if the protocol is effective, and the secret X must be the same. Intuitively, if , the protocol will be effective; otherwise, the protocol fails.
FIGURE 4
5 Security analysis
In this section, we analyze the attack performed by the internal and external malicious nodes.
5.1 Internal attack
Since the internal nodes directly take part in the process of the protocol, the malicious internal nodes can perform more strong attacks than the external ones. Here, we analyzed two types of participant attacks: information leak attacks and forgery attacks.
5.1.1 Information leak attack
Here, we consider information leak attacks and assume that malicious nodes can guess the secret messages together. In order to show that the following three cases are analyzed, without loss of generality, we assume that
rnodes are malicious.
Case 1: Since r malicious nodes conspire, they will send the corresponding particles according to the normal process. Hence, the r particles of r malicious nodes are correctly arranged, and the left (n − r) correct particles are required. As the states of each part come from {| + ⟩, | − ⟩, |0⟩, |1⟩}, the probability of the malicious nodes intuitively guessing one particle is , and the probability of guessing n − r particles is
For the malicious nodes, the successful probability to obtain the secrets is shown in
Figure 5.
Case 2: In the same case, the r malicious nodes can also use other methods to guess the remaining particles. It is observed that the malicious nodes have a total of r × (n − r) particles left in their hands. If malicious nodes want to guess the secrets, they will arrange the remaining r × (n − r) particles correctly, and only one arrangement of particles is correct. Therefore, the successful probability to obtain the secrets is
FIGURE 5
For malicious nodes, the successful probability to obtain the secrets is shown in
Figure 6.
Case 3: Moreover, the r malicious nodes can perform the following different attacks as they give all the particles in their hands to one malicious node. In this sense, the malicious node independently guesses the secrets with the successful probability of
FIGURE 6
For malicious nodes, the successful probability to obtain the secrets is shown in Figure 7.
FIGURE 7
Above all, the probability of malicious nodes guessing the secrets successfully can be shown as
From the analysis mentioned previously, it can be seen for all malicious nodes without all the particles, and the probability to guessing the secrets tends to be 0. According to the property of the LIOP states, our protocol can resist information leak attacks.
5.1.2 Forgery attack
A forgery attack is an easily overlooked but important attack in the QSS protocol. Forgery attack means that malicious nodes can obtain secret messages and successfully forge secret messages so that other nodes get the wrong secret messages. This attack was proposed by Zhang et al. in 2013 [28] and was also mentioned by Sutradhar et al. in 2020 [29]. In the protocol, a forgery attack is also considered. The secrets are encoded as LIOP states, and particles are transmitted between all destination nodes. Therefore, it is possible for malicious nodes to complete the forgery attack.
When malicious nodes change | + ⟩ (| − ⟩) to | − ⟩ (| + ⟩), they have a certain probability to complete the forgery attack. So, the secrets encoded as |ϕ1⟩ (|ϕ4⟩) are forged and are encoded as |ϕ4⟩ (|ϕ1⟩) in Eq. 3. In the multi-party QSS protocol, the secrets are encoded most in the first n states in Eq. 1. The secrets are not encoded in LIOP states with | − ⟩ states. Therefore, this attack is only possible in the four-party QSS protocol.
5.1.2.1 Individual attack
Here, we assume that the malicious node can only perform a forgery attack on its own. When a malicious node gets all and secret messages encoded as |ϕ1⟩or|ϕ4⟩, it can successfully forge secrets 00 to secrets 11 or secrets 11 to secrets 00, as in Eq. 3, i = 1, 2, 3. The probability that the secret messages are encoded as |ϕ1⟩or|ϕ4⟩
Next, we analyze the probability that the malicious node gets all . When we assume that or is a malicious node, we will find that it is impossible for them to get all sequences . Only when it is assumed that is a malicious node and obtains sequence |M3⟩, the individual has a certain probability to acquire all sequences . Therefore, the probability that obtains all is
So, the probability that the individual wants to successfully forge the secrets is
For the n length of the quantum sequences, it is not difficult to see that the probability of the malicious node successfully forging secrets P′ tends to be zero with the increase in n in Eq. 12.
5.1.2.2 Collusion attack
A more serious threat than an individual attack is that some attackers cooperate to forge secrets. Since this attack in this study only exists in the four-party QSS protocol, there are at most two malicious nodes here. When the malicious nodes obtain the secret messages of all sequences and secret messages encoded as |ϕ1⟩ or |ϕ4⟩, the malicious nodes can successfully forge secret messages 00 to secret messages 11 or forge secret messages 11 to secret messages 00. The secret messages are encoded as |ϕ1⟩or|ϕ4⟩ with the probability
We analyze the probability of malicious nodes obtaining all sequences
. A total of three cases were found to be possible to get
.
Case 1: and are malicious nodes.
Case 2: and are malicious nodes.
Case 3: and are malicious nodes.
First, we analyze Case 1, and when they get sequence |M3⟩, they can obtain sequences . The probability of Case 1 is
Next, we see Case 2; when they obtain sequence |M3⟩, the success probability is
Finally, during Case 3, when they receive sequence |M2⟩, the successful probability is
Therefore, the successful probability of malicious nodes forging messages is
For the length of n of the quantum sequences, it is not difficult to see that in Eq. 18, as n increases, the probability of malicious nodes successfully forging secrets tends to be zero, where i = 1, 2, 3.
They want to successfully forge the secret without being discovered is almost impossible. Therefore, the protocol is safe against internal attacks.
5.2 External attack
Unlike internal attackers, external attackers are illegal eavesdroppers from outside. We analyze intercept-replay attacks, intercept-measure-replay attacks, and entangle-measure attacks in the following sections.
5.2.1 Intercept-resend (IR) attack
Eve is an eavesdropper who wants to obtain the secrets of the source node. In order to obtain secrets, he can intercept secrets in Step 4 and Step 6 and complete the attack. Eve prepares large quantities of {| + ⟩, | − ⟩, |0⟩, |1⟩}. Eve intercepts the sequences |Mt⟩ and sends the sequences prepared on his own to at the same time. The probability of Eve guessing one particle is , and the probability of guessing n particles is ; the probability approximates to zero. Therefore, when Na and perform eavesdropping detection, has a high probability of getting wrong measurements, and Na will find that it has eavesdropped. Na will give up sharing secrets, so Eve will not get any secret messages.
5.2.2 Intercept-measure-resend (IMR) attack
Eve receives the sequences |Mt⟩ and measures them in the computational basis. After the measurement, the sequences are resent to . Considering one of the particles in the measured sequence, if measurement basis is the same as Eve′s selection, Eve will get measurement basis, which means Eve will get secrets. However, Eve does not distinguish between secret particles and decoy particles, so they do not get useful secret messages.
Similar to the IR attack and IMR attack, Eve is an external attacker, while in the entanglement and measurement attack, Eve has less information than an internal attacker and, therefore, has a higher probability of failure.
6 Discussion and conclusion
We compare and summarize the QSS protocols based on LIOP states in Table 2. R denotes a rearrangement operation, and H denotes a random three-level Hadamard transform.
TABLE 2
| Protocol | Participant | Local measurement | Operation |
|---|---|---|---|
| Hsu et al. [30] | Three-party | Yes | R, H |
| Yang et al. [22] | Three-party | Yes | R |
| Xu et al. [31] | Three-party | Yes | R, H |
| Our protocol | Multi-party | No | R |
Comparison among some different QSS protocols.
Compared with the existing QSS protocols based on LIOP states, our protocol can be extended to the arbitrary multi-party. In addition, we only use the characteristics of the states themselves to perform arrangement operations and do not require local measurement. In this case, two new QSS protocols based on LIOP states are proposed and may be applied in further quantum networks. The four-party QSS protocol is a special case of the multi-party QSS protocol. However, the secrets are encoded into different forms and attack strategies are different. To improve the efficiency, two more states are introduced in the four-party QSS protocol for encoding. Hence, the necessary forgery attack is discussed. For the multi-party QSS protocol, it is not difficult to see that the forgery attack can be naturally resisted.
In conclusion, combining with the property of LIOP states and obfuscating operation, the source nodes and destination nodes can complete the secret sharing in the quantum network. The destination nodes work together to recover the secrets. Since the LIOP states are more convenient to prepare than the entangled ones, the protocol is easily realized. Moreover, with regard to the property of LIOP states, it is proven that our protocol can be secure against the existing attacks. We hope this can be helpful to the further development of quantum networks.
Statements
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding authors.
Author contributions
Conceptualization, S-JF and K-JZ; methodology, S-JF; software, S-JF; validation, S-JF, K-JZ, LZ, and K-CH; writing—original draft preparation, S-JF; writing—review and editing, S-JF and K-JZ. All authors have read and agreed to the published version of the manuscript.
Funding
This work was supported by the National Natural Science Foundation of China under Grant 61802118 and Natural Science Foundation of Heilongjiang Province under Grant YQ2020F013, LH2019F031. The work of K-JZ was supported by the Advanced Programs of Heilongjiang Province for the overseas scholars and the Outstanding Youth Fund of Heilongjiang University. This research is supported by the Heilongjiang Provincial Key Laboratory of the Theory and Computation of Complex Systems.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1.
ShorPW. Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM Rev Soc Ind Appl Math (1999) 41(2):303–32. 10.1137/S0036144598347011
2.
GroverLK. A fast quantum mechanical algorithm for database search. In: Proceedings of the twenty-eighth annual ACM symposium on Theory of computing (1996). p. 212–9. 10.1145/237814.237866
3.
LongGLLiuXS. Theoretically efficient high-capacity quantum-key-distribution scheme. Phys Rev A (Coll Park) (2002) 65(3):032302. 10.1103/PhysRevA.65.032302
4.
MaHGuoZ. A group quantum communication network using quantum secret sharing. In: 2008 IFIP International Conference on Network and Parallel Computing; 2008 October 18–21; Shanghai, China (2008). p. 549–52. IEEE. 10.1109/NPC.2008.42
5.
ZidanMAbdel-AtyA-HEl-SadekAZanatyEAAbdel-AtyM. Low-cost autonomous perceptron neural network inspired by quantum computation. AIP Conf Proc (2017) 1905:020005. 10.1063/1.5012145
6.
NoorKINoorMAMohamedHM. Quantum approach to starlike functions. Appl Math Inf Sci (2021) 15(4):437–41. 10.18576/amis/150405
7.
BogolyubovNNJr.SoldatovAV. Time-convolutionless master equation for multi-level open quantum systems with initial system-environment correlations. Appl Math Inf Sci (2020) 14(5):771–80. 10.18576/amis/140504
8.
SaidTChouikhABennaiM. N two-transmon-qubit quantum logic gates realized in a circuit QED system. Appl Math Inf Sci (2019) 13(5):839–46. 10.18576/amis/130518
9.
ZidanMAbdel-AtyA-HYounesAEl-khayatIAbdel-AtyM. A novel algorithm based on entanglement measurement for improving speed of quantum algorithms. Appl Math Inf Sci (2018) 12(1):265–9. 10.18576/amis/120127
10.
Abdel-AtyA-HKadryHZidanMZanatyEAAbdel-AtyM. A quantum classification algorithm for classification incomplete patterns based on entanglement measure. J Intell Fuzzy Syst (2020) 38(3):2809–16. 10.3233/JIFS-179566
11.
YeTYLiHKHuJL. Semi-quantum key distribution with single photons in both polarization and spatial-mode degrees of freedom. Int J Theor Phys (Dordr) (2020) 59(9):2807–15. 10.1007/s10773-020-04540-y
12.
YeTYGengMJXuTJChenY. Efficient semiquantum key distribution based on single photons in both polarization and spatial-mode degrees of freedom. Quan Inf Process (2022) 21(4):123. 10.1007/s11128-022-03457-1
13.
HilleryMBuvekVBerthiaumeA. Quantum secret sharing. Phys Rev A (Coll Park) (1999) 59(3):1829–34. 10.1103/PhysRevA.59.1829
14.
KarlssonAKoashiMImotoN. Quantum entanglement for secret sharing and secret splitting. Phys Rev A (Coll Park) (1999) 59(1):162–8. 10.1103/PhysRevA.59.162
15.
XiaoLLongGLDengFGPanJW. Efficient multiparty quantum-secret-sharing schemes. Phys Rev A (Coll Park) (2004) 69(5):052307. 10.1103/PhysRevA.69.052307
16.
QinHDaiY. Dynamic quantum secret sharing by using d-dimensional GHZ state. Quan Inf Process (2017) 16(3):64–13. 10.1007/s11128-017-1525-y
17.
ZhangKJZhangXJiaHYZhangL. A new n-party quantum secret sharing model based on multiparty entangled states. Quan Inf Process (2019) 18(3):81–15. 10.1007/s11128-019-2201-1
18.
MansourMDahbiZ. Quantum secret sharing protocol using maximally entangled multi-qudit states. Int J Theor Phys (Dordr) (2020) 59(12):3876–87. 10.1007/s10773-020-04639-2
19.
HuWZhouRGLiXFanPTanC. A novel dynamic quantum secret sharing in high-dimensional quantum system. Quan Inf Process (2021) 20(5):159–28. 10.1007/s11128-021-03103-2
20.
YuSOhCH. Detecting the local indistinguishability of maximally entangled states (2015). arXiv preprint arXiv:1502.01274. Available at: https://arxiv.org/abs/1502.01274 (Accessed Feb 4, 2015).10.48550/arXiv.1502.01274
21.
GuoGPLiCFShiBSLiJGuoGC. Quantum key distribution scheme with orthogonal product states. Phys Rev A (Coll Park) (2001) 64(4):042301. 10.1103/PhysRevA.64.042301
22.
YangYWenQZhuF. An efficient quantum secret sharing protocol with orthogonal product states. Sci China Ser G: Phys Mech Astron (2007) 50(3):331–8. 10.1007/s11433-007-0028-8
23.
JiangDHWangJLiangXQXuGBQiHF. Quantum voting scheme based on locally indistinguishable orthogonal product states. Int J Theor Phys (Dordr) (2020) 59(2):436–44. 10.1007/s10773-019-04337-8
24.
JiangDHHuQZLiangXQXuGB. A trusted third-party E-payment protocol based on locally indistinguishable orthogonal product states. Int J Theor Phys (Dordr) (2020) 59(5):1442–50. 10.1007/s10773-020-04413-4
25.
WalgateJHardyL. Nonlocality, asymmetry, and distinguishing bipartite states. Phys Rev Lett (2002) 89(14):147901. 10.1103/PhysRevLett.89.147901
26.
XuGBWenQYQinSJYangYHGaoF. Quantum nonlocality of multipartite orthogonal product states. Phys Rev A (Coll Park) (2016) 93(3):032341. 10.1103/PhysRevA.93.032341
27.
FengYShiY. Characterizing locally indistinguishable orthogonal product states. IEEE Trans Inf Theor (2009) 55(6):2799–806. 10.1109/TIT.2009.2018330
28.
ZhangKQinS. The Cryptanalysis of Yuan et al.’s Multiparty Quantum Secret Sharing Protocol. Int J Theor Phys (Dordr) (2013) 52(11):3953–9. 10.1007/s10773-013-1706-0
29.
SutradharKOmH. Efficient quantum secret sharing without a trusted player. Quan Inf Process (2020) 19(2):73–15. 10.1007/s11128-019-2571-4
30.
HsuLYLiCM. Quantum secret sharing using product states. Phys Rev A (Coll Park) (2005) 71(2):022321. 10.1103/PhysRevA.71.022321
31.
XuJChenHWLiuWJLiuZH. An efficient quantum secret sharing scheme based on orthogonal product states. In: IEEE Congress on Evolutionary Computation; 2010 July 18–23; Barcelona, Spain (2010). p. 1–4. IEEE. 10.1109/CEC.2010.5586410
Summary
Keywords
quantum secret sharing, quantum network, quantum secure communication, orthogonal product states, quantum cryptography
Citation
Fu S-J, Zhang K-J, Zhang L and Hou K-C (2022) A new non-entangled quantum secret sharing protocol among different nodes in further quantum networks. Front. Phys. 10:1021113. doi: 10.3389/fphy.2022.1021113
Received
17 August 2022
Accepted
30 September 2022
Published
24 October 2022
Volume
10 - 2022
Edited by
Xiubo Chen, Beijing University of Posts and Telecommunications (BUPT), China
Reviewed by
Mahmoud Abdel-Aty, Sohag University, Egypt
Song Lin, Fujian Normal University, China
Cai Zhang, South China Agricultural University, China
Updates
Copyright
© 2022 Fu, Zhang, Zhang and Hou.
This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Ke-Jia Zhang, zhangkejia@hlju.edu.cn; Long Zhang, lzhang@hlju.edu.cn
†These authors contributed equally to this work
This article was submitted to Quantum Engineering and Technology, a section of the journal Frontiers in Physics
Disclaimer
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.