Design of a Multilayer Dual-Band Balanced Bandpass Filter on a Circular Patch Resonator

This letter presents a novel multilayer dual-band balanced bandpass filter (BPF) design by using two perturbed circular patch resonators. The TM₁₁ mode and TM₂₁ mode of the resonator with odd-symmetric field distributions are explored to realize the desired differential-mode (DM) transmission and common-mode (CM) suppression. Two circular patches are properly coupled in the back-to-back form to realize a dual-passband balanced response by virtue of coupling apertures etched on the ground. In addition to the internal coupling, the above apertures are also further utilized for the undesired degenerate mode harmonic suppression. Besides, slot perturbations on the patch are introduced to perturb the TM₂₁ resonant mode to independently adjust the center frequency of the higher passband, while the lower passband remains almost unchanged. Thus, two passbands can be flexibly controlled by simultaneously tuning the above slots and size of the patch. For validation, a dual-band balanced BPF prototype is implemented. The results indicate 18 and 26% wide fractional bandwidths centered at 5.5 and 7.5 GHz with return loss higher than 20 dB under DM operation and CM suppression higher than 40 dB over an ultra-wide frequency band.

Introduction

Balanced filters play key roles in modern wireless communication systems, attributing to their superior immunity to the electromagnetic interference and environmental noise [1]. In the meantime, great development of modern wireless communication systems brings out an increased requirement for dual-band operations. To the end, dual-band balanced bandpass filters (BPFs) are desired.

Accordingly, much efforts have been made to explore a variety of high-performance dual-band balanced BPFs by using different transmission line structures, such as planar microstrip transmission line resonators [2–5], substrate-integrated waveguide (SIW) resonators [6–9], and dielectric resonators (DRs) [10]. On the contrary, the patch-type resonators are attracting much attention in balanced BPF designs due to their superior advantages of higher power handling capability and lower loss over the transmission line–based resonators and simpler and more straightforward analysis and design compared with the SIW and DR forms. However, to the best of our knowledge, only limited works have been carried out on design of single-band balanced BPFs, e.g., square patch resonator–based balanced BPFs [11–13] and triangular patch resonator–based balanced BPFs [14–16]. How to design a dual-band patch balanced BPF is still rarely reported and remains challenging.

This letter is aimed at presenting a new dual-band balanced BPF design on a circular patch resonator. For this purpose, the TM₁₁ mode and TM₂₁ mode resonant properties of a circular patch are carefully analyzed and investigated with both differential-mode (DM) excitation and common-mode (CM) excitation. Two vertically coupled circular patches, via proper coupling apertures etched on the common ground, are explored to realize two passbands under DM operation. Furthermore, slot perturbations on the patch are designed to adjust the frequency ratio of the two passbands and attain high CM suppression. In order to demonstrate the design concept, a circular patch dual-band balanced filter prototype was designed, manufactured, and tested. Measured and simulated results well coincide with each other.

Design and Analysis of the Proposed Dual-Band Balanced Filter

Figures 1A–E describe the configuration of the proposed dual-band balanced BPF. Two layers of RO4003C substrates (ε_r = 3.55, h = 0.508 mm, and tanδ = 0.0027) are used. Two differential input ports (Ports 1 and 1′) are placed at the top layer, while two output ports (Ports 2 and 2′) are at the bottom layer. Two coupling apertures of length l₂ and width w₂ are arranged along the main diagonal line (A–B) on the square common ground of Figure 1D. Besides, two perturbation slots with length l₁ and width w₁ are placed along the diagonal line (C–D) of a circular patch with radius r as shown in Figures 1C,E. The dual-band response of the proposed balanced BPF is realized by the utilization of two diagonal modes: TM₁₁ mode (Mode 1) and TM₂₁ mode (Mode 3), in the patch resonator. In this context, the first DM passband is made up of a pair of TM₁₁ modes (Mode 1), while the second DM passband is formed by two TM₂₁ modes (Mode 3) from the two top- and bottom-layer patches, respectively. Detailed working mechanisms are illustrated as follows.

FIGURE 1

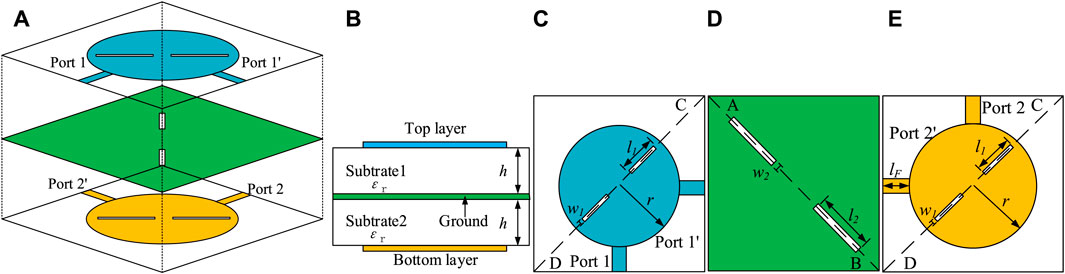
FIGURE 1. (A) 3D view of the proposed dual-band balanced filter, (B) side view, (C) top layer, (D) middle layer, and (E) bottom layer.

Figure 2 and Figure 3 indicate simulated electrical and magnetic fields of the first three resonant modes in the circular patch: a pair of degenerate TM₁₁ modes (Mode 1 and Mode 2) and TM₂₁ mode (Mode 3), respectively. As observed in Figure 2, the electrical-field patterns of these modes are odd-symmetric inside the patch. In other words, almost same intensity and opposite direction can be found with respect to the corresponding symmetric plane. Therefore, when input ports 1 and 1′ are injected with DM signals, the fields of the TM₁₁ mode (Mode 1) and TM₂₁ mode (Mode 3) can be simultaneously excited in the top-layer patch. Meanwhile, the magnetic-field intensity around regions (Area 1 and Area 3) in Figure 3 is high for both the TM₁₁ mode (Mode 1) and the TM₂₁ mode (Mode 3).

FIGURE 2

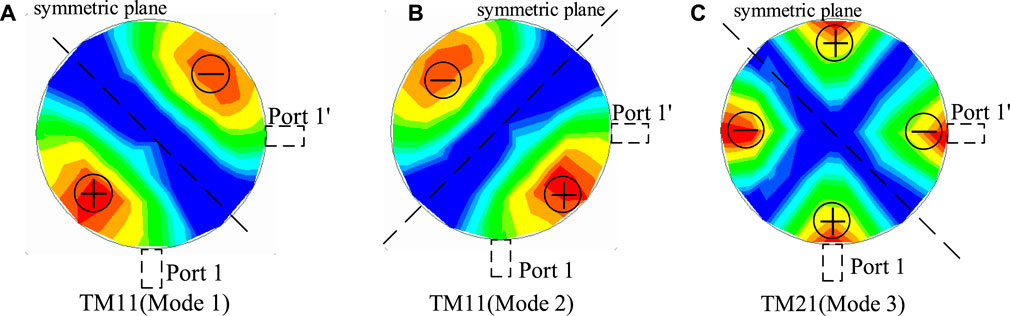
FIGURE 2. Simulated E-fields of the resonant modes in the circular patch. (A) TM₁₁ mode (Mode 1), (B) TM₁₁ mode (Mode 2), and (C) TM₂₁ mode (Mode 3).

FIGURE 3

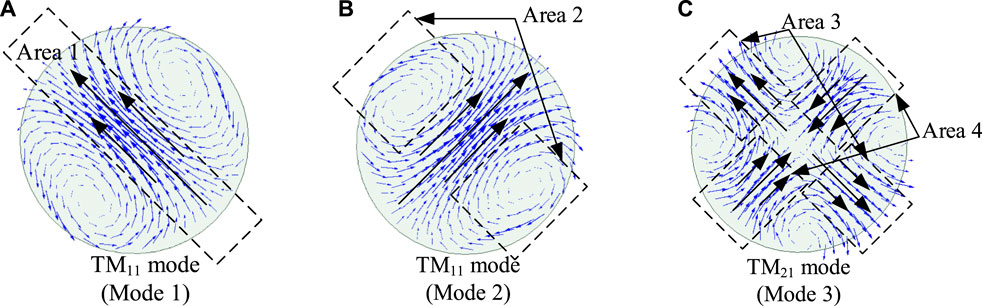
FIGURE 3. Simulated H-fields of the resonant modes in the circular patch. (A) TM₁₁ mode (Mode 1), (B) TM₁₁ mode (Mode 2), and (C) TM₂₁ mode (Mode 3).

Two coupling apertures placed in the above regions are utilized for the internal coupling of both passbands in a vertically stacked form. Accordingly, the same field patterns from the TM₁₁ mode (Mode 1) and TM₂₁ mode (Mode 3) on the bottom-layer patch will be excited. When the feeding output ports are chosen to place at two sides of the symmetrical plane as Ports 2 and 2′, balanced outputs can be attained for both the TM₁₁ mode (Mode 1) and the TM₂₁ mode (Mode 3) in the bottom-layer patch.

It should be mentioned that, under CM excitation, the TM₁₁ mode (Mode 1) and TM₂₁ mode (Mode 3) cannot be supported on the patches, while the TM₁₁ mode (Mode 2) can be activated. Thanks to the fields of Mode 2 that are weak around coupling apertures as shown in Figure 3, the CM signals can hardly be coupled to the bottom-layer resonator. Therefore, the CM suppression will be obtained with this structure.

Based on the above analysis, the corresponding coupling scheme is depicted for the dual-band balanced filter, as shown in Figure 4. In the scheme, 1^A and 2^A represent the coupled TM₁₁ modes (Mode 1) of the top and bottom patch resonators, respectively, to form the first passband. On the contrary, 1^B and 2^B denote the coupled TM₂₁ modes of the top and bottom patch resonators for the second passband. As is known, for the intact circular patch, the resonant frequency (f_nm) of each mode (TM_nm) is determined by the radius r of the circular patch, and the formula is provided as follows [17]:

$f_{nm}=\frac{c{x}_{nm}}{2\pi a\sqrt{{\epsilon }_r}},(1)$

$x_{11}=1.841184,x_{21}=3.054237,(2)$

$a=r{\left[1+\frac{2h}{\pi r{\epsilon }_r}\left(\ln \frac{\pi r}{2h}+1.7726\right)\right]}^{\frac{1}{2}},(3)$

where c is the speed of light.

FIGURE 4

FIGURE 4. Coupling scheme for the dual-band balanced filter.

According to Eqs. 1–3, the two resonant frequencies of the interested modes for the dual-band balanced BPF are simultaneously changed with various radii r. Therefore, it is impossible to independently control two passbands by just changing the radius r. To further improve the flexibility of the design, two slot perturbations on the patch are carefully introduced in the patches as shown in Figure 1 to perturb the TM₂₁ (Mode 3) resonant mode while seldom influencing the TM₁₁ mode (Mode 1), according to the magnetic-field patterns in Figure 3. Therefore, the higher passband can be independently tuned, while the lower passband remains almost unchanged. In other words, two passbands can be flexibly controlled by simultaneously tuning the above slots and size of the patch. For more clear illustration, Figure 5 provides the effect of changing the length of the slot l₁ on the passbands. It can be seen that the first passband stays remained, while the second passband shifts to lower frequency with an increase in slot length l₁.

FIGURE 5

FIGURE 5. Simulated S₂₁ of the filter against varied l₁.

Herein, it is worth mentioning that, with the involvement of the introduced slot perturbations, the field distributions of the TM₁₁ mode (Mode 2) will be affected. As such, the resonant frequency of the TM₁₁ mode (Mode 2) under CM excitation will be shifted away from that of the TM₁₁ mode (Mode 1) under DM excitation. For better understanding of this principle, Figure 6 gives the performance of CM suppressions with and without slot perturbations on the bottom and top patches. As can be seen, due to slot perturbations, the resonant frequency of the TM₁₁ mode (Mode 2) is shifted from 6.0 to 4.5 GHz when compared to the design without slots. The CM suppression has been highly enhanced from 19 to 40 dB for the lower passband, while it remains above 48 dB for the second passband in both cases.

FIGURE 6

FIGURE 6. Simulated frequency responses with and without slot lines on top and bottom metal layers.

To verify this design concept, a dual-band balanced BPF that can operate at the center frequencies of f₁ = 5.5 GHz and f₂ = 7.5 GHz with bandwidths BW₁ = 1,000 MHz and BW₂ = 2,000 MHz, and in-band return loss higher than 20 dB, is designed. The corresponding design parameters are calculated as M^Ⅰ₁₂ = 0.037, Q^Ⅰ_ext = 32.6 and M^Ⅱ₁₂ = 0.049, Q^Ⅱ_ext = 24.4. Superscripts I and II denote the two passbands, respectively. Firstly, the initial value of the radius r is determined by the center frequency f₀₁ of the first passband and Eqs. 1–3. Secondly, the length l₁ of the slot is determined by the center frequency f₀₂ of the second passband. Thirdly, the final parameters of the feedline (l_F), slots (w₁), and internal coupling apertures (w₂ and l₂) are optimized to achieve a compromise for calculated external quality factors (Q_ext) and internal coupling coefficients (M_ij) of both passbands. Herein, it is worth mentioning that as the internal coupling is obtained using the same coupling apertures for both passbands, the two passband bandwidths will not be able to be independently controlled.

Measurement and Discussions

Based on the given specifications and design procedures discussed above, the size of the proposed dual-band balanced filter can be determined as follows: r = 8.0, l₁ = 6.0, l₂ = 4.9, l_F = 5, w₁ = 0.3, and w₂ = 0.68 (unit: mm). A dual-band balanced filter is designed and manufactured. Simulation and measurement results are shown in Figure 7. The measured results show that the CFs are 5.5 and 7.5 GHz and the 3 dB BWs are 1.0 GHz (18%) and 2.0 GHz (26%). In the two passbands, the measured insertion loss is both less than 1.4 dB and the return loss is both higher than 22 dB. Common-mode inhibition is higher than 40 dB.

FIGURE 7

FIGURE 7. Simulated and measured results.

Table 1 compares the proposed dual-band balanced filter with other state-of-the-art designs. The present work not only exhibits a new effective design method for a dual-band balanced patch filter but also achieves nice operation performance in terms of much wider bandwidths, independently controllable center frequencies, better return loss, competitive common-mode suppression, compact size, etc.

TABLE 1

TABLE 1. Comparison with other previous works.

Conclusion

A new dual-band balanced patch filter has been designed and implemented in this letter. The resonant modes of circular patch TM₁₁ mode and TM₂₁ mode are explored to design the dual-band balanced filter. By wisely etching coupling apertures on the ground and introducing slots on the patch, a nice controllable dual-band response and improved CM suppression are attained in the design. Measured results coincide well with simulated ones, verifying the proposed design concept. It is our belief that this design has a prospect of broad application in the application of wireless communication systems.

Data Availability Statement

The original contributions presented in the study are included in the article/supplementary material, and further inquiries can be directed to the corresponding author.

Author Contributions

YX conducted extensive analysis and wrote this paper. ZL gave assistance in the measurement. SW and TC revised this paper. JC contributed to the funding support.

Funding

This work was supported by the National Natural Science Foundation of China (No. 61802055) and the Fundamental Research Funds for the Central Universities (No. N2019001).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

1. Roberto G, G, José-María M, F, Li Y, Dimitra P. Contiguous-channel Dual-Band Balanced Diplexer. IEEE Microw Wirel Compon Lett (2019) 29(5):318–20. doi:10.1109/lmwc.2019.2904396

CrossRef Full Text | Google Scholar

2. Wei F, Jay Guo Y, Qin P-Y, Wei Shi X. Compact Balanced Dual- and Tri-band Bandpass Filters Based on Stub Loaded Resonators. IEEE Microw Wireless Compon Lett (2015) 25(2):76–8. doi:10.1109/lmwc.2014.2370233

CrossRef Full Text | Google Scholar

3. Wu X, Wan F, Ge J. Stub-loaded Theory and its Application to Balanced Dual-Band Bandpass Filter Design. IEEE Microw Wireless Compon Lett (2016) 26(4):231–3. doi:10.1109/lmwc.2016.2537045

CrossRef Full Text | Google Scholar

4. Song Y, Liu HW, Zhao W, Wen P, Wang Z. Compact Balanced Dual-Band Bandpass Filter with High Common-Mode Suppression Using Planar Via-free CRLH Resonator. IEEE Microw Wireless Compon Lett (2018) 28(11):996–8. doi:10.1109/lmwc.2018.2873240

CrossRef Full Text | Google Scholar

5. Ren B, Ma Z, Liu H, Ohira M, Guan X, Wen P. Design of Balanced Dual-Band Superconducting Bandpass Filter with High Selectivity and Deep Common-Mode Suppression. Pro Asia-pacific Micro Confer (2018) 423–5. doi:10.23919/apmc.2018.8617383

CrossRef Full Text | Google Scholar

6. Xu X, Wang J, Zhang G, Chen J. Design of Balanced Dual‐band Bandpass Filter Based on Substrate Integrated Waveguide. Electron Lett (2013) 49(20):1278–80. doi:10.1049/el.2013.2371

CrossRef Full Text | Google Scholar

7. Li P, Chu H, Zhao D, Chen RS. Compact Dual-Band Balanced SIW Bandpass Filter with Improved Common-Mode Suppression. IEEE Microw Wireless Compon Lett (2017) 27(4):347–9. doi:10.1109/lmwc.2017.2678428

CrossRef Full Text | Google Scholar

8. Shen Y, Wang H, Kang W, Wu W. Dual-band SIW Differential Bandpass Filter with Improved Common-Mode Suppression. IEEE Microw Wireless Compon Lett (2015) 25(2):100–2. doi:10.1109/lmwc.2014.2382683

CrossRef Full Text | Google Scholar

9. Zhou K, Kang W, Wu W. Compact Dual‐band Balanced Bandpass Filter Based on Double‐layer SIW Structure. Electron Lett (2016) 52(18):1537–9. doi:10.1049/el.2016.1968

CrossRef Full Text | Google Scholar

10. Chen J-X, Zhan Y, Qin W, Bao Z-H, Xue Q. Novel Narrow-Band Balanced Bandpass Filter Using Rectangular Dielectric Resonator. IEEE Microw Wireless Compon Lett (2015) 25(5):289–91. doi:10.1109/lmwc.2015.2409805

CrossRef Full Text | Google Scholar

11. Janković N, Bengin V, C. Balanced Bandpass Filter Based on Square Patch Resonators. Pro. 12th Int. Conf. Telecom. Mod. Satell., Cable Broadcast. Services (TELSIKS); 14-17 Oct. 2015; Nis, Serbia,IEEE (2015) 14–7. doi:10.1109/telsks.2015.7357766

CrossRef Full Text | Google Scholar

12. Liu Q, Wang J, Zhu L, Zhang G, Wu W. Design of a New Balanced-To-Balanced Filtering Power Divider Based on Square Patch Resonator. IEEE Trans Microwave Theor Techn. (2018) 66(12):5280–9. doi:10.1109/tmtt.2018.2871180

CrossRef Full Text | Google Scholar

13. Zheng S, Wu R, Liu Z. A Balanced Bandpass Filter with Two Transmission Zeros Based on Square Patch Resonators. Proc. IEEE Int. Conf. Ubiquitous Wireless Broadband (ICUWB); 16-19 Oct. 2016; Nanjing, China, IEEE (2016) 1–3. doi:10.1109/icuwb.2016.7790443

CrossRef Full Text | Google Scholar

14. Liu Q, Wang J, Zhang G, Zhu L, Wu W. A New Design Approach for Balanced Bandpass Filters on Right-Angled Isosceles Triangular Patch Resonator. IEEE Microw Wireless Compon Lett (2019) 29(1):5–7. doi:10.1109/lmwc.2018.2884829

CrossRef Full Text | Google Scholar

15. Liu Q, Wang J, He Y. Compact Balanced Bandpass Filter Using Isosceles Right Triangular Patch Resonator. Electron Lett (2017) 53(4):253–4. doi:10.1049/el.2016.2715

CrossRef Full Text | Google Scholar

16. Wu RT, Zheng SY, Feng WJ, Li YX, Long YL. Design of Balanced Filtering Components Based on Isosceles Right-Angled Triangular Patch. IEEE Trans Compon., Packag Manufact Technol (2019) 9(4):736–44. doi:10.1109/tcpmt.2018.2872588

CrossRef Full Text | Google Scholar

17. Chakravarty T, De A. Design of Tunable Modes and Dual-Band Circular Patch Antenna Using Shorting Posts. IEE Proc Microw Antennas Propag (1999) 146(3):224–8. doi:10.1049/ip-map:19990629

CrossRef Full Text | Google Scholar

18. Gupta T, Akhtar MJ, Biswas A. Dual-mode Dual-Band Compact Balanced Bandpass Filter Using Square Patch Resonator, Proc. Asia-Pacific Micro Conf. (APMC); 5-9 Dec. 2016; New Delhi, India,IEEE (2016). doi:10.1109/apmc.2016.7931391

CrossRef Full Text | Google Scholar