Waves that Appear From Nowhere: Complex Rogue Wave Structures and Their Elementary Particles

The nonlinear Schrödinger equation has wide range of applications in physics with spatial scales that vary from microns to kilometres. Consequently, its solutions are also universal and can be applied to water waves, optics, plasma and Bose-Einstein condensate. The most remarkable solution presently known as the Peregrine solution describes waves that appear from nowhere. This solution describes unique events localized both in time and in space. Following the language of mariners they are called “rogue waves”. As thorough mathematical analysis shows, these waves have properties that differ them from any other nonlinear waves known before. Peregrine waves can serve as ‘elementary particles’ in more complex structures that are also exact solutions of the nonlinear Schrödinger equation. These structures lead to specific patterns with various degrees of symmetry. Some of them resemble “atomic like structures”. The number of particles in these structures is not arbitrary but satisfies strict rules. Similar structures may be observed in systems described by other equations of mathematical physics: Hirota equation, Davey-Stewartson equations, Sasa-Satsuma equation, generalized Landau-Lifshitz equation, complex KdV equation and even the coupled Higgs field equations describing nucleons interacting with neutral scalar mesons. This means that the ideas of rogue waves enter nearly all areas of physics including the field of elementary particles.

1 Introduction

The nonlinear Schrödinger equation (NLSE) has wide range of applications in physics with spatial scales that vary from microns to kilometres and even light years. It describes nonlinear wave phenomena in optics [1, 2], oceanography [3, 4], plasmas [5, 6], atmosphere [7], Bose-Einstein condensate [8, 9] and cosmology [10]. Taking into account the lowest order nonlinearity and dispersion, this equation describes nonlinear wave phenomena at the fundamental level. NLSE serves as a basic tool for understanding modulation instability [11, 12], solitons [13], periodic waves [14] and extreme waves [15, 16]. The ideas born in the studies of NLSE solutions can be transferred to many other systems. Despite being studied for nearly 50 years, the NLSE solutions have a rich structure and provide surprises for researchers even today [17].

Being a practical introduction to a special issue, this article provides a basic review of mathematical results on NLSE that are important for understanding the nonlinear phenomena in general. It leaves aside the complexities of inverse scattering technique [13], Darboux transformation [18], theta functions [19] and other sophistications of modern mathematics [20]. Instead, it provides solutions in explicit form so that everyone can appreciate their clarity, simplicity of making useful plots and most importantly, the possibility of using them in applications.

The NLSE describes systems with an infinite number of degrees of freedom. Being integrable, it has an infinite number of solutions that can be presented in analytic form. Among them, there are fundamental ones such as soliton solutions [13], Akhmediev breathers [21–24], the Peregrine solution [26–28] and the general doubly-periodic solutions [17, 22]. These can be considered as fundamental modes of the nonlinear system each with a specific single eigenvalue of the inverse scattering transform [13]. More complicated solutions are nonlinear superpositions of these fundamental ones. Although mathematically, these superpositions may look highly complicated, conceptually, they can be understood as a combination of fundamental solutions. The corresponding spectrum of eigenvalues for complex solutions is a combined set of individual eigenvalues.

Presently, the most studied combinations are multi-soliton solutions [13, 29]. Next in complexity are multi-Akhmediev breathers [25, 31]. Rogue waves can also be superimposed resulting in multi-rogue wave solutions. In contrast to multi-solitons and multi-ABs, multi-rogue waves are degenerate solutions. The eigenvalues corresponding to the individual contributions are located at the same point of the complex plane. Despite this complication, multi-rogue waves are also well studied [32–34] but, perhaps, their physics is less understood [35–37] than the physics of any other solutions of the NLSE. The main reason is the unusual set of rules that control their superposition [35]. On the other hand, the superpositions of elementary doubly-periodic solutions studied in [17, 22] still need to be constructed. This task is mathematically challenging and has not been addressed so far. Existence of “explicit” solutions in terms of theta functions [19] does not provide any clue for solving this highly involved task.

Building higher-order superpositions consisting of the same type of fundamental solutions, say, multi-soliton solutions is relatively simple task [38]. This may be done using techniques such as Darboux transformation [30]. Mixing different types of fundamental solutions is more difficult. However, this has also been done in a few recent works. Mixing them is the way to address problems such as rogue waves on top of a periodic background [39, 40]. The number of possibilities is literally infinite.

After what is said above, it may seem strange that the NLSE model is the simplest one among the existing nonlinear evolution equations. However, this is indeed the case. The NLSE provides the conceptual background for further developments in the science of rogue waves. We should keep in mind that the first known integrable equation which is the real KdV equation [41] does not have rogue wave solutions. It cannot be used as a mathematical platform for rogue wave research. On the contrary, the ideas developed in the studies of NLSE solutions can be further extended to many other systems. These include Hirota equation [42], Sasa-Satsuma equation [43], Davey-Stewartson equations [44–46], Sine-Gordon equation [47], Landau-Lifschitz equation [48] and many others. The basic concepts are valid not only for integrable equations but can be expanded to non-integrable cases [49, 50] and, to some extent, to dissipative systems [51, 52].

The generality of the concept of rogue waves can be further expanded to extreme events in nature. Clearly, the equations that describe natural phenomena are more complex than the NLSE [53]. Nevertheless, these are also evolution equations that can be solved if not analytically, then numerically [54]. Rogue waves must be part of complex evolution of the system with either regular or chaotic initial conditions. These rogue waves may take more complicated forms than a simple Peregrine wave. They can take the form of tornadoes or hurricanes. These are also formations that “appear from nowhere”. Thus, they do belong to the class of rogue waves or “extreme events”.

Our task here is well defined by the subject of the special issue. Therefore, we will concentrate on the Peregrine wave, its analogs and its higher-order combinations. This is a very small subset of the whole set of multi-parameter families of solutions of the NLSE. Nevertheless, this subset plays an important role in explaining extreme events in many physical situations. Understanding variety of complex phenomena starts with the studies of simple examples. These simple examples are listed in the present rendition.

2 NLSE

The relative simplicity of the nonlinear Schrödinger equation, its integrability [13] and its applicability to many weakly nonlinear dispersive systems made it a universal model for wave propagation. The most common applications include deep ocean water waves [3, 4] and waves in an optical fiber [1, 2]. Universality means that this equation can be written in a standard form that is applicable to all major physical settings. Variables in this form are dimensionless and there are no free parameters. Namely,

$i\frac{\partial \psi }{\partial x}+\frac{1}{2}\frac{{\partial }^2\psi }{\partial t^2}+{\left|\psi \right|}^2\psi =0(1)$

Here, we consider x as the propagation distance and t as the retarded time in a reference frame moving with the group velocity. The function ψ means the envelope of the wave packet. Being a complex function, it defines both, the amplitude of the envelope and the phase shift of the carrier wave.

For a given central frequency of narrow banded waves, the group velocity of the waves is well defined by the dispersion relation. In this case, the propagation distance and time in the moving frame are linearly related. This means that the time and the distance can be easily exchanged in Eq. 1 [4]. This replacement creates an alternative form of the equation that has been used in the earlier descriptions of water wave propagation [4]. Evolution in time is also convenient in problems related to Bose-Einstein condensate. For experiments in water tanks and in optical fibers, it is more convenient to stick to the notations taken in Eq. 1. Then waves are evolving along the tank or along the fiber and the shape of the wave envelope can be observed moving along with the wave packet.

All solutions below will be given in dimensionless form that directly satisfy Eq. 1. For practical applications, solutions must be rescaled to dimensional variables, i.e. they must be expressed in meters (kilometres) along the tank or along the fiber, in seconds for the transverse variable and in the amplitude units for ψ. In the case of an optical fiber, this rescaling is given by:

$X=L_{NL}\times x,T=\sqrt{\left|{\beta }_2\right|L_{NL}}\times t,\text{Ψ}=\sqrt{P_P}\times \psi ,(2)$

where $L_{NL}={\left(\gamma P_P\right)}^{-1}$ is the nonlinear length, γ is the nonlinear coefficient defined by the material of the fiber, ${\beta }_2$ is the group velocity dispersion defined by the fiber design, and $P_P$ is the pump power. Coefficients in the rescaling can be transferred to the equation or used only with the transformation (2). The latter choice is more convenient as it allows us to keep the NLSE to be a universal model.

In the case of waves in deep water the rescaling takes the form:

$X=\frac{x}{k{\xi }^2},T=\sqrt{2}\frac{t}{\omega \xi },\text{Ψ}=\frac{\psi \xi }{k},(3)$

where X is dimensional distance along the tank, T is dimensional time in the frame moving with the group velocity $c_g=\frac{\omega }{2k}$, $\text{Ψ}$ is the envelope of the water wave elevation, ω is the carrier frequency and k is carrier wavenumber that satisfies the dispersion relation $\omega =\sqrt{gk}$ with $g=9.81$ m/s² being the gravitational acceleration. Dimensionless parameter ξ is the wave steepness defined as the product of the wave amplitude a and the wavenumber k.

Further adjustment of solutions can be done with the use of scaling transformation

${\psi }^{\prime }\left(x,t\right)=\alpha \psi \left(\alpha x,{\alpha }^{2}t\right)(4)$

Namely, if $\psi \left(x,t\right)$ is a solution of the NLSE (1), then ${\psi }^{\prime }$ is also a solution of the same equation. Eq. 4 provides an additional tool for adjustment of initial conditions to the required levels in optical and hydrodynamic experiments.

3 Peregrine Wave

One of the simplest non-singular rational solutions of the NLSE 1) is given by [15, 16, 26]:

$\psi \left(x,t\right)=\left[4\frac{1+2ix}{1+4x^2+4t^2}-1\right]e^{ix},(5)$

It is known as the Peregrine solution or Peregrine soliton [27] or Peregrine breather [28]. The modulus of this complex solution is shown in Figure 1. The main feature of this solution is the localization of its central peak both in time and in space. The constant background represents a plane wave with an infinite source of energy. This solution has all features of rogue waves in the ocean [15, 16]. It represents an unexpected wave event on an otherwise flat background.

FIGURE 1

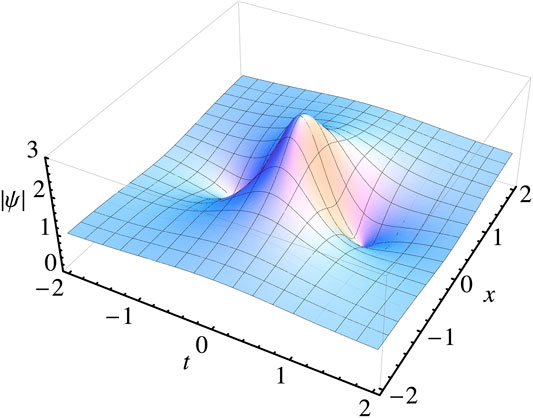
FIGURE 1. A single Peregrine wave of the NLSE [15]. The maximum amplitude at the origin is three while the background amplitude is 1. The solution has two zeros along the t axis at each side of the maximum. This simple wave structure appears in more complicated rogue wave patterns.

Peregrine solution has been observed in various experiments. The most notable ones are in water waves [55] and in optical fibers [27]. This solution appears in field evolution dynamics with variety of initial conditions [56]. Moreover, the Peregrine solution can be considered as a universal structure emerging in any type of intensity localization of high power pulses [57]. We can consider it as an “elementary particle” of more complicated patterns that can appear on a plane wave background. As the NLSE is the envelope equation, the Peregrine solution may describe both the wave of elevation or a depression. The latter is known as the rogue wave hole [58]. Solutions similar to the Peregrine one can also be found in other physical systems [5960–62]. Thus, the phenomenon of rogue wave is even more universal than we can imagine.

4 HIGHER-ORDER Rogue Wave Solutions

There are higher-order rational solutions of the NLSE that we can call rogue waves. The second order solution has been first presented in [14]. Several methods are known for constructing higher-order solutions of integrable equations [18, 32–34]. A hierarchy of rogue wave solutions with progressively increasing central amplitudes are presently known as Akhmediev-Peregrine (AP) breathers [636465–66]. Their general form can be written as:

${\psi }_N\left(x,t\right)=\left[{\left(-1\right)}^N+\frac{G_N\left(x,t\right)+ix{H}_N\left(x,t\right)}{D_N\left(x,t\right)}\right]e^{ix},(6)$

where G, H and D are polynomials, and N is the order of the solution. In the case of the Peregrine solution (5), $N=1$ and we have: $G_1\left(x,t\right)=4,H_1\left(x,t\right)=8,D_1\left(x,t\right)=1+4x^2+4t^2.$

For the second order solution, the polynomials are given by [14, 67]:

$\begin{array}{l}{G}_2=\frac{3}{8}-3t^2-2t^4-9x^2-10x^4-12t^2{x}^2,\\ H_2=\frac{15}{4}+6t^2-4t^4-2t^4-2x^2-4x^4-8t^2{x}^2,\\ D_2=\frac{1}{8}[\frac{3}{4}+9t^2+4t^4+\frac{16}{3}{t}^6+33x^2+36x^4+\frac{16}{3}{x}^6-24t^2{x}^2+16t^4{x}^2+16t^2{x}^4].\end{array}$

This solutions is shown in Figure 2A. While for the first order solution shown in Figure 1 the amplitude is 3 times the background, the maximum amplitude of the second order solution shown in Figure 2A is 5 times the background.

FIGURE 2

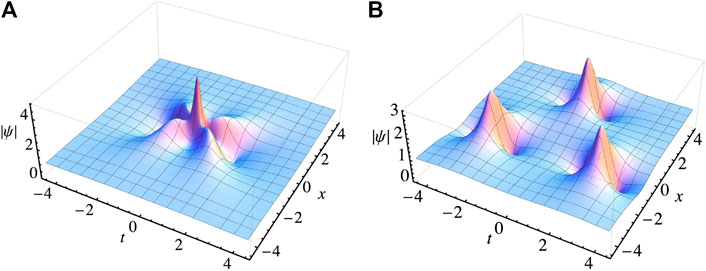
FIGURE 2. (A) AP solution of the second order [15]. (B) Rogue wave triplet with parameters $\gamma =200$ and $\beta =0$ [73]. Here, the second order solution consists of three Peregrine waves.

The second-order solution with free parameters has been given earlier in [32, 73]. The solution intensity ${\left|\psi \right|}^2$ is completely defined by the denominator $D_2\left(x,t\right)$. Namely, $|{\psi }_2\left(x,t\right){|}^2=1+{\left[\text{log}\left(D_2\left(x,t\right)\right)\right]}_{tt}$ [71]. The latter is now given by:

$\begin{array}{c}{D}_2\left(x,t\right)={\beta }^2+{\gamma }^2+64t^6+48t^4\left(4x^2+1\right)-16\beta t^3+12t^2\left(16x^4-24x^2+4\gamma x+9\right)\\ +12t\beta \left(1+4x^2\right)+64x^6+432x^4-16\gamma x^3+396x^2-36\gamma x+9\end{array}$

where β and γ are free real parameters of the solution. This solution is shown in Figure 2B. When $\beta =0$ and $\gamma =0$, the solution reduces to the second-order AP solution shown in 2(a). An interesting fact about the solution shown in Figure 2B is that despite being of the second order it contains three elementary rogue waves rather than two. This is a distinctive feature of multi-rogue wave solutions. They are different from multi-soliton solutions that have the number of solitons equal to the order of the solution [30].

The general form of the third-order rogue wave solution is cumbersome. It has several free parameters. Various forms of the third-order solution have been presented in [71, 73–75] and [76]. Instead of giving the general form, we restrict ourselves with a simpler one-parameter case. As the solution intensity ${\left|{\psi }_3\right|}^2$ is completely defined by the denominator $D_3\left(x,t\right)$, ${\left|{\psi }_3\left(x,t\right)\right|}^2=1+{\left[\text{log}\left(D_3\left(x,t\right)\right)\right]}_{tt}$ [71], we only present the expression for $D_3\left(x,t\right)$ [37, 76]. It has a single free real parameter b:

$D_3\left(x,t\right)= {\displaystyle \sum _{j=0}^{12}{d}_j\left(T\right){\left(2x\right)}^j},(7)$

where the polynomials $d_j\left(T=2t\right)$ are:

$\begin{array}{c}{d}_0=b^2\left(T^2+1\right)+T^{12}+6T^{10}+135T^8+2340T^6+3375T^4+12150T^2+2025,\\ d_1=-10b\left(T^6-9T^4-45T^2+45\right),\\ d_2=b^2+6\left(T^{10}-15T^8+90T^6+2250T^4-6075T^2+15525\right),\\ d_3=10b\left(T^4+18T^2+9\right),d_4=15\left(T^8-12T^6-90T^4+5220T^2+9585\right),\\ d_5=6b\left(3T^2-17\right),d_6=20\left(T^6+3T^4+675T^2+765\right),d_7=-2b,\\ d_8=15\left(T^4+18T^2+249\right),d_9=0,d_{10}=6\left(T^2+21\right),d_{11}=0,d_{12}=1.\end{array}$

The third-order solutions are shown in Figure 3 for the cases $b=0$ and $b=2\times {10}^7$. The third-order solution consists of six Peregrine waves. When $b=0$, all six are located at the origin leading to the central amplitude 7. For nonzero b, the solution splits into six components. Each of them is a Peregrine wave as can be seen from Figure 3B. One of them is located at the origin. Five others are at the corners of an equilateral pentagram. The total number of Peregrine waves is again higher than the order of the solution. Remarkably, the number of Peregrine waves in exact solutions cannot be equal to 2, 4, 5. We can consider the Peregrine wave as the elementary rogue wave solution. Equivalently, we can consider it as a rogue wave quantum or elementary particle of rogue wave patterns.

FIGURE 3

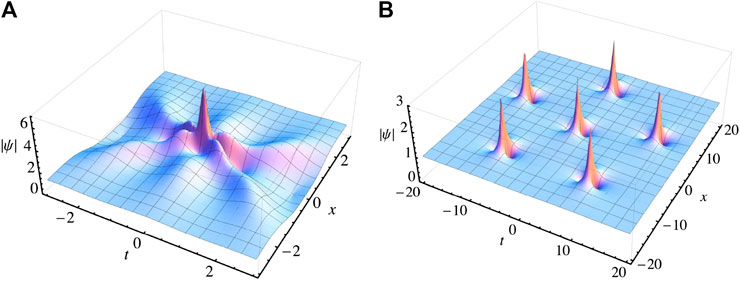
FIGURE 3. (A) Third order rogue wave solution given by Eq. (7) with $b=0$. (B) Third-order rogue wave solution with a circular pattern. It is given by Eq. (7) with $b=2\times {10}^7$. Each ‘elementary particle’ in this structure is a single Peregrine wave.

5 Complex Rogue Wave Patterns

The $N^{th}$ order rogue wave solution always contains $N\left(N+1\right)/2$ Peregrine waves. Namely, solution of the order $N=\mathrm{1,2,3,4,5}\cdots$ contains $\mathrm{1,3,6,10,15},\cdots$ Peregrine waves, respectively. These are known as ‘triangular numbers’ illustrated in Figure 4. They are defined as the total number of points in a regular pattern within a triangle with N points along its edges.

FIGURE 4

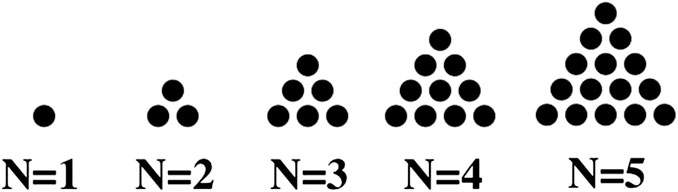
FIGURE 4. The first five triangular numbers. They are represented by the number of solid dots within each triangle.

Rogue wave patterns are defined by the order of the solution, N, and may depend on additional free parameters. Two examples are given above. These free parameters split the solution into individual Peregrine waves. These parameters specify separations and relative positions of the individual components on the $\left(x,t\right)$-plane. Any solution of $N^{th}$ order contains $N\left(N+1\right)/2$ individual components that, when well-separated, can be identified as Peregrine waves. This is a fundamental result [35]: the number of Peregrine waves in a multi-rogue wave solution of the NLSE is given by a triangular number. No other number of Peregrine waves can be present in a general multi-rogue wave solution. This number does not depend on whether the “elementary particles” in the higher-order rogue wave are well-separated or partially separated. In the latter case, when the free parameters are small, the number of Peregrine waves is not visually obvious.

The maximal amplitude of a rogue wave is one of its main characteristics. The maximal amplitude of the higher-order AP solutions when all Peregrine waves are located at the origin is $\left(2N+1\right)$ times the background, where N is the order of the solution [63, 68–70]. This is the highest possible amplitude for all imaginable rogue wave patterns.

A detailed classification of the rogue wave patterns has been given in [72]. The main results of this classification are shown in Figure 5. It shows the calculated rogue wave patterns that increase in order from top to bottom of table. A single Peregrine wave that is the solution of the first order is located in the top left cell. Higher-order AP solutions are located in the left column. The figure shows only solutions of up to 6-th order, although the list can be continued indefinitely. The second column represents regular triangular structures. The third column represents pentagram patterns. The fourth column provides examples of heptagram structures, i.e., patterns with seven Peregrine waves on one or several circles of different radii. Further columns have 9, 11, … Peregrine waves in the outer and inner shells of the structure.

FIGURE 5

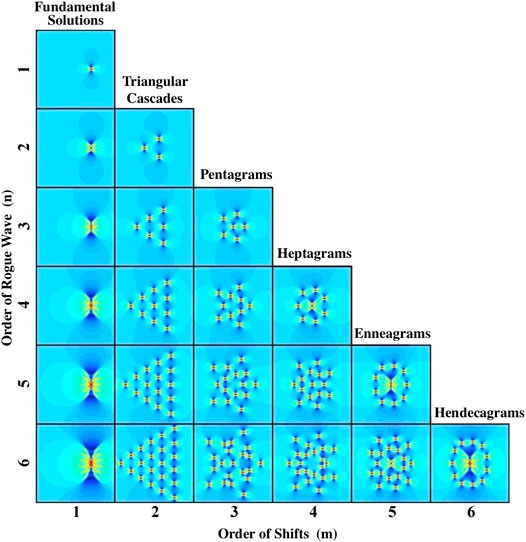
FIGURE 5. Patterns of higher-order rogue wave solutions [72]. Only solutions of up to order six are shown. The Peregrine soliton is on the first row. Second-order solutions are of two types: the AP solution and the triplet. They are located in the second row. Variety of patterns increases with the order. For example, the third order solutions do exist in the AP, triangular and pentagram forms. This happens because of the larger number of free parameters in the solution. Patterns located along the diagonal line are circular. They have a ring of $\left(2N-1\right)$ Peregrine solitons around a central rogue wave of order $N-2$ (for $N>2$). These are basically “atomic structures” with a nucleus and a shell of “electrons”.

“Atomic-like” circular structures are located along the diagonal line. Starting from order 3, they consist of a central nucleus containing $N-2$ elementary rogue waves in the form of an AP solution and $2N-1$ Peregrine waves playing the role of the shell of “electrons”. Patterns in inner cells of the table can be considered as “atomic structures” with several shells of “electrons”. The larger variety of patterns for higher-order solutions is caused by the larger number of free parameters controlling the solution. The rogue wave patterns can also have a lower symmetry than in Figure 5. Some examples can be found in [36, 71].

There is no doubt that these patterns can be observed experimentally. Indeed, a single Peregrine wave has been observed in optics [27], in water waves [55] and in a multicomponent plasma [77]. It may soon be observed in Bose-Einstein condensate [78, 79]. The rogue wave triplet has been observed in a water wave tank [80]. AP solutions up to fifth-order have also been observed in water waves [81]. As the NLSE has a wide range of applications, these solutions may appear in situations that we cannot even predict right now.

Can this approach be used for a description of elementary particles and atomic structures? Obviously, this will need more sophisticated equation than the NLSE. As mentioned, the phenomena described in this review are not unique to the NLSE although, perhaps, the NLSE case is the most studied of all. Rogue waves have been found in systems described by the Hirota and Maxwell-Bloch equations [42, 82], Sasa-Satsuma equation [83], Fokas-Lenells equation [84], in systems with self-steepening effect [85, 86] and even in the case of the complex KdV equation [87, 88]. Patterns of multi-rogue wave solutions similar to those in Figure 5 have been found for the complex modified KdV equation [89], Kundu–Eckhaus equation [90], coupled nonlinear Schrödinger–Boussinesq equations [91], generalized derivative nonlinear Schrödinger equations [92, 93], generalized Landau-Lifshitz equation [48], Manakov equations [94], the three–wave resonant interaction equations [95, 96], discrete Ablowitz-Ladik equations [97] and even in the case of breather collisions [98, 99]. Most recent work [100] shows that the ideas of rogue waves now enter the field of elementary particles. Namely, patterns similar to those in Figure 5 may appear in the case of coupled Higgs field equations describing nucleons interacting with neutral scalar mesons [100]. Further developments of the rogue wave theory along these lines may boost our vision of the complex world and how it is build out of simple fundamental particles.

Author Contributions

The author confirms being the sole contributor of this work and has approved it for publication.

Conflict of Interest

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

1. Hasegawa A, Tappert F. Transmission of stationary nonlinear optical pulses in dispersive dielectric fibers. I. Anomalous dispersion. Appl Phys Lett (1973) 23:142–4. doi:10.1063/1.1654836

CrossRef Full Text | Google Scholar

2. Agrawal GP. Nonlinear fiber optics 4th Edn. NY: Academic Press (2007)

Google Scholar

3. Zakharov VE. Stability of periodic waves of finite amplitude on a surface of deep fluid. J Appl Mech Tech Phys (1968) 9:190–4. doi:10.1007/BF00913182

Google Scholar

4. Osborne A. Nonlinear ocean waves and the inverse scattering transform Academic Press (2010).

Google Scholar

5. Moslem WM, Shukla PK, Eliasson B. Surface plasma rogue waves. Europhys Lett (2011) 96:25002. doi:10.1209/0295-5075/96/25002

CrossRef Full Text | Google Scholar

6. El-Tantawy SA. Rogue waves in electronegative space plasmas: the link between the family of the KdV equations and the nonlinear Schrödinger equation. Astrophys Space Sci (2016) 361:164. doi:10.1007/s10509-016-2754-8

CrossRef Full Text | Google Scholar

7. Stenflo L, Marklund M. Rogue waves in the atmosphere. J Plasma Phys (2010) 76:293. doi:10.1017/S0022377809990481

CrossRef Full Text | Google Scholar

8. Gross EP. Structure of a quantized vortex in boson systems. Nuovo Cimento (1961) 20:454. doi:10.1007/BF02731494

CrossRef Full Text | Google Scholar

9. Pitaevskii LP. Vortex lines in an imperfect Bose gas. Zh Eksp Teor Fiz (1961) 40:646.

Google Scholar

10. Lidsey JE. Scalar field cosmologies hidden within the nonlinear Schrödinger equation (2013). Available at: https://arxiv.org/abs/1309.7181 (Accessed September 27 2013).

Google Scholar

11. Bespalov VI, Talanov VI. Filamentary structure of light beams in nonlinear liquids. Sov Phys JETP Lett (1966) 3:307.

Google Scholar

12. Benjamin TB, Feir J. The disintegration of wave trains on deep water: Part 1. Theory J Fluid Mech (1967) 27:417. doi:10.1017/S002211206700045X

CrossRef Full Text | Google Scholar

13. Zakharov VE, Shabat AB. Exact theory of two-dimensional self-focusing and one-dimensional self-modulation of waves in nonlinear media. J Exp Theor Phys (1972) 34:62–9.

Google Scholar

14. Akhmediev N, Eleonskii VM, Kulagin NE. Generation of periodic trains of picosecond pulses in an optical fiber: exact solutions. Sov Phys JETP (1985) 62(5):894–9.

Google Scholar

15. Akhmediev N, Ankiewicz A, Taki M. Waves that appear from nowhere and disappear without a trace. Phys Lett (2009) 373:675–8. doi:10.1016/j.physleta.2008.12.036

CrossRef Full Text | Google Scholar

16. Shrira VI, Geogjaev VV. What makes the Peregrine soliton so special as a prototype of freak waves? J Eng Math (2009) 67:11–22. doi:10.1007/s10665-009-9347-2

CrossRef Full Text | Google Scholar

17. Conforti M, Mussot A, Kudlinski A, Trillo S, Akhmediev N. Doubly periodic solutions of the focusing nonlinear Schrödinger equation: recurrence, period doubling, and amplification outside the conventional modulation-instability band. Phys Rev (2020) 101:023843. doi:10.1103/PhysRevA.101.023843

CrossRef Full Text | Google Scholar

18. Matveev VB, Salle MA. Darboux transformations and solitons Berlin-Heidelberg: Springer (1991).

CrossRef Full Text | Google Scholar

19. Its OR, Kotlyarov V. Explicit formulas for solutions of the Schrödinger nonlinear equation. Doklady Akad. Nauk Ukrainian SSR (1976) 10:965–8.

Google Scholar

20. Its AR, Rybin AV, Sall MA. Exact integration of nonlinear Schrodinger equation[original in Russian: teor. Theor Math Phys (1988) 69:29–32.

Google Scholar

21. Akhmediev N, Korneev VI. Modulation instability and periodic solutions of nonlinear Schrödinger equation. Theor Math Phys (1986) 69:189–94.

CrossRef Full Text | Google Scholar

22. Akhmediev N, Eleonskii VM, Kulagin NE. Exact first-order solutions of the nonlinear Schrödinger equation. Theor Math Phys (1987) 72:809.

CrossRef Full Text | Google Scholar

23. Yang G, Wu FO, Lopez Aviles HE, Christodoulides D. Optical amplification and transmission of attenuated multi-soliton based on spectral characteristics of Akhmediev breather. Optic Commun (2020) 473:125899.

CrossRef Full Text | Google Scholar

24. Andral U, Kibler B, Dudley JM, Finot C. Akhmediev breather signatures from dispersive propagation of a periodically phase-modulated continuous. wave Motion (2020) 95:102545.

CrossRef Full Text | Google Scholar

25. Akhmediev N, Korneev VI, Mitskevich NV. N-modulation signals in a single-mode optical waveguide under nonlinear conditions. Zh Exp Teor Fiz (1988) 94:159–70.

Google Scholar

26. Peregrine DH. Water waves, nonlinear Schrödinger equations and their solutions. J Australian Math Soc Ser B (1983) 25:16.

CrossRef Full Text | Google Scholar

27. Kibler B, Fatome J, Finot C, Millot G, Dias F, Genty G, et al. The Peregrine soliton in nonlinear fibre optics. Nat Phys (2010) 6:790–5.

CrossRef Full Text | Google Scholar

28. Gaillard P, Gastineau M. The Peregrine breather of order nine and its deformations with sixteen parameters solutions to the NLS equation. Phys Lett (2015) 379:1309–13.

CrossRef Full Text | Google Scholar

29. Ablowitz MJ, Segur H. Solitons and the inverse scattering transform Philadelphia, Pa, USA: SIAM (1981).

CrossRef Full Text | Google Scholar

30. Akhmediev N, Mitskevich NV. Extremely high degree of N-soliton pulse compression in an optical fiber. IEEE J Quantum Electron QE (1991) 27:849–57.

CrossRef Full Text | Google Scholar

31. Akhmediev N, Soto-Crespo JM, Ankiewicz A. Extreme waves that appear from nowhere: on the nature of rogue waves. Phys Lett (2009) 373:2137–45. doi:10.1016/j.physleta.2009.04.023

CrossRef Full Text | Google Scholar

32. Dubard P, Gaillard P, Klein C, Matveev VB. On multi-rogue wave solutions of the NLS equation and positon solutions of the KdV equation. Eur Phys J Spec Top (2010) 185:247–58. doi:10.1140/epjst/e2010-01252-9

CrossRef Full Text | Google Scholar

33. Ohta Y, Yang J. General high-order rogue waves and their dynamics in the nonlinear Schrödinger equation. Proc R Soc A (2012) 468:1716–40.

CrossRef Full Text | Google Scholar

34. Gaillard P. Degenerate determinant representation of solutions of the nonlinear Schrödinger equation, higher order Peregrine breathers and multi-rogue waves. J Math Phys (2013) 54:013504. doi:10.1063/1.4773096

CrossRef Full Text | Google Scholar

35. Ankiewicz A, Akhmediev N. Multi-rogue waves and triangular numbers. Rom Rep Phys (2017) 69:104.

Google Scholar

36. Kedziora DJ, Ankiewicz A, Akhmediev N. Triangular rogue wave cascades. Phys Rev E Stat Nonlinear Soft Matter Phys (2012) 86:056602. doi:10.1103/PhysRevE.86.056602

CrossRef Full Text | Google Scholar

37. Kedziora DJ, Ankiewicz A, Akhmediev N. Circular rogue wave clusters. Phys Rev E Stat Nonlinear Soft Matter Phys (2011) 84:056611. doi:10.1103/PhysRevE.84.056611

CrossRef Full Text | Google Scholar

38. Satsuma J, Yajima N. Initial value problems of one-dimensional self-modulation of nonlinear waves in dispersive media. Prog Theor Phys Suppl (1974) 55:284.

CrossRef Full Text | Google Scholar

39. Kedziora DJ, Ankiewicz A, Akhmediev N. Rogue waves and solitons on a cnoidal background. Eur Phys J Spec Top (2014) 223:43–62. 10.1140/epjst/e2014-02083-4.

CrossRef Full Text | Google Scholar

40. Chen J, Pelinovsky DE, White RE. Rogue waves on the double-periodic background in the focusing nonlinear Schrödinger equation. Phys Rev E (2019) 100:052219. doi:10.1103/PhysRevE.100.052219

PubMed Abstract | CrossRef Full Text | Google Scholar

41. Zabusky NJ, Kruskal MD. Interaction of “solitons” in a collisionless plasma and the recurrence of initial states. Phys Rev Lett (1965) 15:240–3.

CrossRef Full Text | Google Scholar

42. Ankiewicz A, Soto-Crespo JM, Akhmediev N. Rogue waves and rational solutions of the Hirota equation. Phys Rev E Stat Nonlinear Soft Matter Phys (2010) 81:046602. doi:10.1103/PhysRevE.81.046602

CrossRef Full Text | Google Scholar

43. Bandelow U, Akhmediev N. Persistence of rogue waves in extended nonlinear Schrödinger equations: integrable Sasa-Satsuma case. Phys Lett (2012) 376:1558–61. doi:10.1016/J.PHYSLETA.2012.03.032

CrossRef Full Text | Google Scholar

44. Ohta Y, Yang J. Rogue waves in the Davey-Stewartson I equation. Phys Rev E Stat Nonlinear Soft Matter Phys (2012) 86:036604. doi:10.1103/PhysRevE.86.036604

CrossRef Full Text | Google Scholar

45. Ohta Y, Yang JK. Dynamics of rogue waves in the Davey – stewartson II equation. J Phys Math Theor (2013) 46:105202. doi:10.1088/1751-8113/46/10/105202

CrossRef Full Text | Google Scholar

46. Rao J, Cheng Y, He J. Rational and semirational solutions of the nonlocal Davey–Stewartson equations. Stud Appl Math (2017) 139:568–98. doi:10.1111/sapm.12178

CrossRef Full Text | Google Scholar

47. Li R, Geng X. Rogue periodic waves of the sine—Gordon equation. Appl Math Lett (2020) 102:106147. doi:10.1016/j.aml.2019.106147

CrossRef Full Text | Google Scholar

48. Li R, Geng X, Xue B. A generalization of the Landau-Lifschitz equation: breathers and rogue waves. J Nonlinear Math Phys (2020) 27:279–94. doi:10.1080/14029251.2020.1700636

CrossRef Full Text | Google Scholar

49. Ankiewicz A, Devine N, Akhmediev N. Are rogue waves robust against perturbations? Phys Lett (2009) 373:3997–4000. doi:10.1016/j.physleta.2009.08.053

CrossRef Full Text | Google Scholar

50. Ankiewicz A, Soto-Crespo JM, Chowdhury MA, Akhmediev N. Rogue waves in optical fibers in presence of third-order dispersion, self-steepening, and self-frequency shift. J Opt Soc Am B (2013) 30:87–94. doi:10.1364/JOSAB.30.000087

CrossRef Full Text | Google Scholar

51. Zaviyalov A, Egorov O, Iliew R, Lederer F. Rogue waves in mode-locked fibre lasers. Phys Rev (2012) 85:013828. doi:10.1103/PhysRevA.85.013828

CrossRef Full Text | Google Scholar

52. Soto-Crespo JM, Grelu P, Akhmediev N. Dissipative rogue waves: extreme pulses generated by passively mode-locked lasers. Phys Rev E Stat Nonlinear Soft Matter Phys (2011) 84:016604. doi:10.1103/PhysRevE.84.016604

CrossRef Full Text | Google Scholar

53. Lynch P. The origins of computer weather prediction and climate modelling. J Comput Phys (2008) 227:3431–44. doi:10.1016/j.jcp.2007.02.034

CrossRef Full Text | Google Scholar

54. Krishnamurti TN. Numerical weather prediction. Annu Rev Fluid Mech (1995) 27:195–225. doi:10.1146/annurev.fl.27.010195.001211

CrossRef Full Text | Google Scholar

55. Chabchoub A, Hoffmann NP, Akhmediev N. Rogue wave observation in a water wave tank. Phys Rev Lett (2011) 106:204502. doi:10.1103/PhysRevLett.106.204502

PubMed Abstract | CrossRef Full Text | Google Scholar

56. Bertola M, Tovbis A. Universality for the focusing nonlinear Schrödinger equation at the gradient catastrophe point: rational breathers and poles of the tritronqué solution to Painlevé I, Commun. Pure Appl Math (2012) 66:678. doi:10.1002/cpa.21445

Google Scholar

57. Tikan A, Billet C, El G, Tovbis A, Bertola M, Sylvestre T, et al. Universal Peregrine soliton structure in nonlinear pulse compression in optical fiber. Phys Rev Lett (2017) 119:033901. doi:10.1103/PhysRevLett.119.033901

PubMed Abstract | Google Scholar

58. Chabchoub A, Hoffmann NP, Akhmediev N. Observation of rogue wave holes in a water wave tank. J Geophys Res (2012) 117:C00J02. doi:10.1029/2011JC007636

CrossRef Full Text | Google Scholar

59. Chen S, Grelu P, Soto-Crespo JM. Dark- and bright-rogue-wave solutions for media with long-wave-short-wave resonance. Phys Rev E Stat Nonlinear Soft Matter Phys (2014) 89:011201. doi:10.1103/PhysRevE.89.011201

CrossRef Full Text | Google Scholar

60. Baronio F, Degasperis A, Conforti M, Wabnitz S. Solutions of the vector nonlinear Schrödinger equations: evidence for deterministic rogue waves. Phys Rev Lett (2012) 109:044102. doi:10.1103/PhysRevLett.109.044102

PubMed Abstract | CrossRef Full Text | Google Scholar

61. Baronio F. Akhmediev breathers and Peregrine solitary waves in a quadratic medium. Opt Lett (2017) 42:1756. doi:10.1364/OL.42.001756

PubMed Abstract | CrossRef Full Text | Google Scholar

62. Baronio F, Conforti M, Degasperis A, Lombardo S. Rogue waves emerging from the resonant interaction of three waves. Phys Rev Lett (2013) 111:114101. doi:10.1103/PhysRevLett.111.114101

PubMed Abstract | CrossRef Full Text | Google Scholar

63. Gaillard P. Other 2N-2 parameters solutions of the NLS equation and 2N+1 highest amplitude of the modulus of the N-th order Akhmediev-Peregrine breather. J Phys Math Theor (2015) 48:145203. doi:10.1088/1751-8113/48/14/145203

CrossRef Full Text | Google Scholar

64. Dontsop PYG, Essama BGO, Doing JM, Dedzo MM, Atangana J, Yemele D, et al. Akhmediev-Peregrine rogue waves generation in a composite right/left-handed transmission line. Opt Quant Electron (2016) 48:59. doi:10.1007/s11082-015-0333-9

CrossRef Full Text | Google Scholar

65. Chabchoub A, Fink M. Time-reversal generation of rogue waves. Phys Rev Lett (2014) 112:124101. doi:10.1103/PhysRevLett.112.124101

PubMed Abstract | CrossRef Full Text | Google Scholar

66. Vitanov NK, Chabchoub A, Hoffmann N. Deep-water waves: on the nonlinear Schrödinger equation and its solutions. J Theor Appl Mech Sofia (2013) 43:43–54. doi:10.2478/jtam-2013-0013

CrossRef Full Text | Google Scholar

67. Akhmediev N, Ankiewicz A. Solitons, nonlinear pulses and beams London: Chapman & Hall (1997).

Google Scholar

68. Ankiewicz A, Clarkson P, Akhmediev N. Rogue waves, rational solutions, the patterns of their zeros and integral relations. J Phys Math Theor (2010) 43:122002.

CrossRef Full Text | Google Scholar

69. Wang L, Yang C, Wang J, He J. The height of an n-th order fundamental rogue wave for the nonlinear Schrödinger equation. Phys Lett (2017) 381:1714–8. doi:10.1016/j.physleta.2017.03.023

CrossRef Full Text | Google Scholar

70. Chin SA, Ashour OA, Nikolić SN, Belić MR. Maximal intensity higher-order Akhmediev breathers of the nonlinear Schrödinger equation and their systematic generation. Phys Lett (2016) 380:3625–9. doi:10.1016/j.physleta.2016.08.038

CrossRef Full Text | Google Scholar

71. Ling L, Zhao LC. Simple determinant representation for rogue waves of the nonlinear Schrödinger equation. Phys Rev E Stat Nonlinear Soft Matter Phys (2013) 88:043201. doi:10.1103/PhysRevE.88.043201

CrossRef Full Text | Google Scholar

72. Kedziora DJ, Ankiewicz A, Akhmediev N. Classifying the hierarchy of nonlinear-Schrödinger-equation rogue-wave solutions. Phys Rev E - Stat Nonlinear Soft Matter Phys (2013) 88:013207. doi:10.1103/PhysRevE.88.013207

CrossRef Full Text | Google Scholar

73. Ankiewicz A, Kedziora DJ, Akhmediev N. Rogue wave triplets. Phys Lett (2011) 375:2782–5. doi:10.1016/j.physleta.2011.05.047

CrossRef Full Text | Google Scholar

74. Gaillard P. Deformations of third-order Peregrine breather solutions of the nonlinear Schrödinger equation with four parameters. Phys Rev E Stat Nonlinear Soft Matter Phys (2013) 88:042903. doi:10.1103/PhysRevE.88.042903

CrossRef Full Text | Google Scholar

75. Gaillard P. Degenerate determinant representation of solutions of the nonlinear Schrödinger equation, higher order Peregrine breathers and multi-rogue waves. J Math Phys (2013) 54:013504. doi:10.1063/1.4773096

CrossRef Full Text | Google Scholar

76. Akhmediev N, Ankiewicz A, Soto-Crespo JM. Rogue waves and rational solutions of the nonlinear Schrödinger equation. Phys Rev E - Stat Nonlinear Soft Matter Phys (2009) 80:026601. doi:10.1103/PhysRevE.80.026601

CrossRef Full Text | Google Scholar

77. Bailung H, Sharma SK, Nakamura Y. Observation of Peregrine solitons in a multicomponent plasma with negative ions. Phys Rev Lett (2011) 107:255005. doi:10.1103/PhysRevLett.107.255005

PubMed Abstract | CrossRef Full Text | Google Scholar

78. Bludov YV, Konotop VV, Akhmediev N. Matter rogue waves. Phys Rev (2009) 80:033610. doi:10.1103/PhysRevA.80.033610

CrossRef Full Text | Google Scholar

79. Nguyen JHV, Luo D, Hulet RG. Formation of matter-wave soliton trains by modulational instability. Science (2017) 356:422–6. doi:10.1126/science.aal3220

PubMed Abstract | CrossRef Full Text | Google Scholar

80. Chabchoub A, Akhmediev N. Observation of rogue wave triplets in water waves. Phys Lett (2013) 377:2590–3. doi:10.1016/j.physleta.2013.07.027

CrossRef Full Text | Google Scholar

81. Chabchoub A, Hoffmann N, Onorato M, Slunyaev A, Sergeeva A, Pelinovsky E, et al. Observation of a hierarchy of up to fifth-order rogue waves in a water tank. Phys Rev E Stat Nonlinear Soft Matter Phys (2012) 86:056601. doi:10.1103/PhysRevE.86.056601

CrossRef Full Text | Google Scholar

82. Li C, He J, Porsezian K, Porseizan K. Rogue waves of the Hirota and the Maxwell-Bloch equations. Phys Rev E Stat Nonlinear Soft Matter Phys (2013) 87:012913. doi:10.1103/PhysRevE.87.012913

CrossRef Full Text | Google Scholar

83. Gui M, Qin Z, Grimshaw R, Akhmediev N. Intricate dynamics of rogue waves governed by the Sasa-Satsuma equation. Physica D (2020) 402:132252. doi:10.1016/j.physd.2019.132252

CrossRef Full Text | Google Scholar

84. He J, Xu S, Porsezian K. Rogue waves of the Fokas-Lenells equation. J. Phys. Soc. Japan (2012) 81:124007. doi:10.1143/JPSJ.81.124007

CrossRef Full Text | Google Scholar

85. Liu C, Akhmediev N. Super-regular breathers in nonlinear systems with self-steepening effect. Phys Rev E (2019) 100:062201. doi:10.1103/PhysRevE.100.062201

PubMed Abstract | CrossRef Full Text | Google Scholar

86. Chen S, Pan C, Grelu P, Baronio F, Akhmediev N. Fundamental Peregrine solitons of ultrastrong amplitude enhancement through self-steepening in vector nonlinear systems. Phys Rev Lett (2020) 124:113901. doi:10.1103/PhysRevLett.124.113901

PubMed Abstract | CrossRef Full Text | Google Scholar

87. Ankiewicz A, Bokaeeyan M, Akhmediev N. Shallow-water rogue waves: an approach based on complex solutions of the Korteweg–de Vries equation. Phys Rev E (2019) 99:050201. doi:10.1103/PhysRevE.99.050201

PubMed Abstract | CrossRef Full Text | Google Scholar

88. Crabb M, Akhmediev N. Rogue wave multiplets in the complex KdV equation. Rom Rep Phys (2020) 72:118.

Google Scholar

89. He J, Wang L, Li L, Porsezian K, Erdélyi R. Few-cycle optical rogue waves: complex modified Korteweg-de Vries equation. Phys Rev E Stat Nonlinear Soft Matter Phys (2014) 89:062917. doi:10.1103/PhysRevE.89.062917

CrossRef Full Text | Google Scholar

90. Qiu D, He J, Zhang Y, Porsezian K. The Darboux transformation of the Kundu-Eckhaus equation. Proc Royal Soc A Math Phys Eng Sci (2015) 471:20150236. doi:10.1098/rspa.2015.0236

CrossRef Full Text | Google Scholar

91. Zhang , Chen Y. General high-order rogue waves to nonlinear Schrödinger–Boussinesq equation with the dynamical analysis. Nonlinear Dynam (2018) 93:2169–84. doi:10.1007/s11071-018-4317-8

CrossRef Full Text | Google Scholar

92. Yang B, Chen J, Yang J. Rogue waves in the generalised derivative nonlinear Schrödinger equations. J. Nonlin. Science (2020) 30:3027–56.

CrossRef Full Text | Google Scholar

93. Yang B, Yang J. Universal patterns of rogue waves Available at: https://arxiv.org/abs/2009.06060 (Accessed September 13 2020).

Google Scholar

94. Chen S, Mihalache D. Vector rogue waves in the Manakov system: diversity and compossibility. J Phys Math Theor (2015) 48:215202. doi:10.1088/1751-8113/48/21/215202

CrossRef Full Text | Google Scholar

95. Chen S, Soto-Crespo JM, Grelu P. Watch-hand-like optical rogue waves in three-wave interactions. Optic Express (2015) 23:349–59. doi:10.1364/OE.23.000349

CrossRef Full Text | Google Scholar

96. Wang X, Cao J, Chen Y. Higher-order rogue wave solutions of the three–wave resonant interaction equation via the generalized Darboux transformation. Phys Scripta (2015) 90:105201. doi:10.1088/0031-8949/90/10/105201

CrossRef Full Text | Google Scholar

97. Ohta Y, Yang J. General rogue waves in the focusing and defocusing Ablowitz—Ladik equations. J Phys Math Theor (2014) 47:255201. doi:10.1088/1751-8113/47/25/255201

CrossRef Full Text | Google Scholar

98. Kedziora DJ, Ankiewicz A, Akhmediev N. Second-order nonlinear Schrödinger equation breather solutions in the degenerate and rogue wave limits. Phys Rev E Stat Nonlinear Soft Matter Phys (2012) 85:066601. doi:10.1103/PhysRevE.85.066601

CrossRef Full Text | Google Scholar

99. Wang L, He J, Xu H, Wang J, Porsezian K. Generation of higher-order rogue waves from multibreathers by double degeneracy in an optical fiber. Phys Rev E (2017) 95:042217. doi:10.1103/PhysRevE.95.042217

PubMed Abstract | CrossRef Full Text | Google Scholar

100. Zhaqilao . Dynamics of localized wave solutions for the coupled Higgs field equation. Nonlinear Dynam (2020) 101:1181–98.

CrossRef Full Text | Google Scholar