Membrane Formation in Liquids by Adding an Antagonistic Salt

Antagonistic salts are composed of hydrophilic and hydrophobic ions. In a binary mixture, such as water and organic solvent, these ion pairs preferentially dissolve to those phases, respectively, and there is a coupling between the charge density and the composition. The heterogeneous distribution of ions forms a large electric double layer at the interface between these solvents. This reduces the interfacial tension between water and organic solvent, and stabilizes an ordered structure, such as a membrane. These phenomena have been extensively studied from both theoretical and experimental point of view. In addition, the numerical simulations can reproduce such ordered structures.

1. Introduction

The solvation effect of ion is considered to be important in various fields, such as those concerning biochemistry, chemical reactions (e.g., electrode reactions and metal ion complexation), and phase behavior in solutions. In particular, the solvation effect on the phase behavior of binary water/organic solvent mixtures is noteworthy. Experiments have established that the two-phase region of a binary mixture tends to expand with the addition of a hydrophilic salt, e.g., NaCl [1–4]. In such mixtures, both cations and anions preferentially attract water molecules more than the organic solvent molecules, which is a key factor in salt-induced phase separation [3, 4].

On the other hand, the class of salts containing a pair of hydrophilic and hydrophobic ions is known an “antagonistic salt.” An example is sodium tetraphenylborate, NaBPh₄, which is frequently used as a precipitation agent [5]. This chemical is composed of a hydrophilic sodium ion (Na⁺) and a hydrophobic tetraphenylborate ion (${\text{BPh}}_4^{-}$), which has four phenyl rings bonded to a negatively charged boron atom. Because the surrounding phenyl rings are large, hydrogen bonding around this anion is largely deformed and the hydration shell may be destroyed. Thus, the anions preferentially dissolve in an organic solvent (oil) whereas the cations prefer to stay within the water [6, 7]. This means that the cations and anions behave antagonistically and may distribute heterogeneously when added to a binary liquid consisting of water and an organic solvent.

In 2004, Onuki and Kitamura investigated the effect of the preferential solvation of ions on the binary water/organic solvent based on the Ginzburg-Landau theory, and predicted that the long-range periodic structure, so-called the charge-density-wave (CDW) structure, is induced by the addition of antagonistic salts due to strong coupling between the concentration fluctuation of solvents and the preferential solvation of ions [8]. In 2006, Onuki also investigated the ion distribution in the binary water/organic solvent mixture, and showed that the cations and anions of an antagonistic salt tend to adsorb around a water-oil interface (see Figure 1) [14]. In parallel, the aggregation of hydrophilic and hydrophobic ion pairs was experimentally confirmed by Luo et al. by means of X-ray reflectivity for the mixture of water/nitrobenzene with tetrabutylammonium bromide [15]. Under this condition, it was also shown that the interfacial tension decreases with increasing the amount of antagonistic salts [15]. Furthermore, in 2007, the CDW structure predicted by Onuki and Kitamura [8] was confirmed using small-angle neutron scattering (SANS) on the water/3-methylpyridine (3MP)/NaBPh₄ mixture [16].

FIGURE 1

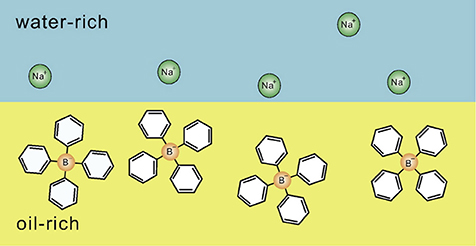
Figure 1. Schematic illustration indicating the distribution of hydrophilic and hydrophobic ion pairs at a water-oil interface, which was clarified by the theoretical studies of Onuki et al. [9–14]. Hydrophilic Na⁺ and hydrophobic ${\text{BPh}}_4^{-}$ are in the water-rich region and the oil-rich region, respectively. The figure is reproduced from Onuki et al. [13] with permission from Copyright 2016 Elsevier Ltd.

Since then, a number of investigations regarding the effect of antagonistic salts have been performed. On the basis of the Ginzburg-Landau theory, Onuki et al. clarified the heterogeneous distribution of solvents and ions in the mixture more in detail [9–14]. Bier et al. estimated the effect of antagonistic salts on the concentration fluctuation of solvents by extending the Ornstein-Zernike theory [17]. Bier et al. also examined the phase behavior of the mixture with antagonistic salts based on the density functional theory [18]. Pousaneh et al. investigated the effective interaction between a pair of colloids based on the Ginzburg-Landau theory, and showed that the oscillatory interaction occurs in the presence of antagonistic salts in the mixture [19, 20]. At the same time, there are several experimental results on the formation of ordered structures (e.g., membranes) induced by the preferential solvation of antagonistic salts [16, 21–33] and on the surface of water. Further, such structures have recently been confirmed by a lattice-based Monte Carlo simulation [34].

We review a series of results on mesoscopic structures induced by antagonistic salts in water/organic solvent mixtures.

2. The Effect of Antagonistic Salts on the Phase Behavior of Water/Organic Solvent Mixtures

As mentioned above, hydrophilic cations and hydrophobic anions tend to adsorb near the interface between water and the organic solvent because of the preferential solvation and the electrostatic attraction. This heteroselective solvation of cations and anions can affect the solubility of water and organic solvent. Figure 2 shows phase diagrams of deuterium oxide (D₂O)/3MP and D₂O/3MP/NaBPh₄ mixtures [24]. The binary mixture of D₂O and 3MP is miscible at room temperature and separates into two phases between T ≈ 310 K (lower critical solution temperature, LCST) and T ≈ 390 K (upper critical solution temperature, UCST), where T denotes the temperature of the mixture [35]. The critical composition is ϕ_3MP = 0.30, where ϕ_3MP denotes the volume fraction of 3MP in the mixture. When NaBPh₄ is dissolved in the mixture, the two-phase region shrinks as the concentration of NaBPh₄ in the mixtures C_salt increases, and disappears at C_salt > 15 mmol/L. That is, the mutual solubility between D₂O and 3MP increases with the addition of NaBPh₄. This result is consistent with the assumption that pairs of antagonistic ions behave as surface-active agents. Similar tendencies, i.e., increase of the mutual solubility between water and organic solvent, were also reported for water/3MP/tetraphenylphosphonium chloride (PPh₄Cl) mixtures [23], water/2,6-dimethylpyridine/ NaBPh₄ mixtures [26], and water/acetonitrile/NaBPh₄ mixtures [31].

FIGURE 2

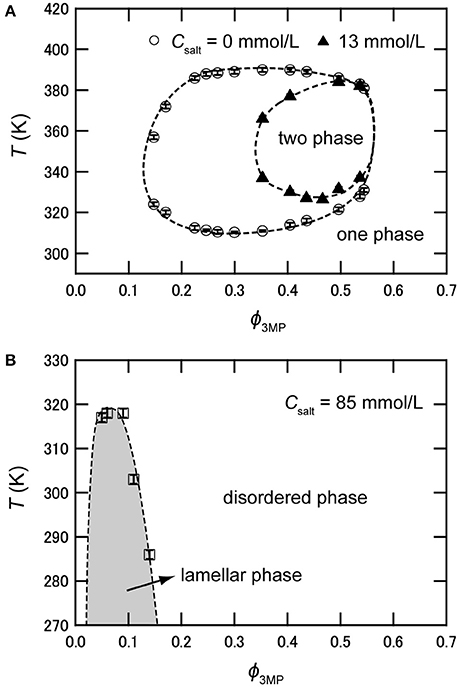
Figure 2. (A) Phase diagram of D₂O/3MP and D₂O/3MP/NaBPh₄ (13 mmol/L). (B) Phase diagram of D₂O/3MP/NaBPh₄ (85 mmol/L). It is noted that the two-phase region does not exist at this salt concentration. The data are taken from Sadakane et al. [24].

3. Membrane Structures Formed in the Water-Rich Regions of the Mixtures

As shown above, the mutual solubility of water and organic solvent increases with the addition of antagonistic salts. In this sense, antagonistic salts play the role of surfactants. Various kinds of ordered structures are formed in surfactant solutions depending on the sample composition and temperature. Therefore, it was expected that antagonistic salts can also induce an ordered structure in the mixture under certain conditions. In fact, the formation of a highly ordered lamellar structure, i.e., a multilamellar vesicle, was discovered in 2009 [21] in the water-rich region of water/3MP/NaBPh₄ mixtures (see Figure 2B).

Figure 3 shows the temperature dependence via optical microscopic images of a mixture of D₂O and 3MP at ϕ_3MP = 0.09 with 85 mmol/L of NaBPh₄. The entire area is homogeneous without visible structures above 318 K (Figure 3A), whereas small droplets with diameter of ca. 20 μm are formed below 318 K (Figures 3B,C); that is, a temperature-induced order/disorder phase transition occurs at 318 K. The left-hand panel of Figure 3B′ shows a magnified view of Figure 3B and the right-hand panel shows the corresponding polarized microscopic image with crossed Nicols. Since Maltese cross patterns are shown in the polarized microscopic image, the formation of multilamellar vesicles is suggested [21]. Similarly, the formation of multilamellar vesicles was observed in water/2,6-dimethylpyridine/NaBPh₄ mixtures [26].

FIGURE 3

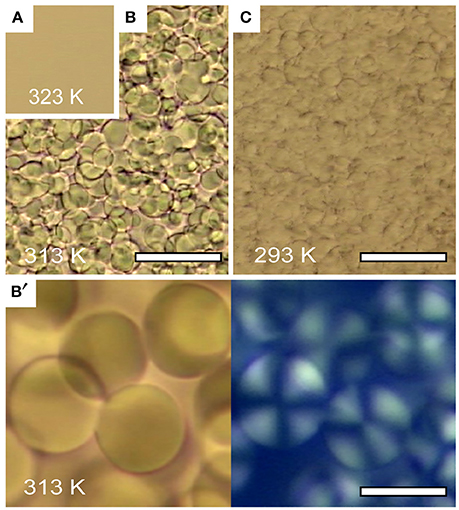
Figure 3. Optical microscopy images of D₂O/3MP/NaBPh₄ mixture at ϕ_3MP = 0.09 and C_salt = 85 mmol/L ϕ_3MP = 0.09: (A) T = 323 K (disordered phase), (B) T = 313 K (lamellar phase), and (C) T = 293 K (lamellar phase). Scale bars in (B,C) indicate 100 μm. (B′) Magnified view of (B) (left) and a birefringent image of the same region (right) with the scale bar being 20 μm. The figure is reproduced from Sadakane et al. [21] (Copyright 2009 by The American Physical Society). As per the copyright holder's policies, no permission is required for the use of figures by the authors of the original article.

Nanoscale structures induced by antagonistic salts were investigated by SANS, which could distinguish water and organic solvent regions. Figure 4A shows the temperature dependences of SANS profiles of a D₂O/3MP/NaBPh₄ mixture (ϕ_3MP = 0.09 and C_salt = 85 mmol/L) [24]. The SANS profiles below 318 K show a sharp peak with higher-order peaks arising from the highly ordered structure, whereas profiles above 318 K show a single broad peak arising from the disordered structure. The sample shows two-phase coexistence at T ≈ 318 K, i.e., a highly ordered phase and a disordered phase are observed in the upper and the lower parts of the sample, respectively, suggesting that a 1st-order phase transition occurs around this temperature. This result is consistent with microscopic observation shown in Figure 3.

FIGURE 4

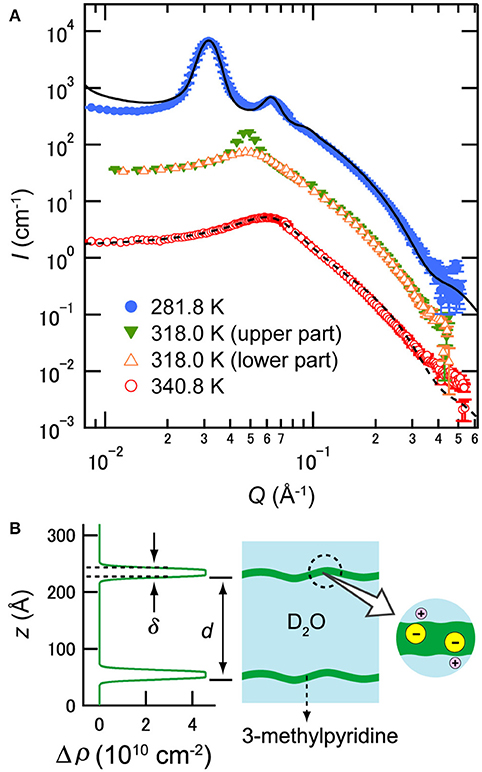
Figure 4. (A) Temperature dependence of SANS profiles of D₂O/3MP/NaBPh₄ mixture (ϕ_3MP = 0.09 and C_salt = 85 mmol/L). The data at lower temperatures have been multiplied by 10 for better visualization. The closed and open symbols indicate data for the lamellar phase and the sponge phase, respectively. The solid and dashed lines show the fitting result according to Equations (1) and (4), respectively. The data are taken from Sadakane et al. [24]. (B) Scattering density distribution at T = 283 K estimated from Equation (1) (left) and schematic picture of the distribution of ingredients (right). The z axis indicates the distance normal to the membrane. (B) is reproduced from Sadakane et al. [21] (Copyright 2009 by The American Physical Society). As per the copyright holder's policies, no permission is required for the use of figures by the authors of the original article.

SANS profiles obtained from the highly ordered phase were analyzed according to the model scattering function of the lamellar structure proposed by Nallet et al. [36] as

$\begin{array}{ll}{I}_{L\text{α}}(Q)=\frac{2\pi P_{L\text{α}}(Q)S_{L\text{α}}(Q)}{d{Q}^2},& (1)\end{array}$

with the form factor of the membrane

$\begin{array}{ll}{P}_{L\text{α}}(Q)=\frac{2{(\Delta \rho )}^2}{Q^2}\left[1-cos(\delta Q)exp\left(-\frac{1}{2}{\Delta \delta }^2{Q}^2\right)\right],& (2)\end{array}$

and structure factor

$\begin{array}{l}{S}_{L\text{α}}(Q)=1+2{\displaystyle \sum _{n=1}^{N-1}}\left(1-\frac{n}{N}\right)cos\left(\frac{dnQ}{1+2\Delta Q^2{d}^{2}g(n)}\right)\\ ·exp\left[-\frac{\Delta Q^2{d}^2{n}^2+2d^{2}g(n)Q^2}{2(1+2\Delta Q^2{d}^{2}g(n))}\right]\frac{1}{\sqrt{1+2\Delta Q^2{d}^{2}g(n)}},& (3)\end{array}$

where I_Lα denotes the SANS intensity of the lamellar phase, Q the momentum transfer, d the intermembrane distance, Δρ the difference in scattering length density distribution between the membrane and bulk water, δ the membrane thickness, Δδ the membrane thickness distribution, N the number of layers, ΔQ the instrumental resolution, and g the correlation function of fluctuating membranes. As a result of the data analysis, it was confirmed that the membrane was composed of 3 MP and ${\text{BPh}}_4^{-}$ ions (see Figure 4B) [21, 24]. For example, the values of d and δ of the mixture at 281.8 K were evaluated as 195.6±0.2 and 14±3 Å, respectively [24]. A similar membrane structure was also observed in water/2,6-dimethylpyridine/NaBPh₄ mixtures [26].

On the other hand, SANS profiles obtained from the disordered phase were analyzed according to the model scattering function for the sponge structure of a surfactant solution, given as [37, 38]

$\begin{array}{ll}{I}_{L3}(Q)=\frac{{\varphi }_{3\text{MP}}+{\varphi }_{\text{BPh}}}{\pi {r_{\text{d}}}^2\delta }{P}_{L3}(Q)S_{L3}(Q),& (4)\end{array}$

with the form factor derived from randomly oriented disks of membranes

$\begin{array}{ll}{P}_{L3}(Q)=4{(\pi {r_{\text{d}}}^2\Delta \rho )}^2\frac{\left[1-cos(\delta Q)exp\left(-{\delta }^2{Q}^2/32\right)\right]/Q^2}{{r_{\text{d}}}^2{Q}^2+2exp\left(-{r_{\text{d}}}^2{Q}^2/6\right)},& (5)\end{array}$

and structure factor

$\begin{array}{l}{S}_{L3}(Q)=1+\frac{C_{1}arctan({\xi }_{\text{io}}Q/2)}{Q}+\frac{C_2}{1/{{\xi }_{\text{c}}}^2+{(Q-2\pi /d)}^2}\\ \approx 1+\frac{C_1\pi }{2Q}+\frac{C_2}{1/{{\xi }_{\text{c}}}^2+{(Q-2\pi /d)}^2},& (6)\end{array}$

where I_L3 denotes the SANS intensity of the sponge phase, ϕ_BPh the volume fraction of a ${\text{BPh}}_4^{-}$ ion in the mixture, r_d the radius of gyration of a disk membrane, ξ_io the inside–outside correlation length (which reflects the membrane scale [39]), and ξ_c the correlation length of cells. The terms C₁ and C₂ are constants. For example, the values of d and δ of the mixture at 340.8 K were evaluated as 92±1 and 16±1 Å, respectively [24].

As shown in Sadakane et al. [24], the lamellar structure in the mixture with antagonistic salt was assumed to be stabilized by the electrostatic interaction arising from ions. On the basis of this idea, Onuki et al. performed a numerical simulation of a water/organic solvent/antagonistic salt mixture and showed that multilamellar vesicles are certainly reproduced (see Figure 5) [13]. Their simulation is based on the Ginzburg-Landau theory considering the electrostatic interaction arising from ions. Here, it was found that the membrane was composed of organic solvent and hydrophobic anions, and hydrophilic cations distribute on the surface of the membrane. In addition, the intermembrane distance d and the membrane thickness η were evaluated as ca. 96 and 18 Å, respectively [13].

FIGURE 5

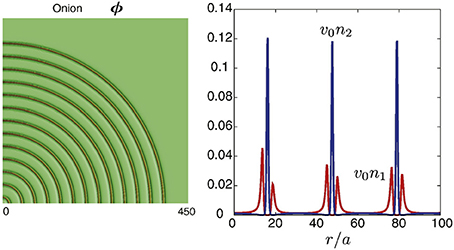
Figure 5. Numerically calculated multilamellar vesicle and charge density in water/organic solvent/antagonistic salt (hydrophilic cation and hydrophobic anion pairs) mixture. ϕ denotes the volume fraction of water, n₁ the number density of cations, and n₂ the number density of anions. $v_0=a^3$ is the molecular volume of solvent. r represents the distance from the center of the multilamellar vesicle. In the mixture, the average volume fraction of water in the mixture, < ϕ >, is set to 0.8. The figure on the left shows cross-sectional profile of ϕ, while the figure on the right shows the charge density around three membranes. The figure is reproduced from Onuki et al. [13] with permission from Copyright 2016 Elsevier Ltd.

In 2017, Tasios et al. performed a Monte Carlo simulation of the water/organic solvent/antagonistic salt mixture [34]. Their simulation was based on an Ising-like lattice model taking into account the effect of the electrostatic interaction arising from ions. As shown in Figure 6A, the lamellar structure was reproduced when the composition of water, x_B, was 0.5. Additionally, some other structures that have not been observed in experiments were also obtained: (Figure 6B) perforated lamellar at x_B = 0.4, (Figure 6C) tubular lamellar at x_B = 0.3 (lower temperature region), (Figure 6D) tubular at x_B = 0.35, (Figure 6E) tubular disordered at x_B = 0.3 (higher temperature region), and (Figure 6F) droplet phases at x_B = 0.2.

FIGURE 6

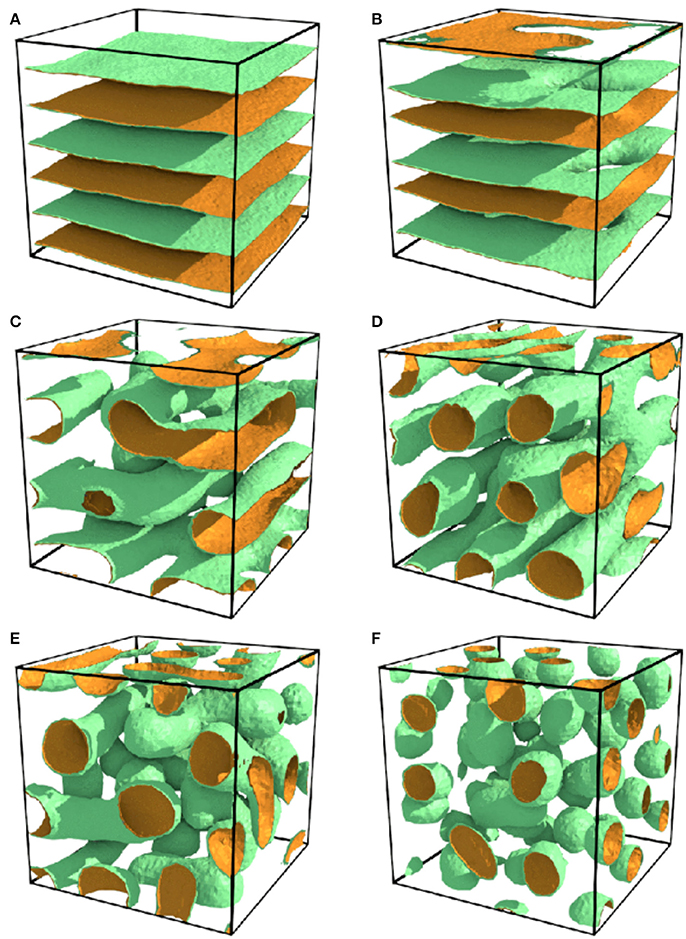
Figure 6. Ordered structures of water/organic solvent/antagonistic salt mixtures obtained by lattice-based Monte Carlo simulation. (A) Lamellar at x_B = 0.5, (B) perforated lamellar at x_B = 0.4, (C) tubular lamellar at x_B = 0.3 (lower temperature region), (D) tubular at x_B = 0.35, (E) tubular disordered at x_B = 0.3 (higher temperature region), and (F) droplet phases at x_B = 0.2. The figure is reproduced from Tasios et al. [34] with permission from Copyright 2017 by The American Physical Society.

Membrane formation in mixtures with antagonistic salts was also confirmed by neutron spin echo (NSE) spectroscopy, which could observe structural fluctuations in the scale of nanometers [24]. Figure 7A indicates an NSE result for D₂O/3MP/NaBPh₄ mixture (ϕ_3MP = 0.09, C_salt = 85 mmol/L, and T = 293.2 K). The observed intermediate correlation function can be explained using the formula proposed by Zilman and Granek [40]:

$\begin{array}{ll}\frac{I(Q,t)}{I(Q,0)}=exp\left[-{(\Gamma t)}^{2/3}\right],& (7)\end{array}$

where Γ and t denote the relaxation rate and Fourier time, respectively. In the case of membrane undulation, Γ is proportional to Q³:

$\begin{array}{ll}\Gamma =0.025{\gamma }_{\text{k}}{\left(\frac{k_{\text{B}}T}{{\kappa }_{\text{NSE}}}\right)}^{1/2}\frac{k_{\text{B}}T}{{\eta }_{\text{vis}}}{Q}^3,& (8)\end{array}$

where κ_NSE and η_vis denote the bending modulus of the membrane and the viscosity of the surrounding medium, respectively. As shown in Figure 7B, Γ is proportional to Q³. This evidence supports the idea that the nanostructure in the mixture with an antagonistic salt behaves as a membrane.

FIGURE 7

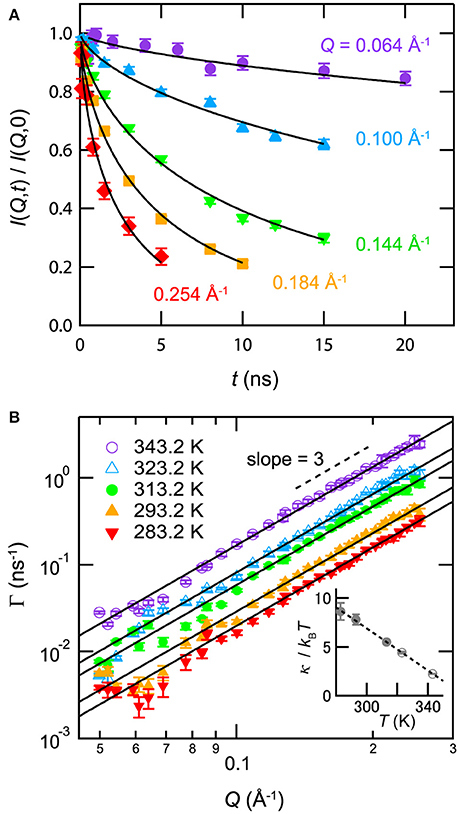
Figure 7. (A) Normalized intermediate correlation functions I(Q, t)/I(Q, 0) for the mixture of D₂O/3MP/NaBPh₄ (C_salt = 85 mmol/L, ϕ_3MP = 0.09, and T = 293.2 K). The solid lines indicate fitting results according to Equation (7). (B) Q dependence of the relaxation rate Γ obtained from the fit of I(Q, t)/I(Q, 0) according to Equation (7) for mixture of D₂O/3MP/NaBPh₄ (C_salt = 85 mmol/L, ϕ_3MP = 0.09, and 283.2K ≤ T ≤ 343.2K). The closed and open symbols indicate data for the lamellar phase and the sponge phase, respectively. The solid lines are the fitting results according to Equation (8). The inset in (B) shows the temperature dependence of κ_NSE/k_BT according to Equation (8). The closed and open symbols indicate data for the lamellar phase and the sponge phase, respectively. The dashed line is a visual guide. Error bars denote ±1 standard deviation. The figure is partly reproduced from Sadakane et al. [24] with permission from Copyright 2013 AIP Publishing LLC.

The inset of Figure 7B shows the temperature dependence of κ_NSE/k_BT according to Equation (7). κ_NSE/k_BT decreases linearly with increasing temperature. It is noted that the value of κ_NSE obtained from Equation (7) in surfactant systems deviates from the actual value because of the difference between the viscosity of water η_vis and the effective viscosity of bulk water near the membrane η_eff: in many cases, κ_NSE approaches a reasonable value when η_eff is substituted with η_eff ≈ 3η_vis [41–45]. Similarly, the values of κ_NSE/k_BT in the present case can deviate from reasonable values. In addition, the long-range electrostatic repulsion between membranes can suppress fluctuation [46], thereby increasing the value of κ_NSE from the actual value.

4. Charge-Density-Wave Structures Formed in Near-Critical Regions of the Mixtures

As shown in Figure 2A, a two-phase region exists in the mixture of D₂O/3MP with NaBPh₄ below 15 mmol/L. In the case of the binary D₂O/3MP mixture without salt, “normal critical opalescence” is shown in the vicinity of the critical point; i.e., the mixture becomes milky-white due to critical concentration fluctuation. On the other hand, it was found in 2007 that a mixture with NaBPh₄ (C_salt < 15 mmol/L) became blue far from the phase separation temperature and that the color changed to green and orange with increasing temperature (see Figure 8) [16]. This result suggests that a periodic structure whose characteristic repeat distance was comparable to the wavelength of visible light was formed, and its repeat distance increased with increasing temperature.

FIGURE 8

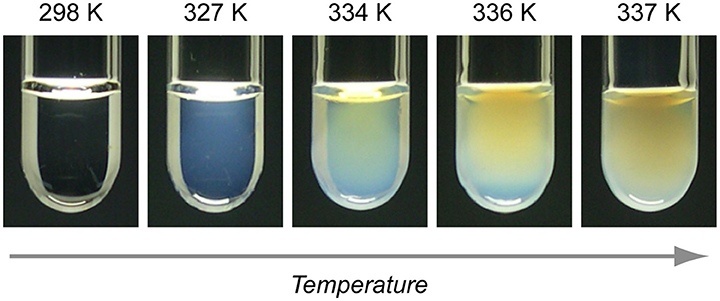
Figure 8. Result of visual inspection of D₂O/3MP/NaBPh₄ mixture (ϕ_3MP = 0.35 and C_salt = 13 mmol/L). The mixture is blue at T = 327K, green at 334 K, and orange at 337 K. The figure is reproduced from Sadakane et al. [16] (Copyright 2007 by The Physical Society of Japan). As per the copyright holder's policies, no permission is required for the use of figures by the authors of the original article.

The nanometer scale structures formed in such mixtures were investigated by SANS in 2011. As shown in Figure 9A, SANS profiles for the mixture of D₂O/3MP (ϕ_3MP = 0.35) follow the Ornstein-Zernike equation [47], suggesting that D₂O-rich and 3MP-rich domains are distributed randomly and the critical concentration fluctuation occurs without forming ordered structure [22]. On the other hand, SANS profiles of D₂O/3MP/NaBPh₄ (ϕ_3MP = 0.35 and C_salt = 6 mmol/L) mixture were explained by the model scattering function of the CDW structure [8, 17, 20]:

$\begin{array}{ll}{I}_{\text{OK}}(Q)=\frac{I_0}{1+\left\{1-{{\gamma }_{\text{p}}}^2/\left(1+{{\lambda }_D}^2{Q}^2\right)\right\}{\xi }^2{Q}^2},& (9)\end{array}$

where I₀ denotes forward scattering proportional to the osmotic compressibility, ξ the correlation length of concentration fluctuation, λ_D the Debye screening length, and γ_p ≥ 0 a dimensionless parameter related to the difference in solubility between cations and anions in polar solvents, i.e., water (see Figure 9B). It is noted that Equation (9) corresponds to the Ornstein-Zernike equation when γ_p = 0. According to the theoretical study by Onuki and Kitamura in 2004 [8], it is suggested that a semi-micro-scale long-range periodic structure is formed as the CDW structure due to strong coupling between the concentration fluctuation of solvents and the preferential solvation of ions.

FIGURE 9

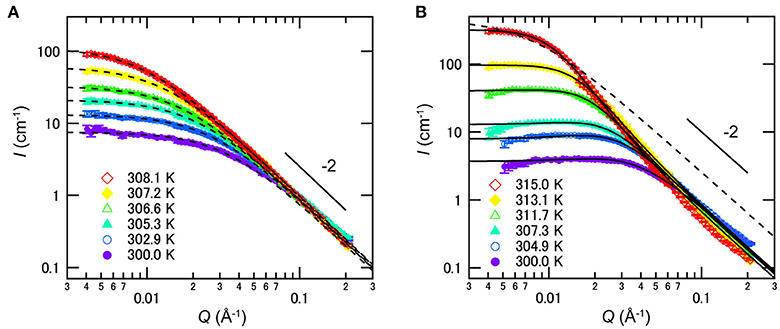
Figure 9. Temperature dependences of SANS profiles of mixtures of D₂O/3MP (ϕ_3MP = 0.35) (A) without NaBPh₄ and (B) with 6 mmol/L of NaBPh₄. The dashed and solid lines are fitting results according to the Ornstein-Zernike equation [47] and Equation (9), respectively. Error bars in the SANS profiles denote ±1 standard deviation. The figure is reproduced from Sadakane et al. [22] (Copyright The Royal Society of Chemistry 2011). As per the copyright holder's policies, no permission is required for the use of figures by the authors of the original article.

Formation of the CDW structure was also observed in the mixtures of water/3MP/PPh₄Cl [23], water/2,6-dimethylpyridine/polystyrene/NaBPh₄ [27], water/ethanol/1-octane/antagonistic salts (NaBPh₄ and sodium thiocyanate) [28], water/2,6-dimethylpyridine/tetraheptylammonium bromide [30], and water/acetonitrile/NaBPh₄ [31] by means of small-angle X-ray scattering (SAXS). Figure 10 shows the example of the SAXS profiles for the H₂O/acetonitrile/NaBPh₄ mixtures [31]. The profiles for the binary H₂O/acetonitrile (Figure 10A) was well explained by the Ornstein-Zernike equation, suggesting that no ordered structure was formed in the mixture. On the other hand, profiles of the mixture of H₂O/acetonitrile with 50 mmol/L NaBPh₄ (Figure 10B) indicated that the CDW structure was formed since the Bragg peak arising from the long-range periodic structure was shown. Here, Bier et al. claimed that the long-range monopole-dipole interaction between solvents and ions is a key factor in the formation of the CDW structure [31].

FIGURE 10

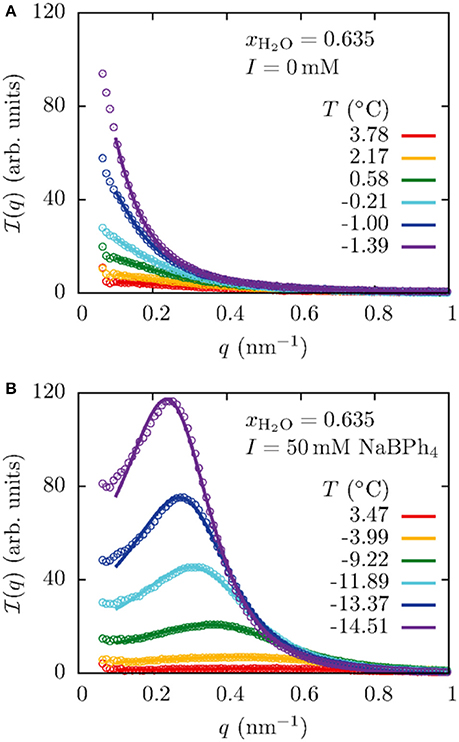
Figure 10. (A) Temperature dependence of the SAXS profiles of the H₂O/acetonitrile mixture. q denotes the momentum transfer and x_H2O the mole fraction of water in the mixture. The solid lines show fitting results according to the Ornstein-Zernike equation. (B) Temperature dependence of the SAXS profiles for the mixture H₂O/acetonitrile with 50 mmol/L of NaBPh₄. The solid lines show the fitting result according to the model scattering function for the charge-density-wave structure, taking into account the effect of the long-range monopole-dipole interaction between solvents and ions [31]. The figure is reproduced from Bier et al. [31] with permission from Copyright 2017 by The American Physical Society.

The detailed morphology of the CDW structure was numerically examined by Araki and Onuki based on the Ginzburg-Landau theory [10, 11, 48, 49]. In their model, large concentration fluctuation of solvents is taken into account, unlike in Figure 5. Typical results of the simulation are shown in Figure 11. The stretched water-rich and organic solvent-rich domains are distributed periodically in the mixture. Then, hydrophilic cations are present on the surfaces of the organic solvent-rich domains, whereas hydrophobic anions exist inside the organic solvent-rich domains. These images are similar to the bicontinuous structures formed in surfactant mixtures. In this case, the organic solvent-rich domains and hydrophobic anions can be regarded as a membrane, similar to the lamellar and sponge structures shown in section 3.

FIGURE 11

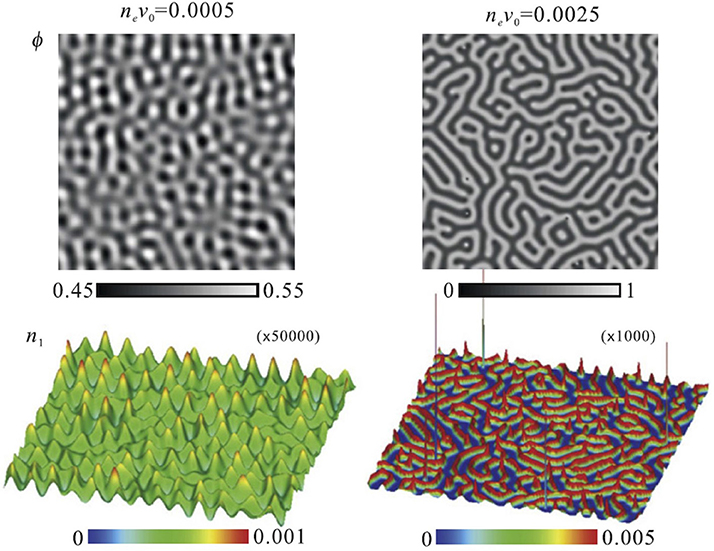
Figure 11. Morphologies of concentration fluctuation (upper figures) and charge density (lower figures) in the charge-density-wave phase obtained by Araki and Onuki by numerical simulation. ϕ, n₁, n_e, and v₀ are the volume fraction of water in the mixture, the cation density, the average ion density, and the solvent molecular volume, respectively. The figure is reproduced from Araki and Onuki [10] with permission from Copyright 2009 IOP 1 Publishing Ltd.

The evidence that water-rich and organic solvent-rich domains are stretched as 2-dimensional planes could be confirmed in the critical phenomena. In the near-critical region, the temperature dependence of ξ follows [47]

$\begin{array}{ll}\xi ={\xi }_0{\left|\frac{T_{\text{c}}-T}{T_{\text{c}}}\right|}^{-\nu },& (10)\end{array}$

where ξ₀ denotes the bare correlation length, T_c the critical temperature, and ν the critical index for ξ. In most cases of simple liquid mixtures (including the D₂O/3MP mixture), ν indicates the value of the 3D-Ising universality class, i.e., ν = 0.63 [50]. On the other hand, SANS measurements of D₂O/3MP mixtures revealed that the value of ν changes from 0.63 (3D-Ising) to 1.00 (2D-Ising) with the addition of an antagonistic salt (see Figure 12) [22]. Thus, it was interpreted that the concentration fluctuation of D₂O and 3MP has the 2-dimensional nature in the presence of NaBPh₄. This tendency is consistent with the idea that water-rich and organic solvent-rich domains are stretched in planes in the CDW, as mentioned above.

FIGURE 12

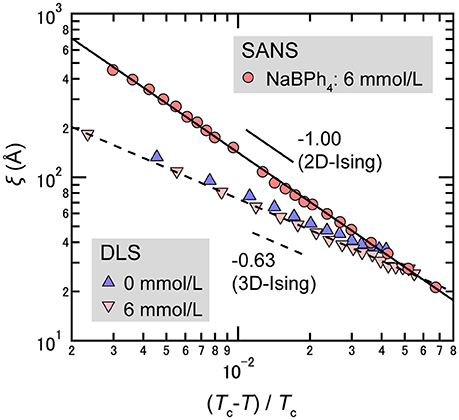
Figure 12. Temperature dependence of ξ for mixtures of D₂O/3MP (ϕ_3MP = 0.35) and D₂O/3MP/NaBPh₄ (ϕ_3MP = 0.35 and C_salt = 85 mmol/L). The circles are data obtained by SANS measurements [22], while the triangles and the inverted triangles are data obtained by DLS measurements [25].

However, it should be pointed out that this interpretation is contradicted by the results of dynamic light scattering (DLS) measurements [25], shown in Figure 12; the value of ν is 0.63 even in the presence of NaBPh₄ (6 mmol/L). A possible reason for the discrepancy in critical behavior could be the fact that the correlation lengths observed from SANS and DLS are quite different. The correlation length observed from SANS may be modified because the values observed are comparable to the length scale of the periodic structure and the Debye correlation length. This point has not yet been clarified and is subject to debate.

5. Summary

In this article, the formation of the ordered structures induced by antagonistic salts in the water/organic solvent mixtures has been reviewed. Hydrophilic and hydrophobic ions preferentially dissolve in polar and less-polar solvents, respectively, and stabilize the interface between these solvents through the Coulomb interaction. Thus, the interfacial tension decreases and a microphase separation is induced. In the water-rich region of the water/organic solvent/antagonistic salt mixtures (e.g., the water/3MP/NaBPh₄ and the water/2,6-dimethylpyridine/NaBPh₄ mixtures), the lamellar and the sponge structures were observed by optical microscopy, small-angle neutron scattering (SANS), and neutron spin echo (NSE). In such phases, it was assumed that the membrane is composed of organic solvent and hydrophobic ions. The formation of the lamellar structure was also confirmed by the numerical simulations taking into account the effect of the electrostatic interaction arising from ions. On the other hand, the formation of the charge-density-wave (CDW) structure was confirmed in near-critical regions of the water/organic solvent/antagonistic salt mixtures (e.g., the water/3MP/NaBPh₄ and the water/acetonitrile/NaBPh₄ mixtures) by SANS and small-angle X-ray scattering (SAXS). Furthermore, it was suggested that the organic solvent-rich domains behave as a membrane in the CDW phase, as in the case of the lamellar or the sponge phase. These series of findings will motivate further theoretical and experimental investigations for exploring the nature of the self-organization of soft materials containing ions or polyelectrolytes.

Author Contributions

KS and HS wrote the paper.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

We would like to express our gratitude to our collaborators, Prof. Onuki, Prof. Shibayama, Prof. Nishida, Prof. Koizumi, Dr. Nagao, Prof. Endo, and the late Dr. Y. B. Melnichenko. We also appreciate various grants to support our research activities and the neutron scattering beamtime awarded.

References

1. Eckfeldt EL, Lucasse WW. The liquid-liquid phase equilibria of the system cyclohexane-methyl alcohol in the presence of various salts as third components. J Phys Chem. (1943) 47:164–83.

Google Scholar

2. Hales BJ, Bertrand GL, Hepler LG. Effects of 3rd components on critical mixing in water-triethylamine system. J Phys Chem. (1966) 70:3970–5.

Google Scholar

3. Takamuku T, Yamaguchi A, Matsuo D, Tabata M, Yamaguchi T, Otomo T, et al. Nacl-induced phase separation of 1,4-dioxane-water mixtures studied by large-angle X-ray scattering and small-angle neutron scattering techniques. J Phys Chem B (2001) 105:10101–10. doi: 10.1021/jp011692w

CrossRef Full Text | Google Scholar

4. Misawa M, Yoshida K, Maruyama K, Munemura H, Hosokawa Y. Salt-induced phase separation in aqueous solution. J Phys Chem Solids (1999) 60:1301–6.

Google Scholar

5. Schall E. Volumetric determination of potassium. Anal Chem. (1957) 29:1044–6.

Google Scholar

6. Marcus Y, (ed.). Ion Solvation. New York, NY: Wiley (1985).

Google Scholar

7. Sadakane K, Fujii K, Tsuzuki S, Watanabe H, Umebayashi Y. Solvation state of sodium tetraphenylborate in 3-methylpyridine and its aqueous solutions. J Mol Liq. (2017) 248:53–9. doi: 10.1016/j.molliq.2017.10.006

CrossRef Full Text | Google Scholar

8. Onuki A, Kitamura H. Solvation effects in near-critical binary mixtures. J Chem Phys. (2004) 121:3143–51. doi: 10.1063/1.1769357

PubMed Abstract | CrossRef Full Text | Google Scholar

9. Onuki A. Surface tension of electrolytes: hydrophilic and hydrophobic ions near an interface. J Chem Phys. (2008) 128:224704. doi: 10.1063/1.2936992

PubMed Abstract | CrossRef Full Text | Google Scholar

10. Araki T, Onuki A. Dynamics of binary mixtures with ions: dynamic structure factor and mesophase formation. J Phys Condens Matter (2009) 21:424116. doi: 10.1088/0953-8984/21/42/424116

PubMed Abstract | CrossRef Full Text | Google Scholar

11. Onuki A, Araki T, Okamoto R. Solvation effects in phase transitions in soft matter. J Phys Condens Matter (2011a) 23:284113. doi: 10.1088/0953-8984/23/28/284113

PubMed Abstract | CrossRef Full Text | Google Scholar

12. Onuki A, Okamoto R. Selective solvation effects in phase separation in aqueous mixtures. Curr Opin Coll Interface Sci. (2011) 16:525–33. doi: 10.1016/j.cocis.2011.04.002

CrossRef Full Text | Google Scholar

13. Onuki A, Yabunaka S, Araki T, Okamoto R. Structure formation due to antagonistic salts. Curr Opin Coll Interface Sci. (2016) 22:59–64. doi: 10.1016/j.cocis.2016.02.007

CrossRef Full Text | Google Scholar

14. Onuki A. Ginzburg-landau theory of solvation in polar fluids: ion distribution around an interface. Phys Rev E Stat Nonlin Soft Matter Phys. (2006) 73:021506. doi: 10.1103/PhysRevE.73.021506

PubMed Abstract | CrossRef Full Text | Google Scholar

15. Luo GM, Malkova S, Yoon J, Schultz DG, Lin BH, Meron M, et al. Ion distributions near a liquid-liquid interface. Science (2006) 311:216–8. doi: 10.1126/science.1120392

PubMed Abstract | CrossRef Full Text | Google Scholar

16. Sadakane K, Seto H, Endo H, Shibayama M. A periodic structure in a mixture of D₂O/3-methylpyridine/NaBPh₄ induced by solvation effect. J Phys Soc Jpn. (2007) 76:113602. doi: 10.1143/JPSJ.76.113602

CrossRef Full Text | Google Scholar

17. Bier M, Harnau L. The structure of fluids with impurities. Z Phys Chem. (2012) 226:807–14. doi: 10.1524/zpch.2012.0260

CrossRef Full Text | Google Scholar

18. Bier M, Gambassi A, Dietrich S. Local theory for ions in binary liquid mixtures. J Chem Phys. (2012) 137:034504. doi: 10.1063/1.4733973

PubMed Abstract | CrossRef Full Text | Google Scholar

19. Pousaneh F, Ciach A, Maciolek A. Effect of ions on confined near-critical binary aqueous mixture. Soft Matter (2012) 8:7567–81. doi: 10.1039/C2SM25461A

CrossRef Full Text | Google Scholar

20. Pousaneh F, Ciach A. The effect of antagonistic salt on a confined near-critical mixture. Soft Matter (2014) 10:8188–201. doi: 10.1039/c4sm01264j

PubMed Abstract | CrossRef Full Text | Google Scholar

21. Sadakane K, Onuki A, Nishida K, Koizumi S, Seto H. Multilamellar structures induced by hydrophilic and hydrophobic ions added to a binary mixture of D₂O and 3-methylpyridine. Phys Rev Lett. (2009) 103:167803. doi: 10.1103/PhysRevLett.103.167803

PubMed Abstract | CrossRef Full Text | Google Scholar

22. Sadakane K, Iguchi N, Nagao M, Endo H, Melnichenko YB, Seto H. 2D-Ising-like critical behavior in mixtures of water and 3-methylpyridine including antagonistic salt or ionic surfactant. Soft Matter (2011) 7:1334–40. doi: 10.1039/C0SM00598C

CrossRef Full Text | Google Scholar

23. Sadakane K, Horikawa Y, Nagao M, Seto H. The effect of tetraphenylphosphonium chloride on phase behavior and nanoscale structures in a mixture of D₂O and 3-methylpyridine. Chem Lett. (2012) 41:1075–7. doi: 10.1246/cl.2012.1075

CrossRef Full Text | Google Scholar

24. Sadakane K, Nagao M, Endo H, Seto H. Membrane formation by preferential solvation of ions in mixture of water, 3-methylpyridine, and sodium tetraphenylborate. J Chem Phys. (2013) 139:234905. doi: 10.1063/1.4838795

PubMed Abstract | CrossRef Full Text | Google Scholar

25. Leys J, Subramanian D, Rodezno E, Hammouda B, Anisimov MA. Mesoscale phenomena in solutions of 3-methylpyridine, heavy water, and an antagonistic salt. Soft Matter (2013) 9:9326–34. doi: 10.1039/C3SM51662H

CrossRef Full Text | Google Scholar

26. Sadakane K, Endo H, Nishida K, Seto H. Lamellar/disorder phase transition in a mixture of water/2,6-dimethylpyridine/antagonistic salt. J Solution Chem. (2014) 43:1722–31. doi: 10.1007/s10953-014-0240-x

CrossRef Full Text | Google Scholar

27. Samin S, Hod M, Melamed E, Gottlieb M, Tsori Y. Experimental demonstration of the stabilization of colloids by addition of salt. Phys Rev Appl. (2014) 2:024008. doi: 10.1103/PhysRevApplied.2.024008

CrossRef Full Text | Google Scholar

28. Marcus J, Touraud D, Prévost S, Diat O, Zemb T, Kunz W. Influence of additives on the structure of surfactant-free microemulsions. Phys Chem Chem Phys. (2015) 17:32528–38. doi: 10.1039/C5CP06364G

PubMed Abstract | CrossRef Full Text | Google Scholar

29. Michler D, Shahidzadeh N, Westbroek M, van Roij R, Bonn D. Are antagonistic salts surfactants? Langmuir (2015) 31:906–11. doi: 10.1021/la504801g

PubMed Abstract | CrossRef Full Text | Google Scholar

30. Witala M, Lages S, Nygård K. Mesoscale ordering in binary aqueous solvents induced by ion size asymmetry. Soft Matter (2016) 12:4778–82. doi: 10.1039/c6sm00580b

PubMed Abstract | CrossRef Full Text | Google Scholar

31. Bier M, Mars J, Li H, Mezger M. Salt-induced microheterogeneities in binary liquid mixtures. Phys Rev E (2017) 96:022603. doi: 10.1103/PhysRevE.96.022603

PubMed Abstract | CrossRef Full Text | Google Scholar

32. Silver BR, Holub K, Marecek V. Spontaneous emulsification at surfactantless liquid/liquid interfaces. J Electroanal Chem. (2017) 805:91–7. doi: 10.1016/j.jelechem.2017.10.027

CrossRef Full Text | Google Scholar

33. Winkler R, Buchecker T, Hastreiter F, Touraud D, Kunz W. PPh₄Cl in aqueous solution–the aggregation behavior of an antagonistic salt. Phys Chem Chem Phys. (2017) 19:25463–70. doi: 10.1039/C7CP02677C

PubMed Abstract | CrossRef Full Text | Google Scholar

34. Tasios N, Samin S, van Roij R, Dijkstra M. Microphase separation in oil-water mixtures containing hydrophilic and hydrophobic ions. Phys Rev Lett. (2017) 119:218001. doi: 10.1103/PhysRevLett.119.218001

PubMed Abstract | CrossRef Full Text | Google Scholar

35. Narayanan T, Kumar A. Reentrant phase-transitions in multicomponent liquid-mixtures. Phys Rep Rev Sect Phys Lett (1994) 249:136–218.

Google Scholar

36. Nallet F, Laversanne R, Roux D. Modeling x-ray or neutron-scattering spectra of lyotropic lamellar phases - interplay between form and structure factors. J Phys II (1993) 3:487–502.

Google Scholar

37. Lei N, Safinya C, Roux D, Liang K. Synchrotron x-ray-scattering studies on the sodium dodecyl sulfate-water-pentanol-dodecane l₃ sponge phase. Phys Rev E (1997) 3:608–13.

38. Porcar L, Hamilton WA, Butler PD, Warr GG. Scaling of structural and rheological response of l-3 sponge phases in the “sweetened” cetylpyridinium/hexanol/dextrose/brine system. Langmuir (2003) 19:10779–94. doi: 10.1021/la034661w

CrossRef Full Text | Google Scholar

39. Roux D, Coulon C, Cates ME. Sponge phases in surfactant solutions. J Phys Chem. (1992) 96:4174–87.

Google Scholar

40. Zilman AG, Granek R. Undulations and dynamic structure factor of membranes. Phys Rev Lett. (1996) 77:4788–91.

PubMed Abstract | Google Scholar

41. Takeda T, Kawabata Y, Seto H, Komura S, Ghosh SK, Nagao M, et al. Neutron spin-echo investigations of membrane undulations in complex fluids involving amphiphiles. J Phys Chem Solids (1999) 60:1375–7.

Google Scholar

42. Yi Z, Nagao M, Bossev DP. Bending elasticity of saturated and monounsaturated phospholipid membranes studied by the neutron spin echo technique. J Phys Condens Matter (2009) 21:155104. doi: 10.1088/0953-8984/21/15/155104

PubMed Abstract | CrossRef Full Text | Google Scholar

43. Nagao M. Observation of local thickness fluctuations in surfactant membranes using neutron spin echo. Phys Rev E (2009) 80:031606. doi: 10.1103/PhysRevE.80.031606

PubMed Abstract | CrossRef Full Text | Google Scholar

44. Watson MC, Brown FLH. Interpreting membrane scattering experiments at the mesoscale: the contribution of dissipation within the bilayer. Biophys J. (2010) 98:L9–11. doi: 10.1016/j.bpj.2009.11.026

PubMed Abstract | CrossRef Full Text | Google Scholar

45. Lee JH, Choi SM, Doe C, Faraone A, Pincus PA, Kline SR. Thermal fluctuation and elasticity of lipid vesicles interacting with pore-forming peptides. Phys Rev Lett. (2010) 105:038101. doi: 10.1103/PhysRevLett.105.038101

PubMed Abstract | CrossRef Full Text | Google Scholar

46. Israelachvili JN, (ed.). Intermolecular and Surface Forces. London: Academic Press (1991).

Google Scholar

47. Stanley HE, (ed.). Introduction to Phase Transitions and Critical Phenomena. Oxford: Oxford University Press (1971).

Google Scholar

48. Onuki A, Okamoto R, Araki T. Phase transitions in soft matter induced by selective solvation. Bull Chem Soc Jpn. (2011b) 84:569–87. doi: 10.1246/bcsj.20110012

CrossRef Full Text | Google Scholar

49. Onuki A, Araki T. Selective solvation in aqueous mixtures: interface deformations and instability. J Phys Soc Jpn. (2012) 81:SA004. doi: 10.1143/JPSJS.81SA.SA004

CrossRef Full Text | Google Scholar

50. Unni PKM. Critical behavior of the aqueous electrolytic system 3-methylpyridine+D₂O+NaBr. J Chem Phys. (2006) 124:054505. doi: 10.1063/1.2167640

CrossRef Full Text | Google Scholar