
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Photonics , 05 April 2023
Sec. Nonlinear Optics
Volume 4 - 2023 | https://doi.org/10.3389/fphot.2023.1051294
This article is part of the Research Topic Frontiers in Photonics: Rising Stars in Europe View all 3 articles
We present the theory of parametric amplification in coupled nonlinear waveguides considering the frequency dependency of the coupling strength. We show that coupling dispersion can indeed compensate for the uncoupled individual waveguides dispersion enabling a substantial tailoring of the gain spectrum. Our theory describes both phase-sensitive and phase-insensitive operational modes, it can be straightforwardly generalized to include arbitrary higher-order waveguide and coupling dispersion and its predictions agree very well with numerical simulations both in the presence and in the absence of waveguide losses. It provides a tool for the design of novel versatile parametric amplifiers based both on coupled integrated waveguides and dual-core fibers too.
Optical parametric amplifiers are devices with great technological potential for signal amplification in optical communications. This is due to their broad bandwidth, low noise figure and exploitation of waveguides intrinsic Kerr nonlinearity, not requiring any particular rare-earth based material doping. Parametric amplification is based on the four-wave mixing process where two photons from a powerful pump wave are annihilated and two photons are created at different frequencies called signal and idler respectively, provided that certain phase-matching conditions are satisfied (Stolen and Bjorkholm, 1982; Mahric, 2007). Parametric amplification has been demonstrated in a variety of configurations in nonlinear optical fibers (Hansryd et al., 2002; Takasaka et al., 2012; Gordienko et al., 2017; Olsson et al., 2018; Andrekson and Karlsson, 2020; Gordienko et al., 2023). However it can be achieved also in silicon and silicon nitride waveguides which are promising for optical amplification in integrated devices (Ye et al., 2021a; Ye et al., 2021b).
Parametric amplification in two coupled nonlinear waveguides has been suggested very generally in the past in the context of nonlinear optical couplers (Mecozzi, 1988; Trillo et al., 1989). It has been recently proposed as a promising practical solution for optical communications featuring: flat gain profile, phase-matching in the normal dispersion regime thanks to the coupling contribution, and 0-dB noise figure in the phase-sensitive operational mode (Ribeiro et al., 2017; 2018). Preliminary experimental results in a dual-core highly nonlinear fiber have been obtained too (Szabo et al., 2021; Ribeiro et al., 2022). Some of the authors of the present paper have furthermore shown that if the coupling is chosen with a proper spatial dependence, it can compensate for the mismatch dynamically arising due to the pump attenuation (Ribeiro and Perego, 2022a; Ribeiro and Perego, 2022b), which could solve a substantial problem in integrated amplifiers where the attenuation is of the order of 1 dB/m (Karlsson et al., 2021). The latter coupled waveguides geometry can lead, for specific parameters choice, to superior performances in terms of bandwidth and bandwidth-gain product, compared both to the standard and to the tapered single waveguide silicon nitride parametric amplifiers (Zhao et al., 2020).
In the present work, we show that the frequency dependency of the coupling, and in particular the coupling dispersion—the second derivative of the coupling strength with respect to frequency evaluated at the pump frequency—can play an important role in compensating for the chromatic dispersion of the individual waveguides. This is due to the collective behavior of the interacting waves propagating along coupled waveguides. The justification of the relevance of this particular effect is grounded on the fact that coupled nonlinear waveguides can be fabricated in such a way that the contribution of the coupling dispersion is strong enough to compensate for the individual waveguide dispersion. Indeed, the role of the frequency dependent coupling strength has been explored in the past especially in the study of coupled silicon waveguides. In that context it enables the existence of solitons and modulation instability in conditions where these phenomena would not be possible in the single uncoupled waveguide (Benton and Skryabin, 2009; de Nobriga et al., 2010; Ding et al., 2012). However, the role of coupling dispersion in parametric amplification has not yet been investigated analytically to the best of our knowledge. The impact on the modulation instability spectrum of the first coefficient in the Taylor expansion of the coupling around the pump frequency has been theoretically studied in the past for two evanescently coupled core fibers (Li et al., 2011; 2012) and also in generalized forms of the two coupled nonlinear Schrödinger equations (Nithyanandan et al., 2013; Nair et al., 2018; Li et al., 2020a; Li et al., 2020b). It has been shown that this term can lead to the generation of additional spectral sidebands both in the normal and in the anomalous dispersion regime, provided that the pump power distribution is asymmetric in the two waveguides. In this work we show a different phenomenon and, namely, that the second-order term in the expansion can enable complete compensation of the group velocity dispersion of the single waveguide in the case when the pump power distribution is symmetric in the two waveguides. We provide analytical formulas for the parametric gain in the phase-sensitive (PS) and phase-insensitive (PI) regime too, considering characteristic parameters for both lossless coupled core fibers and lossy integrated silicon nitride waveguides amplifiers. This provides a novel tool for dispersion engineering to be exploited in the design of broadband, flat gain, low noise figure, and energy efficient parametric amplifiers.
The starting point of our theory consists of the two nonlinear Schrödinger equations which rule the propagation of the electric field amplitudes A1,2 along two identical coupled waveguides:
βn and Cn are the n-th coefficient of the Taylor expansion of the frequency-dependent propagation constant β(ω) and coupling C(ω) respectively, γ and α are the nonlinearity and attenuation coefficients, t is the temporal coordinate and z is the spatial evolution coordinate along the longitudinal waveguides dimension. In the context of degenerate four-wave mixing, the electric field amplitudes A1,2 can be expressed as combinations of pump, signal, and idler waves oscillating with angular frequency ω0, ωs = ω0 + Ω and ωi = ω0 − Ω, and with amplitudes up1,p2, us1,s2 and ui1,i2 respectively, reading
The substitution of Eq. 2 into the coupled NLSEs (Eq. 1a and 1b) yields six coupled equations governing the propagation of the six waves. The equations for waveguide 1 read
where the small sidebands approximation |up|2 ≫|us,i|2 has been considered. Swapping indexes 1 and 2 gives other three equations for waveguide 2.
We focus on the case where the pump waves are identical in the two waveguides with common initial phase ϕ0, i.e.,
where Pp is the total input pump power and
where
with
with
To highlight the role of coupling dispersion in the parametric amplification process we calculated analytically the z-dependent gain for us− = us1 − us2 — the signal of supermode A− —, defined as the ratio between the value of us− square modulus at coordinate z to its value at the input of the amplifier:
where
where
The dependency of the gain on the initial phase ϕs is shown in Figures 3A, B for the lossy amplifier. We then fixed ϕs to maximize the gain, and calculated the gain spectrum both analytically and numerically, which is shown in Figures 3C, D, where the PI gain is also reported as a reference. An excellent agreement between theory and simulations is achieved in this case too. We note that in this work we have considered the signal of “−” supermode as the amplifier output. If the signal of “+” supermode us+ = us1 + us2 is chosen instead, then analogous dispersion compensation effects arise but requiring C2 with the opposite sign.
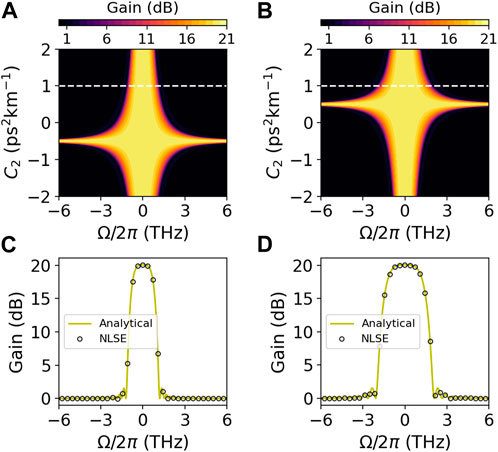
FIGURE 1. Phase insensitive lossless amplifier Map of parametric analytical gain versus frequency and C2 in anomalous (A) and normal (B) dispersion regime; and gain spectrum versus frequency comparison between numerics and theory for anomalous (C) and normal (D) dispersion regime with C2 = 1 ps2km−1 (corresponding to dashed lines in (A,B). Other parameters used are β2 =±0.5 ps2km−1, γ = 10 W−1km−1, Pp = 6 W, C0 = 15 km−1 and z = 0.1 km. The input signal wave into waveguide 1 used in simulations is
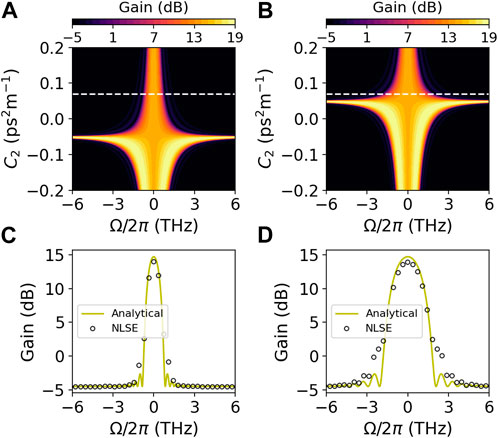
FIGURE 2. Phase insensitive lossy amplifier Map of parametric analytical gain versus frequency and C2 in anomalous (A) and normal (B) dispersion regime; and gain spectrum versus frequency comparison between numerics (circles) and theory (solid lines) for anomalous (C) and normal (D) dispersion regime with C2 = 0.07 ps2m−1 (corresponding to dashed lines in (A,B). Other parameters used are β2 = ±0.05 ps2m−1, γ = 1.2 W−1m−1, Pp = 6 W, C0 = 1.8 m−1, z = 1.5 m and α = 0.69 m−1 corresponding to 3 dBm−1. The input signal wave into waveguide 1 used in simulations is

FIGURE 3. Phase sensitive lossy amplifier Map of parametric gain calculated analytically versus frequency and ϕs in anomalous (A) and normal (B) dispersion regime; gain spectrum versus frequency for anomalous (C) and normal (D) dispersion regime with ϕs = 0.35π (corresponding to dashed lines in (A,B) comparing numerics (diamonds) with analytics (red solid lines). The phase insensitive gain spectrum from Figure 2 is also shown as a reference. Other parameters used are β2 = ±0.05 ps2m−1, γ = 1.2 W−1m−1, Pp = 6 W, C0 = 1.8 m−1, z = 1.5 m, C2 = 0.07 ps2m−1 and α = 0.69 m−1 corresponding to 3 dBm−1. The input signal wave into waveguide 1 used in simulations is
It is furthermore important to mention that the performances of the lossy parametric amplifier with frequency dependent coupling could be improved in terms of gain-bandwidth product by exploiting the benefits of spatially dependent coupling to compensate for pump attenuation induced phase-matching degradation. This has been demonstrated in (Ribeiro and Perego, 2022a; Ribeiro and Perego, 2022b) for coupling having a constant frequency profile. A detailed analysis of the coupled waveguides parametric amplifier with both spatially and frequency dependent coupling will be presented in a future work.
Compared to a recent numerical study of parametric amplification in coupled waveguides, also considering wavelength dependency of the coupling strength (Su and Biaggio, 2022), our work provides elegant, practical and easy-to-use analytical expressions for the design of an effective dual-waveguide parametric amplifier, accounting for coupling dispersion of an arbitrary order. Considering terms of order greater than 2, both in the single waveguide and in the coupling dispersion, is particularly relevant when the net combined contribution of both single waveguide group velocity and second-order coupling dispersion is close to zero, and there is a need to describe parametric amplifiers operating over a larger bandwidth. In that case, higher-order waveguide dispersion and coupling dispersion terms in the expansion must be taken into account and constitute the limiting factors for the gain spectrum. These terms can be straightforwardly included in the theoretical framework presented in this work and their magnitude would depend on the particular fabrication geometry and materials, which should be evaluated on an individual basis. Furthermore our theory can be also generalized to include the effects of free carrier dispersion (Chaturvedi et al., 2017) and two-photon absorption (Tsoy et al., 2001) which are relevant in silicon waveguides.
In conclusion, we have shown that coupling dispersion is a fundamental physical effect that enables dispersion engineering in dual-waveguide parametric amplifiers, determining substantial tailoring of the gain spectrum and offering the possibility of broadband parametric amplification also when the individual waveguides have normal dispersion. This powerful tool adds a substantial degree of versatility to the already promising features of dual-waveguide parametric amplifiers such as flat gain spectrum, 0-dB noise figure, and compensation of loss induced mismatch. Further developments in the study of frequency dependent coupling for dispersion compensation can be relevant for fiber amplifiers with a large number of cores and for arrays of integrated waveguides with different coupling topologies, as well as for other photonic devices that involve multiple coupled modes.
The raw data supporting the conclusion of this article will be made available by the authors, upon reasonable request.
AMP and VR initiated the study, MS performed the analytical calculations and the numerical simulations, all the authors analyzed the data, AMP wrote the manuscript with inputs from VR and MS
All the authors acknowledge support from EPSRC project EP/W002868/1. AMP acknowledges support from the Royal Academy of Engineering through the Research Fellowship scheme.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Alem, M., Soto, M. A., and Thévenaz, L. (2015). Analytical model and experimental verification of the critical power for modulation instability in optical fibers. Opt. Express 23, 29514–29532. doi:10.1364/oe.23.029514
Andrekson, P. A., and Karlsson, M. (2020). Fiber-based phase-sensitive optical amplifiers and their applications. Adv. Opt. Phot. 12, 367–428. doi:10.1364/aop.382548
Benton, C. J., and Skryabin, D. V. (2009). Coupling induced anomalous group velocity dispersion in nonlinear arrays of silicon photonic wires. Opt. Express 17, 5879–5884. doi:10.1364/oe.17.005879
Chaturvedi, D., Mishra, A. K., and Kumar, A. (2017). Analysis of free carrier effects on modulational instability in silicon-on-insulator nano-waveguides. J. Opt. Soc. Am. B 34, 1060–1069. doi:10.1364/josab.34.001060
de Nobriga, C. E., Hobbs, G. D., Wadsworth, W. J., Knight, J. C., Skryabin, D. V., Samarelli, A., et al. (2010). Supermode dispersion and waveguide-to-slot mode transition in arrays of silicon-on-insulator waveguides. Opt. Lett. 35, 3925–3927. doi:10.1364/ol.35.003925
Ding, W., Staines, O. K., Hobbs, G. D., Gorbach, A. V., de Nobriga, C., Wadsworth, W. J., et al. (2012). Modulational instability in a silicon-on-insulator directional coupler: Role of the coupling-induced group velocity dispersion. Opt. Lett. 37, 668–670. doi:10.1364/ol.37.000668
Gordienko, V., Ferreira, F. M., Ribeiro, V., and Doran, N. (2023). Design of an interferometric fiber optic parametric amplifier for the rejection of unwanted four-wave mixing products. Opt. Express 31, 8226–8239. doi:10.1364/oe.476884
Gordienko, V., Stephens, M. F. C., El-Taher, A. E., and Doran, N. J. (2017). Ultra-flat wideband single-pump Raman-enhanced parametric amplification. Opt. Express 25, 4810–4818. doi:10.1364/oe.25.004810
Hansryd, J., Andrekson, P. A., Westlund, M., Li, J., and Hedekvist, P. O. (2002). Fiber-based optical parametric amplifiers and their applications. IEEE J. Sel. Top. Quantum Electron. 8, 506–520. doi:10.1109/JSTQE.2002.1016354
Karlsson, M., Schröder, J., Zhao, P., and Andrekson, P. A. (2021). “Analytic theory for parametric gain in lossy integrated waveguides,” in Conference on lasers and electro-optics (D.C., United States: Optical Society of America). JTh3A.5.
Li, J. H., Chiang, K. S., and Chow, K. W. (2011). Modulation instabilities in two-core optical fibers. J. Opt. Soc. Am. B 28, 1693–1701. doi:10.1364/josab.28.001693
Li, J. H., Chiang, K. S., Malomed, B. A., and Chow, K. W. (2012). Modulation instabilities in birefringent two-core optical fibres. J. Phys. B Atomic, Mol. Opt. Phys. 45, 165404. doi:10.1088/0953-4075/45/16/165404
Li, J. H., Sun, T. T., Ma, Y. Q., Chen, Y., Cao, Z. L., Xian, F. L., et al. (2020a). Modulation instabilities in nonlinear two-core optical fibers with fourth order dispersion. Optik 208, 164134. doi:10.1016/j.ijleo.2019.164134
Li, J. H., Sun, T. T., Ma, Y. Q., Chen, Y. Y., Cao, Z. L., and Xian, F. L. (2020b). The effects of fourth-order dispersion on modulation instabilities in two-core optical fibers with asymmetric CW state. Phys. Scr. 95, 115502. doi:10.1088/1402-4896/abba4c
Mahric, M. E. (2007). Fiber optical parametric amplifiers, oscillators and related devices: Theory, applications, and related devices. Cambridge, United Kingdom: Cambridge University Press.
Mecozzi, A. (1988). Parametric amplification and squeezed-light generation in a nonlinear directional coupler. Opt. Lett. 13, 925–927. doi:10.1364/ol.13.000925
Nair, A. A., Porsezian, K., and Jayaraju, M. (2018). Impact of higher order dispersion and nonlinearities on modulational instability in a dual-core optical fiber. Eur. Phys. J. D. 72, 6. doi:10.1140/epjd/e2017-80437-6
Nithyanandan, K., Raja, R. V. J., and Porsezian, K. (2013). Modulational instability in a twin-core fiber with the effect of saturable nonlinear response and coupling coefficient dispersion. Phys. Rev. A 87, 043805. doi:10.1103/physreva.87.043805
Olsson, S. L. I., Eliasson, H., Astra, E., Karlsson, M., and Andrekson, P. A. (2018). Long-haul optical transmission link using low-noise phase-sensitive amplifiers. Nat. Commun. 9, 2513. doi:10.1038/s41467-018-04956-5
Ribeiro, V., Karlsson, M., and Andrekson, P. (2017). Parametric amplification with a dual-core fiber. Opt. Express 25, 6234–6243. doi:10.1364/oe.25.006234
Ribeiro, V., Lorences-Riesgo, A., Andrekson, P., and Karlsson, M. (2018). Noise in phase-(in)sensitive dual-core fiber parametric amplification. Opt. Express 26, 4050–4059. doi:10.1364/oe.26.004050
Ribeiro, V., and Perego, A. M. (2022a). Parametric amplification in lossy nonlinear waveguides with spatially dependent coupling. Opt. Express 30, 17614–17624. doi:10.1364/oe.457583
Ribeiro, V., and Perego, A. M. (2022b). “Theory of parametric amplification in coupled lossy waveguides,” in Conference on lasers and electro-optics (D.C., United States: Optical Society of America).
Ribeiro, V., Szabó, A. D., Rocha, A. M., Gaur, C. B., Ali, A. A. I., Quiquempois, Y., et al. (2022). Parametric amplification and wavelength conversion in dual-core highly nonlinear fibers. J. Light. Technol. 1, 6013–6020. doi:10.1109/JLT.2022.3186809
Shi, M., Ribeiro, V., and Perego, A. M. (2023a). On the resilience of dual-waveguide parametric amplifiers to pump power and phase fluctuations. Appl. Phys. Lett. 122, 101102. doi:10.1063/5.0136729
Shi, M., Ribeiro, V., and Perego, A. M. (2023b). Parametric amplification based on intermodal four-wave mixing between different supermodes in coupled-core fibers. Opt. Express 31, 9760. doi:10.1364/oe.480480
Stolen, R., and Bjorkholm, J. (1982). Parametric amplification and frequency conversion in optical fibers. IEEE J. Quantum Electron. 18, 1062–1072. doi:10.1109/JQE.1982.1071660
Su, J., and Biaggio, I. (2022). Dual-core optical fibers for efficient mid-infrared generation via third-order frequency mixing and coupling-length phase matching. J. Opt. Soc. Am. B 39, 729–741. doi:10.1364/josab.449934
Szabo, A. D., Ribeiro, V., Gaur, C. B., Ali, A. A. I., Mussot, A., Quiquempois, Y., et al. (2021). Dual-polarization c+l-band wavelength conversion in a twin-core highly nonlinear fibre. Proceedings of the OFC 2021 Virtual conference, 06-10 June 2021, San Francisco, CA, USA, Paper MB5.4
Takasaka, S., Mimura, Y., Takahashi, M., Sugizaki, R., and Ogoshi, H. (2012). “Flat and broad amplification by quasi-phase-matched fiber optical parametric amplifier,” in Optical fiber communication conference (D.C., United States: Optical Society of America). OTh1C.4.
Trillo, S., Wabnitz, S., Stegeman, G. I., and Wright, E. M. (1989). Parametric amplification and modulational instabilities in dispersive nonlinear directional couplers with relaxing nonlinearity. J. Opt. Soc. Am. B 6, 889–900. doi:10.1364/josab.6.000889
Tsoy, E. N., de Sterke, C. M., and Abdullaev, F. K. (2001). Influence of two-photon absorption on modulational instability. J. Opt. Soc. Am. B 18, 1144–1149. doi:10.1364/JOSAB.18.001144
Ye, Z., Zhao, P., Twayana, K., Karlsson, M., Andrekson, P. A., and Torres-Company, V. (2021a). “Ultralow-loss meter-long dispersion-engineered silicon nitride waveguides,” in Conference on lasers and electro-optics (D.C., United States: Optical Society of America). SF1C.5. doi:10.1364/CLEO_SI.2021.SF1C.5
Ye, Z., Zhao, P., Twayana, K., Karlsson, M., Torres-Company, V., and Andrekson, P. A. (2021b). Overcoming the quantum limit of optical amplification in monolithic waveguides. Sci. Adv. 7, eabi8150. eabi8150. doi:10.1126/sciadv.abi8150
Zhao, P., Karlsson, M., and Andrekson, P. A. (2022). Low-noise integrated phase-sensitive waveguide parametric amplifiers. J. Light. Technol. 40, 128–135. doi:10.1109/JLT.2021.3119423
Keywords: parametric amplification, coupled waveguides, coupling dispersion, nonlinear couplers, four wave mixing effects
Citation: Shi M, Ribeiro V and Perego AM (2023) Parametric amplification in coupled nonlinear waveguides: The role of coupling dispersion. Front. Photonics 4:1051294. doi: 10.3389/fphot.2023.1051294
Received: 22 September 2022; Accepted: 21 March 2023;
Published: 05 April 2023.
Edited by:
Alejandro Aceves, Southern Methodist University, United StatesReviewed by:
Nikos Lazarides, Khalifa University, United Arab EmiratesCopyright © 2023 Shi, Ribeiro and Perego. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Auro M. Perego, YS5wZXJlZ28xQGFzdG9uLmFjLnVr
†Present address: Vitor Ribeiro, KETS Quantum Security Ltd., Bristol, United Kingdom
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.