
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Photonics, 29 November 2022
Sec. Quantum Optics
Volume 3 - 2022 | https://doi.org/10.3389/fphot.2022.932944
This article is part of the Research TopicGlobal Excellence in Photonics: EuropeView all 4 articles
Spin systems in solid state materials are promising qubit candidates for quantum information in particular as quantum memories or for quantum sensing. A major prerequisite here is the coherence of spin phase oscillations. In this work, we show a control sequence which, by applying RF pulses of variable detuning, allows to increase the visibility of spin phase oscillations. We experimentally demonstrate the scheme on single NV centers in diamond and analytically describe how the NV electron spin phase oscillations behave in the presence of classical noise models. We hereby introduce detuning as the enabling factor that modulates the filter function of the sequence, in order to achieve a visibility of the Ramsey fringes comparable to or longer than the Hahn-echo T2 time and an improved sensitivity to DC magnetic fields in various experimental settings.
Solid-state qubits are of central importance within the quantum technologies due to their outstanding performance in key fields such as quantum information processing (Stajic, 2013) and quantum magnetic field sensing (Jones et al., 2009; Bal et al., 2012). Several physical systems have been exploited to experimentally realize solid-state qubits such as quantum dots (Hanson and Awschalom, 2008; Shulman et al., 2012; Veldhorst et al., 2017; Strauß et al., 2019; Carmele and Reitzenstein, 2019), superconductive qubits (Wendin, 2017; Dicarlo et al., 2009), nuclear spins in materials (Pla et al., 2013) and electronic spins in molecules or defect-centers in crystals (Smeltzer et al., 2009; Pla et al., 2012; D. et al., 2007; Schlegel et al., 2008). Among the latter, the nitrogen-vacancy (NV) color center in diamond has been extensively investigated due to its exceptional stability and properties observed even at room temperature and in ambient conditions (Kurtsiefer et al., 2000). The NV center has an electron spin triplet that can be optically initialized, readout via fluorescence intensity measurement and controlled with appropriate radiofrequency (RF) pulse trains (Jelezko and Wrachtrup et al., 2006; Kennedy et al., 2003; Ryan et al., 2010). This has allowed the demonstration of quantum information storage (Dutt et al., 2007; Maurer et al., 2012) and of magnetic field measurements with high sensitivity and spatial resolution (Degen et al., 2017; Sushkov et al., 2014; Mamin et al., 2012; Loretz et al., 2014). Furthermore, electron spin relaxometry has also been used to probe the behavior of single magnetic domain particles (Gould et al., 2014; Sadzak et al., 2018) and small ensembles of molecules (Ermakova et al., 2013) on the nanoscale. In order to perform sensing in complex physical environments, dynamical decoupling (DD) schemes have been implemented to filter-out the background magnetic noise from the specific target signals. These schemes rely on sequences of precisely timed RF pulses that act as frequency filters and bandwidth selectors. Some basic DD measurements are Ramsey (1950) and Hahn (1950) schemes, that have been followed by a manifold of other techniques often derived from the nuclear magnetic resonance (NMR) field (Degen et al., 2017), such as CPMG (Naydenov et al., 2011), XY-n (de Lange et al., 2010), UDD (Uhrig, 2007; Wang et al., 2012). Most of the currently available decoupling schemes are focused on prolonging the T2 coherence time and are primarly applied to AC magnetometry. Concerning DC magnetometry, the current approaches are based on using isotopically pure diamonds (Ishikawa et al., 2012), diamond material engineering (Barry et al., 2020) or elaborated schemes that circumvent the problem by using spin bath driving, ancillary spins or diamond mechanical rotation (Wood et al., 2018; Liu et al., 2019; Barry et al., 2020). As these solutions rely on specific experimental configurations, a robust dynamical-decoupling alternative would be particularly interesting for a manifold of applications. Motivated by recent experiments on trapped atoms (Vitanov et al., 2015), we propose here an extension of the Hahn-Ramsey dynamical decoupling scheme, where the detuning of the RF spin control pulses is used to obtain an increased visibility of the electron spin phase oscillations. We demonstrate the protocol on single NV centers in bulk diamond, and show a pronounced increase in the spin oscillations coherence time. Furthermore, we give an analytical description of the filter function and of the sequence and provide an estimation of the scheme sensitivity for DC magnetometry, thereby proving that it can be of great importance in a broad range of applications such as quantum sensing, quantum information processing (Nielsen and Chuang, 2000) and synchronization (Hodges et al., 2013).
We make use of two experimental procedures in order to observe the Hahn-Ramsey properties and demonstrate its effects on NV centers under different conditions and parameters. In the first, we select a stable defect center with long electron spin
where σz is the Pauli spin matrix, U (0, τ) is the free evolution operator,
Where
Here, the pulse length is assumed to be ideal (that is, τp is tuned so that ω1τp = π/2 or ω1τp = π). The central, inversely detuned π pulse, separates the free precession time in two parts, with the first being described by the operator UΔ(0, τ) and the second by U−Δ(τ, 2τ). Given the form of Eq. 2, this implies that the slow contribution of f(t) is canceled out, leaving nevertheless an oscillating spin phase dependent on Δ only. While quantum interactions with other single spins are typically represented via specific interaction operators, such as hyperfine interaction, spin-spin coupling and so on, bath interactions or fluctuating environment fields of classical nature are included in the term f(t) and treated as a stochastic process. For solid-state spin centers, it is appropriate to treat f(t) as a classical magnetic noise described by a compound Poisson process, where the jump times have an exponentially decaying probability density function with a correlation time of 1/λ and the jump intensities have a Gaussian distribution with zero average and Γ variance. By including this in Eq. 3, we obtain an expression that links the fringes decoherence process to detuning and Rabi frequencies, and also to the noise parameters here introduced (see Supplementary Material). After switching to the frequency domain, the expected signal can be expressed as an exponentially decaying function
With a = cos(θ) and b = sin(θ). These three components are weighted in the final signal by detuning-related coefficients (see Figure 1). The term with the spin phase oscillating as
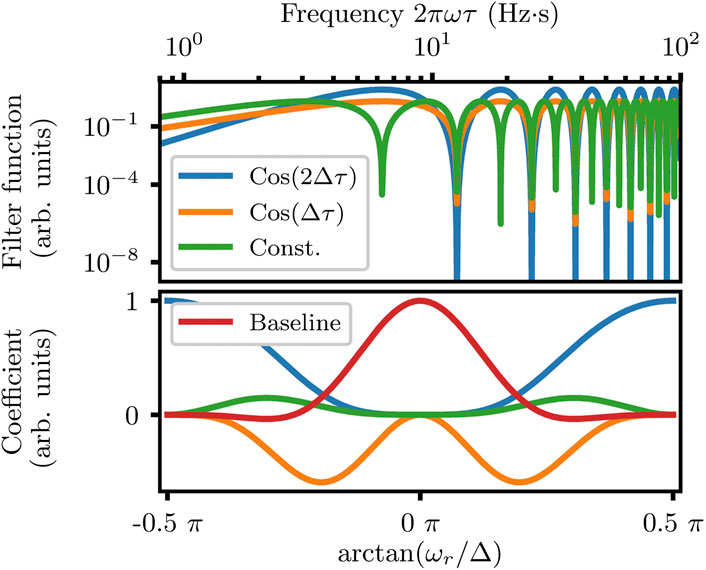
FIGURE 1. The top figure shows the filter functions contributing to the Hahn-Ramsey signal; the colors represent respectively the non-oscillating part (green), the component oscillating in
For our experiment, we use a type [111] CVD-grown delta-doped diamond plate with a 15NV center rich layer. The diamond is placed on a microwave waveguide and the RF pulses are delivered via a 50 µm thick copper wire closely located to the surface. From the bottom side, the diamond is accessible via a high numerical aperture (NA = 1.4) oil immersion objective Olympus UPLANSAPO ×60, that is used to optically initialize the nitrogen-vacancies with a 532 nm diode laser source pulsed by an acousto-optic modulator. The same objective collects the fluorescence light that is sent to a confocal setup and a beamsplitter, and finally collimated on two Perkin-Elmer single photon detectors. The experimental setup and basic ODMR measurements are shown in Figure 2. In order to perform the first demonstration, we carry out several acquisitions of Ramsey and Hahn-Ramsey signals on different NV centers in the diamond plate. After identifying a single NV center via autocorrelation measurements, we apply a ≈ 500 G static magnetic field parallel to the defects quantization axis to achieve a nuclear spin hyperpolarization (Jacques et al., 2009) of 80%–90% (see Figure 2), that allows us to work with an approximate two-level spin-1/2 system. We then proceed to record the Ramsey and Hahn-Ramsey fringes for a specific radiofrequency detuning, as shown in Figure 3. By testing the sequence directly on the NV centers in the natural diamond environment, we would expect this to be effective in prolonging spin phase fringes only if the surrounding is characterized by a non-negligible sub-MHz noise dynamics that affects the spin coherence. The measurements results confirm this picture and show that the Hahn-Ramsey is effective in increasing the visibility of electron spin phase oscillations and the T2,HR time. This is depicted in Figure 3, where an exponential fit shows a change in the oscillation decay timescale from
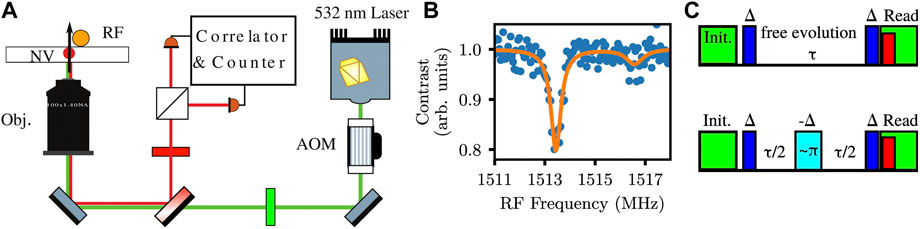
FIGURE 2. (A) Schematic representation of the experimental setup; a [111] diamond plate is illuminated by an AOM-pulsed 532 nm green laser. The emission from the NV centers is collected via an oil immersion objective (NA = 1.40) and sent to a confocal filter and a beamsplitter, terminating on two avalanche photodiodes used for autocorrelation and photon counting measurements. The spins are manipulated by radiofrequency pulses delivered on the diamond by an impedance matched stripline. By applying a static field of ≈ 500 G along the NV centers axis, the 15N nitrogen nuclear spin of the NV center is hyperpolarized in one of its two states with a polarization ratio
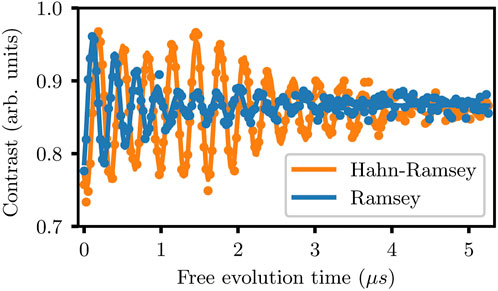
FIGURE 3. Ramsey (blue) and Hahn-Ramsey (orange) measurements on a single NV center in diamond, with the solid lines showing the fit functions. The beatings are due to a second spin weakly interacting with the NV electron spin. The Hahn-Ramsey fit is performed via Eqs 3, 4 as given in the Supplementary Material, provided a nominal detuning of Δ = 4 MHz and θ ≈ 1.10 (corresponding to ΩRabi = 6 MHz) that follows the condition Δ < ΩRabi. An exponentially decaying fit of the form
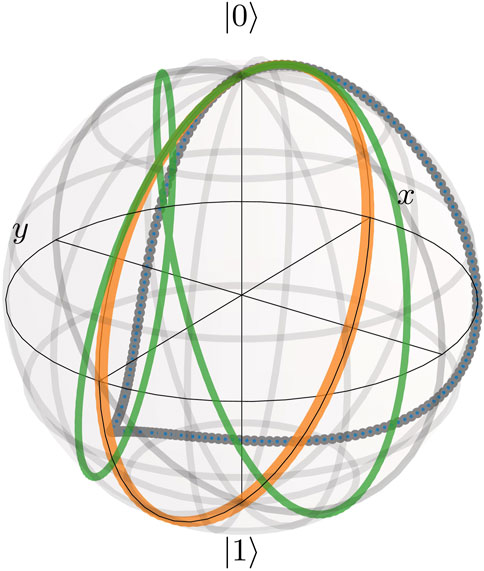
FIGURE 4. Simulations of different trajectories for a single Hahn-Ramsey fringe on the Bloch sphere, given three possible θ = ω1/Δ values. The green line depicts the case of θ ≈ π/2, the orange line θ ≈ 0.4 ⋅ π while the blue dotted line shows the case where θ = 0.2π. By shifting Δ/ω1 away from the ≪ 1 limit, the spin rotation takes place around an off-equator axis, where phase oscillations show a more complex pattern and are less sensitive to noise.
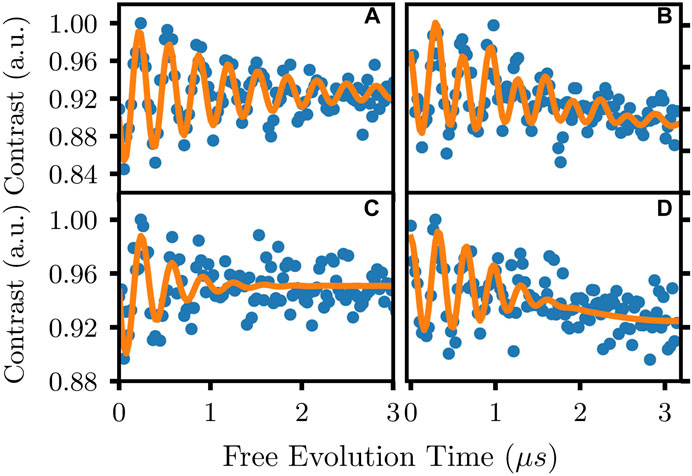
FIGURE 5. The outcomes of Ramsey (A) and Hahn-Ramsey (B) measurements on a single NV center in the diamond compared with (C,D), where the Ramsey and Hahn-Ramsey signals are acquired after adding artificial magnetic noise with Poissonian statistics and correlation time of 0.5 µs, corresponding to a −3 dB bandwidth of 2 MHz, and a strength of Γ ≈ 200 kHz. The complex pattern displayed by the Hahn-Ramsey measurements is well fitted by the model, which shows as well the more pronounced decay for the Ramsey signal after applying the additional noise.
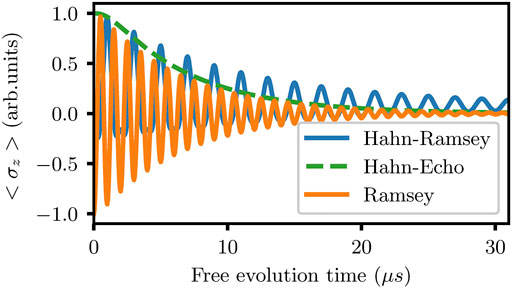
FIGURE 6. Simulation showing the Ramsey, Hahn-Ramsey and Hahn-echo measurements given the parameters of λ = 2.5 1/t, Γ = 2π ⋅ 0.1 1/t and θ = 0.2π. For a detuning comparable to the Rabi frequency, the
Compared to the classic Ramsey interferometry, the Hahn-Ramsey sensitivity scales with the respective T2,HR time and offers an improved performance for physical situations where low-intensity noises are an important contribution to the sensitivity deterioration, and as well in situations where low-power RF are available for the spin manipulation pulses, with improvement factors of up to
We have described and experimentally demonstrated a Hahn-Ramsey type of dynamical decoupling sequence on NV centers in diamond. By opportunely inverting the detunings in the RF control sequence, we are able to show that the HR scheme is effective in providing a better suppression of low-frequency noise with respect to the Ramsey scheme, approaching the Hahn-echo limit when the detuning is smaller than the Rabi frequency. This can be exploited to effectively improve the spin phase oscillation visibility. When the detuning magnitude is instead comparable to the Rabi frequency, the Hahn-Ramsey can provide even longer Ramsey fringes decay times. This in turn may be used for DC magnetometry, providing a better sensitivity than the standard Ramsey interferometry.
The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.
NS conceived the experiment and performed measurements and simulations. AC developed the theoretical analysis. All authors made substantial contributions to the work and to the manuscript preparation.
AK and AC gratefully acknowledge support from the Deutsche Forschungsgemeinschaft (DFG) through the project B2 of the SFB 910 and from the European Union’s Horizon 2020 research and innovation program under the SONAR Grant Agreement No. 734690. NS gratefully acknowledges funding from the Deutsche Forschungsgemeinschaft (DFG) through the SFB 951.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphot.2022.932944/full#supplementary-material
Bal, M., Deng, C., Orgiazzi, J.-L., Ong, F., and Lupascu, A. (2012). Ultrasensitive magnetic field detection using a single artificial atom. Nat. Commun. 3, 1324. doi:10.1038/ncomms2332
Barry, J. F., Schloss, J. M., Bauch, E., Turner, M. J., Hart, C. A., Pham, L. M., et al. (2020). Sensitivity optimization for nv-diamond magnetometry. Rev. Mod. Phys. 92, 015004. doi:10.1103/RevModPhys.92.015004
Biercuk, M. J., Doherty, A. C., and Uys, H. (2011). Dynamical decoupling sequence construction as a filter-design problem. J. Phys. B At. Mol. Opt. Phys. 44, 154002. doi:10.1088/0953-4075/44/15/154002
Carmele, A., and Reitzenstein, S. (2019). Non-markovian features in semiconductor quantum optics: Quantifying the role of phonons in experiment and theory. Nanophotonics 8, 655–683. doi:10.1515/nanoph-2018-0222
de Lange, G., Wang, Z. H., Ristè, D., Dobrovitski, V. V., and Hanson, R. (2010). Universal dynamical decoupling of a single solid-state spin from a spin bath. Science 330, 60–63. doi:10.1126/science.1192739
Degen, C. L., Reinhard, F., and Cappellaro, P. (2017). Quantum sensing. Rev. Mod. Phys. 89, 035002. doi:10.1103/RevModPhys.89.035002
Dicarlo, L., Chow, J. M., Gambetta, J. M., Bishop, L. S., Johnson, B. R., Schuster, D. I., et al. (2009). Demonstration of two-qubit algorithms with a superconducting quantum processor. Nature 460, 240–244. doi:10.1038/nature08121
Dutt, M. V. G., Childress, L., Jiang, L., Togan, E., Maze, J., Jelezko, F., et al. (2007). Quantum register based on individual electronic and nuclear spin qubits in diamond. Science 316, 1312–1316. doi:10.1126/science.1139831
Ermakova, A., Pramanik, G., Cai, J. M., Algara-Siller, G., Kaiser, U., Weil, T., et al. (2013). Detection of a few metallo-protein molecules using color centers in nanodiamonds. Nano Lett. 13, 3305–3309. doi:10.1021/nl4015233
Gould, M., Barbour, R. J., Thomas, N., Arami, H., Krishnan, K. M., and Fu, K. M. C. (2014). Room-temperature detection of a single 19nm super-paramagnetic nanoparticle with an imaging magnetometer. Appl. Phys. Lett. 105, 072406. doi:10.1063/1.4893602
Hanson, R., and Awschalom, D. D. (2008). Coherent manipulation of single spins in semiconductors. Nature 453, 1043–1049. doi:10.1038/nature07129
Hodges, J. S., Yao, N. Y., Maclaurin, D., Rastogi, C., Lukin, M. D., and Englund, D. (2013). Timekeeping with electron spin states in diamond. Phys. Rev. A . Coll. Park. 86, 032118. doi:10.1103/PhysRevA.87.032118
Ishikawa, T., Fu, K. M. C., Santori, C., Acosta, V. M., Beausoleil, R. G., Watanabe, H., et al. (2012). Optical and spin coherence properties of nitrogen-vacancy centers placed in a 100 nm thick isotopically purified diamond layer. Nano Lett. 12, 2083–2087. doi:10.1021/nl300350r
Jacques, V., Neumann, P., Beck, J., Markham, M., Twitchen, D., Meijer, J., et al. (2009). Dynamic polarization of single nuclear spins by optical pumping of nitrogen-vacancy color centers in diamond at room temperature. Phys. Rev. Lett. 102, 057403. doi:10.1103/PhysRevLett.102.057403
Jelezko, F., and Wrachtrup, J. (2006). Single defect centres in diamond: A review. Phys. Stat. Sol. 203, 3207–3225. doi:10.1002/pssa.200671403
Jones, J. A., Karlen, S. D., Fitzsimons, J., Ardavan, A., Benjamin, S. C., Briggs, G. A. D., et al. (2009). Magnetic field sensing beyond the standard quantum limit using 10-spin noon states. Science 324, 1166–1168. doi:10.1126/science.1170730
Kennedy, T. A., Colton, J. S., Butler, J. E., Linares, R. C., and Doering, P. J. (2003). Long coherence times at 300 k for nitrogen-vacancy center spins in diamond grown by chemical vapor deposition. Appl. Phys. Lett. 83, 4190–4192. doi:10.1063/1.1626791
Kurtsiefer, C., Mayer, S., Zarda, P., and Weinfurter, H. (2000). Stable solid-state source of single photons. Phys. Rev. Lett. 85, 290–293. doi:10.1103/PhysRevLett.85.290
Levitt, M. H. (2000). Spin dynamics : Basics of nuclear magnetic resonance. New York, United States: John Wiley & Sons.
Liu, Y. X., Ajoy, A., and Cappellaro, P. (2019). Nanoscale vector dc magnetometry via ancilla-assisted frequency up-conversion. Phys. Rev. Lett. 122, 100501. doi:10.1103/PhysRevLett.122.100501
Loretz, M., Pezzagna, S., Meijer, J., and Degen, C. L. (2014). Nanoscale nuclear magnetic resonance with a 1.9-nm-deep nitrogen-vacancy sensor. Appl. Phys. Lett. 104, 033102. doi:10.1063/1.4862749
Mamin, H. J., Sherwood, M. H., and Rugar, D. (2012). Detecting external electron spins using nitrogen-vacancy centers. Phys. Rev. B 86, 195422. doi:10.1103/PhysRevB.86.195422
Maurer, P. C., Kucsko, G., Latta, C., Jiang, L., Yao, N. Y., Bennett, S. D., et al. (2012). Room-temperature quantum bit memory exceeding one second. Science 336, 1283–1286. doi:10.1126/science.1220513
Naydenov, B., Dolde, F., Hall, L. T., Shin, C., Fedder, H., Hollenberg, L., et al. (2011). Dynamical decoupling of a single-electron spin at room temperature. Phys. Rev. B 83, 081201. doi:10.1103/PhysRevB.83.081201
Nielsen, M. A., and Chuang, I. L. (2000). Quantum computation and quantum information. Cambridge: Cambridge University Press.
Pla, J. J., Tan, K. Y., Dehollain, J. P., Lim, W. H., Morton, J. J., Jamieson, D. N., et al. (2012). A single-atom electron spin qubit in silicon. Nature 489, 541–545. doi:10.1038/nature11449
Pla, J. J., Tan, K. Y., Dehollain, J. P., Lim, W. H., Morton, J. J., Zwanenburg, F. A., et al. (2013). High-fidelity readout and control of a nuclear spin qubit in silicon. Nature 496, 334–338. doi:10.1038/nature12011
Ramsey, N. F. (1950). A molecular beam resonance method with separated oscillating fields. Phys. Rev. 78, 695–699. doi:10.1103/PhysRev.78.695
Ryan, C. A., Hodges, J. S., and Cory, D. G. (2010). Robust decoupling techniques to extend quantum coherence in diamond. Phys. Rev. Lett. 105, 200402. doi:10.1103/PhysRevLett.105.200402
Sadzak, N., Héritier, M., and Benson, O. (2018). Coupling a single nitrogen-vacancy center in nanodiamond to superparamagnetic nanoparticles. Sci. Rep. 8, 8430. doi:10.1038/s41598-018-26633-9
Schlegel, C., Van Slageren, J., Manoli, M., Brechin, E. K., and Dressel, M. (2008). Direct observation of quantum coherence in single-molecule magnets. Phys. Rev. Lett. 101, 147203. doi:10.1103/PhysRevLett.101.147203
Shulman, M. D., Dial, O. E., Harvey, S. P., Bluhm, H., Umansky, V., and Yacoby, A. (2012). Demonstration of entanglement of electrostatically coupled singlet-triplet qubits. Science 336, 202–205. doi:10.1126/science.1217692
Smeltzer, B., McIntyre, J., and Childress, L. (2009). Robust control of individual nuclear spins in diamond. Phys. Rev. A . Coll. Park. 80, 050302. doi:10.1103/PhysRevA.80.050302
Stajic, J. (2013). The future of quantum information processing. Science 339, 1163. doi:10.1126/science.339.6124.1163
Strauß, M., Carmele, A., Schleibner, J., Hohn, M., Schneider, C., Höfling, S., et al. (2019). Wigner time delay induced by a single quantum dot. Phys. Rev. Lett. 122, 107401. doi:10.1103/PhysRevLett.122.107401
Sushkov, A. O., Chisholm, N., Lovchinsky, I., Kubo, M., Lo, P. K., Bennett, S. D., et al. (2014). All-optical sensing of a single-molecule electron spin. Nano Lett. 14, 6443–6448. doi:10.1021/nl502988n
Uhrig, G. S. (2007). Keeping a quantum bit alive by optimized π-pulse sequences. Phys. Rev. Lett. 98, 100504. doi:10.1103/PhysRevLett.98.100504
Veldhorst, M., Eenink, H. G., Yang, C. H., and Dzurak, A. S. (2017). Silicon cmos architecture for a spin-based quantum computer. Nat. Commun. 8, 1766. doi:10.1038/s41467-017-01905-6
Vitanov, N. V., Gloger, T. F., Kaufmann, P., Kaufmann, D., Collath, T., Tanveer Baig, M., et al. (2015). Fault-tolerant hahn-ramsey interferometry with pulse sequences of alternating detuning. Phys. Rev. A . Coll. Park. 91, 033406. doi:10.1103/PhysRevA.91.033406
Wang, Z. H., De Lange, G., Ristè, D., Hanson, R., and Dobrovitski, V. V. (2012). Comparison of dynamical decoupling protocols for a nitrogen-vacancy center in diamond. Phys. Rev. B 85, 155204. doi:10.1103/PhysRevB.85.155204
Wendin, G. (2017). Quantum information processing with superconducting circuits: A review. Rep. Prog. Phys. 80, 106001. doi:10.1088/1361-6633/aa7e1a
Keywords: solid-state qubits, NV, nitrogen-vacancy, quantum sensing, magnetometry, quantum synchronization, dynamical decoupling, noise suppression
Citation: Sadzak N, Carmele A, Widmann C, Nebel C, Knorr A and Benson O (2022) A Hahn-Ramsey scheme for dynamical decoupling of single solid-state qubits. Front. Photonics 3:932944. doi: 10.3389/fphot.2022.932944
Received: 30 April 2022; Accepted: 04 November 2022;
Published: 29 November 2022.
Edited by:
Eilon Poem, Weizmann Institute of Science, IsraelReviewed by:
Kasturi Saha, Indian Institute of Technology Bombay, IndiaCopyright © 2022 Sadzak, Carmele, Widmann, Nebel, Knorr and Benson. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Nikola Sadzak, c2FkemFrQHBoeXNpay5odS1iZXJsaW4uZGU=
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.