
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Nucl. Eng., 14 April 2023
Sec. Radioactive Waste Management
Volume 2 - 2023 | https://doi.org/10.3389/fnuen.2023.1158109
This article is part of the Research TopicSolubility Phenomena in The Context of Nuclear Waste DisposalView all 10 articles
A correction has been applied to this article in:
Corrigendum: The solubility of oxygen in water and saline solutions
Oxygen is one of the key reaction partners for many redox reactions also in the context of nuclear waste disposal. Its solubility influences radionuclides’ behavior, corrosion processes and even microbial activity. Therefore, a reliable calculation of the solubility of molecular oxygen in aqueous solutions is relevant for any safety assessment. Available geochemical speciation and reactive transport programs handle these data very differently. In some codes, the hypothetical equilibrium between dissolved oxygen and water is used to balance redox reactions. Equilibrium constants are given in “temperature grids” for up to 573.15 K. In other cases, temperature functions for the solubility of gaseous oxygen in water are given, without any reference to a valid temperature range. These settings become even more complicated when used in the context of modeling equilibria in high-saline solutions applying the Pitzer formalism. This raised the question about the experimental foundation of equilibrium constants given in such data files and their validity for the solubility of molecular oxygen in saline solutions. For this article, a thorough literature review was conducted with respect to the solubility of molecular oxygen in pure water and saline solutions. From these primary experimental O2 solubility data a temperature-dependent Henry’s law function as well as temperature-dependent binary and ternary Pitzer ion-interaction coefficients were derived. An internally consistent set of thermodynamic data for dissolved oxygen is presented, along with statements about its validity in terms of temperature and, as far as Pitzer interaction coefficients are concerned, of solution composition. This self-consistent activity-fugacity model containing thermodynamic data, Henry’s law temperature equation, and Pitzer interaction coefficients is capable of providing a more accurate description of redox transformations, allowing a reduction of conservatism in safety assessment calculations, not only in the context of a nuclear repository. The model reproduces well the reliable experimental data available, and is capable to predict the oxygen solubility in complex solution media. The temperature functions used to describe Henry’s constant and the Pitzer interaction coefficients are consistent with the implementation in commonly used geochemical computational programs, allowing direct use without further modification.
Redox reactions are important for the description of geochemical reactions in general (e.g., pyrite oxidation) and of solubility limitations of radioactive contaminants in particular. In a final deep geological repository (DGR) for radioactive waste, attainment of anoxic conditions is very likely in the post-closure phase of operation. In the event of any post-closure access of an aqueous solution to the waste, and assuming anoxic conditions, many radionuclides are immobile to a large extent through the formation of poorly soluble (hydro-)oxides in their reduced tetravalent form [e.g., TcO2(am), U(OH)4(am), Np(OH)4(am), PuO2(am/cr), etc.] (Grenthe et al., 2020). However, under strongly reducing conditions even their trivalent forms e.g., Pu(OH)3(am/cr) might exist, showing a higher solubility and thus mobility than the tetravalent actinides (Grenthe et al., 2020). This holds also for higher redox states (penta-, hexa- or heptavalent), which are more soluble in water. Concerning all of these redox-triggered transformations, exact knowledge of the redox potential (EH/pe) is essential, which in turn depends on the correct description of dissolved oxygen. For an assessment of the maximum likely mobilization of radionuclides from the near field of a DGR under the prerequisite of intrusion of water, the mass of available dissolved oxygen (being present, e.g., by diffusional transport or freshwater intrusion) is one key factor for the retardation of radionuclides in aqueous solution.
In geochemical speciation codes such as PHREEQC (Parkhurst and Appelo, 2013), Geochemist’s Workbench (Bethke, 2022), or EQ3/6 (Wolery, 1992), redox reactions of any kind are linked for computational reasons to the hypothetical half-cell reaction
Or, alternatively, to another form involving dissolved oxygen:
Adding the reaction
for which, by definition,
Reactions (Eq. 4) and (Eq. 5) are sometimes referred to as “logK-EH-reaction.” In data files for the above-mentioned codes, equilibrium constants for these reactions are either given as functions of temperature or as “temperature grids,” where pre-calculated values at defined temperatures (usually 0, 25, 60, 100, 150, 200, 250, and 300°C) are displayed. Unfortunately, this is usually done without any references to literature or a hint to the validity limits at all. The situation becomes even more obscure if the reaction (Eq. 5) containing O2(aq) is used as half-cell reaction, because this reaction must in fact be considered as addition of reaction (Eq. 4) and
This poses the question about the experimental foundations for the calculation of the solubility of molecular oxygen in water at temperatures up to 573.15 K. If applied in calculations for high-saline solutions the question arises concerning the impact of ionic strength in general or the presence of specific electrolytes on the solubility of molecular oxygen in particular. In such cases, the Pitzer ion-interaction approach provides a tool for calculating the activity coefficient in concentrated salt solutions (Pitzer, 1991). For this purpose, coefficients for a virial equation are used for binary and ternary interactions between ions as well as uncharged species in aqueous solution, reflecting the deviation from the behavior in pure water. In some data files, e.g., the “Yucca Mountain Pitzer file” (Mariner, 2004) delivered with the EQ3/6 code, Pitzer coefficients for O2(aq) are included, some valid at 298.15 K only, others are given with a temperature function of unknown validity range. Compilations of data from different sources may cause inconsistencies, which, especially when using the interaction coefficients, may have a significant negative impact on the results of the models obtained.
Facing the task of extending a thermodynamic database based on traceable experimental data, simple adoption of precalculated equilibrium constants in temperature grids from parameter files in geochemical codes is not appropriate, particularly since they are provided in many databases without reference to the source of the primary data. Consequently, these data had to be comprehensibly and consistently recalculated from experimental data for the solubility of oxygen at various temperatures in both pure water and salt solutions and from standard formation data.
For this purpose, solubility data of O2 in pure water as well as in a wide variety of electrolyte solutions were critically evaluated and combined with well-established thermodynamic data for liquid water H2O(l), H2(g), and O2(g) to create an internally consistent set of equilibrium constants for reactions (Eqs 4–6). With respect to O2(g) solubility data, the focus was on the system of oceanic salts (including carbonates), which are relevant as potential host rocks implying solutions of high ionic strengths. The alkali ((di-)hydrogen) phosphates were included for inorganic phosphate ions that can form in nuclear waste repositories when phosphate glasses or lanthanide phosphate monazites (LnPO4), as waste forms of highly active waste streams, undergo dissolution processes in contact with water. The acids of the anions contained in the system were included in the data set to represent the case of acid-forming oxidation of minerals (acid mine drainage). Hydroxides were included to allow calculation for alkaline solutions from cement pore waters.
Henry’s law constant (KO2(aq)) using the partial pressure of O2 in the gas phase describes the equilibrium condition for dissolution reaction of O2 in water (Eq. 6) with γO2(aq) being the O2 activity coefficient, mO2(aq) the molal O2 concentration in solution and fO2(aq) the O2 fugacity in the gas phase.
Geochemical codes treat the temperature dependence of equilibrium constants as polynomial functions with several temperature-dependent terms (Eq. 8) or mathematically equivalent transformations thereof.
The Pitzer formalism is widely used to account for the deviation from ideality of aqueous solutions of high ionic strength. It has proven capable of calculating solubilities in saturated solutions in the system of oceanic salts: Na+, K+, Mg2+, Ca2+ – Cl−, SO42−, HCO3− – CO2(g) – H2O(l). The probably most cited paper in this respect is the one by Harvie, Møller and Weare (Harvie et al., 1984), whose results are often referred to as “HMW-database.” The usability of their database was demonstrated for a huge amount of application cases. One example especially relevant for nuclear waste disposal is by Herbert, who successfully modeled dissolution and precipitation processes in complex, saturated salt solutions in German rock salt formations (Herbert et al., 2000). Later, the HMW-database was extended to higher temperatures (Christov and Møller, 2004). Its popularity, as well as that of the Pitzer formalism in general, was promoted by the fact that it got implemented in various geochemical codes, such as PHREEQC, EQ3/6, Geochemist’s Workbench, but also in CHEMAPP (Eriksson and Spencer, 1995; Eriksson et al., 1997), GEMS (Wagner et al., 2012; Kulik et al., 2013), or recently TOUGHREACT (Zhang et al., 2006). In parallel, the HMW-database was further developed and extended in response to the necessities of national programs for the disposal of radioactive waste in salt rock formations with the potential to contain high-saline solutions, e.g., the Yucca Mountain project (United States) (Mariner, 2004), the Waste Isolation Pilot Plant project (United States) (Domski and Nielsen, 2019), or the Oceanic Salt System from Voigt (2020) (Voigt, 2020a; Voigt, 2020b) with the alkali phosphate data from Scharge et al. (2013), Scharge et al. (2015) and the carbonate data from Harvie et al. (1984) as compiled in the THEREDA project in Germany (Moog et al., 2015; THEREDA, 2023). The Pitzer formalism was also applied to marine chemistry. Millero and co-workers over many years worked on a database specifically suited for seawater, e.g., (Pierrot and Millero, 2017). Recently, the Scientific Committee on Ocean Research of the International Council for Science (SCOR) created working group 145 to establish a reference seawater chemical speciation model, which is based on the Pitzer model (Turner et al., 2016).
The Pitzer formalism extends the Debye-Hückel equation with a virial expansion to account for binary and ternary ionic-strength-dependent specific interactions between ions of likewise and opposite charge (Pitzer, 1991). Explicit formulations for binary and ternary solutions of cations and anions are given by Scharge et al. (2012). In the present work, non-ideal interactions between a neutral component O2(aq) and cations or anions are investigated. More specifically, the focus is set on binary λ-interactions (between a neutral and a charged solute) and ternary ζ-interactions (one uncharged and two oppositely charged solutes), η-interactions (one uncharged and two similar charged solutes) and µ-interactions (two different uncharged and one charged solutes). The activity coefficient for a neutral species according to Pitzer (Pitzer, 1991) is:
Note, that in Eq. 9 ηNcc’, ηNaa’, µNn’c and µNn’a refer to interactions between a neutral solute and to two different non-neutral solutes with likewise charge. These interactions are mathematically identical to a ternary ζ-interaction. It is perhaps for this reason, that sometimes they are not properly distinguished in data files and instead referred to as ζ- or even ψ-interactions altogether.
A few publications are available in the literature providing Pitzer interaction coefficients for molecular oxygen in electrolyte solutions. Namely, the works of Clegg and Brimblecombe (1990), Millero et al. (2002a), Millero et al. (2002b), Millero et al. (2003), Millero and Huang (2003), Geng and Duan (2010), and Zheng and Mao (2019) are worth mentioning here. However, combining these data sets with the only existing Pitzer data set valid for higher temperatures and including the whole Oceanic Salt System (THEREDA) leads to inconsistencies. Such inconsistent databases will produce incorrect modeling results. This is demonstrated in Figure 1 by recalculating experimental data of O2 solubility in NaCl solution from literature (Millero et al., 2002a; Millero et al., 2002b; Millero et al., 2003). This does not mean that the various available Pitzer datasets for oxygen are of poor quality. However, it illustrates the importance of consistent data sets.
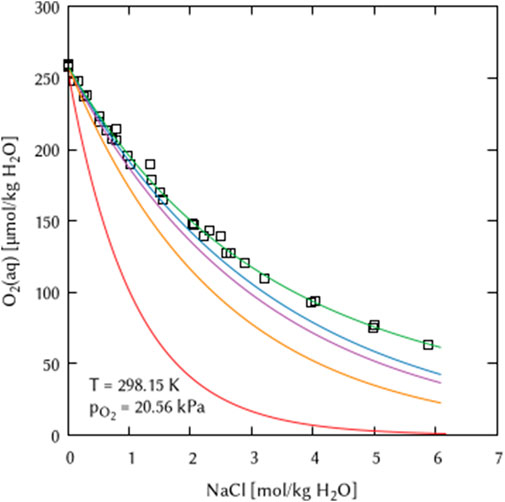
FIGURE 1. Effect of combining inconsistent Pitzer datasets on the example of O2 solubility in NaCl solution. □ Experimental data from Millero et al. (2002a), Millero et al. (2002b), Millero et al. (2003), lines: Calculation using the THEREDA Pitzer dataset (Moog et al., 2015; Voigt, 2020a; Voigt, 2020b; THEREDA, 2023) in combination with: blue) Millero et al. (2003), purple) Clegg and Brimblecombe (1990), orange) Geng and Duan (2010), red) Zheng and Mao (2019), green) this work.
This work focusses on primary experimental results. Respective literature on the solubility of molecular oxygen in pure water as well as in electrolyte solutions was collected, critically assessed, and used to recalibrate the temperature-dependent Henry’s law constant as well as the Pitzer interaction coefficients.
In many publications, the O2 solubility data are given in figures only. In these cases, the data were re-digitized using the software package “Engauge Digitizer” from Mitchell et al. (2020). The uncertainty resulting from this digitization step depends on the quality of the graphics, but in most cases can be neglected compared to the experimental uncertainty.
Experimentally obtained O2 solubility data are published using various different formats and units. Here, all datasets were recalculated to a micromolal scale (µmoles O2 per kg of water) as a function of salt concentration in molal scale (moles salt per kg of water). If the concentration data were available in a volume-related concentration unit, e.g., molar (moles per liter solution), they were converted using the density functions according to Söhnel and Novotný (1985). All solubility data on molecular oxygen in both, pure water and electrolyte solutions are provided in the supporting information.
All parameters for temperature dependency equations—for Henry’s law temperature equation as well as for the Pitzer interaction coefficients—were determined using the geochemical speciation software PHREEQC (batch version 3.7) (Parkhurst and Appelo, 2013) coupled with the parameter estimation software UCODE_2014 (Poeter et al., 2014) that uses a minimization of sum of squared residuals approach.
To determine the numerical values for the parameters of the temperature dependent Henry’s law Eq. 8, data for the oxygen solubility in pure water were collected from the literature. These solubility datasets were used to fit the simplified temperature function equation using parameters A1, A3, and A4 only. The common geochemical codes (e.g., ChemApp, Geochemist’s Workbench, PHREEQC, ToughReact) use six temperature parameters (Eq. 8 or mathematically equivalent transformations thereof) for the temperature-dependent description of reaction constants. Since a complete set of these temperature parameters must be specified in the code-specific parameter files, the other parameters (A2, A5 and A6) are set to zero. Older codes like EQ3/6 use a temperature-dependend grif of up to eight logK values; these can be easily calculated from the parameterized equation.
Most geochemical programs treat the Pitzer coefficients and their temperature parameter in a different way. There, polythermal equations use terms where the temperature is given relative to a reference temperature (mostly T = 298.15 K, sometimes referred to as “25°-centered”), see Eq. 10 or mathematically equivalent transformations thereof.
P is a Pitzer coefficient (here λ, ζ or η), T is the temperature in Kelvin, and Tr is the reference temperature (298.15 K) and ln the natural logarithm.
It was numerically impossible to fit all necessary temperature function parameters for all relevant Pitzer coefficients simultaneously. Therefore, a step-by-step approach was chosen: By definition, the coefficient λ(O2-Cl−) and therefore all temperature parameters for (X0–X5) were set to 0. First, the Pitzer coefficients at T = 298.15 K were fitted using the experimental O2 solubility data for the chemical subsystem H+, Na+, K+ - Cl−, OH− - H2O(l) only. The resulting values of the coefficients were then used in the successive fitting of the chemical subsystems H+, Na+, K+ - SO42− - H2O(l), Na+, K+ - CO32− - H2O(l) and H+, Na+, K+ - PO43− - H2O(l). All obtained interaction coefficients were then used as boundary conditions to fit interaction coefficients within the chemical subsystems of the Earth alkaline chlorides and Earth alkaline sulfates, respectively: Mg2+, Ca2+ - Cl−, OH− - H2O(l) as well as Mg2+ - SO42−, OH− - H2O(l). Finally, the interaction coefficients η(Cl−-SO42−) and η(Na+-Mg2+) for ternary salt solutions (NaCl + Na2SO4, NaCl + MgCl2) were fitted.
During the parameter determination, first all possible combinations of binary (λ) and ternary (ζ/η/µ) Pitzer coefficients should be determined. It became apparent that not all such coefficients were needed to describe the system, or that some combinations of binary and ternary coefficients were strongly cross-correlated. By reducing the number of adjustable coefficients, it was possible to create a data set that was sufficient to describe the system while using a minimum number of coefficients.
The coefficient for the ternary interaction ζ(O2-Na+-OH−) is not needed for the description of the system at 298.15 K. However, for the polythermal description of the system, this interaction cannot be neglected. Therefore, the coefficient was set to zero at 298.15 K.
The values of the Pitzer coefficients at T = 298.15 K correspond to the X0 parameters in Eq. 10. Thus, in the following steps, the Pitzer coefficients valid at T = 298.15 K were used as boundary parameters for the fits of the temperature dependency parameters X1 to X5. It was found that only the first two parameters (X1 and X2) had to be adjusted to fully describe the temperature dependency of the O2(aq) Pitzer coefficients. Thus, all other parameters (X3, X4 and X5) are set to zero.
A simultaneous fit of the temperature equations parameters including all chemical systems was not possible. Therefore, temperature parameters for the systems of solutions of binary electrolytes were iteratively fitted individually and sequentially until none of the parameters changed. Figure 2 shows the fitting scheme for the generation of the Pitzer interaction coefficients temperature parameters. Thus, a consistent set of parameterized temperature equations of the Pitzer interaction coefficients could be deduced.

FIGURE 2. Fitting scheme for the generation of the Pitzer interaction coefficients temperature parameters.
The coefficient for the ternary interaction ζ(O2-K+-HSO4−) could not be retrieved at 298.15 K as there are no experimental data for this temperature. The only O2 solubility data available for the alkali hydrosulfate (NaHSO4 and KHSO4) system were acquired at 310.2 K (Lang and Zander, 1986). The experimental data of the chemical system O2-Na2SO4-H2O could be described without a ternary interaction coefficient. For the description of the experimental data of the chemical system O2-K2SO4-H2O, however, a ternary interaction coefficient ζ(O2-K+-HSO4−) had to be introduced. To do so, the value for the ζ(O2-K+-HSO4−) coefficient was determined only in the context of the fits of the temperature parameters but had to be used as temperature independent one, since only experimental measured values for a single temperature are available. Verifying calculations including this coefficient in the fits of the 298.15 K data (X0 parameter) showed that this coefficient has no influence on the previously determined systems—namely, O2-K2SO4-H2O.
For the chemical systems for which no O2 solubility data are available at temperatures different from T = 298.15 K (carbonate system, phosphate system, ternary salt mixtures) no temperature parameters of the Pitzer coefficients could be obtained. Consequently, these Pitzer coefficients are valid at T = 298.15 K only and not included in Figure 2.
The aim of this work was to create a thermodynamic data set to calculate the oxygen solubility in pure water as well as in solutions containing ions ubiquitous in nature: Na+, K+, Mg2+, Ca2+, Cl−, HSO4−/SO42−, HCO3−/CO32−, H2PO4−/HPO42−/PO43−.
IUPAC published two reviews by Battino et al. (1981) and Clever et al. (2014) on available solubility data of molecular oxygen in pure water as part of the Solubility Data Series. This data collection was used here as a basis to create a temperature function for the solubility of O2. From these reviews, only solubility data within the temperature range from 273.15 up to 373.15 K with an oxygen partial pressure of 101.325 kPa were used. Solubility data at temperatures above 373.15 K were omitted as they are not the focus of the intended future usage of this data set. Moreover, due to the large scattering of the experimental data, using them significantly impaired the quality of the fitting results of the Henry’s law constant’s temperature equation at lower temperatures—specifically below 298.15 K.
For the O2 solubility in salt solutions, the number of publications containing experimental data is quite limited. A critical data evaluation was performed using the following criteria:
• Completeness of experimental conditions given,
• For datasets of electrolyte solutions: Agreement of the O2 solubility in the peripheral system (pure water at the given temperature) with accepted experimental values of the review workers of IUPAC or the calculated values from the temperature function of this work,
• Consistency in the order of magnitude and progression of the O2 solubility decrease with increasing salt concentration between datasets from different sources,
• Total gas pressure up to 101.325 kPa (1 atm): experimental datasets derived at higher pressures were discarded,
• Temperature range: References with experimental O2 solubility were ranked higher when they 1) provide data at T = 298.15 K and 2) provide data over a broader temperature range. This was done in order to create a data set with the highest possible applicability while maintaining consistency with the interaction coefficients valid at T = 298.15 K that were created in the first fitting step.
The literature review and data assessment on O2 solubility in salt solutions is given in Table 1.
The obtained temperature parameters for the logKH,cp of the O2 solubility in pure water are given in Table 2. The resulting temperature dependency equation describes the oxygen solubility in pure water very well (see Figure 3) while using only three of the six possible temperature terms.

TABLE 2. Temperature parameters of the logarithmic Henry’s law constant (logKH,cp) for the O2 solubility in pure water, Eq. 8.
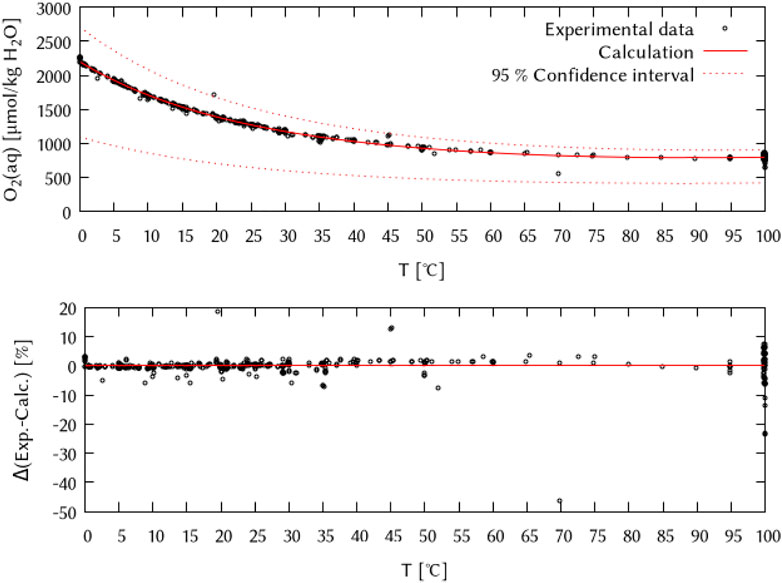
FIGURE 3. Temperature dependency of the logarithmic Henry’s law constant of the O2 solubility in pure water (expressed in µmol/kg H2O∙101.325 kPa). Points: experimental data from literature references given by IUPAC (Battino et al., 1981; Clever et al., 2014), solid line: calculation (this work), dashed line: 95% confidence interval.
Comparing the value at T = 298.15 K calculated with the obtained temperature function of Henry’s constant with values found in other thermodynamic databases shows very good agreement, see Table 3.
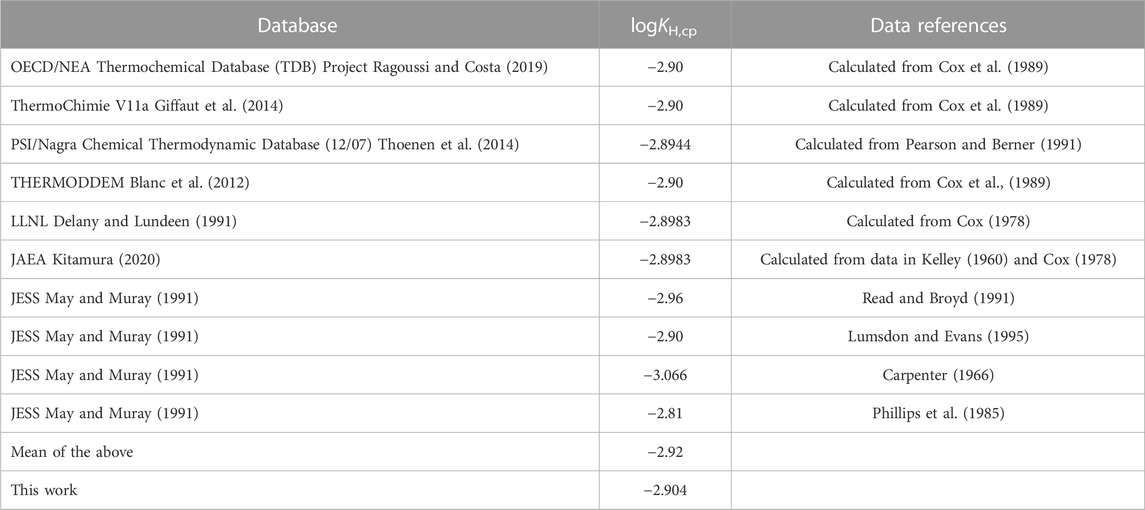
TABLE 3. Comparison of the logarithmic Henry’s law constant (logKH,cp) for the O2 solubility in pure water at T = 298.15 K used in different thermodynamic database projects and other sources.
To create an internally consistent set of equilibrium constants for reactions (Eqs 4, 5) it is necessary to select thermodynamic data for H2O(l), O2(g) and H2(g). The temperature function for the standard molar Gibbs enthalpy of formation is calculated with
Within the frame of this chapter, we adopt the following general temperature function as extension of Eq. 8:
Application of this temperature function for
with
For the corresonding temperature function for O2(aq) the compiled solubility data for oxygen in pure water (Table 2) were used. The equilibrium constant for reaction (Eq. 6) can be expressed as [index numbers of coefficients refer to the general function (Eq. 12)]
Using the corresponding parameters for O2(g) the temperature function for the standard molar Gibbs enthalpy of formation is then calculated with
For the next step, we use the following relations, where all temperature parameters
One notes that the
After evaluation for
with
Selected and calculated thermodynamic data are summarized in Tables 4–6. Standard formation data were adopted from Cox et al. (1989); heat capacities between 280 and 500 K were adopted from Chase (1998). For liquid water
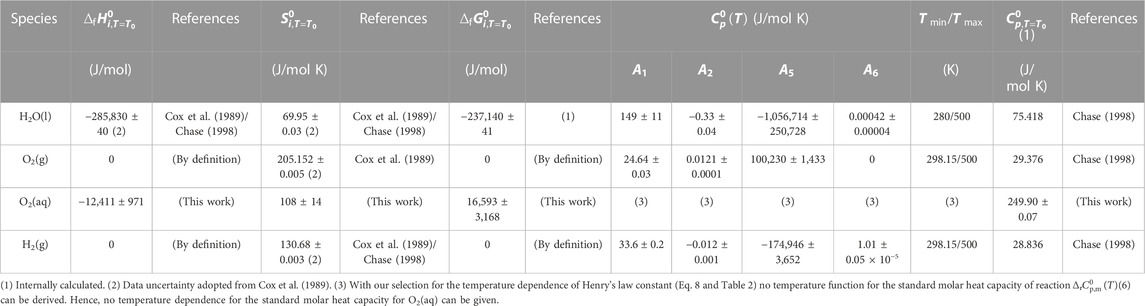
TABLE 5. Standard formation data for H2O(l), O2(g), H2(g), and O2(aq). Temperature parameters for
Uncertainties for standard formation data for O2(g), H2(g), and H2O(l) were adopted from Cox et al. (1989). Uncertainties for standard reaction and standard formation data for O2(aq) were calculated from the error of the temperature parameters for reaction (Eq. 6) in Table 2.
The obtained values are in good agreement with
it was possible to calculate the temperature functions for
The valid range of temperature for reaction (Eq. 4) [formation of O2(g)] is determined by the available heat capacity data for H2O(l). For reaction (Eq. 5) [formation of O2(aq)] it is limited by the availability of solubility data. Note, that for the latter reaction the given validity range is valid for low saline solutions only. In saline solutions where Pitzer coefficients are applied and the geochemical code works with a half-cell reaction involving O2(aq), the valid temperature range can be lower and depends on the particular system, see Section 4.5.
Using the temperature parameters in Table 6 the so-called “temperature grid” for “logK for EH-reaction” for codes like EQ3/6 was re-calculated. The results are given in Table 7. The range of temperature for which our parameters should be used is in fact narrower than is suggested in some data files for EQ3/6 or Geochemist’s Workbench.

TABLE 7. Calculated equilibrium constants for EH reactions (Eqs 4, 5) as a function of temperature. Values in brackets are beyond the recommended range of validity.
Not surprisingly, equilibrium constants given in Table 7 are similar to those currently available in data files for geochemical codes. The values given in this work, however, can be traced back to published standard formation and solubility data.
All binary mixtures of the chemical systems Na+, K+, H+/Cl−, OH− - H2O(l) were fitted simultaneously to obtain the Pitzer coefficients. As an initial coefficient λ(O2(aq)-Cl−) was set to zero. Binary interaction coefficients (λ) for all cations and anions in the system could be obtained. During parameter reduction, it was found that only the ternary interaction coefficients ζ(O2(aq)-Na+-Cl−) and ζ(O2(aq)-K+-Cl−) are necessary to describe the system (Figure 4). Later fits of the temperature dependence parameters showed that a ternary ζ(O2(aq)-Na+-OH−) coefficient becomes necessary at higher temperatures. At T = 298.15 K this coefficient was set to 0.
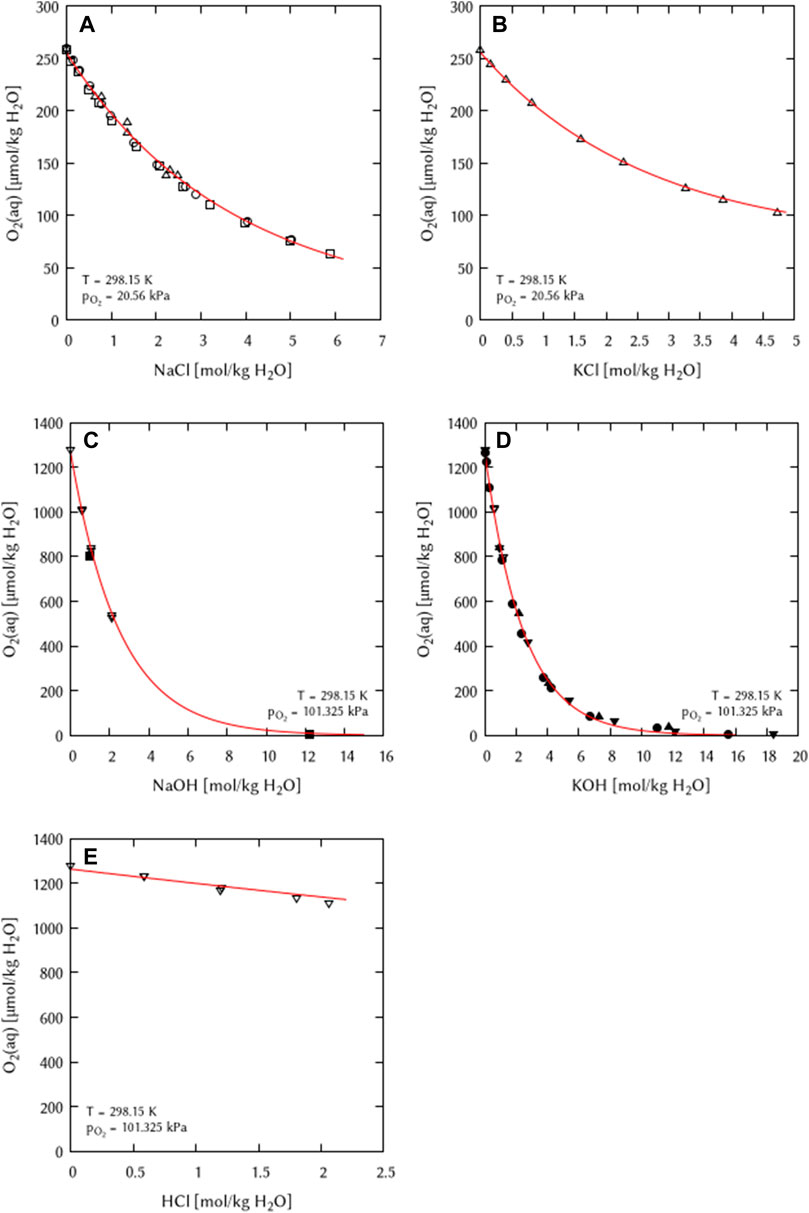
FIGURE 4. Modelling of the O2 solubility in binary solutions of (A) NaCl, (B) KCl, (C) NaOH, (D) KOH, and (E) HCl as a function of the electrolyte concentration at T = 298.15 K (Points: Experimental data from: □ Millero et al. (2002a), ○ Millero et al. (2002b), ▵ Millero et al. (2003), ▿ Geffcken (1904), ■ Chatenet et al. (2000), ● Davis et al. (1967), ▴ Gubbins and Walker (1965), ▾ Shoor et al. (1968), Lines: this work).
The obtained binary interaction coefficients (λ) were used as initial values for the simultaneous fitting of the binary mixtures of the system Na+, K+, H+/HSO4−, SO42− - H2O(l). The full set of binary [λ(O2(aq)-HSO4−) and λ(O2(aq)-SO42−)] and ternary interaction coefficients [ζ(O2(aq)-Na+- SO42−) and ζ(O2(aq)-K+- SO42−)] was found do be necessary for a full description of the system (Figure 5).
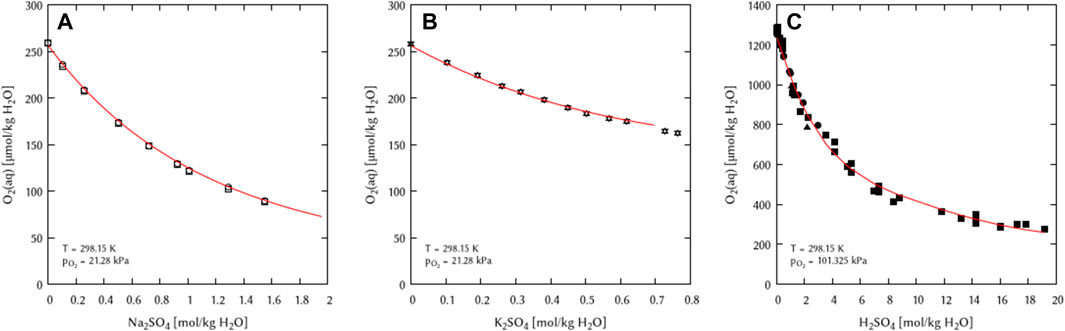
FIGURE 5. Modelling of the O2 solubility in binary solutions of (A) Na2SO4, (B) K2SO4, and (C) H2SO4 as a function of the electrolyte concentration at T = 298.15 K (Points: Experimental data from: □ Millero et al. (2002a), ○ Millero et al. (2002b), ▵ Millero et al. (2003), ▿ Millero and Huang (2003), ■ Das (2005), ● Geffcken (1904), ▴ Narita et al. (1983), Lines: this work).
For the solubility of oxygen in NaHSO4 or KHSO4 solutions, there are no data at T = 298.15 K available in literature. Thus, the ζ(O2(aq)-K+-HSO4−) value was not fitted from data at 298.15 K but from the only available data at 310.2 K from Lang and Zander (1986). The value was derived from a fitting using the polythermal data set (see Section 4.4). Since no temperature dependency could be retrieved, the ζ(O2(aq)-K+-HSO4−) value was set temperature independent. Therefore, the obtained value must be set to the 298.15 K term (A0) in Eq. 10. The implementation of the ζ(O2(aq)-K+-HSO4−) coefficient had no influence of the fitting of the ζ(O2(aq)-K+-SO4−) coefficient, which was counterchecked. No value had to be fitted for ζ(O2(aq)-Na+-HSO4−) in NaHSO4 solutions because the dataset derived in this work was already sufficient to describe the oxygen solubility in this solution without another ternary interaction coefficient (see Section 4.4.2).
The previously obtained binary interaction coefficients (λ) were used as boundary conditions for the simultaneously fitting of the binary mixtures of the system Na+,K+/CO32− - H2O(l). A binary [λ(O2-CO32−)] as well as two ternary interaction coefficients [ζ(O2(aq)-Na+-CO32−) and ζ(O2(aq)-K+-CO32−)] are required to describe the two systems (Figure 6).
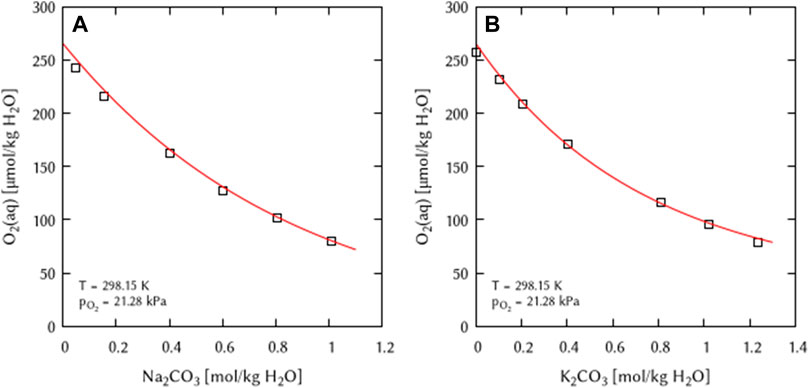
FIGURE 6. Modelling of the O2 solubility in binary solutions of (A) Na2CO3, and (B) K2CO3 as a function of the electrolyte concentration at T = 298.15 K (Points: Experimental data from □ Khomutov and Konnik (1974), Lines: this work).
The O2 solubility in solutions of Na3PO4, K3PO4, K2HPO4, KH2PO4, and H3PO4 were fitted simultaneously to obtain the interaction coefficients. For the System O2-K3PO4 it was found that no ternary interaction coefficient ζ(O2(aq)-K+-PO43−) is necessary to describe the experimental data while a ζ(O2(aq)-Na+-PO43−) was required for the System O2-Na3PO4. Also for the acidic potassium phosphate solutions (KH2PO4 and K2HPO4) as well as for phosphoric acid solutions, ternary interaction coefficients were found to be indispensable to fully describe these systems (Figure 7). No experimental O2 solubility data were available for the acidic sodium phosphate solutions (NaH2PO4 and Na2HPO4). Therefore, it cannot be specified whether or not ternary interaction coefficients are necessary in these subsystems.
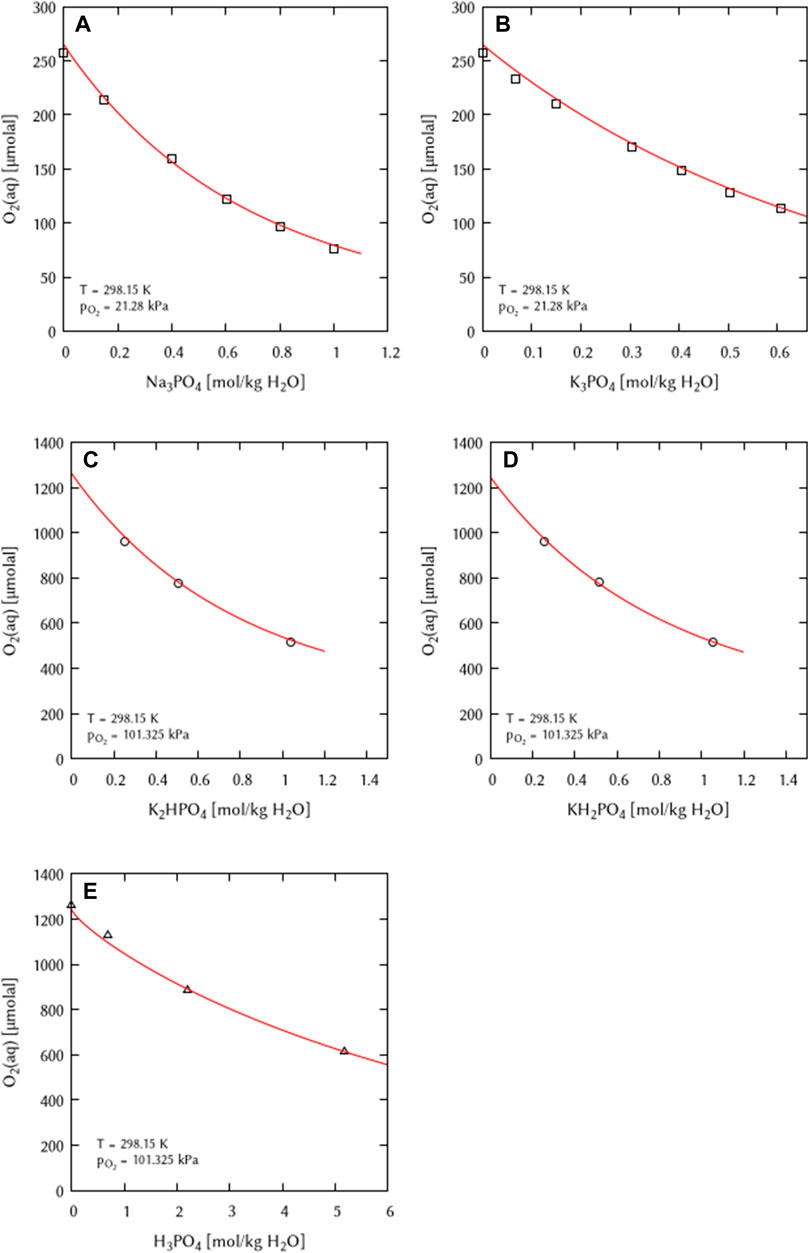
FIGURE 7. Modelling of the O2 solubility in binary solutions of (A) Na3PO4, (B) K3PO4, (C) K2HPO4, (D) KH2PO4, and (E) H3PO4 as a function of electrolyte concentration at T = 298.15 K (Points: Experimental data from □ Khomutov and Konnik (1974), ○ Iwai et al. (1993), ▵ Gubbins and Walker (1965), Lines: this work).
Using the previously determined Pitzer coefficients as boundary conditions, the interaction coefficients for Earth alkaline salts and mixtures of salt solutions were deduced. For all three systems, a binary as well as a ternary interaction coefficient was necessary for a complete description (Figure 8).
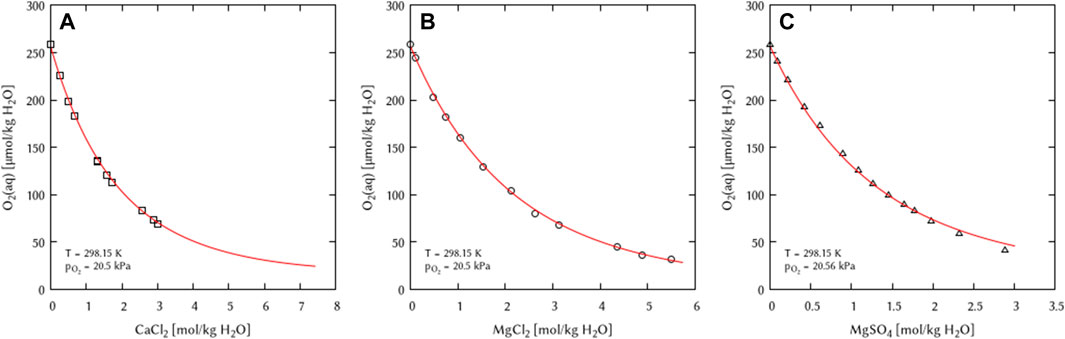
FIGURE 8. Modelling of the O2 solubility in binary solutions of (A) CaCl2, (B) MgCl2, and (C) MgSO4 as a function of electrolyte concentration at T = 298.15 K (Points: Experimental data from □ Millero et al. (2003), ○ Millero et al. (2002a), ▵ Millero et al. (2002b) Lines: this work).
The O2 solubility data in ternary solutions of NaCl + MgCl2 and NaCl + Na2SO4 was used to deduce the coefficients η(O2(aq)-Na+-Mg2+) and η(O2(aq)-Cl−-SO42−). The O2 solubility data in the ternary solution of Na2SO4 + MgSO4 was not used in the fitting procedure but to verify the obtained η coefficient (Figure 9). The obtained calculation results are in good agreement with the experimental data. The O2 solubility data in the ternary solution of MgCl2 + MgSO4 was also not used in the fitting, the authors claimed the usage of two different stock solutions within the experiment (Millero et al., 2002a). Only one data point matches with the calculation suggesting that—in agreement with the primary source—this point belonged to one charge of stock solutions while the other points belong to the experiments with other stock solutions.
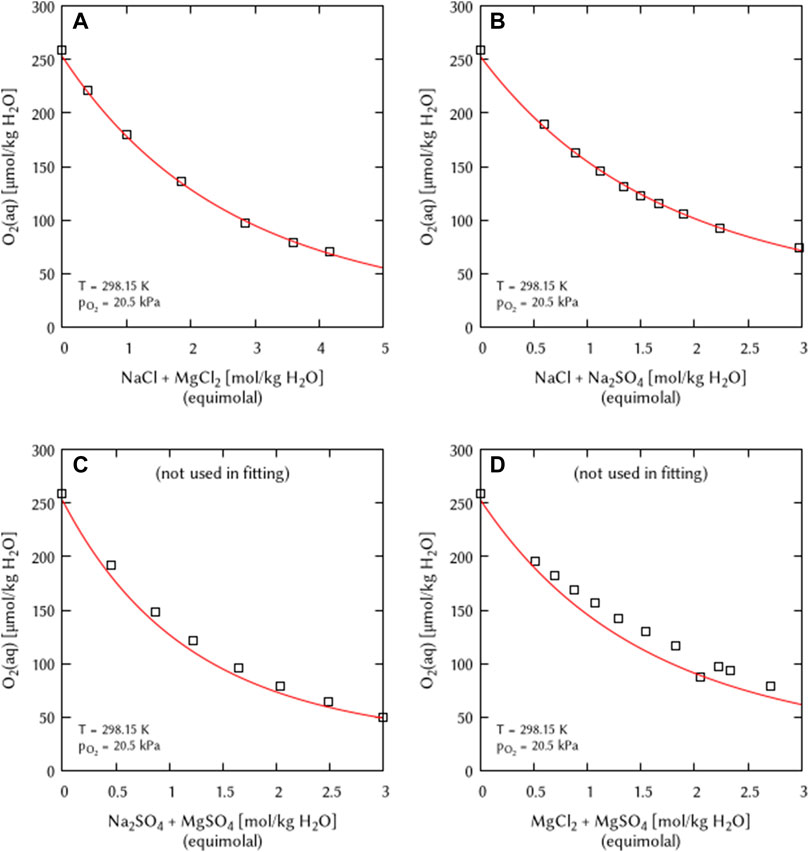
FIGURE 9. Modelling of the O2 solubility in equimolal solutions of (A) NaCl + MgCl2, (B) NaCl + Na2SO4, (C) Na2SO4+MgSO4, and (D) MgCl2 + MgSO4 as a function of electrolyte concentration at T = 298.15 K (Points: Experimental data from □ Millero et al. (2002a), Lines: this work).
Millero et al. (2002a) also experimentally studied O2 solubility in the quaternary-reciprocal systems Na+, Mg2+/Cl−, SO42− - H2O were given. Interaction coefficients for these kind of systems could not be deduced because the Pitzer ion-interaction approach is limited to coefficients for ternary interactions while in this case a coefficient would be necessary for an interaction between four different species (O2-c-a-a’/O2-c-c’-a) in solution.
The obtained Pitzer interaction coefficients—ion-neutral species pairs, λ and ion-neutral species triplets, ζ and η—are given in Table 8.
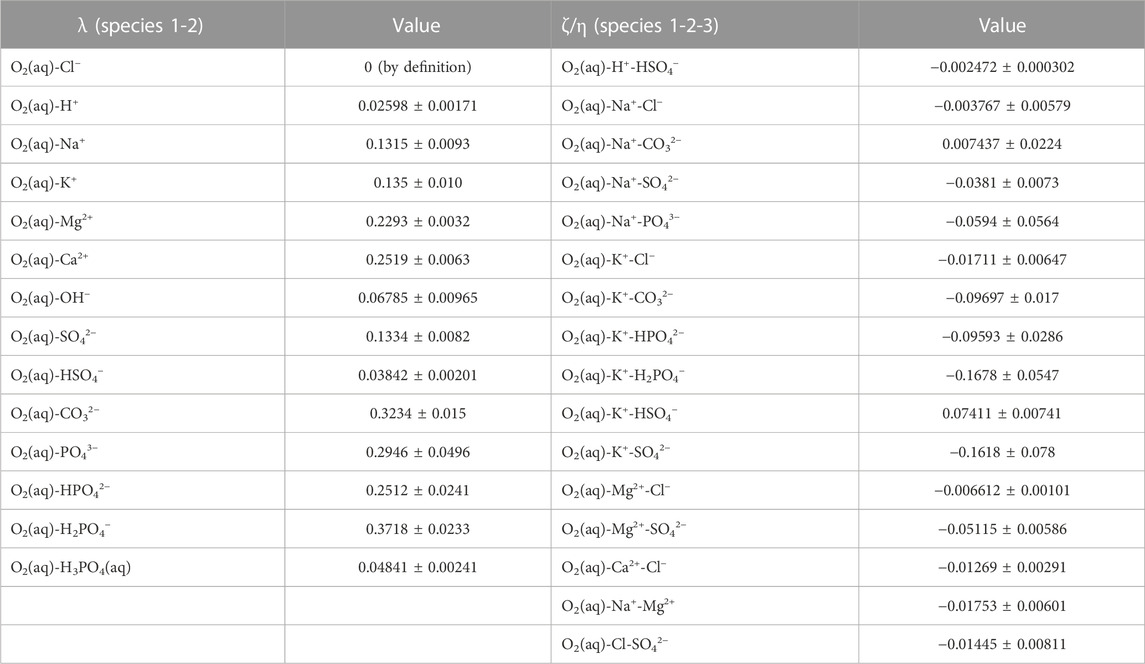
TABLE 8. Binary and ternary ion-neutral species interaction coefficients (λ, ζ, η) for the solubility of O2 in salt solutions at T = 298.15 K. The uncertainty information refers to one standard deviation.
For the polythermal description of O2 solubility in electrolyte solutions, the Pitzer interaction coefficients valid for 298.15 K were used as a boundary condition for fitting of the temperature parameters for Eq. 10 on top of them. These parameter fittings were run individually for each electrolyte and then iterated until no changes were observed anymore.
The binary systems of the alkali metal chlorides, alkali metal hydroxides and hydrochloric acid allowed to determine the temperature dependency for two binary interaction coefficients: λ(O2(aq)-Na+), λ(O2(aq)-K+), and λ(O2(aq)-H+) as well as for two ternary coefficients ζ(O2(aq)-Na+-Cl−) and ζ(O2(aq)-K+-Cl−) (see Figures 10, 11). For each coefficient, the temperature dependency can be described using only two of the six parameters of Eq. 10, which are the
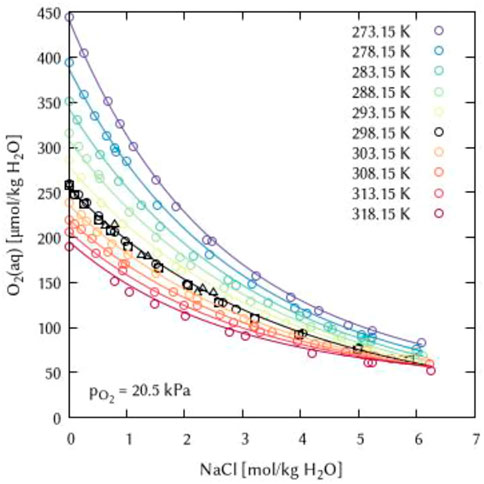
FIGURE 10. Modelling of the O2 solubility in sodium chloride solution as a function of temperature and NaCl concentration. (Points: Experimental data from □ Millero et al. (2002a), ○ Millero et al. (2002b), ▵ Millero et al. (2003), Lines: this work).
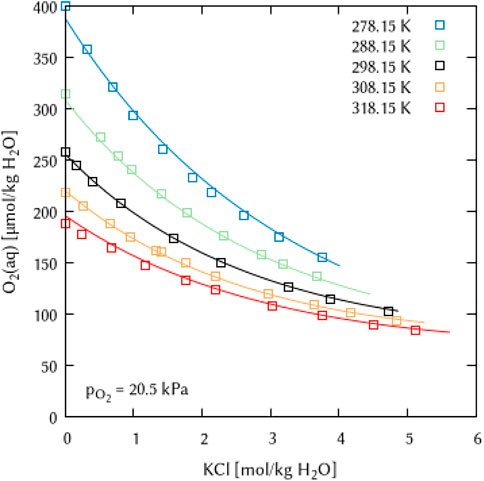
FIGURE 11. Modelling of the O2 solubility in potassium chloride solution as a function of temperature and KCl concentration. (Points: Experimental data from □ Millero et al. (2003), Lines: this work).
For the ternary coefficient ζ(O2(aq)-Na+-OH−) with a value of 0 at T = 298.15 K, only the first parameter of the temperature dependency equation was found to be necessary to adequately describe the experimental points available (Figure 12).
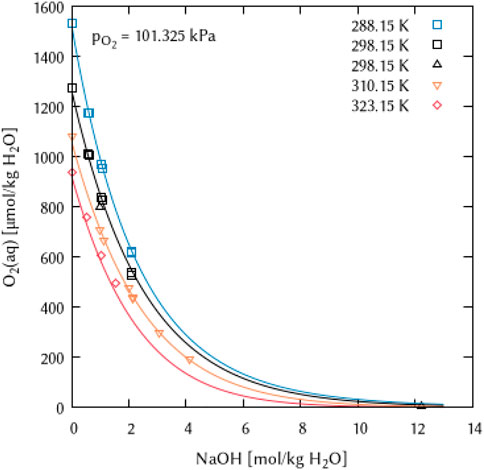
FIGURE 12. Modelling of the O2 solubility in sodium hydroxide solution as a function of temperature and NaOH concentration. (Points: Experimental data from □ Geffcken (1904), ▵ Chatenet et al. (2000), ▿ Lang and Zander (1986) and ⬦ Bruhn et al. (1965), Lines: this work).
Even though the few available experimental points on O2 solubility at higher KOH concentrations and raised temperatures (T = 353.15–373.15 K) are not well reproduced in terms of the expected trend of temperature dependence (Figure 13), the introduction of another ternary coefficient ζ(O2(aq)-K+-OH−) or temperature dependence parameters thereof did not lead to any improvement. Furthermore, it can be assumed that the deviation of the calculation from the expected trend (lowering the O2 solubility with increasing temperature) in this concentration and temperature range is lower than the experimental uncertainty to be expected.
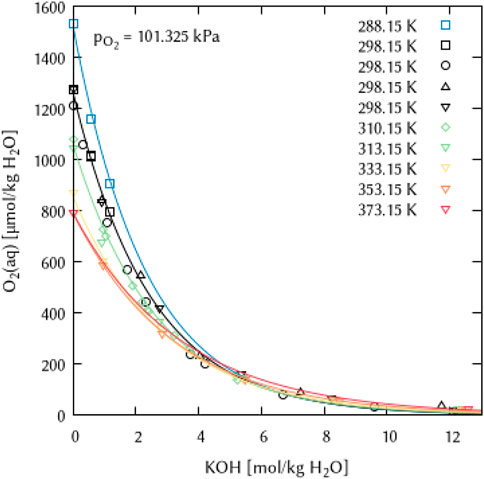
FIGURE 13. Modelling of the O2 solubility in potassium hydroxide solution as a function of temperature and KOH concentration. (Points: Experimental data from □ Geffcken (1904), ○ Davis et al. (1967), ▵ Gubbins and Walker (1965), ▿ Shoor et al. (1968) and ⬦ Lang and Zander (1986), Lines: this work).
For solutions of hydrochloric acid, even in the polythermal system, no ternary interaction coefficient and thus no temperature parameters were necessary to describe the very few points on O2 solubility as a function of temperature and electrolyte concentration (Figure 14).
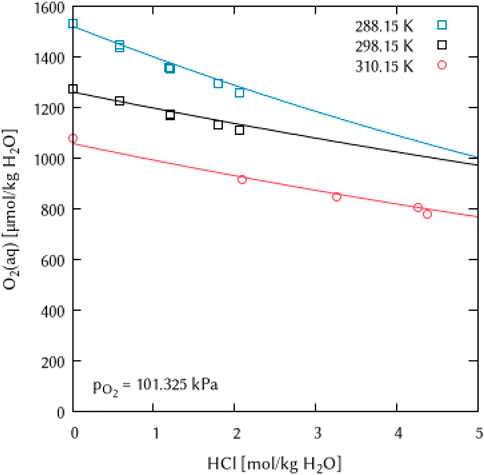
FIGURE 14. Modelling of the O2 solubility in hydrochloric acid solution as a function of temperature and HCl concentration. (Points: Experimental data from □ Geffcken (1904) and ○ Lang and Zander (1986), Lines: this work).
In the systems of alkali metal sulfate and sulfuric acid solutions, it was possible to obtain parameters describing the temperature dependence of the Pitzer interaction coefficients. Again, the fitting of a maximum of two terms was sufficient to describe the available data points for the Na2SO4 and H2SO4 systems (Figures 15, 16).
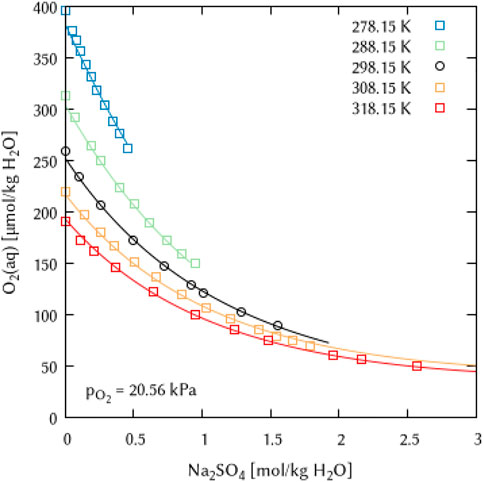
FIGURE 15. Modelling of the O2 solubility in sodium sulfate solution as a function of temperature and Na2SO4 concentration. (Points: Experimental data from ○ Millero et al. (2002a) and □ Millero et al. (2002b), Lines: this work).
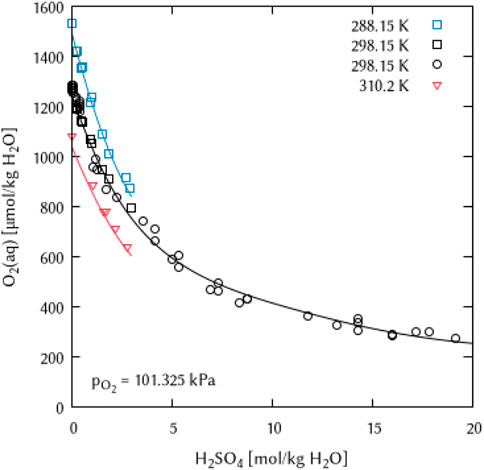
FIGURE 16. Modelling of the O2 solubility in sulfuric acid solution as a function of temperature and H2SO4 concentration. (Points: Experimental data from □ Geffcken (1904), ○ Das (2005) and ▿ Lang and Zander (1986), Lines: this work).
In the K2SO4 solutions, O2 solubility is poorly described at both very low (278.15 K) and very high (318.15 K) temperatures, yielding too high values with increasing electrolyte concentration (Figure 17) in both cases. Since the temperature dependence equation of the Pitzer interaction coefficients in the geochemical codes centers around the value of 298.15 K (see Eq. 10), these effects cannot be compensated, as a fit improvement in the temperature range above 298.15 K causes a corresponding deterioration below 298.15 K and vice versa.
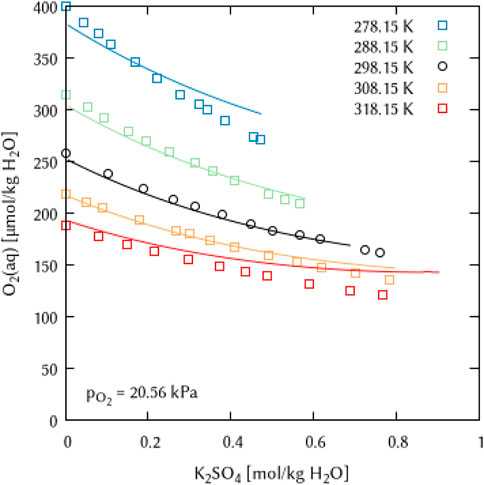
FIGURE 17. Modelling of the O2 solubility in potassium sulfate solution as a function of temperature and K2SO4 concentration. (Points: Experimental data from ○ Millero et al. (2003) and □ Millero and Huang (2003), Lines: this work).
The systems Na+/HSO4− - H2O(l) and K+/HSO4− - H2O(l) could not be used for the estimation of the ternary interaction coefficients ζ(O2(aq)-Na+-HSO4−) or ζ(O2(aq)-K+-HSO4−) because of the lack of O2 solubility data at 298.15 K. The only available experimental datasets are at 310.2 K generated by Lang and Zander (1986). Thus, it is not possible to obtain a ζ coefficient at 298.15 K that is necessary for the fitting with the temperature dependency equation used in this study. The systems Na⁺/HSO4⁻ - H2O(l) and K⁺/HSO4⁻ - H2O(l) were modelled using the obtained dataset. Results indicate that no ternary interaction coefficient ζ(O2(aq)-Na+-HSO4−) is necessary to describe the O2 solubility data in the system Na⁺/HSO4⁻ - H2O(l) (see Figure 18).
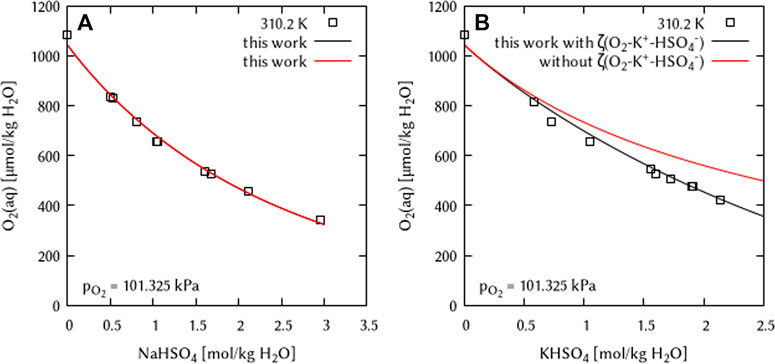
FIGURE 18. Modelling of the O2 solubility in binary solutions of (A) NaHSO4, and (B) KHSO4 as a function of electrolyte concentration at 310.2 K using the Pitzer coefficient set of this work. [Points: Experimental data from □ Lang and Zander (1986), Lines: this work].
Modelling results for the chemical system K⁺/HSO4⁻ - H2O(l) without an ternary interaction coefficient show a discrepancy between the experimental results from Lang and Zander (1986) and the modelling. Thus, a ternary interaction coefficient ζ(O2(aq)-K+-HSO4−) was fitted at T = 310.2 K. Due to the lack of solubility data at other temperatures, no parameters for the temperature function could be derived. Therefore, this ternary coefficient was assumed to be temperature independent.
The binary systems of the alkaline Earth metal salts CaCl2, MgCl2 and MgSO4 were used to derive the temperature dependency parameters for the binary interaction coefficients λ(O2(aq)-Ca2+) and λ(O2(aq)-Mg2+) as well as for the ternary coefficients ζ(O2(aq)-Ca2+-Cl−), ζ(O2(aq)-Mg2+-Cl−) and ζ(O2(aq)-Mg2+-SO42−), see Figures 19–21. For the magnesium salt solutions, the temperature dependency of the interaction coefficients requires two parameters [
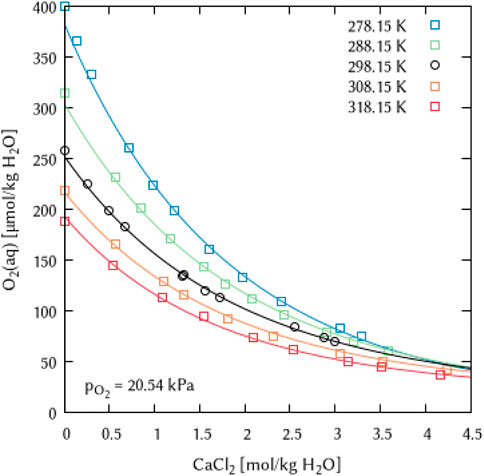
FIGURE 19. Modelling of the O2 solubility in calcium chloride solution as a function of temperature and CaCl2 concentration. [Points: Experimental data from ○ Millero et al. (2003) and □ Millero and Huang (2003), Lines: this work].
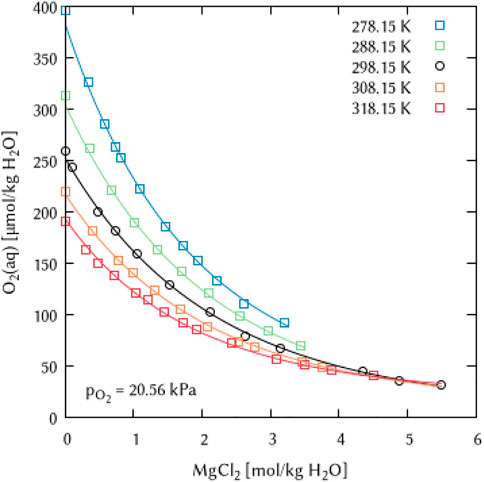
FIGURE 20. Modelling of the O2 solubility in magnesium chloride solution as a function of temperature and MgCl2 concentration. [Points: Experimental data from ○ Millero et al. (2002a) and □ Millero et al. (2002b), Lines: this work].
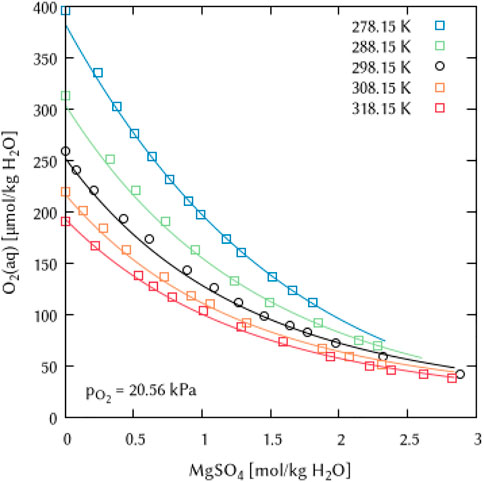
FIGURE 21. Modelling of the O2 solubility in magnesium sulfate solution as a function of temperature and MgSO4 concentration. [Points: Experimental data from ○ Millero et al. (2002a) and □ Millero et al. (2002b), Lines: this work].
The full list of obtained temperature parameters for the polythermal Pitzer interaction coefficients is given in Table 9.
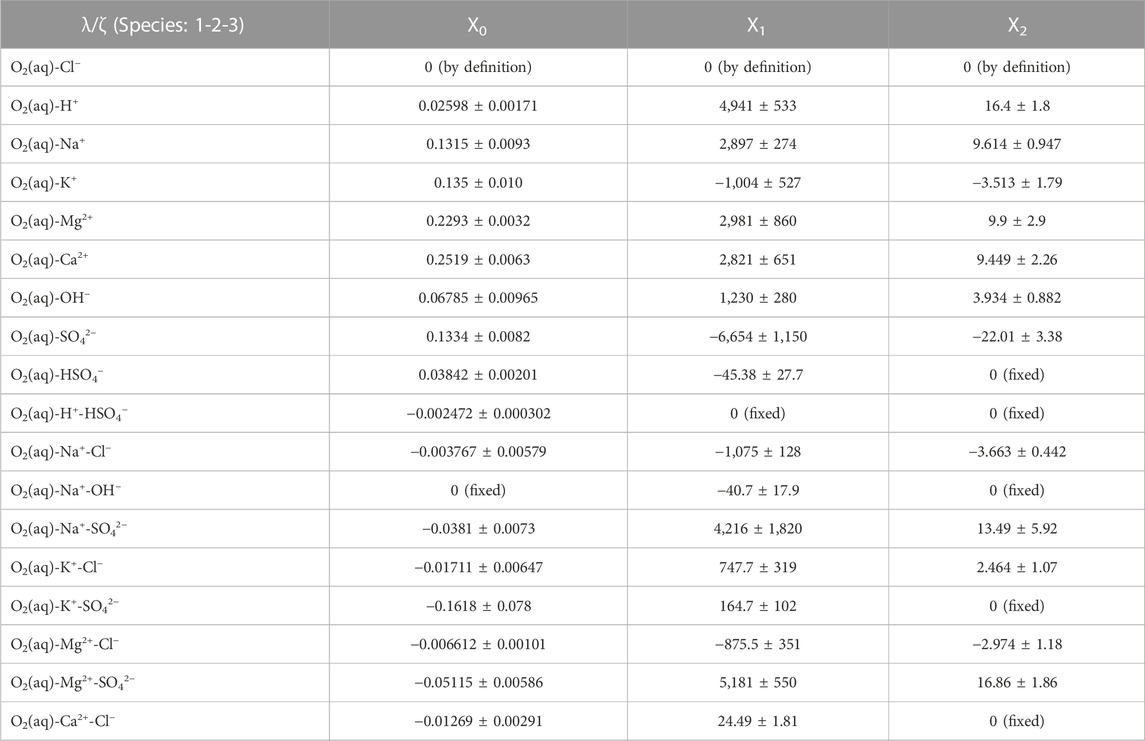
TABLE 9. Parameters for the temperature dependency function of the binary and ternary interaction coefficients (λ, ζ). The uncertainty information refers to one standard deviation.
For the carbonate, the phosphate, and ternary systems in the oceanic salt system, no temperature dependency parameters of the interaction coefficients could be deduced so far due to the very limited data available. Therefore, for polythermal datasets, the temperature function parameters X1–X5 for the Pitzer interaction coefficients are set to zero.
The conservative validity range for this dataset is given as follows:
• Temperature: T = 273–318 K,
• Ionic strength: I ≤ 5 mol/kg H2O,
• O2 partial pressure: p(O2) ≤ 101.325 kPa.
This generalized specification is not valid for all chemical subsystems. The validity ranges of temperature and ionic strength of the individual background electrolyte are given in Table 10 according to the experimental data sets used for the parameter fitting.

TABLE 10. Validity ranges (T, I, p) according to the experimental conditions used for the parameter fitting.
For the O2 solubility in binary solutions of bicarbonates only one experimental dataset (O2 in NaHCO3 solutions with T = 323–423 K and p = 1–5 MPa) is available in literature (Broden and Simonson, 1978) and (Broden and Simonson, 1979). It was not possible to extrapolate to 298.15 K and 101.325 kPa using the temperature and pressure dependency functions given in the articles or other extrapolation methods. Therefore, neither this binary interaction coefficient λ(O2(aq)-HCO3−) nor its temperature function parameters could be deduced so far.
There are no O2 solubility data for other ternary mixtures of salt solutions published in literature beside the work of Millero et al. (2002a) (system Na+, Mg2+/Cl−, SO42− - H2O(l) at 298.15 K). Therefore only the interaction coefficients ζ(O2(aq)-Na+-Mg2+) and ζ(O2(aq)-Cl−-SO42−) for these ternary systems could be deduced. Here, especially the ternary interaction coefficients with hydroxide [ζ(O2(aq)-OH−-Cl−) and ζ(O2(aq)-OH−-SO42−)] would be of interest, since the O2 solubility decreases significantly more in alkaline solutions than in neutral or acidic electrolyte solutions. For all other ternary (or higher) mixtures, no interaction coefficients could be obtained. The same applies to the temperature dependence of the O2 interaction parameters in ternary electrolyte solutions.
Ternary interactions coefficients for systems with alkaline Earth ions (Mg2+, Ca2+) and bivalent or trivalent anions (CO32−, SO42−, PO43−)—except ζ(O2(aq)-Mg2+-SO42−)—are assumed to be unnecessary because of the low solubility of the formed solid phases, e.g., calcite, dolomite, gypsum or apatite. The contributions of these ions in such solutions are sufficiently covered by the binary interaction coefficients.
In this paper, a set of thermodynamic formation data for dissolved oxygen O2(aq) consistent with standard formation data for O2(g), H2(g), H2O(l), and a temperature-dependent Henry’s law constant is presented. In combination with binary and ternary Pitzer coefficients they allow the calculation of oxygen solubility in concentrated salt solutions of the system Na+, K+, H+, Ca2+, Mg2+/Cl−, SO42−, CO32−, PO43−, OH− - H2O(l), including also the associated acids and bases. By combining a very large number of experimental papers, which have been subjected to critical evaluation, the data set is based on a wide range of data, which in turn ensures robustness.
Contrary to values for the so-called logK-EH-grid circulating among geochemical modelers, values given herein can be traced back to experimental solubility data and standard formation data. Moreover, their validity in terms of temperature and solution composition is clearly stated.
The use of the dataset allows a more accurate description of the redox state of saline solutions. This does not only improve the description of the solubility of redox-sensitive radionuclides, but is also applicable to a variety of other processes—from material corrosion to microbial activity. The presented dataset allows modeling with a broader application range than the data compilations previously available in the literature, both in terms of the number of chemical subsystems and the temperature range. With a more precise description of redox conditions, it is possible to further reduce conservatism in safety assessment calculations, not only in the context of a nuclear repository.
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
FB: conceptualization, methodology, data analysis, software, fitting (Henry’s law constant and Pitzer ion-interaction coefficients), writing; HM: methodology and data review (thermodynamics), writing; VB: review and editing, supervision, funding acquisition.
This work was funded by BGE—the federal company for radioactive waste disposal, with the contract number 45181017.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fnuen.2023.1158109/full#supplementary-material
Armenante, P. M., and Karlsson, H. T. (1982). Salting-out parameters for organic acids. J. Chem. Eng. Data 27, 155–156. doi:10.1021/je00028a016
Battino, R., Cargill, R. W., Chen, C. T. A., Clever, H. L., Roth, J. A., Cramer, A. L., et al. (1981). IUPAC solubility data Series vol. 7: Oxygen and ozone. Oxford: Pergamon Press, 533.
Baykut, S., and Aroguz, A. Z. (1989). The effect of different electrolytes on the solubility of gases in water. Chim. Acta Turc. 17, 365–375.
Benson, B. B., Krause, D., and Peterson, M. A. (1979). The solubility and isotopic fractionation of gases in dilute aqueous solution. I. Oxygen. J. Solut. Chem. 8, 655–690. doi:10.1007/BF01033696
Bethke, C. M. (2022). Geochemical and biogeochemical reaction modeling. 3rd Edition. Cambridge: Cambridge University Press. ISBN-13: 978-1-108-79086-4.
Bikov, M. M. (1937). The solubility of gases in solutions of salts under pressure at high temperature. Tr. Voronezhsk. Gos. Univ. 9, 29–57.
Blanc, P., Lassin, A., Piantone, P., Azaroual, M., Jacquemet, N., Fabbri, A., et al. (2012). Thermoddem: A geochemical database focused on low temperature water/rock interactions and waste materials. Appl. Geochem. 27 (10), 2107–2116. doi:10.1016/j.apgeochem.2012.06.002
Bohr, C. (1910). Über die Löslichkeit von Gasen in konzentrierter Schwefelsäure und in Mischungen von Schwefelsäure und Wasser. Z. Phys. Chem. 71, 47–50. doi:10.1515/zpch-1910-7104
Broden, A., and Simonson, R. (1978). Solubility of oxygen Part 1. Solubility of oxygen in water at temperatures ≤150°C and pressures ≤5MPa. Sven. Papperstidn. 81, 541–544.
Broden, A., and Simonson, R. (1979). Solubility of oxygen Part 2. Solubility of oxygen in sodium hydrogen carbonate and sodium hydroxide solutions at temperatures ≤150°C and pressures ≤5MPa. Sven. Papperstidn. 82, 487–491.
Bruhn, G., Gerlach, J., and Pawlek, F. (1965). Untersuchungen über die Löslichkeit von Salzen und Gasen in Wasser und wässrigen Lösungen bei Temperaturen oberhalb von 100 °C (Studies on the solubility of salts and gases in water and aqueous solutions at temperatures above 100 °C). Z. Anorg. Allg. Chem. 337 (1-2), 68–79. doi:10.1002/zaac.19653370111
Carpenter, J. H. (1966). New measurements of oxygen solubility in pure and natural Water. Limnol. Ocean. 11, 264–277. doi:10.4319/lo.1966.11.2.0264
M. W. Chase (Editor) (1998). “Journal of physical and chemical reference data, monograph No. 9, NIST-JANAF thermochemical Tables,” Published by the American chemical society and the American institutes of physics for the national Institute of standards and technology. Fourth Edition (Gaithersburg, Maryland: National Institute of Standards and Technology), 20899.
Chatenet, M., Aurousseau, M., and Durand, R. (2000). Electrochemical measurement of the oxygen diffusivity and solubility in concentrated alkaline media on rotating ring-disk and disk electrodes—Application to industrial chlorine-soda electrolyte. Electrochim. Acta 45, 2823–2827. doi:10.1016/S0013-4686(00)00325-X
Christov, C., and Møller, N. (2004). A chemical equilibrium model of solution behavior and solubility in the H-Na-K-Ca-OH-Cl-HSO4-SO4-H2O system to high concentration and temperature. Geochim. Cosmochim. Acta 68 (18), 3717–3739. doi:10.1016/j.gca.2004.03.006
Clegg, S., and Brimblecombe, P. (1990). The solubility and activity coefficient of oxygen in salt solutions and brines. Geochim. Cosmochim. Acta 54, 3315–3328. doi:10.1016/0016-7037(90)90287-U
Clever, H. L., Battino, R., Miyamoto, H., Yampolski, Y., and Young, C. L. (2014). IUPAC-NIST solubility data Series. 103. Oxygen and ozone in water, aqueous solutions, and organic liquids (supplement to solubility data Series volume 7). J. Phys. Chem. Ref. Data 43, 033102-1–033102-209. doi:10.1063/1.4883876
Cox, J. D. (1978). CODATA recommended key values for thermodynamics, 1977 Report of the CODATA Task Group on key values for thermodynamics, 1977. J. Chem. Thermodyn. 10 (10), 903–906. doi:10.1016/0021-9614(78)90050-2
Cox, J. D., Wagman, D. D., and Medvedev, V. A. (1989). CODATA key values for thermodynamics. New York: Hemisphere Publishing Corp.
Cramer, S. D. (1984). Oxygen solubility in brines. Ind. Eng. Chem. Process. Des. Dev. 23 (3), 618–620. doi:10.1021/i200026a035
Cramer, S. D. (1980). The solubility of oxygen in brines from 0 to 300 °C. Ind. Eng. Chem. Process. Des. Dev. 19 (2), 300–305. doi:10.1021/i260074a018
Das, T. N. (2005). Saturation concentration of dissolved O2 in highly acidic aqueous solutions of H2SO4. Ind. Eng. Chem. Res. 44, 1660–1664. doi:10.1021/ie049539m
Davis, R. E., Horvath, G. L., and Tobias, C. W. (1967). The solubility and diffusion coefficient of oxygen in potassium hydroxide solutions. Electrochim. Acta 12, 287–297. doi:10.1016/0013-4686(67)80007-0
Delany, J. M., and Lundeen, S. R. (1991). The LLNL thermochemical data base - revised data and file format for the EQ3/6 package (No. UCID-21658). Livermore, CA, United States: Lawrence Livermore National Lab (LLNL.
Domski, P., and Nielsen, S. R. (2019). WASTE ISOLATION PILOT PLANT AP-183 Revision 1 analysis plan to update the WIPP geochemical thermodynamic database (DATA0. FM1) to Data0. FM4 for CRA-2019.
Eriksson, G., Hack, K., and Petersen, S. (1997). Chemapp—A programmable thermodynamic calculation interface. Werkst. Woche 96, 47–51.
Eriksson, G., and Spencer, P. (1995). “A general thermodynamic software interface,” in Proceedings 2nd colloquium on process simulation (Otaniemi: Helsinki University of Technology), 113. (Report TKK-V-B104).
Eucken, A., and Hertzberg, G. (1950). Aussalzeffekt und Ionenhydratation. Z. Phys. Chem. 195, 1–23. doi:10.1515/zpch-1950-19502
Geffcken, G. (1904). Beiträge zur Kenntnis der Löslichkeitsbeeinflussung. Z. Phys. Chem. 49, 257–302. doi:10.1515/zpch-1904-4925
Geng, M., and Duan, Z. (2010). Prediction of oxygen solubility in pure water and brines up to high temperatures and pressures. Geochim. Cosmochim. Acta 74, 5631–5640. doi:10.1016/j.gca.2010.06.034
Giffaut, E., Grivé, M., Blanc, P., Vieillard, P., Colàs, E., Gailhanou, H., et al. (2014). Andra thermodynamic database for performance assessment: ThermoChimie. Appl. Geochem. 49, 225–236. doi:10.1016/j.apgeochem.2014.05.007
Glazunov, A. I., Kryazhev, A. M., and Musinsky, S. V. (1997). Растворимость кислорода в растворах гидроокиси натрия (Oxygen solubility in sodium hydroxide solutions). Celljuloza, Bum. karton 7-8, 17–19.
Grenthe, I., Gaona, X., Plyasunov, A. V., Rao, L., Runde, W. H., Grambow, B., et al. (2020). Second update on the chemical thermodynamics of uranium, neptunium, plutonium, americium and technetium. Boulogne-Billancourt, France: OECD Nuclear Energy Agency, Data Bank, 1572.
Gubbins, K. E., and Walker, R. D. (1965). The solubility and diffusivity of oxygen in electrolytic solutions. J. Electrochem. Soc. 112, 469–471. doi:10.1149/1.2423575
Harvie, C. E., Møller, N., and Weare, J. H. (1984). The prediction of mineral solubilities in natural waters: The Na-K-Mg-Ca-H-Cl-SO4-OH-HCO3-CO3-CO2-H2O system to high ionic strengths at 25°C. Geochim. Cosmochim. Acta 48 (4), 723–751. doi:10.1016/0016-7037(84)90098-X
Hayduk, W. (1991). Final Report concerning the solubility of oxygen in sulfuric acid-zinc pressure leaching solutions. Ottawa, Canada: University of Ottawa, Chemical Engineering, 40.
Herbert, H. J. (2000). “Zur Geochemie und geochemischen Modellierung hochsalinarer Lösungen,” in Hannover Bundesanstalt für Geowissenschaften und Rohstoffe (BGR). Editors J. Geologisches, and R. D. Sonderhefte (Stuttgart, Germany: Schweizerbart Science Publishers). ISBN 978-3-510-95845-0.
Iwai, Y., Eya, H., Itoh, Y., Arai, Y., and Takeuchi, K. (1993). Measurement and correlation of solubilities of oxygen in aqueous solutions containing salts and sucrose. Fluid Phase Equilib. 83, 271–278. doi:10.1016/0378-3812(93)87030-5
Iwai, Y., Kohashi, K., Eya, H., Honda, K., and Arai, Y. (1990). Measurement and correlation of oxygen solubilities in aqueous solutions containing salts and sugar. Kagaku Kogaku Ronbun 16, 1247–1251. doi:10.1252/kakoronbunshu.16.1247
Kaskiala, T. (2002). Determination of oxygen solubility in aqueous sulphuric acid media. Min. Eng. 15, 853–857. doi:10.1016/S0892-6875(02)00089-4
Kelley, K. K. (1960). High-temperature heat-content, heat-capacity, and entropy data for the elements and inorganic compounds. U. S. Bureau Mines Bull. 584, 232.
Khomutov, N. E., and Konnik, E. I. (1974). Solubility of oxygen in aqueous electrolyte solutions. Russ. J. Phys. Chem. 48, 21–362. or Zh. Fiz. Khim, 48, (1974), 620–37.
Kitamura, A. (2020). JAEA-TDB-RN in 2020: Update of JAEA’s thermodynamic database for solubility and speciation of radionuclides for performance assessment of geological disposal of high-level and TRU wastes. JAEA-Data/Code, 2020–020. doi:10.11484/jaea-data-code-2020-020
Knaster, M. B., and Apelbaum, L. A. (1964). Растворимость водорода и кислорода в концентрированных растворах едкого кали (Solubility of hydrogen and oxygen in concentrated solutions of potassium hydroxide). Zh. Fiz. Khim. 38 (1), 223–225.
Kulik, D. A., Wanger, T., Dmytrieva, S. V., Kosakowski, G., Hingerl, F. F., Chudneno, K. V., et al. (2013). GEM-selektor geochemical modeling package: Revised algorithm and GEMS3K numerical kernel for coupled simulation codes. Comput. Geosci. 17 (1), 1–24. doi:10.1007/s10596-012-9310-6
Lang, W., and Zander, R. (1986). Salting-out of oxygen from aqueous electrolyte solutions: Prediction and measurement. Ind. Eng. Chem. Fundam. 25, 775–782. doi:10.1021/i100024a050
Li, H. (1994). The solubilities of oxygen in sulphuric acid solutions containing copper and/or nickel sulphates at atmospheric and high pressure. Master thesis (Ottawa, Canada: University of Ottawa), 110.
Lumsdon, D. G., and Evans, L. J. (1995). “Predicting chemical speciation and computer simulation,” in Chemical speciation in the environment (Berlin, Germany: Springer), 86–134.
MacArthur, D. G. (1916). Solubility of oxygen in salt solutions and the hydrates of these salts. J. Phys. Chem. 20, 495–502. doi:10.1021/j150168a003
Mariner, P. (2004). In-drift precipitates/salts model. No. ANL-EBS-MD-000045, REV 02. Las Vegas, Nevada, USA: Yucca Mountain Project.
May, P. M., and Muray, K. (1991). Jess, a joint expert speciation system—II. The thermodynamic database. Talanta 38 (12), 1419–1426. doi:10.1016/0039-9140(91)80290-G
Millero, F. J., Huang, F., and Graham, T. B. (2003). Solubility of oxygen in some 1-1, 2-1, 1-2, and 2-2 electrolytes as a function of concentration at 25°C. J. Solut. Chem. 32, 473–487. doi:10.1023/A:1025301314462
Millero, F. J., Huang, F., and Laferiere, A. L. (2002). Solubility of oxygen in the major sea salts as a function of concentration and temperature. Mar. Chem. 78, 217–230. doi:10.1016/S0304-4203(02)00034-8
Millero, F. J., Huang, F., and Laferiere, A. L. (2002). The solubility of oxygen in the major sea salts and their mixtures at 25°C. Geochim. Cosmochim. Acta 66, 2349–2359. doi:10.1016/S0016-7037(02)00838-4
Millero, F. J., and Huang, F. (2003). Solubility of oxygen in aqueous solutions of KCl, K2SO4, and CaCl2 as a function of concentration and temperature. J. Chem. Eng. Data 48, 1050–1054. doi:10.1021/je034031w
Mitchell, M., Muftakhidinov, B., Winchen, T., van Schaik, B., Wilms, A., Kylesower, , et al. (2020). Engauge digitizer software. Available at: http://markummitchell.github.io/engauge-digitizer (Accessed February 19, 2020). doi:10.5281/zenodo.3558440
Moog, H. C., Bok, F., Marquardt, C. M., and Brendler, V. (2015). Disposal of nuclear waste in host rock formations featuring high-saline solutions - implementation of a thermodynamic reference database (THEREDA). Appl. Geochem. 55, 72–84. doi:10.1016/j.apgeochem.2014.12.016
Narita, E., Lawson, F., and Han, K. H. (1983). Solubility of oxygen in aqueous electrolyte solutions. Hydrometallurgy 10, 21–37. doi:10.1016/0304-386X(83)90074-9
Ohkubo, M. (2000). 製塩工程における高濃度電解質水溶液の溶存酸素濃度測定 (Measurement of solubility of oxygen in electrolyte solution used in salt manufacturing process). Bull. Soc. Sea Water Sci. Jpn. 54 (2), 111–116. doi:10.11457/swsj1965.54.111
Parkhurst, D. L., and Appelo, C. A. J. (2013). Description of input and examples for PHREEQC version 3—a computer program for speciation, batch-reaction, one-dimensional transport, and inverse geochemical calculations. U.S. Geological Survey, Book 6, Chapter 43, Section A of Techniques and Methods, 497.
Pearson, F. J., and Berner, U. R. (1991). Nagra thermochemical data base I. Code data, 80. Nagra, Technical Report 91–17.
Phillips, S. L., Phillips, C. A., and Skeen, J. (1985). Hydrolysis, formation and ionization constants at 25° C, and at high temperature-high ionic strength. Berkeley, CA, USA: Lawrence Berkeley Laboratory, 411. Lawrence Berkeley Laboratory Report No. LBL-14996.
Pierrot, D., and Millero, F. J. (2017). The speciation of metals in natural waters. Aquat. Geochem. 23, 1–20. doi:10.1007/s10498-016-9292-4
Pitzer, K. S. (1991). “Ion interaction approach: Theory and data correlation,” in Activity coefficients in electrolyte solutions. Editor K. S. Pitzer (Boca Raton, Florida: CRC Press), 75–153.
Poeter, E. P., Hill, M. C., Lu, D., Tiedeman, C. R., and Mehl, S. (2014). UCODE_2014, with new capabilities to define parameters unique to predictions, calculate weights using simulated values, estimate parameters with SVD, evaluate uncertainty with MCMC, and More. Report Number: GWMI 2014-02. Indianapolis, Indiana, USA: Integrated Groundwater Modeling Center, 189.
Pospíšol, J., and Lužný, Z. (1960). Absorptionskoeffizienten des Sauerstoffes in Lösungen von Kaliumhydroxyd. Collect. Czech. Chem. Commun. 25, 589–592. doi:10.1135/cccc19600589
Ragoussi, M. E., and Costa, D. (2019). Fundamentals of the NEA Thermochemical Database and its influence over national nuclear programs on the performance assessment of deep geological repositories. J. Environ. Radioact. 196, 225–231. doi:10.1016/j.jenvrad.2017.02.019
Read, D., and Broyd, T. W. (1991). Recent progress in testing chemical equilibrium models: The CHEMVAL project. Radiochim. Acta 52 (2), 453–456. doi:10.1524/ract.1991.5253.2.453
Reynafarje, B., Costa, L. E., and Lehninger, A. L. (1985). O2 solubility in aqueous media determined by a kinetic method. Anal. Biochem. 145, 406–418. doi:10.1016/0003-2697(85)90381-1
Scharge, T., Muñoz, A. G., and Moog, H. C. (2012). Activity coefficients of fission products in highly salinary solutions of Na+, K+, Mg2+, Ca2+, Cl-, and SO42-: Cs+. J. Chem. Eng. Data 57 (6), 1637–1647. doi:10.1021/je200970v
Scharge, T., Munoz, A. G., and Moog, H. C. (2015). Thermodynamic modeling of high salinary phosphate solutions. II. Ternary and higher systems. J. Chem. Thermodyn. 80, 172–183. doi:10.1016/j.jct.2013.12.017
Scharge, T., Munoz, A. G., and Moog, H. C. (2013). Thermodynamic modelling of high salinary phosphate solutions. I. Binary systems. J. Chem. Thermodyn. 64, 249–256. doi:10.1016/j.jct.2013.05.013
Shock, E. L., Helgeson, H. C., and und Sverjensky, D. A. (1989). Calculation of the thermodynamic and transport properties of aqueous species at high pressures and temperatures: Standard partial molal properties of inorganic neutral species. Geochim. Cosmochim. Acta 53, 2157–2183. doi:10.1016/0016-7037(89)90341-4
Shoor, S. K., Walker, R. D., and Gubbins, K. E. (1968). Salting out of nonpolar gases in aqueous potassium hydroxide solutions. J. Phys. Chem. 73 (2), 312–317. doi:10.1021/j100722a006
Söhnel, O., and Novotný, P. (1985). Densities of aqueous solutions of inorganic substances. Amsterdam, Netherlands: Elsevier Publishing Company, 336.
Stephan, E. F., Hatfield, N. S., Peoples, R. S., and Pray, H. A. H. (1956). The solubility of gases in water and in aqueous uranyl salt solutions at elevated temperatures and pressures. Columbus, Ohio: Battelle Memorial Institute. Battelle Memorial Institute Report, BMI-1067.
Tan, Z. Q., Gao, G. H., Yu, Y. X., and Gu, G. (2001). Solubility of oxygen in aqueous sodium carbonate solution at pressures up to 10 MPa. Fluid Phase Equilibria 180, 375–382. doi:10.1016/S0378-3812(01)00371-5
THEREDA (2023). THEREDA – thermodynamic reference database. Available at: https://www.thereda.de/.
Thoenen, T., Hummel, W., Berner, U. R., and Curti, E. (2014). The PSI/nagra chemical thermodynamic database 12/07. PSI Report No. 14-04. ISSN 1019-0643.
Turner, D. R., Achterberg, E. P., Chen, C.-T. A., Clegg, S. L., Hatje, V., Maldonado, M. T., et al. (2016). Toward a quality-controlled and accessible pitzer model for seawater and related systems. Front. Mari. Sci. 3, 1–12. doi:10.3389/fmars.2016.00139
Voigt, W. (2020). Hexary system of oceanic salts – polythermal pitzer dataset (numerical supplement). THEREDA J. 1 (1), 1–9. URN: urn:nbn:de:bsz:105-qucosa2-782361.
Voigt, W. (2020). Temperature extension of NaCl Pitzer coefficients and ∆RG°(NaCl). THEREDA J. 1 (2), 1–6. URN: urn:nbn:de:bsz:105-qucosa2-782374.
Wagner, T., Kulik, D. A., Hingerl, F. F., and Dmytrieva, S. V. (2012). GEM-selektor geochemical modeling package: TSolMod library and data interface for multicomponent phase models. Can. Mineral. 50 (5), 1173–1195. doi:10.3749/canmin.50.5.1173
Wolery, T. (1992). EQ3/6, A software package for the geochemical modelling of aqueous systems. UCRL MA 110662 Part I. Livermore, CA, USA: Lawrence Livermore National Laboratory.
Yasunishi, A. (1977). Solubilities of sparingly soluble gases in aqueous sodium sulfate and sulfite solutions. J. Chem. Eng. Jpn. 10 (2), 89–94. doi:10.1252/jcej.10.89
Yasunishi, A. (1978). Solubility of oxygen in aqueous electrolyte solutions. Kagaku Kogaku Rombunshu 4, 185–189. doi:10.1252/kakoronbunshu.4.185
Zhang, C., Fan, F.-R., and Bard, A. J. (2009). Electrochemistry of oxygen in concentrated NaOH solutions: Solubility, diffusion coefficients, and superoxide formation. J. Am. Chem. Soc. 131, 177–181. doi:10.1021/ja8064254
Zhang, G., Spycher, N., Sonnenthal, E., and Steefel, C. I. (2006). Implementation of a Pitzer activity model into TOUGHREACT for modeling concentrated solutions. TOUGH Symposium 2006, Technical Program and Abstracts, Berkley, May 15–17.
Keywords: oxygen solubility, electrolyte solutions, water, Pitzer ion-interaction approach, EH equation
Citation: Bok F, Moog HC and Brendler V (2023) The solubility of oxygen in water and saline solutions. Front. Nucl. Eng. 2:1158109. doi: 10.3389/fnuen.2023.1158109
Received: 03 February 2023; Accepted: 10 March 2023;
Published: 14 April 2023.
Edited by:
Taishi Kobayashi, Kyoto University, JapanReviewed by:
Tiziana Missana, Centro de Investigaciones Energéticas, Medioambientales y Tecnológicas, SpainCopyright © 2023 Bok, Moog and Brendler. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: F. Bok, Zi5ib2tAaHpkci5kZQ==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.