Depletion attraction in colloidal and bacterial systems

Depletion attraction is a common entropy force observed in colloidal systems. As a common phenomenon in colloidal and bacterial systems, studying the mechanism and application of depletion attraction is of great value for controlling the state of colloidal solutions, regulating the morphology of colloidal particles, disease treatment, and water pollution treatment. Based on the current research status, we briefly introduce the calculation and measurement methods of depletion attractions. And we review the application of depletion attractions in colloidal systems, and summarize the different phenomena and aggregation mechanisms caused by depletion attraction in active colloidal particle-bacterial systems. Understanding the specific role of depletion aggregation in colloidal and bacterial systems provides more possibilities for further exploring depletion aggregation mechanisms and utilizing depletion aggregation phenomena in nature.

1 Introduction

A colloidal dispersion is a complex mixture comprised of particles with dimensions typically ranging from 1 nm to 5 μm that are dispersed in a continuous phase (Nägele, 1989; Russel, et al., 1989; Lee, 2019). Examples of common colloidal dispersions in daily life include paint, milk, and toothpaste. The state of a colloidal system is primarily determined by the interactions that occur between its constituent particles. Within colloids and biological systems, depletion attraction is an important dispersion mechanism that refers to the interaction between particles (e.g., bacteria) and other particles or solid surfaces. Depletion attraction is a phenomenon whereby the presence of certain components in a solution leads to depletion effects when the particles approach one another or come into contact with solid surfaces. This depletion effect causes the particles to be attracted to one another or to the solid surfaces (Goodwin, 2004).

In the context of a stable colloidal system comprised of particles with differing sizes, the second law of thermodynamics and minimization of free energy dictate that larger particles will experience secondary attraction. This is due to the restricted size of particles and the arduous interaction between colloidal particles, which create a circular space similar to the radius of a small ball surrounding the large particles. This space excludes smaller particles and defines the excluded volume of the small ball in relation to the larger particles. When two large particles diffuse to a close proximity and their distances are close enough, the excluded volumes will begin to overlap, thereby providing more room for small particles. If the density of the small balls is sufficiently high, when two large balls are close to one another and generate overlapped excluded volumes, an attractive interaction between the two large balls will ensue. Once the strength of this attraction exceeds k_BT, the two large balls will coalesce and will not be separated by thermal motion. This attraction can lead to instability in the dispersion of large balls, resulting in aggregation or coalescence. The attraction between large balls that is caused by the maximization of system entropy is referred to as depletion attractions, with the small balls being called depletion agents. The depletion interaction is an entropy-induced interaction that is distinct from the four fundamental forces of physics (gravity, electromagnetic force, strong force, and weak force).

In the 1970s, scholars studying the phase behavior of colloidal and polymer mixtures discovered that depletion attraction, previously introduced by Asakura and Oosawa, could explain numerous observations such as lotion droplet flocculation and creaming. As a result, this concept has gained widespread acceptance, leading to further theoretical work aimed at expanding upon their ideas. Investigating the depletion aggregation of colloidal particles holds great significance in controlling colloidal solution stability (Vrij, 1977; Ye et al., 1996; Xing et al., 2012; Lele and Tilton, 2019), regulating the process and product morphology of colloidal synthesis reactions (Kraft et al., 2012; Sacanna et al., 2013; Hueckel et al., 2021), and promoting the self-assembly and morphology regulation of colloidal particles. Bacteria, as active colloidal particles (Wang et al., 2012 Ashton et al., 2013; Du et al., 2020), have important effects on their environment through depletion attractions, impacting their adhesion (Niu et al., 2021), movement (Wilson et al., 2011; Porter et al., 2019), and aggregation (Schwarz-Linek et al., 2010a; Dorken et al., 2012; Sun et al., 2012; Secor et al., 2016; Secor et al., 2018; Cai, 2020; Secor et al., 2021). Therefore, in-depth research on the mechanism and influencing factors of depletion attraction is of paramount importance for further comprehending the physical and physiological properties of colloidal and bacterial systems.

Colloidal and bacterial systems are two distinct systems with unique characteristics, but they both involve interactions between molecules in solution and solid surfaces or between biological macromolecules. This comprehensive review aims to thoroughly summarize and analyze the mechanism, regulation, and application of depletion attraction in both colloidal and bacterial systems. The goal is to provide valuable references for further research in related fields. Additionally, this article will explore current research issues and offer suggestions for future research directions, with the hope of providing guidance and references for future research in this area.

2 How to calculate depletion attraction?

Depletion attraction occurs in stable colloidal systems with distinct populations of two differently sized hard spherical particles in a liquid phase, large particle diameter: “D", small particle diameter: “d.” This entropy-based depletion attraction’s energy depends on particle shapes and small particle volume fraction. Due to finite sizes and non-penetrating interactions, smaller particles can’t enter a spherical region just beyond each large sphere. This defines the boundary of an ‘excluded volume’ of small spheres with respect to a big sphere: “d/2”(small spheres). When large spheres approach and the distance is d/2, overlapped excluded volumes increase to maximum. With the decreasing distance and the two big spheres touch, the minimum potential energy associated with the depletion interaction at contact is: U_dep,min = −(3/2)k_BTφ_d (D/d) (Mao et al., 1995). The well depth in the potential energy between two big spheres arising from the depletion attractions is proportional to the size ratio D/d and the volume fraction of the depletion agent φ_d. Consequently, the depletion attraction forces among the large spheres are linked solely to the size ratio of particles under constant temperature conditions and concentration of depletion agents. It remains unaffected by different materials and different composition. The physical image of depletion attraction is clear, but quantitative theoretical studies are relatively difficult. Currently, most work is based on the results of molecular dynamics simulations. However, since the calculation of depletion attraction and free energy is quite challenging in simulation calculations, the quantitative results currently obtained are not very complete. Constructing an efficient simulation method to calculate depletion attraction and its consequences is a meaningful research topic.

Since Asakura and Oosawa first proposed the theoretical concept of depletion attractions (Asakura and Oosawa, 1954; Asakura and Oosawa, 1958), extensive research has been conducted to investigate their fundamental characteristics in various colloidal systems. Both experimental (Evans and Needham, 1988; Knoben et al., 2007; Yang et al., 2015) and theoretical (Asakura and Oosawa, 1954; Krüger et al., 2011; Wan and Glotzer, 2018) approaches have been employed to explore the relationship between depletion attractions, distance, the concentration of depletion agent, and the geometries of colloidal particles. Computer simulations have played a crucial role in testing the theoretical framework and gaining deeper insight into this phenomenon.

2.1 Computer simulation of depletion attraction

The depletion effect was first predicted theoretically by Sho Asakura and Fumio Oosawa in the 1950s Since then, computer simulations of depletion in various systems have been conducted using molecular dynamics and Monte Carlo methods. Although computer simulations can provide a detailed description of depletion attractions in different systems, the accuracy of the simulation results can depend on the defining model used (Jiménez-Ángeles et al., 2011; Wolters et al., 2017; Wan and Glotzer, 2018; Calero and Pagonabarraga, 2020).

Based on density generalized function theory, Dietrich et al. (Roth et al., 1999) calculated the depletion potential in a small hard sphere system with a volume fraction of η_s and a radius of R_s, and a large hard sphere with a radius of R_b on a curved surface with a radius of R_c. The depletion potential $W_c^{AOa}$ was expressed mathematically as a function of the radius of curvature R_c as follows:

$\beta W_c^{AOa}\left(R_c\right)=-{\eta }_s\left(1+\frac{3R_c\left(R_b/R_s\right)}{R_c+\gamma R_b}\right)(1)$

where β⁻¹ = k_BT, and γ indicated whether the large hard sphere is inside (−1) or outside (+1) of the surface.

As shown in Figure 1A, the solid line indicated the results calculated from the density functional theory and the dashed line corresponds to the A-O approximation. It can be shown from the figure that the calculation results are closer when η_s is smaller. That is, the model description is more accurate when η_s → 0.

FIGURE 1

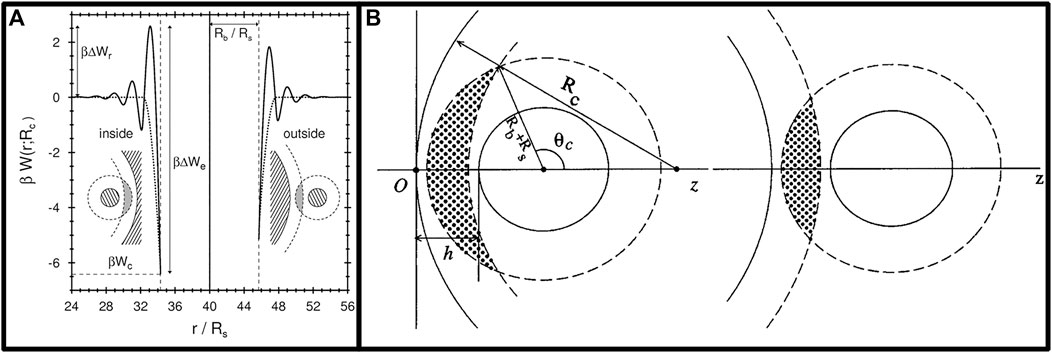
FIGURE 1. (A) Effect of surface curvature on the depletion potential of a single hard sphere (Roth et al., 1999); (B) Schema of overlapped excluded volume by a fixed hard sphere in a fluid of smaller hard spheres located inside (left) and outside (right)of a spherical cavity (Li and Ma, 2002).

Krüger et al. (Krüger et al., 2011) proposed a simple mathematical model that combines A-O theory with Derjaguin’s approximation using differential geometry to evaluate the non-isotropic depletion potential of ellipsoids in terms of the mean and Gaussian curvatures of ellipsoidal particle surfaces. This method yielded an excellent agreement between the theoretical results and the simulated data at low concentrations of depletion agents. In a separate study, Yang et al. (Yang et al., 2015) investigated the depletion attraction of spherical colloidal particles using Monte Carlo simulations in the presence of temperature-sensitive depletion agents on a substrate with a pattern. The simulations produced overlapped excluded volume equations between spherical colloidal particles and between spherical particles and a patterned substrate, allowing for an understanding of how the surface microstructure of the substrate affected the local depletion interactions of spherical particles. Castañeda-Priego et al. (Castañeda-Priego et al., 2006) conducted Monte Carlo simulations to explore the impact of particle shape, system dimensionality, and container surface pattern on depletion attraction. Their findings indicated that the A-O model was not applicable to depletion attractions between two large spheres induced by rod particles as depletion agents, due to the A-O model’s neglect of the contribution of rotation to entropy, which was significant for non-isotropic rod particles. Compared to spherical particles, rod-shaped particles as depletion agents produced stronger and longer-range depletion attraction under similar conditions (Castañeda-Priego et al., 2006). Additionally, some results suggested that interactions between convex regions on the surface of the vessel wall and large particles were weaker, while the attraction between concave regions and large particles was stronger. Figure 1B shows the overlapped excluded volumes between the large particles and the surface in both convex and concave regions, and it could be seen that under the same conditions, the concave vessel wall generated more V_ov with large particles (Li and Ma, 2002). These results have significant implications for the utilization of depletion attractions in colloids.

Through the introduction of several methods for calculating depletion forces, we present images of depletion interactions and general concepts of equivalent interactions, followed by a detailed presentation of several practical methods for calculating hard sphere depletion interactions and various approximate analytical formulas. Due to the scope of this article, the details of calculations and formula derivations are not discussed. For interested readers who wish to delve deeper, a fairly comprehensive list of references is provided for convenience. It is important to note, however, that the references presented here are limited to supporting and supplementing this article, and not a comprehensive list of literature related to colloid depletion interactions.

2.2 Experimental measurements of depletion attraction

In addition to theoretical calculations, direct or indirect experimental measurements of the depletion attraction/potential have been carried out (Evans and Needham, 1988; Lee et al., 1991; Richetti and Kékicheff, 1992; Andrew and Simon, 1995; Ohshima et al., 1997; Rudhardt et al., 1998; Crocker et al., 1999; Piech and Walz, 2002; Knoben et al., 2007; Gong et al., 2011; Kumar et al., 2013). However, possible interactions between large spheres and small spheres, such as van der Waals forces, electrostatic forces, and hydrodynamic effects, hinder the accurate measurement of depletion attractions when two large spheres (plates) are in close proximity. In 1988, Evans and Needham (Evans and Needham, 1988) directly measured the depletion potential by dissolving dextran (molecular weight 10,000–150,000 Da) in water as depletion agents, reducing the distance between two lipid vesicle membranes, and measuring the concentration of dextran in the solution to obtain the adhesion force between two lipid vesicle membranes immersed in it, calculated as:

$∆F=A{\int }_0^{Z_g}\left({\mathit{\Pi }}_B-\mathit{\Pi }\right)dz(2)$

where A is the surface area of the vesicle, Z_g is the distance of between two lipid vesicle membranes. Π is the osmotic pressure of the solution, and Π_B is the osmotic pressure in the gap between the vesicles. The magnitude of the adhesion force is the magnitude of the depletion attraction.

Subsequently, a variety of measurement methods have been used, including atomic force microscope (AFM) (Andrew and Simon, 1995; Piech and Walz, 2002; Knoben et al., 2007), the laser radiation method (Ohshima et al., 1997; Crocker et al., 1999), the total internal reflection microscopy (TIRM) method (Higurashi et al., 1998; Gong et al., 2011), neutron diffraction method (Lee et al., 1991; Kumar et al., 2013). Experimental measurements of sphere-to-sphere and sphere-to-wall depletion attractions have been conducted. AFM can be used to directly measure the depletion attraction between a large sphere and a surface. The method entails immersing a probe with a colloidal sphere in the solution of depletion agents, scanning the substrate surface continuously, and monitoring the deflection of the cantilever with a split photodiode detector and a total reflection laser beam. The curve of the depletion attraction with distance can be obtained directly since the spring constant of the cantilever is known. Figure 2A shows a schematic illustration of the principle of AFM measurement.

FIGURE 2

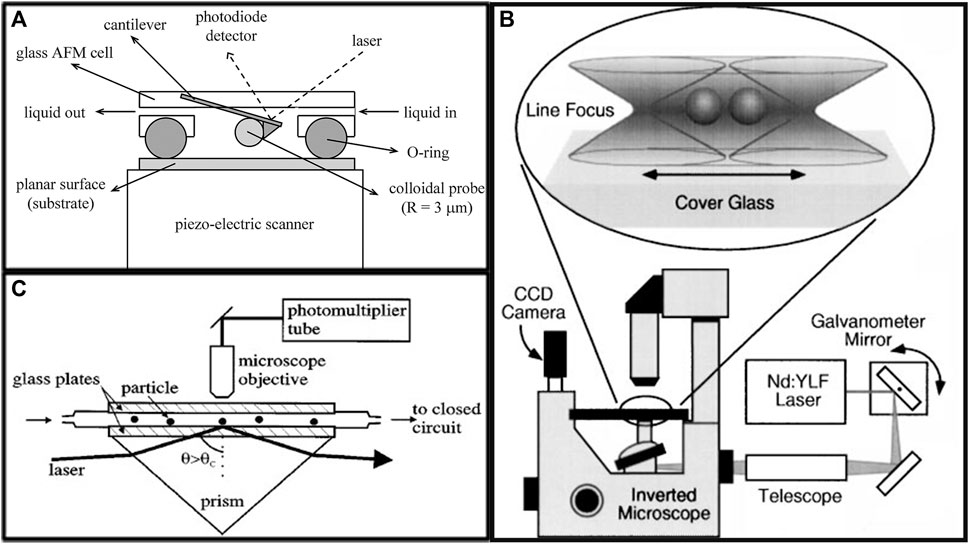
FIGURE 2. Schematic illustration of (A) the use of atomic force microscope (AFM) to measure the depletion attraction between a colloidal particle and a flat plane (Knoben et al., 2007); (B) the use of laser tweezers to measure the depletion attraction between two colloidal particles (Crocker et al., 1999); (C) the use of total internal reflection microscopy (TIRM) to measure the depletion attraction between a colloidal particle and a flat plane (Rudhardt et al., 1998).

Utilizing focused lasers, it was possible to construct optical traps in which minuscule particles were confined through the pressure exerted by the laser (Higurashi et al., 1998; Wilking and Mason, 2008). Ohshima et al. (Ohshima et al., 1997) introduced polystyrene (PS) microspheres possessing a diameter of 1.0 µm into a polyethylene oxide (PEO) solution containing spheres with a radius of 0.101 µm. The PS spheres experienced depletion attractions while approaching the surface of the cuvette and ultimately adhered to it. Subsequently, a focused laser beam was employed to manipulate the PS spheres, with the laser’s intensity gradually increased until the point at which it could displace the PS sphere, at which point the laser’s power was equal to the depletion attraction potential of the PS spheres and the cuvette. Crocker et al. (Crocker et al., 1999) employed poly (methyl methacrylate) (PMMA) particles with a diameter of 1,100 ± 15 nm as their larger spheres and PS particles with a diameter of 83 nm as their depletion agents. A moderate amount of sodium dodecyl sulfate (SDS) and salt were added to the solution to prevent the larger PMMA spheres from aggregating due to van der Waals attraction. Two distinct optical tweezers beams were focused on the two larger spheres, and the depletion attraction between them was directly measured (Osterman, 2010), the main schematic of the experiment is shown in Figure 2B. Consequently, a series of similar works were successively initiated (Perry et al., 2012; Julie et al., 2014; Zosel et al., 2020). Utilizing the energy harnessed from optical tweezers, the researchers immobilized the spherical colloids within the solution matrix and measured the potential of mean force between two large colloidal particles. These results paved the way for a systematic examination of the intricate relation between depletion forces and the spatial separation distance among the large spheres. By calculating the dynamics of the large spheres, assistances were provided in elucidating the nucleation mechanism influenced by depletion attraction, the stability of the solution and the conformational issues of biomacromolecules in crowded environments. Similarly, there is the work of Amano et al. (Amano et al., 2016), who directly measured the depletion attraction potential (Φ_LL) between two large colloidal particles using linear optical tweezers, and then proposed and tested a theory that translates Φ_LL into the number density distribution (g_LS) of small particles around large particles. Their theory would contribute to the structural analysis of colloidal suspensions, which was important for both understanding colloidal properties and developing colloidal products. A recent work by Liu et al. (Liu et al., 2019) captured two silica particles in a point-like optical trap and a linear optical trap using dual-optical tweezers technology, and by controlling the linear trap brought one particle close enough to the other. By modulating the concentration of the ionic surfactant sodium dodecyl benzene sulfonate (SDBS), and concurrently regulating the spatial separation distance, the depletion attraction between the two particles could be measured as the separation distance varies.

AFM and laser radiation represent interventional measurements that unavoidably influence the thermal motion of colloidal spheres. The magnitude of the depletion attraction potential is equivalent to the thermal motion energy ∼ k_BT (Gong et al., 2011), thereby making it effortless for the thermal motion to interfere with the depletion potential measurement. In contrast, total internal reflection microscopy (TIRM) serves as a non-interfering method in essence gauge the inter-surface interactions with the thermal energy k_BT and thus very sensitive to weak forces. The potential measurements are not reliant on laser intensity, which eliminate the impact of light forces. When light is reflected at a solid/fluid interface above the angle of total internal reflection θc (Figure 2C), an evanescent wave is formed which leaks into the fluid. Briefly, in a TIRM measurement, an evanescent wave is engendered at the interface between the solid material and the liquid medium through the mechanism of total internal reflection within the glass substrate. This evanescent wave exhibits an exponential decay pattern relative to the distance from the interface with a characteristic decay length β⁻¹ which depends on the incident angle θ, the wavelength of the light beam, and the refractive indices at the interface. When an object which scatters light, e.g., a colloidal sphere, approaches the surface close enough to enter the evanescent field frustrated total reflection will occur. The scattering intensity of a colloidal sphere I is related to the distance z between the colloidal sphere and the surface; it can be written as I ∝ e^−βz. Measuring the scattered intensity over a long period provides a histogram of the separation distance, the probability p(z) of where the colloidal sphere stays are obtained. Using the Boltzmann distribution function p(z) = e^{−[Φ(z)/ k}_B^T], the potential of depletion attraction Φ(z) between the ball wall can be obtained (Gong et al., 2011). A schematic representation of the principle is illustrated in Figure 2C.

In addition to the above methods, small-angle neutron scattering (SANS) could also be used to measure the depletion attraction in solution (Kumar et al., 2013; Ray and Aswal, 2014; Yadav et al., 2014). Kumar et al. (Kumar et al., 2013) used nano-sized silica dispersed in a solution of 0.1 M NaCl, adding poly(ethylene oxide-poly(propylene oxide-poly(ethylene oxide) triblock copolymers as depletion agents. The depletion attraction caused the aggregation of nanoparticles. The differential coherent scattering intensity per unit volume cross section of silica particles was measured by SANS. It can be determined the magnitude of the potential of depletion attraction.

In the aforementioned experimental measurement systems, the colloidal particles suspended in a polymer matrix provide a model system for studying the behavior of depletion attractions. Measuring depletion forces not only helps in understanding the phase behavior of colloidal particle-polymer systems, but also finds applications and attention in specialized functional material design such as targeted drug delivery and diagnosis, where some colloidal particles have demonstrated the practicality of protein sensor arrays. Many of these applications require an understanding of the interaction parameters in these complex systems. Despite interdisciplinary intersections, a comprehension of the depletion attraction forces and their complex interactions among the microstructure are still necessary.

3 Depletion attraction in colloidal systems

With a constantly growing and diverse library of colloidal materials and an array of intelligent self-assembly methods employing depletion attraction, novel functional materials with tailored properties are being developed. These materials exhibit unique functionalities that cannot be attained without the presence of depletion attractions. The magnitude of depletion attraction within the system can be precisely regulated by manipulating the concentration, size, and shape of depletion agents. Furthermore, the relative strength of the attraction at different locations can be controlled by adjusting the local overlapped excluded volumes through specific surface shapes, microstructures, and roughness. These characteristics of depletion attraction are commonly used in theoretical research (Roth et al., 2002; Castañeda-Priego et al., 2006; König et al., 2008; Jiménez-Ángeles et al., 2011; Ye et al., 2013; Banerjee et al., 2015; Padovani et al., 2015) and applied research (Abbas and Lodge, 2007; Kim D. et al., 2019; Zhao et al., 2019; Dekker et al., 2020; Saez Cabezas et al., 2020). In this section, we will provide a brief survey of some common applications of self-assembled depletion attractions, emphasizing their anisotropic structures.

3.1 Phase separation induced by depletion attraction

When the size of colloidal particles in a dispersion is significantly different, the smaller particles can act as depletion agents, leading to depletion attractions between the larger particles. If these depletion attractions are strong enough, phase separation can occur as demonstrated by several studies (De Hek and Vrij, 1981; Pusey and van Megen, 1986; Yasrebi et al., 1991; Bartlett et al., 1992; Sanyal et al., 1992; Calderon et al., 1993; Duijneveldt et al., 1993; Kaplan et al., 1994; Buitenhuis et al., 1995; Ilett et al., 1995; Imhof and Dhont, 1995; Steiner et al., 1995; Herzfeld, 1996). The effect of overlapped excluded volume has been studied in colloidal solutions for a long time (Hobbie, 1998; Hobbie, 1999; Abbas et al., 2009; Hu et al., 2013; Ray et al., 2015). It has been successfully applied to the separation and purification of substances. For example, the separation of carbon nanotubes and graphene oxide (Padovani et al., 2015), and the separation of “isotopic” particles from colloidal particles (Perry and Manoharan, 2016).

Bibette developed a method for the purification of polydisperse emulsions into monodisperse emulsions using depletion attraction (Bibette, 1991). The addition of SDS above the critical micelle concentration (CMC) to a silicone oil micro-drop emulsion stabilized with surfactant SDS caused droplet flocculation when the SDS micelles act as depletion agents. Since the overlapped depletion volume (V_ov) between large droplets was larger, flocculation was more likely. The volume fraction of the cream formed in the solution was high, and the larger droplets were the primary component while the smaller droplets remained in the solution for further separation. The cream was removed, redispersed in SDS, and a slightly lower SDS concentration was added. The newly flocculated cream was removed again, and the process was repeated to obtain large monodispersed droplets. By adding more SDS to the emulsion after the first removal of the cream, the remaining monodisperse droplets could be obtained by continuously removing the cream (Bibette, 1991).

The shape of larger particles affects V_ov and the magnitude of depletion attraction. Mason revealed the relationship between the shape of large particles and the depletion attraction and used these differences of depletion attractions to separate particles of different shapes (Mason, 2002). For example, spheres could be successfully separated from disks by exploiting the significant differences in the depletion attraction between disk-disk, disk-sphere and sphere-sphere configurations (Figure 3A). Compared with the configuration of the sphere-sphere, there were larger V_ov in rod-sphere system. Phase separation in the rod-sphere system was experimentally investigated by Koenderink et al. (Koenderink et al., 1999). By mixing smaller silica rods with larger silica microspheres, phase separation of the spheres occurred under the effect of depletion attraction from the rods (Figure 3B). In agreement with theoretical predictions, this phase separation could occur at very low concentrations of rods and spheres, which confirmed the theoretical model of A-O that depletion agents of the rods resulted in phase separation faster and better compared to the depletion agents of spheres. In nonactive colloidal systems with Brownian motion, phase separation will occur when the thermal disturbance (∼k_BT) is unable to overcome the binding of the aggregates by the depletion attraction. Therefore, in the system of active matter, efficient depletion attraction is required to facilitate phase separation.

FIGURE 3

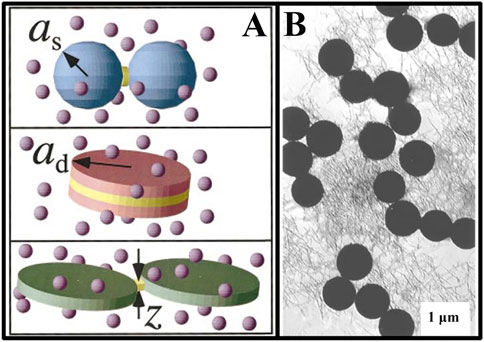
FIGURE 3. (A) Schematic of overlapped excluded volume formed for differently shaped particles (Mason, 2002); (B) Transmission electron micrograph of phase separation in a mixture of silica spheres and silica rods (Koenderink et al., 1999).

3.2 Preparation of colloidal crystals by depletion attraction

Colloidal crystals are structures that are arranged in periodically ordered by colloidal particles, which can be used for the modulation of light and have promising applications in the development of new optical devices, enhanced anti-counterfeiting technology, information storage, sensors and other fields. Depletion attraction has been extensively used in the preparation of colloidal crystals.

Colloidal crystals that are patterned at specific locations can further broaden their applications. Dinsmore et al. (Dinsmore et al., 1996) etched microscopic steps on the container wall added smaller particles as depletion agents and successfully achieved preferential aggregation of colloidal particles at the step edges by exploiting the larger V_ov between the step edges and the colloidal particles. Similarly, using the differences in shape between different parts of the cell wall, it was possible to preferentially form aggregates at specified locations, e.g., V_ov (for large particles in a corner) > V_ov (for its surface on the vessel) > V_ov (between two spherical particles) in the presence of depletion agents, as shown in Figure 4A. Dinsmore et al. (Dinsmore and Yodh, 1999) succeeded in causing large spheres to preferentially form crystal nuclei in the corners of the vessel and grew along them to become crystals using this principle. Subsequently, nucleation and crystallization also occurred on the vessel walls. Ordered microstructures could trap or induce aggregation of particles at specific locations, thus forming specific microstructures that could aid in the preparation of highly ordered microarray structures. Du et al. (Du et al., 2020) successfully achieved the controlled deposition of monolayer colloidal particles on substrates using depletion attractions, combined with patterned substrates.

FIGURE 4

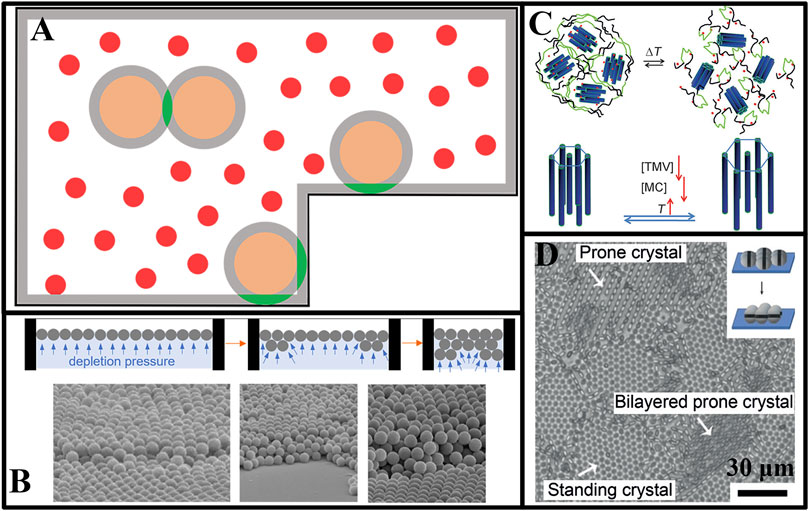
FIGURE 4. (A) Schematic of overlapped excluded volumes of particles at different locations of the container; (B) Silica microspheres self-assemble into multilayer ordered structures at the air-water interface (Kim K. et al., 2019); (C) Schematic illustration showing the superlattices of TMV self-assembled in response to temperature and concentration (Li et al., 2013b); (D) Prone crystals and standing crystals self-assembled by θ-shape SiO₂ particles (Wang L. et al., 2019).

The fabrication of large-area multilayer crystals presents a challenging task due to the tendency of wrinkles and defects in one layer to affect particle alignment in subsequent layers, especially when compared to single-layer crystallization. To overcome this challenge, Kim et al. (Kim K. et al., 2019) prepared colloidal assemblies with multilayer crystal structures at the air-water interface using depletion attractions. As shown in Figure 4B, polyvinyl alcohol (PEG) was added to the aqueous dispersion of silica colloidal microspheres as depletion agents. The depletion attraction generated by PEG in the solution caused the silica microspheres to aggregate into a single-crystal film at the gas-liquid interface, which continuously reduced the distance between the mica flakes at both ends, and the single film was transformed into a multilayer structure. The thickness of the multilayer structure was related to the concentration of PEG and could be simply regulated by the concentration of PEG. This experimental phenomenon could be explained by the classical theory of elastic films at fluid-fluid interfaces, providing a theoretical basis for the fabrication of stable three-dimensional crystals and experimental support for understanding the role of colloidal particles at the interface.

Rod-shaped particles, unlike spherical ones, have the potential to form diverse crystal structures. Baranov et al. (Baranov et al., 2010) assembled nanorods into a two-dimensional hexagonal rotator crystal structure in organic solvents using depletion attractions. This ordered structure could be transferred from the solution to the substrate, yielding a monolayer of vertically standing rod-like particles stacked in a single crystal. Using the similar method, they then prepared three-dimensional crystals in the shape of octopod/tetrapod (Zanella et al., 2011). Dogic and co-workers have explored the structure of the rod-shaped fd virus assembled using glycolic anhydride (Dextran) as depletion agents (Barry and Dogic, 2010). It was found that when the concentration of depletion agents was high, due to the direction dependence of depletion interaction, the single-layer disks assembled by fd virus were superimposed to form smectic filaments. As the concentration of depletion agents decreased, the disks were no longer stacked on top of each other but instead combine laterally to form a single layer of film with a diameter of hundreds of microns. Li et al. have investigated the structure of rod-shaped active matter assemblied by depletion agents in the solution consisting of rods of tobacco mosaic virus (TMV) and methylcellulose (MC) (Li et al., 2013a; Li et al., 2013b). The results showed that TMV was able to form a super-lattice by MC induced depletion attraction, and that the lattice spacing was responsive to temperature and TMV concentration (Figure 4C). This lattice spacing was controllable over a wide range, up to five times the TMV diameter due to the use of depletion attractions.

In addition to rod-like particles, ellipsoidal particles with different chemical properties of the surface can also be assembled in richer structures of colloidal crystals by depletion attractions. Wang et al. (Wang L. et al., 2019) synthesized columnar spherical silica particles with a PS girdle in the middle, as shown in Figure 4D, which have theta-shaped surface chemistry. When situated on a quasi-two-dimensional plane, these particles could form two types of ordered structures through depletion attraction. The first was a steady-state “standing” crystal phase that was predominantly governed by the depletion attraction between the particle and the substrate, while the second was a sub-steady “prone” crystal phase dominated by depletion attractions between particles. When the relative strength of the depletion attraction between the particle and the substrate was adjusted, the two crystal phases could be converted into each other. Liu and co-workers have synthesized ellipsoidal colloidal particles with polystyrene-poly(3 (isobutenyloxy)propyltrimethoxysilyl)-polystyrene (PS-TPM-PS) (Liu et al., 2020). By modifying the properties of their surface chemistry, the colloidal particles bonded to specific parts of each other under the combined effect of depletion attractions and electrostatic repulsion, forming colloidal crystals with special structures such as square lattices and brick-wall type lattices. In addition, the study has explored the assembly of binary mixed colloidal systems of different shapes into crystalline alloys by depletion attractions (Young et al., 2012; Ye et al., 2013; Karas et al., 2016; Brown et al., 2018; Xie et al., 2018; Sung et al., 2019; Li et al., 2020; van Ravensteijn et al., 2020; Liu H. et al., 2021).

3.3 Particles’ roughness controlled depletion attractions

The magnitude of the depletion attraction depends not only on the shape of the large particles in the system, but also on the surface roughness of the large particles (and the vessel walls) (Zhao and Mason, 2008a; Zhao and Mason, 2008b). Figure 5A illustrates the basic principle. For instance, non-spherical and plate-like particles produced through photolithography technology have rough surfaces. If the size of the depletion agent was smaller than the surface roughness of these particles, then there would be a relatively small concentration difference between the depletion agent inside and outside two particles. Consequently, this results in weak depletion attraction that was insufficient to cause particle aggregation. Conversely, if the size of the depletion agent exceeded that of surface roughness on these particles, then there would be a significant concentration difference between its interior and exterior parts leading to strong depletion attraction causing particle aggregation. Therefore, by adjusting the ratio between surface roughness and size of depletion agents we could regulate their strength of attraction. By exploiting the surface roughness of the larger particles, it was feasible to create colloidal assemblies with distinct structures.

FIGURE 5

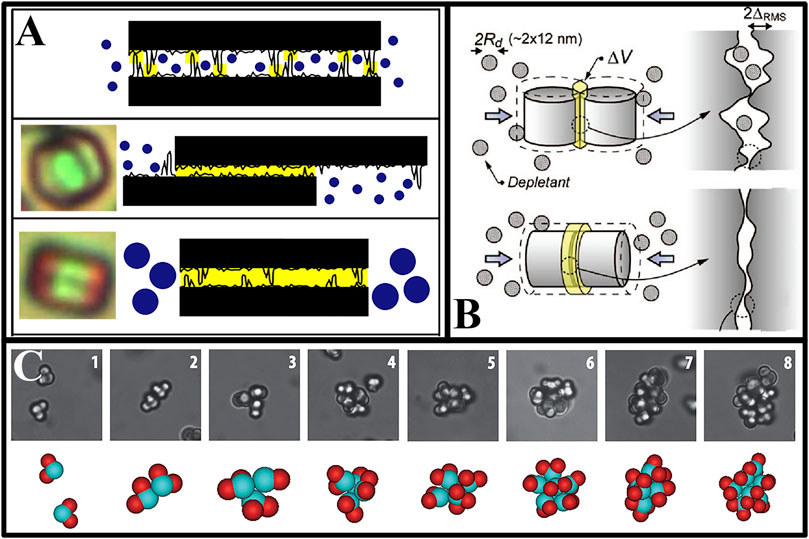
FIGURE 5. Roughness-controlled depletion attractions. (A) Surface asperities influence the excluded volume (Zhao and Mason, 2008b); (B) The excluded volume of side-side and face-face of two cylinders in the presence of depletion agents (Badaire et al., 2008); (C) Complex aggregates of “patched particles” self-assembled by depletion attractions (Wolters et al., 2015).

Zhao and Mason experimentally demonstrated the effect of surface roughness on the depletion attraction of large particles (Zhao and Mason, 2008a; Zhao and Mason, 2008b). They used roughness controlled depletion attractions (RCDAs) to assemble particles prepared by photolithography. The operation steps were as follows: Firstly, the non-spherical colloidal particle system was mixed with the depletion agent, and the non-spherical colloidal particles were confined to the bottom surface of the container by the attractive force of the depletion agent. Then, by tilting the sample, the non-spherical colloidal particles were concentrated by the force of gravity. As the roughness of the upper and lower surfaces of the particles was much smaller than that of the side surfaces, and the size of the depletion agent selected was between these two roughness values, the particles could be adsorbed on the bottom surface of the container by the attractive force of the depletion agent to form a monolayer two-dimensional colloidal system. If the thickness of the particles was not considered, the non-spherical colloidal system constructed by this method was a true two-dimensional colloidal system, and the interaction between the particles in the system was similar to that of hard spheres. Therefore, this system provided a very good research platform for studying some basic thermodynamic problems, such as how shape affects the colloidal thermodynamic properties.

They prepared pentagonal platelets and successfully attracted the two smooth surfaces of the two pentagonal platelets to each other, resulting in a dimer by RCADs, as shown in Figure 5A. To better understand how surface roughness and sub-particle characteristics affected depletion attractions, Zhao and Mason (Zhao and Mason, 2008a) conducted a simulation using platelets with controlled prolate hemi-spheroidal asperities on their faces. They found that taller asperities in the distribution of asperity heights had a significant impact on RCDAs, and that the specific type of lateral distribution and concentration of asperities also affected RCDAs. The simulation also showed that lock-and-key arrangements of mating surface asperities could enhance depletion attractions rather than suppress it. This suggested that precise control over the geometrical features on colloidal building blocks’ surfaces could either suppress or enhance depletion attractions depending on these features’ exact nature. This simulation work (Zhao and Mason, 2008b) confirmed the theoretical concept introduced earlier by Zhao and Mason that shape complementary lock-and-key colloids could increase attractive interactions between portions of their surfaces with complementary geometrical features (Zhao and Mason, 2008a), including positive and negative relief features (protrusions and indentations).

Zhao et al. successfully formed a series of two-dimensional assembled structures using differently shaped platelet colloidal particles selected depletion agents with smaller sizes than the roughness of the sides of the lithographed particles by RCDAs. The interactions between these colloidal particles were hard, and the depletion attraction between the colloidal particles and the substrate could form a better two-dimensional assembly structure. Guided by the RCDAs, triangle, square, rhombic and kite-shaped colloidal platelets were assembled to form rich liquid crystal, crystalline and glass on the plane (Zhao and Mason, 2009; Zhao et al., 2011; Zhao et al., 2012; Zhao and Mason, 2012; Zhao and Mason, 2015; Hou et al., 2019; Liu M. et al., 2021; Liu et al., 2022). The study of the assembly model had been of immense help in advancing the theory of condensed matter physics (Zhao et al., 2012; Zhao and Mason, 2012; Zhao and Mason, 2015). Badaire et al. (Badaire et al., 2008) have prepared cylindrical colloidal particles using photolithography. Similarly, using the different roughness of the upper and lower surfaces of the particles compared to the sides, choosing the right-sized particles as depletion agents could cause the upper and lower surfaces of the particles to attract each other to form chain-like assemblies (Figure 5B). Roughness-controlled depletion attractions could also be used for the self-assembly of colloidal particles on hard curved surfaces (Liu et al., 2022). Thereby providing experimental supported for the development of the theory and applications of curved surface self-assembly. Besides photolithography, colloidal particles with varying surface roughness could also be synthesized chemically. Kraft et al. (Kraft et al., 2012) synthesized PS-dimer particles with one smooth end and one rough end. When depletion agents of the right size were added to the dispersion solution, the smooth surfaces of particles were subjected to a strong depletion attraction, creating a directional attraction between each other. In contrast, the rough surfaces between particles and between rough and smooth surfaces of particles have no depletion attractions, resulting in a specially assembled structure. Similarly, they also synthesized colloidal particles in the shape of “Mickey Mouse,” two rough “ears” set at 90° to a smooth “head.” Particles could form more diverse assemblies structures in the presence of appropriate sized depletion agents (Wolters et al., 2015) (Figure 5C).

Apart from the applications mentioned above, depletion attraction could also be used to prepare micelles (Abbas et al., 2009; Lin et al., 2010; Repollet-Pedrosa and Mahanthappa, 2013; Yang et al., 2019), Pickering emulsions (Kim et al., 2017), and limit crystal growth (Ishibe et al., 2019), among others, but we will not list them all here. Depletion attraction has attracted widespread attention and research since its theoretical proposal in the 1950s, and it has been less than a century since then. With the emergence of numerous colloidal particle preparation techniques, the library of colloidal particle shapes that we have mastered continues to expand. We anticipate utilizing depletion attraction to assemble even more structures, which will undoubtedly drive its application in colloidal systems. From a fundamental research perspective, one of the future research priorities is still the self-assembly of colloidal particles using depletion attractions. Through a combination of experiments and computer simulations, we can study the different assembly structures of colloidal particles under depletion attraction, attempt to summarize general principles, and ultimately answer the fundamental question of the role of depletion attraction in the self-assembly of colloidal particles. Another research hotspot is in interdisciplinary areas, especially in the direction of biology, such as simulating collective behavior of bacterial aggregation. From an applied research perspective, applied research has been relatively lacking over the past few decades. Therefore, in the next 10–20 years, applied research needs to be strengthened, and better guidance of basic research directions through practical needs. From a technical perspective, utilizing depletion attraction to prepare new functional materials is a future development direction, especially in the fields of environment, energy, and communication control. Examples include new energy storage materials for electric vehicles, new photonic bandgap materials for controllable light transmission, and smart materials capable of sensing changes in the environment, all of which can help to address increasingly pressing issues related to people’s production and life, such as environmental pollution and information security.

4 Depletion attraction in bacterial systems

It is now widely accepted that bacteria use two main modes of life: planktonic individuals, or surface-attached biofilms. However, in recent years, medical microbiologists have begun to emphasize that suspended bacterial aggregates are a major form of bacterial community at sites of chronic infection (Bjarnsholt et al., 2009; Bjarnsholt et al., 2013; Secor et al., 2018). Although sharing many similarities with surface-attached biofilms and therefore often defined as biofilm-like aggregates, these non-attached cell clumps show smaller sizes and different formation mechanisms in vivo. The term “aggregates” is used to distinguish between suspended clusters and surface-attached biofilms, which show some degree of similarity, such as antibiotic resistance, but also considerable differences in their phenotypes and regulatory mechanisms in vivo (Cai, 2020). As shown in Figures 6A, B (Schwarz-Linek et al., 2010b; Secor et al., 2018), in a polymer rich environment, aggregates have been proven to form through an increase in entropy force (consumption of aggregates). The formation of bacterial aggregates has the same mechanism as the depletion interactions of colloidal particles, but it has unique biological significance and research value. It is of great significance in the study of antibiotic resistance mechanisms of pathogenic microorganisms, bacterial sedimentation in biotechnology, and water pollution management (Secor et al., 2018; Cai, 2020).

FIGURE 6

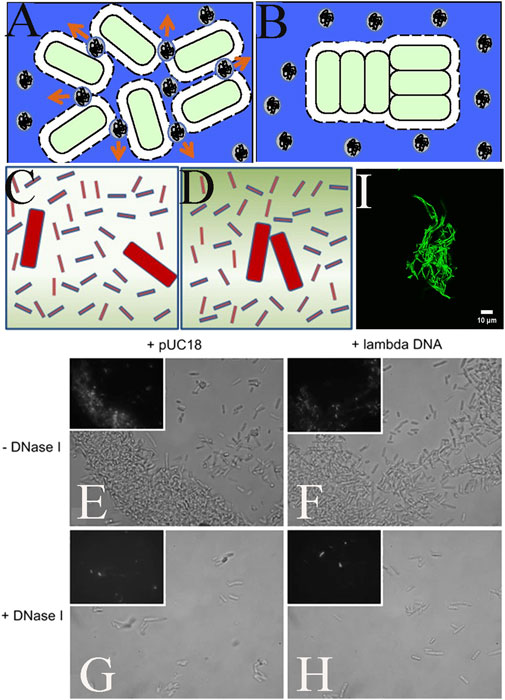
FIGURE 6. Bacterial depletion aggregation caused by different depletion agents. (A) When 2 cells approach each other, the polymer spontaneously moves out and away from the exclusion volume (arrow); (B) When excluding volume overlap, the available volume of the polymer increases, maximizing the system entropy (Secor et al., 2018); (C, D) Consumption effect diagram in a mixture of two large bacteria and small rod-shaped cellulose nanocrystals (Sun et al., 2012); (E–H) DNA in Escherichia coli aggregation body, DNA is labeled with YOYO-1; (G, H) Escherichia coli aggregates treated with DNase I (Liu et al., 2008); (I) Bacillus subtilis cell clusters in MSgg culture, with a concentration of 20 kDa PEG and a concentration of 40 mg/mL (Lobo-Cabrera et al., 2021).

4.1 Bacterial aggregate caused by different depletion agents

The utilization of depletion agents for bacterial aggregate formation is widespread. Currently, they mainly include biological substances (e.g., succinose, alginate, non-adsorbent EPS, Mucin, DNA, Phage, F-actin, Hyaluronan) (Liu et al., 2008; Ghosh et al., 2015; Secor et al., 2018; Secor et al., 2021), chemical polymers (e.g., PEG, sodium polystyrene sulfonate, cellulose nanocrystals) (Eboigbodin et al., 2006; Schwarz-Linek et al., 2010a; Sun et al., 2012). Researchers have studied the particular mechanisms and physiological significance of depletion aggregates utilizing different agents. The type, shape, molecular weight, concentration, hydrophilicity, and salt responsiveness of the polymers impacted the aggregation state and morphology of bacterial aggregates. Sun et al. discovered that rod-shaped nanoparticles were highly effective in depleting flocculation of colloidal-sized bacteria and that phase separation of bacteria could occur in very low concentrations of rod-shaped CNC particles (Figures 6C, D) (Sun et al., 2012). Secor used different mutants of Pseudomonas aeruginosa by employing various depletion agents and producing phase diagrams. It was discovered that high molecular weight DNA had a stronger depletion attraction than low molecular weight DNA, and the mixture of DNA and mucin as depletion agents usually required lower concentrations to allow bacterial aggregation than when DNA alone was the depletion agent. Furthermore, the addition of DNA enzymes to degrade DNA, when DNA was the depletion agent, could cause the depletion aggregation of bacteria to disappear (Figures 6E–H) (Liu et al., 2008; Secor et al., 2018). Lobo-Cabrera found that larger polymer sizes or concentrations led to the development of interesting striated and dendritic colonies, as shown in Figure 6I. The analysis of the results of Brownian kinetic simulations showed the existence of a threshold polymer concentration that distinguished between the two growth mechanisms. Below this threshold, the main force driving polymer-induced compaction was hindering the spread of bacterial cells, while collective effects played a smaller role. Above this threshold, especially for large polymers, polymer-induced compaction was a collective phenomenon driven by depletion forces. Well beyond this concentration threshold, severely limited diffusion drived the formation of filamentous and dendritic colonies (Lobo-Cabrera et al., 2021). Additionally, the introduction of dispersants could also lead to changes in the viscoelasticity of bacterial cultures. Wang et al. added polyethylene glycol (PEG) with different molecular weights and concentrations to two types of Bacillus subtilis cell cultures and observed the cell clusters in cell suspensions. It was found that the viscoelastic changes in cell cultures were the result of depletion interactions and shear force competition. When depletion interactions dominated, cells tended to aggregate more, which led to an increase in viscosity; when shear forces were greater than stripping forces, this leads to dispersion of cell clusters and reduction in viscosity (Wang X. et al., 2019).

4.2 Different bacteria form different depletion aggregates

In addition to bacterial depletion aggregates being influenced by depletion agents, the different properties of bacteria can also affect the formation of aggregates, such as bacterial shape, surface properties, bacterial products and motility, and bacterial growth stage (Hayashi et al., 2003; Dorken et al., 2012; Secor et al., 2016; Ebrahimi et al., 2019; Azimi et al., 2021). Secor found that cells overexpressing the extracellular polysaccharides Pel and Psl maintained aggregation after a short period of exhaustive aggregation, whereas wild-type and mucinous P. aeruginosa did not aggregate. It was demonstrated that after bacteria form aggregates by depletion mechanism, intercellular interactions could be promoted to further form irreversible aggregates (Secor et al., 2021). Dorken et al. found that succinose secreted by Sinorhizobium meliloti promoted the formation of evacuated aggregates, as shown in Figure 7A. A mutant that overproduced the extracellular polysaccharide succinoglycan exhibited enhanced aggregation, resulting in phase separation of the culture. Applying purified succinoglycans from cultures to different concentrations of cells, the same “crowding mechanism” occurred, causing cells to form laterally arranged clusters (Dorken et al., 2012). Azimi et al. found that changes in the O-antigen glycans covering the LPS resulted in different types of aggregate assembly by altering the physicochemical properties of the bacterial cell surface (Figure 7D). In contrast to the lateral aggregates formed by wild-type PAO1, they found that loss of the O-specific antigen (OSA) and alteration of the lipopolysaccharide (LPS) core glycoforms resulted in increased cell surface hydrophobicity that overcame the entropic forces exerted by the host polymer, leading to disordered, irreversible aggregation (Azimi et al., 2021). Secor discussed the implications of the accumulation of filamentous Pf phages produced by P. aeruginosa in the biofilm matrix, as shown in Figure 7B. They interacted with matrix polymers to organize the biofilm into a highly ordered liquid crystal. This structural structure promoted bacterial adhesion, dry survival and antibiotic resistance-all features associated with biofilms (Secor et al., 2016). Eboigbodin used 0.5 to 2.5 wt% of Escherichia coli AB1157 harvested at different growth stages, adding different concentrations of non-adsorbent polymers sodium polystyrene sulfonate (SPS) for depletion aggregation studies. E. coli AB1157 was negatively charged and the cell surface properties changed at different growth stages (Eboigbodin et al., 2006). The cell surface charge was closely related to the non-adsorbent polymer concentration induced depletion aggregation. The differences in bacterial aggregation properties were due to changes in the amount of charge during bacterial growth (Hayashi et al., 2003). Meanwhile, Secor found different effects of depletion aggregation on the interaction of bacillus P. aeruginosa and spherical Staphylococcus aureus in co-culture of two different shapes of bacteria (Figure 7C). As each shape formed different communities, S. aureus enhanced its resistance to P. aeruginosa virulence factors through the formation of aggregates (Secor et al., 2021).

FIGURE 7

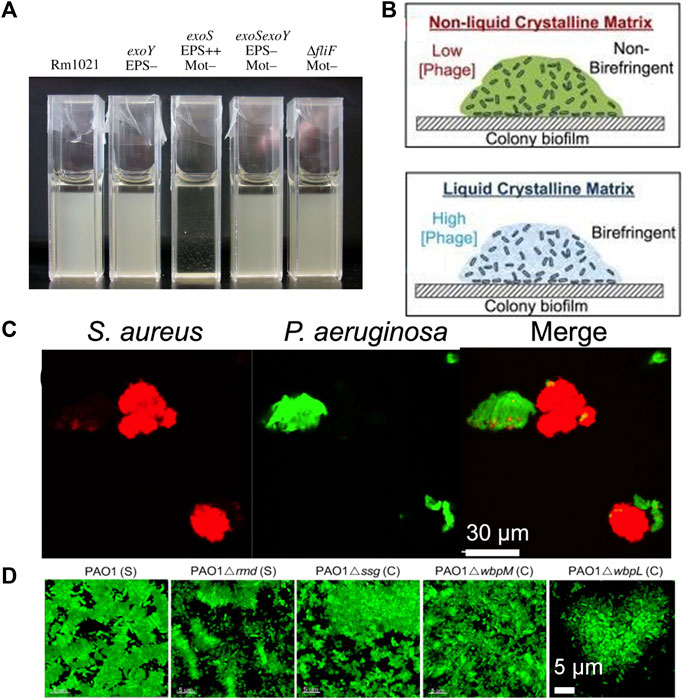
FIGURE 7. Different bacterial aggregation phenomena are different by depletion attractions. (A) Sedimentation screening of Sinorhizobium meliloti Rm1021 and the mutant shown. The strain overexpressing exoS amber sugar settles the fastest within 24 h (Dorken et al., 2012); (B) Pf phages organize the biofilm matrix of Pseudomonas aeruginosa into liquid crystals. Organize the biofilm matrix into liquid crystals to enhance adhesion, antibiotic resistance, and dry survival rate (Secor et al., 2016); (C) Exhaustive aggregation spontaneously separates bacteria with different cell shapes. Showing Pseudomonas aeruginosa expressing GFP and Staphylococcus aureus expressing m-chery (Secor et al., 2021) (D) The loss of common polysaccharide antigen (CPA) (Δrmd) does not change the type of emptying assembly, The loss of O-specific antigen (OSA) (Δwbpm) results in dispersed small aggregates. The absence of CPA and OSA (ΔwbpL) has changed the type of aggregation assembly (Azimi et al., 2021).

4.3 Bacterial movement affects depletion aggregates

The formation of bacterial depletion aggregates is the result of an entropic increase and thus, unlike passive colloidal particles, the presence of flagella and the specific motility of bacteria strongly influence their formation (Schwarz-Linek et al., 2012; Porter et al., 2019). Schwarz-Linek et al. combined experiments, theory and simulations to demonstrate that the use of active colloids (e.g., motile bacteria) can dramatically alter this mixture of evacuated aggregates physical properties. First, significantly stronger interparticle gravitational forces were required to induce phase separation. The force pushing each cell was ≈ 3πησv, where σ ≈ 1 μm and v ≈ 10 μm/s were the typical cell size and velocity, respectively, and η ≈ 1 cP was the viscosity of the aqueous medium; this gived a propulsive force of ≈0.1 pN. Typical forces due to losses can be estimated by the ratio contact strength starting phase separation (≈k_BT, thermal energy) and its range (approximately the size of the polymer, e.g., by its rotational radius, r_g ≈ 10–100 nm), so that k_BT/r_g ≈ 0.05–0.5 pN. Thus, the competition between activity and interparticle attraction should yield interesting and novel physics. Second, the finite-sized aggregates formed under lower interparticle attraction conditions rotate in one direction. Presumably, bacterial suspensions contained a fraction of non-motile organisms (Wilson et al., 2011; Schwarz-Linek et al., 2012). In the presence of polymers, these cells should be the first to aggregate, producing “nuclei” for subsequent aggregation of motile cells. Preliminary BD simulations of motile and non-motile attractive dumbbell mixtures supported the idea that the rotation and translation of clusters were driven by external motile cells. Porter studied the depletion of aggregation of motile and non-motile bacteria in polyethylene glycol solutions in a short time (B10 min) using experimental and numerical calculations. Surface in semi-dilute polymer concentration state and short time scales, the motility of bacteria in dilute solution (Figures 8A–D) made it difficult to form depletion aggregates while greatly promoting the collision rate to enhance the formation of aggregates in a viscous environment, as Figure 8D showed (Porter et al., 2019).

FIGURE 8

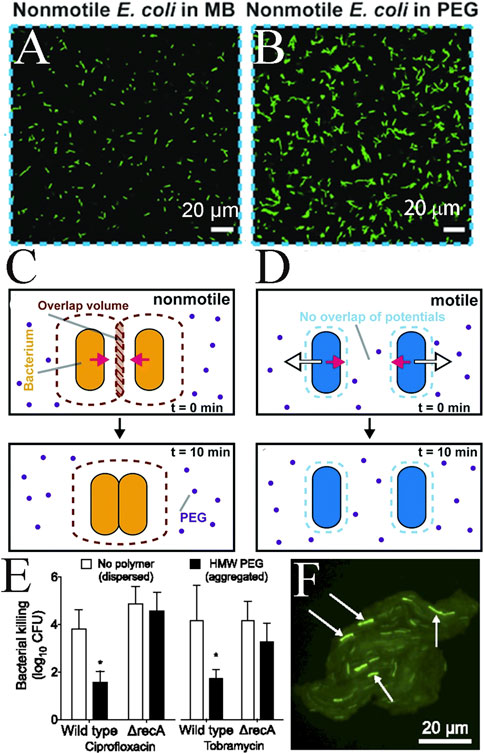
FIGURE 8. Bacterial movement affects depletion attraction, and aggregates have stronger resistance. (A) Representative slices of nonmotile Escherichia coli taken in motility buffer (MB) with no aggregation and (B) in 0.8 mg/mL PEG 1 MDa showing aggregation; (C) A schematic of nonmotile bacteria (orange) in PEG (purple) solution at 0 min and at 10 min. The PEG is excluded from the inter-bacterial volume inducing an effective potential (brown dotted line) due to depletion (pink arrows); (D) A schematic of motile bacteria (blue) in PEG (purple) solution at 0 min and at 10 min. The swim force (white arrows) from bacterial motility decreases the well depth and range of the depletion potential (pink arrows) induced by PEG at a given concentration, preventing aggregation in the dilute PEG concentration regime (Ebrahimi et al., 2019); (E) Antibiotic tolerance of wild-type and ΔrecA Pseudomonas aeruginosa was measured in dispersed (no polymer) or aggregated (HMW PEG) conditions. Bacteria were treated with the indicated antibiotic for 1 h. Killing is represented as the log₁₀ reduction of viable cells treated with antibiotics compared with untreated controls; (F) Representative image showing the fluorescent SOS reporter (recAp::gfp) 1 h after depletion aggregation was induced by HMW PEG (Secor et al., 2018).

The physiological implications of bacterial depletion aggregation due to the presence of non-interacting polymers have been studied extensively. Secor found that bacterial depletion aggregation induced significant antibiotic resistance, which relied on the SOS response, a stress response activated by genotoxic stress, as Figures 8E, F showed. These findings raised the possibility that targeting the conditions that promoted depletion aggregation or depletion-mediated mechanisms of tolerance may lead to new therapeutic approaches to combat chronic bacterial infections (Secor et al., 2018; Secor et al., 2021).

Bacterial aggregates are ubiquitous and their widespread presence in seawater, freshwater, and wastewater treatment systems as well as in chronic infection sites. The formation of bacterial aggregates in marine gel particles (MGP) has been reported to be dependent on the viscosity of plankton-produced polymers (Rochelle-Newall et al., 2010); the large number of polymers secreted by the host found at chronic infection sites may drive bacterial aggregation through entropic forces independent of biofilm formation, which did not require biofilm assembly function or even bacterial viability. Importantly, depletion aggregation significantly and rapidly increased bacterial antibiotic resistance through a mechanism mediated by LexA SOS regulation (Secor et al., 2021). Although extensive research has been conducted on how solid surfaces alter biofilm initiation and formation, studies on bacterial depletion aggregation are still limited due to the complex growth and movement of microorganisms themselves. Current research has focused on the factors affecting bacterial depletion aggregation, and more research is needed to explore further physiological mechanisms of aggregation and effective control of aggregation formation. The next research directions can be focused on the following aspects: 1) Use molecular biology method to dig deeper into the mechanisms of how different polymers affect bacterial aggregation. 2) Focus on the process of bacterial aggregation while swimming. Meanwhile, the physiological processes accompanying aggregation when bacteria growing deserve further exploration. 3) Development strategies of blocking the physiological responses leading to depletion of aggregation-mediated antibiotic resistance or to modify the host environment in a way that reduces the aggregation force generated by host polymers reduce the aggregation and colonization of pathogenic microorganisms.

5 Perspectives and challenges

In this review, we have presented recent progress in understanding the aggregation of colloidal and bacterial systems using depletion attractions. The depletion attractions are related to the size, shape, and concentration of depletion agents. Controlling the concentration of depletion agents can precisely regulate the magnitude of depletion attraction; and introducing appropriate stimulus-responsive particles as depletion agents, which can regulate the magnitude of depletion attraction in situ.

The depletion attraction is related to the shape and roughness of the larger particles and the surface of the container. The development of photolithography has provided specially shaped particles (Zhao and Mason, 2009; Zhao et al., 2011; Zhao et al., 2012; Zhao and Mason, 2012; Wang and Mason, 2015; Zhao and Mason, 2015) and surfaces with microstructures (Boniello et al., 2019). These have given a major boost to the development of shape-matching by depletion attractions. Scientists are using computer simulations to design more special-shaped particles, such as large-area open-ended assemblies that promise to be useful in catalysis and micro and nanodevices (Moore et al., 2021). It is believed that further developments in microfabrication technology will push depletion attractions to play a more prominent role in an increasing number of fields. The refinement of chemical synthesis has also contributed to the development of depletion attractions, not only by developing particles with specific concaves on their surfaces, but also by developing particles with different surface roughness. In recent years, new types of non-isotropic particles have been developed, such as patchy particles and block particles with different surface chemistries (Liu et al., 2020). The combination of depletion attractions with these special particles will exhibit more specific phase behavior, richer assembly structures, and more applications. Controlling the interaction between colloidal particles to achieve the desired self-assembled structure is a fundamental challenge in creating supermaterials via colloidal self-assembly. By investigating the impact of depletion agents, as well as various system parameters such as temperature, concentration, and size, on self-assembly under depletion, general principles can be established. These principles can be used to reverse engineer the desired characteristics of the colloidal system and select an appropriate preparation method. This line of research not only provides a solid scientific foundation for materials science, but also has the potential to significantly contribute to the development of new functional materials in fields such as biology, information technology, and nanotechnology. In the future, this research may play a pivotal role in developing novel photonic bandgap materials for controlling light transmission, intelligent coatings that can detect environmental changes, and new energy storage materials for electric vehicles, thereby promoting sustainable development in the new materials industry.

Physicists studying complex systems and soft matter condensed state physics have discovered self-assembling complex systems based on depletion attractions. These are formed by colloidal particles, which are lifeless particles. Although biologists typically do not focus on depletion aggregation phenomena found in biological systems, the multiple particle aggregation behavior predicted by depletion theory contributes to our understanding of certain biological phenomena. This makes depletion aggregation in biological systems an intriguing topic for physicists to investigate. Bacterial biophysics research under depletion attractions takes place at a scale between macroscopic and microscopic, similar in size to that of colloidal particles. It is related to macroscopic fluid mechanics at large scales and biochemistry, micro-mechanics, and biosensing systems at microscopic scales. We believe that cross-disciplinary cooperation among researchers from various fields is necessary to delve deeper into bacterial biophysics research. Interdisciplinary research in the field of bacteria will play an active role in environmental protection, preventing and eradicating epidemics, and promoting the healthy development of humanity. Depletion attractions are now used in many fields. With the development of synthetic and micro-fabricated technologies by interdisciplinarities, depletion attractions will be shown as advantages and used in more areas.

Author contributions

HZ and HL conceived and designed the project. HZ and HL wrote the original draft. HZ, HL, WZ, and DK wrote the review and editing. HL supervised the project. All authors contributed to the article and approved the submitted version.

Funding

This research was funded by the National Natural Science Foundation of China (grant number 11704276) and the Natural Science Foundation of Tianjin (Grant No. 19JCQNJC14900).

Acknowledgments

We thank Prof. Kun Zhao and Dr. Yiwu Zong for useful discussion.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Abbas, S., Cowman, C. D., and Lodge, T. P. (2009). Effect of added homopolymer on the hexagonal phase formed by cylindrical block copolymer micelles in a selective solvent. Macromol. Rapid Commun. 30 (4-5), 352–361. doi:10.1002/marc.200800599

PubMed Abstract | CrossRef Full Text | Google Scholar

Abbas, S., and Lodge, T. P. (2007). Depletion interactions: A new control parameter for the self-assembly of diblock copolymer micelles. Phys. Rev. Lett. 99 (13), 137802. doi:10.1103/physrevlett.99.137802

PubMed Abstract | CrossRef Full Text | Google Scholar

Amano, K., Iwaki, M., Hashimoto, K., Fukami, K., Nishi, N., Takahashi, O., et al. (2016). Number density distribution of small particles around a large particle: structural analysis of a colloidal suspension. Langmuir 32 (42), 11063–11070. doi:10.1021/acs.langmuir.6b02628

PubMed Abstract | CrossRef Full Text | Google Scholar

Andrew, M., and Simon, B. (1995). Direct measurement of the depletion force using an atomic force microscope. J. Colloid Interface Sci. 170 (2), 604–606. doi:10.1006/jcis.1995.1142

CrossRef Full Text | Google Scholar

Asakura, S., and Oosawa, F. (1958). Interaction between particles suspended in solutions of macromolecules. J. Polym. Sci. 33 (126), 183–192. doi:10.1002/pol.1958.1203312618

CrossRef Full Text | Google Scholar

Asakura, S., and Oosawa, F. (1954). On interaction between two bodies immersed in a solution of macromolecules. J. Chem. Phys. 22 (7), 1255–1256. doi:10.1063/1.1740347

CrossRef Full Text | Google Scholar

Ashton, D. J., Jack, R. L., and Wilding, N. B. (2013). Self-assembly of colloidal polymers via depletion-mediated lock and key binding. Soft Matter 9 (40), 9661–9666. doi:10.1039/c3sm51839f

PubMed Abstract | CrossRef Full Text | Google Scholar

Azimi, S., Thomas, J., Cleland Sara, E., Curtis Jennifer, E., Goldberg Joanna, B., and Diggle Stephen, P. (2021). O-specific antigen-dependent surface hydrophobicity mediates aggregate assembly type in Pseudomonas aeruginosa. mBio 12 (4), e00860–e00821. doi:10.1128/mbio.00860-21

PubMed Abstract | CrossRef Full Text | Google Scholar

Badaire, S., Cottin-Bizonne, C., and Stroock, A. D. (2008). Experimental investigation of selective colloidal interactions controlled by shape, surface roughness, and steric layers. Langmuir 24 (20), 11451–11463. doi:10.1021/la801718j

PubMed Abstract | CrossRef Full Text | Google Scholar

Banerjee, D., Yang, J., and Schweizer, K. S. (2015). Entropic depletion in colloidal suspensions and polymer liquids: role of nanoparticle surface topography. Soft Matter 11 (47), 9086–9098. doi:10.1039/c5sm02072g

PubMed Abstract | CrossRef Full Text | Google Scholar

Baranov, D., Fiore, A., van Huis, M., Giannini, C., Falqui, A., Lafont, U., et al. (2010). Assembly of colloidal semiconductor nanorods in solution by depletion attraction. Nano Lett. 10 (2), 743–749. doi:10.1021/nl903946n

PubMed Abstract | CrossRef Full Text | Google Scholar

Barry, E., and Dogic, Z. (2010). Entropy driven self-assembly of nonamphiphilic colloidal membranes. Proc. Nat. Acad. Sci. U.S.A. 107 (23), 10348–10353. doi:10.1073/pnas.1000406107

PubMed Abstract | CrossRef Full Text | Google Scholar

Bartlett, P., Ottewill, R. H., and Pusey, P. N. (1992). Superlattice Formation in binary mixtures of hard-sphere colloids. Phys. Rev. Lett. 68 (25), 3801–3804. doi:10.1103/physrevlett.68.3801

PubMed Abstract | CrossRef Full Text | Google Scholar

Bibette, J. (1991). Depletion interactions and fractionated crystallization for polydisperse emulsion purification. J. Colloid Interface Sci. 147 (2), 474–478. doi:10.1016/0021-9797(91)90181-7

CrossRef Full Text | Google Scholar

Bjarnsholt, T., Fiandaca, M. J., Pedersen, J., Hansen, C. R., Andersen, C. B., Pressler, T., et al. (2009). Pseudomonas aeruginosa biofilms in the respiratory tract of cystic fibrosis patients. Pediatr. Pulmonol. 44 (6), 547–558. doi:10.1002/ppul.21011

PubMed Abstract | CrossRef Full Text | Google Scholar

Bjarnsholt, T., Jensen, P. O., Alhede, M., Alhede, M., Eickhardt-Sørensen, S. R., Moser, C., et al. (2013). The in vivo biofilm. Trends Microbiol. 21 (9), 466–474. doi:10.1016/j.tim.2013.06.002

PubMed Abstract | CrossRef Full Text | Google Scholar

Boniello, G., Luo, Y., Beller, D. A., Serra, F., and Stebe, K. J. (2019). Colloids in confined liquid crystals: A plot twist in the lock-and-key mechanism. Soft Matter 15 (26), 5220–5226. doi:10.1039/c9sm00788a

PubMed Abstract | CrossRef Full Text | Google Scholar

Brown, S. L., Shah, V. D., Morrell, M. V., Zubich, M., Wagner, A., Denton, A. R., et al. (2018). Superlattice Formation in colloidal nanocrystal suspensions: hard-sphere freezing and depletion effects. Phys. Rev. E. 98 (6), 062616. doi:10.1103/physreve.98.062616

CrossRef Full Text | Google Scholar

Buitenhuis, J., Donselaar, L. N., Buining, P. A., Stroobants, A., and Lekkerkerker, H. N. W. (1995). Phase separation of mixtures of colloidal boehmite rods and flexible polymer. J. Colloid Interface Sci. 175 (1), 46–56. doi:10.1006/jcis.1995.1427

CrossRef Full Text | Google Scholar

Cai, Y.-M. (2020). Non-surface attached bacterial aggregates: A ubiquitous third lifestyle. Front. Microbiol. 11, 557035. doi:10.3389/fmicb.2020.557035

PubMed Abstract | CrossRef Full Text | Google Scholar

Calderon, F. L., Bibette, J., and Biais, J. (1993). Experimental phase diagrams of polymer and colloid mixtures. Europhys. Lett. 23 (9), 653–659. doi:10.1209/0295-5075/23/9/006

CrossRef Full Text | Google Scholar

Calero, C., and Pagonabarraga, I. (2020). Self-assembly of microscopic rods due to depletion interaction. Entropy 22 (10), 1114. doi:10.3390/e22101114

PubMed Abstract | CrossRef Full Text | Google Scholar

Castañeda-Priego, R., Rodríguez-López, A., and Méndez-Alcaraz, J. M. (2006). Entropic forces in dilute colloidal systems. Phys. Rev. E. 73 (5), 051404. doi:10.1103/physreve.73.051404

CrossRef Full Text | Google Scholar

Crocker, J. C., Matteo, J. A., Dinsmore, A. D., and Yodh, A. G. (1999). Entropic attraction and repulsion in binary colloids probed with a line optical tweezer. Phys. Rev. Lett. 82 (21), 4352–4355. doi:10.1103/physrevlett.82.4352

CrossRef Full Text | Google Scholar

De Hek, H., and Vrij, A. (1981). Interactions in mixtures of colloidal silica spheres and polystyrene molecules in cyclohexane: I. Phase separations. J. Colloid Interface Sci. 84 (2), 409–422. doi:10.1016/0021-9797(81)90232-0

CrossRef Full Text | Google Scholar

Dekker, F., González García, Á, Philipse, A. P., and Tuinier, R. (2020). Phase stability of dispersions of hollow silica nanocubes mediated by non-adsorbing polymers. Eur. Phys. J. E. 43(6): 38, doi:10.1140/epje/i2020-11962-y

PubMed Abstract | CrossRef Full Text | Google Scholar

Dinsmore, A. D., and Yodh, A. G. (1999). Entropic confinement of colloidal spheres in corners on silicon substrates. Langmuir 15 (2), 314–316. doi:10.1021/la981243w

CrossRef Full Text | Google Scholar

Dinsmore, A. D., Yodh, A. G., and Pine, D. J. (1996). Entropic control of particle motion using passive surface microstructures. Nature 383 (6597), 239–242. doi:10.1038/383239a0

CrossRef Full Text | Google Scholar

Dorken, G., Ferguson, G., French, C., and Poon, W. (2012). Aggregation by depletion attraction in cultures of bacteria producing exopolysaccharide. J. R. Soc. Interface 9, 3490–3502. doi:10.1098/rsif.2012.0498

PubMed Abstract | CrossRef Full Text | Google Scholar

Du, L., Sun, N., Chen, Z., Li, Y., Liu, X., Zhong, X., et al. (2020). Depletion-mediated uniform deposition of nanorods with patterned, multiplexed assembly. ACS Appl. Mater. Interfaces. 12 (43), 49200–49209. doi:10.1021/acsami.0c13409

PubMed Abstract | CrossRef Full Text | Google Scholar

Duijneveldt, J. S. v., Heinen, A. W., and Lekkerkerker, H. N. W. (1993). Phase separation in bimodal dispersions of sterically stabilized silica particles. Europhys. Lett. 21 (3), 369–374. doi:10.1209/0295-5075/21/3/019

CrossRef Full Text | Google Scholar

Eboigbodin, K., Newton, J., Routh, A. F., and Biggs, C. (2006). Role of nonadsorbing polymers in bacterial aggregation. Langmuir 21, 12315–12319. doi:10.1021/la051740u

PubMed Abstract | CrossRef Full Text | Google Scholar

Ebrahimi, A., Schwartzman, J., and Cordero, O. X. (2019). Multicellular behaviour enables cooperation in microbial cell aggregates. Philos. Trans. R. Soc. Lond. Ser. B 374 (1786), 20190077. doi:10.1098/rstb.2019.0077

PubMed Abstract | CrossRef Full Text | Google Scholar

Evans, E., and Needham, D. (1988). Attraction between lipid bilayer membranes in concentrated solutions of nonadsorbing polymers: comparison of mean-field theory with measurements of adhesion energy. Macromolecules 21 (6), 1822–1831. doi:10.1021/ma00184a049

CrossRef Full Text | Google Scholar

Ghosh, P., Mondal, J., Ben-Jacob, E., and Levine, H. (2015). Mechanically-driven phase separation in a growing bacterial colony. Proc. Nat. Acad. Sci. U.S.A. 112 (17), 2166–2173. doi:10.1073/pnas.1504948112

PubMed Abstract | CrossRef Full Text | Google Scholar

Gong, X.-j., Xing, X.-c., Wei, X.-l., and Ngai, T. (2011). Direct measurement of weak depletion force between two surfaces. Chin. J. Polym. Sci. 29 (1), 1–11. doi:10.1007/s10118-010-1012-8

CrossRef Full Text | Google Scholar

Goodwin, J. W. (2004). “Interactions between colloidal particles,” in Colloids and interfaces with surfactants and polymers – an introduction, 61–93.

Google Scholar

Hayashi, H., Seiki, H., Tsuneda, S., Hirata, A., and Sasaki, H. (2003). Influence of growth phase on bacterial cell electrokinetic characteristics examined by soft particle electrophoresis theory. J. Colloid Interface Sci. 264, 565–568. doi:10.1016/s0021-9797(03)00418-1

PubMed Abstract | CrossRef Full Text | Google Scholar

Herzfeld, J. (1996). Entropically driven order in crowded solutions: from liquid crystals to cell biology. Acc. Chem. Res. 29 (1), 31–37. doi:10.1021/ar9500224

PubMed Abstract | CrossRef Full Text | Google Scholar

Higurashi, E., Sawada, R., and Ito, T. (1998). Optically induced angular alignment of birefringent micro-objects by linear polarization. Appl. Phys. Lett. 73 (21), 3034–3036. doi:10.1063/1.122663

CrossRef Full Text | Google Scholar

Hobbie, E. K. (1999). Depletion-driven phase separation and reversible aggregation in confined colloidal mixtures. Langmuir 15 (26), 8807–8812. doi:10.1021/la990397o

CrossRef Full Text | Google Scholar

Hobbie, E. K. (1998). Metastability and depletion-driven aggregation. Phys. Rev. Lett. 81 (18), 3996–3999. doi:10.1103/physrevlett.81.3996

CrossRef Full Text | Google Scholar

Hou, Z., Zhao, K., Zong, Y., and Mason, T. G. (2019). Phase behavior of two-dimensional brownian systems of corner-rounded hexagons. Phys. Rev. Mater. 3 (1), 015601. doi:10.1103/physrevmaterials.3.015601

CrossRef Full Text | Google Scholar

Hu, S.-W., Sheng, Y.-J., and Tsao, H.-K. (2013). Depletion-induced size fractionation of nanorod dispersions. Soft Matter 9 (30), 7261–7266. doi:10.1039/c3sm50825k

CrossRef Full Text | Google Scholar

Hueckel, T., Hocky, G. M., and Sacanna, S. (2021). Total synthesis of colloidal matter. Nat. Rev. Mater. 6 (11), 1053–1069. doi:10.1038/s41578-021-00323-x

CrossRef Full Text | Google Scholar

Ilett, S. M., Orrock, A., Poon, W. C. K., and Pusey, P. N. (1995). Phase behavior of a model colloid-polymer mixture. Phys. Rev. E. 51 (2), 1344–1352. doi:10.1103/physreve.51.1344

PubMed Abstract | CrossRef Full Text | Google Scholar

Imhof, A., and Dhont, J. K. G. (1995). Experimental phase diagram of a binary colloidal hard-sphere mixture with a large size ratio. Phys. Rev. Lett. 75 (8), 1662–1665. doi:10.1103/physrevlett.75.1662

PubMed Abstract | CrossRef Full Text | Google Scholar

Ishibe, T., Congdon, T., Stubbs, C., Hasan, M., Sosso, G., and Gibson, M. (2019). Enhancement of macromolecular ice recrystallization inhibition activity by exploiting depletion forces. ACS Macro Lett. 8, 1063–1067. doi:10.1021/acsmacrolett.9b00386

PubMed Abstract | CrossRef Full Text | Google Scholar

Jiménez-Ángeles, F., Odriozola, G., and Lozada-Cassou, M. (2011). Entropy effects in self-assembling mechanisms: also a view from the information theory. J. Mol. Liq. 164 (1), 87–100. doi:10.1016/j.molliq.2011.07.013

CrossRef Full Text | Google Scholar

Julie, N., Marit, S., and Kurt Ingar, D. (2014). Stability and interaction forces of oil-in-water emulsions as observed by optical tweezers – A proof-of-concept study. RSC Adv. 4 (94), 52220–52229. doi:10.1039/c4ra07140a

CrossRef Full Text | Google Scholar

Kaplan, P. D., Rouke, J. L., Yodh, A. G., and Pine, D. J. (1994). Entropically driven surface phase separation in binary colloidal mixtures. Phys. Rev. Lett. 72 (4), 582–585. doi:10.1103/physrevlett.72.582

PubMed Abstract | CrossRef Full Text | Google Scholar

Karas, A. S., Glaser, J., and Glotzer, S. C. (2016). Using depletion to control colloidal crystal assemblies of hard cuboctahedra. Soft Matter 12 (23), 5199–5204. doi:10.1039/c6sm00620e

PubMed Abstract | CrossRef Full Text | Google Scholar

Kim, D., Bae, W. K., Kim, S.-H., and Lee, D. C. (2019). Depletion-mediated interfacial assembly of semiconductor nanorods. Nano Lett. 19 (2), 963–970. doi:10.1021/acs.nanolett.8b04198

PubMed Abstract | CrossRef Full Text | Google Scholar

Kim, K., Kim, B. Q., Kim, J. Q., and Choi, S. Q. (2019). New collapse mechanism of colloidal particle monolayers via depletion pressure: formation of large-area particle multilayers at the air–water interface. J. Phys. Chem. C 123 (45), 27862–27867. doi:10.1021/acs.jpcc.9b06963

CrossRef Full Text | Google Scholar

Kim, K., Kim, S., Ryu, J., Jeon, J., Jang, S. G., Kim, H., et al. (2017). Processable high internal phase pickering emulsions using depletion attraction. Nat. Commun. 8 (1), 14305. doi:10.1038/ncomms14305

PubMed Abstract | CrossRef Full Text | Google Scholar

Knoben, W., Besseling, N., and Stuart, M. (2007). Direct measurement of depletion and hydrodynamic forces in solutions of a reversible supramolecular polymer. Langmuir 23, 6095–6105. doi:10.1021/la062656x

PubMed Abstract | CrossRef Full Text | Google Scholar

Koenderink, G. H., Vliegenthart, G. A., Kluijtmans, S. G. J. M., van Blaaderen, A., Philipse, A. P., and Lekkerkerker, H. N. W. (1999). Depletion-induced crystallization in colloidal rod-sphere mixtures. Langmuir 15 (14), 4693–4696. doi:10.1021/la990038t

CrossRef Full Text | Google Scholar

König, P. M., Roth, R., and Dietrich, S. (2008). Lock and key model system. Europhys. Lett. 84 (6), 68006. doi:10.1209/0295-5075/84/68006

CrossRef Full Text | Google Scholar

Kraft, D. J., Ni, R., Smallenburg, F., Hermes, M., Yoon, K., Weitz, D. A., et al. (2012). Surface roughness directed self-assembly of patchy particles into colloidal micelles. Proc. Nat. Acad. Sci. U.S.A. 109 (27), 10787–10792. doi:10.1073/pnas.1116820109

PubMed Abstract | CrossRef Full Text | Google Scholar

Krüger, S., Mögel, H. J., Wahab, M., and Schiller, P. (2011). Depletion force between anisometric colloidal particles. Langmuir 27 (2), 646–650. doi:10.1021/la104141w

PubMed Abstract | CrossRef Full Text | Google Scholar

Kumar, S., Lee, M. J., Aswal, V. K., and Choi, S. M. (2013). Block-copolymer-induced long-range depletion interaction and clustering of silica nanoparticles in aqueous solution. Phys. Rev. E. 87 (4), 042315. doi:10.1103/physreve.87.042315

PubMed Abstract | CrossRef Full Text | Google Scholar

Liu, H.-H., Yang, Y.-R., Shen, X.-C., Zhang, Z.-L., Shen, P., and Xie, Z.-X. (2008). Role of DNA in bacterial aggregation. Curr. Microbiol. 57, 139–144. doi:10.1007/s00284-008-9166-0

PubMed Abstract | CrossRef Full Text | Google Scholar

Lee, E. (2019). Chapter 1 - electrophoresis of a single rigid particle. Interface Sci. Technol. 26, 3–45. doi:10.1016/B978-0-08-100865-2.00001-1

CrossRef Full Text | Google Scholar

Lee, L. T., Guiselin, O., Lapp, A., Farnoux, B., and Penfold, J. (1991). Direct measurements of polymer depletion layers by neutron reflectivity. Phys. Rev. Lett. 67 (20), 2838–2841. doi:10.1103/physrevlett.67.2838

PubMed Abstract | CrossRef Full Text | Google Scholar

Lele, B. J., and Tilton, R. D. (2019). Control of the colloidal depletion force in nonionic polymer solutions by complexation with anionic surfactants. J. Colloid Interface Sci. 553, 436–450. doi:10.1016/j.jcis.2019.06.026

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, S., Wang, L., and Liu, B. (2020). Fabrication of magnetic colloidal bicones and their switchable self-assembly. Soft Matter 16 (34), 8024–8032. doi:10.1039/d0sm00872a

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, T., Zan, X., Sun, Y., Zuo, X., Li, X., Senesi, A., et al. (2013a). Self-assembly of rodlike virus to superlattices. Langmuir 29 (41), 12777–12784. doi:10.1021/la402933q

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, T., Zan, X., Winans, R. E., Wang, Q., and Lee, B. (2013b). Biomolecular assembly of thermoresponsive superlattices of the tobacco mosaic virus with large tunable interparticle distances. Angew. Chem. Int. Ed. 52 (26), 6770–6774. doi:10.1002/ange.201209299

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, W., and Ma, H. R. (2002). Depletion potential near curved surfaces. Phys. Rev. E. 66 (6), 061407. doi:10.1103/physreve.66.061407

PubMed Abstract | CrossRef Full Text | Google Scholar

Lin, W. R., Zheng, C., Wan, X., Liang, D., and Zhou, Q. (2010). Transition of large compound micelles into cylinders in dilute solution: kinetic study. Macromolecules 43, 5405–5410. doi:10.1021/ma1006057

CrossRef Full Text | Google Scholar

Liu, H., Zong, Y., and Zhao, K. (2021). The curvature effect on the diffusion of single brownian squares on a cylindrical surface in the presence of depletion attractions. Langmuir 37 (30), 9264–9268. doi:10.1021/acs.langmuir.1c01540

PubMed Abstract | CrossRef Full Text | Google Scholar

Liu, H., Zong, Y., Zu, M., Mason, T. G., Ye, F., and Zhao, K. (2022). Curvature-assisted self-assembly of brownian squares on cylindrical surfaces. J. Colloid Interface Sci. 605, 863–870. doi:10.1016/j.jcis.2021.07.123

PubMed Abstract | CrossRef Full Text | Google Scholar

Liu, M., Zheng, X., Grebe, V., He, M., Pine, D. J., and Weck, M. (2021). Two-dimensional (2D) or quasi-2D superstructures from DNA-coated colloidal particles. Angew. Chem. Int. Ed. 60 (11), 5744–5748. doi:10.1002/anie.202014045

CrossRef Full Text | Google Scholar

Liu, M., Zheng, X., Grebe, V., Pine, D. J., and Weck, M. (2020). Tunable assembly of hybrid colloids induced by regioselective depletion. Nat. Mater. 19 (12), 1354–1361. doi:10.1038/s41563-020-0744-2

PubMed Abstract | CrossRef Full Text | Google Scholar

Liu, S., Hu, Y., Xia, J., Fang, S., and Duan, M. (2019). In situ measurement of depletion caused by SDBS micelles on the surface of silica particles using optical tweezers. Langmuir 35 (42), 13536–13542. doi:10.1021/acs.langmuir.9b02041

PubMed Abstract | CrossRef Full Text | Google Scholar

Lobo-Cabrera, F., Patti, A., Govantes, F., and Cuetos, A. (2021). Polymer-induced microcolony compaction in early biofilms: A computer simulation study. Phys. Rev. E. 103 (5), 052407. doi:10.1103/physreve.103.052407

PubMed Abstract | CrossRef Full Text | Google Scholar

MaoCates, Y. M. E., and Lekkerkerker, H. N. W. (1995). Depletion force in colloidal systems. Phys. A 222 (1), 10–24. doi:10.1016/0378-4371(95)00206-5

CrossRef Full Text | Google Scholar

Mason, T. G. (2002). Osmotically driven shape-dependent colloidal separations. Phys. Rev. E. 66 (6), 060402. doi:10.1103/physreve.66.060402

PubMed Abstract | CrossRef Full Text | Google Scholar

Moore, T. C., Anderson, J. A., and Glotzer, S. C. (2021). Shape-driven entropic self-assembly of an open, reconfigurable, binary host–guest colloidal crystal. Soft Matter 17 (10), 2840–2848. doi:10.1039/d0sm02073g

PubMed Abstract | CrossRef Full Text | Google Scholar

Nägele, G. (1989). Colloidal hydrodynamics. London and New York: Academic Press.

Google Scholar

Niu, W. A., Rivera, S. L., Siegrist, M. S., and Santore, M. M. (2021). Depletion forces drive reversible capture of live bacteria on non-adhesive surfaces. Soft Matter 17 (35), 8185–8194. doi:10.1039/d1sm00631b

PubMed Abstract | CrossRef Full Text | Google Scholar

Ohshima, Y. N., Sakagami, H., Okumoto, K., Tokoyoda, A., Igarashi, T., Shintaku, K. B., et al. (1997). Direct measurement of infinitesimal depletion force in a colloid-polymer mixture by laser radiation pressure. Phys. Rev. Lett. 78, 3963–3966. doi:10.1103/physrevlett.78.3963

CrossRef Full Text | Google Scholar

Osterman, N. (2010). TweezPal-optical tweezers analysis and calibration software. Comput. Phys. Commun. 181 (11), 1911–1916. doi:10.1016/j.cpc.2010.07.024

CrossRef Full Text | Google Scholar

Padovani, G. C., Petry, R., Holanda, C. A., Sousa, F. A., Saboia, V. M., Silva, C. A., et al. (2015). Mechanisms of colloidal stabilization of oxidized nanocarbons in the presence of polymers: obtaining highly stable colloids in physiological media. J. Phys. Chem. C 119 (32), 18741–18752. doi:10.1021/acs.jpcc.5b04274

CrossRef Full Text | Google Scholar

Perry, R. W., and Manoharan, V. N. (2016). Segregation of isotope particles within colloidal molecules. Soft Matter 12 (11), 2868–2876. doi:10.1039/c5sm02851e

PubMed Abstract | CrossRef Full Text | Google Scholar

Perry, R. W., Meng, G., Dimiduk, T. G., Fung, J., and Manoharan, V. N. (2012). Real-space studies of the structure and dynamics of self-assembled colloidal clusters. Faraday Discuss. 159, 211–234. doi:10.1039/c2fd20061a

CrossRef Full Text | Google Scholar

Piech, M., and Walz, J. Y. (2002). Direct measurement of depletion and structural forces in polydisperse, charged systems. J. Colloid Interface Sci. 253 (1), 117–129. doi:10.1006/jcis.2002.8503

PubMed Abstract | CrossRef Full Text | Google Scholar

Porter, M. K., Preska Steinberg, A., and Ismagilov, R. F. (2019). Interplay of motility and polymer-driven depletion forces in the initial stages of bacterial aggregation. Soft Matter 15 (35), 7071–7079. doi:10.1039/c9sm00791a

PubMed Abstract | CrossRef Full Text | Google Scholar

Pusey, P. N., and van Megen, W. (1986). Phase behaviour of concentrated suspensions of nearly hard colloidal spheres. Nature 320 (6060), 340–342. doi:10.1038/320340a0

CrossRef Full Text | Google Scholar

Ray, D., Aswal, V. K., and Kohlbrecher, J. (2015). Micelle-induced depletion interaction and resultant structure in charged colloidal nanoparticle system. J. Appl. Phys. 117 (16), 164310. doi:10.1063/1.4919359

CrossRef Full Text | Google Scholar

Ray, D., and Aswal, V. K. (2014). Tuning of depletion interaction in nanoparticle-surfactant systems. AIP Conf. Proc. 1591 (1), 175–177. doi:10.1063/1.4872534

CrossRef Full Text | Google Scholar

Repollet-Pedrosa, M., and Mahanthappa, M. (2013). Fusion of poly(vinyl acetate-b-vinyl alcohol) spherical micelles in water induced by poly(ethylene oxide). Soft Matter 9, 7684. doi:10.1039/c3sm51371h

CrossRef Full Text | Google Scholar

Richetti, P., and Kékicheff, P. (1992). Direct measurement of depletion and structural forces in a micellar system. Phys. Rev. Lett. 68 (12), 1951–1954. doi:10.1103/physrevlett.68.1951

PubMed Abstract | CrossRef Full Text | Google Scholar

Rochelle-Newall, E. J., Mari, X., and Pringault, O. (2010). Sticking properties of transparent exopolymeric particles (TEP) during aging and biodegradation. J. Plankton Res. 32, 1433–1442. doi:10.1093/plankt/fbq060

CrossRef Full Text | Google Scholar

Roth, R., Götzelmann, B., and Dietrich, S. (1999). Depletion forces near curved surfaces. Phys. Rev. Lett. 83 (2), 448–451. doi:10.1103/physrevlett.83.448

CrossRef Full Text | Google Scholar

Roth, R., van Roij, R., Andrienko, D., Mecke, K. R., and Dietrich, S. (2002). Entropic torque. Phys. Rev. Lett. 89 (8), 088301. doi:10.1103/physrevlett.89.088301

PubMed Abstract | CrossRef Full Text | Google Scholar

Rudhardt, D., Bechinger, C., and Leiderer, P. (1998). Direct measurement of depletion potentials in mixtures of colloids and nonionic polymers. Phys. Rev. Lett. 81 (6), 1330–1333. doi:10.1103/physrevlett.81.1330

CrossRef Full Text | Google Scholar

Russel, W. B., Saville, D. A., and Schowalter, W. R. (1989). Colloidal dispersions. Cambridge: Cambridge University Press.

Google Scholar

Sanyal, S., Easwar, N., Ramaswamy, S., and Sood, A. K. (1992). Phase separation in binary nearly-hard-sphere colloids: evidence for the depletion force. Europhys. Lett. 18 (2), 107–110. doi:10.1209/0295-5075/18/2/003

CrossRef Full Text | Google Scholar

Sacanna, S., Pine, D. J., and Yi, G.-R. (2013). Engineering shape: the novel geometries of colloidal self-assembly. Soft Matter 9 (34), 8096–8106. doi:10.1039/c3sm50500f

CrossRef Full Text | Google Scholar

Saez Cabezas, C. A., Sherman, Z. M., Howard, M. P., Dominguez, M. N., Cho, S. H., Ong, G. K., et al. (2020). Universal gelation of metal oxide nanocrystals via depletion attractions. Nano Lett. 20 (5), 4007–4013. doi:10.1021/acs.nanolett.0c01311

PubMed Abstract | CrossRef Full Text | Google Scholar

Schwarz-Linek, J., Dorken, G., Winkler, A., Wilson, L. G., Pham, N. T., French, C. E., et al. (2010a). Polymer-induced phase separation in suspensions of bacteria. Europhys. Lett. 89 (6), 68003. doi:10.1209/0295-5075/89/68003

CrossRef Full Text | Google Scholar

Schwarz-Linek, J., Valeriani, C., Cacciuto, A., Cates, M., Marenduzzo, D., Morozov, A., et al. (2012). Phase separation and rotor self-assembly in active particle suspensions. Proc. Nat. Acad. Sci. U.S.A. 109, 4052–4057. doi:10.1073/pnas.1116334109

PubMed Abstract | CrossRef Full Text | Google Scholar

Schwarz-Linek, J., Winkler, A., Wilson, L. G., Pham, N. T., Schilling, T., and Poon, W. C. K. (2010b). Polymer-induced phase separation in Escherichia coli suspensions. Soft Matter 6 (18), 4540–4549. doi:10.1039/c0sm00214c

CrossRef Full Text | Google Scholar

Secor, P., Jennings, L., Michaels, L., Sweere, J., Singh, P., Parks, W., et al. (2016). Biofilm assembly becomes crystal clear – filamentous bacteriophage organize the Pseudomonas aeruginosa biofilm matrix into a liquid crystal. Microb. Cell 3, 49–52. doi:10.15698/mic2016.01.475

PubMed Abstract | CrossRef Full Text | Google Scholar

Secor, P., Michaels, L., Bublitz, D., Jennings, L., and Singh, P. (2021). The depletion mechanism actuates bacterial aggregation by exopolysaccharides and determines species distribution & composition in bacterial aggregates. Front. Cell. Infect. Microbiol. 12, 869736. doi:10.3389/fcimb.2022.869736

CrossRef Full Text | Google Scholar

Secor, P. R., Michaels, L. A., Ratjen, A., Jennings, L. K., and Singh, P. K. (2018). Entropically driven aggregation of bacteria by host polymers promotes antibiotic tolerance in Pseudomonas aeruginosa. Proc. Nat. Acad. Sci. U.S.A. 115 (42), 10780–10785. doi:10.1073/pnas.1806005115

PubMed Abstract | CrossRef Full Text | Google Scholar

Steiner, U., Meller, A., and Stavans, J. (1995). Entropy driven phase separation in binary emulsions. Phys. Rev. Lett. 74 (23), 4750–4753. doi:10.1103/physrevlett.74.4750

PubMed Abstract | CrossRef Full Text | Google Scholar

Sun, X., Danumah, C., Liu, Y., and Boluk, Y. (2012). Flocculation of bacteria by depletion interactions due to rod-shaped cellulose nanocrystals. Chem. Eng. J. 198-199, 476–481. doi:10.1016/j.cej.2012.05.114

CrossRef Full Text | Google Scholar

Sung, B., Wensink, H. H., and Grelet, E. (2019). Depletion-driven morphological transitions in hexagonal crystallites of virus rods. Soft Matter 15 (46), 9520–9527. doi:10.1039/c9sm01207a

PubMed Abstract | CrossRef Full Text | Google Scholar

van Ravensteijn, B. G. P., Hage, P. A., and Voets, I. K. (2020). Framed by depletion. Nat. Mater. 19 (12), 1261–1263. doi:10.1038/s41563-020-00861-9

PubMed Abstract | CrossRef Full Text | Google Scholar

Vrij, A. (1977). “Polymers at interfaces and the interactions in colloidal dispersions,” in Colloid and surface science. Editor E. Wolfram (Pergamon), 471–483.

CrossRef Full Text | Google Scholar

Wan, D., and Glotzer, S. C. (2018). Shapes within shapes: how particles arrange inside a cavity. Soft Matter 14 (16), 3012–3017. doi:10.1039/c8sm00048d

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, L., Lu, J., and Liu, B. (2019). Metastable self-assembly of theta-shaped colloids and twinning of their crystal phases. Angew. Chem. Int. Ed. 58 (46), 16585–16590. doi:10.1002/ange.201908256

CrossRef Full Text | Google Scholar

Wang, P. Y., and Mason, T. G. (2015). Colloidal lock-and-key dimerization reactions of hard annular sector particles controlled by osmotic pressure. J. Am. Chem. Soc. 137 (48), 15308–15314. doi:10.1021/jacs.5b10549

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, X., Shen, X., Wang, Z., and Kong, Y. (2019). Viscoelasticity variation in a biofilm-mediated Bacillus subtilis suspension induced by adding polyethylene glycol. Eur. Biophys. J. 48 (7), 599–608. doi:10.1007/s00249-019-01385-0

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, Y., Wang, Y., Breed, D. R., Manoharan, V. N., Feng, L., Hollingsworth, A. D., et al. (2012). Colloids with valence and specific directional bonding. Nature 491 (7422), 51–55. doi:10.1038/nature11564

PubMed Abstract | CrossRef Full Text | Google Scholar

Wilking, J. N., and Mason, T. G. (2008). Multiple trapped states and angular kramers hopping of complex dielectric shapes in a simple optical trap. Europhys. Lett. 81 (5), 58005. doi:10.1209/0295-5075/81/58005

CrossRef Full Text | Google Scholar

Wilson, L., Martinez, V., Schwarz-Linek, J., Tailleur, J., Bryant, G., Pusey, P., et al. (2011). Differential dynamic microscopy of bacterial motility. Phys. Rev. Lett. 106, 018101. doi:10.1103/physrevlett.106.018101

PubMed Abstract | CrossRef Full Text | Google Scholar

Wolters, J. R., Avvisati, G., Hagemans, F., Vissers, T., Kraft, D. J., Dijkstra, M., et al. (2015). Self-assembly of "mickey Mouse" shaped colloids into tube-like structures: experiments and simulations. Soft Matter 11 (6), 1067–1077. doi:10.1039/c4sm02375g

PubMed Abstract | CrossRef Full Text | Google Scholar

Wolters, J. R., Verweij, J. E., Avvisati, G., Dijkstra, M., and Kegel, W. K. (2017). Depletion-induced encapsulation by dumbbell-shaped patchy colloids stabilize microspheres against aggregation. Langmuir 33 (13), 3270–3280. doi:10.1021/acs.langmuir.7b00014

PubMed Abstract | CrossRef Full Text | Google Scholar

Xie, Y., Li, Y., Wei, G., Liu, Q., Mundoor, H., Chen, Z., et al. (2018). Liquid crystal self-assembly of upconversion nanorods enriched by depletion forces for mesostructured material preparation. Nanoscale 10 (9), 4218–4227. doi:10.1039/c7nr06663e

PubMed Abstract | CrossRef Full Text | Google Scholar

Xing, X., Sun, G., Li, Z., and Ngai, T. (2012). Stabilization of colloidal suspensions: competing effects of nanoparticle halos and depletion mechanism. Langmuir 28 (46), 16022–16028. doi:10.1021/la303547m

PubMed Abstract | CrossRef Full Text | Google Scholar

Yadav, I., Kumar, S., Aswal, V. K., and Kohlbrecher, J. (2014). Small-angle neutron scattering study of differences in phase behavior of silica nanoparticles in the presence of lysozyme and bovine serum albumin proteins. Phys. Rev. E. 89 (3), 032304. doi:10.1103/physreve.89.032304

PubMed Abstract | CrossRef Full Text | Google Scholar

Yang, T., Lei, Z., Yang, S., and Chen, E.-Q. (2019). Depletion driven self-assembly of block copolymer solutions by homopolymers. Phys. Chem. Chem. Phys. 21, 2121–2127. doi:10.1039/c8cp06679e

PubMed Abstract | CrossRef Full Text | Google Scholar

Yang, Y., Edwards, T. D., and Bevan, M. A. (2015). Modeling depletion mediated colloidal assembly on topographical patterns. J. Colloid Interface Sci. 449, 270–278. doi:10.1016/j.jcis.2014.12.002

PubMed Abstract | CrossRef Full Text | Google Scholar

Yasrebi, M., Shih, W. Y., and Aksay, I. A. (1991). Clustering of binary colloidal suspensions: experiment. J. Colloid Interface Sci. 142 (2), 357–368. doi:10.1016/0021-9797(91)90066-h

CrossRef Full Text | Google Scholar

Ye, X., Millan, J. A., Engel, M., Chen, J., Diroll, B. T., Glotzer, S. C., et al. (2013). Shape alloys of nanorods and nanospheres from self-assembly. Nano Lett. 13 (10), 4980–4988. doi:10.1021/nl403149u

PubMed Abstract | CrossRef Full Text | Google Scholar

Ye, X., Narayanan, T., Tong, P., Huang, J. S., Lin, M. Y., Carvalho, B. L., et al. (1996). Depletion interactions in colloid-polymer mixtures. Phys. Rev. E. 54 (6), 6500–6510. doi:10.1103/physreve.54.6500

PubMed Abstract | CrossRef Full Text | Google Scholar

Young, K. L., Jones, M. R., Zhang, J., Macfarlane, R. J., Esquivel-Sirvent, R., Nap, R. J., et al. (2012). Assembly of reconfigurable one-dimensional colloidal superlattices due to a synergy of fundamental nanoscale forces. Proc. Nat. Acad. Sci. U.S.A. 109 (7), 2240–2245. doi:10.1073/pnas.1119301109

PubMed Abstract | CrossRef Full Text | Google Scholar

Zanella, M., Bertoni, G., Franchini, I. R., Brescia, R., Baranov, D., and Manna, L. (2011). Assembly of shape-controlled nanocrystals by depletion attraction. Chem. Commun. 47 (1), 203–205. doi:10.1039/c0cc02477e

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, C., Wang, G., Takarada, T., Liang, X., Komiyama, M., and Maeda, M. (2019). Shape-selective isolation of Au nanoplates from complex colloidal media by depletion flocculation. Colloids Surf. A Physicochem. Eng. Asp. 568, 216–223. doi:10.1016/j.colsurfa.2019.02.019

CrossRef Full Text | Google Scholar

Zhao, K., Bruinsma, R., and Mason, T. G. (2011). Entropic crystal–crystal transitions of brownian squares. Proc. Nat. Acad. Sci. U.S.A. 108 (7), 2684–2687. doi:10.1073/pnas.1014942108

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., Bruinsma, R., and Mason, T. G. (2012). Local chiral symmetry breaking in triatic liquid crystals. Nat. Commun. 3, 801. doi:10.1038/ncomms1803

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., and Mason, T. (2008a). Directing colloidal self-assembly through roughness-controlled depletion attractions. Phys. Rev. Lett. 99, 268301. doi:10.1103/physrevlett.99.268301

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., and Mason, T. G. (2009). Frustrated rotator crystals and glasses of brownian pentagons. Phys. Rev. Lett. 103 (20), 208302. doi:10.1103/physrevlett.103.208302

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., and Mason, T. G. (2015). Shape-designed frustration by local polymorphism in a near-equilibrium colloidal glass. Proc. Nat. Acad. Sci. U.S.A. 112 (39), 12063–12068. doi:10.1073/pnas.1507897112

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., and Mason, T. G. (2012). Twinning of rhombic colloidal crystals. J. Am. Chem. Soc. 134 (43), 18125–18131. doi:10.1021/ja308214m

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, K., and Mason, T. (2008b). Suppressing and enhancing depletion attractions between surfaces roughened by asperities. Phys. Rev. Lett. 101, 148301. doi:10.1103/physrevlett.101.148301

PubMed Abstract | CrossRef Full Text | Google Scholar

Zosel, F., Soranno, A., Buholzer, K. J., Nettels, D., and Schuler, B. (2020). Depletion interactions modulate the binding between disordered proteins in crowded environments. Proc. Nat. Acad. Sci. U.S.A. 117 (24), 13480–13489. doi:10.1073/pnas.1921617117

PubMed Abstract | CrossRef Full Text | Google Scholar