- 1Department of Mathematics, University of Engineering and Technology, Lahore, Pakistan
- 2Department of Mathematics, Minhah University, Lahore, Pakistan
- 3Department of Mathematical Sciences, Faculty of Science and Technology, Universiti Kebangsaan Malaysia, Selangor, Malaysia
- 4Department of Mathematics and Social Sciences, Sukkur IBA University, Sukkur, Sindh Pakistan
- 5Department of Industrial & Systems Engineering, College of Engineering, Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia
- 6Center of Research, Faculty of Engineering, Future University in Egypt, New Cairo, Egypt
- 7Department of Industrial Engineering, College of Engineering, Prince Sattam Bin Abdulaziz University, Alkharj, Saudi Arabia
- 8Industrial Engineering Department, Faculty of Engineering, Zagazig University, Zagazig, Egypt
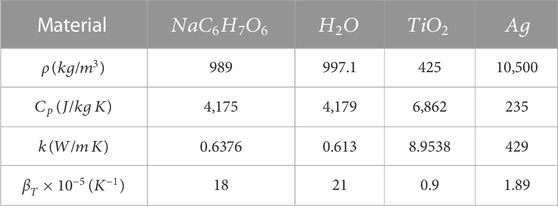
The functional effects of medications, such as those that slow down and calm the body, have been investigated for the polarized diffusion coefficient based on the subsequent increase through magnetism. This study examines generalized Mittag–Lefer kernel-based fractional derivatives in MHD Brinkman-type fluids under bioconvection that contain hybrid titanium dioxide (TiO2) and silver (Ag) nanoparticles with water (H2O) and sodium alginate (NaC6H7O6) as the base fluids. Atangana–Baleanu (AB) and Caputo–Fabrizio (CF) fractional derivatives, which are two contemporary definitions of fractional-order derivatives with a memory effect, were used to explore the modified fractional model utilizing the Laplace transformation and certain numerical algorithms. The impacts of restrictions on various nanoparticles were investigated and graphically displayed. We observed that the volumetric fraction improvement controls the fluid velocity by slowing it down. The water-based hybrid nanofluid has a greater influence on the temperature and momentum fields than the sodium alginate-based hybrid nanofluid due to the physical characteristics of the explored nanoparticles and base fluids. Additionally, the memory effect causes a higher substantial value for the AB-fractional derivative of the velocity profile than the CF-fractional derivative.
1 Introduction
Channel flow has been applied in various industrial fields including chemical reactors in pharmacological manufacturing and thermal exchangers in energy plants. Although various natural phenomena and Newtonian actions in equal stages may be named two-phase movements, there are many related applications where the constant fluid segment displays non-Newtonian movement characteristics. In the last two decades, the impact of numerous heat transfer behaviors in the industry and engineering sectors has benefited from and been improved by nanofluid science. The development of a hybrid nanofluid is another recent advancement in nanotechnology. This new product combines at least two substances with various physical and chemical features such as heat flow and thermal conductivity. In essence, the development of a hybrid nanofluid has completely transformed this new product. Numerous researchers have become interested in nanofluids due to their thermal solid conductivity performance. Examples abound in the biomedical, biochemical, and food distribution industries (Bräuer et al., 2021). Zheng et al. (2021) explored how a vortex maker formed affected fluids and the thermal conversion of HNFs in a channel. D’Ippolito et al. (2021) evaluated the systematic scale conflict of channel movement caused by vegetation. Using the CF derivative, Haq et al. (2020) developed MHD’s fractional viscid fluid impact in a channel across a permeable surface.
The exceptional perception of nanoscience to increase the amount of heat sources has motivated researchers due to the constantly growing requirement for heat storage. For example, thermal transmission properties are claimed in fields ranging from biomedical to manufacturing industries. Improvements in thermal efficiency provide an advantage for plasma research, electronic equipment like computer chips, nuclear reactors, electricity generation, space cooling, and many others. The macroscopic fluid convection motion phenomenon, which is known as “bioconvection,” is caused by the thickness angle formed by the mass of directional swimming microorganisms. Bioconvection was a fundamental principle introduced by Plat in 1961. Bioconvection is used, among other things, in the manufacturing of biological polymers in biotechnology and biosensors and in the testing and lab equipment sectors (Platt, 1961; Asjad et al., 2021). Sisko’s three-dimensional radiative bioconvective stream was used by Ge-JiLe et al. (2021) to analyze nanofluids containing moving microorganisms. Ramzan et al. (2021) showed the occurrence of bioconvection in a three-dimensional meandering hyperbolic partially ionized magnetized nanofluid stream with Cattaneo–Christov heat flux and activation vitality. An examination of magneto-bioconvective enhancement and thermal conductivity in a nanofluid stream containing gyrotactic microorganisms was conducted by Alhussain et al. (2021). Farooq et al. (2021) adjusted Cattaneo–Christov equations and exponential space-based heat sources to account for a thermally radioactive Carreau nanofluid bioconvection flow. Yusuf et al. (2021) investigated the role of gyrotactic bacteria and entropy generation in the movement of Williamson nanofluids across an inclined plate. A Brinkman-type fluid (BTF) fractional model utilizing hybrid nanoparticles was examined by Saqib et al. (2020). Danish Ikram et al. considered the heat transfer of an exponentially moving vertical plate over a viscous fluid containing clay nanoparticles. Using a hybrid fractional operator, Asjad et al. (2020) looked into the thermophysical characteristics of clay nanofluids. Using a constant and proportional Caputo fractional operator, Ikram et al. (2021) established a fractional model of a Brinkman-type fluid transporting hybrid nanoparticles in a confined microchannel.
A specific or isolated nanomaterial might exhibit extraordinary thermophysical or rheologic properties in addition to the excellent and typical requirements for a certain use. HNF is controlled to maintain different properties despite the multiple applications. An advanced NF, which is called a hybrid nanofluid, combines “two or more distributed NPs in the base liquid.” The purpose of examining HNFs is to improve various thermal processes, such as heat transfer, highly well-organized heat conductivity, and solidity, by balancing the benefits and drawbacks of remarkable suspensions. Examples of these processes include the refrigeration of generators, cooling systems for machines, electric refrigeration, refrigeration of converters, and atomic structure refrigeration. HNFs’ capacities to improve thermal conductivity offers an opportunity to account for them in real-valued energy models. An HNF was tested over a porous surface in motion along with alumina NPs in Waini et al. (2019), and studies of the volume fraction for copper NPs were confirmed. To simulate the HNF, two NPs were included in the composite along with water. To study the mobility of HNF (Al2O3-CuO/H2O), Ashwinkumar et al. (2021) used radiation passing through a vertical plate and cone. Their research focused on contrasting two different forms and the characteristics of HNF flow. Samrat et al. (2022) considered heat transmission when analyzing the movement of NF and HNF due to the stretched surface. Characteristic groundwork investigations completed on HNFs have also been conducted (Raza et al., 2019; Anuar et al., 2020; Sulochana et al., 2020; TÜRKYILMAZOĞLU, 2021; Ibraheem et al., 2022; Raza et al., 2022; Turkyilmazoglu, 2022; Zhang et al., 2022; Turkyilmazoglu and Altanji, 2023).
The field of fractional calculus is rapidly expanding due to the wide variety of processes that it can be applied to every day. Numerous definitions of fractional derivatives have been published in the literature due to the curiosity of academics. A fractional-order derivative based on an exponential function was created by Caputo–Fabrizio (CF) to address the challenges with earlier research on the singularity problem of the kernel. However, the fractional model of Caputo and Fabrizio lacks a specific kernel. This idea of fractional order has received significant attention in recent studies in the area of fractional calculus. Understanding the viscoelastic and rheologic properties of HNFs in detail are difficult due to the self-similar properties and memory-taking capacities of fractional operators. They provide a reasonable explanation for the behavior and heat efficiency of nanofluids. These operators have frequently been used to mimic and examine the specifics of various natural formations over the past few years. Fractional derivatives are widely applicable in signal processing, epidemiology, population modeling, economics, dynamic structures, fluid dynamics, electrochemistry, and many other fields. The three most fundamental standard fractional operators in fractional calculus depend on the convolution of regular derivatives and distinctive kernels. The two leading derivatives in this hierarchy are Riemann-Liouville and Caputo’s derivatives, where the first operator complicates a power-law kernel (Podlubny, 1999). Due to its widespread use, the definition of CF has been used by numerous researchers for a variety of scientific inquiries. The kernel’s non-locality, which is a difficulty in the definition of CF, was addressed by Atangana and Nieto (2015) in a new formulation. The most current explanation of fractional derivatives without these problems was given by Atangana (2016). AB derivatives are rarely used in research, and this word is brand-new. Arif et al. (2019) used this most recent concept of AB-fractional derivatives to compare these two ideas, and the current issue is also applied to CF-fractional derivatives. In addition, this study solves the well-known pair stress fluid model with an external pressure gradient in a closed-form channel. Even though they employed the Caputo and CF-fractional model, Akhtar (2016) estimated the closed-form solutions (CSF) in channels. To avoid these obstacles, Atangana and Baleanu produced a novel operator whose mathematical expansion encompasses the convolution of the regular derivative and a general kernel, which is included in the Mittag–Leffler function (Atangana and Baleanu, 2016). Currently, in perceiving the worth of fractional-order operators, an extensive range of investigators has explored real-life phenomena in fractional situations rather than in regular contexts. Asjad et al. (2022) investigated the fractional bioconvection nanofluid solution moving through a channel. Investigations on heat transfer and fluid problems were conducted using local and non-local kernel approaches by Ali et al. (2022a) and Ali et al. (2022b). Tarasov explained how a novel rebellion, “memory revolution,” occurred in present mathematical economics just because of fractional derivatives and corresponding integrals. The investigation of neurology has also been impacted by the initiation of fractional calculus (Tarasov, 2019; Riaz et al., 2022).
In the absence of fractional bioconvection, those previously mentioned were carried out by or lacking fractional approaches. However, the fundamental purpose is to combine these two fascinating topics: bioconvection and fractional operators. In the previous literature, we did not find any investigation on the focus of fractional bioconvection of an applied magnetic field on a naturally occurring Brinkman-type flow of a hybrid nanofluid over two parallel plates with CF- and AB-fractional derivative approaches. Consequently, we applied the Laplace transform method to solve the thermal transmission and fluid flow problems of bioconvection. In addition, graphical analysis was applied to present a graphical description of the diverse flow parameters.
2 Description of the problem
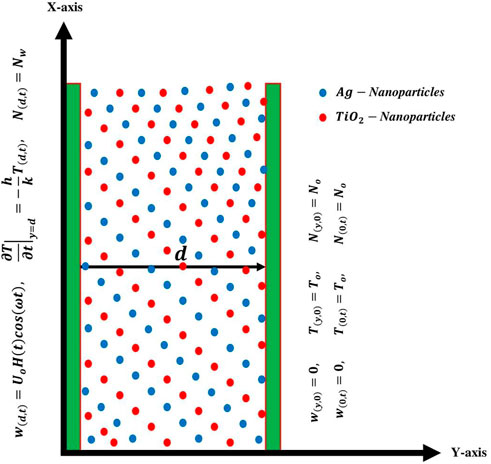
Suppose an incompressible, unsteady, and free convective Brinkman-type hybrid nanofluid moves along the path of two parallel plates with characteristics of mass diffusion and temperature gradient, as described in Figure 1. Both plates are fixed in the xy-plane at a distance d that is vertical to the y-axis and parallel to the x-axis. The whole system, including the fluid and any relevant limitations, is initially at
• The length of the parallel plates is infinite with width d.
• The poured plates are vertical to the y-axis and oriented in the x-direction.
• At
• Different nanoparticles in the mixed hybrid nanofluid accelerate in the x-direction.
• The constant magnetic field of strength
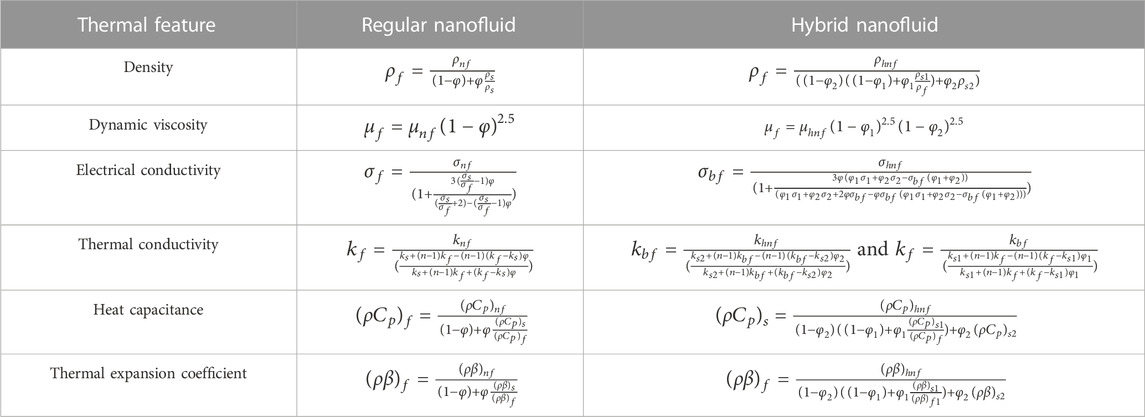
By utilizing Boussinesq’s (Mayeli and Sheard, 2021) and Roseland approximations (Chu et al., 2020), the governed partial differential equations can be formulated as follows (Asjad et al., 2022):
where
The initial conditions of the entire system are considered in Eq. 4 at
and utilizing the aforementioned none-dimensional constraints in the governed equations and conditions (1)–(6), one can obtain the following results by adjusting the abovementioned dimensionless variables while ignoring the ″*″ symbols:
with consistent dimensionless circumstances
where
3 Basic preliminaries
In the fractional modeling of the set, as mentioned previously for the governing equations, the ABC (Atangana and Baleanu, 2016) and CF (Caputo and Fabrizio, 2015) formulations are employed, and these temporal derivatives are specified as follows:
with its LT
The mathematical form of the CF-fractional derivative is (Caputo and Fabrizio, 2015)
and the LT of the CF-fractional derivative is
4 Solution with the AB-fractional derivative
4.1 Solution of the temperature profile
The solution of the non-dimensional equation of the temperature field can be represented as follows in the sense of the AB-time fractional derivative:
with
The result of the temperature field can be found by applying the Laplace transformation to the abovementioned equation and utilizing the relevant conditions as follows:
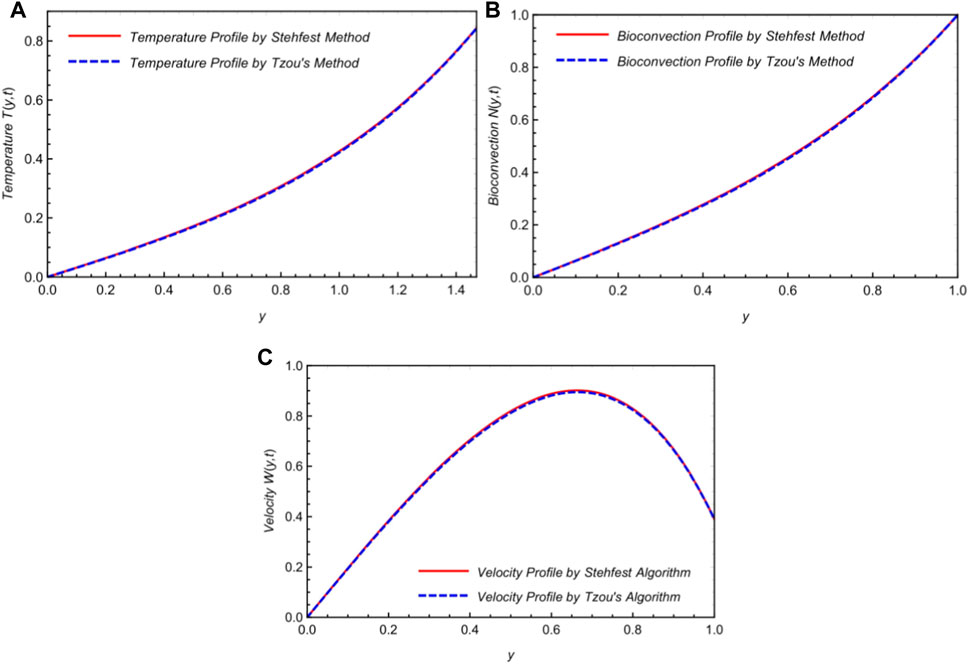
The Laplace inverse of the solution, as described previously, will be numerically evaluated using Stehfest and Tzou’s methods in Tables 3–5.
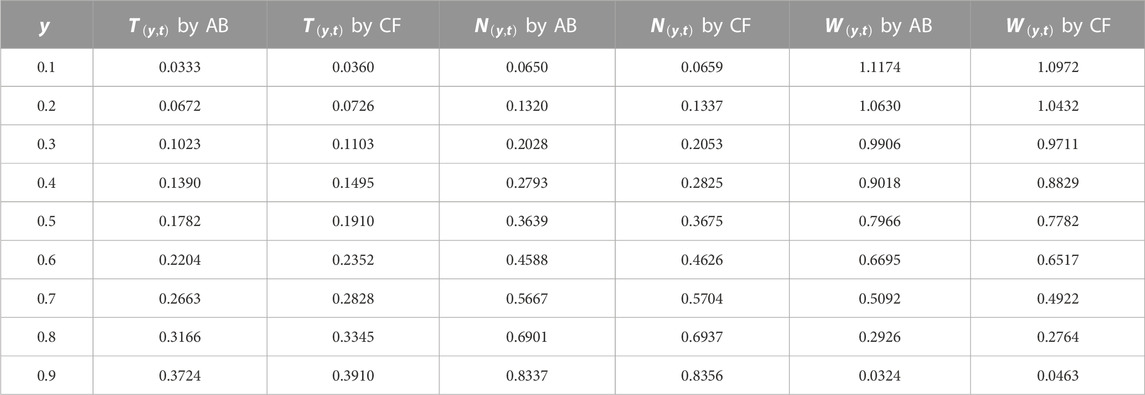
TABLE 4. Numerical analysis of governed solutions with the AB- and CF-fractional derivatives at t = 0.5
4.2 Solution of the bioconvection profile
In terms of the AB-time fractional derivative, the solution of a non-dimensional equation of the bioconvection profile may be attained by employing the Laplace transformation on the governed equations in terms of the AB-time fractional derivative, and we get
with the corresponding conditions
By using the aforementioned conditions and Eq. 16, the solution of the bioconvection profile can be derived as
The Laplace inverse of the aforementioned Eq. 17 will be analyzed numerically in Tables 3–5 with numerical algorithms.
4.3 Solution of the velocity profile
For the solution of the velocity profile by employing the LT on the non-dimensional governed equation of the velocity field in Eq. 7,
with
5 Solution with the CF-fractional derivative
5.1 Solution of the temperature field
The following representation shows the evaluation of the non-dimensional temperature field solution using the CF-time fractional derivative
with
The solution of the temperature field will be obtained by applying Laplace to the preceding equation and employing the appropriate conditions as follows:
Using Stehfest and Tzou’s techniques in Tables 3–5, the Laplace inverse of the aforementioned solution will be numerically examined.
5.2 Solution of the bioconvection field
By using the Laplace transformation on the guided equations in terms of the CF-time fractional derivative, we gain the solution of a non-dimensional equation of the bioconvection profile as follows:
with the following conditions
By using the aforementioned conditions, the solution of the bioconvection profile in terms of the CF-fractional derivative can be derived as follows:
The Laplace inverse of Eq. 21 will be numerically analyzed using the techniques in Tables 3–5.
5.3 Solution of the velocity profile
The solution of the velocity profile is obtained by employing the LT on the non-dimensional governed equation of the velocity field (Eq. 7):
with
Analyzing the results of the momentum, concentration, and temperature profiles is difficult. We also employed numerical approaches for the Laplace inverse, specifically Stehfest and Tzou’s numerical schemes, as numerous authors have previously performed. The mathematical formulations of these algorithms (Raza et al., 2021a; Raza et al., 2021b; Guo et al., 2021) can be characterized as
and
6 Discussion of the results
Bioconvection is investigated by employing the combined effects of porosity and the applied magnetic field on a naturally occurring Brinkman-type flow of a viscous and incompressible HNF (Ag-TiO2-H2O and Ag-TiO2-C6H9NaO7) over two parallel plates. The thermal transmission and fluid flow model is fractionalized with two fractional techniques (AB- and CF-fractional derivatives). The Laplace transformation approach is utilized to solve the governing equations. The impact of different constraints has been analyzed graphically with their ranges as follows:
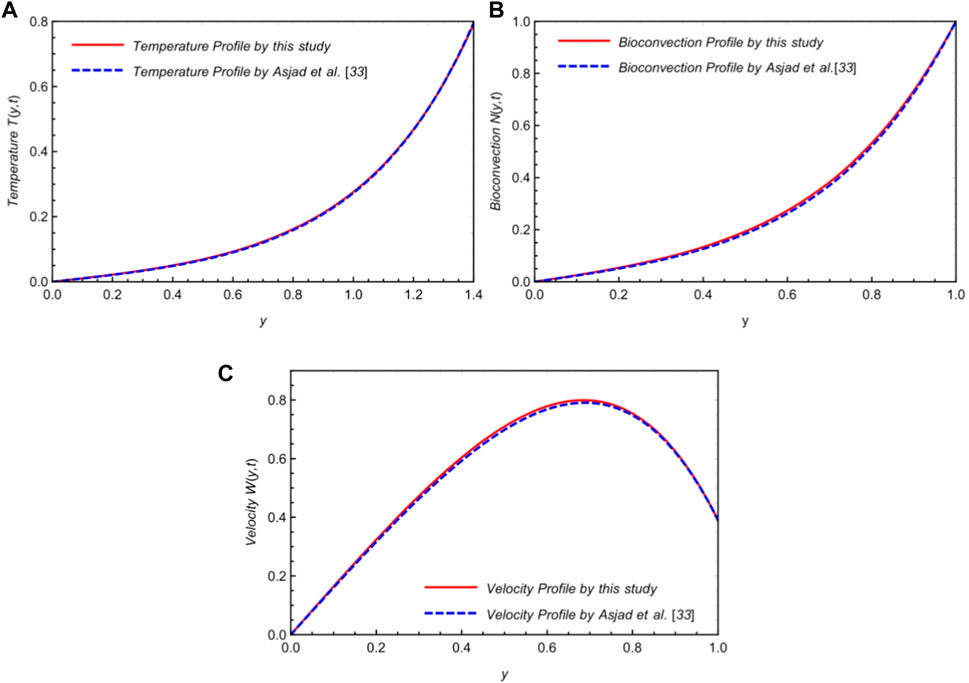
FIGURE 14. Comparison of the validity of governed equations with the attained results of Asjad et al. (2022).
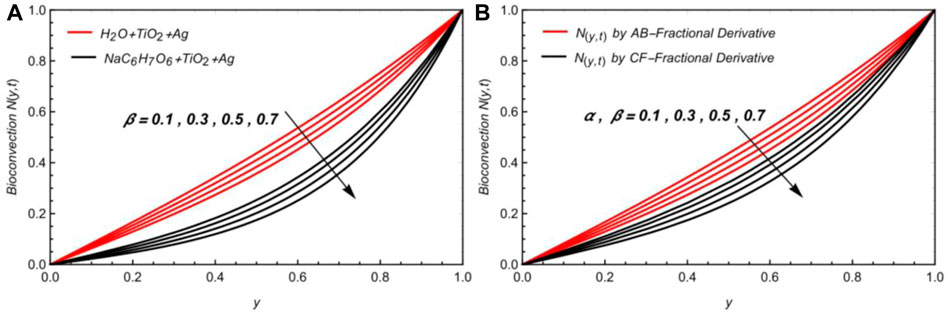
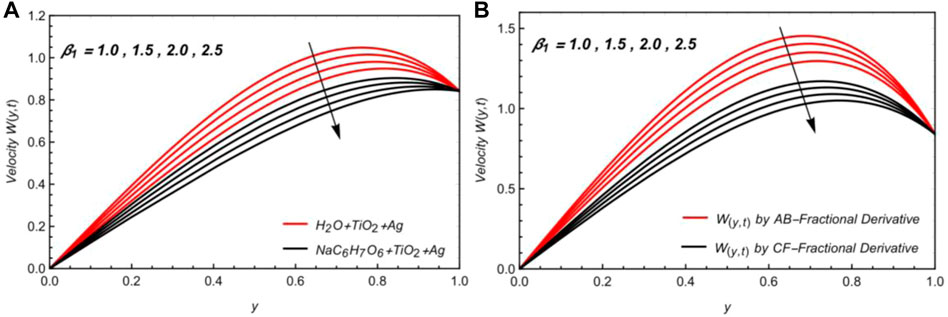
Figure 2A illustrates the influence of the fractional parameter β on bioconvection. The growing value of β causes a decline in bioconvection. This results in a decrease in bioconvection as the boundary layer enlarges. Figure 2B shows the effects of
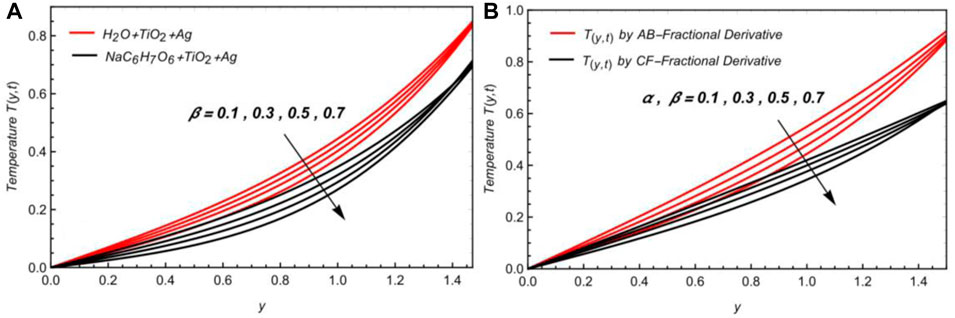
Figure 3A shows that the temperature field declined by increasing β. As the boundary layer becomes extensive, the temperature reduces, which is expected. Figure 3B shows the effects of
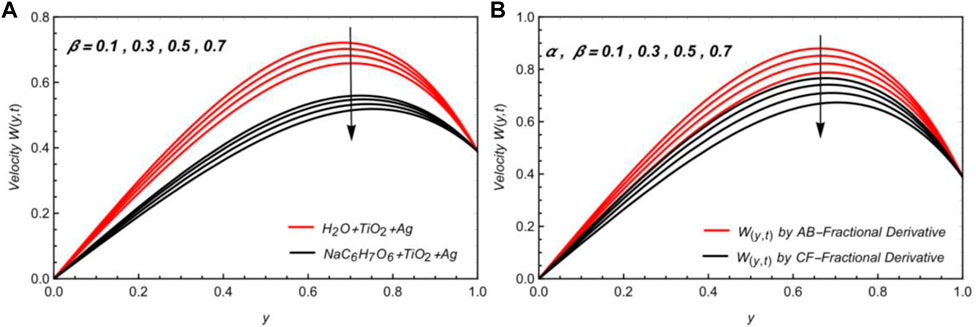
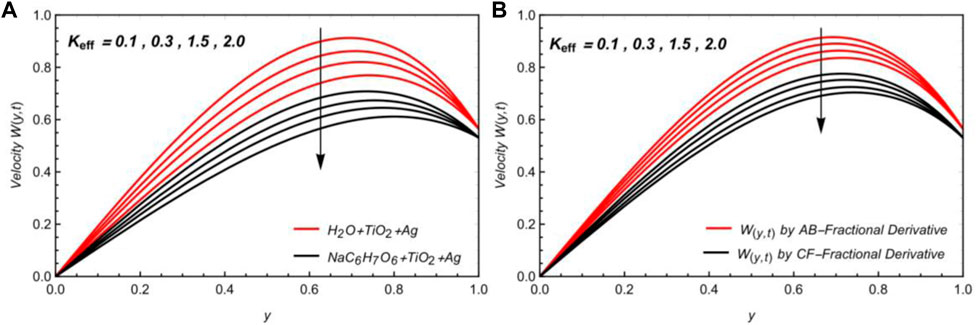
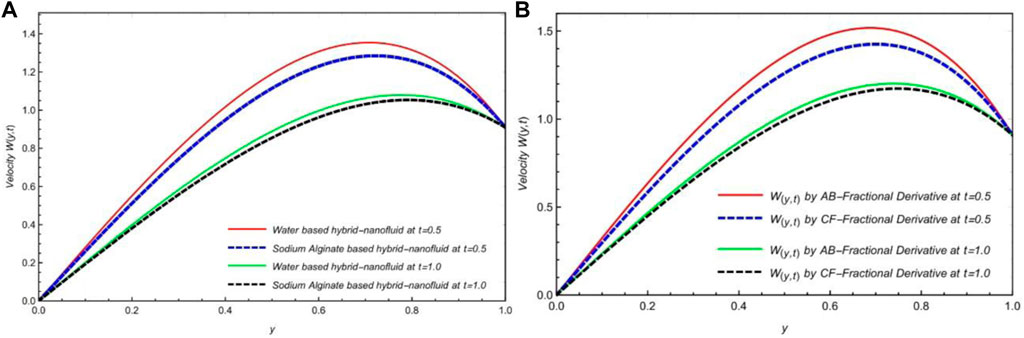
Figure 4A shows that the velocity profile is reduced by increasing β, which is due to the effect of the Mittage–Leffler kernel. Figure 4B shows the effects of
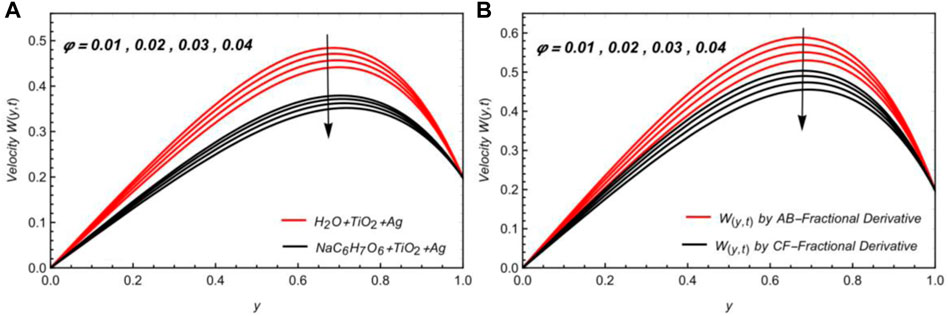
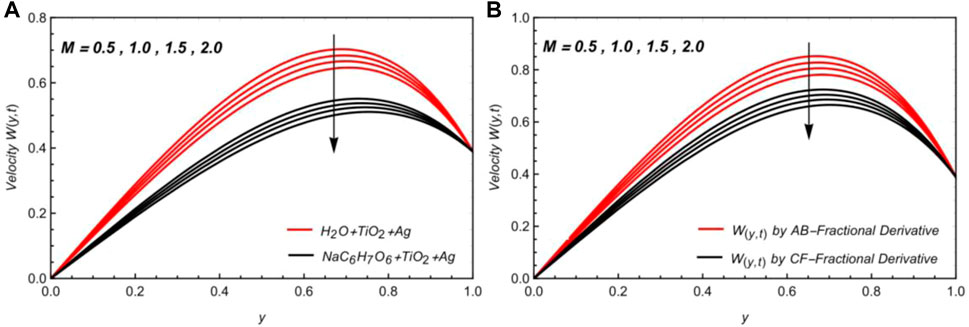
Figures 6A, B represent the velocity diagrams to understand the influence of
The Brinkman parameter
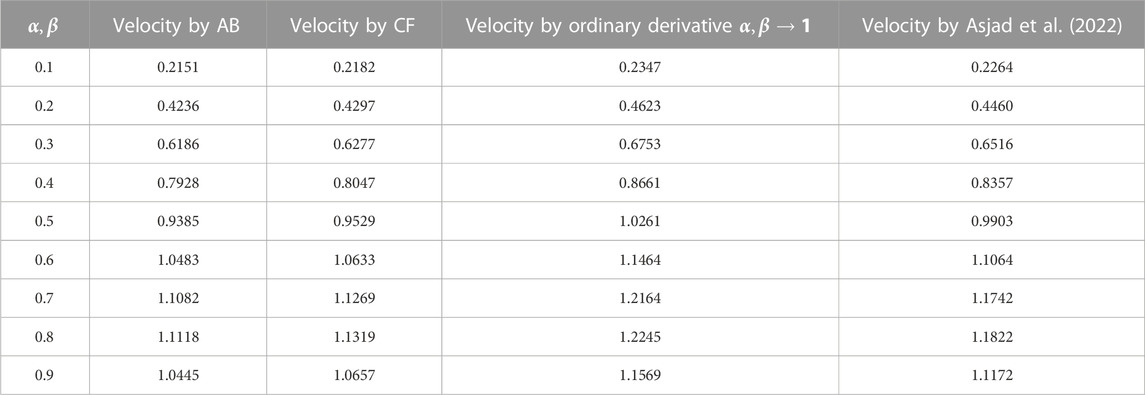
TABLE 6. Numerical analysis of attained results with the ordinary derivative and Asjad et al. (2022).
7 Conclusion
The free convective flow of an unsteady and incompressible Brinkman-type flow mixed with
• This approach may be broadened to include more varied physical science categories with intricate geometries.
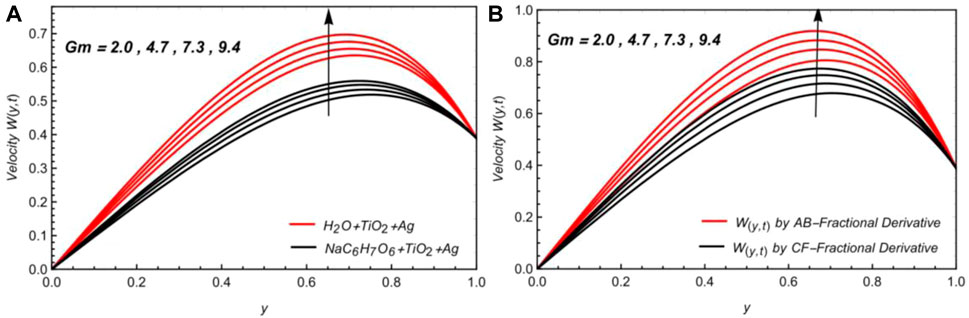
• The enhancing value of
• The bioconvection profile also decelerates by enhancing the value of fractional constraints.
• The momentum field is enhanced by the parameter
• The fractional parameter can control the momentum and thermal boundary layer thickness.
• The discovered solutions can aid in accurately interpreting actual data and serve as a tool for testing potential approximations of solutions as necessary.
• The results of Asjad et al. (2022) and the overlap of both curves from the numerical scheme verify the conclusions of this investigation.
The subsequent recommendations are based on methods, expansions, geometries, and analyses and are intended to indicate a future extension of the issue that this study examines. For example, a horizontal plate of constant length and linear velocity may be used to assess the current issue. A Keller Box scheme analysis of the same issue is also possible.
Data availability statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author contributions
Conceptualization, SME, SE, AR, and UK; methodology, SE, AR, and UK; software, NN, SE, AA, and UK; validation, NN, SE, AR, and UK; formal analysis, AR, SE, and AA; investigation, UK and AA; resources, AR; data curation, AR; writing—original draft preparation, NN, SME, UK, and SE; writing—review and editing, NN, SME, and AA; visualization, SME; supervision, UK; project administration, AA and SE; funding acquisition, SE and AA. All authors have read and agreed to the published version of the manuscript.
Funding
This work received support from Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R163), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. In addition, this study is also funded by Prince Sattam bin Abdulaziz University project number (PSAU/2023/R/1444).
Acknowledgments
The authors are thankful for the support of Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R163), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. Also, this work is supported via funding from Prince Sattam bin Abdulaziz University project number (PSAU/2023/R/1444).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Abbreviations
References
Akhtar, S. (2016). Flows between two parallel plates of couple stress fluids with time-fractional Caputo and Caputo-Fabrizio derivatives. Eur. Phys. J. Plus 131 (11), 401–413. doi:10.1140/epjp/i2016-16401-3
Alhussain, Z. A., Renuka, A., and Muthtamilselvan, M. (2021). A magneto-bioconvective and thermal conductivity enhancement in nanofluid flow containing gyrotactic microorganism. Case Stud. Therm. Eng. 23, 100809. doi:10.1016/j.csite.2020.100809
Ali, Q., Al-Khaled, K., Khan, M. I., Khan, S. U., Raza, A., Oreijah, M., et al. (2022). Diffusion phenomenon for natural convection flow of classical hartmann problem due to a cylindrical tube by generalized fourier’s theories: A fractional analysis. Int. J. Mod. Phys. B, 2350104. doi:10.1142/s0217979223501047
Ali, Q., Al-Khaled, K., Omar, J., Raza, A., Khan, S. U., Khan, M. I., et al. (2022). Analysis for advection–diffusion problem subject to memory effects and local and nonlocal kernels: A fractional operators approach. Int. J. Mod. Phys. B, 2350099. doi:10.1142/s0217979223500996
Anuar, N. S., Bachok, N., Turkyilmazoglu, M., Arifin, N. M., and Rosali, H. (2020). Analytical and stability analysis of MHD flow past a nonlinearly deforming vertical surface in Carbon Nanotubes. Alexandria Eng. J. 59 (1), 497–507. doi:10.1016/j.aej.2020.01.024
Arif, M., Ali, F., Sheikh, N. A., Khan, I., and Nisar, K. S. (2019). Fractional model of couple stress fluid for generalized Couette flow: A comparative analysis of atangana–baleanu and caputo–fabrizio fractional derivatives. IEEE Access 7, 88643–88655. doi:10.1109/access.2019.2925699
Ashwinkumar, G., Samrat, S., and Sandeep, N. (2021). Convective heat transfer in MHD hybrid nanofluid flow over two different geometries. Int. Commun. Heat Mass Transf. 127, 105563. doi:10.1016/j.icheatmasstransfer.2021.105563
Asjad, M. I., Ikram, M. D., Ali, R., Baleanu, D., and Alshomrani, A. S. (2020). New analytical solutions of heat transfer flow of clay-water base nanoparticles with the application of novel hybrid fractional derivative. Therm. Sci. 24 (1), 343–350. doi:10.2298/tsci20s1343a
Asjad, M. I., Ikram, M. D., Sarwar, N., Muhammad, T., Sivasankaran, S., and Subaihi, S. A. A. (2022). Analysis of fractional bioconvection with hybrid nanoparticles in Channel flow. Math. Problems Eng. 2022, 1–14. doi:10.1155/2022/8600591
Asjad, M. I., Sarwar, N., Ali, B., Hussain, S., Sitthiwirattham, T., and Reunsumrit, J. (2021). Impact of bioconvection and chemical reaction on MHD nanofluid flow due to exponential stretching sheet. Symmetry 13 (12), 2334. doi:10.3390/sym13122334
Atangana, A., and Baleanu, D. (2016). New fractional derivatives with nonlocal and non-singular kernel: Theory and application to heat transfer model. arXiv preprint arXiv:1602.03408.
Atangana, A., and Nieto, J. J. (2015). Numerical solution for the model of RLC circuit via the fractional derivative without singular kernel. Adv. Mech. Eng. 7 (10), 168781401561375. doi:10.1177/1687814015613758
Atangana, A. (2016). On the new fractional derivative and application to nonlinear Fisher’s reaction–diffusion equation. Appl. Math. Comput. 273, 948–956. doi:10.1016/j.amc.2015.10.021
Bräuer, F., Trautner, E., Hasslberger, J., Cifani, P., and Klein, M. (2021). Turbulent bubble-laden channel flow of power-law fluids: A direct numerical simulation study. Fluids 6 (1), 40. doi:10.3390/fluids6010040
Caputo, M., and Fabrizio, M. (2015). A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 1 (2), 73–85.
Chu, Y. M., Bilal, S., and Hajizadeh, M. R. (2020). Mathematical methods in the applied sciences.Hybrid ferrofluid along with MWCNT for augmentation of thermal behavior of fluid during natural convection in a cavity
D’Ippolito, A., Calomino, F., Alfonsi, G., and Lauria, A. (2021). Flow resistance in open channel due to vegetation at reach scale: A review. Water 13 (2), 116. doi:10.3390/w13020116
Farooq, U., Waqas, H., Khan, M. I., Khan, S. U., Chu, Y.-M., and Kadry, S. (2021). Thermally radioactive bioconvection flow of Carreau nanofluid with modified Cattaneo-Christov expressions and exponential space-based heat source. Alexandria Eng. J. 60 (3), 3073–3086. doi:10.1016/j.aej.2021.01.050
Ge-JiLe, H., Waqas, H., Khan, S. U., Khan, M. I., Farooq, S., and Hussain, S. (2021). Three-dimensional radiative bioconvective flow of a sisko nanofluid with motile microorganisms. Coatings 11 (3), 335. doi:10.3390/coatings11030335
Guo, B., Raza, A., Al-Khaled, K., Khan, S. U., Farid, S., Wang, Y., et al. (2021). Fractional-order simulations for heat and mass transfer analysis confined by elliptic inclined plate with slip effects: A comparative fractional analysis. Case Stud. Therm. Eng. 28, 101359. doi:10.1016/j.csite.2021.101359
Haq, S. U., Khan, M. A., Khan, Z. A., and Ali, F. (2020). MHD effects on the channel flow of a fractional viscous fluid through a porous medium: An application of the Caputo-Fabrizio time-fractional derivative. Chin. J. Phys. 65, 14–23. doi:10.1016/j.cjph.2020.02.014
Ibraheem, G. H., Turkyilmazoglu, M., and Al-Jawary, M. (2022). Novel approximate solution for fractional differential equations by the optimal variational iteration method. J. Comput. Sci. 64, 101841. doi:10.1016/j.jocs.2022.101841
Ikram, M. D., Asjad, M. I., Akgül, A., and Baleanu, D. (2021). Effects of hybrid nanofluid on novel fractional model of heat transfer flow between two parallel plates. Alexandria Eng. J. 60 (4), 3593–3604. doi:10.1016/j.aej.2021.01.054
Mayeli, P., and Sheard, G. J. (2021). Buoyancy-driven flows beyond the boussinesq approximation: A brief review. Int. Commun. Heat Mass Transf. 125, 105316. doi:10.1016/j.icheatmasstransfer.2021.105316
Platt, J. R. (1961). Bioconvection patterns" in cultures of free-swimming organisms. Science 133 (3466), 1766–1767. doi:10.1126/science.133.3466.1766
Podlubny, I. (1999). An introduction to fractional derivatives, fractional differential equations, to methods of their solution and some of their applications. Math. Sci. Eng. 198, 340.
Ramzan, M., Gul, H., Kadry, S., and Chu, Y.-M. (2021). Role of bioconvection in a three dimensional tangent hyperbolic partially ionized magnetized nanofluid flow with Cattaneo-Christov heat flux and activation energy. Int. Commun. Heat Mass Transf. 120, 104994. doi:10.1016/j.icheatmasstransfer.2020.104994
Raza, A., Almusawa, M. Y., Ali, Q., Haq, A. U., Al-Khaled, K., and Sarris, I. E. (2022). Solution of water and sodium alginate-based casson type hybrid nanofluid with slip and sinusoidal heat conditions: A prabhakar fractional derivative approach. Symmetry 14 (12), 2658. doi:10.3390/sym14122658
Raza, A., Khan, I., Farid, S., My, C. A., Khan, A., and Alotaibi, H. (2021). Non-singular fractional approach for natural convection nanofluid with Damped thermal analysis and radiation. Case Stud. Therm. Eng. 28, 101373. doi:10.1016/j.csite.2021.101373
Raza, A., Khan, S. U., Khan, M. I., Farid, S., Muhammad, T., Khan, M. I., et al. (2021). Fractional order simulations for the thermal determination of graphene oxide(GO)and molybdenum disulphide(MoS2)nanoparticles with slip effects. Case Stud. Therm. Eng. 28, 101453. doi:10.1016/j.csite.2021.101453
Raza, A., Khan, U., Eldin, S. M., Alotaibi, A. M., Elattar, S., Prasannakumara, B. C., et al. (2019). Significance of free convection flow over an oscillating inclined plate induced by nanofluid with porous medium: The case of the prabhakar fractional approach. Micromachines 13 (11), 2019. doi:10.3390/mi13112019
Riaz, S., Amir, M., Memon, I. Q., Ali, Q., and Abro, K. A. (2022). A comparative study for solidification of nanoparticles suspended in nanofluids through non-local kernel approach. Arabian J. Sci. Eng., 1–19. doi:10.1007/s13369-022-07493-y
Samrat, S., Ashwinkumar, G., and Sandeep, N. (2022). Simultaneous solutions for convective heat transfer in dusty-nano-and dusty-hybrid nanoliquids. Proc. Institution Mech. Eng. Part E J. Process Mech. Eng. 236 (2), 473–479.
Saqib, M., Shafie, S., Khan, I., Chu, Y.-M., and Nisar, K. S. (2020). Symmetric MHD channel flow of nonlocal fractional model of BTF containing hybrid nanoparticles. Symmetry 12 (4), 663. doi:10.3390/sym12040663
Sulochana, C., Aparna, S., and Sandeep, N. (2020). Magnetohydrodynamic MgO/CuO-water hybrid nanofluid flow driven by two distinct geometries. Heat. Transf. 49 (6), 3663–3682. doi:10.1002/htj.21794
Tarasov, V. E. (2019). On history of mathematical economics: Application of fractional calculus. Mathematics 7 (6), 509. doi:10.3390/math7060509
Turkyilmazoglu, M., and Altanji, M. (2023). Fractional models of falling object with linear and quadratic frictional forces considering Caputo derivative. Chaos, Solit. Fractals 166, 112980. doi:10.1016/j.chaos.2022.112980
Türkyilmazoğlu, M. (2021). Magnetohydrodynamic moving liquid plug within a microchannel: Analytical solutions. J. BIOMECHANICAL ENGINEERING-TRANSACTIONS ASME 143 (1), 011012. doi:10.1115/1.4048713
Turkyilmazoglu, M. (2022). Transient and passage to steady state in fluid flow and heat transfer within fractional models. Int. J. Numer. Methods Heat Fluid Flow 33, 728–750. no. ahead-of-print. doi:10.1108/hff-04-2022-0262
Waini, I., Ishak, A., and Pop, I. (2019). Hybrid nanofluid flow and heat transfer past a vertical thin needle with prescribed surface heat flux. Int. J. Numer. Methods Heat Fluid Flow 29 (12), 4875–4894. doi:10.1108/hff-04-2019-0277
Yusuf, T. A., Mabood, F., Prasannakumara, B., and Sarris, I. E. (2021). Magneto-bioconvection flow of Williamson nanofluid over an inclined plate with gyrotactic microorganisms and entropy generation. Fluids 6 (3), 109. doi:10.3390/fluids6030109
Zhang, J., Raza, A., Khan, U., Ali, Q., Zaib, A., Weera, W., et al. (2022). Thermophysical study of oldroyd-B hybrid nanofluid with sinusoidal conditions and permeability: A prabhakar fractional approach. Fractal Fract. 6 (7), 357. doi:10.3390/fractalfract6070357
Zheng, Y., Yang, H., Mazaheri, H., Aghaei, A., Mokhtari, N., and Afrand, M. (2021). An investigation on the influence of the shape of the vortex generator on fluid flow and turbulent heat transfer of hybrid nanofluid in a channel. J. Therm. Analysis Calorim. 143 (2), 1425–1438. doi:10.1007/s10973-020-09415-2
Keywords: hybrid nanofluid, parallel plates, Mittage–Leffler function, magnetohydrodynamics, AB and CF derivatives
Citation: Raza A, Nigar N, Khan U, Elattar S, Eldin SM and Abed AM (2023) Comparative investigation of fractional bioconvection and magnetohydrodynamic flow induced by hybrid nanofluids through a channel. Front. Mater. 10:1143612. doi: 10.3389/fmats.2023.1143612
Received: 13 January 2023; Accepted: 24 January 2023;
Published: 16 February 2023.
Edited by:
Ali Saleh Alshomrani, King Abdulaziz University, Saudi ArabiaReviewed by:
Mustafa Turkyilmazoglu, Hacettepe University, TürkiyePrasannakumara B. C., Davangere University, India
Copyright © 2023 Raza, Nigar, Khan, Elattar, Eldin and Abed. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Umair Khan, dW1haXJraGFuQGliYS1zdWsuZWR1LnBr
 Ali Raza
Ali Raza Niat Nigar2
Niat Nigar2 Umair Khan
Umair Khan Samia Elattar
Samia Elattar Sayed M. Eldin
Sayed M. Eldin