
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Mater. , 29 April 2022
Sec. Metamaterials
Volume 9 - 2022 | https://doi.org/10.3389/fmats.2022.901794
This article is part of the Research Topic Editors’ Showcase: Metamaterials View all 5 articles
Phase-gradient metasurfaces (PGMs) have provided unprecedented opportunities for manipulating light. Here, we reexamine ordinary and well-studied subwavelength metallic gratings (OMGs) from the concept of PGMs to provide more insight into their diffraction properties. We will show that due to the existence of gauge invariance in PGMs, i.e., the diffraction law of PGMs is independent of the choice of initial value of abrupt phase shift that induces the phase gradient, the well-studied OMGs can be regarded as a PGM strictly, with its diffraction properties can be fully predicted by generalized diffraction law with phase gradient. In particular, the generalized diffraction law reveals that the phase gradient plays a significant role in the famous effect of Wood’s anomalies and Rayleigh’s conjecture.
In recent years, Phase-gradient metasurfaces (PGMs) have been reported as a way to manipulate electromagnetic (EM) wave propagation, leading to numerous effects or applications (Yu et al., 2011; Kildishev et al., 2013; Xu et al., 2016; Sun et al., 2019). These include efficient focusing (Arbabi et al., 2017), ultrathin cloaking (Ni et al., 2015), photonic spin Hall effects (Yin et al., 2013), metalenses (Chen et al., 2012; Wang et al., 2018), wavefront control (Xie et al., 2014; Ra’di et al., 2017; Fu et al., 2019a), and others (Sun et al., 2012; Li et al., 2015; Tymchenko et al., 2015; Khorasaninejad et al., 2016; Hu et al., 2019). PGMs are periodic arrays of a carefully designed supercell with m unit cells (m is an integer) that discretely introduce a covering 2π abrupt phase shift (APS)
Wood anomalies are well-known effects in the optics community. They were first discovered by Wood in 1902 in experiments on reflection-type OMG (Wood, 1902) and have been investigated and attracted much attention from scientists for more than a century (Rayleigh, 1907; Fano, 1941; Hessel and Oliner, 1965; Maradudin et al., 2016). They have obvious sudden and intense variations in the reflectance/transmittance of various diffracted orders in certain narrow frequency bands or alternatively in a certain narrow range of incident angles for a fixed operating frequency (Maradudin et al., 2016). They are termed anomalies, as ordinary grating theory cannot explain them well. Various efforts (Rayleigh, 1907; Fano, 1941; Hessel and Oliner, 1965) have been made over the years to understand Wood’s anomalies. For instance, Ugo Fano attributed them to the excitations of surface plasmons in periodically corrugated metal interfaces (Fano, 1941). Rayleigh proposed a well-known interpretation based on his conjecture that these anomalies occur at the wavelength at which a diffracted order appears or disappears at a grazing angle (Rayleigh, 1907). Specifically, for the nth diffracted order, the anomaly occurs at the wavelength (Rayleigh, 1907; Maradudin et al., 2016),
where p is the period of grating,
In this work, we start from the gauge invariance of PGMs, revisit the widely studied OMG from the concept of PGMs and present insight into Rayleigh’s conjecture. We will show that ordinary grating theory cannot accurately describe OMG diffractions. In contrast, only the diffraction law including the phase gradient can be used to fully determine their diffraction features. In this diffraction law, the diffraction order of n = 1 is the lowest order, while the 0th order is a higher diffraction order and is difficult to couple. This result completely contrasts with the ordinary understanding of the diffraction law and fully explains the conjecture of WR anomalies, as shown in Eq. 1. More importantly, we also show that WR anomalies can be seen in any OMG, but the physical mechanism can be understood more deeply and clearly by the gauge invariance in a PGM system. Our findings provide a way to study the physics in OMGs from the concept of PGMs, bridging the gap of two fields of optical metasurfaces and plasmonics.
A typical reflection-type PGM with m = 2 is shown in Figure 1A, a textured metallic grating made of a periodic repeated supercell with a period of p. Each supercell contains two unit cells of different grooves. The width of each unit cell is
where v is an integer, G = 2π/p is the reciprocal vector, and
where
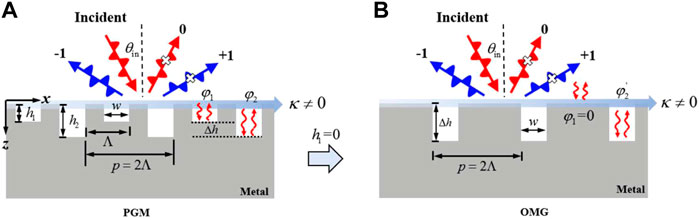
FIGURE 1. (A) Schematic diagram of reflection-type phase-gradient metagrating (PGM) in air, where one period is composed of two air grooves with depths h1 and h2. A TM wave incident to the PGM with an incident angle
In fact, the initial value of APS is not unique for the design of PGMs. If we take the following transformation,
where
With this global gauge invariance in PGMs, we choose
To further reveal the diffraction features, theoretical analyses were performed for the designed PGM and OMG. The total magnetic field in the air region (
where
To clearly illustrate our idea, we first consider a PGM with
In Eq. 7, the order
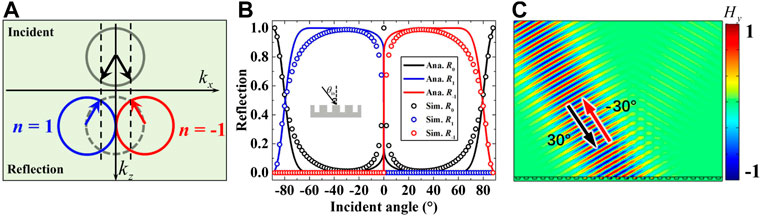
FIGURE 2. The case of
Similar results are obtained for other phase gradients, i.e.,
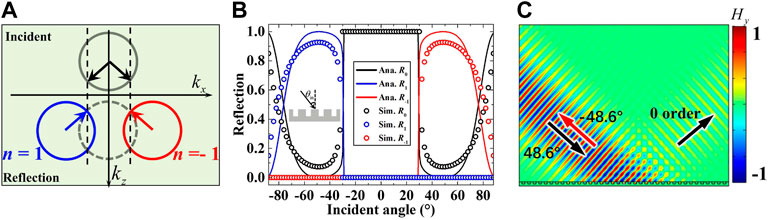
FIGURE 3. The case of
It is believed that WR anomalies can be predicted for any groove depth, but their intensities are closely related to the depth of the grooves. Here, we find that when the APS along the interface covers full
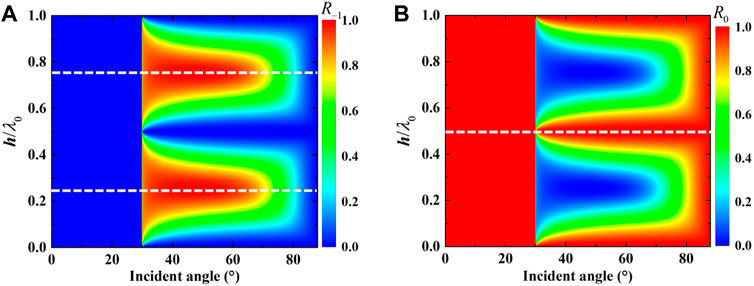
FIGURE 4. Analytical reflectivity as a function of
This physics provides us with guidance to design a high-efficiency retroflector (i.e.,
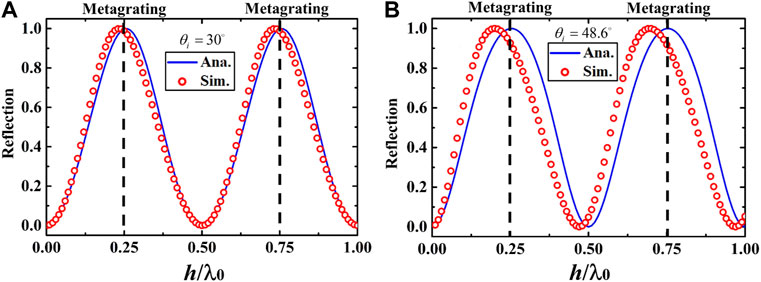
FIGURE 5. Retroreflection efficiency as a function of the groove depth of OMG. (A) is for
In summary, we have demonstrated that the well-studied reflected-type OMGs can be regarded as a PGM with m = 2 due to the existence of gauge invariance in PGMs, with their diffraction properties that can be explained more deeply by the generalized grating diffraction equation derived from PGMs than the ordinary grating diffraction equation. In particular, such a generalized diffraction equation provides insight into Wood-Rayleigh (WR) anomalies, revealing that the phase gradient contributes to the physics of the Rayleigh conjecture. The gauge invariance and our results build a bridge between the fields of metasurfaces and plasmonics, enabling many potential applications of retroreflection, sensors, and wavefront control.
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
JQ and QZ equal contribution including design, research, and simulation. YC and YX contributed by coming up with design ideas and theoretical research.
This work was supported by the National Natural Science Foundation of China (Grant Nos. 11974010 and 12104331), the project funded by the China Postdoctoral Science Foundation (Grant No. 2020M681701), the Postdoctoral Science Foundation of Jiangsu Province (Grant No. 2021K276B) and the Priority Academic Program Development (PAPD) of Jiangsu Higher Education Institutions.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
YX thanks the support from the Key Lab of Modern Optical Technologies of Education Ministry of China, Soochow University. QZ thanks the Postgraduate Research & Practice Innovation Program of Jiangsu Province (No. KYCX21_2936) for this work.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fmats.2022.901794/full#supplementary-material
Aieta, F., Kats, M. A., Genevet, P., and Capasso, F. (2015). Multiwavelength Achromatic Metasurfaces by Dispersive Phase Compensation. Science 347, 1342–1345. doi:10.1126/science.aaa2494
Arbabi, A., Arbabi, E., Horie, Y., Kamali, S. M., and Faraon, A. (2017). Planar Metasurface Retroreflector. Nat. Phot. 11, 415–420. doi:10.1038/nphoton.2017.96
Born, M., and Wolf, E. (1999). Principles of Optics: Electromagnetic Theory of Propagation, Interference, and Diffraction of Light. Cambridge, UK: Cambridge University Press.
Cao, Y., Fu, Y., Zhou, Q., Ou, X., Gao, L., Chen, H., et al. (2019). Mechanism behind Angularly Asymmetric Diffraction in Phase-Gradient Metasurfaces. Phys. Rev. Appl. 12, 024006. doi:10.1103/physrevapplied.12.024006
Cao, Y., Fu, Y., Jiang, J.-H., Gao, L., and Xu, Y. (2021). Scattering of Light with Orbital Angular Momentum from a Metallic Meta-Cylinder with Engineered Topological Charge. ACS Photonics 8, 2027–2032. doi:10.1021/acsphotonics.1c00077
Chen, X., Huang, L., Mühlenbernd, H., Li, G., Bai, B., Tan, Q., et al. (2012). Dual-polarity Plasmonic Metalens for Visible Light. Nat. Commun. 3, 1198. doi:10.1038/ncomms2207
Fano, U. (1941). The Theory of Anomalous Diffraction Gratings and of Quasi-Stationary Waves on Metallic Surfaces (Sommerfeld’s Waves). J. Opt. Soc. Am. 31, 213–222. doi:10.1364/josa.31.000213
Fu, Y., Cao, Y., and Xu, Y. (2019). Multifunctional Reflection in Acoustic Metagratings with Simplified Design. Appl. Phys. Lett. 114, 053502. doi:10.1063/1.5083081
Fu, Y., Shen, C., Cao, Y., Gao, L., Chen, H., Chan, C. T., et al. (2019). Reversal of Transmission and Reflection Based on Acoustic Metagratings with Integer Parity Design. Nat. Commun. 10, 2326. doi:10.1038/s41467-019-10377-9
Hessel, A., and Oliner, A. A. (1965). A New Theory of Wood's Anomalies on Optical Gratings. Appl. Opt. 4, 1275–1297. doi:10.1364/ao.4.001275
Hu, G., Hong, X., Wang, K., Wu, J., Xu, H.-X., Zhao, W., et al. (2019). Coherent Steering of Nonlinear Chiral Valley Photons with a Synthetic Au-WS2 Metasurface. Nat. Photonics 13, 467–472. doi:10.1038/s41566-019-0399-1
Khorasaninejad, M., Chen, W. T., Devlin, R. C., Oh, J., Zhu, A. Y., and Capasso, F. (2016). Metalenses at Visible Wavelengths: Diffraction Limited Focusing and Subwavelength Resolution Imaging. Science 352, 1190–1194. doi:10.1126/science.aaf6644
Kildishev, A. V., Boltasseva, A., and Shalaev, V. M. (2013). Planar Photonics with Metasurfaces. Science 339, 1232009. doi:10.1126/science.1232009
Li, G., Chen, S., Pholchai, N., Reineke, B., Wong, P. W. H., Pun, E. Y. B., et al. (2015). Continuous Control of the Nonlinearity Phase for Harmonic Generations. Nat. Mater. 14, 607–612. doi:10.1038/nmat4267
Maradudin, A. A., Simonsen, I., Polanco, J., and Fitzgerald, R. M. (2016). Rayleigh and Wood Anomalies in the Diffraction of Light from a Perfectly Conducting Reflection Grating. J. Opt. 18, 024004. doi:10.1088/2040-8978/18/2/024004
Ni, X., Wong, Z. J., Mrejen, M., Wang, Y., and Zhang, X. (2015). An Ultrathin Invisibility Skin Cloak for Visible Light. Science 349, 1310–1314. doi:10.1126/science.aac9411
Ra’di, Y., and Alù, A. (2018). Reconfigurable Metagratings. ACS Photonics 5, 1779–1785. doi:10.1021/acsphotonics.7b01528
Ra’di, Y., Sounas, D. L., and Alù, A. (2017). Metagratings: beyond the Limits of Graded Metasurfaces for Wave Front Control. Phys. Rev. Lett. 119, 067404. doi:10.1103/physrevlett.119.067404
Sun, S., He, Q., Xiao, S., Xu, Q., Li, X., and Zhou, L. (2012). Gradient-index Meta-Surfaces as a Bridge Linking Propagating Waves and Surface Waves. Nat. Mater. 11, 426–431. doi:10.1038/nmat3292
Sun, S., He, Q., Hao, J., Xiao, S., and Zhou, L. (2019). Electromagnetic Metasurfaces: Physics and Applications. Adv. Opt. Phot. 11, 380–479. doi:10.1364/aop.11.000380
Tymchenko, M., Gomez-Diaz, J. S., Lee, J., Nookala, N., Belkin, M. A., and Alù, A. (2015). Gradient Nonlinear Pancharatnam-Berry Metasurfaces. Phys. Rev. Lett. 115, 207403. doi:10.1103/physrevlett.115.207403
Wang, S., Wu, P. C., Su, V. –C., Lai, Y. –C., Chen, M. –K., Kuo, H. Y., et al. (2018). A Broadband Achromatic Metalens in the Visible. Nat. Nanotech. 13, 227–232. doi:10.1038/s41565-017-0052-4
Wood, R. W. (1902). XLII. On a Remarkable Case of Uneven Distribution of Light in a Diffraction Grating Spectrum. Lond. Edinb. Dublin Philos. Mag. J. Sci. 4, 396–402. doi:10.1080/14786440209462857
Xie, Y., Wang, W., Chen, H., Konneker, A., Popa, B.-I., and Cummer, S. A. (2014). Wavefront Modulation and Subwavelength Diffractive Acoustics with an Acoustic Metasurface. Nat. Commun. 5, 5553. doi:10.1038/ncomms6553
Xu, Y., Fu, Y., and Chen, H. (2015). Steering Light by a Sub-Wavelength Metallic Grating from Transformation Optics. Sci. Rep. 5, 12219. doi:10.1038/srep12219
Xu, Y., Fu, Y., and Chen, H. (2016). Planar Gradient Metamaterials. Nat. Rev. Mater. 1, 16067. doi:10.1038/natrevmats.2016.67
Yin, X., Ye, Z., Rho, J., Wang, Y., and Zhang, X. (2013). Photonic Spin Hall Effect at Metasurfaces. Science 339, 1405–1407. doi:10.1126/science.1231758
Keywords: phase gradient metasurfaces, diffraction, gauge invariance, ordinary metallic gratings, wood anomalies
Citation: Quan J, Zhou Q, Cao Y and Xu Y (2022) Understanding of Wood Anomalies in Metallic Gratings From Phase Gradient Metasurfaces. Front. Mater. 9:901794. doi: 10.3389/fmats.2022.901794
Received: 22 March 2022; Accepted: 14 April 2022;
Published: 29 April 2022.
Edited by:
Huanyang Chen, Xiamen University, ChinaCopyright © 2022 Quan, Zhou, Cao and Xu. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Yadong Xu, eWR4dUBzdWRhLmVkdS5jbg==
†These authors have contributed equally to this work
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.