Study on the mass transfer of SO2 in ammonia-based desulfurization process

The coal hydrogen production process requires high-temperature steam as a reactant. Coal-fired boilers are usually used to provide high-temperature steam. The ammonia-based desulphurization process is an effective technology to remove SO₂ in the coal-fired flue gas. However, the mass transfer coefficient of SO₂ in such an engineered process is not readily available in the literature. In this paper, experiments were carried out to investigate the mass transfer process of SO₂ absorption. Combing calculating the air and liquid contact area of the droplet and liquid membrane on the tower wall, the mass transfer coefficient contain parameters pH, gas flue rate and liquid to gas ratio was proposed. The comparison results show that the calculated SO₂ mass transfer rate agrees well with the measured data. The relative error of the calculated and experimental values was less than ±20%. This proposed mass transfer coefficient appears to be beneficial for the design and operation of ammonia-based desulfurization FGD system.

1 Introduction

Sulfur dioxide (SO₂) from coal fired power plants is known as a major air pollutant (Sun et al., 2010; Valle-Zermeño et al., 2014). The emission standard of SO₂ is increasingly strict in many countries and the flue gas is required to be treated before being discharged into the atmosphere. Among several desulfurization technologies, ammonia absorption based treatment process appears to be very attractive, as the resulting ammonium sulfate is a valuable by-product while meeting the emission standard of SO₂(Gao et al., 2010; Jia et al., 2010; Wang et al., 2015). Compared with the widely used limestone-gypsum desulfurization method, the investment of ammonia desulfurization technology is about 23% lower and the operating cost is about 25% lower. The chemical reactions involved in the ammonia-based desulfurization processes are as following (He et al., 2003; Long et al., 2006; Jia et al., 2015):

${\mathrm{S}\mathrm{O}}_2+2{\mathrm{N}\mathrm{H}}_3+{\mathrm{H}}_2\mathrm{O}\leftrightarrow {\left({\mathrm{N}\mathrm{H}}_4\right)}_2{\mathrm{S}\mathrm{O}}_3(1)$

${\left({\mathrm{N}\mathrm{H}}_4\right)}_2{\mathrm{S}\mathrm{O}}_3+{\mathrm{S}\mathrm{O}}_2+{\mathrm{H}}_2\mathrm{O}\leftrightarrow 2{\mathrm{N}\mathrm{H}}_4\mathrm{H}{\mathrm{S}\mathrm{O}}_3(2)$

${\mathrm{N}\mathrm{H}}_4\mathrm{H}{\mathrm{S}\mathrm{O}}_3+{\mathrm{N}\mathrm{H}}_3\leftrightarrow {\left({\mathrm{N}\mathrm{H}}_4\right)}_2{\mathrm{S}\mathrm{O}}_3(3)$

${\mathrm{N}\mathrm{H}}_4\mathrm{H}{\mathrm{S}\mathrm{O}}_3+1/2{\mathrm{O}}_2\to {\mathrm{N}\mathrm{H}}_4\mathrm{H}{\mathrm{S}\mathrm{O}}_4(4)$

${\left({\mathrm{N}\mathrm{H}}_4\right)}_2{\mathrm{S}\mathrm{O}}_3+1/2{\mathrm{O}}_2\to {\left({\mathrm{N}\mathrm{H}}_4\right)}_2{\mathrm{S}\mathrm{O}}_4(5)$

In ammonia-based desulfurization process, removal of SO₂ from flue gas by absorption is a major procedure (Ryuichi and Yukio, 1985). Spray scrubber is considered to be among the simplest, most economical and efficient absorption device for air pollution treatment (Javed et al., 2006; Zhong et al., 2008). In ammonia-based desulfurization process, the spray scrubber is also widely employed for removing SO₂ from flue gas. The mass transfer rate of SO₂ across the gas-liquid interface is an important system design parameter (Ryuichi and Yukio, 1985). The mass transfer coefficient can reflect the influence of operational condition on SO₂ absorption rate, and theoretically guide the design and operation of spray scrubber, so as to make the design and operation of ammonia desulfurization system more accurate, and reduce the investment and operation cost. Accordingly, the mass transfer coefficient of SO₂ absorption has attracted much attention of researchers.

By correlating with falling velocity of droplet, Henry constant and physical properties of solution etc., Ryuichi developed a liquid-film mass transfer coefficient of the SO₂ absorption by water droplets. The results of the research show that experimental values are smaller than the theoretical ones at contact times longer than 0.4 s (Ryuichi and Yukio, 1985). Hu also investigated the mass transfer coefficient by correlating with dimensionless number Re, Sc and We (Hu et al., 2008). Marion deduced a gas-film mass transfer coefficient of the SO₂ absorption by water drop, and the mass transfer coefficient was a function of the Sherwood number, molecular diffusivity and droplet diameter (Marion et al., 2006). Hixon and Scott investigated the gas phase volumetric mass transfer coefficient of SO₂ absorption by sodium hydroxide solution, and the empirical equation contains parameters of gas flow rate, liquid flow rate and tower height was proposed (Hixon and Scott, 1935). Schmidt and Stichlmair conducted a research to investigate SO₂ absorption in a spray scrubber and the results show that gas velocity has little influence on the mass transfer rate (Schmidt and Stichlmair, 1991). Milene investigated the absorption of SO₂ by sodium hydroxide solution in a lab-pilot spray tower. The volumetric mass transfer coefficient expression has also been proposed, including three parameters: diameter of the spray nozzle orifice and exit velocity of liquid from the orifice, superficial gas and liquid velocities (Milene and Waldir, 2013).

Dimensionless numbers including Schmidt number Sc, Reynolds number Re and Sherwood number Sh are quantitatively correlated among physicochemical properties of the fluids such as density, viscosity and kinematic viscosity, etc. These physicochemical properties of the fluids are variable along the height of absorption tower during the SO₂ absorption process (Ryuichi and Yukio, 1985). Moreover, the gas-liquid interfacial area in the absorption tower is difficult to measure and the mass transfer coefficient of absorption is generally represented by overall volumetric gas phase mass transfer coefficient (K_Ga, kmol·m⁻²·h⁻¹·Pa⁻¹) (Hixon and Scott, 1935; Milene and Waldir, 2013; Bandyopadhay and Biswas, 1998). However, the specific gas-liquid interfacial area per unit volume of absorption tower is highly depended upon the device and operating conditions. The specific gas-liquid interfacial area per unit volume of absorption tower is diverse in different systems. Although the mass transfer coefficient obtained by correlating with dimensionless number and the volumetric gas phase mass transfer coefficient are important parameters to evaluate the mass transfer process, they could not properly predict mass transfer rate of SO₂ across the gas-liquid interface. On the other hand, extensive studies have been made on the mass transfer coefficient of absorption of SO₂ by water, NaOH or Na₂CO₃ solution, etc., however, there are few investigations about mass transfer coefficient of SO₂ absorption by ammonia solution. Accordingly, an independent study is needed.

Under operating conditions relevant to ammonia-based FGD plants, experiments were conducted to investigate the mass transfer rate of SO₂ absorption by ammonia in a lab-scale spray scrubber in this work. Basing on experiments, a mass transfer coefficient of SO₂ absorption by ammonia was also proposed. This mass transfer coefficient could predict the mass transfer rate of SO₂ across the gas-liquid interface.

2 Experimental

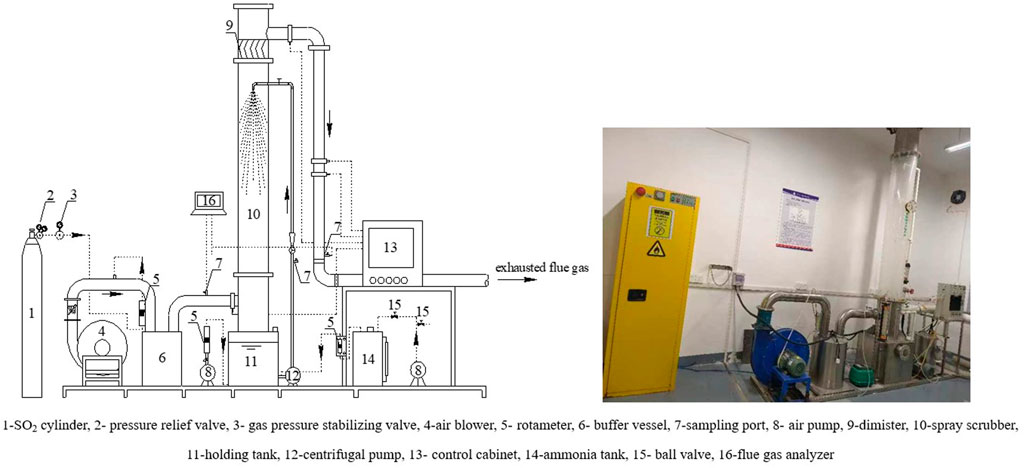
Experiments were performed in a lab-scale spray scrubber. Figure 1 shows the schematic diagram of experimental setup. The spray scrubber was built of plexiglass and stainless steel, with an effective height of absorption zone 2.0 m and inner diameter of 0.3 m. A solid cone spray nozzle with 15º angle opening is installed at the top of the absorption zone. In the buffer vessel, SO₂ was mixed with air to form simulated flue gas. SO₂ (99.99 vol%) was supplied from a gas cylinder and the flow rate was controlled by a pressure stabilizing valve and a rotameter to ensure the desired SO₂ concentration. The simulated flue gas was subsequently introduced into spray scrubber and countercurrently contacted with the descending liquid drops. The liquid drops and its absorbed SO₂ fall into the holding tank located at the bottom of spray scrubber. The absorption liquid in the holding tank was circulated into the spray scrubber by a centrifugal pump. The amount of ammonia (17–20 wt%) was added into the solution at the front of centrifugal pump depended on the pH of solution.

FIGURE 1

FIGURE 1. Diagram of the experimental system for ammonia-based desulfurization.

In this work, experiments were conducted at solution pH level of 5.0–6.0, the gas flow rate ranging from 1.92 to 2.6 m s⁻¹, and the liquid-to-gas ratio was between 3 and 5.5 L m⁻³. The pH of liquid sampled from the sampling port was monitored by pH meter (HANNA, HI198128). The inlet and outlet SO₂ concentrations were monitored using a flue gas analyzer (MRU Vario Plus, Accuracy is ±5 ppm). The simulated flue gas consisted of SO₂ (SO₂ mole fraction is 210 × 10^–6) was stabilized for 1.5 h before measuring the inlet and outlet SO₂ concentration. In order to keep the sulfite concentration constant, a part of the solution was discharged according to the amount of SO₂ absorbed per hour. The total sulfite concentration ($c_{{\mathrm{S}}^{4+}}$) in the solution was about 0.05 mol L⁻¹.

3 Theoretical

3.1 Assumptions

a. The falling drops are considered to be ball in shape and fall along vertical line.

b. The collisions and coalescence between drops are neglected.

c. The radial SO₂ concentration and velocity of flue gas are constant while the axial SO₂ concentration vary along the height of spray scrubber.

d. Temperature is evenly distributed in the spray scrubber.

3.2 Model

The physicochemical equilibrium is assumed at the liquid-gas interface and the mass transfer rate’s equation can be described as Eq. 6 in the process of SO₂ absorption (Colle et al., 2005).

$N=K_G\left(p-p^{*}\right)(6)$

The overall gas-phase mass transfer coefficient K_G could be correlated by Eq. 7 (Liu et al., 2015):

$\frac{1}{K_{\mathrm{G}}}=\frac{1}{k_{\mathrm{G}}}+\frac{1}{H{k}_L}(7)$

Where, k_G is the individual gas phase mass transfer coefficient, k_L is the individual liquid phase mass transfer coefficient and H is the Henry coefficient. SO₂ is easily soluble in water and the numerical value of Henry coefficient H is large. Accordingly, Eq. 7 can be simplified to Eq. 8:

$\frac{1}{K_{\mathrm{G}}}\approx \frac{1}{k_{\mathrm{G}}}(8)$

Combining Eqs.6–8, the mass transfer rate’s equation of SO₂ absorption can be transformed into:

$N=k_G\left(p-p^{*}\right)=k_y\left(y-y^{*}\right)(9)$

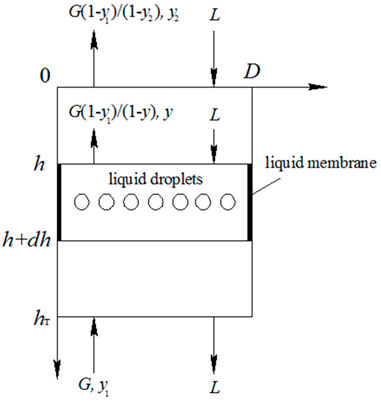
Dividing the absorption zone of spray scrubber into small height incremental volumes (Figure 2), in each element, the material balance for SO₂ absorption could be described as (Colle et al., 2005):

$Na=k_{y}a\cdot \left(S\cdot dz\right)\cdot \left(y-y^{*}\right)=Q\left(1-y_1\right)\frac{y}{1-y}|z+dz-Q\left(1-y_1\right)\frac{y}{1-y}|z(10)$

FIGURE 2

FIGURE 2. Schematic diagram of material balance for SO₂ absorption.

Eq. 10 could be transformed into:

$\frac{k_{y}a\cdot \left(\mathrm{S}\cdot dz\right)\cdot \left(y-y^{*}\right)}{dz}=Q\left(1-y_1\right)\frac{d}{dz}\left(\frac{y}{1-y}\right)(11)$

In ammonia-based desulfurization process, SO₂ is absorbed by dilute ammonia and the total sulfite (SO₂(aq), ${\mathrm{H}\mathrm{S}\mathrm{O}}_3^{-}$ and ${\mathrm{S}\mathrm{O}}_3^{2-}$) is formed in the liquid phase. The distribution coefficients of SO₂(aq) (δ₀), ${\mathrm{H}\mathrm{S}\mathrm{O}}_3^{-}$ (δ₁) and ${\mathrm{S}\mathrm{O}}_3^{2-}$ (δ₂) could be calculated and the distribution coefficients of each species versus pH are shown in Figure 3 (Jia et al., 2010, 2015).

FIGURE 3

FIGURE 3. Distribution coefficient of each species of total sulfite versus pH (T = 298.15K).

In ammonia-based desulfurization process, SO₂ is commonly absorbed in the pH range from 5.0 to 6.0. It can be observed from Figure 3 that the distribution coefficient of SO₂(aq) is nearly zero and the SO₂(aq) concentration in solution could be neglected over the pH range of 5.0–6.0. According to Henry’s Law, it can be concluded that the mole fraction of SO₂ in gas phase is much bigger than the equilibrium mole fraction of SO₂ at gas-liquid interface (y >> y_i). Moreover, the mole fraction of SO₂ in gas phase is much smaller than 1. Therefore, Eq. 11 can be transformed into:

$G\frac{1}{y}dy=k_y\mathit{a}\mathit{d}\mathit{h}(12)$

Integrating Eq. 12 from top to bottom of the absorption zone along the vertical direction, yields:

${\int }_{y_2}^{y_1}G\frac{1}{y}dy={\int }_0^{h_{\mathrm{T}}}{k}_y\mathit{a}\mathit{d}\mathit{h}(13)$

The numerical integration of Eq. 13 allowed to compute the mass transfer rate:

$k_y=\frac{G}{a{h}_{\mathrm{T}}}\ln \left(\frac{y_1}{y_2}\right)(14)$

Where, a is the specific surface area which contains surface area of liquid droplet (A_d) and liquid film (A_m). The specific surface area (a) could be calculated by the following equation:

$a=\left(A_{\mathrm{d}}+A_{\mathrm{m}}\right)/\pi R^2{h}_{\mathrm{T}}(15)$

The surface area of liquid droplet (A_d) in the element can be expressed as:

$A_{\mathrm{d}}=\frac{1}{3600}\times \frac{L_{\mathrm{d}}}{\frac{\pi d^3}{6}}\times \pi d^2\times \frac{dh}{u_{\mathrm{d}}}(16)$

In Eq. 16, d is the Sauter mean diameter of droplets and it can be calculated by Eq. 17; (Wu et al., 1995):

$d=133.0\cdot \left(D/2\right)\cdot W{e}^{-0.74}(17)$

where:

$\mathrm{W}\mathrm{e}=\frac{\rho {\left(u_d^0\right)}^2\left(D/2\right)}{\sigma }(18)$

In Eq. 18, the initial velocity of liquid droplet out of the nozzle $u_d^0$ could be calculated according to the law of conservation of momentum: $m_l{u}_d^0=P_l\pi d_k^2/4$. The equation of motion for describing the movement of falling liquid droplets in the absorption zone could be determined as Eqs.19–22 (Zhong et al., 2008; Michalski, 2000):

$\frac{d{u}_{\mathrm{d}}}{dt}=g\left(\frac{{\rho }_{\mathrm{d}}-{\rho }_g}{{\rho }_{\mathrm{d}}}\right)-\frac{3}{4}\left(\frac{{\rho }_g{\left(u_{\mathrm{d}}-u_g\right)}^2{C}_{\mathrm{d}}}{{\rho }_{\mathrm{d}}g}\right)(19)$

${\int }_0^{t_z}{u}_{\mathrm{d}}dt=dh(20)$

In Eq. 18, the drag coefficient could be calculated by Eq. 20 (Zhong et al., 2008; Michalski, 2000):

$C_d=\frac{24}{{\mathrm{R}\mathrm{e}}_{\mathrm{d}}}\left(1+0.125{\mathrm{R}\mathrm{e}}_{\mathrm{d}}^{0.72}\right)(21)$

where:

${\mathrm{R}\mathrm{e}}_{\mathrm{d}}=\frac{d\left|u_{\mathrm{d}}-u_{\mathrm{g}}\right|{\rho }_{\mathrm{g}}}{{\mu }_{\mathrm{g}}}(22)$

The surface area of liquid film (A_m) in the element could be expressed as:

$A_{\mathrm{m}}=\pi \left(D-2h_{\mathrm{m}}\right)\cdot dh(23)$

The flow of the liquid wall film is assumed to be in the laminar and the thickness of the liquid wall film can be determined as (Dai and Chen, 1988):

$h_{\mathrm{m}}=0.369{\left(\frac{3v^2}{g}\right)}^{\frac{1}{3}}{\mathrm{R}\mathrm{e}}_{\mathrm{m}}^{0.5}(24)$

Combining Eqs.14–16 and Eq. 23 yields:

$k_y=\frac{G}{\frac{(A_{\mathrm{d}}+A_{\mathrm{m}})}{\pi R^2{h}_{\mathrm{T}}}{h}_{\mathrm{T}}}\ln \left(\frac{y_1}{y_2}\right)(25)$

4 Results and discussion

4.1 Determination of the mass transfer coefficient

Frequently, the K_Ga was correlated in the dependency on the flow rate of gas and liquid in literature (Milene and Waldir, 2013). The mass transfer rate of SO₂ absorption by ammonia is influenced by several factors, such as pH of solution (pH), gas flow rate (v) and liquid-to-gas ratio (m). The pH of solution and the gas- and liquid-phase mixing have great influences on the mass transfer rate of SO₂ absorption by ammonia. On basis of experiments, the mass transfer coefficient k_y could be related to pH, v and m by means of multivariable nonlinear regression and the expression is in the following form:

$k_y=k\bullet {\mathrm{p}\mathrm{H}}^a{v}^b{m}^c(26)$

where k, a, b and c are the coefficients for the respective parameters modeled in the proposed equation mentioned above.

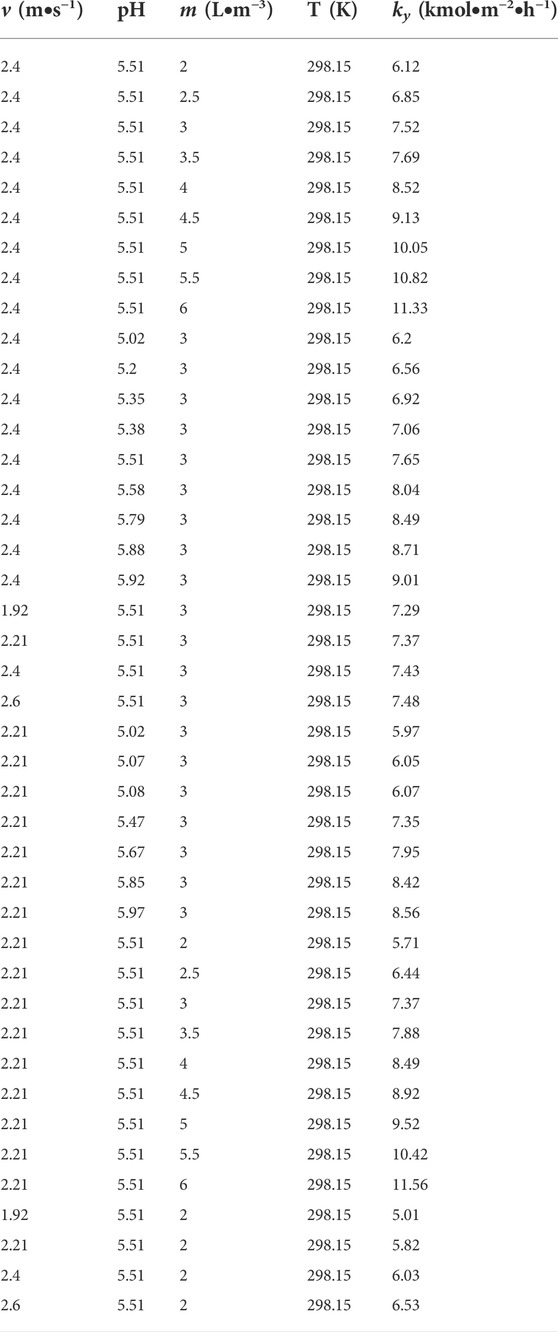
In this work, the inlet and outlet concentration of SO₂ were measured at various operating conditions of pH, v and liquid-to-gas ratio m. Subsequently, the mass transfer rate of SO₂ absorption at different operating conditions were calculated according to Eqs.16, 23 and 25. The experimental conditions and experimental mass transfer rate obtained are presented in Table 1.

TABLE 1

TABLE 1. Experimental mass transfer rate of SO₂ absorption.

Eq. 27 is obtained by taking natural logarithm on both sides of Eq. 26:

${\ln k}_y=\ln k+a\mathrm{l}\mathrm{n}\mathrm{p}\mathrm{H}+b\ln v+c\ln m(27)$

ln(v), ln (pH) and ln(m) are independent variables in the three-variable linear Eq. 27. Basing on data listed in Table 1, the mass transfer coefficient (a, b and c) of SO₂ absorption is related to pH, v and m by means of multivariable linear regression and the expression of Eq. 26 is as follows:

$k_y=5.8\times {10}^{-2}\times v^{0.43}{\mathrm{p}\mathrm{H}}^{2.26}{m}^{0.57}(28)$

4.2 Effect of v on mass transfer performance

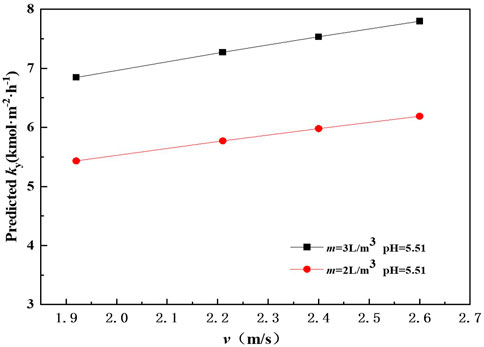
In order to examine the effect of gas flow rate on mass transfer performance, the mass transfer rate was calculated by Eq. 27 at v range from 1.92 to 2.6 m/s. It can be seen from Figure 4 that the predicted mass transfer rate of SO₂ absorption increases as v increases. When pH is 5.51 and m is 2 L m⁻³, the predicted mass transfer rate of SO₂ absorption by ammonia solution is about 5.43 kmol m⁻² h⁻¹ at v 1.92 m s⁻¹. The predicted mass transfer rate reaches 6.19 kmol m⁻² h⁻¹ when u_g increases to 2.6 m s⁻¹ at m 2 L m⁻³. A similar tendency has also been observed by Milene and Waldir (Milene and Waldir, 2013). It could be explained as that raising v results in an increase of gas-liquid mixing. Meanwhile, the residence time of liquid drops in the spray scrubber increase as v increases. An examination of Figure 4 also shows that the predicted mass transfer rate of SO₂ absorption by ammonia at m 3 L m⁻³ is larger than that at m 2 L m⁻³.

FIGURE 4

FIGURE 4. Effect of u_g on mass transfer performance.

Figure 5 compares the mass transfer rate predicted by the proposed correlation Eq. 27 and the experimental data. Approximately 100% of the predicted points lie within an uncertainty of ±10% of the values obtained experimentally. The predicted values agree well with the measured data.

FIGURE 5

FIGURE 5. Deviation of the predicted k_y from the experimental k_y at different u_g.

4.3 Effect of pH on mass transfer performance

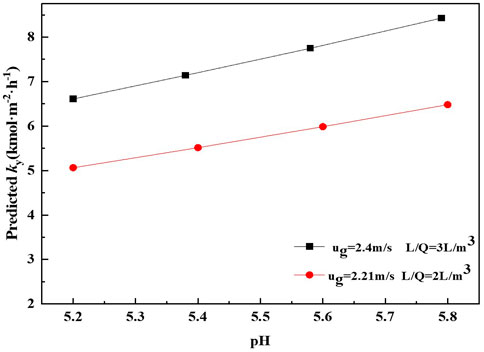
The effect of pH of solution on the mass transfer rate predicted by Eq. 27 is presented in Figure 6.

FIGURE 6

FIGURE 6. Effect of pH on mass transfer performance.

The results show that the mass transfer performance in terms of k_y increases as the pH of solution increases. The similar tendency has also been reported by Mounsef (Mounsef et al., 2015). The possible reason is that the absorption of SO₂ has faster reaction kinetics at higher pH than that at lower pH. The higher the pH of solution, the more sulfite ions could react with SO₂ and hence a more intense mass transfer performance is observed. Regard to the results observed in Figure 6 and Eq. 27, it can be inferred that pH has a significant effect on the mass transfer performance for SO₂ absorption by ammonia. Obviously, it can also be seen from Figure 6 that the mass transfer rate obtained at conditions of v 2.21 m s⁻¹ and m 2 L m⁻³ is lower than that obtained at conditions of v 2.4 m s⁻¹ and m 3 L m⁻³. It can be observed from Eq. 27 that the mass transfer rate is proportional to v and m. Meanwhile, the influence of v on mass transfer rate of SO₂ absorption is more significant than m.

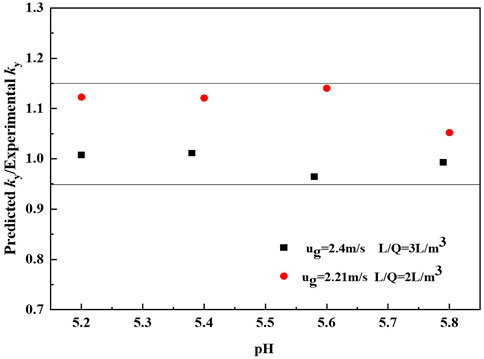
Figure 7 compares the values of predicted k_y and the experimental values. Obviously, nearly 100% of the predicted points lie within an uncertainty of ±15% of the values obtained experimentally.

FIGURE 7

FIGURE 7. Deviation of the predicted k_y from the experimental k_y at different pH of solution.

4.4 Effect of m on mass transfer performance

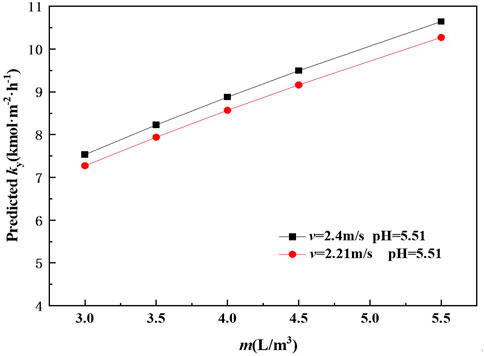
The mass transfer rate of SO₂ absorption by ammonia was also calculated at different liquid-to-gas ratio (m) and the results was present in Figure 8.

FIGURE 8

FIGURE 8. Effect of L/G on mass transfer performance.

Figure 8 shows that the predicted mass transfer rate profile increases as m increases over the range of 3.0–5.5 L m⁻³. When the pH is 5.51 and v is 2.21 m s⁻¹, the predicted mass transfer rate of absorption of SO₂ by ammonia is about 7.53 kmol m⁻²·h⁻¹ at m 3 L m⁻³. The predicted mass transfer rate is about 8.88 kmol m⁻² h⁻¹ as m increases to 4 L m⁻³. The reason for such observation may be explained as, with the increase in the m, the total gas-liquid interfacial area also increased (Ma et al., 2013). However, the power consumption of the recirculating pump increased as m increases. Meanwhile, with the increase in the m, the coalescence of the droplets increased while the specific gas-liquid interfacial area increases slowly. Moreover, Figure 8 also shows that the mass transfer rate obtained at conditions of v 2.21 m s⁻¹ and pH 5.51 is lower than that obtained at conditions of v 2.4 m s⁻¹ and pH 5.51. The possible reason is similar as explained in Sections 4.2 and 4.3.

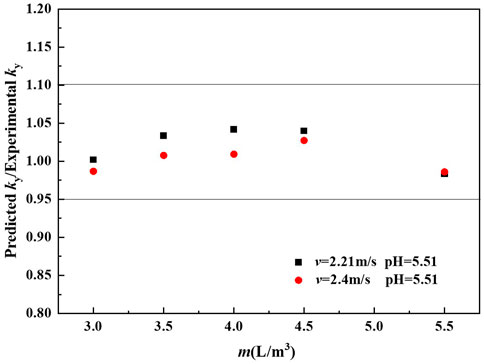
Figure 9 compares the mass transfer rate predicted by the proposed correlation Eq. 27 and the experimental values.

FIGURE 9

FIGURE 9. Deviation of the predicted k_y from the experimental k_y at different L/G.

Approximately 100% of the predicted values lie within the uncertainty of ±5% of the experimental results. Moreover, it can be seen from Figure 9 that the deviation between the predicted values and experimental values increases as m decreases over the range of 3.0–4.5 L m⁻³. It could be reasoned that the droplets are not evenly distributed in the spray scrubber at low m in this study.

4.5 Validation of predicted k_y

The comparison between the experimental mass transfer rate and the predicted mass transfer rate was conducted to verify the reliability of this proposed mass transfer coefficient (Eq. 27). The inlet and outlet concentration of SO₂ were measured at different operating conditions such as v, pH and m, then the mass transfer rate were calculated by Eq. 25. The corresponding predicted mass transfer rate at the same conditions were also calculated by the mass transfer coefficient in Eq. 28. The gas flow rate v ranged from 1.92 to 2.6 m/s, the pH of solution ranged from 5.2 to 5.8 and the liquid-to-gas ratio m ranged from 3 to 5.5 L/m³.

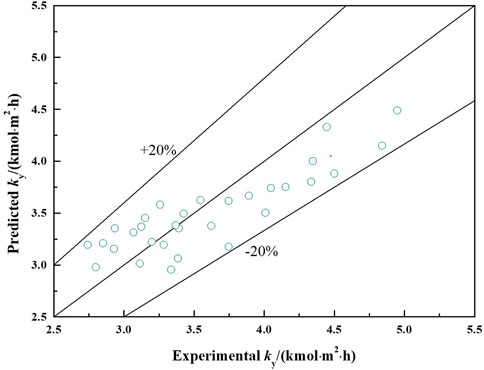
Figure 10 shows the predicted mass transfer rate versus the measured values of a lab-scale ammonia-based desulfurization system. The comparison reveals that the relative error of the predicted values and the measured data is almost within ±20%. The mass transfer rate of absorption of SO₂ by ammonia predicted by Eq. 28 agree well with the measured values. This proposed mass transfer coefficient appears to be beneficial for the design and operation of ammonia-based desulfurization FGD system.

FIGURE 10

FIGURE 10. Predicted k_y in comparison with experimental k_y for all the tested conditions.

5 Conclusion

The mass transfer performance of SO₂ absorption by ammonia has been studied in a lab-scale spray scrubber. Experiments were conducted by varying operating conditions of v, pH and m. On basis of experimental results, the functional relationship between k_y, v and m was deduced by means of multivariable linear regression and the mass transfer coefficient of absorption of SO₂ by ammonia was proposed as following:

$k_y=5.8\times {10}^{-2}\times v^{0.43}{\mathrm{p}\mathrm{H}}^{2.26}{m}^{0.57}(29)$

The mass transfer rate of SO₂ absorption by ammonia at different operating conditions was calculated by the equation mentioned above. The mass transfer coefficient was verified against measured values of a lab-scale ammonia-based desulfurization system. The comparison results show that the predicted mass transfer rate agrees well with the measured data. The relative deviation between predicted mass transfer rate and measured data is almost within ±20%. The mass transfer coefficient proposed could predict the mass transfer rate of SO₂ across the gas-liquid interface. Accordingly, the mass transfer coefficient proposed in this work would be beneficial for the design and operation of ammonia-based desulfurization system.

Data availability statement

The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.

Author contributions

YJ: Conceptualization, Methodology, Investigation. GY: Validation, Formal analysis, Writing—original draft. DW: Validation, Formal analysis, Visualization, Writing—review and editing. YG and YD: Validation, Formal analysis, Visualization. JJ, YC, MZ, and CS: Resources, Writing—review and editing. LG: Validation, Formal analysis.

Funding

This work was financially supported by the Major national R & D projects of China (2017YFB0601805) and National Natural Science Foundation of China (51674002).

Conflict of interest

DW was employed by Xi’'an Aerospace Yuan Dongli Engineering Co., Ltd.

The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Bandyopadhay, A., and Biswas, M. N. (1998). Scrubbing of sulphur dioxide in a dual-flow scrubber. J. India. Assoc. Environ. Manage. 26, 113–133.

Google Scholar

Colle, S., Thomas, D., and Vanderschuren, J. (2005). Process simulation of sulphur dioxide abatement with hydrogen peroxide solutions in a packed column. Chem. Eng. Res. Des. 83, 81–87. doi:10.1205/cherd.03115

CrossRef Full Text | Google Scholar

Dai, G. C., and Chen, M. H. (1988). Chemical engineering fluid mechanics. Beijing: Chemical Industrial Press.

Google Scholar

Gao, X., Ding, H. L., Du, Z., Wu, Z. L., Fang, M. X., Luo, Z. Y., et al. (2010). Gas-liquid absorption reaction between (NH₄)₂SO₃ solution and SO₂ for ammonia-based wet flue gas desulfurization. Appl. Energy 87, 2647–2651. doi:10.1016/j.apenergy.2010.03.023

CrossRef Full Text | Google Scholar

He, B. S., Zheng, X. Y., Wen, Y., Tong, H. L., Chen, M. Q., and Chen, C. H. (2003). Temperature impact on SO2 removal efficiency by ammonia gas scrubbing. Energy Convers. Manag. 44, 2175–2188. doi:10.1016/s0196-8904(02)00230-3

CrossRef Full Text | Google Scholar

Hixon, A. W., and Scott, C. E. (1935). Absorption of gases in spray towers. Ind. Eng. Chem. 27, 307–314. doi:10.1021/ie50303a015

CrossRef Full Text | Google Scholar

Hu, L. S., Wang, X. J., Yu, G. S., Wang, F. C., and Yu, Z. H. (2008). Study on gas-liquid phase mass transfer coefficient of entrained flow reactor. Chem. Eng. J. 141, 278–283. doi:10.1016/j.cej.2007.12.032

CrossRef Full Text | Google Scholar

Javed, K. H., Mahmud, T., and Purba, E. (2006). Enhancement of mass transfer in a spray tower using swirling gas flow. Chem. Eng. Res. Des. 84, 465–477. doi:10.1205/cherd.05119

CrossRef Full Text | Google Scholar

Jia, Y., Yin, L. G., Xu, Y. L., Ding, X. L., and Sheng, G. (2015). A model for performance of sulfite oxidation of ammonia-based flue gas desulfurization system. Atmos. Pollut. Res. 6, 997–1003. doi:10.1016/j.apr.2015.05.005

CrossRef Full Text | Google Scholar

Jia, Y., Zhong, Q., Fan, X. Y., and Wang, X. R. (2010). Kinetics of oxidation of total sulfite in the ammonia-based wet flue gas desulfurization process. Chem. Eng. J. 164, 132–138. doi:10.1016/j.cej.2010.08.041

CrossRef Full Text | Google Scholar

Liu, X. Y., Guo, Z. S., Roache, N. F., Mocka, C. A., Allen, M. R., and Mason, M. A. (2015). Henry’s law constant and overall mass transfer coefficient for formaldehyde emission from small water pools under simulated indoor environmental conditions. Environ. Sci. Technol. 49, 1603–1610. doi:10.1021/es504540c

PubMed Abstract | CrossRef Full Text | Google Scholar

Long, X. L., Li, W., Xiao, W. D., and Yuan, W. (2006). Novel homogeneous catalyst system for the oxidation of concentrated ammonium sulfite. J. Hazard. Mat. 129, 260–265. doi:10.1016/j.jhazmat.2005.08.035

PubMed Abstract | CrossRef Full Text | Google Scholar

Ma, S. C., Zang, B., Song, H. H., Chen, G. D., and Yang, J. H. (2013). Research on mass transfer of CO₂ absorption using ammonia solution in spray tower. Int. J. Heat. Mass Transf. 67, 696–703. doi:10.1016/j.ijheatmasstransfer.2013.08.090

CrossRef Full Text | Google Scholar

Marion, M., Lépinasse, E., and Saboni, A. (2006). SO₂ absorption and desorption by an accelerating water droplet undergoing vaporization. Int. J. Heat. Fluid Flow. 27, 290–297. doi:10.1016/j.ijheatfluidflow.2005.09.001

CrossRef Full Text | Google Scholar

Michalski, J. A. (2000). Aerodynamic characteristics of flue gas desulfurization spray towers-polydispersity consideration. Ind. Eng. Chem. Res. 39, 3314–3324. doi:10.1021/ie990791h

CrossRef Full Text | Google Scholar

Milene, C. C., and Waldir, A. B. (2013). Experimental study of the SO₂ removal efficiency and volumetric mass transfer coefficients in a pilot-scale multi-nozzle spray tower. Int. J. Heat. Mass Transf. 66, 80–89. doi:10.1016/j.ijheatmasstransfer.2013.07.011

CrossRef Full Text | Google Scholar

Mounsef, J. R., Salameh, D., Louka, N., Brandam, C., and Lteif, R. (2015). The effect of aeration conditions, characterized by the volumetric mass transfer coefficient K_La, on the fermentation kinetics of Bacillus thuringiensis kurstaki. J. Biotechnol. 210, 100–106. doi:10.1016/j.jbiotec.2015.06.387

PubMed Abstract | CrossRef Full Text | Google Scholar

Ryuichi, K., Yukio, H., and Kuroda, H. (1985). SO₂ absorption by water droplets. J. Chem. Eng. Jpn. 18, 169–172. doi:10.1252/jcej.18.169

CrossRef Full Text | Google Scholar

Schmidt, B., and Stichlmair, J. (1991). Two-phase flow and mass transfer in scrubbers. Chem. Eng. Technol. 14, 162–166. doi:10.1002/ceat.270140303

CrossRef Full Text | Google Scholar

Sun, Z. G., Zhao, Y., Gao, H. Y., and Hu, G. X. (2010). Removal of SO₂ from flue gas by sodium humate solution. Energy fuels. 24, 1013–1019. doi:10.1021/ef901052r

CrossRef Full Text | Google Scholar

Valle-Zermeño, R. D., Formosa, J., Aparicio, J. A., and Chimenos, J. (2014). Reutilization of low-grade magnesium oxides for flue gas desulfurization during calcination of natural magnesite: A closed-loop process. Chem. Eng. J. 254, 63–72. doi:10.1016/j.cej.2014.05.089

CrossRef Full Text | Google Scholar

Wang, S. J., Zhu, P., Zhang, G., Zhang, Q., Wang, Z. Y., and Zhao, L. (2015). Numerical simulation research of flow field in ammonia-based wet flue gas desulfurization tower. J. Energy Inst. 88, 284–291. doi:10.1016/j.joei.2014.09.002

CrossRef Full Text | Google Scholar

Wu, P. K., Teseng, L. K., and Faeth, G. M. (1995). Primary breakup in gas/liquid mixing layers for turbulent liquids. At. Spr. 2, 295–317. doi:10.1615/atomizspr.v2.i3.60

CrossRef Full Text | Google Scholar

Zhong, Y., Gao, X., Huo, W., Luo, Z. Y., Ni, M. J., and Cen, K. F. (2008). A model for performance optimization of wet flue gas desulfurization systems of power plants. Fuel Process. Technol. 89, 1025–1032. doi:10.1016/j.fuproc.2008.04.004

CrossRef Full Text | Google Scholar

Nomenclature

Abbreviations

a Gas-liquid mass transfer contact area (m²/m³)

A_d The surface area of droplets in a spray scrubber (m²)

A_m Liquid film surface area of spray tower (m²)

C_d Drag coefficient

d Sauter mean diameter of droplet (m)

$d_k$ Inner hole diameter of nozzle, 4 × 10^–3 m

D The diameter of tower (m)

G Flue gas flow rate (kmol·m⁻²·h⁻¹)

g Acceleration of gravity (m·s⁻²)

h_m The thickness of spray wall liquid film (m)

H Henry coefficient

k₀ coefficient

K_G The overall volumetric mass transfer coefficients (kmol·m⁻³·h⁻¹·Pa⁻¹)

k_G The overall mass transfer coefficients in the gas phase (kmol·m⁻³·h⁻¹·Pa⁻¹)

k_L The mass transfer coefficients in the liquid phase (kmol·m⁻³·h⁻¹·Pa⁻¹)

k_y The mass transfer rate (kmol·m⁻³·h⁻¹·Pa⁻¹)

$k_y^{\prime }$ The mass transfer coefficient (kmol·m⁻³·h⁻¹·Pa⁻¹)

L Liquid flow rate (L·h⁻¹)

L_d Slurry spray amount in the presence of liquid drop (L·h⁻¹)

L_m Tower wall slurry flow (kg·s⁻¹)

m_l mass flow rate of liquid from nozzle (kg·s⁻¹)

N The mass transfer rate of SO₂ (kmol·m⁻²·h⁻¹)

p The pressure of SO₂ in gas phase (Pa)

P_l Operating pressure of nozzle (MPa)

p^* Interfacial equilibrium partial pressure of SO₂ in gas phase (Pa)

Re_d Reynolds number

Re_m The Reynolds number of Liquid film flow

$u_d^0$ Initial velocity of droplet (m·s⁻¹)

u_d The velocity of droplet (m·s⁻¹)

u_g The velocity of gas (m·s⁻¹)

We Weber number.

y₁ Inlet mole fraction of SO₂

y₂ Outlet mole fraction of SO₂

y^* Equilibrium mole fraction of SO₂ at gas-liquid interface.

y Mole fraction of SO₂ in gas phase

Z Height of absorption area of spray tower (m)

η SO₂ removal efficiency (%)

ρ Slurry density (kg·m⁻³)

μ Viscosity of slurry (Pa·s)

ν Kinematic viscosity of slurry (m²·s⁻¹)

σ Surface tension of slurry (N·m).