- 1Key Laboratory of Advanced Micro-structure Materials, MOE, School of Physics Science and Engineering, Tongji University, Shanghai, China
- 2Shanxi Provincial Key Laboratory of Microstructure Electromagnetic Functional Materials, Shanxi Datong University, Datong, China
- 3College of Electronic and Information Engineering, Tongji University, Shanghai, China
Recently, linear-crossing metamaterials (LCMMs) in the hyperbolic topological transition of iso-frequency contour, have attracted people’s great attention. Due to the novel linear dispersion, LCMM provides a new platform to control and enhance the light-matter interactions, such as all-angle negative refraction, filters, super-lens, etc. However, the narrow-band working frequency is currently the major limitation in LCMMs. In this work, we propose two methods to realize multiple linear-crossing metamaterials (MLCMMs), including a basic Drude-Lorenz model and an actual step-like multilayer structure. Especially, in order to identify the designed two kinds of MLCMMs, we numerically demonstrate the unique beam splitting and directional refraction of MLCMM at different frequencies. Our findings may not only provide a new platform for the fundamental study of LCMM, but also facilitate some broadband applications.
Introduction
Metamaterials are fundamental building blocks in a number of optical applications due to their ability to efficiently and flexibly manipulate light (Pendry, 2000; Shelby et al., 2001; Engheta and Ziolkowsky, 2006). Among the abundant metamaterials, hyperbolic metamaterials (HMMs) (Poddubny et al., 2013; Smolyaninov, 2018; Guo et al., 2020a; Lee et al., 2022) and zero-index metamaterials (ZIMs) (Liberal and Engheta, 2017; Niu et al., 2018) are undoubtedly remarkable because of their diverse counterintuitive attributes. Recently, linear-crossing metamaterials (LCMMs) with linear dispersion, which combine the properties of both HMM and ZIM, have been proposed in the study of topological phase transition between two kinds of HMMs (Guo et al., 2018a). Take the electric LCMM (
Although LCMMs have some physical properties similar to ZIMs and HMMs, which have been widely studied in the last two decades, the novel linear dispersion also enables LCMMs to have many unique properties that are worth exploring. The light-matter interactions depend on the dispersion in wave-vector space characterized by the isofrequency contours (IFCs). Similar to the Fermi plane in electronic systems, the IFCs of photonic systems determine the transmission properties of light in various materials (Guo et al., 2020a). The complex dispersive control of beam propagation is a very interesting topic (Fan et al., 2016; Tsilipakos et al., 2018; Zhu et al., 2022). It is well known that the normal topological transition of dispersion in anisotropic metamaterials, in which IFC changes from a closed ellipsoid to an open hyperboloid, can lead to many unusual propagation phenomena of electromagnetic waves in media, such as all-angle negative refraction (High et al., 2015), focusing (Martín-Sánchez et al., 2021), and self-collimation (Yu et al., 2016). Especially, the open IFCs of hyperbolic dispersion can support high-k modes and possess an enhanced photonic density of states (Smolyaninov and Narimanov, 2010; Krishnamoorthy et al., 2012), leading to promising applications such as super-resolution imaging that can overcome diffraction limits (Liu et al., 2007; Smolyaninov et al., 2007; Duan et al., 2021a), long-range atom-atom interaction (Guo et al., 2018b; Newman et al., 2018), optical forces engineering (Li et al., 2020a; Li et al., 2020b), sub-wavelength cavities (Yang et al., 2012; Wang et al., 2020; Guo et al., 2022b) and waveguides (Guo et al., 2021a; Fu et al., 2021; Ji et al., 2022). Similar to the normal topological transition, the LCMMs existing in the novel hyperbolic topological transition of dispersion can also be well studied based on IFC, which reveals that LCMMs have some unique properties in the field of designing superior optical devices, such as the super-resolution imaging (Guo et al., 2018a), diffraction free beam (Guo et al., 2020b), and robust spatial filtering (Guo et al., 2020c). It should be emphasized that although LCMM was proposed in the transmission lines system based on effective circuit model, it has recently been extended to photonic crystal (Yang et al., 2019), phononic crystal (Xu et al., 2022), and even the
In this work, we propose the multi-frequency LCMM from the basic Drude-Lorenz model and actual step-like multilayer structure. Specifically, from the perspective of basic dispersive electromagnetic parameters, when the permittivity meets the Drude-Lorenz model, a double-LCMM will be achieved. In addition, multiple structure is a very special topic because it has more physical properties than a single counterpart. When considering an actual composite stepped multilayer structure, a quintuple-LCMM will also be achieved. Since the two open branches of IFC intersect in LCMMs, multi-frequency beam splitting will occur when light is normally incident on LCMMs. Besides, the unidirectional refraction will happen if the light is incident on the material at oblique angles. Based on the findings in this study, a series of important applications based on these novel properties may be applied, such as broadband 50/50 beam splitter, unidirectional refraction, zero-phase delay device and so on.
Results and discussion
The novel physical properties of LCMMs have motivated extensive studies of linear dispersion in photonic and acoustic systems. However, as introduced in previous works, the LCMMs suffer from single frequency response for the near-zero permittivity or permeability, which greatly hamper the related applications. Once the multiple linear-crossing metamaterials (MLCMM) can be realized, broadband directional refraction becomes possible. Due to the special linear dispersion characteristics of MLCMM, it will open up a new way for energy directional collection and filtering when the natural light incident on the material, as shown schematically in Figure 1. Generally speaking, the electromagnetic response of uniaxial materials is described by the permittivity and permeability (Guo et al., 2020a)
where the subscript
where
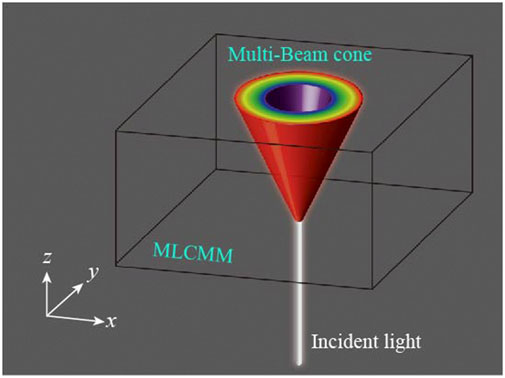
FIGURE 1. Schematic of the multi-beam cone in the MLCMM. The beam splitting will occur when the light incident on the MLCMM.
Considering the TE polarized wave propagating in the x–z plane, the simply dispersion equation of the uniaxial media is expressed as (Guo et al., 2020a)
where
where
where
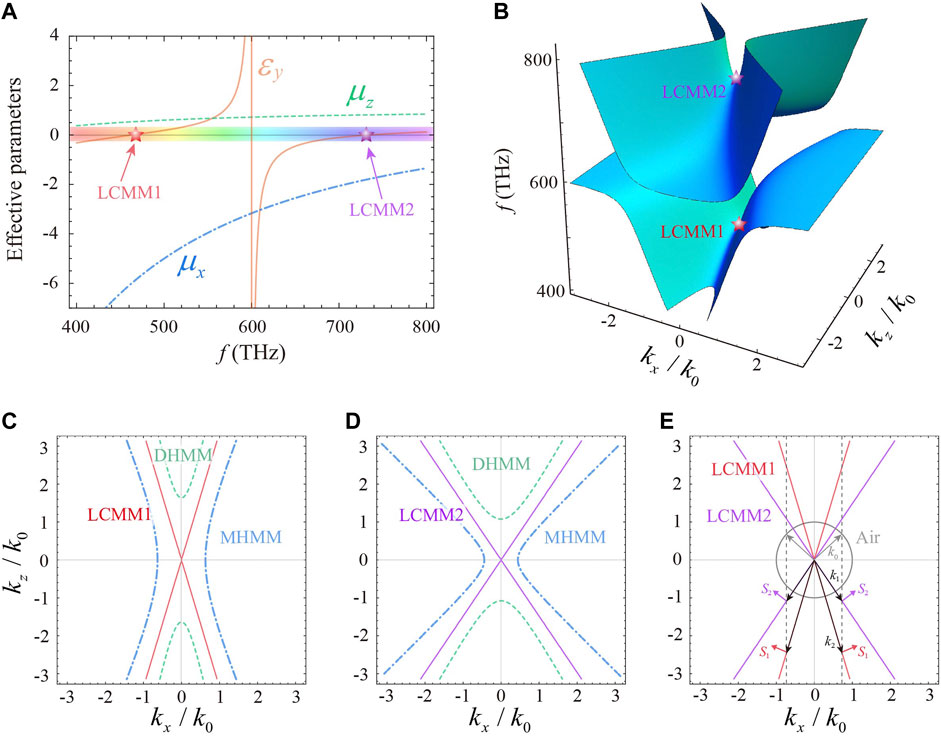
FIGURE 2. MLCMM based on the Drude-Lorenz model. (A) Effective anisotropic electromagnetic parameters of the MLCMM based on the Drude-Lorenz model.
The beam splitting and directional refraction of double-LCMM are clarified from the linear dispersion, which is shown in Figure 2E. The gray arrows and black arrows denote the wave vectors in free space (the incident medium) and double-LCMM (the refracted medium), respectively. According to the boundary conditions and the causality law, we can determine that the energy flow of the refractive waves can only propagate in two fixed directions, which are marked respectively by the red and purple arrows for LCMM1 and LCMM2 in Figure 2E. Because of the novel linear dispersion, when light incident from air to the double-LCMM the refracted angle is fixed as
For the LCMM1 (LCMM2) at 463 THz (733 THz), the anisotropic permeability corresponds to
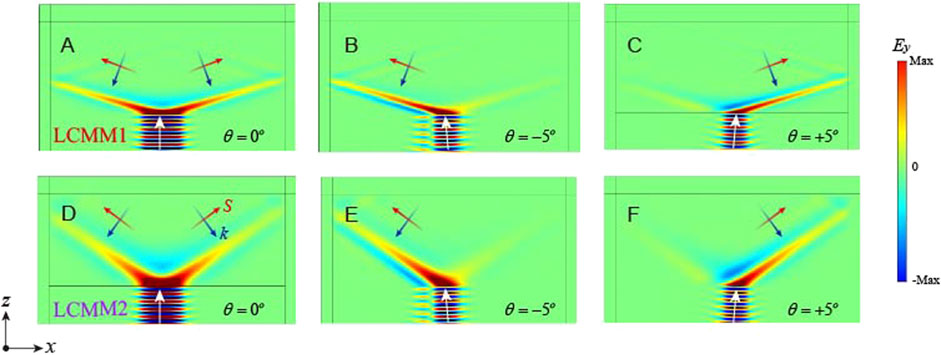
FIGURE 3. Beam splitting and unidirectional refraction of MLCMM based on the Drude-Lorenz model. (A) Beam splitting when the light is normally incident on the LCMM1. (B), (C) The directional refraction of LCMM1 when the incident angle is
From Figure 3, it is clear that incident red and purple light will trigger beam splitting and directional refraction with different refraction angles. In order to further verify the multi-frequency directional refraction of MLCMM, we study the electric field distributions at more incident angles in Figure 4. When the incident angle increases from
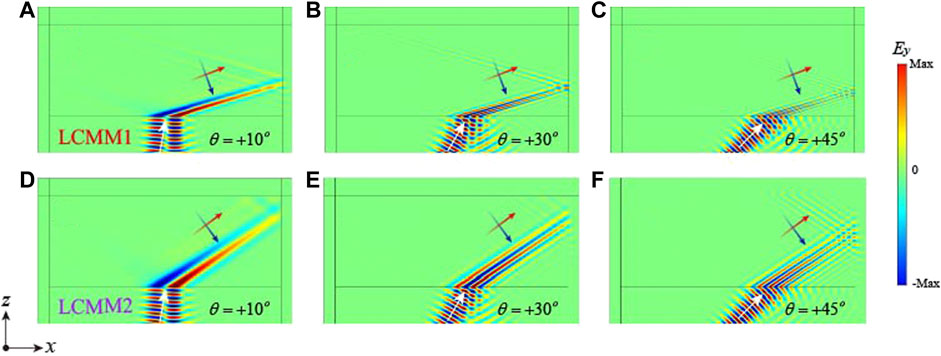
FIGURE 4. Directional refraction of the MLCMM based on the Drude-Lorenz model. (A–C) The positive refraction of LCMM1 with different incident angles: (A)
After using the basic Drude-Lorenz model to study the multi-frequency directional refraction of MLCMM, we also design a practical composite stepped multilayer structure to realize the MLCMM. At present, although multilayered structures containing two kinds of single-negative media have been proposed for the single-frequency LCMM, the multiple-LCMM with broadband properties remains elusive (Guo et al., 2020b). Fortunately, based on the Bergman spectral representation of the effective permittivity, the step-like design has been successfully used in realizing the multi frequency near-zero effective permittivity (Sun et al., 2013). Very recently, the all-angle broadband near-zero effective permittivity property also has been demonstrated in the symmetric step-like multilayer structure (Sun et al., 2022). Inspired by these pioneering works, in this section we propose the composite stepped multilayer structure to realize the MLCMM. The component DPS (
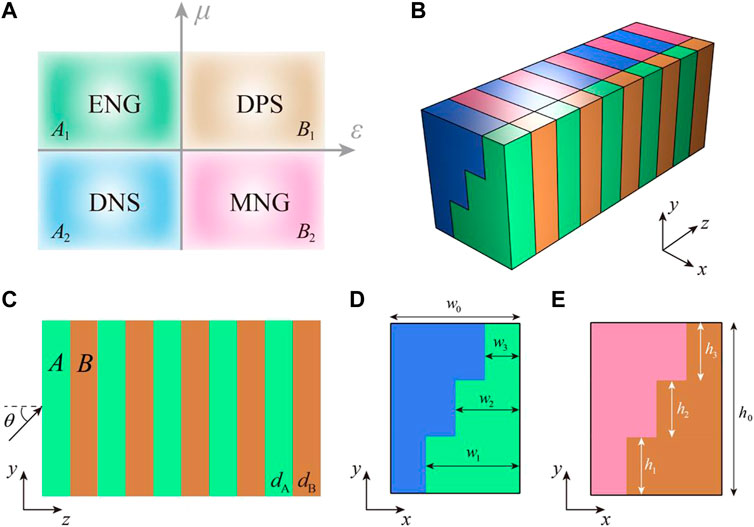
FIGURE 5. Step-like multilayer structure for MLCMM. (A) Electromagnetic parameter space of the isotropic media. DPS (
Considering the simplicity of the structure, we assume that the dispersion electromagnetic parameters are described by the simple Drude model (Guo et al., 2020b)
In the following calculation, we choose
and
respectively. Based on Eqs. 9 and (10), the effective permittivity and anisotropic permeability of the step-like multilayer structure are shown in Figure 6A. The dependence of

FIGURE 6. MLCMM based on the step-like multilayer structure. (A) Effective real part of anisotropic electromagnetic parameters based on the step-like multilayer structure.
For the LCMM1, LCMM2, and LCMM3, the anisotropic permeability in x (z) direction correspond to
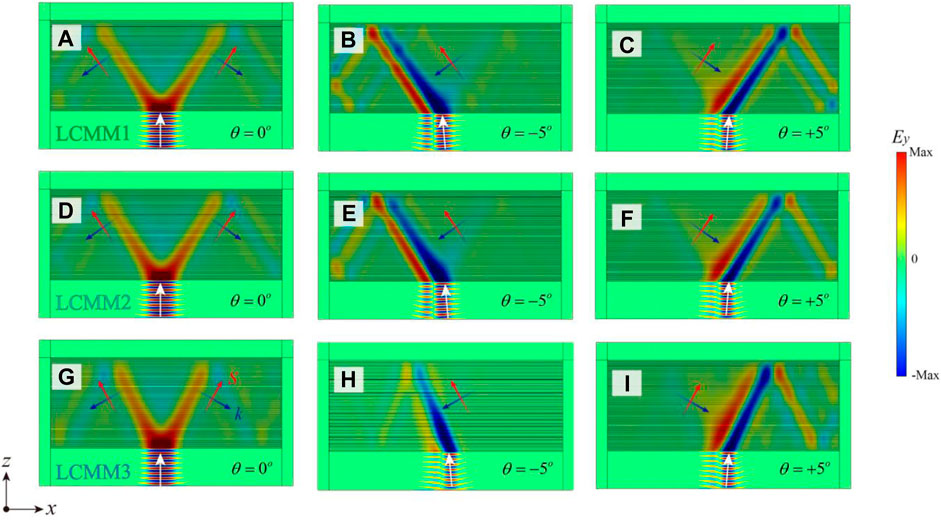
FIGURE 7. Beam splitting and unidirectional refraction of MLCMM based on the step-like multilayer structure. (A) Beam splitting when the light is normally incident on the LCMM1. (B), (C) The directional refraction of LCMM1 when the incident angle is
Similar to the MLCMM based on the basic Drude-Lorenz model in Figure 4, the directional refraction with different refraction angles of MLCMM based on the step-like multilayer structure is demonstrated in Figure 8. When the incident angle increases from
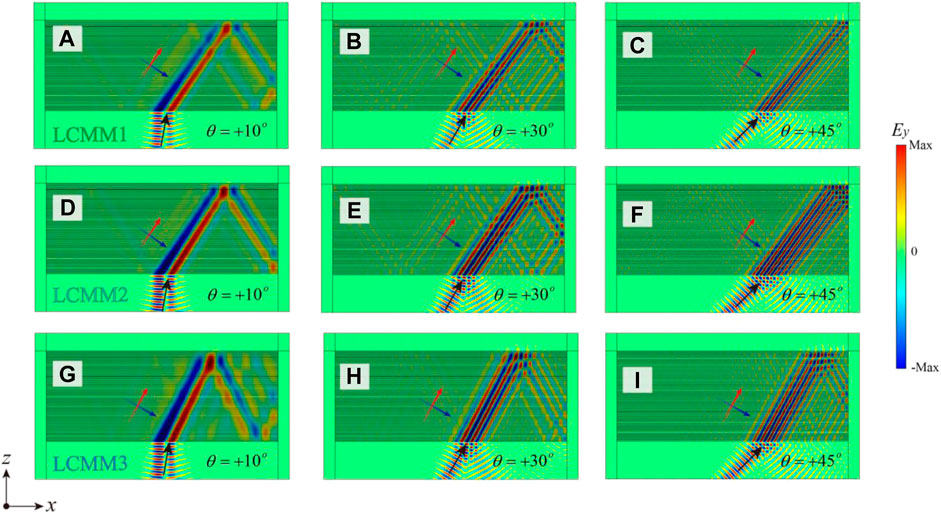
FIGURE 8. Directional refraction of the MLCMM based on the step-like multilayer structure. (A–C) The positive refraction of LCMM1 with different incident angles: (A)
Conclusion
In summary, we theoretically propose the MLCMM in the hyperbolic topological transition. Starting from basic Drude-Lorenz model and the effective composite step-like multilayer structure, we demonstrate that the MLCMMs can be used to realize the multi-frequency beam splitting and directional refraction for the normal and oblique incident beams, respectively. The results not only combine two independent concepts of ZIM and HMM to construct a new multi-frequency optical metamaterial, but also provide a way to design novel optical devices with excellent performance, which overcomes the single-frequency limitation of conventional LCMM.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Author contributions
ZG and HC put forward the initial idea and supervised the project. ZG and YJ performed the theoretical calculations and wrote the manuscript. XW, FD, and LD helped with the theoretical analyses. All authors fully contribute to the research. ZG and YJ contribute equally to this work.
Funding
This work was supported by the National Key R&D Program of China (Grant No. 2021YFA1400602), the National Natural Science Foundation of China (NSFC; Grant Nos. 12004284 and 61621001), the Central Government Guides Local Science and Technology Development Fund Projects (Grant No. YDZJSX 2021B011), the Fundamental Research Funds for the Central Universities (Grant No. 22120210579), and the Shanghai Chenguang Plan (Grant No.21CGA22).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Alù, A., and Engheta, N. (2009). All optical metamaterial circuit board at the nanoscale. Phys. Rev. Lett. 103, 143902. doi:10.1103/physrevlett.103.143902
Chen, M., Lin, X., Dinh, T. H., Zhang, Z., Shen, J., Ma, Q., et al. (2020). Configurable phonon polaritons in twisted α-MoO3. Nat. Mat. 19, 1307–1311. doi:10.1038/s41563-020-0732-6
Deng, F., Li, Y., Sun, Y., Wang, X., Guo, Z., Shi, Y., et al. (2015). Valley-dependent beams controlled by pseudomagnetic field in distorted photonic graphene. Opt. Lett. 40, 3380. doi:10.1364/ol.40.003380
Duan, J., Álvarez-Pérez, G., Tresguerres-Mata, A. I. F., Taboada-Gutiérrez, J., Voronin, K. V., Bylinkin, A., et al. (2021). Planar refraction and lensing of highly confined polaritons in anisotropic media. Nat. Commun. 12, 4325. doi:10.1038/s41467-021-24599-3
Duan, J., Alvarez-Pérez, G., Voronin, K. V., Prieto, I., Taboada-Gutiérrez, J., Volkov, V. S., et al. (2021). Enabling propagation of anisotropic polaritons along forbidden directions via a topological transition. Sci. Adv. 7, eabf2690. doi:10.1126/sciadv.abf2690
DuanCapote-Robayna, J. N., Taboada-Gutiérrez, J., ÁlvarezPérez, G., Prieto, I., Martín-Sánchez, J., Nikitin, A. Y., et al. (2020). Twisted nano-optics: Manipulating light at the nanoscale with twisted phonon polaritonic slabs. Nano Lett. 20, 5323–5329. doi:10.1021/acs.nanolett.0c01673
Engheta, N. (2007). Circuits with light at nanoscales: Optical nanocircuits inspired by metamaterials. Science 317, 1698–1702. doi:10.1126/science.1133268
Engheta, N., and Ziolkowsky, R. W. (2006). Metamaterials, physics and engineering exploration. New York: John Wiley & Sons.
Erçağlar, V., Hajian, H., Serebryannikov, A. E., and Ozbay, E. (2021). Multifunctional tunable gradient metasurfaces for terahertz beam splitting and light absorption. Opt. Lett. 46, 3953. doi:10.1364/ol.435197
Fan, Y., Shen, N. H., Zhang, F., Wei, Z., Li, H., Zhao, Q., et al. (2016). Electrically tunable Goos–Hänchen effect with graphene in the terahertz regime. Adv. Opt. Mat. 4, 1824–1828. doi:10.1002/adom.201600303
Fan, Y., Shen, N., Zhang, F., Zhao, Q., Wu, H., Fu, Q., et al. (2019). Graphene plasmonics: A platform for 2D optics. Adv. Opt. Mat. 7, 1800537. doi:10.1002/adom.201800537
Ferrari, L., Wu, C. H., Lepage, D., Zhang, X., and Liu, Z. W. (2015). Hyperbolic metamaterials and their applications. Prog. Quantum Electron. 40, 1. doi:10.1016/j.pquantelec.2014.10.001
Fu, M., Jin, Y., Evans, J., and He, S. L. (2021). Microwave waveguide-type hyperbolic metamaterials. Adv. Photonics Res. 2, 2000043. doi:10.1002/adpr.202000043
Guo, Z., Jiang, H., and Chen, H. (2020). Abnormal wave propagation in tilted linear-crossing metamaterials. Adv. Photonics Res. 2, 2000071. doi:10.1002/adpr.202000071
Guo, Z., Jiang, H., and Chen, H. (2020). Hyperbolic metamaterials: From dispersion manipulation to applications. J. Appl. Phys. 127, 071101. doi:10.1063/1.5128679
Guo, Z., Jiang, H., and Chen, H. (2022). Linear-crossing metamaterials and their applications. Acta Phot. Sin. 51, 0151111.
Guo, Z., Jiang, H., and Chen, H. (2020). Linear-crossing metamaterials mimicked by multi-layers with two kinds of single negative materials. J. Phys. Photonics 2, 011001. doi:10.1088/2515-7647/ab5ecb
Guo, Z., Jiang, H., and Chen, H. (2022). Zero-index and hyperbolic metacavities: Fundamentals and applications. J. Phys. D. Appl. Phys. 55, 083001. doi:10.1088/1361-6463/ac2e89
Guo, Z., Jiang, H., Li, Y., Chen, H., and Agarwal, G. S. (2018). Enhancement of electromagnetically induced transparency in metamaterials using long range coupling mediated by a hyperbolic material. Opt. Express 26, 627. doi:10.1364/oe.26.000627
Guo, Z., Jiang, H., Sun, Y., Li, Y., and Chen, H. (2018). Actively controlling the topological transition of dispersion based on electrically controllable metamaterials. Appl. Sci. (Basel). 8, 596. doi:10.3390/app8040596
Guo, Z., Jiang, H., Zhu, K., Sun, Y., Li, Y., and Chen, H. (2018). Focusing and super-resolution with partial cloaking based on linear-crossing metamaterials. Phys. Rev. Appl. 10, 064048. doi:10.1103/physrevapplied.10.064048
Guo, Z., Long, Y., Jiang, H., Ren, J., and Chen, H. (2021). Anomalous unidirectional excitation of high-k hyperbolic modes using all-electric metasources. Adv. Phot. 3, 036001. doi:10.1117/1.ap.3.3.036001
Guo, Z., Song, J., Jiang, H., and Chen, H. (2021). Miniaturized backward coupler realized by the circuit-based planar hyperbolic waveguide. Adv. Photonics Res. 2, 2100035. doi:10.1002/adpr.202100035
Guo, Z., Sun, Y., Jiang, H., Ding, Y.-Q., Li, Y., Zhang, Y., et al. (2020). Experimental demonstration of an anomalous Floquet topological insulator based on negative-index media. Available at: https://arxiv.org/abs/2006.12252.
Hashimoto, Y., Seniutinas, G., Balčytis, A., Juodkazis, S., and Nishijima, Y. (2016). Au-Ag-Cu nano-alloys: Tailoring of permittivity. Sci. Rep. 6, 25010. doi:10.1038/srep25010
High, A. A., Devlin, R. C., Dibos, A., Polking, M., Wild, D. S., Perczel, J., et al. (2015). Visible frequency hyperbolic metasurface. Nature 522, 192–196. doi:10.1038/nature14477
Hu, F., Du, S., Li, J., and Gu, C. (2021). Multidimensional image and beam splitter based on hyperbolic metamaterials. Nano Lett. 21, 1792–1799. doi:10.1021/acs.nanolett.0c04795
Hu, G., Ou, Q., Si, G., Wu, Y., Wu, J., Dai, Z., et al. (2020). Topological polaritons and photonic magic angles in twisted α-MoO3 bilayers. Nature 582, 209–213. doi:10.1038/s41586-020-2359-9
Ji, W., Zhou, X., Chu, H., Luo, J., and Lai, Y. (2022). Theory and experimental observation of hyperbolic media based on structural dispersions. Phys. Rev. Mat. 4, 105202. doi:10.1103/physrevmaterials.4.105202
Krishnamoorthy, H. N., Jacob, Z., Narimanov, E., Kretzschmar, I., and Menon, V. M. (2012). Topological transitions in metamaterials. Science 336, 205–209. doi:10.1126/science.1219171
Kruk, S. S., Wong, Z. J., Pshenay-Severin, E., O’Brien, K., Neshev, D. N., Kivshar, Y. S., et al. (2016). Magnetic hyperbolic optical metamaterials. Nat. Commun. 7, 11329. doi:10.1038/ncomms11329
Lee, D., So, S., Hu, G., Kim, M., Badloe, T., Cho, H., et al. (2022). Hyperbolic metamaterials: Fusing artificial structures to natural 2D materials. Elight 2, 1. doi:10.1186/s43593-021-00008-6
Li, H., Cao, Y., Shi, B., Zhu, T., Geng, Y., Feng, R., et al. (2020). Momentum-topology-induced optical pulling force. Phys. Rev. Lett. 124, 143901. doi:10.1103/physrevlett.124.143901
Li, H., Cao, Y., Zhou, L. M., Xu, X., Zhu, T., Shi, Y., et al. (2020). Optical pulling forces and their applications. Adv. Opt. Phot. 12, 288. doi:10.1364/aop.378390
Liberal, I., and Engheta, N. (2017). Near-zero refractive index photonics. Nat. Photonics 11, 149–158. doi:10.1038/nphoton.2017.13
Liu, M., Hwang, H. Y., Tao, H., Strikwerda, A. C., Fan, K., Keiser, G. R., et al. (2012). Terahertz-field-induced insulator-to-metal transition in vanadium dioxide metamaterial. Nature 487, 345–348. doi:10.1038/nature11231
Liu, Y., Ouyang, C., Xu, Q., Su, X., Ma, J., Zhao, J., et al. (2022). Negative refraction in twisted hyperbolic metasurfaces. Nanophotonics 11, 1977–1987. doi:10.1515/nanoph-2021-0561
Liu, Y., Ouyang, C., Xu, Q., Su, X., Yang, Q., Ma, J., et al. (2022). Moiré-driven electromagnetic responses and magicangle in sandwiched hyperbolic metasurface. Phot. Res 10, 2056–2065. doi:10.1364/prj.462119
Liu, Z., Lee, H., Xiong, Y., Sun, C., and Zhang, X. (2007). Far field optical hyperlens magnifying sub-diffraction-limited objects. Science 315, 1686. doi:10.1126/science.1137368
Long, Y., Ren, J., Guo, Z., Jiang, H., Wang, Y., Sun, Y., et al. (2020). Designing all-electric subwavelength metasources for near-field photonic routings. Phys. Rev. Lett. 125, 157401. doi:10.1103/physrevlett.125.157401
Martín-Sánchez, J., Duan, J. H., Taboada-Gutiérrez, J., Álvarez-Pérez, G., Voronin, K. V., Prieto, I., et al. (2021). Focusing of in-plane hyperbolic polaritons in van der Waals crystals with tailored infrared nanoantennas. Sci. Adv. 7, eabj0127. doi:10.1126/sciadv.abj0127
Newman, W. D., Cortes, C. L., Afshar, A., Cadien, K., Meldrum, A., Fedosejevs, R., et al. (2018). Observation of long-range dipole-dipole interactions in hyperbolic metamaterials. Sci. Adv. 4, eaar5278. doi:10.1126/sciadv.aar5278
Niu, X. X., Hu, X. Y., Chu, S. S., and Gong, Q. H. (2018). Epsilon-near-zero photonics: A new platform for integrated devices. Adv. Opt. Mat. 6, 1701292. doi:10.1002/adom.201701292
Pendry, J. B. (2000). Negative refraction makes a perfect lens. Phys. Rev. Lett. 85, 3966–3969. doi:10.1103/physrevlett.85.3966
Phon, R., Kim, Y., Park, E., Jeong, H., and Lim, S. (2021). Mechanical and self-deformable spatial modulation beam steering and splitting metasurface. Adv. Opt. Mat. 9, 2100821. doi:10.1002/adom.202100821
Poddubny, A., Iorsh, I., Belov, P., and Kivshar, Y. (2013). Hyperbolic metamaterials. Nat. Photonics 7, 948–957. doi:10.1038/nphoton.2013.243
Sehmi, H. S., Langbein, W., and Muljarov, E. A. (2017). Optimizing the Drude-Lorentz model for material permittivity: Method, program, and examples for gold, silver, and copper. Phys. Rev. B 95, 115444. doi:10.1103/physrevb.95.115444
Shelby, R. A., Smith, D. R., and Schultz, S. (2001). Experimental verification of a negative index of refraction. Science 292, 77–79. doi:10.1126/science.1058847
Shen, L., Lin, X., Shalaginov, M. Y., Low, T., Zhang, X. M., Zhang, B. L., et al. (2020). Broadband enhancement of on-chip single-photon extraction via tilted hyperbolic metamaterials. Appl. Phys. Rev. 7, 021403. doi:10.1063/1.5141275
Smolyaninov, I. I., Hung, Y.-J., and Davis, C. C. (2007). Magnifying superlens in the visible frequency range. Science 315, 1699–1701. doi:10.1126/science.1138746
Smolyaninov, I. I. (2018). Hyperbolic metamaterials. London: Morgan & Claypool/Institute of Physics.
Smolyaninov, I. I., and Narimanov, E. E. (2010). Metric signature transitions in optical metamaterials. Phys. Rev. Lett. 105, 067402. doi:10.1103/physrevlett.105.067402
Sun, L., Gao, J., and Yang, X. D. (2013). Broadband epsilon-near-zero metamaterials with steplike metal-dielectric multilayer structures. Phys. Rev. B 87, 165134. doi:10.1103/physrevb.87.165134
Sun, L., Lin, Y., Yu, K. W., and Wang, G. P. (2022). All-angle broadband ENZ metamaterials. New J. Phys. 24, 073016. doi:10.1088/1367-2630/ac7d02
Taboada-Gutiérre, J., Álvarez-Pérez, G., Duan, J., Ma, W., Crowley, K., Prieto, I., et al. (2020). Broad spectral tuning of ultra-low-loss polaritons in a van der Waals crystal by intercalation. Nat. Mat. 19, 964–968. doi:10.1038/s41563-020-0665-0
Tsilipakos, O., Koschny, T., and Soukoulis, C. M. (2018). Antimatched electromagnetic metasurfaces for broadband arbitrary phase manipulation in reflection. ACS Photonics 5, 1101–1107. doi:10.1021/acsphotonics.7b01415
Vakil, A., and Engheta, N. (2011). Transformation optics using graphene. Science 332, 1291–1294. doi:10.1126/science.1202691
Wang, Y., Guo, Z., Chen, Y., Chen, X., Jiang, H., and Chen, H. (2020). Circuit-based magnetic hyperbolic cavities. Phys. Rev. Appl. 13, 044024. doi:10.1103/physrevapplied.13.044024
Xu, C., Huang, S., Guo, Z., Jiang, H., Li, Y., Wu, Y., et al. (2022). Acoustic beam splitting and cloaking based on a compressibility-near-zero medium. Phys. Rev. Appl. 17, 054025. doi:10.1103/physrevapplied.17.054025
Yang, X. D., Yao, J., Rho, J., Yin, X. B., and Zhang, X. (2012). Experimental realization of three-dimensional indefinite cavities at the nanoscale with anomalous scaling laws. Nat. Photonics 6, 450–454. doi:10.1038/nphoton.2012.124
Yang, Y., Jia, Z., Xu, T., Luo, J., Lai, Y., and Hang, Z.-H. (2019). Beam splitting and unidirectional cloaking using anisotropic zero-index photonic crystals. Appl. Phys. Lett. 114, 161905. doi:10.1063/1.5088837
Yermakov, O. Y., Hurshkainen, A. A., Dobrykh, D. A., Kapitanova, P. V., Iorsh, I. V., Glybovski, S. B., et al. (2018). Experimental observation of hybrid TE-TM polarized surface waves supported by a hyperbolic metasurface. Phys. Rev. B 98, 195404. doi:10.1103/physrevb.98.195404
Yu, K., Guo, Z. W., Jiang, H. T., and Chen, H. (2016). Loss-induced topological transition of dispersion in metamaterials. J. Appl. Phys. 119, 203102. doi:10.1063/1.4952378
Zhang, D. B., Seifert, G., and Chang, K. (2014). Strain-induced pseudomagnetic fields in twisted graphene nanoribbons. Phys. Rev. Lett. 112, 096805. doi:10.1103/physrevlett.112.096805
Keywords: anisotropic metamaterials, zero-index metamaterials, linear-crossing dispersion, beam splitting, topological transition
Citation: Guo Z, Jian Y, Wu X, Deng F, Dong L and Chen H (2022) Multiple linear-crossing metamaterials for directional refraction. Front. Mater. 9:1001233. doi: 10.3389/fmats.2022.1001233
Received: 23 July 2022; Accepted: 15 August 2022;
Published: 12 September 2022.
Edited by:
Fuyin Ma, Xi’an Jiaotong University, ChinaReviewed by:
Yuancheng Fan, Northwestern Polytechnical University, ChinaJiafu Wang, Air Force Engineering University, China
Wenlong Gao, University of Paderborn, Germany
Bogdan Popa, University of Michigan, United States
Copyright © 2022 Guo, Jian, Wu, Deng, Dong and Chen. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Zhiwei Guo, MjAxNGd1b3poaXdlaUB0b25namkuZWR1LmNu
 Zhiwei Guo
Zhiwei Guo Yiran Jian
Yiran Jian Xian Wu
Xian Wu Fusheng Deng
Fusheng Deng Lijuan Dong
Lijuan Dong Hong Chen
Hong Chen