- 1Key Lab. of Advanced Micro/Nano Electronic Devices & Smart Systems of Zhejiang, College of Information Science and Electronic Engineering, Zhejiang University, Hangzhou, China
- 2National Key Laboratory of Electromagnetic Environment Effects, Shijiazhuang, China
- 3School of Applied Science, Beijing Information Science and Technology University, Beijing, China
- 4Beijing Research Institute of Telemetry, Beijing, China
- 5International Joint Innovation Center, ZJU-UIUC Institute, Zhejiang University, Haining, China
Electromagnetic response of clustered charged particles is the foundation of electromagnetic wave interaction with various natural phenomena, such as sandstorm, cloud, and volcano eruption. Previous studies usually employed assumption of independent charged particles, without considering the coupling between them. Here, we build up a general numerical model considering the multiple scattering effect, and test it with a charged two- and four-particle system. The numerical results show that independence assumption fails, while the number density of clustered charged particles is getting larger. This work may pave the way for deeper understanding on the electromagnetic interaction of clustered charged particles.
Introduction
Sand/dust storm is a natural phenomenon that may cause signals of remote sensing and telecommunication being worsened due to the attenuation of electromagnetic waves (EMWs) by sand particles. To understand the attenuation mechanism, much research has been conducted on the scattering of sand storm (Peterson and Strm, 1974; Ansari and Evans, 1982; Haddad et al., 1983; Ghobrial and Jervase, 1997; Islam et al., 2010) with the Lorenz–Mie theory. Notably, particles in the sand storm can be charged due to many reasons, such as friction and collision between particles (Gill, 1948;Gill and Alfrey, 1949; Lowell and Rose-Innes, 1980; Lacks and Mohan Sankaran, 2011; Xie et al., 2013a; Xie et al., 2013b). Existing research has shown that the scattering properties of charged particles are strikingly different from those of neutral ones (Klačka and Kocifaj, 2007; Zhou and Xie, 2011; Kocifaj, 2013; Klačka et al., 2015b; Kocifaj et al., 2015). For example, Klačka et al. found that scattering and absorption will be significantly enhanced by the surface charge for small particles with a size parameter less than 0.01 (Klačka and Kocifaj, 2007). In 1977, Bohren and Hunt put forward a model to solve the scattering problem of a charged particle through revising the boundary condition by introducing an excess charge surface conductivity (Bohren and Hunt, 1977; Bohren and Huffman, 1998). The Drude model that relates the surface conductivity of charged particles to the charge-to-mass ratio of the charge carriers and the driving frequency can be employed to understand the surface currents on the particles (Klačka and Kocifaj, 2007; Kocifaj, 2013; Klačka et al., 2015b).
However, although there is a large number of research conducted on the scattering properties of individual charged particles of different materials and shapes (Klačka and Kocifaj, 2010; Kocifaj et al., 2016; Liao et al., 2019; Yang et al., 2019; Xue et al., 2020), little attention was given on the interactions between charged particles in clusters. It is reported that conventional analytical methods, which assume the granular medium is homogeneous and neglect multiple scattering effects between particles, will lead to significant overestimation of the attenuation through the medium (Huang et al., 2020; Xie et al., 2020). Recently, Xie et al. improved the independent scattering approximation in conventional radiative transfer theory to deal with the multiple scattering effects in a charged granular system (Xie et al., 2020). In 2020, Chai J W et al. analytically studied the optical response of a symmetric circular array of metallic nanoparticles based on the eigen-decomposition method under the dipole approximation (Fung and Chan, 2008; Chai et al., 2020). In this article, we build up a fully analytical theoretical frame based on the T-matrix formulism (Tsang et al., 2001; Mishchenko et al., 2002, 2006; Huang et al., 2020) to study the electromagnetic response of multi-particle systems consisting of electrically charged spherical particles with multiple scattering effect considered. The article is organized as follows: In the Single charged particle section, before the discussion of the multiple scattering effect, we investigated the influence of various factors on the scattering of a single charged particle, such as the charge density, radius, and relative permittivity of the particle. We would like to state that our theoretical frame is under the assumption of uniform charge distribution on the particle surface. Uniform charge distribution is typical for particles of simple geometry such as spherical particles considered in this work, to which separation of variables method is applicable to find scattered electric and magnetic fields (Klačka et al., 2015a). In the Clustered charged particles section, the T-matrix formulism with modified boundary condition to calculate the electromagnetic response of clustered charged spherical particles is described. Then, in the Result section, the discussions on the numerical results of a single charged particle and clustered particles are presented, with comparison between different charge densities and inter-particle distances. Finally, the convergence performance of the proposed theoretical approach is investigated, and conclusions are given in the Conclusion section.
Single charged Particle
A charged spherical particle with radius
where k1 and k2 denote the wavenumber inside and outside the particle, which satisfy the dispersion relation:
where
where
where
However, when it comes to charged particles, the electrons on the surface will be driven by the electric field on the surface. Hence, there exist surface current
The surface current can be expressed as:
where σs denotes the excess charge surface conductivity, and
where
where
where qs/ms is the charge-to-mass ratio of charge carriers. For electrons, we have
The problem of plane waves scattered by a charged particle can then be solved based on a T-matrix-based approach with modified boundary conditions, which is the generalized version of the Lorenz–Mie theory. The scattering of electromagnetic waves by homogeneous, isotropic sphere, which carries excess charge is tackled under the assumption that surface current is linearly related to the electric field parallel to the surface of the particle. The calculated scattering coefficients are, therefore, formally different from those of the standard Mie theory. We first expand the incident wave into spherical harmonics,
where
where jn and hn are the first and third kinds of spherical Bessel function. Rg stands for the regular solution of the spherical harmonics, which means the solution is regular (finite) at the origin. The vector spherical waves without the prefix Rg are the expressions with jn replaced by hn.
where
The Scattered Field can Be Written as
and the internal field of the particle can be written as
As mentioned above,
Solve the equations, and we can obtain scattering coefficients
where
If we consider the surface charge density to be infinity, which will cause the conductivity
Then, the scattering cross section can be expressed as
and the extinction cross section can be obtained by
The scattering cross section implies the amount of energy removed by the scattering of the incidence wave, while the extinction cross section implies the amount of energy removed from the incident energy due to the sum of scattering and absorption by the particle. These cross sections of a single particle can be significantly altered by surface charges, material properties of the particle, and the environment (Wang et al., 2020).
Clustered Charged Particles
Under the classical assumption of independent scattering, the cross section of the multi-particle system can be written as
A general theoretical frame to study the multiple scattering effect in a multi-particle system has been built in previous research (Tsang et al., 2001; Mishchenko et al., 2002, Mishchenko et al. 2006; Altuncu et al., 2019; Huang et al., 2020). Similar to the solid-state analogy, where waves are scattered by crystals lattice, here, lattice sums of spherical harmonic functions are adopted to model the scattering from discrete particles, and the center transformation is implemented with the T-matrix approach combined with the translational addition theorems, given the differences between the Schrödinger and Maxwell equations (Popov, 2012; Tan and Tsang, 2019). Consider N spherical particles with radii a1, a2, a3, …, aN, respectively. The ith particle has permittivity equal to
As mentioned above, for an incident EMW with electric field polarized in x-axis and propagating in z-axis, it has the form
For an arbitrary particle, e.g., the lth particle, we assume the exciting field consisting of two parts: the first part is the original incident wave, and the other is the scattered wave from other particles. The expression for the exciting fields of the lth particle is
where
For the lth particle, the exciting field consists of the incidence wave, and the part that comes from the scattered field of other particles can be expressed as
The electromagnetic field scattered by the jth particle
where
In order to solve this equation, we need to transform all spherical waves on the right side to be centered at
For
For
The expressions of
for l = 1, 2, 3, …N. These equations can be expressed in matrix form as follows:
where
Considering the incident field as plane wave without loss of generality, the multiple scattering equations can be written as
The above equations can be solved by iteration. After solving for the exciting coefficients
After obtaining all scattering coefficients of all particles, the scattered spherical waves centered at different particles should be transformed to be centered at one particular particle. Here, we set the particular center to be
Then, the scattering cross section in charged particle clusters considering the multiple scattering effects is given by
Result
For an incident EMW with frequency between 10 and 200 GHz with electric field polarized in the x direction and propagating in the z direction, EMW has the form
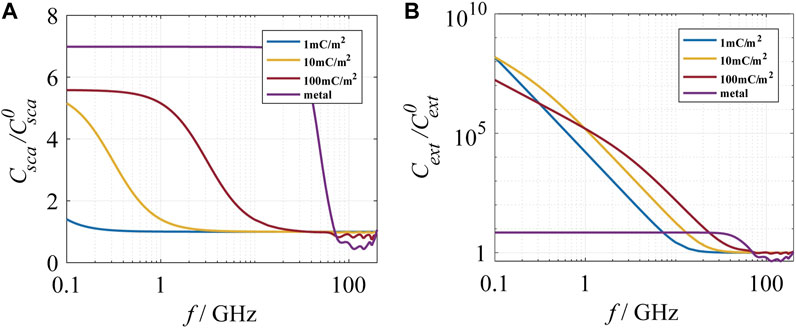
FIGURE 1. The influence of surface charge density on the scattering/extinction cross sections of a single particle. Surface charge densities are set to
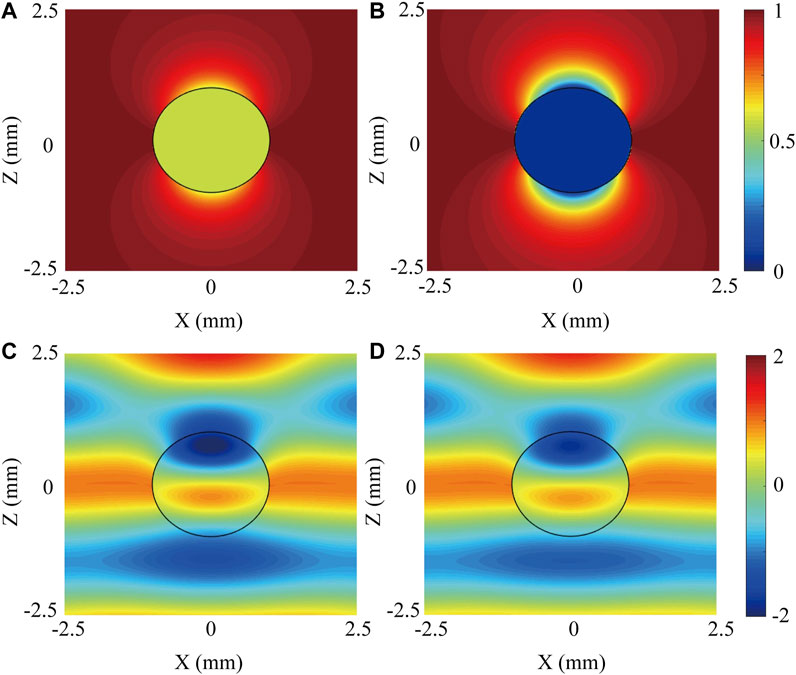
FIGURE 2. Field distributions of neutral and charged particles. The x component of the electric field at 1 GHz (upper panel) and 100 GHz (lower panel) for (A, C) a neutral particle and (B, D) a charged particle with surface charge density
As shown in Figure 1B, compared with the scattering cross section, the extinction cross section is greatly enhanced by the surface charge in the low-frequency range, which satisfies the Rayleigh scattering limit. The enhancement increases when the frequency gets lower. For surface charge density equal to 10 mC/m2, the enhancement of
Next, the influence of the material of the particles on scattering and extinction properties is investigated. For particles with radius
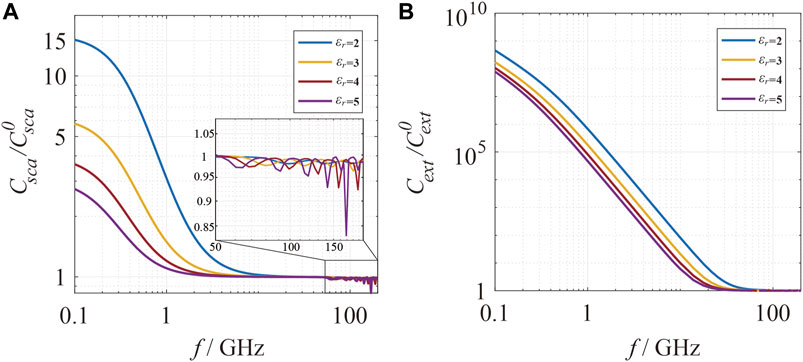
FIGURE 3. The influence of relative permittivity on the scattering/extinction cross sections of a single charged particle. Relative permittivity is set to
The influence of the size of the particles on the scattering and extinction properties is also investigated. For a particle with relative permittivity equal to 3.2 and surface charge density equal to 10 mC/m2, the ratio of scattering cross section and extinction cross section of charged particles to those of the uncharged one are plotted in Figures 4A,B, respectively. The radius of particles varies from 0.01 to 10 mm. It can be seen that for particles with smaller sizes, the enhancement effect by surface charges on the scattering cross section and extinction cross section is enlarged. This is because the enhancement effect is dependent on the bulk complex refractive index
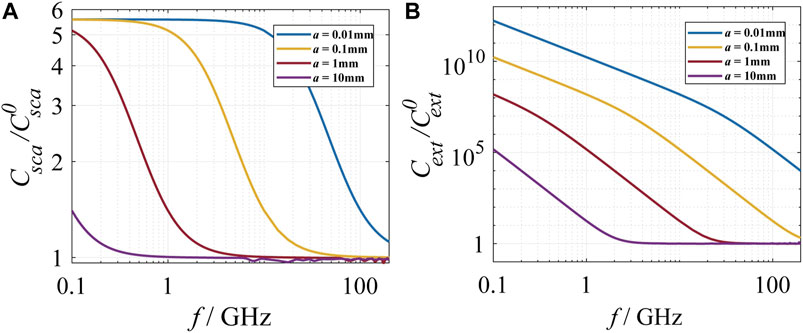
FIGURE 4. The influence of the size of the particles on the scattering/extinction cross sections of a single charged particle. The radii of particles are set to
For a multi-particle system, the dependence of the scattering cross section on the number density and surface charge density of the particles is studied. Under the classical assumption of independent scattering, the scattering cross section can be expressed as
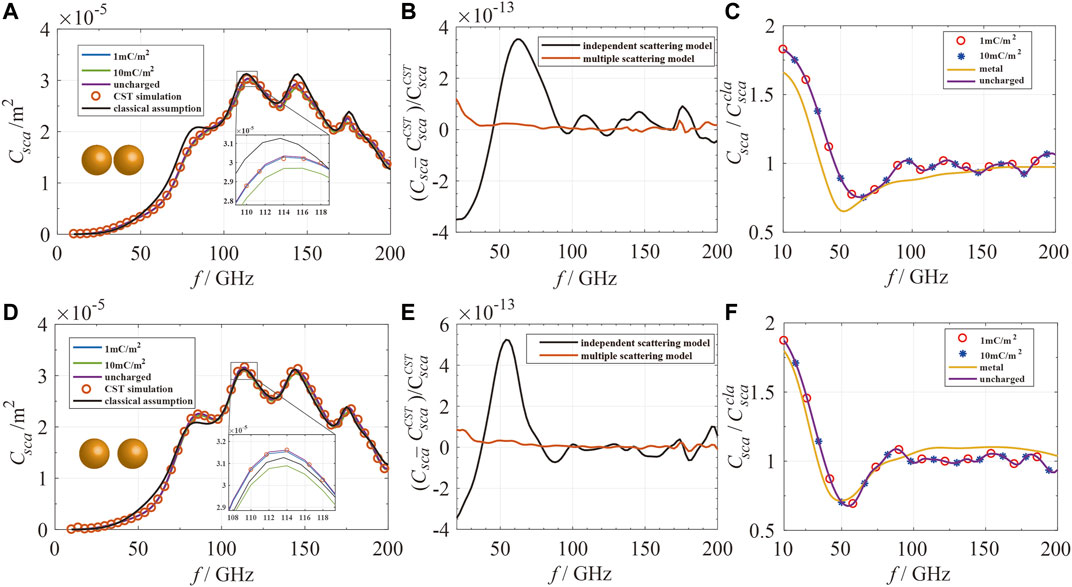
FIGURE 5. The scattering cross sections for two-particle systems with different separations and charge densities. First column: the scattering cross section of two-particle clusters calculated under the multiple scattering method (for uncharged particles charged particles), classical independent scattering assumption, and CST simulation (for uncharged particles) with the radius of particles equal to 1 mm; distances between two particles are (A) 2.2 mm and (D) 3 mm. Second column: the relative differences between the results calculated under classical assumption, multiple scattering model, and CST simulation for uncharged particles for distance equal to (B) 2.2 mm and (E) 3 mm. Third column: the ratio of scattering cross section calculated under the multiple scattering method to the results calculated with the classical assumption for distance equal to (C) 2.2 mm and (F) 3 mm.
There is an obvious difference between the result of multiple scattering method and classical independent scattering method. High accuracy of multiple scattering model can be seen when compared with CST simulation. Using the CST simulation as a benchmark, we calculated the relative difference between the two methods and the simulation to evaluate the accuracy of the multiple scattering model and classical assumption in Figures 5B,E. It can be seen that the multiple scattering model whose result is almost the same as simulation has higher accuracy compared with the classical assumption. As the distance between particles decreases from 3 to 2.2 mm, which is equivalent to increasing the number density, the maximum deviation between the calculated result under classical assumption and the simulation result increase from
Next, to gain deeper insight into the electromagnetic response of clustered charged particles, we investigated the influence of surface charges on the multiple scattering effect. The ratio of the scattering cross section calculated under the multiple scattering model
We then increase the number of particles and validate the T-matrix-based multiple scattering model in a cluster composed of four particles. The pattern of the particle cluster is shown in the inset in Figures 6A,D. Because the distances between two of the particles in the cluster may be different, the distance R from each center of the particle to the center of the cluster is used to indicate the inter-particle distances. As illustrated in Figures 6A,D the scattering cross sections for four-particle clusters with R equals 1.5 and 2 mm, and the particle radius equals 1 mm are almost two times larger compared with the scattering cross section of the two-particle system since the number of particles is doubled. In the inset in Figures 6A,D the scattering spectrum is demonstrated to be suppressed by surface charges, which is in accordance with the results shown in Figures 1A, 5A,D. In Figures 6B,E when R decreases from 2 to 1.5 mm, the mean absolute difference
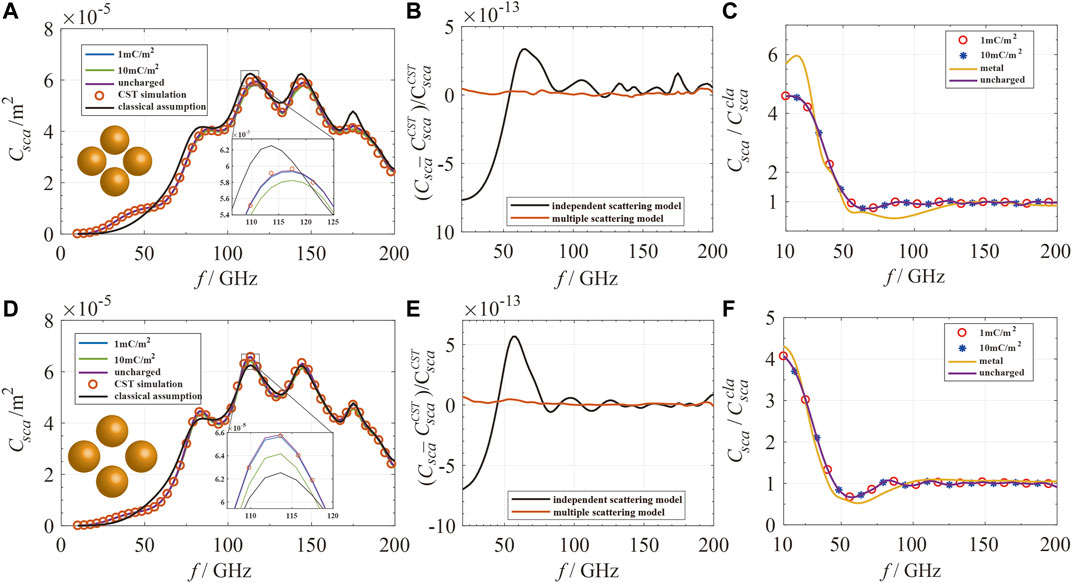
FIGURE 6. The scattering cross sections for four-particle clusters with different separations and charge densities. First column: the scattering cross section of four-particle clusters calculated under the multiple scattering method (for uncharged particles and charged particles), classical independent scattering assumption, and CST simulation (for uncharged particles) with radius of the particles equal to 1 mm, R equals (A) 1.5 mm and (D) 2 mm. Second column: the relative difference between the result calculated under classical assumption, multiple scattering model, and CST simulation for R equal to (A) 1.5 mm and (D) 2 mm. Third column: the ratio of scattering cross section calculated under the multiple scattering method to the results calculated with the classical assumption for R equal to (A) 1.5 mm and (D) 2 mm.
Finally, in Figure 7, we depict the ratio of the scattering cross section calculated under the multiple scattering model to the result under the classical assumption

FIGURE 7. Convergence of the scattering cross section versus the number of iterations and Na. The ratio of scattering cross section calculated under the multiple scattering method to the results calculated with the classical assumption for (A) two-particle clusters and (D) four-particle clusters with different separations between particles indicated by the distance R from the center point of the cluster to an individual particle.
Conclusion
Based on the T-matrix approach, the problem of electromagnetic waves scattered by a single-charged particle can be solved, and the influence of surface charged density and relative permittivity of the particle is investigated. For sizes of particles much smaller than the wavelength of the incident EMWs, the enhancement of extinction cross section by surface charge is enlarged with the higher surface charge density but reduced by higher permittivity of the particle. The enhancement of scattering cross section by surface charge is slightly enlarged by a higher surface charge density and reduced by a higher permittivity of the particle. For a higher-frequency range, the influence of surface charge and relative permittivity on the extinction cross section can be neglected, but the scattering cross section is slightly reduced by higher surface charge and larger relative permittivity of the particle. With the increase in the size of the particles, the enhancement of scattering/extinction cross sections due to the surface charges is smaller.
Next, exploiting the T-matrix approach based on the addition theorem and the iterative method, the multiple scattering problem of clustered charged particles can be solved. The influence of inter-particle distances and surface charge density on the multiple scattering effect is investigated. Scattering cross sections of two-particle systems and four-particle clusters are calculated via the multiple scattering method with varying inter-particle distances. The deviation from the result calculated under the classical independent scattering assumption is obvious when inter-particle distance is smaller than three times that of the particle radius and is further enlarged as particles are getting closer. Surface charge density has little influence on the multiple scattering effect within several hundred mC/m2.
Data Availability Statement
The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.
Author Contributions
WY did the calculation and implementation of the MATLAB program, XH, SZ and CW did the mathematical derivation, JJ, TY and FG did the simulation verification.
Funding
This work was supported by the National Natural Science Foundation of China(NNSFC) under Grants No. 51777213, National Key Laboratory Foundation No. 6142205200402, the Fundamental Research Funds for the Central Universities No. 2020XZZX002-15, Beijing Information science and technology university Research Grants No. 2025037.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Altuncu, Y., Durukan, T., and Akdogan, R. E. (2019). Reconstruction of Two-Dimensional Objects Buried into Three-Part Space with Locally Rough Interfaces via Distorted Born Iterative Method. Pier 166, 23–41. doi:10.2528/PIER19072203
Ansari, A. J., and Evans, B. G. (1982). Microwave Propagation in Sand and Dust Storms. IEE Proc. F Commun. Radar Signal. Process. UK 129, 315–322. doi:10.1049/ip-f-1.1982.0047
Bohren, C. F., and Huffman, D. R. (1998). Absorption and Scattering of Light by Small Particles. Hoboken, NJ: John Wiley & Sons.
Bohren, C. F., and Hunt, A. J. (1977). Scattering of Electromagnetic Waves by a Charged Sphere. Can. J. Phys. 55, 1930–1935. doi:10.1139/p77-235
Bruning, J., and Yuen Lo, L. (1971). Multiple Scattering of EM Waves by Spheres Part I--Multipole Expansion and ray-optical Solutions. IEEE Trans. Antennas Propagat. 19, 378–390. doi:10.1109/TAP.1971.1139944
Chai, J., Ge, L., Hu, P., Xiang, H., and Han, D. (2020). Angle-dependent Optical Response of the Plasmonic Nanoparticle Clusters with Rotational Symmetry. Opt. Express 28, 10425–10437. doi:10.1364/OE.388590
Cruzan, O. R. (1962). Translational Addition Theorems for Spherical Vector Wave Functions. Quart. Appl. Math. 20, 33–40. doi:10.1090/qam/132851
Fung, K. H., and Chan, C. T. (2008). Analytical Study of the Plasmonic Modes of a Metal Nanoparticle Circular Array. Phys. Rev. B 77, 205423. doi:10.1103/PhysRevB.77.205423
Ghobrial, S. I., and Jervase, J. A. (1997). Microwave Propagation in Dust Storms at 10.5 GHz - A Case Study in Khartoum, Sudan, E80-B. Tokyo, Japan: IEICE Transactions on Communications., 1722–1727.
Gill, E. W. B., and Alfrey, G. F. (1949). Frictional Electrification. Nature 163, 172. doi:10.1038/163172a0
Gill, E. W. B. (1948). Frictional Electrification of Sand. Nature 162, 568–569. doi:10.1038/162568b0
Haddad, S., Salman, M. J. H., and Jha, R. K. (1983). Effects of Dust/sandstorm on Some Aspects of Microwave Propagation. Proc. ESA Spec. Publ. 194, 153–162.
Holloway, C. L., Kuester, E. F., and Haddab, A. H. (2019). Retrieval Approach for Determining Surface Susceptibilities and Surface Porosities of a Symmetric Metascreen from Reflection and Transmission Coefficients. Pier 166, 1–22. doi:10.2528/PIER19022305
Huang, H., Tsang, L., Colliander, A., Shah, R., Xu, X., and Yueh, S. (2020). Multiple Scattering of Waves by Complex Objects Using Hybrid Method of T-Matrix and Foldy-Lax Equations Using Vector Spherical Waves and Vector Spheroidal Waves. Pier 168, 87–111. doi:10.2528/PIER20080409
Islam, M. R., Omer Elshaikh, Z. E., Khalifa, O. O., Alam, A. Z., Khan, S., and Naji, A. W. (2010). Prediction of Signal Attenuation Due to Duststorms Using Mie Scattering. Iiumej 11, 71–87. doi:10.31436/iiumej.v11i1.42
Klačka, J., Kocifaj, M., Kundracik, F., Videen, G., and Kohút, I. (2015b). Generalization of Electromagnetic Scattering by Charged Grains through Incorporation of Interband and Intraband Effects. Opt. Lett. 40, 5070–5073. doi:10.1364/OL.40.005070
Klačka, J., Kocifaj, M., Kundracik, F., and Videen, G. (2015a). Optical Signatures of Electrically Charged Particles: Fundamental Problems and Solutions. J. Quantitative Spectrosc. Radiative Transfer 164, 45–53. doi:10.1016/j.jqsrt.2015.05.009
Klacka, J., and Kocifaj, M. (2010). On the Scattering of Electromagnetic Waves by a Charged Sphere. Pier 109, 17–35. doi:10.2528/PIER10072708
Klačka, J., and Kocifaj, M. (2007). Scattering of Electromagnetic Waves by Charged Spheres and Some Physical Consequences. J. Quantitative Spectrosc. Radiative Transfer 106, 170–183. doi:10.1016/j.jqsrt.2007.01.016
Kocifaj, M., Klačka, J., Kundracik, F., and Videen, G. (2015). Charge-induced Electromagnetic Resonances in Nanoparticles. Annalen Der Physik 527, 765–769. doi:10.1002/andp.201500202
Kocifaj, M., Kundracik, F., and Videen, G. (2016). Optical Characterization of Electrically Charged Particles Using Discrete Dipole Approximation. J. Quantitative Spectrosc. Radiative Transfer 184, 161–166. doi:10.1016/j.jqsrt.2016.07.010
Kocifaj, M. (2013). Theoretical Conditions for Charge-Induced normal Modes in Spherical Particles. Laser Phys. Lett. 10, 055901. doi:10.1088/1612-2011/10/5/055901
Lacks, D. J., and Mohan Sankaran, R. (2011). Contact Electrification of Insulating Materials. J. Phys. D: Appl. Phys. 44, 453001. doi:10.1088/0022-3727/44/45/453001
Liao, T.-H., Ding, K.-H., and Tsang, L. (2019). Broadband Green's Function with Higher Order Low Wavenumber Extractions for an Inhomogeneous Waveguide with Irregular Shape. Pier 164, 75–95. doi:10.2528/PIER18102903
Lovat, G., Burghignoli, P., Araneo, R., Celozzi, S., Andreotti, A., Assante, D., et al. (2020). Shielding of an Imperfect Metallic Thin Circular Disk: Exact and Low-Frequency Analytical Solution. Pier 167, 1–10. doi:10.2528/PIER19090908
Lowell, J., and Rose-Innes, A. C. (1980). Contact Electrification. Adv. Phys. 29, 947–1023. doi:10.1080/00018738000101466
Mishchenko, M. I., Travis, L. D., and Lacis, A. A. (2006). Multiple Scattering of Light by Particles: Radiative Transfer and Coherent Backscattering. Cambridge: Cambridge University Press.
Mishchenko, M. I., Travis, L. D., and Lacis, A. A. (2002). Scattering, Absorption, and Emission of Light by Small Particles. Cambridge: Cambridge University Press.
Peterson, B., and Strm, S. (1974). T-matrix Formulation of Electromagnetic Scattering from Multilayered Scatterers. Phys. Rev. D 10. doi:10.1103/physrevd.10.2670
Tan, S., and Tsang, L. (2019). Efficient Broadband Evaluations of Lattice Green's Functions via Imaginary Wavenumber Components Extractions. Pier 164, 63–74. doi:10.2528/PIER18102001
Tsang, L., Kong, J. A., Ding, K. H., and Ao, C. O. (2001). Scattering of Electromagnetic Waves: Numerical Simulations. Hoboken, NJ: John Wiley & Sons.
Tsang, L., Kong, J. A., and Ding, K. H. (2000). Scattering of Electromagnetic Waves: Theories and Applications. Hoboken, NJ: John Wiley & Sons.
Tsang, L., and Kong, J. A. (1983). Scattering of Electromagnetic Waves from a Half Space of Densely Distributed Dielectric Scatterers. Radio Sci. 18, 1260–1272. doi:10.1029/RS018i006p01260
Wang, C., Qian, C., Hu, H., Shen, L., Wang, Z., Wang, H., et al. (2020). Superscattering of Light in Refractive-Index Near-Zero Environments. Pier 168, 15–23. doi:10.2528/PIER20070401
Xie, L., Han, K., Ma, Y., and Zhou, J. (2013a). An Electrification Mechanism of Sand Grains Based on the Diffuse Double Layer and Hertz Contact Theory. Appl. Phys. Lett. 103, 104103. doi:10.1063/1.4819948
Xie, L., Li, G., Bao, N., and Zhou, J. (2013b). Contact Electrification by Collision of Homogenous Particles. J. Appl. Phys. 113, 184908. doi:10.1063/1.4804331
Xie, L., Zhong, H., Du, Z., and Zhou, J. (2020). Monte Carlo Simulation of Electromagnetic Wave Transmittance in Charged Sand/dust Storms. J. Quantitative Spectrosc. Radiative Transfer 241, 106744. doi:10.1016/j.jqsrt.2019.106744
Xue, Z., Wu, Y. M., Chew, W. C., Jin, Y.-Q., and Boag, A. (2020). The Multilevel Fast Physical Optics Method for Calculating High Frequency Scattered Fields. Pier 169, 1–15. doi:10.2528/PIER20071203
Yang, P., Ding, J., Panetta, R. L., Liou, K.-N., Kattawar, G. W., and Mishchenko, M. I. (2019). On the Convergence of Numerical Computations for Both Exact and Approximate Solutions for Electromagnetic Scattering by Nonspherical Dielectric Particles (Invited Review). Pier 164, 27–61. doi:10.2528/PIER18112810
Zhang, J., Hu, X., Chen, H., and Gao, F. (2020). Designer Surface Plasmons Enable Terahertz Cherenkov Radiation (Invited). Pier 169, 25–32. doi:10.2528/PIER20102708
Zhou, J., Dou, X., and Xie, L. (2018). Scattering and Attenuation of Electromagnetic Waves by Partly Charged Particles. J. Quantitative Spectrosc. Radiative Transfer 206, 55–62. doi:10.1016/j.jqsrt.2017.11.006
Keywords: addition theorem, mie scattering, charged particles, multiple scattering, scattering cross section
Citation: Ye W, Hu X, Zhou S, Wang C, Jiang J, Yang T and Gao F (2021) Electromagnetic Response of Clustered Charged Particles. Front. Mater. 8:739116. doi: 10.3389/fmats.2021.739116
Received: 10 July 2021; Accepted: 14 October 2021;
Published: 15 November 2021.
Edited by:
Yu Luo, Nanyang Technological University, SingaporeReviewed by:
Dezhuan Han, Chongqing University, ChinaCai Tong, Zhejiang University, China
Hao Chi Zhang, Southeast University, China
Copyright © 2021 Ye, Hu, Zhou, Wang, Jiang, Yang and Gao. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Xiaofeng Hu, c25vd2ZveDIyNzBAMTYzLmNvbQ==; Jing Jiang, amlhbmdqaW5nMDEzMEBiaXN0dS5lZHUuY24=; Fei Gao, Z2FvZmVpemp1QHpqdS5lZHUuY24=
 Wenzheng Ye1
Wenzheng Ye1 Jing Jiang
Jing Jiang Fei Gao
Fei Gao