Plasmonic Modes in Thin Films: Quo Vadis?

Politano, Antonio; Chiarello, Gennaro

doi:10.3389/fmats.2014.00009

PERSPECTIVE article

Front. Mater., 28 July 2014

Sec. Thin Solid Films

Volume 1 - 2014 | https://doi.org/10.3389/fmats.2014.00009

Plasmonic modes in thin films: quo vadis?

$\r\n Antonio Politano*$ Antonio Politano* $Gennaro Chiarello\r\n$ Gennaro Chiarello

Dipartimento di Fisica, University of Calabria, Cosenza, Italy

Herein, we discuss the status and the prospect of plasmonic modes in thin films. Plasmons are collective longitudinal modes of charge fluctuation in metal samples excited by an external electric field. Surface plasmons (SPs) are waves that propagate along the surface of a conductor with applications in magneto-optic data storage, optics, microscopy, and catalysis. In thin films, the electronic response is influenced by electron quantum confinement. Confined electrons modify the dynamical screening processes at the film/substrate interface by introducing novel properties with potential applications and, moreover, they affect both the dispersion relation of SP frequency and the damping processes of the SP. Recent calculations indicate the emergence of acoustic surface plasmons (ASPs) in Ag thin films exhibiting quantum well states and in graphene films. The slope of the dispersion of ASP decreases with film thickness. We also discuss open issues in research on plasmonic modes in graphene/metal interfaces.

Plasmons in low-dimensional systems never cease to amaze with new astonishing findings, although it has quite a long history, started with the discovery of surface plasmons (SPs) in thin films by Ritchie (1957).

Recently, novel modes, such as sheet (Langer et al., 2011; Politano et al., 2012a), Dirac (Fei et al., 2011; Stauber, 2014), and acoustic surface plasmons (ASPs) (Politano et al., 2011; Yuan et al., 2011) and, moreover, plasmarons (Krstajic and Peeters, 2013), have been observed in low-dimensional systems. Such excitations are supported by the two-dimensional electron gas (2DEG). The great interest toward plasmons arises from the exceptional range of the possible applications of plasmonics.

To date, plasmonic devices based on noble metals (Ag and Au) are widely diffused (Nyga et al., 2008; Pala et al., 2009). Nevertheless, current research is oriented toward the realization of graphene-based plasmonic devices. In fact, plasmons in graphene offer promising prospect of applications covering a wide frequency range, going from terahertz up to the visible (Vicarelli et al., 2012; García de Abajo, 2014).

Nanoscale thin films are an ideal playground for manipulating plasmon properties by peculiar phenomena occurring in thin films, such as quantum size effects (Hamawi et al., 1991; Wei and Chou, 2002) and quantum electron confinement (Ogando et al., 2005; Politano and Chiarello, 2010). Film morphology may originate plasmon confinement within disordered grains (Moresco et al., 1999) or periodic nanodomes (Politano et al., 2013a). Herein, the open challenges regarding plasmons modes in thin films will be presented to the reader, with a particular attention for the cases with higher prospect for plasmonic applications, i.e., noble metal (Ag and Au) and graphene films.

As a general rule, the electromagnetic fields of both sides forming an interface interact in such a way that the SP splits into two plasmonic excitations in which electron may oscillate in phase or not. For a Drude thin slab in vacuum of thickness a (Pitarke et al., 2007), the dispersion relations of these modes can be obtained by applying appropriate boundary conditions and solving Maxwell’s equations (Raether, 1980):

ω = \frac{ω_{p}}{\sqrt{2}} {(1 \pm e^{- qa})}^{1 ∕ 2}

(1)

The high energy plasmon in the Figure 1A has anti-symmetric field distribution, whereas the low-energy one has symmetric field distribution.

FIGURE 1

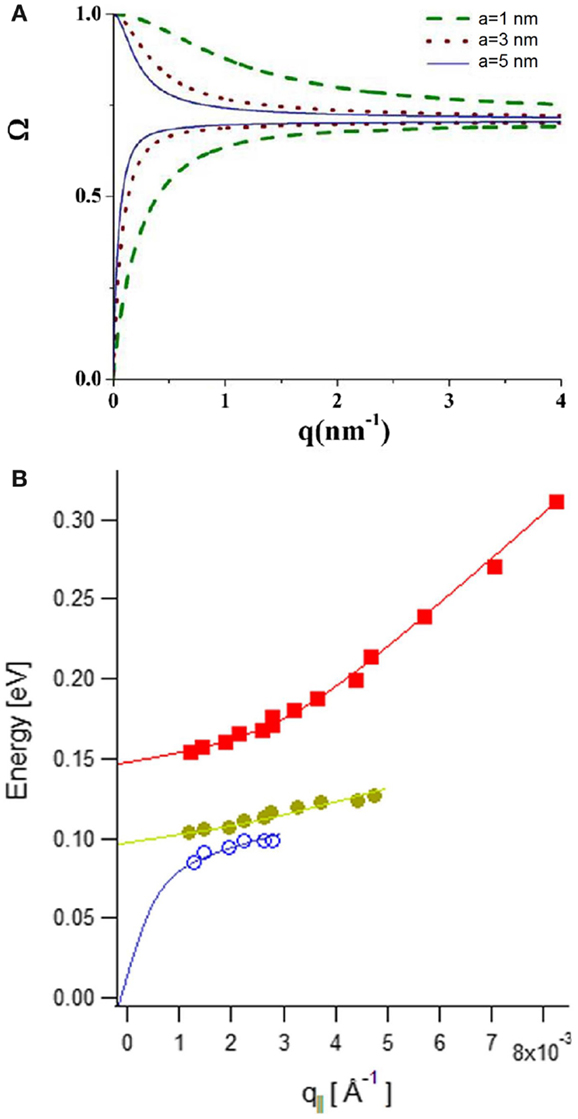
Figure 1. (A) Effects of thickness on the solution of the Eq. 1 for plasmon frequencies in thin films of thickness a. Adapted from Ali and Elham (2013); (B) Plasmon dispersion in graphene on SiO₂. The interaction of optical phonons of SiO₂ with plasmons modifies the dispersion calculated following Eq. 1. Adapted from Yan et al. (2013).

At short wavelengths (qa ≫ 1), the surface waves become decoupled and each surface sustains independent oscillations at the reduced frequency ω_s = ω_p/√ 2 characteristic of a semi-infinite electron gas with a single plane boundary. At long wavelengths (qa ≪ 1), there are normal oscillations at ω_p and tangential 2D oscillations at:

ω_{2 D} = {(2π naq)}^{1 ∕ 2}

(2)

which were later discussed by Stern (1967) and observed in artificially structured semiconductors (Allen et al., 1977) and, more recently, in a metallic surface-state band on a silicon surface (Nagao et al., 2001a,b).

The plasmon dispersion in Eq. 1 is modified by the interaction with phonons. Plasmon–phonon coupling is a striking manifestation of the breakdown of the Born–Oppenheimer approximation (Jablan et al., 2011), with consequences on transport (Tediosi et al., 2007) properties. The plasmon–phonon coupling phenomenon implies the hybridization of the plasmon modes of the 2DEG with the optical phonon modes, giving rise to the coupled plasmon–phonon modes (shown in Figure 1B for the sample case of graphene/SiO₂).

Concerning interfaces, different authors have invoked the existence of interface plasmons (Layet et al., 1986). Ahlqvist et al. (1982) have studied the electrodynamics of the interface between two semi-infinite electron gases, finding that the interface plasmon is characterized by

ω_{i}^{2} = (ω_{1}^{2} + ω_{2}^{2}) ∕ 2

(3)

where ω_i, ω₁, and ω₂ are the frequencies of the interface plasmon and of the two semi-infinite electron gases, respectively. Jewsbury and Summerside (1980) have suggested that an “interface plasmon” is not a pure mode but arises from the electronic band structure at the interface.

However, the traditional theoretical approach used to describe plasmons in thin films, based on Eqs 1 and 2 and on interface plasmons (Eq. 3) is inadequate to describe the extraordinary complexity of plasmon modes at interfaces. Thus, the overall encouraging viewpoint for plasmonic applications is also accompanied by the possibility to carry out many other fascinating fundamental studies.

As an example, the strain resulting from the lattice mismatch between adlayer and substrate (Schell-Sorokin and Tromp, 1990; Sander et al., 1998) may further affect plasmonic excitations. Additional collective electronic modes may be induced by strain, as found by Pellegrino et al. (2010) for the case of graphene. However, experimental studies are still lacking due to the difficulties in following strain effects on plasmonic excitations.

Moreover, the influence of electron quantum confinement (presence of quantum well states, QWS) on the SP is still not clearly established. Theoreticians (Yuan and Gao, 2008) and experimentalists (Yu et al., 2005; Politano et al., 2009) have put in evidence the influence of QWS on the plasmon lifetime in films. Due to the opening of the decay channel of the SP into electron-hole pairs via interband transitions involving QWS, the line-width of the SP assumes an unusual dispersion relation as a function of the momentum transfer, as compared with the case of bulk samples. The effects of QWS on plasmon dispersion have been studied only for a few systems. In Politano et al. (2008) and Politano and Chiarello (2009), it has been shown that the screening properties are influenced by the presence of the modified electron distribution in the presence of QWS. However, rigorous and satisfactory theoretical description is still missing.

The presence of QWS and the subsequent enhanced SP density of states around the Fermi level in thin films may also increase the cross section for the excitation of intrinsically free-electron plasmons, such as the multipole surface plasmon (MP) (Liebsch, 1998). The nature of MP has been understood for alkali metals (Tsuei et al., 1990, 1991; Sprunger et al., 1992; Zielasek et al., 2006), alkaline-earth metals (Sprunger et al., 1992), and aluminum (Chiarello et al., 2000). Unfortunately, contradictory results are reported for the most popular plasmonic systems (Ag and Au). Calculations based on a s–d polarization model by Liebsch (1998) predicted the existence of the Ag and Au MP near ω_m = 0.8 ⋅ ω_p = 7.2 eV (ω_p = 9.0 eV is the s-electron bulk plasmon energy for both Ag and Au) as the density profile at the surface has predominantly s-electron character. Experiments on bulk Ag have not found this mode (Moresco et al., 1996; Barman et al., 2004a,b). In contrast, the Ag MP has been recently measured in Ag films on Ni(111). However, such excitation is revealed only in experimental conditions enhancing the surface sensitivity, i.e., at low impinging energies and grazing incidence (Politano et al., 2013b,d), in agreement with Liebsch’s prediction (Liebsch, 1998). Therefore, electron quantum confinement in Ag 5 sp-derived QWS (Miller et al., 1994) enhances the cross section for Ag MP excitation in thin films compared with semi-infinite media (bulk samples).

Another open issue is related to the possible existence of acoustic plasmon modes in thin films. Unfortunately, to date no experimental works exist on this topic, while from the theoretical side Silkin et al. (2011) have shown that ASP emerge in the electronic response of thin Ag films. The presence of Ag QWS in ultrathin films induces the appearance of ASP, whose dispersion is determined by the QWS band. The slope of the dispersion relation decreases with film thickness.

The surface response function (Figure 2A) for film thickness higher than three layers shows an additional feature at about 2 eV, which correspond to interband transitions between energy-split SS⁺ and SS⁻ electronic states (interband SP, ISP). In contrast with ASP, the ISP energy has finite value at q = 0. Moreover, the ISP energy decreases with increasing thickness and it merges with the ASP at higher thickness.

FIGURE 2

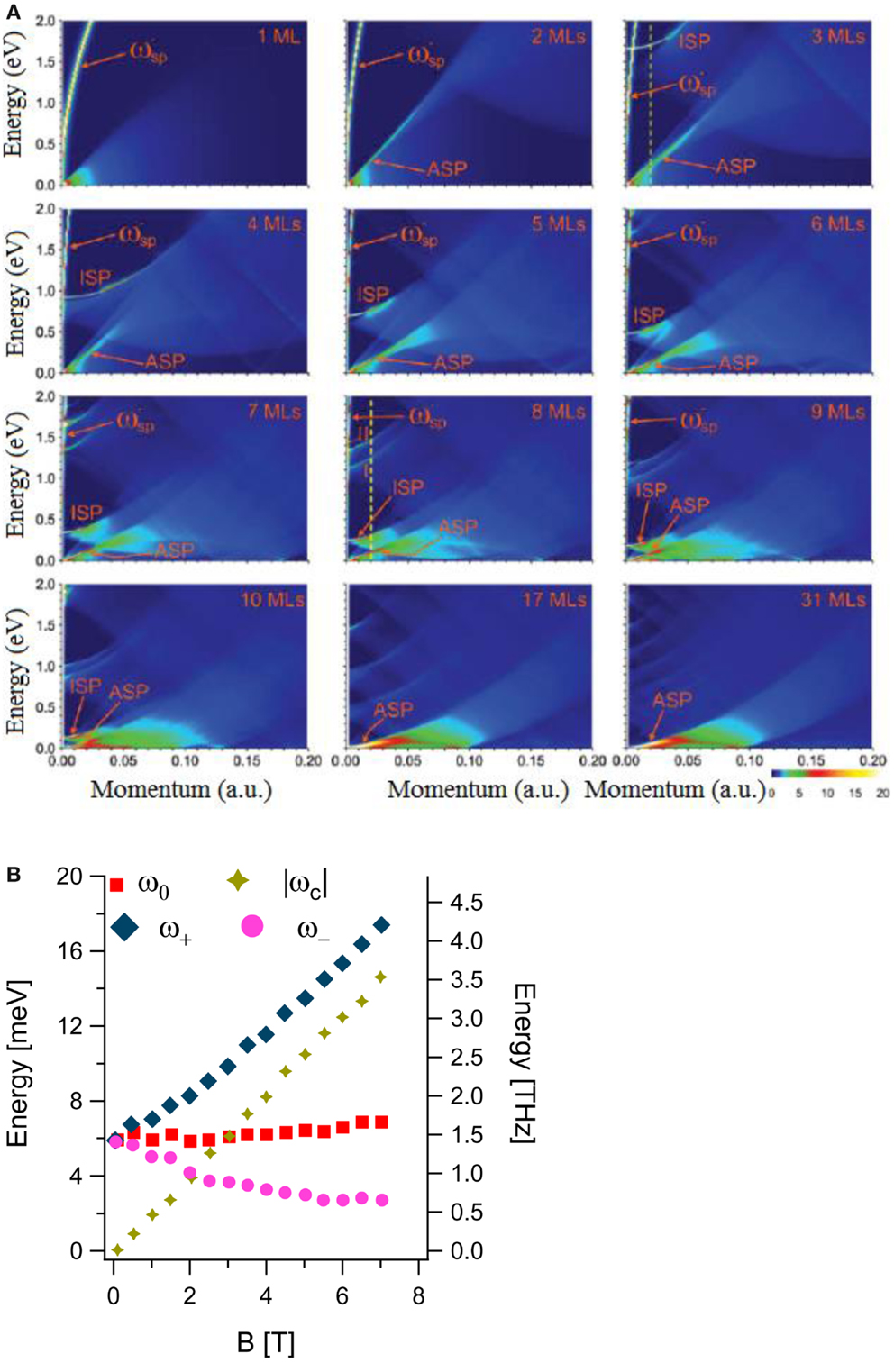
Figure 2. (A) Normalized surface loss function Im[g(q,ω)]/qω for Ag(111) films with thickness ranging from 1 to 31 monolayers (ML) evaluated by using realistic effective masses in energy band dispersions. Note the strongly dispersing mode corresponding to a conventional $ω_{SP}^{-}$ mode of a thin film. Peaks denoted with “ISP” are originated from the interband transition between the energy-split quantum states. Adapted from Silkin et al. (2011). (B) Dependence of the plasmon energy ω₀, the cyclotron resonance energy ω_C and the magnetoplasmon energies ω_± on the magnetic field B. Adapted from Crassee et al. (2012).

Acoustic surface plasmon owes its existence to the spatial coexistence of a 2DEG with a 3D electron gas. It has been also predicted to exist at the K/Be interface (Echeverry et al., 2010; Silkin et al., 2010a,b). The screening by the underlying metal substrate change the square-root-like dispersion of the 2D plasmon into linear.

Concerning graphene films, the most puzzling open issues are related to plasmonic modes in graphene/metal interfaces. Due to the difficulty in the theoretical description of the screening by the underlying metal substrate, accurate theoretical models for plasmons in graphene/metal interfaces are still missing. The out-of-plane charge transfer between graphene and the metal is determined by the difference between the work function of graphene and the metal surface and, in addition, by the metal–graphene chemical interaction that creates an interface dipole lowering the metal work function. The induced electrostatic potential decays weakly with the distance from the metal contact as V(x) ≈ x^−1/2 and ≈x⁻¹ for undoped and doped graphene, respectively (Khomyakov et al., 2010). Instead, current models overestimate the screening by the metal substrate. Likely, the experimental study of plasmons in graphene deposited on jellium surfaces (Al) could help theoreticians to improve our understanding of screening processes at graphene/metals. Unfortunately, such experimental study is complicated by the difficult preparation of graphene on jellium surfaces.

Low-energy intraband plasmon in graphene is currently well understood (Shin et al., 2011; Stauber and Gómez-Santos, 2012b; Stauber, 2014). In contrast, theoretical models hitherto fail to describe the nature of a non-linear mode observed at ~0.5 eV (Politano and Chiarello, 2014) and, moreover, the quadratic dispersion of interband plasmon (Generalov and Dedkov, 2012; Politano et al., 2012b) in graphene/metal interfaces. The dispersion of the interband plasmon is instead linear in both free-standing graphene (Kramberger et al., 2008) and Cs-decoupled graphene/Ni(111) (Cupolillo et al., 2013b; Ligato et al., 2013). The observation of the change of the interband plasmon from linear to quadratic as a function of the number of graphene layers on silicon carbide (Lu et al., 2009) may in principle afford important information for shedding light on the still confusing state-of-the-art of plasmon modes in epitaxial graphene. However, theoretical models describing the increasing wealth of experimental results on interband plasmons are yet missing.

Moreover, experimental studies on plasmons in bilayer graphene grown on metals would be essential to verify and improve current theoretical models for both plasmon dispersion (Wang and Chakraborty, 2007; Sensarma et al., 2010; Stauber and Gómez-Santos, 2012a; Roldán and Brey, 2013) and plasmaron formation (Van-Nham and Holger, 2012; Krstajic and Peeters, 2013).

Finally, another intriguing topic is magnetoplasmonics, which recently is attracting huge interest for its potential applications in technology (Belotelov et al., 2011; Bonanni et al., 2011). The 2D magnetoplasmons are collective excitations between Landau levels (Lozovik and Sokolik, 2012). They can be observed through infrared optical absorption and inelastic light scattering (Kallin and Halperin, 1984; Oji and MacDonald, 1986; Cinà et al., 1999; Eriksson et al., 1999; Bychkov and Martinez, 2002; Li and Zhai, 2011). In layered and doped graphene structures, the instability and unusual dispersion of magnetoplasmon modes have been studied in recent years, within different approaches (Tahir and Sabeeh, 2007; Berman et al., 2008, 2009; Bychkov and Martinez, 2008a,b; Fischer et al., 2009, 2010; Roldán et al., 2009; Tahir et al., 2011; Wu et al., 2011; Bisti and Kirova, 2012; Ferreira et al., 2012; Lozovik and Sokolik, 2012; Wang et al., 2012; Yan et al., 2012; Chamanara et al., 2013a,b; Petkovic et al., 2013). Magnetoplasmons have been observed in graphene epitaxially grown on SiC (Crassee et al., 2012). The Drude absorption is transformed into a strong terahertz plasmonic peak due to nanoscale inhomogeneities, such as substrate terraces and wrinkles. Plasmonic excitations also modify the magneto-optical response and, in particular, the Faraday rotation (Crassee et al., 2012). This makes graphene a unique playground for plasmon-controlled magneto-optical phenomena thanks to a cyclotron mass, which is two orders of magnitude smaller than in conventional plasmonic materials, such as noble metals.

The field-induced splitting of the plasmon peak resembles strikingly the appearance of collective resonances previously observed in other systems (Allen et al., 1983; Glattli et al., 1985; Mast et al., 1985; Kukushkin et al., 2003). The upper and lower branches are attributed to the so-called bulk and edge magnetoplasmons, respectively, with the frequencies

ω_{\pm} = \sqrt{\frac{ω_{c}^{2}}{4} + ω_{0}^{2}} \pm \frac{| ω_{c} |}{2}

where ω₀ is the plasmon frequency at 0 field, ω_c = ±eB/mc is the cyclotron frequency, defined as positive for electrons and negative for holes, m is the cyclotron mass, and c the speed of light. At high fields (|ω_c| ≫ ω₀), the upper branch becomes essentially the usual cyclotron resonance with a linear dependence on magnetic field, while the lower branch represents a collective mode confined to the edges (Fetter, 1985) with the energy inversely proportional to the field (Figure 2B).

In conclusion, issues discussed herein provide the grounds for theoretical studies aimed at characterizing in more details how growth mode, quantum size effects, and the electron quantum confinement within the adlayer influence the dispersion and the lifetime of collective excitations in nanoscale thin films.

The comprehension of plasmonic excitations in thin films (Chiarello et al., 1997a,b), especially of noble metals (Politano and Chiarello, 2009; Politano, 2012a,b, 2013) and graphene (Politano et al., 2011, 2012a,b, 2013a,c; Cupolillo et al., 2012, 2013a,b; Politano and Chiarello, 2013a,b, 2014), could keep active researchers for a long time.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

Ahlqvist, P., Monreal, R., Flores, F., and Garcia-Moliner, F. (1982). Interface plasmons at the boundary of two semi-infinite electron gases. Phys. Scripta 26, 35. doi: 10.1088/0031-8949/26/1/006