- 1Southern Marine Science and Engineering Guangdong Laboratory (Zhuhai), School of Marine Sciences, Sun Yat-sen University, Zhuhai, China
- 2Guangdong Provincial Key Laboratory of Marine Resources and Coastal Engineering, Guangzhou, China
- 3Pearl River Estuary Marine Ecosystem Research Station, Ministry of Education, Zhuhai, China
Reclamation is one of the most prominent anthropogenic activities affecting tidal flat morphology and the related ecosystem service. Two representative types of reclamation are upper-flat enclosure and lower-flat enrockment. From a historical perspective, different type of reclamation was adapted in different areas in ancient China. As previous studies on reclamation are often site-specific, the reason that leads to such a difference is unclear. The intertidal dynamic equilibrium theory (DET) provides a comprehensive framework for this quest. Here, we extend the DET with additional effects of reclamation. A model based on DET (DET-ESTMORF) was applied to investigate the impact of reclamation on tidal flats. The model was validated by comparing our results against previous records and observations. Results show that both types of reclamation induce morphological adjustment by enhancing local accretion, which upset the previous equilibrium. Specifically, upper-flat enclosure drives tidal flats into evolution toward wave dominance, whereas lower-flat enrockment causes adjustment toward tidal dominance. Next, the impact of reclamation on tidal flats in different states (prograding or retreating) was investigated. We show that both enclosure and enrockment can induce accretion despite the varying wave climate and sediment supply, with the mean accretion rate raised by 1.8 cm/year and 1.2 cm/year, respectively. However, the resulting profiles are different in shape, especially on retreating flats. Finally, we point out that tidal range and sediment supply are the potential reasons affecting the choice of reclamation types in practices.
Introduction
Coastal wetlands like tidal flats are experiencing great pressure and becoming more vulnerable due to global climate change, reduced fluvial sediment supply, and intense coastal urbanization (Syvitski et al., 2009; Kirwan and Megonigal, 2013; Murray et al., 2019). Sea level rise and the growing trend of wave height are increasing risks of coastal flooding and storm surges (Vitousek et al., 2017; Young and Ribal, 2019). Construction of dams and reservoirs in river basins over the past 50 years has dramatically decreased sediment reaching estuaries (over 100 billion metric tons), leading to massive erosion of coasts worldwide (Syvitski et al., 2005, 2009). While the consequences of these processes are noticeable on a decadal or centennial scale, direct human activities (e.g., reclamation) in coastal regions driven by economic development can, within a few years or decades, lead to substantial changes in coastal landscapes and shorelines (Tian et al., 2016; Wang et al., 2021). Many countries have reclaimed coastal wetlands for agriculture, aquaculture, industrial use, and urban expansion for centuries, including the United Kingdom (Doody, 2004), the United States (Kennish, 2001), Netherlands (Hoeksema, 2007), and China (Ma et al., 2014; Wang et al., 2014). Around the globe, this practice has caused 25–50% loss of coastal wetlands over the last 50–100 years, and the loss continues at present days, with around 8,000 km2 each year (Pendleton et al., 2012).
Coastal reclamation refers to the process of creating new land from seas and coastal wetlands (Nadzir et al., 2014). Here, we focus on two types of reclamation carried out on tidal flats: upper-flat enclosure and lower-flat enrockment. Other types (e.g., artificial islands) are not considered in this study. Upper-flat enclosure is employed in places where the elevation is high enough to construct dikes readily (i.e., near high waterline), usually on sediment-rich coasts with large-scale tidal flats (e.g., in Jiangsu, China). Since building dikes on the upper flat can directly convert tidal flats into agricultural land, it has been carried out for centuries as a major way to utilize coastal wetlands. Another method is lower-flat enrockment, which facilitates sedimentation by building submerged breakwaters on the lower flat near the low waterline. This practice is often followed by building dikes or planting coastal vegetation to stabilize sediment. It has a long history of practice in the Pearl River Delta since the Song Dynasty and has accelerated the seaward progradation of coastline (Xiong et al., 2020). It is noteworthy that the utilization of the two types of reclamation varied in different regions in ancient China. Specifically, upper-flat enclosure was widely conducted in Yangtze River Estuary, Yellow River Estuary, and the nearby coasts, while lower-flat enrockment was more common in Pearl River Estuary. However, the underlying causes that led to different choices of reclamation are not clear.
Reclamation is bound to influence intertidal morphodynamics (Chen, 1990; Jiang and Feng, 1991; Wang et al., 2012; Lu and Jiang, 2013; Wei et al., 2017). Studies have revealed that reclamation can influence the local tidal dynamics, including amplitude, asymmetry, and tidal currents (Manda and Matsuoka, 2006; Lu et al., 2009; Gao et al., 2014; Lin et al., 2015). Wave actions can be enhanced due to a reduction in intertidal width (Wang et al., 2012; Zhang et al., 2021). Besides, it has also been widely reported that profile shape, bed slope, and sediment grain size can be altered by reclamation (Wang et al., 2012; Li et al., 2016). However, since previous studies are mostly site-specific, the pattern identified in one tidal flat is not necessarily applicable to another. A systemic comparison between the two different reclamation practices is lacking. Generic insights regarding the underlying mechanisms are needed to improve our understanding of tidal flat evolution under human interventions and predict the potential effects.
One possible avenue to explore the generic impact of reclamation is applying the dynamic equilibrium theory (DET; Friedrichs, 2011). An equilibrium state is typically described as zero net sediment transport, characterized by uniform maximum bottom shear stress (Friedrichs and Aubrey, 1996). Building on this, DET reveals that equilibrium profile shape tends to be convex under tide-dominated conditions and concave under wave-dominated conditions. However, this equilibrium concept is rather idealized as tidal flats are constantly subjected to spatially and temporally changing forces. This conflict is solved by defining the equilibrium over annual and longer time scales (Friedrichs, 2011). During such a period, the actual tidal flat approximates a dynamic equilibrium in between purely tide-dominated (convex) and purely wave-dominated (concave) extremes in concert with varying hydrodynamic conditions (Hu et al., 2018). In addition, DET further considers other drivers (e.g., external sediment supply) to systematically explain the morphological evolution of tidal flats (Friedrichs, 2011; Hu et al., 2015). However, the impact of reclamation on tidal flat equilibrium is yet to be explored.
In this study, we apply a model called DET-ESTMORF (Hu et al., 2015) to investigate the response of tidal flats to reclamation. We focus on: (1) the response of tidal flat equilibrium to two types of reclamation, (2) how such response varies in different states (prograding and retreating), and (3) the potential causes that lead to different choices of reclamation in practice. To evaluate our model, we compared the modeling results with previous researches on different reclamation types. After validation, we compared the response of tidal flat equilibrium to upper-flat enclosure and lower-flat enrockment. Finally, we analyze the impacts of changing wave height (H) and suspended sediment concentration (SSC) on reclaimed tidal flats. The results can also reveal how reclaimed tidal flats respond to environmental changes and their sustainability under future threats. Overall, this study extends DET and provides a new perspective on how human activities affect tidal flat morphology.
Materials and Methods
Model Description
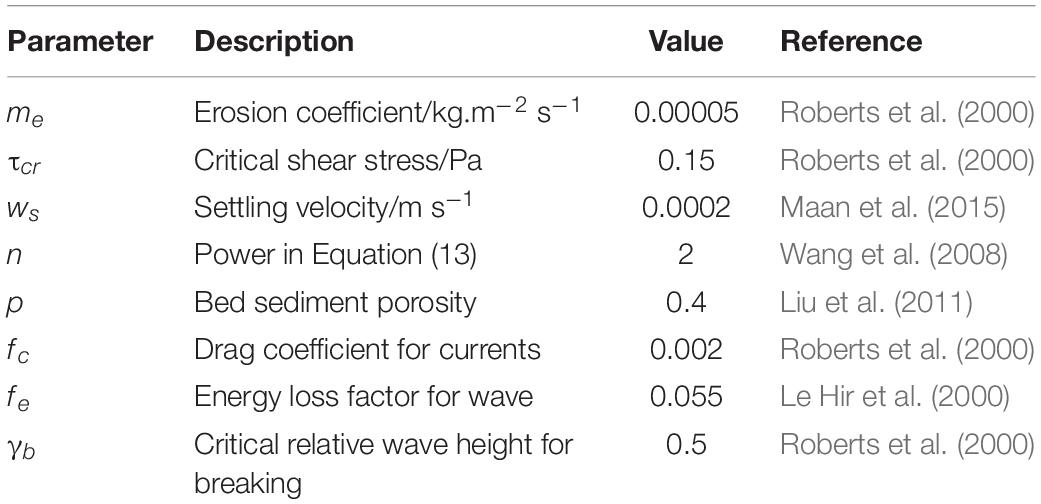
The DET-ESTMORF model is a hybrid-type morphological model that integrates morphological equilibrium relations with hydrodynamics and sediment transport (Hu et al., 2015). In this model, tidal flats in equilibrium are assumed to have uniform distributions of bed shear stress τE and sediment concentration cE across the entire profile. If the actual local shear stress τ is higher than τE, then there is a tendency of erosion (Figure 1A). It means that it demands more sediment in order to prevent erosion and stay in equilibrium. Therefore, the local equilibrium concentration ce is higher than the average level (i.e., cE). If the actual local concentration c is lower than ce, erosion occurs (Figure 1B). The case of sedimentation is similar to that. In summary, the discrepancy between τ and τE triggers the tendency of morphological changes, but whether the erosion or sedimentation will occur or not is ultimately determined by c and ce.
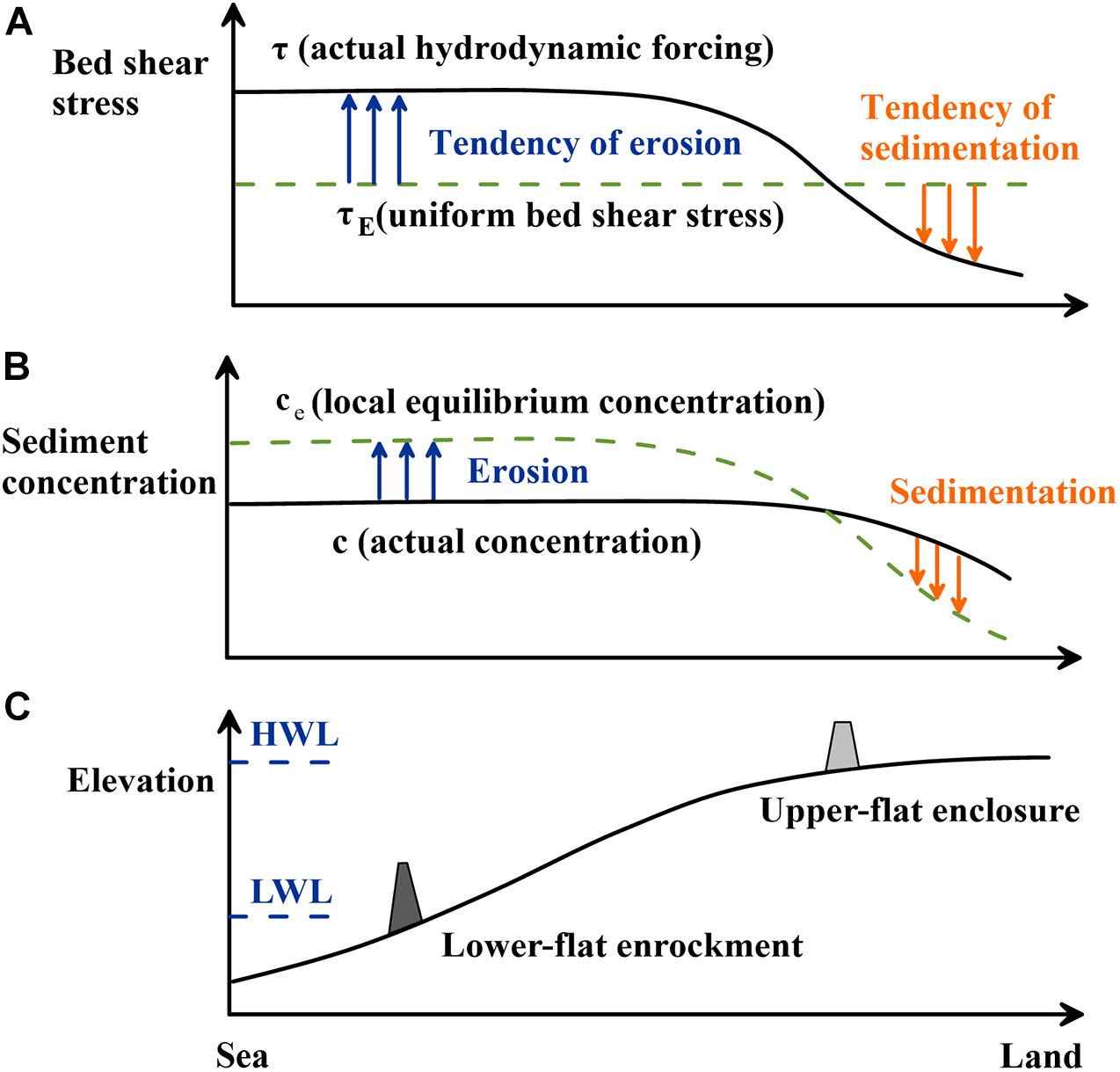
Figure 1. The schematization of the DET-ESTMORF model (modified from Hu et al., 2015) and the two kinds of reclamation. (A) Cross-shore distributions of the actual bed shear stress τ and the equilibrium shear stress τE, showing tendencies of morphological changes. (B) Cross-shore distributions of the actual concentration c and the local equilibrium concentration ce, the difference between which determines the actual morphological changes. (C) An illustration exhibiting upper-flat enclosure and lower-flat enrockment.
Our model considers the impact of both tidal current and wave motion on tidal flats. To derive the tide-induced bed shear stress τc, we use the method described in the original DET-ESTMORF:
where uc is the cross-shore tidal current velocity, ΔV is the volume of water passing through an imaginary vertical plane parallel to the shore within an interval of Δt, x is the cross-flat coordinate, t is the time, h is the water depth, B is the longshore unit width of the flat, ρ is the density of water, and fc is the drag coefficient for currents.
In the wave module, the wave height H at the seaward boundary is Rayleigh distributed with a constant mean value. To derive the cross-shore distribution of H, we solve a series of equations describing the dissipation of wave, rather than using the SWAN model as did in the previous DET-ESTMORF. The wave energy E is dissipated due to bed friction, which can be described by the continuity equation for the wave energy flux (Christoffersen and Jonsson, 1985):
in which g is the acceleration of gravity, cg is the group velocity given by the linear wave theory:
where k is the wave number. The dissipation of wave energy Dw can be evaluated as (Fredsoe and Deigaard, 1992):
fe is the energy loss factor, Uδ is the wave orbital velocity given by:
in which T is the wave period. Besides, wave breaking also plays an essential role in determining the wave height, especially in shallow waters. The maximum wave height is related to the local water depth through a constant γb. Hence,
Then the wave-induced bed shear stress τw can be computed by Callaghan et al. (2010):
which represents the temporal mean for a particular wave. The wave friction factor fw is given by Soulsby (1997):
where the wave orbital semi-excursion at the bed Aδ = Uδ T, bed roughness kb = 2πd50/12, and d50 is the median grain size of sediment particles. Thus, the bed shear stress under combined wave and current action is calculated as (Soulsby, 1995):
in which θ is the angle between directions of tidal flow and wave progradation, τm and τmax represent the mean and maximum value during a wave cycle, respectively. Tidal currents and waves prograde in the cross-shore direction in our modeling (i.e., θ = 0).
In an equilibrium state, there is an overall balance between erosion and sedimentation, which can be described as:
me is the erosion coefficient, τcr is the critical shear stress, and ws is the settling velocity. Here, cE is the SSC at the seaward boundary (see Hu et al., 2015), τE is determined by cE in each simulation via Eq. 12. The local equilibrium concentration ce in this model is defined as:
where τ90 is the 90th percentile of combined wave-current shear tress in a tidal cycle, which is chosen to represent the time-varying hydrodynamic forcing (i.e., τ in Figure 1A). The spatial and temporal distribution of sediment concentration is derived by solving a diffusion equation:
with c the sediment concentration by volume (m3/m3) and D the diffusion coefficient. Then the morphological change can be determined by:
where z is the bed elevation, and p is the bed porosity. The values of parameters and model settings are listed in Table 1 and Supplementary Table 1. Further descriptions of the model can be found in Hu et al. (2015).
Simulations of Upper-Flat Enclosure and Lower-Flat Enrockment
In our model, both types of reclamation are integrated into the bed level (Figure 1C). For upper-flat enclosure, we raise the elevation of the upper part to high water level to keep it unsubmerged. The enclosed area is actually excluded from the computational domain, reducing the intertidal width. Regarding lower-flat enrockment, we choose one point near low water level, raise its elevation and keep it unchanged. However, abrupt changes in topography usually cause fluctuations that tend to be amplified after several years of running, eventually resulting in unrealistic profiles. To avoid that and simplify calculation, we assume the enrockment only dissipates wave energy but does not affect tidal currents. This assumption can be justified by Kuang et al. (2014) and Wen and Liu (2015), which have shown that the construction of low breakwaters does not significantly change the surrounding current field. In our model, waves with large wave height are dampened on reaching the enrockment owing to the limited water depth, while tidal currents go through directly as if the enrockment did not exist there. As the model only considers fundamental processes and the way we simulate reclamation is highly simplified, our results are more general and applicable to other tidal flats. Note that the dikes in figures are not drawn based on actual model settings. The height and the width are adjusted to make the structure look natural. Detailed parameters of the reclamation can be found in Supplementary Table 1.
Results
Model Validation
Our model shows that the morphological adjustment after upper-flat enclosure can be roughly divided into three stages (Figure 2A). First, there is local accretion near the dike while the rest part remains more or less unchanged. Then the accreting tendency continues and gradually extends seawards, resulting in sedimentation across the entire profile. At this stage, the upper flat almost reaches equilibrium while the middle and lower flat continues to experience accretion. The flat becomes stable around 20 years later, with each part of the profile elevated by varying degrees. These results are in line with the morphological adjustments described in Jiang and Feng (1991) (Figure 2B). It shows the general variation pattern but does not contain actual data. Hence, we can only compare the overall shape at each stage as a qualitative validation for our model. Some detailed morphological features, such as the concave slope in t1 and the S-shaped profile in t2, are not fully reproduced by our model. Despite that, the overall similarity between the observed and the modeled morphological changes indicates a good model performance.
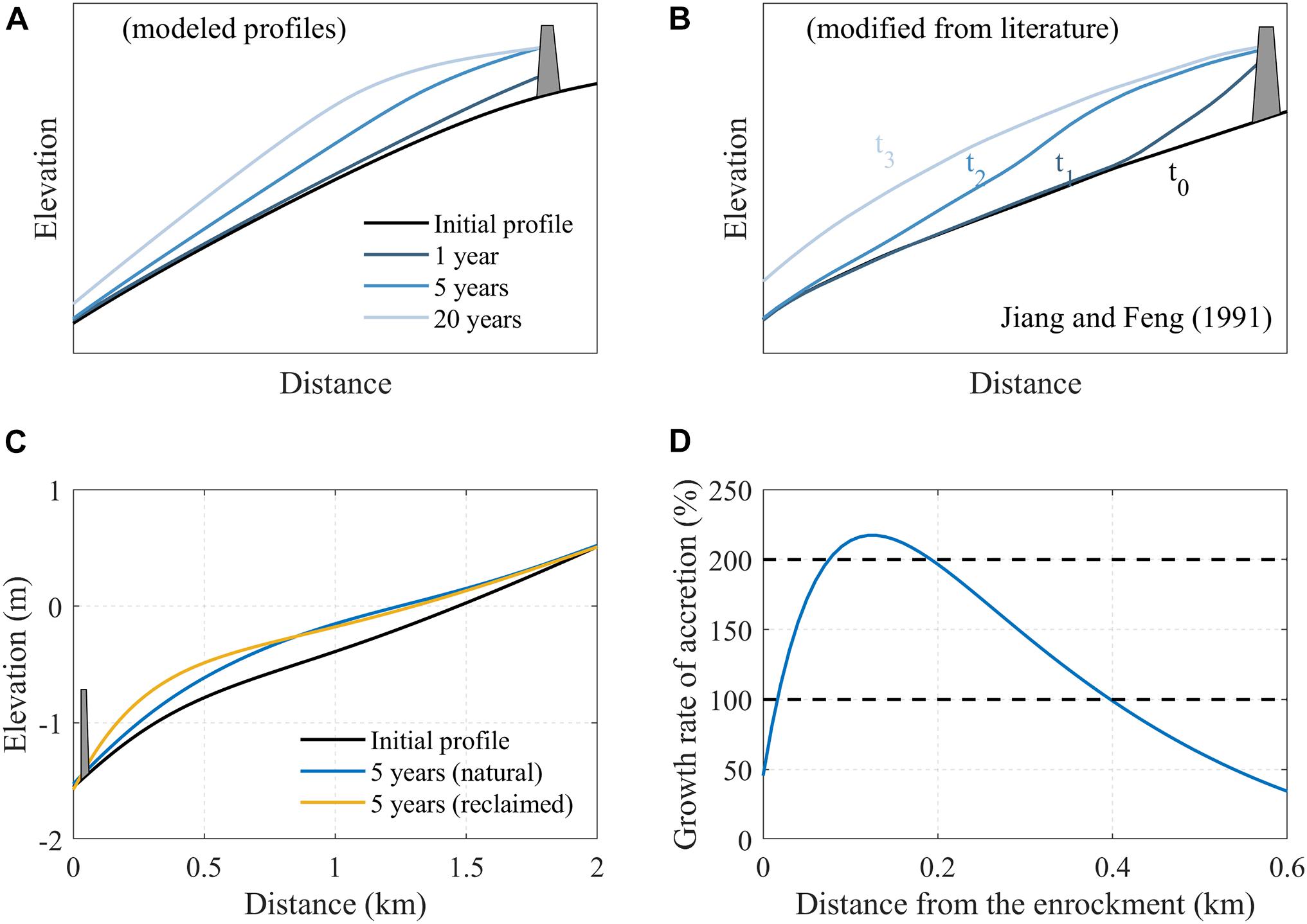
Figure 2. Comparison of model results against previous studies. (A) Simulated tidal flat profiles after enclosure, starting from a convex equilibrium profile. (B) Distinct stages (t0–t3) in the adjustment of tidal flat after enclosure, modified from Jiang and Feng (1991). (C) Simulated profiles under natural state and after enrockment. (D) The effect of enrockment in (C) on enhancing accretion rate, computed by (vr-vn)/vn where vn and vr are the vertical accretion rates on natural and reclaimed tidal flats, respectively. Note that (D) only focuses on the region near the enrockment.
As for lower-flat enrockment, we calculated the accretion rates and compared them with records in previous studies. We simulated tidal flats in the Pearl River Estuary, where enrockment has been widely employed. Model settings were chosen to correspond with the local conditions (see Supplementary Table 1). It is clear that enrockment causes additional sedimentation on the lower flat, i.e., the regional accretion rate is increased (Figure 2C). The degree of increase varies spatially (Figure 2D), which peaks at a distance of around 130 m. According to Dong (1982), the vertical accretion rate experienced an approximate 1–2 times increase within the first few years after enrockment in Pearl River Estuary (e.g., from 0.06–0.08 m/year to 0.1–0.15 m/year). This generally agrees with the modeled results (Figure 2D), which serves as a quantitative validation of our model. More importantly, the agreement with previous observation-based findings also indicates the applicability of DET in reclaimed tidal flats.
Reclamation-Induced Changes in Tidal Flat Morphodynamics
After model validation, we modeled the changes of tidal flat morphology and hydrodynamics with reclamation. The modeling starts from an equilibrium profile so that the adjustment is purely caused by reclamation. The enclosure-induced accretion concentrates on the upper flat and diminishes seaward, resulting in a much steeper profile (Figure 3A). This morphological adjustment can be explained by changes in hydrodynamics in the context of DET. First, the dike directly reduces the tidal prism and blocks the tidal currents near high water level, leading to a drop in τc90 (Figure 3C). This initiates a local imbalance between equilibrium concentration and actual concentration (i.e., ce and c), which first triggers sedimentation near the dike where the impact of enclosure is the most significant. This regional accretion has two main consequences. One is a drop in τw90 on the upper flat due to shallower water depth (Figure 3E), and another is a further decrease in tidal current (but with a smaller degree) in response to the steeper profile. These extend the accretion seawards and cause further morphodynamic adjustment. Twenty years later, when the flat approaches a new equilibrium, τc90 and τw90 experience a 23.3% decline and a 21.9% growth, respectively.
Figure 3. Response of tidal flat morphodynamics to two kinds of reclamation. (A,B) The tidal flat morphological evolution induced by reclamation. (C,D) The distribution of 90th percentile tide-induced shear stress (τc90). (E,F) The distribution of 90th percentile wave-induced shear stress (τw90). The values in the last four panels are averaged over a spring-neap cycle (i.e., 14 tidal cycles) to eliminate the effect of varying water levels on hydrodynamics. The panels on the left side are cases of upper-flat enclosure, whereas those on the right side are for lower-flat enrockment.
Regarding lower-flat enrockment, rapid accretion is also observed near the dike at the early stage, while slight erosion occurs on the upper flat (Figure 3B). Then the accretion gradually moves landward before the flat finally stabilizes in a more convex shape. Again, the underlying mechanism can be described using DET. First, the enrockment largely attenuates the wave energy exerted on the lower flat, leading to a significant drop in τw90 (Figure 3F). Since we assume that the enrockment does not affect tidal current, the decrease in wave forcing means smaller combined shear stress. Like the case of enclosure, the weakened hydrodynamic forcing triggers an imbalance between local ce and c, which results in regional accretion. As more sediments are settling on the lower flat, the supply to the upper flat is marginally reduced, which accounts for the slight erosion there. Meanwhile, the increased convexity on the lower flat leads to a growth in τc90 (Figure 3D). This somewhat compensates for the decrease in τw90 and reduces the tendency of accretion. After 20 years, the flat approaches a new equilibrium in which τc90 and τw90 are 28.0% larger and 18.5% smaller, respectively.
Responses of Natural and Reclaimed Flats to Changing Wave Height and Sediment Supply
Subsequently, we consider the situations where the natural evolution of tidal flats and reclamation-induced adjustment coexist. This is because tidal flats that stay in equilibrium are rather exceptional, and they tend to be prograding or retreating depending on the actual environmental conditions. Here, we change the wave height and SSC to initiate progradation or retreat on an equilibrium profile (Figure 4A and Supplementary Table 1). When further considering the two types of reclamation, we get four different situations (Figures 4B–E). On the prograding flat in Figure 4B, the enclosure promotes the average accretion by 68.0%, most of which occurs on the upper flat. This compensates for the relatively weaker progradation near high water level on the original natural flat and recreates a convex profile similar to the initial profile. Likewise, enrockment on the prograding flat enhances accretion, but the effect is not as strong as enclosure. In Figure 4C, the mean elevation is only increased by 36.7%, with much of the accretion concentrated on the lower flat.
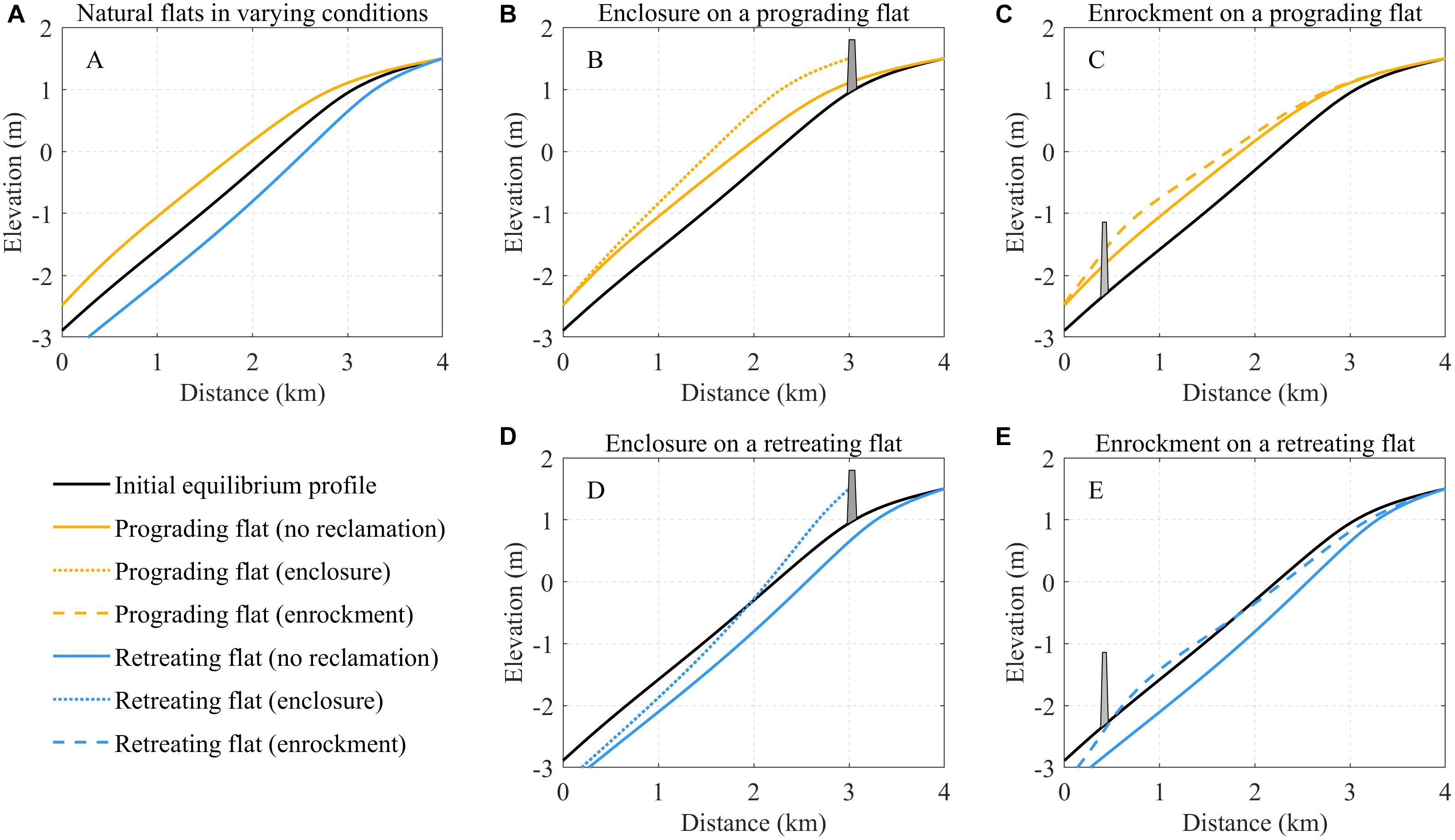
Figure 4. Tidal flat profiles under various situations. (A) Natural profiles in progradation, equilibrium, and retreat. (B,C) Reclamation on a prograding flat. (D,E) Reclamation on a retreating flat. The initial equilibrium profile is the same as in Figure 3.
When it comes to retreating flats, both enclosure and enrockment still maintain their ability to promote accretion, which mitigates the environment-driven erosion (85.4 and 95.7% in Figures 4D,E, respectively). Despite their similar overall effect, the resulting profiles are quite different due to the distribution of accretion. On a retreating natural flat, more sediment is eroded from the lower flat, increasing the profile slope (Figure 4A). This tendency is intensified by enclosure which induces more sediment deposited on the upper flat than the lower flat, resulting in a much steeper profile (Figure 4D). By contrast, the retreating tendency is almost counteracted by enrockment, which traps more sediment on the lower flat and sustains the original shape to a large extent (Figure 4E).
Besides the four representative situations, we have also run multiple cases with wave height and sediment supply changing in certain ranges to see a bigger picture. As shown in Figure 5A, the elevation change rate is proportional to SSC and inversely related to H, meaning that a higher sediment concentration or a smaller wave height favors progradation (the top left region) while the opposite situation leads to retreat (the bottom right region). This correlation does not essentially change when reclamation is considered. However, the elevation change rates on reclaimed flats are generally larger than on natural flats under the same condition, suggesting that reclamation has a universal effect in promoting accretion. Of all the cases we simulated, the accretion rate is raised by 1.8 and 1.2 cm/year on average under upper-flat enclosure and lower-flat enrockment, respectively.
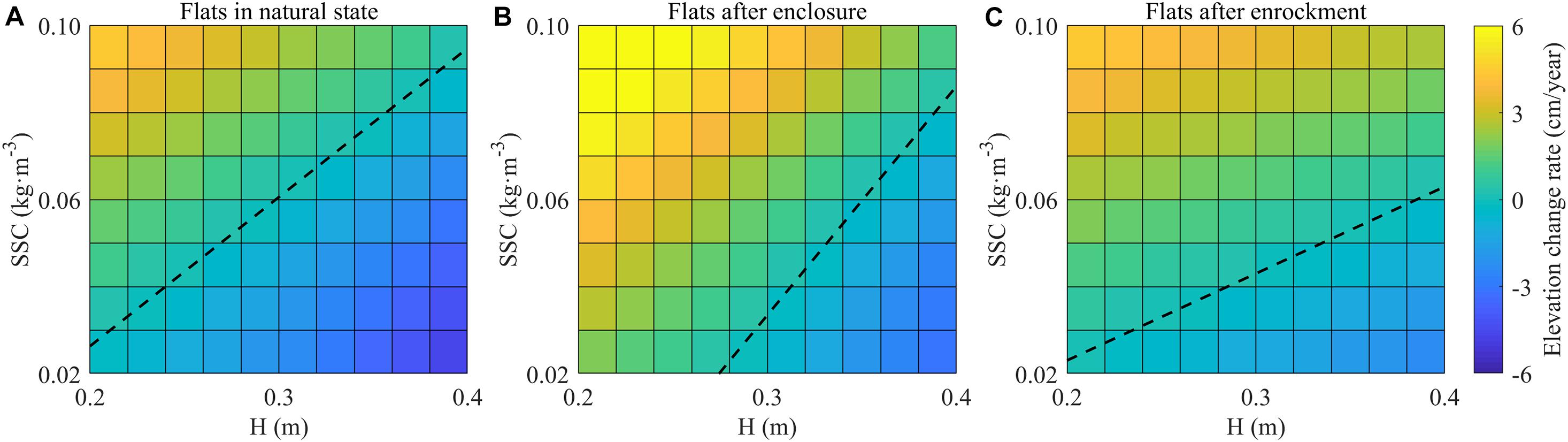
Figure 5. Mean elevation change rate on tidal flats under various conditions. (A) Natural tidal flats. (B) Tidal flats after enclosure. (C) Tidal flats after enrockment. All simulations start from the same initial equilibrium profile as in Figure 3, and the time span is 20 years. The wave climate and sediment supply are varied to simulate tidal flats that are not in equilibrium. The wave height ranges from 0.2 to 0.4 m along the X-axis and SSC from 0.02 to 0.10 kg m–3 along the Y-axis. The color shows how fast the mean elevation changes, with positive and negative values representing progradation and retreat, respectively. The dashed black lines indicate cases with elevation change rates close to zero.
For reclaimed flats with zero net change in elevation (Figures 5B,C), the mean wave height is larger and the mean SSC is smaller than that in natural flat (Figure 5A). This indicates that the reclaimed flat can sustain the elevation under the environment with stronger wave forcing and less sediment supply, which agrees with the above conclusion that reclamation can promote accretion and mitigate erosion. Another point worth noticing is the change in gradient. The line in Figure 5B has a larger gradient than that in Figure 5A. This suggests that enclosed flats demand a higher increase of sediment supply than natural flats to neutralize the erosive effect of an increase in wave height. This is better illustrated by how much the mean elevation changes with wave height under a certain SSC. For example, when SSC = 0.06 kg m–3, a 1 cm increase in wave height leads to an average 5.3 cm thick of sediment eroded from natural flats (Figure 5A), whereas the effect is enhanced to 6.8 cm after enclosure (Figure 5B). Therefore, the enclosed flats are more susceptible to the change in wave climate. However, for flats after enrockment, the opposite is the case. The line in Figure 5C gets smaller in gradient than in Figure 5A, indicating the change in wave height has less impact on intertidal morphology. For the same situation of SSC = 0.06 kg m–3, there is only an average 2.5 cm erosion in response to wave height increase per centimeter. Hence, in this case, the flat has become less sensitive to change in wave height.
Discussion
Impact of Reclamation on Tidal Flat Equilibrium
Intertidal response to reclamation and the underlying mechanism have been investigated by analyzing hydrodynamic and sedimentological processes (Chen, 1990; Jiang and Feng, 1991; Wang et al., 2012; Li et al., 2016). Here, we provide a new perspective by using a model embedded with DET. We show that tidal flat equilibrium is broken by reclamation by weakening the local tidal or wave forcing. The drop in hydrodynamic forcing means a lower equilibrium concentration (i.e., it needs less SSC to stay in equilibrium, see Eq. 13), while the actual concentration is still at the same level before reclamation. This surplus in suspended sediment causes accretion (Figure 1B), breaking the previous balance further. Consequently, the reclamation triggers a chain reaction of tidal flat morphodynamics, which weakens the effect of reclamation until reaching a new equilibrium.
The equilibrium state is typically described as tide-dominated, wave-dominated, or somewhere in between. It is mainly controlled by hydrodynamic forcing (τc and τw) and reflected by profile shape (convex or concave). From section “Reclamation-Induced Changes in Tidal Flat Morphodynamics,” we find that enclosure reduces tidal forcing while increases wave forcing. In addition, the reclaimed profile is less convex than before. The changes in morphology and hydrodynamic forces imply that the tidal flat is moving toward the direction of wave dominance. On the contrary, lower-flat enrockment causes morphodynamic adjustment toward tidal dominance. Note that reclamation only moves the equilibrium toward either of the two directions. It does not necessarily mean that tidal flats will become fully wave-dominated after enclosure or tide-dominated after enrockment. The tidal flat equilibrium is still primarily determined by the natural conditions (e.g., the tidal range, wave height, and sediment supply).
Although this conclusion is drawn by analyzing reclamation on tidal flats in equilibrium, it also holds for flats that have not reached a stable equilibrium. For flats with enclosure, whether they be prograding or retreating, it has been shown that the change in wave height has a larger impact on the bed level (see section “Responses of Natural and Reclaimed Flats to Changing Wave Height and Sediment Supply”). This increase in susceptibility to wave climate indicates the increasing significance of wave in determining intertidal morphology. For flats with enrockment, the reduced sensitivity to wave height change suggests that tidal current may become more dominant.
The extent to which the equilibrium is changed by reclamation depends on many factors, including the height of dikes and the relative location. Lu and Jiang (2013) reported the impact of sequential reclamation on eastern Chongming Island, which shows different accretion rates for different reclamation projects, possibly related to the elevation of enclosure. Vona et al. (2020) pointed out that the sediment accumulation induced by breakwater is inversely proportional to its distance to the shoreline.
Impact of Reclamation on Prograding and Retreating Flats
When tidal flats are not in equilibrium, the natural evolution and the reclamation-induced adjustment are coupled together. On prograding flats, the enclosure accelerates the natural accretion, which has been established in previous studies at different sites (Chen, 1990; Lu and Jiang, 2013; Li et al., 2016). In this case, upper-flat enclosure is desirable because it brings land resources while maintaining the sustainability of tidal flats. However, when the environment favors retreat (e.g., decreasing sediment supply), it is not sustainable to enclose tidal flats. Although the enclosure can cause accretion to a certain extent, the resulting profile is much steeper and slightly concave (see Figure 4D). This change has been observed in Yang et al. (2011). They compared the profiles on the eastern Chongming Island in the Yangtze River Estuary between 2006 and 2010. They found accretion on the upper flat and erosion on the lower flat, which is ascribable to the sequential reclamation (Lu and Jiang, 2013) and a decline in sediment load (Yang et al., 2011; Dai and Liu, 2013; Dai et al., 2016). Additionally, the enclosure directly reduces intertidal width, which cannot be offset by expanding the tidal flat seawards due to the erosion-favored environment. The narrower tidal flat is less effective in mitigating coastal erosion and storm impacts (van Loon-Steensma et al., 2014; Zhang et al., 2021). Moreover, the dikes and the steepened profiles block the inland migration of vegetation, reducing the capability of coastal ecosystems in adapting to rising sea levels (Torio and Chmura, 2013).
As lower-flat enrockment enhances accretion by dampening wave energy, the degree of the impact depends on the dominance of wave forcing relative to other environmental factors in shaping intertidal morphology. For example, on prograding flats in which wave is less dominant than tidal forcing or sediment supply, the impact of enrockment is less significant. However, on retreating flats, the enrockment can markedly reduce wave erosion and weaken the retreating tendency. Therefore, this method can be applied to protect coasts and restore tidal flats (acting as breakwaters). Kirby (2000) conceptualized a method called tidal flat regeneration, which involves simultaneous placing breakwaters offshore and nourishing the tidal flats to create a new convex equilibrium shape. This approach is proved to be viable, according to our results (Figures 4, 5).
Upper-Flat Enclosure or Lower-Flat Enrockment
In the very early stage of reclamation when tidal flats remain largely unexploited, the most convenient and effective way to reclaim is to build dikes on the upper flat. On coasts with wide tidal flats, upper-flat enclosure is the most widely used approach. As the scale of reclamation expands, tidal flats need to prograde fast enough to ensure space for new enclosures. When the tidal flat is relatively small or no more upper flat is available, reclamation is deployed on middle and even lower flats. However, the low elevation, frequent inundation, and soft surface sediment induce considerable difficulties for enclosure. Therefore, lower-flat enrockment is employed to increase the bed level (Chen et al., 2000).
The intertidal width is mainly dependent on the local environmental conditions. Observations have suggested that tidal flats become wider and more convex-up with increased tidal range (Kirby, 2000; Bearman et al., 2010), which means larger upper-flat area. Numerical models have revealed a positive correlation between the width and sediment supply as well (Roberts et al., 2000; Hu et al., 2015). These two factors can account for the large-scale tidal flat in Jiangsu, China. The tidal range there is around 3.9–5.5 m on average, and the coasts receive abundant sediment supply from nearby regions (Wang et al., 2012). This accretion-favored environment creates expansive land for enclosure. In addition, our results have shown that enclosure on prograding flats can enhance accretion, which in turn provides more land available for new enclosures. As a result of natural progradation and reclamation, the current coastline in northern Jiangsu is 50 km away from the Fangong dike, which was once constructed near the mean high tide level (Chen et al., 2000).
Contrastingly, the Pearl River Estuary is characterized by small tidal ranges (1.0–1.7 m on average, Mao et al., 2004). In addition, the tidal flats receive relatively less sediment supply from the river. Under such conditions, tidal flats are just several kilometers wide and prograde at a low pace. Initially, reclamation was carried out on the upper flat. As the population grew due to several waves of human migration over history, people began exploring and developing techniques to utilize the lower flat to tackle land shortage (Chen et al., 2000; Xiong et al., 2020). Consequently, the region transitioned from reclamation on the existing upper flat to the newly formed lower flat (Chen et al., 2000). Hence, the local sediment supply and tidal range are the potential causes leading to the choice of reclamation practice.
Besides, these factors are also closely related to the reclamation-induced impacts on tidal flat ecosystems. Our results have pointed out that wave climate and sediment supply will influence the overall impact of reclamation (at least in terms of morphology). Thus, projection of future environmental changes is needed to evaluate the long-term impact of reclamation. For example, if sediment supply is continuously decreasing (which is the case on many coasts nowadays, Syvitski et al., 2005; Murray et al., 2019), the impact of enclosure that was previously advantageous for progradation will be weakened and even become damaging to the sustainability of tidal flats.
Conclusion
In this study, the response of tidal flat equilibrium to reclamation is investigated using DET-ESTMORF. Reclamation affects intertidal morphology by first reducing the bed shear stress from either waves or tidal currents. This breaks the initial local balance between erosion and sedimentation and leads to a change in tidal flat equilibrium. Specifically, upper-flat enclosure drives tidal flats into evolution toward wave dominance, whereas lower-flat enrockment causes adjustments toward tidal dominance.
Furthermore, we showed that both enclosure and enrockment induce accretion to a certain extent despite the varying wave climate and sediment supply, with the accretion rate raised by 1.8 and 1.2 cm/year on average in our results. The discrepancy mainly lies with the profile shape, especially on retreating flats. By steepening the profile and reducing the width, upper-flat enclosure increases the vulnerability of tidal flats to coastal erosion and climate change. In contrast, lower-flat enrockment can reduce wave erosion and help sustain the profile under the retreat-favored environment, which is beneficial for the sustainability of tidal flats.
We also identified potential factors that may account for the different choices of reclamation practice. By affecting the intertidal width and progradation rate, tidal range and sediment supply are the underlying causes that determine the reclamation type in a region. In addition, the impact of reclamation is also profoundly affected by environmental factors. This warrants consideration of future environmental changes in devising reclamation schemes and estimating the benefits and risks.
This study extends DET with the consideration of reclamation. It provides a new perspective to understand the general pattern underlying the variety of tidal flat adjustment, highlighting the universality of DET in predicting intertidal morphodynamics. It is noted that our results only describe the general evolution of tidal flats driven by a limited number of physical processes. The impact of reclamation is much more complex since many factors influence the intertidal morphodynamics in different spatial and temporal scales (e.g., hydrodynamic conditions, biological processes, and reclamation area). However, our results may inspire more studies on reclamation from different aspects and the new application of DET.
Data Availability Statement
The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.
Author Contributions
ZZ: formal analysis, software, and writing – original draft. ZH: conceptualization, supervision, funding acquisition, project administration, and writing – review and editing. Both authors contributed to the article and approved the submitted version.
Funding
This work was supported by the Joint Research Project of NSFC (No. 51761135022), NWO (ALWSD.2016.026), and EPSRC (EP/R024537/1), Guangdong Provincial Department of Science and Technology (Grant No. 2019ZT08G090), Fundamental Research Funds for the Central Universities of China (20lgzd16), and Innovation Group Project of Southern Marine Science and Engineering Guangdong Laboratory (Zhuhai) (No. 311021004).
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Acknowledgments
The authors would like to thank Haixian Xiong for useful discussions on the reclamation practices in Pearl River Delta.
Supplementary Material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fmars.2021.769077/full#supplementary-material
References
Bearman, J., Friedrichs, C., Jaffe, B., and Foxgrover, A. (2010). Spatial trends in tidal flat shape and associated environmental parameters in South San Francisco Bay. J. Coast. Res. 26, 342–349. doi: 10.2112/08-1094.1
Callaghan, D. P., Bouma, T. J., Klaassen, P., van der Wal, D., Stive, M. J. F., and Herman, P. M. J. (2010). Hydrodynamic forcing on salt-marsh development: distinguishing the relative importance of waves and tidal flows. Estuar. Coast. Shelf Sci. 89, 73–88. doi: 10.1016/j.ecss.2010.05.013
Chen, C. (1990). Changes in mud flat after tideland being enclosed (in Chinese with English abstract). Mar. Sci. Bull. 9, 69–74.
Chen, J., Dai, Z., Li, K., Liu, J., Zhou, J., Xu, H., et al. (2000). Coastal Reclamation in China (in Chinese). Beijing: China Water & Power Press.
Christoffersen, J. B., and Jonsson, I. G. (1985). Bed friction and dissipation in a combined current and wave motion. Ocean Eng. 12, 387–423. doi: 10.1016/0029-8018(85)90002-2
Dai, Z., Fagherazzi, S., Mei, X., and Gao, J. (2016). Decline in suspended sediment concentration delivered by the Changjiang (Yangtze) River into the East China Sea between 1956 and 2013. Geomorphology 268, 123–132. doi: 10.1016/j.geomorph.2016.06.009
Dai, Z., and Liu, J. T. (2013). Impacts of large dams on downstream fluvial sedimentation: an example of the three Gorges Dam (TGD) on the Changjiang (Yangtze River). J. Hydrol. 480, 10–18. doi: 10.1016/j.jhydrol.2012.12.003
Dong, Z. (1982). Experience of reclamation on tidal flats in the Pearl River Estuary (in Chinese). Pearl River 3, 2–11.
Doody, J. P. (2004). ‘Coastal squeeze’ – an historical perspective. J. Coast. Conserv. 10, 129–138. doi: 10.1652/1400-0350(2004)010[0129:CSAHP]2.0.CO;2
Fredsoe, J., and Deigaard, R. (1992). Advanced Series on Ocean Engineering. Tuck Link: World Scientific.
Friedrichs, C. T. (2011). “Tidal flat morphodynamics: a synthesis,” in Treatise on Estuarine and Coastal Science, eds E. Wolanski and D. McLusky (Waltham, MA: Academic Press), 137–170. doi: 10.1016/B978-0-12-374711-2.00307-7
Friedrichs, C. T., and Aubrey, D. G. (1996). “Uniform bottom shear stress and equilibrium hypsometry of intertidal flats,” in Coastal and Estuarine Studies, ed. C. Pattiaratchi (Washington, D. C.: AGU), 405–429. doi: 10.1029/CE050p0405
Gao, G., Wang, X., and Bao, X. (2014). Land reclamation and its impact on tidal dynamics in Jiaozhou Bay, Qingdao, China. Estuar. Coast. Shelf Sci. 151, 285–294. doi: 10.1016/j.ecss.2014.07.017
Hoeksema, R. J. (2007). Three stages in the history of land reclamation in the Netherlands. Irrig. Drain. 56, S113–S126. doi: 10.1002/ird.340
Hu, Z., van der Wal, D., Cai, H., van Belzen, J., and Bouma, T. J. (2018). Dynamic equilibrium behaviour observed on two contrasting tidal flats from daily monitoring of bed-level changes. Geomorphology 311, 114–126. doi: 10.1016/j.geomorph.2018.03.025
Hu, Z., Wang, Z., Zitman, T. J., Stive, M. J. F., and Bouma, T. J. (2015). Predicting long-term and short-term tidal flat morphodynamics using a dynamic equilibrium theory. J. Geophys. Res. 120, 1803–1823. doi: 10.1002/2015JF003486
Jiang, G., and Feng, H. (1991). “The effect of dike on development of tidal flat profiles (in Chinese with English abstract)” Proceedings of the Fourth Chinese Oceanological and Limnological Science Conference, ed. the Chinese Society of Oceanology and Limnology (Beijing: Science Press), 26–33.
Kennish, M. J. (2001). Coastal salt marsh systems in the U.S.: a review of anthropogenic impacts. J. Coast. Res. 17, 731–748.
Kirby, R. (2000). Practical implications of tidal flat shape. Cont. Shelf Res. 20, 1061–1077. doi: 10.1016/s0278-4343(00)00012-1
Kirwan, M. L., and Megonigal, J. P. (2013). Tidal wetland stability in the face of human impacts and sea-level rise. Nature 504, 53–60. doi: 10.1038/nature12856
Kuang, C., He, L., Gu, J., Pan, Y., Zhang, Y., Yang, Y., et al. (2014). Effects of submerged breakwater on hydrodynamics and shoreline change of the East beach of beidaihe, Bohai Bay, China. J. Coast. Res. 30, 598–614. doi: 10.2112/JCOASTRES-D-13-00173.1
Le Hir, P., Roberts, W., and Cazaillet, O. (2000). Characterization of intertidal flat hydrodynamics. Cont. Shelf Res 20, 1433–1459. doi: 10.1016/s0278-4343(00)00031-5
Li, M., Yang, L., Gong, X., Xue, W., Yang, Y., Shi, Y., et al. (2016). The geomorphologic response of accreting intertidal flat to reclamation: a case from Jianggang, Jiangsu (in Chinese with English abstract). Mar. Sci. Bull. 35, 683–693. doi: 10.11840/j.issn.1001-6392.2016.06.011
Lin, L., Liu, Z., Xie, L., Gao, H., Cai, Z., Chen, Z., et al. (2015). Dynamics governing the response of tidal current along the mouth of Jiaozhou Bay to land reclamation. J. Geophys. Res. 120, 2958–2972. doi: 10.1002/2014JC010434
Liu, X., Gao, S., and Wang, Y. (2011). Modeling profile shape evolution for accreting tidal flats composed of mud and sand: a case study of the central Jiangsu coast, China. Cont. Shelf Res. 31, 1750–1760. doi: 10.1016/j.csr.2011.08.002
Lu, B., and Jiang, X. (2013). Reclamation impacts on the evolution of the tidal flat at Chongming Eastern Beach in Changjiang estuary (in Chinese with English version). J. Remote Sens. 17, 335–349.
Lu, Y., Ji, R., and Zuo, L. (2009). Morphodynamic responses to the deep water harbor development in the Caofeidian sea area, China’s Bohai Bay. Coast. Eng. 56, 831–843. doi: 10.1016/j.coastaleng.2009.02.005
Ma, Z. J., Melville, D. S., Liu, J. G., Chen, Y., Yang, H. Y., Ren, W. W., et al. (2014). Rethinking China’s new great wall. Science 346, 912–914. doi: 10.1126/science.1257258
Maan, D. C., van Prooijen, B. C., Wang, Z., and De Vriend, H. J. (2015). Do intertidal flats ever reach equilibrium? J. Geophys. Res. 120, 2406–2436. doi: 10.1002/2014JF003311
Manda, A., and Matsuoka, K. (2006). Changes in tidal currents in the ariake sound due to reclamation. Estuaries Coast. 29, 645–652. doi: 10.1007/BF02784289
Mao, Q., Shi, P., Yin, K., Gan, J., and Qi, Y. (2004). Tides and tidal currents in the Pearl River Estuary. Cont. Shelf Res. 24, 1797–1808. doi: 10.1016/j.csr.2004.06.008
Murray, N. J., Phinn, S. R., DeWitt, M., Ferrari, R., Johnston, R., Lyons, M. B., et al. (2019). The global distribution and trajectory of tidal flats. Nature 565, 222–225. doi: 10.1038/s41586-018-0805-8
Nadzir, N. M., Ibrahim, M., and Mansor, M. (2014). Impacts of coastal reclamation to the quality of life: Tanjung Tokong community, Penang. Proc. Soc. Behav. Sci. 153, 159–168. doi: 10.1016/j.sbspro.2014.10.050
Pendleton, L., Donato, D. C., Murray, B. C., Crooks, S., Jenkins, W. A., Sifleet, S., et al. (2012). Estimating global “blue carbon” emissions from conversion and degradation of vegetated coastal ecosystems. PLoS One. 7:e43542. doi: 10.1371/journal.pone.0043542
Roberts, W., Le Hir, P., and Whithouse, R. J. S. (2000). Investigation using simple mathematical models of the effect of tidal currents and waves on the profile shape of intertidal mudflats. Cont. Shelf Res. 20, 1079–1097. doi: 10.1016/s0278-4343(00)00013-3
Soulsby, R. L. (1995). “Bed shear-stresses due to combined waves and currents,” in Advances in Coastal Morphodynamics, eds M. J. F. Stive, H. J. De Vriend, J. Fredsoe, L. Hamm, R. L. Soulsby, C. Teisson, et al. (Delft: Delft Hydraulics), 20–24.
Soulsby, R. L. (1997). Dynamics of Marine Sands. A Manual for Practical Applications. London: Thomas Telford.
Syvitski, J. P. M., Kettner, A. J., Overeem, I., Hutton, E. W. H., Hannon, M. T., Brakenridge, G. R., et al. (2009). Sinking deltas due to human activities. Nat. Geosci. 2, 681–686. doi: 10.1038/ngeo629
Syvitski, J. P. M., Vörösmarty, C. J., Kettner, A. J., and Green, P. (2005). Impact of humans on the flux of terrestrial sediment to the global coastal ocean. Science 308, 376–380. doi: 10.1126/science.1109454
Tian, B., Wu, W. T., Yang, Z. Q., and Zhou, Y. X. (2016). Drivers, trends, and potential impacts of long-term coastal reclamation in China from 1985 to 2010. Estuar. Coast. Shelf Sci. 170, 83–90. doi: 10.1016/j.ecss.2016.01.006
Torio, D. D., and Chmura, G. L. (2013). Assessing coastal squeeze of tidal Wetlands. J. Coast. Res. 29, 1049–1061. doi: 10.2112/JCOASTRES-D-12-00162.1
van Loon-Steensma, J. M., Slim, P. A., Decuyper, M., and Hu, Z. (2014). Salt-marsh erosion and restoration in relation to flood protection on the wadden sea barrier island terschelling. J. Coast. Conserv. 18, 415–430. doi: 10.1007/s11852-014-0326-z
Vitousek, S., Barnard, P. L., Fletcher, C. H., Frazer, N., Erikson, L., and Storlazzi, C. D. (2017). Doubling of coastal flooding frequency within decades due to sea-level rise. Sci. Rep. 7:1399. doi: 10.1038/s41598-017-01362-7
Vona, I., Gray, M., and Nardin, W. (2020). The impact of submerged breakwaters on sediment distribution along marsh boundaries. Water 12:1016. doi: 10.3390/w12041016
Wang, W., Liu, H., Li, Y. Q., and Su, J. L. (2014). Development and management of land reclamation in China. Ocean Coast. Manage. 102, 415–425. doi: 10.1016/j.ocecoaman.2014.03.009
Wang, X. G., Yan, F. Q., and Su, F. Z. (2021). Changes in coastline and coastal reclamation in the three most developed areas of China, 1980–2018. Ocean Coast. Manage. 204:105542. doi: 10.1016/j.ocecoaman.2021.105542
Wang, Y., Gao, S., Jia, J., Thompson, C. E. L., Gao, J., and Yang, Y. (2012). Sediment transport over an accretional intertidal flat with influences of reclamation, Jiangsu coast, China. Mar. Geol. 291–294, 147–161. doi: 10.1016/j.margeo.2011.01.004
Wang, Z., de Vriend, H., Stive, M., and Townend, I. (2008). “On the parameter setting of semi-empirical long-term morphological models for estuaries and tidal lagoons,” in Proceedings of the 5th IAHR Symposium on River, Coastal and Estuarine Morphodynamics, eds C. M. Dohmen-Janssen and S. J. M. H. Hulscher (London: Taylor & Francis), 103–111. doi: 10.1201/noe0415453639-c14
Wei, W., Dai, Z., Mei, X., Liu, J. P., Gao, S., and Li, S. (2017). Shoal morphodynamics of the Changjiang (Yangtze) estuary: Influences from river damming, estuarine hydraulic engineering and reclamation projects. Mar. Geol. 386, 32–43. doi: 10.1016/j.margeo.2017.02.013
Wen, C., and Liu, T. (2015). “Numerical study on the effect of breakwater construction on tidal flow and sediment,” in Proceedings of the 2015 Sixth International Conference on Intelligent Systems Design and Engineering Applications (ISDEA), (Guiyang: IEEE), 538–541. doi: 10.1109/ISDEA.2015.139
Xiong, H., Zong, Y., Huang, G., and Fu, S. (2020). Human drivers accelerated the advance of Pearl River deltaic shoreline in the past 7500 years. Quat. Sci. Rev. 246:106545. doi: 10.1016/j.quascirev.2020.106545
Yang, S., Milliman, J. D., Li, P., and Xu, K. (2011). 50,000 dams later: erosion of the Yangtze River and its delta. Glob. Planet Change 75, 14–20. doi: 10.1016/j.gloplacha.2010.09.006
Young, I. R., and Ribal, A. (2019). Multiplatform evaluation of global trends in wind speed and wave height. Science 364, 548–552. doi: 10.1126/science.aav9527
Keywords: reclamation, tidal flats, dynamic equilibrium theory, tidal flat equilibrium, morphological response
Citation: Zhong Z and Hu Z (2021) The Impact of Reclamation on Tidal Flat Morphological Equilibrium. Front. Mar. Sci. 8:769077. doi: 10.3389/fmars.2021.769077
Received: 01 September 2021; Accepted: 19 October 2021;
Published: 10 November 2021.
Edited by:
Zheng Gong, Hohai University, ChinaReviewed by:
Dongdong Shao, Beijing Normal University, ChinaLiqin Zuo, Nanjing Hydraulic Research Institute, China
Copyright © 2021 Zhong and Hu. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Zhan Hu, aHV6aDlAbWFpbC5zeXN1LmVkdS5jbg==
 Zehua Zhong
Zehua Zhong Zhan Hu
Zhan Hu