Large Stimulation of Recalcitrant Dissolved Organic Carbon Degradation by Increasing Ocean Temperatures

More than 96% of organic carbon in the ocean is in the dissolved form, most of it with lifetimes of decades to millennia. Yet, we know very little about the temperature sensitivity of dissolved organic carbon (DOC) degradation in a warming ocean. Combining independent estimates from laboratory experiments, oceanographic cruises and a global ocean DOC cycling model, we assess the relationship between DOC decay constants and seawater temperatures. Our results show that the apparent activation energy of DOC decay (E_a) increases by three-fold from the labile (lifetime of days) and semi-labile (lifetime of months) to the semi-refractory (lifetime of decades) DOC pools, with only minor differences between the world's largest ocean basins. This translates into increasing temperature coefficients (Q₁₀) from 1.7–1.8 to 4–8, showing that the generalized assumption of a constant Q₁₀ of ~2 for biological rates is not universally applicable for the microbial degradation of DOC in the ocean. Therefore, rising ocean temperatures will preferentially impact the microbial degradation of the more recalcitrant and larger of the three studied pools. Assuming a uniform 1°C warming scenario throughout the ocean, our model predicts a global decrease of the DOC reservoir by 7 ± 1 Pg C. This represents a 15% reduction of the semi-labile + semi-refractory DOC pools.

Introduction

Dissolved organic carbon (DOC) in the ocean represents one of the largest reservoirs of organic matter on the Earth's surface, containing a similar amount of carbon as atmospheric carbon dioxide (CO₂) (Hedges, 2002). The main processes responsible for the removal of DOC from the ocean water column are: (1) photochemistry, where DOC is degraded directly to CO₂, carbon monoxide (CO) or low molecular weight organic compounds readily available for prokaryote uptake (Moran and Zepp, 1997; Mopper et al., 2015); (2) abiotic aggregation into microparticles (Kerner et al., 2003) or sorption to particles (Chin et al., 1998); (3) abiotic degradation via free radical reactions with oxygen (Rontani et al., 2014); and (4) degradation by marine heterotrophic prokaryotes (Lønborg and Álvarez-Salgado, 2012), with the latter representing the major DOC sink in the ocean (Carlson and Hansell, 2015).

Although the DOC pool comprises a myriad of compounds covering a widespread molecular size-reactivity continuum (Amon and Benner, 1996; Benner and Amon, 2015), it is operationally divided into a biologically labile fraction (DOC_L) that is degraded over hours to days, a semi-labile fraction (DOC_SL) with turnover times from weeks to months, a semi-refractory fraction (DOC_SR) that can be stored for decades, a refractory fraction (DOC_R) with lifetimes of thousands of years, and an ultra-refractory fraction (DOC_UR) resistant to removal for tens of thousands of years (Hansell, 2013). Bioavailability has traditionally been linked to the chemical composition and structure of DOC, but more recently it has been shown to also vary depending on a range of other external factors (Raymond and Spencer, 2015). These factors include nutrient status, redox state, mineral-particle associations, terrestrial inputs, sun-light, biological production of recalcitrant compounds, changing microbial community composition, and the effect of priming or the extreme dilution of individual molecules (e.g., Amon and Benner, 1996; Thingstad et al., 1999; Del-Giorgio and Davies, 2003; Bianchi, 2011; Keil and Mayer, 2014; Arrieta et al., 2015; Letscher et al., 2015). About 4% of the ocean's net primary production escapes degradation in the surface layer to be exported as DOC to the ocean interior at a rate of about 2 Pg C year⁻¹ (Hansell et al., 2012), roughly equalling the annual net ocean uptake of CO₂ (Sarmiento and Gruber, 2006). Therefore, climate induced changes in DOC production, degradation, and/or export to deeper waters could potentially impact the atmospheric CO₂ concentration and the Earth climate system. Previous studies have proposed that changes in the degradation of ocean DOC over global scales could have an important role in regulating the long-term climate, with the rate and magnitude of degradation impacting atmospheric CO₂ levels, which in turn influences the cooling and warming of the Earth surface (Peltier et al., 2007; Swanson-Hysell et al., 2010; Sexton et al., 2011).

The temperature sensitivity of natural organic matter degradation has been studied extensively in soils (Davidson et al., 2000; Davidson and Janssens, 2006), demonstrating that increasing ambient temperature preferentially stimulates the microbial degradation of the more recalcitrant organic matter fractions. These studies suggest that the lability of a substrate can be defined by the change in the activation energy (E_a) needed in order for the degradation to take place; the more recalcitrant the compound, the higher its E_a and, following the Arrhenius law, the higher its temperature sensitivity to microbial degradation (Davidson et al., 2000; Davidson and Janssens, 2006; Sierra, 2012). In the marine environment, studies of the microbial activity response to temperature in sediments have shown that the E_a varies as a function of compound class and the organisms degrading the organic matter (Westrich and Berner, 1988; Middelburg et al., 1996). More recently, the Arrhenius law has been used for calculating the E_a of plankton community metabolism, suggesting that marine autotrophs and heterotrophs might react differently to ocean warming (Yvon-Durocher et al., 2012; Chen and Laws, 2017). It has also been shown that the temperature dependence of heterotrophic prokaryote production is fundamentally different between the upper and deeper layers of the ocean (Lønborg et al., 2016). Dissolved oxygen utilization rates in the ocean, computed from dissolved oxygen concentrations and apparent water age estimates, have also revealed a noteworthy temperature sensitivity of microbial respiration (Brewer and Peltzer, 2016, 2017).

Here, we quantify the E_a associated with the microbial degradation of DOC _L, DOC_SL, and DOC_SR, which partly controls the ocean prokaryotic production and oxygen consumption. To achieve this goal, we combine data from laboratory microbial cultures and latitudinal DOC concentration and water mass age gradients collected during oceanographic cruises, with the outputs from a global ocean DOC cycling model. This analysis allows us to also assess the impact of future ocean warming on the microbial degradation and size of the DOC fractions and the potential implications for the ocean carbon cycle.

Methods

Sensitivity of the DOC_L and DOC_SL decay constants to temperature, on the order of days to months, can be tested with microbial incubation experiments. However, this approach is not feasible for DOC_SR, which turns over in years to decades. In this case, calculating rates using latitudinal gradients of DOC concentrations and water ventilation times obtained during oceanographic cruises are more suitable (Carlson et al., 2010). Furthermore, models that combine ocean circulation with DOC biogeochemical cycling provide insights into the global implications of temperature dependence of DOC_SL and DOC_SR degradation.

Determination of DOC_L and DOC_SL Decay Constants from Incubation Studies

Studies of the degradation of DOC_L usually involve the dilution of a natural microbial community to follow the utilization of DOC over days in controlled laboratory conditions; when the incubation time is extended for months, the kinetics of the degradation of DOC_SL can also be followed (Lønborg and Álvarez-Salgado, 2012). While numerous studies have determined the DOC_L and DOC_SL decay constants (k_C) measuring changes in DOC concentration over time using incubation experiments (see Lønborg and Álvarez-Salgado, 2012; for recent overview) very few were conducted at different temperatures (Table 1). In order to overcome methodological differences between the few available studies, we only included data where changes in DOC concentration over time had been measured at different temperatures. These include laboratory incubations using phytoplankton-derived DOC (Seiki et al., 1991), marine humic substances (Bussmann, 1999), and DOC collected in a coastal system (Lønborg et al., 2009). In one case (Bussmann, 1999), only initial DOC was measured but the time course of dissolved oxygen was followed over time. We included this study in the analysis because of the interest on the degradation of natural humic substances. Therefore, it should be kept in mind that in this case the temperature response of DOC respiration rather than utilization (= growth + respiration) has been followed. DOC samples collected during these experiments were either left unfiltered (Bussmann, 1999) or passed through a 0.45 μm membrane (Seiki et al., 1991) or GF/F filter (Lønborg et al., 2009). All studies but one (Seiki et al., 1991), which used wet oxidation, employed high-temperature combustion to determine the DOC concentration. Note that studies inferring the temperature sensitivity of marine organic matter degradation in long-term microbial incubation experiments using oxygen consumption estimates were not included in our analysis as they either did not distinguish between the particulate and dissolved fraction and/or did not report initial DOC values (e.g., Robinson and Williams, 2005; Hansen and Bendtsen, 2014; Bendtsen et al., 2015).

TABLE 1

Table 1. Summary of values obtained applying the Arrhenius law to experimental DOC degradation.

Estimation of DOC_SR Decay Constants from Latitudinal Gradients

Field data used in this work were collected in June–July 2003 along the CLIVAR section A16N in the North Atlantic Ocean (latitudinal range: 6°S to 63°N; longitude range: 20°-29°W) as part of the US CLIVAR Repeat Hydrography program (See Figure 6 in Hansell, 2013). Hydrographic data were obtained at 73 stations spaced by about 50 km using a conductivity, temperature, and depth (CTD) profiler equipped with 36 twelve-liters Niskin bottles. More than two thousand DOC samples were collected during the cruise. Samples taken in the depth range 0–250 m were passed through an in-line polycarbonate filter cartridge holding a combusted GF/F filter attached directly to the Niskin bottle, while samples below this depth were left unfiltered.

The ventilation time of each sample, an estimate of water apparent age, was estimated from measurements of chlorofluorocarbon (CFC) concentrations as described elsewhere (Carlson et al., 2010). In brief, the water mass ventilation year was estimated by comparing the partial pressure of CFC-12 in each water sample with the annual average of CFC-12 in the atmosphere as a function of time (Bullister and Weiss, 1988; Karstensen and Tomczak, 1998). The accuracy of the CFC-12 method is not only impacted by the analytical error but also by under saturation in surface waters at the time of water masses formation and isopycnal/diapycnal mixing of more recent high-CFC with older zero-CFC water, which adds uncertainty to the ventilation times estimates. The CFC-12 method has an approximate accuracy of 1 year for the water masses with an age range between 3 to 5 years, 0.5 year in the age range 5 to 45 years and 2 years for the waters with an age between 45 and 55 years (Carlson et al., 2010).

Fitting the decline of DOC with water apparent age along isothermal surfaces provides DOC decay constants at different temperatures for the North Atlantic Ocean. Given the apparent age range of the water masses in this hydrographic section, from 3 to 55 years, the DOC_SR pool is studied in this case. For the Pacific Ocean two DOC decay estimates for the recalcitrant pools are available but they were measured at approximately equal temperatures (Hansell et al., 2012). As multiple DOC decay estimates over a range of temperatures are needed, we did not include them in our analysis.

DOC Decay Function

Organic matter consists of a wide spectrum of reactivity, each component with its own rate of degradation; therefore, continuum models, rather than those based on the separation of organic matter into distinct reactivity pools, are the most suitable to study the kinetics of organic matter degradation (e.g., Middelburg et al., 1993. However, most DOC degradation studies have been based on the first-order model developed by(Berner (1964). Following this simple model, we used the following 2–pool first-order exponential decay function to fit the DOC decay in the incubation experiments and latitudinal gradients processed in this study:

$\begin{array}{ll}DOC(t)=b_1·e^{-k_c·t}+b_2& (1)\end{array}$

Where DOC(t) is the concentration of DOC at time t, b₁ is the degraded pool (μmol kg⁻¹), k_C the decay constant (in year⁻¹), t the time (in years) and b₂ the resistant pool (μmol kg⁻¹). For the latitudinal gradients, we fitted DOC and water mass apparent ages (t) to the exponential decay function for different temperature bins (see Table S1).

Arrhenius Law

The temperature sensitivity of k_C is defined by changes in activation energy (E_a):

$\begin{array}{ll}{K}_c=A·e^{-E_a/R·T}& (2)\end{array}$

Where A is the theoretical k_C in the absence of E_a (in year⁻¹); E_a is the energy barrier to be surpassed in order for the reaction to take place (in J mol⁻¹); R is the universal gas constant (8.314 J mol⁻¹ K⁻¹); and T is the temperature in Kelvin (K). The factor $e^{-E_a/R·T}$ is proportional to the fraction of DOC molecules with kinetic energies in excess of E_a (Arrhenius, 1889). The Arrhenius equation suggests that more recalcitrant DOC requires a higher amount of energy to be degraded than labile compounds and therefore its degradation is slower. An estimate of the E_a for DOC degradation was derived from the slope of an Arrhenius plot where the ln of DOC decay constants (ln k_C) is plotted against the inverse absolute temperature (1/T) (Figure 1). The Arrhenius law assumes that DOC degradation is a well-defined enzymatic reaction with a constant E_a and that temperature is the only factor affecting the rate, while factors such as nutrient limitation are ignored. As all the experimental and in situ studies included here had nutrients at non-limiting levels this is a valid assumption (data not shown). Furthermore, as DOC consists of myriad compounds degraded by a variety of microbial populations with different physiologies (Repeta, 2015), the temperature response measured is really the sum of all processes involved and the calculated E_a should be seen as an apparent E_a (Westrich and Berner, 1988; Middelburg et al., 1996). Also as both the estimates from the laboratory experiments and latitudinal gradients were obtained using apparent E_a and apparent Arrhenius pre-exponential factors, the result of this analysis is a likely upper limit to the rate of enzymatic degradation.

FIGURE 1

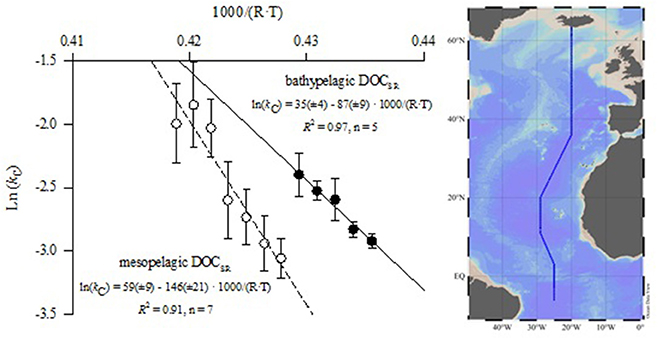
Figure 1. Arrhenius plots of the ln of DOC decay constants (ln k_C) against the inverse absolute temperature (1/T) for the meso- and bathypelagic North Atlantic Ocean along the CLIVAR A16N line (Map of cruise track is shown to the right). Solid and dashed lines represent the corresponding regression lines. Origin intercept and slope of the regression equations and their corresponding standard errors of estimation are provided; R², coefficient of determination; error bars represent standard errors. The map was createdusing Ocean Data View (Schlitzer, 2016).

T-tests were performed to test the significance of differences and Linear regression analyses were used for the Arrhenius plots applying model II regression (Sokal and Rohlf, 1995). Prior to regressions, normality was checked, and the confidence level was set at 95%.

The relationship between temperature and biological rates has been modeled in various ways (Ratkowsky et al., 1983; Ahlgren, 1987), while the factor by which a decay constant increases with a 10°C increase (Q₁₀) is mostly used. Q₁₀ values have previously been calculated using multiple functions (Sierra, 2012). In this study we assumed that the relationship between rates and temperature is exponential and we used the following function (Li and Dickie, 1987):

$\begin{array}{ll}{Q}_{10}=e^{\frac{E_a}{R}·\frac{10}{T_1·T_2}}& (3)\end{array}$

where T₁ and T₂ are the temperatures at which the DOC decay constants were measured.

Describing DOC_SL and DOC_SR Decay Using a Global Ocean DOC Cycling Model

Following the first-order decay model (Equation 1), a linear DOC cycling model that considers two DOC pools, semi-labile (DOC_SL) and semi-refractory (DOC_SR), was coupled with an ocean circulation inverse model (Primeau et al., 2013) constrained by tracer observations of temperature, salinity, sea surface height, heat, and freshwater fluxes, Δ¹⁴C-DIC, CFC-11, and phosphate that accurately represents the transport and ideal age of ocean water masses (Devries and Primeau, 2011). The horizontal resolution of the model is 2° × 2° with 24 vertical layers ranging in thickness from ~36 m near the surface to ~663 m at depth. The temperature sensitive DOC decay parameters A and E_a (Equation 2) were estimated using a Bayesian inversion procedure combined with a numerical optimization technique that finds the most probable value for each parameter conditioned on the global DOC tracer observations from Letscher and Moore (2015). The tracer conservation equations of the two DOC pools are given by:

$\begin{array}{ll}\frac{\partial {\text{DOC}}_{\text{SL}}}{\partial t}+T.{\text{DOC}}_{\text{SL}}=f_1.J_P.r_{CP}-A_1{e}^{-E_{a1}/RT}.{\text{DOC}}_{\text{SL}}& (4)\end{array}$

$\begin{array}{ll}\frac{\partial {\text{DOC}}_{\text{SR}}}{\partial t}+T.{\text{DOC}}_{\text{SR}}=f_2.J_P.r_{CP}-A_2{e}^{-E_{a2}/RT}.{\text{DOC}}_{\text{SR}}& (5)\end{array}$

where T is the 3D advection-diffusion operator of the annually resolved steady-state ocean circulation, J_p is the biological new production rate of organic matter in the euphotic zone (upper 73 m) which has been optimized using the phosphate tracer distribution and is detailed elsewhere (Primeau et al., 2013), r_CP is the ratio of carbon to phosphorus in newly produced organic matter which is assigned a modified Redfield ratio of 117:1, R is the universal gas constant and T, the temperature in degrees Kelvin, is obtained from the ocean circulation inverse model output.

The 6-tuneable parameter set [f₁ f₂ A₁ A₂ E_a1 E_a2] contains the fraction of biological organic matter production that accumulates as DOC_SL (f ₁; unit less) and DOC_SR (f ₂), the theoretical decay constants for DOC_SL (A₁; s⁻¹) and DOC_SR (A₂; s⁻¹) in the absence of an energy barrier, and the apparent activation energies (E_a1; kJ mol⁻¹) and (E_a2; kJ mol⁻¹) for DOC_SL and DOC_SR, respectively. The most probable values of the tuneable parameter set are determined by minimizing an objective function using the Nelder-Mead method (Lagarias et al., 1998), which considers the squared deviations between model-predicted and observed DOC pairs from the global dataset, with additional priors related to the acceptable ranges of each parameter, e.g., 0 ≤ f ≤ 0.5; 0 ≤ E_a ≤ 150 kJ mol⁻¹, etc. An additional constraint, that the global inventory of DOC_SR > DOC_SL (Hansell, 2013), was needed to accurately simulate the known distributions of each DOC pool. The linear Equations (4, 5) are solved by direct matrix inversion with the resulting 3D distributions of DOC_SL + DOC_SR added to the concentration of recalcitrant DOC (DOC_R), which was not simulated, and varied with ocean basin. Concentrations of DOC_R were estimated from the mean concentrations observed below 1500 m as 43.3 μmol kg⁻¹ (North Atlantic; 65°N-0°), 40.5 μmol kg⁻¹ (South Atlantic; 40°S-0°), 38.6 μmol kg⁻¹ (Pacific), 41.4 μmol kg⁻¹ (Indian), 41.7 μmol kg⁻¹ (Arctic; > 65°N), and 40.9 μmol kg⁻¹ (Southern; > 40°S).

We tested the sensitivity of the inferred DOC decay temperature parameters to alternate assumptions with respect to marine DOC production and consumption processes (Table 2, Figures 2, 3). Model I considers both DOC_SL and DOC_SR are produced biologically in the euphotic zone as separate fractions with a modified Redfield r_CP 117:1, and is represented by Equations (4, 5). Model II is based on the size-reactivity continuum concept of DOC cycling with only DOC_SL being produced biologically in the euphotic zone (with r_CP = 117:1) and DOC_SR being produced as a fraction of DOC_SL decay by heterotrophic consumption. The Model II tracer conservation equation for DOC_SR is:

$\begin{array}{l}\frac{\partial \text{DO}{\text{C}}_{\text{SR}}}{\partial t}+\text{T}.\text{DO}{\text{C}}_{\text{SR}}=f_2.(A_1{e}^{-{E_a}_1/RT}.\text{DO}{\text{C}}_{\text{SL}})\\ -A_2{e}^{-Ea2/RT}.\text{DO}{\text{C}}_{\text{SR}}& (6)\end{array}$

With f₂ being the fraction of DOC_SL decay that produces DOC_SR. The Model II tracer conservation equation for DOC_SL is equal to Equation (4). Sensitivity of the model results to variability in the r_CP of DOM production was also tested, using the spatially varying r_CP of upper ocean organic matter export inferred for twelve ocean biomes in Teng et al. (2014). The twelve ocean biomes are nominally separated by the 0.3 μM [PO₄] iso-concentration at the surface from World Ocean Atlas 2009. The spatially varying r_CP values are reported in Table S2. All cases fit the data equally well as, and neither significantly altering the conclusions drawn from, the standard case (Model I, Redfield r_CP) presented above, i.e., A₂ >> A₁; E_a2 > E_a1. The reported model parameter standard error bars were assigned by expansion of the log posterior probability function in a Taylor series about the most probable values and truncating at second order, i.e., Laplace's approximation.

TABLE 2

Table 2. Model-obtained values for the temperature sensitivity of DOC degradation.

FIGURE 2

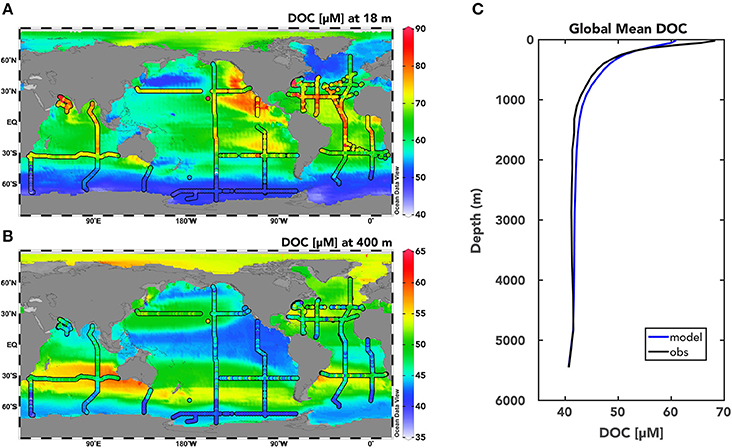
Figure 2. Modeled DOC distributions from the global ocean DOC cycling inverse model with Model I DOC cycling. Model simulated total DOC concentrations [μM] (DOC_SL+DOC_SR+DOC_R) are plotted for the euphotic zone at 18 m (A) and within the mesopelagic zone at 400 m (B) from the solution to the Model I DOC cycling case with Redfield r_CP of DOM production (see Methods for details). Observations of total DOC from the global database at each depth are shown with the colored dots. (C) Global mean simulated (blue) and observed (black) depth profile of DOC concentrations [μM].

FIGURE 3

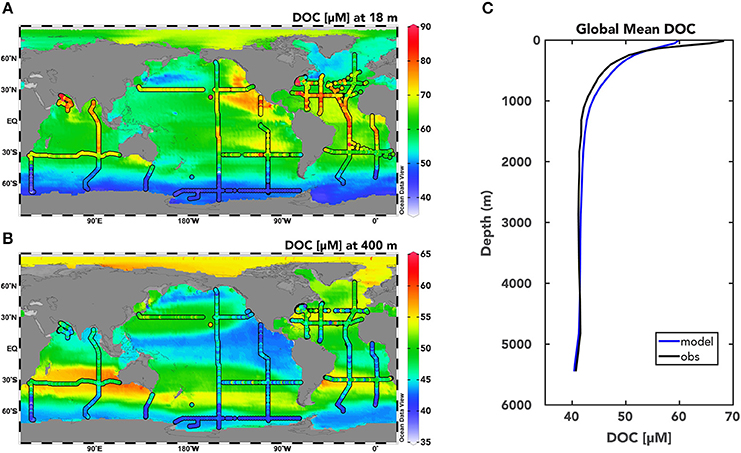
Figure 3. Modeled DOC distributions from the global ocean DOC cycling inverse model with Model II DOC cycling. Model simulated total DOC concentrations [μM] (DOC_SL+DOC_SR+DOC_R) are plotted for the euphotic zone at 18 m (A) and within the mesopelagic zone at 400 m (B) from the solution to the Model II DOC cycling case with Redfield r_CP of DOM production. Observations of total DOC from the global database at each depth are shown with the colored dots. (C) Global mean simulated (blue) and observed (black) depth profile of DOC concentrations [μM].

For all approaches used it should be remembered that we are assuming that geochemical DOC scavenging processes, such as interaction with suspended particles (Druffel et al., 1992), are negligible. As these processes are purely physical/chemical they should have low temperature sensitivity (Q₁₀ around 1; Oelkers, 1991) and the importance of these processes will therefore not be as severely impacted by changing ocean temperature as the biological processes. Furthermore, the major pool of DOC_R, which amounts to 630 ± 32 Pg C and has a half-life of thousands of years (Hansell, 2013), has been excluded from our analysis because the nature of its removal, biotic or geochemical, is still unclear.

Results

Apparent E_a values, temperature coefficients (Q₁₀), and average decay constants (k_C) of DOC degradation estimated from microbial incubation experiments conducted with phytoplankton-derived DOC, marine humic substances, and natural DOC from a coastal embayment are summarized in Table 1. Applying the Arrhenius law, we estimated apparent E_a, values varying from (average ± standard error) 35 ± 10 kJ mol⁻¹ for the DOC_L to 67 ± 11 kJ mol⁻¹ for the DOC_SL pool, while the Q₁₀ increased from 1.7 to 2.7 (Table 1). The corresponding average DOC decay constants ranged from 110 ± 0.1 × 10⁻³ day⁻¹ (half-life, ln2/k_C, 6.3 ± 0.6 days) for the DOC_L to 5 ± 1 × 10⁻³ day⁻¹ (half-life, 152 ± 18 days) for the DOC_SL (Table 1).

The Arrhenius plot of the DOC_SR decay constants, obtained from the DOC concentrations and CFC apparent water ages collected during the CLIVAR A16N cruise, clearly show two distinct data clusters for the bathy- and mesopelagic North Atlantic Ocean (Figure 1) with significantly different (t-test, p < 0.05) apparent E_a (average ± standard error) of 87 ± 9 and 146 ± 21 kJ mol⁻¹, which translate into Q₁₀ values of 3.8 ± 0.5 and 8 ± 3, respectively (Table 1). The most likely reason for this difference is that the bathypelagic North Atlantic is filled with fresher DOC during the massive water mass formation that occurs in the Arctic seas, which constitutes the engine of the meridional overturning circulation. Uncertainties in this analysis of the temperature response of DOC_SR primarily stem from the fact that the surface ocean waters ultimately ventilating the meso- and bathypelagic zones do not have identical initial DOC content; while both waters contain DOC_SR and DOC_R, they are at different concentrations and likely partially different chemical compositions. Note that for the bathypelagic ocean, b₁ (degraded pool) and b₂ (resistant pool) in Equation (1) remained constant at 5.6 ± 0.4 and 40.6 ± 0.7 μmol kg⁻¹ for all temperatures, but for the mesopelagic ocean b₁ decreased and b₂ increased with increasing temperature (Table S1). These changes are most likely explained by an increasing proportion of recalcitrant DOC in subtropical compared with subpolar mode waters, although the influence of decreasing age range with increasing temperature cannot be disregarded (Table S1). In any case, to avoid this effect, we restricted our analysis of the temperature response of the mesopelagic layer to the 8 to 11°C isotherms, where b₁ and b₂ remained constant at 11 ± 2 and 42 ± 2 μmol kg⁻¹ for all temperatures. When extrapolating this analysis, it should be remembered that our dataset is restricted to one CLIVAR section (A16N). Other cruises have not been included as they contained too few data points (2–3) and it was therefore not possible to obtain k_C over a wide temperature range, which is a prerequisite for calculating reliable E_a and Q₁₀ values.

The E_a values of DOC_SR obtained for the North Atlantic could be affected by water mass mixing because the latitudinal gradients of DOC and CFC apparent age were followed along isothermal rather than isopycnal surfaces. Note that although isopycnal surfaces are preferred to minimize the impact of mixing, it is compulsory to use isothermals when studying temperature sensitivities. Therefore, we used a global ocean DOC cycling model that accounts for ocean mixing of DOC to both constrain the validity of our approach (i.e., following gradients along isothermals) and provide estimates of the global impact. The most probable values for the apparent E_a conditioned on the global database of DOC observations were found to be 53.1 ± 1.3 kJ mol⁻¹ (average ± standard error) for DOC_SL and 101.3 ± 31.5 kJ mol⁻¹ for DOC_SR (Table 2). Corresponding Q₁₀ values using a reference temperature of 15°C yield a value of 2.2 ± 0.1 for the DOC_SL pool and 4.4 ± 2.1 for DOC_SR. These estimates are all within the ranges obtained from the incubation studies and the latitudinal gradients of DOC in the north Atlantic (Tables 1, 2). This good agreement between the model and experimental data, shows that the water mass mixing on the latitudinal DOC gradients did not significantly affect the estimate of DOC_SR decay constants, and secondly that our results are generally valid.

The most probable basin-specific apparent E_a values were also tested for the Atlantic and Pacific basin, yielding Atlantic values of 55.2 ± 2.1 kJ mol⁻¹ (Q₁₀ = 2.3 ± 0.1) and 86.2 ± 13.4 kJ mol⁻¹ (Q₁₀ = 3.1 ± 0.7) and Pacific values of 54.6 ± 1.8 kJ mol⁻¹ (Q₁₀ = 2.2 ± 0.1) and 92.7 ± 52.0 kJ mol⁻¹ (Q₁₀ = 3.9 ± 3.3) for the DOC_SL and DOC_SR pools, respectively (data not shown). Thus, apparent E_a (Q₁₀) values for the DOC_SL pool are statistically the same between the Atlantic and Pacific basins. More data are required to reduce the uncertainties in the inferred basin specific E_a for the DOC_SR pool in order to address the possibility of differing temperature sensitivities between ocean basins.

Using our estimates, we calculated the sensitivity of the size of the global ocean DOC reservoir per 1°C of equilibrium ocean warming, by comparing the modern ocean reservoir, computed with the most probable parameter values to the DOC cycling model, with that assessed by uniformly increasing the ocean temperature by 1°C. The modern ocean is found to hold 57.0 Pg C of DOC_SL + DOC_SR and 630.0 Pg C of DOC_R decreasing to 48.5 Pg C of DOC_SL + DOC_SR for a 1°C warming (Table 2; Model I, Redfield r_CP case). Therefore, in terms of size changes of the DOC reservoir for a 1°C warming scenario, our DOC cycling model predicts a decrease by 7 ± 1 Pg C (mean and SD of the four modeled DOC cycling sensitivity tests), i.e., ~15% of the DOC_SL + DOC_SR reservoirs (Table 2). In these calculations, we assume for simplicity a uniform warming throughout the ocean, which approximates the sensitivity of the ocean DOC reservoir to an equilibrium warming of the global ocean by 1°C. We ignore regional differences in warming and that most heat is absorbed by the surface and then slowly redistributed throughout the ocean interior that defines the transient response of the ocean to Earth surface warming (Drijfhout et al., 2014). The impact of changing ocean temperatures on DOC degradation will therefore vary, and would for the deep ocean be delayed relative to both the initial warming and the subsequent uptake of CO₂ and heat. Given the current observed rate of deep ocean (>1,000 m) warming of ~0.01°C per decade (Purkey and Johnson, 2010), the deep ocean would warm by 1°C from present in ~1 millennium, i.e., the 7 ± 1 Pg C reduction in the global ocean DOC reservoir would occur on a similar timescale as deep ocean ventilation. The temperature coefficients, Q₁₀, at a reference temperature of 15°C (see Equation 3 in methods) are 1.7–1.8 for DOC_L, 2–3 for DOC_SL, and 4–8 for DOC_SR, indicating that DOC compounds with higher activation energies have higher temperature dependence.

Discussion

Considering that most of the 662 ± 32 Pg C of DOC in the oceans has half-life times of decades to thousands of years (Hansell, 2013), knowledge on the impact of rising ocean temperatures on the decay constants of the less bioavailable DOC pools is relevant for predicting how climate change will affect the global carbon cycle.

Our combined modeling and experimental approaches indicate that DOC_SR has an apparent E_a of ~83–146 kJ mol⁻¹, which is comparable to values found for soil (67–120 kJ mol⁻¹; Leifeld and Von Lützow, 2013) and mesopelagic ocean dissolved oxygen consumption (86.5 mol⁻¹; Brewer and Peltzer, 2016). Comparatively, DOC_L and DOC_SL have lower apparent E_a of ~35–40 and ~50–70 kJ mol⁻¹, respectively, which are comparable to values estimated for the degradation of glucose (30 kJ mol⁻¹), tannin (70 kJ mol⁻¹), labile soil organic matter (~44 kJ mol⁻¹), and values recently predicted for marine respiration (~56 kJ mol⁻¹) and mesopelagic prokaryote production (~72 kJ mol⁻¹) (Knorr et al., 2005; Davidson and Janssens, 2006; Yvon-Durocher et al., 2012; Lønborg et al., 2016). Overall this suggests that the DOC_SR pool has higher activation energies and temperature dependence than the DOC_L and DOC_SL pools. A recent study demonstrated that in soils the Q₁₀ values scales inversely with temperature, with low temperature environments having a higher Q₁₀ than warmer ones (Koven et al., 2017). In a similar way we find that DOC_SR, mostly present in the deep ocean with lower temperatures, has a higher Q₁₀ of ~3–4, while DOC_SL, with higher concentrations in warmer mesopelagic waters, has a lower Q₁₀ of ~1.8–2. DOC decay in the future ocean will also vary depending on how factors other than temperature will be impacted by global change. These factors, amongst others, include changes in ocean circulation and the supply of inorganic nutrients, UV light exposure, dissolved oxygen levels, bacterial community composition, DOC chemical structure, and/or an extreme dilution of individual organic substrates (Lønborg et al., 2009; Kattner et al., 2011; Mopper et al., 2015; Repeta, 2015). Ocean models have predicted that warming may strengthen vertical stratification, thereby suppressing upward mixing of nutrients from the deep ocean and causing longer exposure to UV-light, with possible consequences for the DOC decay. Currently we do not have sufficient data to determine how this would impact our findings of the temperature sensitivity of DOC cycling. Over the last few years it has been demonstrated that global increases in ocean temperatures will reduce the overall O₂ content (i.e., “ocean deoxygenation”; Keeling et al., 2010) both directly (e.g., decreased solubility) and indirectly (e.g., increased respiration and decreased ocean ventilation). Studies in marine sediments have found that the degradation of organic matter under hypoxic and anoxic conditions is slower and less efficient than under oxic conditions (Zehnder and Stumm, 1988; Fenchel and Finlay, 1995), but due to the lack of data on DOC cycling under different O₂ levels we are not able to conclude how this might influence the relationships found in this study. The deep ocean bacterial community composition has been shown to vary over spatial and temporal scales, which could potentially affect DOC decay rates in the deep Ocean (Delong et al., 2006). However, a major influence seems unlikely as previous studies have suggested that DOC decay is generally independent of the bacterial community composition (Sjöstedt et al., 2013). The chemical composition of DOC in the deep ocean varies spatially (e.g., Martínez-Pérez et al., 2017 and our higher diagnosed activation energy for the DOC_SR than the DOC_SL pool point to a compositional control on the temperature sensitivity, i.e., the molecule types that make up the DOC_SR pool require more energy to be consumed than DOC_SL molecules. A similar conclusion was reached by Brewer and Peltzer (2017), who showed that the activation energy of ocean oxygen consumption varies widely (60.8 to 1662.9 kJ mol⁻¹) which they suggested was partly explained by some compounds being harder to oxidize than others.

Recent studies have also suggested that the dilute nature of individual compounds that constitute the DOC pool control its persistence in the oceans (Kattner et al., 2011; Arrieta et al., 2015). At very low concentrations, molecular diffusion could limit the utilization of DOC. Since molecular diffusion of organic matter is temperature sensitive, this could influence our findings. However, the E_a for molecular diffusion (~15 kJ mol⁻¹; Oelkers, 1991) is low compared with the E_a obtained in this study. The approaches combined here are also biased by different limitations; as an example, the incubation studies do not include the effects of some environmental factors (e.g., sunlight, pressure) and they could also be impacted by so called “bottle effects,” which could lead to different conditions compared with those found in the natural environment. On the other hand, both the DOC latitudinal gradients and modeling approach do not explicitly measure the microbial degradation but infer it indirectly from subsurface spatial gradients in DOC and temperature. However, it can be assumed that these approaches assess the temperature effects on microbial consumption of DOC, as long as abiotic particle-DOC scavenging or solubilization processes are minimal (Druffel et al., 1992, 2016).

Ocean warming varies spatially and over time, partly linked with the steady heat accumulation in the deep ocean, resulting in ~30% of the ocean warming occurring below 1,000 m (Whitney et al., 2007; Meehl et al., 2011; Balmaseda et al., 2013). As the ocean will most likely experience temperature increases of a few (1–4°C) degrees Celsius over the next decades (Meehl et al., 2011), we have estimated that the decay rates of the DOC_L, DOC_SL, and DOC_SR pools will increase by 4–6, 7–12, 15–18% for 1°C of warming. Note that for an increase of 2°C, which is possible for the surface ocean layer by the end of this century, the degradation rate of the DOC_SR pool will increase by 32–40% compared with the current situation. According to the microbial carbon pump (MCP) hypothesis, stimulation of microbial respiration will lead to an increase in the production of DOC_R relative to the production of the other DOC pools, with the magnitude and impact of this stimulation depend on the sensitivity of DOC_R degradation to changing temperature (Jiao et al., 2010; Legendre et al., 2015). In terms of size changes of the DOC reservoir for a 1°C warming scenario, our DOC cycling model predicts a decrease by 7 ± 1 Pg C (~15%) of the DOC_SL + DOC_SR reservoirs. Assuming a warming rate equal to the current sea surface increase of 0.01°C year⁻¹ (Rhein et al., 2013), the annual loss of DOC_SL + DOC_SR would be 0.07 Pg C year⁻¹, which represents about 3.5% of the annual net ocean uptake of CO₂ (Sarmiento and Gruber, 2006). Therefore, the overall loss of bioavailable DOC would significantly, but not massively, counteract the ocean CO₂ sink.

Conclusion

Our study found that, (1) the assumption of a constant Q₁₀ of ~2 is not universally applicable for the microbial degradation of DOC in the open ocean, (2) the empirically estimated Q₁₀ values indicate that increasing ocean temperature will enhance the microbial degradation of the more biologically resistant DOC with a high apparent E_a compared to the more labile DOC fractions with a low apparent E_a, and (3) increasing the equilibrium ocean temperature by just 1°C will result in a net loss of ~7 Pg C of bioavailable DOC. In this analysis we have not tested the temperature sensitivity for the DOC_R pool, since knowledge on the controls for removal of this pool is lacking, however abiotic processes are thought to contribute significantly (Hansell and Carlson, 2013). Further studies are therefore needed to determine the dominant loss processes for DOC_R before the possible temperature sensitivity can be assessed on this largest contributor to the global ocean carbon reservoir.

Author Contributions

CL and XÁ-S conceived the study. CL, XÁ-S, and DH authors contributed equally to the data survey, analysis, and interpretation. RL performed the model simulation, developed new tracer transport diagnostics, and interpreted the model outputs. All authors wrote the paper.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

This study was funded by the Australian Institute of Marine Science and a grant from the Carlsberg Foundation to CL. XÁ-S has been partially funded by the Spanish Ministry of Economy and Competitiveness grant numbers CSD2008–00077 (MALASPINA 2010), CTM2011–30010–C02–02 (HOT-MIX), and CTM2015-69392-C3-2-R (FLUXES), the latter two co-financed with FEDER funds. RL acknowledges support from the U.S. DOE Office of Science (DE-SC0016329). DH was supported by a grant from the US National Science Foundation (OCE-1436748). This work is a contribution of the SCOR Working Group 134 on the Microbial Carbon Pump in the Ocean.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fmars.2017.00436/full#supplementary-material

References

Ahlgren, G. (1987). Temperature functions in biology and their application to algal growth constants. Oikos 49, 177–190. doi: 10.2307/3566025

CrossRef Full Text | Google Scholar

Amon, R. M. W., and Benner, R. (1996). Bacterial utilization of different size classes of dissolved organic matter. Limnol. Oceanogr. 41, 41–51. doi: 10.4319/lo.1996.41.1.0041

CrossRef Full Text | Google Scholar

Arrhenius, S. Z. (1889). Über die reaktionsgeschwindigkeit bei der inversion von rohrzucker durch säuren. Phys. Chem. 4, 226–248.

Google Scholar

Arrieta, J. M., Mayol, E., Hansman, R. L., Herndl, G. J., Dittmar, T., and Duarte, C. M. (2015). Dilution limits dissolved organic carbon utilization in the deep ocean. Science 348, 331–333. doi: 10.1126/science.1258955

PubMed Abstract | CrossRef Full Text | Google Scholar

Balmaseda, M. A., Trenberth, K. E., and Källén, E. (2013). Distinctive climate signals in reanalysis of global ocean heat content. Geophys. Res. Lett. 40, 1754–1759. doi: 10.1002/grl.50382

CrossRef Full Text | Google Scholar

Bendtsen, J., Hilligsøe, K. M., Hansen, J. L. S., and Richardson, K. (2015). Analysis of remineralisation, lability, temperature sensitivity and structural composition of organic matter from the upper ocean. Prog. Oceanogr. 130, 125–145. doi: 10.1016/j.pocean.2014.10.009

CrossRef Full Text | Google Scholar

Benner, R., and Amon, R. M. (2015). The size-reactivity continuum of major bioelements in the ocean. Ann. Rev. Mar. Sci. 7, 185–205. doi: 10.1146/annurev-marine-010213-135126

PubMed Abstract | CrossRef Full Text | Google Scholar

Berner, R. A. (1964). An idealized model of dissolved sulfate distribution in recent sediments Geochim. Cosmochim. Acta 28, 1497–1503. doi: 10.1016/0016-7037(64)90164-4

CrossRef Full Text | Google Scholar

Bianchi, T. S. (2011). The role of terrestrially derived organic carbon in the coastal ocean: a changing paradigm and the priming effect. Proc. Natl. Acad. Sci. U.S.A. 108, 19473–19481. doi: 10.1073/pnas.1017982108

PubMed Abstract | CrossRef Full Text | Google Scholar

Brewer, P. G., and Peltzer, E. T. (2016). Ocean chemistry, ocean warming, and emerging hypoxia: Commentary. J. Geophys. Res. Oceans 121, 3659–3667. doi: 10.1002/2016JC011651

CrossRef Full Text | Google Scholar

Brewer, P. G., and Peltzer, E. T. (2017). Depth perception: the need to report ocean biogeochemical rates as functions of temperature, not depth. Philos. Trans. A Math. Phys. Eng. Sci. 375:20160319. doi: 10.1098/rsta.2016.0319

CrossRef Full Text | Google Scholar

Bullister, J. L., and Weiss, R. F. (1988). Determination of CCI3 and CCl2 F2 in seawater and air. Deep Sea Res. 35, 839–853. doi: 10.1016/0198-0149(88)90033-7

CrossRef Full Text | Google Scholar

Bussmann, I. (1999). Bacterial utilization of humic substances from the Arctic Ocean. Aquat. Microb. Ecol. 19, 37–45. doi: 10.3354/ame019037

CrossRef Full Text | Google Scholar

Carlson, C. A., and Hansell, D. A. (2015). “DOM sources, sinks, reactivity, and budgets,” in Biogeochemistry of Marine Dissolved Organic Matter, eds D. A. Hansell and C. A. Carlson (San Diego, CA: Academic Press), 65–126.

Google Scholar

Carlson, C. A., Hansell, D. A., Nelson, N. B. A., Siegel, D., Smethie, W. M., Khatiwala, S., et al. (2010). Dissolved organic carbon export and subsequent remineralization in the mesopelagic and bathypelagic realms of the North Atlantic basin. Deep Sea Res. II 57, 1433–1445. doi: 10.1016/j.dsr2.2010.02.013

CrossRef Full Text | Google Scholar

Chen, B., and Laws, E. A. (2017). Is there a difference of temperature sensitivity between marine phytoplankton and heterotrophs? Limnol. Oceanogr. 62, 806–817. doi: 10.1002/lno.10462

CrossRef Full Text | Google Scholar

Chin, W. C., Orellana, M. V., and Verdugo, P. (1998). Spontaneous assembly of marine dissolved organic matter into polymer gels. Nature 391, 568–572. doi: 10.1038/35345

CrossRef Full Text | Google Scholar

Davidson, E. A., and Janssens, I. A. (2006). Temperature sensitivity of soil carbon decomposition and feedbacks to climate change. Nature 440, 165–173. doi: 10.1038/nature04514

PubMed Abstract | CrossRef Full Text | Google Scholar

Davidson, E. A., Trumbore, S. E., and Amundson, R. (2000). Soil warming and organic carbon content. Nature 408, 789–790. doi: 10.1038/35048672

PubMed Abstract | CrossRef Full Text | Google Scholar

Del-Giorgio, P., and Davies, J. (2003). “Patterns of dissolved organic matter lability and consumption across aquatic ecosystems,” in Aquatic Ecosystems: Interactivity of Dissolved Organic Matter, eds S. E. G. Findlay and R. L. Sinsabaugh (San Diego, CA: Academic Press), 399–424.

Google Scholar

Delong, E. F., Preston, C. M., Mincer, T., Rich, V., Hallam, S. J., Frigaard, N. U., et al. (2006). Community genomics among stratified microbial assemblages in the ocean's interior. Science 311, 496–503. doi: 10.1126/science.1120250

PubMed Abstract | CrossRef Full Text | Google Scholar

Devries, T., and Primeau, F. (2011). Dynamically and observationally constrained estimates of water-mass distributions and ages in the global ocean. J. Phys. Oceanogr. 41, 2381–2401. doi: 10.1175/JPO-D-10-05011.1

CrossRef Full Text | Google Scholar

Drijfhout, S. S., Blaker, A. T., Josey, S. A., Nurser, A. J. G., Sinha, B., and Balmaseda, M. A. (2014). Surface warming hiatus caused by increased heat uptake across multiple ocean basins. Geophys. Res. Lett. 41, 7868–7874. doi: 10.1002/2014GL061456

CrossRef Full Text | Google Scholar

Druffel, E. R. M., Griffin, S., Coppola, A. I., and Walker, B. D. (2016). Radiocarbon in dissolved organic carbon of the Atlantic Ocean. Geophys. Res. Lett. 43, 5279–5286 doi: 10.1002/2016GL068746

CrossRef Full Text | Google Scholar

Druffel, E. R. M., Williams, P. M., Bauer, J. E., and Ertel, J. (1992). Cycling of dissolved and particulate organic matter in the open ocean. J. Geophys. Res. 97, 15539–15659. doi: 10.1029/92JC01511

CrossRef Full Text | Google Scholar

Fenchel, T., and Finlay, B. J. (1995). Ecology and Evolution in Anoxic Worlds. Oxford: Oxford University Press.

Google Scholar

Hansell, D. A. (2013). Recalcitrant dissolved organic carbon fractions. Ann. Rev. Mar. Sci. 5, 421–445. doi: 10.1146/annurev-marine-120710-100757

PubMed Abstract | CrossRef Full Text | Google Scholar

Hansell, D. A., and Carlson, C. A. (2013). Localized refractory dissolved organic carbon sinks in the deep ocean. Global Biogeochem. Cycles 27, 705–710. doi: 10.1002/gbc.20067

CrossRef Full Text | Google Scholar

Hansell, D. A., Carlson, C. A., and Schlitzer, R. (2012). Net removal of major marine dissolved organic carbon fractions in the subsurface ocean. Global Biogeochem. Cycles 26:GB1016. doi: 10.1029/2011GB004069

CrossRef Full Text | Google Scholar

Hansen, J. L. S., and Bendtsen, J. (2014). Seasonal bottom water respiration in the North Sea–Baltic Sea transition zone: rates, temperature sensitivity and sources of organic material. Marine Ecol. Prog. Ser. 499, 19–34. doi: 10.3354/meps10633

CrossRef Full Text | Google Scholar

Hedges, J. I. (2002). “Why Dissolved organics matter” in Biogeochemistry of Marine Dissolved Organic Matter, eds D. A. Hansell and C. A. Carlson (New York, NY: Academic Press), 1–33.

Google Scholar

Jiao, N., Herndl, G. J., Hansell, D. A., Benner, R., Kattner, G., Wilhelm, S. W., et al. (2010). Microbial production of recalcitrant dissolved organic matter: long-term carbon storage in the global ocean. Nat. Rev. Microbiol. 8, 593–599. doi: 10.1038/nrmicro2386

PubMed Abstract | CrossRef Full Text | Google Scholar

Karstensen, J., and Tomczak, M. (1998). Age determination of mixed water masses using CFC and oxygen data. J. Geophys. Res. Oceans 103, 18599–18609. doi: 10.1029/98JC00889

CrossRef Full Text | Google Scholar

Kattner, G., Simon, M., and Koch, B. P. (2011). “Molecular characterization of dissolved organic matter and constraints for prokaryotic utilization,” in Microbial Carbon Pump in the Ocean, eds N. Jiao, F. Azam, and S. Sansers (Washington, DC: Science/AAAS), 60–61.

Google Scholar

Keeling, R. E., Kortzinger, A., and Gruber, N. (2010). Ocean deoxygenation in a warming world. Ann. Rev. Mar. Sci. 2, 199–229. doi: 10.1146/annurev.marine.010908.163855

PubMed Abstract | CrossRef Full Text | Google Scholar

Keil, R. G., and Mayer, L. M. (2014). “Mineral Matrices and Organic Matter,” in Treatise on Geochemistry, 2nd Edn., eds H. Holland and K. Turekian (Amsterdam: Elsevier Science), 337–359.

Kerner, M., Hohenberg, H., Ertl, S., Reckermann, M., and Spitzy, A. (2003). Self-organization of dissolved organic matter tomicelle-like microparticles in river water. Nature 422, 150–154. doi: 10.1038/nature01469

PubMed Abstract | CrossRef Full Text | Google Scholar

Knorr, W., Prentice, I. C., House, J. I., and Holland, E. A. (2005). Long-term sensitivity of soil carbon turnover to warming. Nature 433, 298–301. doi: 10.1038/nature03226

PubMed Abstract | CrossRef Full Text | Google Scholar

Koven, C. D., Hugelius, G., Lawrence, D. M., and Wieder, W. R. (2017). Higher climatological temperature sensitivity of soil carbon in cold than warm climates. Nat. Clim. Change 7, 817–822. doi: 10.1038/nclimate3421

CrossRef Full Text | Google Scholar

Lagarias, J. C., Reeds, J. A., Wright, M. H., and Wright, P. E. (1998). Convergence properties of the nelder-mead simplex method in low dimensions. SIAM J. Optimiz. 9, 112–147. doi: 10.1137/S1052623496303470

CrossRef Full Text | Google Scholar

Legendre, L., Rivkin, R. B., Weinbauer, M. G., Guidi, L., and Uitz, J. (2015). The microbial carbon pump concept: potential biogeochemical significance in the globally changing ocean. Prog. Oceanogr. 134, 432–450. doi: 10.1016/j.pocean.2015.01.008

CrossRef Full Text | Google Scholar

Leifeld, J., and Von Lützow, M. (2013). Chemical and microbial activation energies of soil organic matter decomposition. Biol. Fertil. Soils 50, 147–153. doi: 10.1007/s00374-013-0822-6

CrossRef Full Text | Google Scholar

Letscher, R. T., Knapp, A. N., James, A. K., Carlson, C. A., Santoro, A. E., and Hansell, D. A. (2015). Microbial community composition and itrogen availability influence DOC remineralization in the South Pacific Gyre. Mar. Chem. 177, 325–334. doi: 10.1016/j.marchem.2015.06.024

CrossRef Full Text | Google Scholar

Letscher, R. T., and Moore, J. K. (2015). Preferential remineralization of dissolved organic phosphorus and non-Redfield DOM dynamics in the global ocean: impacts on marine productivity, nitrogen fixation, and carbon export. Global Biogeochem. Cycles 29, 325–340. doi: 10.1002/2014GB004904

CrossRef Full Text | Google Scholar

Li, W. K. W., and Dickie, P. M. (1987). Temperature characteristics of photosynthetic and heterotrophic activities: seasonal variations in temperate microbial plankton. Appl. Environ. Microbiol. 53, 2282–2295.

PubMed Abstract | Google Scholar

Lønborg, C., Cuevas, L. A., Reinthaler, T., Herndl, G. J., Gasol, J. M., Morán, X., et al. (2016). Depth dependent relationships between temperature and ocean heterotrophic prokaryotic production. Front. Mar. Sci. 3:90. doi: 10.3389/fmars.2016.00090

CrossRef Full Text | Google Scholar

Lønborg, C., Davidson, K., Álvarez-Salgado, X. A., and Miller, A. E. J. (2009). Bioavailability and bacterial degradation rates of dissolved organic matter in a temperate coastal area during an annual cycle. Mar. Chem. 113, 219–226. doi: 10.1016/j.marchem.2009.02.003

CrossRef Full Text | Google Scholar

Lønborg, C., and Álvarez-Salgado, X. A. (2012). Recycling versus export of bioavailable dissolved organic matter in the coastal ocean and efficiency of the continental shelf pump. Global Biogeochem. Cycles 26:GB3018. doi: 10.1029/2012GB004353

CrossRef Full Text | Google Scholar

Martínez-Pérez, A. M., Osterholz, H., Nieto-Cid, M., Álvarez, M., Dittmar, T., and Álvarez-Salgado, X. A. (2017). Molecular composition of dissolved organic matter in the Mediterranean Sea. Limnol. Oceanogr. 26, 2699–2712. doi: 10.1002/lno.10600

CrossRef Full Text

Meehl, G. A., Arblaster, J. M., Fasullo, J. T., Hu, A., and Trenberth, K. E. (2011). Model-based evidence of deep-ocean heat uptake during surface-temperature hiatus periods. Nat. Clim. Change 1, 360–364. doi: 10.1038/nclimate1229

CrossRef Full Text | Google Scholar

Middelburg, J. J., Klaver, G., Nieuwenhuize, J., Wielemaker, A., De Haas, W., Van Der Nat, J. F. W. A., et al. (1996). Organic matter mineralization in intertidal sediments along an estuarine gradient. Mar. Ecol. Prog. Ser. 132, 157–168. doi: 10.3354/meps132157

CrossRef Full Text | Google Scholar

Middelburg, J. J., Vlug, T., and Nat, F. J. W. (1993). Organic matter mineralization in marine systems. Global Planet. Change 8, 47–58. doi: 10.1016/0921-8181(93)90062-S

CrossRef Full Text | Google Scholar

Mopper, K., Kieber, D. J., and Stubbins, A. (2015). “Marine photochemistry of organic matter,” in Biogeochemistry of Marine Dissolved Organic Matter, eds D. A. Hansell and C. A. Carlson (San Diego, CA: Academic Press), 389–450.

Moran, M. A., and Zepp, R. G. (1997). Role of photoreactions in the formation of biologically labile compounds from dissolved organic matter. Limnol. Oceanogr. 42, 1307–1316. doi: 10.4319/lo.1997.42.6.1307

CrossRef Full Text | Google Scholar

Oelkers, E. H. (1991). Calculation of diffusion coefficients for aqueous organic species at temperatures from 0 to 350°C. Geochim. Cosmochim. Acta 55, 3515–3529. doi: 10.1016/0016-7037(91)90052-7

CrossRef Full Text | Google Scholar

Peltier, W. R., Liu, Y., and Crowley, J. W. (2007). Snowball Earth prevention by dissolved organic carbon remineralization. Nature 450, 813–818. doi: 10.1038/nature06354

PubMed Abstract | CrossRef Full Text | Google Scholar

Primeau, F. W., Holzer, M., and Devries, T. (2013). Southern Ocean nutrient trapping and the efficiency of the biological pump. J. Geophys. Res. Oceans 118, 2547–2564. doi: 10.1002/jgrc.20181

CrossRef Full Text | Google Scholar

Purkey, S. G., and Johnson, G. C. (2010). Warming of global abyssal and deep Southern Ocean waters between the 1990s and 2000s: contributions to global heat and sea level rise budgets. J. Clim. 23, 6336–6351. doi: 10.1175/2010JCLI3682.1

CrossRef Full Text | Google Scholar

Ratkowsky, D. A., Lowry, R. K., Mcmeekin, T. A., Stokes, A. N., and Chandler, R. E. (1983). Model for bacterial culture growth rate throughout the entire biokinetic temperature range. J. Bacteriol. 154, 1222–1226.

PubMed Abstract | Google Scholar

Raymond, P. A., and Spencer, R. G. M. (2015). “Riverine DOM,” in Biogeochemistry of Marine Dissolved Organic Matter, eds D. A. Hansell and C. A. Carlson (Amsterdam: Elsevier), 509–533.

Repeta, D. J. (2015). “Chemical characterization and cycling of dissolved organic matter,” in Biogeochemistry of Marine Dissolved Organic Matter, eds D. A. Hansell and C. A. Carlson (San Diego, CA: Academic Press), 21–63.

Google Scholar

Rhein, M., Rintoul, S. R., Aoki, S., Campos, E., Chambers, D., Feely, R. A., et al. (2013). “Observations: Ocean,” in Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change, eds T. F. Stocker, D. Qin, G.-K. Plattner, M. Tignor, S. K. Allen, J. Boschung, et al. (Cambridge; New York, NY: Cambridge University Press), 255–315.

Google Scholar

Robinson, C., and Williams, P. J. L. (2005). “Respiration and its measurement in surface marine waters,” in Respiration in Aquatic Ecosystems, eds P. A. Del Giorgio and P. J. L. Williams (Oxford: Oxford University Press), 147–180.

Google Scholar

Rontani, J.-F., Charrière, B., Sempéré, R., Doxaran, D., Vaultier, F., Vonk, J. E., et al. (2014). Degradation of sterols and terrigenous organic matter in waters of the Mackenzie Shelf, Canadian Arctic. Organ. Geochem. 75, 61–73. doi: 10.1016/j.orggeochem.2014.06.002

CrossRef Full Text | Google Scholar

Sarmiento, J. L., and Gruber, N. (2006). Ocean Biogeochemical Dynamics. Princeton, NJ: Princeton University Press.

Google Scholar

Schlitzer, R. (2016). Ocean Data View. Available online at: https://odv.awi.de

Seiki, T., Date, E., and Izawa, H. (1991). Decomposition characteristics of particulate organic matter in hiroshima bay. J. Oceanogr. 47, 207–220. doi: 10.1007/BF02310036

CrossRef Full Text | Google Scholar

Sexton, P. F., Norris, R. D., Wilson, P. A., Pälike, H., Westerhold, T., and Röhl, U. (2011). Eocene global warming events driven by ventilation of oceanic dissolved organic carbon. Nature 471, 349–353 doi: 10.1038/nature09826

PubMed Abstract | CrossRef Full Text | Google Scholar

Sierra, C. A. (2012). Temperature sensitivity of organic matter decomposition in the Arrhenius equation: some theoretical considerations. Biogeochemistry 108, 1–15. doi: 10.1007/s10533-011-9596-9

CrossRef Full Text | Google Scholar

Sjöstedt, J., Pontarp, M., Tinta, T., Alfredsson, H., Turk, V., Lundberg, P., et al. (2013). Reduced diversity and changed bacterioplankton community composition do not affect utilization of dissolved organic matter in the Adriatic Sea. Aquat. Microb. Ecol. 71, 15–24. doi: 10.3354/ame01660

CrossRef Full Text | Google Scholar

Sokal, F. F., and Rohlf, F. J. (1995). Biometry. New York, NY: Freeman.

Google Scholar

Swanson-Hysell, N. L., Rose, C. V., Calmet, C. C., Halverson, G. P., Hurtgen, M. T., Maloof, A., et al. (2010). Cryogenian glaciation and the onset of carbon-isotope decoupling. Science 328, 608–611. doi: 10.1126/science.1184508

PubMed Abstract | CrossRef Full Text | Google Scholar

Teng, Y.-C., Primeau, F. W., Moore, J. K., Lomas, M. W., and Martiny, A. C. (2014). Global-scale variations of the ratios of carbon to phosphorus in exported marine organic matter. Nat. Geosci. 7, 895–898. doi: 10.1038/ngeo2303

CrossRef Full Text | Google Scholar

Thingstad, T. F., Havskum, H., Kaas, H., Nielsen, T. G., Riemann, B., Lefevre, D., et al. (1999). Bacteria-protist interactions and organic matter degradation under P-limited conditions: analysis of an enclosure experiment using a simple model Limnol. Oceanogr. 44, 62–79. doi: 10.4319/lo.1999.44.1.0062

CrossRef Full Text | Google Scholar

Westrich, J. T., and Berner, R. A. (1988). The effect of temperature on rates of sulfate reduction in marine sediments. Geomicrobiol. J. 6, 99–117. doi: 10.1080/01490458809377828

CrossRef Full Text | Google Scholar

Whitney, F. A., Freeland, H. J., and Robert, M. (2007). Persistently declining oxygen levels in the interior waters of the eastern subarctic Pacific. Prog. Oceanogr. 75, 179–199. doi: 10.1016/j.pocean.2007.08.007

CrossRef Full Text | Google Scholar

Yvon-Durocher, G., Caffrey, J. M., Cescatti, A., Dossena, M., Del Giorgio, P., Gasol, J. M., et al. (2012). Reconciling the temperature dependence of respiration across timescales and ecosystem types. Nature 487, 472–476. doi: 10.1038/nature11205

PubMed Abstract | CrossRef Full Text | Google Scholar

Zehnder, A. J. B., and Stumm, W. (1988). “Geochemistry and biogeochemistry of anaerobic habitat,” in Biology of Anaerobic Microorganisms, ed A. J. B. Zehnder (New York, NY: John Wiley and Sons), 1–38.