Is any awareness necessary for an Ne?

The Error-Related Negativity (Ne or ERN) is a reliable electrophysiological index of error processing, which has been found to be independent of whether a subject is aware of an error or not. A large Ne was equally seen after errors that were consciously detected (Aware errors) and those that were not (Unaware errors), compared to a small negativity for correct responses (CRN). This suggests a dissociation between an automatic, preconscious error processing mechanism and subjective evaluation. A common concern regarding this finding is that subjects could have been somewhat aware of their errors, but did not report them due to lack of confidence. Here we tested this possibility directly using a betting paradigm which allowed us to separate occasions in which the subjects were confident of their response and trials in which they were unsure. In a choice reaction time task, subjects directly judged the accuracy of each response (correct or error) and then bet on this judgment using a high, medium, or low amount of money. The bets were used to determine the level of confidence the subjects had of their response. The average across all subjects regardless of confidence (betting) measure replicated the reported finding of an equal Ne for Aware and Unaware errors which was larger than the CRN. However, when Ne measurement was confined to high confidence (high bet) trials in confident subjects, a prominent Ne was seen only for Aware errors, while confident Unaware errors (i.e., error trials on which subjects made high bets that they were correct) elicited a response that did not differ from the CRN elicited by truly correct answers. In contrast, for low confidence trials in unconfident subjects, an intermediate and equal Ne/CRN was elicited by Correct responses, Aware and Unaware errors. These results provide direct evidence that the Ne is related to error awareness, and suggest the amplitude of the Ne/CRN depends on individual differences in error reporting and confidence.

Introduction

In everyday life, we are sometimes acutely aware of having made an error (the notorious “oops” sensation), but at other times we are oblivious of our errors (e.g., when we make typographical errors). In many cases, effective performance of a task requires that errors will be promptly detected and swiftly corrected (e.g., driving or machine operation). Understanding the conditions that lead to error awareness is important for understanding the executive functions that guide goal-directed activity and learning. One of the most intriguing questions in the area of error awareness is why some errors reach awareness while others stay unnoticed. Yet before this question can be answered, it is crucial to determine whether unnoticed errors are processed as errors at all. Using electrophysiology, it has repeatedly been shown that errors in various tasks and modalities are processed as errors by the brain even if we are unaware of making them (Nieuwenhuis et al., 2001; Endrass et al., 2005, 2007; O'Connell et al., 2007, 2009; Pavone et al., 2009; Shalgi et al., 2009; Dhar et al., 2011; Hughes and Yeung, 2011). In each of these studies, awareness of errors was reported on a single trial basis, and a large Error-Related Negativity (Ne; Falkenstein et al., 1991; or ERN; Gehring et al., 1993), a fronto-central ERP component locked to the incorrect response, was equally seen after errors that were reported (Aware errors) and those that weren't (Unaware errors). In most cases, a much smaller response-locked negativity was elicited by correct responses (Correct-Related Negativity, CRN; Ford, 1999; Vidal et al., 2000; Coles et al., 2001). In contrast to the insensitivity of the Ne to awareness, a later centro-parietal Error Positivity wave (Pe; Falkenstein et al., 1991) was elicited only after Aware errors. Two additional event-related fMRI studies by Hester et al. (2005) and Klein et al. (2007) further supported these findings by showing that the Anterior-Cingulate region associated with the generation of the Ne (Dehaene et al., 1994; Brazdil et al., 2002; Debener et al., 2005) does not show a difference between Aware and Unaware errors (see also Stemmer et al., 2004), while Aware errors only were associated with larger bilateral activation of prefrontal and parietal regions (Hester et al., 2005) or left anterior Insula activity (Klein et al., 2007). These findings led to the widespread notion that error monitoring, as reflected by the Ne, goes on regardless of conscious awareness of making errors (e.g., Simons, 2009). However, findings from a handful of studies that showed that the Ne was smaller for ostensibly unaware errors raise doubts regarding whether the Ne is truly insensitive to awareness.

Maier et al. (2008, Experiment 2), using a masked Flanker task, and Steinhauser and Yeung (2010), using a brightness discrimination task, both asked subjects to judge their own accuracy on a single-trial basis, and found a larger Ne for Aware than for Unaware errors. Woodman (2010) used a visual-search task in which in some trials the targets were rendered invisible by substitution masking. Although the participants were not required to indicate awareness of their errors, errors in masked-target trials were assumed to be Unaware errors because the participants were at chance in detecting the presence of the target. An Ne was generated only by errors committed during the non-masked trials, even though masked targets also attracted attention, as evidenced by the N2pc component. Praamstra et al. (2003) induced errors in a cued tapping task by small or large shifts (15 or 50 ms) in the cue timing. While they also did not require their subjects to report awareness of an error, they assumed that the small shifts are below perception threshold whereas the large shifts are consciously detected. Since only large shifts were followed by an Ne, the results were once again taken to suggest that awareness of the errors is a prerequisite for Ne elicitation.

A major critique for the experiments that showed that the Ne was independent of conscious awareness pertains to the determination of the level of error awareness. Were subjects really unaware of the error or was their criterion for reporting an error too high? This criterion may be influenced by individual tendencies, as well as by task instructions and demand characteristics artifacts. If subjects are in fact under-reporting their awareness, the Unaware Error bin could be contaminated by (unreported) Aware errors which have a larger Ne, leading to an inflated Ne for Unaware errors.

This possibility was in fact directly addressed by Scheffers and Coles (2000) who used certainty ratings instead of error-reporting on a trial-by-trial basis. Their subjects performed a Flanker task and immediately afterwards had to indicate their confidence on a five-point scale from “sure correct,” through “don't know,” to “sure incorrect.” The size of the Ne depended on the subjective confidence of making an error. These results have recently been replicated by Hewig et al. (2011) and suggest that the Ne is an index of subjective, rather than objective error monitoring. This conjecture is supported by studies which used indirect measure of error certainty, such as post-test questionnaires and reaction times (RTs), under the assumption that slower responses indicate uncertainty (Pailing and Segalowitz, 2004a; Wessel et al., 2011). All the above studies that measured the Pe component (Steinhauser and Yeung, 2010; Woodman, 2010; Hewig et al., 2011; Wessel et al., 2011), found that it was larger for Aware errors than Unaware errors. Taken together, these studies support the premise that the Ne, like the Pe, is an index of subjective error processing. They contradict a large body of evidence (including our own, Shalgi et al., 2009 and Shalgi and Deouell, 2010) that showed no effect of awareness on the Ne.

Here, we directly addressed this contradiction by using a paradigm in which we previously found a similar Ne for Aware and Unaware errors (Shalgi and Deouell, 2010), together with a wagering paradigm (see Ullsperger et al., 2010) which was shown to be a more objective measure of awareness then direct introspection (Persaud et al., 2007). Instead of directly asking participants to assess their own certainty, the subjects are required to make a bet of a small, medium, or large amount of money on their accuracy judgment (rather than on the actual response). If the participants' decision about their accuracy is correct, they win this money; otherwise, they lose it. The optimal strategy is to bet high whenever they feel they are not just guessing. In other words, participants are willing “to put their money where their mouth is” (Koch and Preuschoff, 2007). In the current study, we employed this betting paradigm to separate trials in which the participants were sure of their response from trials in which they were unsure without explicitly asking for confidence ratings. We expected that if the Ne is indeed independent of awareness, it should be elicited even when the subjects are highly confident that they are right. Conversely, if Ne is only elicited by Aware errors, it should be eliminated if subjects are confident that they had not made an error.

To summarize, previous studies suggested that the Ne is independent from error awareness, while other findings showed that the Ne is affected by subjective confidence. We aimed to replicate both findings in the same experiment, with better confidence assessment, in order to explain the discrepancy between the former studies.

Materials and Methods

Subjects

The participants were 22 students (14 males, all right handed as a prerequisite due to the lateralized aspect of the task), aged 23–32 (mean = 25.73, SD = 2.7), with reportedly normal or corrected-to-normal vision and no history of neurological disorders. Two additional subjects were tested but were excluded from analysis; one due to a very small number of Unaware errors (see “Analysis of Behavior”) and the other due to a misunderstanding of task instructions. All students were paid a minimum flat fee or given course credits for their participation in the study. In addition, they could make up to an extra 25 NIS (∼7$, the equivalent of lunch at the campus cafeteria) by wagering correctly on their responses in the experiment (see “Procedure”), but they could never lose money (i.e., get less than the minimum fee). Written consent was obtained after the experimental procedures were explained. The study was approved by the Ethics Committee of the Faculty of Social Science at the Hebrew University.

Stimuli and Apparatus

Participants sat in a dimly lit, sound attenuated and echo-reduced chamber (Eckel C-26, UK). Stimuli included seven white shapes (diamond, triangle, pentagon, parallelogram, trapezoid, circle, pie) of four different sizes (subtending visual angles of 1.2°–1.8°) on a gray background, presented on a Viewsonic G75f CRT monitor, 100 cm from the subjects' eyes. A row of three shapes appeared on every trial (see Figure 1). The lateral shapes and the central shape were never the same size. Shapes were spaced 5.08° apart. A cushioned tray was placed on the subjects' lap. On the right of the tray was a two-button response box with the buttons “YES” and “NO,” their side counterbalanced across participants. On the left was a computer mouse used to make the secondary responses (see “Procedure”).

FIGURE 1

Figure 1. The trial procedure and some example trials.

Procedure

We developed the Lateralized Error Awareness Task (LEAT) to enable the separation of left and right errors and to obtain an awareness index of each error (Shalgi and Deouell, 2010). The LEAT is a manual choice-reaction time task in which participants are presented with three horizontally aligned shapes in each trial. In the primary task, the participants are instructed to press the “YES” button if one of the shapes is a designated target shape (Shape target), or if one of the lateral shapes (left or right) is the same shape as the central shape (Matching target), disregarding shape sizes, and to press the “NO” button otherwise.

A small black fixation cross appeared constantly at the center of the screen. Each trial started with the three shapes presented for 120 ms. Four hundred milliseconds after their disappearance, the color of the central fixation changed from black to light gray. The participants were instructed to respond as fast as possible only once the fixation had changed its color, creating a response delay in order to increase the number of Unaware errors (see Shalgi et al., 2007). There was no time limit on the response, so participants were forced to make a choice in each trial, but if their RT was longer than 1320 ms, a screen was displayed which reminded the participants to respond when the color of the fixation changes. Shape presentation time was too short to allow lateral eye movements; participants were required to fixate on the central cross and were advised that this will allow for best performance.

Five to seven hundred milliseconds after the primary response, an “Accuracy Judgment” screen appeared in which the choice the participant had just made (“YES” or “NO”) was displayed, together with two on-screen buttons labeled “Correct” and “Error,” and the participant had to click one of the buttons using the mouse controlled with their left hand. The left hand was used for mouse responses as the right (dominant) hand was always kept on the response box for the primary speeded response. Immediately after the participants made their judgment, a “Betting” screen appeared which informed the participant of the judgment they had just made (“Correct” or “Error”) and asked them to bet on this decision by clicking the mouse on one of three credits: 1 (small bet), 2 (medium bet), or 3 (high bet). The participants were not told how much money each credit was worth, only that a larger number of credits meant more money (as an added incentive, an image of a small, medium, or large money bag was displayed beside each credit amount, see Figure 1). The participants were told that if they had made a correct accuracy judgment they would win the number of credits they had bet on, but in case of an incorrect accuracy judgment they would lose that same amount. Both the Accuracy Judgment and the Betting screens were displayed until the participants made their choice. The black fixation cross stayed on constantly during these screens. After placing a bet, the fixation screen was displayed for 800–1000 ms, followed by the next trial.

Each block consisted of 88 trials, of which 44 were target trials (50%), equally divided between Shape and Matching targets. Each target shape or matching shape appeared equally on the left or on the right (the target shape never appeared as the central shape). The order of the stimuli was randomized across participants. To complicate the task and elicit more errors, every 22 trials (i.e., four times per block), a different shape (one of the seven possible shapes at random) was designated as the Shape target, using a screen that announced the change and displayed the new target shape at the center of the screen for two seconds. Each block lasted approximately six minutes. The results of each block were not displayed to the participants so they could not use them to change or adjust their strategy.

Two practice blocks of 15 trials each preceded the experiment, both with neither the Accuracy judgment screen nor the Betting screen. In the first practice, the participants were introduced only to the Matching targets in order to acquaint them with the different shapes and sizes and to gradually ease them into the task. In the second practice, the Shape target condition was introduced. When the participants were confident in their understanding of the task, they performed 16 consecutive experimental blocks. Due to the length of the experiment, after eight blocks the participants were taken out of the recording chamber for a 5–10 minute rest, without removing the electrode cap.

Following the testing session, participants filled a questionnaire in which they rated task difficulty and estimated their own accuracy. The amount of credits won at the end of the experiment was converted to cash using a pre-formed conversion table.

Analysis of Behavior

Errors judged as “Error” in the Accuracy Judgment screen were considered Aware errors, and Errors judged as “Correct” were considered Unaware errors. Correct responses judged as “Error” were classified as False Errors. The percentage of False Errors for each participant was very low, ranging between 0.1–6% (mean 2.1%, SD 1.6%), and therefore, this type of error was not further analyzed. Trials with primary RTs faster than 50 ms or slower than 2500 ms were excluded from analysis. Accuracy was calculated separately for each bet category (1, 2, or 3). Error Awareness rate was calculated as the number of Aware errors divided by the total number of errors. RTs were calculated across blocks separately for the different response types and for the different bet categories (there were not enough error trials to divide response type by bet). The different measures were compared separately using repeated measures ANOVAs. Greenhouse–Geisser correction was applied when necessary and the uncorrected degrees of freedom are presented along with the Greenhouse–Geisser epsilon where it was <1 (Picton et al., 2000). Contrasts were performed using paired sample t-tests.

EEG Recording

EEG was recorded continuously with Ag/AgCl electrodes from 64 scalp electrodes, left and right mastoid sites, and the tip of the nose, using a BioSemi Active 2 system (Biosemi, Netherlands). Blinks and eye movements were monitored using four additional EOG electrodes located at the outer canthus of the right and left eyes and above and below the center of the right eye. The EEG was continuously sampled at 512 Hz and stored for off-line analysis. Analsyis was conducted using BrainVision Analyzer 2 (Brain Products, Germany), and Matlab R2011a (The MathWorks Inc., Natick, MA). For two participants, data from one malfunctioning electrode (P3 or CP3) was replaced by using spherical spline interpolation (Perrin et al., 1989; Perrin, 1990, implemented by Analyzer 2). The EEG data was digitally referenced to the nose and filtered with a bandpass of 0.5–20 Hz (zero-phase 24 dB/octave Butterworth filter). Blink artifacts were removed using the Independent Component Analysis (ICA) method (Jung et al., 2000; as implemented in Analyzer 2). Note that although we corrected for blink artifacts, we first verified that the participants did not blink during stimulus presentation. This was the case for all subjects and trials, with negligible exceptions. Segments contaminated by other artifacts were discarded (rejection criteria: >100 μV absolute difference between samples within segments of 100 ms; absolute amplitude beyond the ±100 μV range). Artifact-free EEG data was parsed into 900 ms response-locked segments starting 400 ms before the response. An average of 93.6% segments survived artifact rejection (range per participant, per response type 75.7–100%). The inclusion criterion was at least 20 Unaware Error segments which survived artifact rejection.

Response-locked segments were averaged separately for Correct responses, Aware errors, and Unaware errors, and within each category for bets 1 and 3. The 2 (medium) bet was excluded from analysis as it was only used to get a cleaner measure of the participants' confidence in the 1 and 3 bet bins. By including this bet option, we could be more confident that when participants chose to bet on 1 they were unsure of their decision and when they chose to bet on 3 they were confident, as all the intermediate cases would fall into the 2 bin. Potentials were measured relative to a 400–200 ms pre-response baseline period (O'Connell et al., 2007; Shalgi et al., 2009). This baseline period did not include the immediate pre-response period as the neural activity leading to the response naturally starts earlier than the final motor outcome of the button press.

ERP Analysis

The Error Negativity: The Ne amplitude and latency were detected individually for each participant and each subcategory and measured in the response-locked averages as the mean amplitude ±4 ms around the most negative peak at the interval of 0–150 ms post-response at electrode FCz.

The Error Positivity: As the Pe is a more sustained parietal wave, it was pre-defined based on our previous studies as the average amplitude at electrode Pz between 300 and 500 ms post response in the response locked averages. Since inspection of the grand average waveforms (see Figure 3) suggested that the Pe began at around 200 ms, we also performed all analyses of the Pe on the 200–500 ms timeframe, and obtained the same qualitative results. Therefore, only the results of the first analysis will be reported.

To simulate traditional procedures, the Ne and Pe amplitudes were entered separately into One-Way repeated measures ANOVAs for Response Type (Correct, Aware Error, Unaware Error), across all subjects and bets. Greenhouse–Geisser correction was applied where necessary. Contrasts were performed using paired sample t-tests.

The cardinal question of this study was whether the Ne is affected by awareness when subjects are highly confident of being aware or unaware of making an error. To that end, we isolated the group of 12 participants who had enough (defined a-priori as more than 20 EEG segments available for analysis) Aware and Unaware error trials with bet 3, and repeated the above One-Way ANOVA analysis with bet 3 trials only. A similar analysis for bet 1 responses was conducted for those eight subjects who had enough Aware and Unaware errors with bet 1. Three subjects were included in both groups, four participants did not have enough segments to be included in any group and one participant was excluded from this analysis due to noisy data (see “Analysis of the Response-Locked Waveforms After Subtracting the Stimulus-locked Waveform” in Appendix for the number of trials in each group).

Hardly any subjects bet enough on both 1 and 3 for both Aware and Unaware errors to allow a full within subject analysis. Therefore, in order to compare the awareness effect between confident and less confident subjects, we omitted the three subjects who were included in both groups above from the high confidence group, which now included only those nine subjects who had enough bet 3 errors of both types but less than 20 bet 1 errors. The Ne and Pe of the High Confidence (HiC) and Low Confidence (LoC) groups were then compared across all bets using a mixed ANOVA with Group as the between-subject factor and Response Type as the within-subject factor. Contrasts were performed using independent-samples t-tests.

Results

Behavioral results

Accuracy and error awareness

The behavioral results are summarized in Figure 2. Although the subjects rated the task as relatively difficult (mean 3.6/5, SD 0.85), accuracy in the task was quite high (85.9%, SD 4.6%). Overall awareness of errors was 50.1% (SD 14.7%).

FIGURE 2

Figure 2. Behavioral results of all 22 participants.

Overall, bet 3 was the popular bet (67.9%), followed by bet 2 (19.6%), and finally bet 1 (12.3%). Rational calculations predict that due to the high accuracy in the task, a strategy of always betting on 3 would yield the highest result, and participants might have intuitively made this assumption. Figure 2B describes the betting patterns for the different response types. When the participants were correct, they were usually certain about their answer (bet 3). Uncertainty went up with the different types of errors: when participants made an Aware Error they tended to bet less on 3 than when they were correct, and even less so when they made an Unaware error. They were least certain when they (rarely) mistakenly judged a correct response as an error. Primary response accuracies for the three bet categories were 52.8% (SD 12.4) for bet 1, 75.1% (SD 12.2) for bet 2 and 91.1% (SD 4) for bet 3 [F_{(2, 38)} = 152.9, p < 0.001, ε = 0.95]; two subjects were excluded from this analysis as they did not have any bet 2 or any bet 1 choices. Accuracy for bet 1 was lower than for bet 2 [t(19) = 10.55, p < 0.001], which was in turn lower than the accuracy for bet 3 [t(19) = 7.89, p < 0.001].

Reaction times

Reaction times in this task were relatively long (across bets: Correct responses 814.7 ms, SD 42.8; Aware errors 885.8 ms, SD 64; Unaware errors 889 ms, SD 64.9) due to the task instructions to delay responses until the fixation changed its color. Nevertheless, the RTs depended on accuracy [F_{(2, 42)} = 54.67, p < 0.001, ε = 0.98] as the RTs for Correct responses were shorter than those for errors [t(21) = 11.34, p < 0.001]; there was no difference between RTs for Aware and Unaware errors p = 0.71. There was also a significant difference between RTs of responses followed by the different bet categories [F_{(2, 38)} = 66.61, p < 0.001, ε = 0.99]: RTs followed by bet 1 (952.2 ms, SD = 84.9) were significantly longer than RTs followed by bet 2 [885 ms, SD = 98.1; t(19) = 5.47, p < 0.001] and these in turn were significantly longer than RTs followed by bet 3 [796.9 ms SD = 41.4; t(20) = 6.33, p < 0.001]. This supports the assumption that participants bet on 1 when they were less confident of their response (slower RTs due to hesitation) and on 3 when they were confident.

Debriefing

Upon debriefing in the post-test questionnaire, all participants noted they made their bets according to their level of certainty. Taken together with the behavioral results, we can be quite sure that each bet category corresponds to a certainty rating.

ERP Results

Awareness effects regardless of confidence

Replicating the results of traditional studies not assessing confidence, a clear Ne was elicited across subjects and bets by both Aware and Unaware errors, beginning before the response and peaking after the response (Figure 3A). A CRN can be seen at about the same time for Correct responses. A significant effect in a One-Way repeated measures ANOVA for Response Type was found for the Ne amplitude [F_{(2, 42)} = 10.2, p < 0.001, ε = 0.959]. This stemmed from a difference between errors and Correct responses [t(21) = 4.57, p < 0.001] while there was no difference between the Ne amplitude for Aware and Unaware errors [t(21) = 1.79, p = 0.09]. Note that while the peak-to-trough CRN seems to be around the same size or even larger than the Ne, supplementary analysis (see “Analysis of the Response-locked Waveforms After Subtracting the Stimulus-locked Waveform” in Appendix) which corrected for the overlapping effect of the stimulus-locked P3, revealed that the CRN is indeed smaller than the Ne. Figure 3B illustrates the results at electrode Pz, where the Pe can be seen as the slow positivity following the response for Aware errors only. A significant effect in a One-Way repeated measures ANOVA for Response Type was found for the Pe amplitude [F_{(2, 42)} = 35.32, p < 0.001, ε = 0.82], which stemmed from the difference between Aware errors and Correct responses [t(21) = 6.67, p < 0.001] and the difference between Aware errors and Unaware errors [t(21) = 6.47, p < 0.001].

FIGURE 3

Figure 3. Grand average (N = 22) response-locked ERPs to correct and error responses across all bets at electrode (A) FCz and (B) Pz. The bottom panel shows the scalp topography at the time of (A) the CRN/Ne peak and (B) the Pe.

Awareness effects with confident accuracy judgments

The most critical question in our study is whether awareness affects the Ne when subjects are highly confident of having made an error or highly confident that they have not made one, as indicated by their willingness to put a high bet on this decision. Note that when participants make an error and bet the highest bet on having made one, they are confident that an error has been made, that is, they demonstrate high error awareness. Similarly, when subjects place the highest bet on having made a correct response, when in fact they have committed an error, they demonstrate clear unawareness of the error. Thus, we isolated 12 subjects who had enough bet 3 trials for both Aware and Unaware errors (see “Methods”), and measured the Ne and Pe for their bet 3 trials only. Contrary to the previous results, the Ne was larger for Aware errors than for Unaware errors, and Unaware errors did not differ from Correct responses (Figure 4A), main effect: [F_{(2, 22)} = 8.65, p < 0.005, ε = 0.77], Aware errors vs. Unaware errors: [t(11) = 3.26, p < 0.01], Corrects vs. Unaware errors: [t(11) = 0.42, p = 0.68]. Thus, when only clear cases of subjective error awareness or unawareness are taken into consideration, the Ne is found to be strongly affected by error awareness. The Pe too was large only for Aware errors in the bet 3 trials in this group [F_{(2, 22)} = 20.35, p < 0.001, ε = 0.67], Correct Responses vs. Aware errors: [t(11) = 4.67, p < 0.005], Aware vs. Unaware: [t(11) = 5.36, p < 0.001]. Thus, both the Ne and the Pe are larger for Aware errors when subjects are confident of their awareness.

FIGURE 4

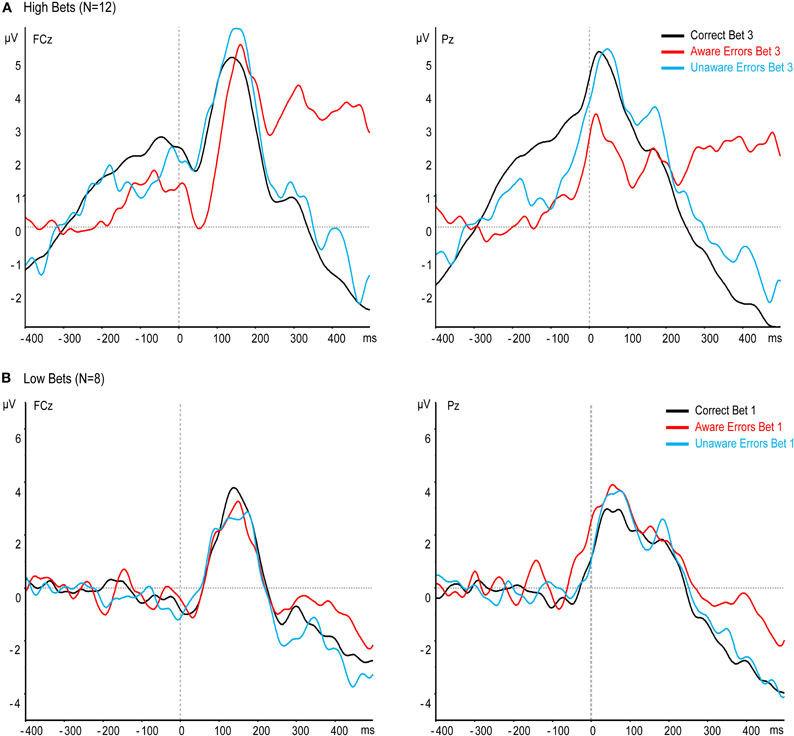
Figure 4. Effect of confidence on response-locked ERPs at electrodes FCz and Pz, when only high (A) or low (B) bets are averaged.

Awareness effects with low-confidence accuracy judgments

To examine the awareness effect when subjects are not sure about their performance, we isolated eight subjects who had enough bet 1 trials for both Aware and Unaware errors (see “Methods”), and measured the Ne and Pe for their bet 1 trials only. When subjects were only willing to place the lowest possible bet on their decision, we surmise that they were not sure of whether they had made an error or not. In other words, they may have had only a trace of awareness of an error when they had made one, and conversely may have had some trace of awareness of an error even if one had not been committed. Indeed, the Ne and Pe were similar for all responses—Correct, Aware errors, and Unaware errors (Figure 4B), [Ne: F_{(2, 14)} < 1, p = 0.47, ε = 0.67; Pe: F_{(2, 14)} = 2.77, p = 0.13, ε = 0.6]. This finding supports the notion that the Ne is affected by the level of subjective error awareness.

Since there were many Correct responses with both bets in this group of subjects, we could directly compare between bet 1 and bet 3. “Analysis of the Correct Response-locked Waveforms of the Low Confidence Group After Subtracting the Stimulus-locked Waveform”in Appendix shows the CRN and Pe of Correct responses for the two bets after correcting for the overlapping effect of the stimulus locked P3. The difference between the CRN for bet 1 and bet 3 was significant [t(7) = 2.38, p < 0.05]. However, the Pe for Correct responses did not significantly differ between bet 1 and 3 [t(7) = 1, p = 0.35].

Direct comparison between low and high confidence groups

As noted in the methods section, the number of errors in each response-type and bet precluded a complete within-subject analysis of high and low bets. However, we could compare high and low confidence subjects, collapsing across their bets (see “Methods”). For this analysis, high confidence subjects were those nine subjects who had made enough bet 3 aware and unaware errors but few (<20) bet 1 errors (HiC group), while low confidence subjects were the eight subjects who had enough bet 1 aware and unaware errors (LoC group). As the groups did not overlap, they could be compared directly (Table 1, Figures 5 and 6). The groups differed significantly in primary task accuracy [t(15) = 2.88, p < 0.05] but not in error awareness rates [t(15) = 1.16, p = 0.26] or subjective rating of task difficulty [t(15) = 1.2, p = 0.25]. As may be expected by the group division criteria, the betting patterns of the groups (Figure 5) were significantly different; a significant main effect of the within-subject factor Bet was moderated by an interaction with the between-subject factor Group in a mixed ANOVA [main effect: F_{(2, 30)} = 28.7, p < 0.001, ε = 0.71; interaction: F_{(2, 30)} = 6.98, p < 0.01]. The interaction stemmed from different usage of the 1 and 3 bets between groups.

TABLE 1

Table 1. Comparison of the behavioral results of the High and Low Confidence Groups.

FIGURE 5

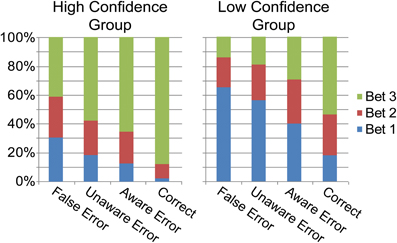
Figure 5. Proportion of each bet category for each response type for the High and Low Confidence groups.

FIGURE 6

Figure 6. Effect of confidence on response-locked ERPs at electrodes FCz and Pz. (A) High confidence group (B) Low confidence group. (C) The interaction between Group and Response Type.

Figure 6 shows a dramatic effect of confidence in the Ne period. While the Ne for Unaware errors aligns with the Ne for Aware errors in the LoC group, there is practically no Ne for Unaware errors in the HiC group. That is, the response for Unaware errors is similar to the CRN elicited by Correct responses. A mixed ANOVA with the within factor Response Type and between factor Group for the Ne amplitude confirmed these results, showing a significant effect of Response Type [F_{(2, 30)} = 8.8, p < 0.005, ε = 0.95] which was moderated by a significant interaction [F_{(2, 30)} = 3.55, p < 0.05]. To elucidate this interaction, we compared the Ne amplitude between groups separately for each response type. There was no significant difference between the Ne for Correct responses [t(15) = 0.77, p = 0.45] or for Aware errors [t(15) = 0.12, p = 0.9], but there was a significantly larger Ne for Unaware errors in the LoC group compared to the HiC group [t(15) = 2.26, p < 0.05].

The same mixed ANOVA for the amplitude of the Pe showed a significant effect of Response Type [F_{(2, 30)} = 23.91, p < 0.001, ε = 0.81] but no interaction [F_{(2, 30)} < 1, p = 0.98]. Follow-up contrasts on the main effect of Response Type revealed a difference between the Pe for Aware and Unaware errors [t(15) = 5.21, p < 0.001] and no difference between the Pe for Correct and Unaware errors [t(15) = 1.75, p = 0.1].

Discussion

Optimizing goal-directed behavior in imperfect conditions likely relies on both implicit and explicit error detection processes. In order to understand the unique contribution of each type of process, an essential initial step is to find physiological indices of aware and unaware error processing. Previous research linked the Ne component, occurring within the first 100 ms after the response, to processing of errors independent of consciousness, while awareness of errors influenced the amplitude of the Pe component at latencies longer than 250 ms post-response (Nieuwenhuis et al., 2001; Endrass et al., 2005, 2007; O'Connell et al., 2007, 2009; Pavone et al., 2009; Shalgi et al., 2009; Dhar et al., 2011; Hughes and Yeung, 2011). This was based on the finding that an Ne was similarly elicited whether subjects were aware of making an error or not, while a large Pe was restricted to errors of which the subjects were aware. Here, we replicated this result across subjects in a new visual choice-reaction time task. A significant Ne was elicited both by errors the subjects reported (Aware errors) and by errors the subjects did not report (Unaware errors). However, awareness is not necessarily a binary situation, and subjects may differ in their criterion for reporting awareness. That is, some subjects may not report awareness of an error even if they have some notion of making one, but are not absolutely sure. In this situation, some putative unaware errors would be contaminated with “partial awareness,” and the elicitation of Ne for these errors would not be evidence for processing of errors without awareness.

In an attempt to get a stricter selection of unaware errors and clarify this question, we used a wagering paradigm, asking subjects to indicate not only whether they had made an error or not, but also to bet money on their answer to this question. The pivotal working assumption was that if subjects report that they have not made an error (when one was made) yet they have some awareness that an error might have been committed, they would be reluctant to bet a large sum of money on their report. Indeed, subjects' debriefing, as well as RTs, suggest that they placed their bets based on their confidence. Based on this working hypothesis, the critical question was whether an Ne would be elicited when subjects were confident that they had not made an error, so much that they were willing to risk losing money. That is, in a condition mitigating the risk of partial awareness. The answer to this question was clear: when participants were highly confident that they had made an error (as indicated by a high bet), a large Ne was elicited, but when they were highly confident that they did not make an Error, the Ne was comparable to the CRN. That is, there was no indication of error processing without awareness in this case. On the other hand, subjects who were more hesitant about their accuracy-judgment responses had an equal Ne for Aware and Unaware errors.

Thus, under stricter selection of Unaware errors, our results support the premise that the Ne is related to subjective awareness of an error. Unlike previous studies that showed this effect (e.g., Scheffers and Coles, 2000; Hewig et al., 2011), our findings cannot be explained by the difficulty of performing the task, i.e., of making mistakes rather than slips (Reason, 1990). Mistakes are errors in which the participant cannot solve the task, for example, when representation of the stimulus is degraded, or when the task is too cognitively demanding, and therefore, a correct response representation cannot be accurately formed. Slips are errors in which the correct response representation can be (easily) evoked, yet an error is made due to premature responding or a momentary lack of attention to the task (Shalgi et al., 2007). Defined this way, if errors are made due to mistakes, no error processing can be expected a-priori, as the correct response is unknown. Thus, the lack of Ne for Unaware errors in Scheffers and Coles (2000; who used reduced stimulus quality) and in Hewig et al. (2011; who used a digit entering task, which relies on a participant's digit span) studies could be attributed to the possibility that errors were of the mistake kind. This possibility is reduced in our current task, as the stimuli were clearly presented and accuracy in the task was high (>85%). The current results, therefore, strengthens the notion that the Ne is related to subjective awareness of an error rather than to error processing per se.

The current results go beyond this conclusion to explain the source of difference between studies that show no awareness effect on the Ne (Nieuwenhuis et al., 2001; Endrass et al., 2005, 2007; O'Connell et al., 2007, 2009; Pavone et al., 2009; Shalgi et al., 2009; Dhar et al., 2011; Hughes and Yeung, 2011) and the studies that show such an effect (Scheffers and Coles, 2000; Praamstra et al., 2003; Pailing and Segalowitz, 2004a; Maier et al., 2008; Steinhauser and Yeung, 2010; Woodman, 2010; Hewig et al., 2011; Wessel et al., 2011). As described above, when we separated our subjects to high and low confidence groups, we obtained both results, depending on individual subjects' error reporting tendencies: across all bets (which parallels the standard procedure for Ne calculation), there was a strong Ne error awareness effect for high confidence subjects, but not for low confidence subjects. Error-reporting experiments likely include a mixture of subjects who are more confident about their performance in the task (i.e., have less instances of partial awareness) and subjects who are less confident. When a large enough group of subjects is unsure about their own accuracy, both the Aware Error and the Unaware Error bins are contaminated by trials that belong to the other bin. A reported error, labeled “Aware,” might be accompanied by a relatively low level of awareness, lowering the average Ne of the Aware errors bin, while an unreported error, labeled “Unaware,” may in fact be accompanied by some awareness and therefore add to the average amplitude of the Unaware errors bin. Consequently, the grand average results, which do not take into account these individual error-reporting differences may not show a significant Ne awareness effect. Indeed, as noted also by Wessel et al. (2011), several previous studies which did not report a statistically significant difference between the Ne to Aware and Unaware errors, did show a numerically smaller Ne in the latter case, as we also show here in the grand average across all subjects and bets. Moreover, we would assume that in difficult tasks, in which the confidence about response accuracy is lower for all subjects compared to their confidence in an easier task, we would be less likely to obtain an Ne awareness effect, as more trials are expected to be in the “gray zone” of awareness.

Error awareness has previously been ascribed mainly to the Pe. Indeed, the Pe, and not the Ne, has already been used to examine the incidence of error awareness in patient studies (Jonkman et al., 2007; Larson and Perlstein, 2009; O'Connell et al., 2009; Wiersema et al., 2009). Our study replicates the sensitivity of the Pe to awareness of errors: Aware errors elicit a higher sustained positivity around 300 ms post-response. In what we see as the critical condition, in which subjects were highly confident of their decision, the Pe for Unaware errors could not be differentiated from the response elicited in Correct trials, as was the case for the Ne. That is, both Ne and Pe were elicited only by Aware errors. The results from the less confident subjects are less clear, however. Like the case of the Ne, the Pe awareness effect was not significant for low bets. Yet unlike in the case of the Ne, the interaction between the high and low confidence groups and error awareness was not significant for the Pe. Also, although one could surmise that for Correct responses with low confidence some Pe would emerge (reflecting some level of false error awareness), no significant difference was found between correct answers followed by bet 1 and 3 in this group (Figure A2D). Since in all previous studies of error awareness, in which a mix of confident and less confident subjects must have been included, a Pe awareness effect was nevertheless found, we can conjecture that the Pe awareness effect reflects a more binary decision regarding the error, whereas the Ne is more sensitive to the level of confidence. We previously proposed that the Pe may not even be a truly response-locked component, but a manifestation of a delayed evaluation (reflected by a P3b) of the stimulus preceding the response (Shalgi et al., 2009). The continuation of that inquiry will be reported elsewhere.

The main shortcoming of our study, like the studies of Scheffers and Coles (2000) and Hewig et al. (2011), is the difficulty of directly comparing between bet 1 and bet 3 responses in the same subjects. Although all our subjects made use of the full range of the betting scale, only three subjects out of 22 (13.6%) made enough 1 and 3 bets for both Aware and Unaware errors. A much larger pool of subjects would have been required to find a substantial group with enough error trials for within-subject comparisons. This obstacle, may suggest in fact that some individuals tend to either be mostly confident about their responses or mostly unsure of them. One might argue that the confidence report, as reflected by the bets, is not really related to differences in the level of error awareness between the subjects, but rather reflects subjects' tendency to be more decisive or daring when making their bets, or their perceived demand characteristics. However, we argue that if this were the case, we would not have found the dissociation we report here at the time window of the Ne (i.e., when the response was made), between high and low confident subjects, and between high and low bets. It would be interesting to study what other traits these individual differences correlate with, and whether we could use these traits to create more homogenous groups for the study of error processing with or without awareness. Recent studies have pointed to the effect of individual differences in demographic characteristics (such as age and sex), personality traits (such as absentmindedness, impulsivity, affective style, anxiety), affective and motivational variables and behavioral performance (e.g., response speed) on error processing (e.g., Hester et al., 2004; Pailing and Segalowitz, 2004b; Boksem et al., 2006; Chang et al., 2010). Attention to individual differences may be critical for advancing our understanding of the role of the ERP components in error processing.

Can error monitoring occur without conscious recognition? Some studies show evidence for error processing without awareness. van Gaal et al. (2010) showed that behavioral conflict adaptation can occur when the response conflict occurs without awareness. Cohen et al. (2009) recently showed using EEG that error monitoring can occur when subjects are not only unaware of their errors, but also of the actual stimuli; when subjects performed a Go/No-Go task, errors following a masked No-Go signal elicited directional synchrony between the ACC and the occipital cortex, akin to the synchrony found when the No-Go signal was consciously perceived. Similarly, Ursu et al. (2009) showed, using fMRI, that the ACC, considered to be the locus of error processing and the origin of the Ne, was active when subjects were presented with a response conflict of which they were seemingly unaware (because a target's location violates an implicitly learned sequence), or when they made errors of which they were unaware. In contrast, other studies suggest that awareness is necessary for conflict adaptation (Kunde, 2003) or for the activation of the ACC (e.g., Dehaene et al., 2003). Whereas unconscious error processing may take place, the question is at what level and what are the prerequisites for error awareness to emerge. The evidence from our study, which controlled error awareness more closely, does not support error processing without awareness at the stage immediately after an error was made, as indexed by the Ne. We suggest that future studies of error awareness must address the methods used for awareness testing.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

We wish to thank Shany Grossman, Assaf Breska, and Tali Shrem for their help in the production of this manuscript. Supported by the National Institute of Psychobiology in Israel founded by the Charles E. Smith family.

References

Boksem, M. A., Tops, M., Wester, A. E., Meijman, T. F., and Lorist, M. M. (2006). Error-related ERP components and individual differences in punishment and reward sensitivity. Brain Res. 1101, 92–101.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Brazdil, M., Roman, R., Falkenstein, M., Daniel, P., Jurak, P., and Rektor, I. (2002). Error processing – evidence from intracerebral ERP recordings. Exp. Brain Res. 146, 460–466.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Chang, W. P., Davies, P. L., and Gavin, W. J. (2010). Individual differences in error monitoring in healthy adults: psychological symptoms and antisocial personality characteristics. Eur. J. Neurosci. 32, 1388–1396.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Cohen, M. X., van Gaal, S., Ridderinkhof, K. R., and Lamme, V. A. (2009). Unconscious errors enhance prefrontal-occipital oscillatory synchrony. Front. Hum. Neurosci. 3:54. doi: 10.3389/neuro.09.054.2009

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Coles, M. G., Scheffers, M. K., and Holroyd, C. B. (2001). Why is there an ERN/Ne on correct trials? Response representations, stimulus-related components, and the theory of error-processing. Biol. Psychol. 56, 173–189.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Debener, S., Ullsperger, M., Siegel, M., Fiehler, K., von Cramon, D. Y., and Engel, A. K. (2005). Trial-by-trial coupling of concurrent electroencephalogram and functional magnetic resonance imaging identifies the dynamics of performance monitoring. J. Neurosci. 25, 11730–11737.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Dehaene, S., Artiges, E., Naccache, L., Martelli, C., Viard, A., Schurhoff, F., Recasens, C., Martinot, M. L., Leboyer, M., and Martinot, J. L. (2003). Conscious and subliminal conflicts in normal subjects and patients with schizophrenia: the role of the anterior cingulate. Proc. Natl. Acad. Sci. U.S.A. 100, 13722–13727.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Dehaene, S., Posner, M. I., and Tucker, D. M. (1994). Localization of a neural system for error-detection and compensation. Psychol. Sci. 5, 303–305.

Dhar, M., Wiersema, J. R., and Pourtois, G. (2011). Cascade of neural events leading from error commission to subsequent awareness revealed using EEG source imaging. PLoS One 6:e19578. doi: 10.1371/journal.pone.0019578

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Endrass, T., Franke, C., and Kathmann, N. (2005). Error awareness in a saccade countermanding task. J. Psychophysiol. 19, 275–280.

Endrass, T., Reuter, B., and Kathmann, N. (2007). ERP correlates of conscious error recognition: aware and unaware errors in an antisaccade task. Eur. J. Neurosci. 26, 1714–1720.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Falkenstein, M., Hohnsbein, J., Hoormann, J., and Blanke, L. (1991). Effects of crossmodal divided attention on late ERP components. II. Error processing in choice reaction tasks. Electroencephalogr. Clin. Neurophysiol. 78, 447–455.

Pubmed Abstract | Pubmed Full Text

Ford, J. M. (1999). Schizophrenia: the broken P300 and beyond. Psychophysiology 36, 667–682.

Pubmed Abstract | Pubmed Full Text

Gehring, W. J., Gross, B., Coles, M., Meyer, D., and Donchin, E. (1993). A neural system for error detection and compensation. Psychol. Sci. 4, 385–390.

Hester, R., Fassbender, C., and Garavan, H. (2004). Individual differences in error processing: a review and reanalysis of three event-related fMRI studies using the GO/NOGO task. Cereb. Cortex 14, 986–994.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Hester, R., Foxe, J. J., Molholm, S., Shpaner, M., and Garavan, H. (2005). Neural mechanisms involved in error processing: a comparison of errors made with and without awareness. Neuroimage 27, 602–608.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Hewig, J., Coles, M. G., Trippe, R. H., Hecht, H., and Miltner, W. H. (2011). Dissociation of Pe and ERN/Ne in the conscious recognition of an error. Psychophysiology 48, 1390–1396.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Hughes, G., and Yeung, N. (2011). Dissociable correlates of response conflict and error awareness in error-related brain activity. Neuropsychologia 49, 405–415.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Jonkman, L. M., van Melis, J. J., Kemner, C., and Markus, C. R. (2007). Methylphenidate improves deficient error evaluation in children with ADHD: an event-related brain potential study. Biol. Psychol. 76, 217–229.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Jung, T. P., Makeig, S., Humphries, C., Lee, T. W., Mckeown, M. J., Iragui, V., and Sejnowski, T. J. (2000). Removing electroencephalographic artifacts by blind source separation. Psychophysiology 37, 163–178.

Pubmed Abstract | Pubmed Full Text

Klein, T. A., Endrass, T., Kathmann, N., Neumann, J., von Cramon, D. Y., and Ullsperger, M. (2007). Neural correlates of error awareness. Neuroimage 34, 1774–1781.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Koch, C., and Preuschoff, K. (2007). Betting the house on consciousness. Nat. Neurosci. 10, 140–141.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Kunde, W. (2003). Sequential modulations of stimulus-response correspondence effects depend on awareness of response conflict. Psychon. Bull. Rev. 10, 198–205.

Pubmed Abstract | Pubmed Full Text

Larson, M. J., and Perlstein, W. M. (2009). Awareness of deficits and error processing after traumatic brain injury. Neuroreport 20, 1486–1490.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Maier, M., Steinhauser, M., and Hubner, R. (2008). Is the error-related negativity amplitude related to error detectability? Evidence from effects of different error types. J. Cogn. Neurosci. 20, 2263–2273.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Nieuwenhuis, S., Ridderinkhof, K. R., Blom, J., Band, G. P., and Kok, A. (2001). Error-related brain potentials are differentially related to awareness of response errors: evidence from an antisaccade task. Psychophysiology 38, 752–760.

Pubmed Abstract | Pubmed Full Text

O'Connell, R. G., Bellgrove, M. A., Dockree, P. M., Lau, A., Hester, R., Garavan, H., Fitzgerald, M., Foxe, J. J., and Robertson, I. H. (2009). The neural correlates of deficient error awareness in attention-deficit hyperactivity disorder (ADHD). Neuropsychologia 47, 1149–1159.

Pubmed Abstract | Pubmed Full Text

O'Connell, R. G., Dockree, P. M., Bellgrove, M. A., Kelly, S. P., Hester, R., Garavan, H., Robertson, I. H., and Foxe, J. J. (2007). The role of cingulate cortex in the detection of errors with and without awareness: a high-density electrical mapping study. Eur. J. Neurosci. 25, 2571–2579.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Pailing, P. E., and Segalowitz, S. J. (2004a). The effects of uncertainty in error monitoring on associated ERPs. Brain Cogn. 56, 215–233.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Pailing, P. E., and Segalowitz, S. J. (2004b). The error-related negativity as a state and trait measure: motivation, personality, and ERPs in response to errors. Psychophysiology 41, 84–95.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Pavone, E. F., Marzi, C. A., and Girelli, M. (2009). Does subliminal visual perception have an error-monitoring system? Eur. J. Neurosci. 30, 1424–1431.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Perrin, F. (1990). Correction. Electroencephalogr. Clin. Neurophysiol. 76, 565.

Perrin, F., Pernier, J., Bertrand, O., and Echallier, J. F. (1989). Spherical splines for scalp potential and current density mapping. Electroencephalogr. Clin. Neurophysiol. 72, 184–187.

Pubmed Abstract | Pubmed Full Text

Persaud, N., McLeod, P., and Cowey, A. (2007). Post-decision wagering objectively measures awareness. Nat. Neurosci. 10, 257–261.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Picton, T. W., Bentin, S., Berg, P., Donchin, E., Hillyard, S. A., Johnson, R. Jr., Miller, G. A., Ritter, W., Ruchkin, D. S., Rugg, M. D., and Taylor, M. J. (2000). Guidelines for using human event-related potentials to study cognition: recording standards and publication criteria. Psychophysiology 37, 127–152.

Pubmed Abstract | Pubmed Full Text

Praamstra, P., Turgeon, M., Hesse, C. W., Wing, A. M., and Perryer, L. (2003). Neurophysiological correlates of error correction in sensorimotor-synchronization. Neuroimage 20, 1283–1297.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Reason, J. T. (1990). Human Error. New York, NY: Cambridge University Press.

Scheffers, M. K., and Coles, M. G. (2000). Performance monitoring in a confusing world: error-related brain activity, judgments of response accuracy, and types of errors. J. Exp. Psychol. Hum. Percept. Perform. 26, 141–151.

Pubmed Abstract | Pubmed Full Text

Shalgi, S., Barkan, I., and Deouell, L. Y. (2009). On the positive side of error processing: error-awareness positivity revisited. Eur. J. Neurosci. 29, 1522–1532.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Shalgi, S., and Deouell, L. Y. (2010). “Is there a hemispatial bias in detecting errors and in error awareness?”, in The Israel Society for Neuroscience 19th Annual Meeting Eilat: Journal of Molecular Neuroscience, vol. 45. (Eilat, Israel: Humana Press), 1–137.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Shalgi, S., O'Connell, R. G., Deouell, L. Y., and Robertson, I. H. (2007). Absent minded but accurate: delaying responses increases accuracy but decreases error awareness. Exp. Brain Res. 182, 119–124.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Simons, R. F. (2009). The way of our errors: theme and variations. Psychophysiology 47, 1–14.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Steinhauser, M., and Yeung, N. (2010). Decision processes in human performance monitoring. J. Neurosci. 30, 15643–15653.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Stemmer, B., Segalowitz, S. J., Witzke, W., and Schonle, P. W. (2004). Error detection in patients with lesions to the medial prefrontal cortex: an ERP study. Neuropsychologia 42, 118–130.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Ullsperger, M., Harsay, H. A., Wessel, J. R., and Ridderinkhof, K. R. (2010). Conscious perception of errors and its relation to the anterior insula. Brain Struct. Funct. 214, 629–643.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Ursu, S., Clark, K. A., Aizenstein, H. J., Stenger, V. A., and Carter, C. S. (2009). Conflict-related activity in the caudal anterior cingulate cortex in the absence of awareness. Biol. Psychol. 80, 279–286.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

van Gaal, S., Lamme, V. A., and Ridderinkhof, K. R. (2010). Unconsciously triggered conflict adaptation. PLoS One 5:e11508. doi: 10.1371/journal.pone.0011508

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Vidal, F., Hasbroucq, T., Grapperon, J., and Bonnet, M. (2000). Is the ‘error negativity’ specific to errors? Biol. Psychol. 51, 109–128.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Wessel, J. R., Danielmeier, C., and Ullsperger, M. (2011). Error awareness revisited: accumulation of multimodal evidence from central and autonomic nervous systems. J. Cogn. Neurosci. 23, 3021–3036.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Wiersema, J. R., van der Meere, J. J., and Roeyers, H. (2009). ERP correlates of error monitoring in adult ADHD. J. Neural. Transm. 116, 371–379.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Woodman, G. F. (2010). Masked targets trigger event-related potentials indexing shifts of attention but not error detection. Psychophysiology 47, 410–414.

Pubmed Abstract | Pubmed Full Text | CrossRef Full Text

Appendix

Number of EEG Segments in the High and Low Confidence Groups

The High Confidence (HiC) group was comprised of the nine subjects who had more than 20 bet 3 segments for both Aware and Unaware Errors and less than 20 bet 1 segments for both error types. The Low Confidence (LoC) Group was comprised of the eight subjects who had more than 20 bet 1 segments for both Aware and Unaware errors. Table A1 summarizes the number of trials in each group.

TABLE A1

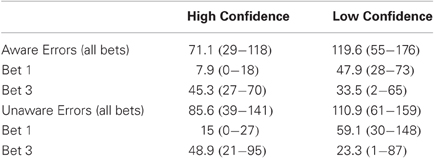
Table A1. Mean number and range of error trials of subjects included in the High and Low Confidence groups.

Analysis of the Response-Locked Waveforms After Subtracting the Stimulus-Locked Waveform

In the response-locked waveform, the peak-to-trough CRN appears to be riding on a slow positive wave starting well before the response, which creates an impression that the onset-to-peak CRN is around the same size or even larger than the Ne (Figure A1A). We hypothesized that the slow positivity is a residual of the jittered stimulus-locked target P3 (Figure A1C). To investigate this hypothesis, we calculated for each subject the mean ERP of the stimulus-locked response, separately for each response type. Then, for each single response-locked segment we subtracted the relevant condition's stimulus-locked ERP, after aligning the two waveforms according to that trial's RT. Trials with RTs longer than 1000 ms could not be included in this procedure as the stimulus-locked grand average was not long enough to be aligned with the response-locked ERP, resulting in the elimination of between 2 and 31 error trials per subject. The grand average result is presented in Figure A1B, which shows the elimination of the slow positivity, and confirms that the CRN is in fact smaller than the Ne. Statistical analysis of the resulting Ne amplitudes replicates the finding from the uncorrected waveforms reported in the Results section of the paper, namely that the Ne for errors is significantly different from the CRN but not different between Aware and Unaware Errors—there was a significant effect in a one-way repeated measures ANOVA for Response Type [F_{(2, 42)} = 8.17, p < 0.005, ε = 0.76] which stemmed from a difference between errors and correct responses [t(21) = 5.27, p < 0.001], while there was no difference between the Ne amplitude for Aware and Unaware Errors [t(21) = 1.47, p = 0.16].

FIGURE A1

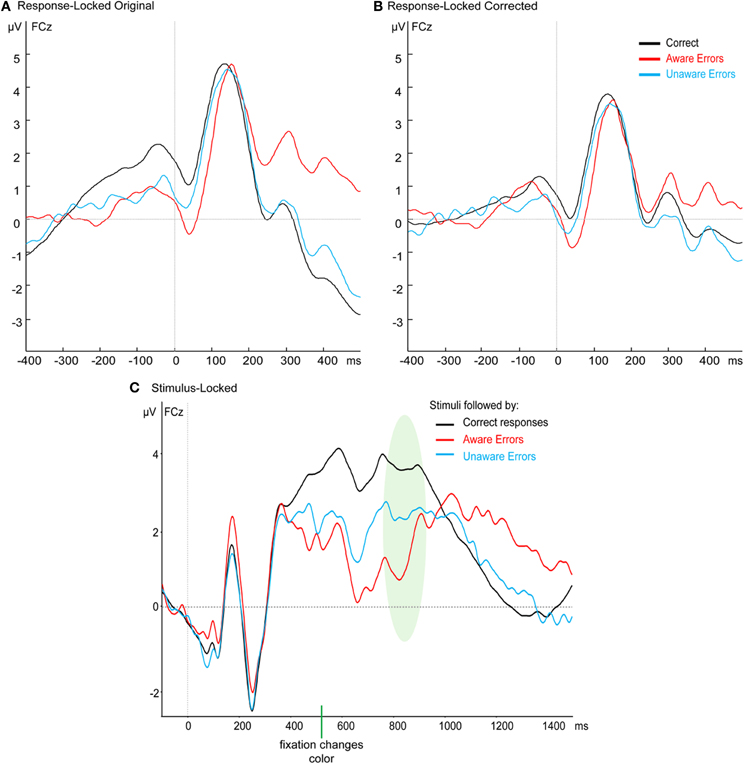
Figure A1. Grand average waveforms at FCz of (A) the original response-locked results, (B) the response-locked results after the subtraction of the stimulus-locked ERPs, and (C) the stimulus-locked waveforms. The shaded area in the stimulus-locked waveforms represents the average reaction time ± one standard deviation.

Analysis of the Correct Response-Locked Waveforms of the Low Confidence Group After Subtracting the Stimulus-Locked Waveform

In the Low Confidence group, there were enough Correct responses with bet 1 and bet 3 for comparison within subjects between bets. However, the response-locked grand average of the Correct response on which subjects bet 3 seems to be riding on a positive pre-response wave (see Figures A2A and A2C). As in Appendix section B, we subtracted the mean of the stimulus-locked response (separately for bet 1 and bet 3) of each subject from each response-locked trial according to its RT. Trials with RTs longer than 1000 ms could not be included in this analysis, resulting in the elimination of between 6 and 128 correct trials per subject (this elimination did not significantly reduce the number of single trials for each subject and bet—range after elimination: 31–960). The grand average result for electrodes FCz and Pz is shown in Figures A2B and A2D. Statistical analysis of the resulting CRN and Pe amplitudes shows that the CRN for bet 3 was significantly smaller than the CRN for bet 1 in these subjects [t(7) = 2.38, p < 0.05] but there was no difference in Pe [t(7) = 1.01, p = 0.35].

FIGURE A2

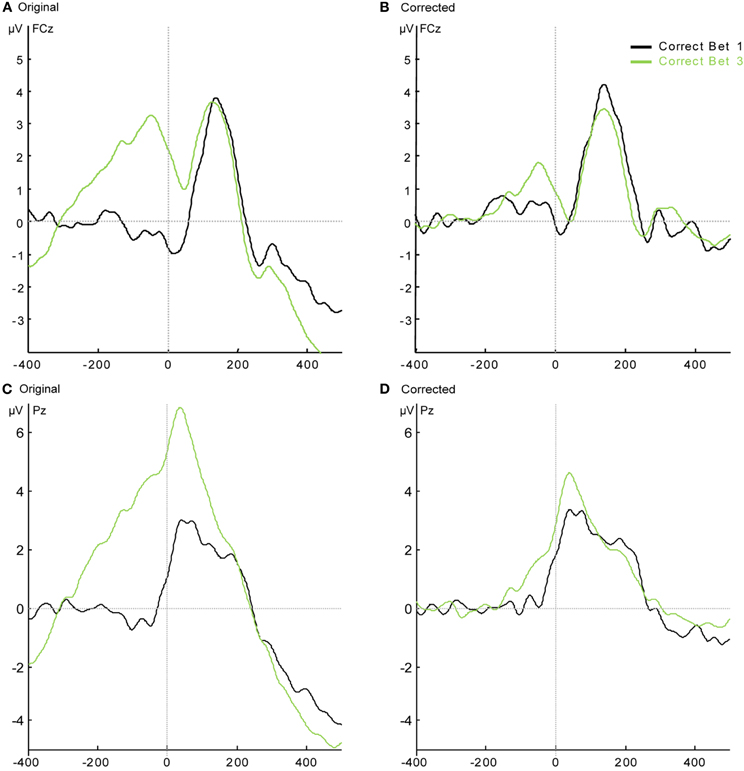
Figure A2. Response-locked grand average waveforms of correct responses of the Low Confidence group. (A) The original results at FCz; (B) the results after the subtraction of the stimulus-locked ERPs at FCz. (C) The original results at Pz; (D) the results after the subtraction of the stimulus-locked ERPs at Pz.