
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. For. Glob. Change, 16 December 2021
Sec. Forest Disturbance
Volume 4 - 2021 | https://doi.org/10.3389/ffgc.2021.737342
This article is part of the Research TopicApplications of Tree Rings in Altered ForestsView all 6 articles
Many studies quantify short-term drought impact on tree growth relative to pre-drought growth averages. However, fewer studies examine the extent to which droughts of differing severity differentially impact tree growth or shape stand dynamics. Focusing on three droughts in high and low density stands of Pinus sylvestris in Scotland, we calculated pre-drought growth averages using climatically standardized antecedent growth years to assess tree level drought and post-drought growth performance as percentage growth change (PGC). We then used mixed-effects models to understand how droughts of differing severity impact tree growth and calculated indices of growth dominance (Gd), size inequality (Si), and size asymmetry (Sa) to detect changes in stand structure. Mixed-effects model results indicate that the magnitude and duration of the growth reduction during and following the more extreme drought was significantly larger compared to less severe droughts, for which we found limited evidence of drought impact. While no changes in Si or Sa were noted following any drought, we found evidence of a difference in Gd after the most extreme drought in both stand densities indicative of a threshold response, with smaller trees contributing proportionally more to stand growth relative to their size. Under less severe droughts, inter-tree variability may have partially buffered against stand-level growth change, however, a small increase in drought severity was associated with a significant reduction in average tree growth, an increase in the number of trees growing at >2SD below pre-drought levels and a shift in Gd toward smaller trees, indicating that a drought severity threshold in P. sylvestris may have been exceeded.
Climate change is expected to increase the frequency, intensity, and duration of extreme drought events globally (Shukla et al., 2019). This predicted increase has resulted in growing concerns regarding the impacts of a hotter climate upon forest ecosystems (Allen et al., 2010, 2015; Anderegg et al., 2013) including negative impacts on tree growth (Anderegg et al., 2015b), shifts in community composition (Suarez and Kitzberger, 2008), and the potential for large scale tree mortality (van Mantgem et al., 2009; Anderegg et al., 2019). As a result, recent work assessing forest vulnerability to drought and its association with particular functional traits (Greenwood et al., 2017; Anderegg et al., 2018; Li et al., 2020) and previous drought performance (Anderegg et al., 2020; DeSoto et al., 2020) has greatly improved our understanding of forest drought susceptibility.
Despite progress in identifying attributes that promote forest resistance and resilience to drought, the impact of drought on stand attributes has been less well documented. Recent evidence indicates that droughts can induce shifts in competitive dominance between species (Cavin et al., 2013), cause persistent shifts in species composition (Suarez and Kitzberger, 2008; Martínez-Vilalta and Lloret, 2016) and will likely lead to changes in forest dynamics under global change (McDowell et al., 2020). Similarly, increases in environmental stressors such as drought may potentially reshape species interactions away from competitive, toward more facilitative processes (He et al., 2013). These changes, coupled with the existence of non-linear threshold-type responses to increasing drought severity (Cavin et al., 2013; Stuart-Haëntjens et al., 2015; Bartlett et al., 2016; Adams et al., 2017) means that understanding how and when drought alters forest structure and function (Haber et al., 2020) is increasingly important. Equally, if we are to implement successful forest management to promote stand-level drought resilience (Sohn et al., 2016), it is essential that we understand the interplay between increasing drought severity, patterns in forest response and the location of thresholds across a range of species, environments, and scales (Choat et al., 2012; Anderegg et al., 2015a; Huang et al., 2015).
Assessments of short-term drought impacts indicate larger trees are commonly more susceptible to drought-induced growth decline (Martínez-Vilalta et al., 2012; Bennett et al., 2015; Ding et al., 2017) including in P. sylvestris (Merlin et al., 2015). However, this pattern is not universal, with basal area having been found to be positively associated with the drought resistance in Norway spruce (Picea abies) and silver fir (Abie alba) (Zang et al., 2014), while the importance of tree sizes on drought recovery can vary depending on the stage of recovery being considered (Ovenden et al., 2021). Similarly, exposure to historic drought may increase future tree vulnerability to extreme drought (Bose et al., 2020), particularly in Pinaceae (Anderegg et al., 2020) while lower historic drought resilience can increase future mortality risk (DeSoto et al., 2020). If larger, older trees do suffer more under drought, this may interact with successional processes, gap dynamics and growth release (Rubio-Cuadrado et al., 2018) to shift forests toward younger, smaller stands (McDowell et al., 2020) by favoring particular tree attributes (small, slow growing, and younger trees) that confer drought resilience.
Characterizing tree response to drought requires an understanding of the influence of pre-drought growth in priming drought year performance (Hilker et al., 2016; Bose et al., 2020; Gessler et al., 2020). While many indices of resistance and resilience exist in the literature (Ingrisch and Bahn, 2018), those introduced by Lloret et al. (2011), where pre-drought and post-drought growth averages are calculated over a pre-defined period (Gazol et al., 2018; Granda et al., 2018), have been widely applied in the forest sciences. While this approach has been instrumental in improving our understanding of the radial growth of trees both during and following drought, the a priori assumption that average growth, calculated from the years immediately preceding a drought accurately represents a “normal” growth rate to which a tree should be expected to return risks omitting some of the climatic context within which a drought is occurring (Anderegg et al., 2015b; Kannenberg et al., 2020; Ovenden et al., 2021).
The standardized precipitation evapotranspiration index (SPEI) (Vicente-Serrano et al., 2010) is widely used to identify drought events in the climate record (Huang et al., 2015; Gazol et al., 2018; DeSoto et al., 2020). Here, we use the SPEI to define a climatically constrained pre-drought growth average. We then use this growth average to calculate the annual percentage growth change (PGC) during and after three droughts of differing severity for individual Pinus sylvestris trees growing at two different stand densities and quantify how many trees show a significant growth impact following each drought. Indices of growth dominance (Gd), size inequality (Si), and size asymmetry (Sa) are then calculated annually to characterize stand behavior before, during and after drought and combined with change point analysis to identify any drought-associated shifts in stand dynamics. This assessment enables us to address the following questions: (a) How are increases in drought severity associated with differences in growth response at the tree and stand levels? (b) Is drought associated with detectable and lasting changes in Gd and stand dynamics?
The present study was conducted with samples taken from a monospecific spacing experiment of Pinus sylvestris L., established in 1935 and situated in the north-east of Scotland (57° 36′ 23″ N, 4° 16′ 50″ W) at 170m a.s.l and two spacing (density) treatments were selected for use: high density (ρH) and low density (ρL).
Sample plots were randomly established so that ten 0.02 ha plots were present in both ρH and ρL. Measurements of tree diameter at breast height (DBH – 1.3 m) were collected for all trees within the sample plots. Sample trees were then selected from within these sample plots using the diameter distribution of measured trees to represent three dominance classes (Dominant, Co-dominant, and Sub-dominant). Dominant trees were selected as those closest to, but above the upper quartile diameter value, co-dominant trees were selected as those closest to, but above the median diameter value and sub-dominant trees were those closest to, but above the lower quartile diameter value across all trees in the 10 sample plots in both ρH and ρL where present. This approach meant 14 dominant, 14 co-dominant and 6 sub-dominant trees in each treatment were felled in the winters of 2002 and 2003, resulting in 34 sample trees from both ρH and ρL. Cross sectional discs were taken from the base of each tree at 0.3m ± 30cm and scanned, resulting in 29 (ρH) and 27 (ρL) usable discs images for analysis (Table 1). Mortality assessments had been conducted in these plots approximately every 5 years from 1955 to 1990 (Supplementary Table 1).
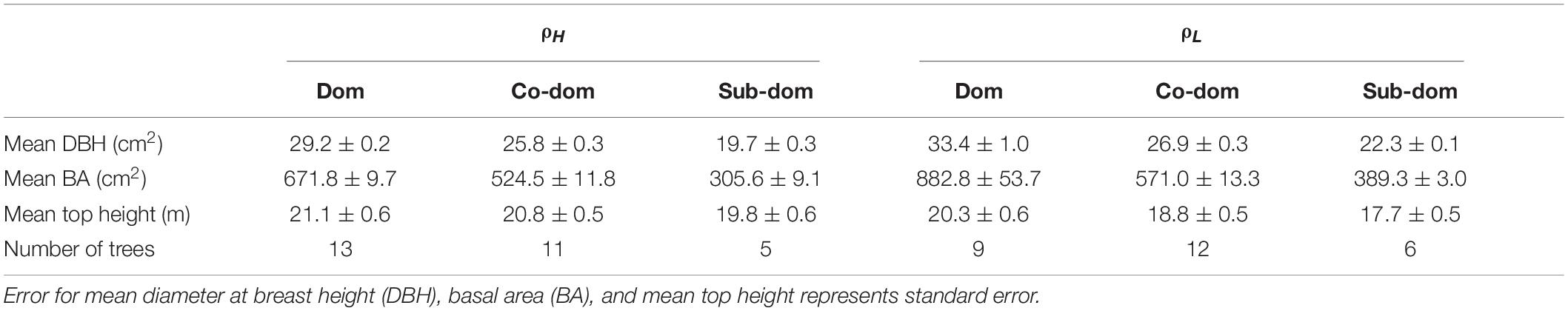
Table 1. Stand characteristics at the time of sampling for felled Dominant (Dom), Co-dominant (Co-dom), and Sub-dominant (Sub-dom) P. sylvestris trees in both the high density (ρH) and low density (ρL) treatments.
Individual tree annual basal area increments (BAI) were calculated from raw ring width data following Eq.1 where R is the radius of the tree in years t and t-1 using the dplR package (Bunn et al., 2019) in R version 3.6.1 (R Core Team, 2019). See Ovenden et al. (2021) for further details of the site and dendroecological methods used to process the collected samples.
To identify notable drought years, we used interpolated climate data at 1 km resolution, obtained from the climate hydrology and ecology research support system (CHESS) meteorology dataset for Great Britain (Robinson et al., 2017) for the study period (1961–2002) to calculate monthly values for both the standardized precipitation evapotranspiration index (SPEI) (Vicente-Serrano et al., 2010) and the climatic water deficit (CWD). SPEI was calculated for August using the five previous months data (i.e., using a 6 month integration period of March–August) (SPEIAug6) across the study period using the SPEI package in R (Beguería et al., 2014). Increasingly negative SPEI values indicate increasingly severe drought conditions. Huang et al. (2015) identified an SPEI threshold of – 1.64 in other species of pine (Pinus edulis and Pinus ponderosa), below which drought was linked to significant growth decline. As such, –1.64 was the thresholds adopted here for defining a drought event using the SPEI (Figure 1).
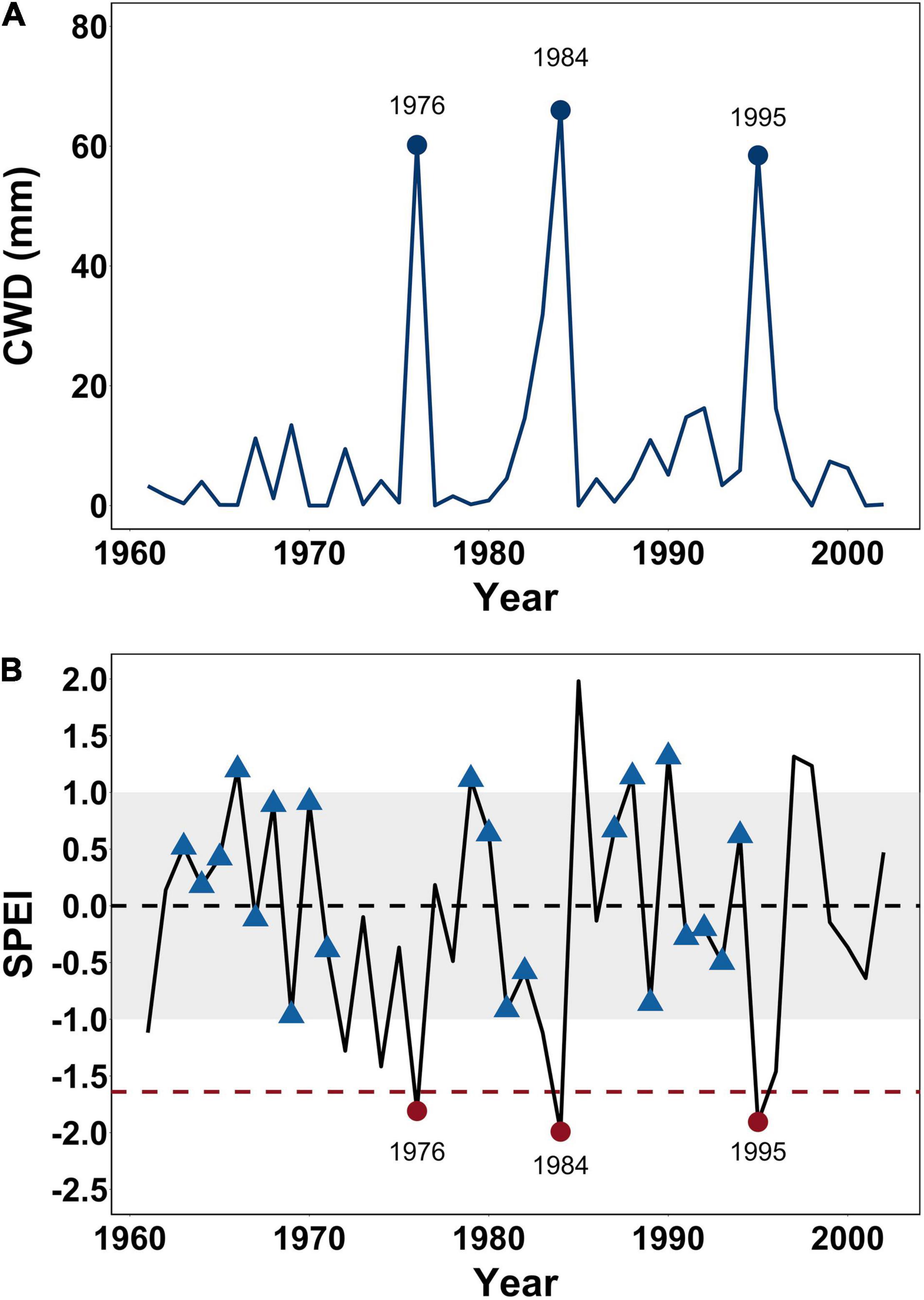
Figure 1. (A) Annual climatic water defect (CWD in mm) summed over 6 months (March – August) for each year during the study period (1961–2002). (B) Annual Standardized Precipitation Evapotranspiration Index (SPEI) values calculated over the same study period (1961–2002). The gray band indicates typical climate years (<1 and >–1). The horizontal red dashed line at an SPEI of –1.64 reflects the threshold for drought linked growth decline in pine species proposed by Huang et al. (2015). The three droughts (1976, 1984, and 1995) considered in this study are indicated by red filled circles and annotated in both (A,B) while blue triangles indicate the years used to calculate the pre-drought growth averages.
Climatic water deficit was calculated for the site at monthly time steps between 1961 and 2002 following Lutz et al. (2010) using R code developed by Redmond (2019). The approach used by Lutz et al. (2010) to calculating CWD applies a Thornthwaite-type water-balance model (Thornthwaite, 1948) which is considered most appropriate when climatic data are limited (e.g., when data on historic wind speed, humidity, and net radiation are absent). As such, CWD was calculated here using slope (degrees), latitude (decimal degrees), folded aspect (degrees) (McCune and Keon, 2002), monthly total precipitation (mm), mean monthly temperature (°C), and the soil available water capacity (mm) in the top 200 cm of the soil. Soil available water capacity for the study site of 143.63 mm was obtained from the James Hutton Institute’s Available Water Capacity (AWC) dataset (Gagkas et al., 2019). Monthly CWD values between March and August were then summed annually to give a single annual CWD value for each year during the study period (Figure 1).
Only years that showed a clear drought signal in the calculations of both SPEI and CWD were selected for analysis, resulting in the identification of three drought events in 1976 (SPEIAug6 of – 1.8, CWD of 60.2 mm), 1984 (SPEIAug6 of –2.0, CWD of 66.0 mm) and 1995 (SPEIAug6 of – 1.9, CWD of 58.5 mm), meaning that both SPEI and CWD indicate that 1984 was the most severe drought in the study period (Figure 1).
We applied a set of exclusion criteria to SPEIAug6 values for the study site across all years preceding each of the three drought events to remove years that indicated unusually dry conditions or potential drought recovery years. This was necessary to ensure that only BAI values from non-drought years or drought recovery years were used to calculate pre-drought growth averages, against which drought responses would be subsequently compared. We then used the corresponding BAI values for the retained SPEI- constrained years to calculate a cumulative moving average (CMA) BAI value at an annual time step (BAIcon). In this way, BAIcon changes over time as additional SPEI-constrained growth years are included in the average. Initially, we identified all years during the study period (1961–2002) where the SPEIAug6 values were between –1 and 1 (Figure 1). This threshold is commonly used in drought studies to indicate non-drought conditions (Hoffmann et al., 2018; Vanhellemont et al., 2018; Slette et al., 2019; Bose et al., 2020). In order to avoid including BAI values that might represent growth during a recovery year [i.e., the year(s) after SPEI values < –1] in the calculation of an SPEI-constrained growth average, we applied thresholds for data exclusion by omitting 1 year of BAI after an observed SPEI value of ≤–1 but >–1.5, 2 years after a SPEI value ≤ –1.5 but > –2.0 and 3 years if the SPEI value was ≤–2, reflecting commonly used thresholds of drought severity (Hoffmann et al., 2018; Vanhellemont et al., 2018). This approach was designed to be conservative (by excluding growth years that may themselves be a recovery year) and reflect the fact that higher stress levels may be more likely to result in physical damage such as hydraulic failure (Adams et al., 2017) and potentially slower recovery (Gessler et al., 2020). Thus, BAIcon was calculated annually from the CMA of all prior years that had not been excluded using one of the above criteria. In this way, SPEI-constrained growth years were defined as having an SPEIAug6 value of > –1, with CMA BAI calculations also omitting any BAI values where growth was potentially recovering from a previous dry year. This approach meant that for each tree separately, the BAI1976con for the 1976 drought was calculated using nine prior annual growth records, the BAI1984con for the 1984 drought was calculated using 13 prior growth records and the BAI1995con for the 1995 drought was calculated using 21 prior growth records from the study period (Figure 1).
Resistance (Rt) is a measure of the immediate impact of drought on tree radial growth relative to a pre-drought growth average calculated over a pre-defined period, often between 2 and 5 years before drought (Lloret et al., 2011). As a result, Rt is calculated following Eq. 2:
where, PreDr is average pre-drought growth and Dr is growth during the drought year, providing a measure of drought impact as the ratio of drought year growth performance relative to a pre-drought growth average. We made a simple modification to Eq. 2 which allowed us to express Rt as PGC following Eq. 3:
where, the pre-drought growth average in the Rt calculation is replaced with individual tree BAIcon values (BAI1976con, BAI1984con, and BAI1995con) and Dr is replaced with BAIt which represents the observed BAI in any year (during or after drought). The use of Eq. 3 over Eq. 2 enabled us to assess BAIt annually against an SPEI-constrained pre-drought growth average for individual drought episodes. The use of Eq. 3 also allowed an assessment of recovery, with an annual PGC value of 0% indicating growth rates have returned to BAI1976con, BAI1984con, or BAI1995con levels. We calculated PGC for the three drought years and for the 4 years following each drought but could not calculate PGC for the years immediately preceding each drought event due to the inclusion of some of these years in the calculations of the pre-drought growth averages (BAI1976con, BAI1984con, and BAI1995con) (Figure 1). We also calculated PGC annually over the same period for all three drought events using ring-width data that had been detrended using a cubic smoothing spline with a 30-year cut-off to ensure our results derived from BAI data were robust.
We fit a mixed-effects model using the nlme package in R (Pinheiro et al., 2020) following Eq. 4 to investigate at an annual resolution whether P. sylvestris trees show differences in growth (indexed by BAI) after droughts of differing severity, at different stand densities and to ascertain how long any differences might persist post-drought.
In Eq. 4, BAIij is the jth measure (representing measurement at each j year) of BAI for the ith tree, X is an n x p matrix of fixed effect variables, where n = the number of observations and p = the fixed effect variables, including drought event, stand density and timepoint (year), with an interaction between drought event and timepoint (year), β is a p x 1 column vector of regression estimates, b0i represents the random effect of tree, where b0i ∼ N(0, σ20) and ε represents the residual error term, where εij ∼ N(0, σ2) (where the error terms are assumed to be normally distributed with a mean of 0 and standard deviation σ2).
Basal area increments values were square root transformed prior to analysis to improve model fit. In order to compare the three drought events, the year of the drought was assigned timepoint “0” (1976, 1984, or 1995). The next four recovery years were then described as years 1–4. Timepoint (year) (zero to four, where zero is the drought year and one to four are the post-drought years) was fitted using third order orthogonal polynomials to accommodate for non-linearities in BAI over time, with the optimal degree of polynomials selected by minimizing AIC values. Tree ID was fitted as a random effect and interaction terms between drought event and year were included. To correct for temporal autocorrelation, the correlation structure was modeled using a corARMA correlation structure of p = 2, q = 0 and year as a time covariate, which also served to detrend the BAI data.
We subsequently compared the association between BAI and the three drought events over time using estimate marginal means calculated using the “emtrends” function in the “emmeans” R package and used the Tukey HSD method to correct for multiple comparisons (Lenth, 2020). Estimated marginal means are the mean response for each factor, averaged across the other variables in the model. When the adjusted marginal means were extracted from the model, the estimates were back-transformed to the original scale and these are the values presented in Figure 2. Adjusted marginal means and unadjusted 95% confidence intervals were also obtained using “emmeans” for each year and drought event. All calculations were performed using R version 3.6.1 (R Core Team 2019).
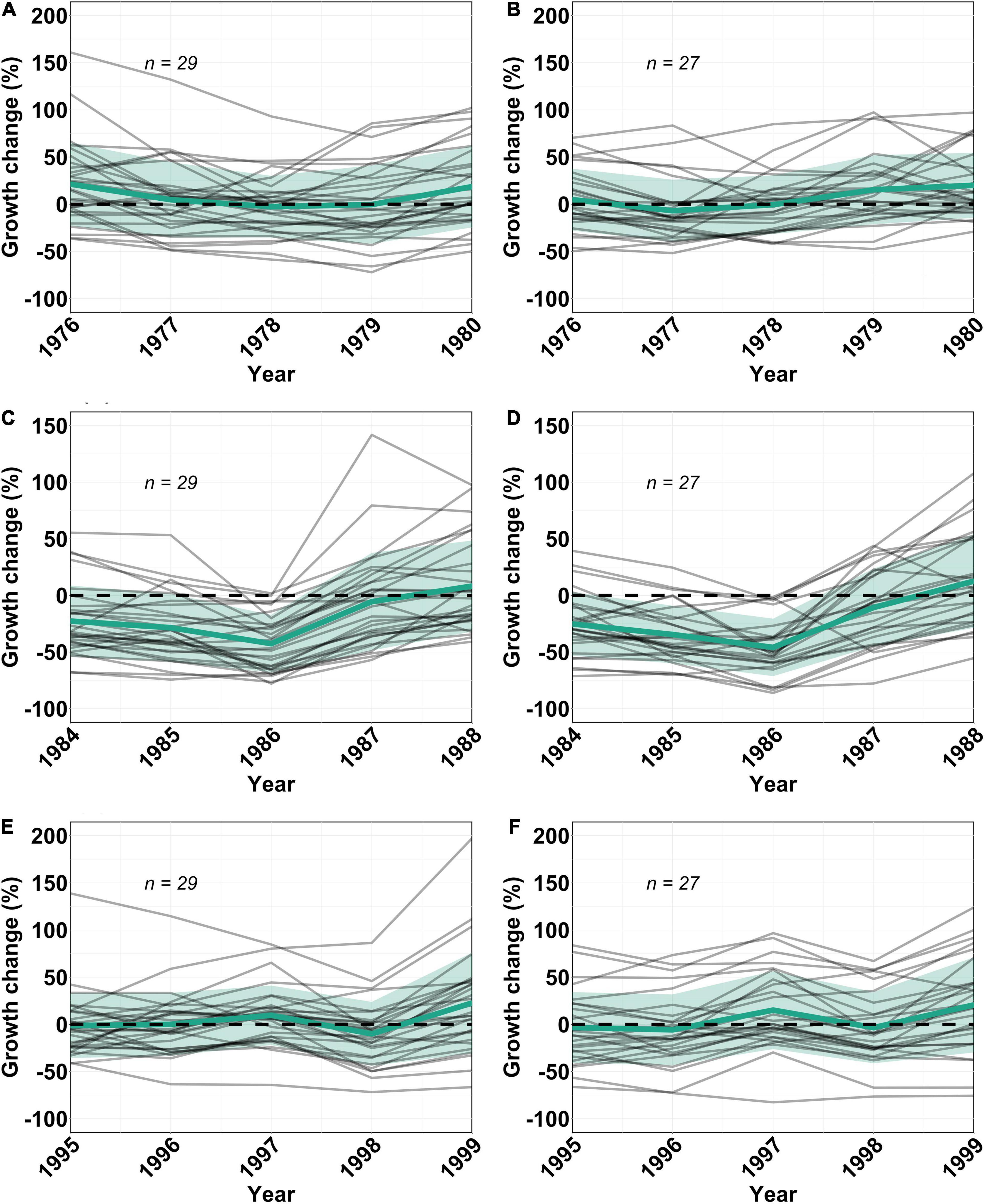
Figure 2. Individual tree (gray lines) and mean (solid green lines) ± 1 SD (green shaded areas) percentage growth change across all trees at a given density relative to BAI1976con, BAI1984con or BAI1995con values for the 1976 (A,B), 1984 (C,D), and 1995 (E,F) droughts, respectively, calculated annually for both high density (ρH – A,C,E; n = 29) and low density (ρL – B,D,F; n = 27) stands. The horizontal black dashed lines indicate no detectable difference between a given year’s growth and BAIcon (growth rates recovered to climatically constrained pre-drought average levels).
We then used a generalized linear mixed model using the “lme4” package to test whether the proportion of trees growing at >2SD below their pre-drought growth average was different during and following the 1976, 1984, and 1995 droughts. Drought event and year were fit as fixed effects along with an interaction between these two variables, while tree ID was fit as a random effect. A 2 degree polynomial was applied to Year. Adjusted marginal means were extracted from the model using the “emmeans” package and the proportions of trees with reduced growth compared at each year. A Bonferroni correction was applied to adjust for multiple comparisons.
To assess the extent to which drought induces shifts in stand structure, we calculated three complementary indices annually for each treatment using all BAI records; Gd, Si, and Sa. Gd was calculated manually in R following Method 3 outlined in West (2018) by plotting cumulative proportional tree basal area (BA) (χ) against the corresponding cumulative proportional tree BAI (γ)and then fitting multiple polynomial functions using ordinary least squared regression and calculating the area under the fitted curve. We selected the best fitting polynomial function using the lowest AIC value with a maximum polynomial order of eight for each year.
Growth dominance measures the degree to which larger or smaller trees are contributing to overall stand growth relative to their size and ranges from –1 to 1, with a hypothetical value of 0 indicating no size class is dominating growth and all trees are growing at rates directly proportional to their size (West, 2018). The closer Gd is to 1, the more larger sized trees are dominating total stand growth, while the closer Gd is to –1, the more smaller trees are dominating total stand growth (West, 2014, 2018).
Size inequality reflects the variability in tree sizes and ranges from 0 to 1, with inequality being zero (i.e., total equality) if all trees are identical in size. Sa is intimately linked to Si, with values ranging from 0 to 2. Sa measures how much larger trees (Sa values ranging from 1 to 2) or smaller trees (Sa values ranging from 0 to 1) in the population contribute to the observed levels of Si. Both Si and Sa were calculated using the Gini coefficient and the Lorenz asymmetry coefficient, respectively, in the ineq package in R (Zeileis and Kleiber, 2014) by plotting cumulative proportional tree size (BA) against cumulative proportional tree frequency.
To identify whether changes in Gd following drought were indicative of a threshold-type response, we initially tested for structural change in Gd over time (separately for high (ρH) and low (ρL) density stands) using the efp function in the strucchange package in R (Zeileis et al., 2002) and specifying “OLS-CUSUM” which runs an empirical fluctuation process of OLS residuals to test whether the null hypothesis of no structural change over time is supported. For Gd in both ρL and ρH the null hypothesis was rejected, indicating the presence of significant structural change at some point(s) in both Gd timeseries (p < 0.05 for Gd in both ρH and ρL). We subsequently used change point analysis [where the term “change point” is synonymous with the term “threshold” (Andersen et al., 2009)] to identify the number and temporal location of any thresholds for Gd in both ρH and ρL. This method has been previously used to assess abrupt decreases in tree growth linked to drought (Vanoni et al., 2016a,b) and does not require the number of potential thresholds to be pre-determined. Similarly, this method can detect both positive (abrupt increases in Gd) or negative (abrupt decreases in Gd) change points. The optimum number of change points was simultaneously estimated using the Bayesian Information Criterion (BIC) and the location of each change points along with their 95% CI were estimated as calendar years using the breakpoints function from the strucchange package in R (Zeileis et al., 2002). Gd values for ρH were also compared to ρL in all three drought years (1976, 1984, and 1995) by permuting the difference between values 10,000 times and using the distribution of these Gd differences to determine the probability of observing a particular value by chance.
During the 1976 drought year, mean PGC was positive in both the high density (ρH) (+21%) and low density (ρL) (+5%) stands but slightly negative in the 1995 drought year for both ρH (−3%) and ρL (−1%) (Figure 3). I contrast, mean PGC was −23% in ρH and −25% in ρL in 1984 (Figure 3) and continued to decline in both stand densities in the 2 years following the 1984 drought, so that by 1986, mean PGC was −43% and −46% in ρH and ρL respectively, meaning that average tree BAI was 43% and 46% lower than BAI1984con in 1986. While the impact of all three drought events was more pronounced for spline detrended ring width data, the general patterns in PGC were the same as those derived from the BAI analysis (Supplementary Figure 1). The annual pairwise comparison between droughts showed that the 1984 drought year was associated with significantly more trees growing at >2SD below average than in the 1995 drought year and in all three post-drought years after both the 1976 and 1995 droughts (p < 0.05 in all cases Supplementary Table 4). By 1986, 16 trees in ρL (55%) and 13 trees in ρH (48%) were growing at rates > 2SD below BAI1984con levels (Supplementary Table 5). Following 1986, the pattern of continued growth decline reversed and mean PGC became positive in 1988 in both ρL and ρH (Figure 3) and the number of trees with BAI > 2SD below BAI1976con, BAI1984con or BAI1995con levels was not statistically different between all three drought events (p > 0.9 in all cases) (Supplementary Table 4).

Figure 3. BAI adjusted marginal means (averaged over high (ρH) and low (ρL) density stands) for the 1976, 1984, and 1995 drought years. Year = 0 represents the drought year while years 1–4 indicate the 4 years post-drought, while error bars represent 95% unadjusted confidence intervals.
Mixed-effects model analysis showed a significant difference in BAI among drought events, but this difference changed over time (p < 0.001) (Figure 2 and Table 2). Post-hoc analyses of estimated marginal means for BAI were used to compare differences between droughts at an annual resolution (Figure 2). BAI differed significantly after the 1984 drought compared to the 1976 and 1995 droughts for 3 years following the event (p < 0.0001 in all cases, Figure 2 and Supplementary Table 3). However, differences in BAI between the 1976 and 1995 drought were only significant (p < 0.05) in the first post-drought year (p = 0.014), but not in the drought year itself or any other post-drought years (p > 0.06 in all cases) (Figure 2 and Supplementary Table 3). As a result, the slightly more extreme drought of 1984 was associated with a greater absolute change in BAI in the drought year and in the following 3 years relative to the less severe 1976 or 1995 droughts.
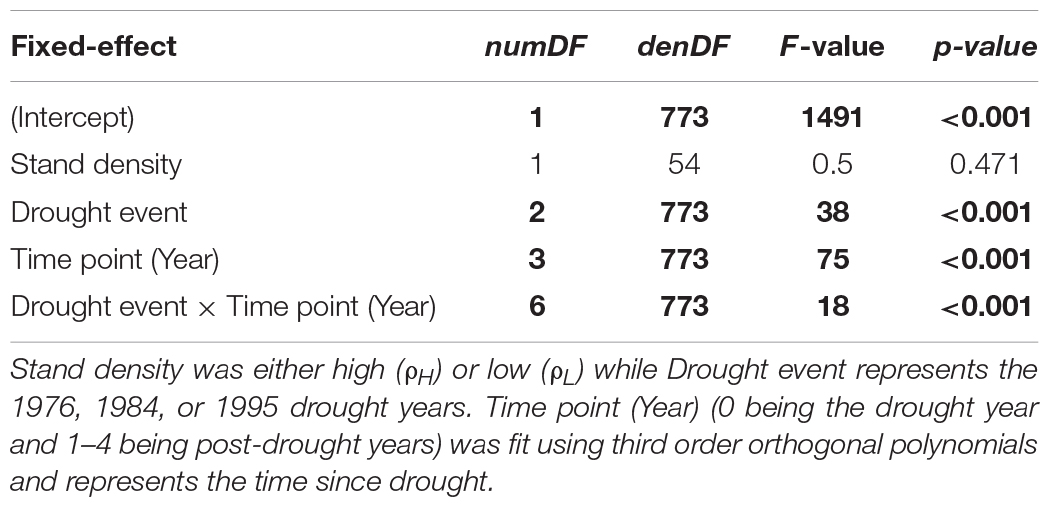
Table 2. ANOVA table for the mixed-effects model of BAI, where numDF = numerator degrees of freedom, denDF = denominator degrees of freedom, interactions are denoted by a × and significant effects (p < 0.05) are highlighted in bold.
Interannual variability in Gd was higher in ρH than ρL across the study period (Figure 4A). Between 1961 and 1973, Gd in ρH indicates that smaller trees were dominating stand growth (small trees were contributing more to total BAI than the same trees were contributing to total BA), but by 1975 larger trees began to dominate growth (larger trees started growing at rates disproportionately fast for their size) (Figure 4A). During the 1976 drought year in ρH, all trees were growing at rates roughly proportional to their size (Gd), however, in the years following 1976 larger trees contributed proportionately more to total stand BAI than their BA contributed to total stand BA (Figure 4A). In contrast, for ρL, Gd remained relatively stable, oscillating around 0 from 1961 to 1985, indicating that all trees were growing at rates roughly proportional to their size (Figure 4A).
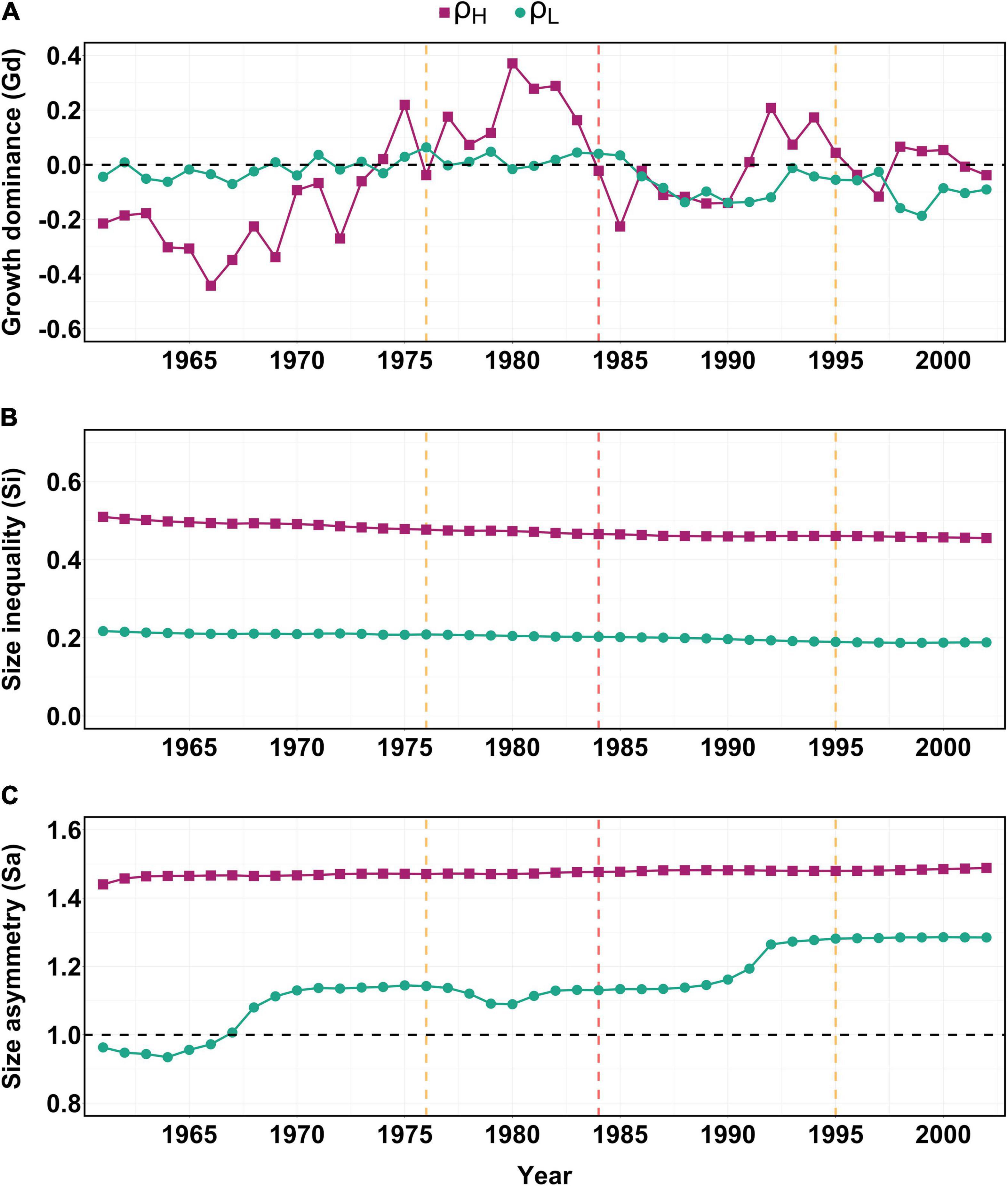
Figure 4. Annual values for (A) Growth dominance (Gd), (B) Size inequality (Si), and (C) Size asymmetry (Sa) calculated annually for both high (ρH) and low (ρL) density treatments. The horizontal black line in (A) indicates that all trees are growing at rates directly proportional to their size, positive values indicate larger trees are dominating growth relative to their size while negative values indicate smaller trees are dominating growth relative to their size in a given year. The dashed horizontal line in (C) indicates both large and small trees are contributing equally to tree size inequality while values >1 or <1 indicate larger or smaller sized trees are contributing more to size inequality, respectively. Orange vertical dashed lines indicate the 1976 and 1995 droughts, while the red vertical dashed line indicates the 1984 drought. Purple squares represent ρH (high density) n = 29, while green circles represent ρL (low density) n = 27.
In 1984 Gd shifted away from larger trees toward smaller trees in ρH, meaning all trees contributed to total stand BAI at rates roughly proportional to their BA. This directional shift in Gd continued into 1985 as smaller trees showed increased Gd, contributing proportionally more to stand level BAI than they did to total stand BA (Figure 4A). Gd briefly returned to roughly zero (all trees were again growing at rates proportional to their size) in 1986, however, in contrast to values of Gd prior to 1984, smaller trees contributed proportionally more to stand level growth from 1987 to 1991. Larger trees again began to dominate growth from 1992 in ρH, a pattern that appeared to temporarily reverse following 1995, where smaller trees dominated growth until 1998. In ρL, Gd became consistently negative from 1986 until 1993 when Gd returned to roughly zero, though Gd never became positive again after 1986 in ρL (Figure 4A). Despite these shifts in Gd, the ten largest trees for all 3 years prior to the 1984 drought were still the same ten largest trees in 1988.
Across the study period (1961–2002), Si was consistently higher for all years in the higher density (ρH) than the lower density (ρL) treatment (Figure 4B) meaning that there was a greater range of tree diameters in the higher density stand. There was also a slight decrease in Si in both ρH and ρL across the study period but no discernible change in Si during or after any of the three drought events (Figure 4B).
In general, Sa remained stable throughout the study period in ρH, while ρL showed a brief depression in Sa between 1978 and 1981, indicating larger trees were temporarily contributing less to Si than before 1978 (Figure 4C). However, Sa returned to pre-1978 levels in ρL by 1982. No immediate change in Sa followed the 1984 drought in either ρH or ρL, however, between 1990 and 1993, Sa shifted to a higher and stable level in ρL, indicating that from 1990 onward in this lower density stand, larger trees were consistently contributing more to size inequality than previously (Figure 4C).
Change point analysis of Gd revealed three change points (i.e., thresholds) for ρH (1973, 1983 and 1990) and two change points for ρL (1970 and 1985) (Supplementary Figure 2). Since the date assigned to each breakpoint is allocated to the last year of the previous period (i.e., 1 year before a change to a lower or higher Gd level) a change point in 1983 indicates a change occurred in 1984. As a result, the extreme drought event of 1984 aligns with a threshold change in Gd in the same year in ρH, while a threshold change in Gd for ρL occurred 2 years later in 1986 (Supplementary Figure 2). Neither the severe drought of 1976 or 1995 fell within the 95% CI of any of the other Gd thresholds identified in either ρH or ρL (Figure 4A and Supplementary Figure 5). Change point analysis was not conducted for Si or Sa due to apparent insensitivity of these indices around all three drought years (Figure 4) and no significant differences in Gd were noted between ρH and ρL in either 1976, 1984 or 1995 (p > 0.613 in all cases).
In this study we developed a standardized method for calculating tree-level pre-drought growth averages using SPEI-constrained growth years, against which drought and post-drought growth performance was assessed for three droughts of differing severity. We then used mixed-effects models, stand level indices of Gd, Si, and Sa calculated annually and change point analysis to investigate whether droughts of differing severity were associated with significantly different growth responses in both absolute (BAI) and relative (PGC) terms, changes in stand dynamics or the magnitude and duration of any drought legacies.
We provide evidence of a threshold response to the most extreme drought (1984) in the study period (1961–2002) which was associated with a large and sustained post-drought growth reduction in both density treatments. In contrast, we found no evidence of a significant impact on average tree growth following the two less severe droughts in 1976 and 1995. This result was mirrored at the stand level with a significant shift in Gd toward smaller trees in both high and low density stands following the most extreme drought in 1984, but neither of the less severe droughts in 1976 or 1995. Collectively, the impact on average tree growth, the proportion of trees impacted and the shift in Gd indicate that a drought severity threshold for P. sylvestris may have been crossed at this site following the 1984 drought.
Many studies calculate resistance, recovery and resilience relative to a pre-drought growth average derived from the years immediately preceding a drought event (Zang et al., 2014; Gazol et al., 2017, 2018). However, recent work has shown that the subjective choice of the number of years to include when calculating these pre-drought growth averages can bias estimates of drought resilience (Schwarz et al., 2020; Ovenden et al., 2021). Here we developed an approach which uses the SPEI to climatically standardize the selection of these pre-drought years to avoid the need for this subjective selection and minimize the risk of including pre-drought year BAI values that may themselves be the product of an abnormally dry year in the calculation of pre-drought average growth.
Using these climatically constrained pre-drought growth averages to address our first objective, we show that the extreme drought of 1984 was associated with a protracted period of low growth and changes in growth dominance at the stand level, consistent with a threshold-type drought response noted in other pine species (Huang et al., 2015; Asbjornsen et al., 2021). However, we did not find any evidence of a similar response following the lower severity droughts on 1976 and 1995, nor did we document any significant differences between the high or low density stands (Table 2), the latter result being in keeping with other recent work on the growth response of P. sylvestris to drought which also documented no effect of tree density in this species (Bello et al., 2019). As such, we found that a small increase in drought severity [measured in both absolute (CWD) and relative (SPEI) terms] in 1984 compared to the 1976 and 1995 droughts was associated with a significant decrease in average tree BAI and a significant increase in the number of trees growing a > 2SD below their pre-drought average for up to three post-drought years (Figure 2 and Supplementary Tables 3, 4). These results reflect other studies that have documented multi-year legacies on tree growth following extreme drought (Anderegg et al., 2015b; Wu et al., 2018). Huang et al. (2015) reported an SPEI threshold of −1.64 for Pinus edulis and Pinus ponderosa, after which progressively more negative SPEI values cause significant declines in forest growth. This value reported by Huang et al. (2015) is slightly less negative than the SPEI recorded for all three droughts in this present study (1976 SPEIAug6 of – 1.8, 1984 SPEIAug6 of –2.0 and a 1995 SPEIAug6 of – 1.9) (Figure 1). As such, our results support other recent work that suggests that thresholds of drought severity likely vary among species (Cavin et al., 2013; Kolb, 2015; Gazol et al., 2020) but also highlights how setting generic, climatologically defined drought thresholds too low can risk including events that may not have been strong enough to elicit an organismal or systemic response (Smith, 2011), potentially clouding our understanding of how different species respond to extreme events. Similarly, the characterization of drought purely in terms of severity using indices (e.g., SPEI or CWD) may also obscure important differences between droughts stemming from the inherent multi-dimensionality of these events (e.g., timing, duration, and intensity). Due to the limited number of drought events in the climate data at this study site, it was not possible to ascertain whether the timing, duration or intensity of drought at this site were linked to patterns in tree growth response. While none of the post-drought years in this study appeared to be abnormally dry (Figure 1), we acknowledge that post-drought climate likely plays an important role in regulating forest recovery dynamics but was not explicitly considered in this study, however, where possible future studies on forest resilience to drought should aim to included post-drought climate to ensure a more complete understanding of drought legacy.
At the stand level, mean PGC in both the high and low density stands remained relatively constant during and following both the 1976 and 1995 droughts with some trees showing reductions in radial growth while others showed radial growth increases. The net effect of this inter-tree variability may have partly buffered against stand-level changes in BAI during these two less severe droughts. While individual tree growth variability is negatively linked to individual drought resilience (Bose et al., 2020), inter-tree variability in pre-drought growth may act as a form of response diversity (Mori et al., 2013) and reflects other recent studies that highlight the potential for intraspecific differences to partly buffer against the impacts of climate change (Oney et al., 2013; Taeger et al., 2015; Moran et al., 2016). In contrast, after the 1984 drought stand growth reduced to levels substantially below BAI1984con (Figure 3), temporarily reduced inter-tree growth variability (Figure 3) and shifted growth dominance (Gd) toward smaller trees (Figure 4), indicating that a threshold for intraspecific variability to buffer against stand level growth loss may have been exceeded.
The drought severity required to cross such thresholds is likely to be linked to the ecophysiological limits of a species hydraulic system to drought stress (Choat et al., 2012; Adams et al., 2017) and pre-drought conditions, which can lead to phenomenon such as structural overshoot (Jump et al., 2017). While it is possible that observed shifts in Gd in this study are partly the result of the death of neighboring trees, assessments of both the high density (ρH) and low density (ρL) treatments in 1985 and 1990 (1 and 6 years after the 1984 extreme drought) show mortality was not abnormally high during these post-drought periods (Supplementary Table 1). While we acknowledge that in some cases mortality might be delayed for many years following drought (Bigler et al., 2007), we show that the crossing of drought thresholds does not necessarily need to result in widespread or elevated tree mortality to be associated with detectable changes in growth dominance and stand dynamics (Stuart-Haëntjens et al., 2015; Batllori et al., 2020), addressing our second research question. Persistent, interspecific shifts in competitive dominance have been documented following extreme drought in other species (Cavin et al., 2013), but such shifts at the population level were not observed in a much larger scale study of P. sylvestris (Martínez-Vilalta et al., 2012).
Collectively, our results demonstrate how small increases in drought severity can be associated with changes in tree growth at different tree densities and may influence stand dynamics to a degree that is not observed under less severe drought conditions. While we did not detect any large changes in Si or Sa in response to drought, this study exclusively looked at even aged, monospecific stands of P. sylvestris. Future work should investigate whether drought induces changes in Si, Sa, and Gd in more structurally diverse, species rich stands of variable age classes to further our understanding of the role of drought in driving novel developmental trajectories and structuring community composition. Similarly, understanding which combinations of drought intensity, timing, duration, and frequency are associated with differences in forest growth and stand development will be key.
Here we demonstrate how extreme drought is associated with a threshold response in Pinus sylvestris trees growing in Scotland at different stand densities. Once crossed, this threshold was associated with decreased BAI which persisted for up to 3 years after drought, a significant increase in the number of trees growing below average and shifts in growth dominance from larger trees to smaller trees in both stand densities. These results suggests that tree level variability may provide some stand level resilience to drought, but also demonstrates how small increases in drought severity may exceed this compensatory mechanism and result in stand level changes that are not manifest under slightly less severe drought conditions.
Publicly available datasets were analyzed in this study. This data can be found here: https://datastorre.stir.ac.uk/handle/11667/163.
TO led conceptual development, methodological approach, analysis, and writing of the manuscript. MP contributed to concept development, manuscript production, and facilitated data availability. T-KC contributed to the methodological approach, analysis, and writing of the manuscript. MM contributed to the manuscript production. AJ contributed to the conceptual development and manuscript production. All authors contributed to the article and approved the submitted version.
This work was funded by the Forest Research, Scottish Forestry Trust, and University of Stirling. The data utilized in this manuscript was from a study supported by the EU Fifth Framework Project (Compression Wood: QLK5-CT-2001-00177) and Forestry Commission of Great Britain.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
We thank Danni Thompson for her support and advice during manuscript preparation, and Brad Duthie and Luc Bussiere of Stirling University for discussion and advice on statistical analysis. We are grateful to Barry Gardiner and colleagues for providing data, Adam Ash for his insight and logistical help, and Michal Petr of Forest Research for his thoughts and guidance early on during the conceptual development of this work.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/ffgc.2021.737342/full#supplementary-material
Adams, H. D., Zeppel, M. J. B., Anderegg, W. R. L., Hartmann, H., Landhäusser, S. M., Tissue, D. T., et al. (2017). A multi-species synthesis of physiological mechanisms in drought-induced tree mortality. Nat. Ecol. Evol. 1, 1285–1291. doi: 10.1038/s41559-017-0248-x
Allen, C. D., Breshears, D. D., and McDowell, N. G. (2015). On underestimation of global vulnerability to tree mortality and forest die-off from hotter drought in the Anthropocene. Ecosphere 6, 1–55. doi: 10.1890/ES15-00203.1
Allen, C. D., Macalady, A. K., Chenchouni, H., Bachelet, D., McDowell, N., Vennetier, M., et al. (2010). A global overview of drought and heat-induced tree mortality reveals emerging climate change risks for forests. For. Ecol. Manag. 259, 660–684. doi: 10.1016/j.foreco.2009.09.001
Anderegg, W. R. L., Anderegg, L. D. L., Kerr, K. L., and Trugman, A. T. (2019). Widespread drought-induced tree mortality at dry range edges indicates climate stress exceeds species’ compensating mechanisms. Glob. Chang. Biol. 25, 3793–3802. doi: 10.1111/gcb.14771
Anderegg, W. R. L., Schwalm, C., Biondi, F., Camarero, J. J., Koch, G., Litvak, M., et al. (2015b). Pervasive drought legacies in forest ecosystems and their implications for carbon cycle models. Science 349, 528–532.
Anderegg, W. R. L., Flint, A., Huang, C. Y., Flint, L., Berry, J. A., Davis, F. W., et al. (2015a). Tree mortality predicted from drought-induced vascular damage. Nat. Geosci. 8, 367–371. doi: 10.1038/ngeo2400
Anderegg, W. R. L., Kane, J. M., and Anderegg, L. D. L. (2013). Consequences of widespread tree mortality triggered by drought and temperature stress. Nat. Clim. Chang. 3, 30–36. doi: 10.1038/nclimate1635
Anderegg, W. R. L., Konings, A. G., Trugman, A. T., Yu, K., Bowling, D. R., Karp, D., et al. (2018). Hydraulic diversity of forests regulates ecosystem resilience during drought. Science 561, 538–541. doi: 10.1038/s41586-018-0539-7
Anderegg, W. R. L., Trugman, A. T., Badgley, G., Konings, A. G., and Shaw, J. (2020). Divergent forest sensitivity to repeated extreme droughts. Nat. Clim. Chang. 10, 1091–1095. doi: 10.1038/s41558-020-00919-1
Andersen, T., Carstensen, J., Hernández-García, E., and Duarte, C. M. (2009). Ecological thresholds and regime shifts: approaches to identification. Trends Ecol. Evol. 24, 49–57. doi: 10.1016/j.tree.2008.07.014
Asbjornsen, H., McIntire, C. D., Vadeboncoeur, M. A., Jennings, K. A., Coble, A. P., and Berry, Z. C. (2021). Sensitivity and threshold dynamics of Pinus strobus and Quercus spp. in response to experimental and naturally occurring severe droughts. Tree Physiol. 41, 1819–1835. doi: 10.1093/treephys/tpab056
Bartlett, M. K., Klein, T., Jansen, S., Choat, B., and Sack, L. (2016). The correlations and sequence of plant stomatal, hydraulic, and wilting responses to drought. Proc. Natl. Acad. Sci. U.S.A. 113, 13098–13103. doi: 10.1073/pnas.1604088113
Batllori, E., Lloret, F., Aakala, T., Anderegg, W. R. L., Aynekulu, E., Bendixsen, D. P., et al. (2020). Forest and woodland replacement patterns following drought-related mortality. Proc. Natl. Acad. Sci. U.S.A. 117, 29720–29729. doi: 10.1073/pnas.2002314117
Beguería, S., Vicente-Serrano, S. M., Reig, F., and Latorre, B. (2014). Standardized precipitation evapotranspiration index (SPEI) revisited: parameter fitting, evapotranspiration models, tools, datasets and drought monitoring. Int. J. Climatol. 34, 3001–3023. doi: 10.1002/joc.3887
Bello, J., Vallet, P., Perot, T., Balandier, P., Seigner, V., Perret, S., et al. (2019). How do mixing tree species and stand density affect seasonal radial growth during drought events? For. Ecol. Manag. 432, 436–445. doi: 10.1016/j.foreco.2018.09.044
Bennett, A. C., Mcdowell, N. G., Allen, C. D., and Anderson-Teixeira, K. J. (2015). Larger trees suffer most during drought in forests worldwide. Nat. Plants 1, 1–5. doi: 10.1038/nplants.2015.139
Bigler, C., Gavin, D. G., Gunning, C., and Veblen, T. T. (2007). Drought induces lagged tree mortality in a subalpine forest in the Rocky Mountains. Oikos 116, 1983–1994. doi: 10.1111/j.2007.0030-1299.16034.x
Bose, A. K., Gessler, A., Bolte, A., Bottero, A., Buras, A., Cailleret, M., et al. (2020). Growth and resilience responses of Scots pine to extreme droughts across Europe depend on predrought growth conditions. Glob. Chang. Biol. 26, 4521–4537. doi: 10.1111/gcb.15153
Bunn, A., Korpela, M., Biondi, F., Campelo, F., Mérian, P., Qeadan, F., et al. (2019). dplR: Dendrochronology Program Library in R. R Package Version 1.7.0.
Cavin, L., Mountford, E. P., Peterken, G. F., and Jump, A. S. (2013). Extreme drought alters competitive dominance within and between tree species in a mixed forest stand. Funct. Ecol. 27, 1424–1435. doi: 10.1111/1365-2435.12126
Choat, B., Jansen, S., Brodribb, T. J., Cochard, H., Delzon, S., Bhaskar, R., et al. (2012). Global convergence in the vulnerability of forests to drought. Nature 491, 752–755. doi: 10.1038/nature11688
DeSoto, L., Cailleret, M., Sterck, F., Jansen, S., Kramer, K., Robert, E. M. R., et al. (2020). Low growth resilience to drought is related to future mortality risk in trees. Nat. Commun. 11:545. doi: 10.1038/s41467-020-14300-5
Ding, H., Pretzsch, H., Schütze, G., and Rötzer, T. (2017). Size-dependence of tree growth response to drought for Norway spruce and European beech individuals in monospecific and mixed-species stands. Plant Biol. 19, 709–719. doi: 10.1111/plb.12596
Gagkas, Z., Lilly, A., Baggaley, N., and Donnelly, D. (2019). Map of Available Water Capacity of Soils in Scotland. Aberdeen: James Hutton Institute.
Gazol, A., Camarero, J. J., Anderegg, W. R. L., and Vicente-Serrano, S. M. (2017). Impacts of droughts on the growth resilience of Northern Hemisphere forests. Glob. Ecol. Biogeogr. 26, 166–176. doi: 10.1111/geb.12526
Gazol, A., Camarero, J. J., Sangüesa-barreda, G., Serra-Maluquer, X., Sánchez-Salguero, R., Coll, L., et al. (2020). Tree species are differently impacted by cumulative drought stress and present higher growth synchrony in dry places. Front. For. Glob. Chang. 3:573346. doi: 10.3389/ffgc.2020.573346
Gazol, A., Camarero, J. J., Vicente-Serrano, S. M., Sánchez-Salguero, R., Gutiérrez, E., de Luis, M., et al. (2018). Forest resilience to drought varies across biomes. Glob. Chang. Biol. 24, 2143–2158. doi: 10.1111/gcb.14082
Gessler, A., Bottero, A., Marshall, J., and Arend, M. (2020). The way back: recovery of trees from drought and its implication for acclimation. New Phytol. 228, 1704–1709. doi: 10.1111/nph.16703
Granda, E., Gazol, A., and Camarero, J. J. (2018). Functional diversity differently shapes growth resilience to drought for co-existing pine species. J. Veg. Sci. 29, 265–275. doi: 10.1111/jvs.12617
Greenwood, S., Ruiz-Benito, P., Martínez-Vilalta, J., Lloret, F., Kitzberger, T., Allen, C. D., et al. (2017). Tree mortality across biomes is promoted by drought intensity, lower wood density and higher specific leaf area. Ecol. Lett. 20, 539–553. doi: 10.1111/ele.12748
Haber, L. T., Fahey, R. T., Wales, S. B., Correa Pascuas, N., Currie, W. S., Hardiman, B. S., et al. (2020). Forest structure, diversity, and primary production in relation to disturbance severity. Ecol. Evol. 10, 4419–4430. doi: 10.1002/ece3.6209
He, Q., Bertness, M. D., and Altieri, A. H. (2013). Global shifts towards positive species interactions with increasing environmental stress. Ecol. Lett. 16, 695–706. doi: 10.1111/ele.12080
Hilker, M., Schwachtje, J., Baier, M., Balazadeh, S., Bäurle, I., Geiselhardt, S., et al. (2016). Priming and memory of stress responses in organisms lacking a nervous system. Biol. Rev. 91, 1118–1133. doi: 10.1111/brv.12215
Hoffmann, N., Schall, P., Ammer, C., Leder, B., and Vor, T. (2018). Drought sensitivity and stem growth variation of nine alien and native tree species on a productive forest site in Germany. Agric. For. Meteorol. 256–257, 431–444. doi: 10.1016/j.agrformet.2018.03.008
Huang, K., Yi, C., Wu, D., Zhou, T., Zhao, X., Blanford, W. J., et al. (2015). Tipping point of a conifer forest ecosystem under severe drought. Environ. Res. Lett. 10:24011. doi: 10.1088/1748-9326/10/2/024011
Ingrisch, J., and Bahn, M. (2018). Towards a comparable quantification of resilience. Trends Ecol. Evol. 33, 251–259. doi: 10.1016/j.tree.2018.01.013
Jump, A. S., Ruiz-Benito, P., Greenwood, S., Allen, C. D., Kitzberger, T., Fensham, R., et al. (2017). Structural overshoot of tree growth with climate variability and the global spectrum of drought-induced forest dieback. Glob. Chang. Biol. 23, 3742–3757. doi: 10.1111/gcb.13636
Kannenberg, S. A., Schwalm, C. R., and Anderegg, W. R. L. (2020). Ghosts of the past: how drought legacy effects shape forest functioning and carbon cycling. Ecol. Lett. 23, 891–901. doi: 10.1111/ele.13485
Kolb, T. E. (2015). A new drought tipping point for conifer mortality. Environ. Res. Lett. 10, 1–3. doi: 10.1088/1748-9326/10/3/031002
Lenth, R. V. (2020). emmeans: Estimated Marginal Means, Aka Least-Squares Means. R Package Version 1.7.0. Available online at: https://cran.r-project.org/web/packages/emmeans/index.html (accessed November 2021).
Li, X., Piao, S., Wang, K., Wang, X., Wang, T., Ciais, P., et al. (2020). Temporal trade-off between gymnosperm resistance and resilience increases forest sensitivity to extreme drought. Nat. Ecol. Evol. 4, 1075–1083. doi: 10.1038/s41559-020-1217-3
Lloret, F., Keeling, E. G., and Sala, A. (2011). Components of tree resilience: Effects of successive low-growth episodes in old ponderosa pine forests. Oikos 120, 1909–1920. doi: 10.1111/j.1600-0706.2011.19372.x
Lutz, J. A., van Wagtendonk, J. W., and Franklin, J. F. (2010). Climatic water deficit, tree species ranges, and climate change in Yosemite National Park. J. Biogeogr. 37, 936–950. doi: 10.1111/j.1365-2699.2009.02268.x
Martínez-Vilalta, J., and Lloret, F. (2016). Drought-induced vegetation shifts in terrestrial ecosystems: the key role of regeneration dynamics. Glob. Planet. Chang. 144, 94–108. doi: 10.1016/j.gloplacha.2016.07.009
Martínez-Vilalta, J., López, B. C., Loepfe, L., and Lloret, F. (2012). Stand- and tree-level determinants of the drought response of Scots pine radial growth. Oecologia 168, 877–888. doi: 10.1007/s00442-011-2132-8
McCune, B., and Keon, D. (2002). Equations for potential annual direct incident radiation and heat load. J. Veg. Sci. 13, 603–606. doi: 10.1111/j.1654-1103.2002.tb02087.x
McDowell, N. G., Allen, C. D., Anderson-teixeira, K., Aukema, B. H., Bond-lamberty, B., Chini, L., et al. (2020). Pervasive shifts in forest dynamics in a changing world. Science 368:eaaz9463. doi: 10.1126/science.aaz9463
Merlin, M., Perot, T., Perret, S., Korboulewsky, N., and Vallet, P. (2015). Effects of stand composition and tree size on resistance and resilience to drought in sessile oak and Scots pine. For. Ecol. Manag. 339, 22–33. doi: 10.1016/j.foreco.2014.11.032
Moran, E. V., Hartig, F., and Bell, D. M. (2016). Intraspecific trait variation across scales: implications for understanding global change responses. Glob. Chang. Biol. 22, 137–150. doi: 10.1111/gcb.13000
Mori, A. S., Furukawa, T., and Sasaki, T. (2013). Response diversity determines the resilience of ecosystems to environmental change. Biol. Rev. 88, 349–364. doi: 10.1111/brv.12004
Oney, B., Reineking, B., O’Neill, G., and Kreyling, J. (2013). Intraspecific variation buffers projected climate change impacts on Pinus contorta. Ecol. Evol. 3, 437–449. doi: 10.1002/ece3.426
Ovenden, T. S., Perks, M. P., Clarke, T., Mencuccini, M., and Jump, A. S. (2021). Life after recovery: Increased resolution of forest resilience assessment sheds new light on post-drought compensatory growth and recovery dynamics. J. Ecol. 109, 3157–3170. doi: 10.1111/1365-2745.13576
Pinheiro, J., Bates, D., DebRoy, S., and Sarkar, D. (2020). R Core Team (2020) nlme: Linear and Nonlinear Mixed Effects Models. R package version 3.1-148.
R Core Team (2019). R: A Language and Environment for Statistical Computing. Vienna: R Foundation for Statistical Computing.
Redmond, M. D. (2019). CWD and AET Function V1.0.1 (Version V1.0.0). Zenodo. doi: 10.5281/zenodo.2530955
Robinson, E. L., Blyth, E., Clark, D. B., Comyn-Platt, E., Finch, J., and Rudd, A. C. (2017). Climate Hydrology and Ecology Research Support System Meteorology Dataset for Great Britain (1961-2015) [CHESS-met] v1.2. NERC Environmental Information Data Centre. Available online at: https://catalogue.ceh.ac.uk/documents/b745e7b1-626c-4ccc-ac27-56582e77b900
Rubio-Cuadrado, Á, Camarero, J. J., del Río, M., Sánchez-González, M., Ruiz-Peinado, R., Bravo-Oviedo, A., et al. (2018). Long-term impacts of drought on growth and forest dynamics in a temperate beech-oak-birch forest. Agric. For. Meteorol. 259, 48–59. doi: 10.1016/j.agrformet.2018.04.015
Schwarz, J. A., Skiadaresis, G., Kohler, M., Kunz, J., Schnabel, F., Vitali, V., et al. (2020). Quantifying growth responses of trees to drought - a critique of the Lloret-indicators and recommendations for future studies. Curr. For. Rep. 185–200. doi: 10.32942/osf.io/5ke4f
Shukla, P. R., Skea, J., Slade, R., van Diemen, R., Haughey, E., Malley, J., et al. (2019). “Technical Summary,” in Climate Change and Land: an IPCC Special Report on Climate Change, Desertification, Land Degradation, Sustainable Land Management, Food Security, and Greenhouse Gas Fluxes in Terrestrial ecosystems, eds P. R. Shukla, J. Skea, E. Calvo Buendia, V. Masson-Delmotte, H.-O. Pörtner, and D. C. Roberts (Geneva: IPPC).
Slette, I. J., Post, A. K., Awad, M., Even, T., Punzalan, A., Williams, S., et al. (2019). How ecologists define drought, and why we should do better. Glob. Chang. Biol. 25, 3193–3200. doi: 10.1111/gcb.14747
Smith, M. D. (2011). An ecological perspective on extreme climatic events: a synthetic definition and framework to guide future research. J. Ecol. 99, 656–663. doi: 10.1111/j.1365-2745.2011.01798.x
Sohn, J. A., Saha, S., and Bauhus, J. (2016). Potential of forest thinning to mitigate drought stress: a meta-analysis. For. Ecol. Manag. 380, 261–273. doi: 10.1016/j.foreco.2016.07.046
Stuart-Haëntjens, E. J., Curtis, P. S., Fahey, R. T., Vogel, C. S., and Gough, C. M. (2015). Net primary production of a temperate deciduous forest exhibits a threshold response to increasing disturbance severity. Ecology 96, 2478–2487. doi: 10.1890/14-1810.1
Suarez, M. L., and Kitzberger, T. (2008). Recruitment patterns following a severe drought: Long-term compositional shifts in Patagonian forests. Can. J. For. Res. 38, 3002–3010. doi: 10.1139/X08-149
Taeger, S., Sparks, T. H., and Menzel, A. (2015). Effects of temperature and drought manipulations on seedlings of Scots pine provenances. Plant Biol. 17, 361–372. doi: 10.1111/plb.12245
Thornthwaite, C. W. (1948). An approach toward a rational classification of climate. Geogr. Rev. 38, 55–102.
van Mantgem, P. J., Stephenson, N. L., Byrne, J. C., Daniels, L. D., Franklin, J. F., Fulé, P. Z., et al. (2009). Widespread increase of tree mortality rates in the Western United States. Science 323, 521–524. doi: 10.1126/science.1165000
Vanhellemont, M., Sousa-Silva, R., Maes, S. L., Van den Bulcke, J., Hertzog, L., De Groote, S. R. E., et al. (2018). Distinct growth responses to drought for oak and beech in temperate mixed forests. Sci. Total Environ. 650, 3017–3026. doi: 10.1016/J.SCITOTENV.2018.10.054
Vanoni, M., Bugmann, H., Nötzli, M., and Bigler, C. (2016a). Drought and frost contribute to abrupt growth decreases before tree mortality in nine temperate tree species. For. Ecol. Manag. 382, 51–63. doi: 10.1016/j.foreco.2016.10.001
Vanoni, M., Bugmann, H., Nötzli, M., and Bigler, C. (2016b). Quantifying the effects of drought on abrupt growth decreases of major tree species in Switzerland. Ecol. Evol. 6, 3555–3570. doi: 10.1002/ece3.2146
Vicente-Serrano, S. M., Beguería, S., and López-Moreno, J. I. (2010). A multiscalar drought index sensitive to global warming: the standardized precipitation evapotranspiration index. J. Clim. 23, 1696–1718. doi: 10.1175/2009JCLI2909.1
West, P. W. (2014). Calculation of a growth dominance statistic for forest stands. For. Sci. 60, 1021–1023.
West, P. W. (2018). Use of the Lorenz curve to measure size inequality and growth dominance in forest populations. Aust. For. 81, 231–238. doi: 10.1080/00049158.2018.1514578
Wu, X., Liu, H., Li, X., Ciais, P., Babst, F., Guo, W., et al. (2018). Differentiating drought legacy effects on vegetation growth over the temperate Northern Hemisphere. Glob. Chang. Biol. 24, 504–516. doi: 10.1111/gcb.13920
Zang, C., Hartl-Meier, C., Dittmar, C., Rothe, A., and Menzel, A. (2014). Patterns of drought tolerance in major European temperate forest trees: climatic drivers and levels of variability. Glob. Chang. Biol. 20, 3767–3779. doi: 10.1111/gcb.12637
Zeileis, A., and Kleiber, C. (2014). Ineq: Measuring Inequality, Concentration, and Poverty. R Package Version 0.2-13.
Keywords: extreme drought, Pinus sylvestris, threshold response, resistance, SPEI, resilience, stand dynamics, global change
Citation: Ovenden TS, Perks MP, Clarke T-K, Mencuccini M and Jump AS (2021) Threshold Response to Extreme Drought Shifts Inter-Tree Growth Dominance in Pinus sylvestris. Front. For. Glob. Change 4:737342. doi: 10.3389/ffgc.2021.737342
Received: 06 July 2021; Accepted: 30 November 2021;
Published: 16 December 2021.
Edited by:
Paul Szejner, Universidad Nacional Autónoma de México, MexicoReviewed by:
Drew Peltier, Northern Arizona University, United StatesCopyright © 2021 Ovenden, Perks, Clarke, Mencuccini and Jump. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Thomas S. Ovenden, dGhvbWFzLm92ZW5kZW5Ac3Rpci5hYy51aw==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.