Enterprise location choice and regional sustainable development based on the theory of stable matching

As an important path of industrial structure adjustment and upgrading, industrial transfer is of great significance to narrow the regional economic development gap in China and promoting the sustainable development of regional economies. The locational choice of firms is one of the main reasons for the transfer of industries. The aim of this study was to obtain an optimal and stable match between firms and local governments. By constructing an evaluation index system for enterprise location selection and an evaluation index system for local government investment attraction, and based on the relevant linguistic evaluation information, this study calculates the satisfaction of enterprises with the location and the satisfaction of local governments with the target investment enterprises and then obtains the optimal stable match between enterprises and local governments based on the constructed stable match model. Most of the previous studies only consider the location choice of enterprises and government’s investment attraction as their respective unilateral choice of location, ignoring the relationship between the two as mutual stakeholders. This study, however, examines firms’ choice of location and the government’s investment promotion from a bilateral matching perspective. The study also studies the mechanism by which the stable matching between enterprises and the government’s inducements to invest is realized. The results show that, based on the stable matching mechanism, a bilateral matching scheme between enterprises and the government can give full play to the decisive role of the markets in resource allocation, maintain long-term stable investment and cooperation between the two sides, and help China’s industries transfer and upgrade their quality in an orderly fashion.

1 Introduction

After more than 40 years of rapid development and liberalization, China’s economic economy has leaped to second place in the world. However, both an imbalance in economic development among different regions and the urgent need for industrial transformation and upgrading have become important problems that restrict China’s economic and social development (Mechanism of realizing industrial structure upgrading through technology-driven regional innovation capacity, 2008; Justin Yifu and Wang, 2021). Industrial transfer is of great significance for the coordination of regional economic development, the vigorous promotion of industrial structure adjustment and upgrades, and the realization of China’s sustainable economic development (Huang et al., 2020; Ding et al., 2022; Liu, 2019). Industrial transfer is realized through both location selection and the subsequent change of location by enterprises in different regions (Michie and Oughton, 2001; Liang et al., 2020). In the process of industrial transfer, enterprises are the main element in transfers, with the selection of enterprise location conducive to enterprises not only making the best use of the superior resources in other places, reducing production costs, and improving the competitiveness of enterprises but also narrowing the gap of regional economic development (Baas and Boons, 2004). As an important component of industrial relocation, enterprises contribute to the sustainable development of the region and improve the quality of life of its inhabitants and contribute to the optimization of four aspects of sustainable development: labor, gender equality, sustainable development of resources, and the equitable distribution of benefits in all areas (Vázquez Maguirre et al., 2018; Yu and Wang, 2021). Therefore, it is of great theoretical and practical significance to study the choice of location by enterprises.

It should be pointed out that, in the past, most research only regarded the choice of enterprise location as an isolated responsibility of the enterprise itself. In other words, it was the unilateral choice of enterprises to settle on a location; research ignored the relationship between the location choice of enterprises and stakeholders. In fact, the location choice of enterprises is closely related to the investment attraction of local governments (Hong, 2009; Li, 2021). Attempts by traditional local governments to attract investment involved a process of choosing from a large number of target enterprises suitable for the local government’s own regional economic development and is a unilateral choice (Belussi and Sammarra, 2012; Budish, 2012; Tian et al., 2019; Fierla, 1998). However, the fact that an enterprise is interested in the target location does not mean that the enterprise meets the policy requirements of the local government for attracting investment; similarly, the enterprises that the local government intends to introduce may not be satisfied with the location, that is, the location selection of enterprises and the local government’s investment attraction are two-way choices and a typical bilateral matching problem (Schiele, 2008). Therefore, the traditional enterprise location selection and local government investment promotion methods belong to a decentralized resource allocation model. Because both enterprises and local governments only consider their own needs and preferences, coupled with the influence of spatial and geographical barriers and asymmetric information, they cannot effectively play a market mechanism resource allocation role. This results in both low efficiency of enterprise location selection and local government investment promotion, with increased matching costs for both parties.

The aim of this study was to find a stable matching mechanism between enterprises and the government to attract investment and to give full play to the decisive role of the market mechanism in resource allocation. Based on this, this study applies the stable matching theory to the location selection of enterprises, so as to realize the efficient and stable matching between enterprises and local governments. This is carried out by using the centralized matching mechanism. The contribution of this study is to build an evaluation index system of enterprise location selection and government investment attraction, put forward a method to calculate satisfaction between enterprises and local governments with linguistic evaluation information, and design a model of stable matching between enterprises and local governments. In this way, a stable matching scheme for both sides is obtained.

2 Literature review

The location selection of enterprises is a complex decision-making process for enterprises. They have to select locations according to their own development needs and the current economic situation, resources, infrastructure, and other idiosyncrasies of relevant locations. For enterprises, what kind of location to choose for production and business activities has an extremely important influence on their own business results and growth and development.

The theory of enterprise location choice can be traced back to The Isolated Country written by Du Neng in 1826 and developed into an industrial location theory proposed by Weber. However, as later scholars continued their research, more factors affecting the location choice of enterprises have since been taken into account. Krugman (1991) discussed the mechanism of industrial agglomeration and diffusion, and Baldwin and Okubo, (2005) analyzed the location selection of heterogeneous enterprises for the first time.

But the earliest theories of location choice always focused first on cost-effectiveness (Predöhl, 1925; Martin, 1987; Cartier, 2002; Fujita, 2010). From this basis, more and more scholars have turned their attention to market analysis and how to maximize the benefits to the firm (Lösch, 1940; Isard, 1956; Gordon and McCann, 2000; Wilson, 2010). The analysis and application of business-related factors have gradually been applied to the disciplines of geography, spatial economics, and geographical economics (Douglas and Marshall, 1932; Ohlin, 1933; Lösch, 1940; Isard, 1956; Gordon and McCann, 2000; Strzelczyk, 2014). Numerous studies have shown that distance is a manifestation of space, and that the spatial proximity of firms brings with it a number of agglomeration benefits, which are of course associated with consumer markets (Ohlin, 1933; Lösch, 1940; Gordon and McCann, 2000). It is this spatial proximity that facilitates the establishment of business relationships and the expansion of business networks between firms and has a direct impact on the formation of clusters (Juliusson et al., 2005; Mosavi, 2010; Lammarino and McCann, 2006). Regardless of the factors that influence the location choice behavior of firms or their location decisions, these studies have been conducted unilaterally from the perspective of the firms and have not taken into account the matching needs of the local government to the firms.

The research on bilateral matching originated when American medical college graduates sought internships at hospitals and hospitals were recruiting interns. In 1962, Gale and Shapley, (1962) studied the problems of stable marriage and university admission and creatively put forward the concept of stable matching. Alvin E. Roth, an economics professor at Harvard University in the United States, constantly improved the stable matching theory from the 1980s and applied the stable matching theory to solve practical problems. He led the design of the stable matching algorithm for couples in NRMP, the New York public school enrollment matching system, the Boston public school enrollment matching system, and the New England kidney trading system (Roth and Vate, 1990). Because of their outstanding contributions to the stable matching theory and market mechanism design, Professor Lloyd Shapley and Professor Irwin Ross shared the 2012 Nobel Prize in Economics. Wan and Li, (2014) designed a bilateral matching method based on the TODIM method for venture capitalists and investment companies. Shen introduced bilateral matching theory into the study of enterprise location selection and proposed an adjustable individual priority WYS algorithm for the design of bilateral matching mechanisms between enterprises and local governments for investment promotion (Shen et al., 2016; Shen and Cui, 2020)^].

On combing and analyzing the related literature, most of the previous studies have shown that the location choice of enterprises and the enterprise choice of local governments are unilateral based on different considerations and different factors. However, so far, no one has studied the bilateral matching between enterprise location choice and local government investment attraction, and it should be pointed out that what has been studied is enterprises and local governments directly giving information about each other’s preferences, and the matching model established by them does not consider the stability of matching. Based on this, this study applies the stable matching theory to the location selection of enterprises and designs a stable matching model with multi-index evaluation information given by enterprises and local governments.

3 Problem description

In the process of enterprise location selection, a centralized matching mechanism brings together both those enterprises with location selection needs and local governments with investment attraction needs through an intermediary platform. It is different from a decentralized market mechanism and is based on bilateral matching whereby it completes the matching of both sides through some matching decision method according to their needs. A typical bilateral matching problem between enterprises and local governments includes two types of subject sets and a market platform. There are two types of subject collections, namely, the collection of enterprises with location selection needs and the collection of local governments with investment attraction needs, in which the first enterprise represents the first local government. The market platform is an investment platform. The function of this platform is to aggregate enterprises and local governments. Through this platform, enterprises can publish location selection information, and local governments at all levels can publish investment information, matching the two parties according to the information provided by both parties. In the bilateral matching problem between enterprises and local governments studied in this study, each enterprise only chooses one location, that is, it only matches with one local government, and each local government only chooses one enterprise to invest in. $E=\left\{E_1,E_2,\cdots ,E_m\right\}$ and $G=\left\{G_1,G_2,\cdots ,G_n\right\}$, where $E_i$ is firm $i$, $G_j$ is government $j$, $i=\mathrm{1,2}\cdots ,m$, and $j=\mathrm{1,2}\cdots ,n$. Market platform $P$ is investment attraction, and firm $E_i$ selects one location, i.e., $1\le q_j$, $j=\mathrm{1,2}\cdots ,n$.

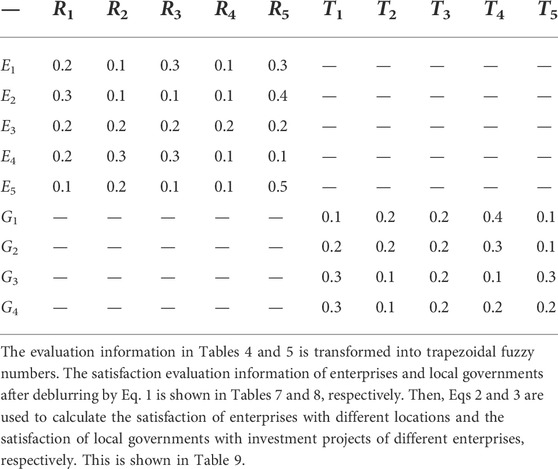
In the process of bilateral matching between enterprises and local governments, enterprises will fully evaluate the location’s good points according to indexes such as regional economic development level, human resource cost, and transportation convenience. In the process of location selection, local governments will also comprehensively evaluate the investment scale of investment enterprises, the number of people driving employment, ecological environment protection, and other indexes. Assuming that the index set is considered by enterprises in choosing a location is the first evaluation index. The weight vector of the evaluation index given by the enterprise represents the weight on the index given by the enterprise. The index set considered by local governments in the process of attracting investment is the first evaluation index. The weight vector of the evaluation index given by the local government expresses the weight of the evaluation index given by the local government. $R=\left\{R_1,R_2,\cdots ,R_h\right\}$, $R_k$ is index $k$, $k=\mathrm{1,2},\dots ,h$; enterprise $E_i$ gives weight vector $u_i=\left(u_i^1,u_i^2,\cdots u_i^h\right)$, $u_i^k$ is weight $R_k$, $0\le u_i^k\le 1$, ${\displaystyle \sum }_{k=1}^h{u}_i^k=1$, $i=\mathrm{1,2}\cdots ,m$, $k=\mathrm{1,2},\dots ,h$. $T=\left\{T_1,T_2,\cdots ,T_f\right\}$ is index $G_j$, $T_d$ is dth index, $d=\mathrm{1,2}\cdots ,f$; $G_j$ gives $v_j=\left(v_j^1,v_j^2,\cdots v_i^f\right)$, $v_j^d$ is weight $T_d$ given by $G_j$, $0\le v_j^d\le 1$, ${\displaystyle \sum }_{k=1}^h{v}_j^d=1$, $j=\mathrm{1,2}\cdots ,n$, $d=\mathrm{1,2}\cdots ,f$.

The satisfaction evaluation matrix of the local government given by the enterprise is $A_i=$, where $a_{ij}^k\left(j=\mathrm{1,2}\cdots ,n,k=\mathrm{1,2},\dots ,h\right)$ represents the satisfaction evaluation value of the local government under the evaluation index $R_k$ given by the enterprise. The satisfaction evaluation matrix of enterprises given by the local government is $B_j=$, where $b_{ij}^d\left(i=\mathrm{1,2}\cdots ,m,d=\mathrm{1,2}\cdots ,f\right)$ represents the satisfaction evaluation value of enterprises given by the local government under the evaluation index.

In this study, the problem to be solved is to use a certain matching mechanism to realize the satisfactory and stable matching between enterprises and local governments according to satisfaction evaluation matrix $A_i$ information and weight vector $u_i$ given by enterprises, the satisfaction evaluation matrix $G_j$ information, and weight vector $v_j$ given by local governments.

4 Stable matching model between enterprises and local governments

4.1 Calculation of satisfaction of enterprises and local governments

In this study, through the analysis of the literature related to enterprise location selection, relevant factors affecting enterprise location selection are extracted, and an index system for comprehensive evaluation of location by enterprises is constructed, as shown in Table 1; through the analysis of the literature related to investment promotion by local governments, relevant factors to be considered in the process of investment promotion by local governments are extracted, and an index system for the comprehensive evaluation of investment enterprises by local governments is constructed (Head et al., 1999; Jordaan, 2012; Lo and Lin, 2015; Gu et al., 2018; Wang et al., 2020; Zhang et al., 2021) (Table 2).

TABLE 1

TABLE 1. Index system for comprehensive evaluation of location by enterprises.

TABLE 2

TABLE 2. Index system for the comprehensive evaluation of investment enterprises by local governments.

4.2 Business and local government satisfaction calculations

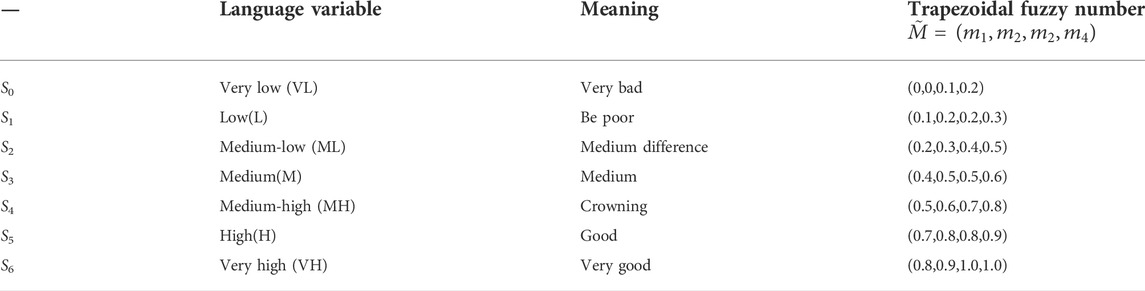
Because linguistic evaluation information is intuitive and easy to express, it is easier for enterprise investment decision-making bodies and local government investment promotion committees to give linguistic evaluation information. The predefined language evaluation index set is $S=\left\{S_0,S_1,\cdots ,S_L\right\}$, where $L$ is an odd number and $S_r$ indicates $r$ language phrases, $r=\mathrm{0,1},\cdots ,$. In this study, enterprises and local governments use seven-granularity language evaluation sets for evaluation. The predefined language evaluation index set is shown in Table 3. Enterprises $E_i$ and local governments $G_j$, respectively, choose a language phrase from the language evaluation index set $S$ to evaluate the evaluation index $R_k$ and $T_d$, that is, the evaluation value $a_{ij}^k$ of local government $G_j$ about the evaluation index given by enterprises $E_i$ is a language phrase, and the evaluation value $b_{ij}^d$ of enterprises concerning the evaluation index given by local governments is a language phrase.

TABLE 3

TABLE 3. Seven granular language evaluation sets.

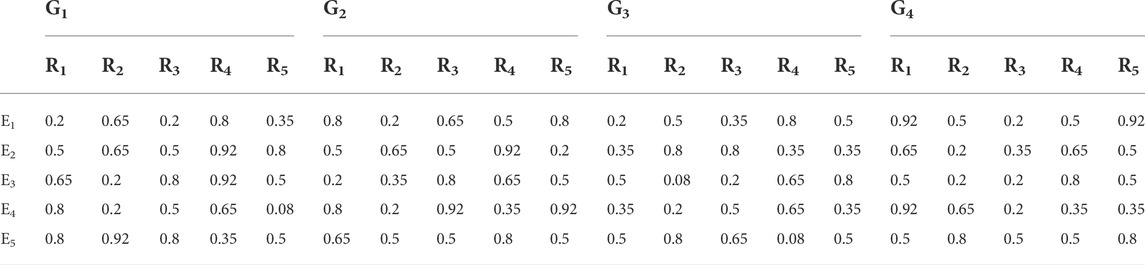
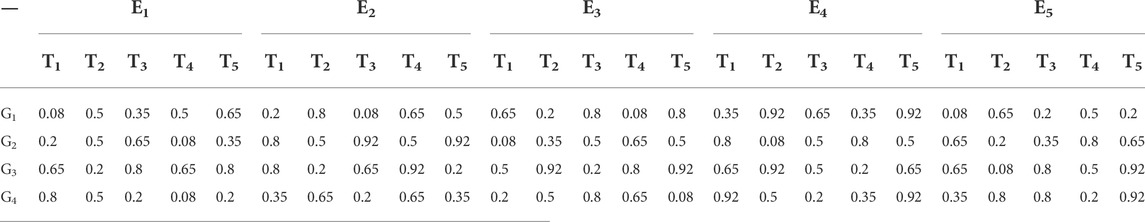
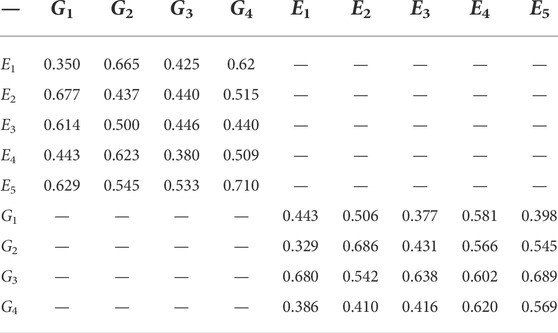
According to Table 3, the matrix $A_i=$ information of language evaluation satisfaction of enterprises and local governments and $B_j=$ is transformed into a trapezoidal fuzzy number matrix ${\stackrel{\sim }{A}}_i=$ and ${\stackrel{\sim }{B}}_j=$. Then, the trapezoidal fuzzy number is deblurred by Eq. 1:

$D\left(\stackrel{\sim }{M}\right)=\frac{1}{3}\left(m_1+m_2+m_3+m_4-\frac{m_3{m}_4-m_1{m}_2}{\left(m_3+m_4\right)-\left(m_1+m_2\right)}\right).(1)$

Then, using Eqs 2 and 3, the degree of satisfaction under different evaluation indexes is weighted and integrated:

$a_{ij}^{{}^{\prime }}={\displaystyle \sum }_{k=1}^h{\stackrel{\sim }{a}}_{ij}^k{u}_i^k,(2)$

$b_{ij}^{{}^{\prime }}={\displaystyle \sum }_{d=1}^f{\stackrel{\sim }{b}}_{ij}^d{v}_j^d.(3)$

Among them, $a_{ij}^{\prime }$ is the overall satisfaction of enterprises $E_i$ to local governments $G_j$ and $b_{ij}^{\prime }$ is the overall satisfaction of local governments $G_j$ to enterprises $E_i$. The satisfaction matrix is $A_i^{\prime }=$ of enterprises $E_i$ to interested local governments; $B_j^{\prime }=$ is the satisfaction matrix of local government $G_j$ to an enterprise that meets the requirements of attracting investment.

4.3 Construction and solution of stable matching model

According to the satisfaction, $B_j^{\prime }=$ of enterprises and local governments and $A_i^{\prime }=$ obtained previously, an optimization model with the maximum satisfaction of enterprises and local policies can be established. Set $x_{ij}$ to 0–1 decision variable and $x_{ij}=1$ means that enterprises $E_i$ and local governments $G_j$ form a matching pair; otherwise, $x_{ij}=0$. Furthermore, the following 0–1 type double-objective integer programming models (4)–(9) can be constructed.

$\mathrm{Max}{Z}_1={\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{a}_{ij}^{\prime }{x}_{ij},(4)$

$\mathrm{Max}{Z}_2={\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^m{b}_{ij}^{\prime }{x}_{ij},(5)$

$s.t.{\displaystyle \sum }_{i=1}^m{x}_{ij}\le 1j=\mathrm{1,2},\cdots ,n,(6)$

${\displaystyle \sum }_{j=1}^n{x}_{ij}\le q_j,i=\mathrm{1,2},\cdots ,m,(7)$

$q_j({\displaystyle \sum }_{a_{ih}^{\prime }>a_{ij}^{\prime }}{x}_{ih}+x_{ij})+{\displaystyle \sum }_{b_{gj}^{\prime }>b_{ij}^{\prime }}{x}_{ih}\ge q_j,i=\mathrm{1,2},\cdots ,m,j=\mathrm{1,2},\cdots ,m,(8)$

$x_{ij}=0\mathrm{o}\mathrm{r}1,i=\mathrm{1,2},\cdots ,m;j=\mathrm{1,2},\cdots ,n.(9)$

In the aforementioned models (4)–(9), Eqs 4, 5 are objective functions, Eq. 4 represents the satisfaction of enterprises that maximize location selection, and Eq. 5 represents the satisfaction of local governments that maximize investment attraction; Eqs 6–9 are constraints, Eq. 6 means that each enterprise $E_i$ can choose only one location for investment, Formula (7) means that each local government $G_j$ can attract not more than $q_j$ projects in $E_i$, and Eq. 8 is a one-to-many stable matching constraints for enterprises and local governments to match.

Models (4)–(9) are multi-objective optimization models, and this study uses the ideal point method to solve them (Zeleny, 1982; Mosavi, 2010). Considering the fairness of matching between enterprises and local governments, let the sum of the objective functions $Z_1$ and $Z_2$ be equally important and take the sum of the optimal solution $Z_1$ and $Z_2$ of the objective functions as the ideal point. Therefore, the multi-objective optimization models (4)–(9) can be transformed into the following single-objective optimization Model (10):

$\mathrm{Min}Z={\left({\left|Z_1-Z_1^{\ast }\right|}^y+{\left|Z_2-Z_2^{\ast }\right|}^y\right)}^{\frac{1}{y}},(10)$

$s.t.,{\displaystyle \sum }_{i=1}^m{x}_{ij}\le 1,j=\mathrm{1,2},\cdots ,n,(11)$

${\displaystyle \sum }_{j=1}^n{x}_{ij}\le q_j,i=\mathrm{1,2},\cdots ,m,(12)$

$(q_j\left({\displaystyle \sum }_{a_{ih}^{\prime }>a_{ij}^{\prime }}{x}_{ih}+x_{ij}\right)+{\displaystyle \sum }_{b_{gj}^{\prime }>b_{ij}^{\prime }}{x}_{ih}\ge q_j,i=\mathrm{1,2},\cdots ,m,j=\mathrm{1,2},\cdots ,m,(13)$

$x_{ij}=0\mathrm{o}\mathrm{r}i=\mathrm{1,2},\cdots ,m;j=\mathrm{1,2},\cdots ,n,(14)$

where $1\le y\le +\infty$.

At that time, when $y=1$, the distance between the optimal solution and the ideal point is the Hamming distance or absolute distance. At that time, when $y=1$, the distance between the optimal solution and the ideal point is the Euclidean distance. At that time, when $y\to \infty$, the distance between the optimal solution and the ideal point was Chebyshev distance. Decision-makers can choose different values for $y$ according to actual needs, and here $y=1$. According to the multi-objective programming theory, the optimal solution of models (10)–(14) is the Pareto efficient solution of models (4)–(9). Because there are $Z_1\le Z_1^{*}$ and $Z_2\le Z_2^{*}$, models (4)–(9) can be converted into models (15)–(19).

$\text{Min}\mathrm{Z}=(Z_1^{\ast }+Z_2^{\ast }-\left(Z_1+Z_2\right),(15)$

$s.t.{\displaystyle \sum }_{i=1}^m{x}_{ij}\le 1,j=\mathrm{1,2},\cdots ,n,(16)$

${\displaystyle \sum }_{j=1}^n{x}_{ij}\le q_j,i=\mathrm{1,2},\cdots ,m,(17)$

$(q_j\left({\displaystyle \sum }_{a_{ih}^{\prime }>a_{ij}^{\prime }}{x}_{ih}+x_{ij}\right)+{\displaystyle \sum }_{b_{gj}^{\prime }>b_{ij}^{\prime }}{x}_{ih}\ge q_j,i=\mathrm{1,2},\cdots ,m,j=\mathrm{1,2},\cdots ,m,(18)$

$x_{ij}=0\mathrm{o}\mathrm{r}1i=\mathrm{1,2},\cdots ,m;j=\mathrm{1,2},\cdots ,n.(19)$

Models (15)–(19) are 0–1 integer programming models, which can be solved by the branch and bound algorithm when the scale of the problem is relatively small. When the scale of the problem is relatively large, CPLEX 12.0, LINGO 11.0, and other software can be used to solve it.

5 Examples

The Jiangsu Province Investment Promotion Information Network is the industry’s leading information publishing platform for industrial projects and a professional organization for industrial park investment promotion services. With the aim of empowering high-tech enterprises to develop, creating industrial agglomeration land, and injecting new industrial kinetic energy into local areas, the Jiangsu Investment Promotion Information Network takes science and technology investment as its core and industrial development as its starting point. It thus provides not only online site selection for enterprises to invest but also investment promotion solutions for the government and industrial parks. The investment service platform can provide online information releases, park promotion services, investment policy, industry information, project docking, investment promotion, and other integrated services. In a period of time, the platform has brought together four local governments (industrial parks) $G=\left\{G_1,G_2,G_3,G_4\right\}$ and five enterprises $E=\left\{E_1,E_2,E_3,E_4,E_5\right\}$ to invest. The number of projects that can attract investment in the four industrial parks is, respectively, $q_1=1$, $q_2=2$, $q_3=1$, and $q_4=1$. According to the evaluation indexes in Table 1, five enterprises giving language evaluation information about five industrial parks is shown in Table 4, and the language evaluation information about the investment projects of five enterprises given by four local governments is shown in Table 5. In addition, the index weights given by enterprises and local governments for their respective evaluation index sets are shown in Table 6.

TABLE 4

TABLE 4. Evaluation information of industrial parks given by enterprises.

TABLE 5

TABLE 5. Evaluation information of investment projects given by local governments.

TABLE 6

TABLE 6. Weight information.

TABLE 7

TABLE 7. Evaluation information of enterprise satisfaction after defuzzification.

TABLE 8

TABLE 8. Satisfaction evaluation information of local government after defuzzification.

TABLE 9

TABLE 9. Overall satisfaction of enterprises and local governments.

On this basis, we can build multi-objective optimization models (4)–(9) of stable matching between enterprises and local governments and then transform them into single-objective optimization models (10)–(14) using the ideal point method. The maximum satisfaction $Z_2^{*}=2.692$ of enterprises and local maximum satisfaction $Z_2^{*}=2.692$ of governments under optimal conditions are calculated using LINGO optimization software. Then, the optimal solutions of the calculation models (15)–(19) are as follows: $x_{11}=x_{23}=x_{34}=x_{42}=x_{52}=1$, the rest $x_{ij}=0$, enterprise $E_1$ and local government $G_1$ match, enterprise $E_2$ and local government $G_3$ match, enterprise $E_3$ and local government $G_4$ match, and enterprise $E_4$ and local government $G_2$ match. Therefore, for enterprises, the optimal investment locations are $G_1,G_3,G_4,G_2,G_2$ for enterprises $E_1,E_2,E_3,E_4,E_5$, and for local governments (industrial parks), the most suitable investment project is $E_1$ for local governments (industrial parks). $G_1$, $E_4$, and $E_5$ for $G_2$, $E_2$ for $G_3$, and $E_3$ for $G_4$.

If the stability of the local government–firm match is not considered, i.e., the stability constraint (8) in models (4)–(9) is not considered, the maximum satisfaction of the firm considering only the interests of the firm is $Z_1^{*}=3.121$, and the maximum satisfaction of the local government considering only the interests of the local government is $Z_2^{*}=2.957$. On this basis, the optimal solution obtained by solving models (15)–(19) is $x_{12}=x_{21}=x_{33}=x_{42}=x_{54}=1$, the rest $x_{ij}=0$, and the overall satisfaction of both parties is 5.726. It is clear that the optimal matching solution obtained by considering stability and not considering stability is different. If the stability of the match between the firm and the local government is not taken into account, the overall satisfaction of both the firm and the local government is higher than that of the case where stability is taken into account. However, from another perspective, if the stable matching condition of matching is not considered, firm $E_3$ and local government $G_2$ would be an unstable matching pair for the matching scheme. Firm$E_3$'s satisfaction from entering into a partnership with local government $G_3$ is 0.446, and local government$G_2$'s satisfaction from entering into a partnership with firm $E_1$ is 0.329, whereas if firm $E_3$ entered into a partnership with local government $G_2$, then $E_3$ and$G_2$'s satisfaction would be 0.500 and 0.431, respectively; in this scenario both $E_3$ and $G_2$ would receive higher satisfaction, and both would have motivation to renege on the original partnership reached with other governments and enterprises, and thus a new partnership between these two, resulting in the invalidation of the original cooperation agreement, will certainly cause other enterprises and local governments to lose the investment promotion work. This shows that the satisfaction and stability of the match between enterprises and local governments in the process of attracting investment is an important factor to be considered.

6 Conclusion

In this study, the stable matching theory is applied to enterprise location selection and local government investment promotion. The evaluation index system of enterprise location selection and local government investment promotion is thus established. Based on the linguistic evaluation information given by enterprises and local governments, the satisfaction of enterprises with location and local governments with target investment enterprises is calculated. The optimal stable matching between enterprises and local governments is then obtained according to the established stable matching model.

6.1 Practical implication of the study

The findings of this study show that by adopting a centralized bilateral matching mechanism and unifying the traditional way in which firms select sites based on the investment attractiveness of local governments, it is possible to improve both the accuracy of firms’ site selection and the efficiency of local governments’ investment attractiveness by changing the past search patterns of firms and local governments. In practical terms, compared to the original situation where local governments at all levels were competing fiercely to try to attract investment, this study concludes that by adopting a stable matching mechanism, local governments can more clearly locate the industrial development goals of their regions and establish more concentrated industrial clusters, thus gaining the favor of enterprises.

6.2 Limitation of this research

The main purpose of this study was to study enterprise’s location choice and government’s investment attraction from the perspective of bilateral matching, proposing a new mechanism to achieve a stable matching between enterprises and government investment attraction, thus changing the decentralized unilateral search mode of enterprises and local governments in the past, which can improve the accuracy of enterprise location choice and the efficiency of local government investment attraction. Companies in Jiangsu Province, China, were chosen as an example for the study. Although the diversity of economies and levels of enterprise development across Jiangsu Province makes the findings highly informative, the lack of data relating to more Asian countries and indeed other enterprises around the world is a limitation of this study.

6.3 Future direction of the study

By comparing and analyzing the matching schemes obtained with and without the stable matching condition, this study finds that the overall satisfaction of firms and local governments is higher when the stable matching condition is not taken into account than when the stable matching condition is taken into account. Disregarding the stable matching condition will give some enterprises and local governments an incentive to break the original partnership and seek better partners, thus leading to ineffective investment promotion efforts by other enterprises and local governments, resulting in wasted resources and ineffective allocation of resources provided by enterprises and local investment promotion. This study further enriches and develops the theory of firm location choice and provides a new matching mechanism for firm location choice and government investment patterns. In the future, based on the research in this study, more stable matching mechanisms can be explored in conjunction with more factors of corporate location choice, and more targeted research will be conducted on more corporate data from various countries around the world, so that more widely used matching mechanisms can be obtained.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding authors.

Author contributions

Conceptualization, LS and DT; methodology, LS; software, LS; validation, LS; formal analysis, LS; investigation, LS; resources, LS; data curation, LS; writing—original draft preparation, LS; writing— review and editing, LS, HK, DT, and VB; supervision, DT and HK; visualization, HK and VB; and project administration, DT. All authors read and agreed to the published version of the manuscript.

Acknowledgments

The authors thank the experts at the Global Economic Organization Big Data Resource Center for their contribution to providing the data used in this study.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Baas, L., and Boons, F. (2004). An industrial ecology project in practice: Exploring the boundaries of decision-making levels in regional industrial systems. J. Of Clean. Prod. 12 (8-10), 1073–1085. doi:10.1016/j.jclepro.2004.02.005

CrossRef Full Text | Google Scholar

Baldwin, R., and Okubo, T. (2005). Heterogeneous firms, agglomeration, and economic geography: Spatial selection and sorting. J. Econ. Geogr. 6 (3), 323–346. doi:10.1093/jeg/lbi020

CrossRef Full Text | Google Scholar

Belussi, F., and Sammarra, A. (2012). Business networks in clusters and industrial districts. London: Routledge.

Google Scholar

Budish, E. (2012). Matching "versus" mechanism design. SIGecom Exch. 11 (2), 4–15. doi:10.1145/2509002.2509005

CrossRef Full Text | Google Scholar

Cartier, C. (2002). Origins and evolution of a geographical idea: The macroregion in China. Mod. China 28 (1), 79–112. doi:10.1177/009770040202800103

CrossRef Full Text | Google Scholar

Ding, R., Shi, F., and Hao, S. (2022). Digital inclusive finance, environmental regulation, and regional economic growth: An empirical study based on spatial spillover effect and panel threshold effect. Sustainability 14 (7), 4340. doi:10.3390/su14074340

CrossRef Full Text | Google Scholar

Douglas, W., and Marshall, J. (1932). A factual study of bankruptcy administration and some suggestions. Columbia Law Rev. 32 (1), 25. doi:10.2307/1115117

CrossRef Full Text | Google Scholar

Fierla, I. (1998). Struktura przestrzenna gospodarki. W: Geografia gospodarcza Polski. Warszawa: Red. I. Fierla. PWE, 11–32.

Google Scholar

Fujita, M. (2010). The evolution of spatial economics: From thünen to the new economic geography. Jpn. Econ. Rev. (Oxf). 61, 1–32. doi:10.1111/j.1468-5876.2009.00504.x

CrossRef Full Text | Google Scholar

Gale, D., and Shapley, L. (1962). College admissions and the stability of marriage. Am. Math. Mon. 69 (1), 9. doi:10.2307/2312726

CrossRef Full Text | Google Scholar

Gordon, I. R., and McCann, P. (2000). Industrial clusters: Complexes, agglomeration and/or social networks? Urban Stud. 37 (3), 513–532. doi:10.1080/0042098002096

CrossRef Full Text | Google Scholar

Gu, J., Yang, Y., and Strange, R. (2018). Location choice, ownership structure and multinational performance. Multinatl. Bus. Rev. 26 (3), 250–276. doi:10.1108/MBR-12-2017-0105

CrossRef Full Text | Google Scholar

Head, C., Ries, J., and Swenson, D. (1999). Attracting foreign manufacturing: Investment promotion and agglomeration. Regional Sci. Urban Econ. 29 (2), 197–218. doi:10.1016/s0166-0462(98)00029-5

CrossRef Full Text | Google Scholar

Hong, J. (2009). Firm heterogeneity and location choices: Evidence from foreign manufacturing investments in China. Urban Stud. 46 (10), 2143–2157. doi:10.1177/0042098009339425

CrossRef Full Text | Google Scholar

Huang, Z., Pan, B., and Teng, F. (2020). Integration of the belt and road initiative and the national strategies for major regional development.China economic transition = dangdai. Zhongguo Jingji Zhuanxing Yanjiu; Beijing 3 (3), 79–87. doi:10.3868/s060-010-020-0032-0

CrossRef Full Text | Google Scholar

Iammarino, S., and McCann, P. (2006). The structure and evolution of industrial clusters: Transactions, technology and knowledge spillovers. Res. Policy 35 (7), 1018–1036. doi:10.1016/j.respol.2006.05.004

CrossRef Full Text | Google Scholar

Isard, W. (1956). Regional science, the concept of region, and regional structure. Pap. Reg. Sci. 2 (1), 13–26. doi:10.1111/j.1435-5597.1956.tb01542.x

CrossRef Full Text | Google Scholar

Jordaan, J. A. (2012). Agglomeration and the location choice of foreign direct investment: New evidence from manufacturing fdi in Mexico. Estud. Económicos 27 (153), 61–97. Available at: http://www.jstor.org/stable/41756359.

Google Scholar

Juliusson, E. Á., Karlsson, N., and Gärling, T. (2005). Weighing the past and the future in decision making. Eur. J. cognitive Psychol. 17 (4), 561–575. doi:10.1080/09541440440000159

CrossRef Full Text | Google Scholar

Justin Yifu, L., and Wang, X. (2021). Dual circulation: A new structural economics view of development. J. Chin. Econ. Bus. Stud., 1–20. doi:10.1080/14765284.2021.1929793

CrossRef Full Text | Google Scholar

Krugman, P. (1991). Increasing returns and economic geography. J. Of Political Econ. 99 (3), 483–499. doi:10.1086/261763

CrossRef Full Text | Google Scholar

Li, Z. (2021). Agglomeration and innovation: An empirical study based on China’s manufacturing data. J. Of Adv. Comput. Intell. Intelligent Inf. 25 (5), 563–573. doi:10.20965/jaciii.2021.p0563

CrossRef Full Text | Google Scholar

Liang, L., Wang, Z., Luo, D., Wei, Y., and Sun, J. (2020). Synergy effects and it’s influencing factors of China’s high technological innovation and regional economy. PLOS ONE 15 (5), e0231335. doi:10.1371/journal.pone.0231335

PubMed Abstract | CrossRef Full Text | Google Scholar

Liu, L. (2019). Research on the productivity effect of inter-regional industry transfer—Taking Guangdong province as an example. Mod. Econ. 10 (08), 1872–1896. doi:10.4236/me.2019.108121

CrossRef Full Text | Google Scholar

Lo, F., and Lin, F. (2015). Advantage transfer on location choice and subsidiary performance. J. Of Bus. Res. 68 (7), 1527–1531. doi:10.1016/j.jbusres.2015.01.046

CrossRef Full Text | Google Scholar

Lösch, A. (1940). Review of Die englische Ernährungslage im Frieden und im Kriege. (Schriften des Deutschen Instituts für Außenpolitische Forschung und des Hamburger Instituts für Auswärtige Politik. H. 13), by H. Decken. Weltwirtschaftliches Arch. 52 (1), 29–31. Available at: http://www.jstor.org/stable/41553165.

Google Scholar

Martin, J. (1987). Handbook of regional and urban economics. Vol1: Elsevier, 21–95. doi:10.1016/S1574-0080(00)80005-XBeckmann, jacques-françois thisse, chapter 2 the location of production activities

CrossRef Full Text | Google Scholar

Mechanism of realizing industrial structure upgrading through technology-driven regional innovation capacity. (2008), 07(09). doi:10.17265/1537-1506/2008.09.008

CrossRef Full Text

Michie, J., and Oughton, C. (2001). Regional innovation strategies: Integrating regional, industrial and innovation policy. New Econ. 8 (3), 164–169. doi:10.1111/1468-0041.00209

CrossRef Full Text | Google Scholar

Mosavi, A. (2010). Multiple criteria decision-making preprocessing using data mining tools. Available at: https://search.ebscohost.com/login.aspx?direct=true&db=edsarx&AN=edsarx.1004.3258&site=eds-live&scope=site.

Google Scholar

Ohlin, B. (1933). Till frågan om penningteoriens uppläggning. Ekon. Tidskr. 35 (2), 45–81. doi:10.2307/3472597

CrossRef Full Text | Google Scholar

Predöhl, A. (1925). Das standortsproblem in der Wirtschaftstheorie. Weltwirtschaftliches Arch. 21, 294–321. http://www.jstor.org/stable/40306335.

Google Scholar

Roth, A., and Vate, J. (1990). Random paths to stability in two-sided matching. Econometrica 58 (6), 1475. doi:10.2307/2938326

CrossRef Full Text | Google Scholar

Schiele, H. (2008). Location, location: The geography of industry clusters. J. Bus. Strategy 29 (3), 29–36. doi:10.1108/02756660810873191

CrossRef Full Text | Google Scholar

Shen, T. Y., and Cui, N. N. (2020). Theory and application of location market design. Reg. Econ. Rev. (01), 63–69. doi:10.14017/j.cnki.2095-5766.2020.0008

CrossRef Full Text | Google Scholar

Shen, T. Y., Qi, Z. X., and &Wang, Y. B. (2016). The market design of Beijing-Tianjin-Hebei industrial interregional orderly transfer-based on bilateral matching algorithm. Economist (04), 42–52. doi:10.16158/j.cnki.51-1312/f.2016.00

CrossRef Full Text | Google Scholar

Strzelczyk, W. (2014). Investment attractiveness measurement of the regions versus general location of the enterprises. Econ. Regional Stud. (Studia Ekonomiczne i Regionalne) 7 (673-2017-2597), 5–25. doi:10.22004/ag.econ.265028

CrossRef Full Text | Google Scholar

Tian, Y., Jiang, G., Zhou, D., Ding, K., Su, S., Zhou, T., et al. (2019). Regional industrial transfer in the Jingjinji urban agglomeration, China: An analysis based on a new “transferring area-undertaking area-dynamic process” model. J. Of Clean. Prod. 235, 751–766. doi:10.1016/j.jclepro.2019.06.167

CrossRef Full Text | Google Scholar

Vázquez Maguirre, M., Portales, L., and Velásquez Bellido, I. (2018). Indigenous social enterprises as drivers of sustainable development: Insights from Mexico and Peru. Crit. Sociol. 44 (2), 323–340. doi:10.1177/0896920516688757

CrossRef Full Text | Google Scholar

Wan, S., and Li, D. F. (2014). Multi-index bilateral matching decision-making method for venture capitalists and investment enterprises with different types of information. China Manag. Sci. 22 (02), 40–47. doi:10.16381/j.cnki.issn1003-207x.2014.02.007

CrossRef Full Text | Google Scholar

Wang, R., Li, D., and Yu, G. (2020). Research on bilateral matching decision method considering attribute association in heterogeneous information environment. J. Of Intelligent Amp; Fuzzy Syst. 38 (4), 4779–4792. doi:10.3233/jifs-191495

CrossRef Full Text | Google Scholar

Wilson, A. (2010). Entropy in urban and regional modelling: Retrospect and prospect. Geogr. Anal. 42 (4), 364–394. doi:10.1111/j.1538-4632.2010.00799.x

CrossRef Full Text | Google Scholar

Yu, X., and Wang, P. (2021). Economic effects analysis of environmental regulation policy in the process of industrial structure upgrading: Evidence from Chinese provincial panel data. Sci. Of Total Environ. 753, 142004. doi:10.1016/j.scitotenv.2020.142004

PubMed Abstract | CrossRef Full Text | Google Scholar

Zeleny, M. (1982). Multiple criteria decision making. New York: McGraw-Hill. Available at: https://www.scirp.org/(S(351jmbntvnsjt1aadkposzje))/reference/ReferencesPapers.aspx?ReferenceID=1600131.

Google Scholar

Zhang, Y., Liu, R., Yang, Y., and Wen, H. (2021). A bilateral matching method of decision-making for customized buses. J. Phys. Conf. Ser. 1976 (1), 012019. doi:10.1088/1742-6596/1976/1/012019

CrossRef Full Text | Google Scholar